Closed Knight’s Tours on (m,n,r)-Ringboards
Abstract
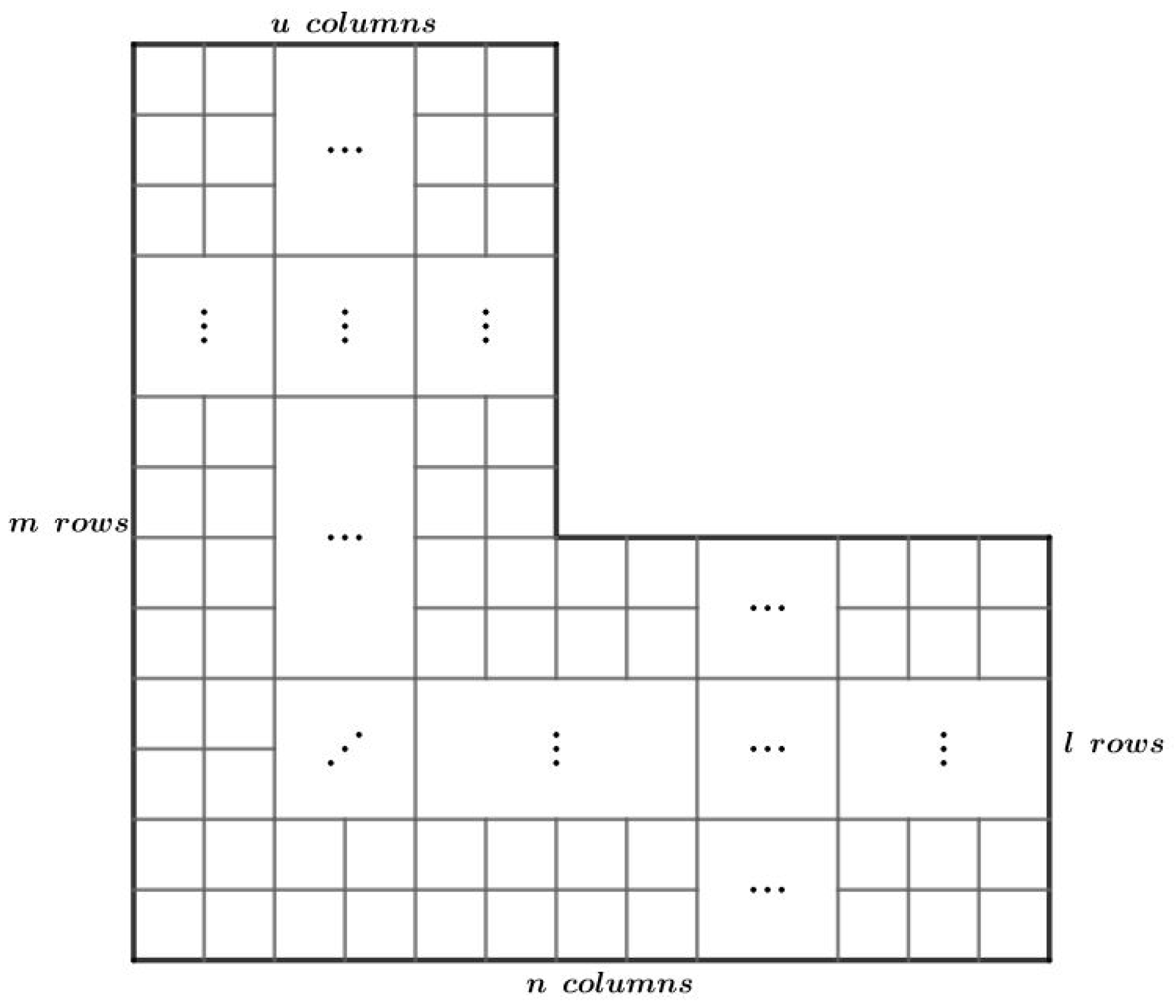
1. Introduction
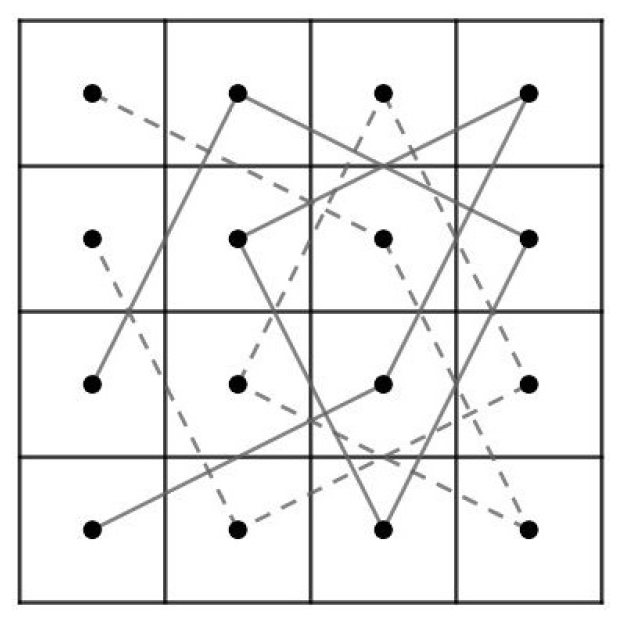
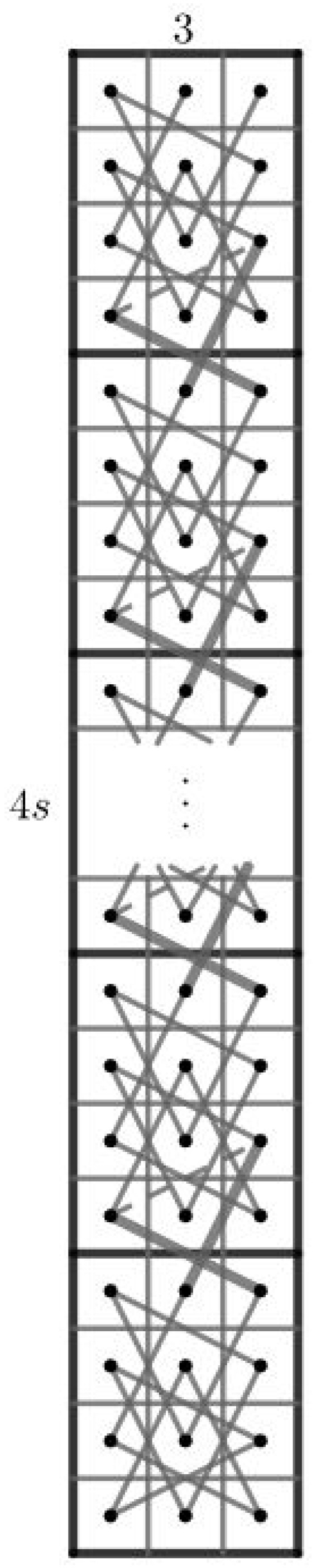
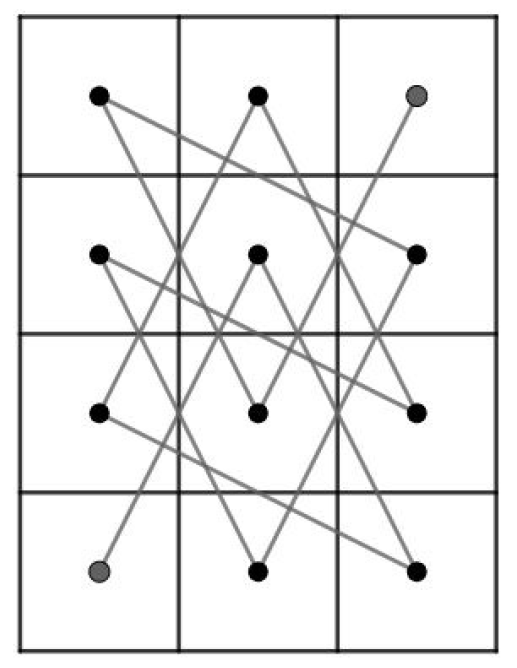
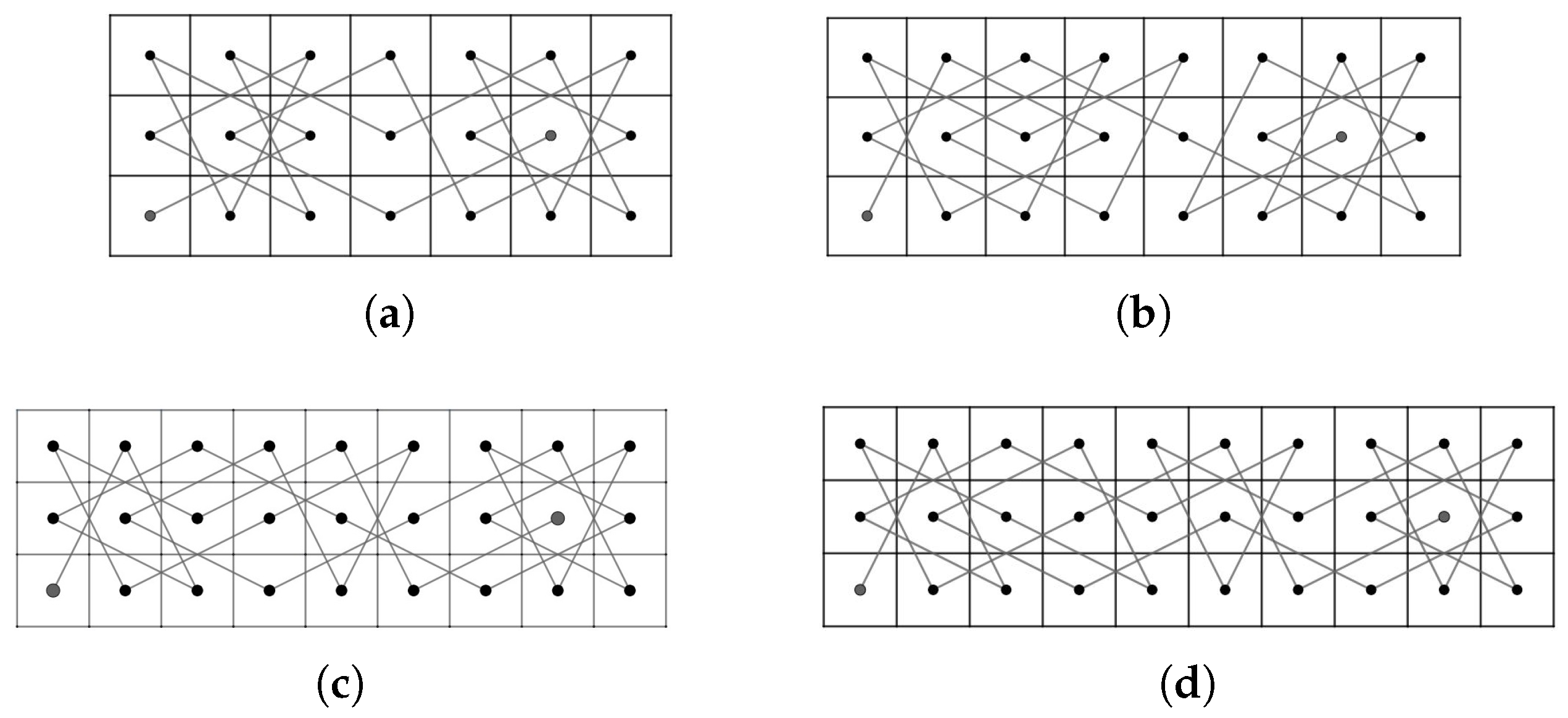
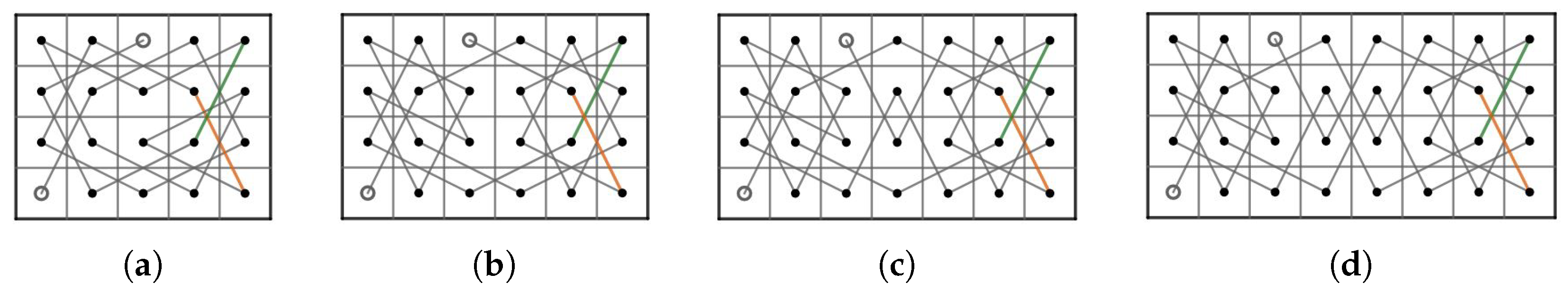
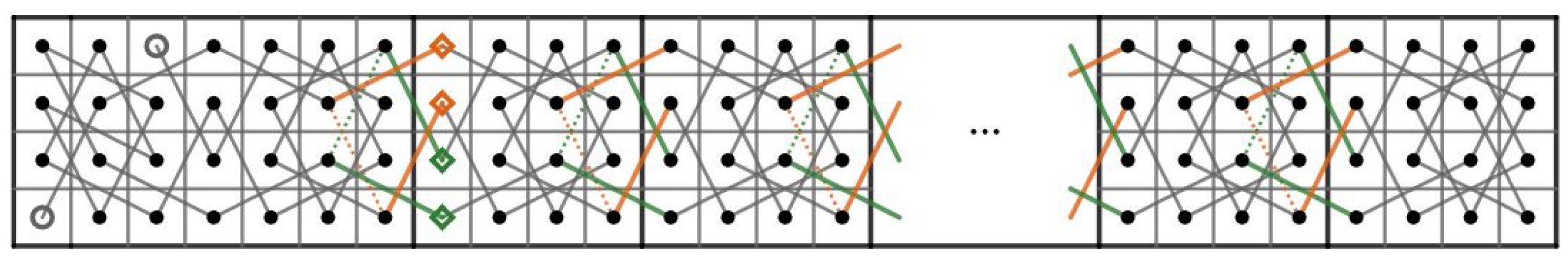
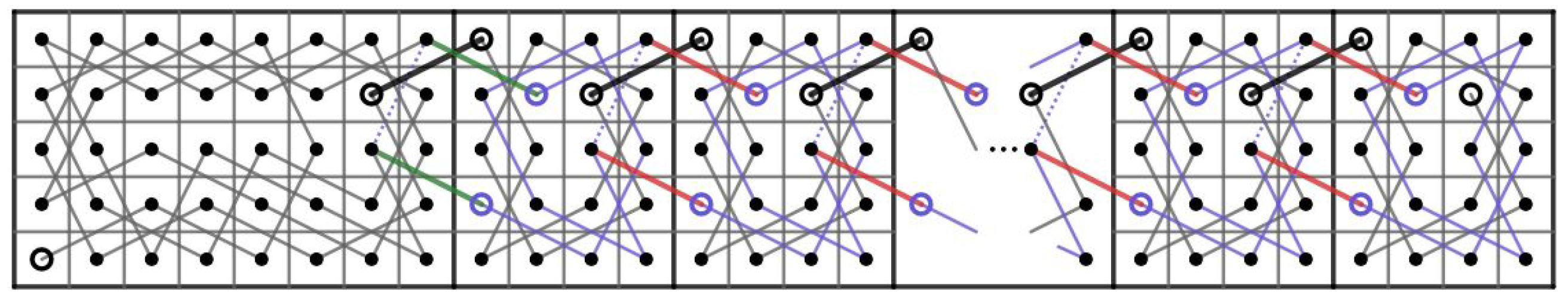
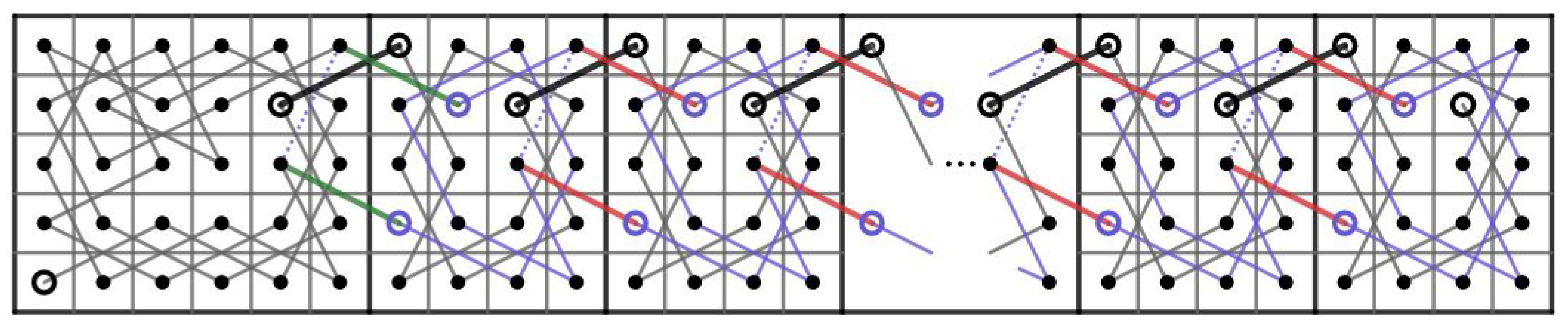
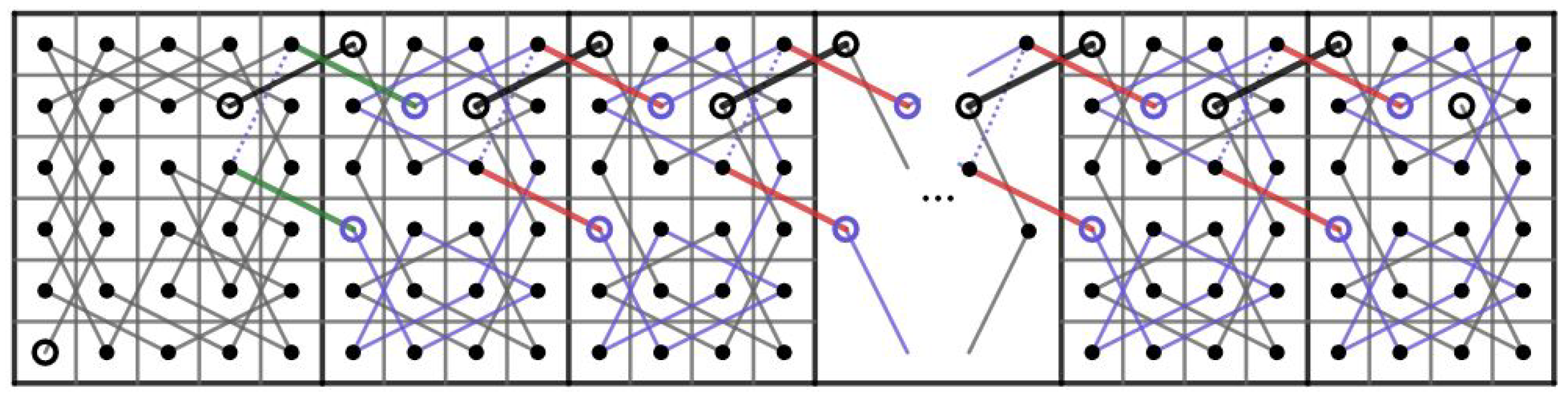
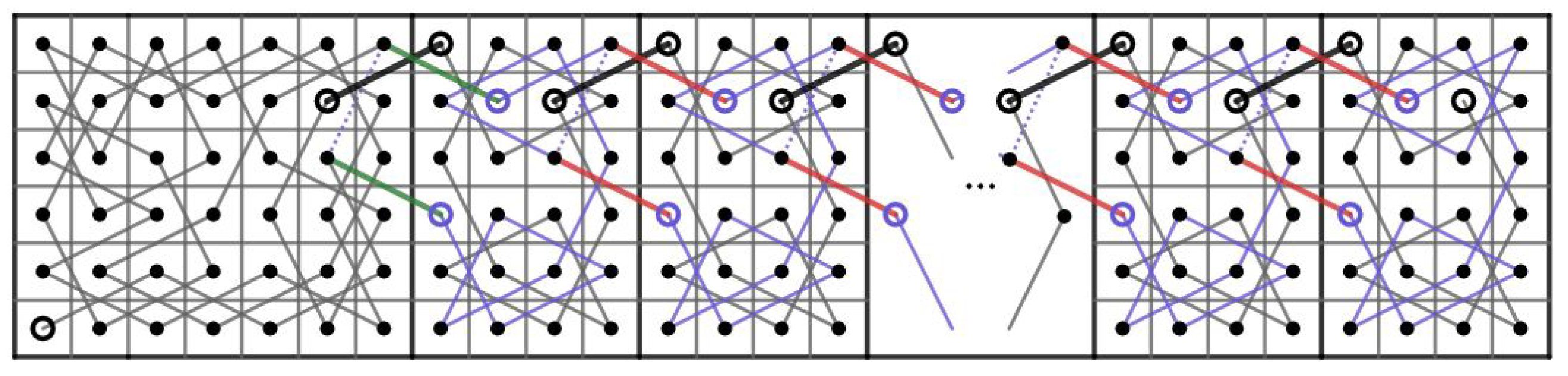
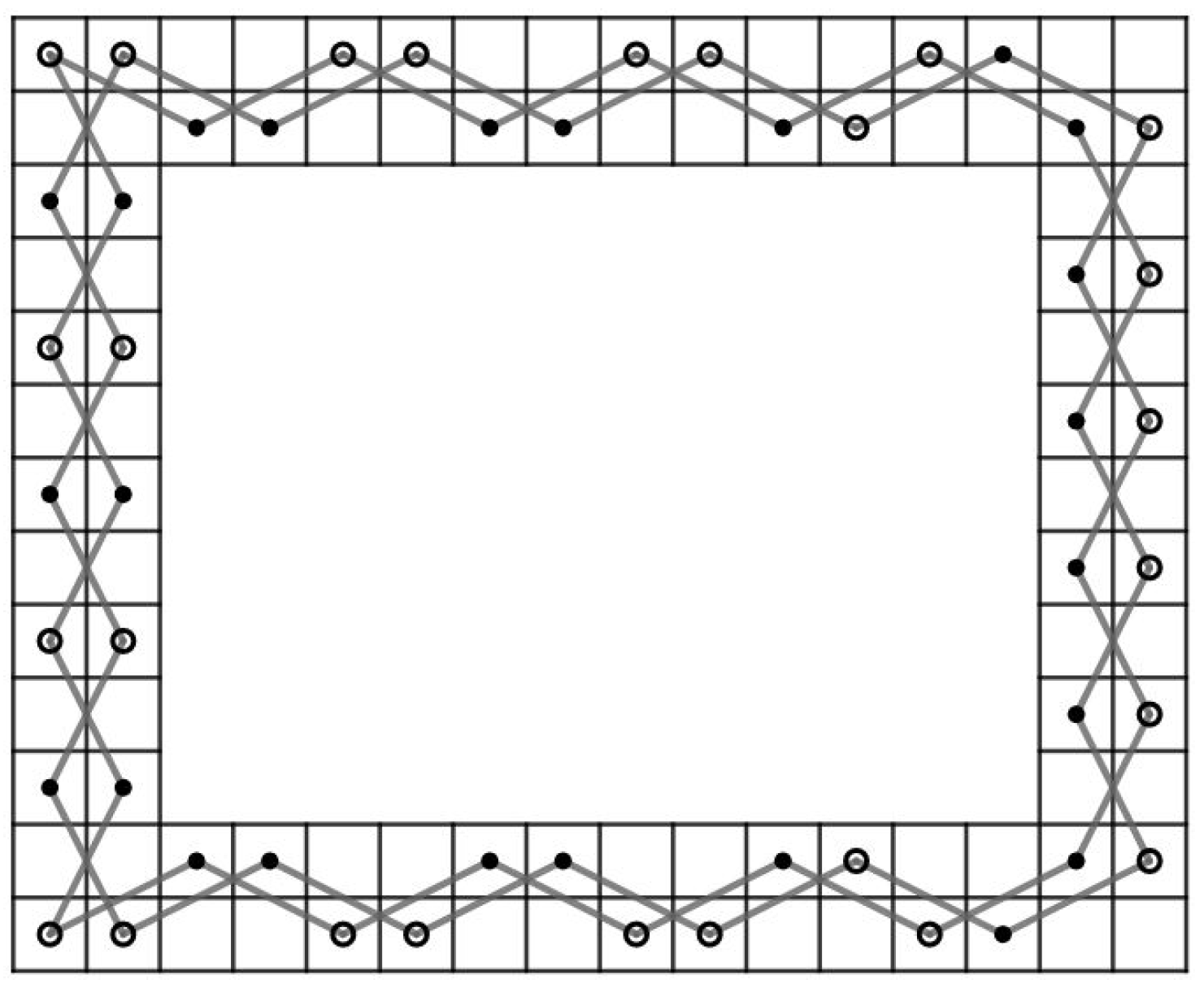
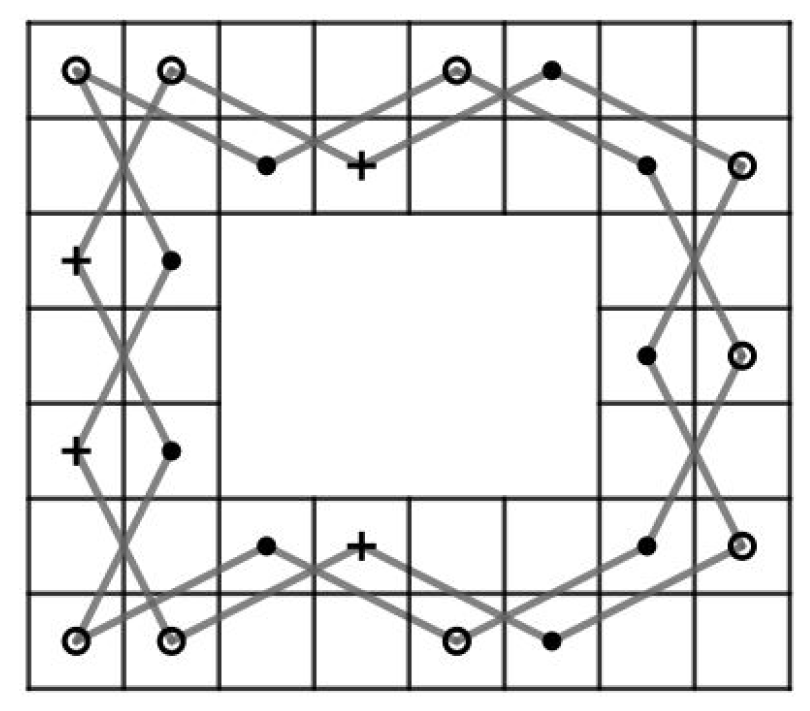
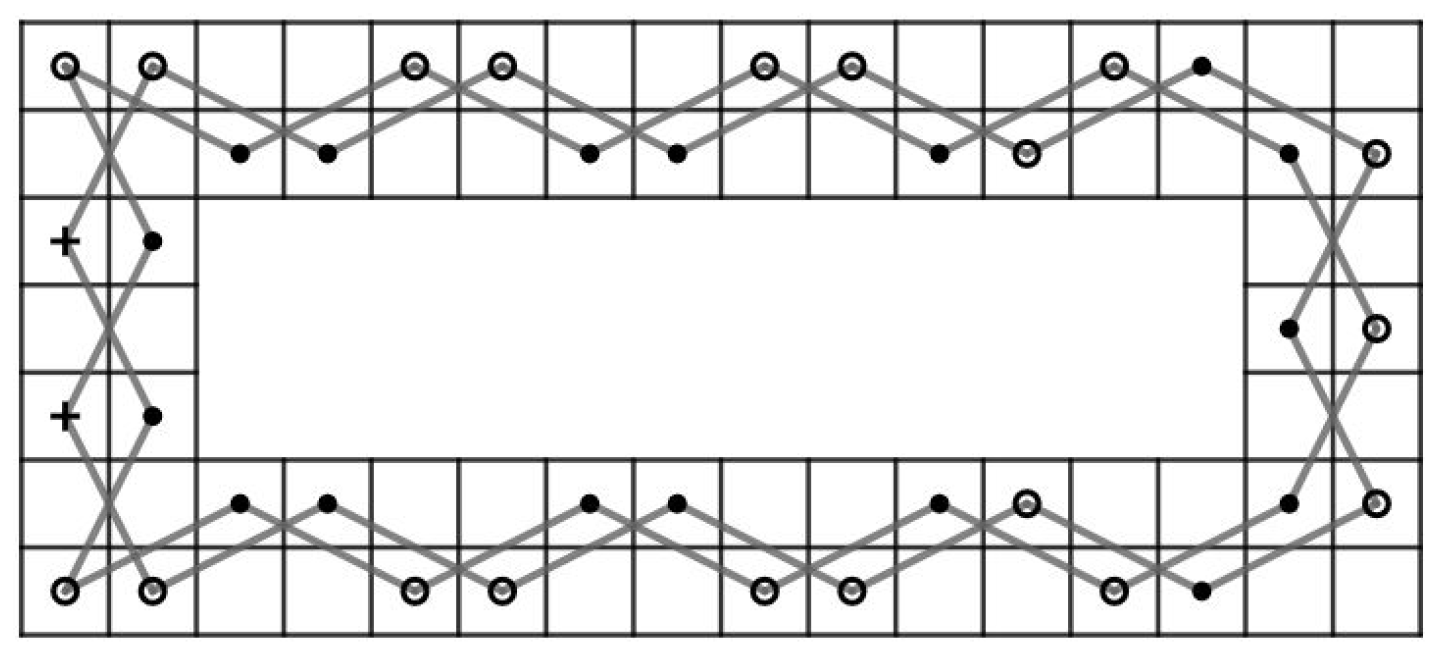
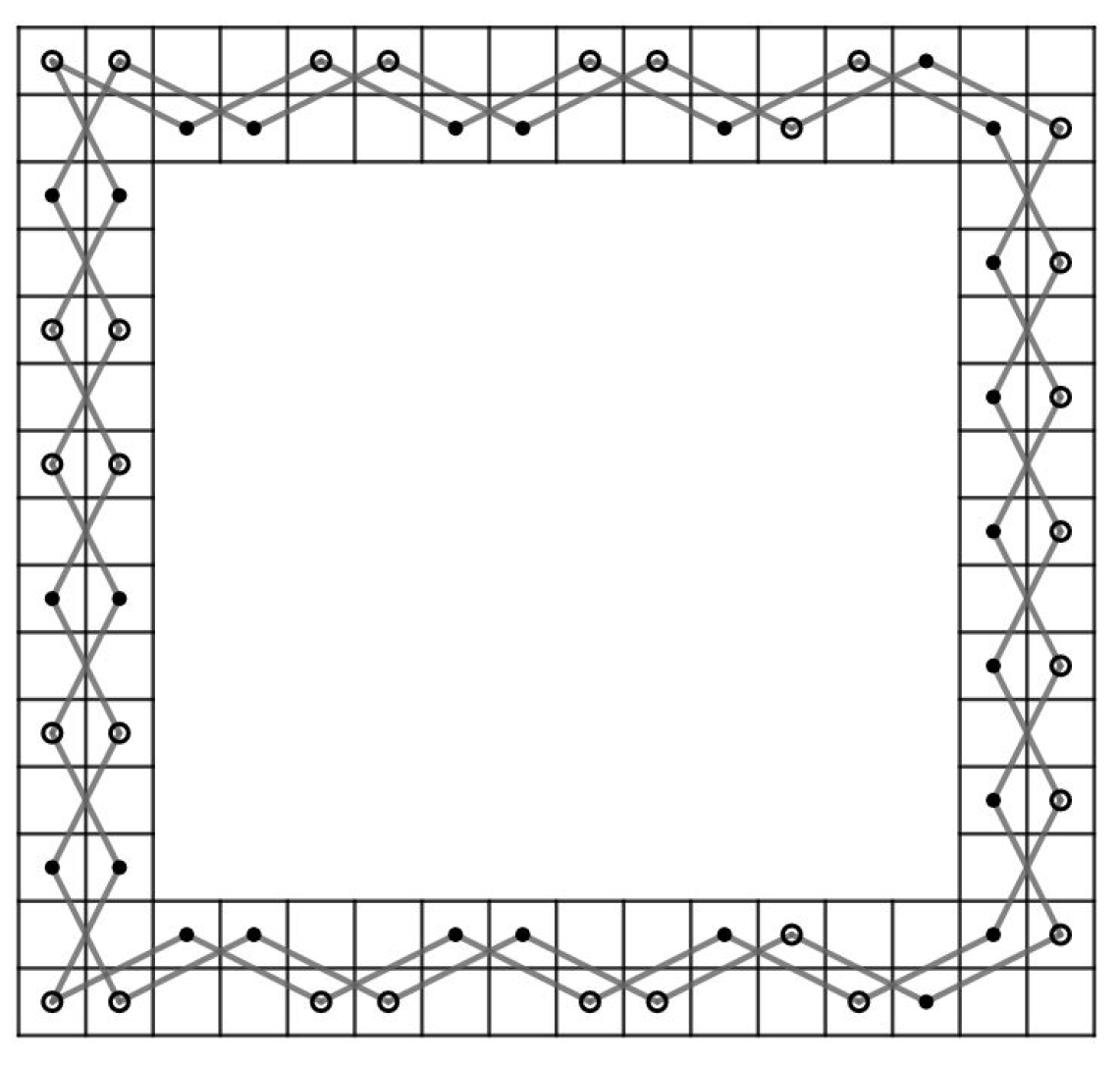
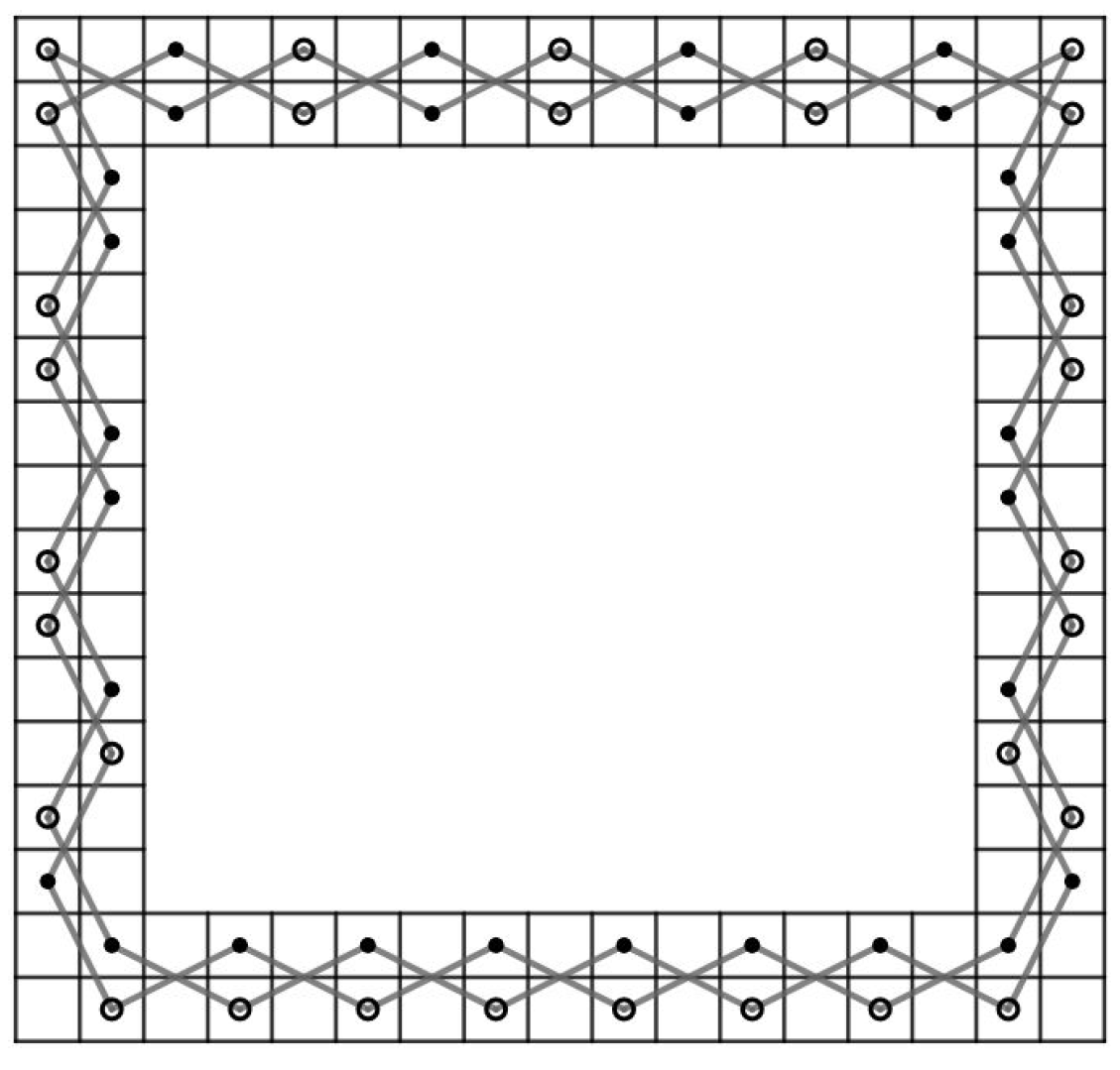
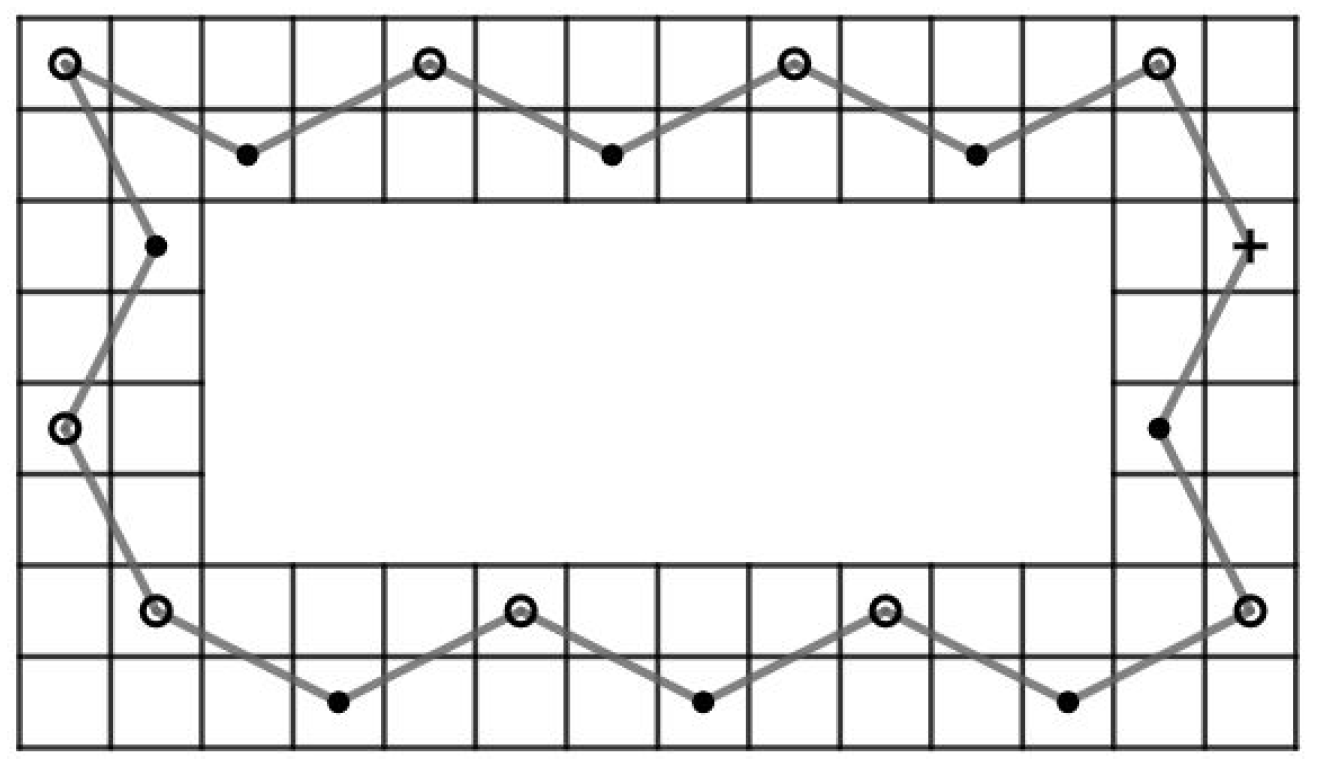
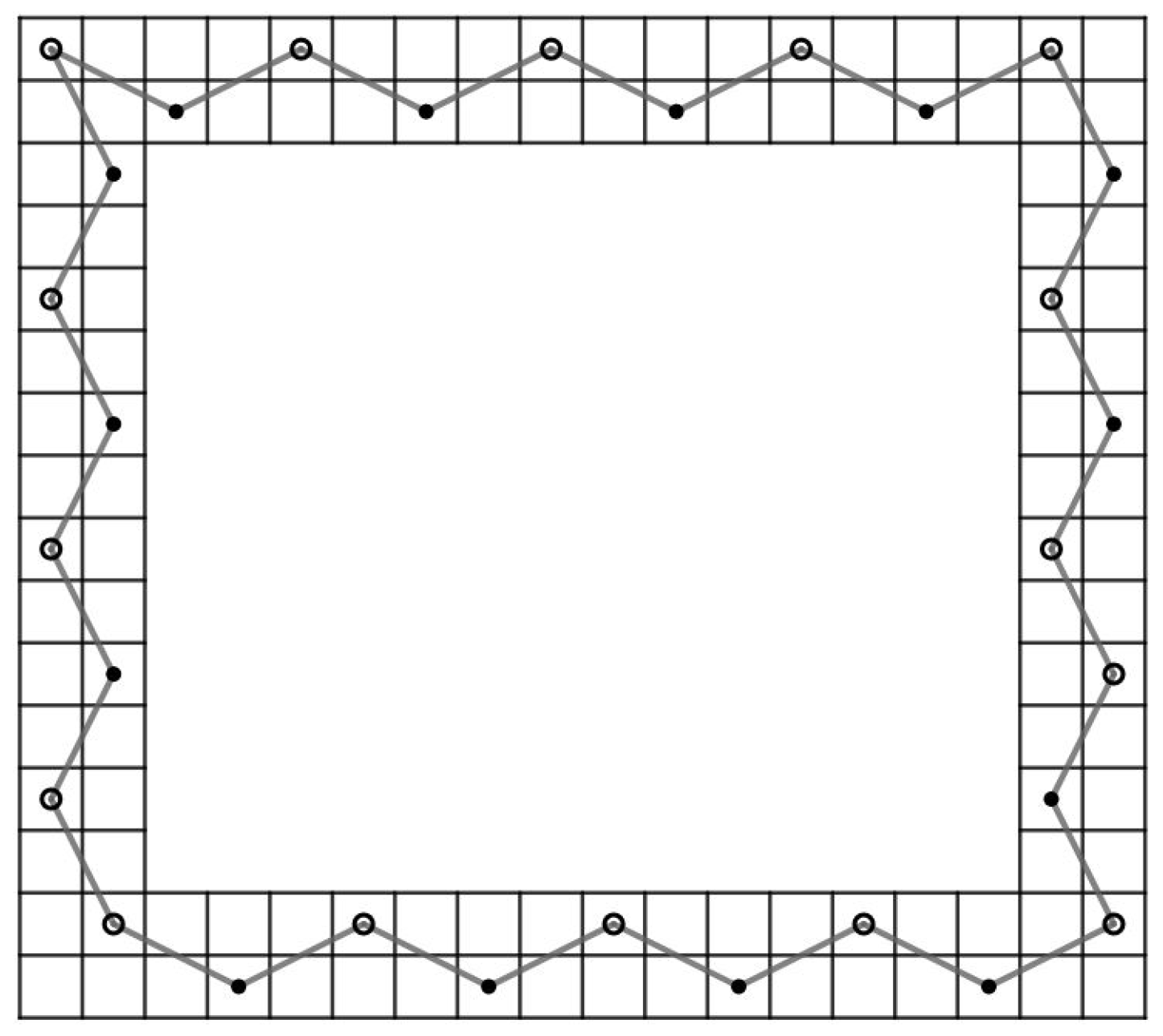
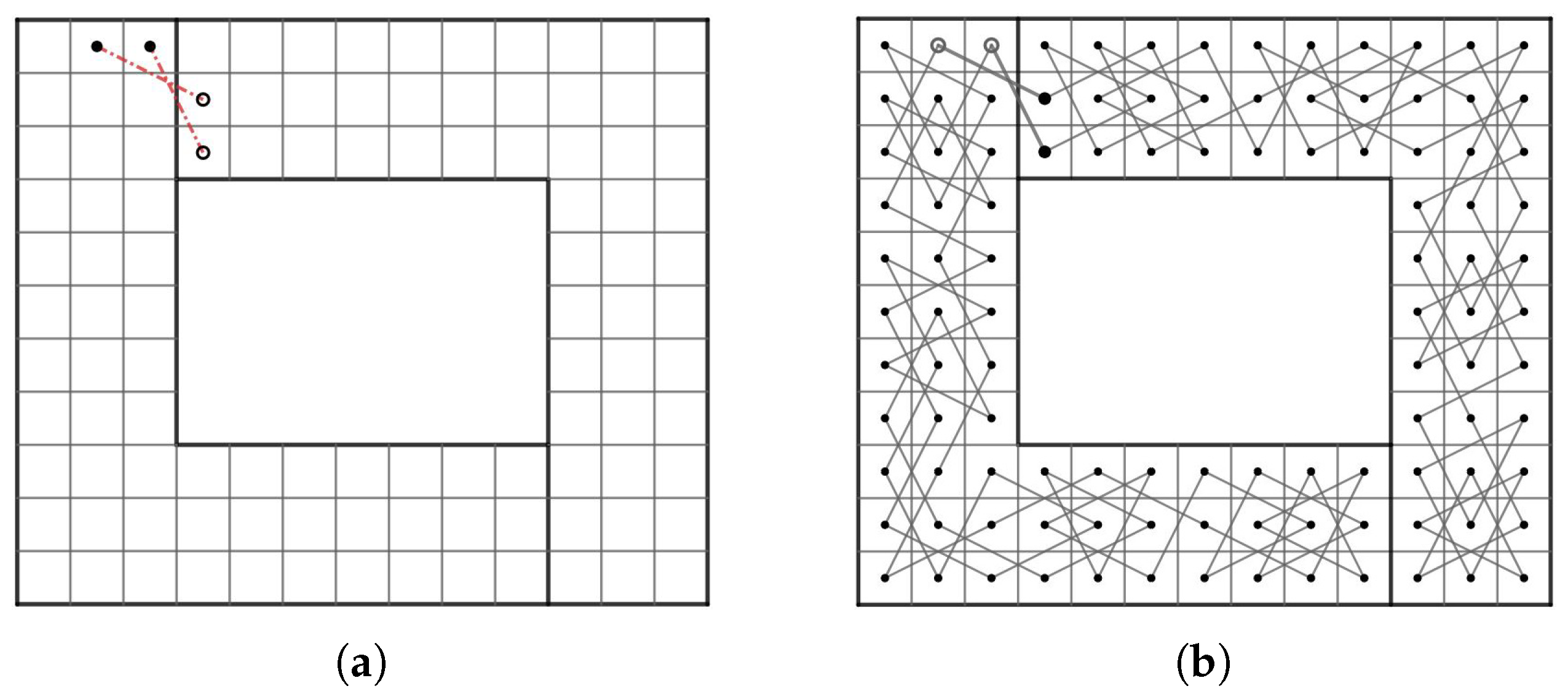
2. CKTs and OKTs on Some LBs and 7Bs
- (i)
- For , delete from and from of the ith CB().
- (ii)
- For , join and of the ith CB() to and of the th CB(), respectively
- (iii)
- For , join and of the ith CB() to and of the th CB(), respectively.
- If m and n are odd integers, then or 3 (mod 4) and or 3 (mod 4).
- If m and n are even integers, then or 2 (mod 4) and or 2 (mod 4).
- If m and n are different parity, then or 3 (mod 4) and or 2 (mod 4); and or 2 (mod 4) and or 3 (mod 4).
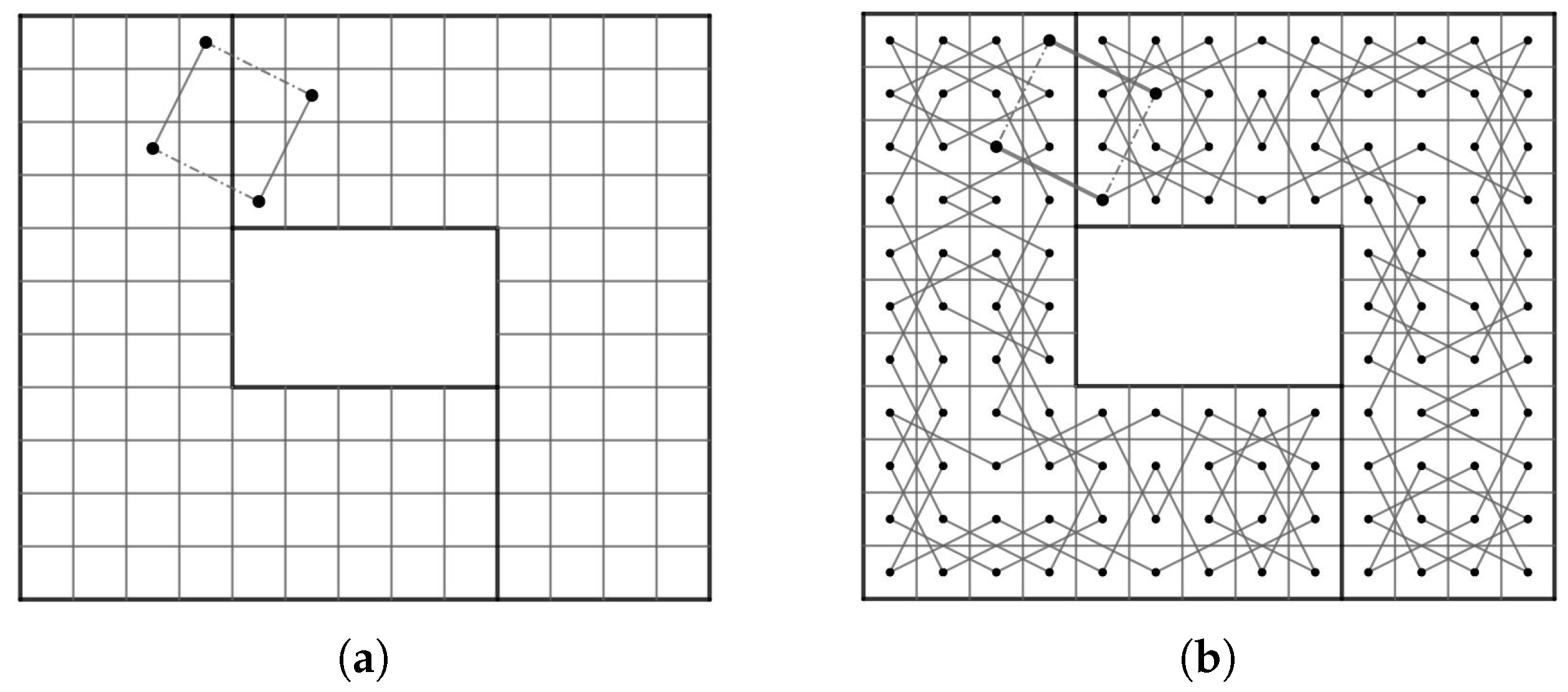
- (i)
- if , then delete and from the CKT of the LB. If , then further delete and from the CKT of the LB.
- (ii)
- If , then join , , and which are four end-points of two paths of the CB() to , , and of the LB, respectively. If , then further join , , and which are four end-points of two paths of the CB() to , , and of the LB, respectively.
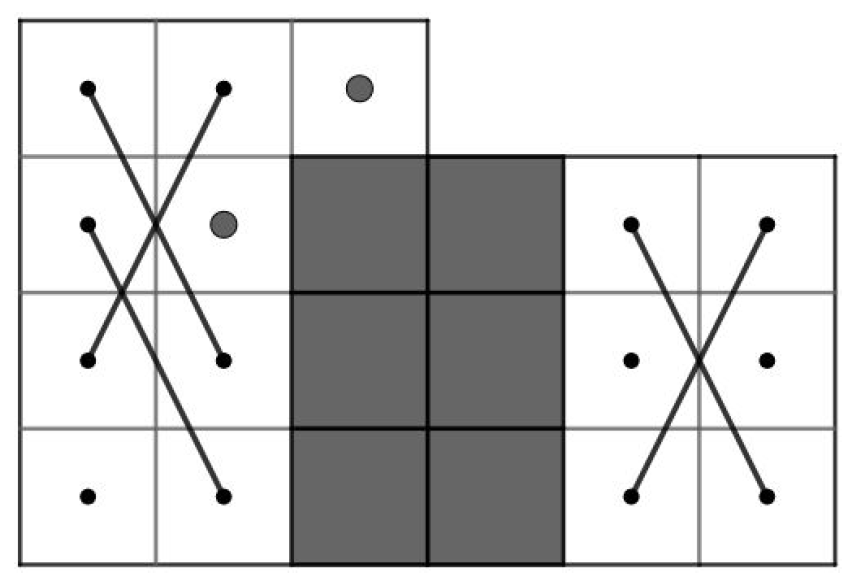
- (a)
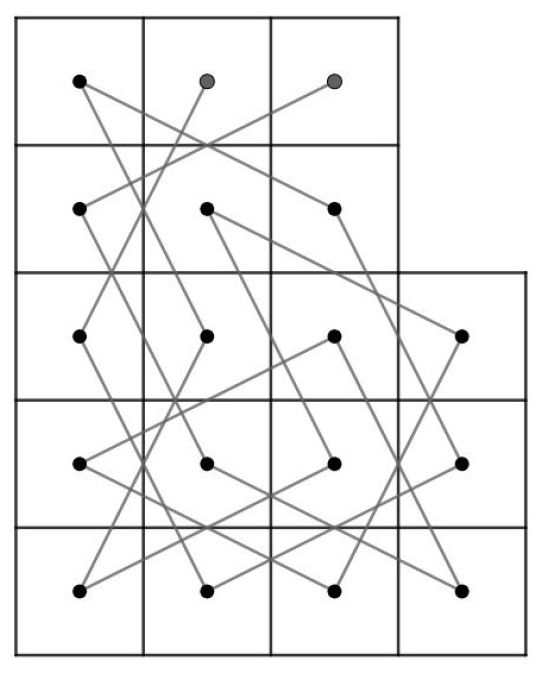
- The LB contains an OKT from to if and only if (i) is odd and or (ii) and .
- (b)
- The LB contains an OKT from to if and only if (i) is even and or (ii) and .
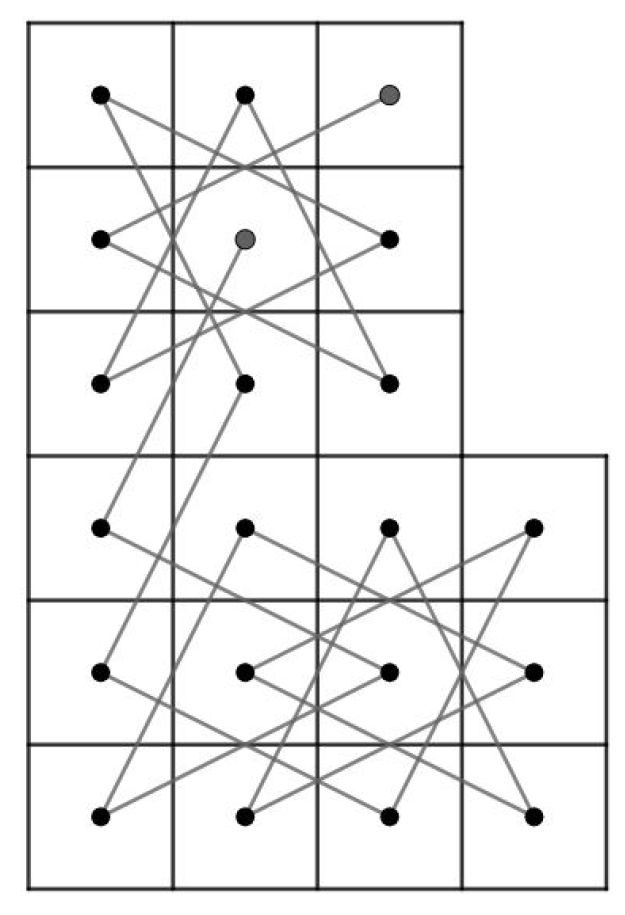
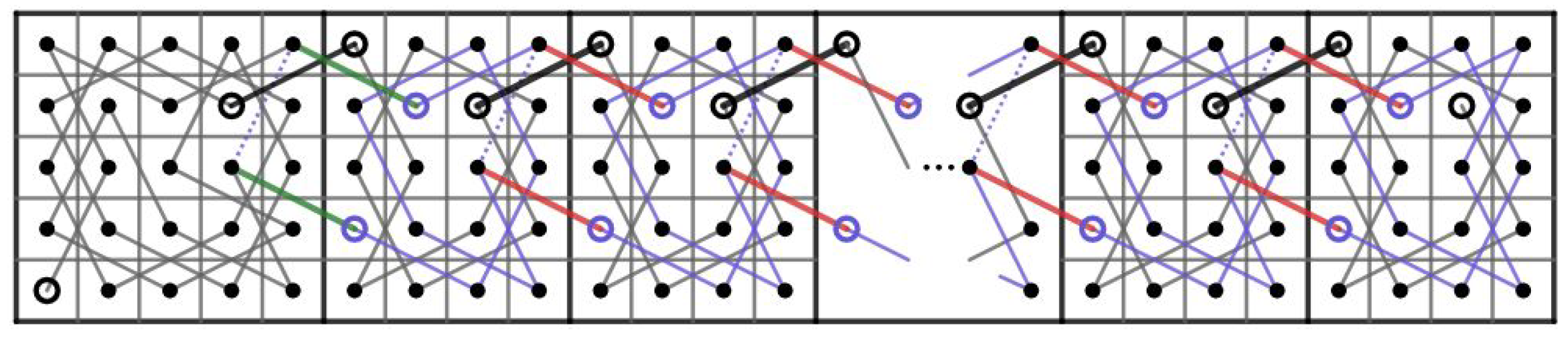
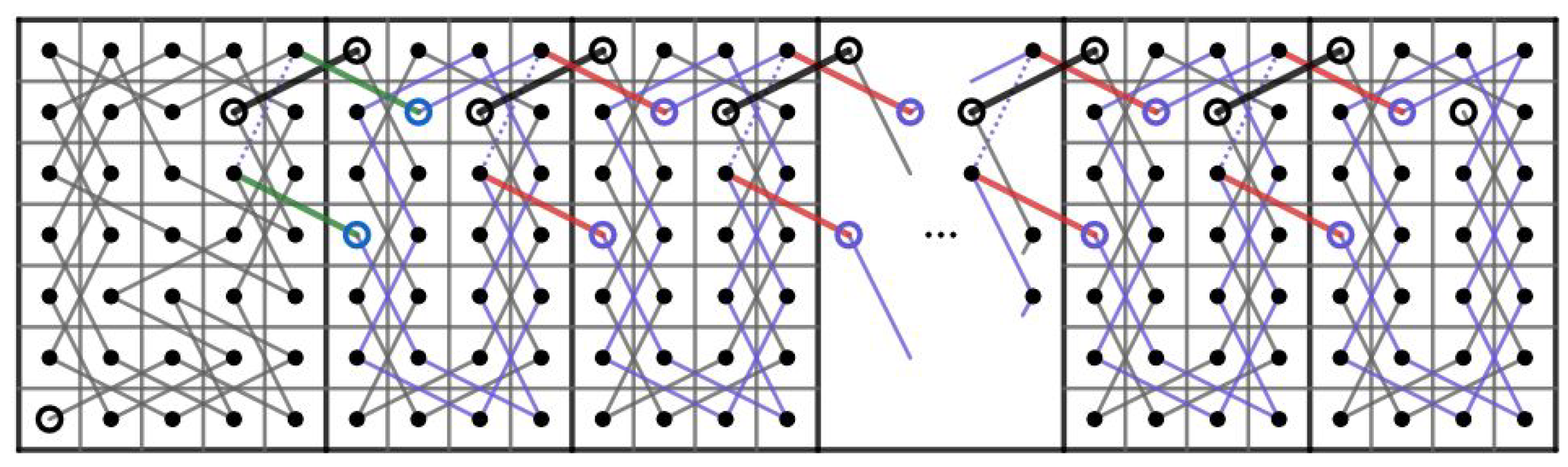
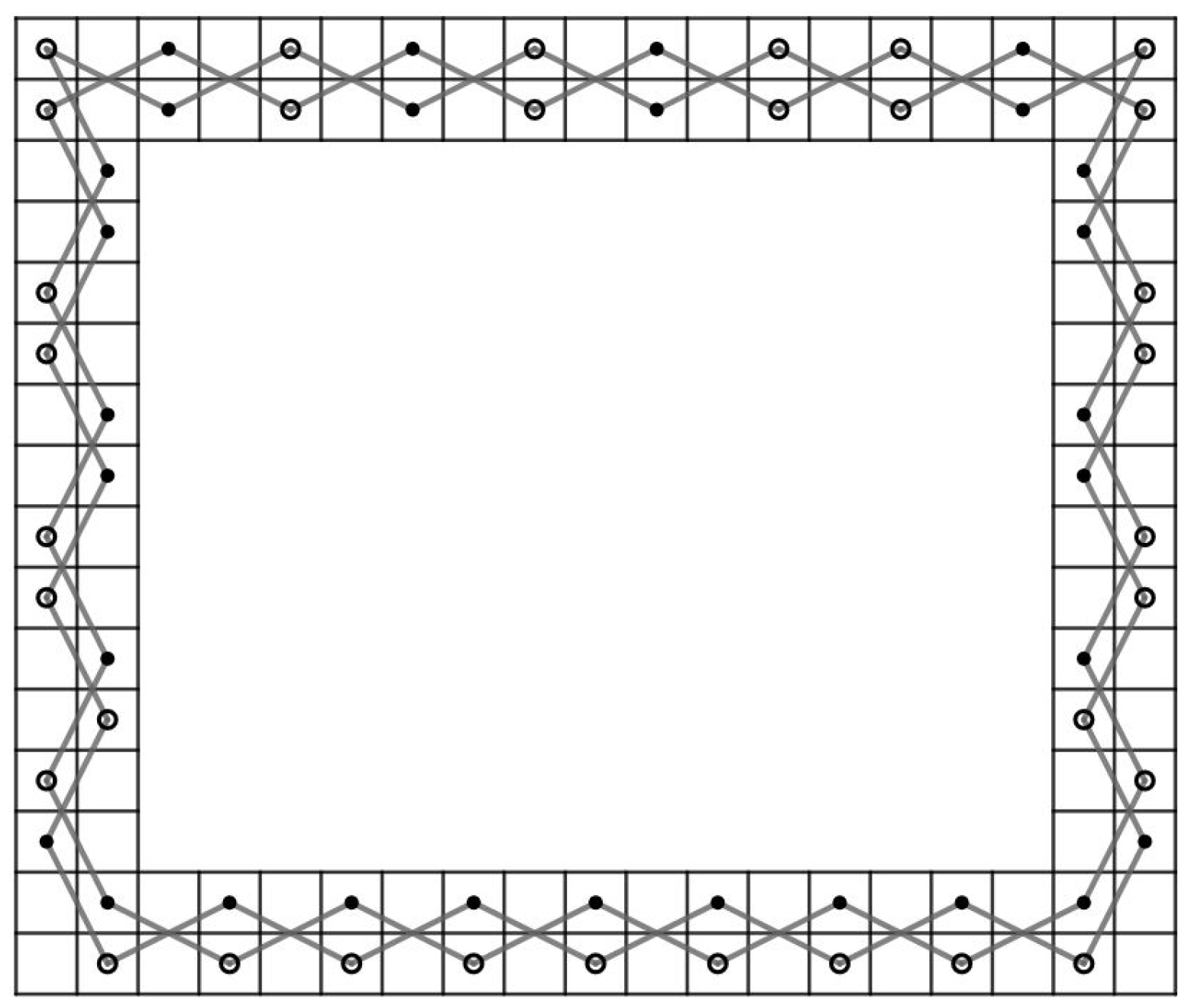
- (i)
- For , delete from the OKT of the ith CB();
- (ii)
- For , join and of the ith CB() to and of the th CB(), respectively.
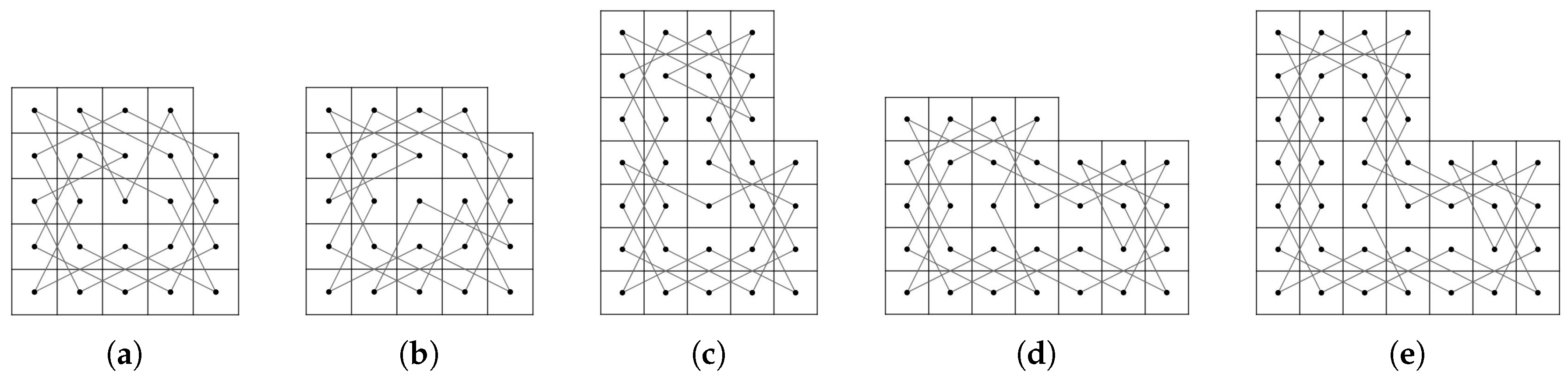
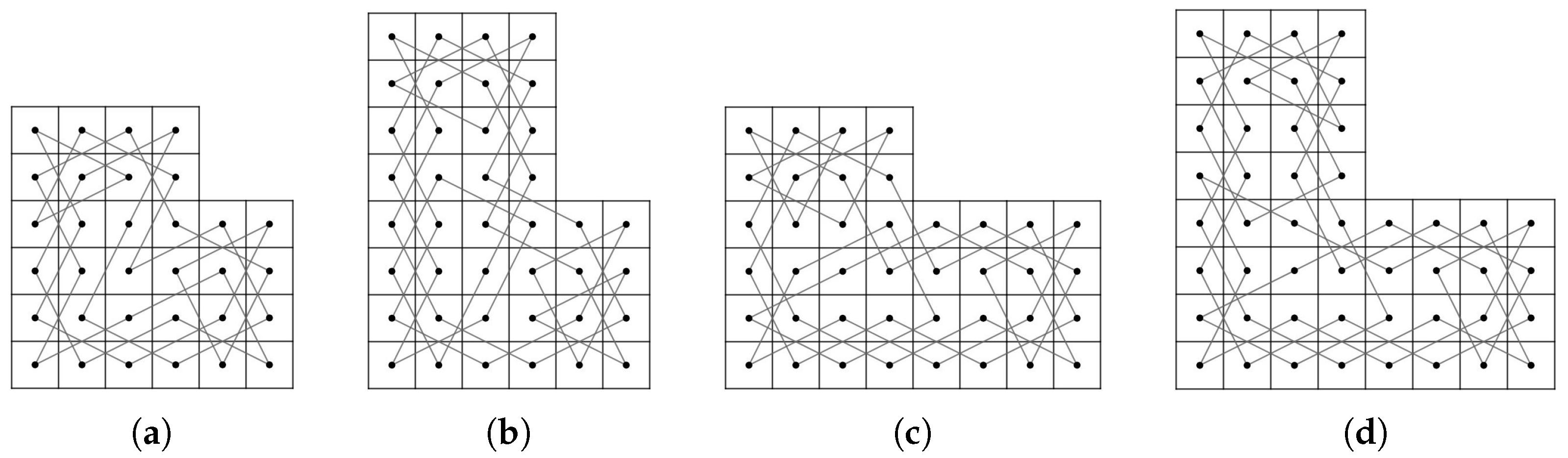
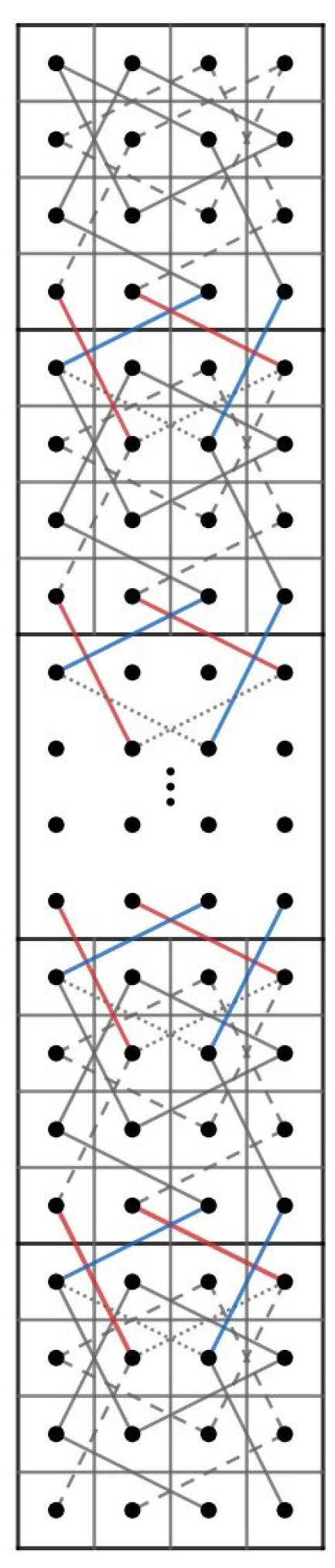
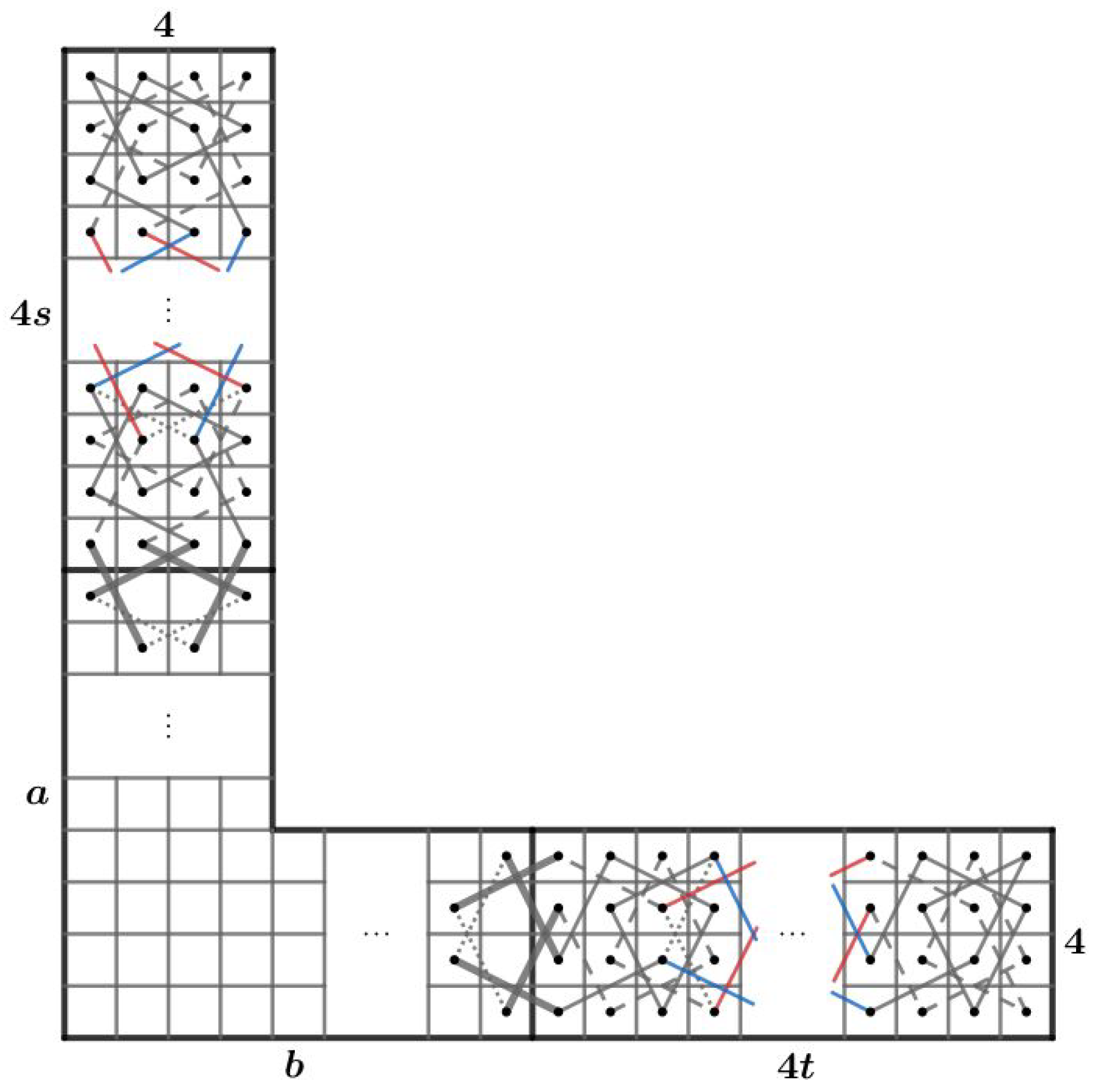
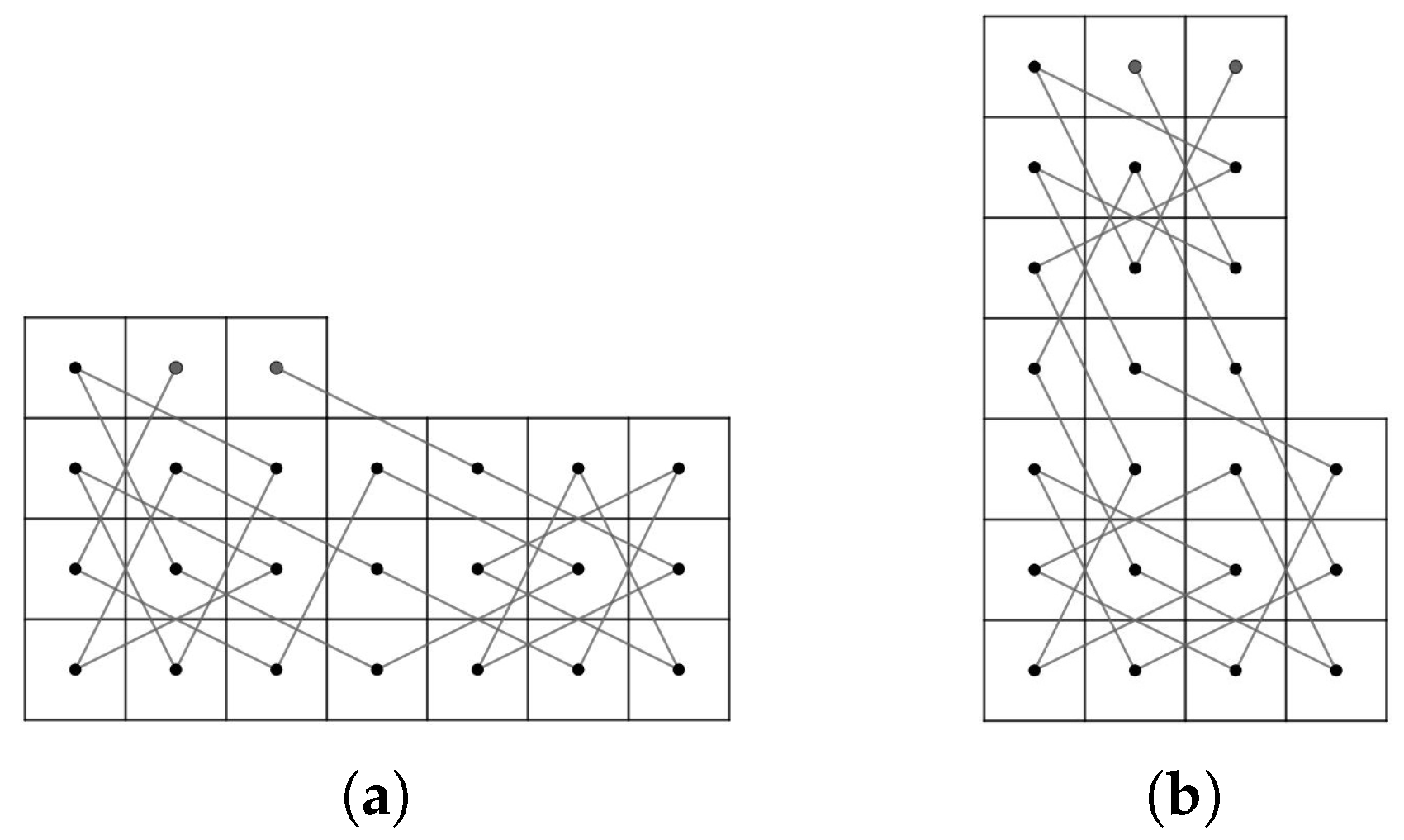
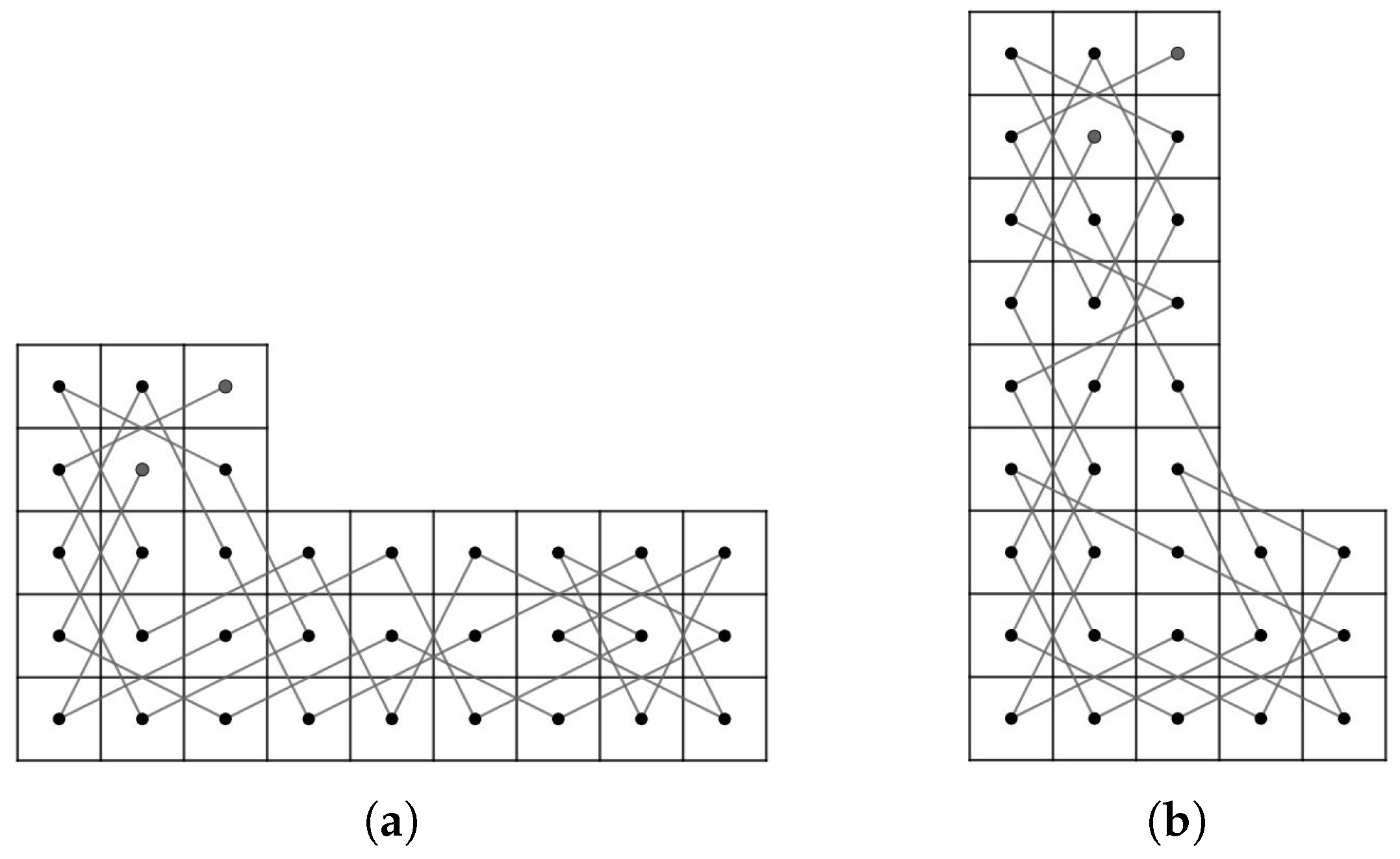
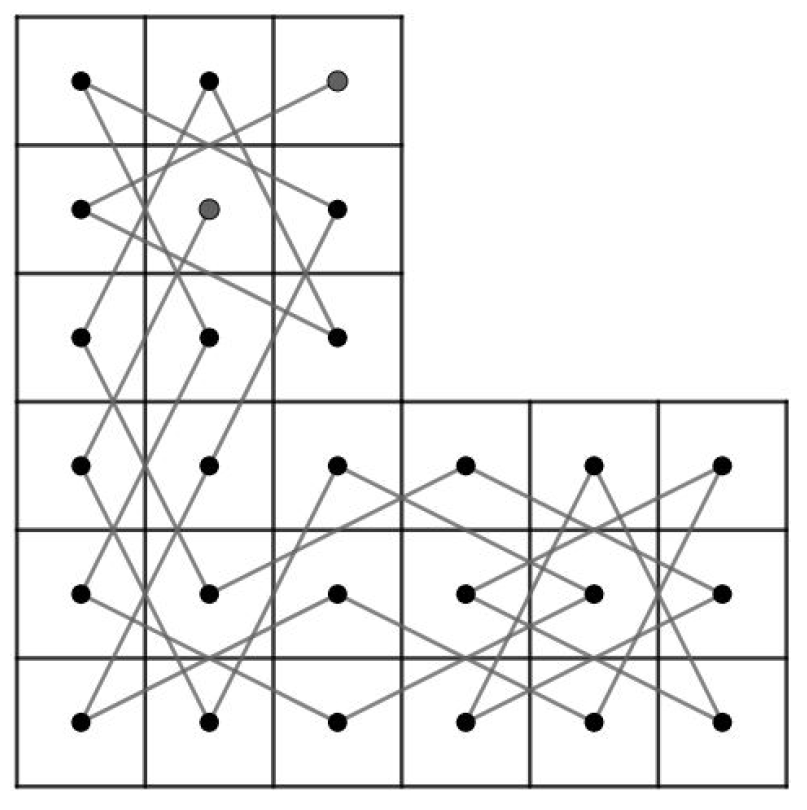
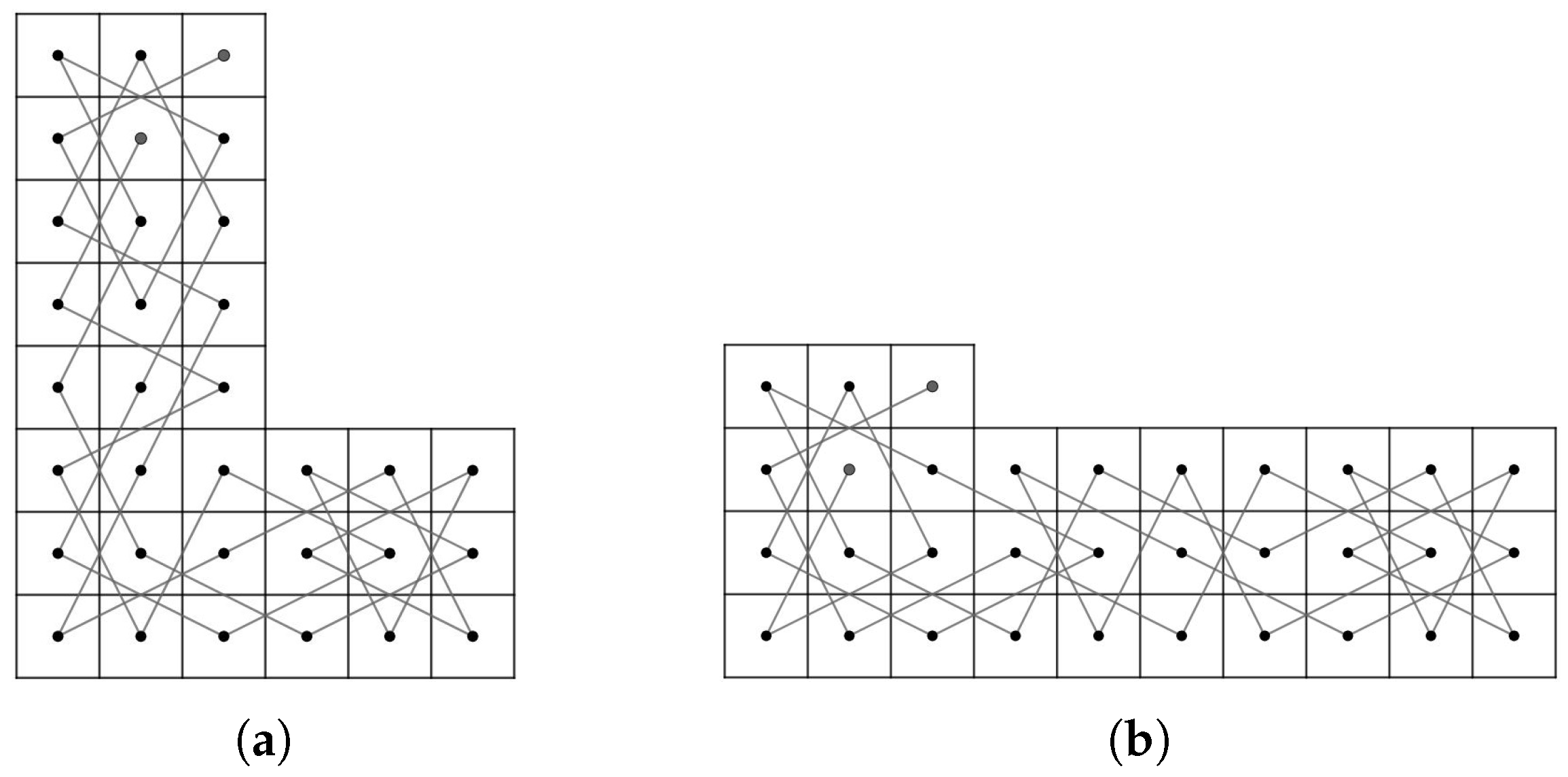
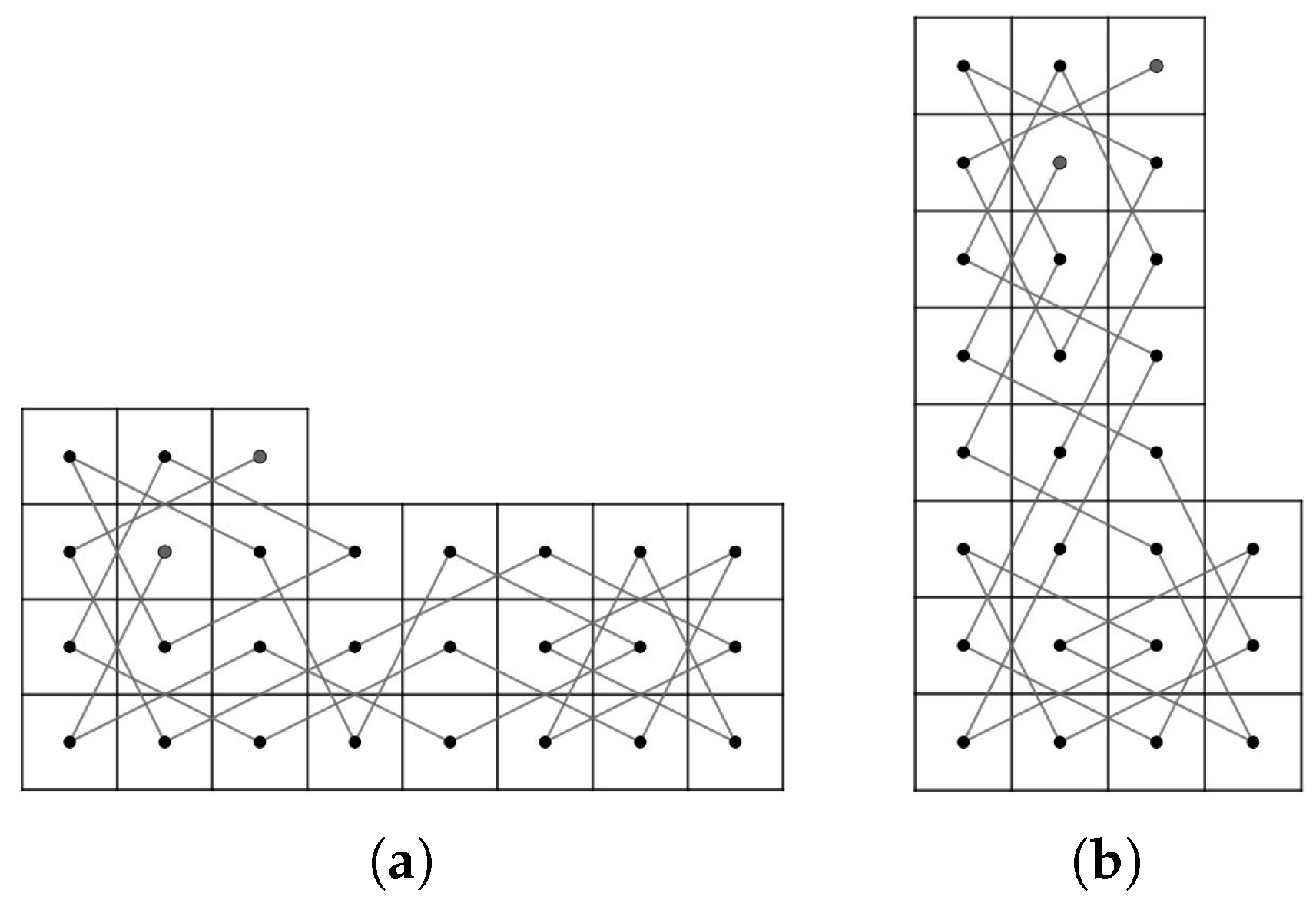
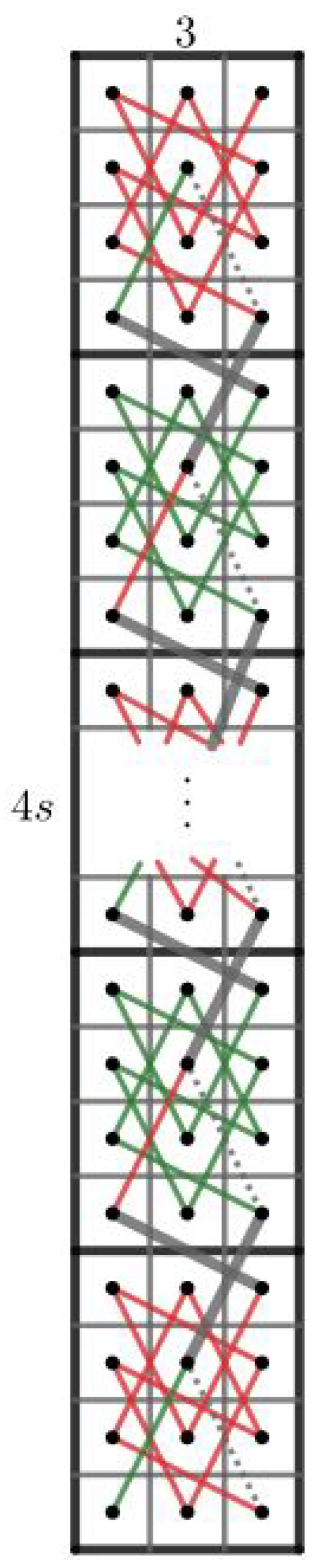
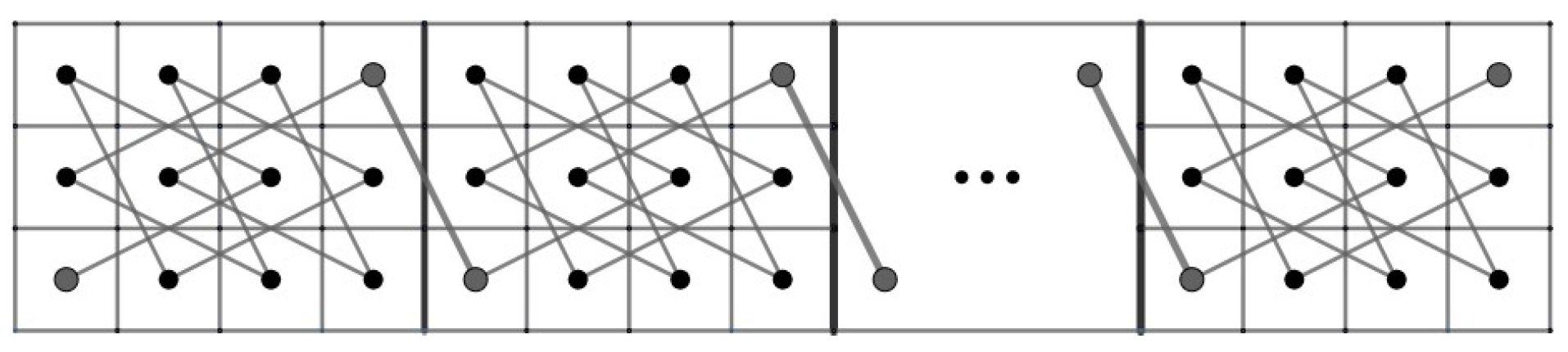
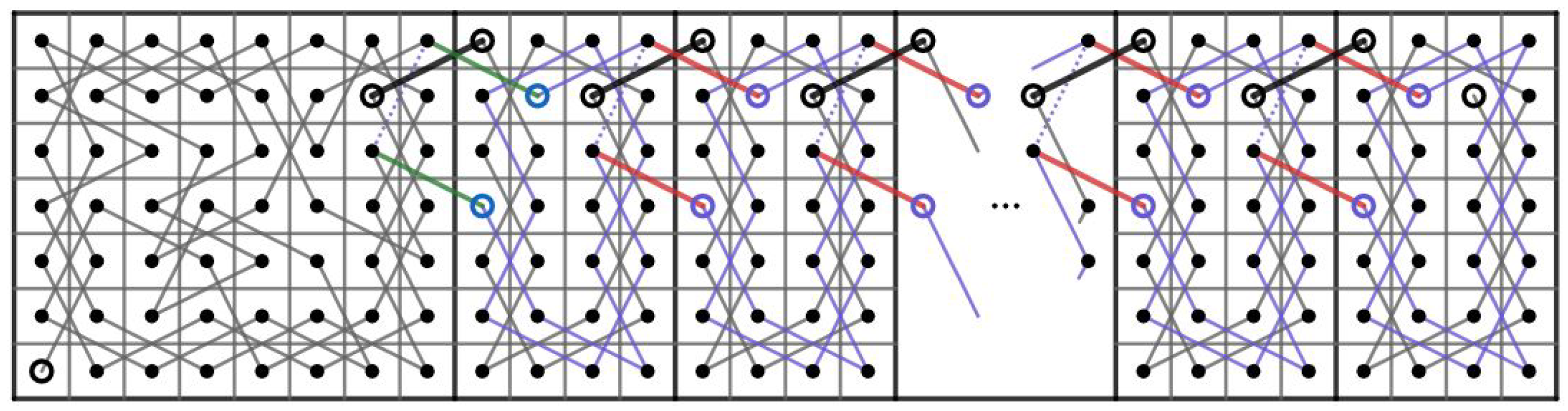
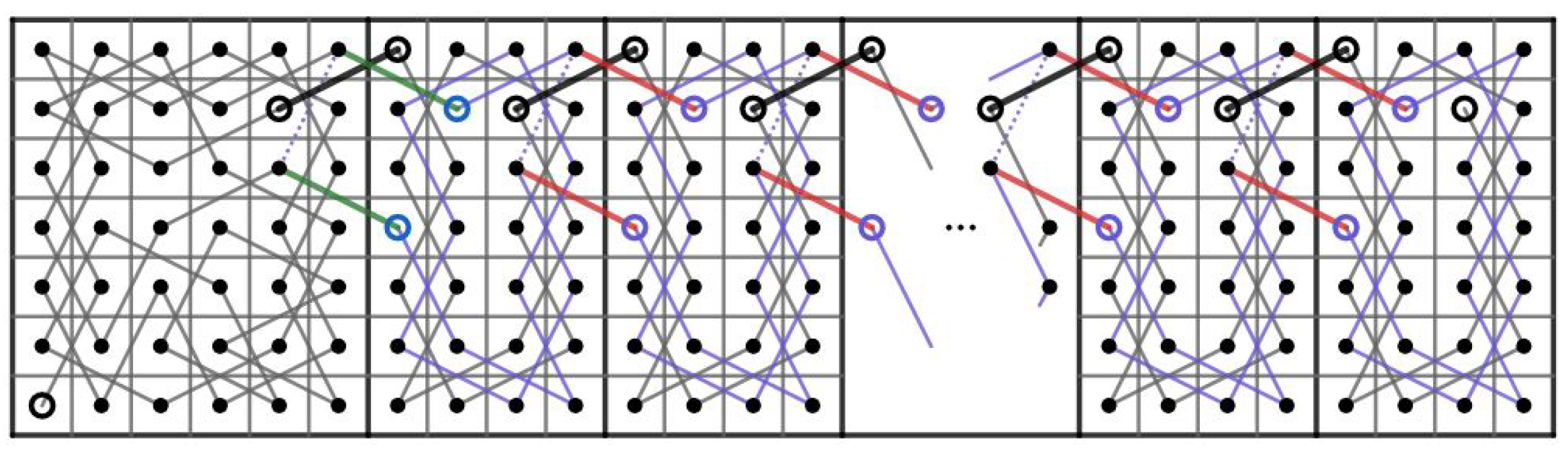
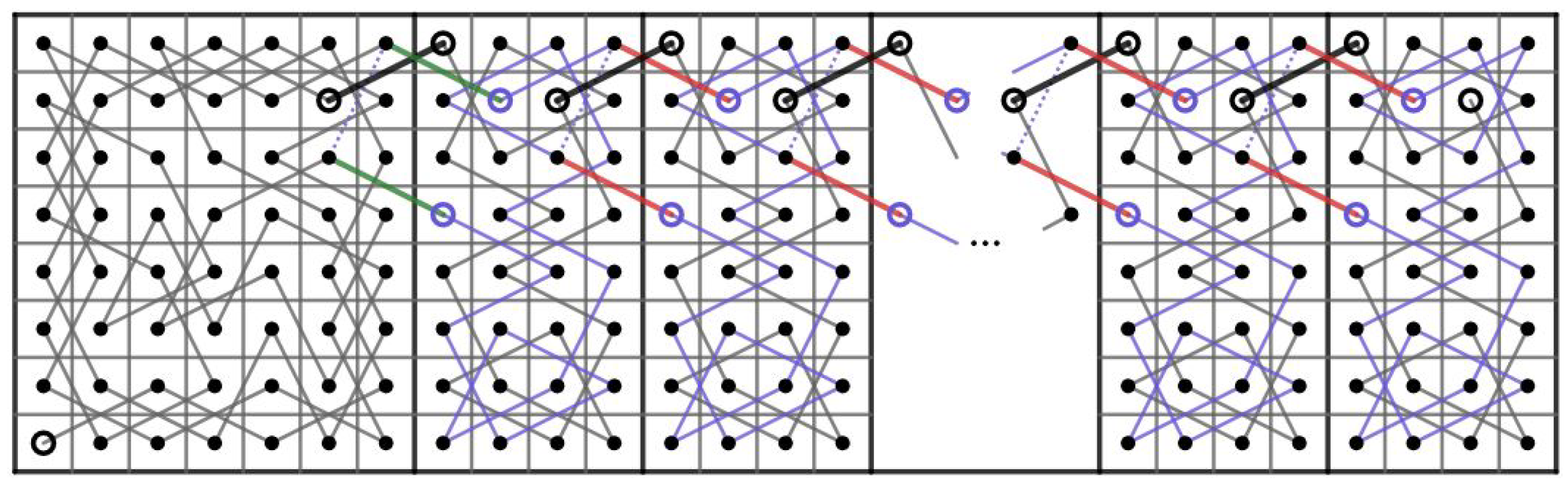
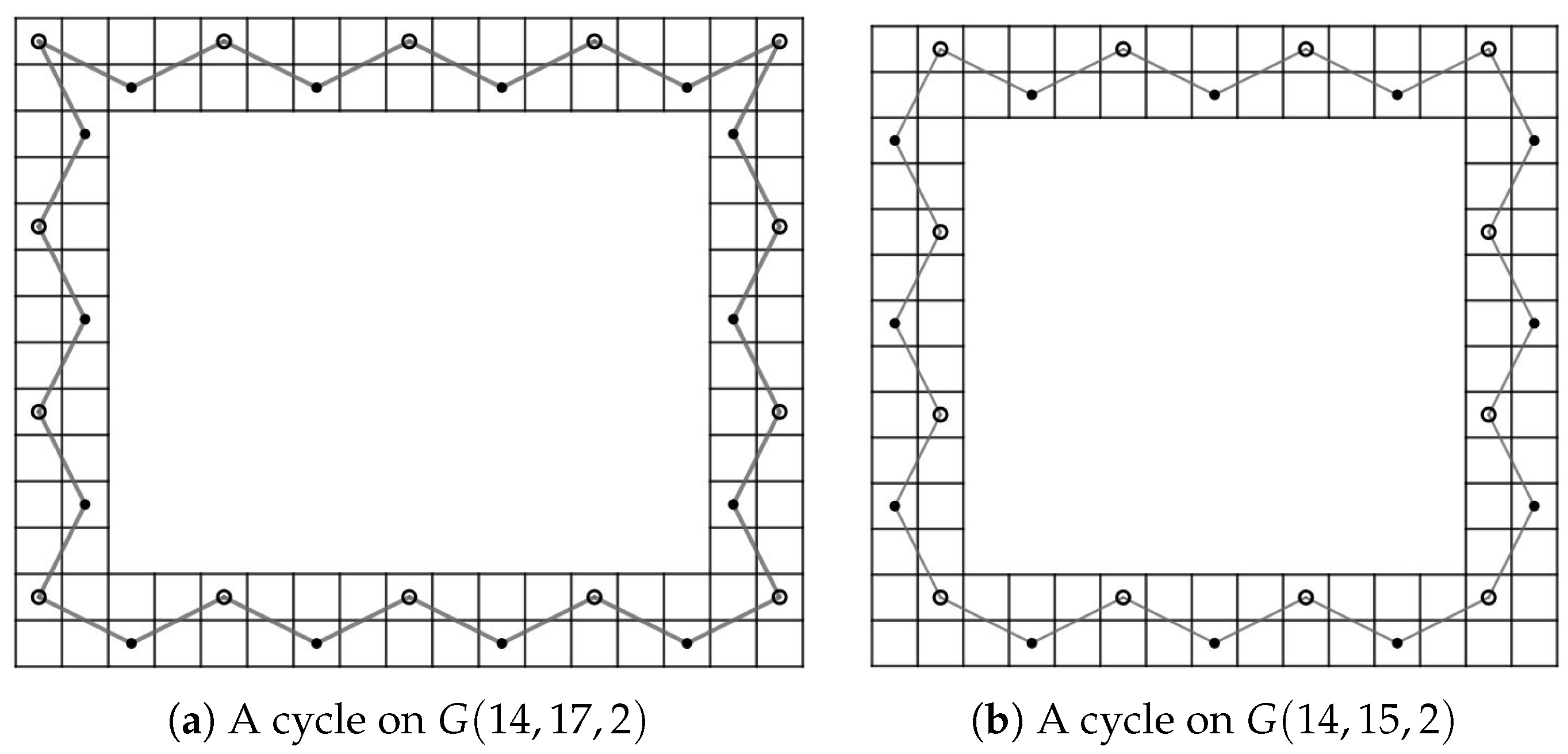
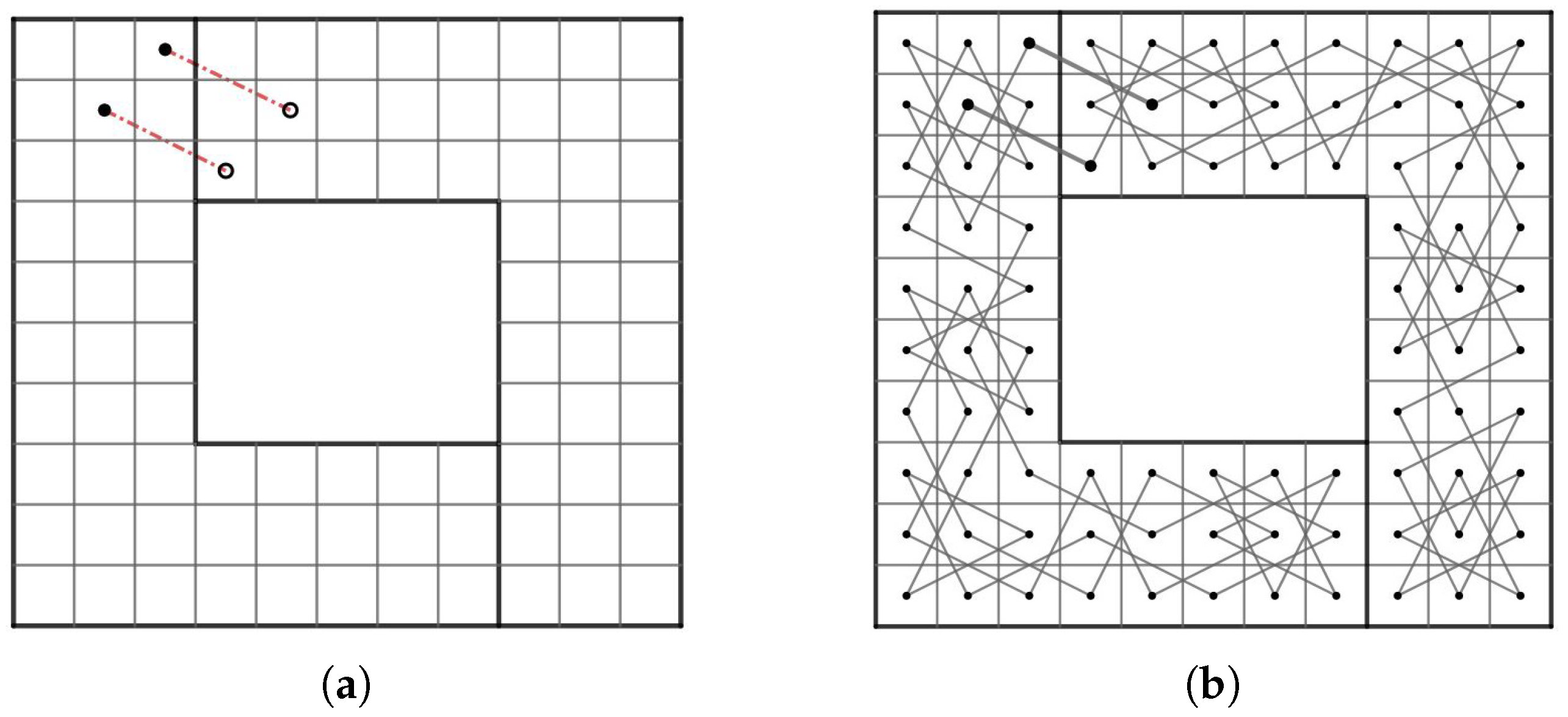
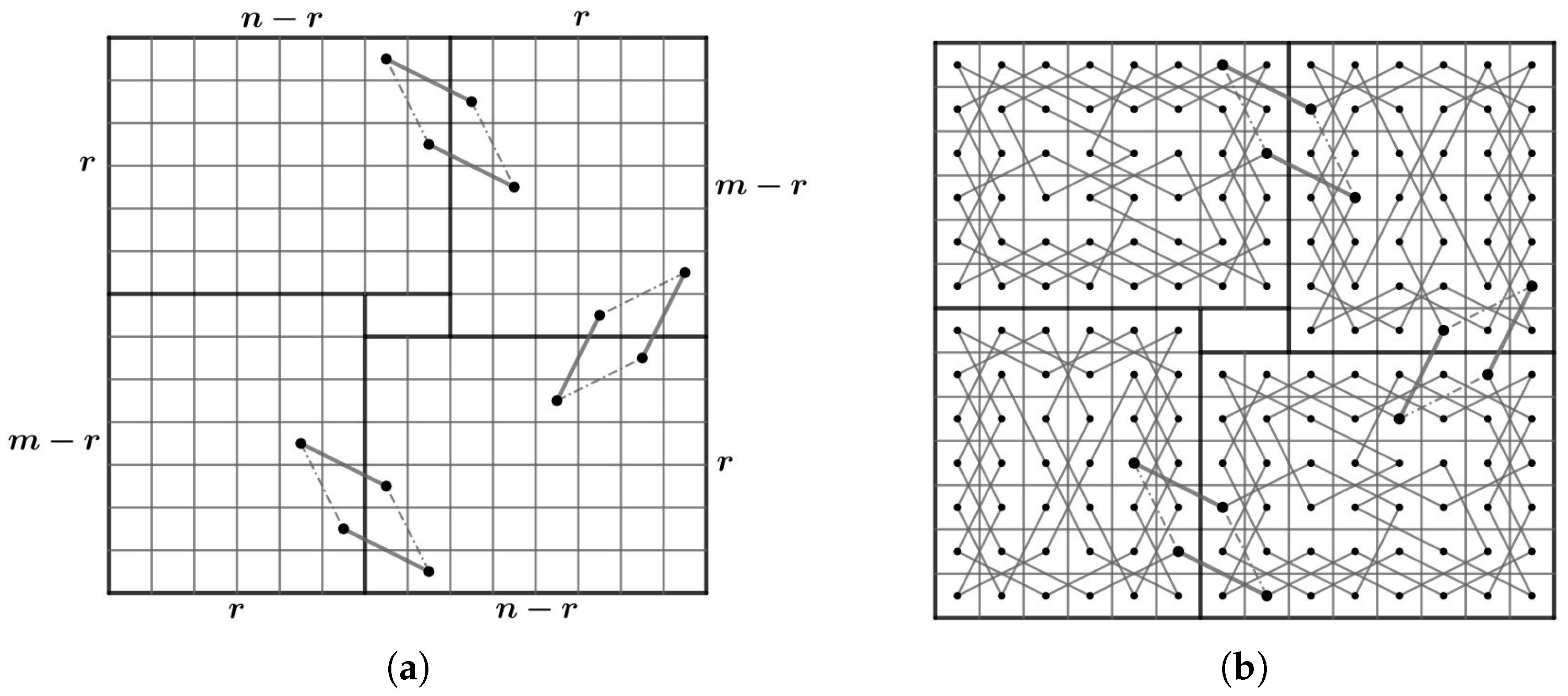
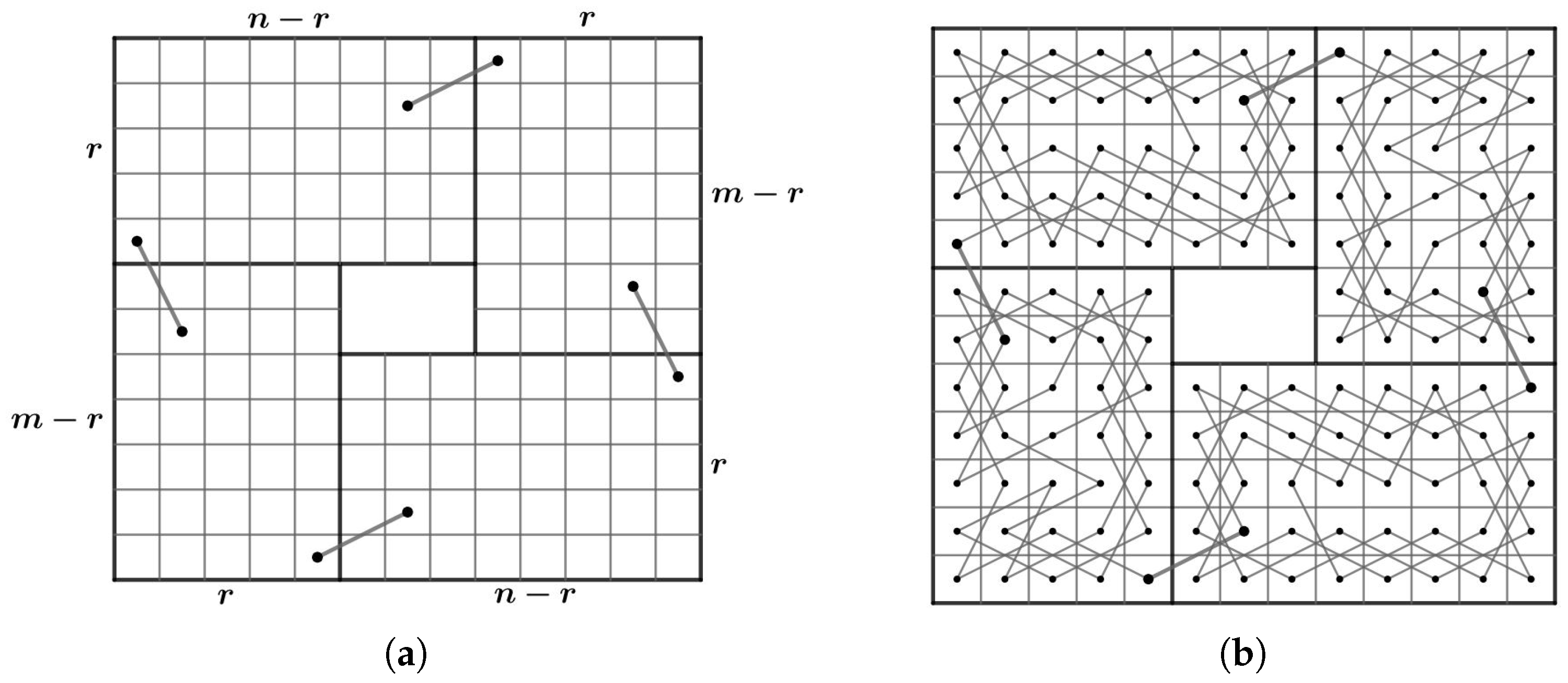
- Case 1: there exist nonnegative integers such that and . We divided the LB into subboards, CB() (Figure 17) and LB (Figure 11a) if and and LB (Figure 11a) and CB() (Figure 16) if and . Otherwise, we divide into three subboards, CB() (Figure 17), LB (Figure 11a) and CB() (Figure 16). Then, we construct the required OKT by the following two steps.
- (i)
- (ii)
- If and , then join and of the CB() to and of the LB, respectively. If and , then join and of the LB to and of the CB() chessboard, respectively. Otherwise, join four pairs of vertices together.
- Case 2: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 11b), and , respectively.
- Case 3: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 12a), and , respectively.
- Case 4: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 12b), and , respectively.
- Case 5: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 13a), and , respectively.
- Case 6: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 13b), and , respectively.
- Case 7: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 10), and , respectively.
- Case 8: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 14a), and , respectively.
- Case 9: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 14b), and , respectively.
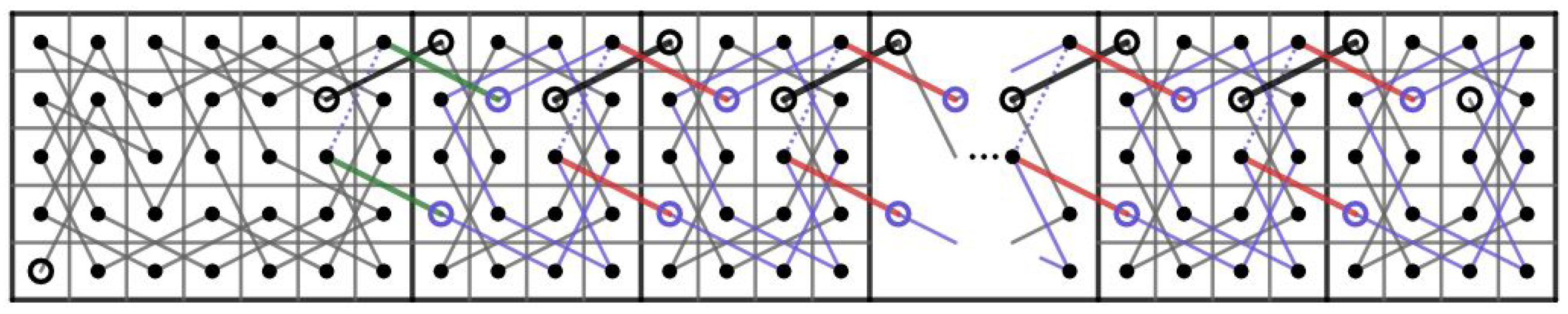
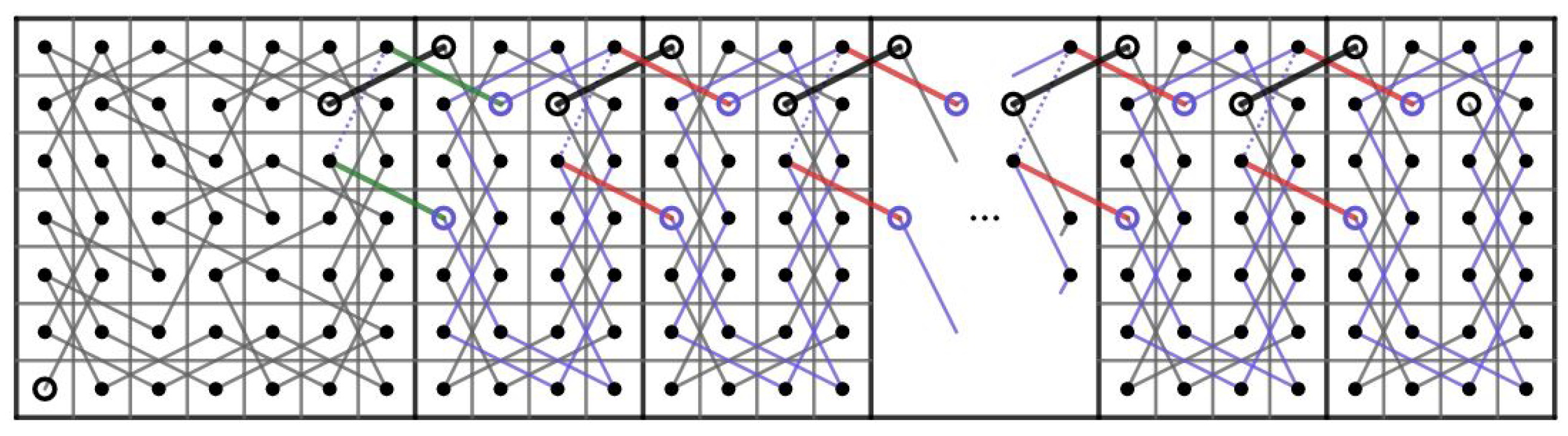
- (i)
- For , delete from the OKT of the ith CB();
- (ii)
- For , join and of the ith CB() to and of the th CB(), respectively.
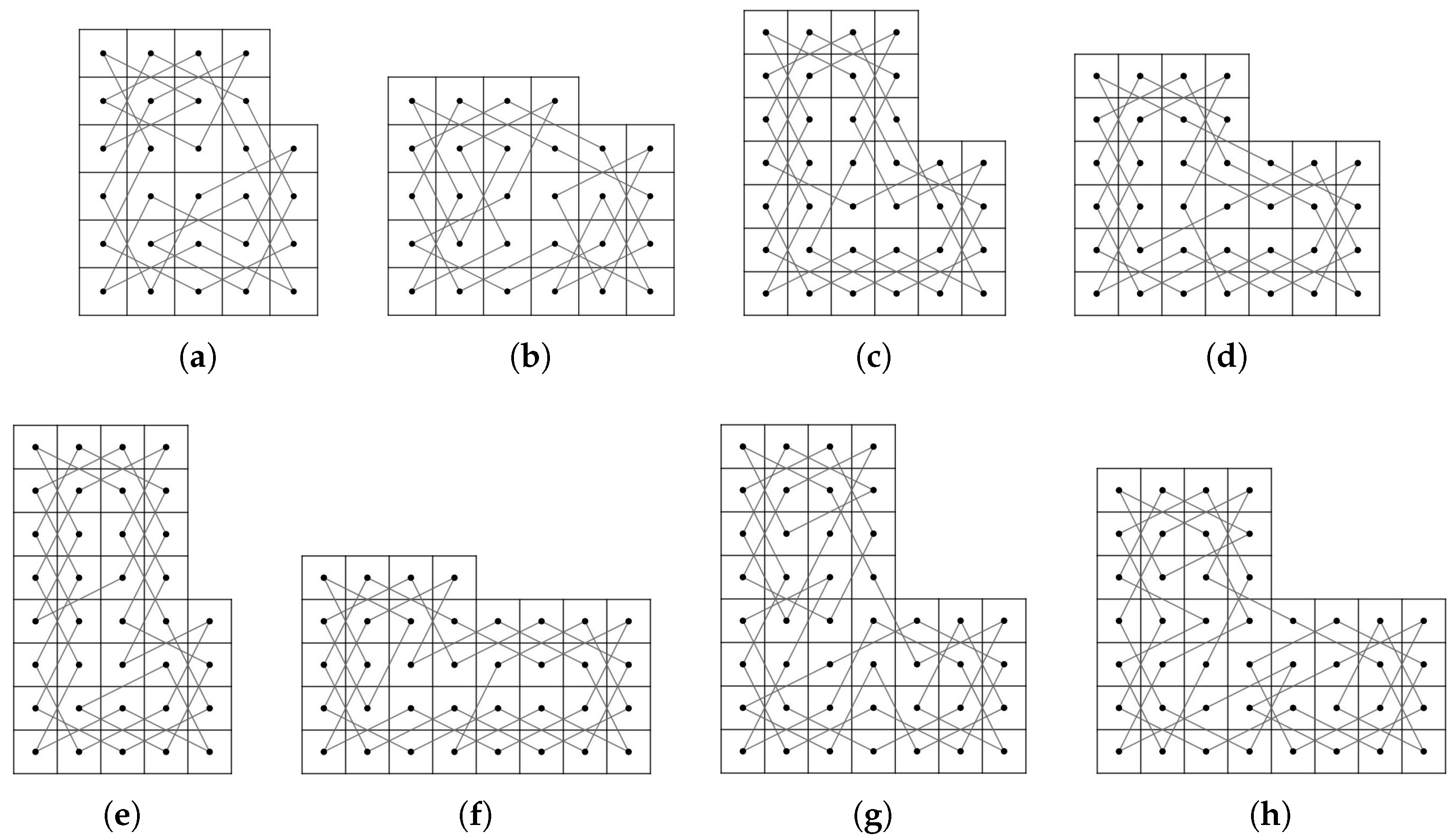
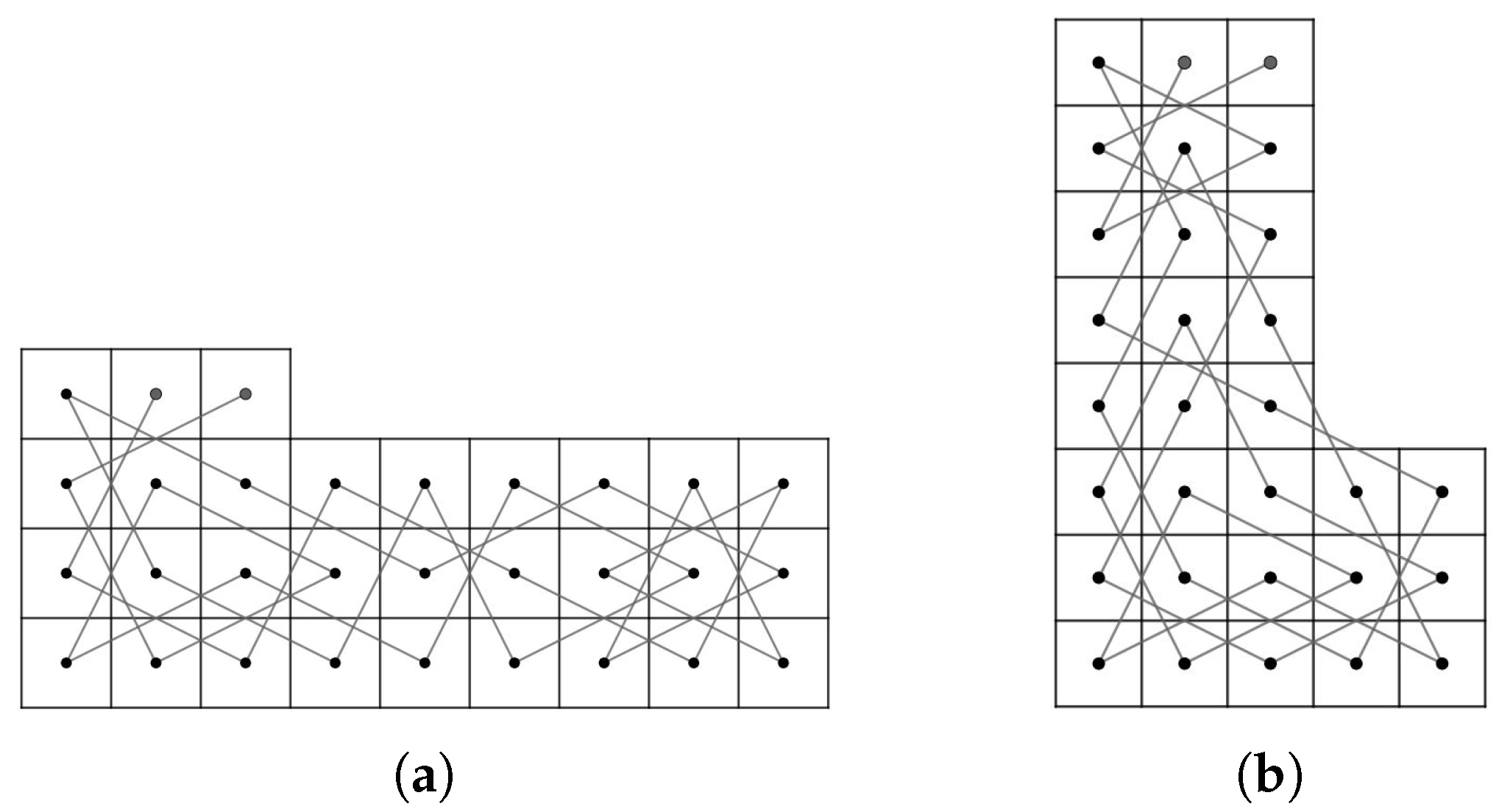
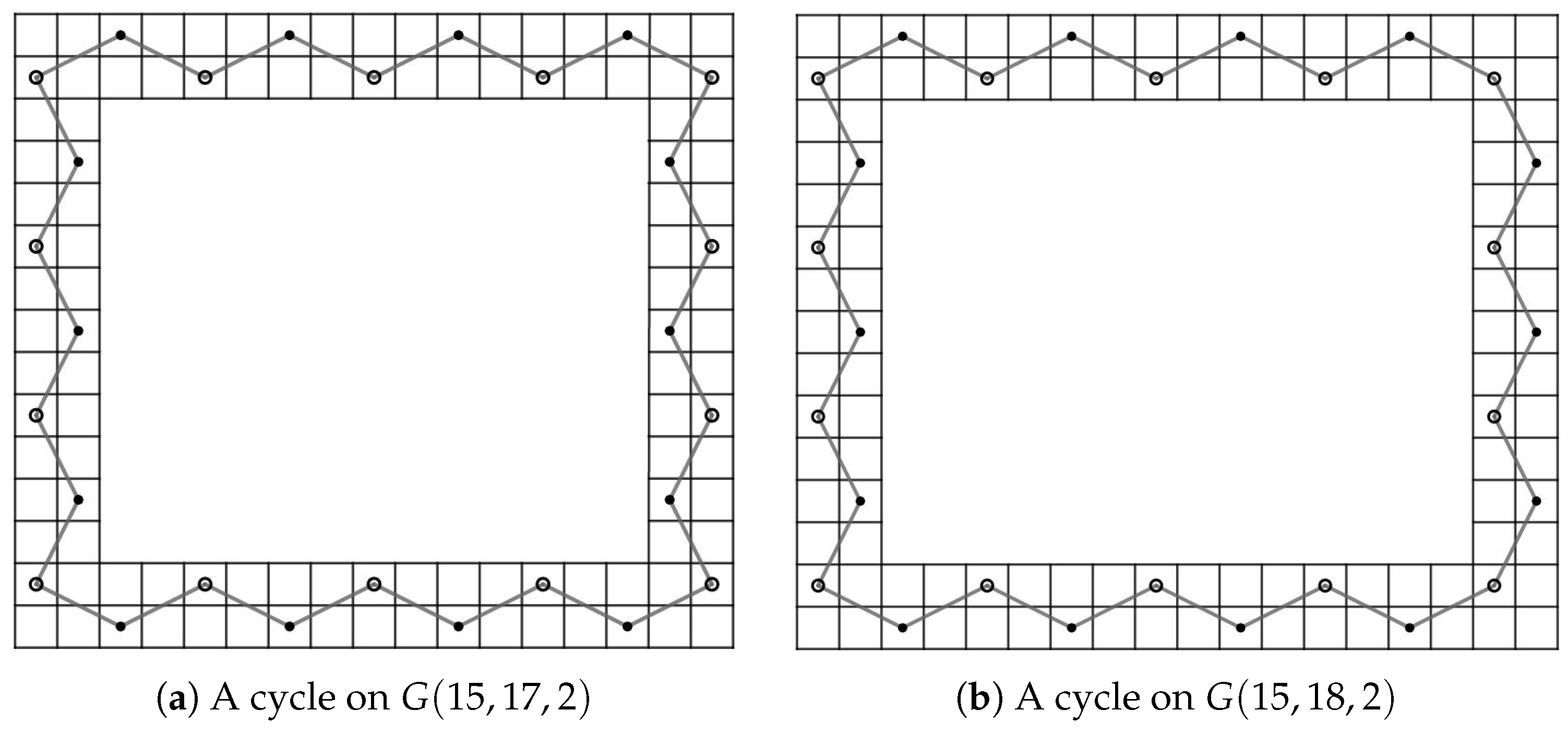
- Case 1: there exist nonnegative integers such that and . We divide the LB into subboards, CB() (Figure 29) and LB (Figure 22a) if and and LB (Figure 22a) and CB() (Figure 16) if and . Otherwise, we divide into three subboards, CB() (Figure 29), LB (Figure 22a) and CB() (Figure 16). Then, we construct the required OKT by the followings.
- Case 2: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 22b), and , respectively.
- Case 3: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 23), and , respectively.
- Case 4: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 24a), and , respectively.
- Case 5: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 24b), and , respectively.
- Case 6: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 25), and , respectively.
- Case 7: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 26a), and , respectively.
- Case 8: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 26b), and , respectively.
- Case 9: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 21), and , respectively.
- Case 10: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 27a), and , respectively.
- Case 11: there exist nonnegative integers such that and . Then, we construct the required OKT by using the same procedure as we did in Case 1 but LB, and are replaced by LB (Figure 27b), and , respectively.
- (a)
- The 7B contains an OKT from to if and only if (i) is odd and or (ii) and .
- (b)
- The 7B contains an OKT from to if and only if (i) is even and or (ii) and .
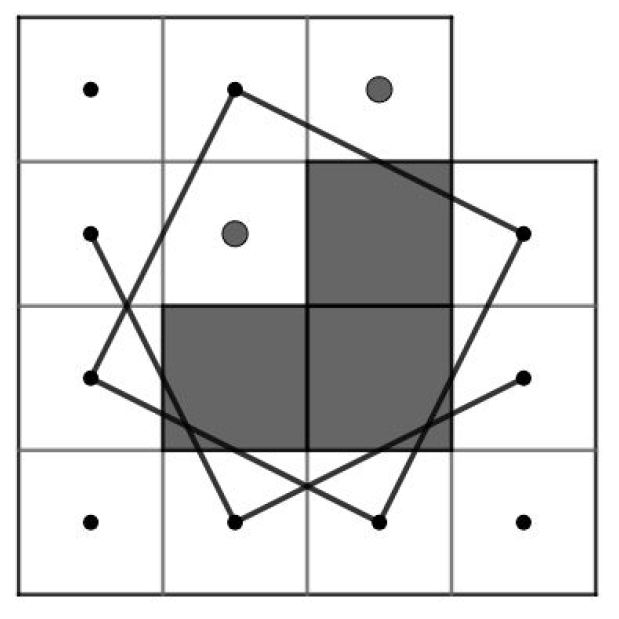
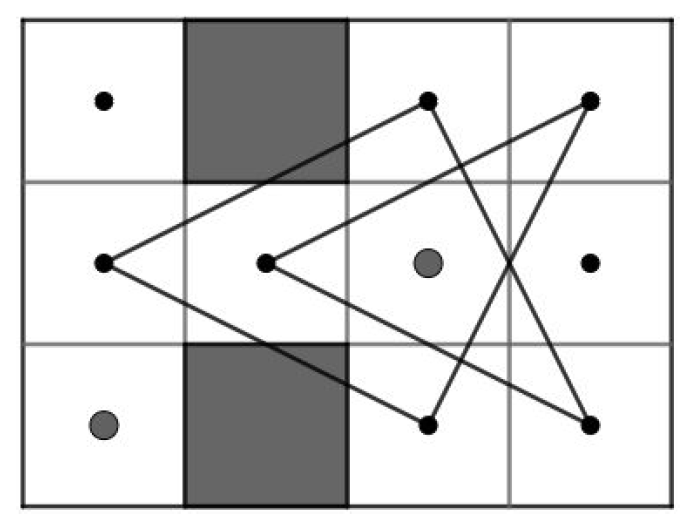
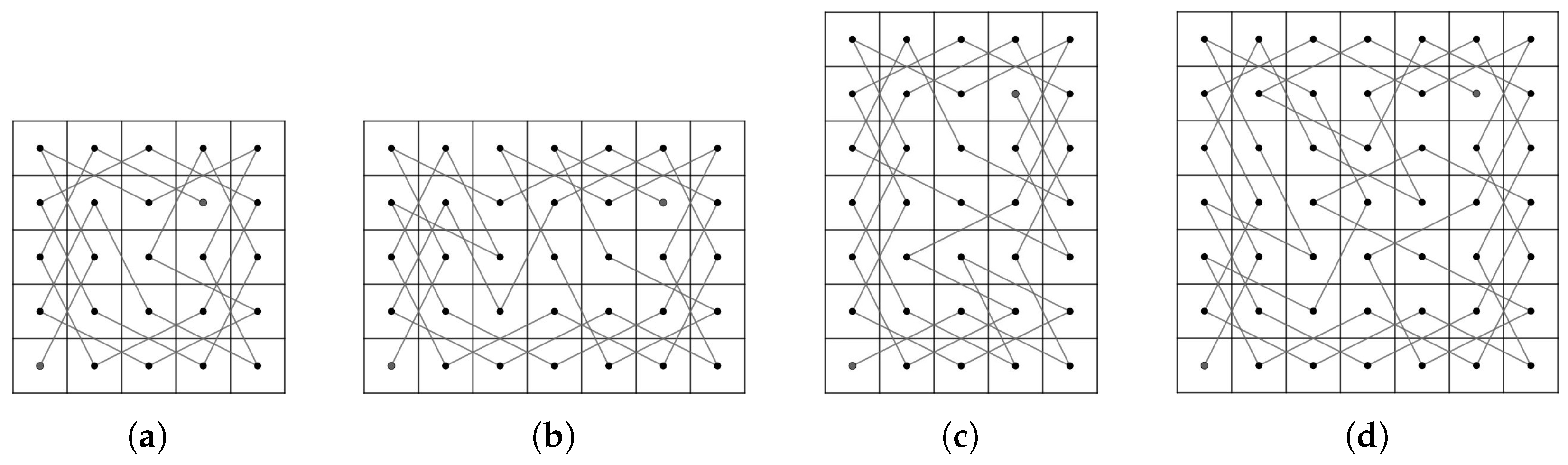
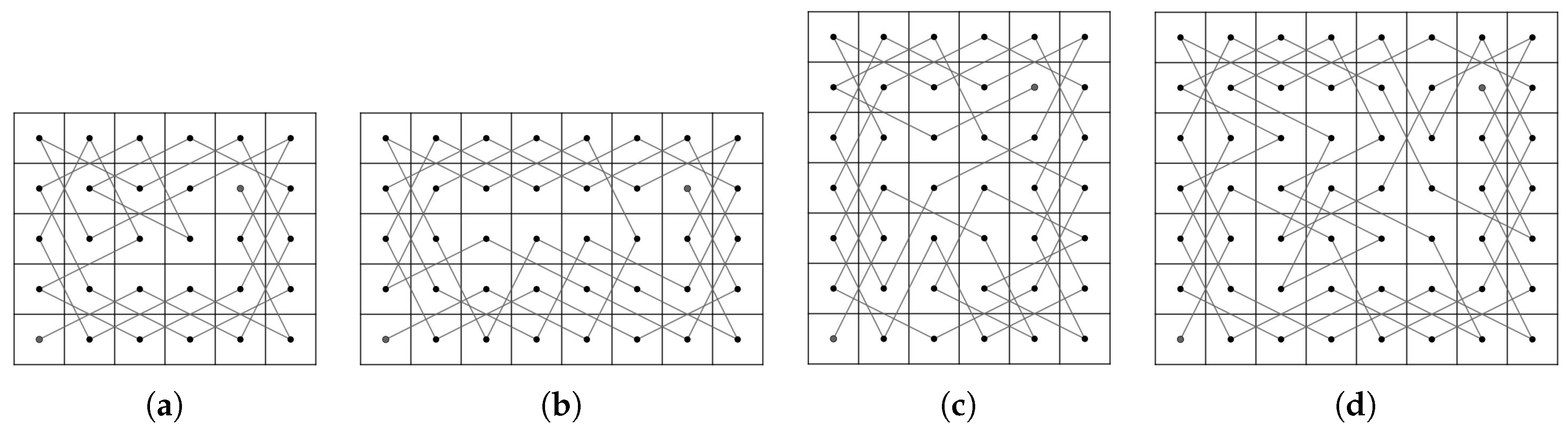
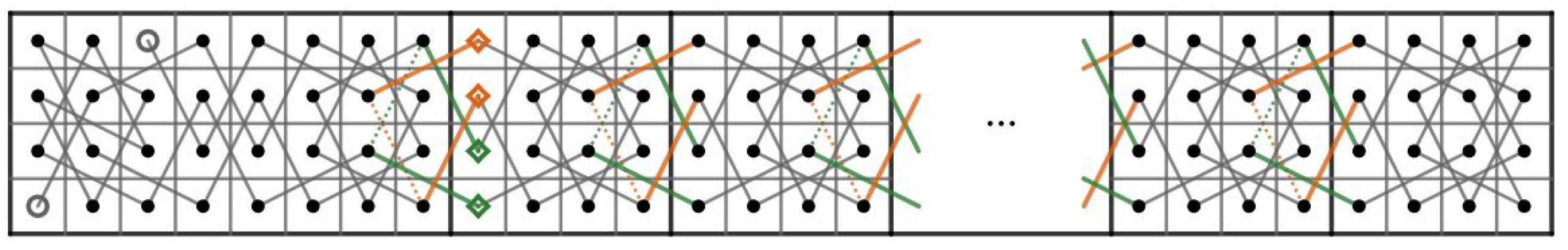
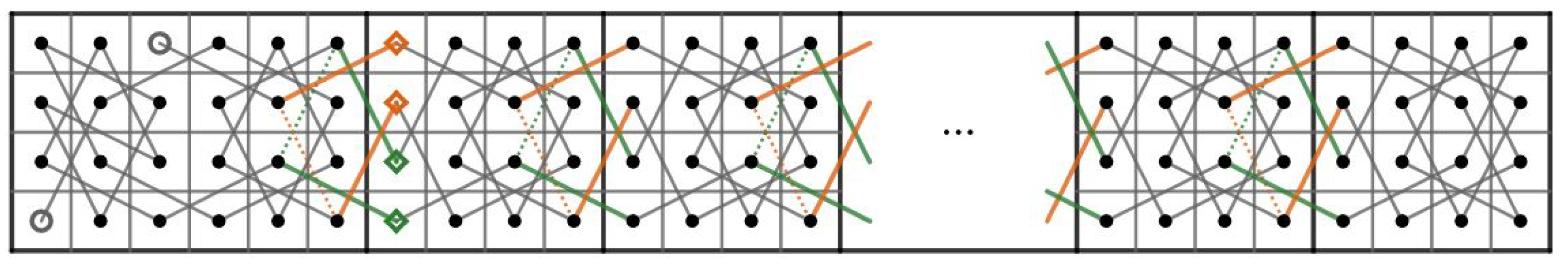
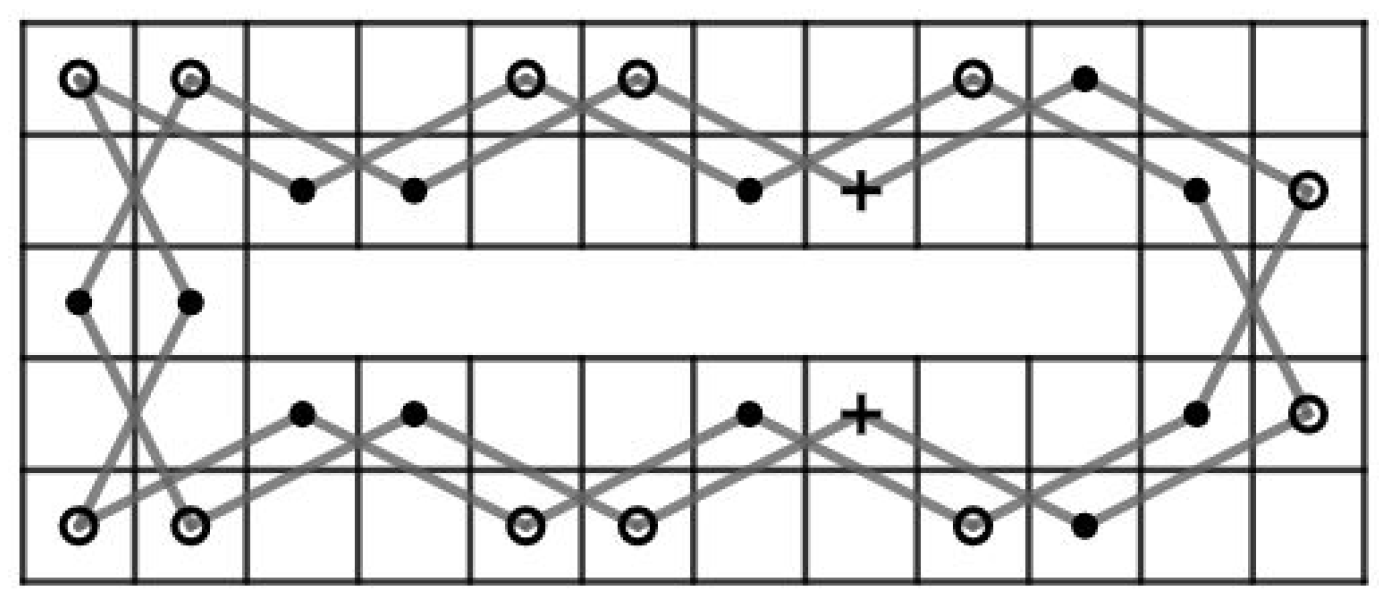
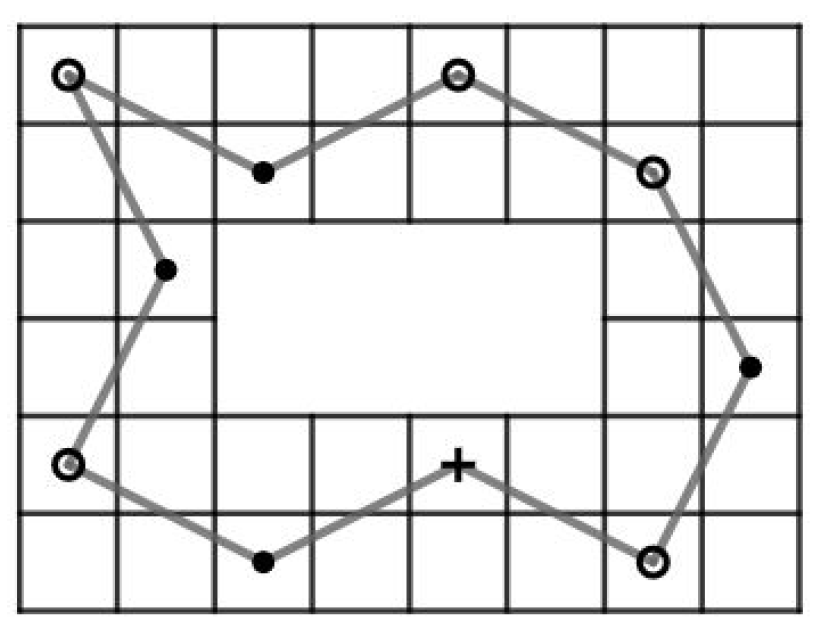
3. Existence of a Special OKT on CB()
- (a)
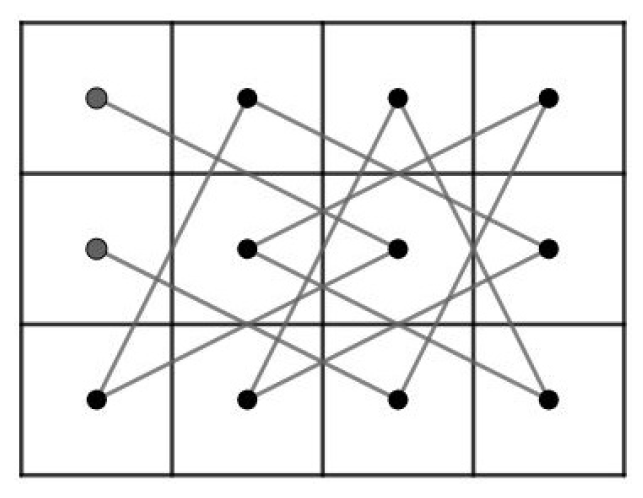
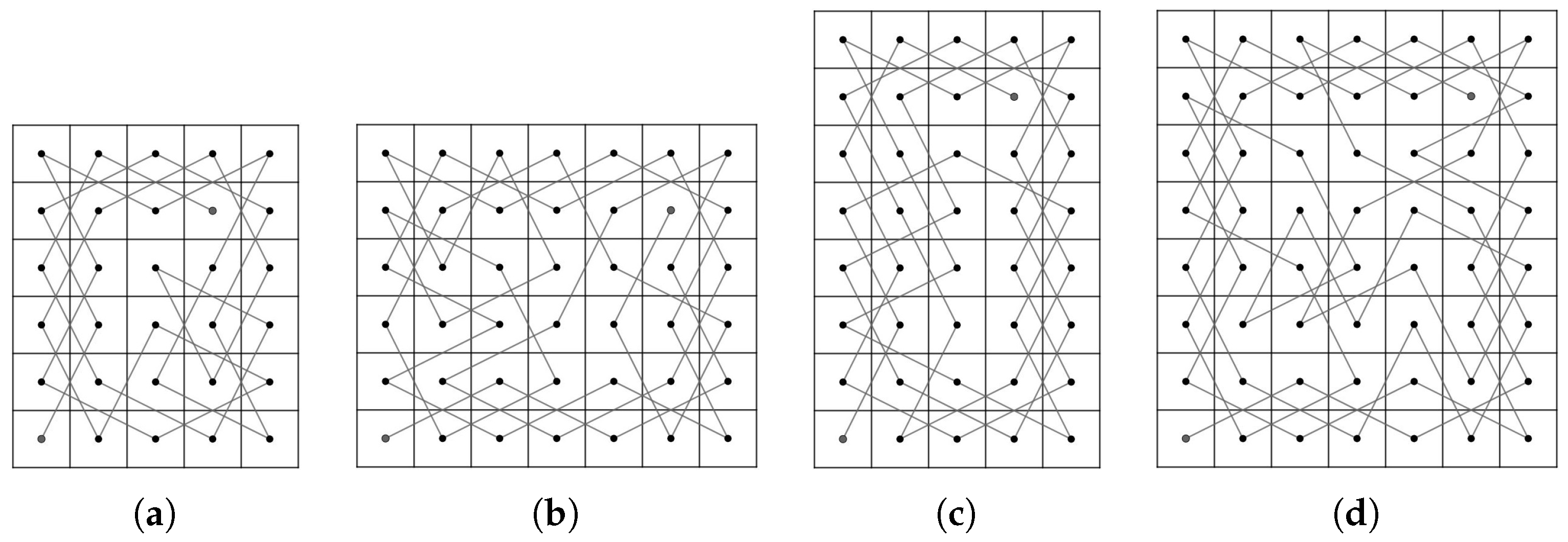
- Let and . Then, a CB() contains an OKT from to if and only if and .
- (b)
- Let . Then, a CB() contains an OKT from to if and only if m and n are not both even.
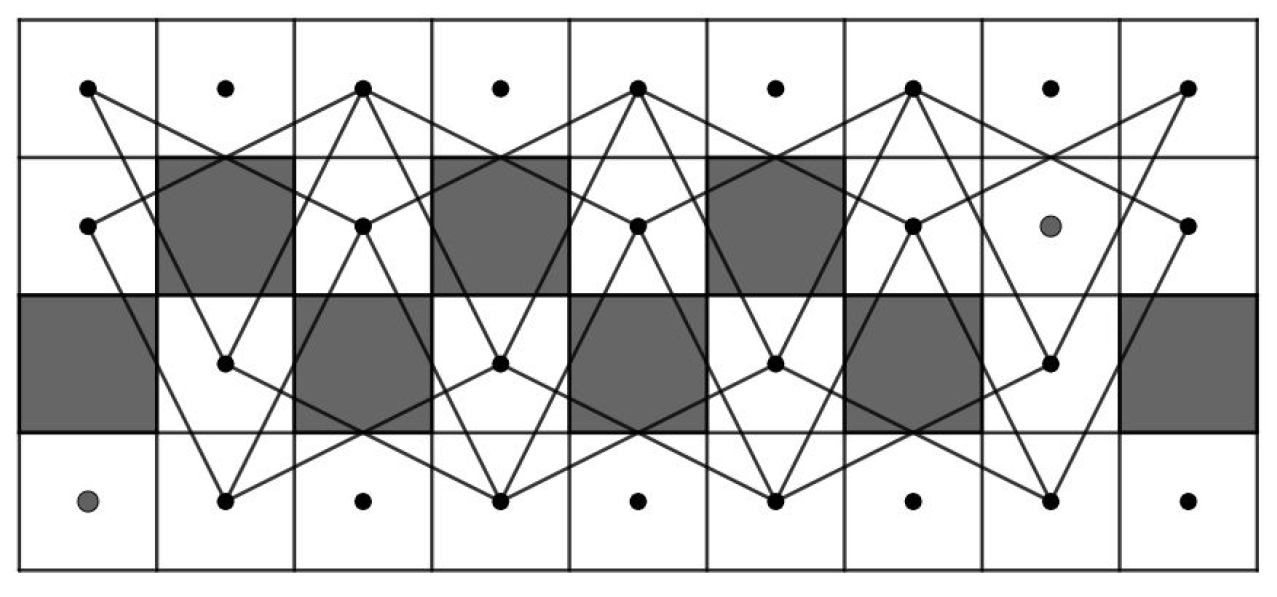
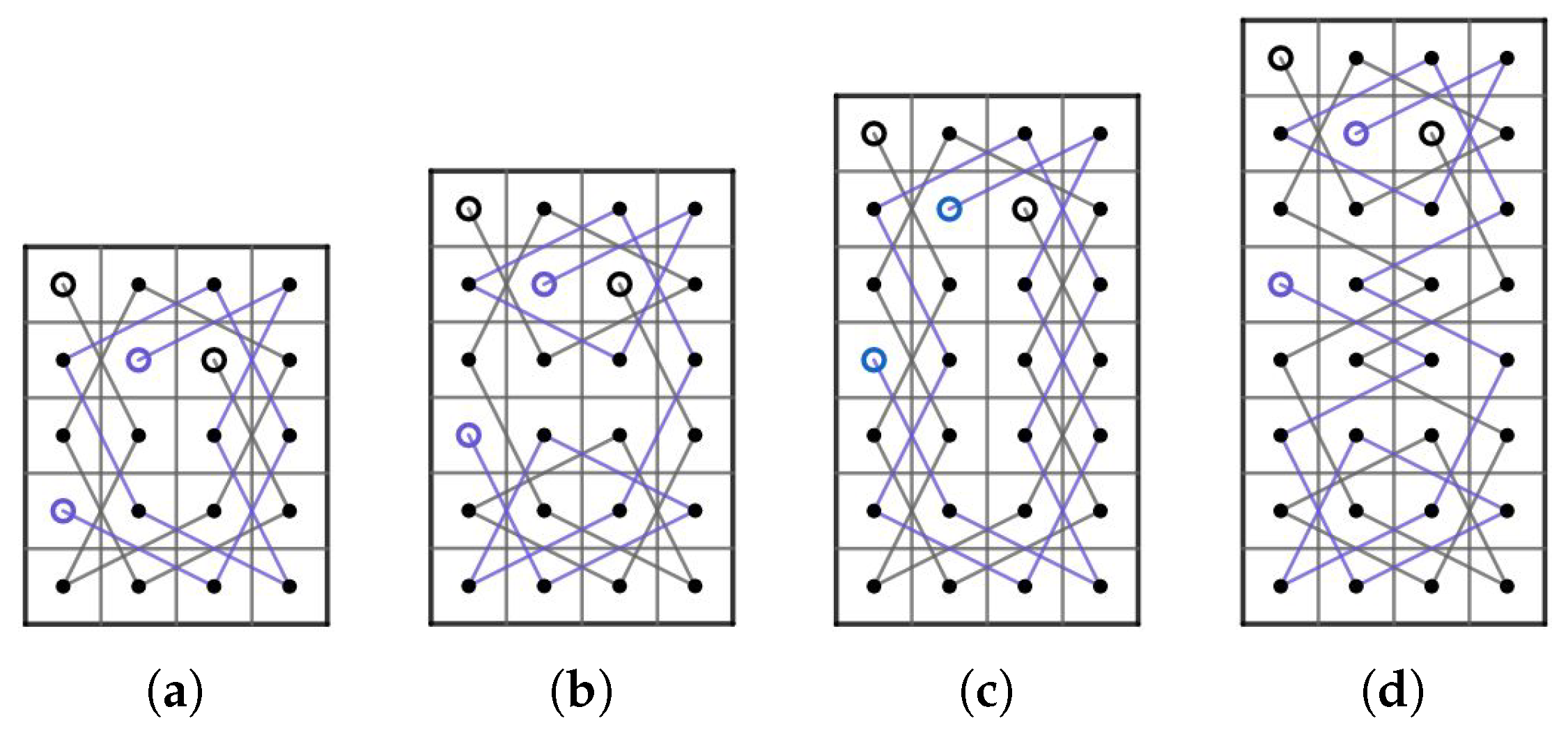
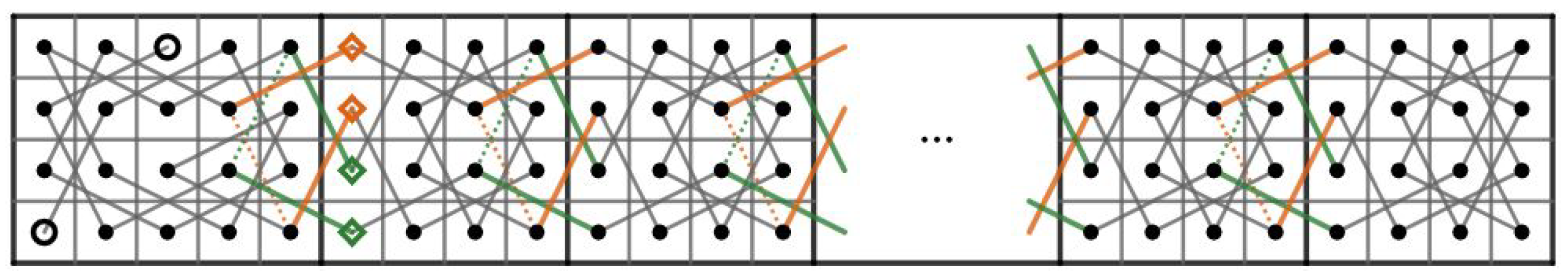
- (i)
- We delete of the OKT on the CB() and connect and of the CB() to and of the 1st CB(), respectively.
- (ii)
- We delete of the second path of the ith CB() for all . Then, we connect and of the ith CB() to and of the th CB().
- (i’)
- (ii’)
- Join of the OKT on CB() to of the 1st CB() shown in Figure 38.
- (iii’)
4. Main Theorem
5. Conclusions and Discussion
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| CKT | Closed Knight’s Tour |
| OKT | Open Knight’s Tour |
| CB | chessboard |
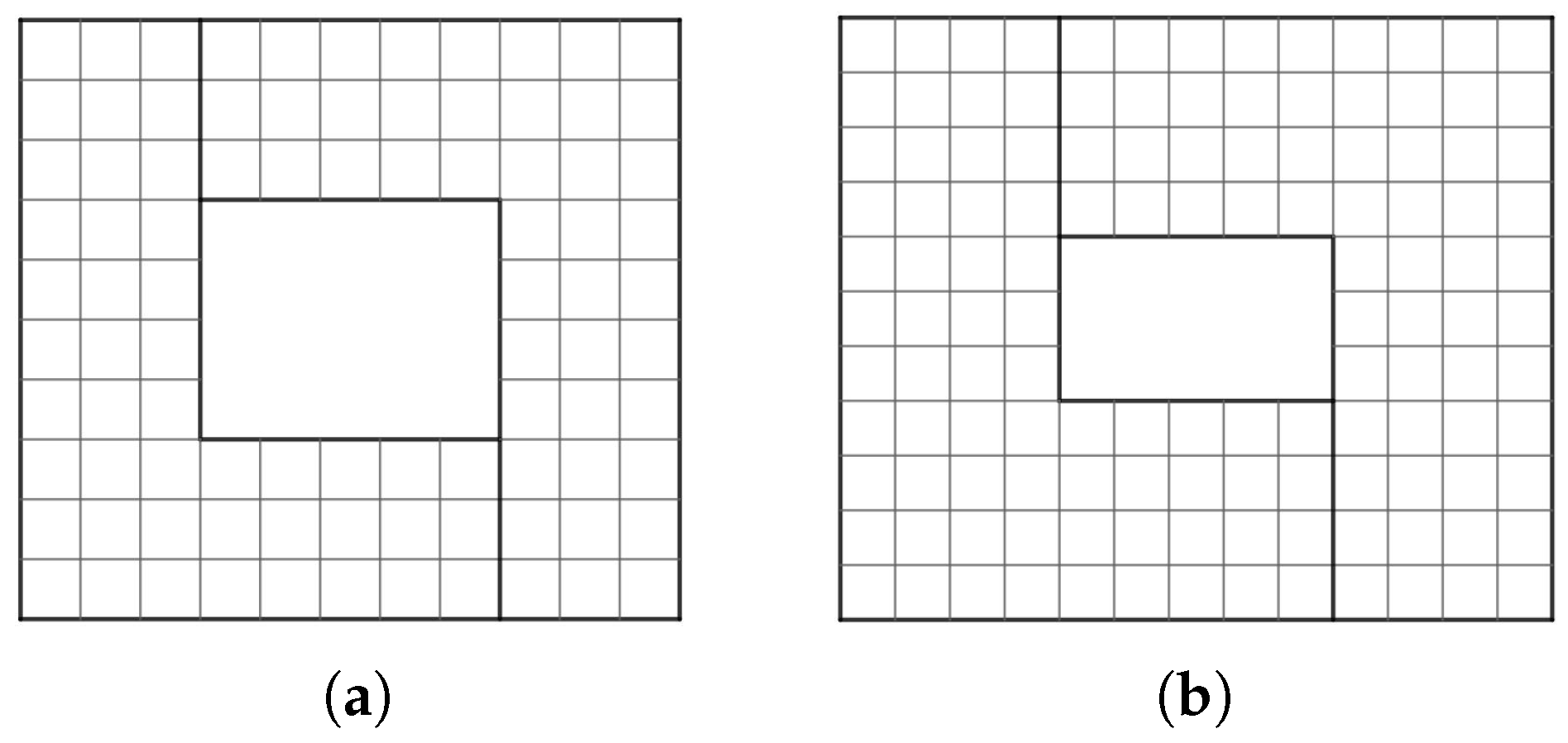
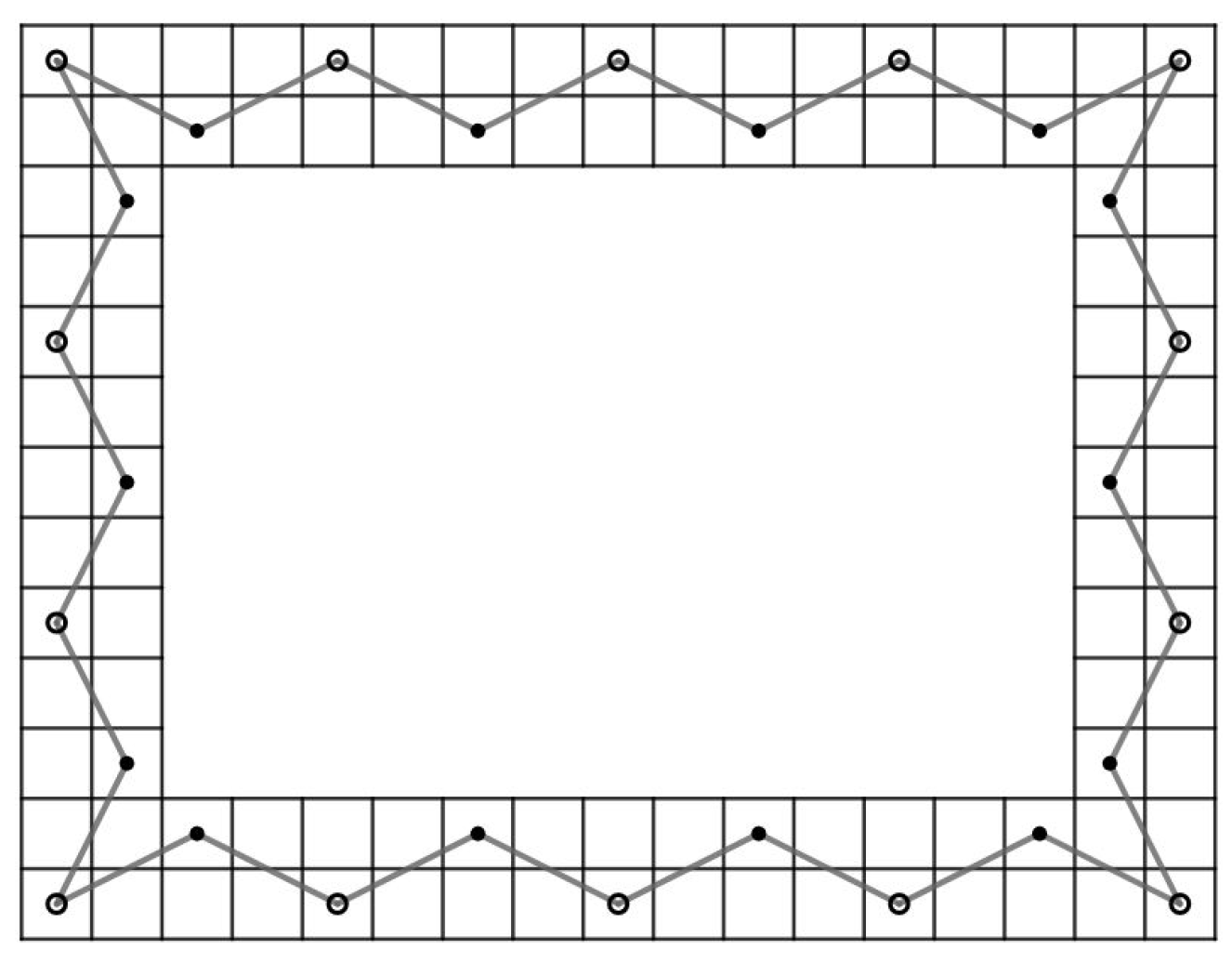
| LB | L-board of size |
| 7B | 7-board of size |
| RB | -ringboard |
References
- Cairns, G. Pillow chess. Math. Mag. 2002, 75, 173–186. [Google Scholar] [CrossRef]
- DeMaio, J. Which chessboards have a closed knight’s tour within the cube? Electron. J. Comb. 2007, 14, R32. [Google Scholar] [CrossRef]
- DeMaio, J.; Bindia, M. Which chessboards have a closed knight’s tour within the rectangular prism? Electron. J. Comb. 2011, 18, 1–8. [Google Scholar] [CrossRef]
- Qing, Y.; Watkins, J.J. Knight’s tours for cubes and boxes. Congr. Numer. 2006, 181, 41–48. [Google Scholar]
- Watkins, J.J. Knight’s tours on a torus. Math. Mag. 1997, 70, 175–184. [Google Scholar] [CrossRef]
- Watkins, J.J. Knight’s tours on cylinders and other surfaces. Congr. Numer. 2000, 143, 117–127. [Google Scholar]
- Singhun, S.; Karudilok, K.; Boonklurb, R. Some Forbidden Rectangular Chessboards with Generalized Knight’s Moves. Thai J. Math. 2020, 133–145. Available online: http://thaijmath.in.cmu.ac.th/index.php/thaijmath/issue/archive (accessed on 2 June 2020).
- Bai, S.; Huang, J. Generalized knight’s tour on 3D chessboards. Discret. Appl. Math. 2010, 158, 1727–1731. [Google Scholar] [CrossRef][Green Version]
- Chia, G.L.; Ong, S.-H. Generalized Knight’s tours on rectangular chessboards. Discret. Appl. Math. 2005, 150, 80–89. [Google Scholar] [CrossRef]
- Schwenk, A.J. Which rectangular chessboards have a knight’s tour? Math. Mag. 1991, 64, 325–332. [Google Scholar] [CrossRef]
- DeMaio, J.; Hippchen, T. Closed Knight’s Tours with Minimal Square Removal for All Rectangular Boards. Math. Mag. 2009, 82, 219–225. [Google Scholar] [CrossRef]
- Bullington, G.; Eroh, L.; Winters, S.J.; Johns, G.L. Knight’s tours on rectangular chessboards using external squares. J. Discret. Math. 2014, 2014, 1–9. [Google Scholar] [CrossRef]
- Miller, A.M.; Farnsworth, D.L. Knight’s Tours on 3 × n Chessboards with a Single Square Removed. Open J. Discret. Math. 2013, 3, 56–59. [Google Scholar] [CrossRef][Green Version]
- Bi, B.; Butler, S.; DeGraaf, S.; Doebel, E. Knight’s tours on boards with odd dimensions. Involve 2015, 8, 615–627. [Google Scholar] [CrossRef]
- Wiitala, H.R. The knight’s tour problem on boards with holes. In Research Experiences for Undergraduates Proceedings; Oregon State University: Corvallis, OR, USA, 1996; pp. 132–151. [Google Scholar]











© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Srichote, W.; Boonklurb, R.; Singhun, S. Closed Knight’s Tours on (m,n,r)-Ringboards. Symmetry 2020, 12, 1217. https://doi.org/10.3390/sym12081217
Srichote W, Boonklurb R, Singhun S. Closed Knight’s Tours on (m,n,r)-Ringboards. Symmetry. 2020; 12(8):1217. https://doi.org/10.3390/sym12081217
Chicago/Turabian StyleSrichote, Wasupol, Ratinan Boonklurb, and Sirirat Singhun. 2020. "Closed Knight’s Tours on (m,n,r)-Ringboards" Symmetry 12, no. 8: 1217. https://doi.org/10.3390/sym12081217
APA StyleSrichote, W., Boonklurb, R., & Singhun, S. (2020). Closed Knight’s Tours on (m,n,r)-Ringboards. Symmetry, 12(8), 1217. https://doi.org/10.3390/sym12081217





