Goal Programming Models with Linear and Exponential Fuzzy Preference Relations
Abstract
1. Introduction
2. Goal Programming Formulation
2.1. Representation of Fuzzy Preference Relations as Membership Functions
2.2. Proposed Approach
- Model 1:
- This model is derived from Arenas-Parra et al. [35] and considers a linear membership function for fuzzy preference relations.
- Model 2:
- This model is an extension of Model 1 with an integration of the proposed formulated exponential membership functions for linguistic preferences among the objectives. This model uses nonlinearities in preferences among the objectives.
- Model 3:
- It is another extension of Model 1, with a new objective function considering a linear membership function for linguistic preferences between the objectives.
- Model 4:
- It is an extension of Model 2, incorporating a new objective function and an exponential membership function for linguistic preferences.
2.3. Comparison with Other Approaches
- Comparison with respect to the objective function
- 2.
- Comparison with respect to Fuzzy preference membership function
- 3.
- Comparison with respect to GP models
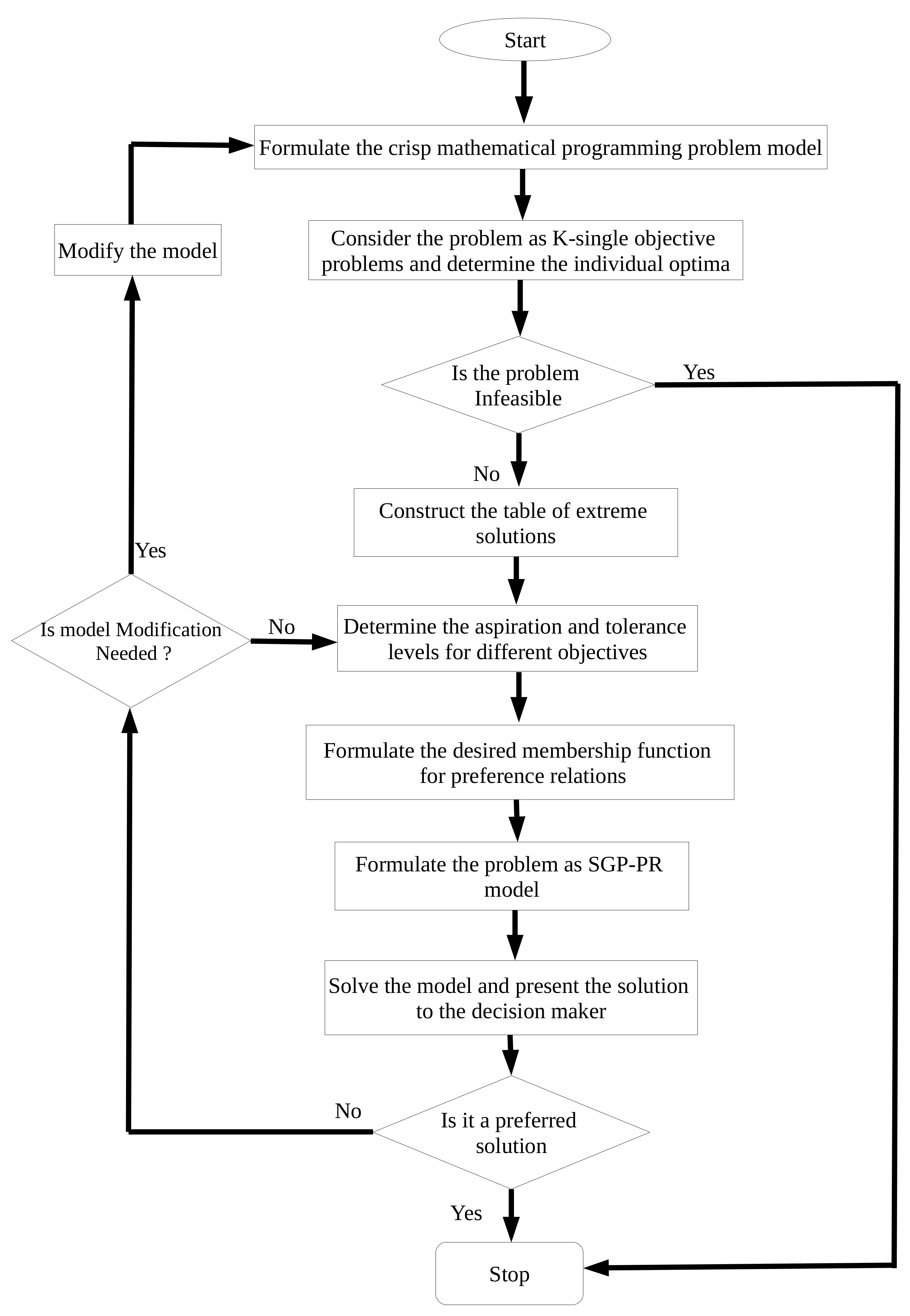
3. Solution Approach
4. Experimental Study
- Goal 1 is significantly more important than Goal 2.
- Goal 2 is significantly more important than Goal 4.
- Goal 2 is significantly more important than Goal 5.
- Goal 3 is fully more important than Goal 2.
4.1. Results and Discussion
- Goal 1 is significantly more important than Goal 2.
- Goal 2 is significantly more important than Goal 4.
- Goal 2 is significantly more important than Goal 5.
- Goal 3 is fully more important than Goal 2.
- Goal 1 is significantly more important than Goal 2.
- Goal 2 is significantly more important than Goal 4.
- Goal 2 is significantly more important than Goal 5.
- Goal 3 is significantly more important than Goal 2.
- Goal 1 is significantly more important than Goal 2.
- Goal 2 is partially equal to Goal 4.
- Goal 2 is significantly more important than Goal 5.
- Goal 3 is fully more important than Goal 2.
- Goal 1 is significantly more important than Goal 2.
- Goal 2 is completely more important than Goal 4.
- Goal 2 is moderately more important than Goal 5.
- Goal 3 is fully more important than Goal 2.
- Goal 1 is significantly more important than Goal 2.
- Goal 2 is significantly more important than Goal 4.
- Goal 2 is significantly more important than Goal 5.
- Goal 3 is partially more important than Goal 2.
4.2. Efficiency Analysis
5. Conclusions, Limitations, and Future Directions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Hwang, C.L.; Masud, A.S.M. Multiple Objective Decision Making—Methods and Applications: A State-of-the-Art Survey; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012; Volume 164. [Google Scholar]
- Lai, Y.J.; Hwang, C.L. Fuzzy multiple objective decision making. In Fuzzy Multiple Objective Decision Making; Springer: Berlin/Heidelberg, Germany, 1994; pp. 139–262. [Google Scholar]
- Sakawa, M.; Nishizaki, I.; Katagiri, H. Fuzzy Stochastic Multiobjective Programming; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2011; Volume 159. [Google Scholar]
- Sakawa, M.; Yano, H.; Nishizaki, I.; Nishizaki, I. Linear and Multiobjective Programming with Fuzzy Stochastic Extensions; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
- Tzeng, G.H.; Huang, J.J. Fuzzy Multiple Objective Decision Making; Chapman and Hall/CRC: London, UK, 2016. [Google Scholar]
- Charnes, A.; Cooper, W.W. Management models and industrial applications of linear programming. Manag. Sci. 1957, 4, 38–91. [Google Scholar] [CrossRef]
- Charnes, A.; Cooper, W.W.; Ferguson, R.O. Optimal estimation of executive compensation by linear programming. Manag. Sci. 1955, 1, 138–151. [Google Scholar] [CrossRef]
- Caballero, R.; Gómez, T.; Ruiz, F. Goal programming: Realistic targets for the near future. J. Multi-Criteria Decis. Anal. 2009, 16, 79–110. [Google Scholar] [CrossRef]
- Chang, C.T. Efficient structures of achievement functions for goal programming models. Asia-Pac. J. Oper. Res. 2007, 24, 755–764. [Google Scholar] [CrossRef]
- Ignizio, J.P. A review of goal programming: A tool for multiobjective analysis. J. Oper. Res. Soc. 1978, 29, 1109–1119. [Google Scholar] [CrossRef]
- Ignizio, J.P. Generalized goal programming An overview. Comput. Oper. Res. 1983, 10, 277–289. [Google Scholar] [CrossRef]
- Romero, C. Handbook of Critical Issues in Goal Programming; Elsevier Science: Amsterdam, The Netherlands, 1991; pp. 1–12. [Google Scholar] [CrossRef]
- Romero, C. A general structure of achievement function for a goal programming model. Eur. J. Oper. Res. 2003, 153, 675–686. [Google Scholar] [CrossRef]
- Zadeh, L. Fuzzy sets. Inf. Control 1965, 8, 338–353. [Google Scholar] [CrossRef]
- Bellman, R.; Zadeh, L. Decision Making in a Fuzzy Environment. Manag. Sci. 1970, 17, 141–164. [Google Scholar] [CrossRef]
- Aköz, O.; Petrovic, D. A fuzzy goal programming method with imprecise goal hierarchy. Eur. J. Oper. Res. 2007, 181, 1427–1433. [Google Scholar] [CrossRef]
- Anukokila, P.; Radhakrishnan, B.; Anju, A. Goal programming approach for solving multi-objective fractional transportation problem with fuzzy parameters. RAIRO-Oper. Res. 2019, 53, 157–178. [Google Scholar] [CrossRef]
- Chen, L.H.; Tsai, F.C. Fuzzy goal programming with different importance and priorities. Eur. J. Oper. Res. 2001, 133, 548–556. [Google Scholar] [CrossRef]
- Cheng, H.W. A satisficing method for fuzzy goal programming problems with different importance and priorities. Qual. Quant. 2013, 47, 485–498. [Google Scholar] [CrossRef]
- Dalman, H.; Bayram, M. Interactive goal programming algorithm with Taylor series and interval type 2 fuzzy numbers. Int. J. Mach. Learn. Cybern. 2019, 10, 1563–1579. [Google Scholar] [CrossRef]
- Díaz-Madroñero, M.; Pérez-Sánchez, M.; Satorre-Aznar, J.R.; Mula, J.; López-Jiménez, P.A. Analysis of a wastewater treatment plant using fuzzy goal programming as a management tool: A case study. J. Clean. Prod. 2018, 180, 20–33. [Google Scholar] [CrossRef]
- Hocine, A.; Kouaissah, N.; Bettahar, S.; Benbouziane, M. Optimizing renewable energy portfolios under uncertainty: A multi-segment fuzzy goal programming approach. Renew. Energy 2018, 129, 540–552. [Google Scholar] [CrossRef]
- Jadidi, O.; Zolfaghari, S.; Cavalieri, S. A new normalized goal programming model for multi-objective problems: A case of supplier selection and order allocation. Int. J. Prod. Econ. 2014, 148, 158–165. [Google Scholar] [CrossRef]
- Jamalnia, A.; Soukhakian, M.A. A hybrid fuzzy goal programming approach with different goal priorities to aggregate production planning. Comput. Ind. Eng. 2009, 56, 1474–1486. [Google Scholar] [CrossRef]
- Jiménez, M.; Bilbao-Terol, A.; Arenas-Parra, M. A model for solving incompatible fuzzy goal programming: An application to portfolio selection. Int. Trans. Oper. Res. 2018, 25, 887–912. [Google Scholar] [CrossRef]
- Pramanik, S.; Roy, T.K. Multiobjective Transportation Model with Fuzzy Parameters: Priority based Fuzzy Goal Programming Approach. J. Transp. Syst. Eng. Inf. Technol. 2008, 8, 40–48. [Google Scholar] [CrossRef]
- Jana, R.K.; Sharma, D.K.; Chakraborty, B. A hybrid probabilistic fuzzy goal programming approach for agricultural decision-making. Int. J. Prod. Econ. 2016, 173, 134–141. [Google Scholar] [CrossRef]
- Petrovic, D.; Aköz, O. A fuzzy goal programming approach to integrated loading and scheduling of a batch processing machine. J. Oper. Res. Soc. 2008, 59, 1211–1219. [Google Scholar] [CrossRef]
- Torabi, S.A.; Moghaddam, M. Multi-site integrated production-distribution planning with trans-shipment: A fuzzy goal programming approach. Int. J. Prod. Res. 2012, 50, 1726–1748. [Google Scholar] [CrossRef]
- Khalili-Damghani, K.; Sadi-Nezhad, S. A decision support system for fuzzy multi-objective multi-period sustainable project selection. Comput. Ind. Eng. 2013, 64, 1045–1060. [Google Scholar] [CrossRef]
- Díaz-Madroñero, M.; Mula, J.; Jiménez, M. Fuzzy goal programming for material requirements planning under uncertainty and integrity conditions. Int. J. Prod. Res. 2014, 52, 6971–6988. [Google Scholar] [CrossRef]
- Sheikhalishahi, M.; Torabi, S. Maintenance supplier selection considering life cycle costs and risks: A fuzzy goal programming approach. Int. J. Prod. Res. 2014, 52, 7084–7099. [Google Scholar] [CrossRef]
- Bilbao-Terol, A.; Jiménez, M.; Arenas-Parra, M. A group decision making model based on goal programming with fuzzy hierarchy: An application to regional forest planning. Ann. Oper. Res. 2016, 245, 137–162. [Google Scholar] [CrossRef]
- Bilbao-Terol, A.; Arenas-Parra, M.; Cañal-Fernández, V.; Jiménez, M. A sequential goal programming model with fuzzy hierarchies to Sustainable and responsible portfolio selection problem. J. Oper. Res. Soc. 2016, 67, 1259–1273. [Google Scholar] [CrossRef]
- Arenas-Parra, M.; Bilbao-Terol, A.; Jiménez, M. Standard goal programming with fuzzy hierarchies: A sequential approach. Soft Comput. 2016, 20, 2341–2352. [Google Scholar] [CrossRef]
- Hasan, M.G.; Qayyum, Z.; Hasan, S.S. Multi-Objective Annualized Hours Manpower Planning Model: A Modified Fuzzy Goal Programming Approach. Ind. Eng. Manag. Syst. 2019, 18, 52–66. [Google Scholar] [CrossRef]
- Hashmi, N.; Jalil, S.A.; Javaid, S. A model for two-stage fixed charge transportation problem with multiple objectives and fuzzy linguistic preferences. Soft Comput. 2019, 1–15. [Google Scholar] [CrossRef]
- Khalili-Damghani, K.; Sadi-Nezhad, S.; Tavana, M. Solving multi-period project selection problems with fuzzy goal programming based on TOPSIS and a fuzzy preference relation. Inf. Sci. 2013, 252, 42–61. [Google Scholar] [CrossRef]
- Khalili-Damghani, K.; Tavana, M.; Amirkhan, M. A fuzzy bi-objective mixed-integer programming method for solving supply chain network design problems under ambiguous and vague conditions. Int. J. Adv. Manuf. Technol. 2014, 73, 1567–1595. [Google Scholar] [CrossRef]
- Anoop, K.D.; Moskowitz, H. Application of fuzzy theories to multiple objective decision making in system design. Eur. J. Oper. Res. 1991, 53, 348–361. [Google Scholar] [CrossRef]
- Peidro, D.; Vasant, P. Transportation planning with modified S-curve membership functions using an interactive fuzzy multi-objective approach. Appl. Soft Comput. 2011, 11, 2656–2663. [Google Scholar] [CrossRef]
- Zangiabadi, M.; Maleki, H. Fuzzy goal programming technique to solve multiobjective transportation problems with Some non-linear membership functions. Iran. J. Fuzzy Syst. 2013, 10, 61–74. [Google Scholar]
- Gupta, P.; Mehlawat, M.; Mittal, G. A fuzzy approach to multicriteria assignment problem using exponential membership functions. Int. J. Mach. Learn. Cybern. 2013, 4. [Google Scholar] [CrossRef]
- Ehsani, E.; Kazemi, N.; Olugu, E.U.; Grosse, E.E.H.; Schwindl, K. Applying fuzzy multi-objective linear programming to a project management decision with nonlinear fuzzy membership functions. Neural Comput. Appl. 2016, 28, 2193–2206. [Google Scholar] [CrossRef]
- Dhodiya, J.M.; Tailor, A.R. Genetic algorithm based hybrid approach to solve fuzzy multi-objective assignment problem using exponential membership function. SpringerPlus 2016, 5. [Google Scholar] [CrossRef]
- Singh, S.; Yadav, S. Intuitionistic fuzzy multi-objective linear programming problem with various membership functions. Ann. Oper. Res. 2017. [Google Scholar] [CrossRef]
- Torabi, S.A.; Hassini, E. An interactive possibilistic programming approach for multiple objective supply chain master planning. Fuzzy Sets Syst. 2008, 159, 193–214. [Google Scholar] [CrossRef]
- Schrage, L.E.; LINDO Systems, I. Optimization Modeling with LINGO; Duxbury Press: Belmont, CA, USA, 1997. [Google Scholar]
- Tan, R.R.; Aviso, K.B.; Promentilla, M.A.B.; Yu, K.D.S.; Santos, J.R. Programming in LINGO. In Input-Output Models for Sustainable Industrial Systems; Springer: Berlin/Heidelberg, Germany, 2019; pp. 29–46. [Google Scholar]
- Server, N. State-of-the-Art Solvers for Numerical Optimization. 2016. Available online: https://neos-server.org/neos/index.html (accessed on 30 May 2020).
- Fourer, R.; Gay, D.M.; Kernighan, B.W. AMPL-A Modeling Language for Mathematical Programming, 2nd ed.; Cengage Learning; Cengage Learning: Stamford, CT, USA, 2003. [Google Scholar]
- Gropp, W.; Moré, J. Optimization environments and the NEOS server. In Approximation Theory and Optimization; Buhman, M.D., Iserles, A., Eds.; Cambridge University Press: Cambridge, UK, 1997; pp. 167–182. [Google Scholar]
- Czyzyk, J.; Mesnier, M.P.; More, J.J. NEOS server. IEEE Comput. Sci. Eng. 1998, 5, 68–75. [Google Scholar] [CrossRef]
- Dolan, E. The NEOS Server 4.0 Administrative Guide; Technical Report, Memorandum ANL/MCS-TM-250; Mathematics and Computer Science Division, Argonne National Laboratory: Argonne, IL, USA, 2001. [Google Scholar]
- Drud, A.S. CONOPT—Alarge-scale GRG code. ORSA J. Comput. 1994, 6, 207–216. [Google Scholar] [CrossRef]
- Pramanik, S.; Roy, T.K. Fuzzy goal programming approach to multilevel programming problems. Eur. J. Oper. Res. 2007, 176, 1151–1166. [Google Scholar] [CrossRef]
- Zheng, Y.; Liu, J.; Wan, Z. Interactive fuzzy decision making method for solving bilevel programming problem. Appl. Math. Model. 2014, 38, 3136–3141. [Google Scholar] [CrossRef]
- Zhao, X.; Zheng, Y.; Wan, Z. Interactive intuitionistic fuzzy methods for multilevel programming problems. Expert Syst. Appl. 2017, 72, 258–268. [Google Scholar] [CrossRef]

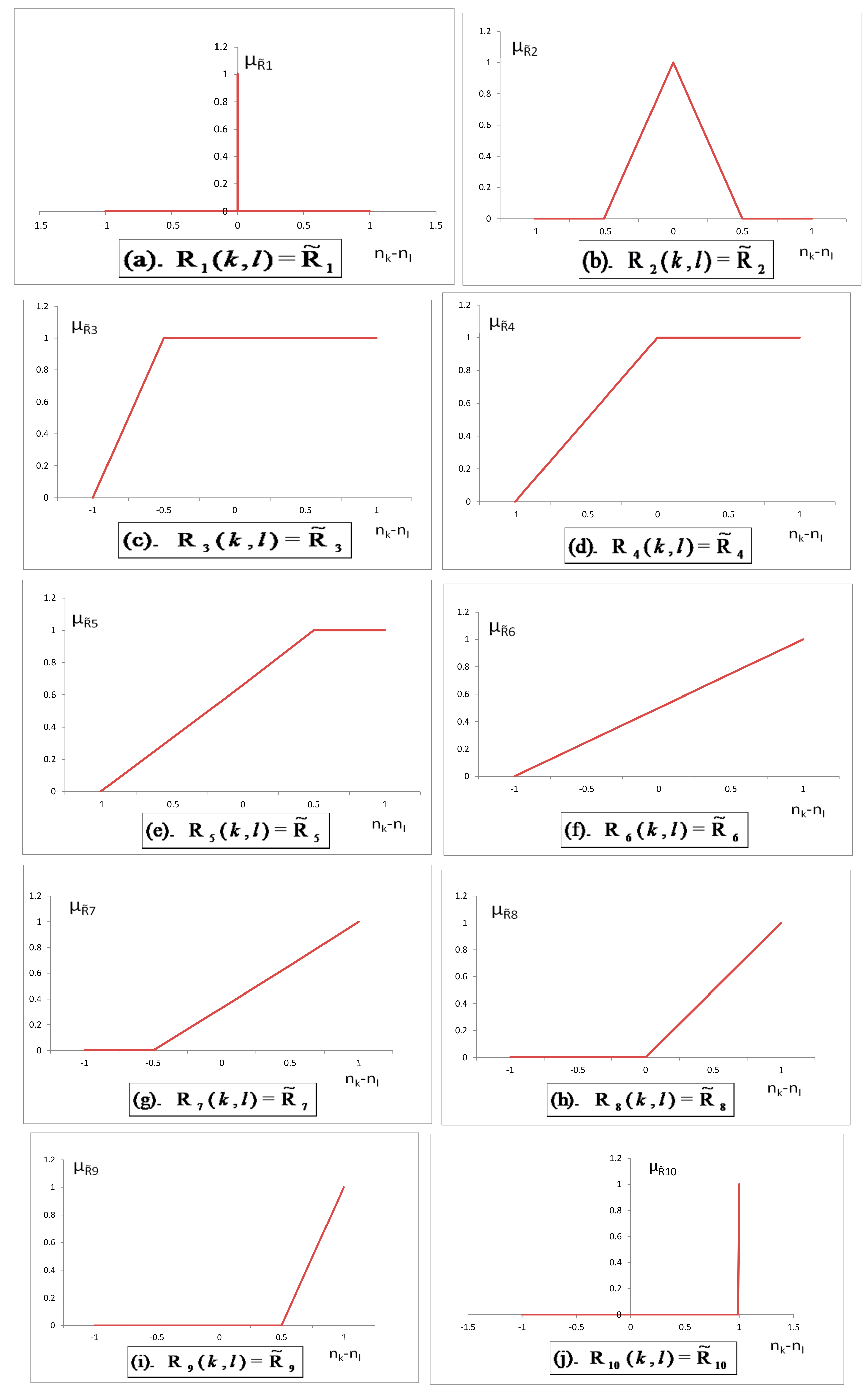
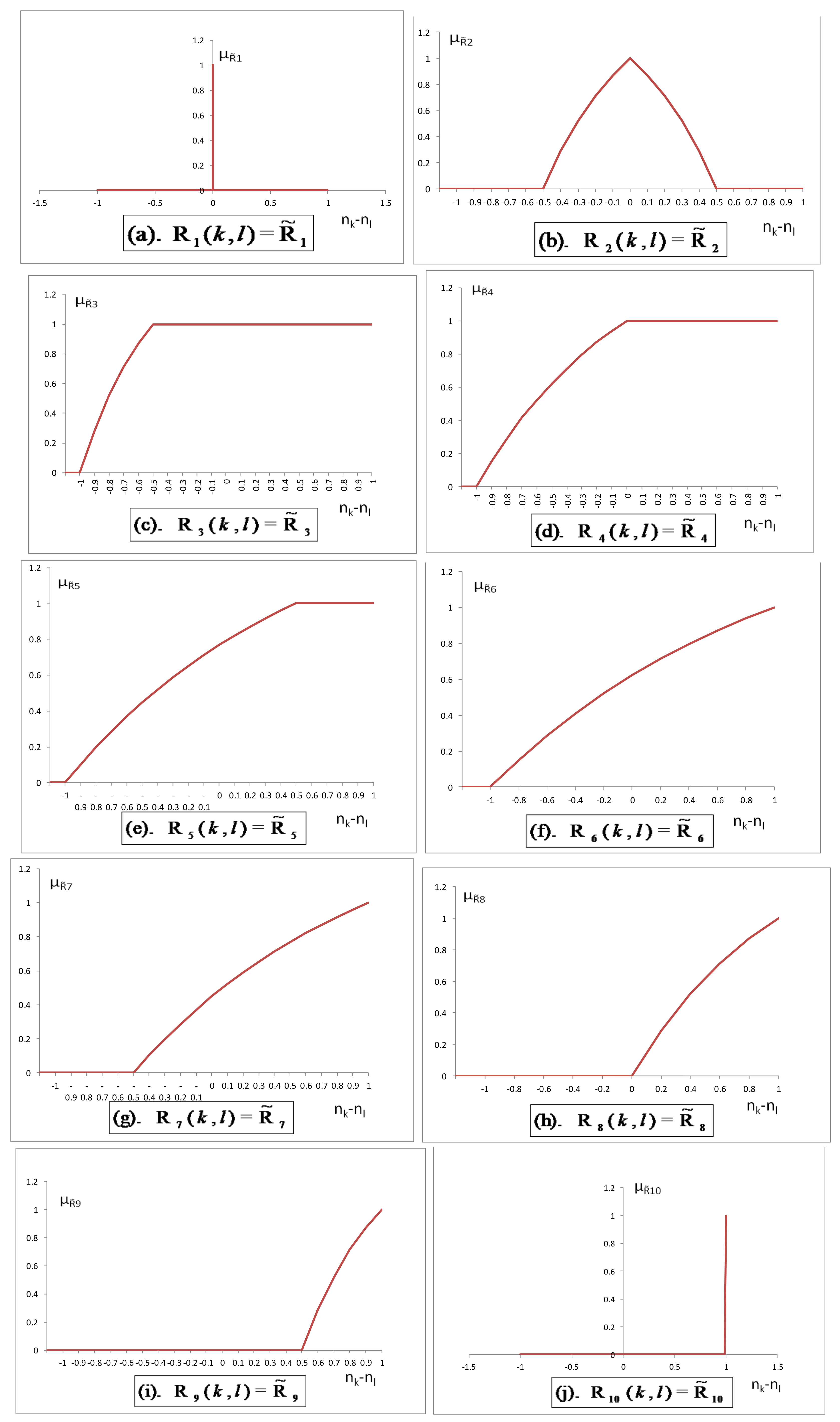

| Linguistic Term | Fuzzy Relation | Membership Values | Transform Function |
|---|---|---|---|
| Exactly equal | |||
| Partially equal | |||
| Partially more important than | |||
| Slightly more important than | |||
| Moderately more important than | |||
| Significantly more important than | |||
| Completely more important than | |||
| Fully more important than | |||
| Extremely more important than | |||
| Incomparable |
| Variables | Model 1 (Linear) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 | |
| Z | 2.7085 | 2.696 | 2.6834 | 2.67086 | 2.8468 | 3.1662 | 3.486 | 3.8059 | 4.1257 | 4.4456 | 4.7655 |
| 12 | 12 | 12 | 12 | 45.9139 | 46.3816 | 46.3816 | 46.3816 | 46.3816 | 46.3816 | 46.3816 | |
| 24 | 24 | 24 | 24 | 100 | 100.526 | 100.526 | 100.526 | 100.526 | 100.526 | 100.526 | |
| 120 | 120 | 120 | 120 | 120 | 120 | 120 | 120 | 120 | 120 | 120 | |
| 24 | 24 | 24 | 24 | 57.0168 | 57.1053 | 57.1053 | 57.1053 | 57.1053 | 57.1053 | 57.1053 | |
| 0 | 0 | 0 | 0 | 39.6639 | 40 | 40 | 40 | 40 | 40 | 40 | |
| 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 0 | 0 | 0 | 0 | 8.2563 | 8.2894 | 8.2894 | 8.2894 | 8.2894 | 8.2894 | 8.2894 | |
| 0 | 0 | 0 | 0 | 1.6596 | 1.7105 | 1.7105 | 1.7105 | 1.7105 | 1.7105 | 1.7105 | |
| 12 | 12 | 12 | 12 | 16.1239 | 16.1184 | 16.1184 | 16.1184 | 16.1184 | 16.1184 | 16.1184 | |
| 1 | 1 | 1 | 1 | 0.9517 | 0.9497 | 0.9497 | 0.9497 | 0.9497 | 0.9497 | 0.9497 | |
| 0.24 | 0.24 | 0.24 | 0.24 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | |
| 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | |
| 0.3428 | 0.3428 | 0.3428 | 0.3428 | 0.8145 | 0.8157 | 0.8157 | 0.8157 | 0.8157 | 0.8157 | 0.8157 | |
| 0 | 0 | 0 | 0 | 0.9915 | 1 | 1 | 1 | 1 | 1 | 1 | |
| 0.88 | 0.88 | 0.88 | 0.88 | 0.4758 | 0.4748 | 0.4748 | 0.4748 | 0.4748 | 0.4748 | 0.4748 | |
| 0.4485 | 0.4485 | 0.4485 | 0.4485 | 0.5927 | 0.5921 | 0.5921 | 0.5921 | 0.592105 | 0.5921 | 0.5921 | |
| 0.76 | 0.76 | 0.76 | 0.76 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 0.62 | 0.62 | 0.62 | 0.62 | 0.5042 | 0.5 | 0.5 | 0.5 | 0.5 | 0.5 | 0.5 | |
| 2.5828 | 2.5828 | 2.5828 | 2.5828 | 4.7579 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | |
| 2.7085 | 2.7085 | 2.7085 | 2.7085 | 1.5728 | 1.5669 | 1.5669 | 1.5669 | 1.5669 | 1.5669 | 0 | |
| Variables | Model 2 (Exponential) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 | |
| Z | 3.0707 | 3.0219 | 2.9731 | 2.9474 | 3.0969 | 3.3464 | 3.6302 | 3.914 | 4.1978 | 4.4816 | 4.7655 |
| 12 | 12 | 12 | 35 | 35 | 46.3816 | 46.3816 | 46.3816 | 46.3816 | 46.3816 | 46.3816 | |
| 24 | 24 | 24 | 45.7463 | 84.8031 | 100.526 | 100.526 | 100.526 | 100.526 | 100.526 | 100.526 | |
| 120 | 120 | 120 | 134.416 | 120 | 120 | 120 | 120 | 120 | 120 | 120 | |
| 24 | 24 | 24 | 27.1662 | 54.1798 | 57.1053 | 57.1053 | 57.1053 | 57.1053 | 57.1053 | 57.1053 | |
| 0 | 0 | 0 | 13.3127 | 31.0515 | 40 | 40 | 40 | 40 | 40 | 40 | |
| 0 | 0 | 0 | 0 | 0.2852 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 0 | 0 | 0 | 0.6944 | 6.9784 | 8.2894 | 8.2894 | 8.2894 | 8.2894 | 8.2894 | 8.2894 | |
| 0 | 0 | 0 | 2.6337 | 0.4991 | 1.7105 | 1.7105 | 1.7105 | 1.7105 | 1.7105 | 1.7105 | |
| 12 | 12 | 12 | 12.5414 | 15.909 | 16.1184 | 16.1184 | 16.1184 | 16.1184 | 16.1184 | 16.1184 | |
| 1 | 1 | 1 | 1 | 1 | 0.9497 | 0.9497 | 0.9497 | 0.9497 | 0.9497 | 0.9497 | |
| 0.24 | 0.24 | 0.24 | 0.4574 | 0.848 | 1 | 1 | 1 | 1 | 1 | 1 | |
| 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | |
| 0.3428 | 0.3428 | 0.3428 | 0.3880 | 0.7739 | 0.8157 | 0.8157 | 0.8157 | 0.8157 | 0.8157 | 0.8157 | |
| 0 | 0 | 0 | 0.3328 | 0.7762 | 1 | 1 | 1 | 1 | 1 | 1 | |
| 0.9258 | 0.9258 | 0.9258 | 0.8504 | 0.6926 | 0.598 | 0.5980 | 0.5980 | 0.5980 | 0.5980 | 0 | |
| 0.5718 | 0.5718 | 0.5718 | 0.6551 | 0.6573 | 0.7068 | 0.7068 | 0.7068 | 0.7068 | 0.7068 | 0 | |
| 0.8421 | 0.8421 | 0.8421 | 0.6624 | 0.223 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 0.7309 | 0.7309 | 0.7309 | 0.6804 | 0.6562 | 0.6224 | 0.6224 | 0.6224 | 0.6224 | 0.6224 | 0 | |
| 2.5828 | 2.5828 | 2.5828 | 3.1783 | 4.3983 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | |
| 3.0707 | 3.0707 | 3.0707 | 2.8484 | 2.2293 | 1.9273 | 1.9273 | 1.9273 | 1.9273 | 1.9273 | 0 | |
| Variables | Model 3 (Linear) | |||||
|---|---|---|---|---|---|---|
| () | c1 | c2 | c3 | c4 | c5 | c6 |
| Z | 2.4251 | 2.4785 | 4.0506 | 2.1444 | 2.9408 | 2.0899 |
| 12 | 35 | 46.3816 | 46.3816 | 46.3816 | 65.1907 | |
| 24 | 87.7187 | 100.526 | 100.526 | 100.526 | 121.692 | |
| 120 | 120 | 120 | 120 | 120 | 120 | |
| 24 | 54.9527 | 57.1053 | 57.1053 | 57.1053 | 60.6625 | |
| 0 | 31.8203 | 40 | 40 | 40 | 53.5177 | |
| 0 | 0 | 0 | 0 | 0 | 0 | |
| 0 | 7.4822 | 8.2894 | 8.2894 | 8.2894 | 9.62345 | |
| 0 | 0.4728 | 1.7105 | 1.7105 | 1.7105 | 3.7559 | |
| 12 | 16.253 | 16.1184 | 16.1184 | 16.1184 | 15.8961 | |
| 0 | 0.7850 | 0.8157 | 0.8157 | 0.8157 | 0.8666 | |
| 1 | 1 | 0.9497 | 0.9497 | 0.9497 | 0.8666 | |
| 0.24 | 0.8771 | 1 | 1 | 1 | 1 | |
| 1 | 1 | 1 | 1 | 1 | 1 | |
| 0.3428 | 0.7850 | 0.8157 | 0.8157 | 0.8157 | 0.8666 | |
| 0 | 0.7955 | 1 | 1 | 1 | 1 | |
| 0.88 | 0.5614 | 0.4748 | 0.4748 | 0.4748 | 0.4333 | |
| 0.4485 | 0.5460 | 0.5921 | 0.5921 | 0.5921 | 0.5666 | |
| 0.76 | 0.1228 | 0 | 0 | 0 | 0 | |
| 0.62 | 0.5408 | 0.5 | 0.5 | 0.5 | 0.5 | |
| 2.5828 | 4.4577 | 4.7655 | 4.7655 | 4.7655 | 4.7332 | |
| 2.7085 | 1.7711 | 1.5669 | 1.5669 | 1.5669 | 1.5 | |
| Variables | Model 4 (Exponential) | |||||
|---|---|---|---|---|---|---|
| () | c1 | c2 | c3 | c4 | c5 | c6 |
| Z | 2.7148 | 2.7444 | 4.0867 | 2.2526 | 3.0129 | 2.1262 |
| 12 | 35 | 46.3816 | 46.3816 | 46.3816 | 65.1907 | |
| 24 | 70.4306 | 100.526 | 100.526 | 100.526 | 121.692 | |
| 120 | 120 | 120 | 120 | 120 | 120 | |
| 24 | 50.3698 | 57.1053 | 57.1053 | 57.1053 | 60.6625 | |
| 0 | 27.2614 | 40 | 40 | 40 | 53.5177 | |
| 0 | 1.6916 | 0 | 0 | 0 | 0 | |
| 0 | 4.4949 | 8.2894 | 8.2894 | 8.2894 | 9.6234 | |
| 0 | 0.6287 | 1.7105 | 1.7105 | 1.7105 | 3.7559 | |
| 12 | 14.2134 | 16.1184 | 16.1184 | 16.1184 | 15.8961 | |
| 0 | 0.6815 | 0.8157 | 0.8157 | 0.8157 | 0.8666 | |
| 1 | 1 | 0.9497 | 0.9497 | 0.9497 | 0.8666 | |
| 0.24 | 0.7043 | 1 | 1 | 1 | 1 | |
| 1 | 1 | 1 | 1 | 1 | 1 | |
| 0.3428 | 0.7195 | 0.8157 | 0.8157 | 0.8157 | 0.8666 | |
| 0 | 0.6815 | 1 | 1 | 1 | 1 | |
| 0.9258 | 0.7543 | 0.5980 | 0.5980 | 0.5980 | 0.5562 | |
| 0.5718 | 0.6151 | 0.7068 | 0.7068 | 0.7068 | 0.6843 | |
| 0.8421 | 0.4049 | 0 | 0 | 5.38E-17 | 0 | |
| 0.7309 | 0.6333 | 0.6224 | 0.6224 | 0.6224 | 0.6224 | |
| 2.5828 | 4.1054 | 4.7655 | 4.7655 | 4.7655 | 4.7332 | |
| 3.0707 | 2.4077 | 1.9273 | 1.9273 | 1.9273 | 1.8631 | |
| PR Type | Model 1 (Linear) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 | ||
| PRT1 | 2.5828 | 2.5828 | 2.5828 | 2.5828 | 4.7579 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | |
| 2.7085 | 2.7085 | 2.7085 | 2.70857 | 1.5728 | 1.5669 | 1.56696 | 1.56696 | 1.56696 | 1.56696 | 0 | ||
| PRT2 | 2.5828 | 4.4577 | 4.4577 | 4.4577 | 4.7579 | 4.7655 | 4.7655 | 4.7655 | 4.786 | 4.786 | 4.786 | |
| 2.8285 | 2.2097 | 2.2097 | 2.2097 | 2.0728 | 2.0669 | 2.0669 | 2.0669 | 2.012 | 2.012 | 0 | ||
| PRT3 | 2.8 | 2.8 | 2.8 | 2.8 | 4.1554 | 4.1554 | 4.6364 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | |
| 3.1 | 3.1 | 3.1 | 3.1 | 2.4222 | 2.4222 | 1.8249 | 1.6064 | 1.6064 | 1.6064 | 0 | ||
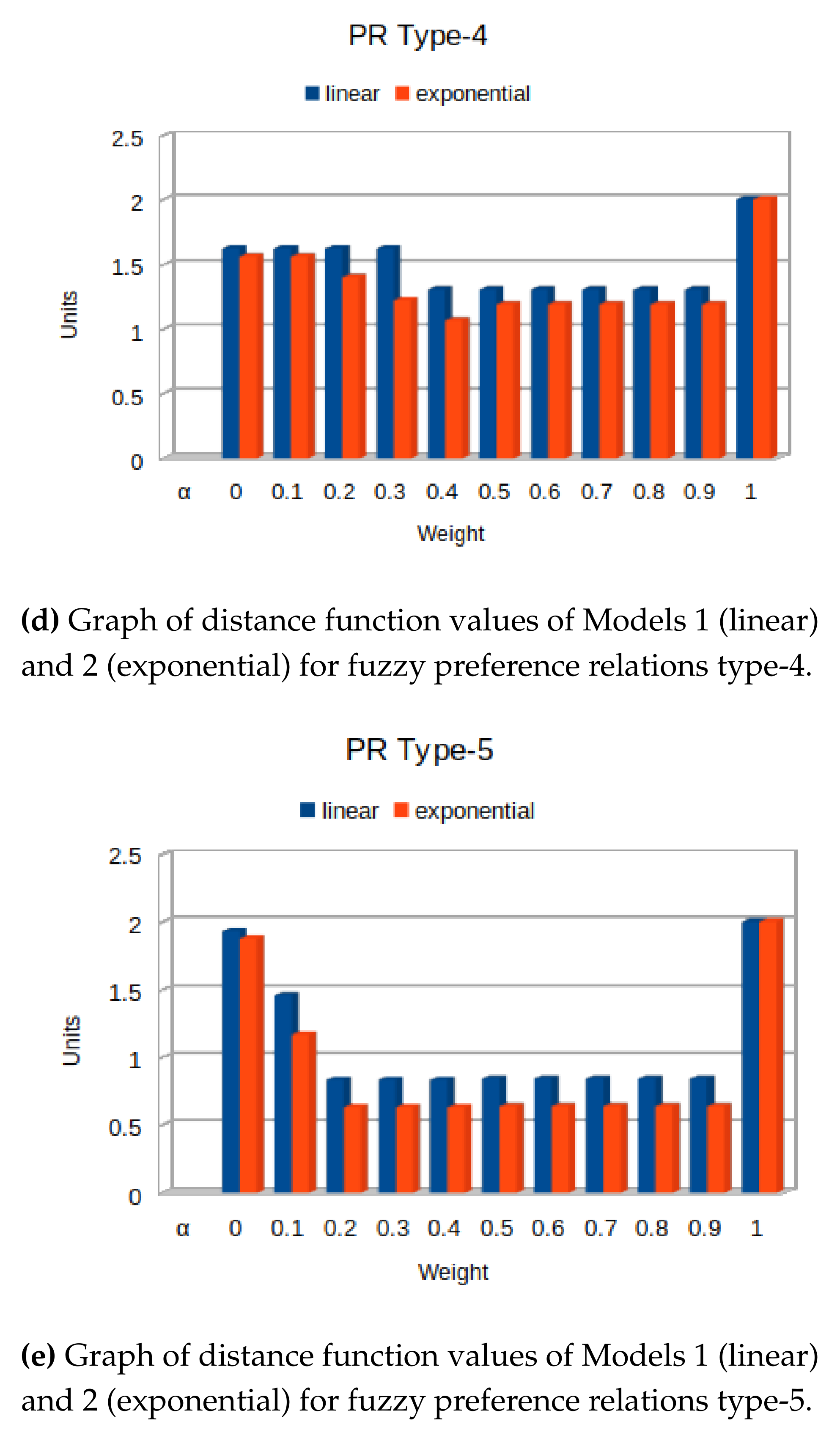
| PRT4 | 2.5828 | 2.5828 | 2.5828 | 2.5828 | 4.7579 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | |
| 2.7314 | 2.7314 | 2.7314 | 2.7314 | 1.6051 | 1.5976 | 1.5976 | 1.5976 | 1.5976 | 1.5976 | 0 | ||
| PRT5 | 1.4949 | 2.85 | 4.7616 | 4.7616 | 4.7616 | 4.786 | 4.786 | 4.786 | 4.786 | 4.786 | 4.786 | |
| 3 | 2.925 | 2.5759 | 2.5759 | 2.5759 | 2.5576 | 2.5576 | 2.5576 | 2.5576 | 2.5576 | 0 | ||
| PR Type | Model 2 (Exponential) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 | ||
| PRT1 | 2.5828 | 2.5828 | 2.5828 | 3.17837 | 4.3983 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | |
| 3.0707 | 3.0707 | 3.0707 | 2.84845 | 2.2293 | 1.9273 | 1.92737 | 1.92737 | 1.92737 | 1.92737 | 0 | ||
| PRT2 | 2.5828 | 2.5828 | 3.2 | 4.5716 | 4.7579 | 4.7655 | 4.7655 | 4.7655 | 4.786 | 4.786 | 4.786 | |
| 3.1543 | 3.1543 | 3.0372 | 2.6378 | 2.5554 | 2.5498 | 2.5498 | 2.5498 | 2.4987 | 2.4987 | 0 | ||
| PRT3 | 2.7526 | 2.8 | 2.8 | 3.1477 | 4.1554 | 4.1554 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | |
| 3.3765 | 3.3757 | 3.3757 | 3.2372 | 2.757 | 2.757 | 1.9612 | 1.9612 | 1.9612 | 1.9612 | 0 | ||
| PRT4 | 2.5828 | 2.5828 | 2.8261 | 3.1876 | 4.4577 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | |
| 3.0257 | 3.0257 | 2.9734 | 2.8471 | 2.1912 | 1.9472 | 1.9472 | 1.9472 | 1.9472 | 1.9472 | 0 | ||
| PRT5 | 1.4949 | 3.2 | 4.7616 | 4.7616 | 4.7616 | 4.786 | 4.786 | 4.786 | 4.786 | 4.786 | 4.786 | |
| 3.2449 | 3.1955 | 2.9382 | 2.9382 | 2.9382 | 2.921 | 2.921 | 2.921 | 2.921 | 2.921 | 0 | ||
| Variables | Model 3 (Linear) | ||||||
|---|---|---|---|---|---|---|---|
| PR Type | () | c1 | c2 | c3 | c4 | c5 | c6 |
| PRT1 | 2.5828 | 4.4577 | 4.7655 | 4.7655 | 4.7655 | 4.7332 | |
| 2.7085 | 1.77113 | 1.5669 | 1.5669 | 1.5669 | 1.5 | ||
| PRT2 | 2.5828 | 4.7579 | 4.786 | 4.786 | 4.7851 | 4.7556 | |
| 2.8285 | 2.0728 | 2.012 | 2.012 | 1.9965 | 1.9592 | ||
| PRT3 | 2.8 | 4.1554 | 4.7655 | 4.4987 | 4.7332 | 4.7332 | |
| 3.1 | 2.4222 | 1.6064 | 2.0085 | 1.6665 | 1.6665 | ||
| PRT4 | 2.5828 | 4.4577 | 4.7655 | 4.7655 | 4.7655 | 4.7655 | |
| 2.7314 | 1.8001 | 1.5976 | 1.5976 | 1.5976 | 1.5976 | ||
| PRT5 | 3.2 | 4.7616 | 4.786 | 4.7851 | 4.7851 | 4.7851 | |
| 2.8812 | 2.5759 | 2.5576 | 2.5485 | 2.5485 | 2.5485 | ||
| Variables | Model 4 (Exponential) | ||||||
|---|---|---|---|---|---|---|---|
| PR Type | () | c1 | c2 | c3 | c4 | c5 | c6 |
| PRT1 | 2.5828 | 4.1054 | 4.7655 | 4.7655 | 4.7655 | 4.7332 | |
| 3.0707 | 2.4077 | 1.9273 | 1.9273 | 1.9273 | 1.8631 | ||
| PRT2 | 2.99 | 4.7579 | 4.786 | 4.786 | 4.7851 | 4.7556 | |
| 3.0787 | 2.5554 | 2.4987 | 2.4987 | 2.4839 | 2.4483 | ||
| PRT3 | 4.7154 | 4.7174 | 4.7655 | 4.7332 | 4.7332 | 4.7332 | |
| 2.0184 | 2.0165 | 1.9612 | 2.0008 | 2.0008 | 2.0008 | ||
| PRT4 | 2.7121 | 4.097 | 4.7655 | 4.7655 | 4.7655 | 4.7332 | |
| 3.0001 | 2.4024 | 1.9472 | 1.9472 | 1.9472 | 1.8709 | ||
| PRT5 | 4.4126 | 4.7616 | 4.786 | 4.7851 | 4.7851 | 4.7835 | |
| 3.0136 | 2.9382 | 2.921 | 2.9126 | 2.9126 | 2.91 | ||
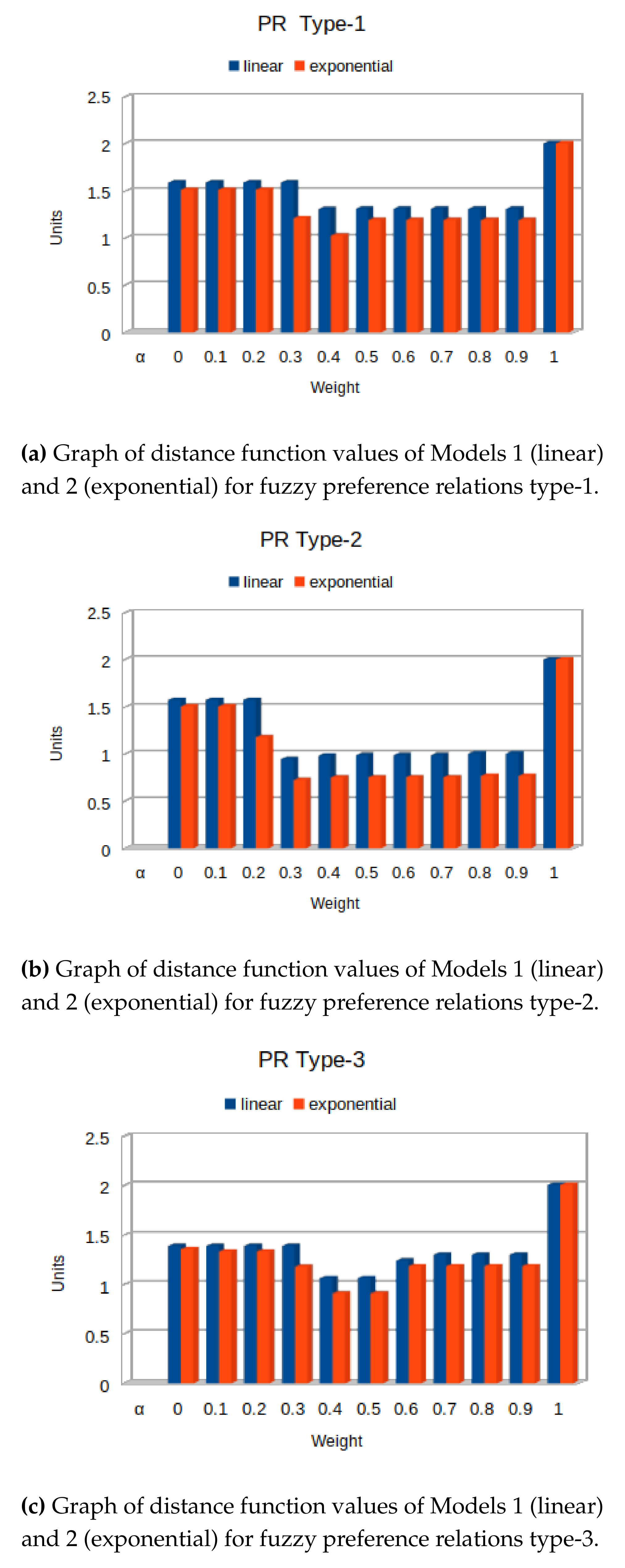
| PR Type | Distance Measure for Model 1 and 2 | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 | ||
| PRT1 | Model 1 | 1.5905 | 1.5905 | 1.5905 | 1.5905 | 1.3126 | 1.3147 | 1.3147 | 1.3147 | 1.3147 | 1.3147 | 2.009 |
| Model 2 | 1.5151 | 1.5151 | 1.5151 | 1.2129 | 1.0285 | 1.1943 | 1.1943 | 1.1943 | 1.1943 | 1.1943 | 2.009 | |
| PRT2 | Model 1 | 1.5769 | 1.5769 | 1.5769 | 0.9511 | 0.9864 | 0.9892 | 0.9892 | 0.9892 | 1.0079 | 1.0079 | 2.0056 |
| Model 2 | 1.5087 | 1.5087 | 1.1816 | 0.7274 | 0.7518 | 0.7543 | 0.7543 | 0.7543 | 0.7687 | 0.7687 | 2.0056 | |
| PRT3 | Model 1 | 1.3909 | 1.3909 | 1.3909 | 1.3909 | 1.0663 | 1.0663 | 1.2424 | 1.303 | 1.303 | 1.303 | 2.009 |
| Model 2 | 1.3591 | 1.3345 | 1.3345 | 1.1789 | 0.9084 | 0.9084 | 1.1865 | 1.1865 | 1.1865 | 1.1865 | 2.009 | |
| PRT4 | Model 1 | 1.6285 | 1.6285 | 1.6285 | 1.6285 | 1.3091 | 1.3111 | 1.3111 | 1.3111 | 1.3111 | 1.3111 | 2.009 |
| Model 2 | 1.5657 | 1.5657 | 1.4032 | 1.2258 | 1.069 | 1.1949 | 1.1949 | 1.1949 | 1.1949 | 1.1949 | 2.009 | |
| PRT5 | Model 1 | 1.9378 | 1.4626 | 0.838 | 0.838 | 0.838 | 0.8475 | 0.8475 | 0.8475 | 0.8475 | 0.8475 | 2.0056 |
| Model 2 | 1.8815 | 1.171 | 0.6339 | 0.6339 | 0.6339 | 0.6423 | 0.6423 | 0.6423 | 0.6423 | 0.6423 | 2.0056 | |
| PR Type | Distance Measure for Model 3 and 4 | ||||||
|---|---|---|---|---|---|---|---|
| () | c1 | c2 | c3 | c4 | c5 | c6 | |
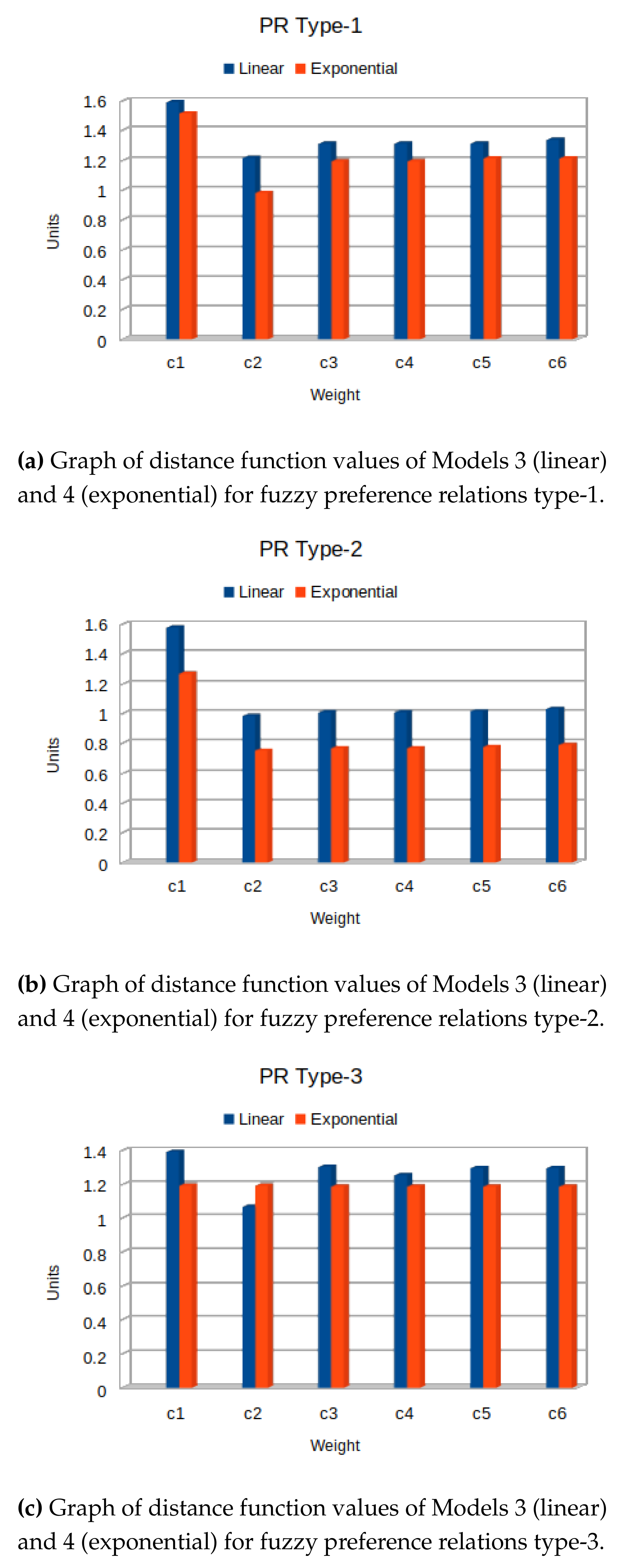
| PRT1 | Model 3 | 1.5905 | 1.2172 | 1.3147 | 1.3147 | 1.3147 | 1.3395 |
| Model 4 | 1.5151 | 0.982 | 1.1943 | 1.1943 | 1.2143 | 1.2143 | |
| PRT2 | Model 3 | 1.5769 | 0.9864 | 1.0079 | 1.0079 | 1.0151 | 1.0322 |
| Model 4 | 1.2682 | 0.7518 | 0.7687 | 0.7687 | 0.7754 | 0.7912 | |
| PRT3 | Model 3 | 1.3909 | 1.0663 | 1.303 | 1.2545 | 1.2953 | 1.2953 |
| Model 4 | 1.1928 | 1.1918 | 1.1865 | 1.186 | 1.186 | 1.186 | |
| PRT4 | Model 3 | 1.6285 | 1.2284 | 1.3111 | 1.3111 | 1.3111 | 1.3422 |
| Model 4 | 1.4763 | 1.0258 | 1.1949 | 1.1949 | 1.1949 | 1.2217 | |
| PRT5 | Model 3 | 1.258 | 0.838 | 0.8475 | 0.8519 | 0.8519 | 0.8519 |
| Model 4 | 0.6669 | 0.6339 | 0.6423 | 0.6461 | 0.6461 | 0.6472 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, M.F.; Hasan, M.G.; Quddoos, A.; Fügenschuh, A.; Hasan, S.S. Goal Programming Models with Linear and Exponential Fuzzy Preference Relations. Symmetry 2020, 12, 934. https://doi.org/10.3390/sym12060934
Khan MF, Hasan MG, Quddoos A, Fügenschuh A, Hasan SS. Goal Programming Models with Linear and Exponential Fuzzy Preference Relations. Symmetry. 2020; 12(6):934. https://doi.org/10.3390/sym12060934
Chicago/Turabian StyleKhan, Mohammad Faisal, Md. Gulzarul Hasan, Abdul Quddoos, Armin Fügenschuh, and Syed Suhaib Hasan. 2020. "Goal Programming Models with Linear and Exponential Fuzzy Preference Relations" Symmetry 12, no. 6: 934. https://doi.org/10.3390/sym12060934
APA StyleKhan, M. F., Hasan, M. G., Quddoos, A., Fügenschuh, A., & Hasan, S. S. (2020). Goal Programming Models with Linear and Exponential Fuzzy Preference Relations. Symmetry, 12(6), 934. https://doi.org/10.3390/sym12060934






