Dynamical Approach to Decays of XYZ States
Abstract
1. Introduction
2. Confined Covariant Quark Model
2.1. Interaction Lagrangian
2.2. Compositeness Condition
2.3. Infrared Confinement
2.4. Electromagnetic Interactions
2.5. Selected Computational Aspects
3. Strong Decays of
3.1. Decays , , and
3.2. Implications of in the Charm Dissociation Process by Light Mesons
4. Radiative Decays of
5. Nature of
6. The Nature of
7. Bottomonium-Like States and
- For transitions, the matrix element can be parameterized with two Lorentz structures:The invariant amplitudes A and B can be combined into the helicity amplitudes:which are practical to express the decay width. For the derivation of the latter, it is useful to work in the rest frame of the initial particle, where is the three-momentum and is the energy of the final state vector. Furthermore, the on-mass-shell character of the initial and final state particles is taken into account by , , , and . One arrives at:
- The matrix element for the transitions is expressed through one covariant term only:The decay with the formula can be written as:where one can note the p-wave suppression factor .
- As shown in [81], the matrix element for decay can be parameterized using three amplitudes:The relation between the helicity amplitudes () and the invariant amplitudes can be shown to be:The rate of the decay , finally, reads:
- matrix elements parametrized as in Equation (85):
- matrix elements parametrized as in Equation (89):
8. Summary and Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gell–Mann, M. A Schematic Model of Baryons and Mesons. Phys. Lett. 1964, 8, 214–215. [Google Scholar] [CrossRef]
- Bai, J.Z.; Ban, Y.; Bian, J.G.; Cai, X.; Chang, J.F.; Chen, H.F.; Chen, H.S.; Chen, J.; Chen, J.C. Observation of a near threshold enhancement in the mass spectrum from radiative J/ψ→γ decays. Phys. Rev. Lett. 2003, 91, 022001. [Google Scholar]
- Aubert, B.; Barate, R.; Boutigny, D.; Gaillard, J.-M.; Hicheur, A.; Karyotakis, Y.; Lees, J.P.; Robbe, P.; Tisserand, V.; Zghiche, A.; et al. Observation of a narrow meson decaying to at a mass of 2.32-GeV/c2. Phys. Rev. Lett. 2003, 90, 242001. [Google Scholar] [CrossRef] [PubMed]
- Choi, S.K.; Olsen, S.L.; Abe, K.; Abe, T.; Adachi, I.; Ahn, B.S.; Aihara, H.; Akai, K.; Akatsu, M.; Akemoto, M.; et al. Observation of a narrow charmonium-like state in exclusive B±→K±π+π−J/ψ decays. Phys. Rev. Lett. 2003, 91, 262001. [Google Scholar] [CrossRef]
- Acosta, D.; Affolder, T.; Ahn, M.H.; Akimoto, T.; Albrow, M.G.; Ambrose, D.; Amerio, S.; Amidei, D.; Anastassov, A.; Anikeev, K.; et al. Observation of the narrow state X(3872)→J/ψπ+π− in collisions at s = 1.96 TeV. Phys. Rev. Lett. 2004, 93, 072001. [Google Scholar] [CrossRef]
- Abazov, V.M.; Abbott, B.; Abolins, M.; Acharya, B.S.; . Adams, D.L.; Adams, M.; Adams, T.; Agelou, M.; Agram, J.L.; Ahmed, S.N.; et al. Observation and properties of the X(3872) decaying to J/ψπ+π− in collisions at TeV. Phys. Rev. Lett. 2004, 93, 162002. [Google Scholar] [CrossRef]
- Aaij, R.; Abellan Beteta, C.; Adeva, B.; Adinolfi, M.; Adrover, C.; Affolder, A.; Ajaltouni, Z.; Albrecht, J.; Alessio, F. Observation of X(3872) production in pp collisions at TeV. Eur. Phys. J. C 2012, 72, 1972. [Google Scholar] [CrossRef]
- Ablikim, M.; Achasov, M.N.; Ai, X.C.; Albayrak, O.; Ambrose, D.J.; An, F.F.; An, Q.; Bai, J.Z.; Baldini Ferroli, R.; Ban, Y.; et al. Observation of e+e− → γX(3872) at BESIII. Phys. Rev. Lett. 2014, 112, 092001. [Google Scholar] [CrossRef]
- Abulencia, A.; Adelman, J.; Affolder, T.; Akimoto, T.; Albrow, M.G.; Ambrose, D.; Amerio, S.; Amidei, D.; Anastassov, A.; Anikeev, K.; et al. Analysis of the quantum numbers JPC of the X(3872). Phys. Rev. Lett. 2007, 98, 132002. [Google Scholar] [CrossRef]
- Aaltonen, T.; Adelman, J.; Akimoto, T.; Alvarez Gonzalezt, B.; Amerioz, S.; Amidei, D.; Anastassov, A.; Annovi, A.; Antos, J.; Apollinari, G.; et al. Precision Measurement of the X(3872) Mass in J/ψπ+π− Decays. Phys. Rev. Lett. 2009, 103, 152001. [Google Scholar] [CrossRef]
- Choi, S.K.; Olsen, L.; Trabelsi, K.; Adachi, I.; Aihara, H.; Arinstein, K.; Asner, D.M.; Aushev, T.; Bakich, A.M.; Barberio, E.; et al. Bounds on the width, mass difference and other properties of X(3872)→π+π−J/ψ decays. Phys. Rev. D 2011, 84, 052004. [Google Scholar] [CrossRef]
- Aaij, R.; Abellan Beteta, C.; Adeva, B.; Adinolfi, M.; Adrover, C.; Affolder, A.; Ajaltouni, Z.; Albrecht, J.; Alessio, F.; Alexander, M.; et al. Determination of the X(3872) meson quantum numbers. Phys. Rev. Lett. 2013, 110, 222001. [Google Scholar] [CrossRef] [PubMed]
- Tanabashi, M.; Hagiwara, K.; Hikasa, K.; Nakamura, K.; Sumino, Y.; Takahashi, F.; Tanaka, J.; Agashe, K.; Aielli, G.; Allanach, B.C.; et al. Review of Particle Physics. Phys. Rev. D 2018, 98, 030001. [Google Scholar] [CrossRef]
- Aubert, B.; Barate, R.; Boutigny, D.; Couderc, F.; Karyotakis, Y.; Lees, J.P.; Poireau, V.; Tisserand, V.; Zghiche, A.; Grauges, E.; et al. Observation of a broad structure in the π+π−J/ψ mass spectrum around 4.26-GeV/c2. Phys. Rev. Lett. 2005, 95, 142001. [Google Scholar] [CrossRef] [PubMed]
- He, Q.; Insler, J.; Muramatsu, H.; Park, C.S.; Thorndike, E.H.; Yang, F.; Coan, T.E.; Gao, Y.S.; Artuso, M.; Blusk, S.; et al. Confirmation of the Y(4260) resonance production in ISR. Phys. Rev. D 2006, 74, 091104. [Google Scholar] [CrossRef]
- Yuan, C.Z.; Shen, C.P.; Wang, P.; McOnie, S.; Adachi, I.; Aihara, H.; Aulchenko, V.; Aushev, T.; Bahinipati, S.; Balagura, V.; et al. Measurement of e+e−→π+π−J/ψ cross-section via initial state radiation at Belle. Phys. Rev. Lett. 2007, 99, 182004. [Google Scholar] [CrossRef]
- Ablikim, M.; Achasov, M.N.; Ai, X.C.; Albayrak, O.; Ambrose, D.J.; An, F.F.; An, Q.; Bai, J.Z.; Baldini Ferroli, R.; Ban, Y.; et al. Observation of a Charged Charmoniumlike Structure in e+e−→π+π−J/ψ at = 4.26 GeV. Phys. Rev. Lett. 2013, 110, 252001. [Google Scholar] [CrossRef]
- Lees, J.P.; Poireau, V.; Tisserand, V.; Garra Tico, J.; Grauges, E.; Palanoab, A.; Eigen, G.; Stugu, B.; Brown, D.N.; Kerth, L.T.; et al. tudy of the reaction e+e−→J/ψπ+π− via initial-state radiation at BaBar. Phys. Rev. D 2012, 86, 051102. [Google Scholar]
- Ablikim, M.; Achasov, M.N.; Ahmed, S.; Ai, X.C.; Albayrak, O.; Albrecht, M.; Ambrose, D.J.; Amoroso, A.; An, F.F.; An, Q.; et al. Precise measurement of the e+e−→π+π−J/ψ cross-section at center-of-mass energies from 3.77 to 4.60 GeV. Phys. Rev. Lett. 2017, 118, 092001. [Google Scholar]
- Cronin-Hennessy, D.; Gao, K.Y.; Hietala, J.; Kubota, Y.; Klein, T.; Lang, B.W.; Poling, R.; Scott, A.W.; Zweber, P.; Dobbs, S.; et al. Measurement of Charm Production Cross Sections in e+e− Annihilation at Energies between 3.97 and 4.26-GeV. Phys. Rev. D 2009, 80, 072001. [Google Scholar] [CrossRef]
- Pakhlova, G.; Abe, K.; Adachi, I.; Aihara, H.; Anipko, D.; Aulchenko, V.; Aushev, T.; Bakich, A.M.; Balagura, V.; Barberio, E.; et al. Measurement of the near-threshold e+e−→D(*)±D*∓ cross-section using initial-state radiation. Phys. Rev. Lett. 2007, 98, 092001. [Google Scholar] [CrossRef] [PubMed]
- Aubert, B.; Barate, R.; Bona, M.; Boutigny, D.; Couderc, F.; Karyotakis, Y.; Lees, J.P.; Poireau, V.; Tisserand, V.; Zghiche, A.; et al. Study of the Exclusive Initial-State Radiation Production of the DD¯System. Phys. Rev. D 2007, 76, 111105. [Google Scholar] [CrossRef]
- Aubert, B.; Karyotakis, Y.; Lees, J.P.; Poireau, V.; Prencipe, E.; Prudent, X.; Tisserand, V.; Garra Tico, J.; Grauges, E.; Lopez, L.; et al. Exclusive Initial-State-Radiation Production of the , , and Systems. Phys. Rev. D 2009, 79, 092001. [Google Scholar] [CrossRef]
- del Amo Sanchez, P.; Lees, J.P.; Poireau, V.; Prencipe, E.; Tisserand, V.; Garra Tico, J.; Grauges, E.; Martinelli, M.; Palano, A.; Pappagallo, M.; et al. Exclusive Prod. , , via e+E− Annihil. Initial-State Phys. Rev. D 2010, 82, 052004. [Google Scholar]
- Liu, Z.Q.; Shen, C.P.; Yuan, C.Z.; Adachi, I.; Aihara, H.; Asner, D.M.; Aulchenko, V.; Aushev, T.; Aziz, T.; Bakich, A.M.; et al. Study of e+e−→π+π−J/ψ and Observation of a Charged Charmoniumlike State at Belle. Phys. Rev. Lett. 2013, 110. [Google Scholar] [CrossRef]
- Xiao, T.; Dobbs, S.; Tomaradze, A.; Seth, K.K. Observation of the Charged Hadron and Evidence for the Neutral in e+e−→ππJ/ψ at = 4170 MeV. Phys. Lett. B 2013, 727, 366. [Google Scholar] [CrossRef]
- Ablikim, M.; Achasov, M.N.; Albayrak, O.; Ambrose, D.J.; An, F.F.; An, Q.; Bai, J.Z.; Baldini Ferroli, R.; Ban, Y.; Becker, J.; et al. Observation of a charged mass peak in at = 4.26 GeV. Phys. Rev. Lett. 2014, 112, 022001. [Google Scholar] [CrossRef]
- Ablikim, M.; Achasov, M.N.; Ai, X.C.; Albayrak, O.; Albrecht, M.; Ambrose, D.J.; Amoroso, A.; An, F.F.; An, Q.; Bai, J.Z.; et al. Observation of Zc(3900)0 in e+e−→π0π0J/ψ. Phys. Rev. Lett. 2015, 115, 112003. [Google Scholar] [CrossRef]
- Ablikim, M.; Achasov, M.N.; Ai, X.C.; Albayrak, O.; Albrecht, M.; Ambrose, D.J.; Amoroso, A.; An, F.; An, Q.; Bai, J.Z.; et al. Determination of the Spin and Parity of the Zc(3900). Phys. Rev. Lett. 2017, 119, 072001. [Google Scholar] [CrossRef]
- Ablikim, M.; Achasov, M.N.; Ahmed, S.; Albrecht, M.; Alekseev, M.; Amoroso, A.; An, F.F.; An, Q.; Bai, Y.; Bakina, O.; et al. Study of e+e−→π+π−π0ηc and evidence for Zc(3900)± decaying into ρ±ηc. Phys. Rev. D 2019, 100, 111102. [Google Scholar] [CrossRef]
- Abazov, V.M.; Abbott, B.; Acharya, B.S.; Adams, M.; Adams, T.; Agnew, J.P.; Alexeev, G.D.; Alkhazov, G.; Altona, A.; Askew, A.; et al. Evidence for in semi-inclusive decays of b-flavored hadrons. Phys. Rev. D 2018, 98, 052010. [Google Scholar]
- Abazov, V.M.; Abbott, B.; Acharya, B.S.; Adams, M.; Adams, T.; Agnew, J.P.; Alexeev, G.D.; Alkhazov, G.; Altona, A.; Askew, A.; et al. Properties of Produced in Collision. Phys. Rev. D 2019, 100, 012005. [Google Scholar] [CrossRef]
- Bondar, A.; Garmash, A.; Mizuk, R.; Santel, D.; Kinoshita, K.; Adachi, I.; Aihara, H.; Arinstein, K.; Asner, D.M.; Aushev, T.; et al. Observation of two charged bottomonium-like resonances in Y(5S) decays. Phys. Rev. Lett. 2012, 108, 122001. [Google Scholar] [CrossRef]
- Garmash, A.; Bondar, A.; Kuzmin, A.; Abdesselam, A.; Adachi, I.; Aihara, H.; Al Said, S.; Asner, D.M.; Aulchenko, V.; Aushev, T.; et al. Amplitude analysis of e+e−→Υ(nS)π+π− at GeV. Phys. Rev. D 2015, 91, 072003. [Google Scholar] [CrossRef]
- Garmash, A.; Abdesselam, A.; Adachi, I.; Aihara, H.; Asner, D.M.; Aushev, T.; Ayad, R.; Aziz, T.; Babu, V.; Badhrees, I.; et al. Observation of Zb(10610) and Zb(10650) Decaying to B Mesons. Phys. Rev. Lett. 2016, 116, 212001. [Google Scholar] [CrossRef]
- Klempt, E.; Zaitsev, A. Glueballs, Hybrids, Multiquarks. Experimental facts versus QCD inspired concepts. Phys. Rep. 2007, 454, 1. [Google Scholar] [CrossRef]
- Godfrey, S.; Olsen, S.L. The Exotic XYZ Charmonium-like Mesons. Ann. Rev. Nucl. Part. Sci. 2008, 58, 51. [Google Scholar] [CrossRef]
- Olsen, S.L. A New Hadron Spectroscopy. Front. Phys. 2015, 10, 121. [Google Scholar] [CrossRef]
- Hosaka, A.; Iijima, T.; Miyabayashi, K.; Sakai, Y.; Yasui, S. Exotic hadrons with heavy flavors: X,Y,Z, and related states. PTEP 2016, 2016, 062C01. [Google Scholar]
- Richard, J.M. Exotic hadrons: Review and perspectives. Few Body Syst. 2016, 57, 1185. [Google Scholar]
- Chen, H.X.; Chen, W.; Liu, X.; Zhu, S.L. The hidden-charm pentaquark and tetraquark states. Phys. Rep. 2016, 639, 1. [Google Scholar] [CrossRef]
- Esposito, A.; Pilloni, A.; Polosa, A.D. Multiquark Resonances. Phys. Rep. 2017, 668, 1. [Google Scholar] [CrossRef]
- Lebed, R.F.; Mitchell, R.E.; Swanson, E.S. Heavy-Quark QCD Exotica. Prog. Part. Nucl. Phys. 2017, 93, 143. [Google Scholar] [CrossRef]
- Ali, A.; Lange, J.S.; Stone, S. Exotics: Heavy Pentaquarks and Tetraquarks. Prog. Part. Nucl. Phys. 2017, 97, 123. [Google Scholar] [CrossRef]
- Olsen, S.L.; Skwarnicki, T.; Zieminska, D. Nonstandard heavy mesons and baryons: Experimental evidence. Rev. Mod. Phys. 2018, 90, 015003. [Google Scholar] [CrossRef]
- Karliner, M.; Rosner, J.L.; Skwarnicki, T. Multiquark States. Ann. Rev. Nucl. Part. Sci. 2018, 68, 17. [Google Scholar] [CrossRef]
- Yuan, C.Z. The XYZ states revisited. Int. J. Mod. Phys. A 2018, 33, 1830018. [Google Scholar] [CrossRef]
- Brambilla, N.; Eidelman, S.; Hanhart, C.; Nefediev, A.; Shen, C.; Thomas, C.E.; Vairo, A.; Yuan, C.Z. The XYZ states: Experimental and theoretical status and perspectives. arXiv 2019, arXiv:1907.07583. [Google Scholar] [CrossRef]
- Agaev, S.; Azizi, K.; Sundu, H. Four-quark exotic mesons. Turk. J. Phys. 2020, 44, 95. [Google Scholar] [CrossRef]
- Guo, F.K.; Hidalgo-Duque, C.; Nieves, J.; Valderrama, M.P. Consequences of Heavy Quark Symmetries for Hadronic Molecules. Phys. Rev. D 2013, 88, 054007. [Google Scholar] [CrossRef]
- Karliner, M.; Rosner, J.L. New Exotic Meson and Baryon Resonances from Doubly-Heavy Hadronic Molecules. Phys. Rev. Lett. 2015, 115, 122001. [Google Scholar] [CrossRef] [PubMed]
- Guo, F.K.; Hanhart, C.; Meißner, U.G.; Wang, Q.; Zhao, Q.; Zou, B.S. Hadronic molecules. Rev. Mod. Phys. 2018, 90, 015004. [Google Scholar] [CrossRef]
- Nielsen, M.; Navarra, F.S.; Lee, S.H. New Charmonium States in QCD Sum Rules: A Concise Review. Phys. Rep. 2010, 497, 41. [Google Scholar] [CrossRef]
- Kleiv, R.T.; Steele, T.G.; Zhang, A.; Blokland, I. Heavy-light diquark masses from QCD sum rules and constituent diquark models of tetraquarks. Phys. Rev. D 2013, 87, 125018. [Google Scholar] [CrossRef]
- Albuquerque, R.M.; Dias, J.M.; Khemchandani, K.P.; Martínez Torres, A.; Navarra, F.S.; Nielsen, M.; Zanetti, C.M. QCD sum rules approach to the X,Y and Z states. J. Phys. G 2019, 46, 093002. [Google Scholar] [CrossRef]
- Ebert, D.; Faustov, R.N.; Galkin, V.O. Masses of heavy tetraquarks in the relativistic quark model. Phys. Lett. B 2006, 634, 214. [Google Scholar] [CrossRef]
- Ebert, D.; Faustov, R.N.; Galkin, V.O.; Lucha, W. Masses of tetraquarks with two heavy quarks in the relativistic quark model. Phys. Rev. D 2007, 76, 114015. [Google Scholar] [CrossRef]
- Li, B.Q.; Chao, K.T. Higher Charmonia and X,Y,Z states with Screened Potential. Phys. Rev. D 2009, 79, 094004. [Google Scholar] [CrossRef]
- Brodsky, S.J.; Hwang, D.S.; Lebed, R.F. Dynamical Picture for the Formation and Decay of the Exotic XYZ Mesons. Phys. Rev. Lett. 2014, 113, 112001. [Google Scholar] [CrossRef]
- Cleven, M.; Guo, F.K.; Hanhart, C.; Wang, Q.; Zhao, Q. Employing spin symmetry to disentangle different models for the XYZ states. Phys. Rev. D 2015, 92, 014005. [Google Scholar] [CrossRef]
- Braaten, E.; Langmack, C.; Smith, D.H. Born-Oppenheimer Approximation for the XYZ Mesons. Phys. Rev. D 2014, 90, 014044. [Google Scholar] [CrossRef]
- Liu, Y.R.; Chen, H.X.; Chen, W.; Liu, X.; Zhu, S.L. Pentaquark and Tetraquark states. Prog. Part. Nucl. Phys. 2019, 107, 237. [Google Scholar] [CrossRef]
- Eichten, E.J.; Lane, K.; Quigg, C. New states above charm threshold. Phys. Rev. D 2006, 73, 014014. [Google Scholar] [CrossRef]
- Ma, L.; Liu, X.H.; Liu, X.; Zhu, S.L. Strong decays of the XYZ states. Phys. Rev. D 2015, 91, 034032. [Google Scholar] [CrossRef]
- Guo, F.K.; Liu, X.H.; Sakai, S. Threshold cusps and triangle singularities in hadronic reactions. Prog. Part. Nucl. Phys. 2020, 112, 103757. [Google Scholar] [CrossRef]
- Guo, F.K.; Hanhart, C.; Wang, Q.; Zhao, Q. Could the near-threshold XYZ states be simply kinematic effects? Phys. Rev. D 2015, 91, 051504. [Google Scholar] [CrossRef]
- Efimov, G.V.; Ivanov, M.A. Confinement and quark structure of light hadrons. Int. J. Mod. Phys. A 1989, 4, 2031. [Google Scholar] [CrossRef]
- Efimov, G.V.; Ivanov, M.A. The Quark Confinement Model of Hadrons; CRC Press: Boca Raton, FL, USA, 1993. [Google Scholar]
- Branz, T.; Faessler, A.; Gutsche, T.; Ivanov, M.A.; Körner, J.G.; Lyubovitskij, V.E. Relativistic constituent quark model with infrared confinement. Phys. Rev. D 2010, 81, 034010. [Google Scholar] [CrossRef]
- Terning, J. Gauging nonlocal Lagrangians. Phys. Rev. D 1991, 44, 887–897. [Google Scholar] [CrossRef]
- Gutsche, T.; Ivanov, M.A.; Körner, J.G.; Lyubovitskij, V.E.; Santorelli, P. Light baryons and their electromagnetic interactions in the covariant constituent quark model. Phys. Rev. D 2012, 86, 074013. [Google Scholar] [CrossRef]
- Gutsche, T.; Ivanov, M.A.; Körner, J.G.; Kovalenko, S.; Lyubovitskij, V.E. Nucleon tensor form factors in a relativistic confined quark model. Phys. Rev. D 2016, 94, 114030. [Google Scholar]
- Lyubovitskij, V.E.; Faessler, A.; Gutsche, T.; Ivanov, M.A.; Körner, J.G. Heavy baryons in the relativistic quark model. Prog. Part. Nucl. Phys. 2003, 50, 329. [Google Scholar]
- Faessler, A.; Gutsche, T.; Ivanov, M.A.; Körner, J.G.; Lyubovitskij, V.E. Semileptonic decays of double heavy baryons in a relativistic constituent three-quark model. Phys. Rev. D 2009, 80, 034025. [Google Scholar] [CrossRef]
- Faessler, A.; Gutsche, T.; Ivanov, M.A.; Körner, J.G.; Lyubovitskij, V.E. Decay properties of double heavy baryons. AIP Conf. Proc. 2010, 1257, 311. [Google Scholar]
- Branz, T.; Faessler, A.; Gutsche, T.; Ivanov, M.A.; Körner, J.G.; Lyubovitskij, V.E.; Oexl, B. Radiative decays of double heavy baryons in a relativistic constituent three–quark model including hyperfine mixing. Phys. Rev. D 2010, 81, 114036. [Google Scholar]
- Ivanov, M. Dynamical picture for the exotic XYZ states. EPJ Web Conf. 2018, 192, 00042. [Google Scholar] [CrossRef]
- Dubnicka, S.; Dubnickova, A.Z.; Ivanov, M.A.; Körner, J.G. Quark model description of the tetraquark state X(3872) in a relativistic constituent quark model with infrared confinement. Phys. Rev. D 2010, 81, 114007. [Google Scholar] [CrossRef]
- Dubnicka, S.; Dubnickova, A.Z.; Ivanov, M.A.; Körner, J.G.; Santorelli, P.; Saidullaeva, G.G. One-photon decay of the tetraquark state X(3872) → γ + J/ψ in a relativistic constituent quark model with infrared confinement. Phys. Rev. D 2011, 84, 014006. [Google Scholar] [CrossRef]
- Goerke, F.; Gutsche, T.; Ivanov, M.A.; Körner, J.G.; Lyubovitskij, V.E.; Santorelli, P. Four-quark structure of Zc(3900), Z(4430) and Xb(5568) states. Phys. Rev. D 2016, 94, 094017. [Google Scholar] [CrossRef]
- Goerke, F.; Gutsche, T.; Ivanov, M.A.; Körner, J.G.; Lyubovitskij, V.E. Zb(10610) and decays in a covariant quark model. Phys. Rev. D 2017, 96, 054028. [Google Scholar]
- Gutsche, T.; Ivanov, M.A.; Körner, J.G.; Lyubovitskij, V.E.; Xu, K. Test of the multiquark structure of a1(1420) in strong two-body decays. Phys. Rev. D 2017, 96, 114004. [Google Scholar]
- Gutsche, T.; Ivanov, M.A.; Körner, J.G.; Lyubovitskij, V.E. Isospin-violating strong decays of scalar single-heavy tetraquarks. Phys. Rev. D 2016, 94, 094012. [Google Scholar]
- Anikin, I.V.; Ivanov, M.A.; Kulimanova, N.B.; Lyubovitskij, V.E. The Extended Nambu-Jona-Lasinio model with separable interaction: Low-energy pion physics and pion nucleon form-factor. Z. Phys. C 1995, 65, 681. [Google Scholar] [CrossRef]
- Salam, A. Lagrangian theory of composite particles. Nuovo Cim. 1962, 25, 224–227. [Google Scholar] [CrossRef]
- Weinberg, S. Elementary particle theory of composite particles. Phys. Rev. 1963, 130, 776–783. [Google Scholar]
- Mandelstam, S. Quantum electrodynamics without potentials. Ann. Phys. 1962, 19, 1–24. [Google Scholar] [CrossRef]
- Vermaseren, J.A.M. New features of FORM. arXiv 2020, arXiv:math-ph/0010025. [Google Scholar]
- Voloshin, M.B. Interference and binding effects in decays of possible molecular component of X(3872). Phys. Lett. B 2004, 579, 316. [Google Scholar]
- Wong, C.Y. Molecular states of heavy quark mesons. Phys. Rev. C 2004, 69, 055202. [Google Scholar]
- AlFiky, M.T.; Gabbiani, F.; Petrov, A.A. X(3872): Hadronic molecules in effective field theory. Phys. Lett. B 2006, 640, 238. [Google Scholar] [CrossRef]
- Fleming, S.; Kusunoki, M.; Mehen, T.; van Kolck, U. Pion interactions in the X(3872). Phys. Rev. D 2007, 76, 034006. [Google Scholar] [CrossRef]
- Bignamini, C.; Grinstein, B.; Piccinini, F.; Polosa, A.D.; Sabelli, C. Is the X(3872) Production Cross Section at Tevatron Compatible with a Hadron Molecule Interpretation? Phys. Rev. Lett. 2009, 103, 162001. [Google Scholar] [CrossRef] [PubMed]
- Hidalgo-Duque, C.; Nieves, J.; Valderrama, M.P. Light flavor and heavy quark spin symmetry in heavy meson molecules. Phys. Rev. D 2013, 87, 076006. [Google Scholar]
- Braaten, E.; Lu, M. Line shapes of the X(3872). Phys. Rev. D 2007, 76, 094028. [Google Scholar] [CrossRef]
- Lee, I.W.; Faessler, A.; Gutsche, T.; Lyubovitskij, V.E. X(3872) as a molecular DD* state in a potential model. Phys. Rev. D 2009, 80, 094005. [Google Scholar] [CrossRef]
- Chiu, T.-W.; Hsieh, T.-H. X(3872) in lattice QCD with exact chiral symmetry. Phys. Lett. B 2007, 646, 95. [Google Scholar] [CrossRef]
- Barnes, T.; Godfrey, S. Charmonium options for the X(3872). Phys. Rev. D 2004, 69, 054008. [Google Scholar] [CrossRef]
- Eichten, E.J.; Lane, K.; Quigg, C. Charmonium levels near threshold and the narrow state X(3872)→π+π−J/ψ. Phys. Rev. D 2004, 69, 094019. [Google Scholar] [CrossRef]
- Kalashnikova, Y.S. Coupled-channel model for charmonium levels and an option for X(3872). Phys. Rev. D 2005, 72, 034010. [Google Scholar] [CrossRef]
- Matheus, R.D.; Navarra, F.S.; Nielsen, M.; Zanetti, C.M. QCD Sum Rules for the X(3872) as a mixed molecule-charmoniun state. Phys. Rev. D 2009, 80, 056002. [Google Scholar]
- Ferretti, J.; Galata, G.; Santopinto, E. Interpretation of the X(3872) as a charmonium state plus an extra component due to the coupling to the meson-meson continuum. Phys. Rev. C 2013, 88, 015207. [Google Scholar] [CrossRef]
- Takizawa, M.; Takeuchi, S. X(3872) as a hybrid state of charmonium and the hadronic molecule. PTEP 2013, 2013, 093D01. [Google Scholar] [CrossRef]
- Ferretti, J.; Galata, G.; Santopinto, E. Quark structure of the X(3872) and χb(3P) resonances. Phys. Rev. D 2014, 90, 054010. [Google Scholar] [CrossRef]
- Zhang, O.; Meng, C.; Zheng, H.Q. Ambiversion of X(3872). Phys. Lett. B 2009, 680, 453. [Google Scholar] [CrossRef][Green Version]
- Swanson, E.S. Diagnostic decays of the X(3872). Phys. Lett. B 2004, 598, 197. [Google Scholar]
- Swanson, E.S. Short range structure in the X(3872). Phys. Lett. B 2004, 588, 189. [Google Scholar] [CrossRef]
- Suzuki, M. The X(3872) boson: Molecule or charmonium. Phys. Rev. D 2005, 72, 114013. [Google Scholar] [CrossRef]
- Liu, Y.R.; Liu, X.; Deng, W.Z.; Zhu, S.L. Is X(3872) Really a Molecular State? Eur. Phys. J. C 2008, 56, 63. [Google Scholar] [CrossRef]
- Coito, S.; Rupp, G.; van Beveren, E. X(3872) is not a true molecule. Eur. Phys. J. C 2013, 73, 2351. [Google Scholar]
- Seth, K.K. An Alternative Interpretation of X(3872). Phys. Lett. B 2005, 612, 1. [Google Scholar] [CrossRef][Green Version]
- Hogaasen, H.; Richard, J.M.; Sorba, P. A Chromomagnetic mechanism for the X(3872) resonance. Phys. Rev. D 2006, 73, 054013. [Google Scholar] [CrossRef]
- Ortega, P.G.; Ruiz Arriola, E. Is X(3872) a bound state? Chin. Phys. C 2019, 43, 124107. [Google Scholar] [CrossRef]
- Li, B.A. Is X(3872) a possible candidate of hybrid meson. Phys. Lett. B 2005, 605, 306. [Google Scholar] [CrossRef]
- Close, F.E.; Page, P.R. The D*0 anti-D0 threshold resonance. Phys. Lett. B 2004, 578, 119. [Google Scholar] [CrossRef]
- Prelovsek, S.; Leskovec, L. Evidence for X(3872) from DD* scattering on the lattice. Phys. Rev. Lett. 2013, 111, 192001. [Google Scholar] [CrossRef]
- Padmanath, M.; Lang, C.B.; Prelovsek, S. X(3872) and Y(4140) using diquark-antidiquark operators with lattice QCD. Phys. Rev. D 2015, 92, 034501. [Google Scholar] [CrossRef]
- Matheus, R.D.; Narison, S.; Nielsen, M.; Richard, J.M. Can the X(3872) be a 1++ four quark state? Phys. Rev. D 2007, 75, 014005. [Google Scholar] [CrossRef]
- Narison, S.; Navarra, F.S.; Nielsen, M. On the nature of the X(3872) from QCD. Phys. Rev. D 2011, 83, 016004. [Google Scholar] [CrossRef]
- Gamermann, D.; Oset, E. Isospin breaking effects in the X(3872) resonance. Phys. Rev. D 2009, 80, 014003. [Google Scholar] [CrossRef]
- Gamermann, D.; Nieves, J.; Oset, E.; Ruiz Arriola, E. Couplings in coupled channels versus wave functions: Application to the X(3872) resonance. Phys. Rev. D 2010, 81, 014029. [Google Scholar]
- Danilkin, I.V.; Simonov, Y.A. Dynamical origin and the pole structure of X(3872). Phys. Rev. Lett. 2010, 105, 102002. [Google Scholar] [CrossRef] [PubMed]
- Maiani, L.; Piccinini, F.; Polosa, A.D.; Riquer, V. Diquark-antidiquarks with hidden or open charm and the nature of X(3872). Phys. Rev. D 2005, 71, 014028. [Google Scholar] [CrossRef]
- Tan, Y.; Ping, J. X(3872) in an unquenched quark model. Phys. Rev. D 2019, 100, 034022. [Google Scholar] [CrossRef]
- Hanhart, C.; Kalashnikova, Y.S.; Kudryavtsev, A.E.; Nefediev, A.V. Reconciling the X(3872) with the near-threshold enhancement in the final state. Phys. Rev. D 2007, 76, 034007. [Google Scholar] [CrossRef]
- Chen, Y.; Zhu, S.L. The Vector and Axial-Vector Charmonium-like States. Phys. Rev. D 2011, 83, 034010. [Google Scholar] [CrossRef]
- Wallbott, P.C.; Eichmann, G.; Fischer, C.S. X(3872) as a four quark state in a Dyson-Schwinger/Bethe-Salpeter approach. Phys. Rev. D 2019, 100, 014033. [Google Scholar] [CrossRef]
- Bigi, I.; Maiani, L.; Piccinini, F.; Polosa, A.; Riquer, V. Four-quark mesons in non-leptonic B decays: Could they resolve some old puzzles? Phys. Rev. D 2005, 72, 114016. [Google Scholar] [CrossRef]
- Abe, K.; Abe, K.; Adachi, I.; Aihara, H.; Aoki, K.; Arinstein, K.; Asano, Y.; Aso, T.; Aulchenko, V.; Aushev, T. Evidence for X(3872)→γJ/ψ and the sub-threshold decay X(3872)→ωJ/ψ. arXiv 2005, arXiv:hep-ex/0505037. [Google Scholar]
- Uhlemann, C.; Kauer, N. Narrow-width approximation accuracy. Nucl. Phys. B 2009, 814, 195–211. [Google Scholar] [CrossRef]
- Voloshin, M. Heavy quark spin selection rule and the properties of the X(3872). Phys. Lett. B 2004, 604, 69–73. [Google Scholar] [CrossRef]
- Voloshin, M. X(3872) diagnostics with decays to . Int. J. Mod. Phys. A 2006, 21, 1239–1250. [Google Scholar] [CrossRef]
- Dubynskiy, S.; Voloshin, M.B. Pionic transitions from X(3872) to χ(cJ). Phys. Rev. D 2008, 77, 014013. [Google Scholar] [CrossRef]
- Maiani, L.; Polosa, A.; Riquer, V. Indications of a Four-Quark Structure for the X(3872) and X(3876) Particles from Recent Belle and BABAR Data. Phys. Rev. Lett. 2007, 99. [Google Scholar] [CrossRef] [PubMed]
- Ivanov, M.A.; Körner, J.G.; Santorelli, P. The J/ψ dissociation cross-sections in a relativistic quark model. Phys. Rev. D 2004, 70, 014005. [Google Scholar] [CrossRef]
- Barnes, T. Charmonium cross-sections and the QGP. Eur. Phys. J. A 2003, 18, 531. [Google Scholar]
- Aubert, B.; Barate, R.; Bona, M.; Boutigny, D.; Couderc, F.; Karyotakis, Y.; Lees, J.P.; Poireau, V.; Tisserand, V.; Zghiche, A.; et al. Search for B+→X(3872)K+, X3872→J/ψγ. Phys. Rev. D 2006, 74, 071101. [Google Scholar] [CrossRef]
- Aubert, B.; Bona, M.; Karyotakis, Y.; Lees, J.P.; Poireau, V.; Prencipe, E.; Prudent, X.; Tisserand, V.; Garra Tico, J.; Grauges, E.; et al. Evidence for X(3872)→ψ2Sγ in B±→X3872K± decays, and a study of . Phys. Rev. Lett. 2009, 102, 132001. [Google Scholar] [CrossRef]
- Bhardwaj, V.; Trabelsi, K.; Singh, J.B.; Choi, S.-K.; Olsen, S.L.; Adachi, I.; Adamczyk, K.; Asner, D.M.; Aulchenko, V.; Aushev, T.; et al. Observation of X(3872)→J/ψγ and search for X(3872)→ψ′γ in B decays. Phys. Rev. Lett. 2011, 107, 091803. [Google Scholar] [CrossRef]
- Aaij, R.; Adeva, B.; Adinolfi, M.; Affolder, A.; Ajaltouni, Z.; Albrecht, J.; Alessio, F.; Alexander, M.; Ali, S.; Alkhazov, G.; et al. Evidence for the decay X(3872)→ψ(2S)γ. Nucl. Phys. B 2014, 886, 665–680. [Google Scholar] [CrossRef]
- Colangelo, P.; De Fazio, F.; Nicotri, S. X(3872)→ decays and the structure of X3872. Phys. Lett. B 2007, 650, 166–171. [Google Scholar] [CrossRef]
- Dong, Y.; Faessler, A.; Gutsche, T.; Kovalenko, S.; Lyubovitskij, V.E. X(3872) as a hadronic molecule and its decays to charmonium states and pions. Phys. Rev. D 2009, 79, 094013. [Google Scholar]
- Nielsen, M.; Zanetti, C. Radiative decay of the X(3872) as a mixed molecule-charmonium state in QCD Sum Rules. Phys. Rev. D 2010, 82, 116002. [Google Scholar] [CrossRef]
- Wang, T.; Wang, G. Radiative E1 decays of X(3872). Phys. Lett. B 2011, 697, 233–237. [Google Scholar] [CrossRef]
- Ke, H.; Li, X. What do the radiative decays of X(3872) tell us. Phys. Rev. D 2011, 84, 114026. [Google Scholar] [CrossRef]
- Takizawa, M.; Takeuchi, S.; Shimizu, K. Radiative X(3872) Decays in Charmonium-Molecule Hybrid Model. Few Body Syst. 2014, 55, 779. [Google Scholar] [CrossRef]
- Badalian, A.; Simonov, Y.; Bakker, B. interaction above threshold and the radiative decay X(3872)→J/ψγ. Phys. Rev. D 2015, 91, 056001. [Google Scholar]
- Takeuchi, S.; Takizawa, M.; Shimizu, K. Radiative Decays of the X(3872) in the Charmonium-Molecule Hybrid Picture. JPS Conf. Proc. 2017, 17, 112001. [Google Scholar]
- Cincioglu, E.; Ozpineci, A. Radiative decay of the X(3872) as a mixed molecule-charmonium state in effective field theory. Phys. Lett. B 2019, 797, 134856. [Google Scholar] [CrossRef]
- Ivanov, M.A.; Locher, M.; Lyubovitskij, V.E. Electromagnetic form-factors of nucleons in a relativistic three quark model. Few Body Syst. 1996, 21, 131. [Google Scholar] [CrossRef]
- Körner, J.G.; Mauser, M.C. One loop corrections to polarization observables. Lect. Notes Phys. 2004, 647, 212. [Google Scholar]
- Dias, J.M.; Navarra, F.S.; Nielsen, M.; Zanetti, C.M. (3900) decay width in QCD sum rules. Phys. Rev. D 2013, 88, 016004. [Google Scholar]
- Wang, Z.G. The magnetic moment of the Zc(3900) as an axialvector tetraquark state with QCD sum rules. Eur. Phys. J. C 2018, 78, 297. [Google Scholar] [CrossRef]
- Wang, Z.G.; Zhang, J.X. The decay width of the Zc(3900) as an axialvector tetraquark state in solid quark-hadron duality. Eur. Phys. J. C 2018, 78, 14. [Google Scholar] [CrossRef]
- Deng, C.; Ping, J.; Wang, F. Interpreting Zc(3900) and Zc(4025)/Zc(4020) as charged tetraquark states. Phys. Rev. D 2014, 90, 054009. [Google Scholar]
- Ke, H.W.; Wei, Y.; Li, X.Q. Is Zc(3900) a molecular state. Eur. Phys. J. C 2013, 73, 2561. [Google Scholar] [CrossRef]
- Wilbring, E.; Hammer, H.W.; Meißner, U.-G. Electromagnetic Structure of the Zc(3900). Phys. Lett. B 2013, 726, 326. [Google Scholar] [CrossRef]
- Zhang, J.R. Improved QCD sum rule study of Zc(3900) as a molecular state. Phys. Rev. D 2013, 87, 116004. [Google Scholar] [CrossRef]
- Cui, C.Y.; Liu, Y.L.; Chen, W.B.; Huang, M.Q. Could Zc(3900) be a IGJP = 1+1+ molecular state? J. Phys. G 2014, 41, 075003. [Google Scholar] [CrossRef][Green Version]
- Patel, S.; Shah, M.; Vinodkumar, P.C. Mass spectra of four quark states in the hidden charm sector. Eur. Phys. J. A 2014, 50, 131. [Google Scholar] [CrossRef][Green Version]
- Chen, D.Y.; Dong, Y.B. Radiative decays of the neutral Zc(3900). Phys. Rev. D 2016, 93, 014003. [Google Scholar] [CrossRef]
- Ortega, P.G.; Segovia, J.; Entem, D.R.; Fernandez, F. The Zc structures in a coupled-channels model. Eur. Phys. J. C 2019, 79, 78. [Google Scholar] [CrossRef]
- Xiao, L.Y.; Wang, G.J.; Zhu, S.L. Hidden-charm strong decays of the Zc states. Phys. Rev. D 2019, 101, 054001. [Google Scholar]
- Prelovsek, S.; Leskovec, L. Search for (3900) in the 1+− Channel on the Lattice. Phys. Lett. B 2013, 727, 172. [Google Scholar] [CrossRef]
- Chen, Y.; Gong, M.; Lei, Y.; Li, N.; Liang, J.; Liu, C.; Liu, H.; Liu, J.; Liu, L.; Liu, Y.; et al. Low-energy scattering of the system and the resonance-like structure Zc(3900). Phys. Rev. D 2014, 89, 094506. [Google Scholar] [CrossRef]
- Prelovsek, S.; Lang, C.B.; Leskovec, L.; Mohler, D. Study of the channel using lattice QCD. Phys. Rev. D 2015, 91, 014504. [Google Scholar] [CrossRef]
- Chen, T.; Chen, Y.; Gong, M.; Liu, C.; Liu, L.; Liu, Y.; Liu, Z.; Ma, J.; Werner, M.; Zhang, J. A coupled-channel lattice study on the resonance-like structure Zc(3900). Chin. Phys. C 2019, 43, 103103. [Google Scholar]
- Ikeda, Y.; Aoki, S.; Doi, T.; Gongyo, S.; Hatsuda, T.; Inoue, T.; Iritani, T.; Ishii, N.; Murano, K.; Sasaki, K. Fate of the Tetraquark Candidate Zc(3900) from Lattice QCD. Phys. Rev. Lett. 2016, 117, 242001. [Google Scholar] [CrossRef]
- Ikeda, Y. The tetraquark candidate Zc(3900) from dynamical lattice QCD simulations. J. Phys. G 2018, 45, 024002. [Google Scholar] [CrossRef]
- Liu, C.; Liu, L.; Zhang, K.L. Towards the understanding of Zc(3900) from lattice QCD. Phys. Rev. D 2020, 101, 054502. [Google Scholar] [CrossRef]
- Braaten, E. How the Zc(3900) Reveals the Spectra of Quarkonium Hybrid and Tetraquark Mesons. Phys. Rev. Lett. 2013, 111, 162003. [Google Scholar] [CrossRef]
- Voloshin, M.B. Zc(3900)—What is inside? Phys. Rev. D 2013, 87, 091501. [Google Scholar] [CrossRef]
- Zhao, L.; Deng, W.Z.; Zhu, S.L. Hidden-Charm Tetraquarks and Charged Zc States. Phys. Rev. D 2014, 90, 094031. [Google Scholar] [CrossRef]
- Li, G. Hidden-charmonium decays of Zc(3900) and Zc(4025) in intermediate meson loops model. Eur. Phys. J. C 2013, 73, 2621. [Google Scholar] [CrossRef][Green Version]
- Chen, D.Y.; Liu, X.; Matsuki, T. Reproducing the Zc(3900) structure through the initial-single-pion-emission mechanism. Phys. Rev. D 2013, 88, 036008. [Google Scholar] [CrossRef]
- Maiani, L.; Riquer, V.; Faccini, R.; Piccinini, F.; Pilloni, A.; Polosa, A.D. A JPG = 1++ Charged Resonance in the Y(4260)→π+π−J/ψ Decay? Phys. Rev. D 2013, 87, 111102. [Google Scholar] [CrossRef]
- Aceti, F.; Bayar, M.; Oset, E.; Martinez Torres, A.; Khemchandani, K.P.; Dias, J.M.; Navarra, F.S.; Nielsen, M. Prediction of an I = 1 state and relationship to the claimed Zc(3900), Zc(3885). Phys. Rev. D 2014, 90, 016003. [Google Scholar] [CrossRef]
- Agaev, S.S.; Azizi, K.; Sundu, H. Strong (3900)→J/ψπ+;ηcρ+ decays in QCD. Phys. Rev. D 2016, 93, 074002. [Google Scholar] [CrossRef]
- Albaladejo, M.; Guo, F.K.; Hidalgo-Duque, C.; Nieves, J. Zc(3900): What has been really seen? Phys. Lett. B 2016, 755, 337. [Google Scholar] [CrossRef]
- Agaev, S.S.; Azizi, K.; Sundu, H. Treating Zc(3900) and Z(4430) as the ground-state and first radially excited tetraquarks. Phys. Rev. D 2017, 96, 034026. [Google Scholar] [CrossRef]
- Ozdem, U.; Azizi, K. Magnetic and quadrupole moments of the Zc(3900). Phys. Rev. D 2017, 96, 074030. [Google Scholar] [CrossRef]
- Pillonia, A.; Fernandez-Ramirez, C.; Jackurac, A.; Mathieu, V.; Mikhasenko, M.; Nys, J.; Szczepaniak, A.P. Amplitude analysis and the nature of the Zc(3900). Phys. Lett. B 2017, 772, 200. [Google Scholar] [CrossRef]
- He, J.; Chen, D.Y. Zc(3900)/Zc(3885) as a virtual state from interaction. Eur. Phys. J. C 2018, 78, 94. [Google Scholar] [CrossRef]
- Chen, H.X.; Chen, W. Settling the Zc(4600) in the charged charmoniumlike family. Phys. Rev. D 2019, 99, 074022. [Google Scholar] [CrossRef]
- Chiu, T.-W.; Hsieh, T.-H. Y(4260) on the lattice. Phys. Rev. D 2006, 73, 094510. [Google Scholar] [CrossRef]
- Albuquerque, R.M.; Nielsen, M. QCD sum rules study of the J(PC) = 1– charmonium Y mesons. Nucl. Phys. A 2009, 815, 53. [Google Scholar] [CrossRef]
- Ding, G.J. Are Y(4260) and Z+(2) are D(1) D or D(0) D* Hadronic Molecules? Phys. Rev. D 2009, 79, 014001. [Google Scholar] [CrossRef]
- Wang, Q.; Cleven, M.; Guo, F.K.; Hanhart, C.; Meißner, U.G.; Wu, X.G.; Zhao, Q. Y(4260): Hadronic molecule versus hadro-charmonium interpretation. Phys. Rev. D 2014, 89, 034001. [Google Scholar] [CrossRef]
- Xue, S.R.; Jing, H.J.; Guo, F.K.; Zhao, Q. Disentangling the role of the Y(4260) in and via line shape studies. Phys. Lett. B 2018, 779, 402. [Google Scholar] [CrossRef]
- Chen, Y.H.; Dai, L.Y.; Guo, F.K.; Kubis, B. Nature of the Y(4260): A light-quark perspective. Phys. Rev. D 2019, 99, 074016. [Google Scholar] [CrossRef]
- Qiao, C.F. One explanation for the exotic state Y(4260). Phys. Lett. B 2006, 639, 263. [Google Scholar] [CrossRef]
- Yuan, C.Z.; Wang, P.; Mo, X.H. The Y(4260) as an omega chi(c1) molecular state. Phys. Lett. B 2006, 634, 399. [Google Scholar] [CrossRef]
- Liu, X.; Li, G. Exploring the threshold behavior and implications on the nature of Y(4260) and Zc(3900). Phys. Rev. D 2013, 88, 014013. [Google Scholar] [CrossRef]
- Cleven, M.; Wang, Q.; Guo, F.K.; Hanhart, C.; Meißner, U.G.; Zhao, Q. Y(4260) as the first S-wave open charm vector molecular state? Phys. Rev. D 2014, 90, 074039. [Google Scholar]
- Liu, X.; Zeng, X.; Li, X. Possible molecular structure of the newly observed Y(4260). Phys. Rev. D 2005, 72, 054023. [Google Scholar]
- Li, X.; Voloshin, M. Suppression of the S-wave production of (3/2)+ + (1/2)− heavy meson pairs in e+e− annihilation. Phys. Rev. D 2013, 88, 034012. [Google Scholar] [CrossRef]
- Zhu, S.L. The Possible interpretations of Y(4260). Phys. Lett. B 2005, 625, 212. [Google Scholar] [CrossRef]
- Llanes-Estrada, F.J. Y(4260) and possible charmonium assignment. Phys. Rev. D 2005, 72, 031503. [Google Scholar]
- Kou, E.; Pene, O. Suppressed decay into open charm for the Y(4260) being an hybrid. Phys. Lett. B 2005, 631, 164. [Google Scholar] [CrossRef]
- Close, F.E.; Page, P.R. Gluonic charmonium resonances at BaBar and BELLE? Phys. Lett. B 2005, 628, 215–222. [Google Scholar] [CrossRef]
- Maiani, L.; Riquer, V.; Piccinini, F.; Polosa, A.D. Four quark interpretation of Y(4260). Phys. Rev. D 2005, 72, 031502. [Google Scholar]
- Dong, Y.; Faessler, A.; Gutsche, T.; Lyubovitskij, V.E. Selected strong decay modes of Y(4260). Phys. Rev. D 2014, 89, 034018. [Google Scholar] [CrossRef]
- Wang, Z.G. Tetraquark state candidates: Y(4260), Y(4360), Y(4660) and Zc(4020/4025). Eur. Phys. J. C 2016, 76, 387. [Google Scholar] [CrossRef]
- Tan, Y.; Ping, J. Y(4626) in a chiral constituent quark model. Phys. Rev. D 2020, 101, 054010. [Google Scholar]
- Zhang, J.R.; Huang, M.Q. The P-wave tetraquark state: Y(4260) or Y(4660)? Phys. Rev. D 2011, 83, 036005. [Google Scholar] [CrossRef]
- Martinez Torres, A.; Khemchandani, K.P.; Gamermann, D.; Oset, E. The Y(4260) as a system. Phys. Rev. D 2009, 80, 094012. [Google Scholar] [CrossRef]
- Dubnička, S.; Dubničková, A.; Issadykov, A.; Ivanov, M.A.; Liptaj, A. Y(4260) as four quark state. arXiv 2020, arXiv:2003.04142. [Google Scholar]
- Sun, Z.F.; He, J.; Liu, X.; Luo, Z.G.; Zhu, S.L. Zb(10610)± and Zb(10650)± as the and molecular states. Phys. Rev. D 2011, 84, 054002. [Google Scholar] [CrossRef]
- Bondar, A.E.; Garmash, A.; Milstein, A.I.; Mizuk, R.; Voloshin, M.B. Heavy quark spin structure in Zb resonances. Phys. Rev. D 2011, 84, 054010. [Google Scholar] [CrossRef]
- Dong, Y.; Faessler, A.; Gutsche, T.; Lyubovitskij, V.E. Decays of and ) as Hadronic Molecules. J. Phys. G 2013, 40, 015002. [Google Scholar]
- Li, X.; Voloshin, M.B. Zb(10610) and Zb(10650) decays to bottomonium plus pion. Phys. Rev. D 2012, 86, 077502. [Google Scholar] [CrossRef]
- Cleven, M.; Wang, Q.; Guo, F.K.; Hanhart, C.; Meißner, U.G.; Zhao, Q. Confirming the molecular nature of the Zb(10610) and the Zb(10650). Phys. Rev. D 2013, 87, 074006. [Google Scholar] [CrossRef]
- Voloshin, M.B. Enhanced mixing of partial waves near threshold for heavy meson pairs and properties of Zb(10610) and Zb(10650) resonances. Phys. Rev. D 2013, 87, 074011. [Google Scholar] [CrossRef]
- Wang, Z.G. Reanalysis of the Y(3940), Y(4140), Zc(4020), Zc(4025) and Zb(10650) as molecular states with QCD sum rules. Eur. Phys. J. C 2014, 74, 2963. [Google Scholar] [CrossRef]
- Baru, V.; Epelbaum, E.; Filin, A.A.; Hanhart, C.; Nefediev, A.V. Spin partners of the Zb(10610) and Zb(10650) revisited. JHEP 2017, 1706, 158. [Google Scholar] [CrossRef]
- Baru, V.; Epelbaum, E.; Filin, A.A.; Hanhart, C.; Nefediev, A.V.; Wang, Q. Spin partners WbJ from the line shapes of the Zb(10610) and Zb(10650). Phys. Rev. D 2019, 99, 094013. [Google Scholar]
- Ali, A.; Hambrock, C.; Wang, W. Tetraquark Interpretation of the Charged Bottomonium-like states and and Implications. Phys. Rev. D 2012, 85, 054011. [Google Scholar]
- Wang, Z.G.; Huang, T. The Zb(10610) and Zb(10650) as axial-vector tetraquark states in the QCD sum rules. Nucl. Phys. A 2014, 930, 63. [Google Scholar]
- Agaev, S.S.; Azizi, K.; Sundu, H. Spectroscopic parameters and decays of the resonance Zb(10610). Eur. Phys. J. C 2017, 77, 836. [Google Scholar] [CrossRef]
- Ozdem, U.; Azizi, K. Magnetic dipole moment of Zb(10610) in light-cone QCD. Phys. Rev. D 2018, 97, 014010. [Google Scholar] [CrossRef]
- Li, M.T.; Wang, W.L.; Dong, Y.B.; Zhang, Z.Y. Zb(10650) and Zb(10610) States in A Chiral Quark Model. J. Phys. G 2013, 40, 015003. [Google Scholar] [CrossRef]
- Chen, D.Y.; Liu, X.; Matsuki, T. Interpretation of Zb(10610) and Zb(10650) in the ISPE mechanism and the Charmonium Counterpart. Chin. Phys. C 2014, 38, 053102. [Google Scholar] [CrossRef]
- Swanson, E.S. Zb and Zc Exotic States as Coupled Channel Cusps. Phys. Rev. D 2015, 91, 034009. [Google Scholar] [CrossRef]
- Kang, X.W.; Guo, Z.H.; Oller, J.A. General considerations on the nature of Zb(10610) and Zb(10650) from their pole positions. Phys. Rev. D 2016, 94, 014012. [Google Scholar] [CrossRef]
- Wang, Q.; Baru, V.; Filin, A.A.; Hanhart, C.; Nefediev, A.V.; Wynen, J.-L. Line shapes of the Zb(10610) and Zb(10650) in the elastic and inelastic channels revisited. Phys. Rev. D 2018, 98, 074023. [Google Scholar] [CrossRef]
- Voloshin, M.B. Radiative transitions from Upsilon(5S) to molecular bottomonium. Phys. Rev. D 2011, 84, 031502. [Google Scholar]
- Zhang, J.R.; Zhong, M.; Huang, M.Q. Could Zb(10610) be a B*B¯ molecular state? Phys. Lett. B 2011, 704, 312. [Google Scholar]
- Cui, C.; Liu, Y.; Huang, M. Investigating different structures of the Zb(10610) and Zb(10650). Phys. Rev. D 2012, 85, 074014. [Google Scholar] [CrossRef]
- Yang, Y.; Ping, J.; Deng, C.; Zong, H.S. Dynamical study of the Zb(10610) and Zb(10650) as molecular states. J. Phys. G 2012, 39, 105001. [Google Scholar] [CrossRef]
- Danilkin, I.V.; Orlovsky, V.D.; Simonov, Y.A. Hadron interaction with heavy quarkonia. Phys. Rev. D 2012, 85, 034012. [Google Scholar] [CrossRef]
- Chen, D.Y.; Liu, X.; Zhu, S.L. Charged bottomonium-like states Zb(10610) and Zb(10650) and the Υ(5S)→Υ(2S)π+π− decay. Phys. Rev. D 2011, 84, 074016. [Google Scholar] [CrossRef]
- Chen, D.; Liu, X. Zb(10610) and Zb(10650) structures produced by the initial single pion emission in the Υ(5S) decays. Phys. Rev. D 2011, 84, 094003. [Google Scholar] [CrossRef]
- Liu, X.; Chen, D. Charged Bottomonium-Like Structures Zb(10610) and Zb(10650). Few Body Syst. 2013, 54, 165–170. [Google Scholar] [CrossRef]
- Cleven, M.; Guo, F.K.; Hanhart, C.; Meißner, U.G. Bound state nature of the exotic Zb states. Eur. Phys. J. A 2011, 47, 120. [Google Scholar] [CrossRef]
- Guo, T.; Cao, L.; Zhou, M.Z.; Chen, H. The possible candidates of tetraquark: Zb(10610) and Zb(10650). arXiv 2011, arXiv:1106.2284. [Google Scholar]
- Navarra, F.S.; Nielsen, M.; Richard, J.M. Exotic Charmonium and Bottomonium-like Resonances. J. Phys. Conf. Ser. 2012, 348, 012007. [Google Scholar] [CrossRef]
- Li, G.; Shao, F.; Zhao, C.W.; Zhao, Q. and hbπ decays in intermediate meson loops model. Phys. Rev. D 2013, 87, 034020. [Google Scholar] [CrossRef]
- Ohkoda, S.; Yasui, S.; Hosaka, A. Decays of Zb→Υπ via triangle diagrams in heavy meson molecules. Phys. Rev. D 2014, 89, 074029. [Google Scholar] [CrossRef]
- Dias, J.M.; Aceti, F.; Oset, E. Study of and interactions in I=1 and relationship to the Zb(10610), Zb(10650) states. Phys. Rev. D 2015, 91, 076001. [Google Scholar] [CrossRef]
- Huo, W.S.; Chen, G.Y. The nature of Zb states from a combined analysis of Υ(5S)→hb(mP)π+π− and . Eur. Phys. J. C 2016, 76, 172. [Google Scholar] [CrossRef]
- Gutsche, T.; Lyubovitskij, V.E.; Schmidt, I. Tetraquarks in holographic QCD. Phys. Rev. D 2017, 96, 034030. [Google Scholar] [CrossRef]

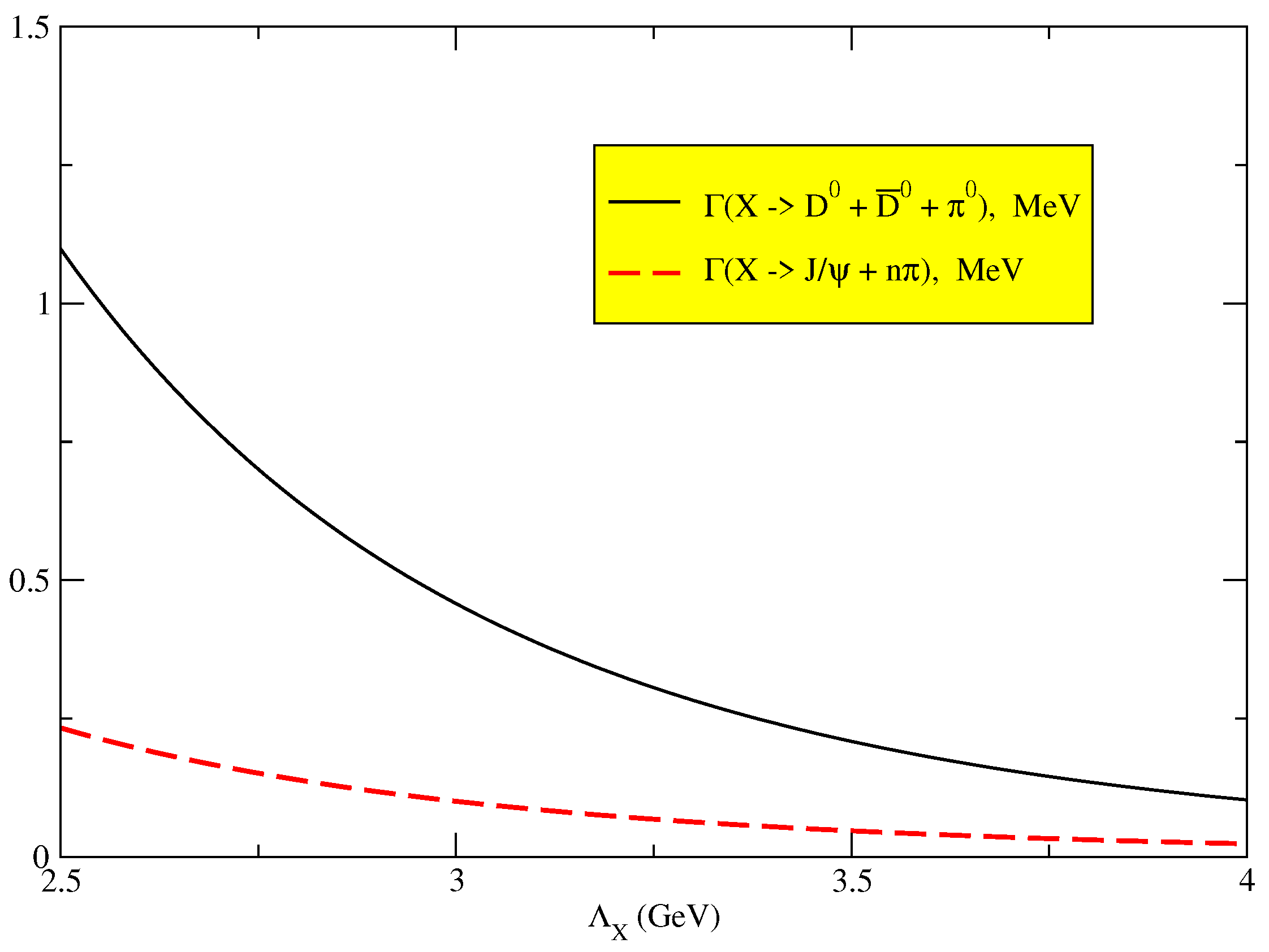

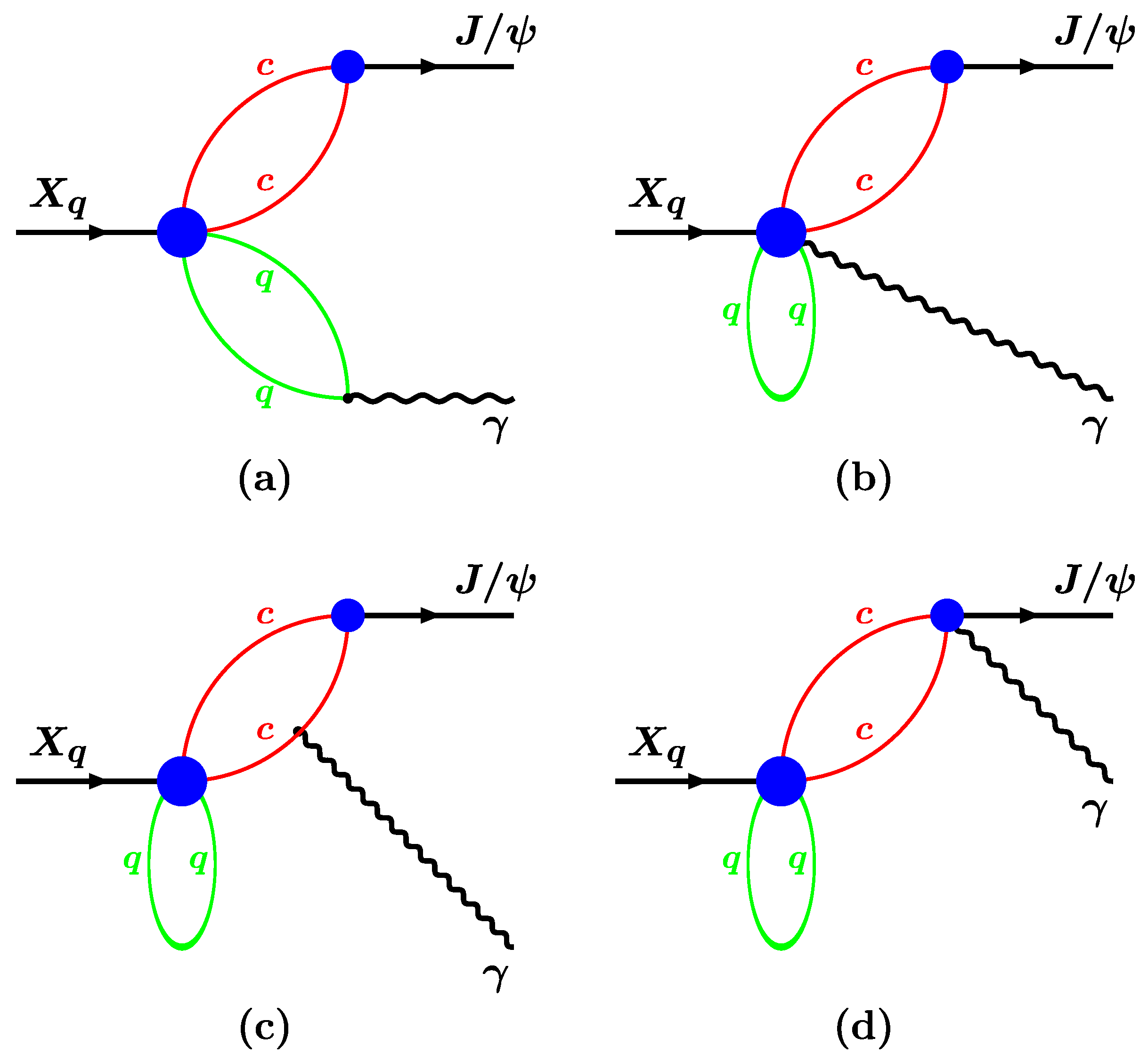
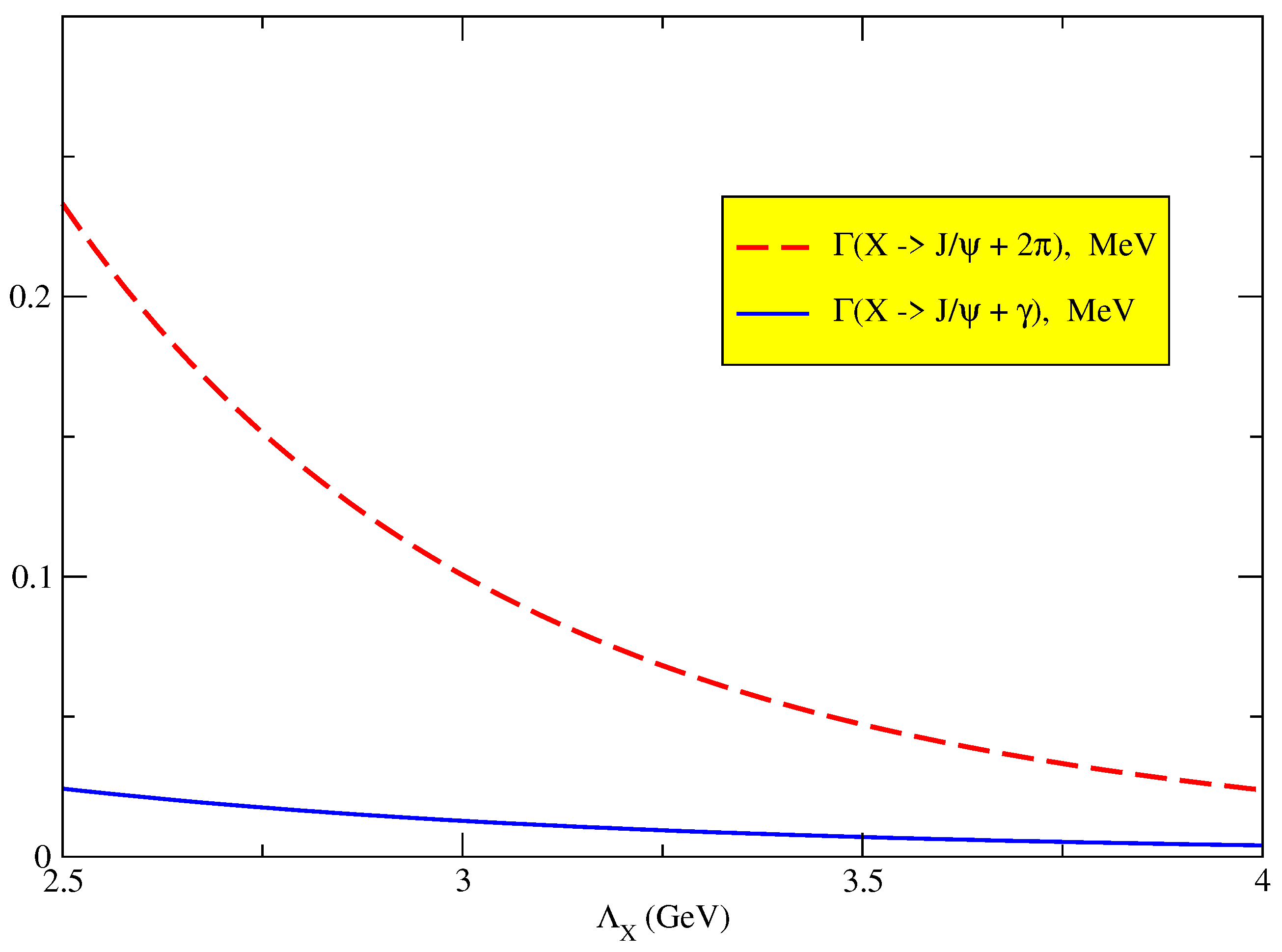

| Mode | Molecular-Type Current | Tetraquark Current |
|---|---|---|
| 0 |
| Quantum Number | Name | Quark Current | Mass (MeV) |
|---|---|---|---|
| () | |||
| () | |||
| () | |||
| () | |||
| () |
| Channel | Widths, MeV | |
|---|---|---|
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dubnička, S.; Dubničková, A.Z.; Ivanov, M.A.; Liptaj, A. Dynamical Approach to Decays of XYZ States. Symmetry 2020, 12, 884. https://doi.org/10.3390/sym12060884
Dubnička S, Dubničková AZ, Ivanov MA, Liptaj A. Dynamical Approach to Decays of XYZ States. Symmetry. 2020; 12(6):884. https://doi.org/10.3390/sym12060884
Chicago/Turabian StyleDubnička, Stanislav, Anna Zuzana Dubničková, Mikhail A. Ivanov, and Andrej Liptaj. 2020. "Dynamical Approach to Decays of XYZ States" Symmetry 12, no. 6: 884. https://doi.org/10.3390/sym12060884
APA StyleDubnička, S., Dubničková, A. Z., Ivanov, M. A., & Liptaj, A. (2020). Dynamical Approach to Decays of XYZ States. Symmetry, 12(6), 884. https://doi.org/10.3390/sym12060884





