Superfields, Nilpotent Superfields and Superschemes ^†

Abstract

We interpret superfields in a functorial formalism that explains the properties that are assumed for them in the physical applications. We study the non-trivial relation of scalar superfields with the defining sheaf of the supermanifold of super spacetime. We also investigate in the present work some constraints that are imposed on the superfields, which allow for non-trivial solutions. They give rise to superschemes that, generically, are not regular, that is they do not define a standard supermanifold.

1. Introduction

Two equivalent approaches are used by mathematicians to understand supervarieties and supermanifolds. The classical one is by means of sheaves of superalgebras that properly generalize similar definitions of standard algebraic geometry. This approach started with Berezin, Leites, Kostant, and Manin [1,2,3,4]. We will be more precise in Section 2 and Section 4.1, but let us make a rough approximation here. From the point of view of algebraic geometry, spaces are described in terms of rings. Essentially, one constructs first a topological space associated with the ring. The category of such spaces is then (contravariantly) equivalent to the category of rings considered. One can proceed by replacing commutative rings with commutative (or supercommutative)superrings and most of the geometric structures generalize, although new phenomena appear. The generalization is successful because, even when superalgebras are non-commutative algebras, their non-commutativity is of a very particular kind, and one does not need indeed to go into the troubles of the full non-commutative geometry. Useful reviews of this approach are for example Refs [5,6,7,8].

On the other hand, the functorial approach to supergeometry was started by Schwarz [9]. A superspace is described as the association of a set to any arbitrary Grassmann algebra $\mathsf{\Lambda }$. The elements in each set are called $\mathsf{\Lambda }$-points. The sets of $\mathsf{\Lambda }$-points, though, have to behave properly under homomorphisms of superalgebras $\mathsf{\Lambda }\to {\mathsf{\Lambda }}^{\prime }$. This means that the assignation has to be functorial, so a superspace is a covariant functor from the category of Grassmann algebras to the category of sets.

The two approaches are related. A space M can also be described in terms of the morphisms $S\to M$ from any other space S in the same category to M. Each of these morphisms is called an S-point of M. The association with each S of the set $M\left(S\right):=\mathrm{Hom}(S,M)$ is a contravariant functor called the functor of points of M. A morphism between two spaces $f:M\to N$ can be given as a set of maps $M\left(S\right)\to N\left(S\right)$ between S-points that is functorial in S. This is in fact the content of Yoneda’s lemma.

Using the equivalence of categories between spaces and rings, the functor can be given over the rings corresponding to the global sections of the superspaces, so we will have a covariant functor $\mathcal{O}\left(S\right)\to \mathrm{Hom}\left(\mathcal{O}\right(M),\mathcal{O}(S\left)\right)$.

The construction, with its own peculiarities, can be carried over to the category of superrings. Then, the functor of points (A didactic exposition of the functorial approach in modern terms was given in the talk by P.Deligne linked in [10].), when restricted to the subcategory of Grassmann algebras, can be interpreted as the functor of Schwarz [9]. The original idea was developed further in [11], where mappings among superspaces, that is natural transformations of the functors, were connected with the more standard approach of sheaf theory.

Yet another approach to formalize the theory of supermanifolds was proposed some time ago by Rogers and De Witt [12,13]. The supergeometry was introduced here by generalizing the numbers (real or complex numbers) to supernumbers, which are elements of a Grassmann algebra, and devising a calculus over them. Many geometric concepts that were being used by physicists in a heuristic definition appeared to have a more sound meaning; indeed, the language used was close to the language used by physicists.

In [14], a comparison of the three approaches was done. An important result was that the Rogers–De Witt point of view could be reconciled with the mathematical one if one assumed that, rather than working with a particular, infinite dimensional Grassmann algebra, the geometric structures were defined functorially over the whole category of superalgebras. It seems to us that the functorial behavior of supergeometry is the very key point to assure the consistency of all the calculations done by physicists.

Currently, there seems to be a consensus on what supergeometry is, what is a superspace, a supermanifold, or a supervariety, and how to put geometric structures on them. The mathematical description has become quite clear. Our goal in this paper is not to redefine these concepts, nor to reformulate supergeometry. Our more modest goal is to understand what is the nature of superfields. Often, mathematicians say that superfields are maps of super spacetime to a manifold (it could also be a supermanifold in some cases). We will see in a moment, with a simple example, why there is an issue with this definition. The problem was formulated for example in [13] in the Rogers–De Witt approach, or more recently in [15], so it is not new at all. It was also mentioned in these references that in the sheaf-theoretical approach, one would need to appeal to auxiliary odd variables. We will analyze this statement in the present paper.

The definition of “even” or “bosonic” fields offers no difficulty. They can be functions on spacetime valued in a finite-dimensional manifold, sections of a vector bundle, connections over it, etc. All these objects have a precise geometrical meaning and offer no ambiguity both in their physics usage and in their mathematical formulation.

An “odd” or “fermionic” field is more difficult to interpret. In physics, it is vaguely stated that it is an “odd function on spacetime”, that is, in the simplest case, fermionic fields are functions with values in some super vector space (in its odd part) or superalgebra. However, physicists use properties of odd fields that cannot be reconciled with this naive point of view.

Let us take the simplest example of an odd field on a spacetime ${\mathbb{R}}^n$ (we do not worry here about Lorentz invariance), say $\psi \left(x\right)$, $x\in {\mathbb{R}}^n$. It is sometimes stated that $\psi$ can be seen as a function $f:{\mathbb{R}}^n\to \mathbb{R}$ multiplied by an odd parameter or odd auxiliary variable $\eta$, so $\psi \left(x\right)=f\left(x\right)\eta$. More rigorously, this can be interpreted as a map between affine superspaces over $\mathbb{R}$:

$$\begin{array}{ccc}{A}^{n|1}& \stackrel{\psi }{⟶}& A^{0|1},\end{array}$$

whose dual map on the corresponding superalgebras is given in terms of the global coordinates (see Remark 1 about the chart theorem):

$$\begin{array}{ccc}\wedge \left[\varphi \right]& \stackrel{{\psi }^{♯}}{⟶}& {\mathcal{C}}^{\infty }\left({\mathbb{R}}^n\right)\otimes \wedge \left[\eta \right]\\ \varphi & \stackrel{}{⟶}& {\psi }^{♯}\left(\varphi \right)=f\left(x\right)\eta .\end{array}$$

(1)

The symbol $\wedge \left[\varphi \right]$ stands for the Grassmann or exterior algebra in the variable $\varphi$. In physics, one needs to compute quantities such as:

$$\psi \left(x\right),\dots ,\psi \left(x_1\right)\psi \left(x_2\right)\dots \psi \left(x_m\right),\dots ,\frac{\partial \psi \left(x\right)}{\partial x^{\mu }},\dots ,\frac{{\partial }^{n_1}\psi \left(x\right)}{\partial {\left(x^{{\mu }_1}\right)}^{n_1}}\dots \frac{{\partial }^{n_k}\psi \left(x\right)}{\partial {\left(x^{{\mu }_k}\right)}^{n_k}},\dots$$

(2)

where the superindex labels the coordinates of spacetime and the subindex labels different points of spacetime.

These quantities, with products of any number of derivatives, appear in Lagrangians and other observables, and products of fields at different points of spacetime are the classical limit of correlation functions. They are always assumed to be, generically, different from zero.

It is clear that these calculations could not be reproduced with a simple object such as $\psi \left(x\right)=f\left(x\right)\eta$. All the quantities written above would be identically zero. It seems that physicists and mathematicians, although not disagreeing on the nature of supermanifolds and supervarieties, are not considering the same object when speaking about superfields or just odd fields. Often, this subtlety has been overlooked, but we think that it deserves special attention. There must be an appropriate object, in the context of mathematicians’ approach to supergeometry, that reproduces the properties of a superfield. In the Rogers–de Witt approach, it was proposed that super spacetime is a bundle with basis spacetime and fiber over each point a copy of a Grassmann algebra. These Grassmann algebras are considered to be different at each point of spacetime. This solves the first part of (2), but it is not clear how to compute derivatives with respect to the coordinates of spacetime. Furthermore, one may wonder why these Grassmann algebras leave no trace in the field content of the theory.

Nevertheless, they were on the right track about the need for infinitely many odd variables. As a first objective of this paper, we propose a complete solution to the problem, and Section 2 is devoted to it. In advance, we can say that the important object to consider is the superspace of superfields.

Not always supersymmetric theories are formulated in terms of superfields: for a high number of supersymmetries (that is, many odd coordinates in super spacetime), there appear too many component fields, which would have to be constrained. Since it is not easy to find appropriate constraints (one can even wonder if they exist), physicists devised other methods to implement supersymmetry in field theory. Nevertheless, whenever it is possible, superfield techniques are very powerful and highly desirable. In that case, the primordial objects from which everything else is derived, including the geometry of superspaces at play, are superfields. Presumably, one should be able to recover the original definition of a supermanifold with its sheaf of superalgebras in terms of superfields. In Section 3, we will demonstrate that this is possible with the use of the even rules principle explained in that same section.

In the second part of the paper, Section 4 and Section 5, we deal with a variety of constraints imposed on the space of superfields. These are constraints that have been used in some supergravity inspired cosmological models (the system studied in Section 4.5 appeared for the first time in a preliminary version of this paper). They provide a wonderful example on how one has to be careful when speaking about superfields. We will see that the use of superschemes is necessary to provide an adequate mathematical framework for the constraints and to clarify its behavior under supersymmetry transformations.

Finally, in Section 6, we comment on the interpretation of fermionic observables in the classical and quantum realms.

In the text, we tried to introduce the basic notions of algebraic geometry that are required to understand the generalization to the super setting. Some more basic concepts, as the definition or sheaf, etc., are given in the Appendix A. We tried to give a consistent account of these concepts as a guide for the reader, but this paper is not a suitable place to introduce one’s self to algebraic geometry, for which many good textbooks exist (particularly useful for us has been [16]). For the super setting, introductory references are [5,6,8], and a more detailed monograph is [7]. Physics conventions regarding spinor notation and supersymmetry transformations are given in Appendices Appendix B and Appendix C.

2. Superspaces and Scalar Superfields

2.1. Some Mathematical Definitions

In this section, we will consider the simplest case: an unconstrained, scalar superfield. The ground field will be $\mathbb{R}$.

We need first some mathematic terminology. In Appendix A, we recall the standard definitions of sheaf over a topological space and of morphism of sheaves (Definitions A2 and A4), which are used in what follows. The reader interested in a more complete and deep treatment of the subject in the formalism that we use can consult for example [5,6,7,8].

Definition 1.

A superspace $S=\left(\right|S|,{\mathcal{O}}_S)$ is a topological space $\left|S\right|$ endowed with a sheaf of superalgebras ${\mathcal{O}}_S$ such that the stalk at each point $x\in \left|S\right|$, denoted as ${\mathcal{O}}_{S,x}$, is a local superalgebra (it has a unique maximal ideal). The sheaf ${\mathcal{O}}_S$ is the structural sheaf of the superspace S.

The elements of ${\mathcal{O}}_S\left(U\right)$, for $U{\subset }_{\mathrm{open}}\left|S\right|$, are local sections over U. If the open set is the total space $\left|S\right|$, then the elements of ${\mathcal{O}}_S\left(\right|S\left|\right)$ are called global sections. The superalgebra ${\mathcal{O}}_S\left(\right|S\left|\right)$ is also called the coordinate superalgebra of the superspace S, and it is denoted simply as $\mathcal{O}\left(S\right)$.

Example 1.

The affine superspace $A^{m|n}$ consists of the topological space ${\mathbb{R}}^m$ with the sheaf of superalgebras that, for any open set $U\in {\mathbb{R}}^m$, attaches the superalgebra ${\mathcal{O}}^{m|n}\left(U\right):=C^{\infty }\left(U\right)\otimes \wedge [{\theta }^1,\dots ,{\theta }^n].$

Let $x^1,\dots ,x^m$ be global coordinates on ${\mathbb{R}}^m$. Then, we say that $x^1,\dots ,x^m,{\theta }^1,\dots ,{\theta }^n\in \mathcal{O}\left(A^{m|n}\right)$ are global coordinates on $A^{m|n}$. Here, and as a general rule, Latin letters denote even (commuting) quantities, and Greek letters denote odd (anticommuting) quantities.

It is important to pay attention to the definition of morphisms of superspaces. One can define them as morphisms of the corresponding sheaves, but first, one has to put them over the same basis. This is done by using the pullback sheaf.

Definition 2.

A morphism $f:S\to T$ of superspaces is given by a pair $\left(\right|f|,f^{♯}:)$ where $\left|f\right|:\left|S\right|\to \left|T\right|$ is a continuous function and $f^{♯}:{\mathcal{O}}_T\to {\left|f\right|}_{*}{\mathcal{O}}_S$ is a morphism of sheaves that preserves the maximal ideal of the stalks. The sheaf ${\left|f\right|}_{*}{\mathcal{O}}_S$ is the pullback by $\left|f\right|$ of the sheaf ${\mathcal{O}}_S$, defined, for any open $U\subset T$, as:

$${\left|f\right|}_{*}{\mathcal{O}}_S\left(U\right)={\mathcal{O}}_S{\left(\right|f|}^{-1}\left(U\right)).$$

Remark 1.

It is not difficult to show that a morphism $f:A^{m|n}\to A^{p|q}$ is determined by the images of the global coordinates $(x^1,\dots ,x^p;{\theta }^1,\dots ,{\theta }^q)$ of $A^{p|q}$ under $f^{♯}$:

$$\begin{array}{ccc}{\mathcal{O}}^{p|q}\left({\mathbb{R}}^p\right)& \stackrel{f^{♯}}{⟶}& {\mathcal{O}}^{m|n}\left({\mathbb{R}}^m\right)\\ x^1,\dots ,x^p& \stackrel{}{⟶}& f^1,\dots ,f^p\\ {\theta }^1,\dots ,{\theta }^q& \stackrel{}{⟶}& {\eta }^1,\dots ,{\eta }^q,\end{array}$$

where $f^1,\dots ,f^p$ are even elements of ${\mathcal{O}}^{m|n}\left({\mathbb{R}}^m\right)$ and ${\eta }^1,\dots ,{\eta }^q$ are odd elements of ${\mathcal{O}}^{m|n}\left({\mathbb{R}}^m\right)$. This is the chart theorem (see, for example, Theorem 4.1.11 in Ref. [7]).

We will denote as $\left(\mathrm{sspaces}\right)$ the category of superspaces with their morphisms as defined above.

Definition 3.

A supermanifold of dimension $m|n$ is a superspace that is locally isomorphic to $A^{m|n}$.

Of special importance for us will be the concept of the functor of points associated with supermanifolds (and, later on, to superschemes).

Definition 4.

Functor of points. Let us denote as $\left(\mathrm{smanifolds}\right)$ the category of supermanifolds with morphisms the superspace morphisms such that, in the notation of Definition 2, $\left|f\right|:\left|S\right|\to \left|T\right|$, is a $C^{\infty }$ function. Let S and X be supermanifolds. A morphism $\phi :S\to X$ is called an S-point of X. The set of S-points of X is the set $\mathrm{Hom}(S,X)$.

The association:

$$\begin{array}{ccc}\left(\mathrm{smanifolds}\right)& \stackrel{F_X}{⟶}& \left(\mathrm{sets}\right)\\ S& \stackrel{}{⟶}& \mathrm{Hom}(S,X)\end{array}$$

is a contravariant functor in S called the functor of points of X.

When S is taken as the spectrum of the field $k=\mathbb{R}$, one is left with ordinary points of the topological space underlying the supermanifold. While these points are enough to recover an ordinary manifold as a set, it is not so with a supermanifold, where, in principle, one would need the S-points for any supermanifold S.

If Y is another supermanifold and we have a morphism $f:X\to Y$, we can associate with each S-point of X, $\phi :S\to X$, an S-point of Y by composition, namely $f\circ \phi$. Therefore, one can talk about the map f as we do in the classical case, as sending “points of X” to “points of Y”. It follows from Yoneda’s lemma that it is equivalent to give the actual map f as a map of ringed spaces or the natural transformation between the functors of points.

2.2. Superfields

In order to introduce the discussion on superfields, we will first describe heuristically a toy model.

Scalar superfields on $A^{1|1}$

We consider the simplest model of superspace having both even and odd components, the affine superspace over $\mathbb{R}$, $A^{1|1}=(\mathbb{R},{\mathcal{O}}^{1|1})$ with structural sheaf:

$${\mathcal{O}}^{1|1}\left(U\right)=C^{\infty }\left(U\right)\otimes \wedge \left[\theta \right],U{\subset }_{\mathrm{open}}\mathbb{R}.$$

The algebra of global sections will be denoted as:

$$\mathcal{O}\left(A^{1|1}\right):=C^{\infty }\left(\mathbb{R}\right)\otimes \wedge \left[\theta \right],$$

with x and $\theta$ the global coordinates on $A^{1|1}$. Generically, a global section can be written as:

$$\tilde{\mathsf{\Phi }}=\tilde{A}+\tilde{G}\theta ,$$

(3)

with $\tilde{A},\tilde{G}\in C^{\infty }\left(\mathbb{R}\right)$.

Formally, (3) looks like the superfields that appear in the physics literature, except for the fact that both $\tilde{A}$ and $\tilde{G}$ are ordinary (even) smooth functions on $\mathbb{R}$. One can add elements with different parity since they belong to the same algebra.

Let us now consider $S_n=A^{0|n}$, a superspace whose topological space is just a point, and the structural sheaf is given by the Grassmann algebra over $\mathbb{R}$ in n generators $\wedge [{\xi }^1,\dots ,{\xi }^n]$ (a superpoint). We consider a morphism:

$$\begin{array}{ccc}{S}_n\times M& \stackrel{\varphi }{⟶}& \mathbb{R}.\end{array}$$

(4)

Using the chart Theorem 1, we can express it terms of the algebras and global coordinates as:

$$\begin{array}{ccc}{C}^{\infty }\left(\mathbb{R}\right)& \stackrel{{\varphi }^{♯}}{⟶}& {\mathcal{C}}^{\infty }\left(\mathbb{R}\right)\otimes \wedge \left[\theta \right]\otimes \wedge [{\xi }^1,\dots {\xi }^n]\\ z& \stackrel{}{⟶}& \mathsf{\Phi }:={\varphi }^{♯}\left(z\right)=A+\chi \theta \end{array}$$

where $A\in {\left({\mathcal{C}}^{\infty }\left(\mathbb{R}\right)\otimes \wedge [{\xi }^1,\dots {\xi }^n]\right)}_0$ and $\chi \in {\left({\mathcal{C}}^{\infty }\left(\mathbb{R}\right)\otimes \wedge [{\xi }^1,\dots {\xi }^n]\right)}_1$.

A and $\chi$ are quantities that could represent the component fields of the superfield $\mathsf{\Phi }$, being A a bosonic (even) field and $\chi$ a fermionic (odd) field. We are aware that we have introduced extra odd variables ${\xi }_1,\dots ,{\xi }_n$, and we will argue why they are spurious, that is they have no physical meaning.

For the moment, let us see how this helps with the problem stated in the Introduction. Let us consider $n=2$ and compute some of the quantities in (2) for the odd field $\chi$. We have:

$$A\left(x\right)=A_0\left(x\right)+A_{12}\left(x\right){\xi }^1{\xi }^2,\chi \left(x\right)=G_1\left(x\right){\xi }^1+G_2\left(x\right){\xi }^2,$$

with $A_0,A_{12},G_1,G_2\in {\mathcal{C}}^{\infty }\left(\mathbb{R}\right)$:

$$\begin{array}{c}\chi \left(x\right)\chi \left(x^{\prime }\right)=(G_1\left(x\right)G_2\left(x^{\prime }\right)-G_2\left(x\right)G_1\left(x^{\prime }\right)){\xi }^1{\xi }^2,\hfill \\ \chi \left(x\right)\dot{\chi }\left(x\right)=(G_1\left(x\right){\dot{G}}_2\left(x\right)-G_2\left(x\right){\dot{G}}_1\left(x\right)){\xi }^1{\xi }^2,\hfill \end{array}$$

which, generically, are different from zero. These properties are used in classical field theory and have a (deformed, as $\hslash \ne 0$) counterpart in quantum field theory.

It is clear now that if one wants to have products of the same field in n different points or products of the field and its derivatives that are not identically zero, one just needs to increase the number of odd generators ${\xi }^i$ up to n. In order to achieve full generality, one is then led to consider all the Grassmann algebras. This is reminiscent of the use of infinitely many variables in that DeWitt–Rogers approach, introduced to give a meaning to the calculations done by physicists. It is seems clear from this example that one cannot escape from considering infinitely many odd variables that are non-physical in some way.

The purpose of defining superfields is better served if one considers in (4) arbitrary, finite dimensional supermanifolds S instead of only Grassmann algebras (or rather, the superpoints they define) and then require functoriality in S. This natural approach to superfields, that generalizes and improves our previous definition in terms of Grassmann algebras, is not completely unknown to mathematicians, and it was suggested to us by P. Deligne (We are grateful to him for this valuable input.) (see also [17]). However, in our experience, it is not widespread knowledge. Certainly, it is ignored by the physics community, and we think that a bridge between different ways of thinking is worthwhile. We will dedicate some time to explain it in detail, giving rigorous meaning to the heuristic explanation that we have provided here.

On the other hand, and perhaps paradoxically in view of (2), it is useful to consider first the case where there is no dependence on the coordinates of spacetime: this means that spacetime is just a point, and the structural sheaf of M is given, in the only open set (the point), by a certain finite dimensional superalgebra.

Spacetime is a point

We first need to introduce the concept of the adjoint of a functor.

Definition 5.

Given two categories $\mathcal{C}$ and $\mathcal{D}$ and two functors:

$$\begin{array}{ccc}\hfill \mathcal{C}& \underset{G}{\overset{F}{\rightleftarrows }}& \mathcal{D},\hfill \end{array}$$

we say that the functor G is a right adjoint to the functor F or that F is a left adjoint to the functor G if there is a bijection:

$${\mathrm{Hom}}_D(F\left(X\right),Y)\cong {\mathrm{Hom}}_C(X,G\left(Y\right)),$$

(5)

for all objects X in $\mathcal{C}$ and all objects Y in $\mathcal{D}$. Varying X and Y, we have a family of bijections:

$${\mathrm{Hom}}_D(F(\_),\_)\cong {\mathrm{Hom}}_C(\_,G(\_)).$$

Example 2.

Let us consider the category (sets) of sets. Let A be a fixed set, and consider the functor that takes the Cartesian product by A

$$\begin{array}{ccc}\left(\mathrm{sets}\right)& \stackrel{F}{⟶}& \left(\mathrm{sets}\right)\\ X& \stackrel{}{⟶}& X\times A.\end{array}$$

We have:

$$\mathrm{Hom}(X\times A,Y)\cong \mathrm{Hom}\left(X,\mathrm{Hom}(A,Y)\right),$$

Therefore, the functor:

$$\begin{array}{ccc}\left(\mathrm{sets}\right)& \stackrel{G}{\to }& \left(\mathrm{sets}\right)\\ Y& \stackrel{}{⟶}& \mathrm{Hom}(A,Y)\end{array}$$

is the right adjoint to F.

Example 3.

Let A be a commutative superalgebra, and let $\mathcal{C}$ be the category of A-modules. $\mathcal{C}$ has a tensor product defined in it [5]. For a certain A-module N, we consider the functor:

$$\begin{array}{ccc}\mathcal{C}& \stackrel{F}{⟶}& \mathcal{C}\\ M& \stackrel{}{⟶}& M{\otimes }_{A}N.\end{array}$$

This functor has a right adjoint:

$$\begin{array}{ccc}\mathcal{C}& \stackrel{G}{⟶}& \mathcal{C}\\ P& \stackrel{}{⟶}& \mathcal{H}o{m}_A(N,P),\end{array}$$

where $\mathcal{H}o{m}_A(N,P)$ is the set of all A-linear maps $N\to P$. Notice that we have used a different notation than for the usual module morphisms ${\mathrm{Hom}}_A(N,P)$, which preserve parity. The set $\mathcal{H}o{m}_A(N,P)$ is itself an A-module. Because of this, its elements are called inner morphisms (the set of inner morphisms is inside the category of A-modules $\mathcal{C}$). The even part of $\mathcal{H}o{m}_A(N,P)$ is just the standard module morphisms. The adjunction formula reads:

$${\mathrm{Hom}}_A(M{\otimes }_{A}N,P)=\mathrm{Hom}(M,\mathcal{H}om(N,P)).$$

A very similar construction is the one that we will use for supermanifolds.

The category of supermanifolds admits categorical products. We will not enter into the details here, which can be found, for example, in Ref. [6], page 137. Essentially, if X and Y are two supermanifolds, we have that $|X\times Y|=\left|X\right|\times \left|Y\right|$, and the sheaf can be determined by gluing the local tensor products ${\mathcal{O}}_X\left(U\right)\widehat{\otimes }{\mathcal{O}}_Y\left(V\right)$, where U and V are rectangular open sets admitting global coordinates and $\widehat{\otimes }$ is the completion of the tensor product.

The cases that we will treat here, spacetime being a point, are especially easy in that respect.

Therefore, let M be a superspace with the underlying topological space a point. We define the functor F as:

$$\begin{array}{ccc}\left(\mathrm{smanifolds}\right)& \stackrel{F}{⟶}& \left(\mathrm{smanifolds}\right)\\ S& \stackrel{}{⟶}& S\times M.\end{array}$$

(6)

F just takes the Cartesian product by M.

This functor has a right adjoint since $\left(\mathrm{smanifolds}\right)$ is a closed monoidal category [17]. The adjoint functor associates with every supermanifold Y the supermanifold of inner morphisms from M to Y, denoted as $\mathcal{H}om(M,Y)$:

$$\begin{array}{ccc}\left(\mathrm{smanifolds}\right)& \stackrel{G}{⟶}& \left(\mathrm{smanifolds}\right)\\ Y& \stackrel{}{⟶}& \mathcal{H}om(M,Y),\end{array}$$

which is defined through the formula (5):

$$\mathrm{Hom}(S\times M,Y)\cong \mathrm{Hom}(S,\mathcal{H}om(M,Y\left)\right).$$

The functor $S\to \mathrm{Hom}(S\times M,Y)$ that we were considering heuristically in (4) is in fact the functor of points (Definition 4) of the supermanifold of inner morphisms $\mathcal{H}om(M,Y)$.

It is clearer now why we have chosen M to be of such a restricted type first. For M an arbitrary supermanifold a superspace like $\mathcal{H}om(M,Y)$ would be infinite-dimensional, which is considerably more difficult to describe. In Ref. [17], for example, infinite-dimensional superspaces of maps between supermanifolds were studied in depth, but we will stay with this simpler description. We will come back to this issue later.

It is instructive to compute it for a particularly simple example.

Example 4.

Let us set $M=A^{0|1}$ with coordinate θ and $Y=\mathbb{R}$ with coordinate z. A morphism $S\times A^{0|1}\to \mathbb{R}$ is given in terms of the global coordinates as:

$$\begin{array}{ccc}\mathcal{O}\left(\mathbb{R}\right)& \stackrel{}{⟶}& \mathcal{O}\left(S\right)\otimes {\mathcal{O}}^{0|1}\left(\mathbb{R}\right)\\ z& \stackrel{}{⟶}& f+\eta \theta ,\end{array}f\in \mathcal{O}{\left(S\right)}_0,\eta \in \mathcal{O}{\left(S\right)}_1.$$

(7)

It is then clear that $\mathcal{H}om(M,\mathbb{R})\cong A^{1|1}$, since any morphism $S\to A^{1|1}$ is given in terms of the global coordinates as:

$$\begin{array}{ccc}{\mathcal{O}}^{1|1}\left(\mathbb{R}\right)& \stackrel{}{⟶}& \mathcal{O}\left(S\right)\\ t,\tau & \stackrel{}{⟶}& f,\eta .\end{array}$$

It amounts to the same to give a map $S\times A^{0|1}\to \mathbb{R}$ as to give a map $S\to A^{1|1}$.

More generally, we can take $M=A^{0|q}$, with coordinates ${\theta }_1,{\theta }_2,\dots ,{\theta }_q$ and target manifold $\mathbb{R}$. A morphism $S\times A^{0|q}\to \mathbb{R}$ is given by:

$$\begin{array}{ccc}\mathcal{O}\left(\mathbb{R}\right)& \stackrel{}{⟶}& \mathcal{O}\left(S\right)\otimes {\mathcal{O}}^{0|q}\left(\mathbb{R}\right)\\ z& \stackrel{}{⟶}& \mathsf{\Phi },\end{array}$$

with:

$$\mathsf{\Phi }={\mathsf{\Phi }}_0+{\mathsf{\Phi }}_i{\theta }^i+{\mathsf{\Phi }}_{ij}{\theta }^i{\theta }^j+{\mathsf{\Phi }}_{ijk}{\theta }^i{\theta }^j{\theta }^k+\dots ,$$

(8)

(the sum over repeated indices is understood). We can restrict the sum to $i<j<k<\dots$ due to the antisymmetry of ${\theta }^i{\theta }^j{\theta }^k\dots$. The component fields are:

$${\mathsf{\Phi }}_0,{\mathsf{\Phi }}_{ij},\dots \in \mathcal{O}{\left(S\right)}_0,{\mathsf{\Phi }}_i,{\mathsf{\Phi }}_{ijk},\dots \in \mathcal{O}{\left(S\right)}_1,$$

(9)

and S runs over all supermanifolds. Then, $\mathcal{H}om(A^{0|q},\mathbb{R})\cong A^{2^{q-1}|2^{q-1}}$.

We observe that the coordinates in S play the role of parameters. In order to see this, it is useful to take first an S that is an ordinary manifold. Then, its coordinates are all even. Choosing a value for them, the expression (8) produces a particular inner morphism $M\to \mathbb{R}$, which, in this case, would be a supermanifold morphism. In order to obtain the rest of the inner morphisms, it is necessary to introduce an S with odd coordinates. We then generalize the concept of “parameter” to include also the odd coordinates. We say that (8) is a family of morphisms $M\to \mathbb{R}$ parametrized by S [10].

Let us now denote $\mathcal{H}=\mathcal{H}om(M,\mathbb{R})\cong A^{2^{q-1}|2^{q-1}}$, the superspace of superfields with target $\mathbb{R}$. We can consider the family (8) also over $\mathcal{H}$, that is the family $\mathcal{H}\times A^{0|q}\to \mathbb{R}$:

$$\begin{array}{ccc}\mathcal{O}\left(\mathbb{R}\right)& \stackrel{}{⟶}& \mathcal{O}\left(\mathcal{H}\right)\otimes {\mathcal{O}}^{0|q}\left(\mathbb{R}\right)\end{array}.$$

(10)

In (8), the coefficients ${\mathsf{\Phi }}_{ijk\dots }$ can be taken as coordinates of $\mathcal{H}$. This family is universal in the sense that any family of maps (8) parametrized by S is determined, up to isomorphism, by a map $S\to \mathcal{H}$.

We may observe here a similarity with the naive approach (1), where for each of the odd components ${\mathsf{\Phi }}_{ijk\dots }$ of the superfield, one would introduce an auxiliary odd variable. With this interpretation, in terms of the adjoint functor, we see that the interesting object (the universal one) has also even coordinates, one for each even ${\mathsf{\Phi }}_{ijkl\dots }$.

In summary, to speak about superfields, we have to speak about the superspace of such superfields, and we have clarified how to define it in terms of its functor of points. The undetermined number of “auxiliary variables” in other approaches are just the coordinates in S, and they appear because of the functorial description.

In the toy model considered (spacetime is a point), one can explicitly give the superspace of superfields in terms of sheaves, as in Definition 1. That means that in this case, one is able to describe the superspace of superfields without recurring to the auxiliary variables. This description is clear mathematically, but perhaps more difficult to implement in a supersymmetric field theory, where the functorial formalism is natural. However, its very existence implies that the auxiliary variables that appear in S, because of the functoriality, are spurious and cannot have a physical meaning attached. Therefore, they do not generate new component fields. In this approach, then, it is quite clear that the odd variables in S and the odd variables in super spacetime play a completely different role.

Presumably, this spuriousness would be maintained also when super spacetime is not a point.

What we find remarkable is that the object that physicists have been using all the time, without giving an explanation, was exactly the functor of points of the superspace of inner morphisms. This is just another example of how the language of the functor of points converges with the physicist’s point of view of supergeometry (Another clear example is the definition of supergroups, which in all references is given explicitly in terms of the functor of points without mentioning it.). We hope to contribute with these simple examples to the understanding of the concept of the superfield.

If spacetime is not a point and M is a supermanifold modeled on $A^{p|q}$, the space of inner morphisms becomes infinite-dimensional, and we may have trouble describing it. We can, though, preserve the idea of a family of maps $M\to \mathbb{R}$ parametrized by a supermanifold S. This presents no interpretation problem. For M an affine space $A^{p|q}$, we will have an expansion like (8), where now, all the component fields will acquire a dependence on the even coordinates of M, $(x^1,\dots ,x^p)$. Then, the very definition of superfield in terms of parametrized families takes care of all the properties that physicists use. One can construct Lagrangians, functional actions, and use the calculus of variations to determine the field equations, very much in the same way that they are computed in ordinary field theory.

Since in several cases the constraints that we are going to investigate are algebraic, that is they do not involve spacetime derivatives, the dependence on the even coordinates can be factored out, and everything works as if spacetime would shrink to a point. Even there, the definition of the superfield that we give will make non-trivial solutions of the constraints appear, with a little difficulty added: the superspaces that we will have to consider are not always smooth supermanifolds, nor algebraic varieties, and we will have to allow for singular superschemes.

3. The Even Rules Principle

In this section we explain the even rules principle, a result due to Deligne and Morgan [5], and see its implications for superfields.

We set $k=\mathbb{R},\mathbb{C}$. The algebras and superalgebras that we consider here are k-algebras, unless otherwise stated, and always have a unit.

We will denote by $\left(\mathrm{svector}\mathrm{spaces}\right)$ the category of super vector spaces and by $\left(\mathrm{c}\mathrm{salgebras}\right)$ the category of commutative (or supercommutative) superalgebras with a unit, both over k.

Let $\mathcal{A}$ be a commutative superalgebra and $M_{\mathcal{A}}$ a left $\mathcal{A}$-module, that is $M_{\mathcal{A}}$ is a super vector space with a morphism of super vector spaces:

$$\begin{array}{ccc}\mathcal{A}\otimes M_{\mathcal{A}}& \stackrel{}{⟶}& M_{\mathcal{A}}\\ a\otimes m& \stackrel{}{⟶}& a·m,\end{array}$$

satisfying:

$$a·(b·m)=\left(ab\right)·m,a,b\in \mathcal{A},m\in M_{\mathcal{A}}.$$

Left $\mathcal{A}$-modules for commutative superalgebras be also right $\mathcal{A}$-modules by setting:

$$\begin{array}{ccc}{M}_{\mathcal{A}}\otimes \mathcal{A}& \stackrel{}{⟶}& M_{\mathcal{A}}\\ m\otimes a& \stackrel{}{⟶}& m·a:={(-1)}^{p\left(m\right)p\left(a\right)}a·m,\end{array}$$

where $p\left(m\right)$ and $p\left(a\right)$ are the parities of m and a, respectively. This satisfies:

$$(m·b)·a=m·(b·a),a,b\in \mathcal{A},m\in M_{\mathcal{A}},$$

We will just call them modules.

Let V be an object in $\left(\mathrm{svector}\mathrm{spaces}\right)$ and $\mathcal{B}$ and object in $\left(\mathrm{c}\mathrm{salgebras}\right)$. We denote as $V\left(\mathcal{B}\right):=\mathcal{B}\otimes V$ the extension of the scalars of V by $\mathcal{B}$ (see Definition A1). Let $h:\mathcal{B}\to {\mathcal{B}}^{\prime }$ be a morphism of commutative superalgebras. Then, $V\left({\mathcal{B}}^{\prime }\right)$ is also a $\mathcal{B}$-module by further extending the scalars to ${\mathcal{B}}^{\prime }$. There is a morphism of $\mathcal{B}$-modules:

$$\begin{array}{ccc}V\left(\mathcal{B}\right)& \stackrel{V\left(h\right)}{⟶}& V\left({\mathcal{B}}^{\prime }\right)\\ b\otimes v& \stackrel{}{⟶}& h\left(b\right)\otimes v.\end{array}$$

This is well defined since h is a superalgebra morphism.

We can as well take the even part of the module. If $V=V_0+V_1$ and $\mathcal{B}={\mathcal{B}}_0+{\mathcal{B}}_1$ are the splitting in even and odd parts, we denote $V{\left(\mathcal{B}\right)}_0$ the even part of $V\left(\mathcal{B}\right)$,

$$V{\left(\mathcal{B}\right)}_0={\mathcal{B}}_0\otimes V_0\oplus {\mathcal{B}}_1\otimes V_1,$$

which is a ${\mathcal{B}}_0$-module. Given a morphism of superalgebras $h:\mathcal{B}\to {\mathcal{B}}^{\prime }$, then $V{\left(h\right)}_0$ is a morphism of ${\mathcal{B}}_0$-modules:

$$\begin{array}{ccc}V{\left(\mathcal{B}\right)}_0& \stackrel{V{\left(h\right)}_0}{⟶}& V{\left({\mathcal{B}}^{\prime }\right)}_0\\ b\otimes v& \stackrel{}{⟶}& h\left(b\right)\otimes v.\end{array}$$

Remark 2.

It will be useful here to recall the definition of superalgebra in terms of commutative diagrams.

Let V be a super vector space. An associative superalgebra structure on V is given by a linear map, the product:

$$\begin{array}{ccc}V\otimes V& \stackrel{\pi }{⟶}& V\end{array}$$

such that

$$p(\left(\pi (u\otimes v)\right)=p\left(u\right)+p\left(v\right)$$

and satisfying the associativity property, that is that the diagram: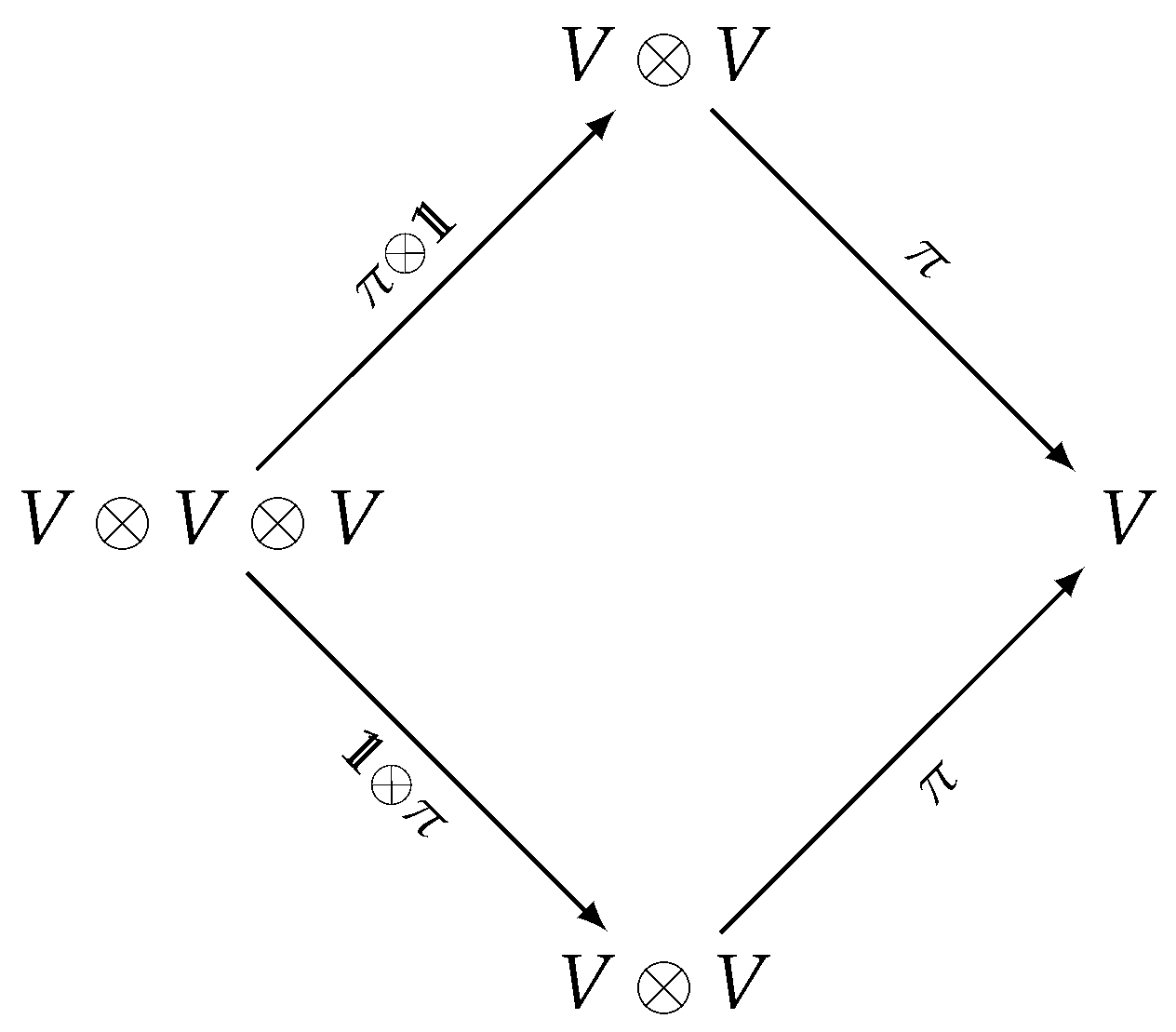is commutative. On the other hand, we say that the superalgebra is commutative if, given the flip map,

$$\begin{array}{ccc}V\otimes V& \stackrel{c_{V,V}}{⟶}& V\otimes V\\ v\otimes w& \stackrel{}{⟶}& {(-1)}^{p_v{p}_w}w\otimes v,\end{array}$$

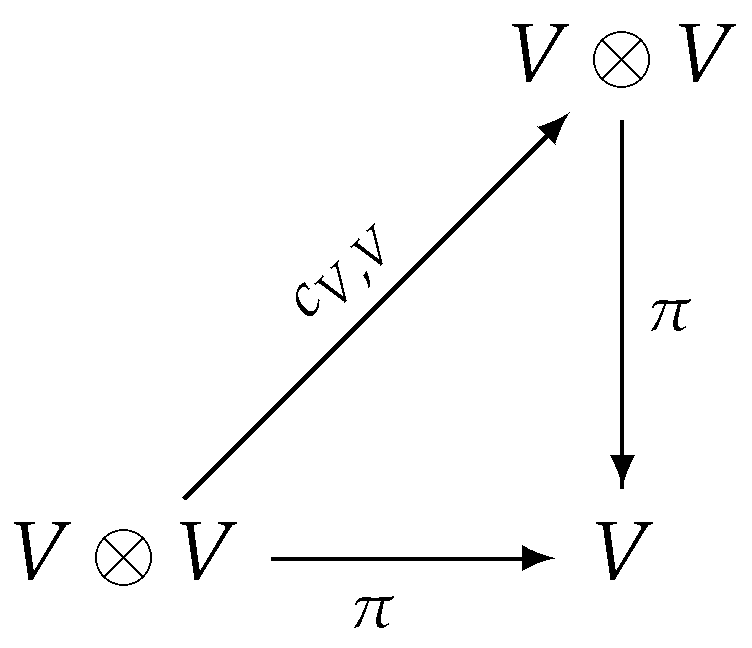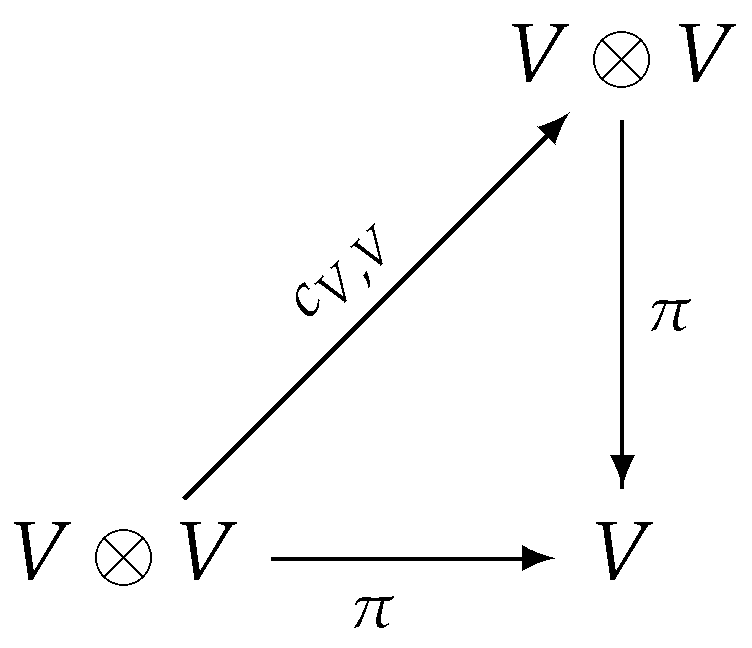the diagram:commutes.

If V has a k-superalgebra structure, then $V\left(\mathcal{B}\right)$ has a $\mathcal{B}$-algebra structure naturally: Let $\pi :V\otimes V\to V$ be the product on V, then we have a product (We use here the direct product and not the tensor product because the bilinearity is in ${\mathcal{B}}_0$ and not only in k.):

$$\begin{array}{ccc}V\left(\mathcal{B}\right)\times V\left(\mathcal{B}\right)& \stackrel{{\pi }_{\mathcal{B}}}{⟶}& V\left(\mathcal{B}\right)\end{array}$$

(11)

given simply by

$${\pi }_{\mathcal{B}}\left(b_1\otimes v_1,b_2\otimes v_2\right)={(-1)}^{p\left(v_1\right)p\left(b_2\right)}{b}_1{b}_2\pi (v_1\otimes v_2).$$

It is straightforward to check that the associativity and commutativity properties are satisfied.

Definition 6.

Let V and W be two superspaces. We say that a family of morphisms:

$$\left\{f_{\mathcal{B}}:V\left(\mathcal{B}\right)\to W\left(\mathcal{B}\right),\mathcal{B}\in \left(\mathrm{c}\mathrm{salgebras}\right)\right\}$$

is functorial in $\mathcal{B}$ if given a superalgebra morphism:

$$\begin{array}{ccc}\mathcal{B}& \stackrel{h}{⟶}& {\mathcal{B}}^{\prime }\end{array}$$

the diagram:

$$\begin{array}{ccc}V\left(\mathcal{B}\right)& \stackrel{f_{\mathcal{B}}}{⟶}& W\left(\mathcal{B}\right)\\ V\left(h\right)\downarrow \phantom{V\left(h\right)}& & \phantom{W\left(h\right)}\downarrow W\left(h\right)\\ V\left({\mathcal{B}}^{\prime }\right)& \stackrel{f_{{\mathcal{B}}^{\prime }}}{⟶}& W\left({\mathcal{B}}^{\prime }\right).\end{array}$$

commutes.

In particular, if V has a superalgebra structure, it is not difficult to see that the family $\{{\pi }_{\mathcal{B}}:V\left(\mathcal{B}\right)\otimes V\left(\mathcal{B}\right)\to V\left(\mathcal{B}\right)\}$ is functorial in $\mathcal{B}$. The same is true for the families of maps appearing in the associativity and commutativity diagrams for the algebras $V\left(\mathcal{B}\right)$.

The following theorem of Deligne and Morgan ([5], page 56) will allow us to recover the defining sheaf in terms of scalar superfields.

Theorem 1.

Even rules principle. Let ${\left\{V_i\right\}}_{i\in I}$, $I=1,\dots ,n$ be a family of super vector spaces, V another super vector space, and $\mathcal{B}={\mathcal{B}}_0\oplus {\mathcal{B}}_1$ a commutative superalgebra. As before, we denote $V_i{\left(\mathcal{B}\right)}_0={(\mathcal{B}\otimes V_i)}_0$ and $V{\left(\mathcal{B}\right)}_0={(\mathcal{B}\otimes V)}_0$.

Any family of ${\mathcal{B}}_0$-multilinear maps:

$$\begin{array}{ccc}{V}_1{\left(\mathcal{B}\right)}_0\times \dots \times V_n{\left(\mathcal{B}\right)}_0& \stackrel{f_{\mathcal{B}}}{⟶}& V{\left(\mathcal{B}\right)}_0\end{array}$$

which is functorial in $\mathcal{B}$ comes from a unique morphism:

$$\begin{array}{ccc}{V}_1\otimes \dots \otimes V_n& \stackrel{f}{⟶}& V\end{array}$$

as in (11), that is

$$f_{\mathcal{B}}(b_1\otimes v_1,b_2\otimes v_2,\dots ,b_n\otimes v_n)={(-1)}^p{b}_1\dots b_{n}f(v_1\otimes \dots \otimes v_n),$$

where p is the number of pairs $(i,j)$ with $i<j$ and $v_i,v_j$ odd.

Proof. 

We will not prove the theorem here (see [5]), but it is instructive to see how the map f can be recovered from the family of maps $f_{\mathcal{B}}$. Let us consider the simple case of a family of maps:

$$\begin{array}{ccc}V{\left(\mathcal{B}\right)}_0\otimes V{\left(\mathcal{B}\right)}_0& \stackrel{f_{\mathcal{B}}}{⟶}& V{\left(\mathcal{B}\right)}_0,\end{array}$$

then we have three possible cases:

• $v_1,v_2$ even. Then, we may take $b_1=b_2=1$ (in an arbitrary algebra $\mathcal{B}$) and:

  $$f(v_1\otimes v_2):=f_{\mathcal{B}}(v_1\otimes v_2).$$
• $v_1$ even, $v_2$ odd. Then, we take for example $\mathcal{B}=\mathsf{\Lambda }\left[\xi \right]$, $b_1=1$, and $b_2=\xi$. The equality:

  $$f_{\mathcal{B}}(v_1\otimes \xi v_2)=\xi f(v_1\otimes v_2)$$

  determines $f(v_1\otimes v_2)$.
• $v_1$ odd, $v_2$ odd. It is enough to consider $\mathcal{B}=\mathsf{\Lambda }[{\xi }^1,{\xi }^2]$, and the equality:

  $$f_{\mathcal{B}}({\xi }^1{v}_1\otimes {\xi }^2{v}_2)=-{\xi }^1{\xi }^{2}f(v_1\otimes v_2)$$

  determines $f(v_1\otimes v_2)$.

 □

One way to give a structure of superalgebra to V is to give a ${\mathcal{B}}_0$-algebra structure on $V{\left(\mathcal{B}\right)}_0$, which varies functorially with $\mathcal{B}$. From the commutative diagrams in Remark 2, it is clear that the superalgebra will be associative or commutative if the algebra structures on $V{\left(\mathcal{B}\right)}_0$ are so.

Example 5.

Toy model. Let us consider the vector space over $k=\mathbb{R},\mathbb{C}$ with basis one even vector e and one odd vector θ.

$$V^{1|1}={\mathrm{span}}_k\{e,\theta \}.$$

Let $\mathcal{B}$ be a commutative superalgebra. An element of $V_0^{1|1}\left(\mathcal{B}\right)$ will be of the form:

$${\mathsf{\Phi }}_{\mathcal{B}}=b_0\otimes e+b_1\otimes \theta ,b_0\in {\mathcal{B}}_0,b_1\in {\mathcal{B}}_1.$$

Let ${\mathsf{\Psi }}_{\mathcal{B}}=a_0\otimes e+b{a}_1\otimes \theta$ be another element of $V^{1|1}{\left(\mathcal{B}\right)}_0$. We can define a product on $V^{1|1}{\left(\mathcal{B}\right)}_0$ as:

$${\mathsf{\Psi }}_{\mathcal{B}}{•}_{\mathcal{B}}{\mathsf{\Phi }}_{\mathcal{B}}=b_0{a}_0\otimes e+(b_0{a}_1+a_1{b}_0)\otimes \theta .$$

It is immediate to check the functorial property (Definition 6) of the whole family of products $\left\{{•}_{\mathcal{B}}\right\}$: for a morphism $h:\mathcal{B}\to {\mathcal{B}}^{\prime }$, we have the map:

$$\begin{array}{ccc}{V}^{1|1}{\left(\mathcal{B}\right)}_0& \stackrel{V^{1|1}{\left(h\right)}_0}{\to }& V^{1|1}{\left({\mathcal{B}}^{\prime }\right)}_0\end{array},$$

that, for short and without risk of confusion, we will call simply h:

$$\begin{array}{c}h\left({\mathsf{\Psi }}_{\mathcal{B}}{•}_{\mathcal{B}}{\mathsf{\Phi }}_{\mathcal{B}}\right)=h\left(b_0{a}_0\right)\otimes e+h(b_0{a}_1+a_1{b}_0)\otimes \theta =\hfill \\ h\left(b_0\right)h\left(a_0\right)\otimes e+\left(h\left(b_0\right)h\left(a_1\right)+h\left(a_1\right)h\left(b_0\right)\right)\otimes \theta =\hfill \\ h\left({\mathsf{\Phi }}_{\mathcal{B}}\right){•}_{{\mathcal{B}}^{\prime }}h\left({\mathsf{\Psi }}_{\mathcal{B}}\right).\hfill \end{array}$$

One can also check the functoriality for the associativity and commutativity diagrams (Remark 2). The algebra structure defined in the superspace $V^{1|1}$ converts it into the Grassmann algebra in one variable $\wedge \left[\theta \right]$.

Remark 3

([5]). The same result can be obtained if, instead of considering arbitrary commutative superalgebras, one restricts to the subcategory of Grassmann algebras $\mathcal{B}=\wedge [{\xi }^1,\dots ,{\xi }^n]$, for short ${\wedge }^n$, for n arbitrarily large, but finite. For V a superalgebra (as in (5)), the object ${({\wedge }^n\otimes V)}_0$ (without specifying n), appears in the literature as the Grassmann envelope of the superalgebra V.

Let M be, as above, super spacetime. We consider two families of maps parametrized by S, $S\times M\to \mathbb{C}$, which in terms of the algebras read:

$$\begin{array}{ccc}\mathcal{O}\left(\mathbb{C}\right)& \stackrel{}{⟶}& \mathcal{O}\left(S\right)\otimes \mathcal{O}\left(M\right)\\ z& \stackrel{}{⟶}& \mathsf{\Phi }\end{array}\begin{array}{ccc}\mathcal{O}\left(\mathbb{C}\right)& \stackrel{}{⟶}& \mathcal{O}\left(S\right)\otimes \mathcal{O}\left(M\right)\\ z& \stackrel{}{⟶}& \mathsf{\Psi }\end{array}$$

where $\mathsf{\Phi }$ and $\mathsf{\Psi }$ are even sections of $\mathcal{O}\left(S\right)\otimes \mathcal{O}\left(M\right)$. The section given by the product $\mathsf{\Phi }·\mathsf{\Psi }$ defines another such family. By identifying $\mathcal{O}\left(S\right)=\mathcal{B}$ and $\mathcal{O}\left(M\right)=V$ and applying Theorem 1, we see that it is the same giving the product in V as giving the functorial family of maps:

$$\begin{array}{ccc}V{\left(\mathcal{B}\right)}_0\otimes V{\left(\mathcal{B}\right)}_0& \stackrel{f_{\mathcal{B}}}{⟶}& V{\left(\mathcal{B}\right)}_0\\ \mathsf{\Phi }\otimes \mathsf{\Psi }& \stackrel{}{⟶}& \mathsf{\Phi }·\mathsf{\Psi }.\end{array}$$

In physics, it is the second option that is chosen, and it is called the product of superfields, although the nature of $\mathcal{B}$ and the functoriality of $f_{\mathcal{B}}$ are not clarified.

4. How to Deal with Algebraic Constraints

The examples that we would like to analyze are the result of some algebraic constraints imposed on a certain set of $N=1$, $D=4$ chiral superfields. In physics, one needs to consider the complexification of the affine space $A^{4|2}$ over the reals. This is because the action of the Lorentz group: the two odd components form a Weyl spinor, and this representation is complex. In order to obtain a real form, one needs to consider $A^{4|4}$ over the complex numbers, and then, one can impose a reality condition compatible with the action of the Lorentz group. Two of the odd variables transform as a chiral (Weyl) spinor and the other two as an antichiral spinor (See for example Ref. [18]). Therefore, in what follows, we will consider complex, affine superalgebras. This does not mean that we are considering algebras of holomorphic functions: we will consider all the real analytic ones, so we see ${\mathbb{C}}^4\cong {\mathbb{R}}^8$.

The super spacetime under consideration is $M=A^{4|2}$, the chiral superspace, with global sections:

$$\mathcal{O}\left(A^{4|2}\right)=C^{\infty }\left({\mathbb{C}}^4\right)\otimes \wedge [{\theta }^1,{\theta }^2].$$

A chiral superfield associates with each supermanifold S a family of morphisms parametrized by S:

$$\begin{array}{ccc}S\times M& \stackrel{}{⟶}& \mathbb{C},\end{array}$$

given in terms of an even section of $\mathcal{O}\left(S\right)\otimes \mathcal{O}\left(M\right)$ as in (8):

$$\mathsf{\Phi }=A+{\theta }^{\alpha }{\chi }_{\alpha }+{\theta }^{\alpha }{\theta }_{\alpha }F,\alpha =1,2.$$

(12)

The index notation is the usual in physics, and it is explained in Appendix B; in particular, the sum over repeated indices is understood. The component fields $A,F$, and ${\chi }_{\alpha }$ depend on the even coordinates of the chiral superspace, although we do not write it explicitly.

We want now to study some algebraic constraints on chiral superfields. Spacetime dependence is untouched by the constraints that we will consider, so one can effectively consider that spacetime is reduced to a point: the full picture is recovered by tensoring with $C^{\infty }\left({\mathbb{C}}^4\right)$ when needed.

If spacetime is a point, then the superspace of superfields, that is the space of inner morphisms $M\to \mathbb{C}$ can be identified with $\mathcal{H}=A^{2|2}$, being the even global coordinates A and F in (12) and ${\chi }_{\alpha }$, $\alpha =1,2$ the odd ones. The constraints will give relations among these coordinates. The restricted space is not necessarily an affine superspace or a supermanifold. In the cases we will deal with, it is in fact an affine superscheme. In the next subsection, we try to give a brief summary on the principal results on schemes and superschemes. In the non-super case, a complete treatment can be found in any textbook on algebraic geometry (see for example the first chapter of [16]). For the super case, there is a thorough treatment in [7].

4.1. Schemes and Superschemes

An affine algebraic variety $Y\subset {\mathbb{C}}^n$ is the zero locus of some polynomials. One defines the algebra F of polynomials over Y as the restriction of the polynomials $\mathbb{C}[x^1,\dots ,x^n]$ to Y. In fact, F can be viewed as the quotient:

$$F=\mathbb{C}[x^1,\dots ,x^n]/I$$

where I is the ideal of functions that vanish on Y, so two polynomials are considered equivalent if they differ by a polynomial that is zero on Y. Each point of Y has associated a maximal ideal of F. In general, an ideal of F corresponds to an algebraic subset (zero locus in Y of sets of polynomials in F). The Zariski topology on Y is such that the closed subsets of Y are precisely the algebraic subsets. Then, the open subsets are the complements of closed subsets.

We are going to define now the structural sheaf of Y, ${\mathcal{O}}_Y$ (see Appendix A2). We have to associate with each open set in Y a ring. Let $U\subset Y$ be an open subset of Y. We define:

$${\mathcal{O}}_Y\left(U\right)=\left\{\frac{f}{g}|f,g\in F,g\left(x\right)\ne 0\forall x\in U\right\}.$$

This procedure is called the localization of F at U. One can check that it satisfies all the conditions to be a sheaf. The stalk at a point $x\in U$ with maximal ideal $\mathfrak{p}$ is just the algebra:

$$F_{\mathfrak{p}}:=\left\{\frac{f}{g}|f\in F,g\in F-\mathfrak{p}\right\}$$

and it is a local algebra, that is it has a unique maximal ideal. One recovers the algebra A as the algebra of global sections ${\mathcal{O}}_Y\left(Y\right)=F$. We will use interchangeably the notation ${\mathcal{O}}_Y$ and ${\mathcal{O}}_F$.

It is convenient to add some points to the topological space Y and consider also all the irreducible subvarieties of Y. This is equivalent to consider, not only the maximal ideals, but all the prime ideals (The reason is that the preimage under an algebra morphisms of a prime ideal is a prime ideal, while this is not true for maximal ideals.). The topological space that results is called the spectrum of F, and it is denoted as $\mathrm{Spec}\left(F\right)$. The Zariski topology is still well defined. The pair $\mathrm{Spec}\left(F\right)$ together with its structural sheaf will be denoted as:

$$\underline{\mathrm{Spec}}\left(F\right):=(\mathrm{Spec}\left(F\right),{\mathcal{O}}_F)=\left(\right|Y|,{\mathcal{O}}_Y),$$

where $\left|Y\right|$ denotes Y understood as a topological space.

Given a field k, an affine algebra is a finitely generated, Noetherian, associative, commutative, unital k-algebra without nilpotent elements. The procedure that we have described above establishes an equivalence of categories between affine algebras and affine varieties.

One can apply the same procedure to an arbitrary commutative algebra F. It can, for example, contain nilpotents: $\mathrm{Spec}\left(F\right)$ and ${\mathcal{O}}_F$ still make sense. The nilpotent elements of a commutative algebra form an ideal N, so one can define the reduced algebra $F_{\mathrm{red}}:=F/N$. Since N sits inside every prime ideal, we have that $\mathrm{Spec}\left(F_{\mathrm{red}}\right)\cong \mathrm{Spec}\left(F\right)$ as topological spaces. Nevertheless, the sheaves ${\mathcal{O}}_F$ and ${\mathcal{O}}_{F_{\mathrm{red}}}$ will be, in general, different. In particular, $F_{\mathrm{red}}$ may be an affine algebra even if F is not.

The following example will be used later on.

Example 6.

Ring of dual numbers. Let us consider the algebra of polynomials in one even variable, $\mathbb{C}\left[ϵ\right]$, and let $\left(ϵ\right)$ be the ideal generated by the element ${ϵ}^2$. The quotient $F=\mathbb{C}\left[ϵ\right]/\left({ϵ}^2\right)$ is not an affine algebra, since it contains a nilpotent, namely the element ϵ. A generic element of F will be of the form:

$$f_0+ϵf_1,f_0,f_1\in \mathbb{C}.$$

The solution of the polynomial equation ${ϵ}^2=0$ over $\mathbb{C}$ is just $ϵ=0$, and in fact, $F_{\mathrm{red}}\cong \mathbb{C}$. Nevertheless, the algebra F keeps track of the double multiplicity of the solution, so it has more information. The only prime ideal is $\mathfrak{p}=\left(ϵ\right)$, and the stalk of the sheaf at such point is:

$$F_{\mathfrak{p}}=\left\{\frac{f_0+ϵf_1}{g_0+ϵg_1}|f_i,g_i\in \mathbb{C},g_0\ne 0\right\}.$$

Working with the reduced algebra, there is only one point in the spectrum, $\left(0\right)$, and the stalk at that point is simply $\mathbb{C}$.

We are led to the following definition:

Definition 7.

An affine scheme X is a topological space $\left|X\right|$ together with a sheaf of algebras ${\mathcal{O}}_X$, which is isomorphic to $\underline{\mathrm{Spec}}\left(F\right)$ for some algebra F.

It may seem odd that the category of affine schemes relates to non-affine algebras, but the adjective “affine” on the noun “scheme” is used to distinguish it from a projective scheme, a generalization that we will not need in this paper.

Since superalgebras inevitably contain nilpotents, the concept of a scheme seems suitable for extension to superalgebras. A superalgebra $\mathcal{A}={\mathcal{A}}_0+{\mathcal{A}}_1$ is an affine superalgebra if its even part ${\mathcal{A}}_0$ is finitely generated as an algebra, its odd part ${\mathcal{A}}_1$ is finitely generated as an ${\mathcal{A}}_0$-module, and taking the quotient by the ideal generated by the odd nilpotents $\mathcal{J}$, $\tilde{\mathcal{A}}:=\mathcal{A}/\mathcal{J}$, one obtains an affine algebra, so $\mathcal{A}$ contains no further nilpotents. For any superalgebra, we will denote as ${\mathcal{A}}_{\mathrm{red}}$ the superalgebra modulo the ideal generated by all the nilpotents. One can also construct the topological space $\mathrm{Spec}\left(\mathcal{A}\right)=\mathrm{Spec}(\mathcal{A}/\mathcal{J})$ and equip it with a sheaf of superalgebras obtained by localization. We then have:

Definition 8.

An affine superscheme is a superspace $S=\left(\right|S|,{\mathcal{O}}_S)$, which is isomorphic to $\underline{\mathrm{Spec}}\left(\mathcal{A}\right)$ for a superalgebra $\mathcal{A}$ not necessarily affine.

Given an affine superscheme S with superalgebra $\mathcal{A}$, there is always an affine scheme $S_{\mathrm{red}}$ associated with the reduced algebra ${\mathcal{A}}_{\mathrm{red}}$. It is the reduced scheme of the superscheme, a concept that is similar to the concept of a reduced manifold of a supermanifold or a reduced algebraic variety of an algebraic supervariety.

4.2. Constraint ${\mathsf{\Phi }}^2=0$

We want to consider the chiral superfield (12) satisfying ${\mathsf{\Phi }}^2=0$. This means:

$${\mathsf{\Phi }}^2=A^2+2A{\theta }^{\alpha }{\chi }_{\alpha }+(2AF-\frac{1}{2}{\chi }^{\alpha }{\chi }_{\alpha }){\theta }^{\beta }{\theta }_{\beta }=0,$$

where $A,F\in {\left(\mathcal{O}\left(S\right)\otimes \mathcal{O}\left(A^{4|2}\right)\right)}_0$ and ${\chi }_{\alpha }\in {\left(\mathcal{O}\left(S\right)\otimes \mathcal{O}\left(A^{4|2}\right)\right)}_1$.

The solutions to the constraint are indeed the superspace of inner morphisms from M to the spectrum of the ring of dual numbers (6):

$$\begin{array}{ccc}M& \stackrel{}{⟶}& \underline{\mathrm{Spec}}\left(\mathbb{C}\left[ϵ\right]/\left({ϵ}^2\right)\right).\end{array}$$

This spectrum is not a manifold, but a scheme, so we have to allow for families of morphisms parametrized by superschemes S, instead of simply supermanifolds or algebraic supervarieties, in order to describe the space of inner morphisms:

$$\begin{array}{ccc}S\times M& \stackrel{}{⟶}& \underline{\mathrm{Spec}}\left(\mathbb{C}\left[ϵ\right]/\left({ϵ}^2\right)\right).\end{array}$$

We obtain then a system of equations restricting the coordinates of $\mathcal{H}=A^{2|2}$:

$$A^2=0,A{\chi }_{\alpha }=0,4AF-{\chi }^{\alpha }{\chi }_{\alpha }=0.$$

(13)

They define an affine superscheme that we denote as $\mathcal{L}$. The superalgebra of global sections, $\mathcal{O}\left(\mathcal{L}\right)$, is:

$$\mathcal{O}\left(\mathcal{L}\right)=C^{\infty }\left({\mathbb{C}}^2\right)[{\chi }_1,{\chi }_2]/\left(A^2,A{\chi }_{\alpha },2AF-{\chi }_1{\chi }_2\right).$$

In order to study this scheme, we start considering the algebra obtained by quotienting $\mathcal{O}\left(\mathcal{L}\right)$ by the odd nilpotents, that is putting the odd coordinates to zero. The affine scheme that we obtain $\tilde{\mathcal{L}}$ satisfies the quadratic relations:

$$A^2=0,AF=0,$$

(14)

so the algebra defining the affine scheme is:

$$\mathcal{O}\left(\tilde{\mathcal{L}}\right)=C^{\infty }\left({\mathbb{C}}^2\right)/\left(A^2,AF\right),(A,F)\in {\mathbb{C}}^2.$$

Since A is an even nilpotent, the scheme is not a supermanifold (nor an algebraic supervariety).

There is, however, an open set where the scheme is isomorphic to an affine space. It corresponds to the points where F is invertible, that is to the prime ideals of $\mathcal{O}\left(\tilde{\mathcal{L}}\right)$ that do not contain F. We will denote the localization of $\mathcal{O}\left(\tilde{\mathcal{L}}\right)$ at these points as $\mathcal{O}{\left(\tilde{\mathcal{L}}\right)}_{F\ne 0}$. This essentially means that we can set $A=0$ from the second equation in (14); then, the first one is satisfied identically:

$$\mathcal{O}{\left(\tilde{\mathcal{L}}\right)}_{F\ne 0}\simeq C^{\infty }\left({\mathbb{C}}^{\times }\right).$$

(15)

This is the regular or smooth part of the scheme, represented by the object ${\mathbb{C}}^{\times }=\mathbb{C}-\left\{0\right\}$.

Going back to the superscheme, we can do a change of variables for the coordinates:

$$A^{\prime }=4AF-{\chi }^{\alpha }{\chi }_{\alpha },F^{\prime }=F{\chi }_{\alpha }^{\prime }={\chi }_{\alpha },$$

(16)

with Jacobian:

$$J=\left(\begin{array}{cccc}4F& 4A& -2{\chi }_2& +2{\chi }_1\\ 0& 1& 0& 0\\ 0& 0& 1& 0\\ 0& 0& 0& 1\end{array}\right),$$

which is non-singular for $F\ne 0$. The inverse transformation is:

$$A=\frac{A^{\prime }+{\chi }^{\alpha }{\chi }_{\alpha }}{4F},F=F^{\prime },{\chi }_{\alpha }={\chi }_{\alpha }^{\prime },$$

and the smooth part of the superscheme is just given by the ring:

$$C^{\infty }\left({\mathbb{C}}^2\right){[{\chi }_1,{\chi }_2]}_{F\ne 0}/\left(A^{\prime }\right)\simeq C^{\infty }\left({\mathbb{C}}^{\times }\right)[{\chi }_1,{\chi }_2].$$

This model appeared in its non-linear form in [19], so it is called the Volkov–Akulov multiplet. In terms of superfields, it appeared in [20,21,22].

We want to stress that imposing the constraint ${\tilde{\mathsf{\Phi }}}^2=0$ for:

$$\tilde{\mathsf{\Phi }}=\tilde{A}+{\theta }^{\alpha }{\tilde{\psi }}_{\alpha }+{\theta }^{\alpha }{\theta }_{\alpha }\tilde{F}$$

a section of the structural sheaf as in (3), with $\tilde{A}$, $\tilde{F}$, and ${\tilde{\chi }}_{\alpha }$ standard (even) functions on spacetime, will give only the trivial solution:

$$\tilde{A}=\tilde{F}={\tilde{\chi }}_{\alpha }=0.$$

The anticommutativity of ${\chi }^{\alpha }$ is crucial to obtain a non-trivial solution.

The lesson to learn is that super spacetime is still the chiral superspace, but we have chosen a distinguished subalgebra of the algebra of scalar superfields (seen as a family of maps parametrized by an arbitrary supermanifold. This is reflected in the fact that the superspace of superfields is now a subspace of the inner morphisms, $\mathcal{H}=A^{2|2}$, and it happens to be a genuine scheme.

In the examples that follow, we will encounter schemes that do not have a regular part: these are the ones that “break” supersymmetry.

4.3. General Constraint $f\left(\mathsf{\Phi }\right)=0$

Let f be a polynomial in one variable. We consider now the more general constraint:

$$f\left(\mathsf{\Phi }\right)=0.$$

Because of the nilpotency of ${\theta }^{\alpha }$, this reduces to:

$$f\left(\mathsf{\Phi }\right)=f\left(A\right)+{\theta }^{\alpha }{\chi }_{\alpha }{f}^{\prime }\left(A\right)+{\theta }^{\alpha }{\theta }_{\alpha }\left(f^{\prime }\left(A\right)F-\frac{1}{4}{f}^{\prime \prime }\left(A\right){\chi }^{\alpha }{\chi }_{\alpha }\right).$$

For example, let us take $f\left(\mathsf{\Phi }\right)={\mathsf{\Phi }}^n$. Then, the constraints that define the affine superscheme $\mathcal{L}$ are:

$$A^n=0,A^{n-1}{\chi }_{\alpha }=0,A^{n-2}\left(4AF-(n-1){\chi }^{\alpha }{\chi }_{\alpha }\right)=0.$$

(17)

The reduced affine scheme in this case is defined by the ring:

$$\mathcal{O}\left(\tilde{\mathcal{L}}\right)=C^{\infty }\left({\mathbb{C}}^2\right)/\left(A^n,A^{n-1}F\right),(A,F)\in {\mathbb{C}}^2.$$

We can still localize at F invertible, and we get:

$$\mathcal{O}{\left(\tilde{\mathcal{L}}\right)}_{F\ne 0}=C^{\infty }{\left({\mathbb{C}}^2\right)}_{F\ne 0}/\left(A^{n-1}\right)\simeq C^{\infty }(\mathbb{C}\times {\mathbb{C}}^{\times })/\left(A^{n-1}\right).$$

Differently from (15), this ring still has the nilpotent A everywhere, so it does not have smooth points.

Remark 4.

Following what some authors in physics do, one could hand-impose an extra constraint:

$$A=a{\chi }^{\alpha }{\chi }_{\alpha }$$

(18)

(physicists would argue “what else can it be?”). The coefficient a can depend on F, but not on ${\chi }^{\alpha }$, because then A would be identically zero.

For $n=2$, this followed from the constraints (13) only assuming that F was invertible. Then, a was determined to be $a=1/4F$. The same trick would not work for $n\ge 3$, so (18) is an extra constraint, not coming from (17), which nevertheless allows solving trivially (17). Moreover, a is arbitrary, so there are indeed solutions to ${\mathsf{\Phi }}^3=0$ that do not solve ${\mathsf{\Phi }}^2=0$. For $n=3,4,5,\dots$, the sets of solutions that we obtain in this way are identical.

The constraint (18), when putting the odd variables to zero, gives $A=0$, which leaves us with the affine algebra $C^{\infty }\left(\mathbb{C}\right)$, something similar to what happened in Example 6. The superalgebra would be $C^{\infty }\left(\mathbb{C}\right)[{\chi }_1,{\chi }_2]$, that is the superalgebra of the affine superspace $A^{1|2}$ $A^{1|2}$.

These types of constraints appear in [23].

Remark 5.

In Appendix C, we write the infinitesimal supertranslation algebra, which acts on the affine superspace $A^{4|2}$. Actually, the supertranslation generators (A2) act on $A^{4|4}$, the complexified Minkowski superspace. There is of course a real form of this superspace and of the supertranslation algebra, which are the usual in physics. On the chiral affine superspace, $A^{4|2}$ only acts one the superalgebra generated by the generators $P_{\mu }$ and $Q_{\alpha }$.

The sets of Equations (13) and (17) are supersymmetric since ${\mathsf{\Phi }}^n=0$ is a supersymmetric constraint. While in the $n=2$ case, the solution obtained by inverting F is a supersymmetric solution, in the $n\ge 3$ case, the solution obtained by imposing (18) is not supersymmetric. This can be checked by explicit calculation. Nevertheless, the constraints being supersymmetric, the space of solutions of (17) has an action of the supertranslation algebra. It is then mandatory to keep the nilpotent A with $A^{n-1}=0$ if one wants to preserve supersymmetry. Although we do not know yet the physical interpretation of such fields, it is remarkable that one is led to maintain genuinely even nilpotents (that is, nilpotents that survive when putting the odd variables to zero) in order to preserve the supersymmetry.

In this situation, we do not have enough odd variables for the problem with $n>3$ to be interesting. One can add more odd variables by going to extended supersymmetry. Physically, though, it is more difficult to give meaning to superspace and superfields in extended supersymmetry. We could also consider real superfields, which have four real odd variables. Finally, one can use several superfields.

In the next section, we see how we can satisfy cubic constraints with two superfields.

4.4. Cubic Constraint with Two Superfields

Let us start with two superfields:

$${\mathsf{\Phi }}_1=A_1+{\theta }^{\alpha }{\chi }_{1\alpha }+{\theta }^{\alpha }{\theta }_{\alpha }{F}_1,{\mathsf{\Phi }}_2=A_2+{\theta }^{\alpha }{\chi }_{2\alpha }+{\theta }^{\alpha }{\theta }_{\alpha }{F}_2.$$

The quantities $A_1,A_2,F_1,F_2$ (even) and ${\chi }_{1\alpha },{\chi }_{2\alpha }$, $\alpha =1,2$ (odd) are coordinates in the superspace $A^{4|4}$. On these coordinates, we want to impose the constraint ${\mathsf{\Phi }}_1·{\mathsf{\Phi }}_2=0$:

$${\mathsf{\Phi }}_1·{\mathsf{\Phi }}_2=A_1{A}_2+{\theta }^{\alpha }(A_1{\chi }_{2\alpha }+A_2{\chi }_1^{\alpha })+{\theta }^{\alpha }{\theta }_{\alpha }(A_1{F}_2+A_2{F}_1-\frac{1}{2}{\chi }_1^{\alpha }{\chi }_{2\alpha })=0,$$

which implies:

$$\begin{array}{cc}\hfill & A_1{A}_2=0,\hfill \\ \hfill & A_1{\chi }_{2\alpha }+A_2{\chi }_{1\alpha }=0,\hfill \\ \hfill & A_1{F}_2+A_2{F}_1-\frac{1}{2}\left({\chi }_1{\chi }_2\right)=0.\hfill \end{array}$$

(19)

To ease the notation, we have written $\left({\chi }_1{\chi }_2\right):={\chi }_1^{\alpha }{\chi }_{2\alpha }$.

This defines an affine superscheme $\mathcal{L}$ with superalgebra:

$$\mathcal{O}\left(\mathcal{L}\right):=C^{\infty }\left({\mathbb{C}}^4\right)[{\chi }_{1\alpha },{\chi }_{2\alpha }]/(A_1{A}_2,A_1{\chi }_{2\alpha }+A_2{\chi }_{1\alpha },A_1{F}_2+A_2{F}_1-\frac{1}{2}\left({\chi }_1{\chi }_2\right)).$$

Let us compute the scheme, $\tilde{\mathcal{L}}$. Setting to zero the odd coordinates in (19), we get:

$$A_1{A}_2=0,A_1{F}_2+A_2{F}_1=0,$$

(20)

so

$$\mathcal{O}\left(\tilde{\mathcal{L}}\right)=C^{\infty }\left({\mathbb{C}}^4\right)/\left(A_1{A}_2,A_1{F}_2+A_2{F}_1\right).$$

We can now localize at $F_1\ne 0$ and solve for $A_2$. Then, (20) becomes:

$$F_2{A}_1^2=0,A_2=-F_1^{-1}{F}_2{A}_1.$$

If we are willing to restrict also to the points $F_2\ne 0$, we would get:

$$A_1^2=0,A_2=-F_1^{-1}{F}_2{A}_1,$$

so $A_1$ and $A_2$ are even nilpotents. The ring would become:

$$\mathcal{O}{\left(\tilde{\mathcal{L}}\right)}_{F_1,F_2\ne 0}=C^{\infty }({\mathbb{C}}^{\times }\times {\mathbb{C}}^{\times }\times \mathbb{C})/\left(A_1^2\right).$$

The scheme is not regular.

We now reintroduce the odd variables, but we localize only at $F_1\ne 0$. Then, we can solve for $A_2$:

$$A_2=-\frac{F_2}{F_1}{A}_1+\frac{1}{2F_1}\left({\chi }_1{\chi }_2\right).$$

Inserting into the first and second equations in (19), we get:

$$\begin{array}{cc}\hfill & F_2{A}_1^2-\frac{1}{2}\left({\chi }_1{\chi }_2\right)A_1=0\hfill \\ \hfill & A_1\left({\chi }_{2\alpha }-\frac{F_2}{F_1}{\chi }_{1\alpha }+\frac{1}{2F_1}\left({\chi }_1{\chi }_2\right){\chi }_{1\alpha }\right)=0.\hfill \end{array}$$

(21)

If $F_2\ne 0$, one can see that $A_1^4=0$, so $A_1$ is nilpotent.

One may consider, as in (18), the extra condition that $A_1$ is an even function of the odd variables,

$$A_1=a\left({\chi }_1^2\right)+b\left({\chi }_2^2\right)+c\left({\chi }_1{\chi }_2\right),$$

(22)

with a, b, and c coefficients that can also be functions of the other fields. As before, we stress that the meaning is that when putting the odd variables to zero, we take $A_1=0$, which solves trivially $A_1^2=0$. Inserting now the ansatz in (21) and after some calculations, we get:

$$a=\frac{1}{4F_1}-\frac{F_2^2}{F_1^2}b,c=-\frac{2F_2}{F_1}b,$$

and b is free. We have made use of the identities:

$$\left({\chi }_1{\chi }_2\right){\chi }_{1\alpha }=-\frac{1}{2}\left({\chi }_1^2\right){\chi }_{2\alpha },\left({\chi }_1{\chi }_2\right){\chi }_{2\alpha }=-\frac{1}{2}\left({\chi }_2^2\right){\chi }_{1\alpha }.$$

Therefore, the superfields become:

$$\begin{array}{cc}\hfill A_1& =\left(\frac{1}{4F_1}+\frac{F_2^2}{F_1^2}b\right)\left({\chi }_1^2\right)+b\left({\chi }_2^2\right)-2\frac{F_2}{F_1}b\left({\chi }_1{\chi }_2\right),\hfill \\ \hfill A_2& =\left(-\frac{F_2}{4F_1^2}-\frac{F_2^3}{F_1^3}b\right)\left({\chi }_1^2\right)-\left(2\frac{F_2^2}{F_1^2}b-\frac{F_2}{F_1}b\left({\chi }_2^2\right)+\frac{1}{2F_1}\right)\left({\chi }_1{\chi }_2\right),\hfill \end{array}$$

(23)

with ${\chi }_1$ and ${\chi }_2$ free, $F_2$ free, and $F_1\ne 0$. Since the Equation (19) are symmetric under the exchange $1\rightleftarrows 2$, one can obtain a similar solution by inverting $F_2$.

If $b=0$, the terms proportional to b in ${\mathsf{\Phi }}_1$ vanish, and we get ${\mathsf{\Phi }}_1^2=0$. With this choice, we obtain for ${\mathsf{\Phi }}_2$:

$${\mathsf{\Phi }}_2^2=-\frac{1}{8F_1}{\chi }_1^2{\chi }_2^2\ne 0.$$

It is not difficult to see that the particular set of solutions with $b=0$ are equivalent to the system:

$${\mathsf{\Phi }}_1^2=0,{\mathsf{\Phi }}_1{\mathsf{\Phi }}_2=0,$$

(24)

once we have inverted $F_1$. One obtains:

$$A_1=\frac{1}{4F_1}{\left({\chi }_1\right)}^2,A_2=\frac{\left({\chi }_1{\chi }_2\right)}{2F_1}-\frac{F_2{\left({\chi }_1\right)}^2}{4F_1^2},$$

and the remaining coordinates free (note that $F_2$ is not required to be invertible). This system has supersymmetry, since it has been cast in the supersymmetric form (24).

We check now the cubic constraints for generic b:

$${\mathsf{\Phi }}_1^2{\mathsf{\Phi }}_2={\mathsf{\Phi }}_1{\mathsf{\Phi }}_2^2=0$$

by virtue of ${\mathsf{\Phi }}_1{\mathsf{\Phi }}_2=0$. On the other hand, one gets also:

$${\mathsf{\Phi }}_1^3=0,{\mathsf{\Phi }}_2^3=0.$$

From the three equations in (17) with $n=3$, the first two are trivially satisfied because they have order greater than four in the odd variables, and we only have four of them. The third one is only of order four in the odd variables, and the terms must cancel exactly. It is not difficult to check that this happens for both superfields.

Nevertheless, the system

$${\mathsf{\Phi }}_1{\mathsf{\Phi }}_2=0,{\mathsf{\Phi }}_1^3=0,{\mathsf{\Phi }}_2^3=0$$

gives rise to a superscheme that is not regular. The constraints are:

$$\begin{array}{cc}\hfill & A_1{A}_2=0,A_1{\psi }_2+A_2{\psi }_1=0,A_1{F}_2+A_2{F}_1-\frac{1}{2}\left({\psi }_1{\psi }_2\right)=0,\hfill \\ \hfill & A_1^3=0,A_2^3=0,A_1^2{\psi }_{1\alpha }=0,A_2^2{\psi }_{2\alpha }=0,\hfill \\ \hfill & A_1\left(A_1{F}_1-\frac{1}{2}{\left({\psi }_1\right)}^2\right)=0,A_2\left(A_2{F}_2-\frac{1}{2}{\left({\psi }_2\right)}^2\right)=0.\hfill \end{array}$$

The scheme $\tilde{\mathcal{L}}$ is given by the constraints:

$$A_1{A}_2=0,A_1{F}_2+A_2{F}_1=0,A_1^3=0,A_2^3=0,F_1{A}_1^2=0,F_2{A}_2^2=0.$$

Even restricting to $F_1\ne 0$, one obtains:

$$A_2=-\frac{F_2}{F_1}{A}_1,A_1^2=0,$$

so a nilpotent remains that cannot be put directly to zero. The solutions obtained in (23) by imposing the ansatz (22) do not reflect the whole solution space, and consequently, they are not supersymmetric.

These constraints appeared in [22,24,25].

4.5. Cubic Constraint with an Arbitrary Number of Superfields

The system (24) can be generalized by adding more chiral superfields. We consider the following system:

$$\begin{array}{cccc}\hfill & X=A+\left(\theta \chi \right)+{\theta }^{2}F,\hfill & \hfill & X^2=0\hfill \\ \hfill & Y_i=A_i+\left(\theta {\psi }_i\right)+{\theta }^2{F}_i,\hfill & \hfill & X{Y}_i=0\hfill \end{array}$$

(25)

for $i=1,\dots ,n$ and n arbitrary. From (13) and (19), the constraints are equivalent to the system:

$$\begin{array}{cccccc}\hfill & A^2=0,\hfill & \hfill & A{\chi }_{\alpha }=0,\hfill & \hfill & 4AF-{\psi }^2=0\hfill \\ \hfill & A{A}_i=0,\hfill & \hfill & A{\psi }_{i\alpha }+A_i{\chi }_{\alpha }=0,\hfill & \hfill & A{F}_i+A_{i}F-\frac{1}{2}\left(\chi {\psi }_i\right)=0.\hfill \end{array}$$

Putting the odd variables to zero, the constraints become:

$$\begin{array}{cccc}\hfill & A^2=0,\hfill & \hfill & AF=0\hfill \\ \hfill & A{A}_i=0,\hfill & \hfill & A{F}_i+A_{i}F=0.\hfill \end{array}$$

Localizing at F invertible, we can solve

$$A=0,A_i=0,$$

which means that the scheme has a smooth part:

$$\mathbb{C}{\left[\tilde{\mathcal{L}}\right]}_{F\ne 0}={\mathcal{C}}^{\infty }({\mathbb{C}}^{\times }\times {\mathbb{C}}^n).$$

Reinserting the fermions, we get:

$$A=\frac{{\psi }^2}{4F},A_i=-\frac{{\chi }^2{F}_i}{4F^2}+\frac{1}{2F}\left(\chi {\psi }_i\right),$$

and the remaining equations are satisfied trivially.

In this case, we can use the same method as in Section 4.2. We perform a change of variables:

$$\begin{array}{c}\hfill A^{\prime }=4AF-{\chi }^2,A_i^{\prime }=A{F}_i+A-iF-\frac{1}{2}\chi {\psi }_i,\\ \hfill F^{\prime }=F,F_i^{\prime }=F_i,{\chi }^{\prime }=\chi ,{\psi }_i^{\prime }={\psi }_i,\end{array}$$

whose Jacobian is invertible if F is so. The constraints are then:

$$A^{\prime }=0,A_i^{\prime }=0,$$

and the superscheme at F invertible becomes:

$$\mathbb{C}{\left[\mathcal{L}\right]}_{F\ne 0}\simeq {\mathcal{C}}^{\infty }({\mathbb{C}}^{\times }\times {\mathbb{C}}^n)[{\chi }_{\alpha },{\psi }_{i\alpha }],$$

with $\alpha =1,2,$ and $i=1,\dots ,n$. The superfields $Y_i$, $i=1,\dots ,n$ satisfy:

$$Y_i{Y}_j{Y}_k=0,\forall i,j,k=1,\dots ,n.$$

(26)

In order to prove this, we used the following Fierz identity:

$$\left({\psi }_1{\psi }_2\right){\psi }_3+\left({\psi }_3{\psi }_1\right){\psi }_2+\left({\psi }_2{\psi }_3\right){\psi }_1=0.$$

We note that the system (25) is not the most general solution to (26). For $n=1$, the solution presented in (23) with ${\mathsf{\Phi }}_1=X$ and ${\mathsf{\Phi }}_2=Y$ is a more general one ($b\ne 0$). In general, imposing a constraint as (22) will break supersymmetry.

The case $n=3$ was presented in [26,27].

5. A Non-Algebraic Constraint

In this section, we are going to consider both chiral and antichiral superfields. Up to now, we were considering only chiral superfields, so the description of Section 4 was the simplest one. Moreover, the spacetime coordinates would not appear explicitly in the discussion of the constraints, so it was as if spacetime was reduced to a point. In this section, we will not be in that case anymore, and the spacetime variables would play a role.

We shall start with a (complexified) super spacetime $M=A^{4|4}$, with:

$$x^{\mu },\mu =0,\dots ,3,{\theta }^{\alpha },{\overline{\theta }}^{\dot{\alpha }},\alpha ,\dot{\alpha }=1,2$$

being its global coordinates. As the notation suggests, ${\theta }^{\alpha }$ and ${\overline{\theta }}^{\dot{\alpha }}$ are related by an antilinear involution that defines the standard (real) super Minkowski space, for which the coordinates $x^{\mu }$ are real. However, for our purposes, it is better to keep the super spacetime complexified, so ${\theta }^{\alpha }$ and ${\overline{\theta }}^{\dot{\alpha }}$ are independent odd coordinates and $x^{\mu }$ are even, complex coordinates.

According to what we expressed at the end of Section 2.2, we will now work with families of morphisms $S\times M\to \mathbb{C}$ parametrized by S. In terms of the algebras, we will have, as before:

$$\begin{array}{ccc}\mathcal{O}\left(\mathbb{C}\right)& \stackrel{}{⟶}& \mathcal{O}\left(S\right)\otimes \mathcal{O}\left(M\right)\\ z& \stackrel{}{⟶}& \mathsf{\Phi },\end{array}$$

where:

$$\begin{array}{cc}\hfill \mathsf{\Phi }=& F+\left(\theta \chi \right)+\left(\overline{\theta }\overline{\chi }\right)+\left(\theta \theta \right)M+\left(\overline{\theta }\overline{\theta }\right)N+\left(\theta {\sigma }^{\mu }\overline{\theta }\right)V_{\mu }\hfill \end{array}$$

$$\begin{array}{c}\hfill +\left(\theta \theta \right)\left(\overline{\theta }\overline{\lambda }\right)+\left(\overline{\theta }\overline{\theta }\right)\left(\theta \mathsf{\Psi }\right)+\left(\theta \theta \right)\left(\overline{\theta }\overline{\theta }\right)D.\end{array}$$

(27)

If there were no dependence on the coordinates $x^{\mu }$ of spacetime, then the superspace of superfields would be $\mathcal{H}=A^{8|8}$, with coordinates:

$$(F,M,N,V_{\mu },D|{\varphi }^{\alpha },{\overline{\chi }}^{\dot{\alpha }},{\overline{\lambda }}^{\dot{\alpha }},{\psi }^{\alpha }),\mu =0,\dots 4,\alpha ,\dot{\alpha }=1,2.$$

The symbols ${\sigma }^{\mu }$ stand for the Pauli matrices, and the notation for the spinor (odd) fields is explained in Appendix B.

When spacetime is not a point, the coordinates above depend on $x^{\mu }$ and are the component fields of the superfield. This suffices to describe the family of maps parametrized by S.

We consider now two odd derivations on $\mathcal{O}\left(M\right)={\mathcal{O}}^{4|4}\left({\mathbb{C}}^4\right)$:

$$D_{\alpha }={\partial }_{\alpha }+\mathrm{i}{\sigma }_{\alpha \dot{\alpha }}^{\mu }{\overline{\theta }}^{\dot{\alpha }}{\partial }_{\mu },{\overline{D}}_{\dot{\alpha }}=-{\partial }_{\dot{\alpha }}-\mathrm{i}{\theta }^{\alpha }{\sigma }_{\alpha \dot{\alpha }}^{\mu }{\partial }_{\mu },$$

where:

$${\partial }_{\alpha }=\frac{\partial }{\partial {\theta }^{\alpha }},{\partial }_{\dot{\alpha }}=\frac{\partial }{\partial {\overline{\theta }}^{\dot{\alpha }}},{\partial }_{\mu }=\frac{\partial }{\partial x^{\mu }}.$$

$D_{\alpha }$ and ${\overline{D}}_{\dot{\alpha }}$ are indeed the right invariant vector fields of the action of the supertranslation generators (see Appendix C).

The derivations above can be applied to $\mathcal{O}\left(S\right)\otimes \mathcal{O}\left(M\right)$, provided that the rule of signs is appropriately taken into account. We now define chiral superfields in terms of a constraint on superfields, as it is done in physics. We say that the superfield $\mathsf{\Phi }$ (27) is chiral if

$${\overline{D}}_{\dot{\alpha }}\mathsf{\Phi }=0.$$

From the fact that:

$${\overline{D}}_{\dot{\alpha }}\left(x^{\mu }+\mathrm{i}\left(\theta {\sigma }^{\mu }\overline{\theta }\right)\right)=0,$$

it is easy to see that, under the change of variables:

$$(x^{\mu },{\theta }^{\alpha },{\overline{\theta }}^{\dot{\alpha }})⟶(y^{\mu }=x^{\mu }+\mathrm{i}\left(\theta {\sigma }^{\mu }\overline{\theta }\right),{\theta }^{\alpha },{\overline{\theta }}^{\dot{\alpha }}),\left(\theta {\sigma }^{\mu }\overline{\theta }\right):={\theta }^{\alpha }{\sigma }_{\alpha \dot{\alpha }}^{\mu }{\overline{\theta }}^{\dot{\alpha }},$$

a chiral superfield ${\mathsf{\Phi }}^{\mathrm{ch}}$ can be written as:

$${\mathsf{\Phi }}^{\mathrm{ch}}=A^{\mathrm{ch}}\left(y\right)+\left(\theta {\chi }^{\mathrm{ch}}\left(y\right)\right)+{\theta }^2{F}^{\mathrm{ch}}\left(y\right).$$

Instead, under the change of variables:

$$(x^{\mu },{\theta }^{\alpha },{\overline{\theta }}^{\dot{\alpha }})⟶({\overline{y}}^{\mu }=x^{\mu }-\mathrm{i}\left(\theta {\sigma }^{\mu }\overline{\theta }\right),{\theta }^{\alpha },{\overline{\theta }}^{\dot{\alpha }}),$$

the antichiral superfield:

$$D_{\alpha }{X}^{\mathrm{ach}}=0,$$

is expressed as:

$$X^{\mathrm{ach}}=A^{\mathrm{ach}}\left(\overline{y}\right)+\left(\overline{\theta }{\chi }^{\mathrm{ach}}\left(\overline{y}\right)\right)+{\overline{\theta }}^2{F}^{\mathrm{ach}}\left(\overline{y}\right).$$

$y^{\mu }$ and ${\overline{y}}^{\mu }$ are related by complex conjugation (as the notation suggests). The complex conjugate of a chiral superfield is an antichiral superfield, and we also have:

$${\overline{y}}^{\mu }=y^{\mu }-2\mathrm{i}\left(\theta {\sigma }^{\mu }\overline{\theta }\right).$$

(28)

Let us now consider now two chiral superfields:

$$X=A\left(y\right)+\theta \chi \left(y\right)+{\theta }^{2}F\left(y\right),Y=B\left(y\right)+\theta \psi \left(y\right)+{\theta }^{2}G\left(y\right),$$

and assume that $X^2=0$ as in Section 4.2. Then, if F is invertible:

$$X=\frac{\chi {\left(y\right)}^2}{4F\left(y\right)}+\theta \chi \left(y\right)+{\theta }^{2}F\left(y\right).$$

We write the complex conjugate of X as:

$$\overline{X}=\frac{\overline{\chi }{\left(\overline{y}\right)}^2}{4\overline{F}\left(\overline{y}\right)}+\overline{\theta }\overline{\chi }\left(\overline{y}\right)+{\theta }^2\overline{F}\left(\overline{y}\right),$$

where the notation (the usual one in physics) means:

$$\overline{A}\left(\overline{y}\right):=\overline{A\left(y\right)},{\overline{\chi }}_{\dot{\alpha }}\left(\overline{y}\right):=\overline{{\chi }_{\alpha }\left(y\right)},\overline{F}\left(\overline{y}\right):=\overline{F\left(y\right)}.$$

The constraint that we intend to impose is [22]:

$$\overline{X}Y=\mathrm{antichiral}.$$

(29)

In order to do that, one writes the superfield Y in terms of the variable ${\overline{y}}^{\mu }$, using (28) and expanding in Taylor series, which is finite because of the nilpotency of the odd variables. One gets:

$$Y=B+\theta \psi +{\theta }^{2}G+2\mathrm{i}{\partial }_{\mu }B\left(\theta {\sigma }^{\mu }\overline{\theta }\right)-\mathrm{i}{\theta }^2\left({\partial }_{\mu }\psi {\sigma }^{\mu }\overline{\theta }\right),$$

where all the component fields are evaluated at ${\overline{y}}^{\mu }$. In order to impose the constraint (29), the only components of $\overline{X}Y$ that can survive are the ones proportional to one, ${\overline{\theta }}^{\alpha }$ and ${\overline{\theta }}^2$. After some calculations, we get (recall that F is invertible):

$$\begin{array}{cccc}\hfill & {\overline{\chi }}^2{\psi }_{\alpha }=0,\hfill & \hfill & {\overline{\chi }}^{2}G=0\hfill \\ \hfill & \frac{{\partial }^{2}B}{4\overline{F}}{\overline{\chi }}^2+\frac{\mathrm{i}}{2}\left({\partial }_{\mu }\psi {\sigma }^{\mu }\overline{\chi }\right)+\overline{F}G=0,\hfill & \hfill & -\mathrm{i}{\partial }_{\mu }B{\sigma }_{\alpha \dot{\alpha }}^{\mu }{\overline{\chi }}^{\dot{\alpha }}+{\psi }_{\alpha }\overline{F}=0,\hfill \\ \hfill & -\mathrm{i}\left({\partial }_{\mu }{\psi }^{\alpha }{\sigma }_{\alpha \dot{\alpha }}^{\mu }\right)\frac{{\overline{\chi }}^2}{4\overline{F}}+{\overline{\chi }}_{\dot{\alpha }}G=0,\hfill & \hfill & \frac{\mathrm{i}{\partial }_{\mu }B{\chi }^2}{2\overline{F}}{\sigma }_{\alpha \dot{\alpha }}^{\mu }+{\overline{\chi }}_{\dot{\alpha }}{\psi }_{\alpha }=0.\hfill \end{array}$$

(30)

Putting the odd variables to zero, we get $G=0$ and B undetermined. The constraints are algebraic, and the scheme that we obtain is:

$$\mathcal{O}\left(\tilde{\mathcal{L}}\right)\simeq C^{\infty }({\mathbb{C}}^{\times }\times \mathbb{C}).$$

The full constraints can be considerably simplified using the fact that F is invertible. For example, one can isolate G and ${\psi }_{\alpha }$:

$$G=-\frac{{\partial }^{2}B}{4{\overline{F}}^2}{\overline{\chi }}^2-\frac{\mathrm{i}}{2\overline{F}}\left({\partial }_{\mu }\chi {\sigma }^{\mu }\overline{\chi }\right),{\psi }_{\alpha }=\mathrm{i}{\partial }_{\mu }B{\sigma }_{\alpha \dot{\alpha }}^{\mu }{\overline{\chi }}^{\dot{\alpha }},$$

and the remaining constraints are satisfied. Although the constraints involve derivatives, the superscheme can be given algebraically, in its complex version, as:

$$\mathcal{O}\left(\mathcal{L}\right)\simeq C^{\infty }({\mathbb{C}}^{\times }\times \mathbb{C})[{\chi }_{\alpha },{\overline{\chi }}_{\dot{\alpha }}].$$

This example is also illustrative of the properties of the odd fields. All through the calculations, one has to assume that an odd field, say ${\chi }_{\alpha }$, and its spacetime derivative ${\partial }_{\mu }{\chi }_{\alpha }$ have a product that is different from zero. Finally, the superscheme has a smooth part, on which the supersymmetry transformations have a well defined action.

6. Observables and Nilpotent Variables

Let F be an algebra, and consider the scheme $\underline{\mathrm{Spec}}\left(F\right)$. Let $\mathfrak{p}$ be a prime ideal in F, so $\mathfrak{p}\in \left|X\right|=\mathrm{Spec}\left(F\right)$, and consider the quotient $F/\mathfrak{p}$. This is an integral domain (the product of two non-zero elements is a non-zero element). Moreover, if we consider the localization of F over $\mathfrak{p}$,

$$F_{\mathfrak{p}}=\left\{\frac{f}{g}|f,g\in F,g\notin \mathfrak{p}\right\},$$

and we quotient with the ideal $F_{\mathfrak{p}}·\mathfrak{p}$, the result $\kappa \left(\mathfrak{p}\right):=F_{\mathfrak{p}}/(F_{\mathfrak{p}}·\mathfrak{p})$ is a field, since every non-zero element in $\kappa \left(\mathfrak{p}\right)$ has an inverse. The field $\kappa \left(\mathfrak{p}\right)$ is called the residue field of $\left|X\right|$ at $\mathfrak{p}$.

Example 7.

1.

We consider the ring of polynomials in one variable $F=\mathbb{C}\left[x\right]$. The prime ideals of F are of the form ${\mathfrak{p}}_a=(x-a)$, $a\in \mathbb{C}$ or the ideal $\left(0\right)$. It is not difficult to see that the residue field at ${\mathfrak{p}}_a$ is $\kappa \left({\mathfrak{p}}_a\right)\cong \mathbb{C}$ and $\kappa \left(\left(0\right)\right)$ is the field of rational functions.

2.

If the ground field is $\mathbb{R}$, we have that every irreducible polynomial in $\mathbb{R}\left[x\right]$ generates a prime ideal. We still have the maximal ideals as ${\mathfrak{p}}_a=(x-a)$ that give all the points of $\mathbb{R}$. At them, the residue field is $\kappa \left({\mathfrak{p}}_a\right)\cong \mathbb{R}$. However, for example, the irreducible polynomial $x^2+1$ also generates a prime ideal. It is not difficult to realize that the elements of the residue field are of the form $a+xb$, with $a,b\in \mathbb{R}$ and x such that $x^2+1=0$, so $\kappa \left((x^2+1)\right)\cong \mathbb{C}$.

As we have seen, affine schemes have residue fields that can vary from point to point. Let F be an algebra, not necessarily affine. For every element $f\in F$, we can define a “function” on $\mathrm{Spec}\left(F\right)$ with values in the residue field via the canonical maps:

$$\begin{array}{ccccc}F& \stackrel{}{⟶}& F_{\mathfrak{p}}& \stackrel{}{⟶}& \kappa \left(\mathfrak{p}\right)\\ f& \stackrel{}{⟶}& f& \stackrel{}{⟶}& f\left(\mathfrak{p}\right).\end{array}$$

In an affine variety, with affine algebra F, one recovers in this way the original interpretation of F as the algebra of functions on the algebraic variety. The same holds in the case of differentiable manifolds and smooth functions.

If F contains a nilpotent element, say n, then $n\in \mathfrak{p}$ for all prime ideals $\mathfrak{p}$ so $n\left(\mathfrak{p}\right)=0$. In other words, n is sent to the zero function, and one cannot reproduce the original algebra F starting from an algebra of functions on $\mathrm{Spec}\left(F\right)$. This is something that we already knew (see Example 6), but now, we can read it from a physical point of view.

A classical mechanics system is commonly described in terms of a symplectic manifold called phase space, whose points represent the possible states of the system. Classical observables are smooth functions on phase space. There is a special observable, the Hamiltonian, which governs the time evolution of the system: given the initial state in an instant of time $t_0$, the system evolves in future times t by following the integral curve of the Hamiltonian vector field associated with the Hamiltonian, passing through the initial state.

This picture can be more or less carried over classical field theory by substituting the phase space for an infinite-dimensional space of maps from spacetime to a target manifold or of sections of some bundle over spacetime, which are the fields. Most of the time, one uses variational calculus to approach classical field theory instead of trying to give some comprehensive study of these infinite-dimensional spaces, which can be very involved. Nevertheless, the idea of an observable is mimicked from classical mechanics: observables are (a special class of) functionals on the space of fields. The time evolution of the system is also governed by some partial differential equations (usually second order) for the fields.

The idea that we want to convey can be already understood at the classical mechanics level. Suppose that we want to generalize the classical phase space to some sort of affine scheme whose algebra contains nilpotents. The usual way to obtain “numbers” (results of a measurement) from the sections of the scheme is by the evaluation procedure explained above. Nilpotent elements go to zero by this map, so they do not represent observables.

Now, one could do the same for a superscheme: even a smooth supermanifold or regular algebraic variety contains nilpotents generated by the odd elements which could not be seen in any “classical” measurement. What we are affirming is that in this interpretation of the observables, classical, odd degrees of freedom could not be seen in experiments.

In quantum mechanics, things are very different. States are rays in a Hilbert space, and observables are Hermitian operators on it. The results of measurements are eigenvalues of these operators, and they appear with a certain probability distribution. The algebra of operators on a Hilbert space is non-commutative, so sometimes, it is said, very roughly, that “quantizing” a system corresponds to substituting the commutative algebra of observables by a non-commutative one, such that when taking the limit $\hslash \to 0$, the original commutative algebra is recovered.

Let us consider the simplest case possible, a two-dimensional phase space ${\mathbb{R}}^2$ with canonical coordinates $(q,p)\in {\mathbb{R}}^2$ and symplectic form $\mathrm{d}q\wedge \mathrm{d}p$. The induced Poisson bracket on the coordinates is:

$${\{q,p\}}_{-}=1.$$

(31)

As a quantum system, one considers the Hilbert space of square integrable functions on the variable q, ${\mathcal{L}}^2\left(\mathbb{R}\right)$. One considers the position and momentum operators:

$$Qf\left(q\right)=qf\left(q\right),Pf\left(q\right)=-\mathrm{i}\hslash \frac{\partial f}{\partial q},$$

whose the commutation rule is:

$${[Q,P]}_{-}=\mathrm{i}\hslash \mathrm{id}.$$

Taking $\hslash \to 0$, the commutation relation is reverted to the commutativity of q and p as ordinary functions on phase space. The fact that the term of order one in ℏ is proportional to the Poisson bracket (substituting the constant function “1;’ by the identity) is not casual, but a requirement of the quantization.

Let us assume that the phase space is now substituted by a superspace, for example $A^{2|2}$, with superalgebra $C^{\infty }\left({\mathbb{R}}^2\right)\otimes \wedge [\theta ,\pi ]$ (this type of “super quantum mechanics” has been studied from the point of view of superfields in [28]). There is also a super Poisson structure on it:

$${\{\theta ,\pi \}}_{+}=1,{\{q,p\}}_{-}=1,$$

and the rest zero. The Poisson bracket of two odd quantities is symmetric.

As in the non-super case, the superalgebra $C^{\infty }\left({\mathbb{C}}^2\right)\otimes \wedge [\theta ,\pi ]$ admits a deformation with parameter ℏ.

Let us focus now on the deformation of the Grassmann algebra $\wedge [\theta ,\pi ]$. Mimicking the procedure with the even variables, we get a non-commutative superalgebra with generators $\mathsf{\Theta }$ and $\mathsf{\Pi }$ satisfying the commutation rules:

$${[\mathsf{\Theta },\mathsf{\Pi }]}_{+}=\mathrm{i}\hslash \mathrm{id}{[\mathsf{\Theta },\mathsf{\Theta }]}_{+}=0,{[\mathsf{\Pi },\mathsf{\Pi }]}_{+}=0,$$

which is the algebra of the odd quantum oscillator with its creation and destruction operators. From them, one can construct the Hilbert space and the quantum observables.

There is a linear change of variables:

$$\mathsf{\Gamma }=\mathsf{\Theta }+\mathrm{i}\mathsf{\Pi },\mathsf{{\rm Y}}=\mathsf{\Theta }-\mathrm{i}\mathsf{\Pi },$$

which shows that this algebra is isomorphic to the Clifford algebra $C(1,1)$ [29,30,31,32]:

$${[\mathsf{\Gamma },\mathsf{\Gamma }]}_{+}=\hslash \mathrm{id},{[\mathsf{{\rm Y}},\mathsf{{\rm Y}}]}_{+}=-\hslash \mathrm{id},{[\mathsf{\Gamma },\mathsf{{\rm Y}}]}_{+}=0.$$

(32)

The key point here is the symmetry (versus the antisymmetry for the even variables) of the odd part of the super Poisson bracket.

The conclusion is that, in their quantum version, odd variables can give rise to meaningful observables. The classical limit $\hslash \to 0$ leaves the superspace mathematical structure, but it does not produce classical, odd observables.

Some of the schemes that we describe in this paper have a regular part, and physicists have restricted themselves to study such a regular part. However, we believe that restrictions such as $F\left(x\right)\ne 0$, for F a scalar field, are quite unnatural. What would happen if one considers the full solution, the one with the even nilpotents taken into account? In particular, what could happen at a quantum level?

One may wonder if the new nilpotent variables will also go through the process of becoming observables in the quantum realm.

More modestly, one may try to deform non-reduced superalgebras as in deformation quantization: formally, in terms of powers of an undetermined parameter that may be the Planck constant. Deformation quantization of manifolds and algebraic varieties has been studied [33,34,35]. It could be interesting to see, with a simple example such as the ones we encounter solving the constraints, if one can obtain a deformation quantization, not intending to deal in complete generality with the deformation of schemes or superschemes.

7. Conclusions

In this paper, we reviewed the concept of the superfield, realizing that there is a superspace of superfields given by the inner morphisms from super spacetime to $\mathbb{R}$ or $\mathbb{C}$ (for a scalar superfield). This superspace is used in physics via its functor of points, and it was given here by an adjunction formula. We then considered the toy model when spacetime is a point: then, the superspace of superfields is explicitly computed in terms of supersheaves. For a spacetime of dimension D, the superspace of superfields becomes infinite-dimensional, and then more difficult to deal with. Nevertheless, using the functor of points approach, one can restrict one’s self to consider a family of maps from super spacetime to the field of scalars, parametrized by an (arbitrary) supermanifold. This point of view can be extended without problem when spacetime has dimension D, and then, the usual variational calculus can be used in the standard, field theoretical way.

The fact that the extra variables in the arbitrary supermanifold appear only when the functor of points is used is an indication that they are spurious and have no physical meaning. They also do not alter the field content of the superfield. One may presume that this spurious character is maintained when spacetime has dimension D, even if in those cases, we are not able to compute explicitly the superspace of superfields in terms of sheaves.

We also showed how to recover the defining sheaf of super spacetime once the superspace of scalar superfields is given.

We analyzed some algebraic constraints imposed on one or more scalar superfields that cannot come from a restriction of superspace itself. The easiest of them is a superfield squared equal zero, so it is a nilpotent superfield. This is reflected then in the space of superfields, which has a genuinely even nilpotent variable, not coming from products of odd variables. There is then the need to define the functor of points over the category of superschemes. We identify two different types of constraints, those that give rise to a scheme that has a regular part and those who do not. Usually in physics, the last ones are said to be “non-supersymmetric”, because in order to solve the constraints, an extra constraint on the component fields is hand imposed. Restricting in that way the space of solutions is what breaks the supersymmetry, not the original constraint. One could investigate further the possibility of allowing non-regular superschemes as superspaces of superfields.

We finally expose some thoughts on the nature of nilpotent variables and the impossibility to measure them at a classical level. For example, the canonical commutation relations of odd variables give rise, in the quantum realm, to a Clifford algebra from which true observables can be extracted. Therefore, it is only when the superalgebra is deformed in the quantum theory that these variables appear as observables. One may wonder how the even nilpotents appearing in our superschemes behave under quantization or, simply, what could be a deformation of such superalgebras.