Wannier States of FCC Symmetry Qualifying Paramagnetic NiO to Be a Mott Insulator
Abstract
1. Introduction
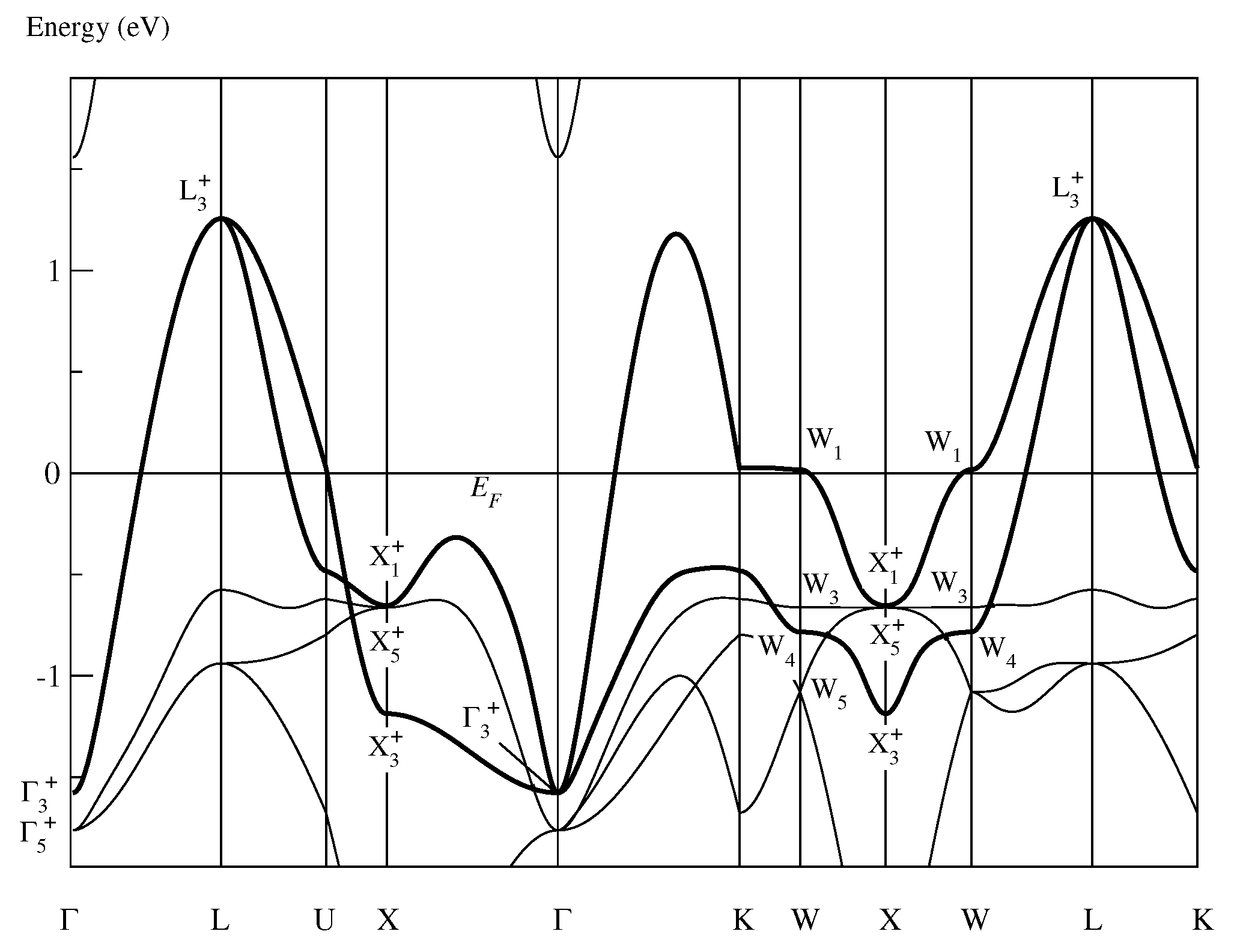
2. Nonadiabatic Atomic-Like Motion in Paramagnetic NiO
- as well localized as possible (according to Definition 5 of [5]);
- centered at the Ni (Table a) or O (Table b) atoms; and
- symmetry-adapted to (according to Equation (10) of [5]).
3. Discussion
3.1. Antiferromagnetic NiO
3.2. Paramagnetic NiO
4. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Krüger, E. Structural Distortion Stabilizing the Antiferromagnetic and Insulating Ground State of NiO. Symmetry 2019, 12, 56. [Google Scholar] [CrossRef]
- Brandow, B. Electronic structure of Mott insulators. Adv. Phys. 1977, 26, 651–808. [Google Scholar] [CrossRef]
- Trimarchi, G.; Wang, Z.; Zunger, A. Polymorphous band structure model of gapping in the antiferromagnetic and paramagnetic phases of the Mott insulators MnO, FeO, CoO, and NiO. Phys. Rev. B 2018, 97, 035107. [Google Scholar] [CrossRef]
- Hubbard, J. Elelectron correlations in narrow energy bands. Proc. R. Soc. Lond. Ser. A 1963, 276, 238–257. [Google Scholar]
- Krüger, E.; Strunk, H.P. Group Theory of Wannier Functions Providing the Basis for a Deeper Understanding of Magnetism and Superconductivity. Symmetry 2015, 7, 561–598. [Google Scholar] [CrossRef]
- Krüger, E. Nonadiabatic extension of the Heisenberg model. Phys. Rev. B 2001, 63, 144403-1-13. [Google Scholar] [CrossRef]
- Bradley, C.; Cracknell, A.P. The Mathematical Theory of Symmetry in Solids; Claredon: Oxford, UK, 1972. [Google Scholar]
- Austin, I.G.; Mott, N.F. Metallic and Nonmetallic Behavior in Transition Metal Oxides. Science 1970, 168, 71–77. [Google Scholar] [CrossRef] [PubMed]
| (a) | Ni(000) | X | L | W | |
| Band 1 | |||||
| Band 2 | |||||
| Band 3 | |||||
| Band 4 | |||||
| Band 5 | + | + | |||
| Band 6 | + | + | |||
| Band 7 | + | + | + | ||
| Band 8 | + | + | + | ||
| Band 9 | + | + | + | ||
| Band 10 | + | + | + | ||
| (b) | O() | ||||
| Band 1 | |||||
| Band 2 | |||||
| Band 3 | |||||
| Band 4 | |||||
| Band 5 | + | + | |||
| Band 6 | + | + | |||
| Band 7 | + | + | + | ||
| Band 8 | + | + | + | ||
| Band 9 | + | + | + | ||
| Band 10 | + | + | + |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Krüger, E. Wannier States of FCC Symmetry Qualifying Paramagnetic NiO to Be a Mott Insulator. Symmetry 2020, 12, 687. https://doi.org/10.3390/sym12050687
Krüger E. Wannier States of FCC Symmetry Qualifying Paramagnetic NiO to Be a Mott Insulator. Symmetry. 2020; 12(5):687. https://doi.org/10.3390/sym12050687
Chicago/Turabian StyleKrüger, Ekkehard. 2020. "Wannier States of FCC Symmetry Qualifying Paramagnetic NiO to Be a Mott Insulator" Symmetry 12, no. 5: 687. https://doi.org/10.3390/sym12050687
APA StyleKrüger, E. (2020). Wannier States of FCC Symmetry Qualifying Paramagnetic NiO to Be a Mott Insulator. Symmetry, 12(5), 687. https://doi.org/10.3390/sym12050687






