Symmetry Classes and Matrix Representations of the 2D Flexoelectric Law
Abstract
1. Introduction
- What types of anisotropies can flexoelectric tensors model?
- How does one characterize flexoelectric materials independently of their spatial orientation?
- How does one parametrize the different characteristics (anisotropy, coupling, etc.) of flexoelectric materials in an intrinsic way?
2. Notation
- the group of invertible matrices over ;
- the group of matrices satisfying and , where and stand for the inverse and the transpose of the matrix . This group is called the orthogonal group;
- the subgroup of of matrices of determinant 1, called the special orthogonal group.
- the identity group;
- the cyclic group with k elements, generated by . For , we have ;
- the dihedral group with elements, generated by and . For , the group will be denoted by .
- Centrosymmetric (denoted by ) if its contains the inversion , and non-centrosymmetric () otherwise;
- Chiral (denoted by ) if its does not contain reflection or mirror , and achiral () otherwise.
- ⊗ denotes the usual tensor product of two tensors or vector spaces;
- denotes the n-th power of the tensor product, e.g., (n copies);
- denotes the symmetrized tensor product;
- indicates the twisted tensor product defined by:
- is the space of n-th order tensors with no index symmetry on ;
- is the space of completely symmetric (by completely symmetric we mean symmetric with respect to all permutations of indices) n-th order tensors on ;
- is the space of n-th order harmonic tensors (i.e., completely symmetric and traceless tensors), with
- is the space of scalars;
- is the space of pseudo-scalars (i.e., scalars which change sign under improper transformations).
- is the second-order identity tensor with components , where is the Kronecker delta;
- is the fourth-order identity tensor on ;
- denotes the 2D Levi–Civita tensor defined by:
- is the space of dimensional square matrices;
- is the space of dimensional symmetric square matrices;
- is the space of rectangular matrices.
- ⊕ direct sum;
- ≃ denotes hereafter an isomorphism.
3. Flexoelectricity Law
3.1. Constitutive Equations
- is a fourth-order elasticity tensor;
- is a fifth-order elasticity tensor;
- is the fifth-order elasticity tensor defined as the transpose of in the following sense ;
- is the sixth-order elasticity tensor.
- is the dielectric susceptibility tensor;
- is the piezoelectric tensor;
- is the transpose of the piezoelectric tensor . The transposition is defined as ;
- is the flexoelectric tensor;
- is the transpose of the flexoelectric tensor . The transposition is defined as .
- State tensors: , , , , , and ;
- Constitutive tensors: , , , , , and .
3.2. Notions of Symmetry Group and Symmetry Class
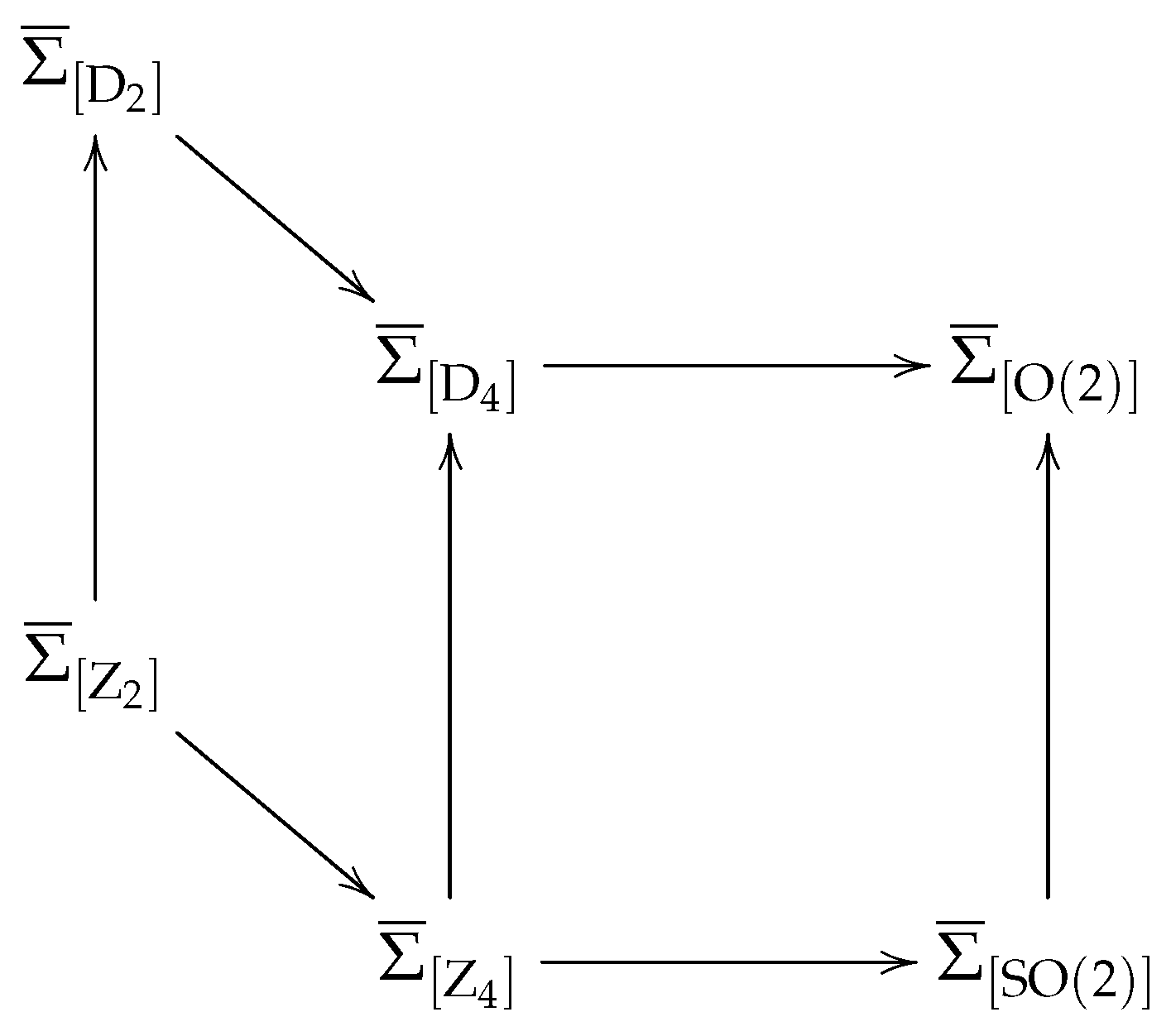
3.3. Symmetry Classes of the Complete Flexoelectric Law
3.4. Isotypic Decomposition of the Space of Flexoelectric Tensors
| ⊗ | |||
| × | × | ||
| × | × | ||
| × | × |
4. Matrix Representations
4.1. Matrix Representations of the Flexoelectric Tensor
4.1.1. -Class
4.1.2. -Class
4.1.3. -Class
4.1.4. -Class
4.1.5. -Class
4.1.6. -Class
4.2. Matrix Representations of the Complete Flexoelectric Law
- Any odd-order tensor vanishes for the centrosymmetric symmetry classes: , ;
- An even-order tensor can not see an odd-order material invariance (in 2D), the invariance seen will be twice the order of the former (see [36] (Theorem 5)).
5. Harmonic Parametrization of the Flexoelectric Tensor
5.1. Decomposition of the State Tensor Space
5.2. The Harmonic Basis
5.3. Explicit Harmonic Decomposition of the Flexoelectric Tensor
5.4. Associated Matrix Representations
6. Discussion
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Kogan, S.M. Piezoelectric effect during inhomogeneous deformation and acoustic scattering of carriers in crystals. Sov. Phys.-Solid State 1964, 5, 2069–2070. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Electrodynamics of Continuous Media; Elsevier: Amsterdam, The Netherlands, 1982; Volume 8. [Google Scholar]
- Royer, D.; Dieulesaint, E. Elastic Waves in Solids II: Generation, Acousto-Optic Interaction, Applications; Springer Science & Business Media: New York, NY, USA, 1999. [Google Scholar]
- Nguyen, T.D.; Mao, S.; Yeh, Y.W.; Purohit, P.K.; McAlpine, M.C. Nanoscale flexoelectricity. Adv. Mater. 2013, 25, 946–974. [Google Scholar] [CrossRef] [PubMed]
- Yudin, P.; Tagantsev, A. Fundamentals of flexoelectricity in solids. Nanotechnology 2013, 24, 432001. [Google Scholar] [CrossRef] [PubMed]
- Sharma, N.D.; Maranganti, R.; Sharma, P. On the possibility of piezoelectric nanocomposites without using piezoelectric materials. J. Mech. Phys. Solids 2007, 55, 2328–2350. [Google Scholar] [CrossRef]
- Eremeyev, V.A.; Ganghoffer, J.F.; Konopińska-Zmysłowska, V.; Uglov, N.S. Flexoelectricity and apparent piezoelectricity of a pantographic micro-bar. Int. J. Eng. Sci. 2020, 149, 103213. [Google Scholar] [CrossRef]
- Chu, B.; Salem, D.R. Flexoelectricity in several thermoplastic and thermosetting polymers. Appl. Phys. Lett. 2012, 101, 103905. [Google Scholar] [CrossRef]
- Deng, Q.; Liu, L.; Sharma, P. Flexoelectricity in soft materials and biological membranes. J. Mech. Phys. Solids 2014, 62, 209–227. [Google Scholar] [CrossRef]
- Vasquez-Sancho, F.; Abdollahi, A.; Damjanovic, D.; Catalan, G. Flexoelectricity in bones. Adv. Mater. 2018, 30, 1705316. [Google Scholar] [CrossRef]
- Sancho, F.N.V. Flexoelectricity in Biomaterials. Ph.D. Thesis, Universitat Autònoma de Barcelona, Barcelona, Spain, 2018. [Google Scholar]
- Nanthakumar, S.S.; Zhuang, X.; Park, H.S.; Rabczuk, T. Topology optimization of flexoelectric structures. J. Mech. Phys. Solids 2017, 105, 217–234. [Google Scholar] [CrossRef]
- Yvonnet, J.; Liu, L.P. A numerical framework for modeling flexoelectricity and Maxwell stress in soft dielectrics at finite strains. Comput. Methods Appl. Mech. Eng. 2017, 313, 450–482. [Google Scholar] [CrossRef]
- Javvaji, B.; He, B.; Zhuang, X. The generation of piezoelectricity and flexoelectricity in graphene by breaking the materials symmetries. Nanotechnology 2018, 29, 225702. [Google Scholar] [CrossRef] [PubMed]
- Huang, S.; Qi, L.; Huang, W.; Shu, L.; Zhou, S.; Jiang, X. Flexoelectricity in dielectrics: Materials, structures and characterizations. J. Adv. Dielectr. 2018, 8, 1830002. [Google Scholar] [CrossRef]
- Ranaivomiarana, N.; Irisarri, F.X.; Bettebghor, D.; Desmorat, B. Concurrent optimization of material spatial distribution and material anisotropy repartition for two-dimensional structures. Contin. Mech. Thermodyn. 2019, 31, 133–146. [Google Scholar] [CrossRef]
- Le Quang, H.; He, Q.C. The number and types of all possible rotational symmetries for flexoelectric tensors. Proc. R. Soc. A Math. Phys. Eng. Sci. 2011, 467, 2369–2386. [Google Scholar] [CrossRef]
- Spencer, A.J.M. A note on the decomposition of tensors into traceless symmetric tensors. Int. J. Eng. Sci. 1970, 8, 475–481. [Google Scholar] [CrossRef]
- Mindlin, R.D. Micro-structure in linear elasticity. Arch. Ration. Mech. Anal. 1964, 16, 51–78. [Google Scholar] [CrossRef]
- Mindlin, R.D.; Eshel, N. On first strain-gradient theories in linear elasticity. Int. J. Solids Struct. 1968, 4, 109–124. [Google Scholar] [CrossRef]
- Auffray, N.; Dirrenberger, J.; Rosi, G. A complete description of bi-dimensional anisotropic strain-gradient elasticity. Int. J. Solids Struct. 2015, 69, 195–206. [Google Scholar] [CrossRef]
- Auffray, N.; Bouchet, R.; Bréchet, Y. Derivation of anisotropic matrix for bi-dimensional strain-gradient elasticity behavior. Int. J. Solids Struct. 2009, 46, 440–454. [Google Scholar] [CrossRef]
- Sahin, E.; Dost, S. A strain-gradients theory of elastic dielectrics with spatial dispersion. Int. J. Eng. Sci. 1988, 26, 1231–1245. [Google Scholar] [CrossRef]
- Bredon, G.E. Introduction to Compact Transformation Groups; Academic Press: Cambridge, MA, USA, 1972; Volume 46. [Google Scholar]
- Zheng, Q.S.; Boehler, J.P. The description, classification, and reality of material and physical symmetries. Acta Mech. 1994, 102, 73–89. [Google Scholar] [CrossRef]
- Forte, S.; Vianello, M. Symmetry classes for elasticity tensors. J. Elast. 1996, 43, 81–108. [Google Scholar] [CrossRef]
- Abud, M.; Sartori, G. The geometry of spontaneous symmetry breaking. Ann. Phys. 1983, 150, 307–372. [Google Scholar] [CrossRef]
- Auffray, N.; Kolev, B.; Olive, M. Handbook of bi-dimensional tensors-Part I: Harmonic decomposition and symmetry classes. Math. Mech. Solids 2017, 22, 1847–1865. [Google Scholar] [CrossRef]
- Vannucci, P. The polar analysis of a third order piezoelectricity-like plane tensor. Int. J. Solids Struct. 2007, 44, 7803–7815. [Google Scholar] [CrossRef]
- Auffray, N.; Desmorat, B. Invariant-Approach To Bidimensional Piezo-Electricity. 2020. in progress. [Google Scholar]
- He, Q.C.; Zheng, Q.S. On the symmetries of 2D elastic and hyperelastic tensors. J. Elast. 1996, 43, 203–225. [Google Scholar] [CrossRef]
- Golubitsky, M.; Stewart, I.; Schaeffer, D. Singularities and groups in bifurcation theory. In Applied Mathematical Sciences; Springer: New York, NY, USA, 1988; Volume II. [Google Scholar]
- Auffray, N. Géométrie des Espaces de Tenseurs, Application à l’élasticité Anisotrope Classique et Généralisée. Habilitation Thesis, Université Paris-Est, Champs-sur-Marne, France, 2017. [Google Scholar]
- Eremeyev, V.A.; Cloud, M.J.; Lebedev, L.P. Applications of Tensor Analysis in Continuum Mechanics; World Scientific: Singapore, 2018. [Google Scholar]
- Auffray, N. Démonstration du théorème d’Hermann à partir de la méthode Forte–Vianello. C. R. Mécanique 2008, 336, 458–463. [Google Scholar] [CrossRef]
- Auffray, N.; Bouchet, R.; Brechet, Y. Class-jump phenomenon for physical symmetries in bi-dimensional space. In Mechanics of Microstructured Solids; Springer: Berlin/Heidelberg, Germany, 2009; pp. 1–11. [Google Scholar]
- Auffray, N.; Desmorat, B.; Abdoul-Anziz, H. Explicit harmonic structure of bidimensional strain-gradient elasticity. 2020. in progress. [Google Scholar]
- Vianello, M. An integrity basis for plane elasticity tensors. Arch. Mech. 1997, 49, 197–208. [Google Scholar]
- Forte, S.; Vianello, M. A unified approach to invariants of plane elasticity tensors. Meccanica 2014, 49, 2001–2012. [Google Scholar] [CrossRef]
- Germain, P. La méthode des puissances virtuelles en mécanique des milieux continus, I. La théorie du second gradient. J. Mécanique 1973, 12, 235–274. [Google Scholar]
- Toupin, R.A. Elastic materials with couple-stresses. Arch. Ration. Mech. Anal. 1962, 11, 385–414. [Google Scholar] [CrossRef]
- Mindlin, R. Influence of couple-stresses on stress concentrations. Exp. Mech. 1963, 3, 1–7. [Google Scholar] [CrossRef]
- Toupin, R.A. Theories of elasticity with couple-stress. Arch. Ration. Mech. Anal. 1964, 17, 85–112. [Google Scholar] [CrossRef]
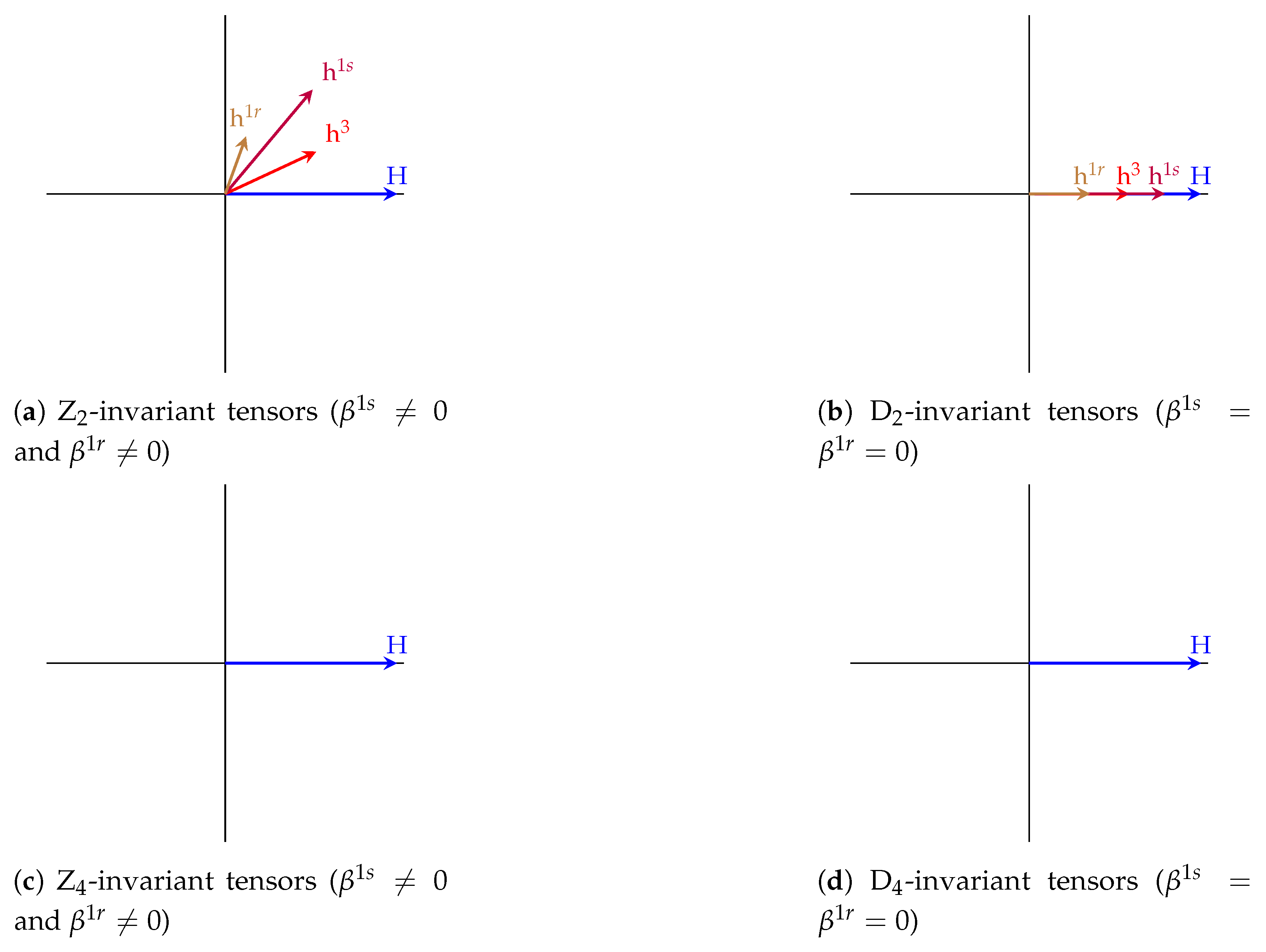
| Name | Digonal | Orthotropic | Tetrachiral | Tetragonal | Hemitropic | Isotropic |
|---|---|---|---|---|---|---|
| #indep () | 6 | 3 | 4 | 2 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdoul-Anziz, H.; Auffray, N.; Desmorat, B. Symmetry Classes and Matrix Representations of the 2D Flexoelectric Law. Symmetry 2020, 12, 674. https://doi.org/10.3390/sym12040674
Abdoul-Anziz H, Auffray N, Desmorat B. Symmetry Classes and Matrix Representations of the 2D Flexoelectric Law. Symmetry. 2020; 12(4):674. https://doi.org/10.3390/sym12040674
Chicago/Turabian StyleAbdoul-Anziz, Houssam, Nicolas Auffray, and Boris Desmorat. 2020. "Symmetry Classes and Matrix Representations of the 2D Flexoelectric Law" Symmetry 12, no. 4: 674. https://doi.org/10.3390/sym12040674
APA StyleAbdoul-Anziz, H., Auffray, N., & Desmorat, B. (2020). Symmetry Classes and Matrix Representations of the 2D Flexoelectric Law. Symmetry, 12(4), 674. https://doi.org/10.3390/sym12040674





