Multiple Lump Novel and Accurate Analytical and Numerical Solutions of the Three-Dimensional Potential Yu–Toda–Sasa–Fukuyama Equation

Abstract

The accuracy of novel lump solutions of the potential form of the three–dimensional potential Yu–Toda–Sasa–Fukuyama (3-Dp-YTSF) equation is investigated. These solutions are obtained by employing the extended simplest equation (ESE) and modified Kudryashov (MKud) schemes to explore its lump and breather wave solutions that characterizes the dynamics of solitons and nonlinear waves in weakly dispersive media, plasma physics, and fluid dynamics. The accuracy of the obtained analytical solutions is investigated through the perspective of numerical and semi-analytical strategies (septic B-spline (SBS) and variational iteration (VI) techniques). Additionally, matching the analytical and numerical solutions is represented along with some distinct types of sketches. The superiority of the MKud is showed as the fourth research paper in our series that has been beginning by Mostafa M. A. Khater and Carlo Cattani with the title “Accuracy of computational schemes”. The functioning of employed schemes appears their effectual and ability to apply to different nonlinear evolution equations.

1. Introduction

Soliton is one of the most well-known properties that characterize the integrability of nonlinear evolution equations [1,2]. Recently, the rogue waves or monster waves, freak waves, abnormal waves have been observed as one of the soliton waves which are an exceptional type of nonlinear waves that are restricted in just one direction and have much significance in a variety of physical branches [3,4]. These waves are first figured out in the deep ocean [5,6]. The applications of this wave appear in the oceanography and optical fibers [7,8,9]. Nowadays, a new branch of the rogue wave is known as a lump wave which is defined by a restricted rogue wave in every direction in space [10,11,12].

Nowadays and based on computer revolution that has a great effect on deriving computational, semi-analytical and numerical schemes, many schemes have derived such as sech-tanh expansion method, auxiliary equation method, direct algebraic equation method, iteration method, exponential expansion method, B-spline schemes, Kudryashov methods, Adomian decomposition method, Khater methods, $\left(\frac{{\phi }^{\prime }\left(\zeta \right)}{\phi \left(\zeta \right)}\right)$-expansion methods and so on [13,14,15,16,17,18,19], the lump solutions of many nonlinear phenomena have been investigated [20,21,22,23,24].

In this context, our paper aims to investigate novel lump solutions of the 3-Dp-YTSF equation through the perspective of ESE and MKud analytical techniques [25,26]. Additionally, The another goal of this manuscript limits to check semi-analytical and numerical solutions of the considered model to explain the accuracy of the obtained analytical solutions and employed analytical schemes [27,28]. The 3-Dp-YTSF equation is given by [29,30,31,32,33,34]

$$\begin{array}{c}\hfill -{\mathcal{Y}}_{xt}+{\mathcal{Y}}_{xxxz}+4{\mathcal{Y}}_x{\mathcal{Y}}_{xz}+2{\mathcal{Y}}_{xx}{\mathcal{Y}}_z+3{\mathcal{Y}}_{yy}=0.\end{array}$$

(1)

where $\mathcal{Y}=\mathcal{Y}(\zeta ,t),\zeta =(x,y,z)$ explains the dynamics of solitons and nonlinear waves. Employing the next wave transformation $\mathcal{Y}=\mathcal{Y}(x,y,z,t)=\mathcal{T}\left(\phi \right)$ where $\phi =\delta +\lambda t,\delta =x+y+z$ converts Equation (1) into the following ordinary differential equation. Integrating the obtained equation once with zero constant of integration, get

$$\begin{array}{c}\hfill (3-\lambda ){\mathcal{T}}^{\prime }+{\mathcal{T}}^{‴}+3{\left({\mathcal{T}}^{\prime }\right)}^2=0.\end{array}$$

(2)

Handling Equation (2) through the perspective of the above-mentioned analytical schemes and the homogeneous balance principles, gives $n=1$. Thus, the general solutions of Equation (2) are evaluated by

$$\begin{array}{c}\hfill \mathcal{T}\left(\phi \right)=\left\{\begin{array}{c}\sum _{i=-n}^n{a}_i\mathcal{C}{\left(\phi \right)}^i=a_1\mathcal{C}\left(\phi \right)+\frac{a_{-1}}{\mathcal{C}\left(\phi \right)}+a_0,\\ \\ \sum _{i=0}^n{a}_i\mathcal{L}{\left(\phi \right)}^i=a_1\mathcal{L}\left(\phi \right)+a_0,\end{array}\right.\end{array}$$

(3)

where $a_0,a_1,a_{-1}$ are arbitrary constants.

The structure of the other sections in this manuscript are given as follows; Section 2 investigates lump solutions of the 3-Dp-YTSF model. Additionally, checking the accuracy of the obtained solution through the above-mentioned semi-analytical and numerical schemes. Section 3 explains the obtained solutions and achieved goals of our research paper. Section 4 gives a summary of the manuscript.

2. Analytical and Numerical Matching for the 3-Dp-YTSF Equation

This section investigates the analytical, semi-analytical and numerical simulations for the 3-Dp-YTSF equation. The headline of this section can be summarized in the following order:

• Applying ESE and MKud schemes to Equation (2) to get lump wave solutions then getting the initial and boundary conditions to investigate semi-analytical and numerical solutions trough the SBS and VI techniques.
• Checking the accuracy of the obtained solutions along calculating the absolute value of error between exact and semi-analytical & numerical solutions.

2.1. ESE Analytical vs. SBS and VI Numerical Techniques along 3-Dp-YTSF Equation

Applying the ESE scheme’s framework and its auxiliary $\left({\mathcal{C}}^{\prime }\left(\phi \right)=h_3\mathcal{C}{\left(\phi \right)}^2+h_2\mathcal{C}\left(\phi \right)+h_1\right)$, where $h_i,i=1,2,3$ are arbitrary constants to be determined later, to Equation (2), obtains the following sets of the above-mentioned parameters:

Set I

$$\begin{array}{c}\hfill \begin{array}{c}\hfill a_{-1}\to 2h_1,a_1\to 0,\lambda \to h_2^2-4h_1{h}_3+3.\end{array}\end{array}$$

Set II

$$\begin{array}{c}\hfill \begin{array}{c}\hfill a_{-1}\to 0,a_1\to -2h_3,\lambda \to h_2^2-4h_1{h}_3+3.\end{array}\end{array}$$

Therefore, the solitary solutions of (2+1)D-3-Dp-YTSF equation are constructed in the following formulas.

For $h_2=0,h_1{h}_3>0$, we get

$$\begin{array}{c}\hfill {\mathcal{Y}}_{\mathrm{I},1}(\zeta ,t)=a_0+2\sqrt{h_1{h}_3}cot\left(\sqrt{h_1{h}_3}\left(\eta +\left(3-4h_1{h}_3\right)t+\delta \right)\right),\end{array}$$

(4)

$$\begin{array}{c}\hfill {\mathcal{Y}}_{\mathrm{I},2}(\zeta ,t)=a_0+2\sqrt{h_1{h}_3}tan\left(\sqrt{h_1{h}_3}\left(\eta +\left(3-4h_1{h}_3\right)t+\delta \right)\right),\end{array}$$

(5)

$$\begin{array}{c}\hfill {\mathcal{Y}}_{\mathrm{II},1}(\zeta ,t)=a_0-2\sqrt{h_1{h}_3}tan\left(\sqrt{h_1{h}_3}\left(\eta +\left(3-4h_1{h}_3\right)t+\delta \right)\right),\end{array}$$

(6)

$$\begin{array}{c}\hfill {\mathcal{Y}}_{\mathrm{II},2}(\zeta ,t)=a_0-2\sqrt{h_1{h}_3}cot\left(\sqrt{h_1{h}_3}\left(\eta +\left(3-4h_1{h}_3\right)t+\delta \right)\right).\end{array}$$

(7)

For $h_2=0,h_1{h}_3<0$, we get

$$\begin{array}{c}\hfill {\mathcal{Y}}_{\mathrm{I},3}(\zeta ,t)=a_0-2\sqrt{-h_1{h}_3}coth\left(\sqrt{-h_1{h}_3}\left(\left(3-4h_1{h}_3\right)t+\delta \right)\mp \frac{log\left(\eta \right)}{2}\right),\end{array}$$

(8)

$$\begin{array}{c}\hfill {\mathcal{Y}}_{\mathrm{I},4}(\zeta ,t)=a_0-2\sqrt{-h_1{h}_3}tanh\left(\sqrt{-h_1{h}_3}\left(\left(3-4h_1{h}_3\right)t+\delta \right)\mp \frac{log\left(\eta \right)}{2}\right),\end{array}$$

(9)

$$\begin{array}{c}\hfill {\mathcal{Y}}_{\mathrm{II},3}(\zeta ,t)=a_0-2\sqrt{-h_1{h}_3}tanh\left(\sqrt{-h_1{h}_3}\left(\left(3-4h_1{h}_3\right)t+\delta \right)\mp \frac{log\left(\eta \right)}{2}\right),\end{array}$$

(10)

$$\begin{array}{c}\hfill {\mathcal{Y}}_{\mathrm{II},4}(\zeta ,t)=a_0-2\sqrt{-h_1{h}_3}coth\left(\sqrt{-h_1{h}_3}\left(\left(3-4h_1{h}_3\right)t+\delta \right)\mp \frac{log\left(\eta \right)}{2}\right).\end{array}$$

(11)

For $h_1=0,h_2>0$, we get

$$\begin{array}{c}\hfill {\mathcal{Y}}_{\mathrm{II},5}(\zeta ,t)=a_0+\frac{2h_2}{h_3{e}^{h_2\left(\eta +\left(h_2^2+3\right)t+\delta \right)}-1}+2h_2.\end{array}$$

(12)

For $h_1=0,h_2<0$, we get

$$\begin{array}{c}\hfill {\mathcal{Y}}_{\mathrm{II},6}(\zeta ,t)=a_0-\frac{2h_3}{h_3{e}^{h_2\left(\eta +\left(h_2^2+3\right)t+\delta \right)}+1}+2h_3.\end{array}$$

(13)

For $4h_1{h}_3>h_2^2$, we get

$$\begin{array}{c}\hfill \begin{array}{c}\hfill {\mathcal{Y}}_{\mathrm{I},5}(\zeta ,t)=a_0-\frac{4h_1{h}_3}{h_2-\sqrt{4h_1{h}_3-h_2^2}tan\left(\frac{1}{2}\sqrt{4h_1{h}_3-h_2^2}\left(\eta +\left(h_2^2-4h_1{h}_3+3\right)t+\delta \right)\right)},\end{array}\end{array}$$

(14)

$$\begin{array}{c}\hfill \begin{array}{c}\hfill {\mathcal{Y}}_{\mathrm{I},6}(\zeta ,t)=a_0-\frac{4h_1{h}_3}{h_2-\sqrt{4h_1{h}_3-h_2^2}cot\left(\frac{1}{2}\sqrt{4h_1{h}_3-h_2^2}\left(\eta +\left(h_2^2-4h_1{h}_3+3\right)t+\delta \right)\right)},\end{array}\end{array}$$

(15)

$$\begin{array}{c}\hfill \begin{array}{c}\hfill {\mathcal{Y}}_{\mathrm{II},7}(\zeta ,t)=a_0-\sqrt{4h_1{h}_3-h_2^2}tan\left(\frac{1}{2}\sqrt{4h_1{h}_3-h_2^2}\left(\eta +\left(h_2^2-4h_1{h}_3+3\right)t+\delta \right)\right)+h_2,\end{array}\end{array}$$

(16)

$$\begin{array}{c}\hfill \begin{array}{c}\hfill {\mathcal{Y}}_{\mathrm{II},8}(\zeta ,t)=a_0+\sqrt{4h_1{h}_3-h_2^2}\left(-cot\left(\frac{1}{2}\sqrt{4h_1{h}_3-h_2^2}\left(\eta +\left(h_2^2-4h_1{h}_3+3\right)t+\delta \right)\right)\right)+h_2.\end{array}\end{array}$$

(17)

Matching between Analytical and Numerical

Here, we employ two recent numerical and semi-analytical schemes to verify the accuracy of our analytical obtained solutions:

• Applying the SBS numerical technique with the following initial condition $\mathcal{Y}(x,0,0,0)=-2tanh\left(x\right)$ gets the following shown analytical and numerical solutions’ values with respect to different values of x in

  Table 1. Analytical and numerical matching through ESE and SBS schemes.

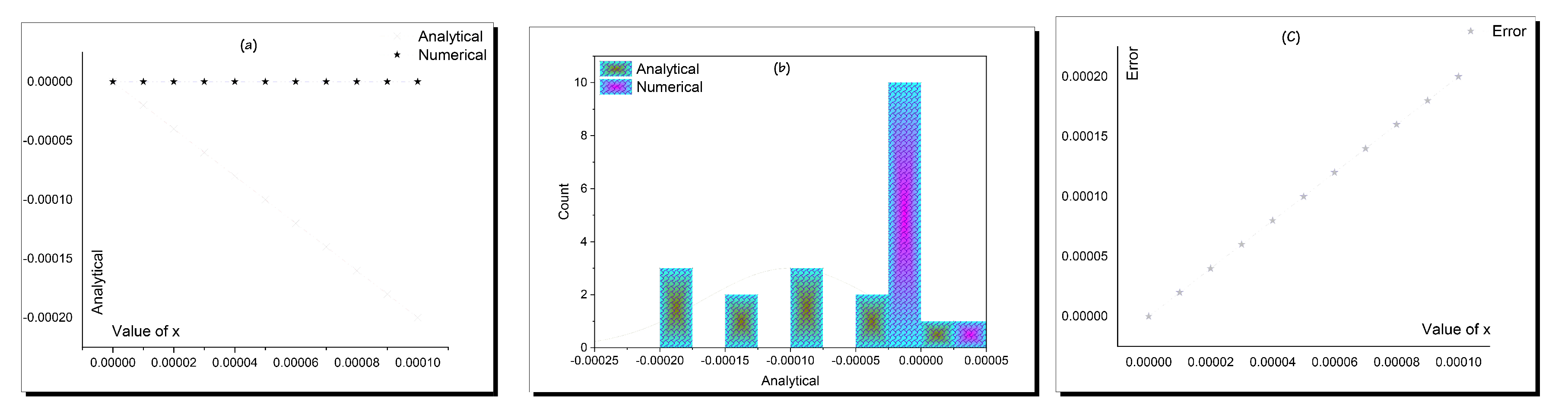
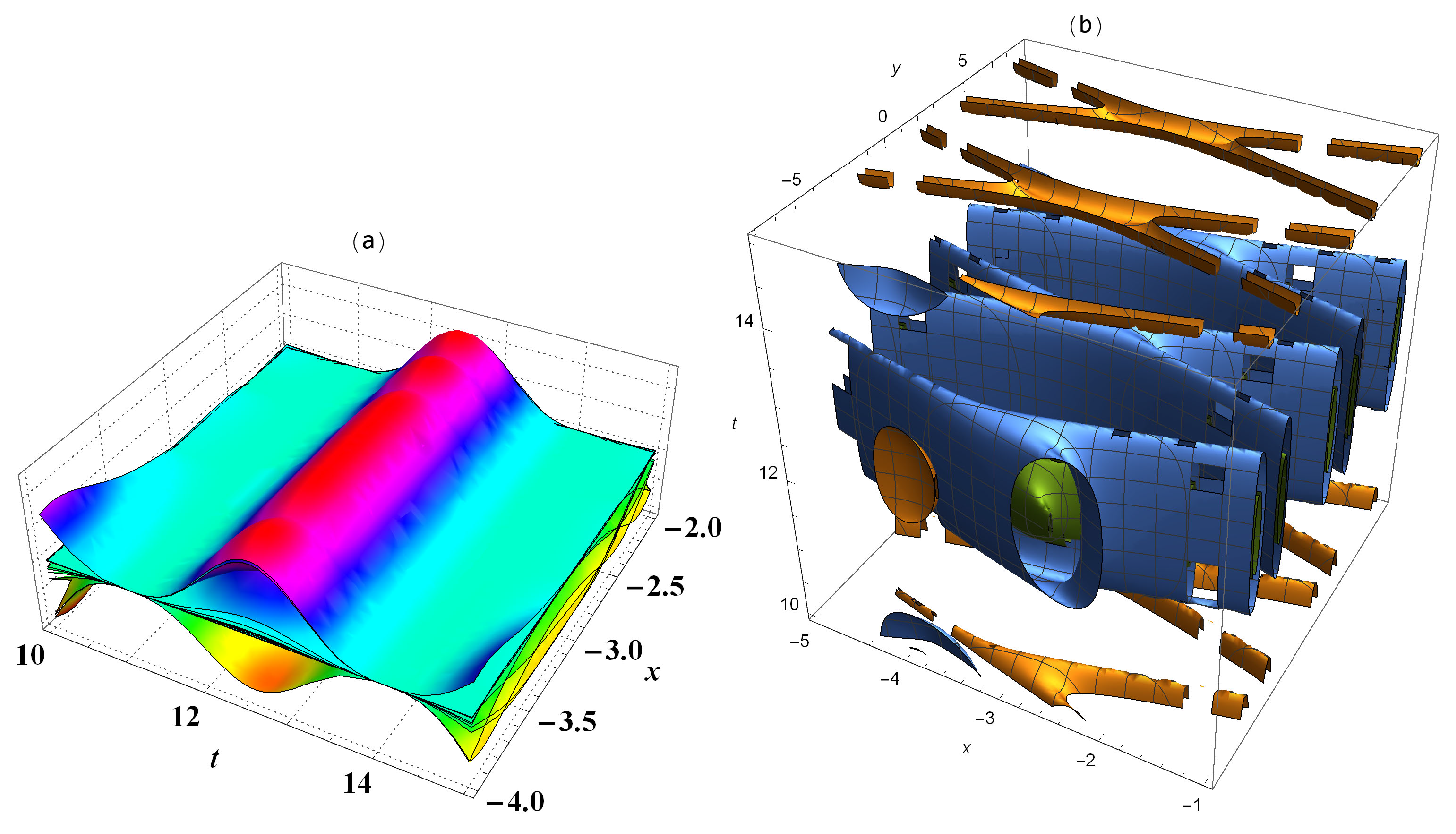
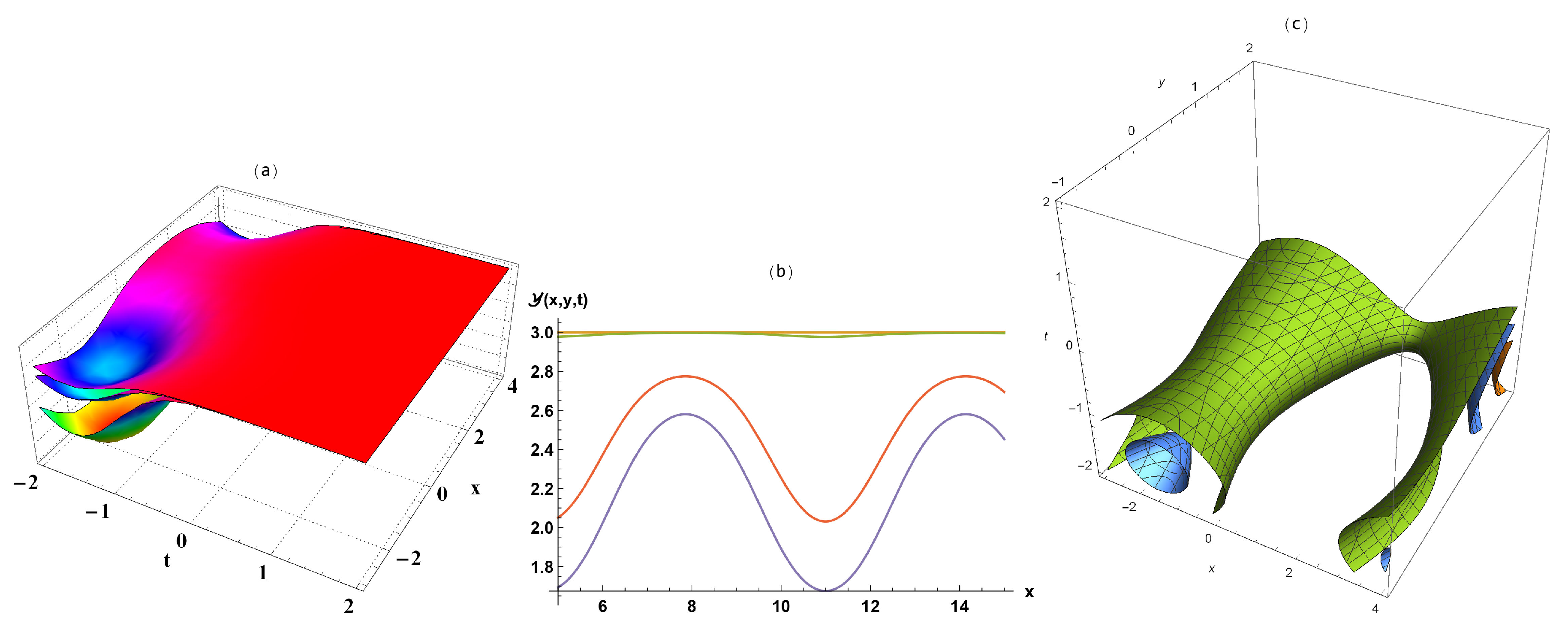
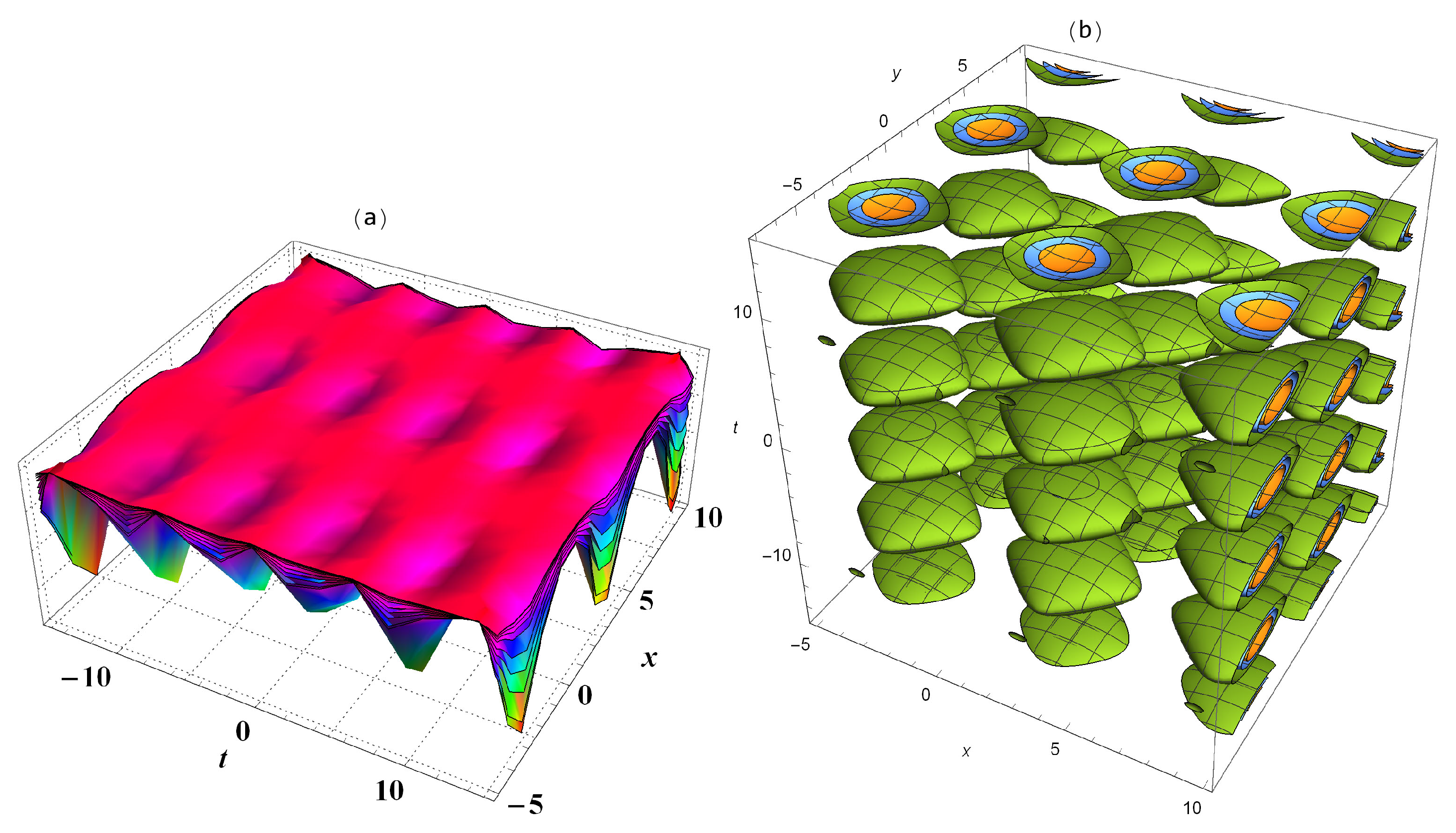
  Value of x	Analytical	Numerical	Absolute Error
  0	0	2.59953 $\times {10}^{-20}$	2.59953 $\times {10}^{-20}$
  0.00001	−0.00002	−4.94237 $\times {10}^{-14}$	0.00002
  0.00002	−0.00004	−6.43149 $\times {10}^{-14}$	0.00004
  0.00003	−0.00006	−1.08723 $\times {10}^{-13}$	0.00006
  0.00004	−0.00008	−1.13736 $\times {10}^{-13}$	0.00008
  0.00005	−0.0001	−1.48758 $\times {10}^{-13}$	0.0001
  0.00006	−0.00012	−1.44334 $\times {10}^{-13}$	0.00012
  0.00007	−0.00014	−1.69924 $\times {10}^{-13}$	0.00014
  0.00008	−0.00016	−1.56069 $\times {10}^{-13}$	0.00016
  0.00009	−0.00018	−1.72219 $\times {10}^{-13}$	0.00018
  0.0001	−0.0002	−1.49012 $\times {10}^{-13}$	−0.0002

• Applying the VI method to Equation (1), gets the following semi-analytical solutions:

  $$\begin{array}{c}\hfill {\mathcal{Y}}_{n+1}={\mathcal{Y}}_n-{\int }_0^t\left(-{\left({\mathcal{Y}}_n\right)}_{xs}+{\left({\mathcal{Y}}_n\right)}_{xxxz}+4{\left({\mathcal{Y}}_n\right)}_x{\left({\mathcal{Y}}_n\right)}_{xz}+2{\left({\mathcal{Y}}_n\right)}_{xx}{\left({\mathcal{Y}}_n\right)}_z+3{\left({\mathcal{Y}}_n\right)}_{yy}\right)ds.\end{array}$$

  (18)
  Using Equation (18)

  $$\begin{array}{c}\hfill {\mathcal{Y}}_0(\zeta ,t)=-2tanh\left(\delta \right),\end{array}$$

  (19)

  $$\begin{array}{c}\hfill {\mathcal{Y}}_1(\zeta ,t)=2tanh\left(\delta \right)\left(48t{\mathrm{sech}}^4\left(\delta \right)-14t{\mathrm{sech}}^2\left(\delta \right)-1\right),\end{array}$$

  (20)

  $$\begin{array}{c}\hfill \begin{array}{cc}\hfill {\mathcal{Y}}_2(\zeta ,t)=& 2tanh\left(\delta \right)\left(4t{\mathrm{sech}}^2\left(\delta \right)\right({\mathrm{sech}}^2\left(\delta \right)(1404t(9-28t)\hfill \\ & \times {\mathrm{sech}}^2\left(\delta \right)+48t{\mathrm{sech}}^4\left(\delta \right)(450t(3-13cosh\left(2\left(\delta \right)\right))\hfill \\ & \times {\mathrm{sech}}^4\left(\delta \right)+5683t-270)+t(1568t-2475)+24)+49t-7)-1).\hfill \end{array}\end{array}$$

  (21)
  Using same steps we can get ${\mathcal{Y}}_i,i=3,4,5,\cdots .$

2.2. Kud Analytical vs. SBS and VI Numerical Techniques along 3-Dp-YTSF Equation

Applying the MKud scheme’s framework and its auxiliary $\left({\mathcal{L}}^{\prime }\left(\phi \right)=ln\left(k\right)\left(\mathcal{L}{\left(\phi \right)}^2-\mathcal{L}\left(\phi \right)\right)\right)$, where k is arbitrary constant to be evaluated later, to Equation (2), obtains the following sets of the above-mentioned parameters:

Set I

$$\begin{array}{c}\hfill a_0=-1,a_1=2,r=2,\mu =-\frac{1}{{log}^2\left(k\right)}.\end{array}$$

Set II

$$\begin{array}{c}\hfill a_0=0,a_1=1,r=\frac{4log\left(k\right)-3}{2log\left(k\right)},\mu =-\frac{1}{4{log}^2\left(k\right)}.\end{array}$$

Set III

$$\begin{array}{c}\hfill a_0=1,a_1=-1,r=\frac{4log\left(k\right)+3}{2log\left(k\right)},\mu =-\frac{1}{4{log}^2\left(k\right)}.\end{array}$$

Therefore, the solitary solutions of 3-Dp-YTSF equation are constructed in the following formulas:

$$\begin{array}{c}\hfill \mathcal{Y}(\zeta ,t)=a_0-\frac{2log\left(k\right)}{1\pm k^{t\left({log}^2\left(k\right)+3\right)+\delta }}.\end{array}$$

(22)

Matching between Analytical, Semi-Analytical and Numerical Solutions

Here, we employ two recent numerical and semi-analytical schemes to verify the accuracy of our analytical obtained solutions:

• Applying the SBS numerical technique with the following initial condition $\mathcal{Y}(x,0,0,0)=tanh\left(\frac{x}{2}\right)$ gets the following shown analytical and numerical solutions’ values with respect to different values of x in

  Table 2. Analytical and numerical matching through MKud and SBS schemes.

  Value of x	Analytical	Numerical	Error
  0	0	2.98907 $\times {10}^{-21}$	2.98907 $\times {10}^{-21}$
  0.00001	5 $\times {10}^{-6}$	1.02496 $\times {10}^{-13}$	5$\times {10}^{-6}$
  0.00002	0.00001	1.31139 $\times {10}^{-13}$	0.00001
  0.00003	0.000015	2.19425 $\times {10}^{-13}$	0.000015
  0.00004	0.00002	2.28909 $\times {10}^{-13}$	0.00002
  0.00005	0.000025	2.98536 $\times {10}^{-13}$	0.000025
  0.00006	0.00003	2.89309 $\times {10}^{-13}$	0.00003
  0.00007	0.000035	3.40231 $\times {10}^{-13}$	0.000035
  0.00008	0.00004	3.12301 $\times {10}^{-13}$	0.00004
  0.00009	0.000045	3.44501 $\times {10}^{-13}$	0.000045
  0.0001	0.00005	2.98023 $\times {10}^{-13}$	0.00005

• Applying VI method along Equation (18) to Equation (1), obtains

  $$\begin{array}{c}\hfill {\mathcal{Y}}_0(\zeta ,t)=tanh\left(\frac{1}{2}\left(\delta \right)\right),\end{array}$$

  (23)

  $$\begin{array}{c}\hfill {\mathcal{Y}}_1(\zeta ,t)=\frac{1}{2}tanh\left(\frac{1}{2}\left(\delta \right)\right){\mathrm{sech}}^2\left(\frac{1}{2}\left(\delta \right)\right)(4t+cosh\left(\delta \right)+1),\end{array}$$

  (24)

  $$\begin{array}{c}\hfill \begin{array}{cc}\hfill {\mathcal{Y}}_2(\zeta ,t)=& \frac{1}{128}tanh\left(\frac{1}{2}\left(\delta \right)\right){\mathrm{sech}}^8\left(\frac{1}{2}\left(\delta \right)\right)(4\left(8t\left(4t^2+6t+3\right)+7\right)\hfill \\ & \times cosh\left(2\left(\delta \right)\right)+32t\left(84t^2+4t+5\right)+8(2t(7t(3-16t)+15)+7)\hfill \\ & \times cosh\left(\delta \right)+8(1-2(t-1\left)t\right)cosh\left(3\right(\delta \left)\right)+cosh\left(4\right(\delta \left)\right)+35),\hfill \end{array}\end{array}$$

  (25)
  Using same steps we can get ${\mathcal{Y}}_i,i=3,4,5,\cdots .$

3. Results’ Interpretation

This section studies the originality and novelty of our research paper. It also shows the accuracy of the obtained analytical solutions. The ESE and MKud computational schemes were applied to the 3-Dp-YTSF equation for constructed solitary wave solutions. Many distinct wave solutions have been obtained, and some of them were demonstrated through some sketches in 2D, 3D, and contour plots to explain the dynamic behaviour of the soliton waves in weakly dispersive media. Comparing our solutions with those that were obtained in previously published papers shows our solutions are completely different from that have been evaluated in [29,30,31,32,33], but some of our solutions are matching the obtained solutions in [34] when $\left(a_0=(A_0+1),h_1{h}_3=\frac{-(3l^2+4c)}{4m},c=\lambda ,\eta =1\right)$ Equation (33) [34] are equal to Equation (9), however the authors in that paper have studied the same model with modified simplest equation method.

The SBS and VI schemes have been applied to the considered model based on the obtained computational solutions. The absolute error between the analytical and numerical solutions was calculated to show the accuracy of the solutions and used methods (Table 1 and Table 2; Figure 1 and Figure 2) while Figure 3 shows lump solitary wave solutions. While Figure 4 represents the matching between analytical and numerical solutions of Equation (1) based on Table 2. Additionally, Figure 5 demonstrates the semi–analytical solutions of Equation (1) in two and three–dimensional plots. This calculating shows the arrogance of the MKud method over the ESE method where its absolute values of error are much smaller than that have been obtained by the ESE method (Figure 6).

4. Conclusions

This research paper successfully handled the nonlinear 3-Dp-YTSF equation arising in fluid dynamics, plasma physics and weakly dispersive media. The ESE, MKud, SBS, and VI schemes were employed to find accurate novel solitary wave solutions of the considered model. Lump wave solutions have been represented in three various types of sketches. The accuracy of the MKud method has been verified over the ESE method where it is our fourth paper in series with title accuracy of the computational schemes. This series aims to determine the accuracy of all computational schemes.