Evolution of EC8 Seismic Design Rules for X Concentric Bracings
Abstract
1. Introduction
2. Ductility Classes and Behavioral Factors
2.1. Current EN 1998-1
2.2. prEN 1998-1-2:2019.3
- qS is the behavior factor component accounting for overstrength due to all other sources;
- qR is the behavior factor component accounting for overstrength due to the redistribution of
- seismic action effects in redundant structures;
- qD is the behavior factor component accounting for the deformation capacity and energy
- dissipation capacity.
3. Overstrength Factors
3.1. Current EN 1998-1
3.2. prEN 1998-1-2:2019.3
4. Design of Dissipative Members
4.1. Current EN 1998-1
4.2. prEN 1998-1-2:2019.3
- Circular hollow sections should verify , where D is the external diameter and t is the thickness of the cross section, γmr is the material randomness coefficient and .
- For rectangular hollow sections, the maximum local slenderness c/t should not be greater than , c being the side width and t the thickness of the cross section.
5. Design of Non-Dissipative Members
5.1. Current EN 1998-1
- Npl,Rd(MEd) is the design resistance to axial force of the beam or column calculated in accordance with EN 1993:1-1 [14], accounting for the interaction with the design value of bending moment;
- MEd, in the seismic design situation;
- NEd,G is the axial force in the beam or in the column due to the non-seismic actions in the seismic design situation;
- NEd,E is the axial force in the beam or in the column due to the design seismic action;
- γov is the material overstrength factor;
- Ω is the minimum overstrength ratio
5.2. prEN 1998-1-2:2019.3
- (i)
- The resistance and stability of both beams and columns should be verified in compression against the following actions:where γrm is the material randomness coefficient (depending on the steel grade); γsh is the factor accounting for hardening of the dissipative zone; and Ωd is the minimum design overstrength .
- (ii)
- The internal forces are calculated by mean of plastic mechanism analysis, namely considering a free-body distribution of axial forces in both tension and compression diagonals with values equal to their expected buckling resistance equal to ;
- (iii)
- The internal forces are calculated by mean of plastic mechanism analysis, considering a free-body distribution of axial forces in which the braces under tension transmit a force equal to and the braces under compression attain their post-buckling resistance equal to .
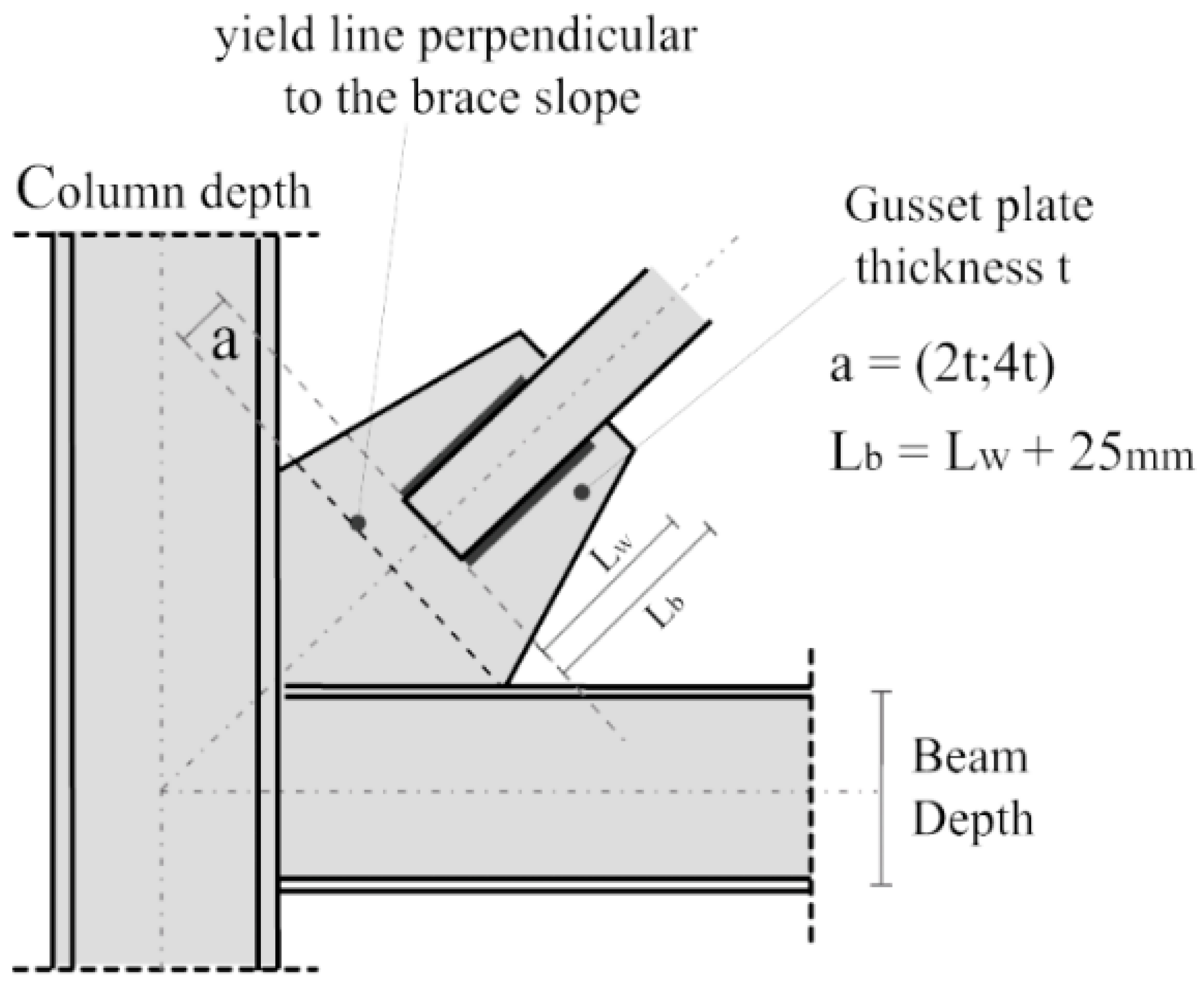
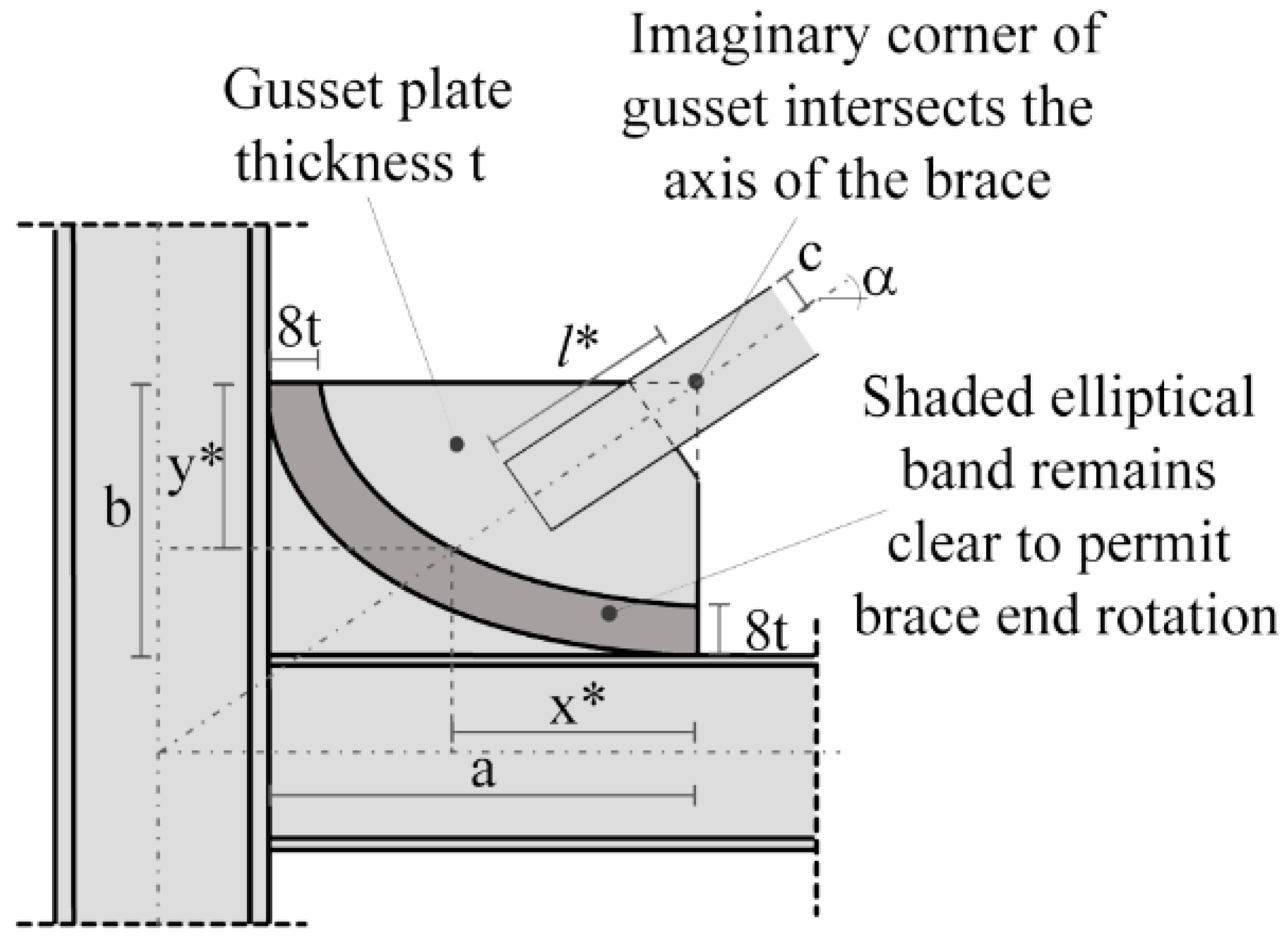
6. Design of Brace-to-Frame Connections
6.1. Current EN 1998-1
6.2. prEN 1998-1-2:2019.3
- Npl,Rd is the design plastic resistance of the brace in tension;
- Nb,Rd is the design buckling resistance of the brace in compression;
- Mpl,Rd is the design plastic moment of the cross section of the brace;
- γrm and γsh are the material randomness and the hardening factors, respectively.
7. Conclusive Remarks
- the use of a tension-only diagonal scheme;
- the requirement of diagonal slenderness;
- the homogeneity condition of the overstrength factor;
- the design of brace-to-frame connections.
Author Contributions
Funding
Conflicts of Interest
References
- CEN. EN 1998-1, Design of Structures for Earthquake Resistance—Part 1: General Rules, Seismic Actions and Rules for Buildings; The European Union Per Regulation: Bruxelles, Belgium, 2005. [Google Scholar]
- Costanzo, S.; D’Aniello, M.; Landolfo, R. Seismic design criteria for chevron CBFs: European vs North American codes (PART-1). J. Constr. Steel Res. 2017, 135, 83–96. [Google Scholar] [CrossRef]
- Costanzo, S.; D’Aniello, M.; Landolfo, R. Seismic design criteria for chevron CBFs: Proposals for the next EC8 (PART-2). J. Constr. Steel Res. 2017, 138C, 17–37. [Google Scholar] [CrossRef]
- Elghazouli, A.Y. Seismic design of steel framed structures to Eurocode 8. Struct. Eng. 2017, 85, 26–31. [Google Scholar]
- Tenchini, A.; D’Aniello, M.; Rebelo, C.; Landolfo, R.; da Silva, L.; Lima, L. High strength steel in chevron concentrically braced frames designed according to Eurocode 8. Eng. Struct. 2016, 124, 167–185. [Google Scholar] [CrossRef]
- Brandonisio, G.; Toreno, M.; Grande, E.; Mele, E.; De Luca, A. Seismic design of concentric braced frames. J. Constr. Steel Res. 2012, 78, 22–37. [Google Scholar] [CrossRef]
- Bosco, M.; Brandonisio, G.; Marino, E.M.; Mele, E.; De Luca, A. Ω* method: An alternative to Eurocode 8 procedure for seismic design of X-CBFs. J. Constr. Steel Res. 2017, 134, 135–147. [Google Scholar] [CrossRef]
- Marino, E.M. A unified approach for the design of high ductility steel frames with concentric braces in the framework of Eurocode. Earthq. Eng Struct. Dyn. 2013, 43, 97–118. [Google Scholar] [CrossRef]
- Marino, E.M.; Nakashima, M. Seismic performance and new design procedure for chevron-braced frames. Earthquake Eng. Struct. Dyn. 2016, 35, 433–452. [Google Scholar] [CrossRef]
- Costanzo, S.; D’Aniello, M.; Landolfo, R. Proposal of design rules for ductile X-CBFS in the framework of EUROCODE 8. Earthq. Eng. Struct. Dyn. 2019, 48, 124–151. [Google Scholar] [CrossRef]
- Longo, A.; Montuori, R.; Piluso, V. Plastic design of V-braced frames. J. Earthq. Eng. 2008, 12, 1246–1266. [Google Scholar] [CrossRef]
- Freddi, F.; Tubaldi, E.; Zona, A.; Dall’Asta, A. Seismic performance of dual systems coupling moment-resisting and buckling-restrained braced frames. Earthq. Eng. Struct. Dyn. 2020, 1–25. [Google Scholar] [CrossRef]
- Morfuni, F.; Freddi, F.; Galasso, C. Seismic performance of dual systems with BRBs under mainshock-aftershock sequences. In Proceedings of the 13th International Conference on Applications of Statistics and Probability in Civil Engineering ICASP, Seoul, Korea, 26–30 May 2019. [Google Scholar]
- Kotoky, N.; Freddi, F.; Ghosh, J.; Raghunandan, M. BRBs uncertainty propagation in seismic retrofit of RC structures. In Proceedings of the 13th International Conference on Applications of Statistics and Probability in Civil Engineering ICASP, Seoul, Korea, 26–30 May 2019. [Google Scholar]
- Qie, Y.; Barbagallo, F.; Marino, E.M.; Du, C.; Wang, T. Full-scale hybrid test for realistic verification of a seismic upgrading technique of RC frames by BRBs. Earthq. Eng. Struct. Dyn. 2020, 49, 1452–1472. [Google Scholar] [CrossRef]
- Barbagallo, F.; Bosco, M.; Marino, E.M.; Rossi, P.P. Seismic design and performance of dual structures with BRBs and semi-rigid connections. J. Constr. Steel Res. 2019, 158, 306–316. [Google Scholar] [CrossRef]
- Barbagallo, F.; Bosco, M.; Marino, E.M.; Rossi, P.P. Achieving a more effective concentric braced frame by the double-stage yield BRB. Eng. Struct. 2019, 186, 484–497. [Google Scholar] [CrossRef]
- Barbagallo, F.; Bosco, M.; Marino, E.M.; Rossi, P.P. Behavior factor of dual systems with BRBs and semi-rigid connections. Key Eng. Mater. 2018, 763, 949–956. [Google Scholar] [CrossRef]
- Bosco, M.; Ghersi, A.; Marino, E.M.; Rossi, P.P. Generalized corrective eccentricities for nonlinear static analysis of buildings with framed or braced structure. Bull. Earthq. Eng. 2017, 15, 4887–4913. [Google Scholar] [CrossRef]
- Montuori, R.; Nastri, E.; Piluso, V.; Todisco, P. A simplified performance based approach for the evaluation of seismic performances of steel frames. Eng. Struct. 2020, 224, 111222. [Google Scholar] [CrossRef]
- Dell’Aglio, G.; Montuori, R.; Nastri, E.; Piluso, V. Consideration of second-order effects on plastic design of steel moment resisting frames. Bull. Earthq. Eng. 2019, 17, 3041–3070. [Google Scholar] [CrossRef]
- Montuori, R.; Piluso, V.; Streppone, S. Design and seismic assessment of MRFS and dual CBFS equipped with friction dampers. Compdyn Proc. 2019, 3, 4213–4225. [Google Scholar]
- Wang, Y.; Nastri, E.; Tirca, L.; Montuori, R.; Piluso, V. Comparative response of earthquake resistant CBF buildings designed according to Canadian and European code provisions. Key Eng. Mater. 2018, 763, 1155–1163. [Google Scholar] [CrossRef]
- Dell’Aglio, G.; Montuori, R.; Nastri, E.; Piluso, V. A critical review of plastic design approaches for failure mode control of steel moment resisting frames. Ing. Sismica 2017, 34, 82–102. [Google Scholar]
- Montuori, R.; Nastri, E.; Piluso, V. Influence of the bracing scheme on seismic performances of MRF-EBF dual systems. J. Constr. Steel Res. 2017, 132, 179–190. [Google Scholar] [CrossRef]
- Longo, A.; Montuori, R.; Piluso, V. Moment frames—Concentrically braced frames dual systems: Analysis of different design criteria. Struct. Infrastruct. Eng. 2016, 12, 122–141. [Google Scholar] [CrossRef]
- Longo, A.; Montuori, R.; Piluso, V. Seismic design of chevron braces cupled with MRF fail safe systems. Earthq. Struct. 2015, 8, 1215–1239. [Google Scholar] [CrossRef]
- Elghazouli, A.Y.; Castro, J.M.; Izzuddin, B.A. Seismic performance of composite moment-resisting frames. Eng. Struct. 2008, 30, 1802–1819. [Google Scholar] [CrossRef]
- Araújo, M.; Castro, J.M. On the quantification of local deformation demands and adequacy of linear analysis procedures for the seismic assessment of existing steel buildings to EC8-3. Bull. Earthq. Eng. 2016, 14, 1613–1642. [Google Scholar] [CrossRef]
- Peres, R.; Castro, J.M.; Bento, R. An extension of an improved forced based design procedure for 3D steel structures. Steel Compos. Struct. 2016, 22, 1115–1140. [Google Scholar] [CrossRef]
- Araújo, M.; Castro, J.M. Simplified procedure for the estimation of local inelastic deformation demands for seismic performance assessment of buildings. Earthq. Eng. Struct. Dyn. 2017, 46, 491–514. [Google Scholar] [CrossRef]
- Silva, A.; Santos, L.; Ribeiro, T.; Castro, J.M. Improved Seismic Design of Concentrically X-Braced Steel Frames to Eurocode 8. J. Earthq. Eng. 2018. [Google Scholar] [CrossRef]
- Macedo, L.; Silva, A.; Castro, J.M. From design to earthquake loss assessment of steel moment-resisting frames. Key Eng. Mater. 2018, 763, 124–130. [Google Scholar] [CrossRef]
- Araújo, M.; Castro, J.M. A Critical Review of European and American Provisions for the Seismic Assessment of Existing Steel Moment-Resisting Frame Buildings. J. Earthq. Eng. 2018, 22, 1336–1364. [Google Scholar] [CrossRef]
- Silva, A.; Castro, J.M.; Monteiro, R. On the importance of brace connection modelling for seismic performance assessment of steel CBFs. Compdyn Proc. 2019, 3, 4907–4915. [Google Scholar]
- Macedo, L.; Silva, A.; Castro, J.M. A more rational selection of the behaviour factor for seismic design according to Eurocode 8. Eng. Struct. 2019, 188, 69–86. [Google Scholar] [CrossRef]
- Silva, A.; Castro, J.M.; Monteiro, R. Practical considerations on the design of concentrically-braced steel frames to Eurocode 8. J. Constr. Steel Res. 2019, 158, 71–85. [Google Scholar] [CrossRef]
- Macedo, L.; Castro, J.M. Earthquake loss assessment of steel moment-resisting frames designed according to Eurocode 8. Soil Dyn. Earthq. Eng. 2019, 124, 58–71. [Google Scholar] [CrossRef]
- Silva, A.; Castro, J.M.; Monteiro, R. Implications of modelling simplifications on the seismic performance of concentrically-braced steel framed buildings. In Proceedings of the 9th International Conference on Advances in Steel Structures, ICASS, Hong Kong, China, 5–7 December 2018. [Google Scholar]
- Silva, A.; Macedo, L.; Monteiro, R.; Castro, J.M. Earthquake-induced loss assessment of steel buildings designed to Eurocode 8. Eng. Struct. 2020, 208, 110244. [Google Scholar] [CrossRef]
- Silva, A.; Castro, J.M.; Monteiro, R. A rational approach to the conversion of FEMA P-58 seismic repair costs to Europe. Earthq. Spectra 2020, 36, 1607–1618. [Google Scholar] [CrossRef]
- Silva, A.; Castro, J.M.; Monteiro, R. Brace-to-frame connection modelling effects on seismic loss assessment of steel concentrically-braced frames. J. Constr. Steel Res. 2020, 172, 106230. [Google Scholar] [CrossRef]
- Di Sarno, L.; Elnashai, A.S.; Nethercot, D.A. Seismic response of stainless steel braced frames. In Proceedings of the International Colloquium on Stability and Ductility of Steel Structures, SDSS 2006, Lisbon, Portugal, 6–8 September 2006. [Google Scholar]
- Di Sarno, L.; Elnashai, A.S. Seismic performance of retrofitted steel moment resisting frames. High Performance. Struct. Mater. 2004, 7, 643–652. [Google Scholar]
- Di Sarno, L.; Elnashai, A.S.; Nethercot, D.A. Seismic performance assessment of stainless steel frames. J. Constr. Steel Res. 2003, 59, 1289–1319. [Google Scholar] [CrossRef]
- Di Sarno, L.; Elnashai, A.S.; Nethercot, D.A. Seismic retrofitting of framed structures with stainless steel. J. Constr. Steel Res. 2006, 62, 93–104. [Google Scholar] [CrossRef]
- Di Sarno, L. Bracing systems for seismic retrofitting of steel frames. In Proceedings of the 5th International Conference on Behaviour of Steel Structures in Seismic Areas—Stessa, Yokohama, Japan, 17 July 2006; pp. 821–826. [Google Scholar]
- Di Sarno, L.; Elnashai, A.S.; Nethercot, D.A. Seismic response of stainless steel braced frames. J. Constr. Steel Res. 2008, 64, 914–925. [Google Scholar] [CrossRef]
- Di Sarno, L.; Elnashai, A.S. Bracing systems for seismic retrofitting of steel frames. J. Constr. Steel Res. 2009, 65, 452–465. [Google Scholar] [CrossRef]
- Di Sarno, L. Ductile response of composite steel and concrete frames. In Proceedings of the SDSS’ Rio 2010: International Colloquium Stability and Ductility of Steel Structures, Janeiro, Brazil, 8–10 September 2010; Volume 2, pp. 831–838. [Google Scholar]
- Di Sarno, L.; Paolacci, F.; Sextos, A.G. Seismic performance assessment of existing steel buildings: A case study. Key Eng. Mater. 2018, 763, 1067–1076. [Google Scholar] [CrossRef]
- Di Sarno, L.; Wu, J.-R. Seismic assessment of existing steel frames with masonry infills. J. Constr. Steel Res. 2020, 169, 106040. [Google Scholar] [CrossRef]
- Pnevmatikos, N.G.; Papagiannopoulos, G.A.; Papavasileiou, G.S. Fragility curves for mixed concrete/steel frames subjected to seismic excitation. Soil Dyn. Earthq. Eng. 2019, 116, 709–713. [Google Scholar] [CrossRef]
- Papavasileiou, G.S.; Pnevmatikos, N.G. The seismic performance of steel buildings retrofitted with steel cables against progressive collapse. Compdyn Proc. 2019, 2, 2167–2173. [Google Scholar]
- Papavasileiou, G.S.; Charmpis, D.C. Earthquake-resistant buildings with steel or composite columns: Comparative assessment using structural optimization. J. Build. Eng. 2020, 27, 100988. [Google Scholar] [CrossRef]
- Papavasileiou, G.S.; Charmpis, D.C.; Lagaros, N.D. Optimized seismic retrofit of steel-concrete composite buildings. Eng. Struct. 2020, 213, 110573. [Google Scholar] [CrossRef]
- Balazadeh-Minouei, Y.; Tremblay, R.; Koboevic, S. Seismic Retrofit of an Existing 10-Story Chevron-Braced Steel-Frame. J. Struct. Eng. 2018, 144, 04018180. [Google Scholar] [CrossRef]
- Balazadeh-Minouei, Y.; Koboevic, S.; Tremblay, R. Seismic Assessment of Existing Steel Chevron Braced Frames. J. Struct. Eng. 2018, 144, 040180461. [Google Scholar] [CrossRef]
- Balazadeh-Minouei, Y.; Koboevic, S.; Tremblay, R. Seismic evaluation of a steel braced frame using NBCC and ASCE 41. J. Constr. Steel Res. 2017, 135, 110–124. [Google Scholar] [CrossRef]
- Toutant, G.; Minouei, Y.B.; Imanpour, A.; Koboevic, S.; Tremblay, R. Stability of steel columns in steel concentrically braced frames subjected to seismic loading. In Structures Congress 2017: Buildings and Special Structures—Selected Papers from the Structures Congress; ASCE: Denver, CO, USA, 2017; pp. 143–154. [Google Scholar]
- Imanpour, A.; Tremblay, R.; Davaran, A.; Stoakes, C.; Fahnestock, L.A. Seismic Performance Assessment of Multitiered Steel Concentrically Braced Frames Designed in Accordance with the 2010 AISC Seismic Provisions. J. Struct. Eng. 2016, 142, 04016135. [Google Scholar] [CrossRef]
- Imanpour, A.; Auger, K.; Tremblay, R. Seismic design and performance of multi-tiered steel braced frames including the contribution from gravity columns under in-plane seismic demand. Adv. Eng. Softw. 2016, 101, 106–122. [Google Scholar] [CrossRef]
- Imanpour, A.; Tremblay, R. Seismic design and response of steel multi-tiered concentrically braced frames in Canada. Can. J. Civ. Eng. 2016, 43, 908–919. [Google Scholar] [CrossRef]
- Tirca, L.; Chen, L.; Tremblay, R. Assessing collapse safety of CBF buildings subjected to crustal and subduction earthquakes. J. Constr. Steel Res. 2015, 115, 4287. [Google Scholar] [CrossRef]
- Davaran, A.; Gélinas, A.; Tremblay, R. Inelastic Buckling Analysis of Steel X-Bracing with Bolted Single Shear Lap Connections. J. Struct. Eng. 2015, 141, 04014204. [Google Scholar] [CrossRef]
- Sizemore, J.; Davaran, A.; Fahnestock, L.; Tremblay, R.; Hines, E. Seismic behavior of low-ductility concentrically-braced frames. In Proceedings of the 2014 Structures Congress, Boston, MA, USA, 3–5 April 2014; pp. 2369–2380. [Google Scholar]
- Davaran, A.; Tremblay, R.; Beland, T.; Fahnestock, L.A.; Hines, E.M. Experimental behavior of low-ductility brace connection limit states. In Proceedings of the 2014 Structures Congress, Boston, MA, USA, 3–5 April 2014; pp. 2429–2441. [Google Scholar]
- Gélinas, A.; Tremblay, R.; Davaran, A. Buckling response of bolted mid-connections in steel X-bracing. In Proceedings of the Annual Conference—Canadian Society for Civil Engineering, Montreal, QC, Canada, 29 May–1 June 2013; Volume 3, pp. 2448–2457. [Google Scholar]
- Tremblay, R.; Davaran, A.; Gélinas, A. Stability of x-bracing systems with traditional bolted connections. Structures congress 2013: Bridging your passion with your profession. In Proceedings of the 2013 Structures Congress, Pittsburgh, PA, USA, 2–4 May 2013; pp. 2662–2674. [Google Scholar]
- Gélinas, A.; Tremblay, R.; Davaran, A. Seismic behaviour of steel HSS X-bracing of the conventional construction category. In Proceedings of the 2012 Structures Congress, Chicago, IL, USA, 29–31 March 2012; pp. 1649–1660. [Google Scholar]
- Agüero, A.; Izvemari, C.; Tremblay, R. Modeling of the seismic response of concentrically braced steel frames using the Opensees analysis environment. Adv. Steel Constr. 2006, 2, 242–274. [Google Scholar]
- Lacerte, M.; Tremblay, R. Making use of brace overstrength to improve the seismic response of multistorey split-X concentrically braced steel frames. Can. J. Civ. Eng. 2006, 33, 1005–1021. [Google Scholar] [CrossRef]
- Tremblay, R.; Poncet, L. Seismic performance of concentrically braced steel frames in multistory buildings with mass irregularity. J. Struct. Eng. 2005, 131, 1363–1375. [Google Scholar] [CrossRef]
- Tremblay, R. Fundamental periods of vibration of braced steel frames for seismic design. Earthq. Spectra 2005, 21, 833–860. [Google Scholar] [CrossRef]
- Tremblay, R.; Poncet, L. Improving the seismic stability of concentrically braced steel frames. In Proceedings of the Annual Stability Conference, Structural Stability Research Council, Long Beach, CA, USA, 24–27 March 2004; pp. 19–38. [Google Scholar]
- Tremblay, R.; Archambault, M.-H.; Filiatrault, A. Seismic response of concentrically braced steel frames made with rectangular hollow bracing members. J. Struct. Eng. 2003, 129, 1626–1636. [Google Scholar] [CrossRef]
- Tremblay, R. Achieving a stable inelastic seismic response for multi-story concentrically braced steel frames. Eng. J. 2003, 40, 111–129. [Google Scholar]
- Tremblay, R. Inelastic seismic response of steel bracing members. J. Constr. Steel Res. 2002, 58, 665–701. [Google Scholar] [CrossRef]
- Tremblay, R. Seismic behavior and design of concentrically braced steel frames. Eng. J. 2001, 38, 148–166. [Google Scholar]
- Tremblay, R.; Robert, N. Seismic performance of low- and medium-rise chevron braced steel frames. Can. J. Civ. Eng. 2001, 28, 699–714. [Google Scholar] [CrossRef]
- Filiatrault, A.; Tremblay, R. Design of Tension-Only Concentrically Braced Steel Frames for seismic induced impact loading. Eng. Struct. 1998, 20, 1087–1096. [Google Scholar] [CrossRef]
- Tremblay, R.; Filiatrault, A. Seismic impact loading in inelastic tension-only concentrically braced steel frames: Myth or reality? Earthq. Eng Struct. Dyn. 1996, 25, 1373–1389. [Google Scholar] [CrossRef]
- Tirca, L.; Serban, O.; Lin, L.; Wang, M.; Lin, N. Improving the Seismic Resilience of Existing Braced-Frame Office Buildings. J. Struct. Eng. 2016, 142, 4015003. [Google Scholar] [CrossRef]
- Tirca, L.; Chen, L. Numerical simulation of inelastic cyclic response of HSS braces upon fracture. Adv. Steel Constr. 2014, 10, 442–462. [Google Scholar]
- Tirca, L.; Chen, L. The influence of lateral load patterns on the seismic design of zipper braced frames. Eng. Struct. 2012, 40, 536–555. [Google Scholar] [CrossRef]
- Sizemore, J.G.; Fahnestock, L.A.; Hines, E.M. Seismic Performance Assessment of Low-Ductility Concentrically Braced Frames. J. Struct. Eng. 2019, 145, 04019016. [Google Scholar] [CrossRef]
- Grabner, K.D.M.; Fahnestock, L.A. Seismic stability of special concentrically braced frames in a moderate seismic region. In Proceedings of the Structural Stability Research Council Annual Stability Conference, Louis, MI, USA, 2–5 April 2019; Volume 1, pp. 307–320. [Google Scholar]
- Sizemore, J.G.; Fahnestock, L.A.; Hines, E.M.; Bradley, C.R. Parametric Study of Low-Ductility Concentrically Braced Frames under Cyclic Static Loading. J. Struct. Eng. 2017, 143, 04017032. [Google Scholar] [CrossRef]
- Bradley, C.R.; Fahnestock, L.A.; Hines, E.M.; Sizemore, J.G. Full-Scale Cyclic Testing of Low-Ductility Concentrically Braced Frames. J. Struct. Eng. 2017, 143, 04017029. [Google Scholar] [CrossRef]
- Stoakes, C.D.; Fahnestock, L.A. Cyclic flexural testing of concentrically braced frame beam-column connections. J. Struct. Eng. 2011, 137, 739–747. [Google Scholar] [CrossRef]
- Landolfo, R.; Mazzolani, F.M.M.; Dubina, D.; da Silva, L.S.; D’Aniello, M. Design of Steel Structures for Buildings in Seismic Areas: Eurocode 8: Design of Structures for Earthquake Resistance. Part 1-1—General RULES, Seismic Actions and Rules for Buildings, 1st ed.; Wilhelm Ernst & Sohn Verlag für Architektur und Technische Wissenschaften GmbH & Co. KG: Berlin, Germany; WILEY: Hoboken, NJ, USA, 2017; ISBN 978-3-433-03010-3. [Google Scholar]
- CEN. CEN prEN 1998-1-2: Draft; 2019. In Eurocode 8: Design of Structures for Earthquake Resistance—Part 1-2: Rules for New Buildings; CEN: Bruxelles, Belgium; The European Union Per Regulation: Brussel, Belgium, 2019. [Google Scholar]
- CEN. EN 1993:1-1, Eurocode 3: Design of Steel Structures—Part 1-1: General Rules and Rules for Buildings; CEN: Bruxelles, Belgium; The European Union Per Regulation: Brussel, Belgium, 2005. [Google Scholar]
- Latour, M.; Rizzano, G. Design of X-shaped double split tee joints accounting for moment-shear interaction. J. Constr. Steel Res. 2015, 104, 115–126. [Google Scholar] [CrossRef]
- Francavilla, A.B.; Latour, M.; Piluso, V.; Rizzano, G. Design of full-strength full-ductility extended end-plate beam-to-column joints. J. Constr. Steel Res. 2018, 148, 77–96. [Google Scholar] [CrossRef]
- Francavilla, A.B.; Latour, M.; Piluso, V.; Rizzano, G. Design criteria for beam-to-column connections equipped with friction devices. J. Constr. Steel Res. 2020, 172, 106240. [Google Scholar] [CrossRef]
- Ferrante Cavallaro, G.; Francavilla, A.B.; Latour, M.; Piluso, V.; Rizzano, G. Cyclic response of low yielding connections using different friction materials. Soil Dyn. Earthq. Eng. 2018, 114, 404–423. [Google Scholar] [CrossRef]
- Lemos, A.; da Silva, L.S.; Latour, M.; Rizzano, G. Numerical modelling of innovative DST steel joint under cyclic loading. Arch. Civ. Mech. Eng. 2018, 18, 687–701. [Google Scholar] [CrossRef]
- Chisari, C.; Francavilla, A.B.; Latour, M.; Piluso, V.; Rizzano, G.; Amadio, C. Critical issues in parameter calibration of cyclic models for steel members. Eng. Struct. 2017, 132, 123–138. [Google Scholar] [CrossRef]
- Latour, M.; Rizzano, G. Mechanical modelling of exposed column base plate joints under cyclic loads. J. Constr. Steel Res. 2019, 162, 105726. [Google Scholar] [CrossRef]
- Iannone, F.; Latour, M.; Piluso, V.; Rizzano, G. Experimental analysis of bolted steel beam-to-column connections: Component identification. J. Earthq. Eng. 2011, 15, 214–244. [Google Scholar] [CrossRef]
- Latour, M.; Rizzano, G. Experimental behavior and mechanical modeling of dissipative T-stub connections. J. Struct. Eng. 2012, 138, 170–182. [Google Scholar] [CrossRef]
- Francavilla, A.B.; Latour, M.; Piluso, V.; Rizzano, G.; Jaspart, J.P.; Demonceau, J.F. On the Robustness of Earthquake-Resistant Moment-Resistant Frames: Influence of Innovative Beam-to-Column Joints. Open Constr. Build. Technol. J. 2018, 12, 101–111. [Google Scholar] [CrossRef]
- Di Benedetto, S.; Latour, M.; Rizzano, G. Stiffness prediction of connections between CHS tubes and externally welded I-beams: Fe analyses and analytical study. Materials 2020, 13, 3030. [Google Scholar] [CrossRef]
- Di Benedetto, S.; Latour, M.; Rizzano, G. Chord failure resistance of 3D cut welded connections with CHS columns and through I-BEAMS. Thin Walled Struct. 2020, 154, 106821. [Google Scholar] [CrossRef]
- Di Benedetto, S.; Latour, M.; Rizzano, G. Assessment of the stiffness of 3D cut welded connections with CHS columns and through I-BEAMS. Structures 2020, 27, 247–258. [Google Scholar] [CrossRef]
- Poursadrollah, A.; D’Aniello, M.; Landolfo, R.; De Martino, A. Preliminary study on the seismic performance of hybrid steel structures with truss lightweight girders and plug-and-play connections. Ing. Sismica Int. J. Earthq. Eng. 2020, 37, 103–113. [Google Scholar]
- Santos, A.F.; Santiago, A.; Latour, M.; Rizzano, G.; da Silva, L.S. Response of friction joints under different velocity rates. J. Constr. Steel Res. 2020, 168, 106004. [Google Scholar] [CrossRef]
- Di Benedetto, S.; Francavilla, A.B.; Latour, M.; Ferrante Cavallaro, G.; Piluso, V.; Rizzano, G. Pseudo-dynamic testing of a full-scale two-storey steel building with RBS connections. Eng. Struct. 2020, 212, 110494. [Google Scholar] [CrossRef]
- Di Lorenzo, G.; Formisano, A.; Landolfo, R.; Mazzolani, F.M.; Terracciano, G. On the use of cold-formed thin walled members for vertical addition of existing masonry buildings. In Proceedings of the SDSS’ Rio 2010: International Colloquium Stability and Ductility of Steel Structures, Janeiro, Brazil, 8–10 September 2010; Volume 2, pp. 945–952. [Google Scholar]
- Di Lorenzo, G.; Formisano, A.; Landolfo, R. On the origin of I beams and quick analysis on the structural efficiency of hot-rolled steel members. Open Civ. Eng. J. 2017, M3, 332–344. [Google Scholar] [CrossRef]
- Formisano, A.; Di Lorenzo, G.; Iannuzzi, I.; Landolfo, R. Seismic vulnerability and fragility of existing Italian industrial steel buildings. Open Civ. Eng. J. 2017, 11, 1122–1137. [Google Scholar] [CrossRef]
- Rizzo, F.; Di Lorenzo, G.; Formisano, A.; Landolfo, R. Time-Dependent Corrosion Wastage Model for Wrought Iron Structures. J. Mater. Civ. 2019, 31, 4019165. [Google Scholar] [CrossRef]
- Di Lorenzo, G.; Babilio, E.; Formisano, A.; Landolfo, R. Innovative steel 3D trusses for preservating archaeological sites: Design and preliminary results. J. Constr. Steel Res. 2019, 154, 250–326. [Google Scholar] [CrossRef]
- Tartaglia, R.; D’Aniello, M. Nonlinear Performance of Extended Stiffened End Plate Bolted Beam-to-column Joints Subjected to Column Removal. Open Civ. Eng. J. 2017, 11, 369–383. [Google Scholar] [CrossRef]
- Tartaglia, R.; D’Aniello, M.; De Martino, A. Ultimate performance of external end-plate bolted joints under column loss scenario accounting for the influence of the transverse beam. Open Constr. Build. Technol. J. 2017, 12, 132–139. [Google Scholar] [CrossRef]
- Tartaglia, R.; D’Aniello, M.; Rassati, G.A. Proposal of AISC-compliant seismic design criteria for ductile partially-restrained end-plate bolted joints. J. Constr. Steel Res. 2019, 159, 364–383. [Google Scholar] [CrossRef]
- Tartaglia, R.; D’Aniello, M.; Zimbru, M. Experimental and numerical study on the T-Stub behaviour with preloaded bolts under large deformations. Structures 2020, 27, 2137–2155. [Google Scholar] [CrossRef]
- D’Aniello, M.; Tartaglia, R.; Cassiano, D. Experimental investigation of the inelastic tensile behaviour of non-preloadable grade 8.8 bolts. Ing. Sismica, Int. J. Earthq. Eng. 2020, 2, 92–110. [Google Scholar]
- Tartaglia, R.; D’Aniello, M.; Landolfo, R.; Rassati, G.A.; Swanson, J. Finite element analyses on seismic response of partial strength extended stiffened joints. COMPDYN 2017. In Proceedings of the 6th International Conference on Computational Methods in Structural Dynamics and Earthquake Engineering, Rhodes Island, Greece, 15–17 June 2017; Volume 2, pp. 4952–4964. [Google Scholar]
- Tartaglia, R.; D’Aniello, M. Influence of transverse beams on the ultimate behaviour of seismic resistant partial strength beam-to-column joints. Ing. Sismica Int. J. Earthq. Eng. 2020, 3, 50–66. [Google Scholar]
- Tartaglia, R.; D’Aniello, M.; Rassati, G.A.; Swanson, J.A.; Landolfo, R. Full strength extended stiffened end-plate joints: AISC vs recent European design criteria. Eng. Struct. 2018, 159, 155–171. [Google Scholar] [CrossRef]
- Tartaglia, R.; D’Aniello, M.; Landolfo, R. The influence of rib stiffeners on the response of extended end-plate joints. J. Constr. Steel Res. 2018, 148, 669–690. [Google Scholar] [CrossRef]
- Tartaglia, R.; D’Aniello, M.; Rassati, G.A.; Swanson, J.; Landolfo, R. Influence of composite slab on the nonlinear response of extended end-plate beam-to-column joints. Key Eng. Mater. 2017, 763, 818–825. [Google Scholar] [CrossRef]
- Tartaglia, R.; D’Aniello, M.; Zimbru, M.; Landolfo, R. Finite element simulations on the ultimate response of extended stiffened end-plate joints. Steel Compos Struct. 2018, 27, 727–745. [Google Scholar]
- Cassiano, D.; D’Aniello, M.; Rebelo, C. Seismic behaviour of gravity load designed flush end-plate joints. Steel Compos. Struct. 2018, 26, 621–634. [Google Scholar] [CrossRef]
- Cassiano, D.; D’Aniello, M.; Rebelo, C. Parametric finite element analyses on flush end-plate joints under column removal. J. Constr. Steel Res. 2017, 137, 77–92. [Google Scholar] [CrossRef]
- D’Aniello, M.; Güneyisi, E.M.; Landolfo, R.; Mermerdaş, K. Analytical prediction of available rotation capacity of cold-formed rectangular and square hollow section beams. Thin Walled Struct. 2014, 77, 141–152. [Google Scholar] [CrossRef]
- Güneyisi, E.M.; D’Aniello, M.; Landolfo, R.; Mermerdaş, K. Prediction of the flexural overstrength factor for steel beams using artificial neural network. Steel Compos Struct. 2014, 17, 215–236. [Google Scholar] [CrossRef]
- D’Aniello, M.; Güneyisi, E.M.; Landolfo, R.; Mermerdaş, K. Predictive models of the flexural overstrength factor for steel thin-walled circular hollow section beams. Thin Walled Struct. 2015, 94, 67–78. [Google Scholar] [CrossRef]
- Ascione, L.; Berardi, V.P.; Giordano, A.; Spadea, S. Macro-scale analysis of local and global buckling behavior of T and C composite sections. Mech. Res. Commun. 2014, 58, 105–111. [Google Scholar] [CrossRef]
- Razaqpur, A.G.; Ascione, F.; Lamberti, M.; Spadea, S.; Malagic, M. GFRP hollow column to built-up beam adhesive connection: Mechanical behaviour under quasi-static, cyclic and fatigue loading. Compos. Struct. 2019, 224, 111069. [Google Scholar] [CrossRef]
- Ascione, F.; Lamberti, M.; Razaqpur, A.G.; Spadea, S.; Malagic, M. Pseudo-ductile failure of adhesively joined GFRP beam-column connections: An experimental and numerical investigation. Compos. Struct. 2018, 200, 864–873. [Google Scholar] [CrossRef]
- Ascione, L.; Berardi, V.P.; Giordano, A.; Spadea, S. Buckling failure modes of FRP thin-walled beams. Compos. Part B Eng. 2013, 47, 357–364. [Google Scholar] [CrossRef]
- Ascione, L.; Berardi, V.P.; Giordano, A.; Spadea, S. Local buckling behavior of FRP thin-walled beams: A mechanical model. Compos. Struct. 2013, 98, 111–120. [Google Scholar] [CrossRef]
- Ascione, L.; Berardi, V.P.; Giordano, A.; Spadea, S. Pre-buckling imperfection sensitivity of pultruded FRP profiles. Compos. Part B Eng. 2015, 72, 206–212. [Google Scholar] [CrossRef]
- Ascione, L.; Giordano, A.; Spadea, S. Lateral buckling of pultruded FRP beams. Compos. Part B Eng. 2011, 42, 819–824. [Google Scholar] [CrossRef]
| Current EN 1998-1 | Next EN 1998-1 | ||
|---|---|---|---|
| Ductility Class | Reference q | Ductility Class | Reference q |
| DCL | 1.5–2 | DC1 | 1.5 |
| DCM | 4 | DC2 | 2.5 |
| DCH | 4 | DC3 | 4 |
| Current EN 1998-1 | Next Rules | ||
|---|---|---|---|
| DCM | Resistance: | DC2 (TO) | Resistance: |
| Global slenderness: | Global slenderness: | ||
| Local slenderness: class 1, 2 | Local slenderness: class 1, 2 | ||
| Overstrength variation: | Overstrength variation: none | ||
| DCH | Resistance: | DC3 | Resistance: ith story: * roof story: |
| Global slenderness: | Global slenderness: | ||
| Local slenderness: class 1 | Local slenderness: class 1 CHS: SHS: | ||
| Overstrength variation: | Overstrength variation: | ||
| Current EN 1998-1 DCH/DCM | |||
|---|---|---|---|
| DCM/DCH | Beams and columns should be designed to verify: , where | ||
| Next rules | |||
| DC2 | Beams and columns should be designed to withstand: where | DC3 | Beams and columns should be designed to withstand: (i) where (ii) Plastic mechanism analysis with: (iii) Plastic mechanism analysis with and |
| Columns should also verify combined action of | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Campiche, A.; Costanzo, S. Evolution of EC8 Seismic Design Rules for X Concentric Bracings. Symmetry 2020, 12, 1807. https://doi.org/10.3390/sym12111807
Campiche A, Costanzo S. Evolution of EC8 Seismic Design Rules for X Concentric Bracings. Symmetry. 2020; 12(11):1807. https://doi.org/10.3390/sym12111807
Chicago/Turabian StyleCampiche, Alessia, and Silvia Costanzo. 2020. "Evolution of EC8 Seismic Design Rules for X Concentric Bracings" Symmetry 12, no. 11: 1807. https://doi.org/10.3390/sym12111807
APA StyleCampiche, A., & Costanzo, S. (2020). Evolution of EC8 Seismic Design Rules for X Concentric Bracings. Symmetry, 12(11), 1807. https://doi.org/10.3390/sym12111807





