An Anisotropic Model for the Universe
Abstract
1. Introduction
2. Anisotropic ΛCDM Model
2.1. Model Setup
2.2. Anisotropy-Scale Relation Interpretation
3. Redshift in Anisotropic Models
3.1. Comoving Distance
3.2. Redshift Calculation
3.3. Angle Averaging and Scale-Small Anisotropic Deviation Redshift Relation
4. Hubble Law in Anisotropic Models
4.1. Generalized Hubble Parameter
4.2. Hubble-Scale-Redshift Relation
4.3. Hubble-Redshift Relation
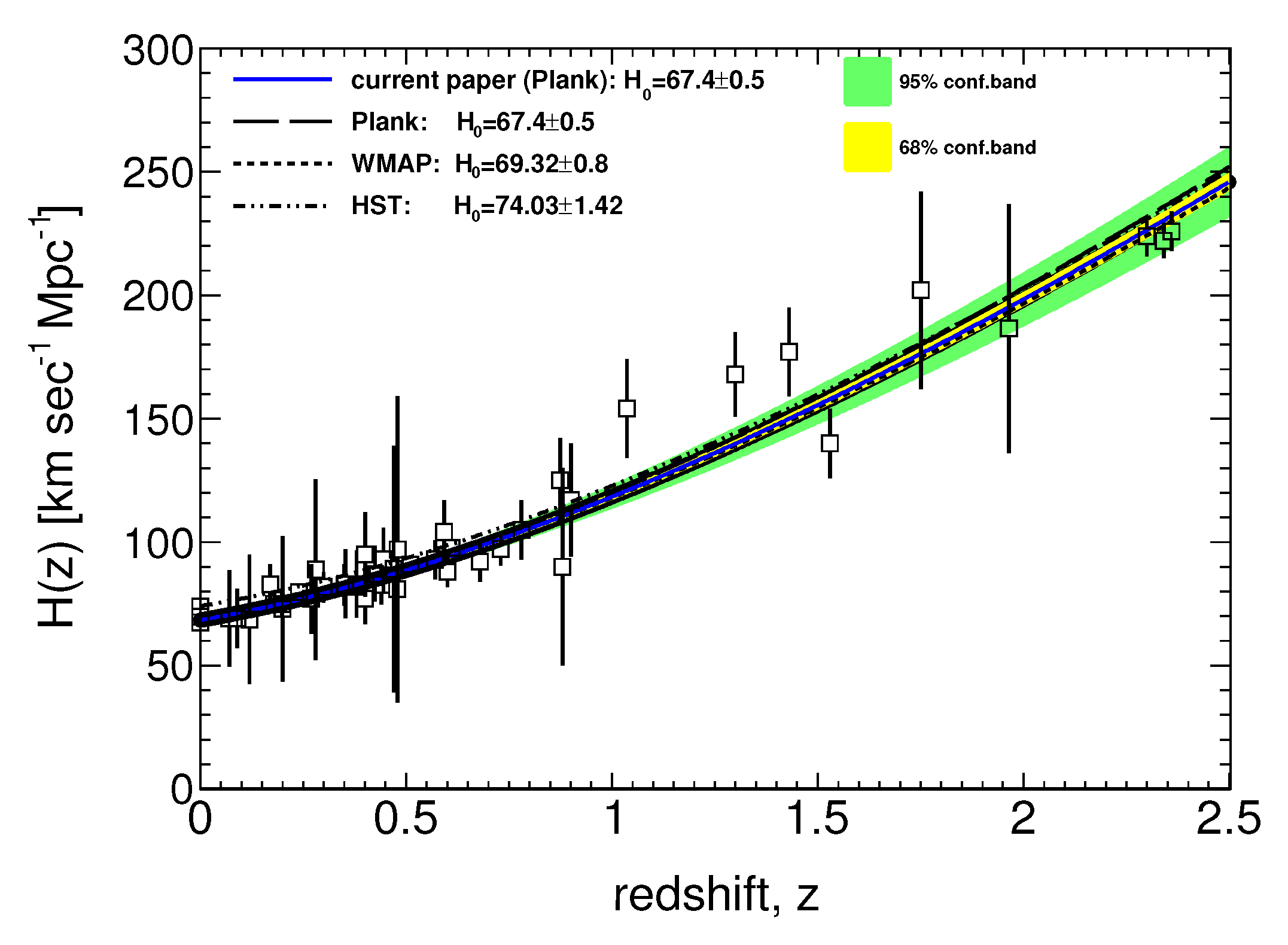
5. Preliminary Confrontation with Observations
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Solutions to Anisotropic Scale Parameters
Appendix A.1. Solutions with Ω0
Appendix A.2. Solutions with Ωm
Appendix B. Anisotropic Redshift Hubble Calculations
Appendix B.1. Anisotropic Redshift Terms
Appendix B.2. Anisotropic Hubble Parameter
References
- Rasanen, S. Dark energy from back-reaction. J. Cosmol. Astropart. Phys. 2004, 2, 3. [Google Scholar] [CrossRef]
- Rasanen, S. Accelerated expansion from structure formation. J. Cosmol. Astropart. Phys. 2006, 11, 3. [Google Scholar] [CrossRef]
- Marozzi, G.; Uzan, J.P. Late time anisotropy as an imprint of cosmological backreaction. Phys. Rev. D 2012, 86, 063528. [Google Scholar] [CrossRef]
- Flanagan, E.E. Can superhorizon perturbations drive the acceleration of the universe? Phys. Rev. D 2005, 71, 103521. [Google Scholar] [CrossRef]
- Misner, C.W. The isotropy of the universe. Astrophys. J. 1968, 151, 431. [Google Scholar] [CrossRef]
- Gibbons, G.W.; Hawking, S.W. Action integrals and partition functions in quantum gravity. Phys. Rev. D 1977, 15, 2752. [Google Scholar] [CrossRef]
- Penzias, A.A.; Wilson, R.W. A measurement of excess antenna temperature at 4080 Mc/s. Astrophys. J. 1965, 142, 419. [Google Scholar] [CrossRef]
- Dicke, R.H.; Peebles, P.J.E.; Roll, P.G.; Wilkinson, D.T. Cosmic Black-Body Radiation. Astrophys. J. 1965, 142, 414. [Google Scholar] [CrossRef]
- Stoeger, S.J.; Araujo, W.R.M.; Gebbie, T. The limits on cosmological anisotropies and inhomogeneities from COBE data. Astrophys. J. 1997, 476, 435. [Google Scholar] [CrossRef][Green Version]
- Maartens, R.; Ellis, G.F.R.; Stoeger, W.R. Limits on anisotropy and inhomogeneity from the cosmic background radiation. Phys. Rev. D 1995, 51, 1525. [Google Scholar] [CrossRef]
- Maartens, R.; Ellis, G.F.R.; Stoeger, W.R. Improved limits on anisotropy and inhomogeneity from the cosmic background radiation. Phys. Rev. D 1995, 51, 5942. [Google Scholar] [CrossRef] [PubMed]
- Friedman, A. On space curvature. Z. Phys. 1992, 10, 377. [Google Scholar] [CrossRef]
- Lemaitre, G. Expansion of the universe, The expanding universe. Mon. Not. R. Astron. Soc. 1931, 91, 490. [Google Scholar] [CrossRef]
- Robertson, H.P. Relativistic cosmology. Rev. Mod. Phys. 1933, 5, 62. [Google Scholar] [CrossRef]
- Walker, A.G. Distance in an expanding universe. Mon. Not. R. Astron. Soc. 1933, 94, 159. [Google Scholar] [CrossRef][Green Version]
- Collins, C.B.; Hawking, S.W. The rotation and distortion of the universe. Mon. Not. R. Astron. Soc. 1973, 162, 307. [Google Scholar] [CrossRef]
- Mather, J.C.; Cheng, E.S.; Eplee, R.E., Jr.; Isaacman, R.B.; Meyer, S.S.; Shafer, R.A.; Weiss, R.; Wright, E.L.; Bennett, C.L.; Boggess, N.W.; et al. A preliminary measurement of the cosmic microwave background spectrum by the Cosmic Background Explorer (COBE) satellite. Astrophys. J. 1990, 354, L37–L40. [Google Scholar] [CrossRef]
- Bennett, C.L.; Bay, M.; Halpern, M.; Hinshaw, G.; Jackson, C.; Jarosik, N.; Kogut, A.; Limon, M.; Meyer, S.S.; Page, L.; et al. The Microwave Anisotropy Probe Mission. Astrophys. J. 2003, 583, 1–23. [Google Scholar] [CrossRef]
- Team, P.H.C.; Ade, P.A.R.; Aghanim, N.; Ansari, R.; Arnaud, M.; Ashdown, M.; Aumont, J.; Banday, A.J.; Bartelmann, M.; Bartlett, J.G.; et al. Planck early results. IV. First, assessment of the High Frequency Instrument in-flight performance. Astron. Astrophys. 2011, 536, A4. [Google Scholar] [CrossRef]
- Eriksen, H.K.; Hansen, F.K.; Banday, A.J.; Gorski, K.M.; Lilje, P.B. Asymmetries in the Cosmic Microwave Background anisotropy field. Astrophys. J. 2004, 605, 14. [Google Scholar] [CrossRef]
- Hansen, F.K.; Banday, A.J.; Gorski, K.M. Testing the cosmological principle of isotropy: Local power-spectrum estimates of the WMAP data. Mon. Not. R. Astron. Soc. 2004, 354, 641. [Google Scholar] [CrossRef]
- Jaffe, T.R.; Banday, A.J.; Eriksen, H.K.; Gorski, K.M.; Hansen, F.K. Evidence of vorticity and shear at large angular scales in the WMAP data: A violation of cosmological isotropy? Astrophys. J. 2005, 629, L1. [Google Scholar] [CrossRef]
- Hoftuft, J.; Eriksen, H.K.; Banday, A.J.; Gorski, K.M.; Hansen, F.K.; Lilje, P.B. Increasing evidence for hemispherical power asymmetry in the five-year WMAP data. Astrophys. J. 2009, 699, 985. [Google Scholar] [CrossRef]
- Ade, P.A.R.; Aghanim, N.; Armitage-Caplan, C.; Arnaud, M.; Ashdown, M.; Atrio-Barandela, F.; Aumont, J.; Baccigalupi, C.; Banday, A.J.; Barreiro, R.B.; et al. Planck2013 results. XXIII. Isotropy and statistics of the CMB. Astron. Astrophys. 2014, 571, A23. [Google Scholar] [CrossRef]
- Akrami, Y.; Fantaye, Y.; Shafieloo, A.; Eriksen, H.K.; Hansen, F.K.; Banday, A.J.; Górski, K.M. Power asymmetry in WMAP and Planck temperature sky maps as measured by a local variance estimator. Astrophys. J. 2014, 784, L42. [Google Scholar] [CrossRef]
- Zhao, W.; Santos, L. The Weird Side of the Universe: Preferred Axis. In Proceedings of the 7th International Workshop on Astronomy and Relativistic Astrophysics (IWARA 2016), International Journal of Modern Physics: Conference Series, Gramado, Brazil, 9–13 October 2016; Volume 45, p. 1760009. [Google Scholar] [CrossRef]
- Contreras, D.; Hutchinson, J.; Moss, A.; Scott, D.; Zibin, J.P. Closing in on the large-scale CMB power asymmetry. Phys. Rev. D 2018, 97, 063504. [Google Scholar] [CrossRef]
- O’Dwyer, M.; Copi, C.J.; Nagy, J.M.; Netterfield, C.B.; Ruhl, J.; Starkman, G.D. Hemispherical Variance Anomaly and Reionization Optical Depth. arXiv 2019, arXiv:1912.02376. [Google Scholar]
- Schwarz, D.J.; Starkman, G.D.; Huterer, D.; Copi, C.J. Is the low-l microwave background cosmic? Phys. Rev. Lett. 2004, 93, 221301. [Google Scholar] [CrossRef]
- Copi, C.J.; Huterer, D.; Schwarz, D.J.; Starkman, G.D. On the large-angle anomalies of the microwave sky. Mon. Not. R. Astron. Soc. 2006, 367, 79. [Google Scholar] [CrossRef]
- Copi, C.; Huterer, D.; Schwarz, D.; Starkman, G. Uncorrelated universe: Statistical anisotropy and the vanishing angular correlation function in WMAP years 1–3. Phys. Rev. D 2007, 75, 023507. [Google Scholar] [CrossRef]
- Copi, C.J.; Huterer, D.; Schwarz, D.J.; Starkman, G.D. Large-angle anomalies in the CMB. Adv. Astron. 2010, 2010, 847541. [Google Scholar] [CrossRef]
- Copi, C.J.; Huterer, D.; Schwarz, D.J.; Starkman, G.D. Large-scale alignments from WMAP and Planck. Mon. Not. R. Astron. Soc. 2015, 449, 3458. [Google Scholar] [CrossRef]
- Marcos-Caballero, A.; Martínez-González, E. Scale-dependent dipolar modulation and the quadrupole-octopole alignment in the CMB temperature. J. Cosmol. Astropart. Phys. 2019, 1910, 53. [Google Scholar] [CrossRef]
- Cruz, M.; Martinez-Gonzalez, E.; Vielva, P.; Cayon, L. Detection of a non-Gaussian spot in WMAP. Mon. Not. R. Astron. Soc. 2005, 356, 29. [Google Scholar] [CrossRef]
- Cruz, M.; Cayon, L.; Martinez-Gonzalez, E.; Vielva, P.; Jin, J. The non-gaussian cold spot in the 3 year Wilkinson microwave anisotropy probe data. Astrophys. J. 2007, 655, 11. [Google Scholar] [CrossRef]
- Cruz, M.; Tucci, M.; Martinez-Gonzalez, E.; Vielva, P. The non-Gaussian cold spot in Wilkinson Microwave Anisotropy Probe: Significance, morphology and foreground contribution. Mon. Not. R. Astron. Soc. 2006, 369, 57. [Google Scholar] [CrossRef]
- Bolejko, K. Emerging spatial curvature can resolve the tension between high-redshift CMB and low-redshift distance ladder measurements of the Hubble constant. Phys. Rev. D 2018, 97, 103529. [Google Scholar] [CrossRef]
- Planck; Aghanim, N.; Akrami, Y.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; Bartolo, N.; et al. Planck 2018 results. VI. Cosmological parameters. arXiv 2018, arXiv:1807.06209. [Google Scholar]
- Riess, A.G.; Casertano, S.; Yuan, W.; Macri, L.; Anderson, J.; MacKenty, J.W.; Bowers, J.B.; Clubb, K.I.; Filippenko, A.V.; Jones, D.O.; et al. New Parallaxes of Galactic Cepheids from Spatially Scanning theHubble Space Telescope: Implications for the Hubble Constant. Astrophys. J. 2018, 855, 136. [Google Scholar] [CrossRef]
- Riess, A.G.; Casertano, S.; Yuan, W.; Macri, L.; Bucciarelli, B.; Lattanzi, M.G.; MacKenty, J.W.; Bowers, J.B.; Zheng, W.; Filippenko, A.V.; et al. Milky Way Cepheid Standards for Measuring Cosmic Distances and Application to Gaia DR2: Implications for the Hubble Constant. Astrophys. J. 2018, 861, 126. [Google Scholar] [CrossRef]
- Riess, A.G.; Casertano, S.; Yuan, W.; Macri, L.M.; Scolnic, D. Large Magellanic Cloud Cepheid standards provide a 1% foundation for the determination of the Hubble constant and stronger evidence for physics beyond ΛCDM. Astrophys. J. 2019, 876, 85. [Google Scholar] [CrossRef]
- Bennett, C.L.; Hill, R.S.; Hinshaw, G.; Larson, D.; Smith, K.M.; Dunkley, J.; Gold, B.; Halpern, M.; Jarosik, N.; Kogut, A.; et al. Seven-year wilkinson microwave anisotropy probe (WMAP*) observations: Are there cosmic microwave background anomalies? Astrophys. J. Suppl. 2011, 192, 17. [Google Scholar] [CrossRef]
- Rameez, M.; Sarkar, S. Is there really a Hubble tension? arXiv 2019, arXiv:1911.06456. [Google Scholar]
- Pontzen, A.; Challinor, A. Bianchi model CMB polarization and its implications for CMB anomalies. Mon. Not. R. Astron. Soc. 2007, 380, 1387. [Google Scholar] [CrossRef]
- Sung, R.; Short, J.; Coles, P. Statistical characterization of cosmic microwave background temperature patterns in anisotropic cosmologies. Mon. Not. R. Astron. Soc. 2011, 412, 492–502. [Google Scholar] [CrossRef]
- Russell, E.; Kılınc, C.B.; Pashaev, O.K. Bianchi I model: An alternative way to model the present-day Universe. Mon. Not. R. Astron. Soc. 2014, 442, 2331–2341. [Google Scholar] [CrossRef]
- Macpherson, H.J. Inhomogeneous Cosmology in an Anisotropic Universe. Ph.D. Thesis, Monash University, Clayton, Australia, 2019. [Google Scholar]
- Cea, P. Eur. Confronting the ellipsoidal universe to the Planck 2018 data. Phys. J. Plus 2020, 135, 150. [Google Scholar] [CrossRef]
- Jacobs, K.C. Bianchi Type I Cosmological Models. Ph.D. Thesis, Caltech University, Pasadena, CA, USA, 1968. [Google Scholar]
- Smoot, G.F.; Bennett, C.L.; Kogut, A.; Wright, E.L.; Aymon, J.; Boggess, N.W.; Cheng, E.S.; De Amici, G.; Gulkis, S.; Hauser, M.; et al. Structure in the COBE differential microwave radiometer first-year maps. Astrophys. J. 1992, 396, L1. [Google Scholar] [CrossRef]
- Simon, J.; Verde, L.; Jimenez, R. Constraints on the redshift dependence of the dark energy potential. Phys. Rev. D 2005, 71, 123001. [Google Scholar] [CrossRef]
- Moresco, M.; Cimatti, A.; Jimenez, R.; Pozzetti, L.; Zamorani, G.; Bolzonella, M.; Dunlopd, J.; Lamareillee, F.; Mignolic, M.; Pearced, H.; et al. Improved constraints on the expansion rate of the Universe up to z 1.1 from the spectroscopic evolution of cosmic chronometers. J. Cosmol. Astropart. Phys. 2012, 8, 6. [Google Scholar] [CrossRef]
- Anderson, L.; Aubourg, E.; Bailey, S.; Beutler, F.; Bolton, A.S.; Brinkmann, J.; Brownstein, J.R.; Chuang, C.-H.; Cuesta, A.J.; Dawson, K.S.; et al. The clustering of galaxies in the SDSS-III Baryon Oscillation Spectroscopic Survey: Measuring DA and H at z = 0.57 from the baryon acoustic peak in the Data Release 9 spectroscopic Galaxy sample. Mon. Not. R. Astron. Soc. 2014, 439, 83. [Google Scholar] [CrossRef]
- Yu, H.; Ratra, B.; Wang, F.-Y. Hubble parameter and Baryon Acoustic Oscillation measurement constraints on the Hubble constant, the deviation from the spatially flat ΛCDM model, the deceleration-acceleration transition redshift, and spatial curvature. Astrophys. J. 2018, 856, 3. [Google Scholar] [CrossRef]
- Sharov, G.; Vorontsova, E. Parameters of cosmological models and recent astronomical observations. J. Cosmol. Astropart. Phys. 2014, 10, 57. [Google Scholar] [CrossRef]
- Antcheva, I.; Ballintijn, M.; Bellenot, B.; Biskup, M.; Brun, R.; Buncic, N.; Canal, P.; Casadei, D.; Couet, O.; Fine, V.; et al. ROOT—A C++ framework for petabyte data storage, statistical analysis and visualization. Comput. Phys. Commun. 2009, 180, 2499. [Google Scholar] [CrossRef]
- Komatsu, E.; Smith, K.M.; Dunkley, J.; Bennett, C.L.; Gold, B.; Hinshaw, G.; Jarosik, N.; Larson, D.; Nolta, M.R.; Page, L.; et al. Seven-year Wilkinson microwave anisotropy probe (WMAP) observations: Cosmological interpretation. Astrophys. J. Suppl. 2011, 192. [Google Scholar] [CrossRef]
- Bolejko, K.; Nazer, M.A.; Wiltshire, D.L. Differential cosmic expansion and the Hubble flow anisotropy. J. Cosmol. Astropart. Phys. 2016, 6, 35. [Google Scholar] [CrossRef]
| Fit Method | Plank | WMAP | HST | |||
|---|---|---|---|---|---|---|
| Log-Likelihood (with/without weighting empty bins) | 0.25 | 0.37 | 0.13 | 0.12 | 0.25 | 0.37 |
| (without weighting empty bins) | 0.13 | 1.32 | 0.25 | 4.03 | 0.13 | 1..31 |
| (with weighting empty bins) | 0.25 | 0.37 | 0.13 | 0.12 | 0.25 | 0.37 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Delliou, M.L.; Deliyergiyev, M.; del Popolo, A. An Anisotropic Model for the Universe. Symmetry 2020, 12, 1741. https://doi.org/10.3390/sym12101741
Delliou ML, Deliyergiyev M, del Popolo A. An Anisotropic Model for the Universe. Symmetry. 2020; 12(10):1741. https://doi.org/10.3390/sym12101741
Chicago/Turabian StyleDelliou, Morgan Le, Maksym Deliyergiyev, and Antonino del Popolo. 2020. "An Anisotropic Model for the Universe" Symmetry 12, no. 10: 1741. https://doi.org/10.3390/sym12101741
APA StyleDelliou, M. L., Deliyergiyev, M., & del Popolo, A. (2020). An Anisotropic Model for the Universe. Symmetry, 12(10), 1741. https://doi.org/10.3390/sym12101741






