Adaptation of a Cost Overrun Risk Prediction Model to the Type of Construction Facility
Abstract
1. Introduction
2. Literature Review
3. Concept of a Cost Overrun Risk Prediction Model
3.1. Main Assumptions of the Model
- exceeding the time and cost of construction investments [34],
- occupational risks on construction sites [37],
- level of safety of construction workers [38],
- technological, financial, political, environmental, and legal risk factors in the life cycle of buildings [39],
- technological risk factors for old buildings [40].
3.2. Block of Fuzzification
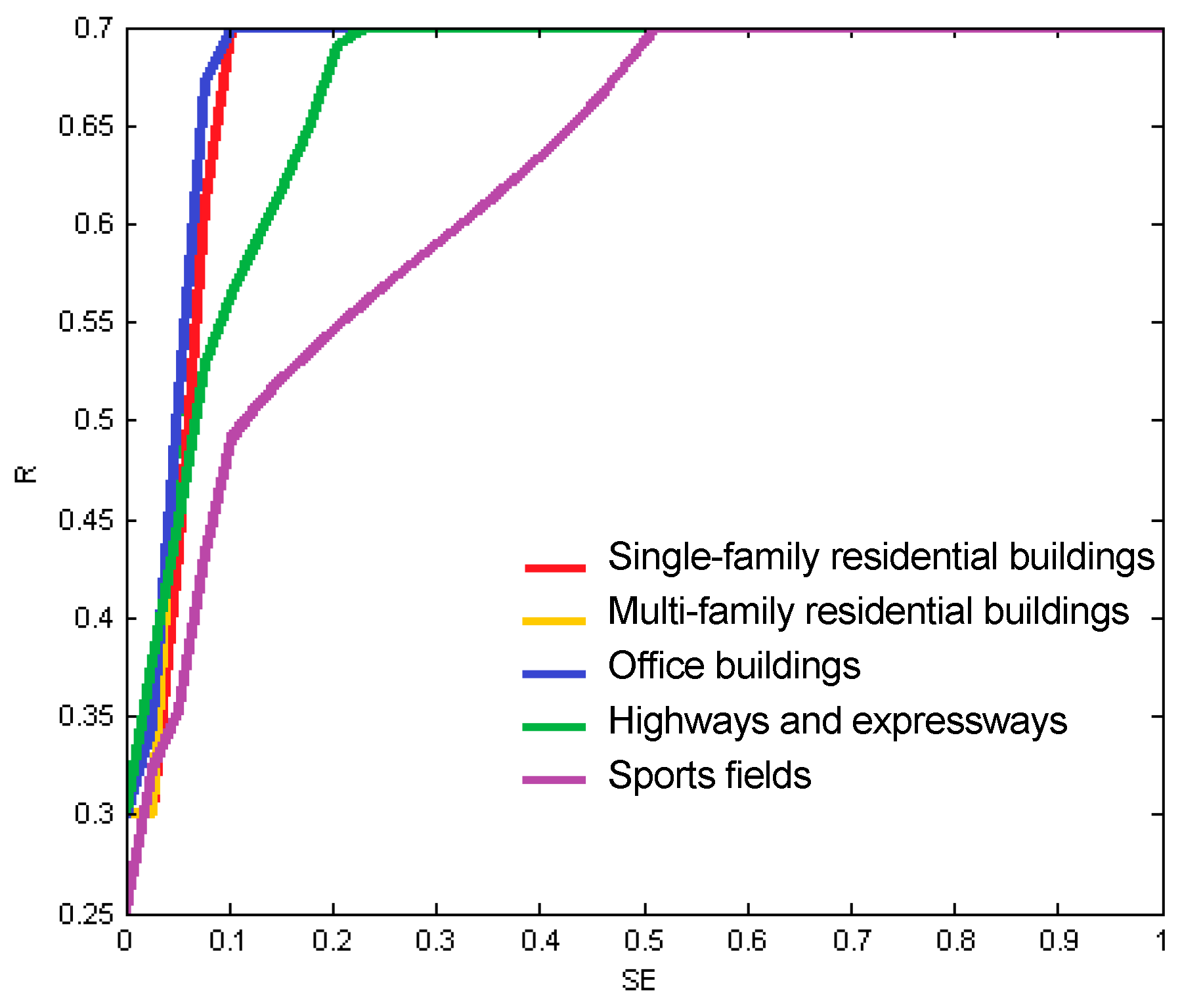
- single-family residential buildings,
- multi-family residential buildings,
- office buildings,
- highways and expressways,
- sports fields.
- “high”—description of the variable would relate to the value “about or above quartile Q3”,
- “average”—description of the variable would relate to the value “about median”,
- “low”—description of the variable would relate to the value “about or below quartile Q1”.
3.3. Block of Inference
3.4. Block of Defuzzification
- are not able to implement the assumption adopted for the purposes of building the rule base, that with the increase in the share of element costs in the building costs (SE), predicted changes in the number of works (WC), and expected changes in the unit price (PC), the value of the risk level of exceeding the costs of a given element of the construction investment (R) will naturally and smoothly increase,
- result in sharp values, which will not in every case adequately represent the output fuzzy set, which is caused by the impact on the sharp result of only the most activated fuzzy set of the output variable.
4. Discussion
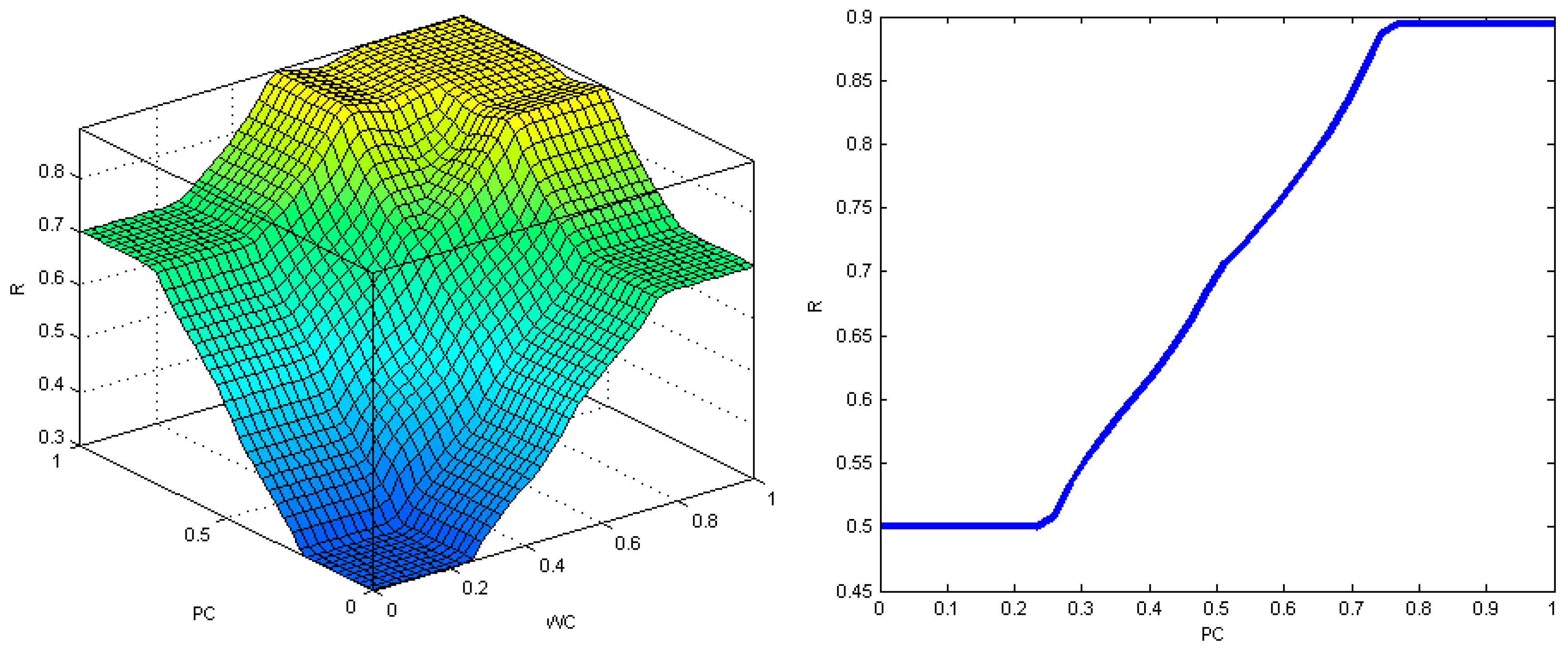
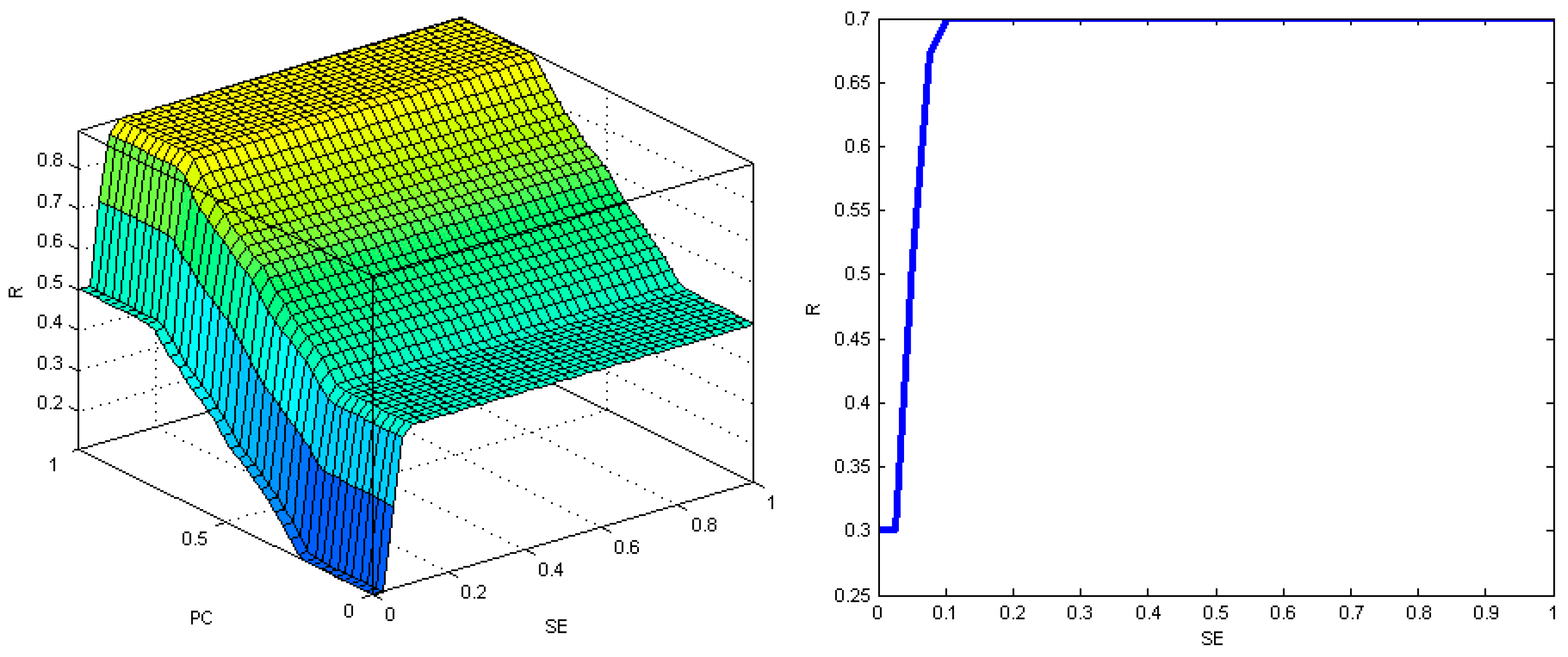
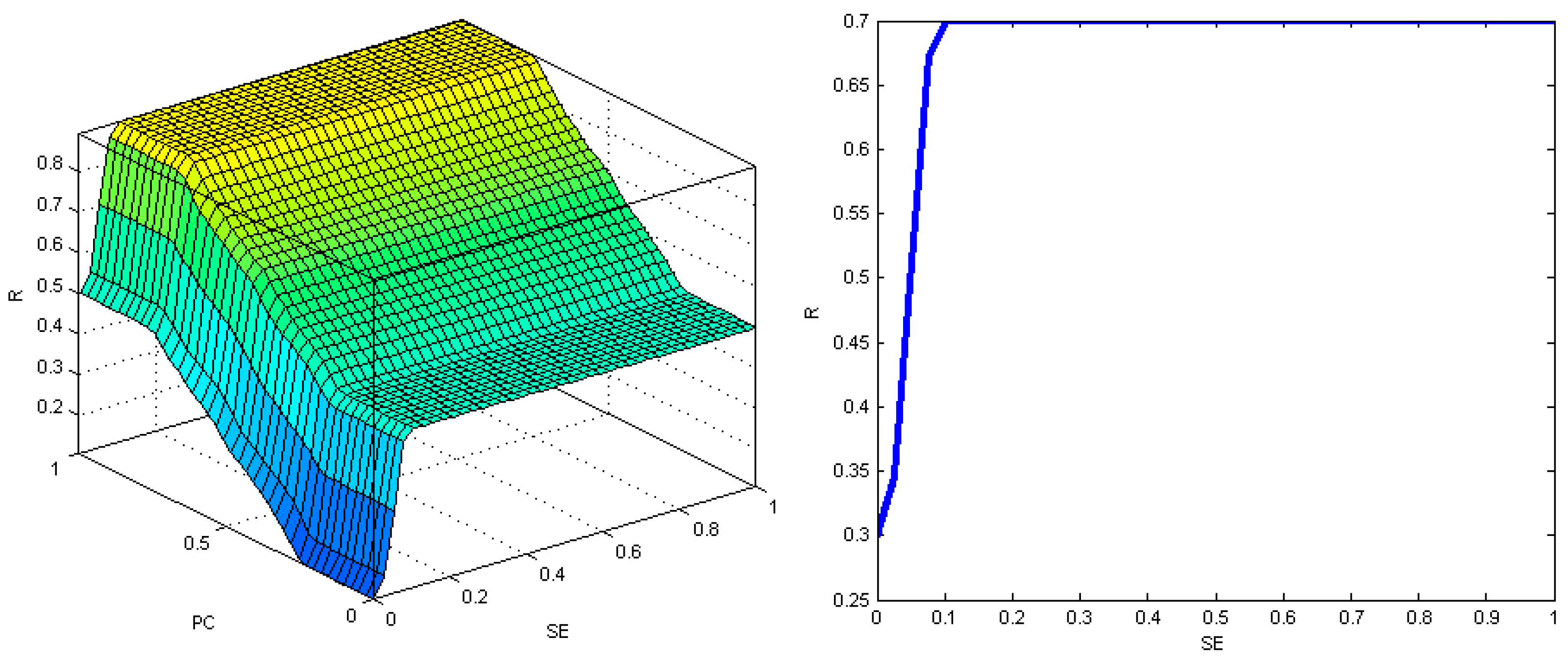
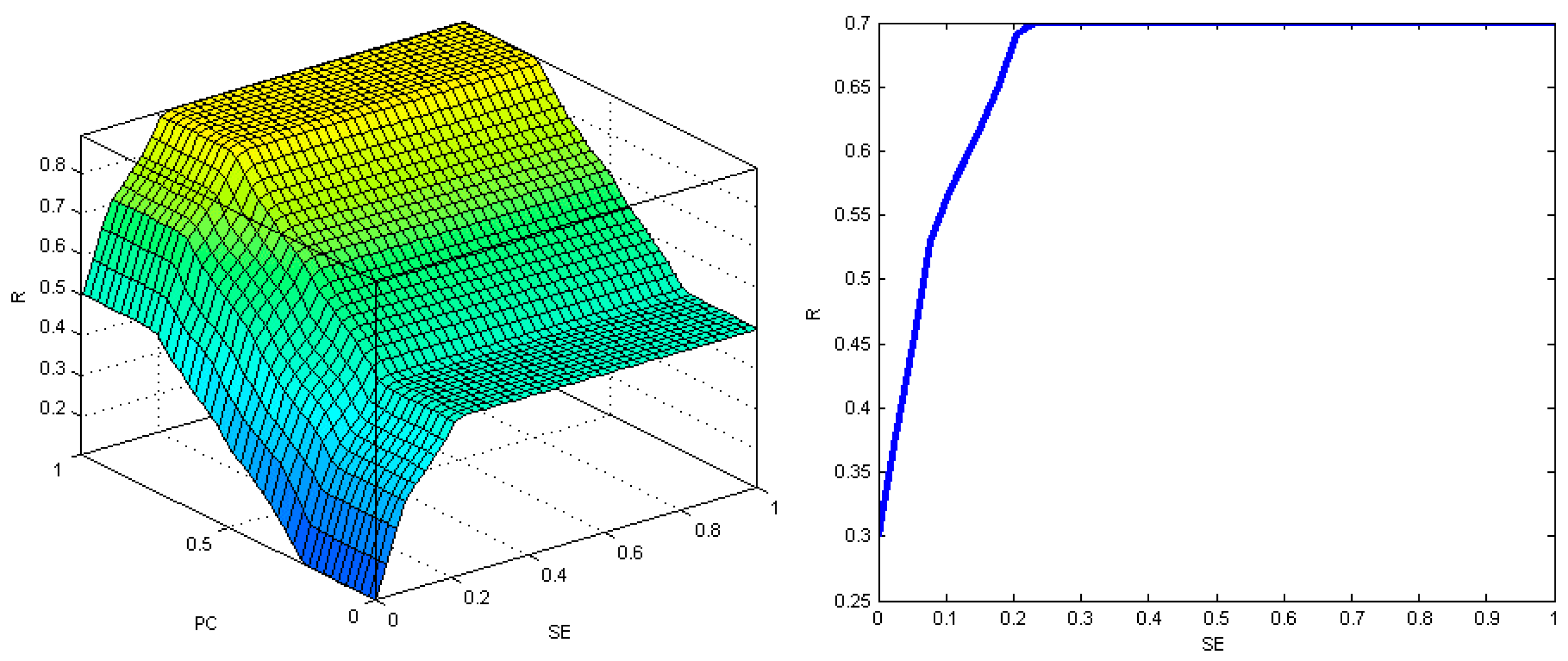
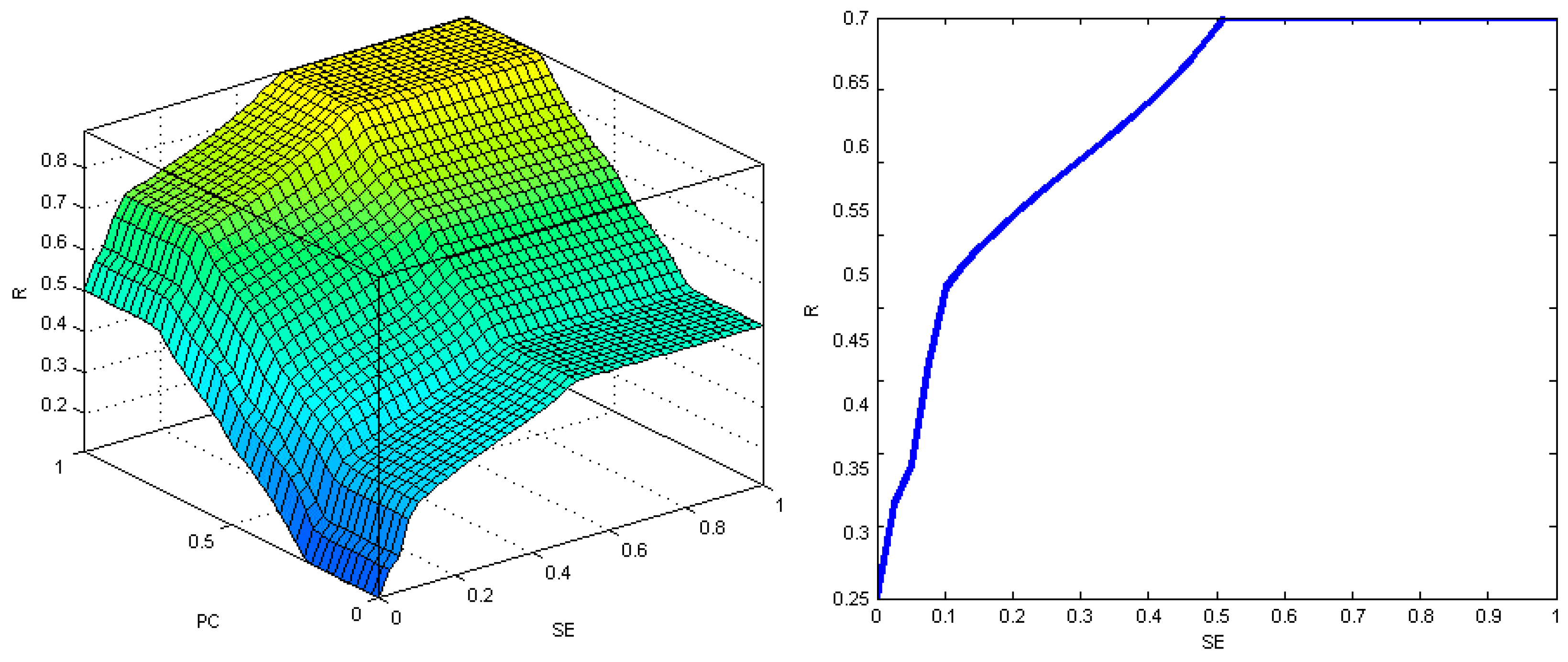
- diagrams of the result area for the output variable (R) due to the influence of the input variables PC and SE in the cross-section, when WC = 0.5, and WC and SE in the cross-section, when PC = 0.5,
- diagrams of the result area for the output variable (R) taking into account the set of input variables PC and WC in the cross-section, when SE = 0.5,
- flat diagrams of the resultant curves for the output variable (R) due to the influence of PC input variables in the cross-section, when WC = SE = 0.5, WC in the cross-section, when PC = SE = 0.5, and SE in the cross-section, when PC = WC = 0.5.
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Love, P.E.D.; Wang, X.; Sing, C.; Tiong, R.L.K. Determining the Probability of Cost Overruns. J. Constr. Eng. Manag. 2013, 139, 321–330. [Google Scholar] [CrossRef]
- Andrić, J.M.; Mahamadu, A.; Wang, J.; Zou, P.X.W.; Zhong, R. The cost performance and causes of overruns in infrastructure development projects in Asia. J. Civ. Eng. Manag. 2019, 25, 203–214. [Google Scholar] [CrossRef]
- Senouci, A.; Ismail, A.; Eldin, N. Time Delay and Cost Overrun in Qatari Public Construction Projects. Procedia Eng. 2016, 164, 368–375. [Google Scholar] [CrossRef]
- Larsen, J.K.; Shen, G.Q.; Lindhard, S.M.; Ditlev, T. Factors Affecting Schedule Delay, Cost Overrun, and Quality Level in Public Construction Projects. J. Manag. Eng. 2016, 32, 04015032. [Google Scholar] [CrossRef]
- Plebankiewicz, E. Model of predicting cost overrun in construction projects. Sustainability 2018, 10, 4387. [Google Scholar] [CrossRef]
- Chen, Y.; Hu, Z. Exploring the properties of cost overrun risk propagation network (CORPN) for promoting cost management. J. Civ. Eng. Manag. 2019, 25, 1–18. [Google Scholar] [CrossRef]
- Cantarelli, C.C.; Flyvbjerg, B.; Molin, J.E.E.; van Wee, B. Cost Overruns in Large-Scale Transportation Infrastructure Projects: Explanations and their Theoretical Embeddedness. Eur. J. Transp. Infrastruct. Res. 2010, 10, 21. [Google Scholar]
- Phama, H.; Luub, T.-V.; Kimc, S.-Y.; Viend, D.-T. Assessing the Impact of Cost Overrun Causes in Transmission Lines Construction Projects. Ksce J. Civ. Eng. 2020, 24, 1029–1036. [Google Scholar] [CrossRef]
- Shaikh, F.A. Financial Mismanagement: A Leading Cause of Time and Cost Overrun in Mega Construction Projects in Pakistan. Eng. Technol. Appl. Sci. Res. 2020, 10, 5247–5250. [Google Scholar]
- Gunduz, M.; Maki, O.L. Assessing the risk perception of cost overrun through importance rating. Technol. Econ. Dev. Econ. 2018, 24, 1829–1844. [Google Scholar] [CrossRef]
- El-Kholy, A.M. Predicting Cost Overrun in Construction Projects. Int. J. Constr. Eng. Manag. 2015, 4, 95–105. [Google Scholar]
- Sohu, S.; Abdullah, A.H.; Nagapan, S.; Rind, T.A.; Jhatial, A.A. Controlling Measures for Cost Overrun Causes in Highway Projects of Sindh Province Engineering. Technol. Appl. Sci. Res. 2019, 9, 4276–4280. [Google Scholar]
- Catalão, F.P.; Cruz, C.O.; Sarmento, J.M. The determinants of cost deviations and overruns in transport projects, an endogenous models approach. Transp. Policy 2019, 74, 224–238. [Google Scholar] [CrossRef]
- Ahiaga-Dagbui, D.D.; Gordon, R.; Love, P.E.D.; Smith, S.D.; Ackermann, F. Toward a Systemic View to Cost Overrun Causation in Infrastructure Projects: A Review and Implications for Research. Proj. Manag. J. 2017, 48, 88–98. [Google Scholar] [CrossRef]
- Huo, T.; Ren, H.; Cai, W.; Shen, G.Q.; Liu, B.; Zhu, M.; Wu, H. Measurement and dependence analysis of cost overruns in megatransport infrastructure projects: Case study in Hong Kong. J. Constr. Eng. Manag. 2018, 144, 05018001. [Google Scholar] [CrossRef]
- França, A.; Haddad, A. Causes of Construction Projects Cost Overrun in Brazil. Int. J. Sustain. Constr. Eng. Technol. 2018, 9, 69–83. [Google Scholar] [CrossRef]
- Johnson, J. Comparing the Effects of ABC and BIM in Construction Projects and Choose the Best Solution to Minimise the Delay and Cost Overrun Using MADMA. PM World J. 2019, 8, 1–18. [Google Scholar]
- Keng, T.C.; Mansor, N.; Ching, Y.K. An Exploration of Cost Overrun in Building Construction Projects. Glob. Bus. Manag. Res. Int. J. 2018, 10, 638–646. [Google Scholar]
- Mahamid, I. Study of relationship between cost overrun and labour productivity in road construction projects. Int. J. Product. Qual. Manag. 2018, 24, 143–164. [Google Scholar] [CrossRef]
- Akinradewo, O.; Aghimien, D.; Aigbavboa, C. Comparative Analysis of Cost Overrun on Road Construction Projects Executed by Indigenous and Expatriate Contractors. In Proceedings of the International Conference on Industrial Engineering and Operations Management, Dubai, United Arab Emirates, 10–12 March 2020. [Google Scholar]
- Cavalieri, M.; Cristaudo, R.; Guccio, C. On the magnitude of cost overruns throughout the project life-cycle: An assessment for the Italian transport infrastructure projects. Transp. Policy 2019, 79, 21–36. [Google Scholar] [CrossRef]
- Derakhshanalavijeh, R.; Teixeira, J.M.C. Cost overrun in construction projects in developing countries, Gas-Oil industry of Iran as a case study. J. Civ. Eng. Manag. 2017, 23, 125–136. [Google Scholar] [CrossRef]
- Cantarelli, C.C.; Molin, E.J.E.; van Wee, B.; Flyvbjerg, B. Characteristics of cost overruns for Dutch transport infrastructure projects and the importance of the decision to build and project phases. Transp. Policy 2012, 22, 49–56. [Google Scholar] [CrossRef]
- Islam, M.S.; Nepal, M.P.; Skitmore, M.; Kabir, G. A knowledge-based expert system to assess power plant project cost overrun risks. Expert Syst. Appl. 2019, 136, 12–32. [Google Scholar]
- Sharma, S.; Goyal, P.K. Fuzzy assessment of the risk factors causing cost overrun in construction industry. Evol. Intell. 2019, 1–13. [Google Scholar] [CrossRef]
- Marzouk, M.; Amin, A. Predicting Construction materials prices using fuzzy logic and neural networks. J. Constr. Eng. Manag. 2013, 139, 1190–1198. [Google Scholar] [CrossRef]
- Knight, K.; Robinson-Fayek, A. Use of fuzzy logic of predicting design cost overruns on building projects. J. Constr. Eng. Manag. 2002, 128, 503–512. [Google Scholar] [CrossRef]
- Ghazal, M.M.; Hammad, A.M. Data Acquisition Model for Analyzing Cost Overrun in Construction Projects using KDD. In Proceedings of the International Conference on Industrial Engineering and Operations Management, Bandung, Indonesia, 6–8 March 2018; pp. 2255–2266. [Google Scholar]
- Sanchez, F.; Bonjour, E.; Micaelli, J.-P.; Monticolo, D. An Approach Based on Bayesian Network for Improving Project Management Maturity: An Application to Reduce Cost Overrun Risks in Engineering Projects. Comput. Ind. 2020, 119, 103227. [Google Scholar] [CrossRef]
- Abu Hammad, A.A.; Ali, S.M.A.; Sweis, G.J.; Basher, A. Prediction Model for Construction Cost and Duration in Jordan. Jordan J. Civ. Eng. 2008, 2, 250–266. [Google Scholar]
- Juszczyk, M.; Leśniak, A.; Zima, K. ANN based approach for estimation of construction costs of sports fields. Complexity 2018, 2018, 7952434. [Google Scholar] [CrossRef]
- Ji, S.H.; Park, M.; Lee, H.S. Cost Estimation Model for Building Projects Using Case-Based Reasoning. Can. J. Civ. Eng. 2011, 38, 570–581. [Google Scholar] [CrossRef]
- Leśniak, A.; Zima, K. Cost Calculation of Construction Projects Including Sustainability Factors Using the Case Based Reasoning (CBR) Method. Sustainability 2018, 10, 1608. [Google Scholar] [CrossRef]
- Ibadov, N.; Kulejewski, J. The assessment of construction project risks with the use of fuzzy sets theory. Tech. Trans. 2014, 1-B, 175–182. [Google Scholar]
- Nieto-Morote, A.; Ruz-Vila, F. A fuzzy approach to construction project risk assessment. Int. J. Proj. Manag. 2011, 29, 220–231. [Google Scholar] [CrossRef]
- Tavakolan, M.; Mohammadi, A. Construction risk management framework using fuzzy sets and failure mode and effect analysis. In Proceedings of the 51st ASC Annual International Conference, The Associated Schools of Construction, College Station, TX, USA, 22–25 April 2015. [Google Scholar]
- Debnath, J.; Biswas, A.; Sivan, P.; Sen, K.N.; Sahu, S. Fuzzy inference model for assessing occupational risks in construction sites. Int. J. Ind. Ergon. 2016, 55, 114–128. [Google Scholar] [CrossRef]
- Othman, M.H.H.; Arbaiy, N.; Lah, M.S.C.; Lin, P.C. Mean-Variance Model With Fuzzy Random Data. J. Crit. Rev. 2020, 7, 1347–1352. [Google Scholar]
- Plebankiewicz, E.; Wieczorek, D. Rozmyta ocena ryzyka w cyklu życia obiektów budowlanych. Mater. Bud. 2016, 6, 59–61. [Google Scholar] [CrossRef]
- Konior, J. Technical assessment of old buildings by fuzzy approach. Arch. Civil. Eng. 2019, 65, 130–141. [Google Scholar] [CrossRef]
- Hovde, P.J.; Moser, K. Performance based Methods for Service Life Prediction; State of the Art Reports; CIB Report; Trondheim Publication: Trondheim, Norway, 2004; p. 294. [Google Scholar]
- Wieczorek, D. Fuzzy risk assessment in the life cycle of building object—Selection of the right defuzzification method. In AIP Conference Proceedings; AIP Publishing: Melville, NY, USA, 2018; Volume 1978, p. 240005. [Google Scholar]


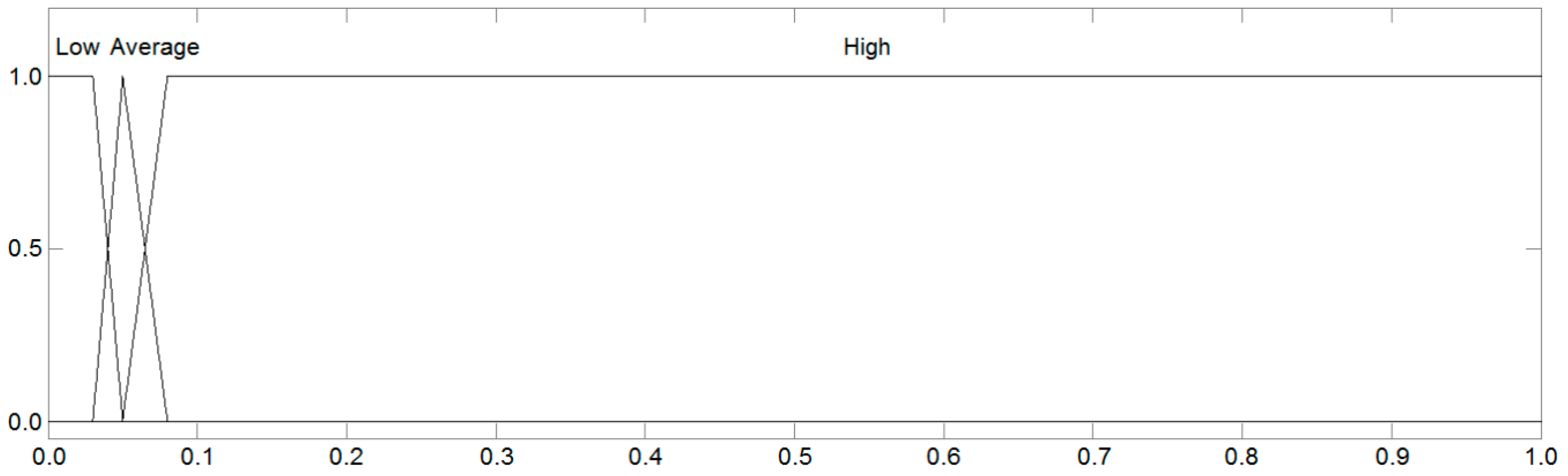

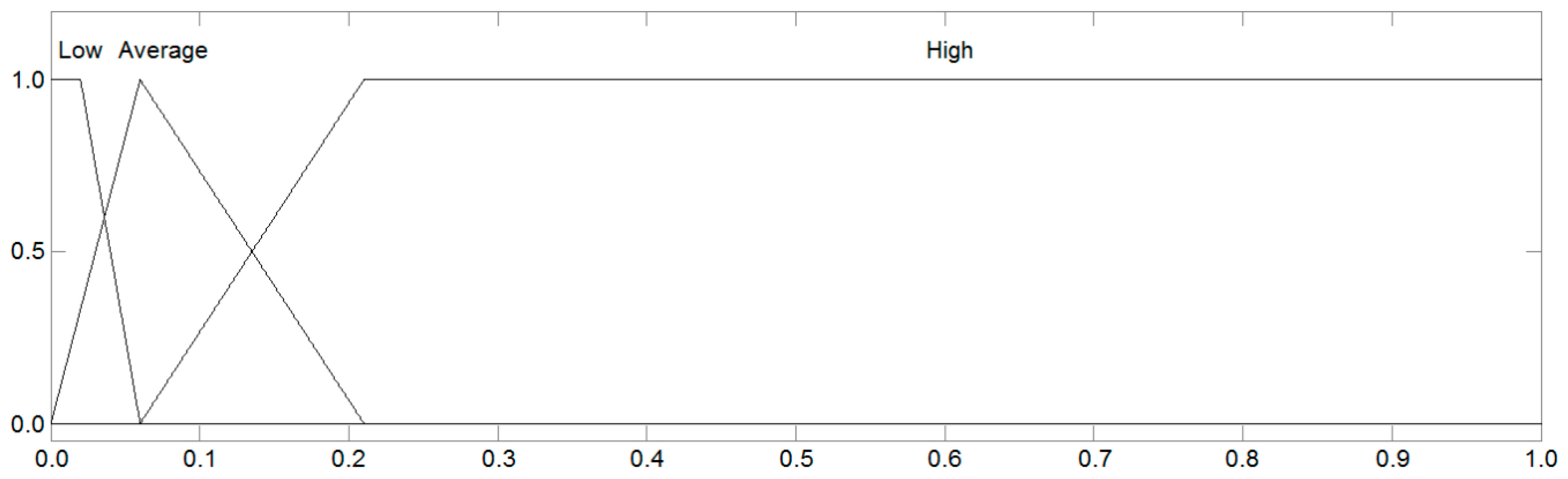
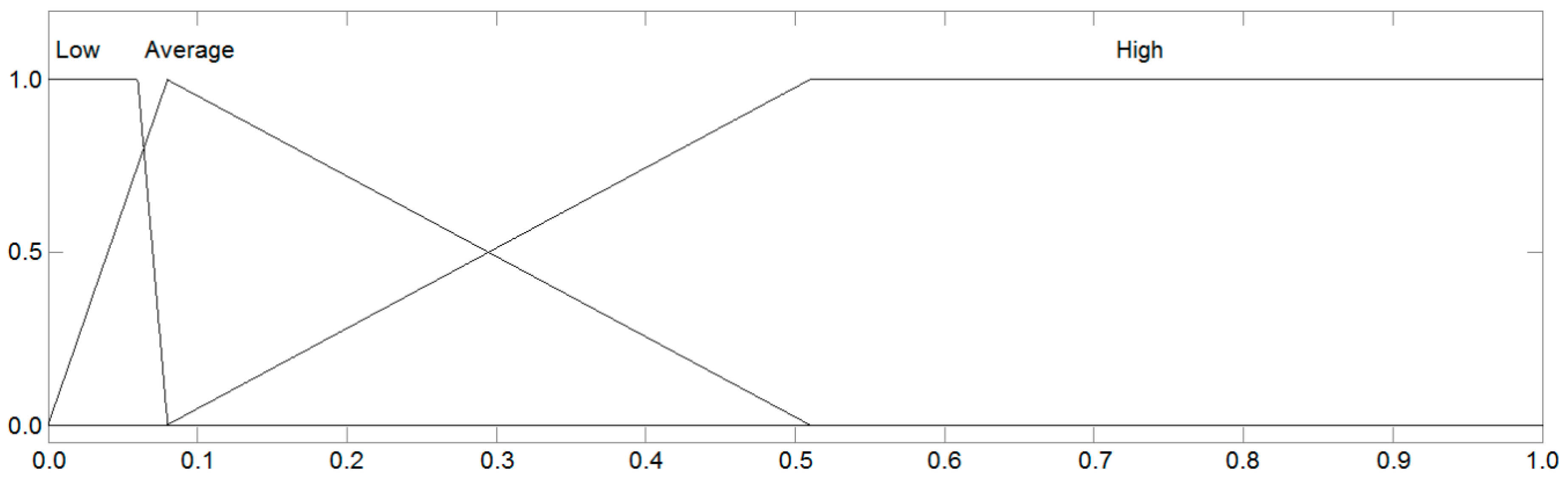


| Fuzzy Set of Linguistic Values for WC or PC | Description of the Variables x2 or x3 | Fuzzy Evaluation of Membership μ(x2) or μ(x3) | |
|---|---|---|---|
| High | Hi | About or above 75.0% | (0.5; 0.75; 1.0; 1.0) |
| Average | Av | About 50.0% | (0.25; 0.5; 0.5; 075) |
| Low | Lo | About or below 25.0% | (0.0; 0.0; 0.25; 0.5) |
| Type of Building | Cost Elements |
|---|---|
| Cubature facilities (single- and multi-family residential buildings, office buildings) | Earthworks, foundations (including walls and insulation of the ground floor of the building), ground walls, ceilings, stairs, partition walls, roof (construction and covering), sleepers and canals inside the building, insulation of the ground, plaster and interior cladding, windows and doors, painting work, floors (with layer), facades with works outside the building, water and sewage installations, central heating installations and electrical installations. |
| Highways and expressways | Preparatory works, earthworks, drainage of road body, substructures, surfaces, finishing works, traffic safety equipment, street and road elements and other works. |
| Sports fields | Site preparation and earthworks, substructures, sports surfaces, landscaping and equipment. |
| Type of Building | Quartile Q1 | Median | Quartile Q3 |
|---|---|---|---|
| Single-family residential buildings | 3 | 6 | 9 |
| Multi-family residential buildings | 3 | 5 | 8 |
| Office buildings | 2 | 5 | 8 |
| Highways and expressways | 2 | 6 | 21 |
| Sports fields | 6 | 18 | 51 |
| Fuzzy Set of Linguistic Values for SE | Description of the Variable x1 | Fuzzy Evaluation of Membership μ(x1) | |
|---|---|---|---|
| Single-family residential buildings | |||
| High | Hi | About or above 9.0% | (0.06; 0.09; 1.0; 1.0) |
| Average | Av | About 6.0% | (0.0; 0.06; 0.06; 0.09) |
| Low | Lo | About or below 3.0% | (0.0; 0.0; 0.03; 0.06) |
| Multi-family residential buildings | |||
| High | Hi | About or above 8.0% | (0.05; 0.08; 1.0; 1.0) |
| Average | Av | About 5.0% | (0.0; 0.05; 0.05; 0.08) |
| Low | Lo | About or below 3.0% | (0.0; 0.0; 0.03; 0.05) |
| Office buildings | |||
| High | Hi | About or above 8.0% | (0.05; 0.08; 1.0; 1.0) |
| Average | Av | About 5.0% | (0.0; 0.05; 0.05; 0.08) |
| Low | Lo | About or below 2.0% | (0.0; 0.0; 0.02; 0.05) |
| Highways and expressways | |||
| High | Hi | About or above 21.0% | (0.06; 0.21; 1.0; 1.0) |
| Average | Av | About 6.0% | (0.0; 0.06; 0.06; 0.21) |
| Low | Lo | About or below 2.0% | (0.0; 0.0; 0.02; 0.06) |
| Sports fields | |||
| High | Hi | About or above 51.0% | (0.08; 0.51; 1.0; 1.0) |
| Average | Av | About 8.0% | (0.0; 0.08; 0.08; 0.51) |
| Low | Lo | About or below 6.0% | (0.0; 0.0; 0.06; 0.08) |
| Rule No. | If (SE) | And (WC) | And (PC) | Then (R) | ||||
|---|---|---|---|---|---|---|---|---|
| LV | Weight | LV | Weight | LV | Weight | Product | Concl. | |
| 1 | Lo | 1 | Lo | 1 | Lo | 1 | 1 | Vl |
| 2 | Lo | 1 | Lo | 1 | Av | 2 | 2 | Vl |
| 3 | Lo | 1 | Lo | 1 | Hi | 3 | 3 | Ql |
| 4 | Lo | 1 | Av | 2 | Lo | 1 | 2 | Vl |
| 5 | Lo | 1 | Av | 2 | Av | 2 | 4 | Ql |
| 6 | Lo | 1 | Av | 2 | Hi | 3 | 6 | Av |
| 7 | Lo | 1 | Hi | 3 | Lo | 1 | 3 | Ql |
| 8 | Lo | 1 | Hi | 3 | Av | 2 | 6 | Av |
| 9 | Lo | 1 | Hi | 3 | Hi | 3 | 9 | Qh |
| 10 | Av | 2 | Lo | 1 | Lo | 1 | 2 | Vl |
| 11 | Av | 2 | Lo | 1 | Av | 2 | 4 | Ql |
| 12 | Av | 2 | Lo | 1 | Hi | 3 | 6 | Av |
| 13 | Av | 2 | Av | 2 | Lo | 1 | 4 | Ql |
| 14 | Av | 2 | Av | 2 | Av | 2 | 8 | Av |
| 15 | Av | 2 | Av | 2 | Hi | 3 | 12 | Qh |
| 16 | Av | 2 | Hi | 3 | Lo | 1 | 6 | Av |
| 17 | Av | 2 | Hi | 3 | Av | 2 | 12 | Qh |
| 18 | Av | 2 | Hi | 3 | Hi | 3 | 18 | Vh |
| 19 | Hi | 3 | Lo | 1 | Lo | 1 | 3 | Ql |
| 20 | Hi | 3 | Lo | 1 | Av | 2 | 6 | Av |
| 21 | Hi | 3 | Lo | 1 | Hi | 3 | 9 | Qh |
| 22 | Hi | 3 | Av | 2 | Lo | 1 | 6 | Av |
| 23 | Hi | 3 | Av | 2 | Av | 2 | 12 | Qh |
| 24 | Hi | 3 | Av | 2 | Hi | 3 | 18 | Vh |
| 25 | Hi | 3 | Hi | 3 | Lo | 1 | 9 | Qh |
| 26 | Hi | 3 | Hi | 3 | Av | 2 | 18 | Vh |
| 27 | Hi | 3 | Hi | 3 | Hi | 3 | 27 | Vh |
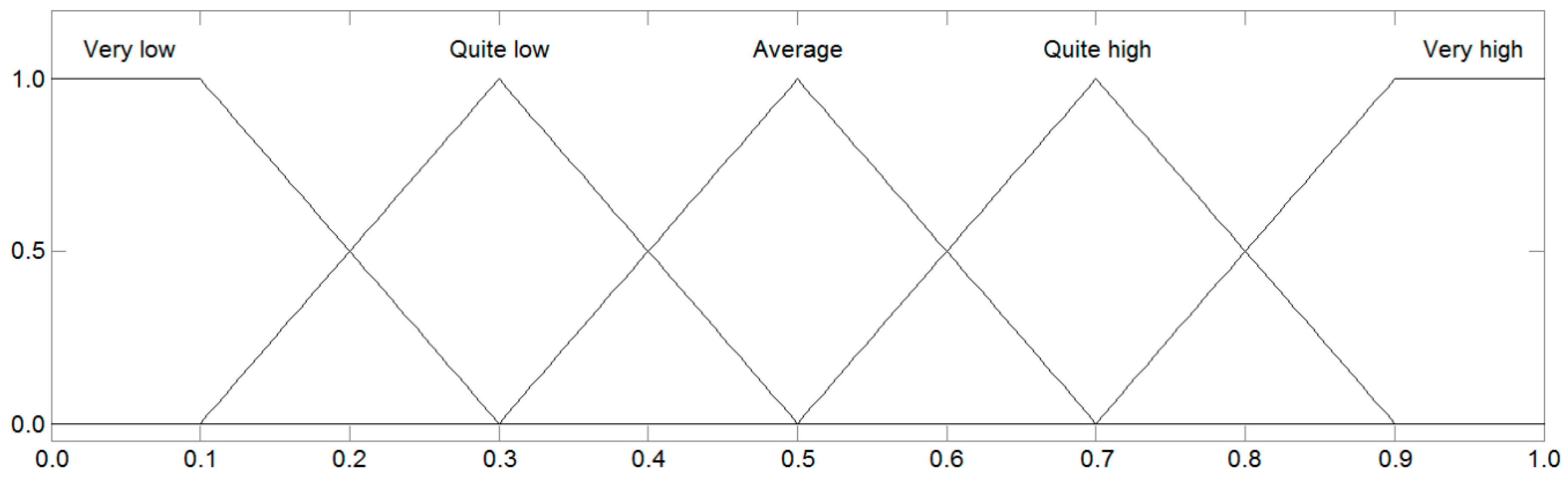
| Fuzzy Set of Linguistic Values for R | Description of the Variable y | Fuzzy Evaluation of Membership μ(y) | |
|---|---|---|---|
| Very high | Vh | About or above 0.9 | (0.7; 0.9; 1.0; 1.0) |
| Quite high | Qh | About 0.7 | (0.5; 0.7; 0.7; 0.9) |
| Average | Av | About 0.5 | (0.3; 0.5; 0.5; 0.7) |
| Quite low | Ql | About 0.3 | (0.1; 0.3; 0.3; 0.5) |
| Very low | Vl | About or below 0.1 | (0.0; 0.0; 0.1; 0.3) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Plebankiewicz, E.; Wieczorek, D. Adaptation of a Cost Overrun Risk Prediction Model to the Type of Construction Facility. Symmetry 2020, 12, 1739. https://doi.org/10.3390/sym12101739
Plebankiewicz E, Wieczorek D. Adaptation of a Cost Overrun Risk Prediction Model to the Type of Construction Facility. Symmetry. 2020; 12(10):1739. https://doi.org/10.3390/sym12101739
Chicago/Turabian StylePlebankiewicz, Edyta, and Damian Wieczorek. 2020. "Adaptation of a Cost Overrun Risk Prediction Model to the Type of Construction Facility" Symmetry 12, no. 10: 1739. https://doi.org/10.3390/sym12101739
APA StylePlebankiewicz, E., & Wieczorek, D. (2020). Adaptation of a Cost Overrun Risk Prediction Model to the Type of Construction Facility. Symmetry, 12(10), 1739. https://doi.org/10.3390/sym12101739





