Truncated-Exponential-Based Appell-Type Changhee Polynomials
Abstract
:1. Introduction and Preliminaries
2. Truncated-Exponential-Based Appell-Type Changhee Polynomials
- (i)
- .
- (ii)
- is neither an even nor odd function for .
- (iii)
- .
- (iv)
- .
- (v)
- where
- (vi)
- where
3. Differential Formulas
4. Integral Formulas
5. Inequalities Involving Integrals
- (i)
- [Inequality for the arithmetic and geometric mean]where and ;
- (ii)
- [Hermite–Hadamard type inequality]where and .
- (iii)
- where and ;
- (iv)
- where and .
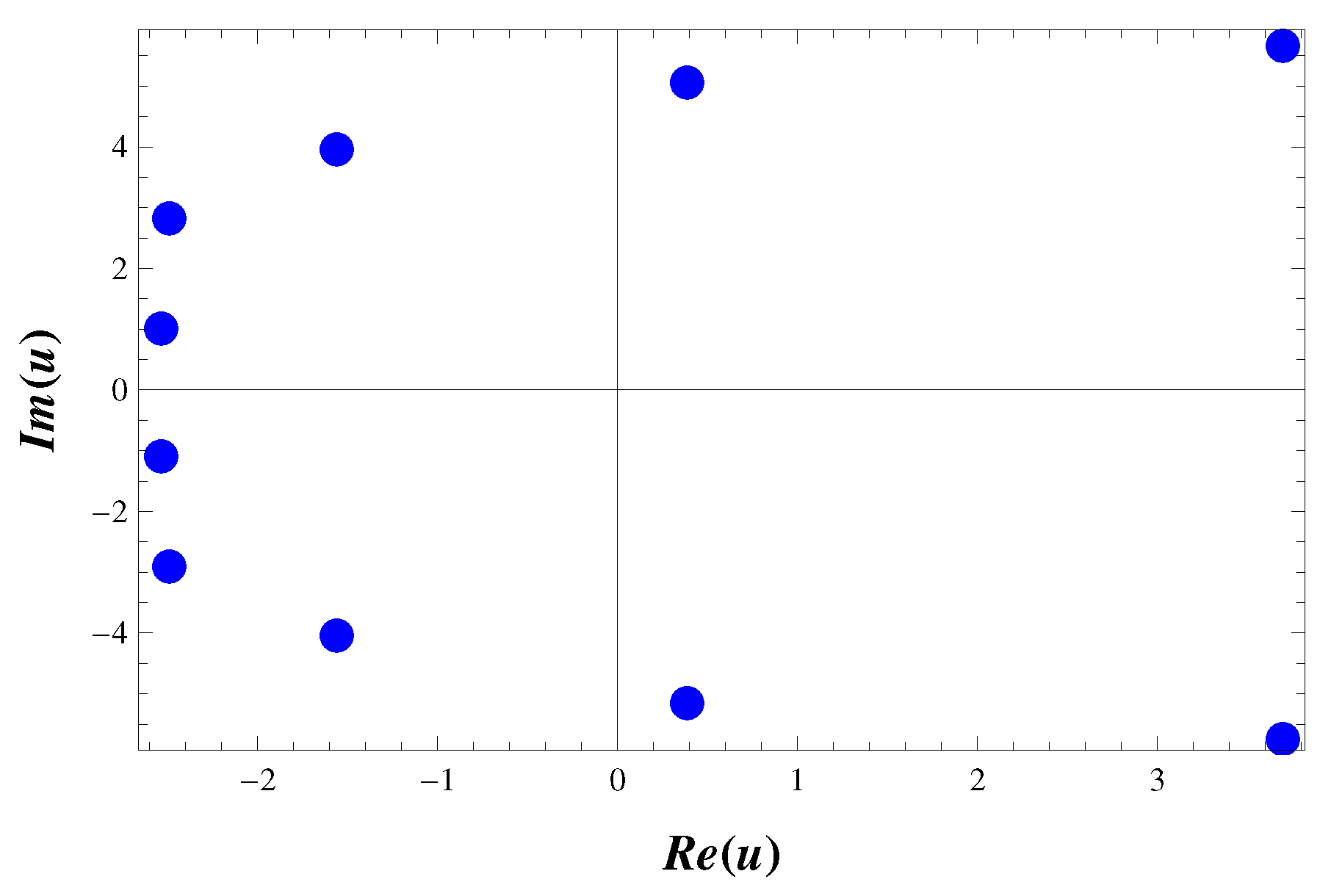
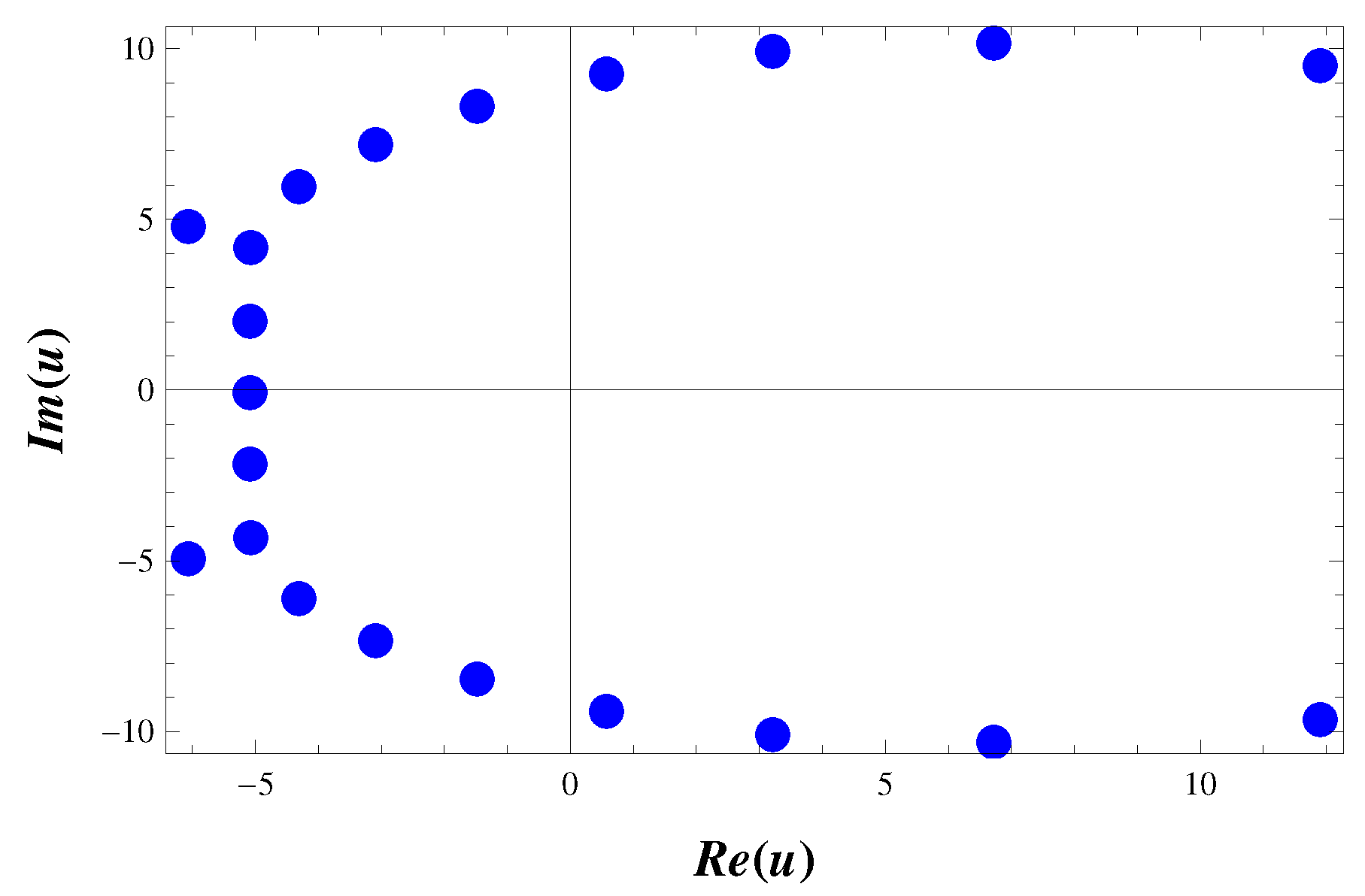
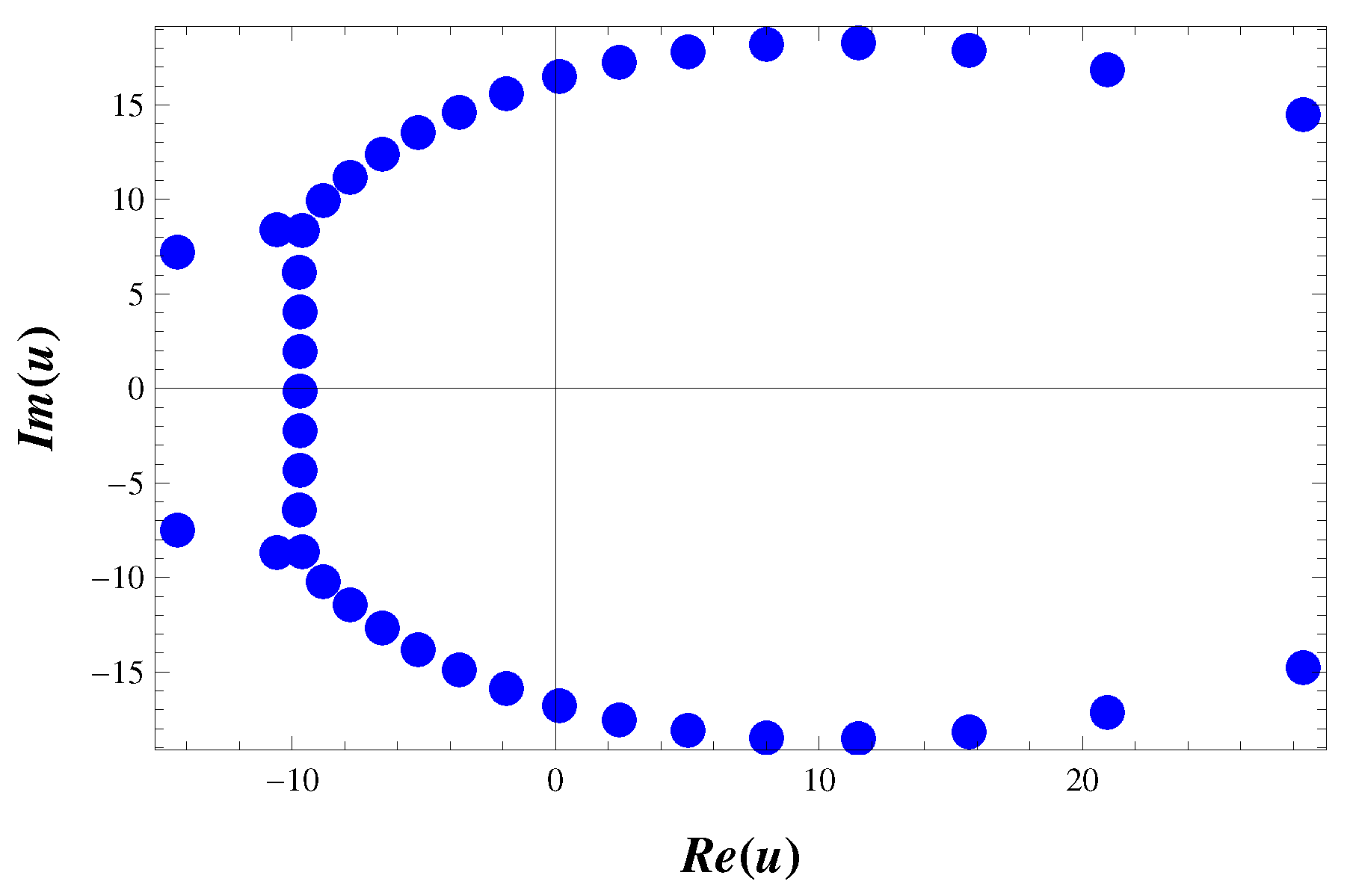
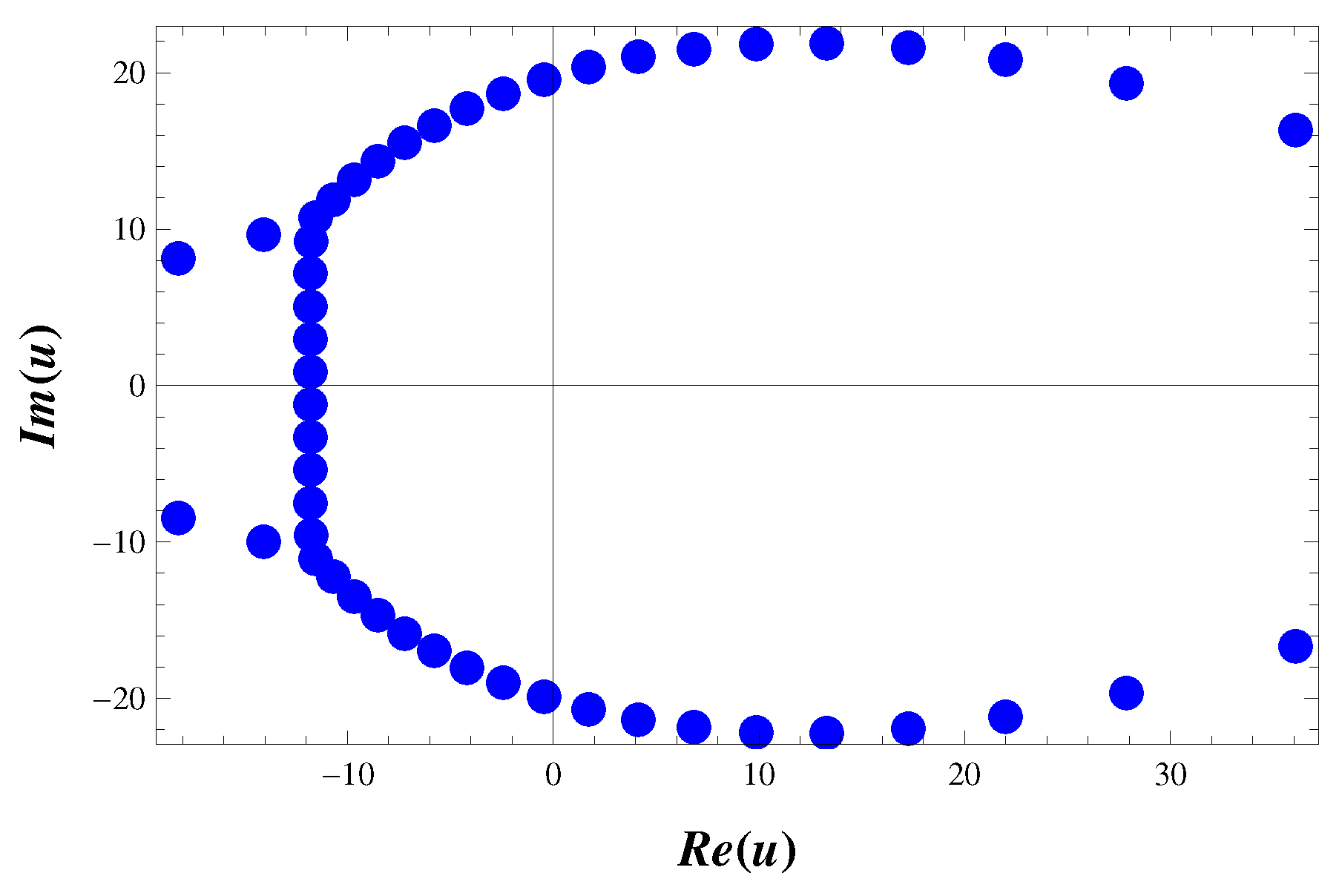
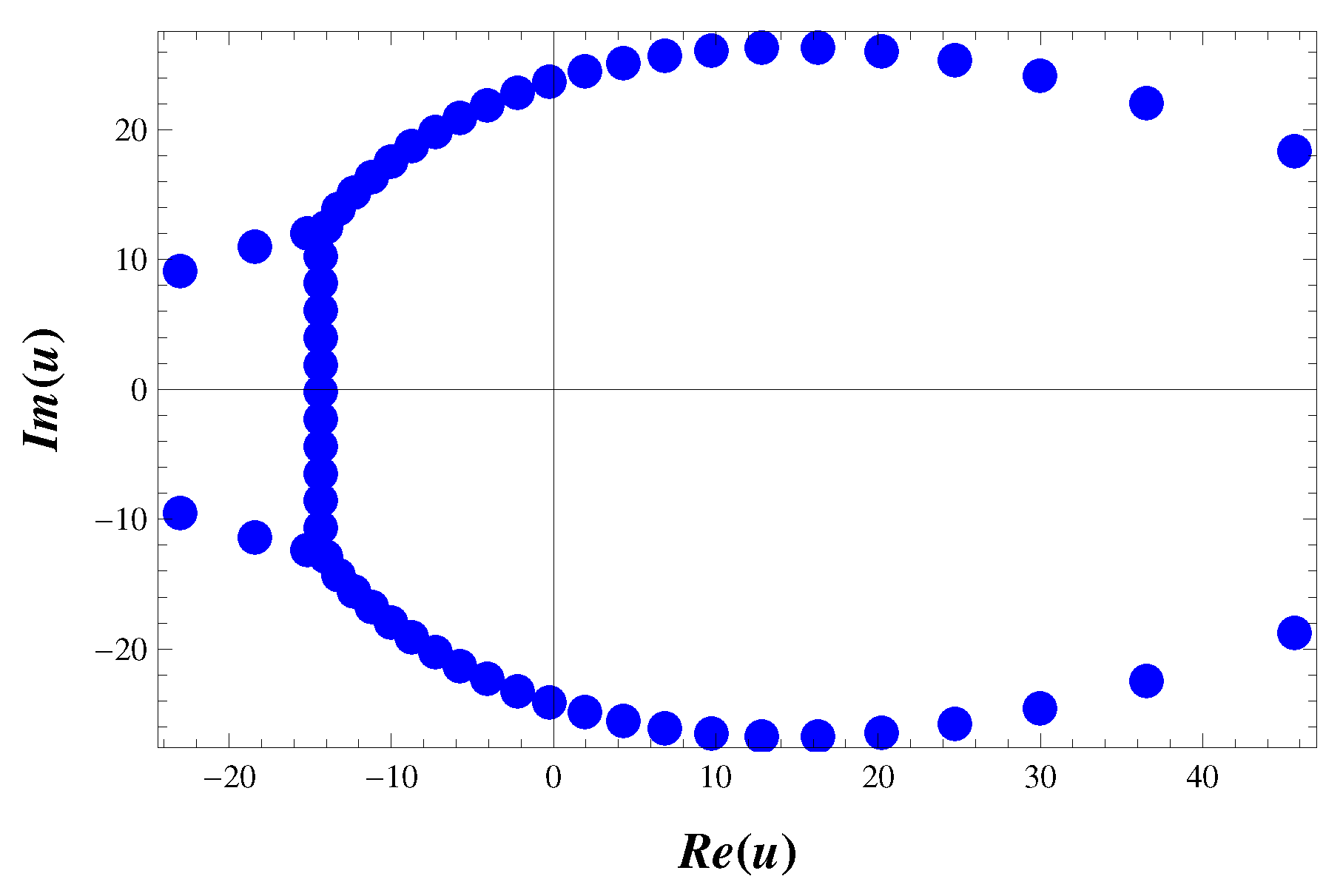
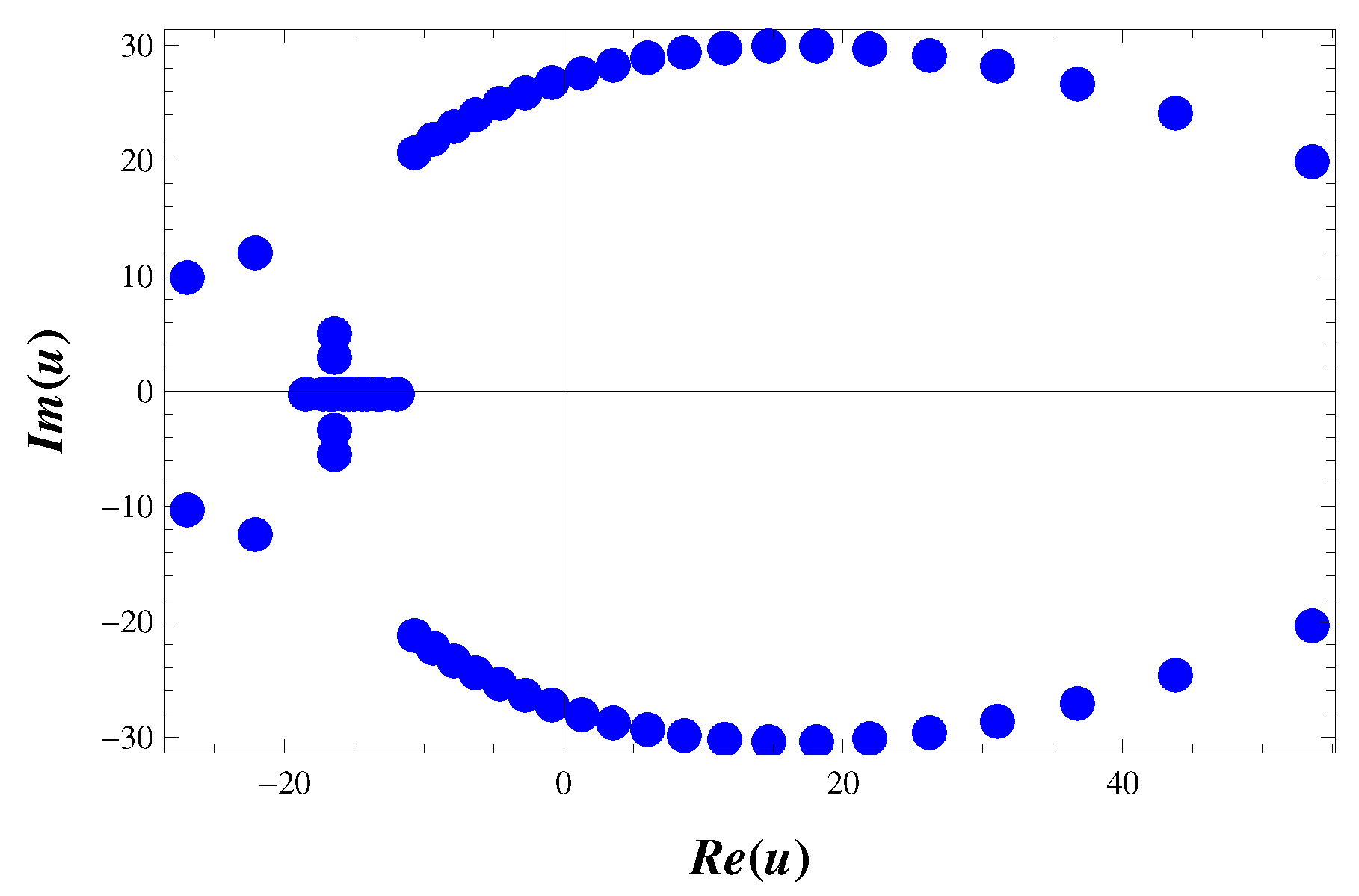
6. Zero Distributions of the Polynomials Via Graphical Approach
- (i)
- All possible zeros of are located in symmetric places with respect to the real axis.Indeed, let be a zero of . Thenwhere denotes the conjugate of . This implies that is also a zero of .
- (ii)
- The polynomial in (57) is a polynomial in the variable u of degree n, all of whose coefficients are positive rational numbers. So, has n zeros, counting multiplicities, in the finite complex plane. Explicitly,whereIn particular,Indeed, we can rewrite (57) as follows:where are such that . The right-handed series of is a geometric series with common ratio , the first term , and the number of terms . We thus have the above explicit expression (58) with (59). The first statement follows immediately from this explicit expression. The second one is implied by fundamental theorem of algebra (see, e.g., ([61], p. 1), ([41], p. 77)).
- (iii)
- We have observed the 78 graphs of zeros of the polynomials from to . We choose to demonstrate only 9 graphs as below Figure 1, Figure 2, Figure 3, Figure 4, Figure 5, Figure 6, Figure 7, Figure 8 and Figure 9.In view of those graphs, we find the followings:
- (a)
- If = 0, then .
- (b)
- If =0, then .
- (c)
- The number of zeros of with is less than the number of zeros of with when .
- (d)
- The longest straight distance from the origin of zeros of with is greater than that of zeros of with , when . That is,where .
- (iv)
- [Approximation of the real zero ofIn view of (i) and (ii) in this section, the polynomials are found to have one real zero. are real-valued infinitely differentiable functions on the interval . Indeed, here, only twice differentiability is sufficient. To approximate the real zeros of , we can use Newton–Raphson’s theorem (see, e.g., [58], pp. 262–263). We try to apply this theorem to find the real zero of the polynomialFirst set in to get . We see that is very near at the real zero c, say. Let . Then consider the sequence given byFrom this recursive relation, we computeFrom this, we may guess that the sequence is increasing and converges to the real zero as .
7. Conclusions, Remarks, and Open Questions
- (i)
- All of the zeros of are observed to be simple, that is, all the zeros are distinct. Are the zeros of , when is greater than 80, are distinct?
- (ii)
- Can the observations (a), (b), (c), and (d) in Section 6 as well as some other ones (if any) be generalized when is greater than 80?.
- (iii)
- From (60), how can we determine (or approximate) and such thatfor ?For example, since are zeros of , and satisfy
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Bayad, A.; Hamahata, Y. Poly-Euler polynomials and Arakawa-Kaneko type zeta functions. Funct. Approx. Comment. Math. 2014, 51, 7–22. [Google Scholar]
- Dattoli, G.; Cesarano, C.; Sacchetti, D. A note on truncated polynomials. Appl. Math. Comput. 2003, 134, 595–605. [Google Scholar] [CrossRef]
- Khan, S.; Nahid, T. Finding non-linear differential equations and certain identities for the Bernoulli-Euler and Bernoulli-Genocchi numbers. SN Appl. Sci. 2019, 1, 217. [Google Scholar] [CrossRef] [Green Version]
- Khan, N.; Usman, T.; Choi, J. A new class of generalized polynomials. Turk. J. Math. 2018, 42, 1366–1379. [Google Scholar]
- Kim, T. p-adic q-integrals associated with the Changhee-Barnes’ q-Bernoulli polynomials. Integral Transform. Spec. Funct. 2004, 15, 415–420. [Google Scholar] [CrossRef]
- Kim, T.; Kim, D.S. Differential equations associated with Catalan-Daehee numbers and their applications. RACSAM 2017, 111, 1071–1081. [Google Scholar] [CrossRef]
- Kim, T.; Kim, D.S.; Kwon, H.I.; Seo, J.J. Revisit nonlinear differential equations associated with Bernoulli numbers of the second kind. Glob. J. Pure Appl. Math. 2016, 12, 1893–1901. [Google Scholar]
- Kim, D.S.; Kim, T.; Seo, J.J. A note on Changhee polynomials and numbers. Adv. Stud. Theor. Phys. 2013, 7, 993–1003. [Google Scholar] [CrossRef]
- Kim, D.S.; Kim, T.; Seo, J.J.; Lee, S.-H.; Schork, M. Higher-order Changhee numbers and polynomials. Adv. Stud. Theor. Phys. 2014, 8, 365–373. [Google Scholar] [CrossRef] [Green Version]
- Kim, T.; Kim, D.S. A note on nonlinear Changhee differential equations. Russ. J. Math. Phys. 2016, 23, 88–92. [Google Scholar] [CrossRef]
- Kurt, B. Notes on the poly-Korobov type polynomials and related polynomials. Filomat 2020. accepted. [Google Scholar]
- Kurt, V. On the generalized q-poly-Euler polynomials of second kind. Filomat 2020. accepted. [Google Scholar]
- Kurt, B.; Simsek, Y. Notes on generalization of the Bernoulli type polynomials. Appl. Math. Comput. 2011, 218, 906–911. [Google Scholar] [CrossRef]
- Lee, J.G.; Jang, L.-C.; Seo, J.-J.; Choi, S.-K.; Kwon, H.I. On Appell-type Changhee polynomials and numbers. Adv. Differ. Equ. 2016, 2016, 160. [Google Scholar] [CrossRef] [Green Version]
- Luo, Q.M.; Guo, B.N.; Qi, F.; Debnath, L. Generalization of Bernoulli numbers and polynomials. Int. J. Math. Math. Sci. 2003, 61, 3769–3776. [Google Scholar] [CrossRef] [Green Version]
- Ozden, H.; Cangul, I.N.; Simsek, Y. Generalized q-Stirling numbers and their interpolation functions. Axioms 2013, 2, 10–19. [Google Scholar] [CrossRef]
- Simsek, Y. New families of special numbers for computing negative order Euler numbers and related numbers and polynomials. Appl. Anal. Discret. Math. 2018, 12, 1–35. [Google Scholar] [CrossRef]
- Wang, N.L.; Li, H. Some identities on the higher-order Daehee and Changhee numbers. Pure Appl. Math. J. 2015, 5, 33–37. [Google Scholar]
- Andrews, L.C. Special Functions for Engineers and Applied Mathematicians; Macmillan Publishing Company: New York, NY, USA, 1985. [Google Scholar]
- Schur, I. Einige Sätze über primzahlen mit anwendungen auf irreduzibilitätsfragen I. Sitzungsberichte Preuss. Akad. Wiss. Phys.-Math. Klasse 1929, 125–136, Also in Gesammelte Abhandlungen, Band III, 140–151. [Google Scholar]
- Schur, I. Einige Sätze über primzahlen mit anwendungen auf irreduzibilitätsfragen II. Sitzungsberichte Preuss. Akad. Wiss. Phys.-Math. Klasse 1929, 370–391, Also in Gesammelte Abhandlungen, Band III, 152–173. [Google Scholar]
- Coleman, R. On the Galois groups of the exponential Taylor polynomials. Enseign. Math. 1987, 33, 183–189. [Google Scholar]
- Erdös, P. A theorem of Sylvester and Schur. J. Lond. Math. Soc. 1934, 9, 282–288. [Google Scholar] [CrossRef]
- Ali, M.; Khan, S. Finding results for certain relatives of the Appell polynomials. Bull. Korean Math. Soc. 2019, 56, 151–167. [Google Scholar] [CrossRef]
- Araci, S.; Riyasat, M.; Nahid, T.; Khan, S. Certain results for unified Apostol type-truncated exponential-Gould-Hopper polynomials and their relatives. arXiv 2006, arXiv:2006.12970v1. [Google Scholar]
- Barakat, R. Evaluation of the incomplete Gamma function of imaginary argument by Chebyshev polynomials. Math. Comput. 1961, 15, 7–11. [Google Scholar] [CrossRef]
- Belingeri, C.; Dattoli, G.; Khan, S.; Ricci, P.E. Monomiality and multi-index multi-variable special polynomials. Integral Transforms Spec. Funct. 2007, 18, 449–458. [Google Scholar] [CrossRef]
- Choi, J.; Jabee, S.; Shadab, M. Some identities associated with 2-variable truncated exponential based Sheffer polynomial sequences. Commun. Korean Math. Soc. 2020, 35, 533–546. [Google Scholar] [CrossRef]
- Chung, W.S.; Hassanabadi, H. Truncated exponential polynomials and truncated coherent states. Eur. Phys. J. Plus 2020, 135, 556. [Google Scholar] [CrossRef]
- Dattoli, G.; Migliorati, M. The truncated exponential polynomials, the associated Hermite forms and applications. Int. J. Math. Math. Sci. 2006, 2006, 98175. [Google Scholar] [CrossRef] [Green Version]
- Dattoli, G.; Ricci, P.E.; Marinelli, L. Generalized truncated exponential polynomials and applications. Rend. Istit. Mat. Univ. Trieste 2002, 34, 9–18. [Google Scholar]
- Dattoli, G.; Torre, A.; Carpanese, M. The Hermite-Bessel functions: A new point of view on the theory of generalized Bessel functions. Radiat. Phys. Chem. 1998, 3, 221–228. [Google Scholar]
- Gori, F. Flattened Gaussian beams. Opt. Commun. 1994, 107, 335–341. [Google Scholar] [CrossRef]
- Joung, H. Asymptotic expansions of recursion coefficients of orthogonal polynomials with truncated exponential weights. Nagoya Math. J. 2002, 165, 79–89. [Google Scholar] [CrossRef] [Green Version]
- Khan, S.; Yasmin, G.; Ahmad, N. On a new family related to truncated exponential and Sheffer polynomials. J. Math. Anal. Appl. 2014, 418, 921–937. [Google Scholar] [CrossRef]
- Khan, S.; Yasmin, G.; Ahmad, N. A Note on truncated exponential-based Appell polynomials. Bull. Malays. Math. Sci. Soc. 2017, 40, 373–388. [Google Scholar] [CrossRef]
- Kumam, W.; Srivastava, H.M.; Wani, S.A.; Araci, S.; Kumam, P. Truncated-exponential-based Frobenius-Euler polynomials. Adv. Differ. Equ. 2019, 2019, 530. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Araci, S.; Khan, W.A.; Acikgöz, M. A note on the truncated-exponential based Apostol-type polynomials. Symmetry 2019, 11, 538. [Google Scholar] [CrossRef] [Green Version]
- Srivastava, H.M.; Choi, J. Zeta and q-Zeta Functions and Associated Series and Integrals; Elsevier Science Publishers: Amsterdam, The Netherlands; London, UK; New York, NY, USA, 2012. [Google Scholar]
- Lim, D.; Qi, F. On the Appell type λ-Changhee polynomials. J. Nonlinear Sci. Appl. 2016, 9, 1872–1876. [Google Scholar] [CrossRef] [Green Version]
- Conway, J.B. Functions of One Complex Variable, 2nd ed.; Springer: New York, NY, USA; Berlin/Heidelberg, Germany, 1978. [Google Scholar]
- Appell, P. Sur une classe de polynômes. Ann. Sci. École Norm. Sup. 1880, 9, 119–144. [Google Scholar] [CrossRef] [Green Version]
- Boas, R.P.; Buck, R.C. Polynomial Expansions of Analytic Functions; Springer: Berlin/Heidelberg, Germany, 1964. [Google Scholar]
- Bretti, G.; Cesarano, C.; Ricci, P.E. Laguerre-type exponential and generalized Appell polynomials. Comput. Math. Appl. 2004, 48, 833–839. [Google Scholar] [CrossRef] [Green Version]
- Bretti, G.; He, M.X.; Ricci, P.E. On quadrature rules associated with Appell polynomials. Int. J. Appl. Math. 2002, 11, 1–14. [Google Scholar]
- Bretti, G.; Natalini, P.; Ricci, P.E. Generalizations of the Bernoulli and Appell polynomials. Abstr. Appl. Anal. 2004, 7, 613–623. [Google Scholar] [CrossRef] [Green Version]
- Bretti, G.; Ricci, P.E. Multidimensional extensions of the Bernoulli and Appell polynomials. Taiwanese J. Math. 2004, 8, 415–428. [Google Scholar] [CrossRef]
- Dattoli, G.; Ricci, P.E.; Cesarano, C. Differential equations for Appell type polynomials. Fract. Calc. Appl. Anal. 2002, 5, 69–75. [Google Scholar]
- Douak, K. The relation of the d-orthogonal polynomials to the Appell polynomials. J. Comput. Appl. Math. 1996, 70, 279–295. [Google Scholar] [CrossRef] [Green Version]
- He, M.X.; Ricci, P.E. Differential equation of Appell polynomials via the factorization method. J. Comput. Appl. Math. 2002, 139, 231–237. [Google Scholar] [CrossRef] [Green Version]
- Ismail, M.E.H. Remarks on differential equation of Appell polynomials via the factorization method. J. Comput. Appl. Math. 2003, 154, 243–245. [Google Scholar] [CrossRef] [Green Version]
- Roman, S. The Umbral Calculus; Academic Press: New York, NY, USA, 1984. [Google Scholar]
- Sheffer, I.M. A differential equation for Appell polynomials. Bull. Am. Math. Soc. 1935, 41, 914–923. [Google Scholar] [CrossRef] [Green Version]
- Shohat, J. The relation of the classical orthogonal polynomials to the polynomials of Appell. Am. J. Math. 1936, 58, 453–464. [Google Scholar] [CrossRef]
- Szegö, G. Orthogonal Polynomials; American Mathematical Society: Providence, RI, USA, 1939. [Google Scholar]
- Hardy, G.H.; Littlewood, J.E.; Pólya, G. Inequalities, 2nd ed.; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Set, E.; Choi, J.; Çelık, B. Certain Hermite–Hadamard type inequalities involving generalized fractional integral operators. RACSAM 2018, 112, 1539–1547. [Google Scholar] [CrossRef]
- Wade, W.R. An Introduction to Analysis, 4th ed.; Pearson Education: Upper Saddle River, NJ, USA, 2010. [Google Scholar]
- Kuijlaars, A.B.J.; Assche, W.V. The asymptotic zero distribution of orthogonal polynomials with varying recurrence coefficients. J. Approx. Theor. 1999, 99, 167–197. [Google Scholar] [CrossRef] [Green Version]
- Martínez-Finkelshteina, A.; Martínez-González, P.; Orive, R. On asymptotic zero distribution of Laguerre and generalized Bessel polynomials with varying parameters. J. Comput. Appl. Math. 2001, 133, 477–487. [Google Scholar] [CrossRef] [Green Version]
- Sheil-Small, T. Complex Polynomials; Cambrige University Press: Cambridge, UK, 2002. [Google Scholar]
- Dragomir, S.S.; Pearce, C.E.M. Selected Topics on Hermite–Hadamard Inequalities and Applications; RGMIA Monographs; Victoria University: Melbourne, Australia, 2000. [Google Scholar]
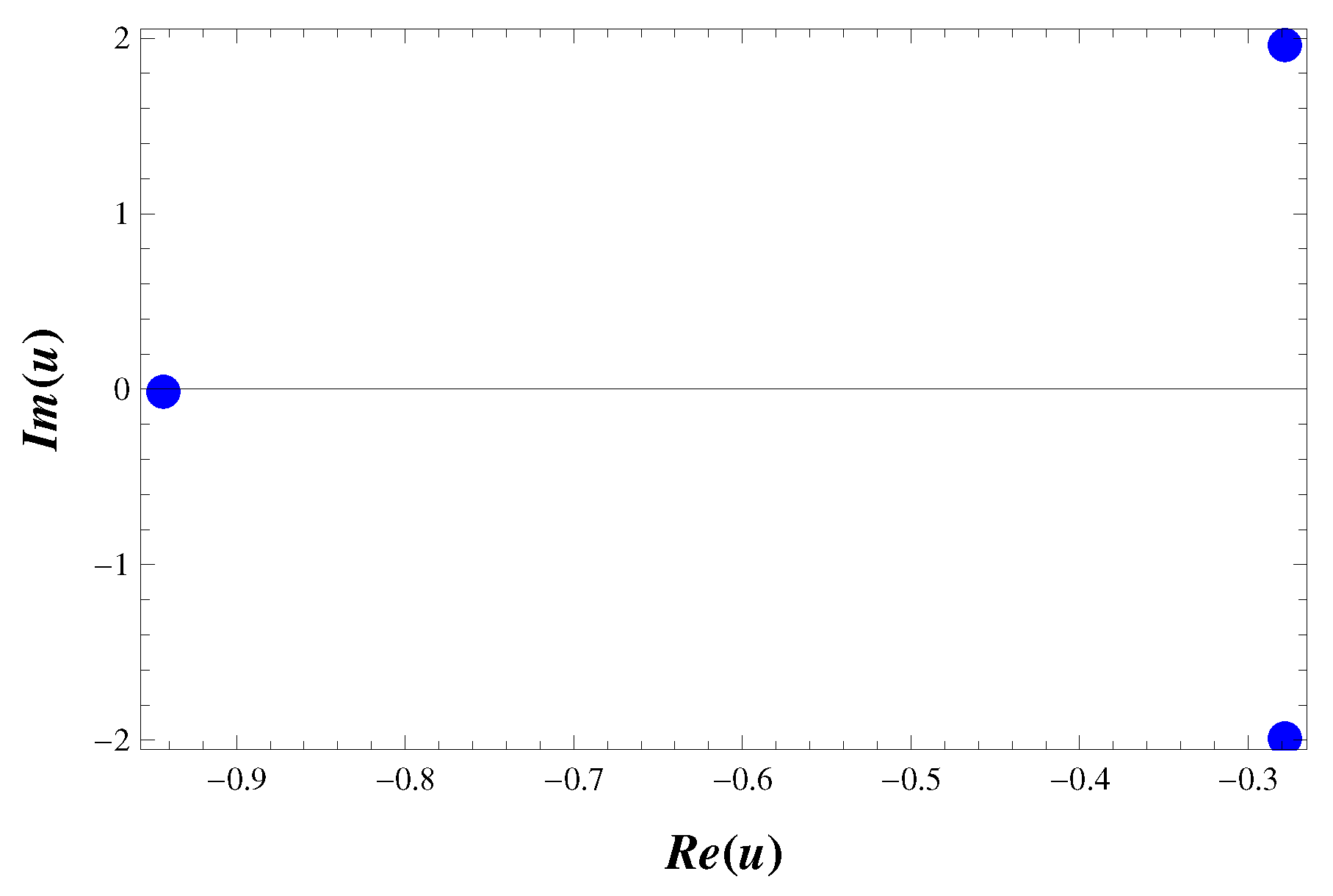


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nahid, T.; Alam, P.; Choi, J. Truncated-Exponential-Based Appell-Type Changhee Polynomials. Symmetry 2020, 12, 1588. https://doi.org/10.3390/sym12101588
Nahid T, Alam P, Choi J. Truncated-Exponential-Based Appell-Type Changhee Polynomials. Symmetry. 2020; 12(10):1588. https://doi.org/10.3390/sym12101588
Chicago/Turabian StyleNahid, Tabinda, Parvez Alam, and Junesang Choi. 2020. "Truncated-Exponential-Based Appell-Type Changhee Polynomials" Symmetry 12, no. 10: 1588. https://doi.org/10.3390/sym12101588
APA StyleNahid, T., Alam, P., & Choi, J. (2020). Truncated-Exponential-Based Appell-Type Changhee Polynomials. Symmetry, 12(10), 1588. https://doi.org/10.3390/sym12101588





