1. Introduction
Graph theory serves as an exceptionally useful tool in solving combinatorial problems in different areas including geometry, algebra, number theory, topology, and social systems. A graph is basically a model of relations, and it is used to embody the real-life problems entailing the relationships between objects. The vertices and edges of the graph are employed to represent the objects and the relations between objects, respectively. In several optimization problems, the available information is inexact or imprecise for various reasons such as the loss of information, a lack of evidence, imperfect statistical data and insufficient information. Generally, the uncertainty in real-life problems may be present in the information that defines the problem. Fuzzy graph (FG) models are advantageous mathematical tools for treating the combinatorial problems in various domains encompassing research, optimization, algebra, computing, environmental science and topology. Fuzzy graphical models are obviously better than graphical models because of the natural existence of vagueness and ambiguity. Originally, fuzzy set theory is required to deal with many complex issues having incomplete information. In 1965, Zadeh [
1] at first suggested the fuzzy set theory. Fuzzy set theory is a very powerful mathematical tool for solving approximate reasoning related problems. These notions effectively illustrate complex phenomena, which are not accurately described using classical mathematics. In a fuzzy set, each object is assigned a single value in the interval [0,1] reflecting its grade of membership. This single value does not allow a separation of evidence for membership and evidence against membership. Hence, Gau and Buehrer [
2] organized the fuzzy set theory by presenting the VS notion through changing the value of an element in a set with a sub-interval of
. Specifically, a true membership function of
and false membership function of
are used to define the boundaries of the membership degree. This kind of reasoning is also called interval membership, as opposed to point membership in the context of fuzzy sets. The VSs describe more possibilities than fuzzy sets. A VS is more initiative and helpful due to the existence of false membership degree. VSs are higher order fuzzy sets. The application of higher order fuzzy sets complicates the solution procedure, but if the complexity on computation time, computation volume, or memory space is not a matter of concern, then better results can be achieved. There are some interesting features for handling vague data that are unique to vague sets. For example, VSs allow for a more intuitive graphical representation of vague data, which significantly facilitates better analysis in data relationships, incompleteness, and similarity measures.
Many real-word phenomena provided motivation to describe the FGs. Kauffman [
3] define fuzzy graphs using Zadeh’s fuzzy relation [
4,
5]. Akram et al. [
6,
7,
8,
9,
10,
11] introduced several concepts in FGs. Rashmanlou et al. [
12,
13,
14,
15,
16] introduced some properties in FGs. Product operations on graphs play a very important role in graph theory. They have applications in different branches, such as coding theory, network designs, chemical graph theory and others. Many scholars discussed product operations on various generalized FGs. Mordeson and Peng [
17] defined some of these product operations on FGs. Subsequently, utilizing these operations, the degree of the vertices is obtained from two FGs in [
18]. Sahoo and Pal [
19] presented some operations on intuitionistic fuzzy graphs. FG theory is growing rapidly, with numerous applications in many domains, including networking, communication, data mining, planning, and scheduling. However, Rosenfeld [
20] described another detailed definition including fuzzy vertex and fuzzy edges and various fuzzy analogs of graph theoretic concepts such as paths, cycles, connectedness etc. A vague graph (VG) is a generalized structure of a fuzzy graph that gives more precision, flexibility and compatibility to a system when compared with systems that are designed using fuzzy graphs. In addition, a VG can focus on resolving the uncertainty associated with the inconsistent and indeterminate information of any real-world problem, where fuzzy graphs may not bring about satisfactory results. Ramakrishna [
21] recommended the VG notion and evaluated some of their features. Borzooei and Rashmanlou [
22,
23,
24,
25] investigated different concepts of VG. Irregularity definitions have been of high significance in the study of network heterogeneity, which has implications in networks found across biology, ecology, technology, and economy. Several graph statistics have existed that have been proposed, many of which are based on the number of vertices in a graph and their degrees. The concepts of the irregularity play a significant role in both graph theory and application in the vague environment. The characterization of highly irregular graphs has also been applied to the question of heterogeneity, yet all of these fail to shed enough light on real-world situations. Efforts continue to be made to find appropriate ways to quantify network heterogeneity; so, Gani and Radha [
26,
27,
28,
29] introduced irregular FGs, some properties of conjunction of FGs, and new concepts in regular FGs. Samanta and Pal [
30] described irregular bipolar fuzzy graphs. A graph structure (GS) is a generalization of simple graphs. GSs are very useful in the study of different domains of computer science and computational intelligence. GS was introduced first by Sampathkumar [
31]. Fuzzy graph structures (FGSs) are more useful than GSs because they deal with the uncertainty and ambiguity of many real-world phenomena, and they are widely useful in the study of some structures, like graphs, signed graphs, semi graphs, edge-colored graphs and edge-labeled graphs. FGSs have many applications in solving various problems of several domains, including communication, data mining, image capturing, and image segmentation. Dinesh [
32] defined the concept of FGSs and investigated some related concepts. Ramakrishna and Dinesh [
33,
34] studied generalized FGSs. Shao et al. [
35] introduced new concepts in intuitionistic fuzzy graphs.
VGSs are the generalization of FGSs and extremely useful in the study of some structures, like graphs, colored graphs, signed graphs, and edge-labeled graphs. VGSs are more useful than GSs because they deal with the uncertainty and ambiguity of many real-word phenomena. Hence, in this paper we introduced three operations on VGSs, namely, maximal product, rejection, residue product, and represented some results concerning their degrees and total degrees. As the concept of regularity led to many developments in the structural theory of graphs, meanwhile, the irregular graphs have also been significant while dealing with network heterogeneity, which as many applications across biology, economy, and technology. So, in this research, we explained special concepts of irregular-VGSs with several key properties. Decision making in medical diagnosis is a vast research field in medical science. Hence, we described an application of VGS by applying the new distance measure for medical diagnosis.
3. Vague Graph Structures
Product operations on graphs play a very important role in graph theory. In various situations they present a suitable construction means. The first definition of product in fuzzy graphs was introduced in [
17]. However, for the first time in this section, two types of products called maximal product, and residue product between the two VGSs were being introduced, which are widely employed in computer networks, and accordingly their properties were evaluated. In addition, another new operation called rejection is introduced, too. Note that the degree and the total degree of vertices that were calculated in the maximal product, rejection and residue product; are presented in (
Appendix A).
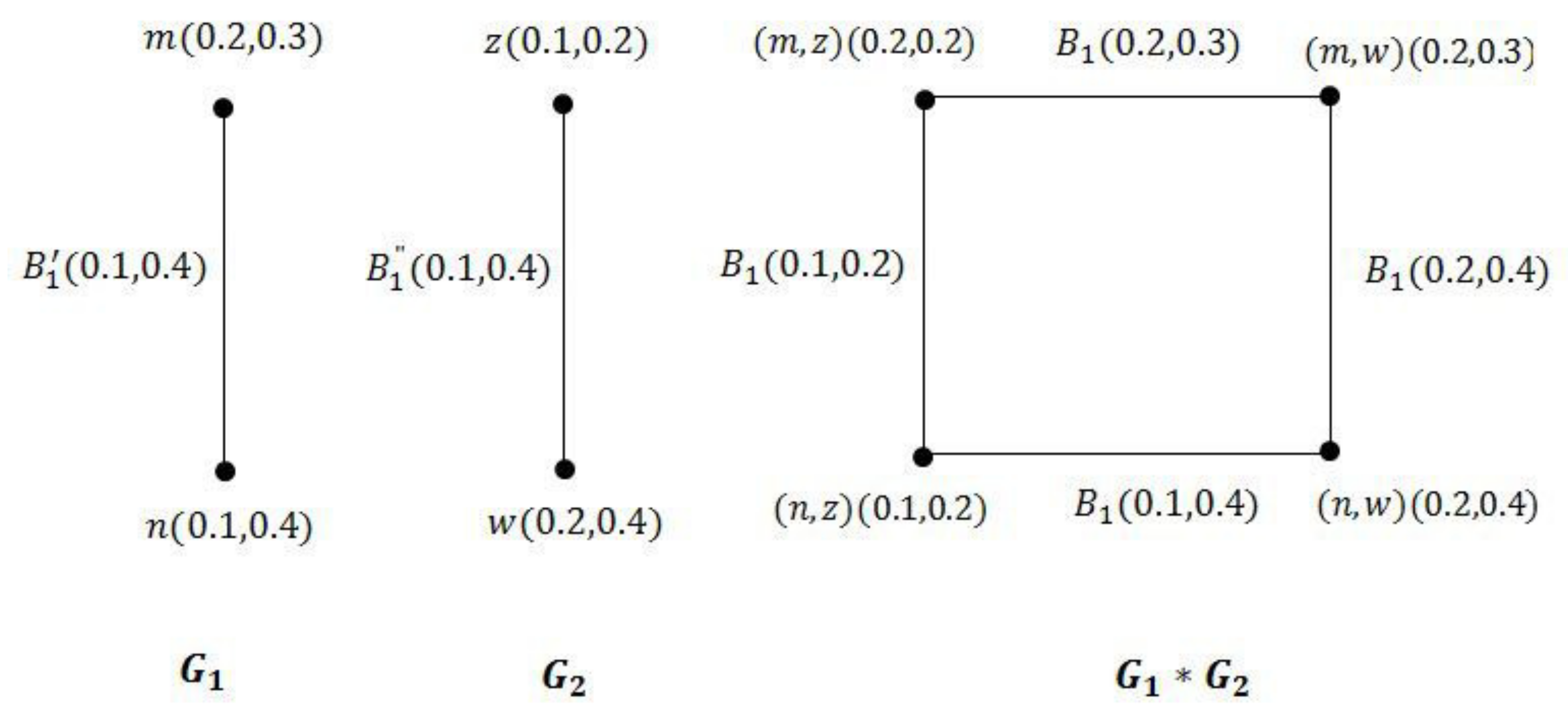
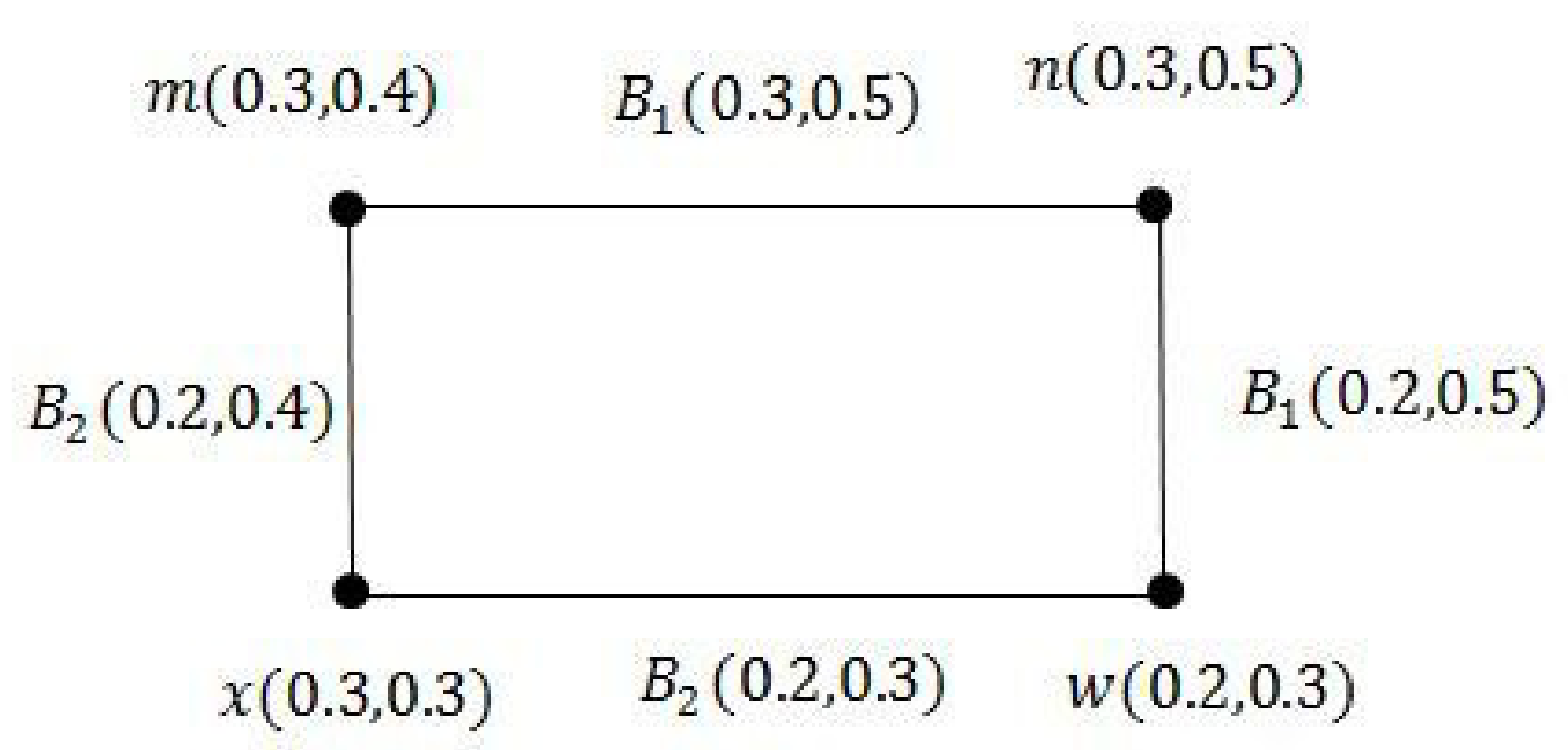
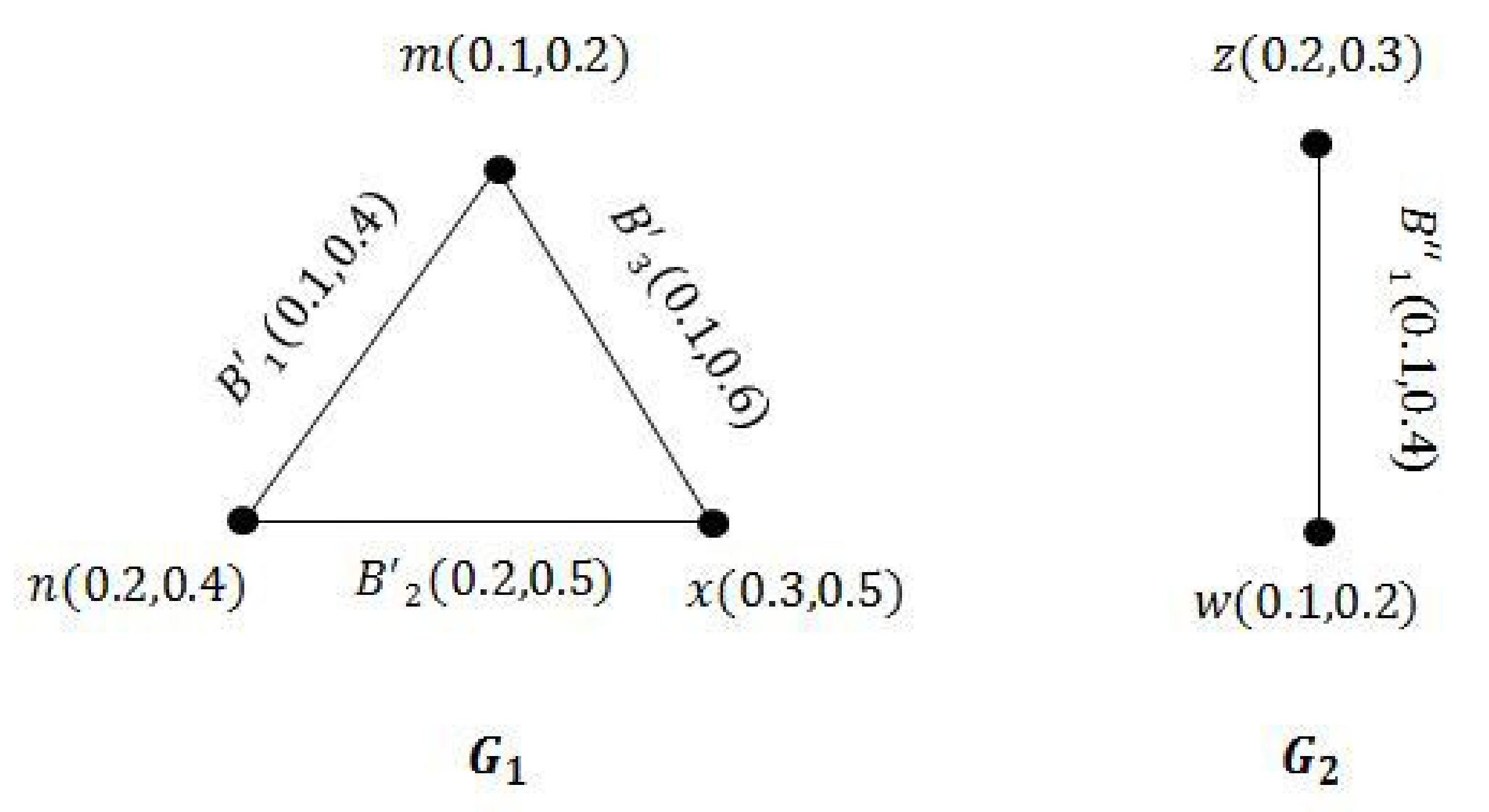
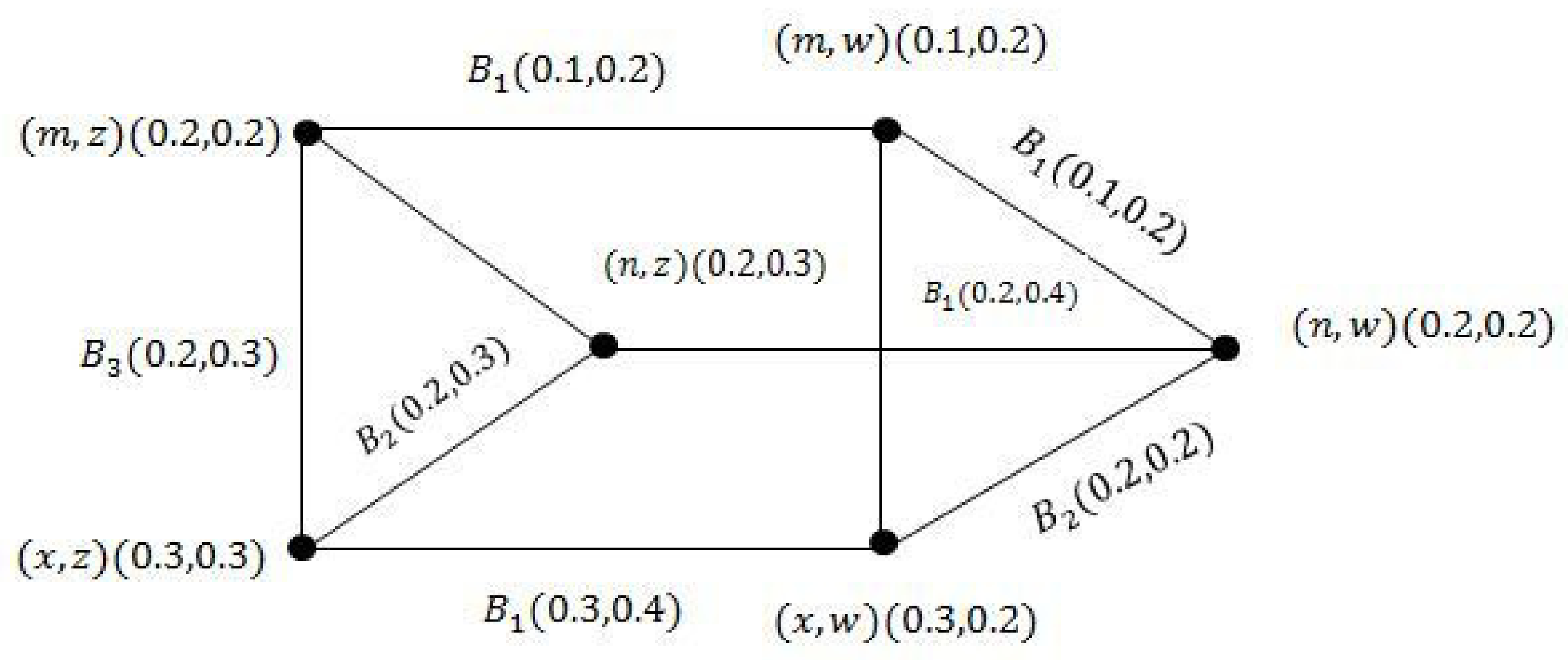
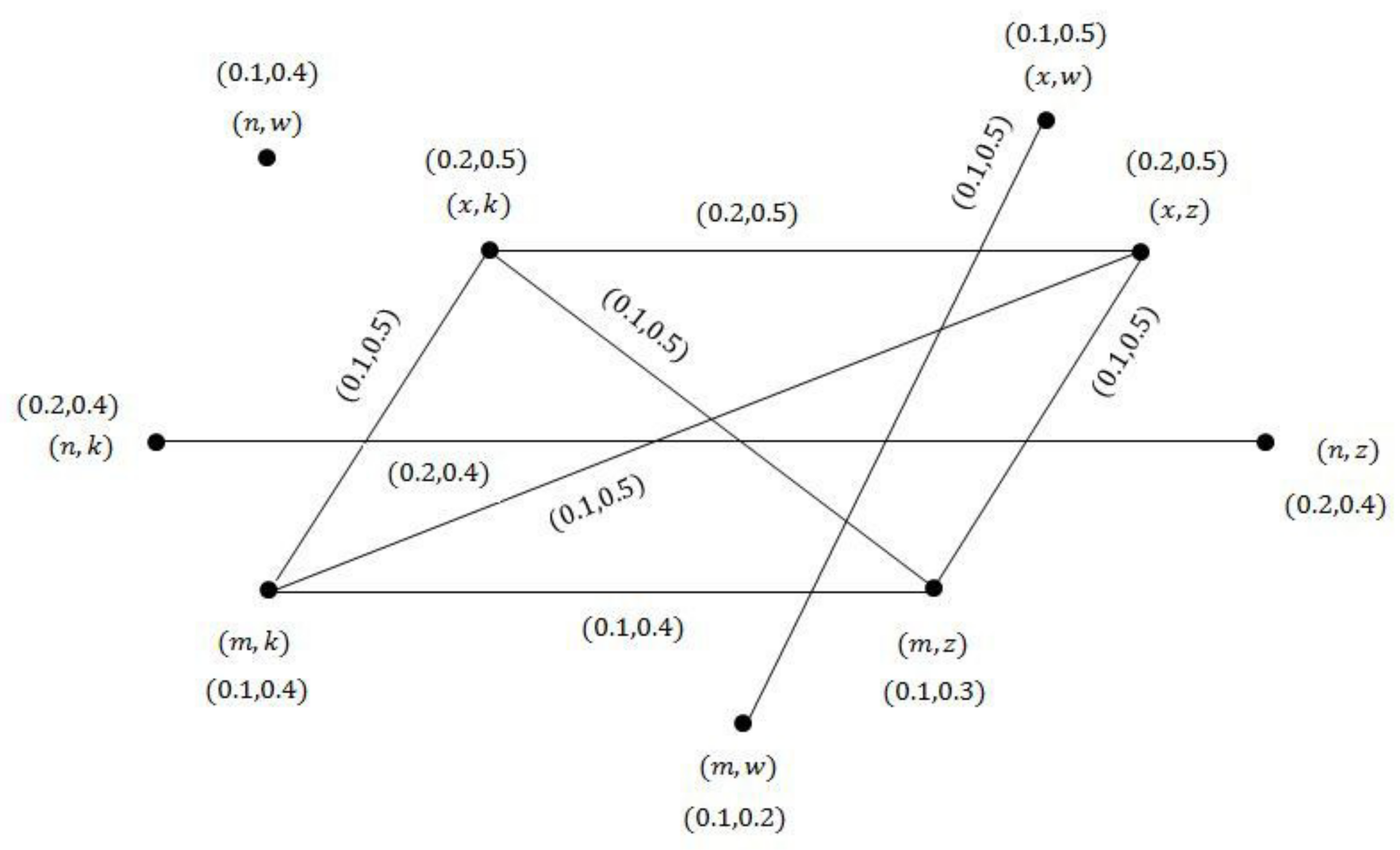
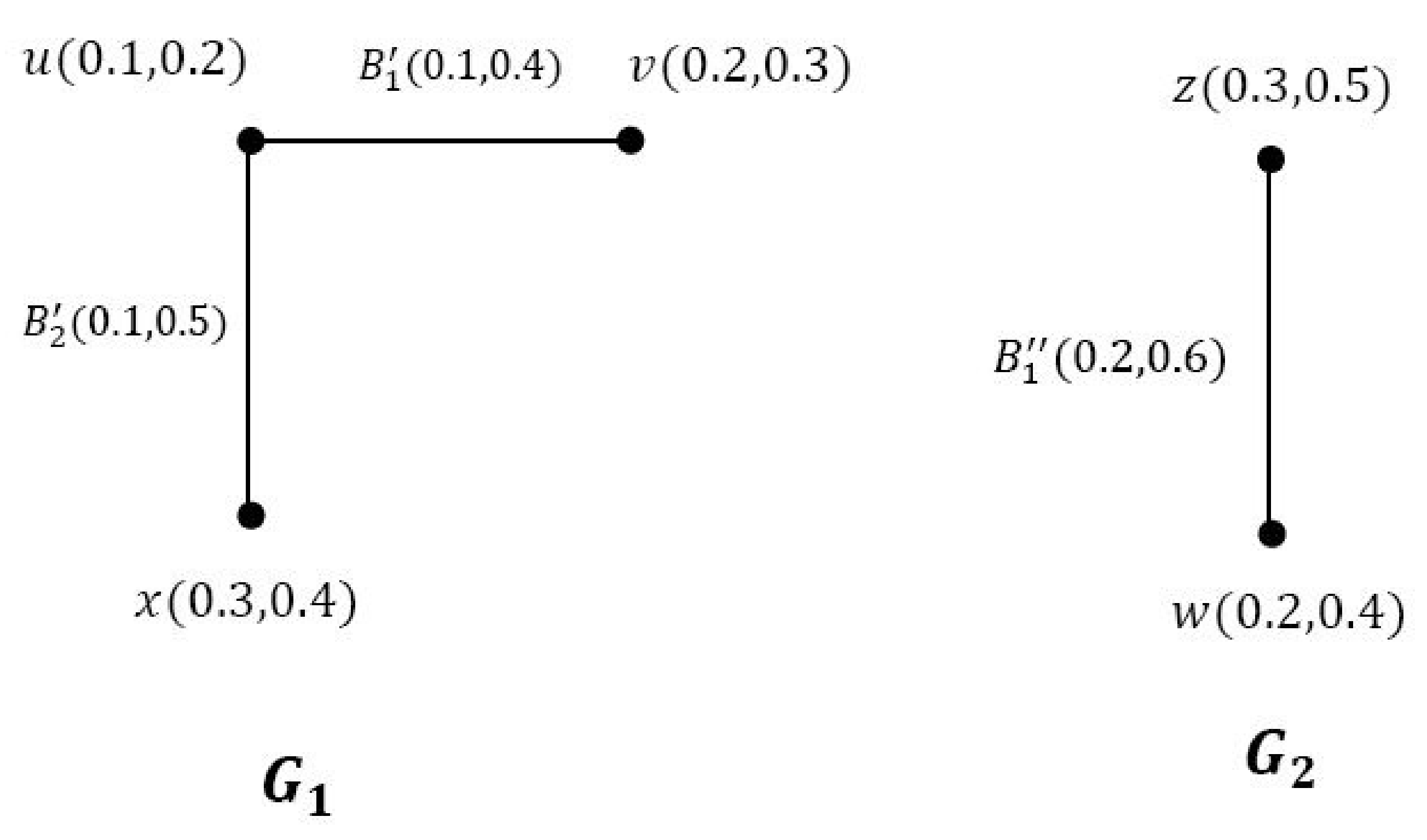
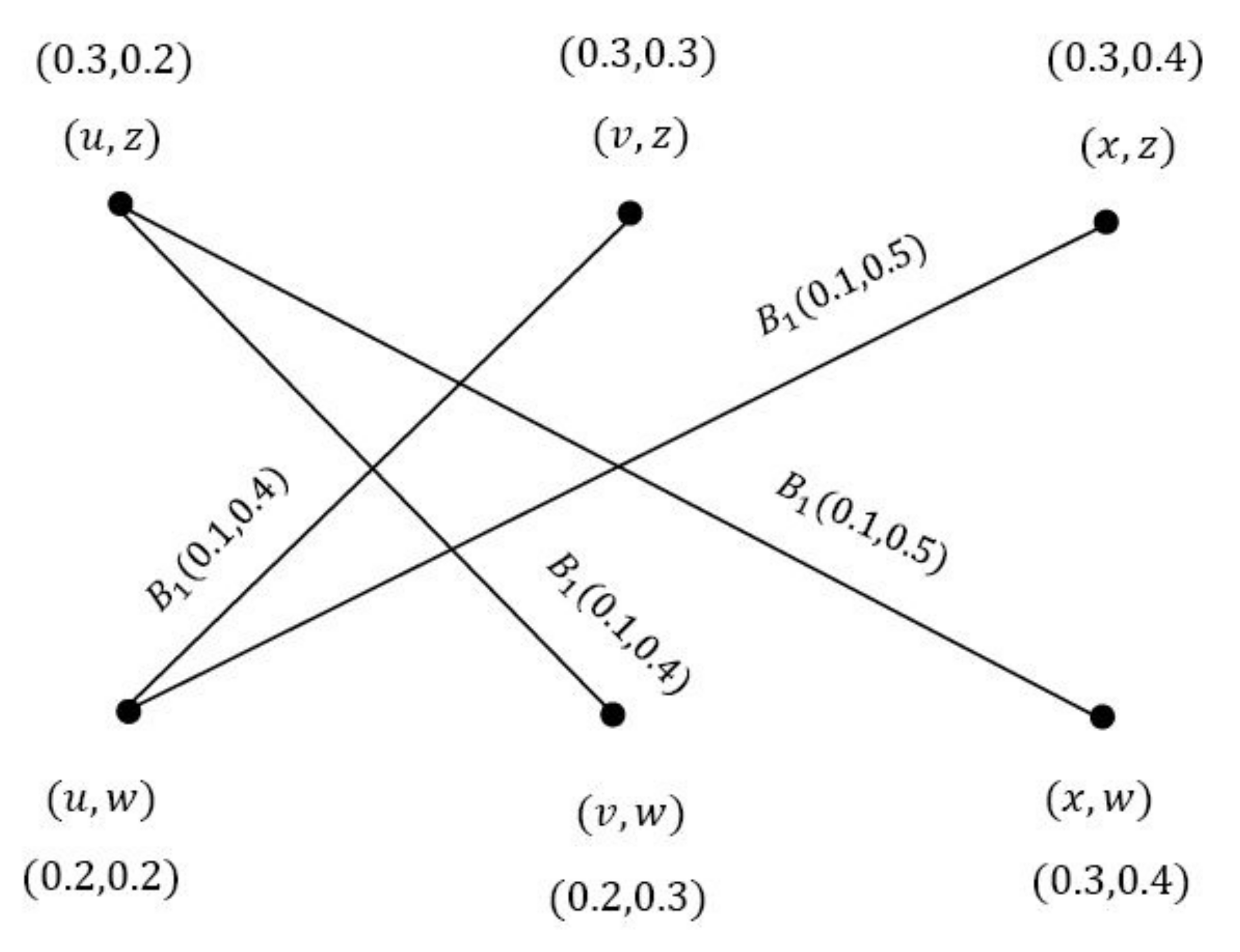
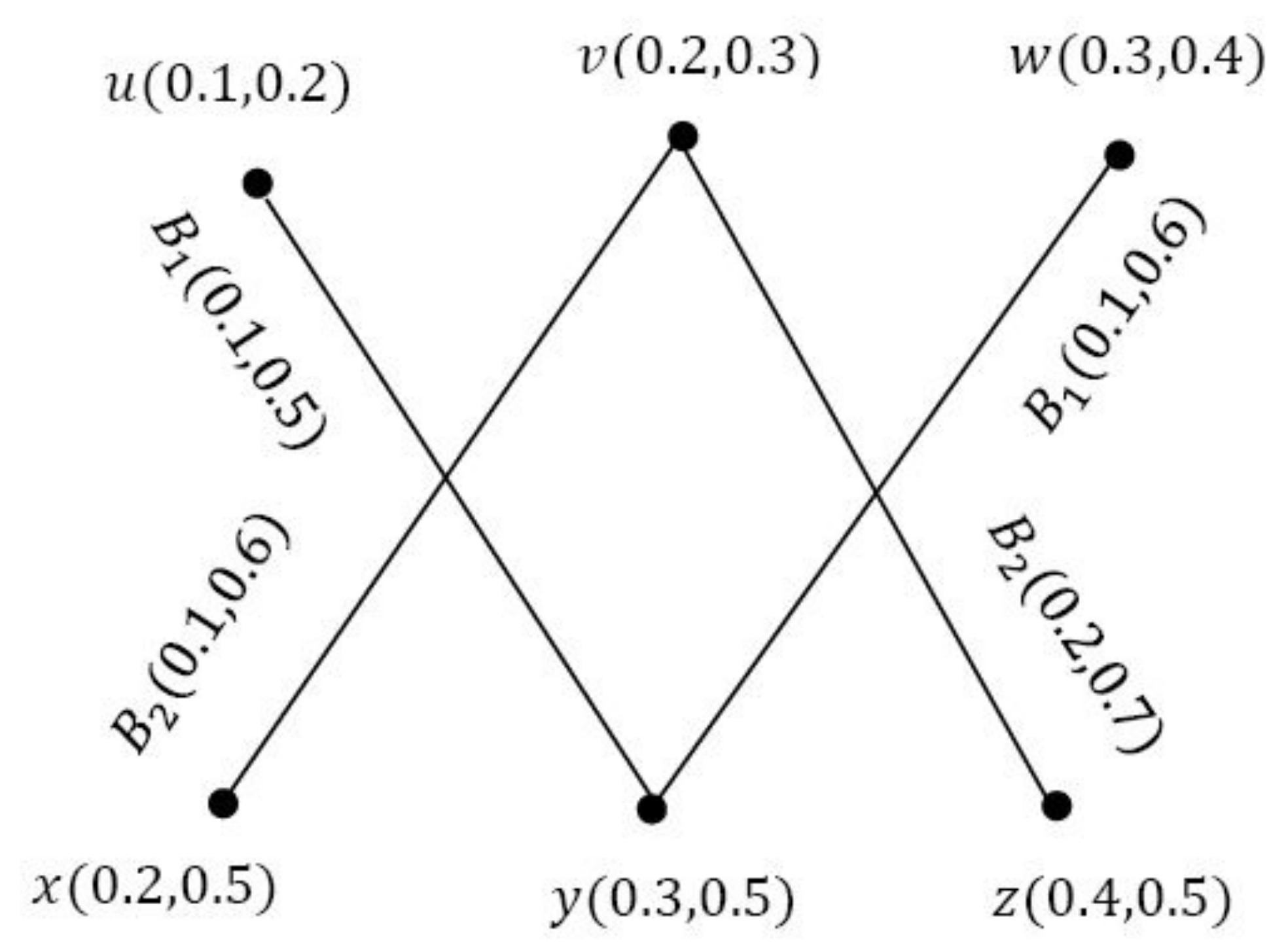
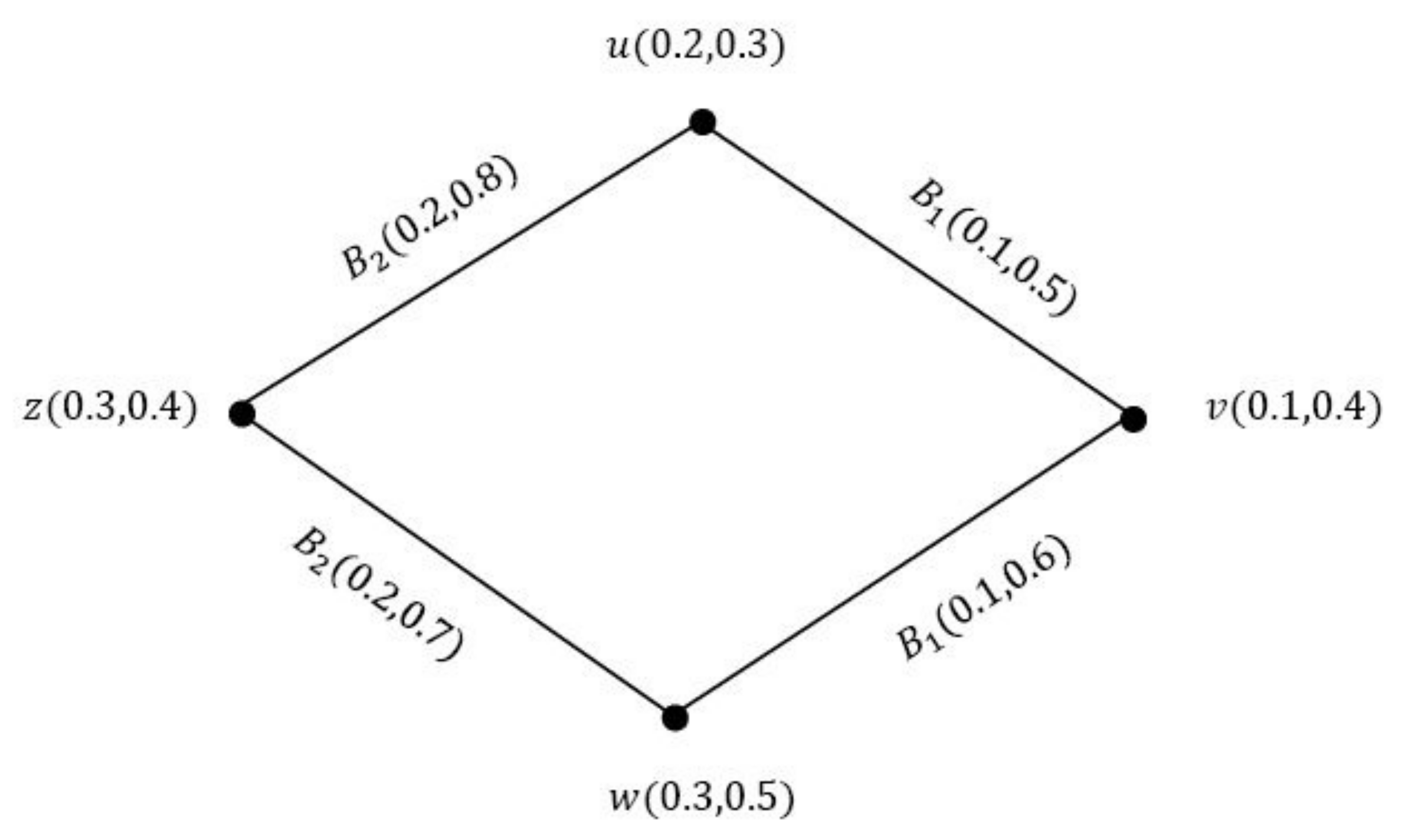
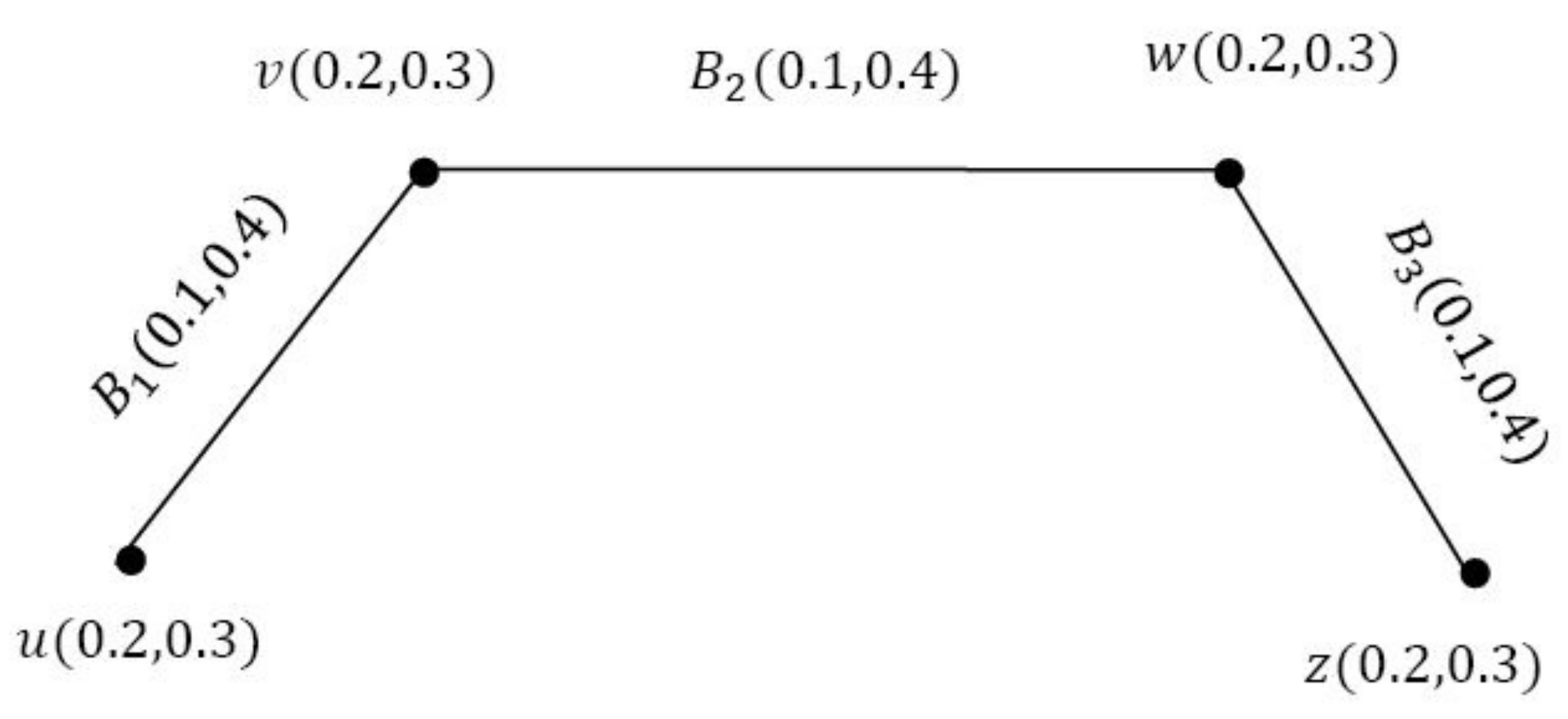
Definition 4. is called a VGS of a GS , if is a vague set on V and for each ; is a vague set on such that:. Note that , for all and , , , where V and are called underlying vertex set and underlying i-edge set of G, respectively. Example 2. Let be a graph structure such that , and . Let A, , and be vague subsets of V, , and respectively such that:Then is a VGS of as shown in Figure 2. Definition 5. Let and be two vague graph structures with underlying crisp graph structures and , respectively. is called maximal vague graph structure with underlying crisp graph structure , where and . Vague vertex set A and vague relation in maximal product are defined as: ,. Example 3. Consider two VGSs and as shown in Figure 3. Their maximal product is shown in Figure 4. For vertex , we find both true membership and false membership value as follows:for and . For edge , we find both true membership value and false membership value.for and . Similarly, we can find both true membership and false membership value for all remaining vertices and edges.
Theorem 1. The maximal product of two VGSs and , is a VGS, too.
Proof. Let and be two VGSs and , . Then by Definition 4, we have two cases:
Hence, is a VGS. □
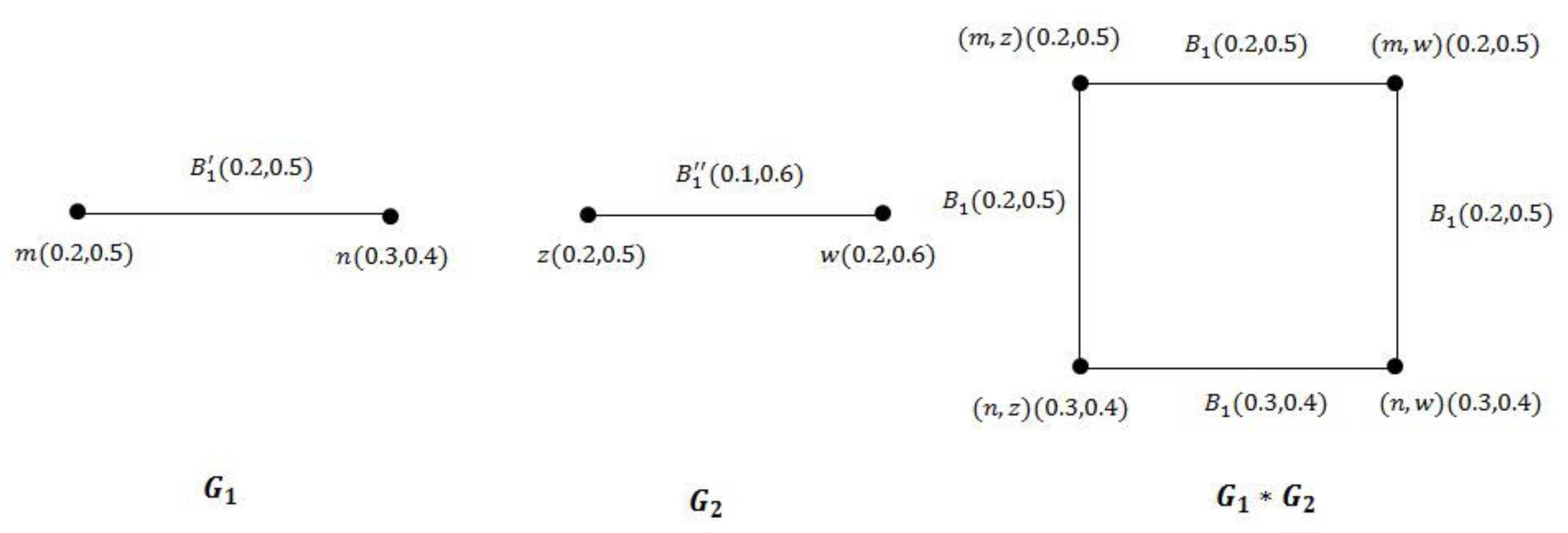
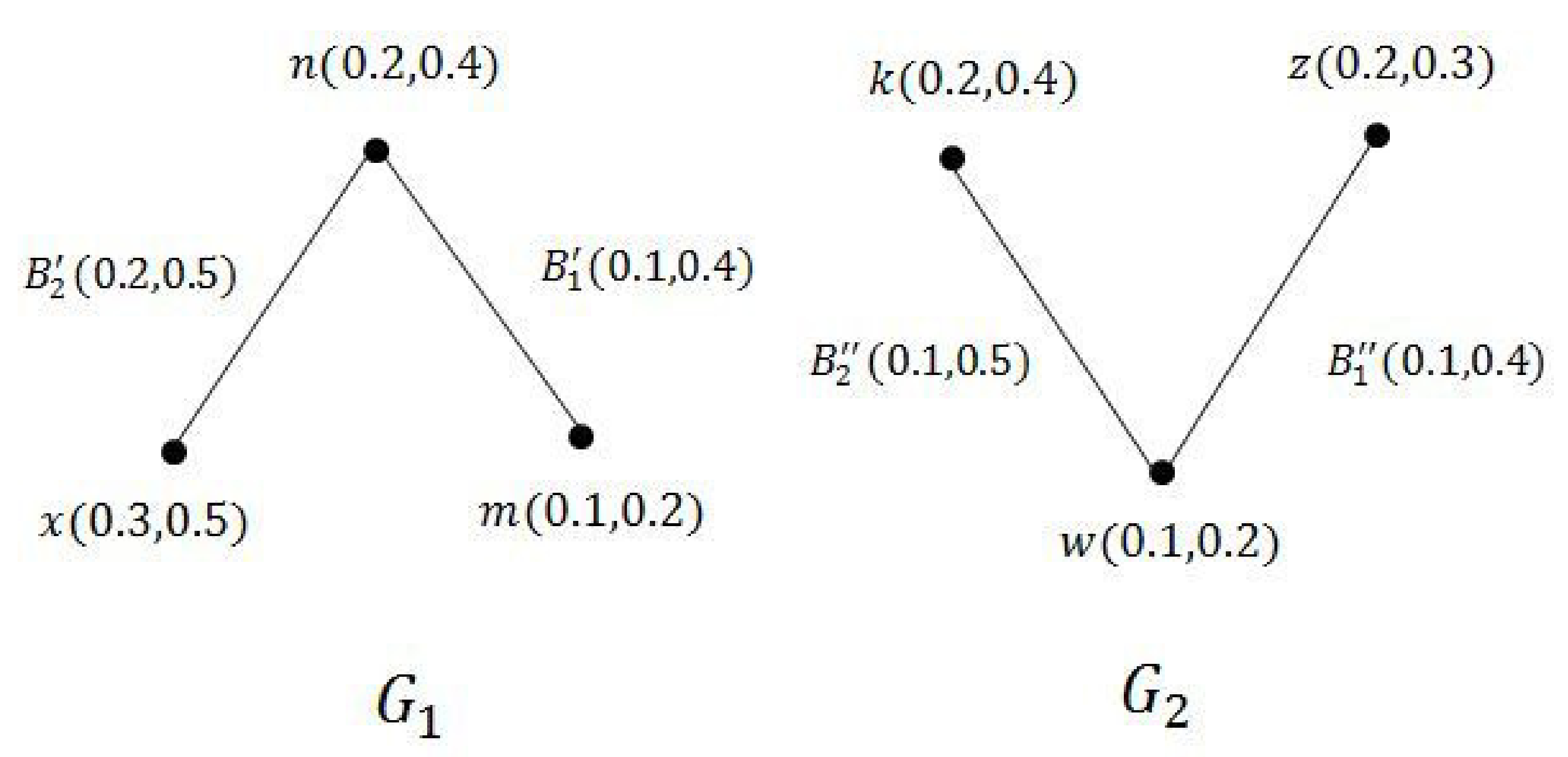
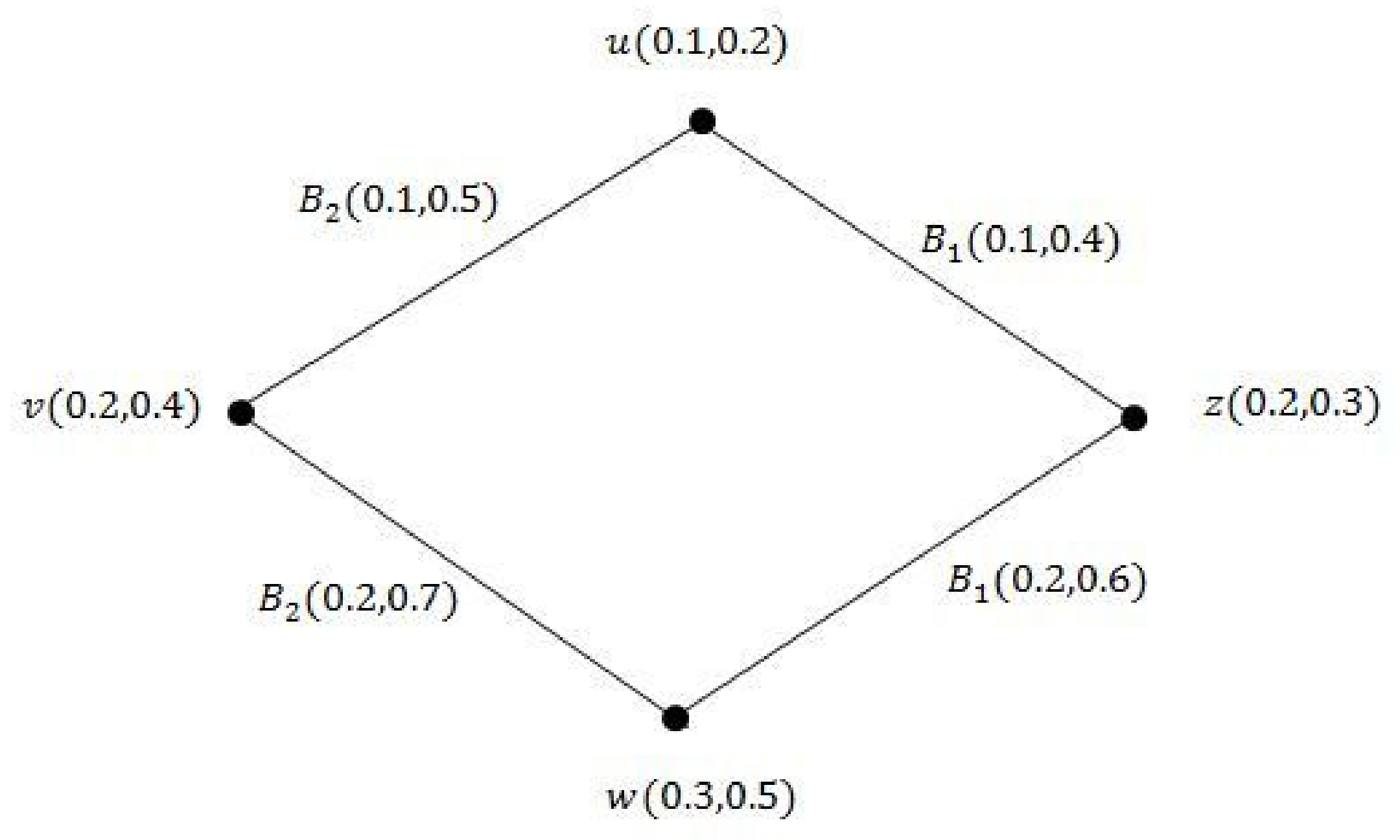
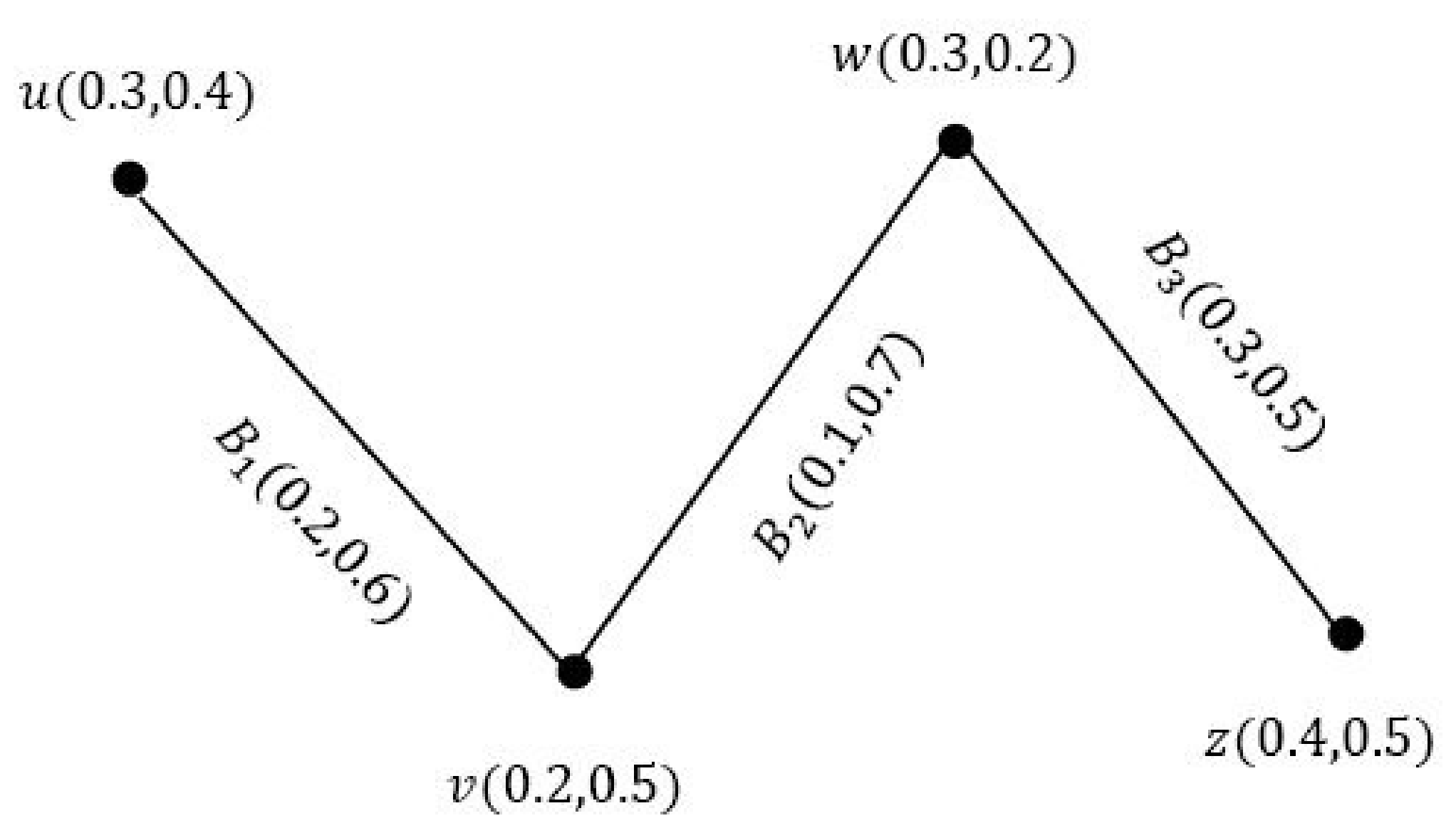
Definition 6. The rejection of two VGSs and is defined as: Example 4. Consider the VGSs and as in Figure 5. We can see that the rejection of two VGSs and , that is in Figure 6. For vertex , we find both membership value and non-membership value as follows:for and . For edge , we find both membership value and non-membership value.for and . Now, for edge we have:for and . Similarly, we can find both membership value and non-membership value for all remaining vertices and edges.
Proposition 1. The rejection of two VGSs and , is a VGS.
Proof. Let and be two VGSs and , . Then by Definition 6 we have:
(i) If
and
,
(ii) If
and
(iii) If
and
Therefore, is a VGS. □
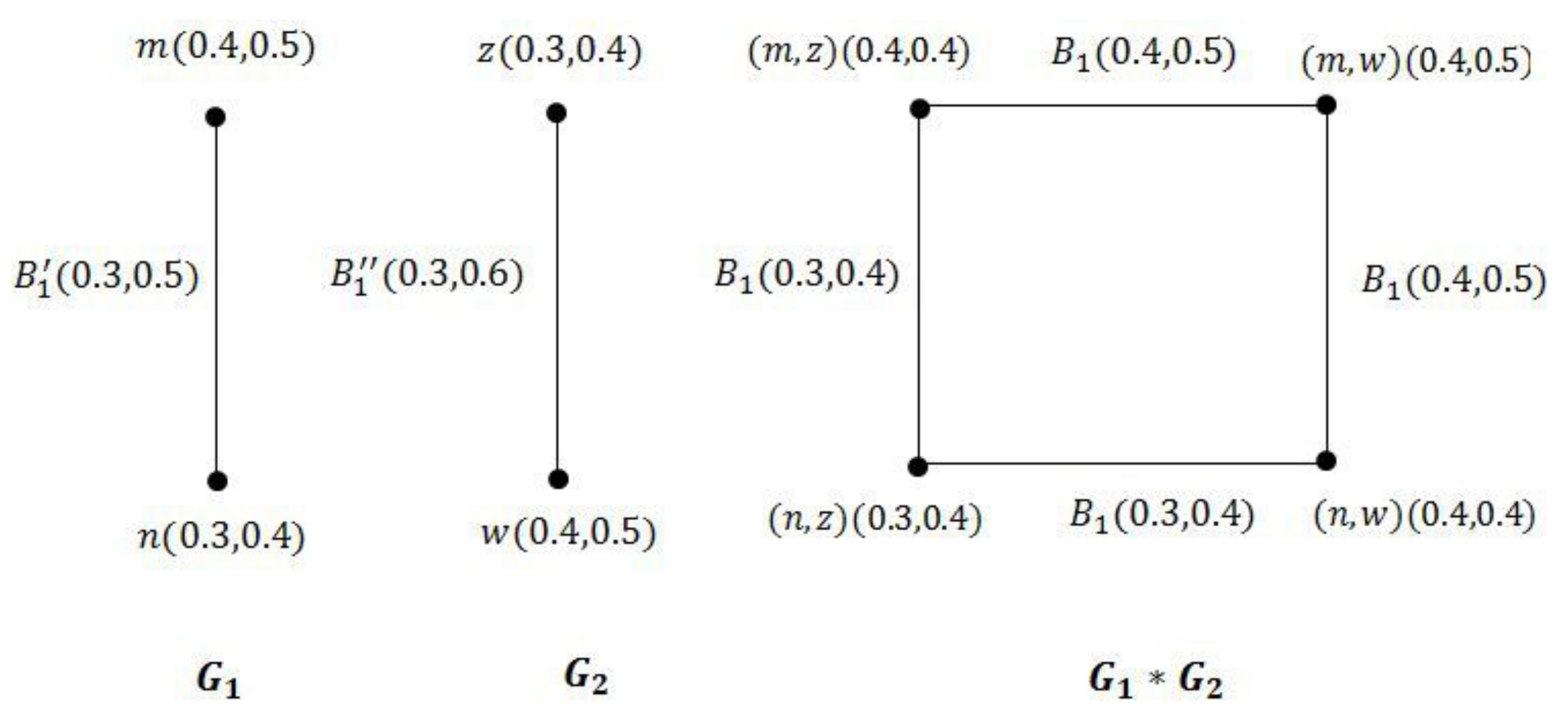
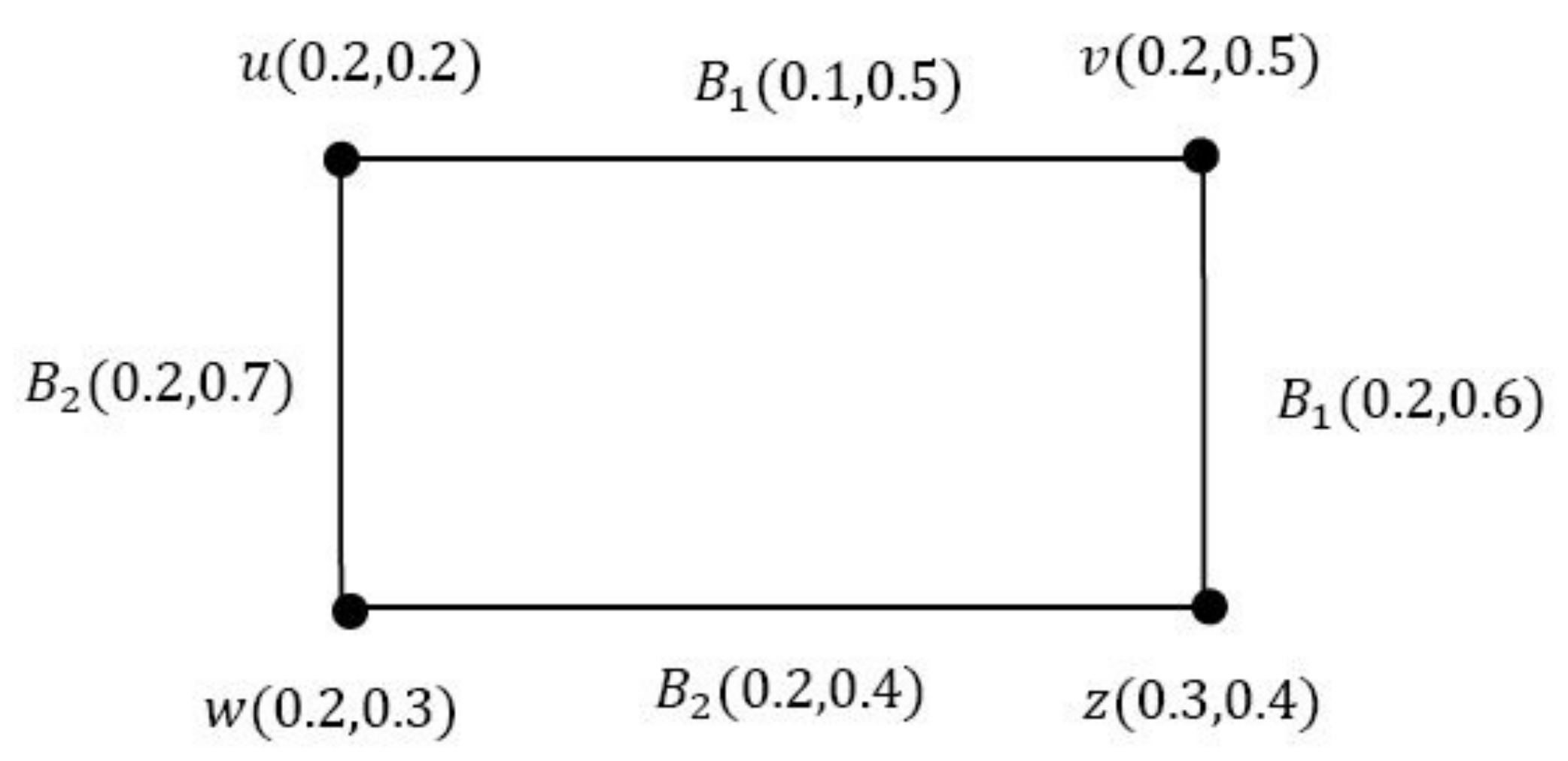
Definition 7. The residue product of two VGSs and is defined as:for all .for all , . Example 5. Consider the VGSs and as in Figure 7. The residue product of and shown in Figure 8. For vertex , we find both membership value and non-membership value as follows:for and . Now, for edge we have:for and . In the same way, we can find both membership value and non-membership value for all remaining vertices and edges.
Proposition 2. The residue product of two VGSs and is a VGS.
Proof. Let
and
be two VGSs and
,
. If
and
, then we have:
□
4. Irregularity in VGSs
The irregularity concepts play an important role in both the graph theory application and theory in the vague environment. The characterizations of highly irregular and neighborly irregular graphs have also been applied to the question of heterogeneity. One of the most broadly studied classes of FGs is IFGs. They are being applied in many contexts, for example, the r-irregular (FGs) with connectivity and edge-connectivity equal to r play a key role in designing reliable communication networks. This idea inspires us to present different types of IVGSs such as totally irregular-VGS (TI-VGS), highly irregular-VGS (HI-VGS), strongly irregular-VGS (SI-VGS), strongly totally irregular-VGS (STI-VGS), highly totally irregular-VGS (HTI-VGS), neighborly edge irregular-VGS (NEI-VGS), neighborly edge totally irregular-VGS (NETI-VGS), and strongly edge totally irregular-VGS (SETI-VGS) and their related theorems.
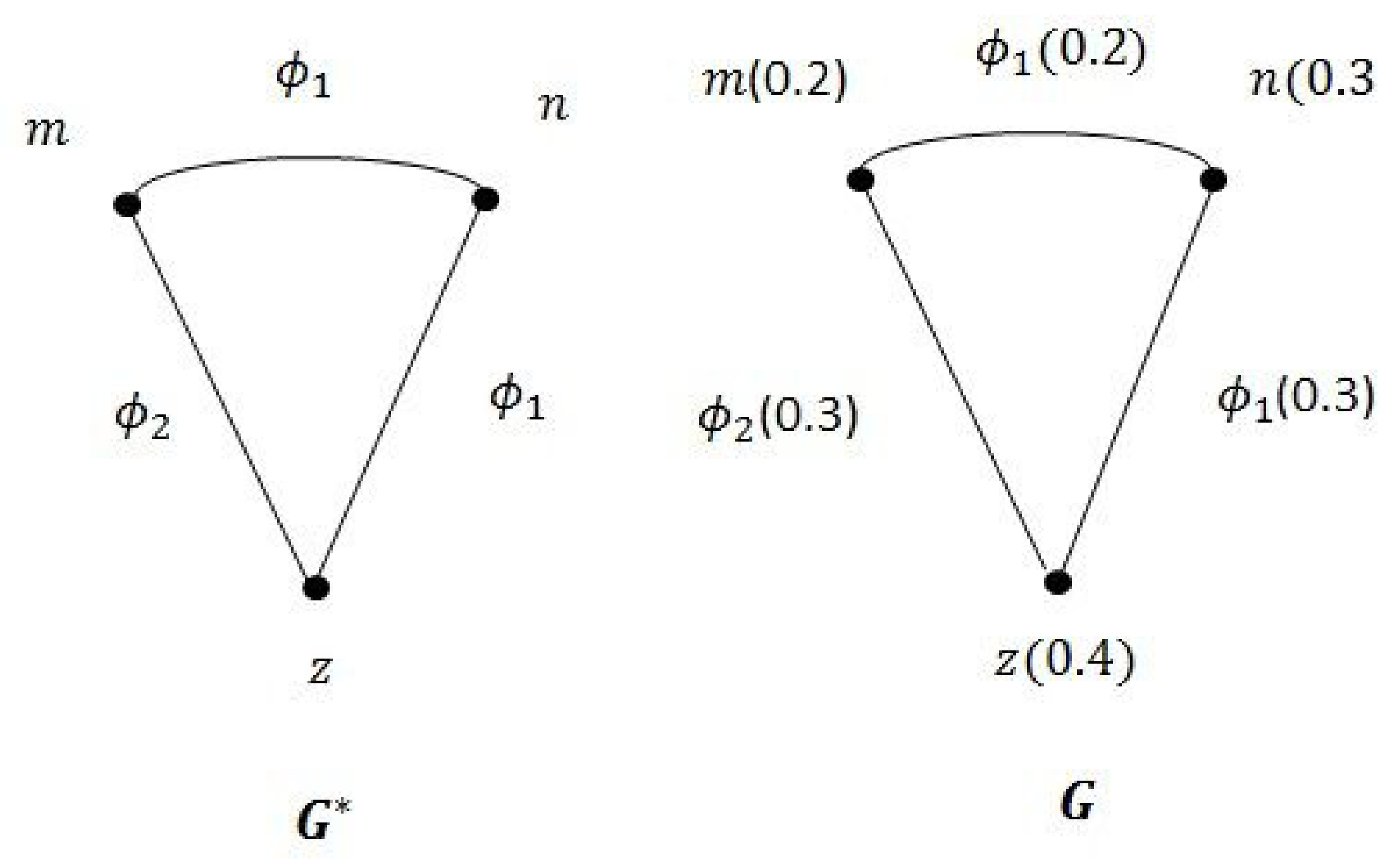
Definition 8. An VGS G is said to be an irregular-VGS if there is a vertex which is adjacent to vertices with distinct degrees.
Example 6. Consider a VGS as shown in Figure 9. By direct calculation, we have , , , . From this, we see that v is adjacent to vertices of different degrees. Hence, G is an I-VGS.
Definition 9. A VGS G is said to be a totally irregular-VGS if ∃ a vertex which is adjacent to vertices with different total degrees.
Example 7. Consider a VGS as shown in Figure 10. By direct calculation, we have , , , and . From this, we see that u is adjacent to vertices of different degrees. Hence, G is a TI-VGS.
Definition 10. A VGS G is said to be a strongly I-VGS if every vertex has a different degree.
Example 8. Consider a VGS as shown in Figure 11. It is easy to see that , , , , , . We can see that every vertex has a different degree. Hence, G is strongly I-VGS.
The first definition of neighborly irregular in fuzzy graph was introduced in [
29]. Neighborly irregular has not been much discussed although the totally irregular and edge irregular concepts are very important and they can be useful in computer science and the problem of finding the shortest path in a computer network. Therefore, the following definitions are provided to highlight the issue.
Definition 11. A VGS G is said to be a strongly TI-VGS if every vertex has a different total degree.
Example 9. By direct calculation we have , , , , , . From this, we see that every vertex has a different total degree. Hence, G is strongly TI-VGS.
Definition 12. A VGS G is said to be highly I-VGS if every vertex in G is adjacent to vertices of different degrees.
Example 10. Consider the VGS G as shown in Figure 9. It is easy to see that every vertex is adjacent to vertices of different degrees. Hence, G is highly I-VGS. Definition 13. A VGS G is said to be highly TI-VGS if every vertex in G is adjacent to vertices of different total degrees.
Example 11. Consider VGS as shown in Figure 12. By simple calculation, we have , , , and . So, every vertex is adjacent to vertices of different degrees. Hence, G is a highly TI-VGS.
Definition 14. The degree and the total degree of an edge of a VGS G are defined by , and , respectively, and we have: Example 12. Consider the VGS as shown in Figure 13. By direct calculation, we have , , , . The degree of every edge is given as:Hence, , , . The total degree of every edge is given as:So, , , and . Definition 15. A connected VGS G is said to be a neighborly edge irregular-VGS (NEI-VGS), if every pair of adjacent edges in G has different degrees.
Example 13. Consider the VGS G as shown in Figure 13. From this, it is easy to see that every pair of adjacent edges has different degrees. Hence, G is an NEI-VGS. Definition 16. A connected VGS G is said to be a neighborly edge totally irregular-VGS (NETI-VGS), if every pair of adjacent edges has different total degrees.
Example 14. Consider the VGS G as shown in Figure 13. From it, we see that every pair of adjacent edges has different total degrees. Therefore, G is an NETI-VGS. Definition 17. A VGS G is said to be a strongly edge irregular-VGS (SEI-VGS), if every edge in G has a different degree.
Example 15. Consider the VGS as shown in Figure 14. By a simple calculation, we have , , and . The degree of each edge is given as:Since that each edge has different degree, so G is an SEI-VGS. Definition 18. A VGS G is said to be a strongly edge totally irregular-VGS (SETI-VGS), if every edge in G has a different total degree.
Example 16. Consider the VGS as shown in Figure 15. By a simple calculation, we have , , , .
The degree of every edge is given as:The total degree of each edges is given as:It shows that every edge in G has a different total degree. Hence, G is an SETI-VGS. Remark 1. The SEI-VGS G may not be SETI-VGS.
Example 17. Consider a VGS as shown in Figure 16. By direct calculation, we have:Since every edge in G has a different degree, so G is an SEI-VGS. Now, we calculate the total degree of every edge as follows:Hence, G is not an SETI-VGS. Theorem 2. If is a strongly edge irregular connected VGS (SEIC-VGS), where , is a constant function. Then G is an SETI-VGS.
Proof. Let G be an SEIC-VGS. Consider as a constant function. Then and , for all , where , and are constants. Consider two edges and in . Since G is an SEI-VGS so , where and are two edges in . This shows that . This implies that . Hence, , where, and are two edges in . Since the edges and were taken to be arbitrary, this shows every two edge in G have different total degrees. Therefore, G is an SETI-VGS. □
Theorem 3. If is a SETIC-VGS, where is a constant function. Then, G is an SEI-VGS.
Proof. Let G be an SETIC-VGS. Consider , is a constant function. Then, , , for all , where and are constants. Consider the edges and in . Since G is an SETI-VGS, so , where and are edges in . This results that . This shows that . So, , where and are two edges in . Since that and were arbitrary edges, so every two edges in G have different degrees. Therefore, G is an SEI-VGS. □
Theorem 4. Let be an SEI-VGS. Then G is an NEI-VGS.
Proof. Let G be an SEI-VGS. Then, every edge in G has a different degree. This shows that every two neighbor edges have different degrees. Hence, G is an NEI-VGS. □
Theorem 5. Let be an SETI-VGS. Then, G is an NETI-VGS.
Proof. Let G be an SETI-VGS. Then, each edge in G has a different total degree. This shows that every two neighbor edges in G have different total degrees. Hence, G is an NETI-VGS. □
Remark 2. If G is an NEI-VGS, then, it is not compulsory that G is an SEI-VGS.
Example 18. Consider the VGS as shown in Figure 17. By simple calculation we have:The degree of each edge is , , and . Since each two neighbor edges have different degrees, i.e., and . Hence, G is an NEI-VGS. Here, we can see that . Hence, G is not an SEI-VGS. Theorem 6. Let be an SEIC-VGS, with as constant function. Then, G is an I-VGS.
Proof. Let G be an SEIC-VGS, with as constant function. Then, and , for every edge , where and are constants. Likewise, every edge in G has a different degree, so G is an SEI-VGS. Suppose that and be any two neighbor edges in G such that . This shows that . This results that . This implies that . Hence, there is a vertex v in G which is neighbor to the vertices with different degrees. This shows that G is an I-VGS. □
Theorem 7. Let be an SETIC-VGS, with , as constant function. Then, G is an I-VGS.
Proof. Let be an SETIC-VGS, with as constant function. Then, and , for every edge , where and are constants and every edge in G has a different total degree. Hence G is an SETI-VGS. Suppose that and be any two neighbor edges in G such that . This shows that . This results that . This represents that . This shows that . Hence, there is a vertex v in G which is neighbor to the vertices with different degrees. This indicates that G is an I-VGS. □
5. Application of VGS in Medical Diagnosis
In this section, an attempt was made to run through a fuzzy decision-making approach using (VS). In addition, an interesting distance measure on VSs has been introduced.
Definition 19. Let be the universe of discourse. Let and be two VSs. The the new distance measure can be defined as: To be a distance measure, it is easy to show that all four conditions are satisfied (See
Appendix A, Remark A5).
Suppose that
be a set of number of possible diseases and
be a set of
n number of patients. Let,
be the symptoms the disease
and
be the symptoms of patient
expressed in VSs. Hence, the distance between the symptoms disease
and symptoms of patient
can be evaluated as follows using the proposed distance measure:
where
and
.
We can describe the distances between every pair of disease and patients using the following matrix:
Applying the fact that, less distance between two VSs shows more similarity between them, therefore, it can be described that for the patient
the disease most possibly suffered by him or her is the disease corresponding to
.
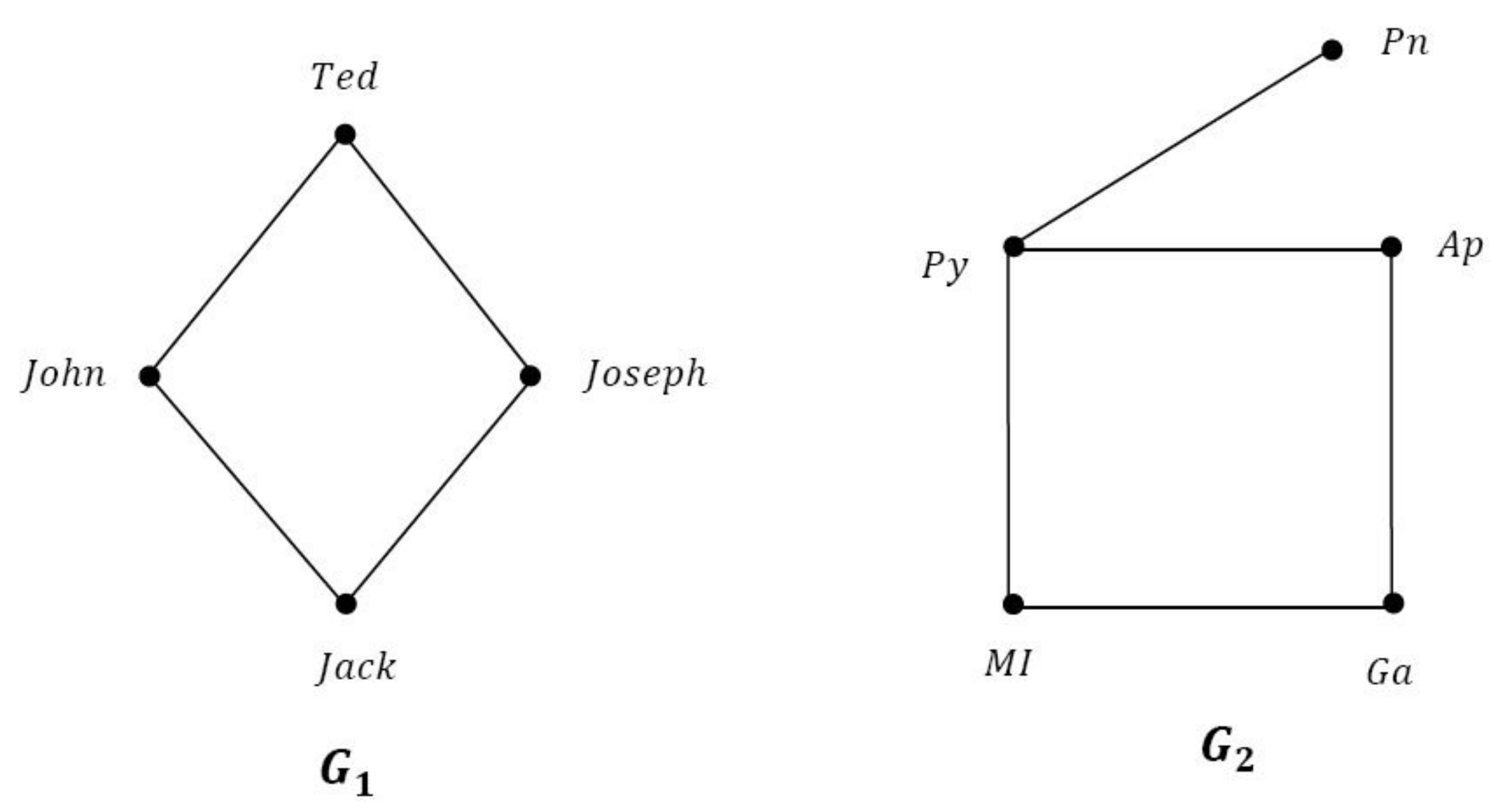
Here, we consider a set of symptoms S, a set of diagnosis D, and a set of patients P. Consider , , and . Our aim is to make the right diagnosis decision for each patient, from the set of symptoms, for each disease.
Now, in
Figure 18, we assume
is the VGS of the set of patients, and
is the VGS of the set of diagnosis where
and
.
The relation between symptom and disease, and the relation between pain and symptom is given in
Table 1 and
Table 2, respectively.
The five diagnoses are now described as vague sets.
Likewise, the patients as vague sets can be described as follows:
Now the vague distance is calculated between the disease and the patients based on their symptoms.
Similarly, the vague distance measure between the patients and the diseases can be found as:
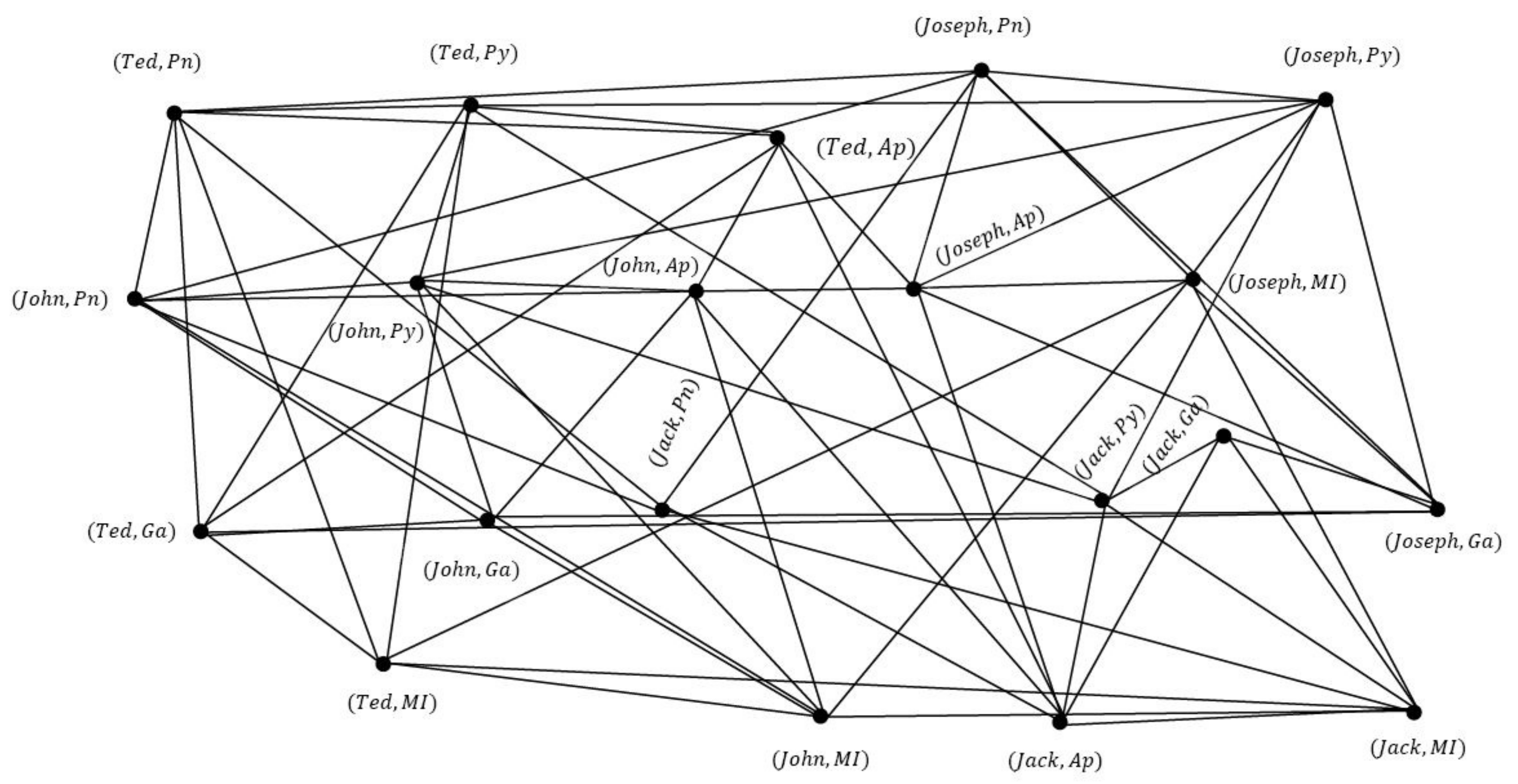
Vague graph structure
is shown in
Figure 19.
In this figure, we clearly see that people who have at least one similar symptom, are closer to each other in terms of diagnosis, and this is also acceptable in medical science. Therefore, with the help of maximized multiplication graph, we can find the right diagnosis for different people, with the same symptoms.
Now by applying the following distance matrix, it can be shown to what extent the symptoms of disease differ by the symptoms of patients.
Less distance between patient and disease implies more possibility of having the disease; we can predict which disease is suffered by the four people. Considering the distance matrix, one can see that, if the doctor agrees, Ted suffers from pyelonephritis, John suffers from appendicitis, Joseph suffers from pneumonia, and Jack suffers from gastroenteritis.