Models for Tree Taper Form: The Gompertz and Vasicek Diffusion Processes Framework
Abstract
1. Introduction
2. Materials and Methods
2.1. SDE Taper Framework
2.1.1. SDE Stem Taper Models
2.1.2. SDE Parameters Estimation
2.1.3. Standard Errors of the Parameter Estimates of the SDE Models
2.1.4. Random Effects Calibration
2.2. Nonlinear Regression Stem Taper Models
2.2.1. Segmented Polynomial
2.2.2. Segmented q-Exponential
2.2.3. Variable Exponential
2.3. Nonlinear and Linear Regression Stem Volume Models
2.4. Evaluation of Models to Data
- Adjusted coefficient of determination:
- Mean prediction error (percent prediction error, %):
- Mean absolute prediction error (percent absolute prediction error, %):
- Root-mean-square error (root-mean-square error, %):
3. Results and Discussion
3.1. Estimation Results
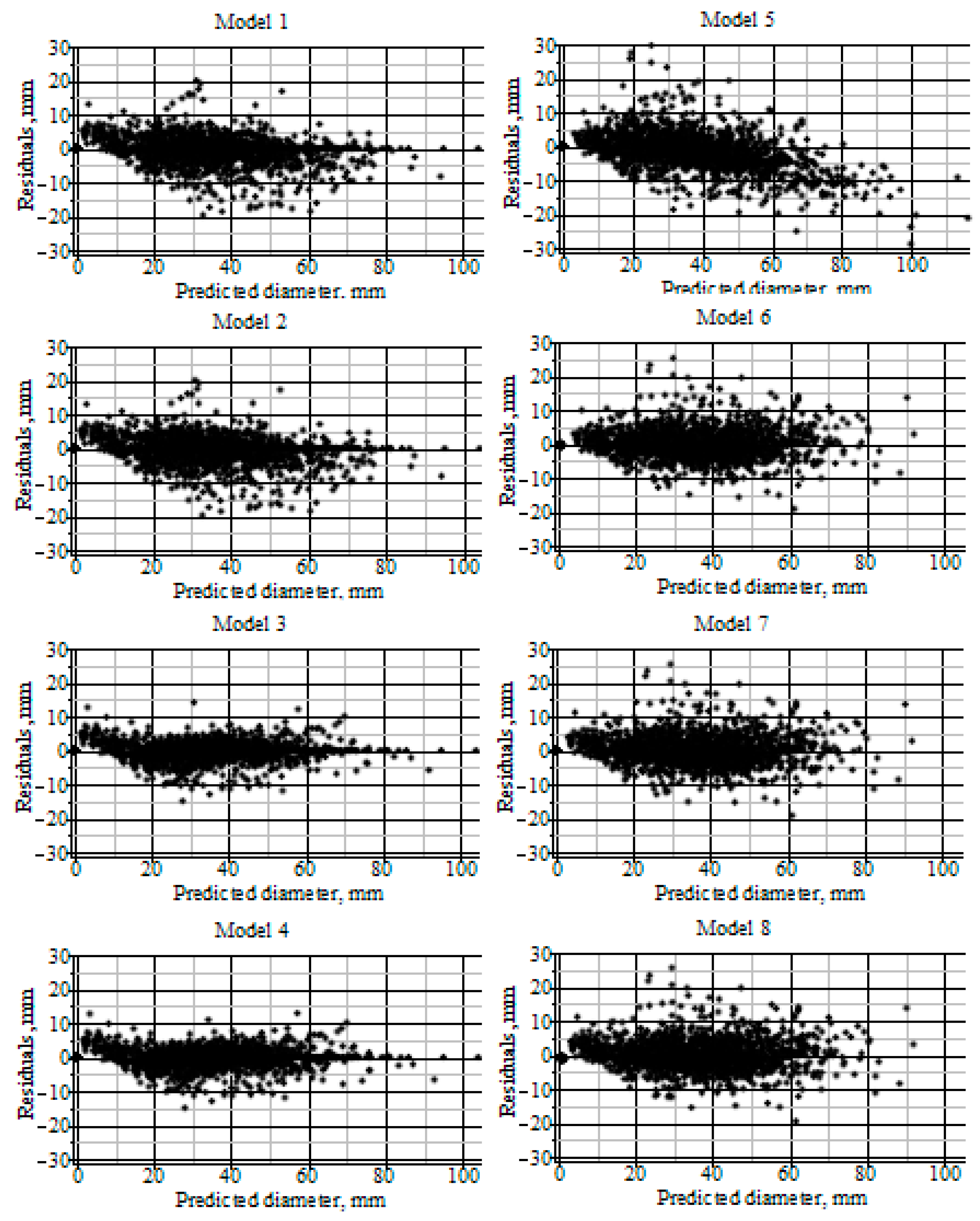
3.2. Comparison of Stem Taper Models
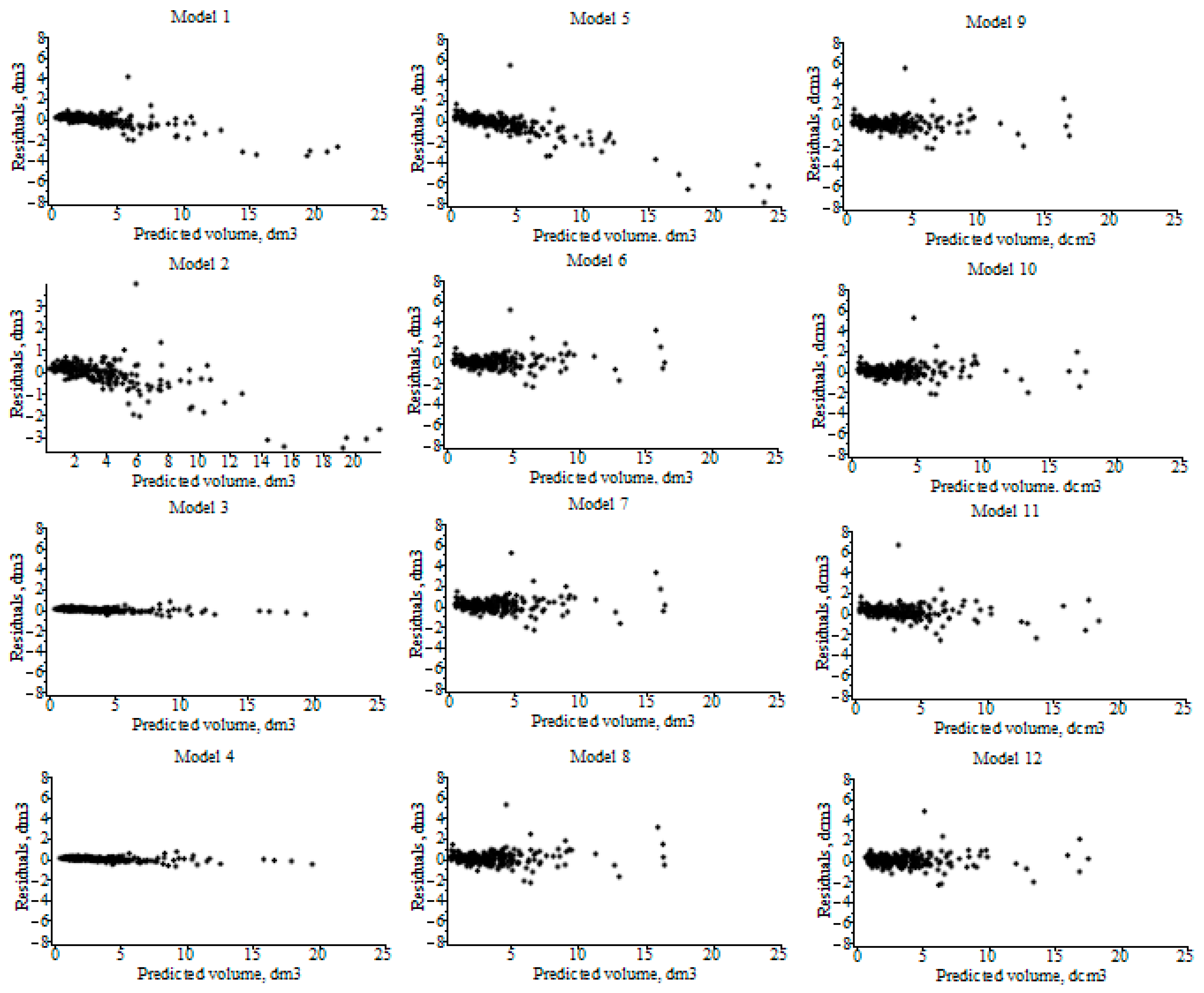
3.3. Comparison of Stem Volume Models
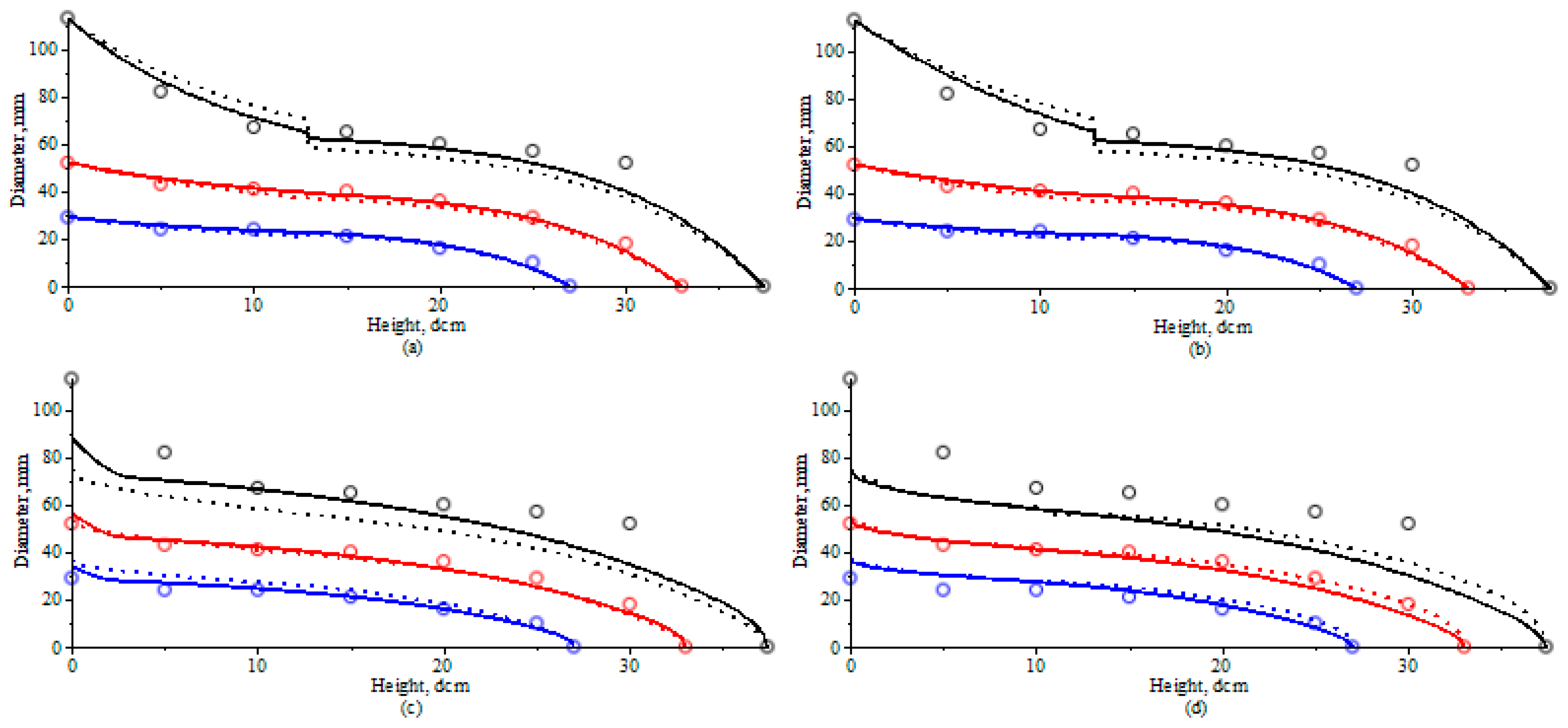
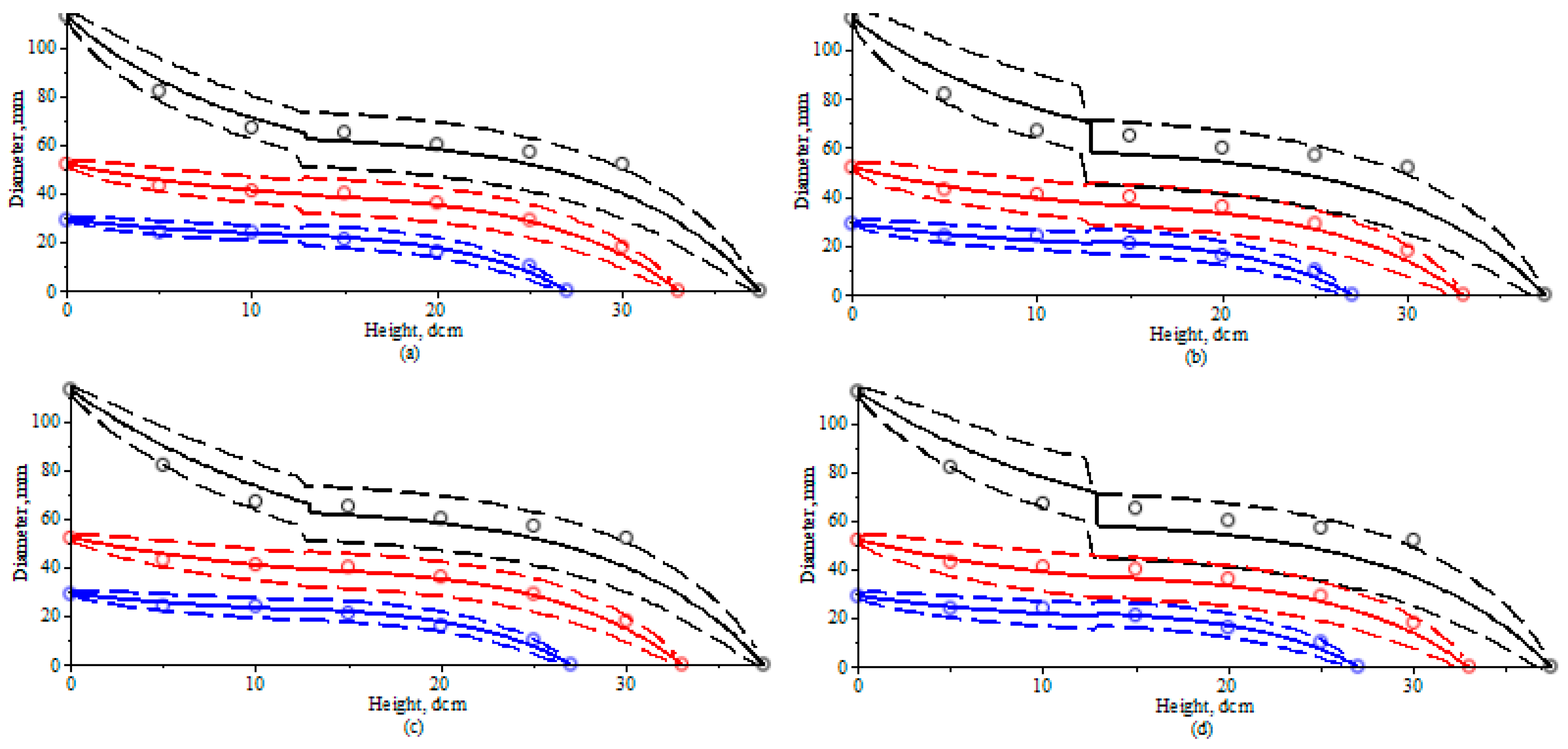
3.4. Illustration of Tree Stem Tapers
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Max, T.A.; Burkhart, H.E. Segmented Polynomial Regression Applied to Taper Models. For. Sci. 1976, 22, 283–289. [Google Scholar]
- Kozak, A. My Last Words on Taper Equations. For. Chron. 2004, 80, 507–515. [Google Scholar] [CrossRef]
- Garcia, O. Dynamic Modelling of Tree Form. Math. Comput. For. Nat. Res. Sci. 2015, 7, 9–15. [Google Scholar]
- Sloboda, B.; Saborowski, J. Stand Taper Form as a Stochastic Process with an Example of Application. In Proceedings of the XVII IUFRO-World Congress, Kyoto, Japan, 6–17 September 1981; pp. 137–150. [Google Scholar]
- Rupšys, P. Univariate and Bivariate Diffusion Models: Computational Aspects and Applications to Forestry. In Stochastic Differential Equations: Basics and Applications; Deangelo, T.G., Ed.; Nova Science Publisher’s: New York, NY, USA, 2018; pp. 1–77. [Google Scholar]
- Gray, H.R. Form and Taper of Forest-Tree Stems; Paper No. 32; Imperial Forestry Institute University of Oxford: Oxford, UK, 1956; p. 79. [Google Scholar]
- Kozak, A.; Mundro, D.D.; Smith, J.H.G. Taper Functions and their Application in Forest Inventory. For. Chron. 1969, 45, 278–283. [Google Scholar] [CrossRef]
- Chiba, Y. A Quantitative Analysis of Stem Form and Crown Structure: The S-Curve and its Application. Tree Physiol. 1990, 7, 169–182. [Google Scholar] [CrossRef]
- Petrauskas, E.; Bartkevičius, E.; Rupšys, P.; Memgaudas, R. The use of stochastic differential equations to describe stem taper and volume. Baltic For. 2013, 19, 43–151. [Google Scholar]
- Diblík, J.; Dzhalladova, I.; Růžičková, M. A Dynamical System with Random Parameters as a Mathematical Model of Real Phenomena. Symmetry 2019, 11, 1338. [Google Scholar] [CrossRef]
- Rupšys, P. The Use of Copulas to Practical Estimation of Multivariate Stochastic Differential Equation Mixed Effects Models. AIP Conf. Proc. 2015, 1684, 080011. [Google Scholar]
- Suzuki, T. Forest transition as a stochastic process. Mitt. Forstl. Bundesversuchsanstalt Wien 1971, 91, 69–86. [Google Scholar]
- Sloboda, B. Mathematische und stochastische Modelle zur Beschreibung der Dynamik von Bäumen und Beständen, insbesondere das bestandesspezifische Wachstum als stochastischer Prozeß; Habilitations-schrift, Universität-Freiburg: Freiburg, Germany, 1976; 310p. [Google Scholar]
- Rupšys, P. New insights into tree height distribution based on mixed effects univariate diffusion processes. PLoS ONE 2016, 11, e0168507. [Google Scholar] [CrossRef] [PubMed]
- Rupšys, P.; Petrauskas, E. A new paradigm in modelling the evolution of a stand via the distribution of tree sizes. Sci. Rep. 2017, 7, 15875. [Google Scholar] [CrossRef] [PubMed]
- Rupšys, P.; Petrauskas, E. Evolution of bivariate tree diameter and height distribution via stand age: Von Bertalanffy bivariate diffusion process approach. J. For. Res. Jpn. 2019, 24, 16–26. [Google Scholar] [CrossRef]
- Rupšys, P. Modeling Dynamics of Structural Components of Forest Stands Based on Trivariate Stochastic Differential Equation. Forests 2019, 10, 506. [Google Scholar] [CrossRef]
- Rupšys, P.; Petrauskas, E. A Linkage among Tree Diameter, Height, Crown Base Height, and Crown Width 4-variate Distribution and Their Growth Models: A 4-variate Diffusion Process Approach. Forests 2017, 8, 479. [Google Scholar] [CrossRef]
- Rupšys, P. Understanding the Evolution of Tree Size Diversity within the Multivariate Nonsymmetrical Diffusion Process and Information Measures. Mathematics 2019, 7, 761. [Google Scholar] [CrossRef]
- Itô, K. On stochastic processes. Jpn. J. Math. 1942, 18, 261–301. [Google Scholar] [CrossRef]
- Bronisz, K.; Zasada, M. Comparison of Fixed- and Mixed-Effects Approaches to Taper Modeling for Scots Pine in West Poland. Forests 2019, 10, 975. [Google Scholar] [CrossRef]
- Fisher, R.A. On the mathematical foundations of theoretical statistics. Philos. Trans. R. Soc. Lond. Ser. A 1922, 222, 309–368. [Google Scholar] [CrossRef]
- Lee, W.K.; Seo, J.H.; Son, Y.M.; Lee, K.H.; von Gadow, K. Modeling stem profiles for Pinus densiflora in Korea. For. Ecol. Manag. 2003, 72, 69–77. [Google Scholar] [CrossRef]
- Duan, A.; Zhang, S.; Zhang, X.; Zhang, J. Development of a stem taper equation and modelling the effect of stand density on taper for Chinese fir plantations in Southern China. PeerJ 2016, 4, e1929. [Google Scholar] [CrossRef]
- Tsallis, C. What are the numbers that experiments provide? Quim. Nova 1994, 17, 468. [Google Scholar]
- Tsallis, C. Nonadditive entropy and nonextensive statistical mechanics-an overview after 20 years. Braz. J. Phys. 2009, 39, 337–356. [Google Scholar] [CrossRef]
- Rupšys, P.; Petrauskas, E. Development of q-exponential models for tree height, volume and stem profile. Int. J. Phys. Sci. 2010, 5, 2369–2378. [Google Scholar]
- Berhe, L.; Arnoldsson, G. Tree taper models for Cupressus lusitanica plantations in Ethiopia. South For. 2008, 70, 193–203. [Google Scholar] [CrossRef]
- Zianis, D.; Muukkonen, P.; Mäkipää, R.; Mencuccini, M. Biomass and stem volume equations for tree species in Europe. Silva Fenn. 2005, 4, 1–63. [Google Scholar]
- Schumacher, F.X.; Hall, F.S. Logarithmic expression of timber-tree volume. J. Agric. Res. 1933, 47, 719–734. [Google Scholar]
- Petrauskas, E.; Rupšys, P.; Memgaudas, R. Q-exponential Variable-form of a Steam Taper and Volume Model for Scots Pine (Pinus sylvesteris L.) in Lithuania. Baltic For. 2011, 17, 118–127. [Google Scholar]
- Honer, T.G. A new total cubic foot volume function. For. Chron. 1965, 41, 476–493. [Google Scholar] [CrossRef]
- Visalga, G.; Rupšys, P.; Petrauskas, E. Influence of Noise on Decay Predictions in Standing Trees. AIP Conf. Proc. 2017, 1895, 030006. [Google Scholar]
- Bühlmann, P.; van de Geer, S. Statistics for High-Dimensional Data: Methods, Theory and Applications; Springer: Heidelberg, Germany, 2011; 558p. [Google Scholar]
- Monagan, M.B.; Geddes, K.O.; Heal, K.M.; Labahn, G.; Vorkoetter, S.M.; McCarron, J.; DeMarco, P. Maple Advanced Programming Guide; Maplesoft: Ontario, ON, Canada, 2007; 388p. [Google Scholar]

| Data | Number of Stems | Min | Max | Mean | St. Dev. | Number of Stems | Min | Max | Mean | St. Dev. |
|---|---|---|---|---|---|---|---|---|---|---|
| Estimation | Validation | |||||||||
| d (mm) | 217 | 15.0 | 78.0 | 37.51 | 11.59 | 102 | 20.0 | 84.0 | 36.93 | 9.25 |
| h (dcm) | 217 | 17.0 | 71.4 | 35.10 | 9.86 | 102 | 2.3 | 60.4 | 34.63 | 7.09 |
| v (dcm3) | 217 | 0.471 | 18.966 | 3.828 | 2.943 | 102 | 0.910 | 24.734 | 3.511 | 2.742 |
| Parameters of SDE Models | |||||||||
| αB | βB | σB | αT | βT | σT | σ1 | σ2 | ||
| 1 | −0.6209 (0.0009) | 1.9619 (0.0038) | 0.2586 (0.0002) | 1.0262 (0.0002) | 4.7321 (0.0030) | 0.4161 (0.0002) | − | − | |
| 2 | 0.7386 (0.0006) | 2.6620 (0.0033) | 0.3369 (0.0003) | 1.0213 (0.0002) | 4.7729 (0.0030) | 0.4196 (0.0002) | − | − | |
| 3 | 0.5429 (0.0009) | 2.1504 (0.0034) | 0.1918 (0.0002) | 1.0212 (0.0001) | 4.8294 (0.0025) | 0.3584 (0.0002) | 0.3755 (0.0005) | 0.1098 (0.0001) | |
| 4 | 0.7218 (0.0007) | 2.4400 (0.0032) | 0.2576 (0.0002) | 1.0212 (0.0001) | 4.8294 (0.0025) | 0.3584 (0.0002) | 0.1689 (0.0002) | 0.1098 (0.0001) | |
| Model | Parameters of Regression Models | ||||||||
| β1 | β2 | β3 | β4 | β5 | β6 | β7 | β8 | β9 | |
| 5 | −4.8466 (2.1724) | 1.7202 (1.2734) * | −2.6159 (1.5277) * | 78.601 (7.8595) | 0.7825 (0.0070) | 0.0940 (0.0030) | − | − | − |
| 6 | 0.3996 (0.2601) | 5.3867 (0.2206) | 0.7490 (0.0101) | −0.5407 (0.0379) | 0.5292 (0.1046) | −0.9364 (0.0633) | 4.9294 (0.4817) | −11.9415 (0.8444) | 4.7264 (0.2347) |
| 7 | 3.5740 (0.1237) | 0.7461 (0.0092) | 0.6350 (0.0571) | −0.5765 (0.0687) | 0.4593 (0.0218) | − | − | − | − |
| 8 | 2.6297 (0.1602) | 0.7508 (0.0162) | 0.0548 (0.0214) | 0.3480 (0.0186) | 0.1285 (0.0597) | 0.2683 (0.0218) | −1.4783 (0.6825) | −0.0024 (0.0017) * | 0.0231 (0.0406) * |
| 9 | 0.6339 (0.1514) | 1.6095 (0.0527) | 0.9114 (0.0553) | − | − | − | − | − | − |
| 10 | −0.3883 (0.4794) * | 0.0549 (0.0174) | 0.8402 (0.0911) | 2.3440 (0.5437) | −0.3139 (0.0641) | − | − | − | − |
| 11 | 0.0148 (0.0016) | 1.0312 (0.0741) | − | − | − | − | − | − | − |
| 12 | 0.0789 (0.2482) | 0.1942 (0.2260) | 34.4302 (12.0618) | −17.8971 (8.6782) | 0.0405 (0.0535) | 1.6661 (1.6431) | − | − | − |
| Model | Estimation Data Set | Validation Data Set | ||||||
|---|---|---|---|---|---|---|---|---|
| B (%B) | AB (%AB) | RMSE (%RMSE) | R2 | B (%B) | AB (%AB) | RMSE (%RMSE) | R2 | |
| 1 | −0.5368 (−1.683) | 2.5766 (8.075) | 4.0599 (12.728) | 0.9533 | −0.2505 (−0.814) | 2.1768 (7.076) | 3.3176 (10.784) | 0.9671 |
| 2 | −0.4925 (−1.544) | 2.5761 (8.076) | 4.0498 (12.697) | 0.9535 | −0.1969 (−0.640) | 2.1972 (7.142) | 3.3457 (10.889) | 0.9664 |
| 3 | −0.1875 (−0.587) | 1.7065 (5.350) | 2.6995 (8.463) | 0.9794 | −0.8018 (−2.606) | 1.9522 (6.346) | 3.0499 (9.914) | 0.9722 |
| 4 | −0.1935 (−0.606) | 1.7610 (5.521) | 2.7448 (8.605) | 0.9787 | −0.8515 (−2.768) | 1.9883 (6.464) | 3.0697 (9.978) | 0.9718 |
| 5 | −1.3717 (−4.301) | 3.7903 (11.883) | 5.4298 (17.023) | 0.9165 | −1.0559 (−3.432) | 3.0086 (9.780) | 4.2303 (13.751) | 0.9464 |
| 6 | 2.4 × 10−9 (7.6 × 10−8) | 2.9359 (9.205) | 4.2083 (13.194) | 0.9499 | −0.1667 (−0.542) | 2.8242 (9.180) | 4.2461 (13.802) | 0.9461 |
| 7 | 0.0856 (0.268) | 2.9056 (9.110) | 4.2197 (13.230) | 0.9495 | 0.0013 (0.004) | 2.7404 (8.908) | 4.1211 (13.396) | 0.9492 |
| 8 | −0.0173 (−0.054) | 2.9790 (9.340) | 4.2235 (13.242) | 0.9495 | −0.1936 (−0.629) | 2.8909 (9.397) | 4.2282 (13.744) | 0.9465 |
| Model | Estimation Data Set | Validation Data Set | ||||||
|---|---|---|---|---|---|---|---|---|
| B (%B) | AB (%AB) | RMSE (%RMSE) | R2 | B (%B) | AB (%AB) | RMSE (%RMSE) | R2 | |
| 1 | −0.1735 (−4.53) | 0.4490 (11.72) | 0.7794 (20.35) | 0.9302 | −0.0603 (−1.71) | 0.2791 (7.96) | 0.4060 (11.56) | 0.9782 |
| 2 | −0.1588 (−4.15) | 0.4393 (11.47) | 0.7599 (19.85) | 0.9336 | −0.0461 (1.33) | 0.2742 (7.81) | 0.3975 (11.32) | 0.9792 |
| 3 | −0.0333 (−0.87) | 0.1222 (3.19) | 0.1737 (4.53) | 0.9965 | −0.1710 (−4.87) | 0.2553 (7.27) | 0.3360 (9.57) | 0.9851 |
| 4 | −0.0363 (−0.95) | 0.1347 (3.51) | 0.1899 (4.96) | 0.9958 | −0.1867 (−5.32) | 0.2595 (7.39) | 0.3336 (9.50) | 0.9853 |
| 5 | −0.3765 (−9.83) | 0.7338 (19.17) | 1.3362 (34.90) | 0.7947 | −0.2446 (−6.98) | 0.5111 (14.55) | 0.7915 (22.54) | 0.9174 |
| 6 | 0.0426 (1.11) | 0.4494 (11.74) | 0.7105 (18.56) | 0.9420 | 0.0560 (1.59) | 0.4850 (13.81) | 1.0163 (28.94) | 0.8639 |
| 7 | 0.0812 (2.12) | 0.4542 (11.86) | 0.7107 (18.56) | 0.9419 | 0.0925 (2.64) | 0.4865 (13.86) | 1.0024 (28.55) | 0.8676 |
| 8 | 0.0427 (1.11) | 0.4423 (11.55) | 0.7076 (18.48) | 0.9424 | 0.0545 (1.55) | 0.4760 (13.56) | 0.9931 (28.29) | 0.8701 |
| 9 | 0.0204 (0.53) | 0.4319 (11.28) | 0.6941 (18.13) | 0.9446 | 0.0925 (2.64) | 0.4865 (13.86) | 1.0024 (28.55) | 0.8676 |
| 10 | 1.1 × 10−9 (3.0 × 10−8) | 0.4420 (11.55) | 0.6865 (17.93) | 0.9458 | 0.0033 (−0.09) | 0.4588 (13.07) | 0.8081 (23.01) | 0.9139 |
| 11 | 0.1321 (3.45) | 0.4691 (12.26) | 0.7559 (19.74) | 0.9343 | 0.1604 (4.57) | 0.4029 (11.48) | 0.6661 (18.97) | 0.9415 |
| 12 | 2.2 × 10−10 (5.8 × 10−9) | 0.4467 (11.67) | 0.6741 (17.81) | 0.9477 | 0.0330 (0.94) | 0.4677 (13.32) | 0.8200 (23.36) | 0.9114 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Narmontas, M.; Rupšys, P.; Petrauskas, E. Models for Tree Taper Form: The Gompertz and Vasicek Diffusion Processes Framework. Symmetry 2020, 12, 80. https://doi.org/10.3390/sym12010080
Narmontas M, Rupšys P, Petrauskas E. Models for Tree Taper Form: The Gompertz and Vasicek Diffusion Processes Framework. Symmetry. 2020; 12(1):80. https://doi.org/10.3390/sym12010080
Chicago/Turabian StyleNarmontas, Martynas, Petras Rupšys, and Edmundas Petrauskas. 2020. "Models for Tree Taper Form: The Gompertz and Vasicek Diffusion Processes Framework" Symmetry 12, no. 1: 80. https://doi.org/10.3390/sym12010080
APA StyleNarmontas, M., Rupšys, P., & Petrauskas, E. (2020). Models for Tree Taper Form: The Gompertz and Vasicek Diffusion Processes Framework. Symmetry, 12(1), 80. https://doi.org/10.3390/sym12010080





