Cosmology and Matter-Induced Branes
Abstract
1. Introduction
2. The Extra Dimensions
2.1. From D Dimensions to 4 Dimensions: General Remark
2.2. The Planck Mass and the Extra Space Structure
2.2.1. Kaluza–Klein Model
2.2.2. Hyperbolic Extra Dimensions
2.2.3. f(R) Theories
2.2.4. Brane Models
2.3. Brane as a Clump of Matter?
3. Matter-Induced Branes
3.1. Matter Distribution within Extra Space
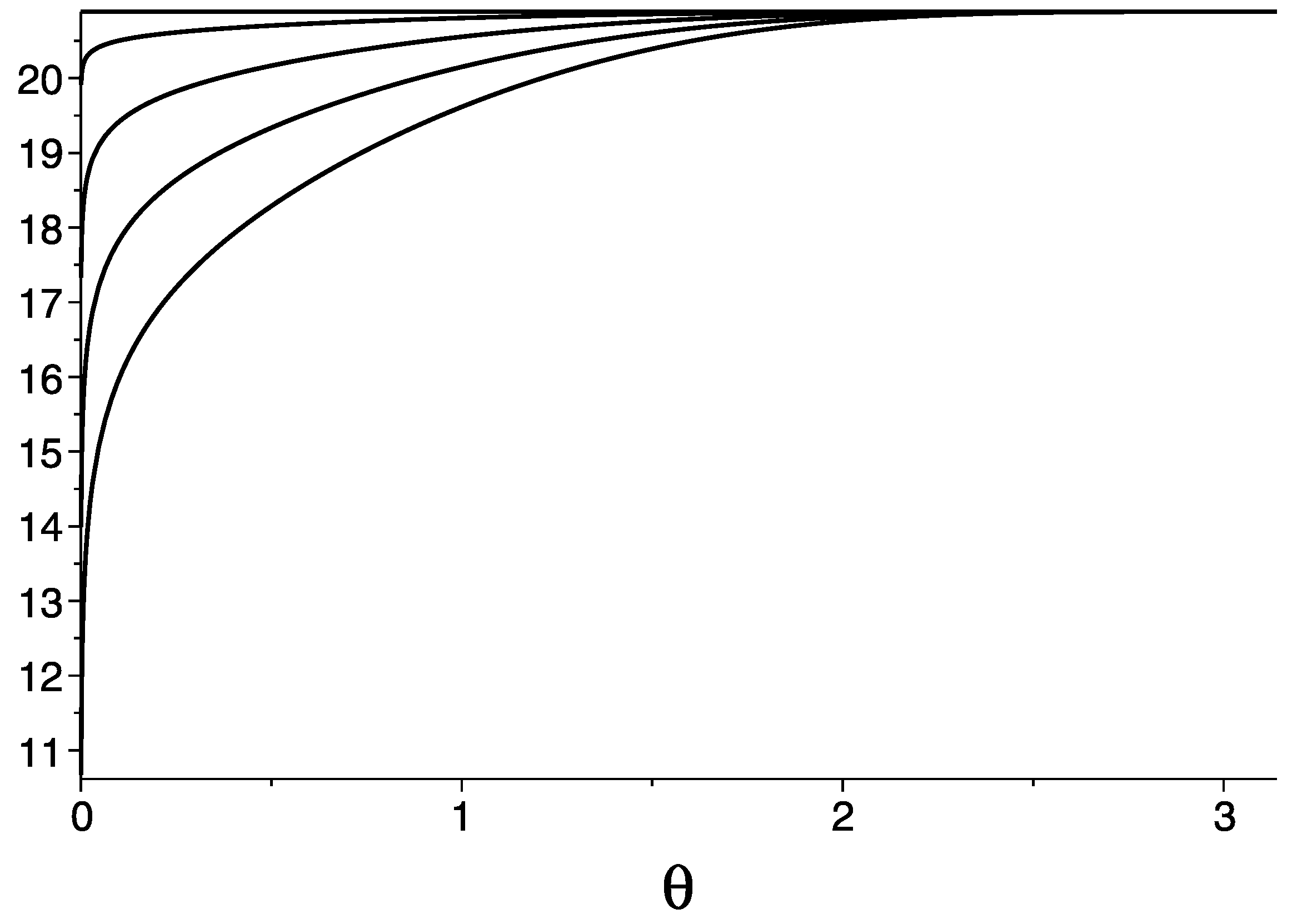
3.2. Matter-Induced Branes and Variation of 4-Dimensional Physical Parameters
4. Fine-Tuning of the Lambda Term and Matter-Induced Branes
5. Conclusions
Funding
Conflicts of Interest
References
- Dolgov, A.D.; Zeldovich, Y.B. Cosmology and elementary particles. Rev. Mod. Phys. 1981, 53, 1–41. [Google Scholar] [CrossRef]
- Khlopov, M.Y.; Rubin, S.G. Cosmological Pattern of Microphysics in the Inflationary Universe; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2004. [Google Scholar]
- Donoghue, J.F. The fine-tuning problems of particle physics and anthropic mechanisms. In Universe or Multiverse? Carr, B., Ed.; Cambridge University Press: Cambridge, UK, 2007; p. 231. [Google Scholar]
- Page, D.N. Anthropic estimates of the charge and mass of the proton. Phys. Lett. 2009, B675, 398–402. [Google Scholar] [CrossRef][Green Version]
- Van de Bruck, C.; Christopherson, A.J.; Robinson, M. Stabilizing the Planck mass shortly after inflation. Phys. Rev. 2015, D91, 123503. [Google Scholar] [CrossRef]
- Bronnikov, K.A.; Melnikov, V.N. Conformal frames and D-dimensional gravity. In The Gravitational Constant: Generalized Gravitational Theories and Experiments; Springer: Dordrecht, The Netherlands, 2003; pp. 39–64. [Google Scholar] [CrossRef][Green Version]
- Gogberashvili, M. Our world as an expanding shell. Europhys. Lett. 2000, 49, 396–399. [Google Scholar] [CrossRef]
- Lyakhova, Y.; Popov, A.A.; Rubin, S.G. Classical evolution of subspaces. Eur. Phys. J. 2018, C78, 764. [Google Scholar] [CrossRef]
- Sahni, V.; Starobinsky, A.A. The Case for a positive cosmological Lambda term. Int. J. Mod. Phys. 2000, D9, 373–444. [Google Scholar] [CrossRef]
- Dienes, K.R.; Dudas, E.; Gherghetta, T. Grand unification at intermediate mass scales through extra dimensions. Nucl. Phys. B 1999, 537, 47–108. [Google Scholar] [CrossRef]
- Fischbach, E.; Klimchitskaya, G.L.; Krause, D.E.; Mostepanenko, V.M. On the validity of constraints on light elementary particles and extra-dimensional physics from the Casimir effect. Eur. Phys. J. 2010, C68, 223–226. [Google Scholar] [CrossRef][Green Version]
- Bolokhov, S.V.; Bronnikov, K.A. On Cosmology in Nonlinear Multidimensional Gravity with Multiple Factor Spaces. Gravit. Cosmol. 2018, 24, 154–160. [Google Scholar] [CrossRef]
- Nasri, S.; Silva, P.J.; Starkman, G.D.; Trodden, M. Radion stabilization in compact hyperbolic extra dimensions. Phys. Rev. D 2002, 66, 045029. [Google Scholar] [CrossRef]
- Starobinsky, A.A. A New Type of Isotropic Cosmological Models Without Singularity. Phys. Lett. 1980, B91, 99–102. [Google Scholar] [CrossRef]
- Bamba, K.; Makarenko, A.N.; Myagky, A.N.; Nojiri, S.; Odintsov, S.D. Bounce cosmology from F(R) gravity and F(R) bigravity. J. Cosmol. Astropart. Phys. 2014, 1, 8. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D.; Tretyakov, P.V. Dark energy from modified F(R)-scalar-Gauss Bonnet gravity. Phys. Lett. B 2007, 651, 224–231. [Google Scholar] [CrossRef]
- Sokolowski, L.M. Metric gravity theories and cosmology:II. Stability of a ground state in f(R) theories. Class. Quantum Gravity 2007, 24, 3713–3734. [Google Scholar] [CrossRef][Green Version]
- Nojiri, S.; Odintsov, S.D. Unified cosmic history in modified gravity: From theory to Lorentz non-invariant models. Phys. Rep. 2011, 505, 59–144. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.; Oikonomou, V. Modified gravity theories on a nutshell: Inflation, bounce and late-time evolution. Phys. Rep. 2017, 692, 1–104. [Google Scholar] [CrossRef]
- Bronnikov, K.A.; Rubin, S.G. Self-stabilization of extra dimensions. Phys. Rev. 2006, D73, 124019. [Google Scholar] [CrossRef]
- Arkani-Hamed, N.; Dimopoulos, S.; Dvali, G.R. The Hierarchy problem and new dimensions at a millimeter. Phys. Lett. 1998, B429, 263–272. [Google Scholar] [CrossRef]
- Randall, L.; Sundrum, R. Large Mass Hierarchy from a Small Extra Dimension. Phys. Rev. Lett. 1999, 83, 3370–3373. [Google Scholar] [CrossRef]
- Cabrer, J.A. Studies on Generalized Warped Five-Dimensional Models. arXiv 2012, arXiv:1201.0614. [Google Scholar]
- Shifman, M. Large Extra Dimensions: Becoming acquainted with an alternative paradigm. Int. J. Mod. Phys. 2010, A25, 199–225. [Google Scholar] [CrossRef]
- Rubakov, V.A.; Shaposhnikov, M.E. Do We Live Inside a Domain Wall? Phys. Lett. 1983, 125B, 136–138. [Google Scholar] [CrossRef]
- Bauer, F.; Sola, J.; Stefancic, H. Dynamically avoiding fine-tuning the cosmological constant: The ‘Relaxed Universe’. JCAP 2010, 1012, 29. [Google Scholar] [CrossRef]
- Krause, A. A Small cosmological constant and back reaction of nonfinetuned parameters. J. High Energy Phys. 2003, 9, 16. [Google Scholar] [CrossRef][Green Version]
- Green, A.M.; Mazumdar, A. Dynamics of a large extra dimension inspired hybrid inflation model. Phys. Rev. D 2002, 65, 105022. [Google Scholar] [CrossRef]
- Peyravi, M.; Riazi, N.; Lobo, F.S.N. Soliton models for thick branes. Eur. Phys. J. 2016, C76, 247. [Google Scholar] [CrossRef]
- Rubin, S.G. Scalar field localization on deformed extra space. Eur. Phys. J. 2015, C75, 333. [Google Scholar] [CrossRef][Green Version]
- Khlopov, M.; Malomed, B.A.; Zeldovich, I.B. Gravitational instability of scalar fields and formation of primordial black holes. Mon. Not. R. Astron. Soc. 1985, 215, 575–589. [Google Scholar] [CrossRef]
- Gani, V.A.; Dmitriev, A.E.; Rubin, S.G. Deformed compact extra space as dark matter candidate. Int. J. Mod. Phys. 2015, D24, 1545001. [Google Scholar] [CrossRef]
- Rubin, S.G. The role of initial conditions in the universe formation. Grav. Cosmol. 2015, 21, 143–151. [Google Scholar] [CrossRef]
- Bronnikov, K.A.; Budaev, R.I.; Grobov, A.V.; Dmitriev, A.E.; Rubin, S.G. Inhomogeneous compact extra dimensions. J. Cosmol. Astropart. Phys. 2017, 10, 001. [Google Scholar] [CrossRef][Green Version]
- Holman, R.; Kolb, E.W.; Vadas, S.L.; Wang, Y. Extended inflation from higher-dimensional theories. Phys. Rev. D 1991, 43, 995–1004. [Google Scholar] [CrossRef] [PubMed]
- Kirillov, A.A.; Korotkevich, A.A.; Rubin, S.G. Emergence of symmetries. Phys. Lett. 2012, B718, 237–240. [Google Scholar] [CrossRef]
- Carneiro, S.; Fabris, J.C. Scalar field black holes. Eur. Phys. J. 2018, C78, 676. [Google Scholar] [CrossRef]
- Trigiante, M. Gauged Supergravities. Phys. Rep. 2017, 680, 1–175. [Google Scholar] [CrossRef]
- Yurov, A.V.; Yurov, V.A. Quantum creation of a universe with varying speed of light: Lambda-problem and instantons. arXiv 2005, arXiv:0812.4738. [Google Scholar]
- Garriga, J.; Vilenkin, A. Solutions to the cosmological constant problems. Phys. Rev. 2001, D64, 023517. [Google Scholar] [CrossRef]
- Weinberg, S. Anthropic Bound on the Cosmological Constant. Phys. Rev. Lett. 1987, 59, 2607. [Google Scholar] [CrossRef]
- Loeb, A. An Observational Test for the Anthropic Origin of the Cosmological Constant. J. Cosmol. Astropart. Phys. 2006, 605, 9. [Google Scholar] [CrossRef]
- Wetterich, C. Naturalness of exponential cosmon potentials and the cosmological constant problem. Phys. Rev. 2008, D77, 103505. [Google Scholar] [CrossRef]
- Bousso, R.; Polchinski, J. Quantization of four form fluxes and dynamical neutralization of the cosmological constant. J. High Energy Phys. 2000, 6, 6. [Google Scholar] [CrossRef]
- Brown, A.R.; Dahlen, A.; Masoumi, A. Compactifying de Sitter space naturally selects a small cosmological constant. Phys. Rev. 2014, D90, 124048. [Google Scholar] [CrossRef]
- Burgess, C.P. The Cosmological Constant Problem: Why it’s hard to get Dark Energy from Micro-physics. In Proceedings of the 100th Les Houches Summer School: Post-Planck Cosmology, Les Houches, France, 8 July–2 August 2013; pp. 149–197. [Google Scholar] [CrossRef]
- Susskind, L. The Anthropic landscape of string theory. arXiv 2003, arXiv:hep-th/0302219. [Google Scholar]
- Rubin, S.G. Inhomogeneous extra space as a tool for the top-down approach. Adv. High Energy Phys. 2018, 2018, 2767410. [Google Scholar] [CrossRef]
- Liu, Y.X. Introduction to Extra Dimensions and Thick Braneworlds. In Memorial Volume for Yi-Shi Duan; World Scientific: Singapore, 2018; pp. 211–275. [Google Scholar] [CrossRef]
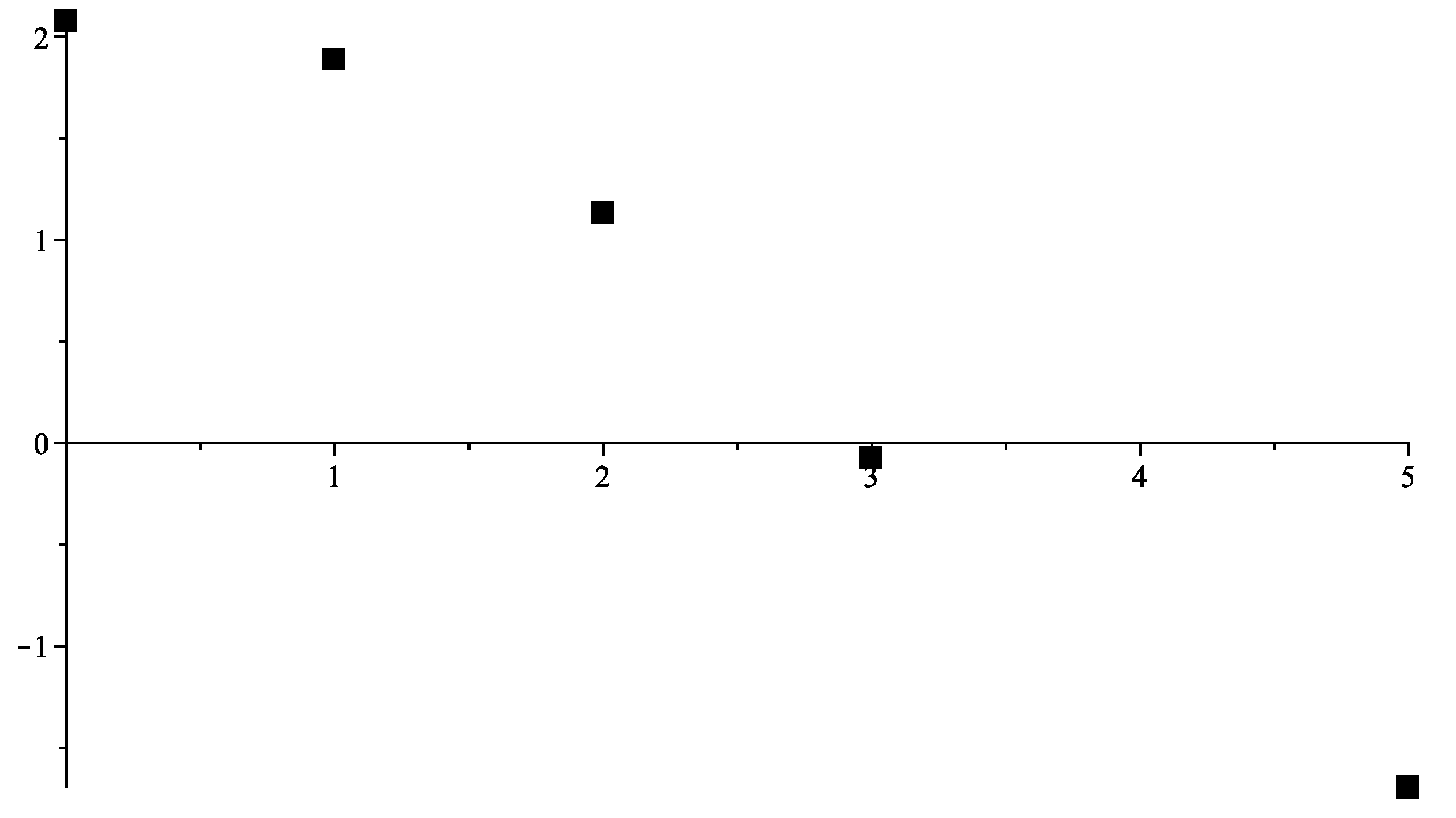
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rubin, S.G. Cosmology and Matter-Induced Branes. Symmetry 2020, 12, 45. https://doi.org/10.3390/sym12010045
Rubin SG. Cosmology and Matter-Induced Branes. Symmetry. 2020; 12(1):45. https://doi.org/10.3390/sym12010045
Chicago/Turabian StyleRubin, Sergey G. 2020. "Cosmology and Matter-Induced Branes" Symmetry 12, no. 1: 45. https://doi.org/10.3390/sym12010045
APA StyleRubin, S. G. (2020). Cosmology and Matter-Induced Branes. Symmetry, 12(1), 45. https://doi.org/10.3390/sym12010045



