A Hybrid Active Filter Using the Backstepping Controller for Harmonic Current Compensation
Abstract
1. Introduction
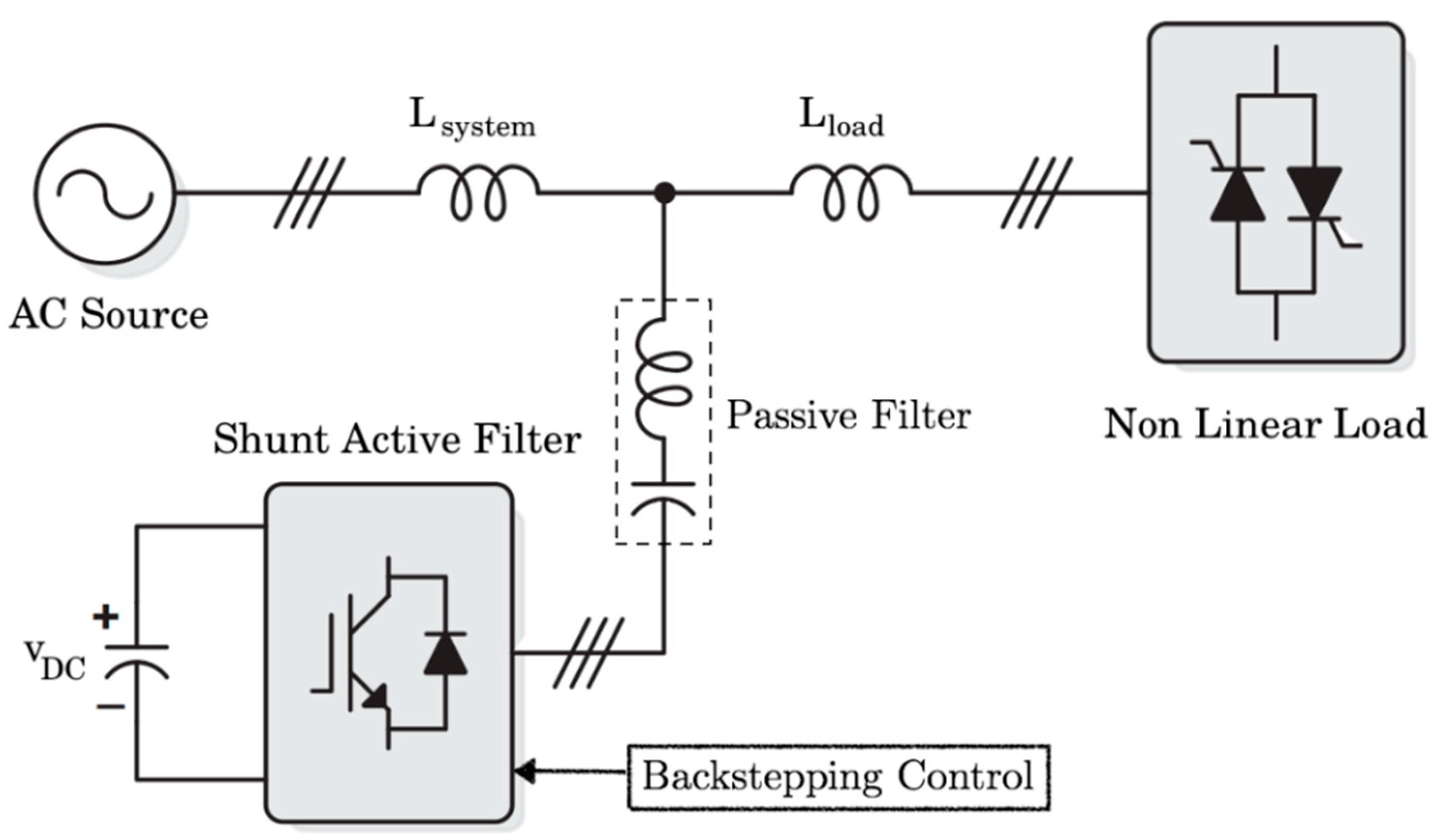
2. Proposed Hybrid Filter
- Serial active filter with parallel passive filter;
- Serial active filter connected in series with parallel passive filter;
- Parallel active filter in series with a passive filter.
2.1. Current Reference Algorithm Using p-q Theory
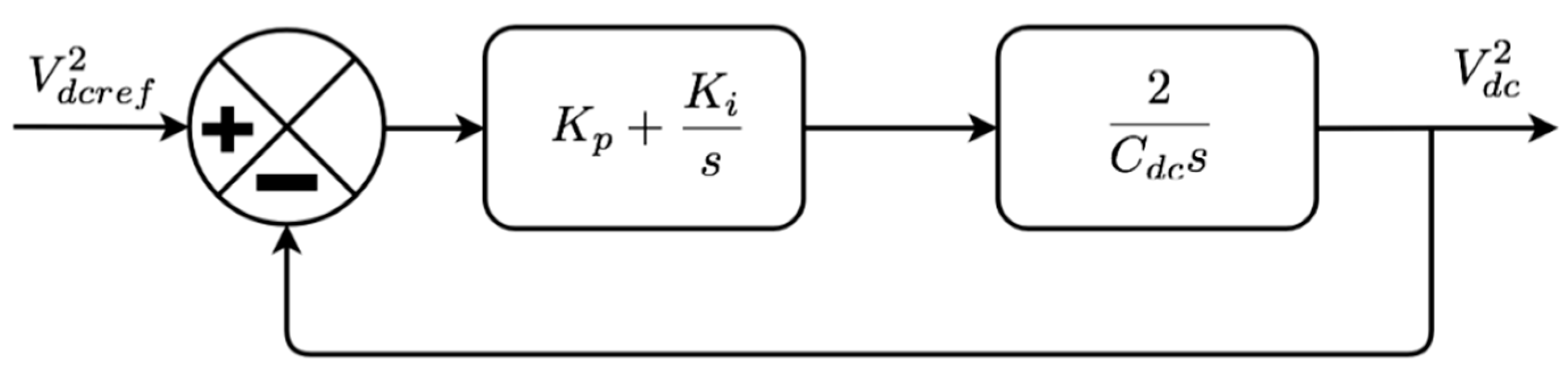
2.2. DC Bus Voltage Regulation
2.3. Regulation of the Current Injected by the Filter
2.4. Fuzzy Logic Control
3. Proposed Backstepping Control
3.1. Subsystem 1
3.2. Subsystem 2
3.3. Subsystem 3
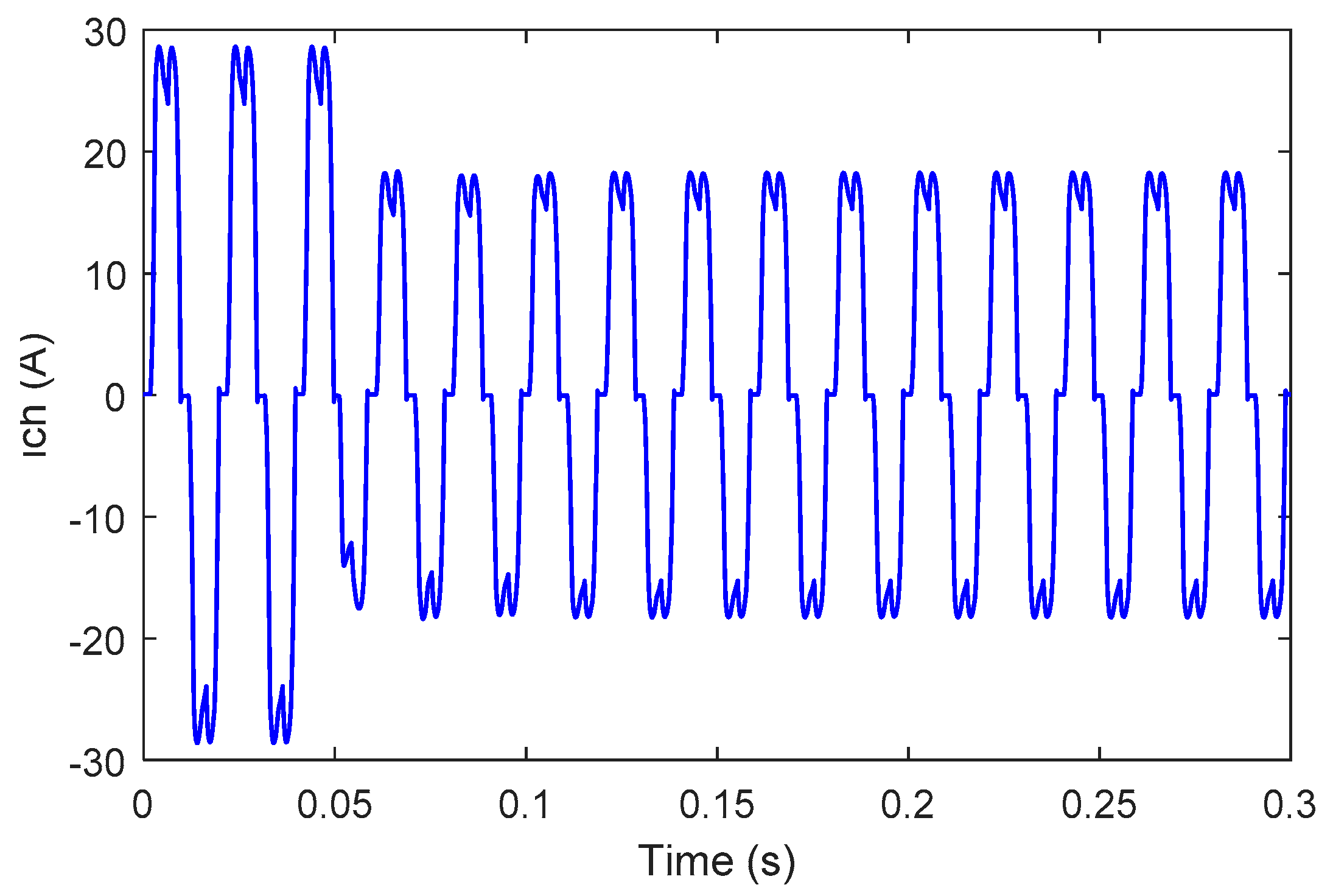
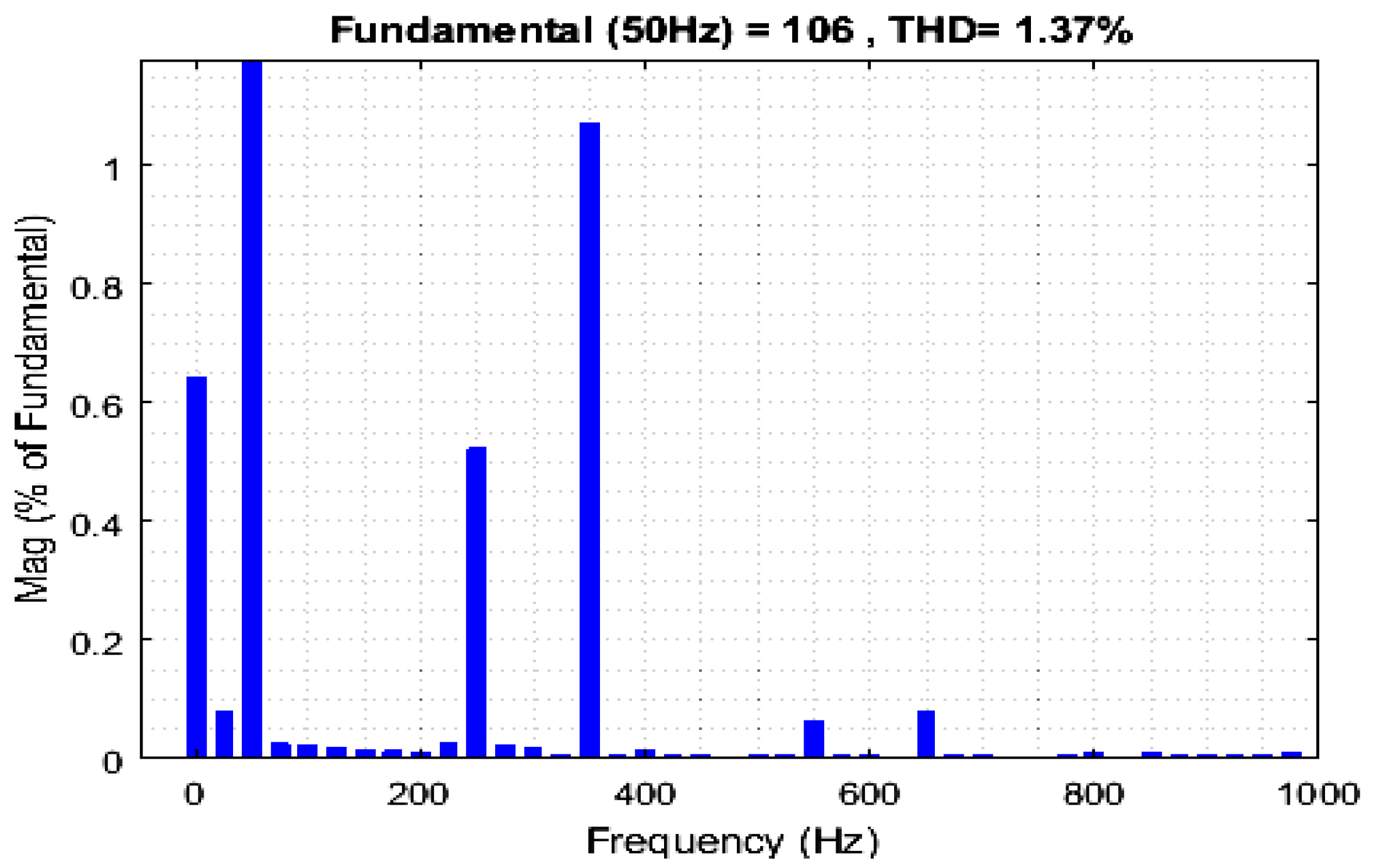
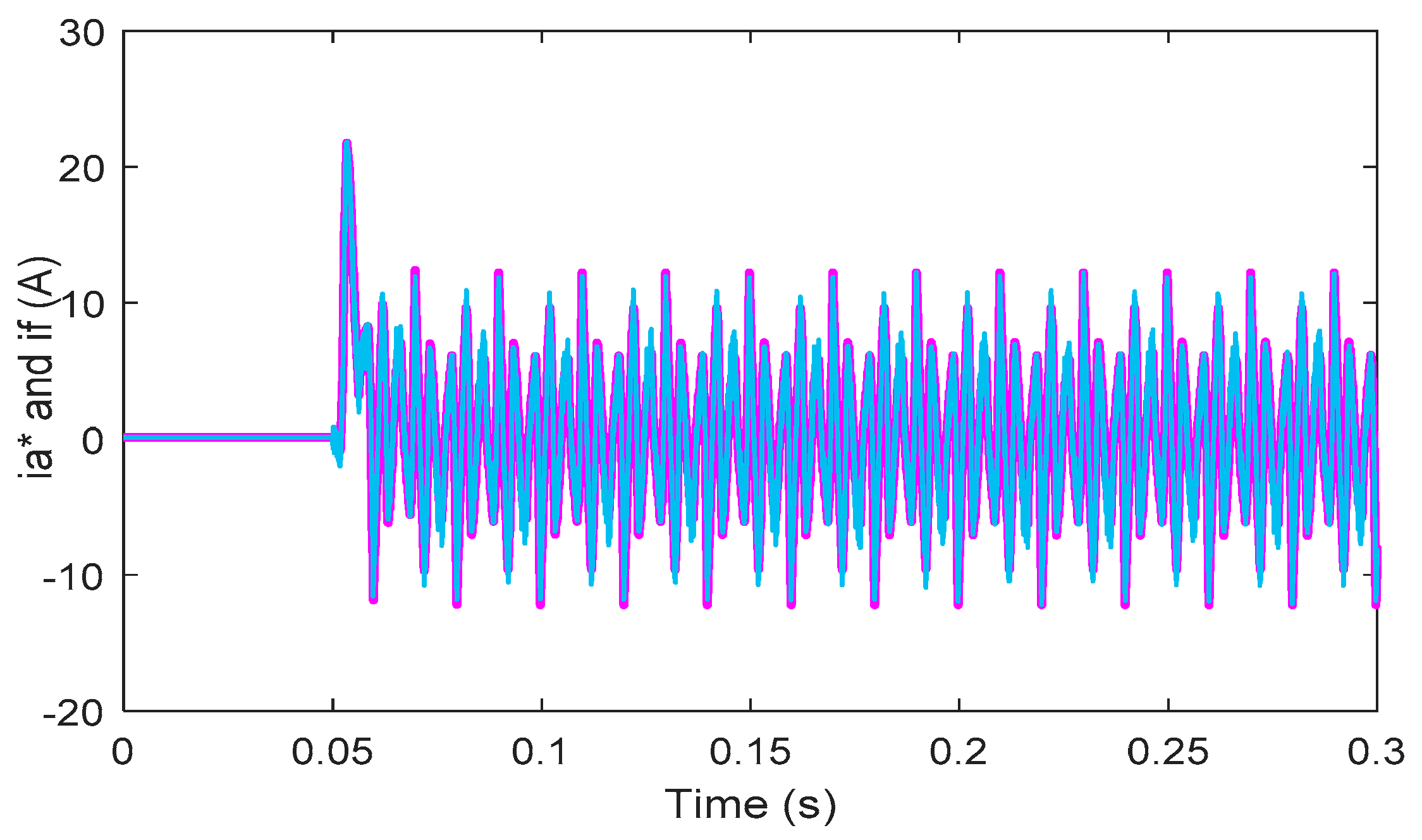
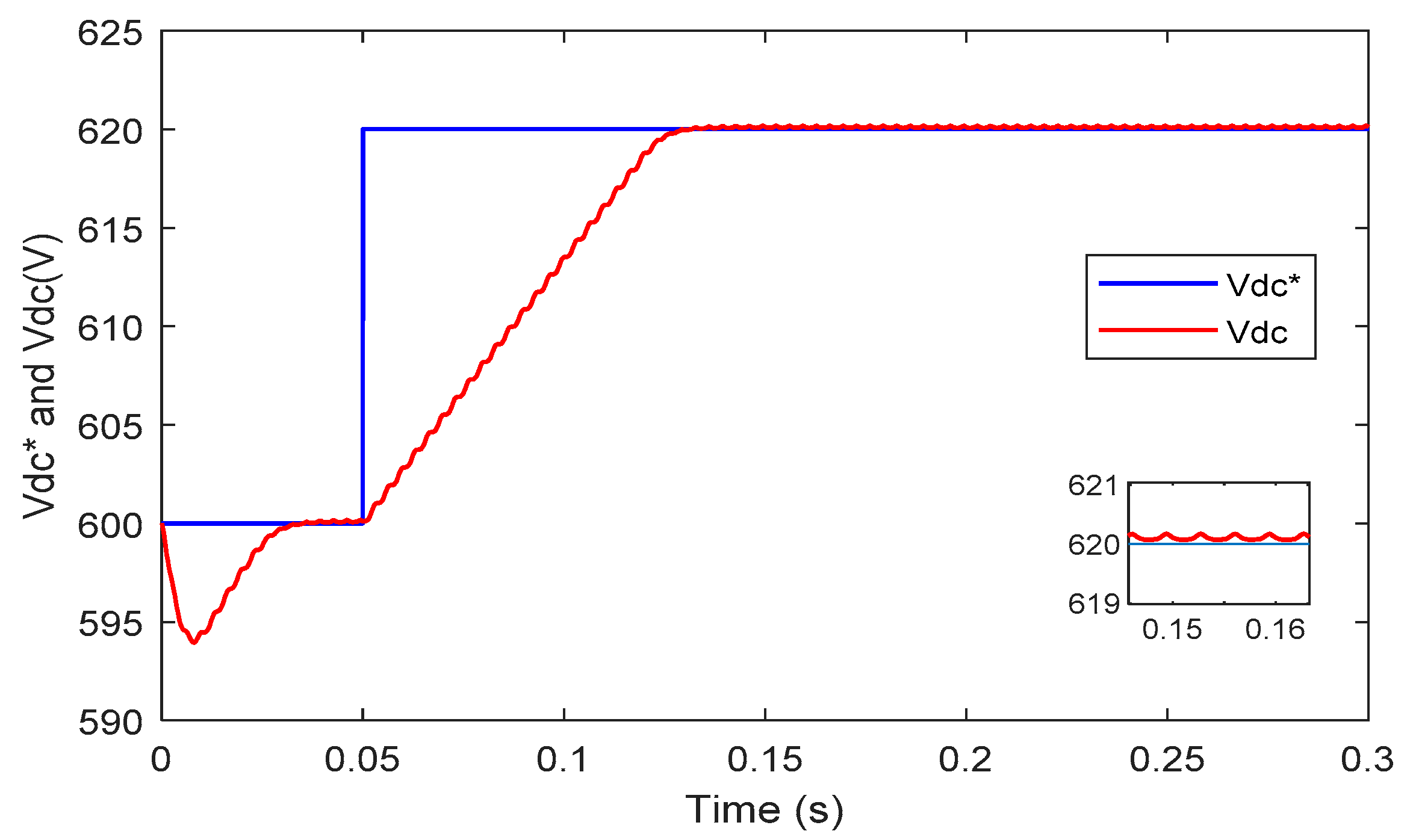
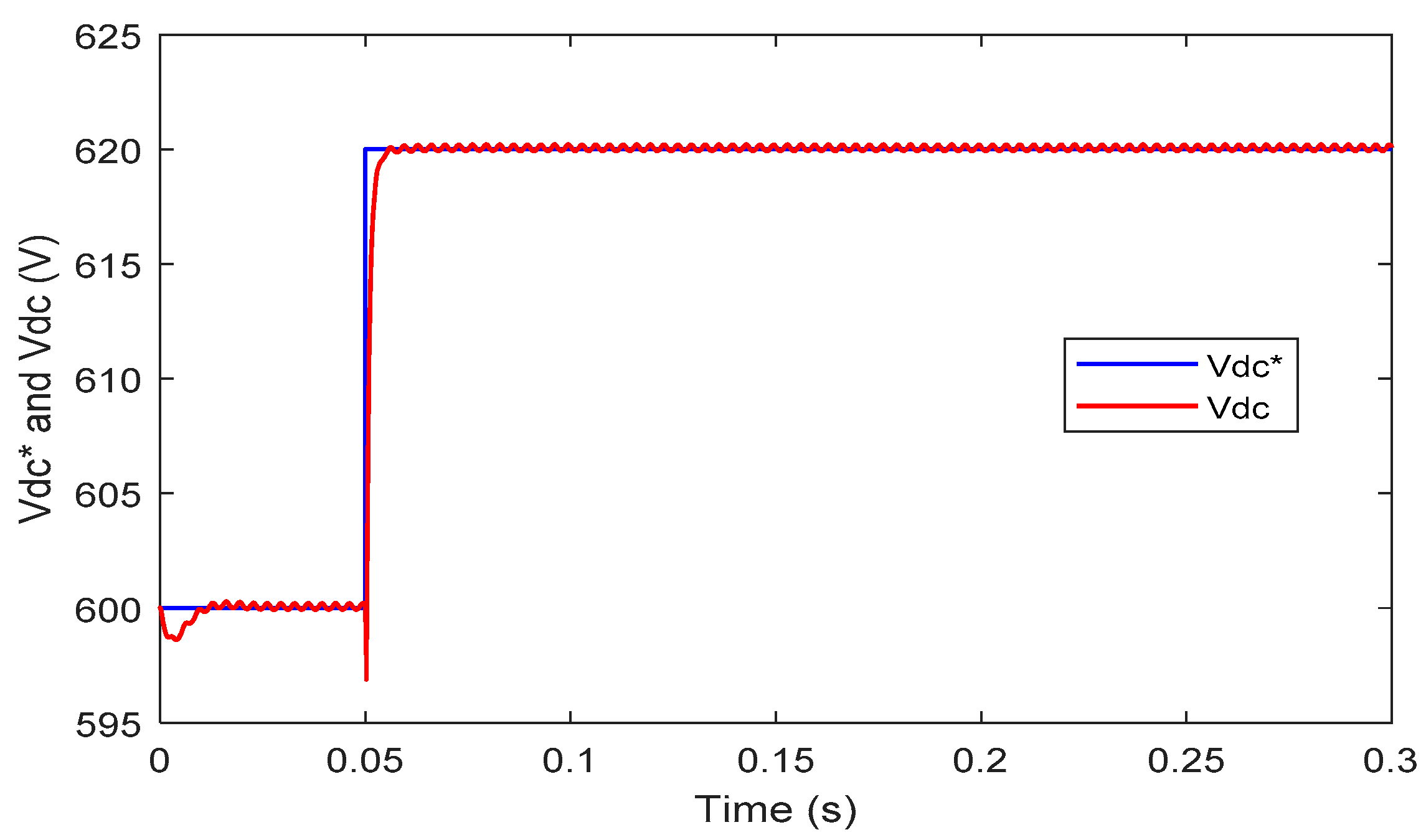
4. Simulation and Interpretation
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Akagi, H. Large static converters for industry and utility applications. Proc. IEEE 2001, 89, 976–983. [Google Scholar] [CrossRef]
- Krastev, I.; Tricoli, P.; Hillmansen, S.; Chen, M. Future of Electric Railways: Advanced Electrification Systems with Static Converters for ac Railways. IEEE Electrif. Mag. 2016, 4, 6–14. [Google Scholar] [CrossRef]
- Emanuel, A.E. On the assessment of harmonic pollution [of power systems]. IEEE Trans. Power Deliv. 1995, 10, 1693–1698. [Google Scholar] [CrossRef]
- Redl, R.; Tenti, P.; Daan van Wyk, J. Power electronics’ polluting effects. IEEE Spectr. 1997, 34, 32–39. [Google Scholar] [CrossRef]
- Singh, B.; Al-Haddad, K.; Chandra, A. A review of active filters for power quality improvement. IEEE Trans. Ind. Electron. 1999, 46, 960–971. [Google Scholar] [CrossRef]
- Rahmani, S.; Al-Haddad, K.; Kanaan, H.Y. A comparative study of shunt hybrid and shunt active power filters for single-phase applications: Simulation and experimental validation. Math. Comput. Simul. 2006, 71, 345–359. [Google Scholar] [CrossRef]
- Peng, F.Z.; Adams, D.J. Harmonic sources and filtering approaches-series/parallel, active/passive, and their combined power filters. In Proceedings of the Conference Record of the 1999 IEEE Industry Applications Conference: Thirty-Forth IAS Annual Meeting (Cat. No.99CH36370), Phoenix, AZ, USA, 3–7 October 1999; Volume 1, pp. 448–455. [Google Scholar]
- Peng, F.Z. Application issues of active power filters. IEEE Ind. Appl. Mag. 1998, 4, 21–30. [Google Scholar] [CrossRef]
- Kim, S.; Enjeti, P.N. A new hybrid active power filter (APF) topology. IEEE Trans. Power Electron. 2002, 17, 48–54. [Google Scholar]
- Graovac, D.; Katic, V.; Rufer, A. Power Quality Problems Compensation With Universal Power Quality Conditioning System. IEEE Trans. Power Deliv. 2007, 22, 968–976. [Google Scholar] [CrossRef]
- Routimo, M.; Salo, M.; Tuusa, H. Comparison of Voltage-Source and Current-Source Shunt Active Power Filters. IEEE Trans. Power Electron. 2007, 22, 636–643. [Google Scholar] [CrossRef]
- Benchaita, L.; Saadate, S.; Salem nia, A. A comparison of voltage source and current source shunt active filter by simulation and experimentation. IEEE Trans. Power Syst. 1999, 14, 642–647. [Google Scholar] [CrossRef]
- Ghadbane, I.; Benchouia, T.; Tahar, G. Comparative study of backstepping and Proportional Integral Controller to Compensating Current Harmonics. In Proceedings of the International Conference on Systems and Processing Information, Guelma, Algeria, 12–14 May 2013. [Google Scholar]
- Zelloma, L.; Rabhi, B.; Saad, S.; Benaissa, A.; Benkhoris, M.F. Fuzzy logic controller of five levels active power filter. Energy Procedia 2015, 74, 1015–1025. [Google Scholar] [CrossRef]
- Abdusalam, M.; Poure, P.; Saadate, S. Control of hybrid active filter without phase locked loop in the feedback et feedforward loops. In Proceedings of the ISIE, IEEE International Symposium on Industrial Electronics, Cambridge, UK, 30 June–2 July 2008. [Google Scholar]
- El-Habrouk, M.; Darwish, M.K.; Mehta, P. Active power filters: A review. IEE Proc. Electr. Power Appl. 2000, 147, 403. [Google Scholar] [CrossRef]
- Akagi, H. Control strategy and site selection of a shunt active filter for damping of harmonic propagation in power distribution systems. IEEE Trans. Power Deliv. 1997, 12, 354–363. [Google Scholar] [CrossRef]
- Marques, G.D. A comparison of active power filter control methods in unbalanced and non-sinusoidal conditions. In Proceedings of the 24th Annual Conference of the IEEE Industrial Electronics Society (Cat.No.98CH36200), IECON ’98, Aachen, Germany, 31 August–4 September 1998. [Google Scholar]
- Sahnouni, K.; Godfroid, H.; Berthon, A. An optimised variable structure control of a shunt active filter. In Proceedings of the IEEE International Electric Machines and Drives Conference: IEMDC’99. Proceedings (Cat. No.99EX272), Seattle, WA, USA, 9–12 May 1999; pp. 682–684. [Google Scholar]
- Jasim, W.; Gu, D. Integral backstepping controller for quadrotor path tracking. In Proceedings of the 2015 International Conference on Advanced Robotics (ICAR), Istanbul, Turkey, 27–31 July 2015; pp. 593–598. [Google Scholar]
- Ouchatti, A.; Abbou, A.; Akherraz, M.; Taouni, A. Induction motor controller using fuzzy MRAS and backstepping approach. Int. Rev. Electr. Eng. 2014, 9, 511–518. [Google Scholar]
- Hou, S.; Fei, J. Adaptive fuzzy backstepping control of three-phase active power filter. Control Eng. Pract. 2015, 45, 12–21. [Google Scholar] [CrossRef]
- Benchouia, M.T.; Ghadbane, I.; Golea, A.; Srairi, K.; Benbouzid, M.E.H. Implementation of Adaptive Fuzzy Logic and PI Controllers to Regulate the DC Bus Voltage of Shunt Active Power Filter. Appl. Soft Comput. 2015, 28, 125–131. [Google Scholar] [CrossRef]
- Ait Chihab, A.; Ouadi, H.; Giri, F.; El Majdoub, K. Adaptive Backstepping Control of Three-Phase Four-Wire Shunt Active Power Filters for Energy Quality improvement. J. Control Autom. Electr. Syst. 2016, 27, 144–156. [Google Scholar] [CrossRef]
- Campanhol, L.B.G.; da Silva, S.A.; Goedtel, A. Application of Shunt Active Power Filter for Harmonic Reduction and Reactive Power Compensation in Three-Phase Four-Wire Systems. IET Power Electron. 2014, 7, 2825–2836. [Google Scholar] [CrossRef]













© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Daou, N.; Montoya, F.G.; Ababssi, N.; Djeghader, Y. A Hybrid Active Filter Using the Backstepping Controller for Harmonic Current Compensation. Symmetry 2019, 11, 1161. https://doi.org/10.3390/sym11091161
Daou N, Montoya FG, Ababssi N, Djeghader Y. A Hybrid Active Filter Using the Backstepping Controller for Harmonic Current Compensation. Symmetry. 2019; 11(9):1161. https://doi.org/10.3390/sym11091161
Chicago/Turabian StyleDaou, Nora, Francisco G. Montoya, Najib Ababssi, and Yacine Djeghader. 2019. "A Hybrid Active Filter Using the Backstepping Controller for Harmonic Current Compensation" Symmetry 11, no. 9: 1161. https://doi.org/10.3390/sym11091161
APA StyleDaou, N., Montoya, F. G., Ababssi, N., & Djeghader, Y. (2019). A Hybrid Active Filter Using the Backstepping Controller for Harmonic Current Compensation. Symmetry, 11(9), 1161. https://doi.org/10.3390/sym11091161




