Magnetohydrodynamics Stagnation-Point Flow of a Nanofluid Past a Stretching/Shrinking Sheet with Induced Magnetic Field: A Revised Model
Abstract
1. Introduction
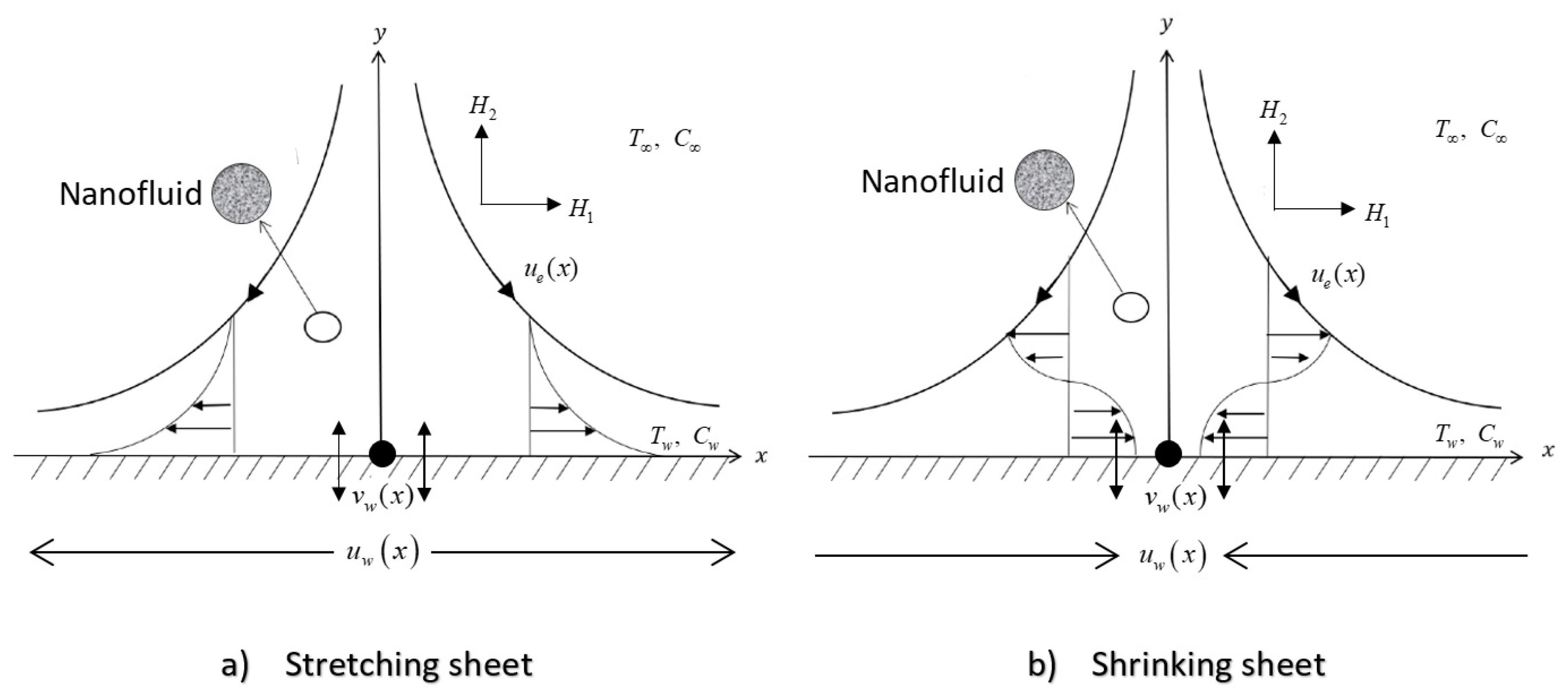
2. Problem Formulation
3. Stability Analysis
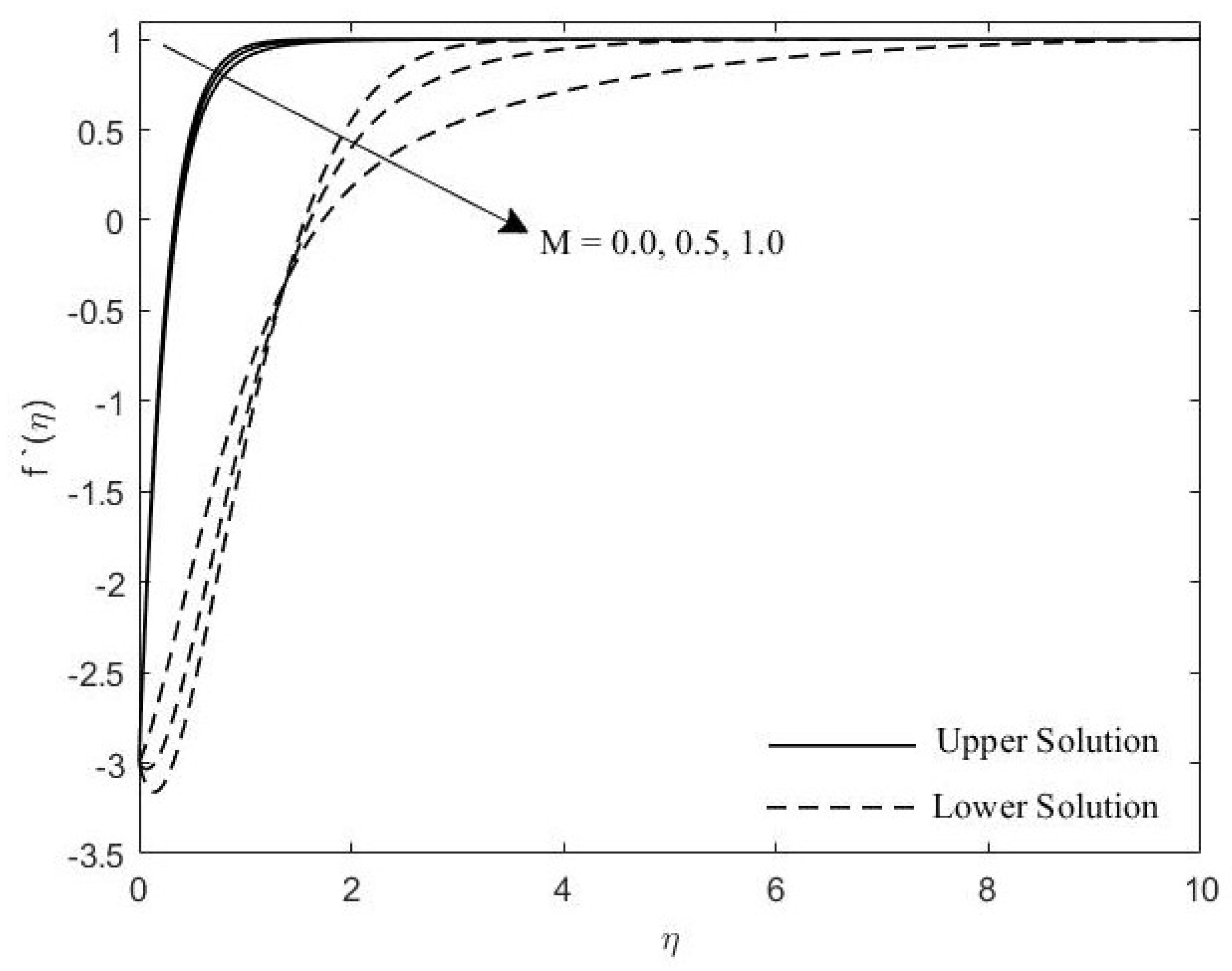
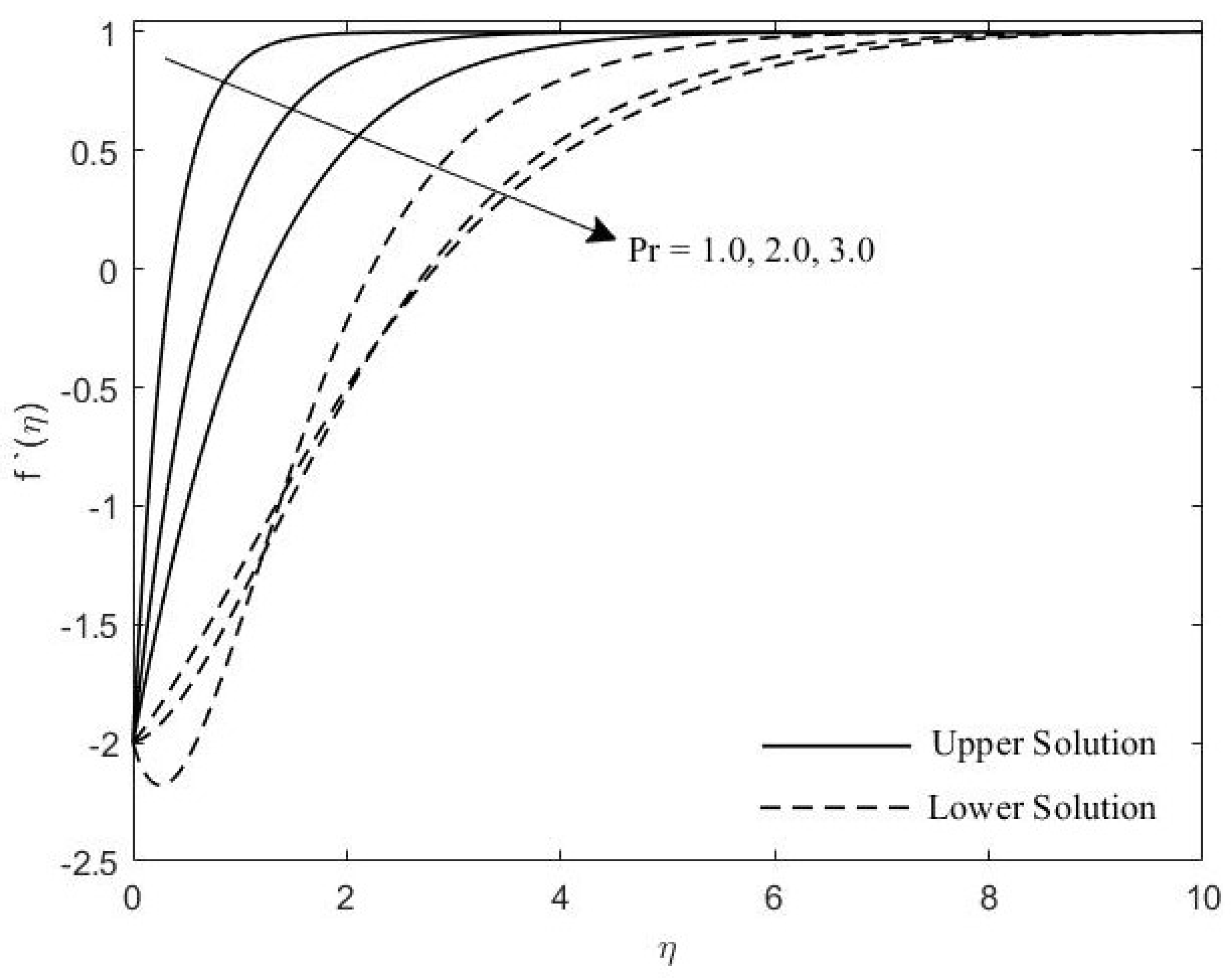
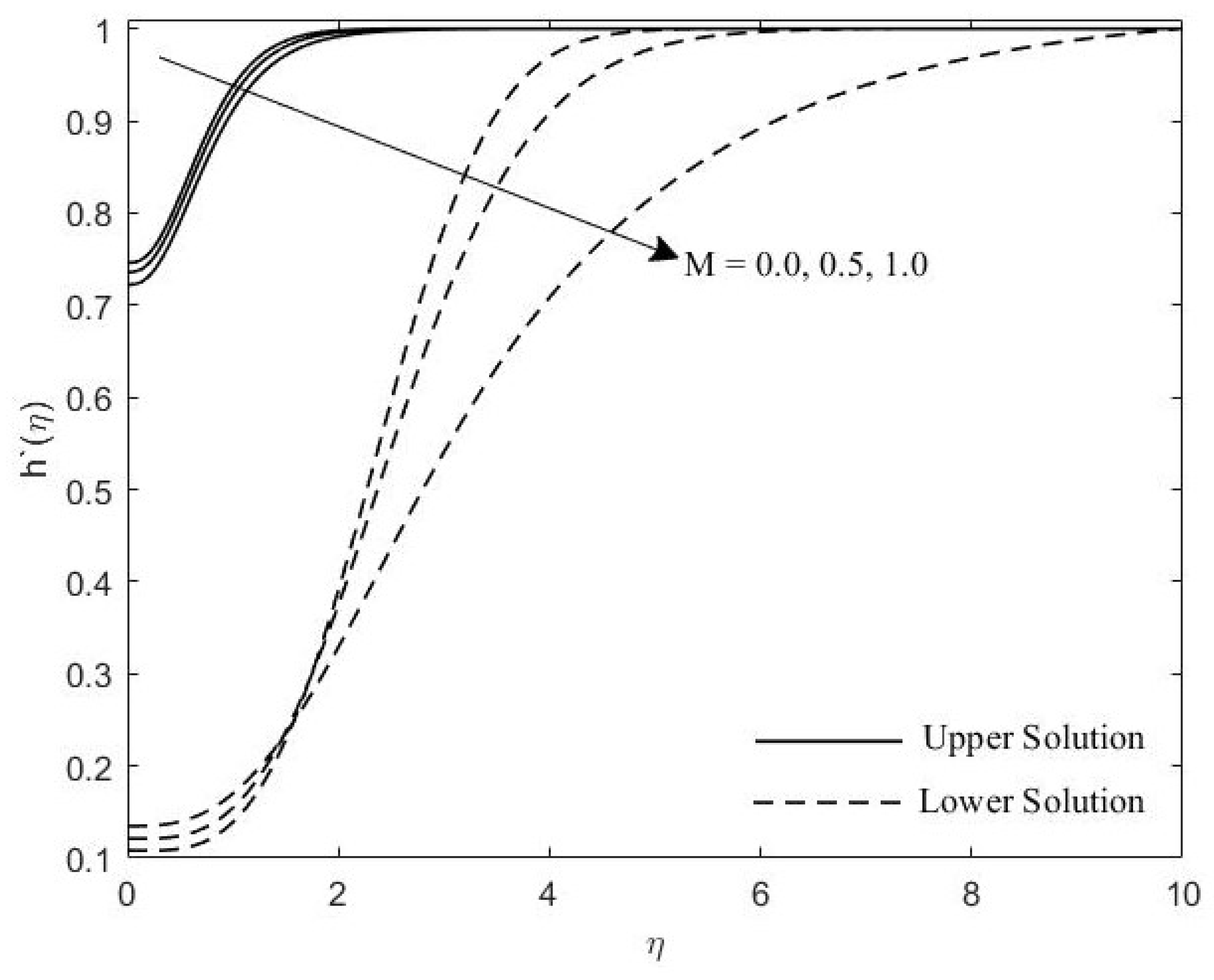
4. Results and Discussion
5. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
Abbreviations
| Roman Letters | |
| a | constant variable |
| C | nanoparticle volume fraction |
| skin friction coefficient | |
| specific heat capacity | |
| Brownian diffusion coefficient | |
| thermophoresis diffusion coefficient | |
| dimensionless stream function | |
| dimensionless induced magnetic field | |
| applied magnetic field | |
| magnetic field at the edge | |
| induced magnetic field components along the x and y directions, respectively | |
| k | thermal conductivity |
| Lewis number | |
| M | magnetic parameter |
| Brownian motion parameter | |
| thermophoresis parameter | |
| local Nusselt number | |
| Prandtl number | |
| surface heat flux | |
| local Reynolds number | |
| S | suction/injection parameter |
| local Sherwood number | |
| t | time |
| T | temperature of the nanofluid |
| velocity at the edge of the boundary layer | |
| velocity components along the x and y directions, respectively | |
| constant mass velocity | |
| Cartesian coordinates | |
| Greek Symbols | |
| thermal diffusivity of the nanofluid | |
| eigenvalue | |
| smallest eigenvalue | |
| ratio of nanoparticle heat capacity to the base fluid heat capacity | |
| similarity variable | |
| dimensionless temperature | |
| stretching/shrinking parameter | |
| magnetic permeability | |
| magnetic diffusivity | |
| kinematic viscosity | |
| density | |
| dimensionless time | |
| surface shear stress | |
| dimensionless nanoparticle volume fraction | |
| reciprocal of the magnetic Prandtl number | |
| Subscripts | |
| w | condition at the surface |
| ∞ | condition outside of boundary layer |
| c | critical value |
| f | base fluid |
| p | nanoparticle |
| Superscripts | |
| differentiation with respect to | |
References
- Fisher, E.G. Extrusion of Plastics, 3rd ed.; Wiley: New York, NY, USA, 1976. [Google Scholar]
- Tsou, F.K.; Sparrow, E.M.; Goldstein, R.J. Flow and heat transfer in the boundary layer on a continuous moving surface. Int. J. Heat Mass Transf. 1967, 10, 219–235. [Google Scholar] [CrossRef]
- Sakiadis, B.C. Boundary-layer behavior on continuous solid surfaces: I. Boundary-layer equations for two-dimensional and axisymmetric flow. AIChE J. 1961, 7, 26–28. [Google Scholar] [CrossRef]
- Crane, L.J. Flow past a stretching plate. J. Appl. Math. Phys. (ZAMP) 1970, 21, 645–647. [Google Scholar] [CrossRef]
- Miklavčič, M.; Wang, C.Y. Viscous flow due to a shrinking sheet. Q. Appl. Math. 2006, 64, 283–290. [Google Scholar] [CrossRef]
- Wang, C.Y. Stagnation flow towards a shrinking sheet. Int. J. Non-Linear Mech. 2008, 43, 377–382. [Google Scholar] [CrossRef]
- Shercliff, J.A. A Textbook of Magnetohydrodynamics; Pergamon Press: Oxford, UK; New York, NY, USA, 1965. [Google Scholar]
- Branover, G.G.; Tinober, A.B. Magnetohydrodynamics of Incompressible Media (in Russian); Nauka: Moscow, Russia, 1970. [Google Scholar]
- Cramer, K.R.; Pai, S.I. Magneto Fluid Dynamics For Engineers and Applied Physicists; McGraw-Hill Book Company: Washington, DC, USA, 1973. [Google Scholar]
- Apelblat, A. Application of the Laplace transform to the solution of the boundary layer equations. III: Magnetohydrodynamic Falkner-Skan problem. J. Phys. Soc. Jpn. 1969, 27, 235–239. [Google Scholar] [CrossRef]
- Ingham, D.B. Impulsively started viscous flows past a finite flat plate with and without an applied magnetic field. Int. J. Numer. Methods Eng. 1973, 6, 521–527. [Google Scholar] [CrossRef]
- Liron, N.; Wilhelm, H.E. Integration of the Magnetohydrodynamic boundary-layer equations by Meksyn’s method. J. Appl. Math. Mech. (ZAMM) 1974, 54, 27–37. [Google Scholar] [CrossRef]
- Watanabe, T.; Pop, I. Magnetohydrodynamic free convection flow over a wedge in the presence of a transverse magnetic field. Int. Commun. Heat Mass Trans. 1993, 20, 871–881. [Google Scholar] [CrossRef]
- Gul, A.; Khan, I.; Shafie, S.; Khalid, A.; Khan, A. Heat transfer in MHD mixed convection flow of a ferrofluid along a vertical channel. PLoS ONE 2015, 10, e0141213. [Google Scholar] [CrossRef]
- Kishore, P.M.; Rajesh, V.; Verma, V. Effects of heat transfer and viscous dissipation on MHD free convection flow past an exponentially accelerated vertical plate with variable temperature. J. Nav. Archit. Mar. Eng. 2010, 7, 101–110. [Google Scholar] [CrossRef]
- Shehzad, S.A.; Abdullah, Z.; Alsaedi, A.; Abbasi, F.M.; Hayat, T. Thermally radiative three-dimensional flow of Jeffrey nanofluid with internal heat generation and magnetic field. J. Magn. Magn. Mater. 2016, 397, 108–114. [Google Scholar] [CrossRef]
- Hayat, T.; Waqas, M.; Khan, M.I.; Alsaedi, A. Impacts of constructive and destructive chemical reactions in magnetohydrodynamic (MHD) flow of Jeffrey liquid due to nonlinear radially stretched surface. J. Mol. Liq. 2017, 225, 302–310. [Google Scholar] [CrossRef]
- Tashtoush, B.; Magableh, A. Magnetic field effect on heat transfer and fluid flow characteristics of blood flow in multi-stenosis arteries. Heat Mass Trans. 2008, 44, 297–304. [Google Scholar] [CrossRef]
- Mukhopadhyay, S. MHD boundary layer flow and heat transfer over an exponentially stretching sheet embedded in a thermally stratified medium. Alexendria Eng. J. 2013, 52, 259–265. [Google Scholar] [CrossRef]
- Tian, X.; Li, B.; Zhang, J. The effects of radiation optical properties on the unsteady 2D boundary layer MHD flow and heat transfer over a stretching plate. Int. J. Heat Mass Transf. 2017, 105, 109–112. [Google Scholar] [CrossRef]
- Ali, F.M.; Nazar, R.; Arifin, N.M.; Pop, I. Dual solutions in MHD flow on a nonlinear porous shrinking sheet in a viscous fluid. Bound. Value Probl. 2013, 1, 32. [Google Scholar] [CrossRef]
- Jusoh, R.; Nazar, R.; Pop, I. Magnetohydrodynamic rotating flow and heat transfer of ferrofluid due to an exponentially permeable stretching/shrinking sheet. J. Magn. Magn. Mater. 2018, 465, 365–374. [Google Scholar] [CrossRef]
- Ali, F.M.; Nazar, R.; Arifin, N.M.; Pop, I. MHD mixed convection boundary layer flow toward a stagnation point on a vertical surface with induced magnetic field. ASME J. Heat Transf. 2011, 133, 022502. [Google Scholar] [CrossRef]
- Ali, F.M.; Nazar, R.; Arifin, N.M.; Pop, I. MHD boundary layer flow and heat transfer over a stretching sheet with induced magnetic field. Heat Mass Trans. 2011, 47, 155–162. [Google Scholar] [CrossRef]
- Choi, S.U.S.; Eastman, J.A. Enhancing thermal conductivity of fluids with nanoparticles. In Proceedings of the 1995 International Mechanical Engineering Congress and Exposition, San Francisco, CA, USA, 12–17 November 1995; Volume 66, pp. 99–105. [Google Scholar]
- Xie, H.; Wang, J.; Xi, T.; Liu, Y.; Ai, F.; Wu, Q. Thermal conductivity enhancement of suspensions containing nanosized alumina particles. J. Appl. Phys. 2002, 91, 4568–4572. [Google Scholar] [CrossRef]
- Das, S.; Putra, N.; Thiesen, P.; Roetzel, W. Temperature dependence of thermal conductive enhancement for nanofluids. J. Heat Transf. 2003, 125, 567–574. [Google Scholar] [CrossRef]
- Lee, D.; Kim, J.; Kim, B. A new parameter to control heat transport in nanofluide: Surface charge state of the particle in suspension. J. Phys. Chem. B 2006, 110, 4323–4328. [Google Scholar] [CrossRef] [PubMed]
- Lee, J.; Hwang, K.; Jang, S.; Lee, B.; Kim, J.; Choi, S.; Choi, C. Effective viscosities and thermal conductivities of aqueous nanofluids containing low volume concentrations of Al2O3 nanoparticles. Int. J. Heat Mass Transf. 2008, 51, 2651–2656. [Google Scholar] [CrossRef]
- Minsta, H.; Roy, G.; Nguyen, C.; Doucet, D. New temperature dependent thermal conductivity data for water based nanofluids. Int. J. Heat Mass Transf. 2009, 48, 363–371. [Google Scholar]
- Bondareva, N.S.; Sheremet, M.A.; Oztop, H.F.; Abu-Hamdeh, N. Heatline visualization of natural convection in a thick walled open cavity filled with a nanofluid. Int. J. Heat Mass Transf. 2017, 109, 175–186. [Google Scholar] [CrossRef]
- Saidur, R.; Leong, K.Y.; Mohammad, H.A. A review on applications and challenges of nanofluids. Renew. Sustain. Energy Rev. 2011, 15, 1646–1668. [Google Scholar] [CrossRef]
- Das, S.K.; Choi, S.U.S.; Yu, W.; Pradeep, Y. Nanofluids: Science and Technology; Wiley: Hoboken, NJ, USA, 2008. [Google Scholar]
- Nield, D.A.; Bejan, A. Convection in Porous Media, 4th ed.; Springer: New York, NY, USA, 2013. [Google Scholar]
- Minkowycz, W.J.; Sparrow, E.M.; Abraham, J.P. Nanoparticle Heat Transfer and Fluid Flow; CRC Press, Taylor and Francis Group: New York, NY, USA, 2013. [Google Scholar]
- Shenoy, A.; Sheremet, M.; Pop, I. Convective Flow and Heat Transfer from Wavy Surfaces: Viscous Fluids, Porous Media and Nanofluids; CRC Press, Taylor and Francis Group: New York, NY, USA, 2016. [Google Scholar]
- Buongiorno, J.; Venerus, D.C.; Prabhat, N.; McKrell, T.; Townsend, J.; Christianson, R.; Tolmachev, Y.V.; Keblinski, P.; Hu, L.; Alvarado, J.L.; et al. A benchmark study on the thermal conductivity of nanofluids. J. Appl. Phys. 2009, 106, 094312. [Google Scholar] [CrossRef]
- Kakaç, S.; Pramuanjaroenkij, A. Review of convective heat transfer enhancement with nanofluids. Int. J. Heat Mass Transf. 2009, 52, 3187–3196. [Google Scholar] [CrossRef]
- Manca, O.; Jaluria, Y.; Poulikakos, D. Heat transfer in nanofluids. Adv. Mech. Eng. 2010, 2, 380826. [Google Scholar] [CrossRef]
- Fan, J.; Wang, L. Review of heat conduction in nanofluids. ASME J. Heat Transf. 2011, 133, 040801. [Google Scholar] [CrossRef]
- Mahian, O.; Kianifar, A.; Kalogirou, S.A.; Pop, I.; Wongwises, S. A review of the applications of nanofluids in solar energy. Int. J. Heat Mass Transf. 2013, 57, 582–594. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Ganji, D.D. Nanofluid convective heat transfer using semi analytical and numerical approaches: A review. J. Taiwan Inst. Chem. Eng. 2016, 65, 43–77. [Google Scholar] [CrossRef]
- Myers, T.G.; Ribera, H.; Cregan, V. Does mathematics contribute to the nanofluid debate? Int. J. Heat Mass Transf. 2017, 111, 279–288. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Mehryan, S.A.M.; Shafee, A.; Sheremet, M.A. Variable magnetic forces impact on magnetizable hybrid nanofluid heat transfer through a circular cavity. J. Mol. Liq. 2019, 277, 388–396. [Google Scholar] [CrossRef]
- Mahapatra, T.R.; Nandy, S.K. Stability of dual solutions in stagnation-point flow and heat transfer over a porous shrinking sheet with thermal radiation. Meccanica 2013, 48, 23–32. [Google Scholar] [CrossRef]
- Hussaini, M.Y.; Lakin, W.D. Existence and non-uniqueness of similarity solutions of a boundary-layer problem. Quart. J. Mech. Appl. Math. 1986, 39, 15–24. [Google Scholar] [CrossRef][Green Version]
- Magyari, E.; Ali, M.E.; Keller, B. Heat and mass transfer characteristics of the self-similar boundary-layer flows induced by continous surfaces stretched with rapidly decreasing velocities. Heat Mass Transf. 2001, 38, 65–75. [Google Scholar] [CrossRef]
- Merrill, K.; Beauchesne, M.; Previte, J.; Paullet, J.; Weidman, P. Final steady flow near a stagnation-point on a vertical surface in a porous medium. Int. J. Heat Mass Transf. 2006, 49, 4681–4686. [Google Scholar] [CrossRef]
- Merkin, J.H. On dual solutions occuring in mixed convection in a porous medium. J. Eng. Math. 1985, 20, 171–179. [Google Scholar] [CrossRef]
- Junoh, M.M.; Ali, F.M.; Pop, I. MHD stagnation-point flow of a nanofluid past a stretching/shrinking sheet with induced magnetic field. J. Eng. Appl. Sci. 2018, 13, 10474–10481. [Google Scholar]
- Kuznetsov, A.V.; Nield, D.A. The Cheng–Minkowycz problem for natural convective boundary layer flow in a porous medium saturated by a nanofluid: A revised model. Int. J. Heat Mass Transf. 2013, 65, 682–685. [Google Scholar] [CrossRef]
- Davies, T.V. The magneto-hydrodynamic boundary layer in two-dimensional steady flow past a semi-infinite flat plate I. Uniform conditions at infinity. Proc. R. Soc. A 1962, 273, 496–508. [Google Scholar]
- Kuznetsov, A.V.; Nield, D.A. Natural convective boundary-layer flow of a nanofluid past a vertical plate. Int. J. Therm. Sci. 2010, 49, 243–247. [Google Scholar] [CrossRef]
- Harris, S.D.; Ingham, D.B.; Pop, I. Mixed convection boundary-layer flow near the stagnation point on a vertical surface in a porous medium: Brinkman model with slip. Transp. Porous Media 2009, 77, 267–285. [Google Scholar] [CrossRef]
- Aman, F.; Ishak, A.; Pop, I. Magnetohydrodynamic stagnation-point flow towards a stretching/shrinking sheet with slip effects. Int. Commun. Heat Mass Transf. 2013, 47, 68–72. [Google Scholar] [CrossRef]
- Bhattacharyya, K.; Mukhopadhyay, S.; Layek, G.C. Slip effects on boundary layer stagnation-point flow and heat transfer towards a shrinking sheet. Int. J. Heat Mass Transf. 2011, 54, 308–313. [Google Scholar] [CrossRef]
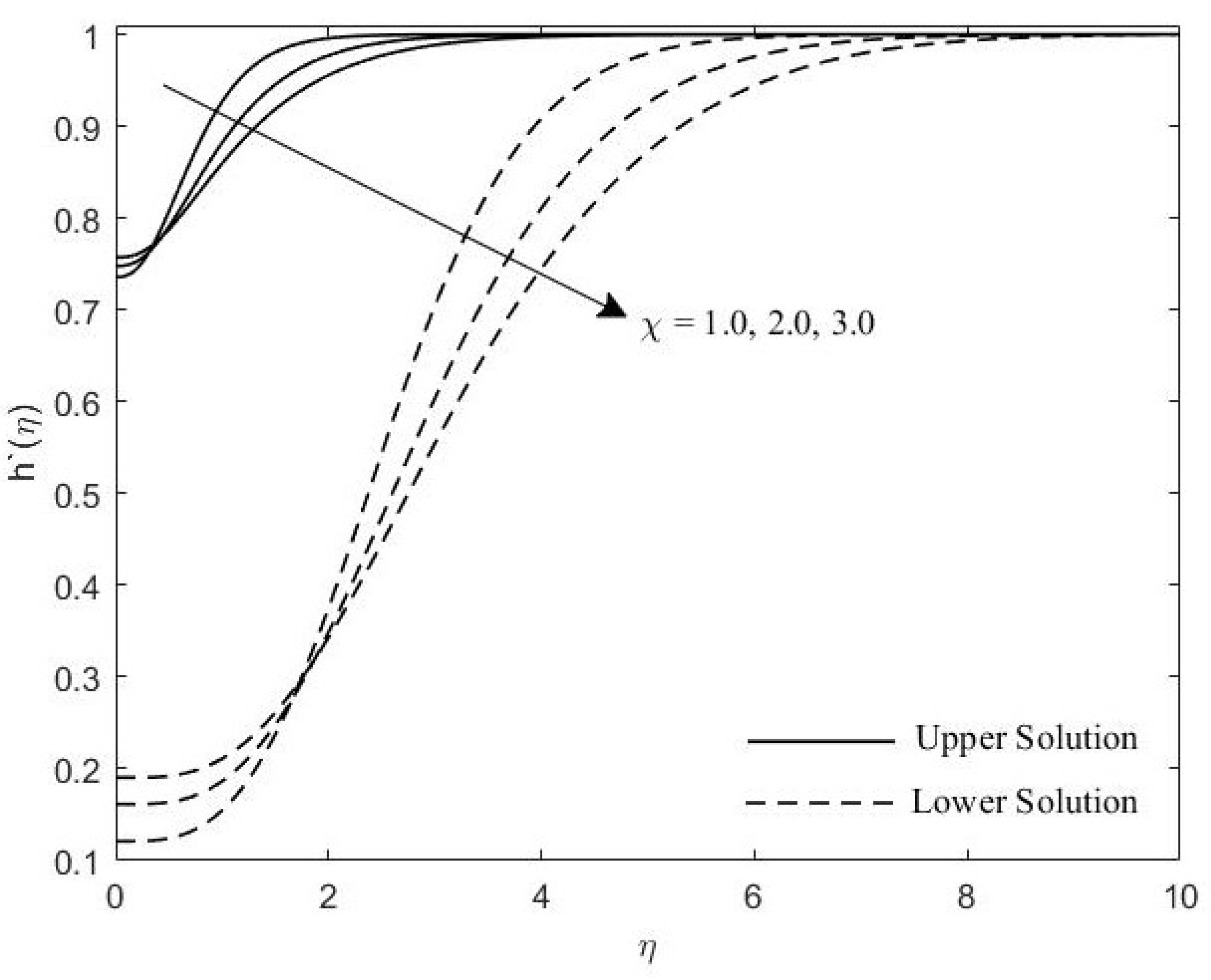
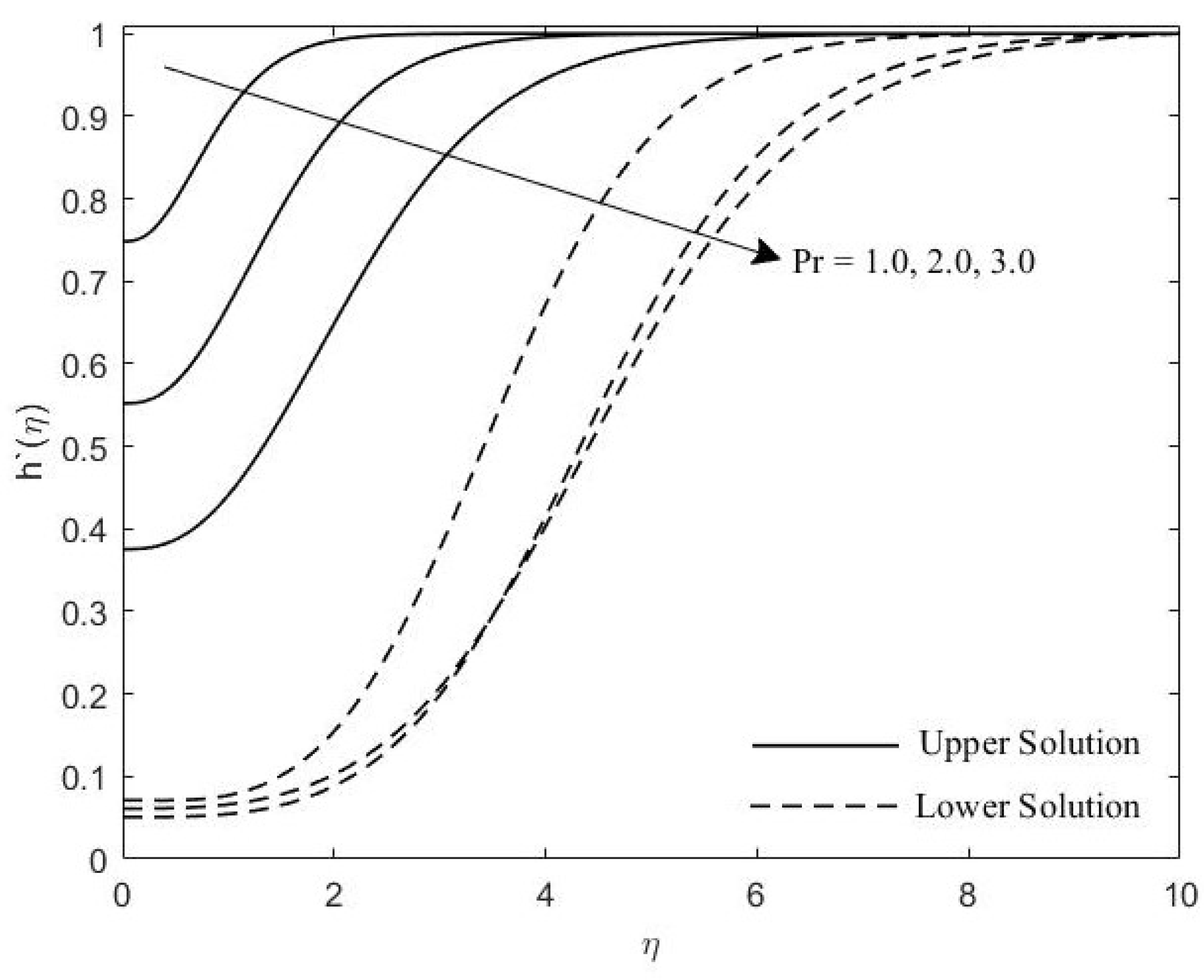


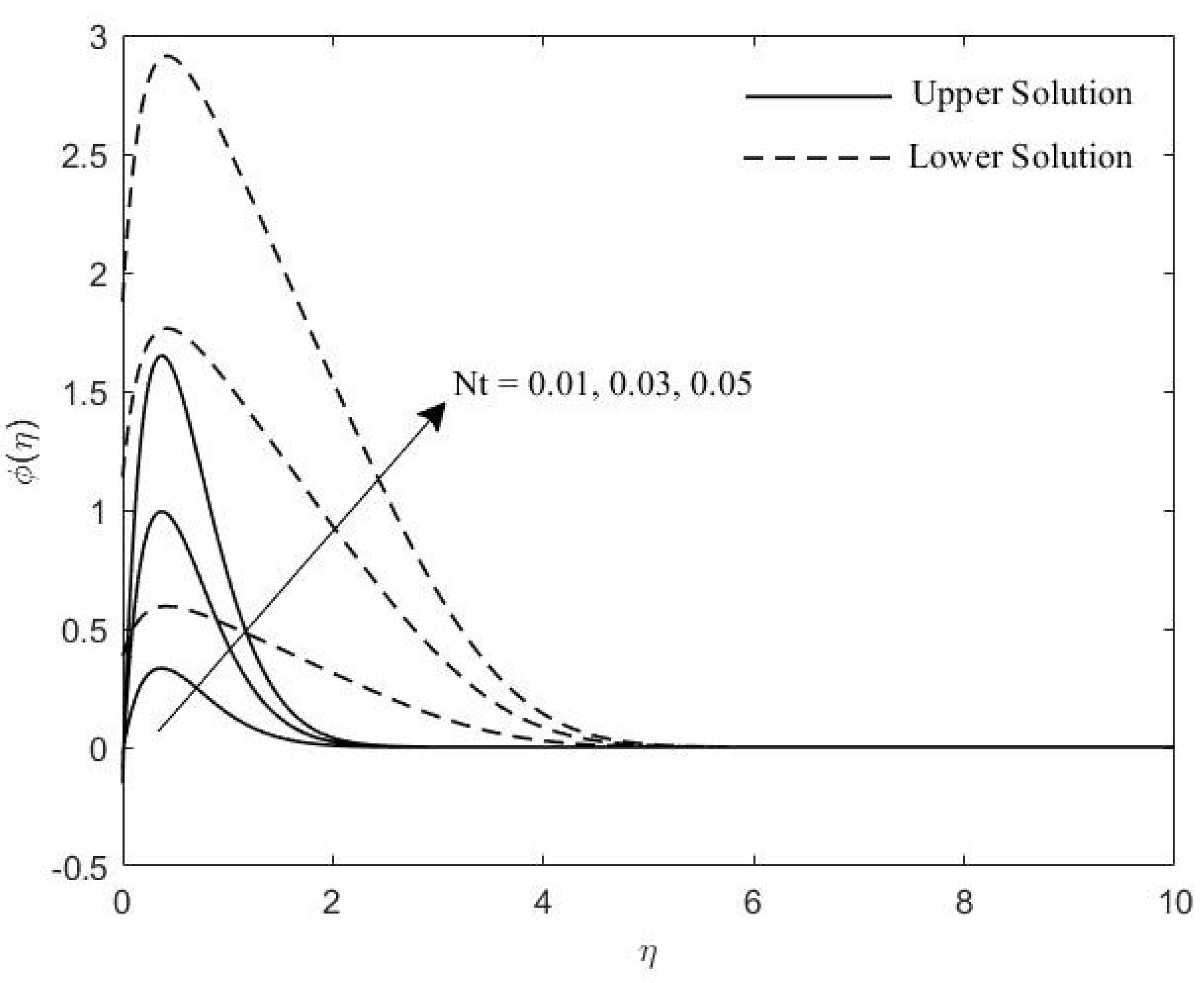
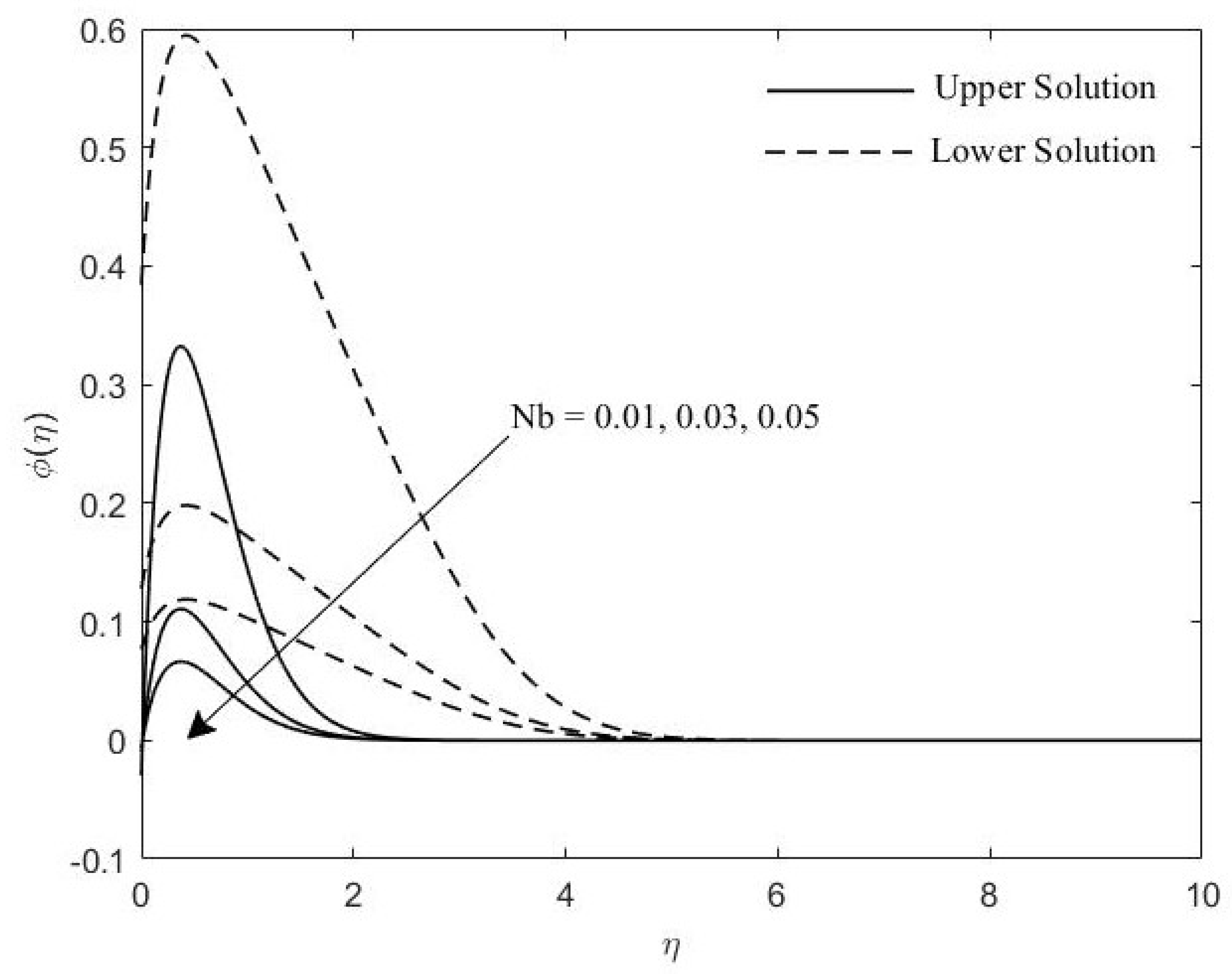


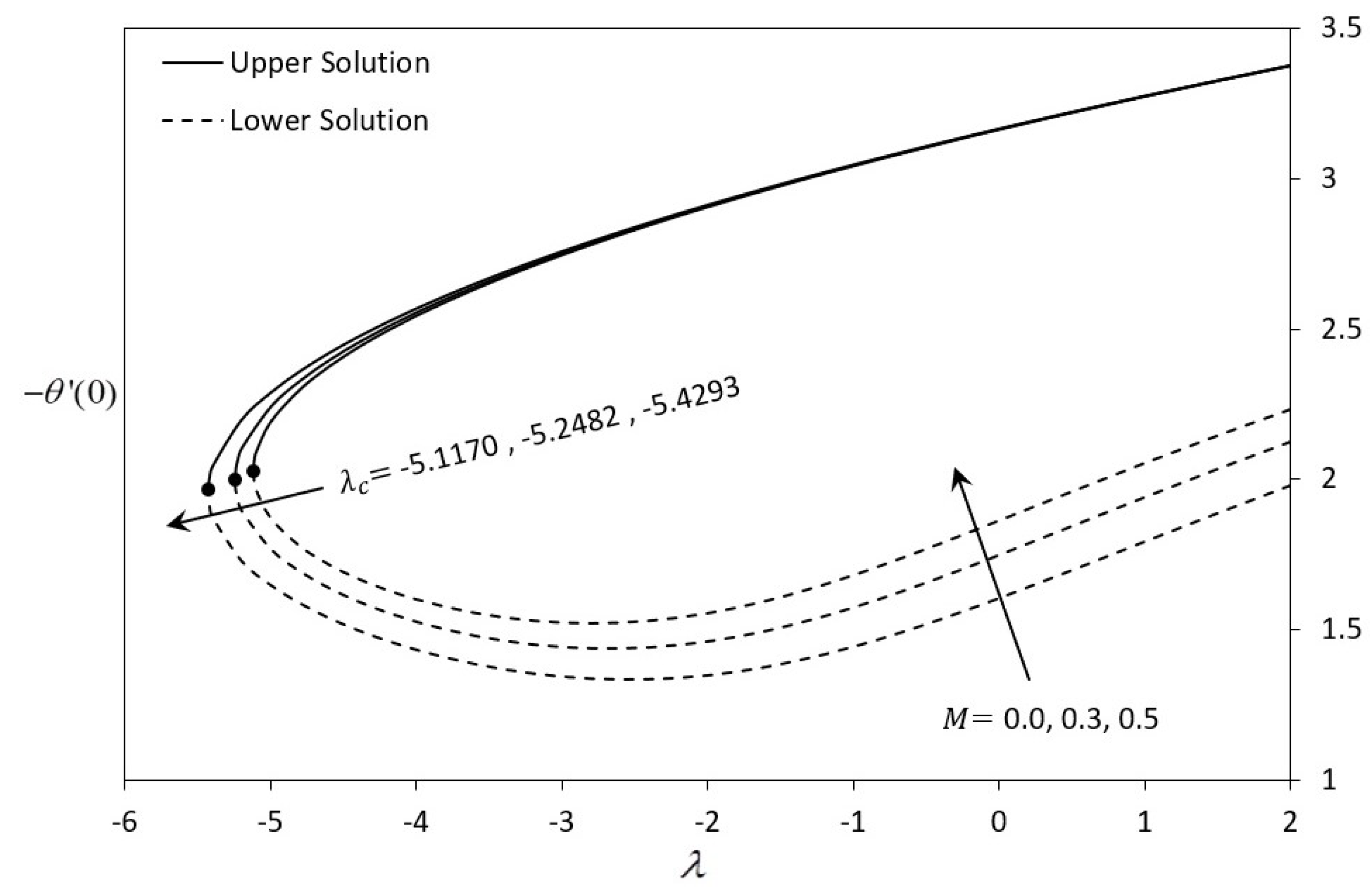
| Present Result | Aman et al. [55] | Bhattacharyya et al. [56] | ||||
|---|---|---|---|---|---|---|
| Upper Branch | Lower Branch | Upper Branch | Lower Branch | Upper Branch | Lower Branch | |
| −0.25 | 1.40224 | 1.4022 | 1.40224051 | |||
| −0.3 | 1.42758 | 1.4276 | ||||
| −0.4 | 1.46861 | 1.4686 | ||||
| −0.5 | 1.49567 | 1.4957 | 1.49566948 | |||
| −0.615 | 1.50724 | 1.5072 | 1.50724089 | |||
| −0.75 | 1.48929 | 1.4893 | 1.48929834 | |||
| −1.0 | 1.32881 | 0 | 1.3288 | 0 | 1.32881689 | 0 |
| −1.15 | 1.08223 | 0.11670 | 1.0822 | 0.1167 | 1.08223164 | 0.11667340 |
| −1.18 | 1.00045 | 0.17836 | 1.0004 | 0.1784 | ||
| −1.2465 | 0.55430 | 0.55430 | 0.5543 | 0.5543 | 0.55428565 | 0.55428565 |
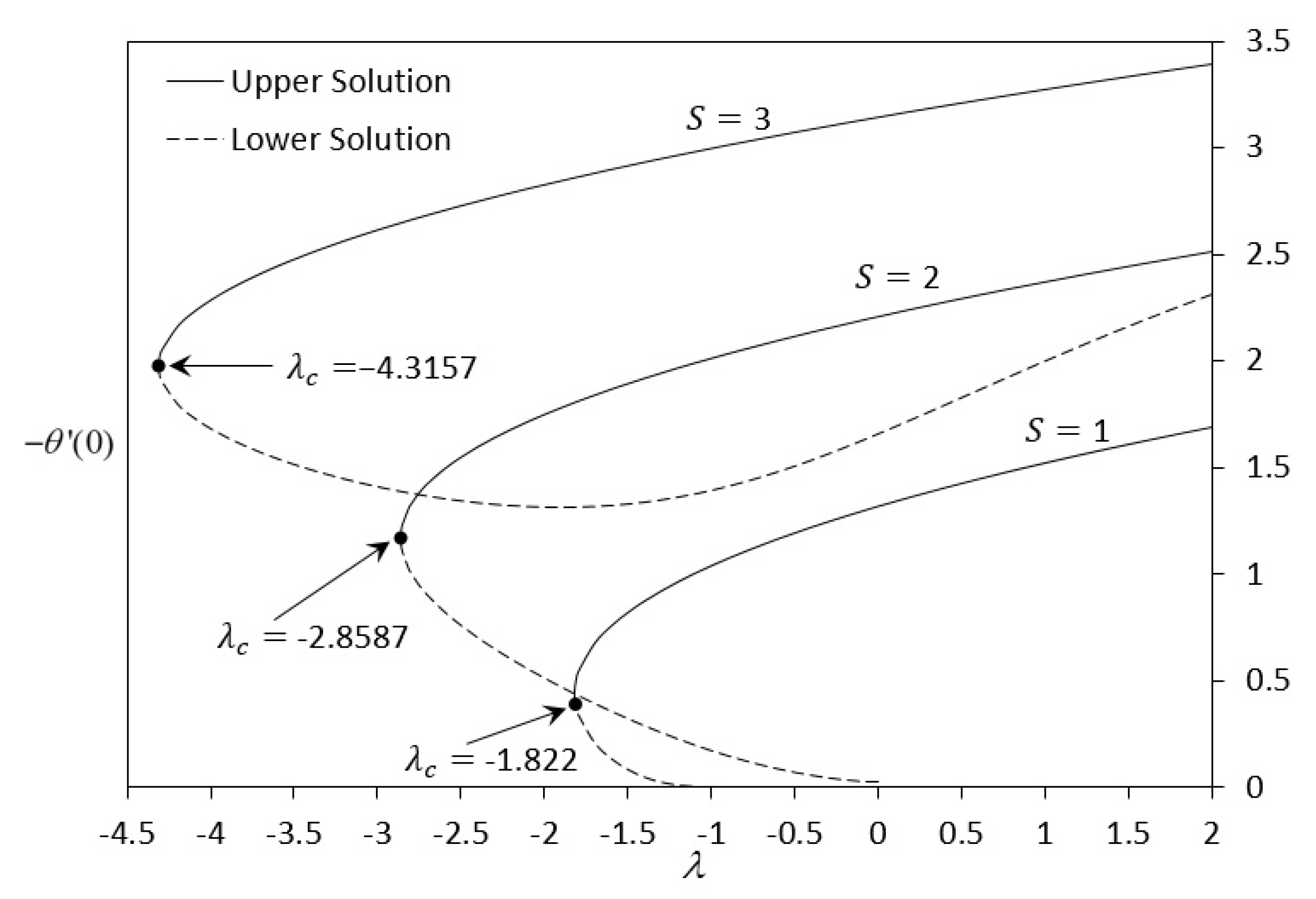
| S | First Solution | Second Solution | |
|---|---|---|---|
| 1 | −1.8 | 0.40484 | −0.39610 |
| −1.82 | 0.12550 | −0.12458 | |
| −1.822 | 0.03052 | −0.03049 | |
| 2 | −2.8 | 0.73421 | −0.71159 |
| −2.85 | 0.28333 | −0.27970 | |
| −2.858 | 0.08134 | −0.08104 | |
| 3 | −4.3 | 0.42854 | −0.42058 |
| −4.31 | 0.25712 | −0.25423 | |
| −4.315 | 0.08807 | −0.08773 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Junoh, M.M.; Md Ali, F.; Pop, I. Magnetohydrodynamics Stagnation-Point Flow of a Nanofluid Past a Stretching/Shrinking Sheet with Induced Magnetic Field: A Revised Model. Symmetry 2019, 11, 1078. https://doi.org/10.3390/sym11091078
Junoh MM, Md Ali F, Pop I. Magnetohydrodynamics Stagnation-Point Flow of a Nanofluid Past a Stretching/Shrinking Sheet with Induced Magnetic Field: A Revised Model. Symmetry. 2019; 11(9):1078. https://doi.org/10.3390/sym11091078
Chicago/Turabian StyleJunoh, Mohamad Mustaqim, Fadzilah Md Ali, and Ioan Pop. 2019. "Magnetohydrodynamics Stagnation-Point Flow of a Nanofluid Past a Stretching/Shrinking Sheet with Induced Magnetic Field: A Revised Model" Symmetry 11, no. 9: 1078. https://doi.org/10.3390/sym11091078
APA StyleJunoh, M. M., Md Ali, F., & Pop, I. (2019). Magnetohydrodynamics Stagnation-Point Flow of a Nanofluid Past a Stretching/Shrinking Sheet with Induced Magnetic Field: A Revised Model. Symmetry, 11(9), 1078. https://doi.org/10.3390/sym11091078





