London Penetration Depth as a Test of Order Parameter Symmetry in Sodium Cobaltate Superconductors
Abstract
1. Introduction
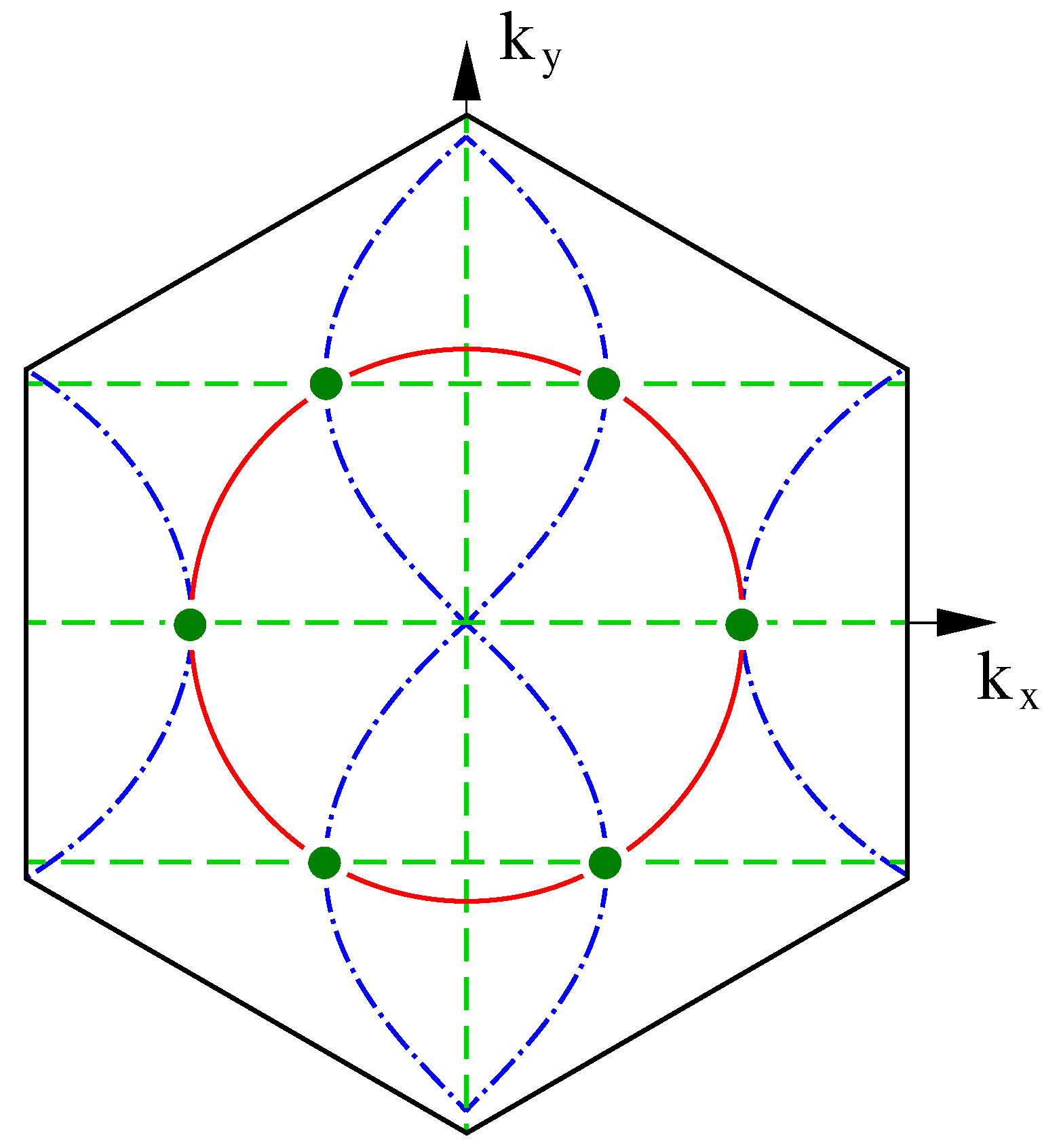
2. Model
3. Equation for the London Penetration Depth
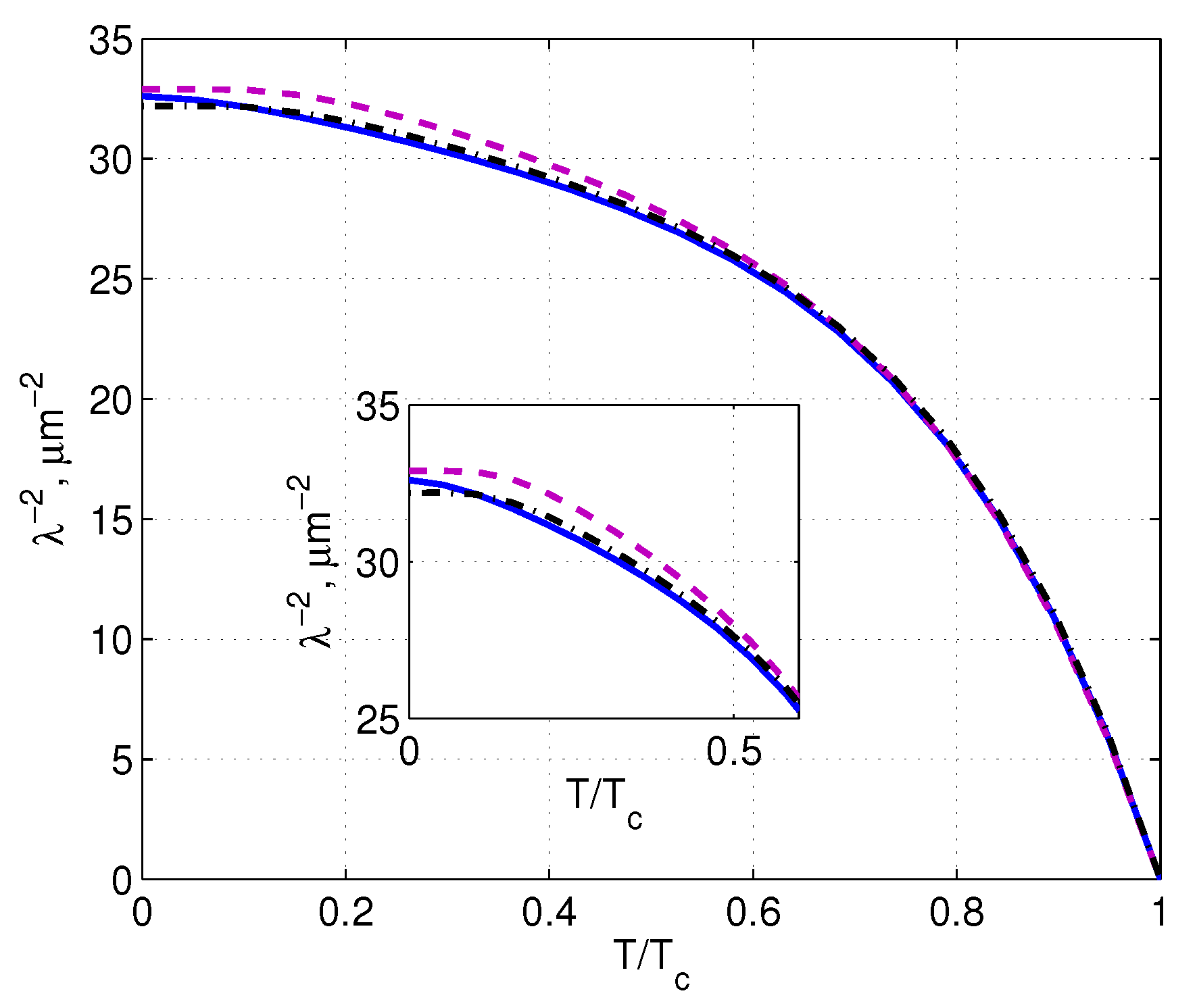
4. Temperature Dependence of the London Penetration Depth for the Chiral Order Parameter
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| HTSC | High Temperature Super Conductor |
| ARPES | Angle-Resolved Photo Emission Spectroscopy |
| LDA | Local Density Approximation |
| DMFT | Dynamical Mean-Field Theory |
| FRG | Functional Renormalization Group |
Appendix A
Appendix B
References
- Ivanova, N.N.; Ovchinnikov, S.G.; Korshunov, M.M.; Eremin, I.M.; Kazak, N.V. Specific features of spin, charge, and orbital ordering in cobaltites. Phys. Usp. 2009, 52, 789–810. [Google Scholar] [CrossRef]
- Sakurai, H.; Ihara, Y.; Takada, K. Superconductivity of cobalt oxide hydrate Nax(H3O)zCoO2·yH2O. Phys. C Supercoductivity Appl. 2015, 514, 378–387. [Google Scholar] [CrossRef]
- Takada, K.; Sakurai, H.; Takayama-Muromachi, E.; Izumi, F.; Dilanian, R.; Sasaki, N. Superconductivity in two-dimensional CoO2 layers. Nature 2003, 422, 53–55. [Google Scholar] [CrossRef]
- Schaak, R.E.; Klimczuk, T.; Foo, M.L.; Cava, R.J. Superconductivity phase diagram of NaxCoO2·1.3H2O. Nature 2003, 42, 527–529. [Google Scholar] [CrossRef] [PubMed]
- Jin, R.; Sales, B.C.; Khalifah, P.; Mandrus, D. Observation of bulk superconductivity in NaxCoO2·yH2O and NaxCoO2·yD2O powder and single crystals. Phys. Rev. Lett. 2003, 91, 217001. [Google Scholar] [CrossRef]
- Ishida, K.; Ihara, Y.; Kitagawa, K.; Murakawa, H.; Maeno, Y.; Michioka, C.; Kato, M.; Yoshimura, K.; Takada, K.; Sasaki, T.; et al. Unconventional Superconductivity and Nearly Ferromagnetic Spin Fluctuations in NaxCoO2·yH2O. J. Phys. Soc. Jpn. 2003, 72, 3041. [Google Scholar] [CrossRef]
- Fujimoto, T.; Zheng, G.; Kitaoka, Y.; Meng, R.L.; Cmaidalka, J.; Chu, C.W. Unconventional Superconductivity and Electron Correlations in the Cobalt Oxyhydrate Na0.35CoO2·yH2O. Phys. Rev. Lett. 2004, 92, 047004. [Google Scholar] [CrossRef] [PubMed]
- Zheng, G.-Q.; Matano, K.; Meng, R.L.; Cmaidalka, J.; Chu, C.W. Na content dependence of superconductivity and the spin correlations in NaxCoO2·1.3H2O. J. Phys. Condens. Matter 2006, 18, L63. [Google Scholar] [CrossRef]
- Ihara, Y.; Takeya, H.; Ishida, K.; Ikeda, H.; Michioka, C.; Yoshimura, K.; Takada, K.; Sasaki, T.; Sakurai, H.; Takayama-Muromachi, E. Unconventional Superconductivity Induced by Quantum Critical Fluctuations in Hydrate Cobaltate Nax(H3O)zCoO2·yH2O—Relationship between Magnetic Fluctuations and Superconductivity Revealed by Co Nuclear Quadrupole Resonance. J. Phys. Soc. Jpn. 2006, 75, 124714. [Google Scholar] [CrossRef]
- Michioka, C.; Ohta, H.; Itoh, Y.; Yoshimura, K. 59Co Nuclear Quadrupole Resonance Studies of Superconducting and Nonsuperconducting Bilayer Water Intercalated Sodium Cobalt Oxides NaxCoO2·yH2O. J. Phys. Soc. Jpn. 2006, 75, 063701. [Google Scholar] [CrossRef]
- Lorenz, B.; Cmaidalka, J.; Meng, R.L.; Chu, C.W. Thermodynamic properties in the normal and superconducting states of NaxCoO2·yH2O powder measured by heat capacity experiments. Physica C 2004, 402, 106–113. [Google Scholar] [CrossRef][Green Version]
- Yang, H.D.; Lin, J.-Y.; Sun, C.P.; Kang, Y.C.; Huang, C.L.; Takada, K.; Sasaki, T.; Sakurai, H.; Takayama-Muromachi, E. Evidence of nodal superconductivity in Na0.35CoO2·1.3H2O: A specific-heat study. Phys. Rev. B 2005, 71, 020504. [Google Scholar] [CrossRef]
- Jin, R.; Sales, B.C.; Li, S.; Mandrus, D. Dependence of the specific heat of NaxCoO2·yH2O/D2O on sodium and water concentrations. Phys. Rev. B 2005, 72, 060512R. [Google Scholar] [CrossRef]
- Zheng, G.-Q.; Matano, K.; Chen, D.P.; Lin, C.T. Spin singlet pairing in the superconducting state of NaxCoO2·1.3H2O: Evidence from a 59Co Knight shift in a single crystal. Phys. Rev. B 2006, 73, 180503. [Google Scholar] [CrossRef]
- Kobayashi, Y.; Moyoshi, T.; Watanabe, H.; Yokoi, M.; Sato, M. Magnetic Properties of Superconducting Cobalt Oxides NaxCoO2·yH2O. J. Phys. Soc. Jpn. 2006, 75, 074717. [Google Scholar] [CrossRef]
- Ihara, Y.; Ishida, K.; Ohta, H.; Yoshimura, K. Co-NMR Measurements on Crystalline Sample of the Bilayere Hydrate Nax(H3O)zCoO2·yH2O. J. Phys. Soc. Jpn. 2008, 77, 073702. [Google Scholar] [CrossRef]
- Onoda, M.; Takao, K.; Ikeda, T. Spin-singlet superconductivity and antiferromagnetic correlations for the field-aligned powder of the triangular lattice NaxCoO2·yH2O. Physica C 2009, 469, 162–167. [Google Scholar] [CrossRef]
- Zhou, S.; Wang, Z. Nodal d + id pairing and topological phases on the triangular lattice of NaxCoO2·yH2O: evidence for an unconventional superconducting state. Phys. Rev. Lett. 2008, 100, 217002. [Google Scholar] [CrossRef] [PubMed]
- Valkov, V.V.; Valkova, T.A.; Mitskan, V.A. Superconducting Phase with the (d + id) Order Parameter in an Ensemble of Hubbard Fermions on the Triangular Lattice. JETP Lett. 2015, 102, 399. [Google Scholar] [CrossRef]
- Korshunov, M.M.; Eremin, I.; Shorikov, A.; Anisimov, V.I.; Renner, M.; Brenig, W. Itinerant in-plane magnetic fluctuations and many-body correlations in NaxCoO2. Phys. Rev. B 2007, 75, 094511. [Google Scholar] [CrossRef]
- Bourgeois, A.; Aligia, A.A.; Rozenberg, M.J. Dynamical Mean Field Theory of an Effective Three-Band Model for NaxCoO2. Phys. Rev. Lett. 2009, 102, 066402. [Google Scholar] [CrossRef]
- Kiesel, M.L.; Platt, C.; Hanke, W.; Thomale, R. Model Evidence of an Anisotropic Chiral d+id-Wave Pairing State for the Water-Intercalated NaxCoO2·yH2O Superconductor. Phys. Rev. Lett. 2013, 111, 097001. [Google Scholar] [CrossRef]
- Lee, K.; Kunes, J.; Pickett, W.E. Charge disproportionation and spin ordering tendencies in NaxCoO2. Phys. Rev. B 2004, 70, 045104. [Google Scholar] [CrossRef]
- Johannes, M.D.; Mazin, I.I.; Singh, D.J.; Papaconstantopoulos, D.A. Erratum: Nesting, Spin Fluctuations, and Odd-Gap Superconductivity in NaxCoO2·yH2O. Phys. Rev. Lett. 2005, 94, 169901. [Google Scholar] [CrossRef]
- Qian, D.; Wray, L.; Hsieh, D.; Viciu, L.; Cava, R.J.; Luo, J.L.; Wu, D.; Wang, N.L.; Hasan, M.Z. Complete d-Band Dispersion Relation in Sodium Cobaltates. Phys. Rev. Lett. 2006, 97, 186405. [Google Scholar] [CrossRef]
- Shimojima, T.; Ishizaka, K.; Tsuda, S.; Kiss, T.; Yokoya, T.; Chainani, A.; Shin, S.; Badica, P.; Yamada, K.; Togano, K. Angle-resolved photoemission study of the cobalt oxide superconductor NaxCoO2·yH2O: observation of the Fermi surface. Phys. Rev. Lett. 2006, 97, 267003. [Google Scholar] [CrossRef]
- Geck, J.; Borisenko, S.V.; Berger, H.; Eschrig, H.; Fink, J.; Knupfer, M.; Koepernik, K.; Koitzsch, A.; Kordyuk, A.A.; Zabolotnyy, V.B.; et al. Anomalous Quasiparticle Renormalization in Na0.73CoO2: Role of Interorbital Interactions and Magnetic Correlations. Phys. Rev. Lett. 2007, 99, 046403. [Google Scholar] [CrossRef]
- Korshunov, M.M.; Eremin, I. Dynamical magnetic susceptibility in the lamellar cobaltate superconductor NaxCoO2·yH2O. Phys. Rev. B 2008, 77, 064510. [Google Scholar] [CrossRef]
- Hanisch, T.; Uhrig, G.S.; Muller-Hartmann, E. Lattice dependence of saturated ferromagnetism in the Hubbard model. Phys. Rev. B 1997, 56, 13960. [Google Scholar] [CrossRef]
- Bardeen, J.; Cooper, L.N.; Schrieffer, J.R. Theory of Superconductivity. Phys. Rev. 1957, 108, 1175. [Google Scholar] [CrossRef]
- Schrieffer, J.R. Theory of Superconductivity; Benjamin: New York, NY, USA, 1964. [Google Scholar]
- Tinkham, M. Introduction to Superconductivity; McGraw-Hill: New York, NY, USA, 1996. [Google Scholar]
- London, F. Superfluids; Dover Publications: New York, NY, USA, 1961. [Google Scholar]
- Misawa, S. London penetration depth in a tight-binding model of layered narrow-band anisotropic superconductors. Phys. Rev. B 1995, 51, 11791. [Google Scholar] [CrossRef]
- Benfatto, L.; Caprara, S.; Castro, C.D. Gap and pseudogap evolution within the charge-ordering scenario for superconducting cuprates. Eur. Phys. J. B 2000, 17, 95. [Google Scholar] [CrossRef]
- Sheehy, D.E.; Davis, T.P.; Franz, M. Unified theory of the ab-plane and c-axis penetration depths of underdoped cuprates. Phys. Rev. B 2004, 70, 054510. [Google Scholar] [CrossRef]
- Eremin, M.V.; Larionov, I.A.; Lyubin, I.E. London penetration depth in the tight binding approximation: Orthorhombic distortion and oxygen isotope effects in cuprates. J. Phys. Condens. Matter 2010, 22, 185704. [Google Scholar] [CrossRef][Green Version]
- Sigrist, M.; Ueda, K. Phenomenological theory of unconventional superconductivity. Rev. Mod. Phys. 1991, 63, 239. [Google Scholar] [CrossRef]
- Hardy, W.N.; Bonn, D.A.; Morgan, D.C.; Liang, R.; Zhang, K. Precision measurements of the temperature dependence of λ in YBa2Cu3O6.95: Strong evidence for nodes in the gap function. Phys. Rev. Lett. 1993, 70, 3999. [Google Scholar] [CrossRef]
- Kanegel, A.; Keren, A.; Patlagan, L.; Chashka, K.B.; King, P.; Amato, A. Muon Spin Relaxation Measurements of NaxCoO2·yH2O. Phys. Rev. Lett. 2004, 92, 257007. [Google Scholar] [CrossRef]
- Higemoto, W.; Oshishi, K.; Koda, A.; Kadono, R.; Ishida, K.; Takada, K.; Sakurai, H.; Takayama-Muromachi, E.; Sasaki, T. Possible unconventional superconductivity in NaxCoO2·yH2O probed by muon spin rotation and relaxation. Phys. Rev. B 2004, 70, 134508. [Google Scholar] [CrossRef]
- Feynman, R.; Leighton, R.; Sands, M. The Feynman Lectures on Physics; Addison-Wesley: London, UK, 1964. [Google Scholar]
- Mineev, V.P.; Samohin, K.V. Introduction to Unconventional Superconductivity; MIPT publishing: Moscow, Russia, 1998. [Google Scholar]
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dzebisashvili, D.M.; Klyuchantsev, A.B. London Penetration Depth as a Test of Order Parameter Symmetry in Sodium Cobaltate Superconductors. Symmetry 2019, 11, 633. https://doi.org/10.3390/sym11050633
Dzebisashvili DM, Klyuchantsev AB. London Penetration Depth as a Test of Order Parameter Symmetry in Sodium Cobaltate Superconductors. Symmetry. 2019; 11(5):633. https://doi.org/10.3390/sym11050633
Chicago/Turabian StyleDzebisashvili, Dmitry M., and Andrey B. Klyuchantsev. 2019. "London Penetration Depth as a Test of Order Parameter Symmetry in Sodium Cobaltate Superconductors" Symmetry 11, no. 5: 633. https://doi.org/10.3390/sym11050633
APA StyleDzebisashvili, D. M., & Klyuchantsev, A. B. (2019). London Penetration Depth as a Test of Order Parameter Symmetry in Sodium Cobaltate Superconductors. Symmetry, 11(5), 633. https://doi.org/10.3390/sym11050633




