Multipurpose Quantum Simulator Based on a Hybrid Solid-State Quantum Device
Abstract
1. Introduction
2. Basic Theory
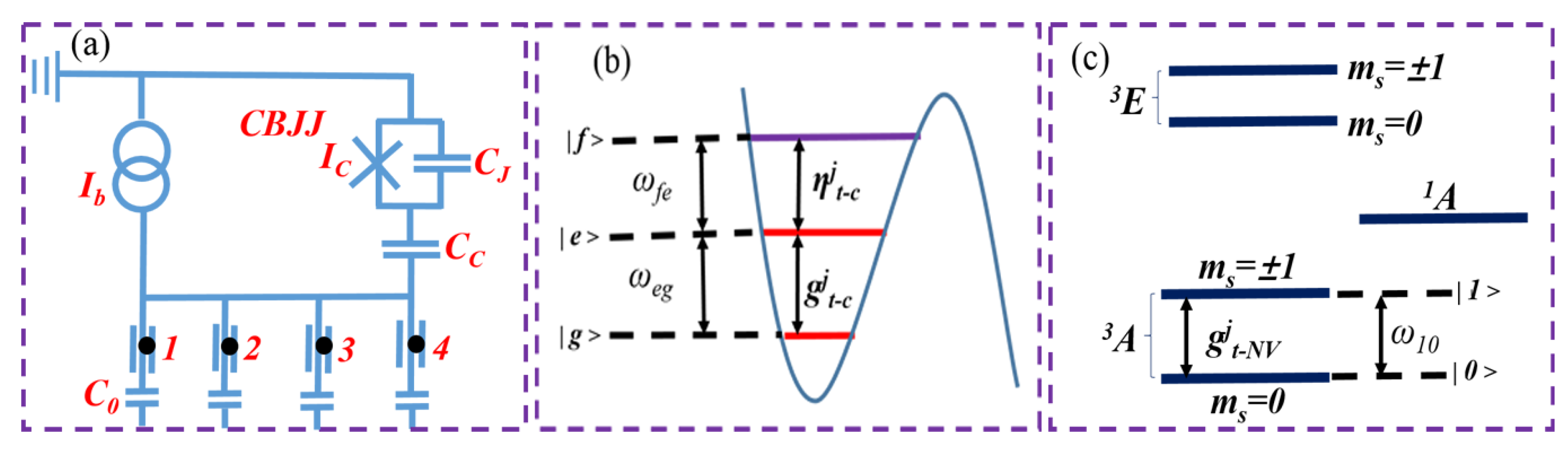
2.1. CBJJ–TLR Off-Resonant Interaction
2.2. N-V Center–TLR Resonant Interaction
2.3. CBJJ–TLR–N-V Center Off-Resonant Interaction
3. Implementation of a Multipurpose Quantum Simulator
4. Implementation of Quantum Anticloning
5. Discussion
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Wootters, W.K.; Zurek, W.H. A single quantum cannot be cloned. Nature 1982, 299, 802. [Google Scholar] [CrossRef]
- Buzek, V.; Hillery, M. Quantum copying: Beyond the no-cloning theorem. Phys. Rev. A 1996, 54, 1844. [Google Scholar] [CrossRef]
- Duan, L.M.; Guo, G.C. A probabilistic cloning machine for replicating two non-orthogonal states. Phys. Lett. A 1998, 243, 261–264. [Google Scholar] [CrossRef]
- Milman, P.; Ollivier, H.; Raimond, J.M. Universal quantum cloning in cavity QED. Phys. Rev. A 2003, 67, 57–63. [Google Scholar] [CrossRef]
- Zou, X.B.; Pahlke, K.; Mathis, W. Scheme for the implementation of a universal quantum cloning machine via cavity-assisted atomic collisions in cavity QED. Phys. Rev. A 2003, 67, 024304. [Google Scholar] [CrossRef]
- Ye, L.; Guo, G.C. Scheme for implementing quantum dense coding in cavity QED. Phys. Rev. A 2005, 71, 034304. [Google Scholar] [CrossRef]
- Fang, B.L.; Wu, T.; Ye, L. Realization of a general quantum cloning machine via cavity-assisted interaction. Europhys. Lett. 2012, 97, 60002. [Google Scholar] [CrossRef]
- Rui, P.S.; Zhang, W.; Liao, Y.L.; Zhang, Z.Y. Probabilistic quantum cloning of a subset of linearly dependent states. Eur. Phys. J. D 2018, 72, 26. [Google Scholar] [CrossRef]
- Bruß, D.; DiVincenzo, D.P.; Ekert, A.; Fuchs, C.A.; Macchiavello, C.; Smolin, J.A. Optimal universal and state-dependent quantum cloning. Phys. Rev. A 1998, 57, 2368. [Google Scholar] [CrossRef]
- Pan, X.Y.; Liu, G.Q.; Yang, L.L.; Fan, H. Solid-state optimal phase-covariant quantum cloning machine. Appl. Phys. Lett. 2011, 99, 051113. [Google Scholar] [CrossRef]
- Bruss, D.; Cinchetti, M.; D’Ariano, G.M.; Macchiavello, C. Phase-covariant quantum cloning. Phys. Rev. A 2000, 62, 012302. [Google Scholar] [CrossRef]
- Knoll, L.T.; Grande, I.H.; Larotonda, M.A. Photonic quantum simulator for unbiased phase covariant cloning. Appl. Phys. B 2018, 124, 3. [Google Scholar] [CrossRef]
- Aguilar, J.C.; Misawa, M.; Matsuda, K.; Rehman, S.; Yasumoto, M.; Suzuki, Y.; Takeuchi, A.; Berriel-Valdos, L.R. Starlet transform applied to digital Gabor holographic microscopy. Appl. Opt. 2016, 24, 6617–6624. [Google Scholar] [CrossRef]
- Meng, F.X.; Yu, X.T.; Zhang, Z.C. An economical state-dependent telecloning for a multiparticle GHZ state. Quantum Inf. Process 2018, 17, 66. [Google Scholar] [CrossRef]
- Wu, H.J.; Zhao, J.; Zhu, A.D. Protection of Telecloning Over Noisy Channels with Environment-Assisted Measurements and Weak Measurements. Int. J. Theor. Phys. 2018, 57, 1235. [Google Scholar] [CrossRef]
- Ma, P.C.; Chen, G.B.; Li, X.W.; Zhan, Y.B. Quantum jointly assisted cloning of an unknown three-dimensional equatorial state. Laser Phys. 2018, 28, 025201. [Google Scholar] [CrossRef]
- Bouchard, F.; Fickler, R.; Boyd, R.; Karimi, E. High-dimensional quantum cloning and applications to quantum hacking. Sci. Adv. 2017, 3, e1601915. [Google Scholar] [CrossRef]
- Bartkiewicz, K.; Ćernoch, A.; Chimczak, G.; Lemr, K.; Miranowicz, A.; Nori, F. Experimental quantum forgery of quantum optical money. npj Quantum Inf. 2017, 7, 1. [Google Scholar] [CrossRef]
- Hu, Y.; Xiao, Y.F.; Zhou, Z.W.; Guo, G.C. Controllable coupling of superconducting transmission-line resonators. Phys. Rev. A 2007, 75, 012314. [Google Scholar] [CrossRef]
- Clarke, J.; Cleland, A.N.; Devoret, M.H.; Esteve, D.; Martinis, J.M. Quantum mechanics of a macroscopic variable: the phase difference of a josephson junction. Science 1988, 239, 992. [Google Scholar] [CrossRef]
- You, J.Q.; Nori, F. Superconducting Circuits and Quantum Information. Phys. Today 2005, 58, 42–47. [Google Scholar] [CrossRef]
- Neeley, M.; Ansmann, M.; Bialczak, R.C.; Hofheinz, M.; Katz, N.; Lucero, E.; O’Connell, A.; Wang, H.; Cleland, A.N.; Martinis, J.M. Process tomography of quantum memory in a Josephson-phase qubit coupled to a two-level state. Nat. Phys. 2008, 4, 523. [Google Scholar] [CrossRef]
- Zagoskin, A.M.; Ashhab, S.; Johansson, J.R.; Nori, F. Quantum two-level systems in Josephson junctions as naturally formed qubits. Phys. Rev. Lett. 2006, 97, 077001. [Google Scholar] [CrossRef]
- Xiong, S.J.; Zhang, Y.; Sun, Z.; Yu, L.; Su, Q.; Xu, X.Q.; Jin, J.S.; Xu, Q.J.; Chen, K.F.; Yang, C.P. Experimental simulation of a quantum channel without the rotating-wave approximation: Testing quantum temporal steering. Optica 2017, 4, 1065–1072. [Google Scholar] [CrossRef]
- Yang, C.P.; Zheng, S.B.; Nori, F. Multiqubit tunable phase gate of one qubit simultaneously controlling n qubits in a cavity. Phys. Rev. A 2010, 82, 062326. [Google Scholar] [CrossRef]
- Liu, Y.; You, J.Q.; Wei, L.F.; Sun, C.P.; Nori, F. Optical selection rules and phase-dependent adiabatic state control in a superconducting quantum circuit. Phys. Rev. Lett. 2005, 95, 087001. [Google Scholar] [CrossRef]
- Guo, G.C.; Zheng, S.B. Generation of Schrödinger cat states via the Jaynes-Cummings model with large detuning. Phys. Lett. A 1996, 223, 332. [Google Scholar] [CrossRef]
- Brune, M.; Hagley, E.; Dreyer, J.; Maitre, X.; Maali, A.; Wunderlich, C.; Raimond, J.M.; Haroche, S. Observing the progressive decoherence of the “meter” in a quantum measurement. Phys. Rev. Lett. 1996, 77, 4887. [Google Scholar] [CrossRef]
- Sandberg, M.; Wilson, C.M.; Persson, F.; Bauch, T.; Johansson, G.; Shumeiko, V.; Duty, T.; Delsing, P. Tuning the field in a microwave resonator faster than the photon lifetime. Appl. Phys. Lett. 2008, 92, 203501. [Google Scholar] [CrossRef]
- Palacios-Laloy, A.; Nguyen, F.; Mallet, F.; Bertet, P.; Vion, D.; Esteve, D. Tunable resonators for quantum circuits. J. Low Temp. Phys. 2008, 151, 1034–1042. [Google Scholar] [CrossRef]
- Johansson, J.R.; Johansson, G.; Wilson, C.M.; Nori, F. Dynamical Casimir effect in a superconducting coplanar waveguide. Phys. Rev. Lett. 2009, 103, 147003. [Google Scholar] [CrossRef]
- Liao, J.Q.; Gong, Z.R.; Zhou, L.; Liu, Y.X.; Sun, C.P.; Nori, F. Controlling the transport of single photons by tuning the frequency of either one or two cavities in an array of coupled cavities. Phys. Rev. A 2010, 81, 042304. [Google Scholar] [CrossRef]
- Clarke, J.; Wilhelm, F.K. Superconducting quantum bits. Nature 2008, 453, 1031. [Google Scholar] [CrossRef]
- Li, Y.; Bruder, C.; Sun, C.P. Quantum nondemolition measurements of photon number by atomic beam deflection. Phys. Rev. Lett. 2007, 75, 032302. [Google Scholar] [CrossRef]
- Zhu, M.Z.; Ye, L. Implementing a quantum cloning machine in separate cavities via the optical coherent pulse as a quantum communication bus. Phys. Rev. A 2015, 91, 127901. [Google Scholar] [CrossRef]
- Yang, C.P. Preparation of n-qubit Greenberger-Horne-Zeilinger entangled states in cavity QED: An approach with tolerance to nonidentical qubit-cavity coupling constants. Phys. Rev. A 2011, 83, 062302. [Google Scholar] [CrossRef]
- Cerf, N.J. Asymmetric quantum cloning machines. Opt. Acta Int. J. Opt. 2004, 47, 187–209. [Google Scholar] [CrossRef]
- Olaya-Castro, A.; Johnson, N.F.; Quiroga, L. Robust one-step catalytic machine for high fidelity anticloning and W-state generation in a multiqubit system. Phys. Rev. Lett. 2005, 94, 110502. [Google Scholar] [CrossRef]
- Gisin, N.; Popescu, S. Spin flips and quantum information for antiparallel spins. Phys. Rev. Lett. 1999, 83, 432. [Google Scholar] [CrossRef]
- DiCarlo, L.; Reed, M.D.; Sun, L.; Johnson, B.R.; Chow, J.M.; Gambetta, J.M.; Frunzio, L.; Girvin, S.M.; Devoret, M.H.; Schoelkopf, R.J. Preparation and measurement of three-qubit entanglement in a superconducting circuit. Nature 2010, 467, 574. [Google Scholar] [CrossRef]
- Yang, W.L.; Yin, Z.Q.; Hu, Y.; Feng, M.; Du, J.F. High-fidelity quantum memory using nitrogen-vacancy center ensemble for hybrid quantum computation. Phys. Rev. A 2011, 84, 3442–3448. [Google Scholar] [CrossRef]
- Manson, N.B.; Harrison, J.P.; Sellars, M.J. Nitrogen-vacancy center in diamond: Model of the electronic structure and associated dynamics. Phys. Rev. B 2006, 74, 104303. [Google Scholar] [CrossRef]
- Gao, M.; Wu, C.W.; Deng, Z.J.; Zou, W.J.; Zhou, L.G.; Li, C.Z.; Wang, X.B. Controllable strong coupling between individual spin qubits and a transmission line resonator via nanomechanical resonators. Phys. Lett. A 2012, 376, 595. [Google Scholar] [CrossRef]
- Jin, P.Q.; Marthaler, M.; Shnirman, A.; Schön, G. Strong coupling of spin qubits to a transmission line resonator. Phys. Rev. Lett. 2012, 108, 190506. [Google Scholar] [CrossRef]
- Yu, Y. Coherent temporal oscillations of macroscopic quantum states in a Josephson junction. Science 2002, 296, 889–892. [Google Scholar] [CrossRef]
- Zhao, Y.J.; Fang, X.M.; Zhou, F.; Song, K.H. A scheme for realizing quantum information storage and retrieval from quantum memory based on nitrogenvacancy centers. Phys. Rev. A 2012, 86, 052325. [Google Scholar] [CrossRef]
- Neumman, P.; Mizuochi, N.; Rempp, F.; Hemmer, P.; Watanabe, H.; Yamasaki, S.; Jacques, V.; Gaebel, T.; Jelezko, F.; Wrachtrup, J. Multipartite entanglement among single spins in diamond. Science 2008, 320, 1326–1329. [Google Scholar] [CrossRef]
- Harrison, J.; Sellars, M.J.; Manson, N.B. Measurement of the optically induced spin polarisation of NV centres in diamond. Diam. Relat. Mater. 2006, 15, 1326–1329. [Google Scholar] [CrossRef]
- Balasubramanian, G.; Neumann, P.; Twitchen, D.; Markham, M.; Kolesov, R.; Mizuochi, N.; Isoya, J.; Achard, J.; Beck, J.; Tissler, J.; et al. Ultralong spin coherence time in isotopically engineered diamond. Nat. Mater. 2009, 8, 383–387. [Google Scholar] [CrossRef]

© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, T.; Zhou, F.; Chen, S.; Xiang, S.; Song, K.; Zhao, Y. Multipurpose Quantum Simulator Based on a Hybrid Solid-State Quantum Device. Symmetry 2019, 11, 467. https://doi.org/10.3390/sym11040467
Yuan T, Zhou F, Chen S, Xiang S, Song K, Zhao Y. Multipurpose Quantum Simulator Based on a Hybrid Solid-State Quantum Device. Symmetry. 2019; 11(4):467. https://doi.org/10.3390/sym11040467
Chicago/Turabian StyleYuan, Tingting, Fang Zhou, Shengping Chen, Shaohua Xiang, Kehui Song, and Yujing Zhao. 2019. "Multipurpose Quantum Simulator Based on a Hybrid Solid-State Quantum Device" Symmetry 11, no. 4: 467. https://doi.org/10.3390/sym11040467
APA StyleYuan, T., Zhou, F., Chen, S., Xiang, S., Song, K., & Zhao, Y. (2019). Multipurpose Quantum Simulator Based on a Hybrid Solid-State Quantum Device. Symmetry, 11(4), 467. https://doi.org/10.3390/sym11040467




