Isoperimetric Numbers of Randomly Perturbed Intersection Graphs

Abstract

Social networks describe social interactions between people, which are often modeled by intersection graphs. In this paper, we propose an intersection graph model that is induced by adding a sparse random bipartite graph to a given bipartite graph. Under some mild conditions, we show that the vertex–isoperimetric number and the edge–isoperimetric number of the randomly perturbed intersection graph on n vertices are $\mathsf{\Omega }(1/lnn)$ asymptomatically almost surely. Numerical simulations for small graphs extracted from two real-world social networks, namely, the board interlocking network and the scientific collaboration network, were performed. It was revealed that the effect of increasing isoperimetric numbers (i.e., expansion properties) on randomly perturbed intersection graphs is presumably independent of the order of the network.

1. Introduction

Complex large-scale network structures arise in a variety of natural and technological settings [1,2], and they pose numerous challenges to computer scientists and applied mathematicians. Many interesting ideas in this area come from the analysis of social networks [3], where each vertex (actor) is associated with a set of properties (attributes), and pairs of sets with nonempty intersections correspond to edges in the network. Complex and social networks represented by such intersection graphs are copious in the real world. Well-known examples include the film actor network [4], where actors are linked by an edge if they performed in the same movie, the academic co-authorship network [5], where two researchers are linked by an edge if they have a joint publication, the circle of friends in online social networks (e.g., Google+), where two users are declared adjacent if they share a common interest, and the Eschenauer–Gligor key predistribution scheme [6] in secure wireless sensor networks, where two sensors establish secure communication over a link if they have at least one common key. Remarkably, it was shown in Reference [7] that all graphs are indeed intersection graphs.

To understand statistical properties of intersection graphs, a probability model was introduced in References [8,9] as a generalization of the classical model $G(n,p)$ of Erdős and Rényi [10]. Formally, let $n,m$ be positive integers and let $p\in [0,1]$. We start with a random bipartite graph $B(n,m,p)$ with independent vertex sets $V=\{v_1,\cdots ,v_n\}$ and $W=\{w_1,\cdots ,w_m\}$ and edges between V and W existing independently with probability p. In terms of social networks, V is interpreted as a set of actors and W a set of attributes. We then define the random intersection graph $G(n,m,p)$ with vertex set V and vertices $v_i,v_j\in V$ adjacent if and only if there exists some $w\in W$ such that both $v_i$ and $v_j$ are adjacent to w in $B(n,m,p)$. Several variant models of random intersection graphs have been proposed, and many graph-theoretic properties of $G(n,m,p)$, such as degree distribution, connected components, fixed subgraphs, independence number, clique number, diameter, Hamiltonicity and clustering, have been extensively studied [8,9,11,12,13,14]. We refer the reader to References [15,16] for an updated review of recent results in this prolific field.

In light of the above list of properties studied, it is, perhaps, surprising that there has been little work regarding isoperimetric numbers of random intersection graphs. The isoperimetric numbers, which measure the expansion properties of a graph (see Section 2 below for precise definitions), have a long history in random graph theory [17,18,19] and are strongly related to the graph spectrum and expanders [20]. They have found a wide range of applications in theoretical computer science, including algorithm design, data compression, rapid mixing, error correcting codes, and robust computer networks [21]. Social networks such as co-authorship networks are commonly believed to have poor expansion properties (i.e., small isoperimetric numbers), which indicate the existence of bottlenecks (e.g., cuts with small size) inside the networks, because of their modular and community organization [22,23]. In this paper, we hope to show that it is possible to increase the isoperimetric numbers by a gentle perturbation of the original bipartite graph structure underlying the intersection graphs.

In recent times, there has been an effort to study the effect of random perturbation on graphs. The most mathematically famous example is perhaps the Newman–Watts small-world network [1,24], which is a random instance obtained by adding random edges to a cycle, exhibiting short average distance and high clustering coefficient, namely, the so-called small-world phenomenon. A random graph model $G\cup R$ [25] with general connected base graph G on n vertices and R being a sparse Erdos-Rényi random graph $G(n,\epsilon /n)$ where $\epsilon >0$ is some small constant has been introduced in [26], and its further properties, such as connectivity, fixed subgraphs, Hamiltonicity, diameter, mixing time, vertex and edge expansion, have been intensively examined; see, e.g., [27,28,29,30,31,32,33,34] and references therein. For instance, in Reference [29], a necessary condition for the base graph is given under which the perturbed graph $G\cup R$ is an expander a.a.s. (asymptomatically almost surely); for a connected base graph G, it is shown in Reference [30] that, a.a.s. the perturbed graph has an edge–isoperimetric number $\mathsf{\Omega }(1/lnn)$, diameter $O(lnn)$, and vertex–isoperimetric number $\mathsf{\Omega }(1/lnn)$, where for the last property G is assumed to have bounded maximum degree. Here, we say that $G\cup R$ possesses a graph property $\mathcal{P}$ asymptotically almost surely, or a.a.s. for brevity, if the probability that $G\cup R$ possesses $\mathcal{P}$ tends to 1 as n goes to infinity. In this paper, to go a step further in this line of research, we investigate the bipartite graph type perturbation, where random edges are only added to the base (bipartite) graph between the two independent sets. We provide lower bounds for the isoperimetric numbers of random intersection graphs induced by such perturbations.

The rest of the paper is organized as follows. In Section 2, we state and discuss the main results, with proofs relegated to Section 4. In Section 3, we give numerical examples based upon real network data, complementing our theoretical results in small network sizes. Section 5 contains some concluding remarks.

2. Results

Let $G=(V,E)$ be a graph with vertex set V and edge set E. If $S\subseteq V$ is a set of vertices, then ${\partial }_{G}S$ denotes the set of edges of G having one end in S and the other end in $V\setminus S$. Given $S\subseteq V$, write $G\left[S\right]$ for the subgraph of G induced by S. We use $N_G\left(S\right)$ to denote the collection of vertices of $V\setminus S$ which are adjacent to some vertex of S. For a vertex $v\in V$, $N_G\left(v\right)$ is the neighborhood of v, and we denote by $N_G^2\left(v\right)=N_G\left(N_G\left(v\right)\right)$ the second neighborhood of v. The above subscript G will be omitted when no ambiguity may arise. For a graph G, its edge–isoperimetric number, $c\left(G\right)$ (also called its Cheeger constant), is given by:

$$c\left(G\right)=\underset{\genfrac{}{}{0pt}{}{S\subseteq V}{0<\left|S\right|\le \left|V\right|/2}}{min}\frac{|{\partial }_{G}S|}{\left|S\right|}.$$

The vertex–isoperimetric number of G, $\iota \left(G\right)$, can be defined similarly as:

$$\iota \left(G\right)=\underset{\genfrac{}{}{0pt}{}{S\subseteq V}{0<\left|S\right|\le \left|V\right|/2}}{min}\frac{|N_G\left(S\right)|}{\left|S\right|}.$$

It is well-known that $c\left(G\right)/∆\left(G\right)\le \iota \left(G\right)\le c\left(G\right)$ [35], where $∆\left(G\right)$ is the maximum degree of G.

We will consider the following model of randomly perturbed intersection graphs. Given a fixed bipartite graph $B=B(V,W,E)$ with two independent vertex sets V ($\left|V\right|=n$) and W ($\left|W\right|=m$), the intersection graph derived from B is denoted by $G\left(B\right)$. That is, $G\left(B\right)$ is a graph on the vertex set V with two vertices adjacent if they have a common neighbor in B. For each pair of vertices $v\in V$ and $w\in W$, we add the edge $\{v,w\}$ to B independently with probability p. The resulting bipartite graph, denoted $B\cup R$, can be viewed as the union of B and a bipartite graph $R\sim B(n,m,p)$, meaning that R is a random graph distributed according to $B(n,m,p)$. We write $G(B\cup R)$, the intersection graph derived from $B\cup R$. Clearly, if the base graph $B(V,W,E)$ is taken to be the empty bipartite graph, our model $G(B\cup R)$ reduces to the random intersection graph $G(n,m,p)$.

Throughout the paper, the standard Landau asymptotic notations will be utilized (see, e.g., [10]). Let $\lfloor ·\rfloor$ be the round-down operator. As customary in the theory of random intersection graphs, we take $m=\lfloor n^{\alpha }\rfloor$ for a fixed real $\alpha \in (0,\infty )$, which allows for a natural progression from sparse to dense graphs. Recall that we say that $G(B\cup R)$ possesses a graph property $\mathcal{P}$ a.a.s. if the probability that $G(B\cup R)$ possesses $\mathcal{P}$ tends to 1 as n goes to infinity.

We are now ready to formulate the main results of this paper.

Theorem 1.

Let $B=B(V,W,E)$ be a bipartite graph with $\left|V\right|=n$ and $\left|W\right|=m=\lfloor n^{\alpha }\rfloor$ such that any two vertices in V are connected by a path and $∆:={max}_{v\in V}{N}_B^2\left(v\right)$ is a constant (i.e., independent of n). For any $\epsilon >0$, let $R\sim B(n,m,p)$ with $p=\epsilon /n$ if $\alpha \le 1$ and $p=\epsilon /\sqrt{nm}$ if $\alpha >1$. Then there exists some constant $\delta >0$ satisfying $\iota \left(G\right(B\cup R\left)\right)\ge \delta /lnn$ a.a.s.

A couple of remarks are in order.

Remark 1.

The local effects of the perturbation are quite mild, as a small ε is of interest. Nonetheless, the global influence on the vertex–isoperimetric number can be prominent. To see this, note that any connected (intersection) graph G has $\iota \left(G\right)=\mathsf{\Omega }(1/n)$. In particular, if G is a tree, we have $\iota \left(G\right)=\mathsf{\Theta }(1/n)$ (see e.g., [36]).

Remark 2.

It is easy to check that the maximum degree of $G\left(B\right)$ is Δ. In fact, $v\in V$ and $v_1\in V$ are adjacent in $G\left(B\right)$ if and only if they have a common neighbor $w\in W$, namely, $w\in N_B\left(v\right)$ and $v_1\in N_B\left(w\right)$. Hence, the degree of v is $N_B\left(N_B\left(v\right)\right)$. The assumption that Δ is a constant cannot be removed in general. Indeed, when $\alpha \ge 1$, consider the bipartite graph $B(V,W,E)$ with $V=\{v_1,\cdots ,v_n\}$, $W=\{w_1,\cdots ,w_m\}$, and the edge set $E=\{\{v_1,w_i\},\{v_j,w_{j-1}\}|i=1,\cdots ,n-1,j=2,\cdots ,n\}$. It is clear that $G\left(B\right)$ is a star with center $v_1$ over the vertex set V. There are no more than $n^{2}p$ edges over $V\setminus \left\{v_1\right\}$ in the graph $G(B\cup R)$, which covers at most $2n^{2}p$ vertices. In $G(B\cup R)$, there will be an independent set S (meaning that $G(B\cup R)\left[S\right]$ is empty) of order at least:

$$n-2n^{2}p=n\left(1-2\epsilon \sqrt{\frac{n}{m}}\right)$$

and $N_{G(B\cup R)}\left(S\right)=1$. Therefore, $\iota \left(G(B\cup R)\right)\le 1/\left(n(1-2\epsilon \sqrt{n/m})\right)=O(1/n)$. When $\alpha <1$, consider the bipartite graph $B(V,W,E)$ with the edge set $E=\{\{v_1,w_i\},\{v_j,w_{j-1}\},$ $\{v_l,w_m\}|$ $i=1,\cdots ,m$, $j=2,\cdots ,m+1,l=m+2,\cdots ,n\}$. Then $G\left(B\right)$ can be thought of as the joining of a star $K_{1,m}$ having center $v_1$ and a complete graph $K_{n-m+1}$ by identifying $v_1$ with any vertex of $K_{n-m+1}$. After adding $nm\epsilon /n=m\epsilon$ edges to B, in $G(B\cup R)$, there will be an independent set S of order at least $m-1-2m\epsilon$ and $N_{G(B\cup R)}\left(S\right)=1$. Therefore, $\iota \left(G\right(B\cup R\left)\right)\le 1/(m-1-2m\epsilon )=O(1/m)$.

Recall that the inequality $c\left(G\right)\ge \iota \left(G\right)$ holds for any graph G. Therefore, a direct corollary of Theorem 1 reads $c\left(G\right(B\cup R\left)\right)\ge \delta /lnn$ a.a.s. for some $\delta >0$. The following theorem shows that this lower bound for edge–isoperimetric number actually holds without any assumption on $∆$.

Theorem 2.

Let $B=B(V,W,E)$ be a bipartite graph with $\left|V\right|=n$ and $\left|W\right|=m=\lfloor n^{\alpha }\rfloor$ such that any two vertices in V are connected by a path. For any $\epsilon >0$, let $R\sim B(n,m,p)$ with $p=\epsilon /\sqrt{nm}$. Then there exists some constant $\delta >0$ satisfying $c\left(G\right(B\cup R\left)\right)\ge \delta /(1+lnn)$ a.a.s.

Theorems 1 and 2 hold in the sense of large n limit. In the next section, we shall demonstrate that the isoperimetric numbers can be improved as well for small randomly perturbed intersection graphs based upon real network data.

3. Illustration on Small Networks

To find the exact isoperimetric numbers, one needs to calculate the minimum fraction of neighboring vertices or edges over the nodes inside the subset for all possible subsets of vertices with order at most $\left|V\right|/2$. Since this is an NP-hard problem, it is intractable to compute the exact values for general graphs [21,35]. It is well known that Cheeger’s inequality, also known as the Alon–Milman inequality, provides bounds for the isoperimetric numbers using graph Laplacian eigenvalues. On the other hand, standard algorithms in linear algebra can be used to efficiently compute the spectrum of a given large graph. Here, instead of evaluating “approximate” values involving other parameters such as eigenvalues, we are interested in obtaining exact values of $\iota \left(G\right(B\cup R\left)\right)$ and $c\left(G\right(B\cup R\left)\right)$ for small networks.

Two intersection-based social networks are considered here: (i) The Norwegian interlocking directorate network Nor-Boards [37], where two directors are adjacent if they are sitting on the board of the same company based on the Norwegian Business Register on 5 August 2009. The underlying bipartite graph $\overline{B}(\overline{V},\overline{W},\overline{E})$ contains $|\overline{V}|=1495$ directors, $|\overline{W}|=367$ companies, and $|\overline{E}|=1834$ edges indicating the affiliation relations; (ii) the co-authorship network ca-CondMat [5] based on preprints posted to the Condensed Matter Section of arXiv E-Print Archive between 1995 and 1999. The underlying bipartite graph $\overline{B}(\overline{V},\overline{W},\overline{E})$ contains $|\overline{V}|=$ 16,726 authors, $|\overline{W}|=$ 22,016 papers, and $|\overline{E}|=$ 58,596 edges indicating authorship.

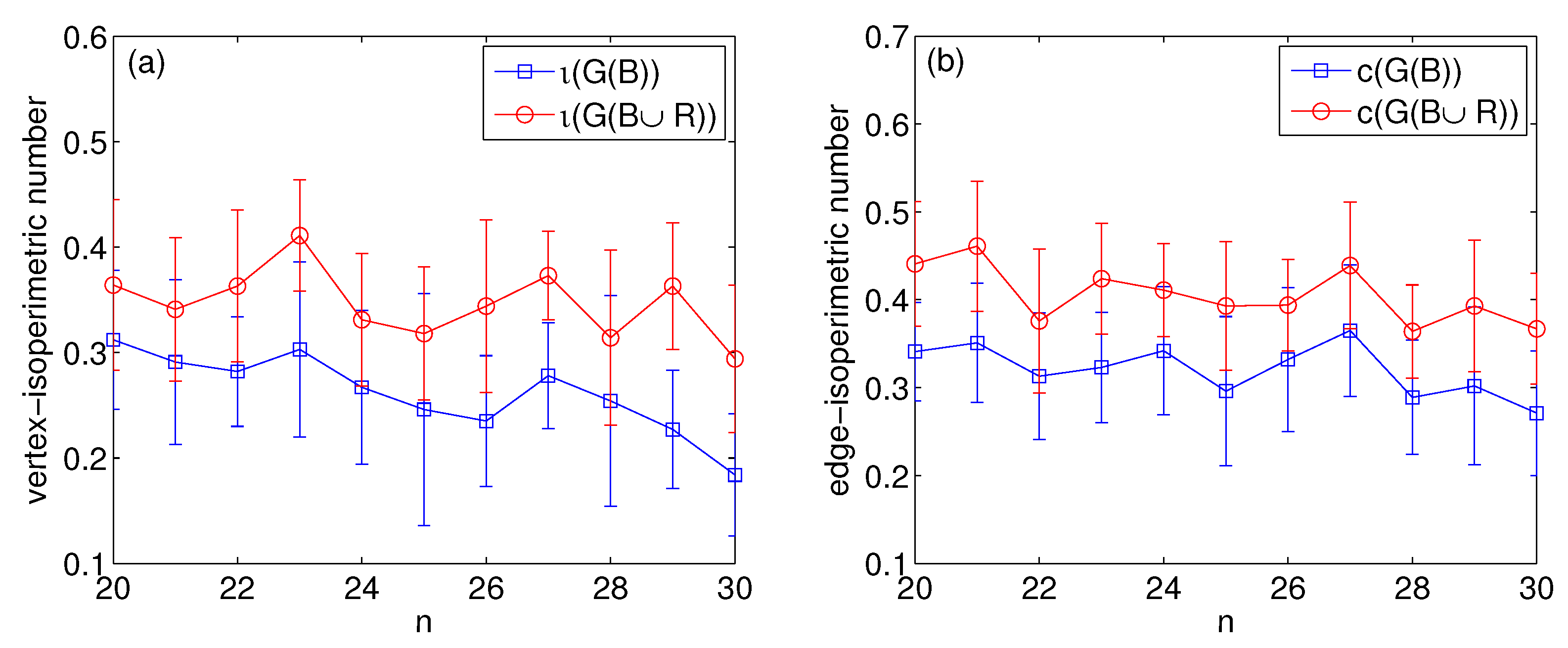
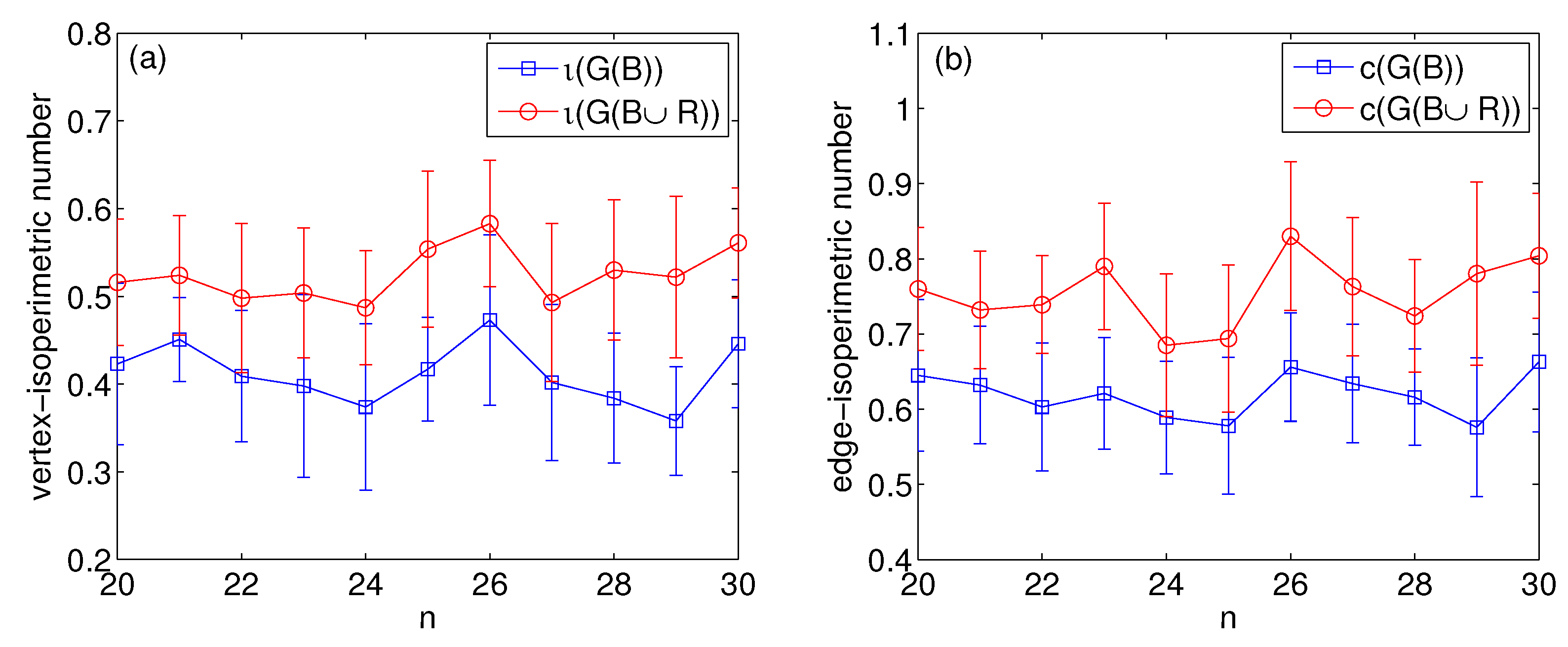
Figure 1 and Figure 2 report the vertex–isoperimetric numbers and edge–isoperimetric numbers for subsets of Nor-Boards and ca-CondMat, respectively. For a given $n\in [20,30]$, we first take a subgraph $B=B(V,W,E)$ from $\overline{B}(\overline{V},\overline{W},\overline{E})$ with $\left|V\right|=n$ so that $G\left(B\right)$ is connected and calculate its vertex–isoperimetric and edge–isoperimetric numbers. Each data point (blue square) in Figure 1 and Figure 2 is obtained by means of an ensemble averaging of 30 independently taken graphs. For each chosen bipartite graph B, we then perturb it following the rules specified in Theorems 1 and 2 with $\epsilon =1$ to get the perturbed intersection graph $G(B\cup R)$. Each data point (red circle) in Figure 1 and Figure 2 is obtained by means of a mixed ensemble averaging of 50 independently-implemented perturbations for 30 graphs. From a statistics viewpoint, it is clear that our random perturbation scheme increases both the vertex–isoperimetric and the edge–isoperimetric number for both cases. This, together with the theoretical results, suggests that the quantitative effect of random perturbations is independent of the order of the network.

Remark 3.

It is worth stressing that the theoretical results (Theorems 1 and 2) are in the large limit of the network size n. In other words, the form $\frac{1}{lnn}$ only makes sense as $n\to \infty$. The simulation results presented in Figure 1 and Figure 2 are for very small networks. Therefore, these results have no bearing on the $\frac{1}{lnn}$ dependence (although a slight decline tendency for $\iota \left(G\right(B\cup R\left)\right)$ can be seen in Figure 1a). The main phenomenon we observe from Figure 1 and Figure 2 is that the random perturbation increases both vertex– and edge–isoperimetric numbers for all the cases considered. The numerical results (for small finite graphs) are a nice complement to the theoretical results (for infinite graphs). However, our numerical observations neither prove the $\frac{1}{lnn}$ dependence would hold for small graphs nor show that such an increase of isoperimetric numbers would be universal in any sense. (A practical issue stems from graph sampling. To establish a proper model fit to the data, Akaike information criteria and Bayesian information criteria need to be applied.) The establishment of correlation between isoperimetric numbers and graph size n for finite intersection graphs is an interesting future work.

4. Proofs

In this section, we prove Theorems 1 and 2. Our idea behind this is somewhat simple: If the network can be carefully decomposed into some subnetworks so that the resulting super-network (with these subnetworks being super-vertices) is sparse and highly connected, then its isoperimetric numbers are expected to be high. Similar approaches have been applied in, e.g., References [29,30,31].

Proof of Theorem 1.

Set $s=C∆(lnn)/\epsilon$ for some constant $C=C\left(\epsilon \right)>0$ to be determined. By assumption, $G\left(B\right)$ is connected. Following Reference [38] (Proposition 4.5), we can divide the vertex set V into disjoint sets $V_1,V_2,\cdots ,V_{\theta }$ satisfying $s\le |V_i|\le ∆s$ and $G\left(B\right)\left[V_i\right]$ connected for each i. Clearly, $n/(∆s)\le \theta \le n/s$. Let $\left[\theta \right]=\{1,2,\cdots ,\theta \}$. For a graph $G=(V,E)$, we say two sets $S_1,S_2\subseteq V$ have common neighbors in G if there exist $v_1\in S_1$, $v_2\in S_2$, and $v\in V$ such that $\{v_1,v\}\in E$ and $\{v_2,v\}\in E$ hold.

We will first show the following property for the random bipartite graph R holds a.a.s.: For every $\mathsf{\Theta }\subseteq \left[\theta \right]$ with $0<\left|\mathsf{\Theta }\right|\le \theta /2$, there exist at least $\left|\mathsf{\Theta }\right|/2$ many of $V_i$ ($i\in \left[\theta \right]\setminus \mathsf{\Theta }$) which have common neighbors with ${\cup }_{i\in \mathsf{\Theta }}{V}_i$ in R.

Indeed, the probability that two sets $V_i$ and $V_j$ have no common neighbors in R can be computed as ${\left\{1-[1-{(1-p)}^{|V_i|}][1-{(1-p)}^{|V_j|}]\right\}}^m$. Hence, the probability that there exists a set $\mathsf{\Theta }\subseteq \left[\theta \right]$ with $0<\left|\mathsf{\Theta }\right|\le \theta /2$ such that no more than $\left|\mathsf{\Theta }\right|/2$ many of $V_i$ ($i\in \left[\theta \right]\setminus \mathsf{\Theta }$) have common neighbors with ${\cup }_{i\in \mathsf{\Theta }}{V}_i$ in R is upper bounded by:

$$\begin{array}{cc}& \sum _{1\le j\le \theta /2}\left(\genfrac{}{}{0pt}{}{\theta }{j}\right)\left(\genfrac{}{}{0pt}{}{\theta -j}{\lfloor \frac{j}{2}\rfloor }\right){\left\{1-[1-{(1-p)}^{js}][1-{(1-p)}^{(\theta -\lfloor 3j/2\rfloor )s}]\right\}}^m,\hfill \\ \le & \sum _{1\le j\le \theta /2}{\left(\frac{e\theta }{j}\right)}^j{\left(\frac{2e(\theta -j)}{j}\right)}^{j/2}{\left(1-\frac{s^{2}j(\theta -3j/2)p^2}{4}\right)}^m,\hfill \end{array}$$

where $\left(\genfrac{}{}{0pt}{}{\theta }{j}\right)\left(\genfrac{}{}{0pt}{}{\theta -j}{\lfloor \frac{j}{2}\rfloor }\right)$ counts the choice of $\mathsf{\Theta }$ (with $\left|\mathsf{\Theta }\right|=j$) and the corresponding sets $\left\{V_i\right\}$ described above, the estimate $|V_i|\ge s$ for all $i\in \left[\theta \right]$ is utilized in the multiplicative probabilities (i.e., there are at least $(\theta -\lfloor 3j/2\rfloor )$ sets in the union ${\cup }_{i\in \mathsf{\Theta }}{V}_i$), and the upper bound comes from a direct application of inequalities ([10], p. 386). The above probability is further upper bounded by ${(C(lnn)/n)}^m{\sum }_{1\le j\le \theta /2}{(2\theta /j)}^{3j/2}=o\left(1\right)$ when $\alpha \le 1$, and is upper bounded by ${\sum }_{1\le j\le \theta /2}{\theta }^{3j/2+2}exp(-C\epsilon ∆jlnn)=o\left(1\right)$ when $\alpha >1$ for a sufficiently large C. Therefore, the above property for the random bipartite graph R holds a.a.s. In the following, we will condition on such an R.

Fix a set $S\subseteq V$ with $\left|S\right|\le n/2$. Define three sets of indices: ${\mathsf{\Theta }}_0=\{i\in \left[\theta \right]|V_i\subseteq S\}$, ${\mathsf{\Theta }}_1=\{i\in \left[\theta \right]|0<|V_i\cap S|<|V_i\left|\right\}$, and ${\mathsf{\Theta }}_2=\{i\in \left[\theta \right]\setminus {\mathsf{\Theta }}_0|N_{G(B\cup R)}\left(V_i\right)\cap S\ne \varnothing \}$. Note that ${\mathsf{\Theta }}_0$ and ${\mathsf{\Theta }}_1$ are deterministic, but ${\mathsf{\Theta }}_2$ is a random set. If $|{\mathsf{\Theta }}_0|\le \theta /2$, $|{\mathsf{\Theta }}_2|\ge |{\mathsf{\Theta }}_0|/2$ a.a.s. by the above assumed property of R. Similarly, if $|{\mathsf{\Theta }}_0|>\theta /2$, we have $|{\mathsf{\Theta }}_2|\ge |\mathsf{\Theta }|/2=(\theta -|{\mathsf{\Theta }}_0|-|{\mathsf{\Theta }}_2\left|\right)/2$ a.a.s., where $\mathsf{\Theta }=\left[\theta \right]\setminus ({\mathsf{\Theta }}_0\cup {\mathsf{\Theta }}_2)$. Hence, $|{\mathsf{\Theta }}_2|\ge min\{|{\mathsf{\Theta }}_0|/2,(\theta -|{\mathsf{\Theta }}_0\left|\right)/3\}$ a.a.s. Recall that $\left|S\right|\le n/2$. We derive that $n/2\le |V\setminus S|\le |{\cup }_{i\notin {\mathsf{\Theta }}_0}{V}_i|\le (\theta -|{\mathsf{\Theta }}_0\left|\right)∆s\le (\theta -|{\mathsf{\Theta }}_0\left|\right)∆n/\theta$, and thus, $\theta -|{\mathsf{\Theta }}_0|\ge \theta /(2∆)$. Therefore, we have a.a.s.:

$$|{\mathsf{\Theta }}_2|\ge min\left\{\frac{|{\mathsf{\Theta }}_0|}{2},\frac{\theta }{6∆}\right\}\ge \frac{|{\mathsf{\Theta }}_0|}{6∆}.$$

By definition, we have $S\subseteq {\cup }_{i\in {\mathsf{\Theta }}_0\cup {\mathsf{\Theta }}_1}{V}_i$. Thus, $\left|S\right|\le \left(\right|{\mathsf{\Theta }}_0|+|{\mathsf{\Theta }}_1\left|\right)∆s$. Since $G\left(B\right)\left[V_i\right]$ for $i\in {\mathsf{\Theta }}_1$ is connected, $|N_{G(B\cup R)}\left(S\right)|\ge |{\mathsf{\Theta }}_1\cup {\mathsf{\Theta }}_2|$. Now we consider two cases. If $|{\mathsf{\Theta }}_1|\ge |{\mathsf{\Theta }}_0|$, then $|N_{G(B\cup R)}\left(S\right)|\ge |{\mathsf{\Theta }}_1|\ge |S|/(2∆s)$. If $|{\mathsf{\Theta }}_1|\le |{\mathsf{\Theta }}_0|$, then $|N_{G(B\cup R)}\left(S\right)|\ge |{\mathsf{\Theta }}_2|\ge |{\mathsf{\Theta }}_0|/(6∆)\ge |S|/\left(12{∆}^{2}s\right)$ a.a.s. Therefore:

$$\frac{|N_{G(B\cup R)}\left(S\right)|}{\left|S\right|}\ge min\left\{\frac{1}{2∆s},\frac{1}{12{∆}^{2}s}\right\}a.a.s.$$

Recall the definition of s at the beginning of the proof, and we complete the proof by taking $\delta =\epsilon /\left(12{∆}^{3}C\right)$. □

We have made no attempt to optimize the constants in the proof. It is easy to check that the condition that $G\left(B\right)$ is connected in Theorem 1 can be weakened. For example, the above proof holds if each connected component of $G\left(B\right)$ is of order at least $C∆(lnn)/\epsilon$.

Let $G=(V,E)$ be a graph of order n. For integers a, b, and c, define $\mathcal{S}(a,b,c)$ as a collection of all sets $S\subseteq V$ such that $\left|S\right|=a$ and there exists a partition $S=S_1\cup \cdots \cup S_b$, where each $G\left[S_i\right]$ is connected, there are no edges in E connecting different $S_i$, and $|N_G\left(S_1\right)|+\cdots +|N_G\left(S_b\right)|=c$. The next lemma gives an upper bound of the size of $\mathcal{S}(a,b,c)$.

Lemma 1.

([30])

$$\left|\mathcal{S}(a,b,c)\right|\le {\left(\frac{en}{b}\right)}^b{\left(\frac{ea}{b}\right)}^b{\left(\frac{ec}{b}\right)}^b{\left(\frac{e(a+c)}{c}\right)}^c.$$

Proof of Theorem 2.

Consider the family $\mathcal{S}(a,b,c)$ of sets defined in graph $G\left(B\right)$. Since $G\left(B\right)$ is connected, we have for each $S\in \mathcal{S}(a,b,c)$, $|{\partial }_{G\left(B\right)}S|\ge c\ge b$. Note that $|{\partial }_{G(B\cup R)}S|\ge |{\partial }_{G\left(R\right)}S|$ holds. It suffices to show that the following property for the random bipartite graph R holds a.a.s.: There are constants $K,\delta >0$ such that for any $Klnn\le a\le n/2$, we have:

$$|{\partial }_{G\left(R\right)}S|\ge \frac{\delta a}{1+lnn},$$

for each $S\in \mathcal{S}(a,b,c)$ with $b\le c\le \delta a/(1+lnn)$. Indeed, when $\left|S\right|=a\le Klnn$, we can choose a small $\delta$ such that $2K\delta \le 1$. Thus, $|{\partial }_{G(B\cup R)}S|\ge |{\partial }_{G\left(B\right)}\left(S\right)|\ge 1\ge \delta a/(1+lnn)$.

It follows from Lemma 1 and $b\le c\le \delta a/(1+lnn)\le a$ that:

$$\begin{array}{ccc}\hfill \left|\mathcal{S}\right(a,b,c\left)\right|& \le & {\left(\frac{2e^{4}n{a}^2}{c^3}\right)}^c\le {\left(\frac{2e^{4}n{(1+lnn)}^3}{{\delta }^{3}a}\right)}^{\delta a/(1+lnn)}\le e^{C\delta aln(1/\delta )},\hfill \end{array}$$

for some constant $C>0$, where the first inequality holds since $f\left(x\right)={(e\rho /x)}^x$ is increasing on $(0,\rho ]$ and the second inequality holds since $g\left(x\right)={(\rho /x^3)}^x$ is increasing on $(0,{\rho }^{1/3}]$.

Note that $m{p}^2\to 0$ and $1-{(1-p^2)}^m\sim m{p}^2$. For a fixed S with $\left|S\right|=a\le n/2$, we obtain:

$$\begin{array}{ccc}\hfill \mathbb{P}\left(\right|{\partial }_{G\left(R\right)}S|<\delta a)& \lesssim & \mathbb{P}(Bin(a(n-a),m{p}^2)<\delta a)\le \mathbb{P}\left(Bin\left(\frac{na}{2},m{p}^2\right)<\delta a\right)\hfill \\ & \le & exp\left(-\frac{a{\epsilon }^2}{16}\right),\hfill \end{array}$$

provided $\delta <{\epsilon }^2/4$, where the first inequality relies on Reference [9] (Theorem 2.2) and the last line uses a standard Chernoff’s bound (e.g., [10]). Hence:

$$\begin{array}{cc}& \mathbb{P}\left(|{\partial }_{G\left(R\right)}S|<\frac{\delta a}{1+lnn},\exists S\in \mathcal{S}(a,b,c),b\le c\le \frac{\delta a}{1+lnn},Klnn\le a\le \frac{n}{2}\right)\hfill \\ \le & \mathbb{P}\left(|{\partial }_{G\left(R\right)}S|<\delta a,\exists S\in \mathcal{S}(a,b,c),b\le c\le n,Klnn\le a\le n\right)\hfill \\ \le & n^{3}exp\left(C\delta aln\left(\frac{1}{\delta }\right)-\frac{a{\epsilon }^2}{16}\right).\hfill \end{array}$$

By taking $C\delta ln(1/\delta )\le {\epsilon }^2/32$ and $K\ge 100/{\epsilon }^2$, the last line above is upper bounded by $n^{3}exp(-{\epsilon }^{2}a/32)\le n^{3}exp(-{\epsilon }^{2}K(lnn)/32)\le n^{3}exp(-25(lnn)/8)=o\left(1\right)$ as $n\to \infty$. The proof is complete. □

5. Concluding Remarks

In this paper, we presented a model of randomly perturbed intersection graphs. The intersection graph is induced by a given bipartite graph (base graph) plus a binomial random bipartite graph. We proved that a.a.s., the vertex–isoperimetric number and the edge–isoperimetric number of the randomly perturbed intersection graphs are of order $\mathsf{\Omega }(1/lnn)$ under some mild conditions. It would be interesting to investigate path length, diameter, and clustering coefficient of this model, which are important characteristics of real-life complex and social networks.

Another intriguing direction is to examine more general intersection graph models, such as active and passive intersection graphs [39]. In particular, if two vertices in one independent set V are declared adjacent when they have at least $k\ge 1$ common neighbors in the other independent set W, what role will k play in estimating the isoperimetric numbers, clustering, and path length of the resulting perturbed intersection graphs? Other perturbation mechanisms are also of research interest.