Application of the Extended HOMED (Harmonic Oscillator Model of Aromaticity) Index to Simple and Tautomeric Five-Membered Heteroaromatic Cycles with C, N, O, P, and S Atoms
Abstract
1. Introduction
2. Methodology
3. Results and Discussion
3.1. HOMED Procedure
3.2. HOMED Parametrization
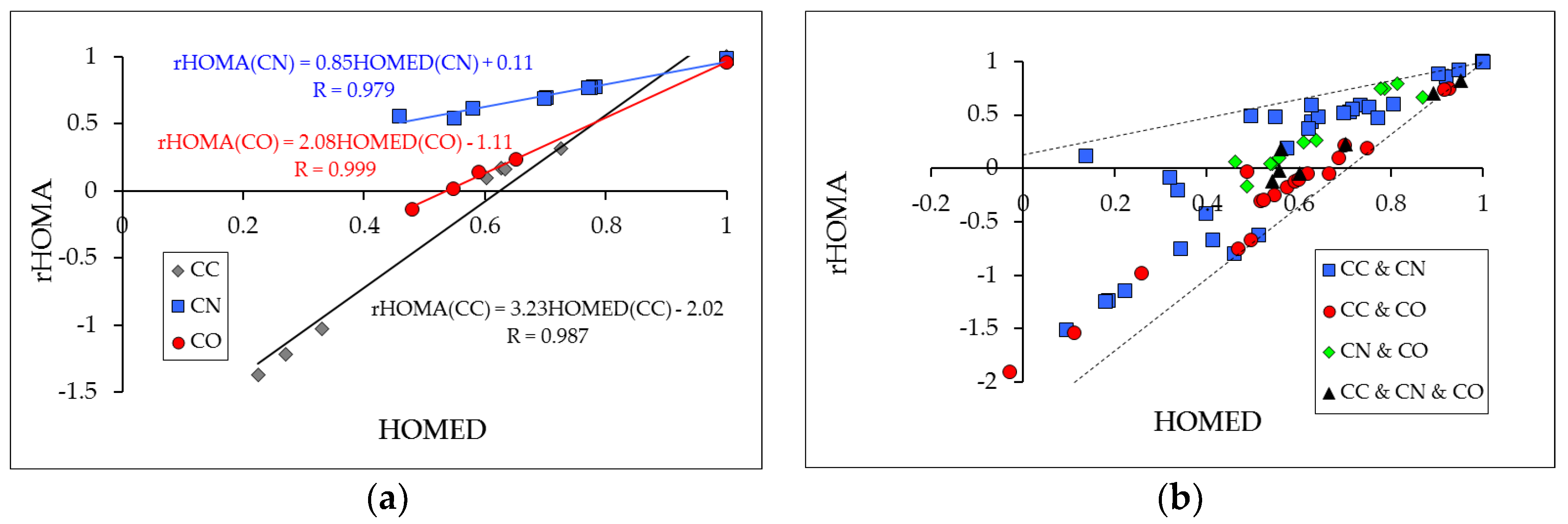
3.3. Differences in the HOMED and rHOMA Scales
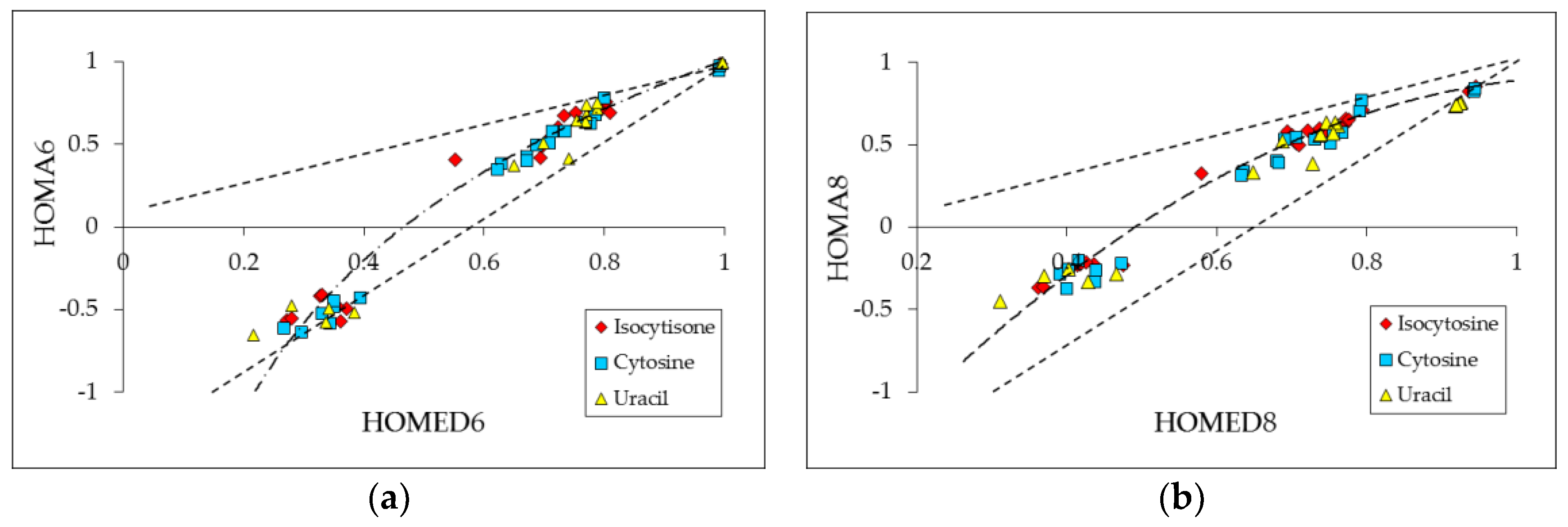
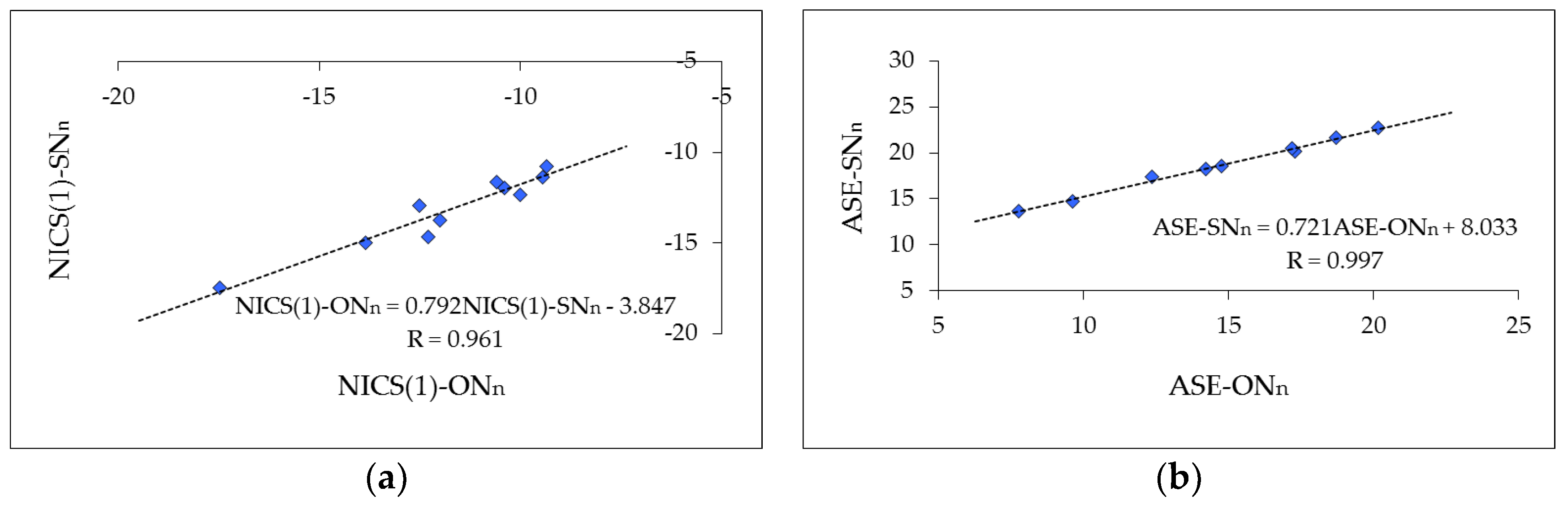
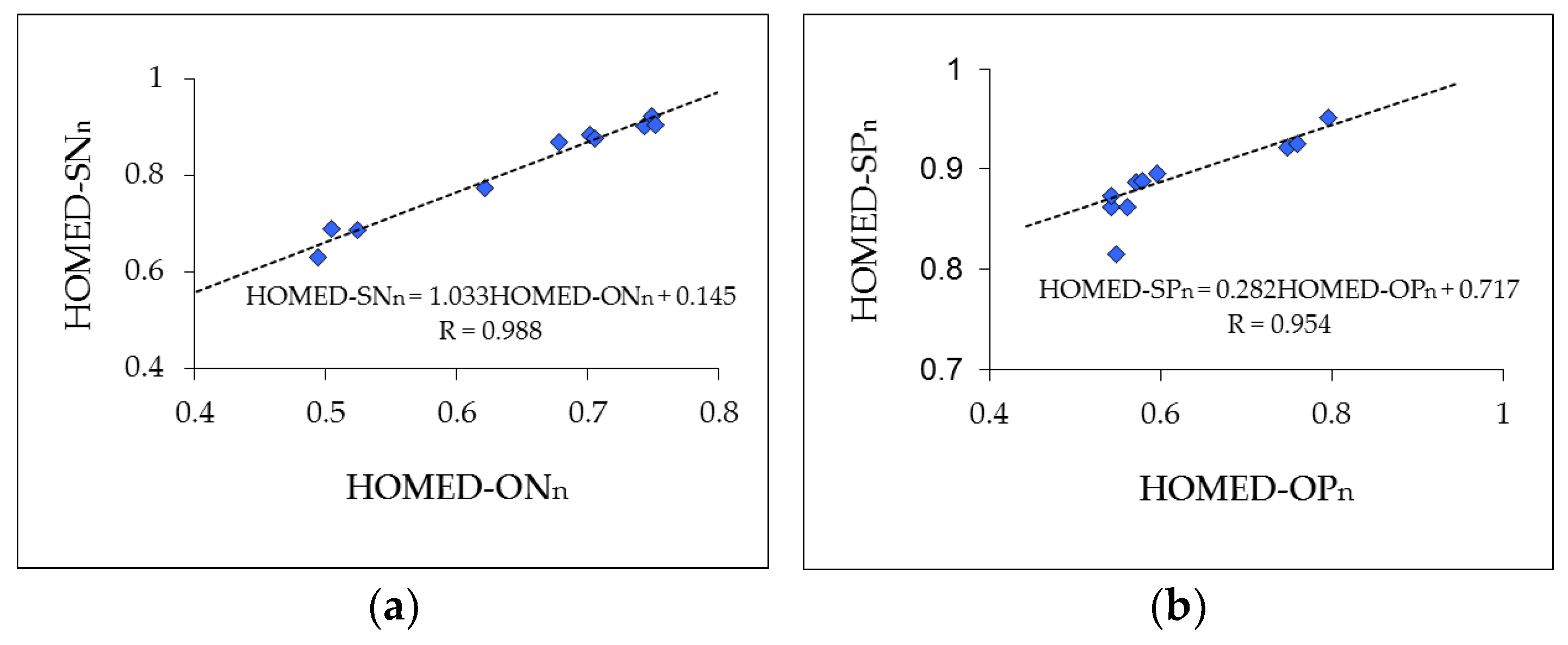
3.4. From Linear Tendencies to Scatter Plots Between rHOMA and HOMED Indices
3.5. HOMED Application to Furan, Thiophene and Their N- and P-Derivatives
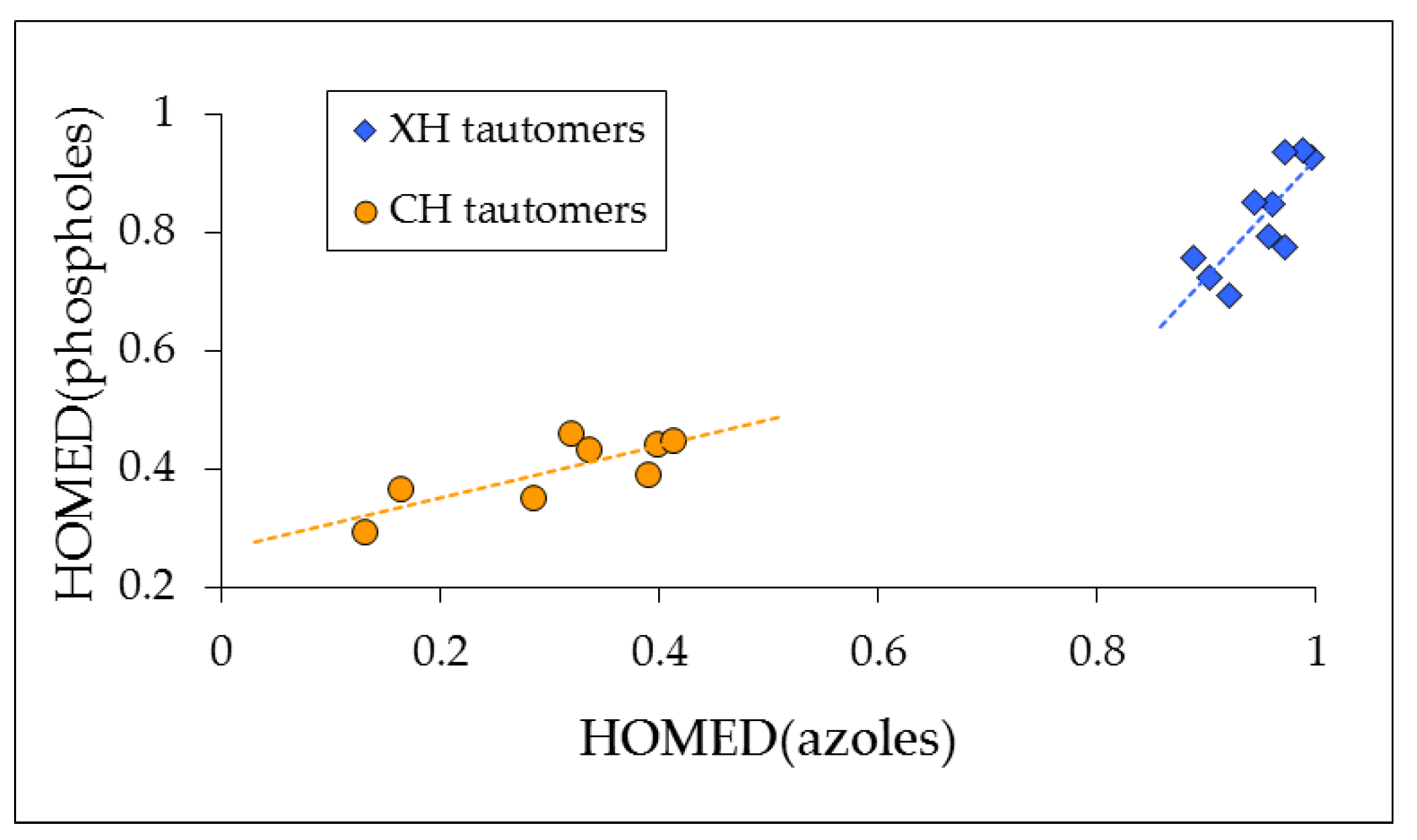
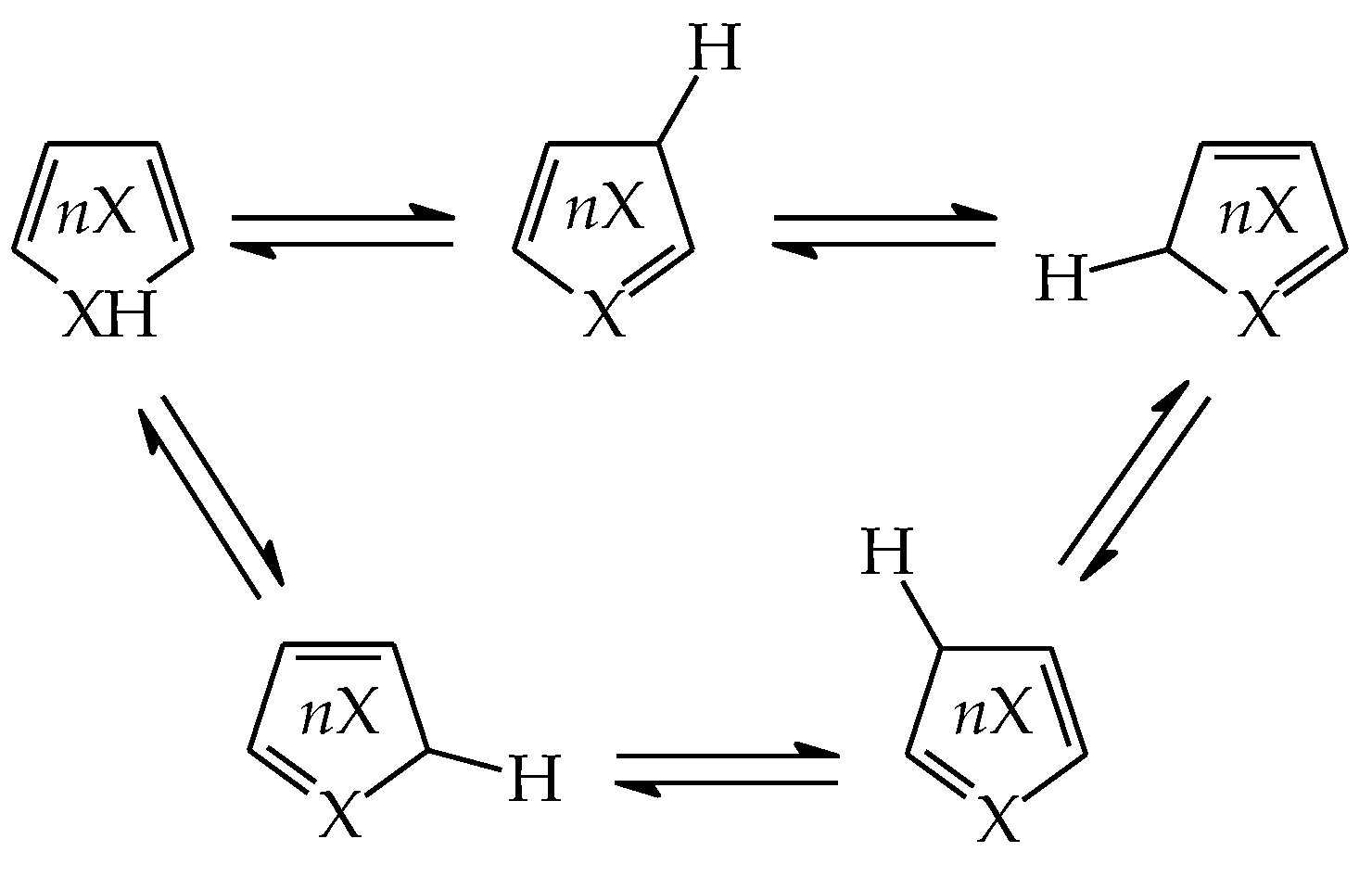
3.6. HOMED Application to Tautomeric Azoles and Phospholes
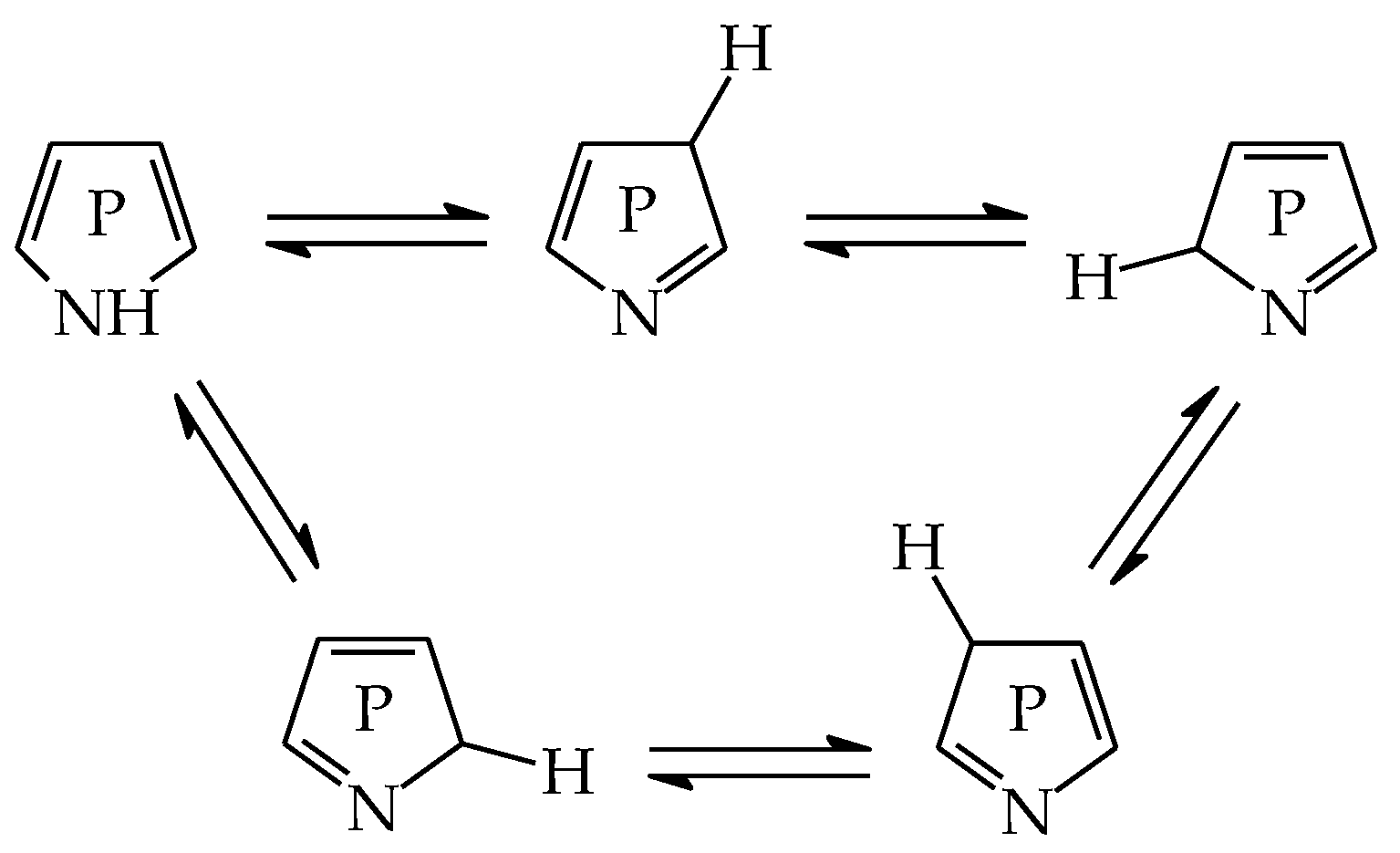
3.7. HOMED Application to Tautomeric Phosphazoles
4. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Kruszewski, J.; Krygowski, T.M. Harmonic oscillator approach to the definition of aromaticity. Bull. Acad. Pol. Sci. Chim. 1972, 20, 907–915. [Google Scholar]
- Kruszewski, J.; Krygowski, T.M. Definition of aromaticity basing on the harmonic oscillator model. Tetrahedron Lett. 1972, 3839–3842. [Google Scholar] [CrossRef]
- Krygowski, T.M.; Kruszewski, J. Bond reactivity index (BRI) in terms of simple harmonic oscillator theory. Bull. Acad. Pol. Sci. Chim. 1973, 21, 409–412. [Google Scholar]
- Krygowski, T.M.; Kruszewski, J. Aromaticity of thiophene, pyrrole and furan in terms of aromaticity indices and Hammett σ constants. Bull. Acad. Pol. Sci. Chim. 1974, 22, 871–876. [Google Scholar]
- Jug, K.; Koester, A. Influence of σ and π electrons on aromaticity. J. Am. Chem. Soc. 1990, 112, 6772–6777. [Google Scholar] [CrossRef]
- Krygowski, T.M. Crystallographic studies of inter- and intramolecular interactions reflected in aromatic character of π-electron systems. J. Chem. Inform. Comput. Sci. 1993, 33, 70–78. [Google Scholar] [CrossRef]
- Krygowski, T.M.; Cyrański, M.K. Structural aspects of aromaticity. Chem. Rev. 2001, 101, 1385–1419. [Google Scholar] [CrossRef]
- Cyrański, M.K. Energetic aspects of cyclic pi-electron delocalization: Evaluation of the methods of estimating aromatic stabilization energies. Chem. Rev. 2005, 105, 3773–3811. [Google Scholar] [CrossRef]
- Raczyńska, E.D.; Kosińska, W.; Ośmiałowski, B.; Gawinecki, R. Tautomeric equilibria in relation to pi-electron delocalization. Chem. Rev. 2005, 105, 3561–3612. [Google Scholar] [CrossRef]
- Raczyńska, E.D.; Krygowski, T.M.; Zachara, J.E.; Ośmiałowski, B.; Gawinecki, R. Tautomeric equilibria, H-bonding and π-electron delocalization in o-nitrosophenol. A B3LYP/ 6-311+G(2df,2p) study. J. Phys. Org. Chem. 2005, 18, 892–897. [Google Scholar] [CrossRef]
- Ośmiałowski, B.; Raczyńska, E.D.; Krygowski, T.M. Tautomeric equilibria and pi electron delocalization for some monohydroxyarenes–Quantum chemical studies. J. Org. Chem. 2006, 71, 3727–3736. [Google Scholar] [CrossRef] [PubMed]
- Krygowski, T.M.; Szatyłowicz, H.; Stasyuk, O.A.; Dominikowska, J.; Paluszak, M. Aromaticity from the viewpoint of molecular geometry: Application to planar systems. Chem. Rev. 2014, 114, 6383–6422. [Google Scholar] [CrossRef] [PubMed]
- Krygowski, T.M.; Szatyłowicz, H. Aromaticity: What does it mean? ChemTexts 2015, 1, 12:1–12:10. [Google Scholar] [CrossRef] [PubMed]
- Szatyłowicz, H.; Stasyuk, O.A.; Krygowski, T.M. Calculating the aromaticity of heterocycles. Adv. Heterocycl. Chem. 2016, 120, 301–327. [Google Scholar] [CrossRef]
- Stasyuk, O.A.; Szatyłowicz, H.; Krygowski, T.M. Effect of the H-bonding on aromaticity of purine tautomers. J. Org. Chem. 2012, 77, 4035–4045. [Google Scholar] [CrossRef] [PubMed]
- Stasyuk, O.A.; Szatyłowicz, H.; Krygowski, T.M. Effect of H-bonding and complexation with metal ions on the pi-electron structure of adenine tautomers. Org. Biomol. Chem. 2014, 12, 456–466. [Google Scholar] [CrossRef] [PubMed]
- Stasyuk, O.A.; Szatyłowicz, H.; Krygowski, T.M. Metal complexation and H-bonding effects on electronic structure of cytosines studied in the gas phase. Croat. Chem. Acta 2014, 87, 335–342. [Google Scholar] [CrossRef]
- Stasyuk, O.A.; Szatyłowicz, H.; Krygowski, T.M. Tautomerisation of thymine acts against the Hűckel 4N + 2 rule. The effect of metal ions and H-bond complexations on the electronic structure of thymine. Org. Biomol. Chem. 2014, 12, 6476–6483. [Google Scholar] [CrossRef] [PubMed]
- Stasyuk, O.A.; Szatyłowicz, H.; Krygowski, T.M. Aromaticity of H-bonded and metal complexes of guanine tautomers. Struct. Chem. 2016, 27, 111–118. [Google Scholar] [CrossRef]
- Raczyńska, E.D.; Krygowski, T.M.; Duczmal, K.; Hallmann, M. On geometry-based HOMED (a measure of hyperconjugation, n-π, and π-π conjugation) and HOMA index (a measure of aromaticity). In Proceedings of the XVIII International Conference on Physical Organic Chemistry, Warsaw, Poland, 20–25 August 2006; p. 31. [Google Scholar]
- Raczyńska, E.D.; Hallmann, M.; Kolczyńska, K.; Stępniewski, T.M. On the harmonic oscillator model of electron delocalization (HOMED) index and its application to heteroatomic π-electron systems. Symmetry 2010, 2, 1485–1509. [Google Scholar] [CrossRef]
- Raczyńska, E.D.; Makowski, M.; Hallmann, M.; Kamińska, B. Geometric and energetic consequences of prototropy for adenine and its structural models—A review. RSC Adv. 2015, 5, 36587–36604. [Google Scholar] [CrossRef]
- Raczyńska, E. Quantum-chemical studies on the favored and rare isomers of isocytosine. Comput. Theor. Chem. 2017, 1121, 58–67. [Google Scholar] [CrossRef]
- Raczyńska, E.D.; Gal, J.-F.; Maria, P.-C.; Michalec, P.; Zalewski, M. Exceptionally high proton and lithium cation gas-phase basicity of the anti-diabetic drug metformin. J. Phys. Chem. A 2017, 121, 8706–8718. [Google Scholar] [CrossRef] [PubMed]
- Frizzo, C.P.; Martins, M.A.P. Aromaticity in heterocycles: New HOMA index parametrization. Struct. Chem. 2012, 23, 375–380. [Google Scholar] [CrossRef]
- Bird, C.W. A new aromaticity index and its application to five-membered ring heterocycles. Tetrahedron 1985, 41, 1409–1414. [Google Scholar] [CrossRef]
- Bird, C.W. The application of a new aromaticity index to six-membered ring heterocycles. Tetrahedron 1986, 42, 89–92. [Google Scholar] [CrossRef]
- Bird, C.W. The application of a new aromaticity index to some bicyclic heterocycles. Tetrahedron 1987, 43, 4725–4730. [Google Scholar] [CrossRef]
- Bird, C.W. Heteroaromaticity. 4. The status of phosphorus and arsenic as heteroatoms. Tetrahedron 1990, 46, 5697–5702. [Google Scholar] [CrossRef]
- Bird, C.W. Heteroaromaticity. 5. A unified aromaticity index. Tetrahedron 1992, 48, 335–340. [Google Scholar] [CrossRef]
- Bird, C.W. Heteroaromaticity. 6. The effect of molecular distortion on aromaticity. Tetrahedron 1992, 48, 1675–1682. [Google Scholar] [CrossRef]
- Bird, C.W. Heteroaromaticity. 7. Some quantitative aspects of the tautomerism of hydroxy- and mercaptoazines. Tetrahedron 1992, 48, 7857–7862. [Google Scholar] [CrossRef]
- Bird, C.W. Heteroaromaticity. 8. The influence of N-oxide formation on heterocyclic Aromaticity. Tetrahedron 1993, 49, 8441–8448. [Google Scholar] [CrossRef]
- Bird, C.W. The relation of classical and magnetic criteria of aromaticity. Tetrahedron 1996, 52, 9945–9952. [Google Scholar] [CrossRef]
- Bird, C.W. Heteroaromaticity. 10. The direct calculation of resonance energies of azines and azoles from molecular dimentions. Tetrahedron 1997, 53, 13111–13118. [Google Scholar] [CrossRef]
- Katritzky, A.R.; Barczyński, P.; Musumurra, G.; Pisano, D.; Szafran, M. Aromaticity as a quantitative concept. 1. A statistical demonstration of the orthogonality of ‘classical’ and ‘magnetic’ aromaticity in five- and six-membered heterocycles. J. Am. Chem. Soc. 1989, 11, 7–15. [Google Scholar] [CrossRef]
- Katritzky, A.R.; Jug, K.; Oniciu, D.C. Quantitative measures of aromaticity for mono-, bi-, and tricyclic penta- and hexaatomic heteroaromatic ring systems and their interrelations. Chem. Rev. 2001, 101, 1421–1450. [Google Scholar] [CrossRef] [PubMed]
- Balaban, A.T.; Oniciu, D.C.; Katritzky, A.R. Aromaticity as a cornestone of heterocyclic chemistry. Chem. Rev. 2004, 104, 2777–2812. [Google Scholar] [CrossRef]
- Minkin, V.I.; Glukhovtsev, M.N.; Simkin, B.Y. Aromaticity and Antiaromaticity. Electronic and Structural Aspects; John Wiley & Sons, Inc.: New York, NY, USA, 1994; ISBN 0471593826. [Google Scholar]
- Schleyer, P.v.R.; Maerker, C.; Dransfield, A.; Jiao, H.; van Eikema Hommes, N.J.R. Nucleus-independent chemical shifts: A simple and efficient aromaticity probe. J. Am. Chem. Soc. 1996, 118, 6317–6318. [Google Scholar] [CrossRef]
- Schleyer, P.v.R.; Jiao, H. What is aromaticity? Pure Appl. Chem. 1996, 68, 209–218. [Google Scholar] [CrossRef]
- Chen, Z.; Wannere, C.S.; Corminboeuf, C.; Puchta, R.; Schleyer, P.v.R. Nucleus-independent chemical shifts (NICS) as aromaticity criterion. Chem. Rev. 2005, 105, 3842–3888. [Google Scholar] [CrossRef]
- Poater, J.; Duran, M.; Solà, M.; Silvi, B. Theoretical evaluation of electron delocalization in aromatic molecules by means of atoms in molecules (AIM) and electron localization function (ELF) topological approaches. Chem. Rev. 2005, 105, 3911–3947. [Google Scholar] [CrossRef]
- Feixas, F.; Matito, E.; Poater, J.; Solà, M. On the performance of some aromaticity indices: A critical assessment using a test set. J. Comput. Chem. 2008, 29, 1543–1554. [Google Scholar] [CrossRef] [PubMed]
- D’Auria, M. A new proposal for the estimation of the aromatic character. Lett. Org. Chem. 2013, 10, 277–282. [Google Scholar] [CrossRef]
- D’Auria, M. A new index for the estimation of the aromatic character—II. Lett. Org. Chem. 2014, 11, 250–258. [Google Scholar] [CrossRef]
- D’Auria, M. A new index for the estimation of the aromatic character—III. Lett. Org. Chem. 2014, 11, 731–735. [Google Scholar] [CrossRef]
- D’Auria, M. A new index for the estimation of the aromatic character—IV. Lett. Org. Chem. 2014, 11, 657–663. [Google Scholar] [CrossRef]
- D’Auria, M. A new index for the estimation of the aromatic character—V. Lett. Org. Chem. 2015, 12, 233–236. [Google Scholar] [CrossRef]
- D’Auria, M. A new index for the estimation of the aromatic character—VI. Lett. Org. Chem. 2015, 12, 482–490. [Google Scholar] [CrossRef]
- D’Auria, M. A new index for the estimation of the aromatic character—VII. Lett. Org. Chem. 2015, 12, 402–406. [Google Scholar] [CrossRef]
- D’Auria, M. A new index for the estimation of the aromatic character—VIII. Lett. Org. Chem. 2015, 12, 549–559. [Google Scholar] [CrossRef]
- D’Auria, M. A new index for the estimation of the aromatic character—IX. Lett. Org. Chem. 2016, 13, 33–43. [Google Scholar] [CrossRef]
- D’Auria, M. A new index for the estimation of the aromatic character—X. Lett. Org. Chem. 2016, 13, 368–373. [Google Scholar] [CrossRef]
- D’Auria, M. An approach to the aromaticity based on the energy of the occupied π orbitals. Curr. Org. Chem. 2016, 20, 971–983. [Google Scholar] [CrossRef]
- Wheland, G.W. The Theory of Resonance and Its Application to Organic Chemistry; John Wiley and Sons, Inc.: New York, NY, USA, 1955. [Google Scholar]
- Pauling, L. The Nature of the Chemical Bond, 3rd ed.; Cornell University Press: Ithaca, NY, USA, 1960; ISBN 0801403332. [Google Scholar]
- Glendening, E.D.; Weinhold, F. Natural resonance theory: I. General formalism. J. Comput. Chem. 1998, 19, 593–609. [Google Scholar] [CrossRef]
- Glendening, E.D.; Weinhold, F. Natural resonance theory: II. Natural bond order and valency. J. Comput. Chem. 1998, 19, 610–627. [Google Scholar] [CrossRef]
- Glendening, E.D.; Badenhoop, J.K.; Weinhold, F. Natural resonance theory: III. Chemical applications. J. Comput. Chem. 1998, 19, 628–646. [Google Scholar] [CrossRef]
- Parr, R.G.; Yang, W. Density Functional Theory of Atoms and Molecules; Oxford University Press: New York, NY, USA, 1989; ISBN 0-19-504279-4. [Google Scholar]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. 1988, B37, 785–789. [Google Scholar] [CrossRef]
- Becke, A.D. Density-functional exchange-energy approximation with correct asymptotic behavior. Phys. Rev. 1988, A38, 3098–3100. [Google Scholar] [CrossRef]
- Jensen, F. Introduction to Computational Chemistry, 3rd ed.; John Wiley & Sons: New York, NY, USA, 2017; ISBN 978-1-118-82599-0. [Google Scholar]
- Curtiss, L.A.; Redfern, P.C.; Raghavachari, K. Gn theory. WIREs Comput. Mol. Sci. 2011, 1, 810–825. [Google Scholar] [CrossRef]
- Raczyńska, E.D. On the Basicity and π-Electron Delocalization of ‘Hexaazabenzene’ N6—Quantum-Chemical Studies. Comput. Theor. Chem. 2011, 971, 38–41. [Google Scholar] [CrossRef]
- Firsch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Montgomery, J.A., Jr.; Vreven, T.; Kudin, K.N.; Burant, J.C.; et al. Gaussian-03, Revision E.01; Gaussian, Inc.: Wallingford, CT, USA, 2004. [Google Scholar]
- Andrzejak, M.; Kubisiak, P.; Zborowski, K.K. Avoiding pitfalls of a theoretical approach: The harmonic oscillator measure of aromaticity index from quantum chemistry calculations. Struct. Chem. 2013, 24, 1171–1184. [Google Scholar] [CrossRef]
- Ostrowski, S.; Dobrowolski, J.C. What does the HOMA index really measure? RSC Adv. 2014, 4, 44158–44161. [Google Scholar] [CrossRef]
- Dobrowolski, J.C.; Ostrowski, S. On the HOMA index of some acyclic and conducting systems. RSC Adv. 2015, 5, 9467–9471. [Google Scholar] [CrossRef]
- Raczyńska, E.D.; Kamińska, B. Variations of the tautomeric preferences and π-electron delocalization for the neutral and redox forms of purine when proceeding from the gas phase (DFT) to water (PCM). J. Mol. Model. 2013, 19, 3947–3960. [Google Scholar] [CrossRef] [PubMed]
- Raczyńska, E.D.; Zientara, K.; Stępniewski, T.M.; Kolczyńska, K. Stability, polarity, intramolecular interactions and π-electron delocalization for all eighteen tautomers-rotamers of uracil. DFT studies in the gas phase. Collect. Czech. Chem. Commun. 2009, 74, 57–72. [Google Scholar] [CrossRef]
- Raczyńska, E.D.; Sapuła, M.; Zientara-Rytter, K.; Kolczyńska, K.; Stępniewski, T.M.; Hallmann, M. DFT Studies on the favored and rare tautomers of neutral and redox cytosine. Struct. Chem. 2016, 27, 133–143. [Google Scholar] [CrossRef]
- Raczyńska, E.D.; Juras, W. Effects of ionization and proton-transfer on bond length alternation in favored and rare isomers of isocytosine. Comput. Theor. Chem. 2019, 1148, 16–26. [Google Scholar] [CrossRef]
- Cyrański, M.K.; Krygowski, T.M.; Katritzky, A.R.; Schleyer, P.v.R. To what extent can aromaticity be defined uniquely? J. Org. Chem. 2002, 67, 1333–1338. [Google Scholar] [CrossRef]
- Elguero, J.; Marzin, C.; Katritzky, A.R.; Linda, P. The Tautomerism of Heterocycles, Advances in Heterocyclic Chemistry; Supplement 1; Academic Press: New York, NY, USA, 1976; ISBN 9780120206513. [Google Scholar]
- Minkin, V.I.; Garnovskii, A.D.; Elguero, J.; Katritzky, A.R.; Denisko, O.V. The tautomerism of heterocycles: Five-membered rings with two or more heteroatoms. Adv. Heterocycl. Chem. 2000, 76, 157–323. [Google Scholar] [CrossRef]
- Trifonov, R.E.; Alkorta, I.; Ostrovskii, V.A.; Elguero, J. A theoretical study of the tautomerism and ionization of 5-substituted NH-tetrazoles. J. Mol. Struct. (Theochem) 2004, 668, 123–132. [Google Scholar] [CrossRef]
- de la Hoz, A.; Sanchez-Migallon, A.; Mateo, A.C.; Prieto, P.; Infantes, L.; Elguero, J. The unusual transformation of an aromatic 1H-imidazole into a non-aromatic 2H-imidazole. Struct. Chem. 2005, 16, 485–490. [Google Scholar] [CrossRef]
- Alkorta, I.; Elguero, J.; Liebman, J.F. The annular tautomerism of imidazoles and pyrazoles: The possible existence of nonaromatic forms. Struct. Chem. 2006, 17, 439–444. [Google Scholar] [CrossRef]
- Raczyńska, E.D. Semiempirical (AM1) studies of gas-phase basicity and acidity of simple cyclic nitrogen compounds: Imidazole, 1-, 2- and 4(5)-methylimidazoles. Structural effects: Isomerism and tautomerism. Polish J. Chem. 1996, 70, 795–808. [Google Scholar]
- Raczyńska, E.D. Application of semiempirical method (AM1) to the study of tautomeric equilibria in the gas phase for simple compounds containing the amidine group: 4(5)-substituted imidazoles. Anal. Chim. Acta 1997, 348, 431–441. [Google Scholar] [CrossRef]
- Raczyńska, E.D. Quantum-chemical studies of the consequences of one-electron oxidation and one-electron reduction for imidazole in the gas phase and water. Comput. Theor. Chem. 2012, 993, 73–79. [Google Scholar] [CrossRef]
- Luque, F.J.; López-Bes, J.M.; Cemeli, J.; Aroztegui, M. Orozco, M. Solvent effects on tautomerism equilibria in heterocycles. Theor. Chem. Acc. 1997, 96, 105–113. [Google Scholar] [CrossRef]
- Kurzepa, M.; Dobrowolski, J.C.; Mazurek, A.P. Theoretical studies on tautomerism and IR spectra of C-5 substituted imidazole. J. Mol. Struct. 2001, 565–566, 107–113. [Google Scholar] [CrossRef]
- Jarończyk, M.; Dobrowolski, J.C.; Mazurek, A.P. Theoretical studies on tautomerism and IR spectra of pyrazole derivatives. J. Mol. Struct. (Theochem) 2004, 673, 17–28. [Google Scholar] [CrossRef]
- Ozimiński, W.P.; Dobrowolski, J.C.; Mazurek, A.P. DFT studies on tautomerism of C5-substituted 1,2,3-triazoles. J. Mol. Struct. 2003, 652–653, 697–704. [Google Scholar] [CrossRef]
- Ozimiński, W.P.; Dobrowolski, J.C.; Mazurek, A.P. DFT studies on tautomerism of C5-substituted 1,2,4-triazoles. J. Mol. Struct. (Theochem) 2004, 680, 107–115. [Google Scholar] [CrossRef]
- Ozimiński, W.P.; Dobrowolski, J.C. On the tautomerism, planarity, and vibrations of phospholes. Chem. Phys. 2005, 313, 123–132. [Google Scholar] [CrossRef]
- Cyrański, M.K.; Schleyer, P.v.R.; Krygowski, T.M.; Jiao, H.; Hohleicher, G. Facts and artifacts about aromatic stability estimation. Tetrahedron 2003, 59, 1657–1665. [Google Scholar] [CrossRef]
- Ramsden, C.A. The influence of aza-substitution on azole aromaticity. Tetrahedron 2010, 66, 2695–2699. [Google Scholar] [CrossRef]
- Krygowski, T.M.; Oziminski, W.; Ramsden, C.A. Sigma-and pi-electron structure of aza-azoles. J. Mol. Model. 2011, 17, 1427–1433. [Google Scholar] [CrossRef]
- Raczyńska, E.D.; Stępniewski, T.M.; Kolczyńska, K. DFT studies on one-electron oxidation and one-electron reduction for 2- and 4-amiopyridines. J. Mol. Model. 2012, 18, 4367–4380. [Google Scholar] [CrossRef] [PubMed]
- Raczyńska, E.D. Effects of positive and negative ionization for 2-aminopyrimidine in the gas phase and in water solution. Comput. Theoret. Chem. 2014, 1031, 56–63. [Google Scholar] [CrossRef]
- Raczyńska, E.D.; Kolczyńska, K.; Stępniewski, T.M. Consequences of one-electron oxidation and one-electron reduction for 4-aminopyrimidine—DFT studies. J. Mol. Model. 2012, 18, 3523–3533. [Google Scholar] [CrossRef] [PubMed]
- Raczyńska, E.D.; Kolczyńska, K.; Stępniewski, T.M.; Kamińska, B. On relation between prototropy and electron delocalization for neutral and redox adenine—DFT studies. Comput. Theor. Chem. 2013, 1022, 35–44. [Google Scholar] [CrossRef]
- Raczyńska, E.D. Electron delocalization and relative stabilities for the favored and rare tautomers of hydroxyazines in the gas phase—A comparison with aminoazines. Comput. Theor. Chem. 2014, 1042, 8–15. [Google Scholar] [CrossRef]
- Nyulászi, L. Effects of substituents on the aromatization of phosphole. J. Phys. Chem. 1995, 99, 586–591. [Google Scholar] [CrossRef]
- Nyulászi, L. Pentaphosphole: An aromatic ring with a planar σ3-phosphorus. Inorg. Chem. 1996, 35, 4690–4693. [Google Scholar] [CrossRef]
- Nyulászi, L. Toward a planar σ3-phosphorus. J. Phys. Chem. 1996, 100, 6194–6198. [Google Scholar] [CrossRef]
- Glukhovtsev, M.N.; Dransfeld, A.; Schleyer, P.v.R. Why pentaphosphole, P5H, is planar in contrast to phosphole, (CH)4PH? J. Phys. Chem. 1996, 100, 13447–13454. [Google Scholar] [CrossRef]
- Dransfeld, A.; Nyulászi, L.; Schleyer, P.v.R. The aromaticity of polyphosphaphospholes decreases with the pyramidality of the tricoordinate phosphorus. Inorg. Chem. 1998, 37, 4413–4420. [Google Scholar] [CrossRef] [PubMed]
- Nyulászi, L. Aromatic compounds with planar tricoordinate phosphorus. Tetrahedron 2000, 56, 79–84. [Google Scholar] [CrossRef]
- Nyulászi, L. Aromaticity of phosphorus heterocycles. Chem. Rev. 2001, 101, 1229–1246. [Google Scholar] [CrossRef] [PubMed]
- Nyulászi, L.; Hollóczki, O.; Lescop, C.; Hissler, M.; Réau, R. An aromatic–antiaromatic switch in P-heteroles. A small change in delocalisation makes a big reactivity difference. Org. Biomol. Chem. 2006, 4, 996–998. [Google Scholar] [CrossRef]
- Pelloni, S.; Lazzeretti, P. Magnetotropicity of phosphole and its arsenic analogue. Theor. Chem. Acc. 2007, 118, 89–97. [Google Scholar] [CrossRef]
- Chesnut, D.B.; Quin, L.D. The important role of the phosphorus lone pair in phosphole aromaticity. Heteroatom Chem. 2007, 18, 754–758. [Google Scholar] [CrossRef]
- Wang, L.; Wang, H.J.; Dong, W.B.; Ge, Q.Y.; Lin, L. Using three major criteria to evaluate aromaticity of five-member C-containing rings and their Si-, N-, and P-substituted aromatic heterocyclics. Struct. Chem. 2007, 18, 25–31. [Google Scholar] [CrossRef]
- Vessally, E. Aromatic stability energy studies on five-membered heterocyclic C4H4M (M = O, S, Se, Te, NH, PH, AsH AND SbH): DFT calculations. J. Struct. Chem. 2008, 49, 979–985. [Google Scholar] [CrossRef]
- Josa, D.; Peña-Gallego, A.; Rodríguez-Otero, J.; Cabaleiro-Lago, E. A MP2 and DFT study of the aromatic character of polyphosphaphospholes. Is the pyramidality the only factor to take into consideration? J. Mol. Model. 2011, 17, 1267–1272. [Google Scholar] [CrossRef] [PubMed]
- Zagidullin, A.A.; Bezkishko, I.A.; Miluykov, V.A.; Sinyashin, O.G. Phospholes—Development and recent advances. Mendeleev Commun. 2013, 23, 117–130. [Google Scholar] [CrossRef]
- Quin, L.D.; Keglevich, G.; Ionkin, A.S.; Kalgutkar, R.; Szalontai, G. Phospholes with reduced pyramidal character from steric crowding. I. Synthesis and NMR characterization of 1-(2,4-di-tert-butyl-6-methyl)phenyl-3-methylphosphole. J. Org. Chem. 1996, 61, 7801–7807. [Google Scholar] [CrossRef]
- Nyulászi, L.; Keglevich, G.; Quin, L.D. Phospholes with reduced pyramidal character from steric crowding. II. Photoelectron spectral evidence for electron delocalization in 1-(2,4-di-tert-butyl-6-methylphenyl)-3-methylphosphole. J. Org. Chem. 1966, 61, 7808–7812. [Google Scholar] [CrossRef]
- Keglevich, G.; Quin, L.D.; Böcskei, Z.; Keserű, G.M.; Kalgutkar, R.; Lahti, P.M. Phospholes with reduced pyramidal character from steric crowding. III. NMR and X-ray diffraction studies on 1-(2,4,6-tri-isopropylphenyl)-3-methylphosphole. J. Organomet. Chem. 1997, 532, 109–116. [Google Scholar] [CrossRef]
- Ozimiński, W. Tautomeric equilibria and aromaticity of phosphodiazoles: An ab initio study. Comput. Theor. Chem. 2012, 980, 92–100. [Google Scholar] [CrossRef]

| Bond | Molecule | Rs | Molecule | Rd | Molecule | Ro |
|---|---|---|---|---|---|---|
| CC | H3C–CH3 | 1.5300 1 | H2C=CH2 | 1.3288 1 |  | 1.3943 1 |
| CN | H3C–NH2 | 1.4658 1 | H2C=NH | 1.2670 1 | 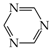 | 1.3342 1 |
| CP | H3C−PH2 | 1.8729 2 | H2C=PH | 1.6704 2 | 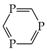 | 1.7364 2 |
| CO | H3C–OH | 1.4238 1 | H2C=O | 1.2017 1 | (HO)2C=OH+ | 1.2811 1 |
| CS | H3C−SH | 1.8352 2 | H2C=S | 1.6154 2 | H3C–C(SH)=SH+ | 1.6975 2 |
| NN | 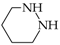 | 1.4742 2 | 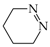 | 1.2348 2 |  | 1.3193 3 |
| NP |  | 1.7747 2 | 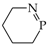 | 1.5751 2 | 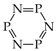 | 1.6398 2,4 |
| NO | 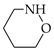 | 1.4510 2 | H3C−N=O | 1.2019 2 | 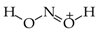 | 1.2605 2 |
| NS |  | 1.7616 2 | H3C−N=S | 1.5759 2 | 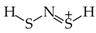 | 1.6123 2 |
| PP | 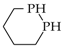 | 2.2568 2 | 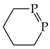 | 2.0406 2 | 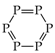 | 2.1332 2,4 |
| PO | 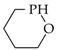 | 1.6942 2 | H3C−P=O | 1.4975 2 | 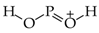 | 1.5635 2 |
| PS | 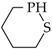 | 2.1778 2 | H3C−P=S | 1.9483 2 | 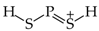 | 2.0496 2 |
| Bond | rHOMA | HOMED | |||
|---|---|---|---|---|---|
| α1 | α2i2 | α1d + 2s3 | α2d + 3s3 | α3d + 4s3 | |
| CC | 257.7 4 | 88.09 5 | 72.96 5 | 78.34 5 | 80.90 5 |
| CN | 93.52 4 | 91.60 5 | 76.62 6 | 81.98 5 | 84.52 5 |
| CP | 118.91 4 | 87.00 7 | 72.08 7 | 77.39 7 | 79.91 7 |
| CO | 157.38 4 | 75.00 5 | 63.79 5 | 67.84 5 | 69.74 5 |
| CS | 94.09 4 | 77.82 7 | 67.17 7 | 71.06 7 | 72.87 7 |
| NN | 130.33 4 | 64.24 7 | 54.42 7 | 57.96 7 | 59.63 7 |
| NP | - | 89.35 7 | 73.92 7 | 79.41 7 | 82.01 7 |
| NO | 57.21 4 | 50.35 7 | 39.47 7 | 43.20 7 | 45.03 6 |
| NS | - | 84.69 7 | 65.35 7 | 71.92 7 | 75.16 7 |
| PP | - | 83.85 7 | 76.67 7 | 79.39 7 | 80.62 7 |
| PO | - | 93.29 7 | 77.88 7 | 83.39 7 | 86.00 7 |
| PS | - | 74.91 7 | 69.55 7 | 71.60 7 | 72.52 7 |
| Structure | HOMED | Structure | HOMED | Structure | HOMED | Structure | HOMED |
|---|---|---|---|---|---|---|---|
 | 0.749 1 | 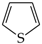 | 0.922 |  | 0.749 |  | 0.922 |
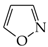 | 0.743 |  | 0.902 | 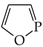 | 0.571 | 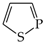 | 0.887 |
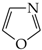 | 0.702 1 | 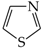 | 0.884 | 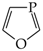 | 0.760 | 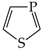 | 0.925 |
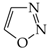 | 0.494 | 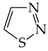 | 0.629 | 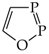 | 0.543 | 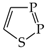 | 0.862 |
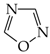 | 0.706 | 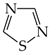 | 0.876 | 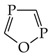 | 0.596 | 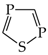 | 0.896 |
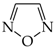 | 0.752 | 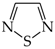 | 0.905 | 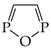 | 0.579 | 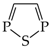 | 0.888 |
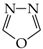 | 0.678 | 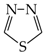 | 0.868 | 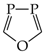 | 0.797 | 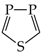 | 0.951 |
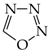 | 0.504 | 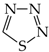 | 0.690 | 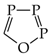 | 0.543 | 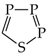 | 0.873 |
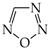 | 0.622 |  | 0.774 | 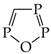 | 0.561 | 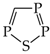 | 0.862 |
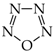 | 0.524 | 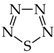 | 0.686 | 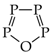 | 0.548 | 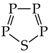 | 0.815 |
| Structure | ΔG | HOMED | Structure | ΔG | HOMED | Structure | ΔG | HOMED |
|---|---|---|---|---|---|---|---|---|
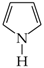 | 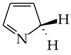 | 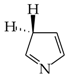 | ||||||
| 0.0 1 | 0.921 1 | 14.9 1 | 0.398 1 | 16.6 1 | 0.413 1 | |||
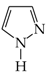 | 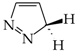 | 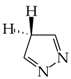 | ||||||
| 0.0 | 0.972 | 27.1 | 0.285 | 23.0 | 0.391 | |||
 | 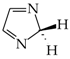 |  | ||||||
| 0.0 1 | 0.903 1 | 16.8 1 | 0.320 1 | 16.6 1 | 0.337 1 | |||
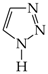 | 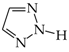 | 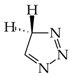 | ||||||
| 4.2 | 0.956 | 0.0 | 0.996 | 27.9 | 0.131 | |||
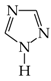 | 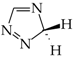 |  | ||||||
| 0.0 | 0.960 | 28.5 | 0.165 | 6.2 | 0.888 | |||
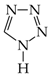 |  | 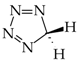 | ||||||
| 2.4 | 0.943 | 0.0 | 0.988 | not stable | not stable | |||
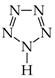 | ||||||||
| 0.0 | 0.971 | |||||||
| Structure | ΔG | HOMED | Structure | ΔG | HOMED | Structure | ΔG | HOMED |
|---|---|---|---|---|---|---|---|---|
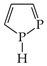
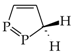
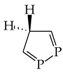
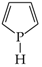 | 2.2 1 | 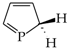 | 0.0 1 | 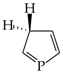 | 4.1 1 | |||
| 2.2 2 | 0.694 1 | 0.0 2 | 0.440 1 | 3.1 2 | 0.448 1 | |||
| 2.2 3 | 0.0 3 | 3.1 3 | ||||||
| 2.1 4 | 0.0 4 | 3.1 4 | ||||||
 |  |  | ||||||
| 12.0 1 | 0.775 1 | 0.0 1 | 0.350 1 | 8.4 1 | 0.390 1 | |||
| 3.0 4 | 0.0 4 | 8.0 4 | ||||||
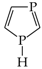 |  | 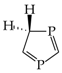 | ||||||
| 5.1 1 | 0.722 1 | 0.0 1 | 0.459 1 | 5.0 1 | 0.433 1 | |||
| 4.3 4 | 0.0 4 | 4.0 4 | ||||||
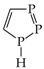 |  | 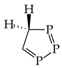 | ||||||
| 0.1 1 | 0.769 1 | 0.0 1 | 0.927 1 | 3.2 1 | 0.292 1 | |||
| 0.0 4 | 0.6 4 | 2.8 4 | ||||||
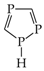 |  | 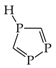 | ||||||
| 4.4 1 | 0.846 1 | 0.0 1 | 0.364 1 | 6.6 1 | 0.755 1 | |||
| 4.3 4 | 0.0 4 | 6.3 4 | ||||||
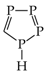 |  | 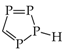 | ||||||
| 2.7 1 | 0.850 1 | 0.3 1 | 0.177 1 | 0.0 1 | 0.939 1 | |||
| 1.7 4 | 0.3 4 | 0.0 4 | ||||||
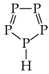 | ||||||||
| 0.0 1 | 0.936 1 | |||||||
| Structure | ΔG | HOMED | Structure | ΔG | HOMED |
|---|---|---|---|---|---|
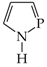 | 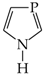 | ||||
| 0.0 | 0.851 | 0.0 | 0.921 | ||
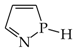 | 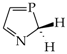 | ||||
| 21.1 | 0.635 | 15.7 | 0.336 | ||
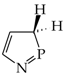 | 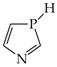 | ||||
| 24.5 | 0.435 | 19.6 | 0.598 | ||
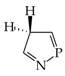 | 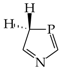 | ||||
| 23.9 | 0.275 | 17.0 | 0.423 | ||
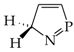 | 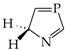 | ||||
| 26.5 | 0.415 | 19.6 | 0.285 | ||
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Raczyńska, E.D. Application of the Extended HOMED (Harmonic Oscillator Model of Aromaticity) Index to Simple and Tautomeric Five-Membered Heteroaromatic Cycles with C, N, O, P, and S Atoms. Symmetry 2019, 11, 146. https://doi.org/10.3390/sym11020146
Raczyńska ED. Application of the Extended HOMED (Harmonic Oscillator Model of Aromaticity) Index to Simple and Tautomeric Five-Membered Heteroaromatic Cycles with C, N, O, P, and S Atoms. Symmetry. 2019; 11(2):146. https://doi.org/10.3390/sym11020146
Chicago/Turabian StyleRaczyńska, Ewa D. 2019. "Application of the Extended HOMED (Harmonic Oscillator Model of Aromaticity) Index to Simple and Tautomeric Five-Membered Heteroaromatic Cycles with C, N, O, P, and S Atoms" Symmetry 11, no. 2: 146. https://doi.org/10.3390/sym11020146
APA StyleRaczyńska, E. D. (2019). Application of the Extended HOMED (Harmonic Oscillator Model of Aromaticity) Index to Simple and Tautomeric Five-Membered Heteroaromatic Cycles with C, N, O, P, and S Atoms. Symmetry, 11(2), 146. https://doi.org/10.3390/sym11020146