Equilibrium of Two-Dimensional Cycloidal Pantographic Metamaterials in Three-Dimensional Deformations
Abstract
1. Introduction
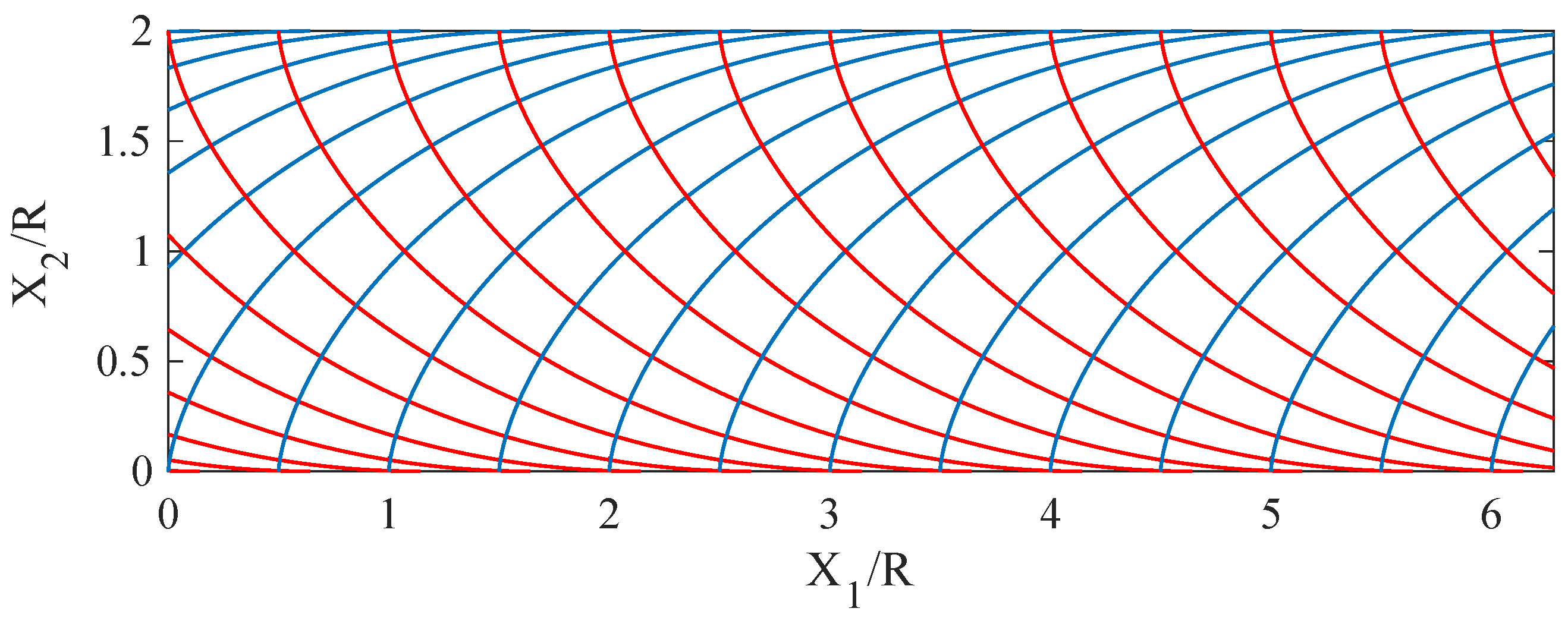
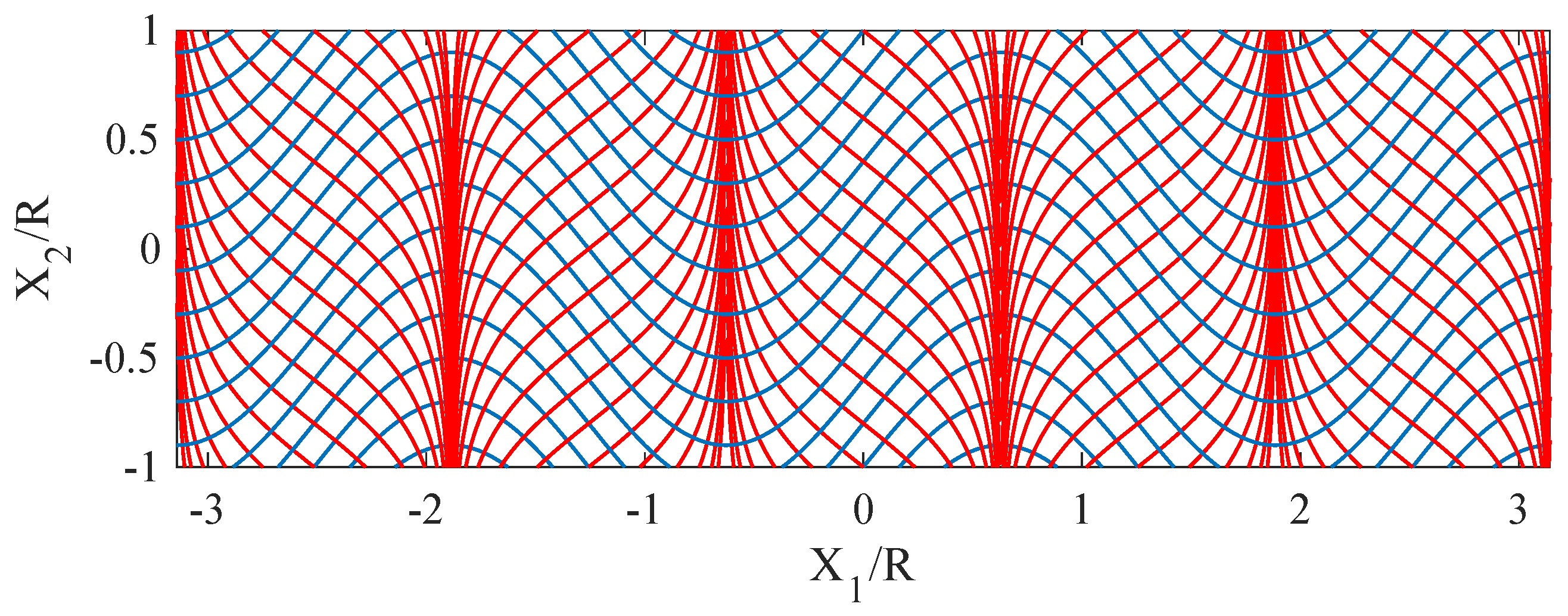
2. Pantographic Sheets with Initially Cycloidal Fibers
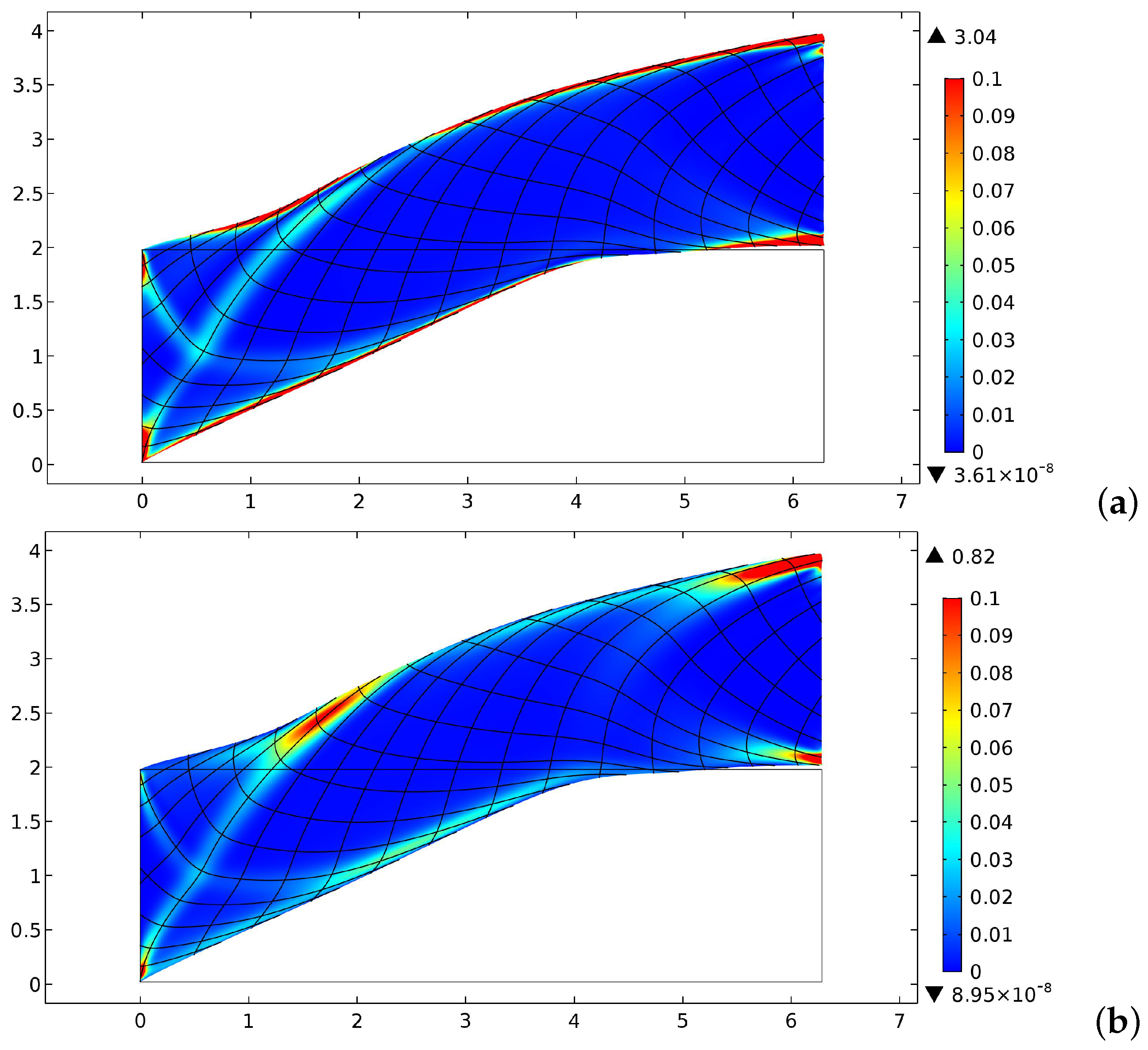
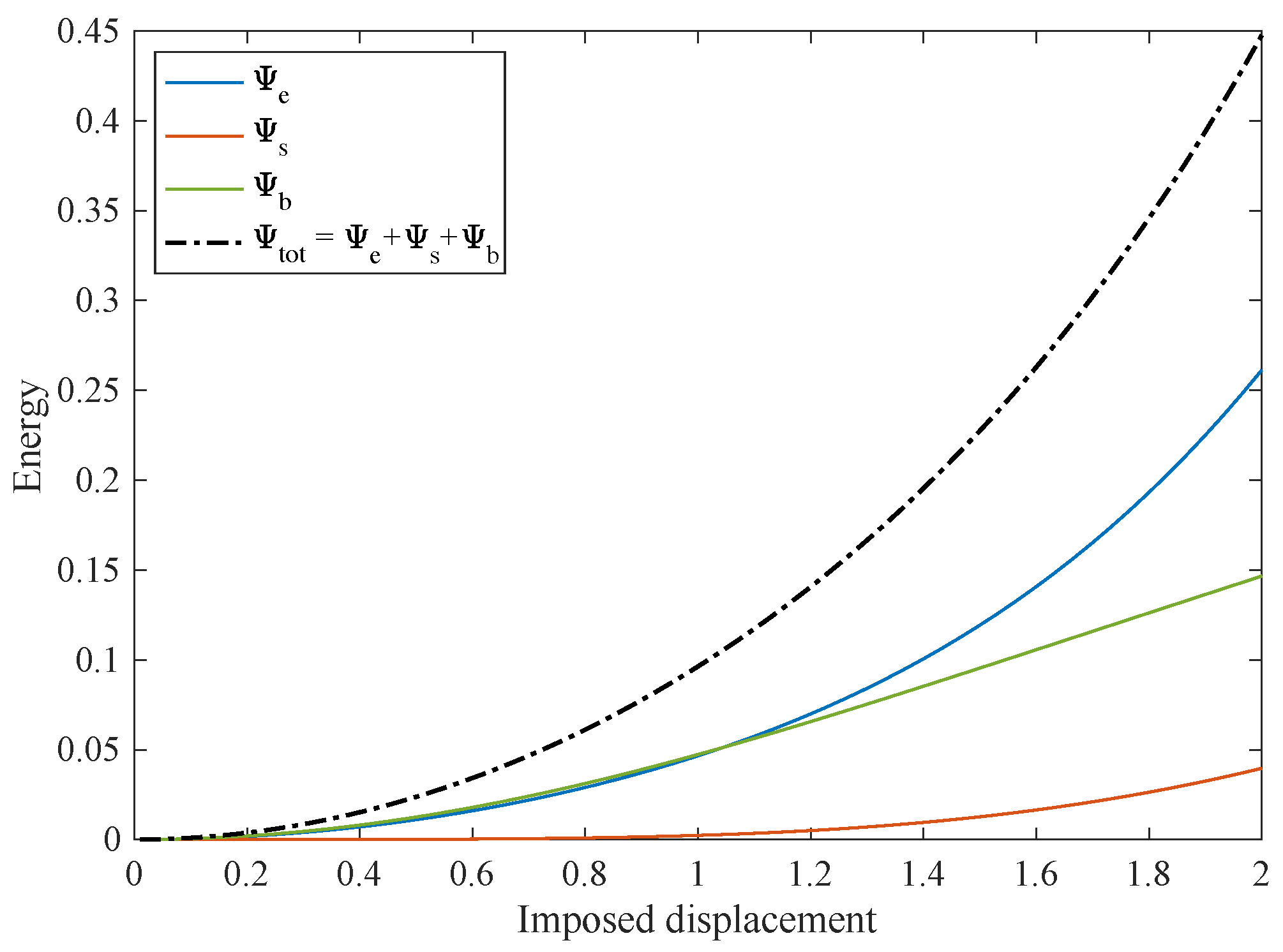
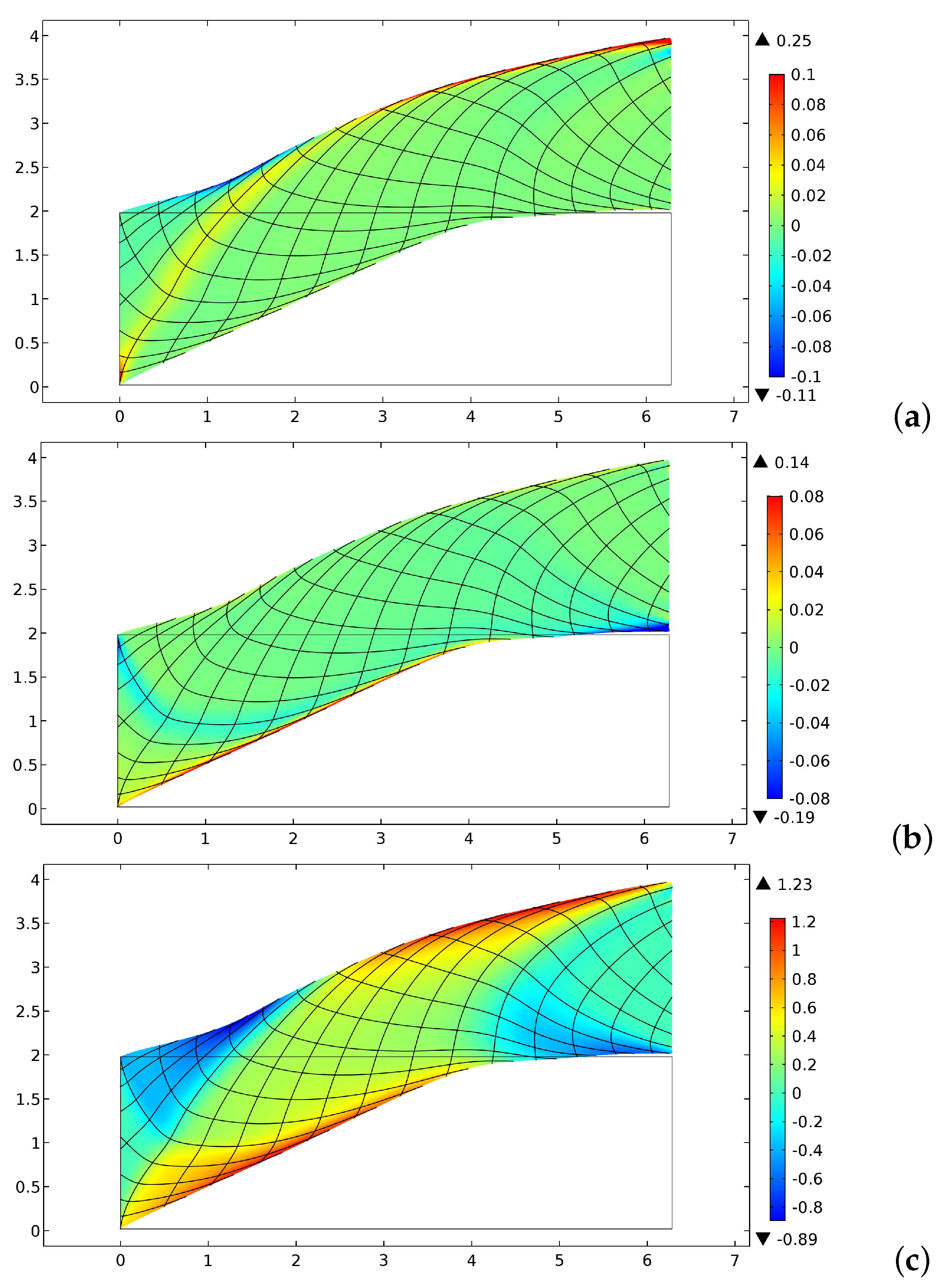
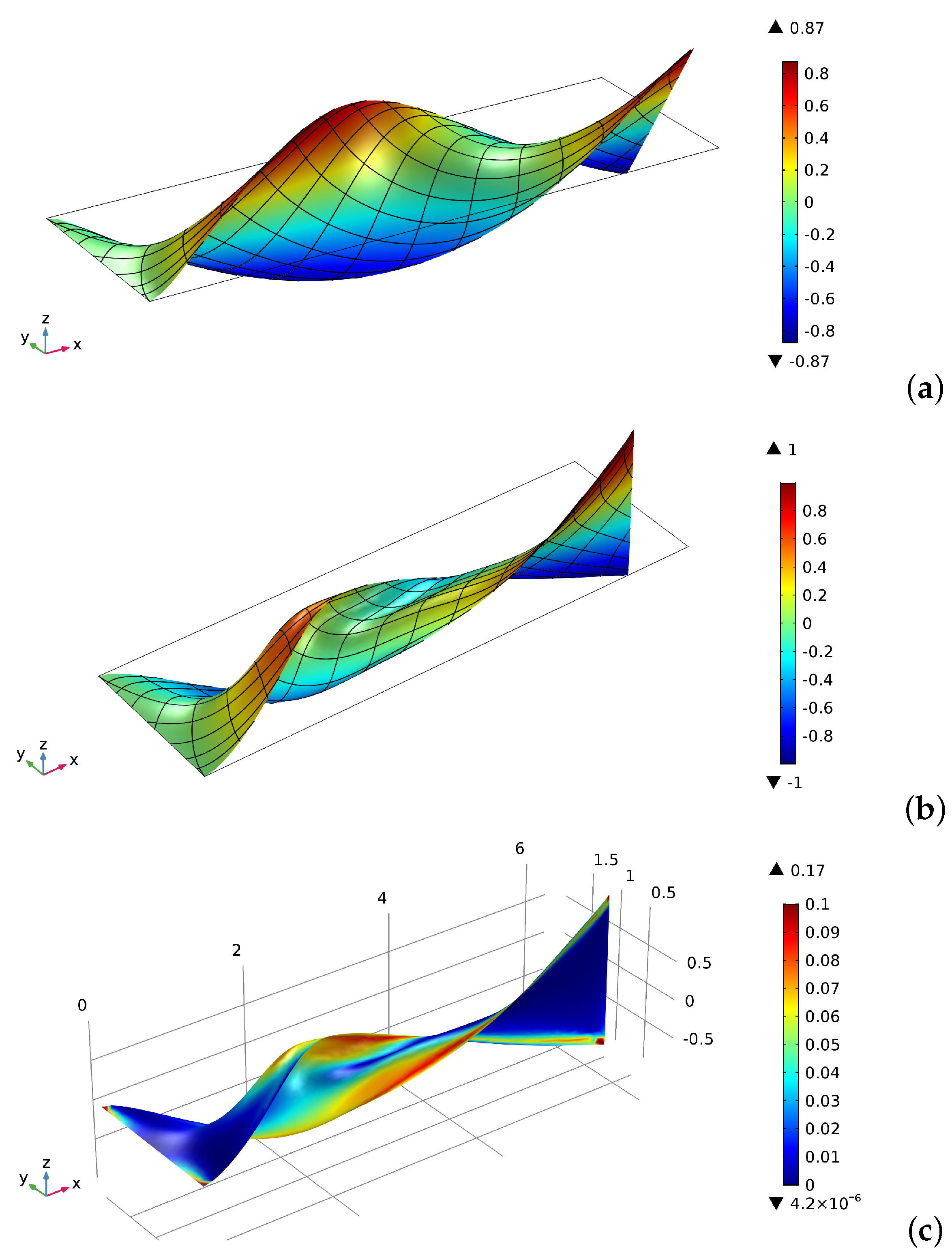
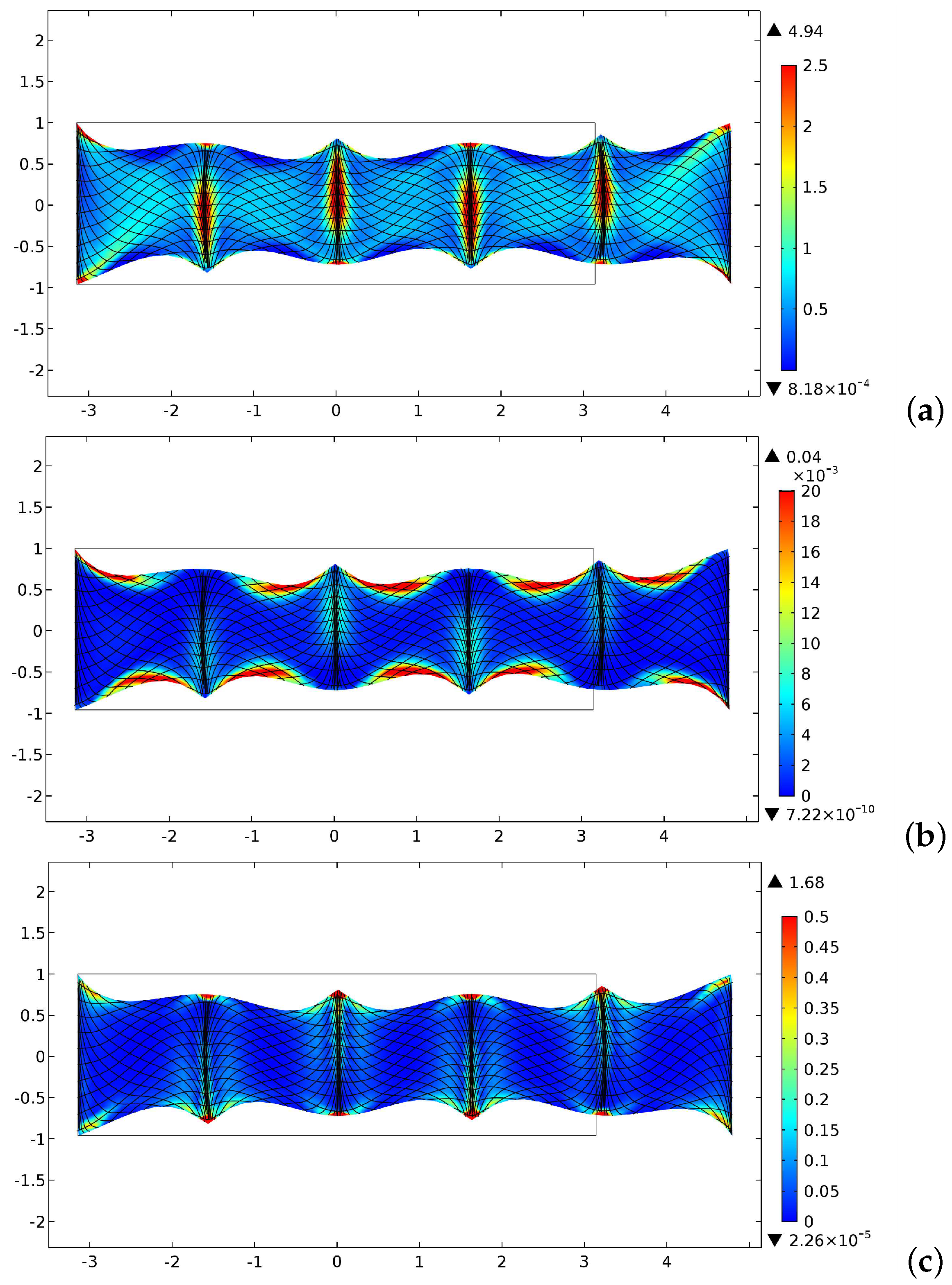
3. Numerical Examples of Equilibrium Shapes
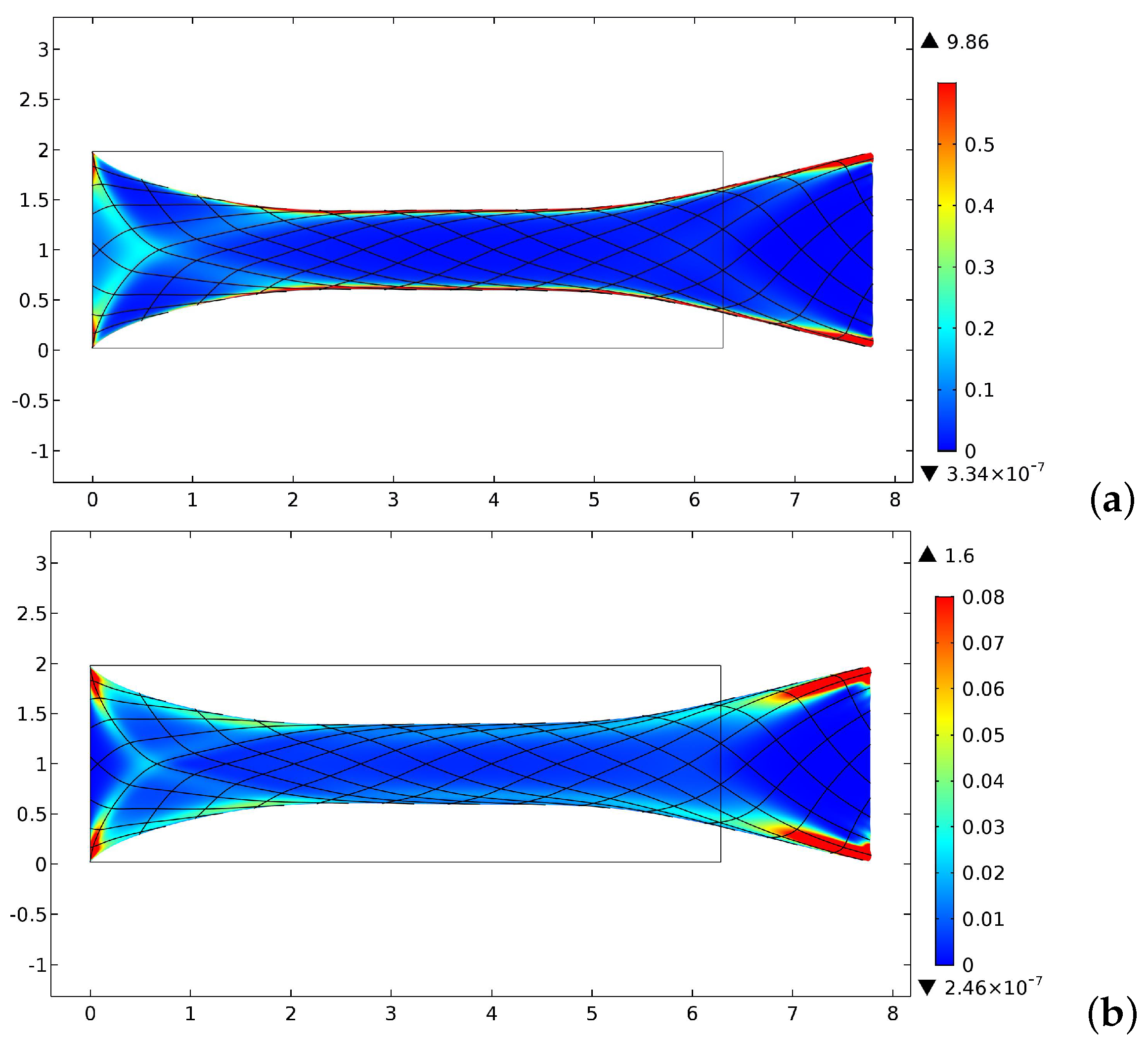
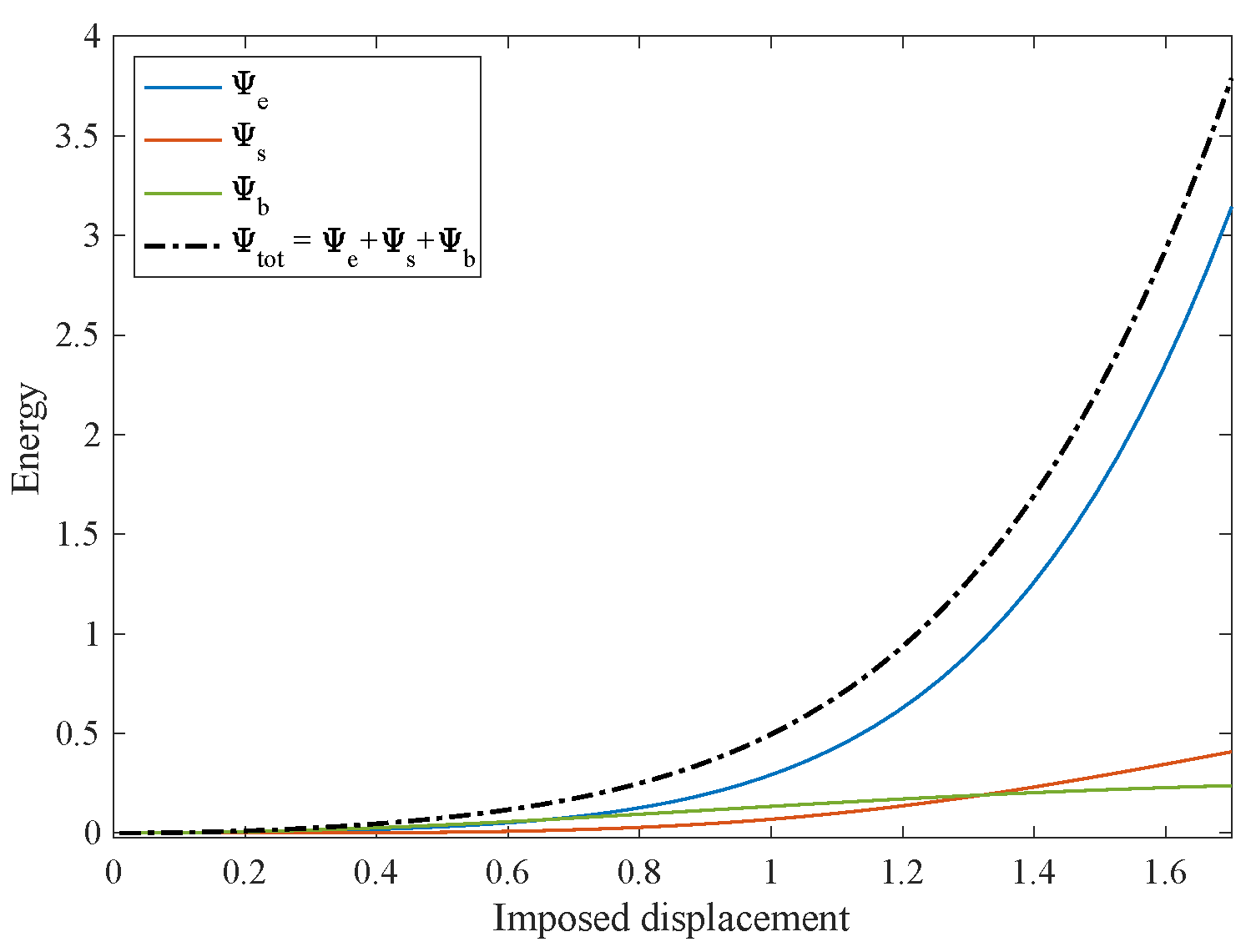
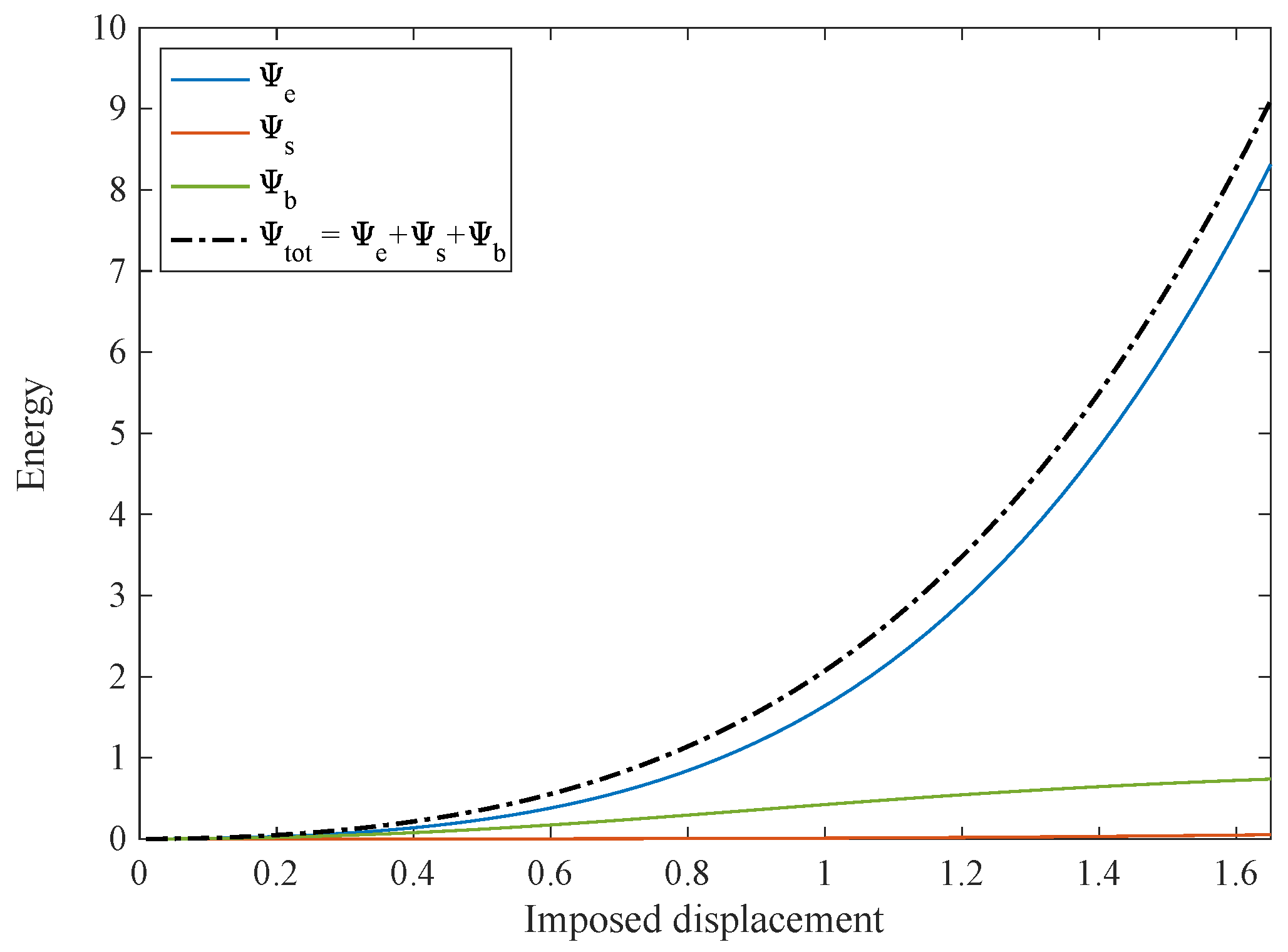
3.1. Bias Extension Test
3.2. Bias Compression Test
3.3. Shear Test
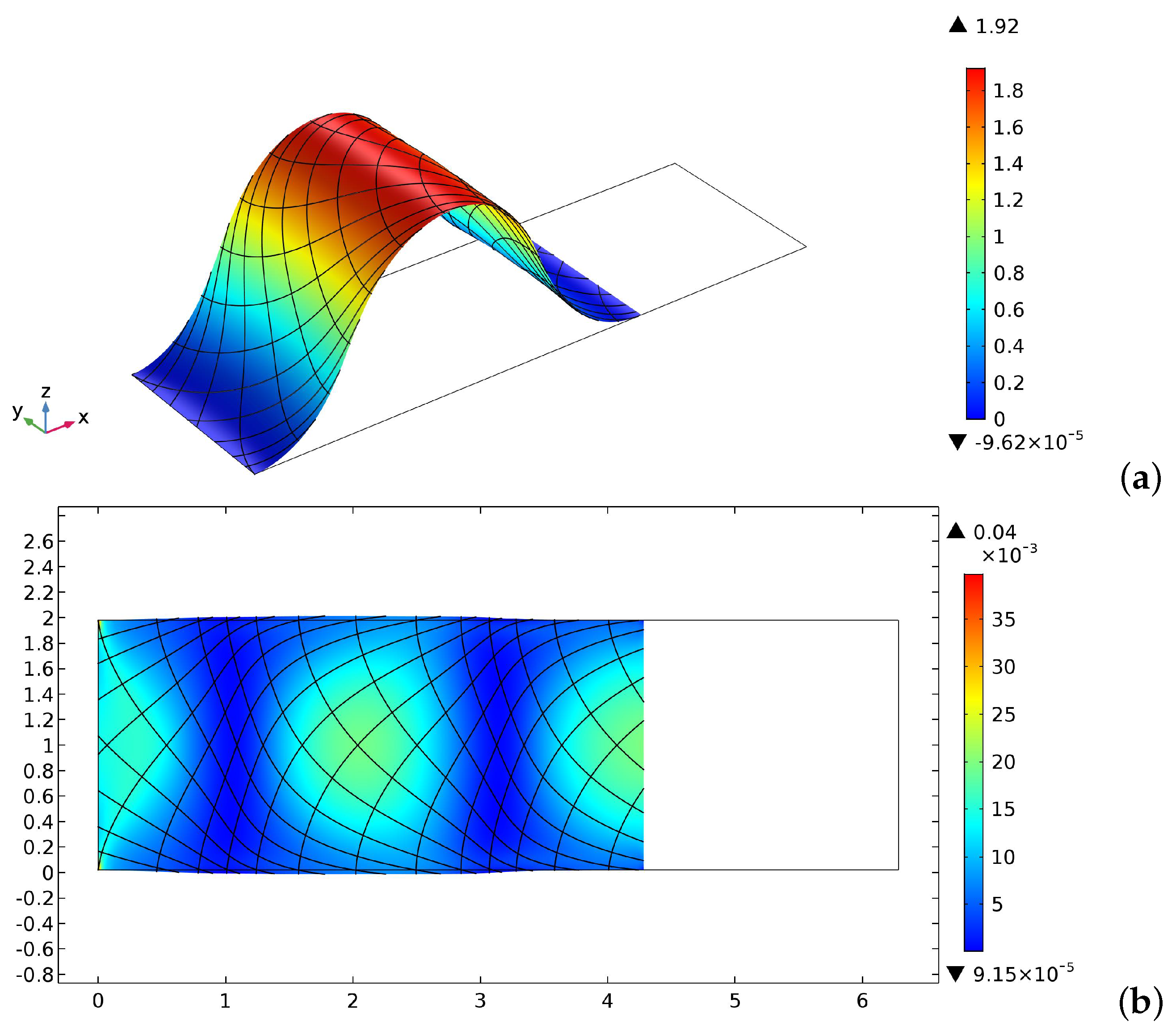
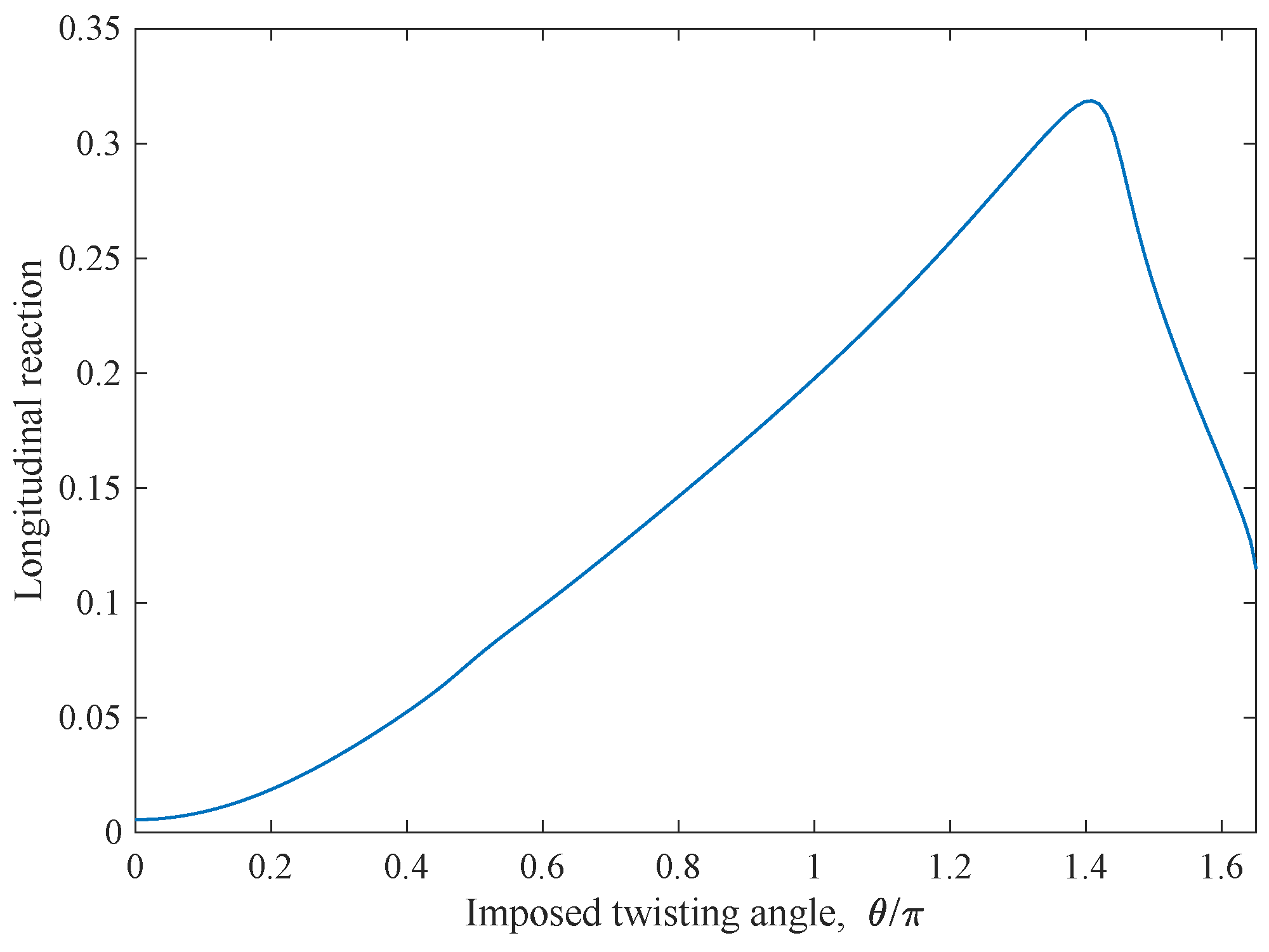
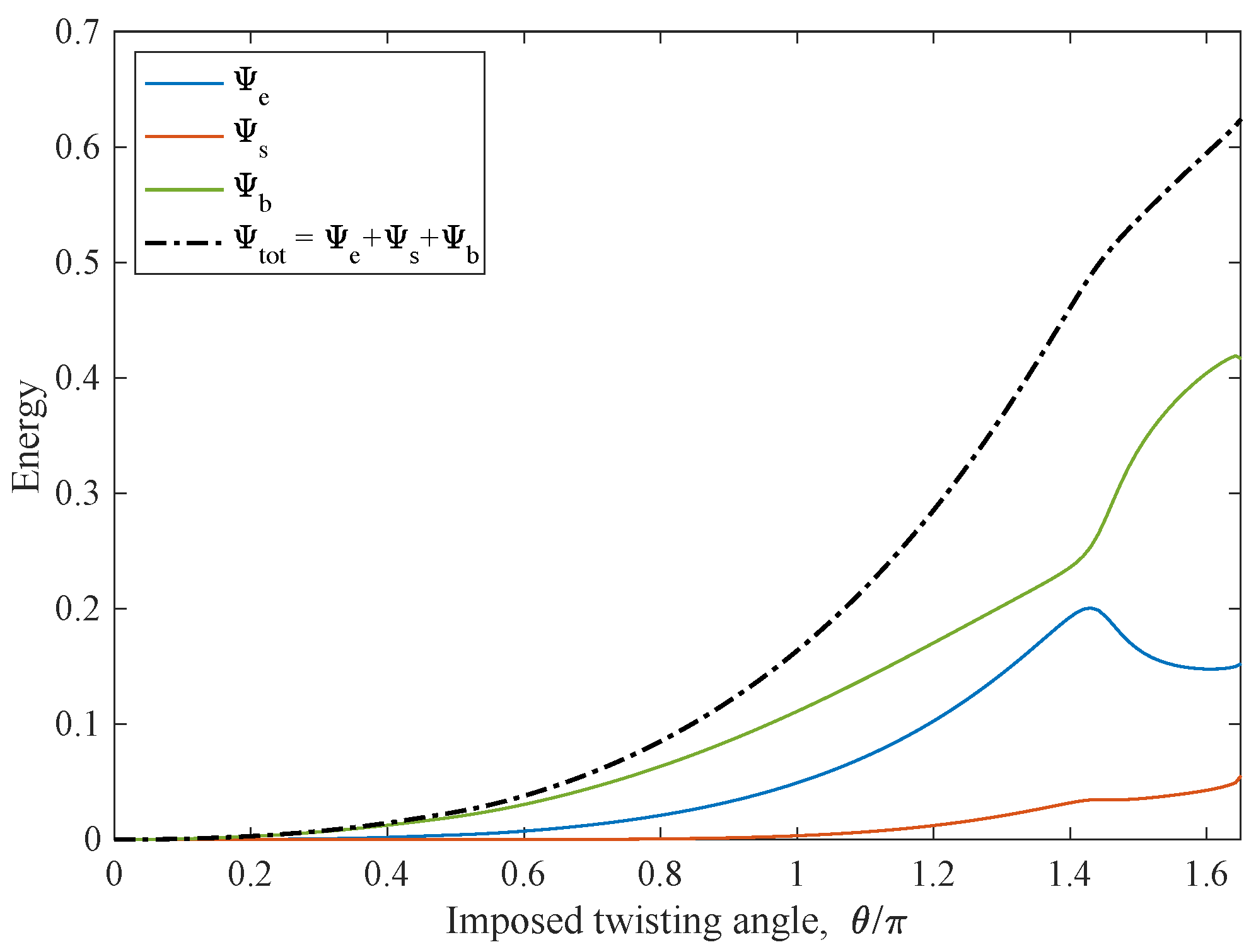
3.4. Torsion Test
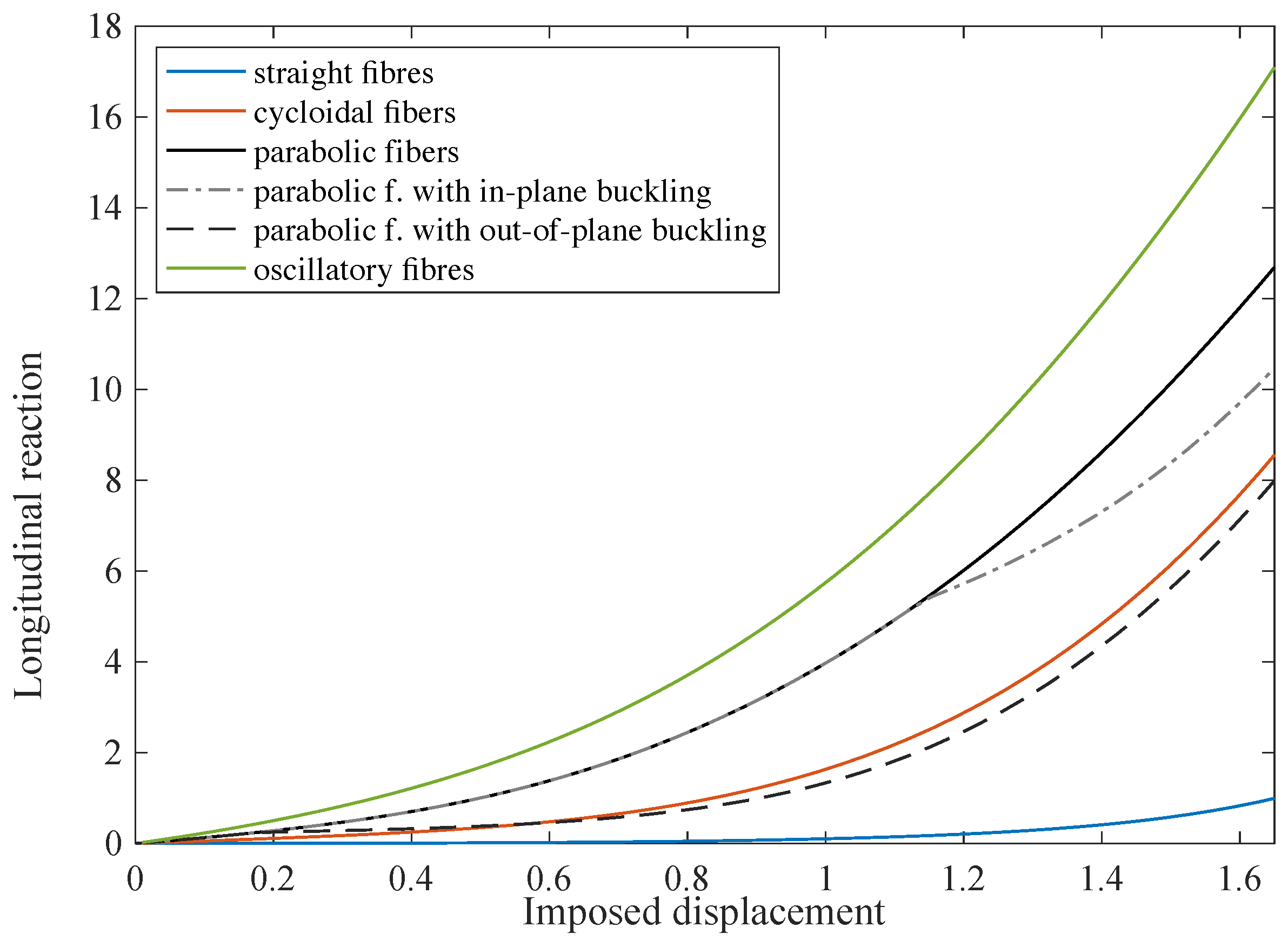
4. Comparison among the Pantographic Sheets with Orthogonal Lattices
Author Contributions
Acknowledgments
Conflicts of Interest
References
- El Sherbiny, M.G.; Placidi, L. Discrete and continuous aspects of some metamaterial elastic structures with band gaps. Arch. Appl. Mech. 2018, 88, 1725–1742. [Google Scholar] [CrossRef]
- Nejadsadeghi, N.; Placidi, L.; Romeo, M.; Misra, A. Frequency band gaps in dielectric granular metamaterials modulated by electric field. Mech. Res. Commun. 2019, 95, 96–103. [Google Scholar] [CrossRef]
- Vangelatos, Z.; Komvopoulos, K.; Grigoropoulos, C.P. Vacancies for controlling the behavior of microstructured three-dimensional mechanical metamaterials. Math. Mech. Solids 2019, 24, 511–524. [Google Scholar] [CrossRef]
- Laudato, M.; De Angelo, M. Workshop on Encounter of the third kind on Generalized continua and microstructures in Arpino, 3–7 April 2018: A review of presentations and discussions. Math. Mech. Solids 2019, 25, 117–126. [Google Scholar] [CrossRef]
- Barchiesi, E.; Spagnuolo, M.; Placidi, L. Mechanical metamaterials: A state of the art. Math. Mech. Solids 2019, 24, 212–234. [Google Scholar] [CrossRef]
- Yildizdag, M.E.; Tran, C.A.; Barchiesi, E.; Spagnuolo, M.; dell’Isola, F.; Hild, F. A Multi-disciplinary Approach for Mechanical Metamaterial Synthesis: A Hierarchical Modular Multiscale Cellular Structure Paradigm. In State of the Art and Future Trends in Material Modeling; Advanced Structured Materials; Altenbach, H.O.A., Ed.; Springer: Cham, Switzerland, 2019; Volume 100, pp. 485–505. [Google Scholar]
- Milton, G.; Briane, M.; Harutyunyan, D. On the possible effective elasticity tensors of 2-dimensional and 3-dimensional printed materials. Math. Mech. Complex Syst. 2017, 5, 41–94. [Google Scholar] [CrossRef]
- Placidi, L.; Barchiesi, E.; Turco, E.; Rizzi, N.L. A review on 2D models for the description of pantographic fabrics. Z. Angew. Math. Phys. 2016, 67, 121. [Google Scholar] [CrossRef]
- Barchiesi, E.; Placidi, L. A review on models for the 3D statics and 2D dynamics of pantographic fabrics. In Wave Dynamics and Composite Mechanics for Microstructured Materials and Metamaterials; Advanced Structured Materials; Sumbatyan, M., Ed.; Springer: Singapore, 2017; Volume 59, pp. 239–258. [Google Scholar]
- Cuomo, M.; dell’Isola, F.; Greco, L.; Rizzi, N.L. First versus second gradient energies for planar sheets with two families of inextensible fibres: Investigation on deformation boundary layers, discontinuities and geometrical instabilities. Compos. Part Eng. 2017, 115, 423–448. [Google Scholar] [CrossRef]
- De Angelo, M.; Spagnuolo, M.; D’Annibale, F.; Pfaff, A.; Hoschke, K.; Misra, A.; Dupuy, C.; Peyre, P.; Dirrenberger, J.; Pawlikowski, M. The macroscopic behavior of pantographic sheets depends mainly on their microstructure: Experimental evidence and qualitative analysis of damage in metallic specimens. Contin. Mech. Thermodyn. 2019, 31, 1181–1203. [Google Scholar] [CrossRef]
- Cuomo, M.; dell’Isola, F.; Greco, L. Simplified analysis of a generalized bias test for fabrics with two families of inextensible fibres. Z. Angew. Math. Phys. 2016, 67, 61. [Google Scholar] [CrossRef]
- Spagnuolo, M.; Barcz, K.; Pfaff, A.; dell’Isola, F.; Franciosi, P. Qualitative pivot damage analysis in aluminum printed pantographic sheets: Numerics and experiments. Mech. Res. Commun. 2017, 83, 47–52. [Google Scholar] [CrossRef]
- Barchiesi, E.; Ganzosch, G.; Liebold, C.; Placidi, L.; Grygoruk, R.; Müller, W.H. Out-of-plane buckling of pantographic fabrics in displacement-controlled shear tests: Experimental results and model validation. Contin. Mech. Thermodyn. 2019, 31, 33–45. [Google Scholar] [CrossRef]
- Turco, E.; Golaszewski, M.; Cazzani, A.; Rizzi, N.L. Large deformations induced in planar pantographic sheets by loads applied on fibers: Experimental validation of a discrete Lagrangian model. Mech. Res. Commun. 2016, 76, 51–56. [Google Scholar] [CrossRef]
- Alibert, J.J.; Seppecher, P.; dell’Isola, F. Truss modular beams with deformation energy depending on higher displacement gradients. Math. Mech. Solids 2003, 8, 51–73. [Google Scholar] [CrossRef]
- Dell’Isola, F.; Lekszycki, T.; Pawlikowski, M.; Grygoruk, R.; Greco, L. Designing a light fabric metamaterial being highly macroscopically tough under directional extension: First experimental evidence. Z. Angew. Math. Phys. 2015, 66, 3473–3498. [Google Scholar] [CrossRef]
- Dell’Isola, F.; Andreaus, U.; Placidi, L. At the origins and in the vanguard of peridynamics, non-local and higher-gradient continuum mechanics: An underestimated and still topical contribution of Gabrio Piola. Math. Mech. Solids 2015, 20, 887–928. [Google Scholar] [CrossRef]
- Franciosi, P.; Spagnuolo, M.; Salman, O.U. Mean Green operators of deformable fiber networks embedded in a compliant matrix and property estimates. Contin. Mech. Thermodyn. 2019, 31, 101–132. [Google Scholar] [CrossRef]
- Spagnuolo, M.; Franciosi, P.; dell’Isola, F. A Green operator-based elastic modeling for two-phase pantographic-inspired bi-continuous materials. Int. J. Solids Struct. 2019. [Google Scholar] [CrossRef]
- Avella, M.; Casale, L.; Dell’Erba, R.; Martuscelli, E. Broom fibers as reinforcement for thermoplastic matrices. In Macromolecular Symposia; Hüthig & Wepf Verlag: Basel, Switzerland, 1998. [Google Scholar]
- Avella, M.; Dell’Erba, R.; Martuscelli, E. Fiber reinforced polypropylene: Influence of iPP molecular weight on morphology, crystallization, and thermal and mechanical properties. Polym. Compos. 1996, 17, 288–299. [Google Scholar] [CrossRef]
- Haseganu, E.M.; Steigmann, D.J. Equilibrium analysis of finitely deformed elastic networks. Comput. Mech. 1996, 17, 359–373. [Google Scholar] [CrossRef]
- Atai, A.A.; Steigmann, D.J. On the nonlinear mechanics of discrete networks. Arch. Appl. Mech. 1997, 67, 303–319. [Google Scholar] [CrossRef]
- Steigmann, D.J. Continuum theory for elastic sheets formed by inextensible crossed elasticae. Int. J. Non-Linear Mech. 2018, 106, 324–329. [Google Scholar] [CrossRef]
- Eugster, S.; dell’Isola, F.; Steigmann, D.J. Continuum theory for mechanical metamaterials with a cubic lattice substructure. Math. Mech. Complex Syst. 2019, 7, 75–98. [Google Scholar] [CrossRef]
- Eremeyev, V.A. Two-and three-dimensional elastic networks with rigid junctions: Modeling within the theory of micropolar shells and solids. Acta Mech. 2019, 230, 3875–3887. [Google Scholar] [CrossRef]
- Eremeyev, V.A. A Nonlinear Model of a Mesh Shell. Mech. Solids 2018, 53, 464–469. [Google Scholar] [CrossRef]
- Boutin, C.; Hans, S.; Chesnais, C. Generalized beams and continua. Dynamics of reticulated structures. In Mechanics of Generalized Continua; Advances in Mechanics and Mathematics; Maugin, G., Metrikine, A., Eds.; Springer: New York, NY, USA, 2010; Volume 21, pp. 131–141. [Google Scholar]
- Pideri, C.; Seppecher, P. A second gradient material resulting from the homogenization of an heterogeneous linear elastic medium. Contin. Mech. Thermodyn. 1997, 9, 241–257. [Google Scholar] [CrossRef]
- Abdoul-Anziz, H.; Seppecher, P.; Bellis, C. Homogenization of frame lattices leading to second gradient models coupling classical strain and strain-gradient terms. Math. Mech. Solids 2019, 24, 3976–3999. [Google Scholar] [CrossRef]
- Abdoul-Anziz, H.; Seppecher, P. Strain gradient and generalized continua obtained by homogenizing frame lattices. Math. Mech. Complex Syst. 2018, 6, 213–250. [Google Scholar] [CrossRef]
- Berrehili, Y.; Marigo, J.J. The homogenized behavior of unidirectional fiber-reinforced composite materials in the case of debonded fibers. Math. Mech. Complex Syst. 2014, 2, 181–207. [Google Scholar] [CrossRef]
- Placidi, L.; dell’Isola, F.; Barchiesi, E. Heuristic Homogenization of Euler and Pantographic Beams. In Mechanics of Fibrous Materials and Applications; CISM International Centre for Mechanical Sciences (Courses and Lectures); Picu, C., Ganghoffer, J.F., Eds.; Springer: Cham, Switzerland, 2020; Volume 596, pp. 123–155. [Google Scholar]
- Dos Reis, F.; Ganghoffer, J.F. Equivalent mechanical properties of auxetic lattices from discrete homogenization. Comput. Mater. Sci. 2012, 51, 314–321. [Google Scholar] [CrossRef]
- Dos Reis, F.; Ganghoffer, J.F. Construction of micropolar continua from the asymptotic homogenization of beam lattices. Comput. Struct. 2012, 112, 354–363. [Google Scholar] [CrossRef]
- Eremeyev, V.A.; Pietraszkiewicz, W. Material symmetry group and constitutive equations of micropolar anisotropic elastic solids. Math. Mech. Solids 2016, 21, 210–221. [Google Scholar] [CrossRef]
- Eremeyev, V.A.; Pietraszkiewicz, W. Material symmetry group of the non-linear polar-elastic continuum. Int. J. Solids Struct. 2012, 49, 1993–2005. [Google Scholar] [CrossRef]
- Altenbach, H.; Eremeyev, V. On the constitutive equations of viscoelastic micropolar plates and shells of differential type. Math. Mech. Complex Syst. 2015, 3, 273–283. [Google Scholar] [CrossRef]
- Battista, A.; Cardillo, C.; Del Vescovo, D.; Rizzi, N.L.; Turco, E. Frequency shifts induced by large deformations in planar pantographic continua. Nanosci. Technol. Int. J. 2015, 6, 161–178. [Google Scholar] [CrossRef]
- Laudato, M.; Barchiesi, E. Non-linear Dynamics of Pantographic Fabrics: Modelling and Numerical Study. In Wave Dynamics, Mechanics and Physics of Microstructured Metamaterials; Advanced Structured Materials; Sumbatyan, M., Ed.; Springer: Cham, Switzerland, 2019; Volume 109, pp. 241–254. [Google Scholar]
- Eremeyev, V.A.; Sharma, B.L. Anti-plane surface waves in media with surface structure: Discrete vs. continuum model. Int. J. Eng. Sci. 2019, 143, 33–38. [Google Scholar] [CrossRef]
- Scala, I.; Rosi, G.; Placidi, L.; Nguyen, V.H.; Naili, S. Effects of the microstructure and density profiles on wave propagation across an interface with material properties. Contin. Mech. Thermodyn. 2019, 31, 1165–1180. [Google Scholar] [CrossRef]
- Engelbrecht, J.; Berezovski, A. Reflections on mathematical models of deformation waves in elastic microstructured solids. Math. Mech. Complex Syst. 2015, 3, 43–82. [Google Scholar] [CrossRef]
- Eremeyev, V.A. Strongly anisotropic surface elasticity and antiplane surface waves. Philos. Trans. R. Soc. 2019, 378, 1–14. [Google Scholar] [CrossRef]
- Moretti, P.F.; Grzybowski, B.A.; Basios, V.; Fortunato, E.; Diez, M.S.; Speck, O.; Martins, R. STEM materials: A new frontier for an intelligent sustainable world. BMC Mater. 2019, 1, 1–3. [Google Scholar] [CrossRef]
- Altenbach, H.; Eremeyev, V.A. Direct approach-based analysis of plates composed of functionally graded materials. Arch. Appl. Mech. 2008, 78, 775–794. [Google Scholar] [CrossRef]
- Seppecher, P.; Alibert, J.J.; dell’Isola, F. Linear elastic trusses leading to continua with exotic mechanical interactions. J. Phys. 2011, 319, 012018. [Google Scholar] [CrossRef]
- Dell’Isola, F.; Turco, E.; Misra, A.; Vangelatos, Z.; Grigoropoulos, C.; Melissinaki, V.; Farsari, M. Force–displacement relationship in micro-metric pantographs: Experiments and numerical simulations. Comptes Rendus Mec. 2019, 347, 397–405. [Google Scholar] [CrossRef]
- Nejadsadeghi, N.; De Angelo, M.; Drobnicki, R.; Lekszycki, T.; dell’Isola, F.; Misra, A. Parametric Experimentation on Pantographic Unit Cells Reveals Local Extremum Configuration. Exp. Mech. 2019, 59, 927–939. [Google Scholar] [CrossRef]
- Barchiesi, E.; Eugster, S.R.; Placidi, L.; dell’Isola, F. Pantographic beam: A complete second gradient 1D-continuum in plane. Z. Angew. Math. Phys. Angew. Math. Phys. 2019, 70, 135. [Google Scholar] [CrossRef]
- Dell’Isola, F.; Steigmann, D. A two-dimensional gradient-elasticity theory for woven fabrics. J. Elast. 2015, 118, 113–125. [Google Scholar] [CrossRef]
- Steigmann, D.J.; dell’Isola, F. Mechanical response of fabric sheets to three-dimensional bending, twisting, and stretching. Acta Mech. Sin. 2015, 31, 373–382. [Google Scholar] [CrossRef]
- Steigmann, D.J.; Pipkin, A.C. Equilibrium of elastic nets. Philos. Trans. R. Soc. London. Ser. Phys. Eng. Sci. 1991, 335, 419–454. [Google Scholar]
- Scerrato, D.; Giorgio, I.; Rizzi, N.L. Three-dimensional instabilities of pantographic sheets with parabolic lattices: Numerical investigations. Z. Angew. Math. Phys. 2016, 67, 53. [Google Scholar] [CrossRef]
- Placidi, L.; Barchiesi, E.; Della Corte, A. Identification of two-dimensional pantographic structures with a linear D4 orthotropic second gradient elastic model accounting for external bulk double forces. In Mathematical Modelling in Solid Mechanics; Advanced Structured Materials; dell’Isola, F., Sofonea, M., Steigmann, D., Eds.; Springer: Singapore, 2017; Volume 69, pp. 211–232. [Google Scholar]
- Harrison, P. Modelling the forming mechanics of engineering fabrics using a mutually constrained pantographic beam and membrane mesh. Compos. Part Appl. Sci. Manuf. 2016, 81, 145–157. [Google Scholar] [CrossRef]
- Harrison, P.; Alvarez, M.F.; Anderson, D. Towards comprehensive characterisation and modelling of the forming and wrinkling mechanics of engineering fabrics. Int. J. Solids Struct. 2018, 154, 2–18. [Google Scholar] [CrossRef]
- Rosi, G.; Placidi, L.; Auffray, N. On the validity range of strain-gradient elasticity: A mixed static-dynamic identification procedure. Eur. J. Mech. A Solids 2018, 69, 179–191. [Google Scholar] [CrossRef]
- Abali, B.E.; Wu, C.C.; Müller, W.H. An energy-based method to determine material constants in nonlinear rheology with applications. Contin. Mech. Thermodyn. 2016, 28, 1221–1246. [Google Scholar] [CrossRef]
- Abali, B.E.; Müller, W.H.; Eremeyev, V.A. Strain gradient elasticity with geometric nonlinearities and its computational evaluation. Mech. Adv. Mater. Mod. Process. 2015, 1, 4. [Google Scholar] [CrossRef]
- Misra, A.; Poorsolhjouy, P. Identification of higher-order elastic constants for grain assemblies based upon granular micromechanics. Math. Mech. Complex Syst. 2015, 3, 285–308. [Google Scholar] [CrossRef]
- Argyris, J.H.; Fried, I.; Scharpf, D.W. The TUBA family of plate elements for the matrix displacement method. Aeronaut. J. 1968, 72, 701–709. [Google Scholar] [CrossRef]
- Gustafsson, T.; Stenberg, R.; Videman, J. A posteriori estimates for conforming Kirchhoff plate elements. SIAM J. Sci. Comput. 2018, 40, A1386–A1407. [Google Scholar] [CrossRef]
- Giorgio, I.; Della Corte, A.; dell’Isola, F.; Steigmann, D.J. Buckling modes in pantographic lattices. Comptes Rendus Mec. 2016, 344, 487–501. [Google Scholar] [CrossRef]
- Wang, C.M.; Zhang, H.; Gao, R.P.; Duan, W.H.; Challamel, N. Hencky bar-chain model for buckling and vibration of beams with elastic end restraints. Int. J. Struct. Stab. Dyn. 2015, 15, 1540007. [Google Scholar] [CrossRef]
- Turco, E. Discrete is it enough? The revival of Piola–Hencky keynotes to analyze three-dimensional Elastica. Contin. Mech. Thermodyn. 2018, 30, 1039–1057. [Google Scholar] [CrossRef]
- Turco, E.; dell’Isola, F.; Cazzani, A.; Rizzi, N.L. Hencky-type discrete model for pantographic structures: Numerical comparison with second gradient continuum models. Z. Angew. Math. Phys. 2016, 67, 85. [Google Scholar] [CrossRef]
- Turco, E.; Misra, A.; Pawlikowski, M.; dell’Isola, F.; Hild, F. Enhanced Piola–Hencky discrete models for pantographic sheets with pivots without deformation energy: Numerics and experiments. Int. J. Solids Struct. 2018, 147, 94–109. [Google Scholar] [CrossRef]
- Andreaus, U.; Spagnuolo, M.; Lekszycki, T.; Eugster, S.R. A Ritz approach for the static analysis of planar pantographic structures modeled with nonlinear Euler–Bernoulli beams. Contin. Mech. Thermodyn. 2018, 30, 1103–1123. [Google Scholar] [CrossRef]
- Greco, L.; Cuomo, M.; Contrafatto, L. Two new triangular G1-conforming finite elements with cubic edge rotation for the analysis of Kirchhoff plates. Comput. Methods Appl. Mech. Eng. 2019, 356, 354–386. [Google Scholar] [CrossRef]
- Greco, L.; Cuomo, M.; Contrafatto, L. A quadrilateral G1-conforming finite element for the Kirchhoff plate model. Comput. Methods Appl. Mech. Eng. 2019, 346, 913–951. [Google Scholar] [CrossRef]
- Cuomo, M.; Greco, L. An implicit strong G1-conforming formulation for the analysis of the Kirchhoff plate model. Contin. Mech. Thermodyn. 2018. [Google Scholar] [CrossRef]
- Greco, L.; Cuomo, M.; Contrafatto, L. A reconstructed local B formulation for isogeometric Kirchhoff–Love shells. Comput. Methods Appl. Mech. Eng. 2018, 332, 462–487. [Google Scholar] [CrossRef]
- Turco, E.; Caracciolo, P. Elasto-plastic analysis of Kirchhoff plates by high simplicity finite elements. Comput. Methods Appl. Mech. Eng. 2000, 190, 691–706. [Google Scholar] [CrossRef]
- Niiranen, J.; Kiendl, J.; Niemi, A.H.; Reali, A. Isogeometric analysis for sixth-order boundary value problems of gradient-elastic Kirchhoff plates. Comput. Methods Appl. Mech. Eng. 2017, 316, 328–348. [Google Scholar] [CrossRef]
- Cazzani, A.; Malagù, M.; Turco, E.; Stochino, F. Constitutive models for strongly curved beams in the frame of isogeometric analysis. Math. Mech. Solids 2016, 21, 182–209. [Google Scholar] [CrossRef]
- Cazzani, A.; Malagù, M.; Turco, E. Isogeometric analysis of plane-curved beams. Math. Mech. Solids 2016, 21, 562–577. [Google Scholar] [CrossRef]
- Niiranen, J.; Balobanov, V.; Kiendl, J.; Hosseini, S.B. Variational formulations, model comparisons and numerical methods for Euler–Bernoulli micro-and nano-beam models. Math. Mech. Solids 2019, 24, 312–335. [Google Scholar] [CrossRef]
- Balobanov, V.; Niiranen, J. Locking-free variational formulations and isogeometric analysis for the Timoshenko beam models of strain gradient and classical elasticity. Comput. Methods Appl. Mech. Eng. 2018, 339, 137–159. [Google Scholar] [CrossRef]
- Maurin, F.; Greco, F.; Desmet, W. Isogeometric analysis for nonlinear planar pantographic lattice: discrete and continuum models. Contin. Mech. Thermodyn. 2019, 31, 1051–1064. [Google Scholar] [CrossRef]
- Yildizdag, M.E.; Demirtas, M.; Ergin, A. Multipatch discontinuous Galerkin isogeometric analysis of composite laminates. Contin. Mech. Thermodyn. 2018. [Google Scholar] [CrossRef]
- Khakalo, S.; Balobanov, V.; Niiranen, J. Modelling size-dependent bending, buckling and vibrations of 2D triangular lattices by strain gradient elasticity models: Applications to sandwich beams and auxetics. Int. J. Eng. Sci. 2018, 127, 33–52. [Google Scholar] [CrossRef]
- Cazzani, A.; Serra, M.; Stochino, F.; Turco, E. A refined assumed strain finite element model for statics and dynamics of laminated plates. Contin. Mech. Thermodyn. 2018. [Google Scholar] [CrossRef]
- Cazzani, A.; Rizzi, N.L.; Stochino, F.; Turco, E. Modal analysis of laminates by a mixed assumed-strain finite element model. Math. Mech. Solids 2018, 23, 99–119. [Google Scholar] [CrossRef]
- Spagnuolo, M.; Andreaus, U. A targeted review on large deformations of planar elastic beams: Extensibility, distributed loads, buckling and post-buckling. Math. Mech. Solids 2019, 24, 258–280. [Google Scholar] [CrossRef]
- Dell’Isola, F.; Seppecher, P.; Alibert, J.J.; Lekszycki, T.; Grygoruk, R.; Pawlikowski, M.; Gołaszewski, M. Pantographic metamaterials: An example of mathematically driven design and of its technological challenges. Contin. Mech. Thermodyn. 2019, 31, 851–884. [Google Scholar] [CrossRef]



© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Scerrato, D.; Giorgio, I. Equilibrium of Two-Dimensional Cycloidal Pantographic Metamaterials in Three-Dimensional Deformations. Symmetry 2019, 11, 1523. https://doi.org/10.3390/sym11121523
Scerrato D, Giorgio I. Equilibrium of Two-Dimensional Cycloidal Pantographic Metamaterials in Three-Dimensional Deformations. Symmetry. 2019; 11(12):1523. https://doi.org/10.3390/sym11121523
Chicago/Turabian StyleScerrato, Daria, and Ivan Giorgio. 2019. "Equilibrium of Two-Dimensional Cycloidal Pantographic Metamaterials in Three-Dimensional Deformations" Symmetry 11, no. 12: 1523. https://doi.org/10.3390/sym11121523
APA StyleScerrato, D., & Giorgio, I. (2019). Equilibrium of Two-Dimensional Cycloidal Pantographic Metamaterials in Three-Dimensional Deformations. Symmetry, 11(12), 1523. https://doi.org/10.3390/sym11121523





