Tsallis Holographic Dark Energy in f(G,T) Gravity
Abstract
1. Introduction
2. Reconstruction of THDE Models
- ,
- ,
2.1. Conserved EMT Based Reconstruction
2.2. Non-Conserved EMT Based Reconstruction
3. Cosmological Analysis
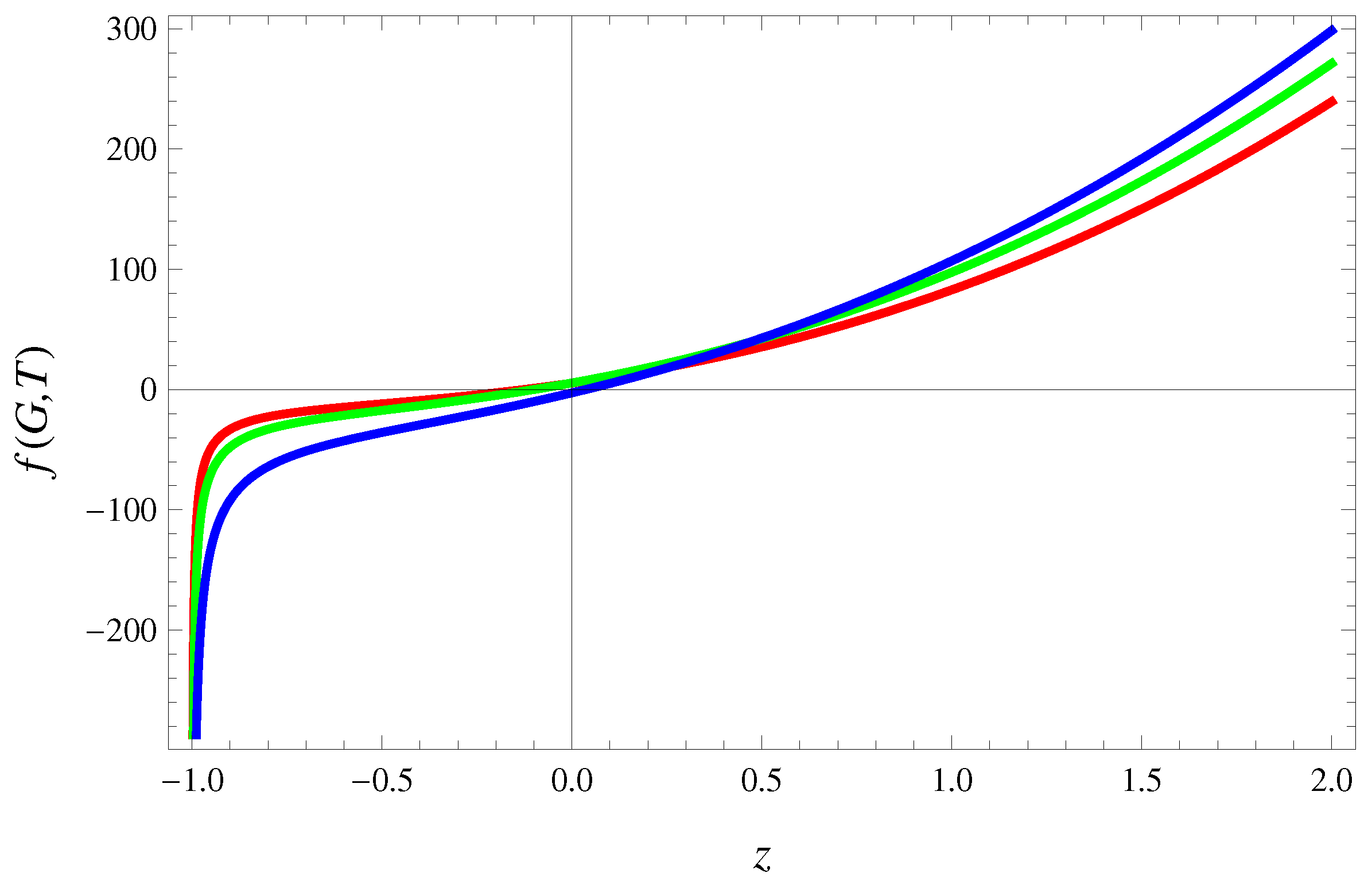
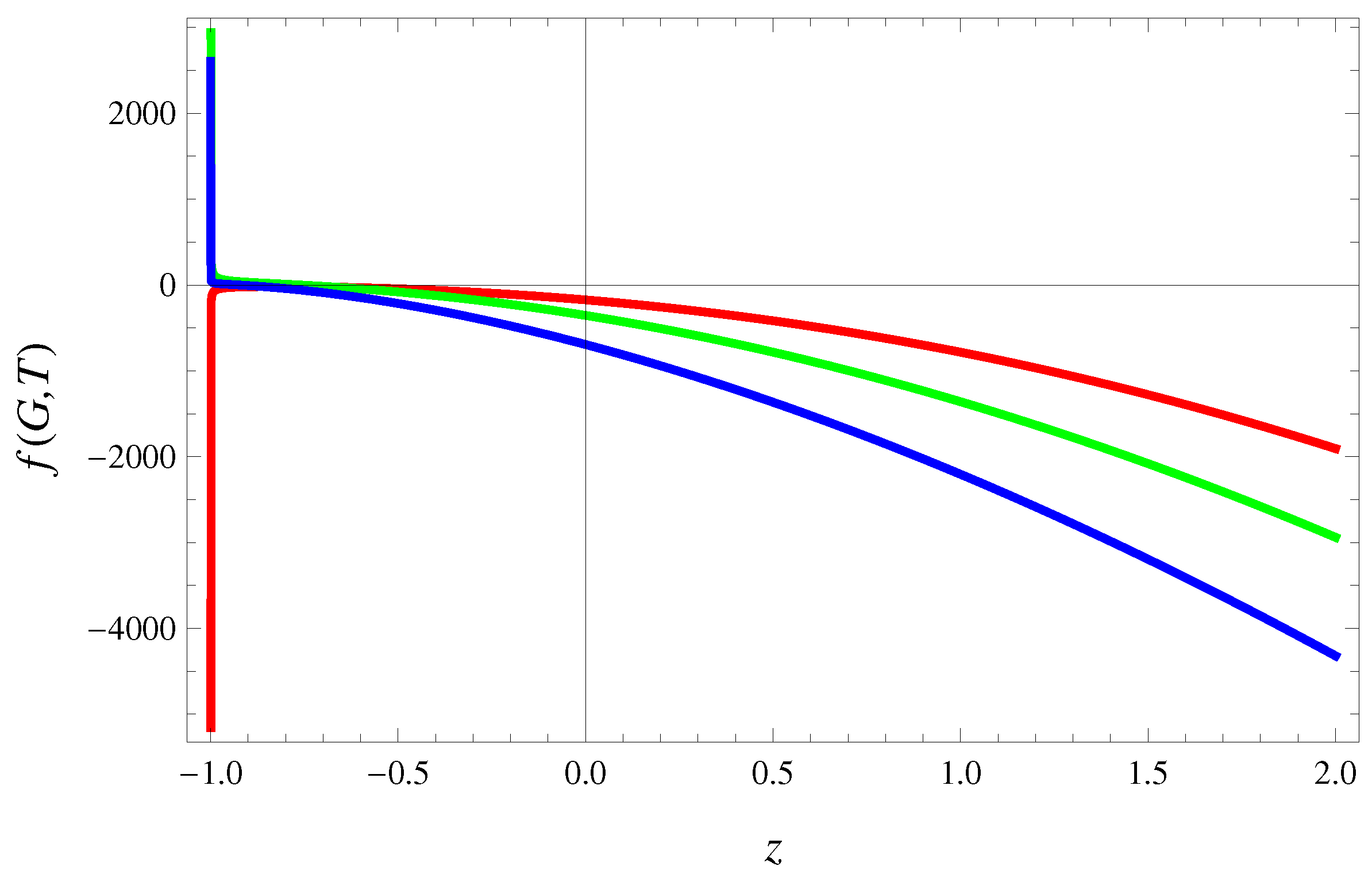
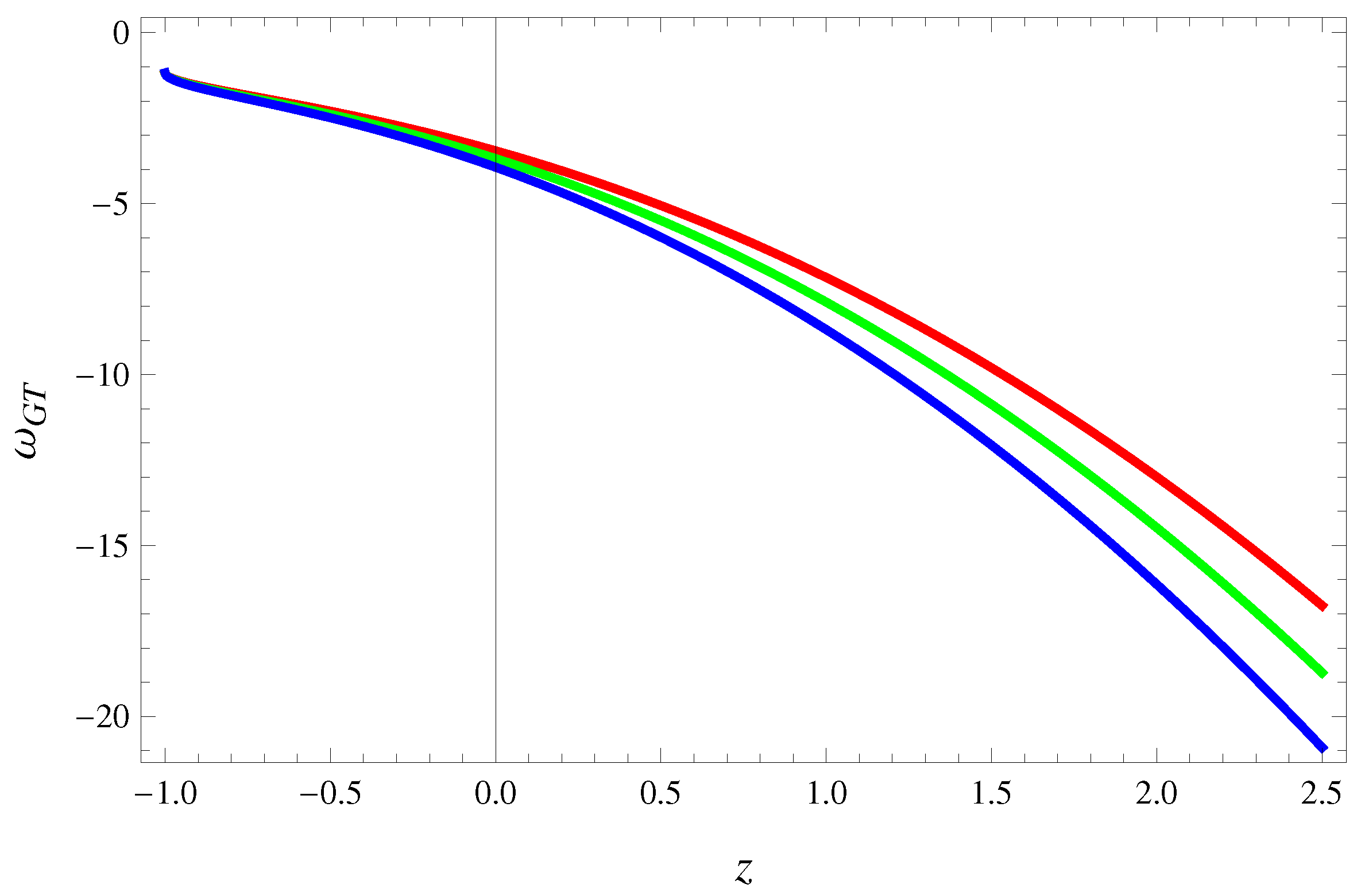
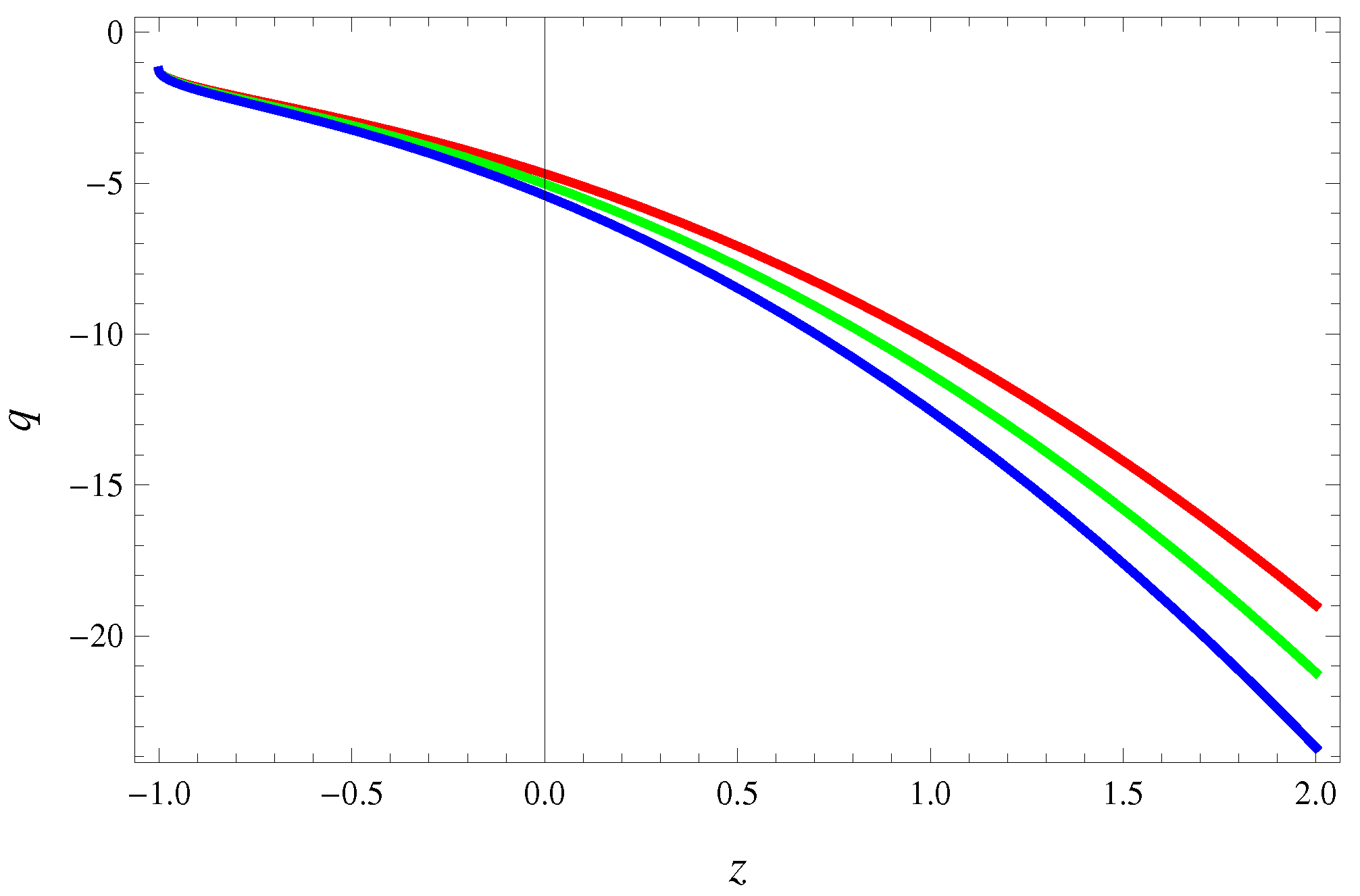
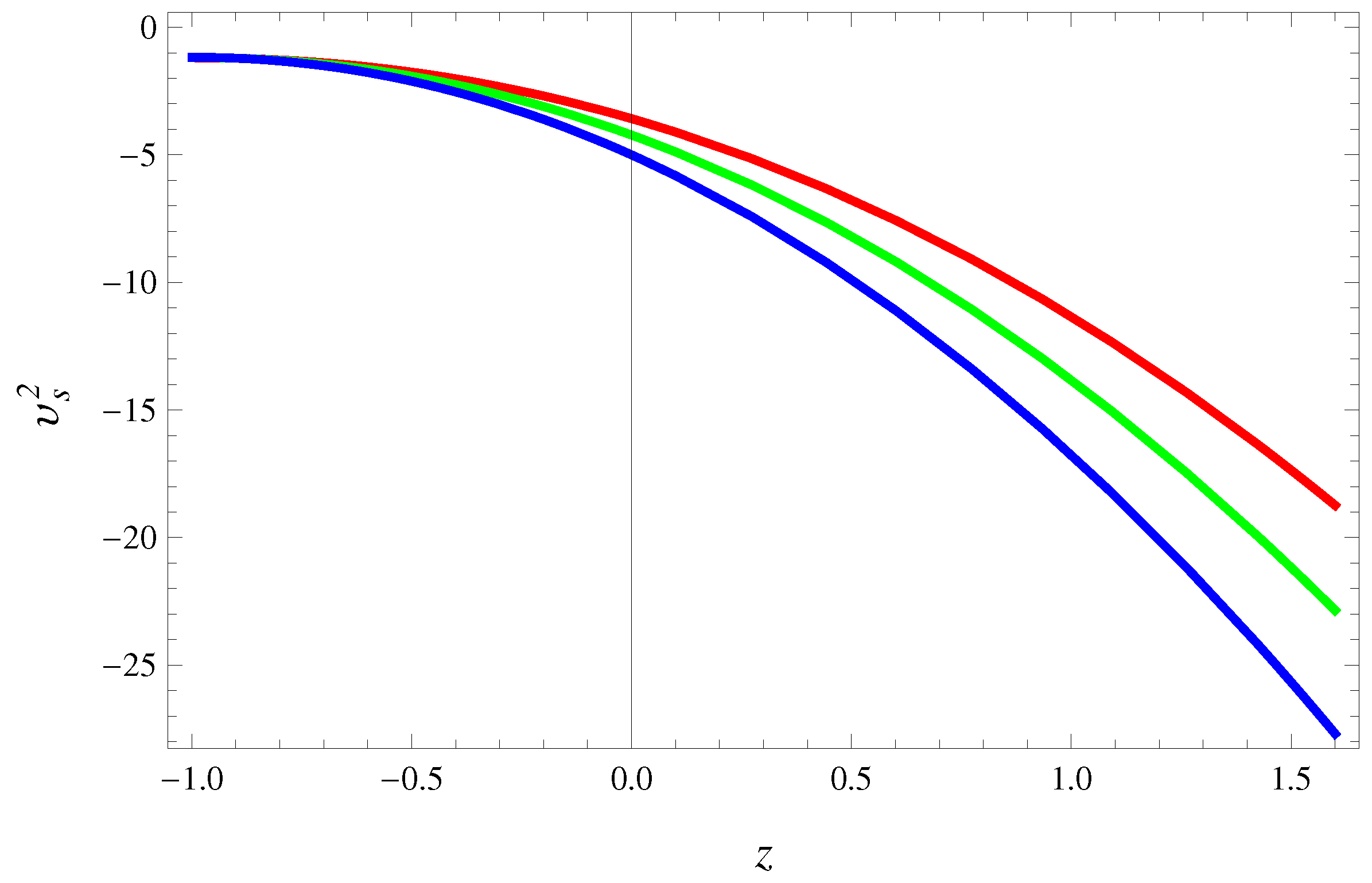
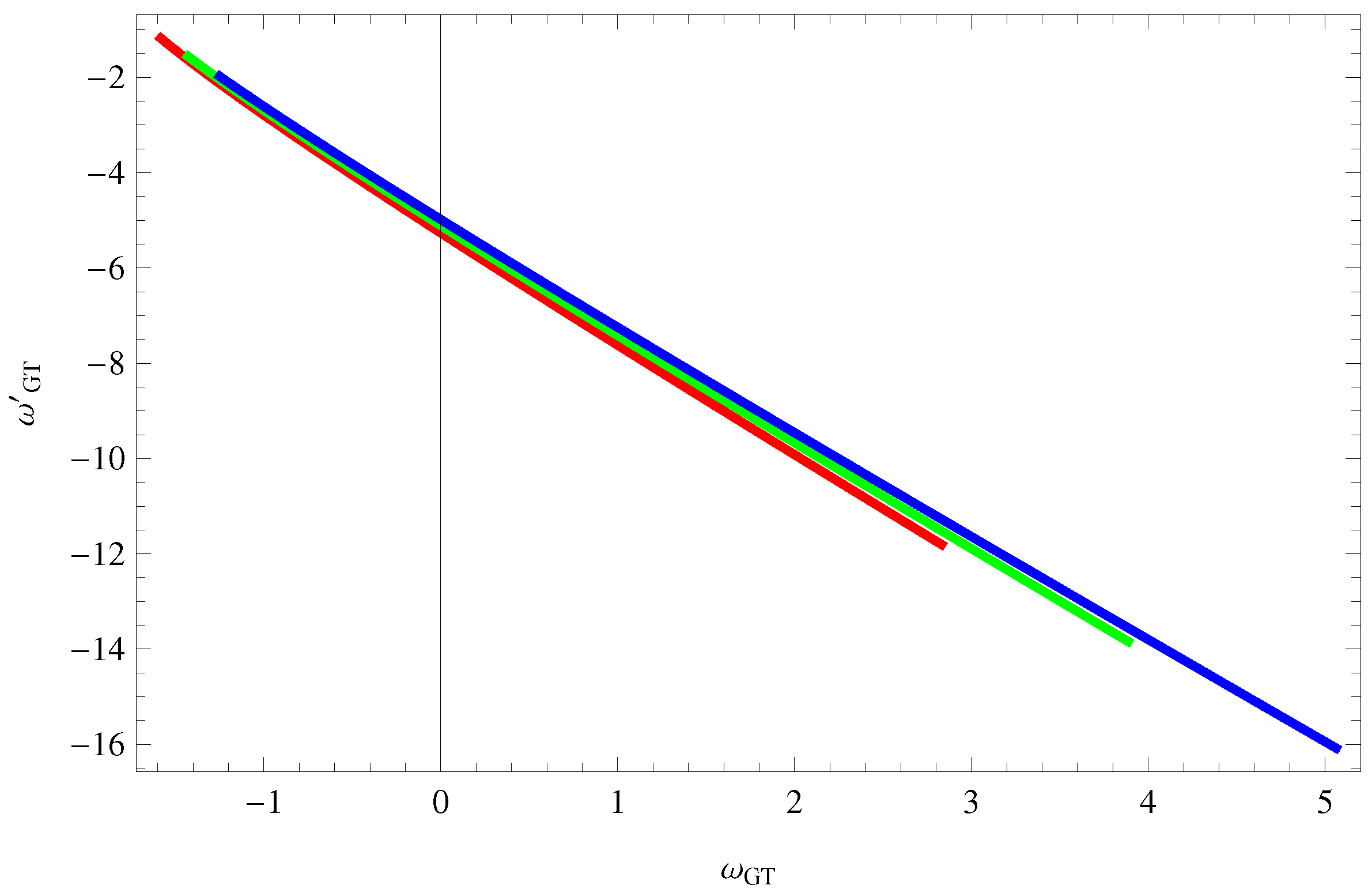
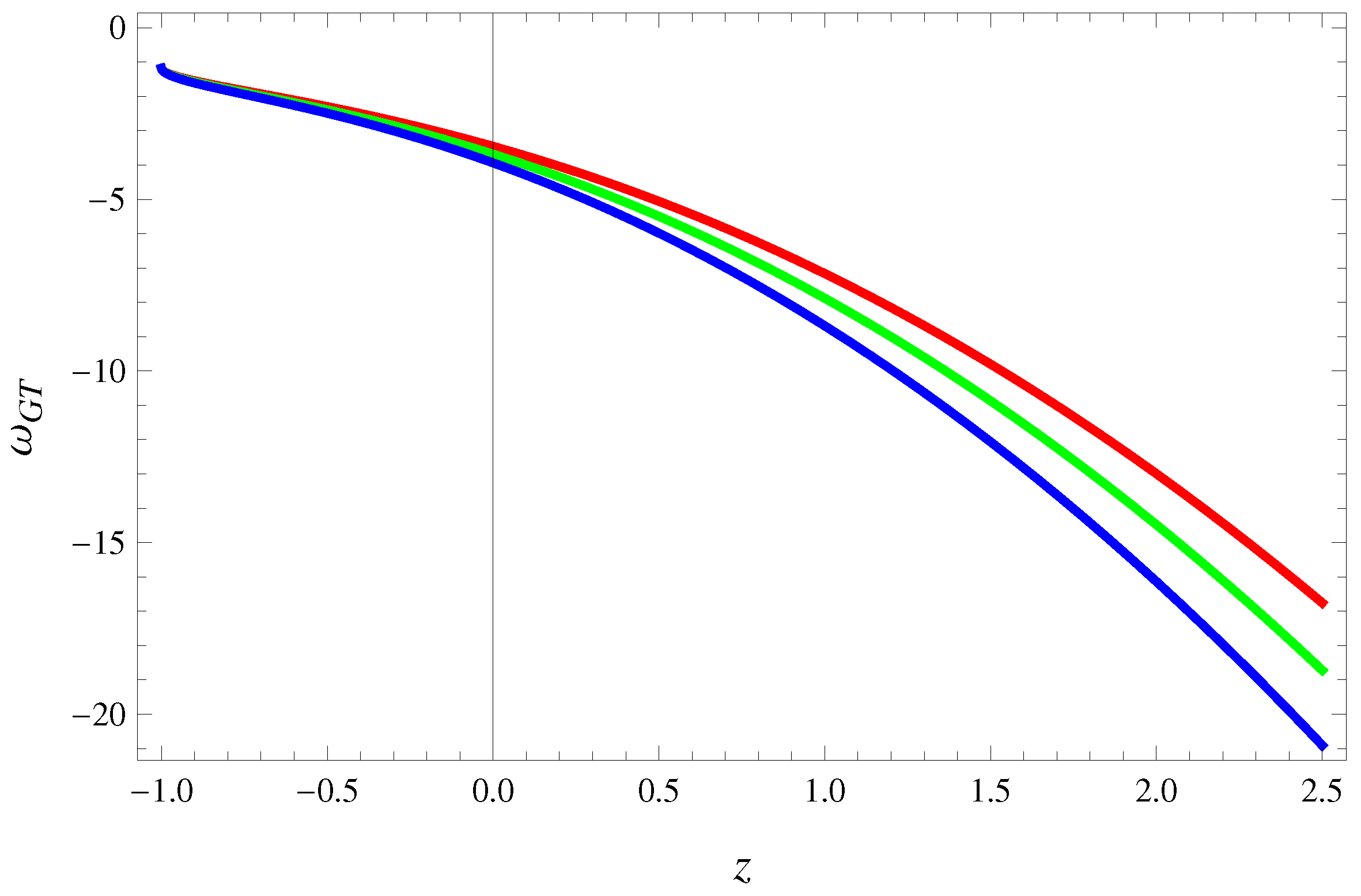
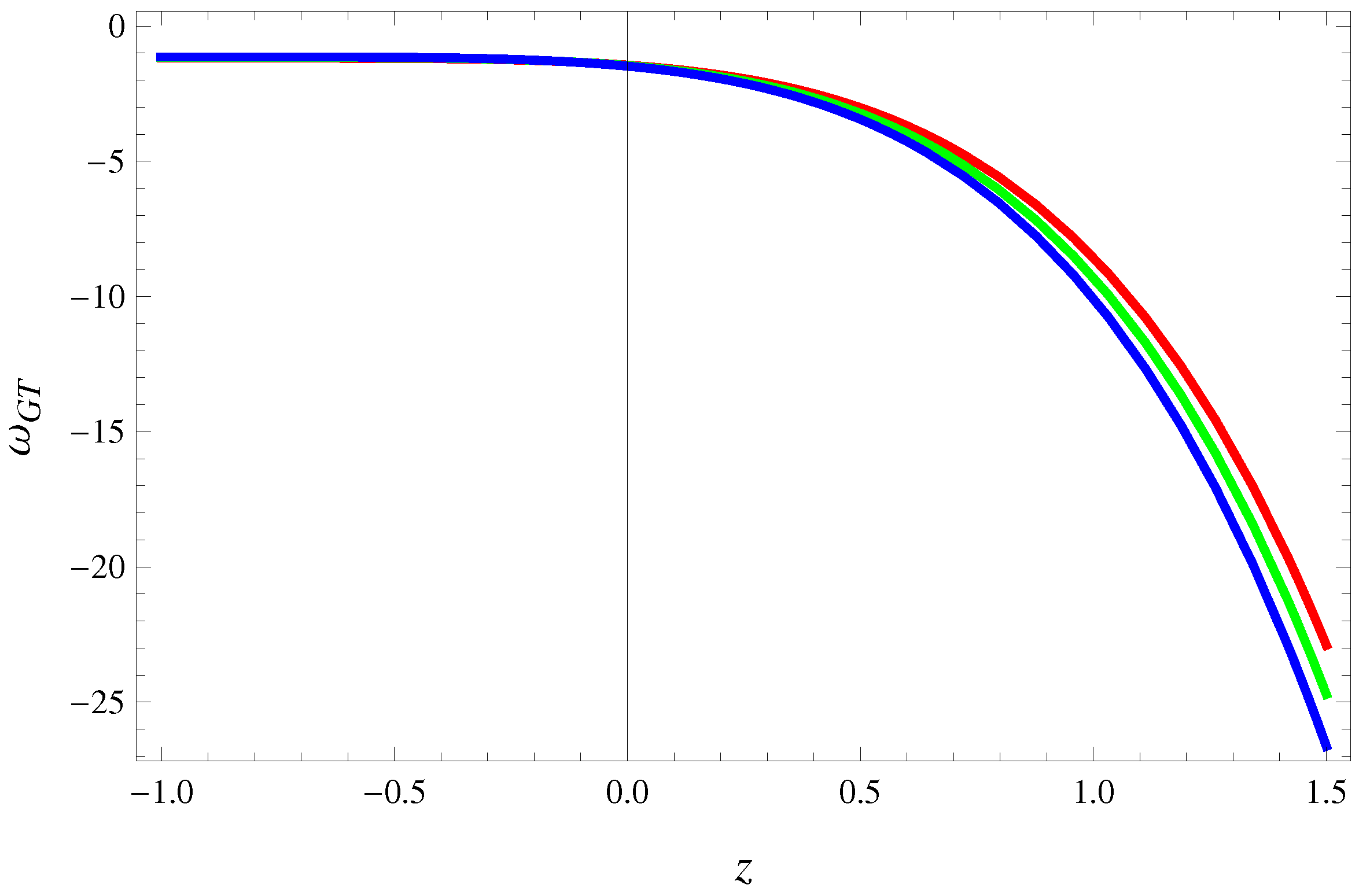
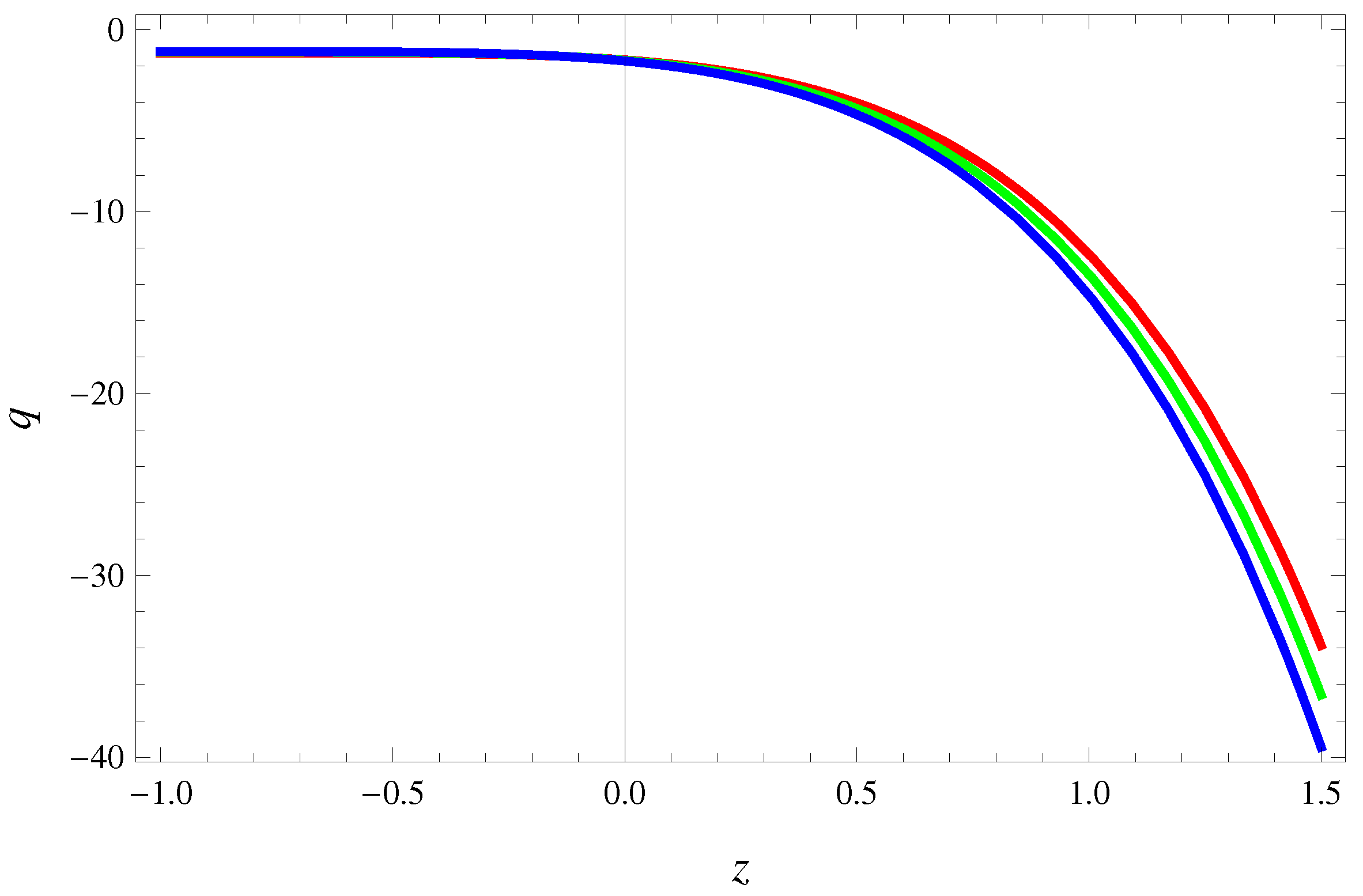
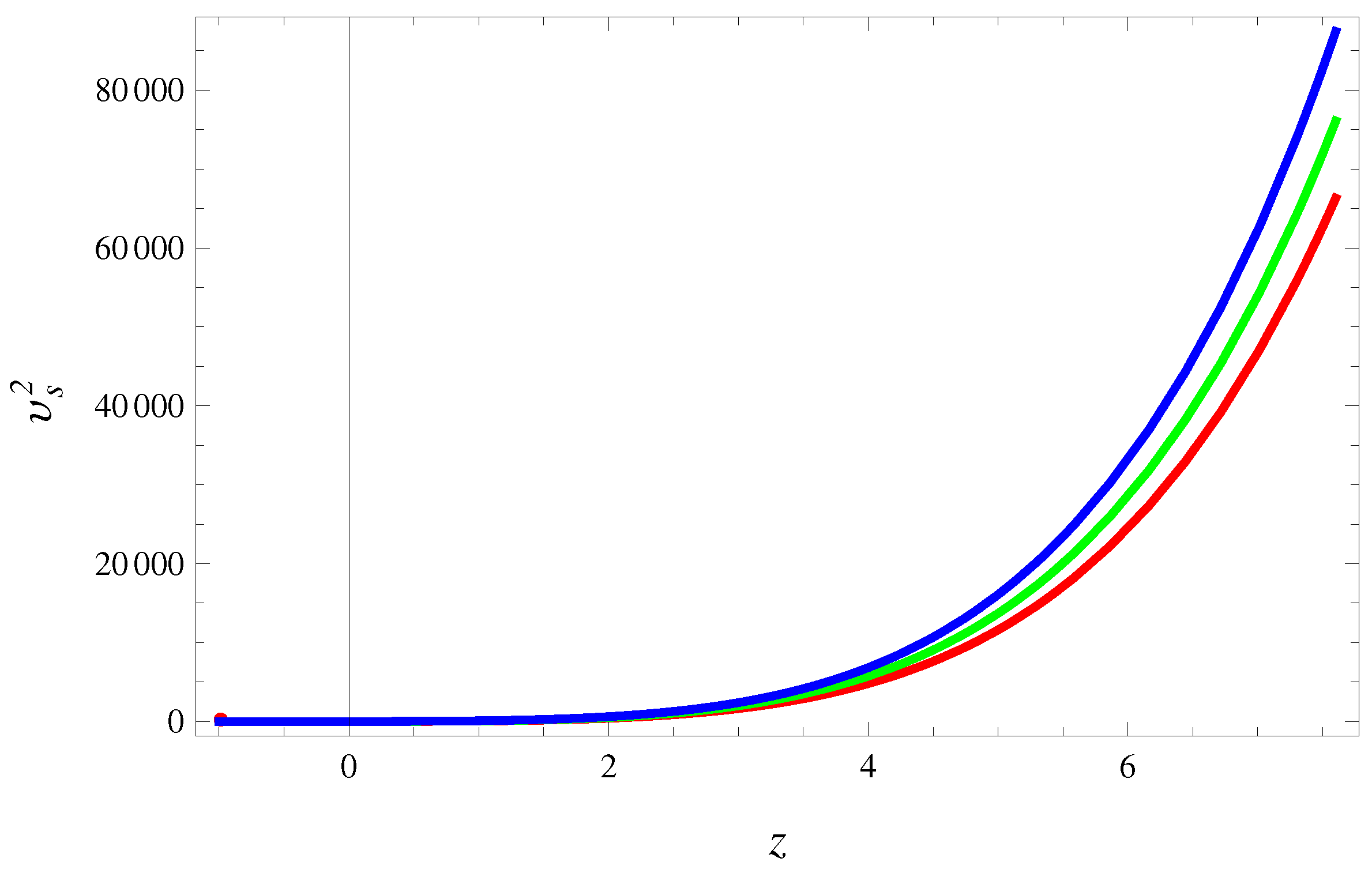
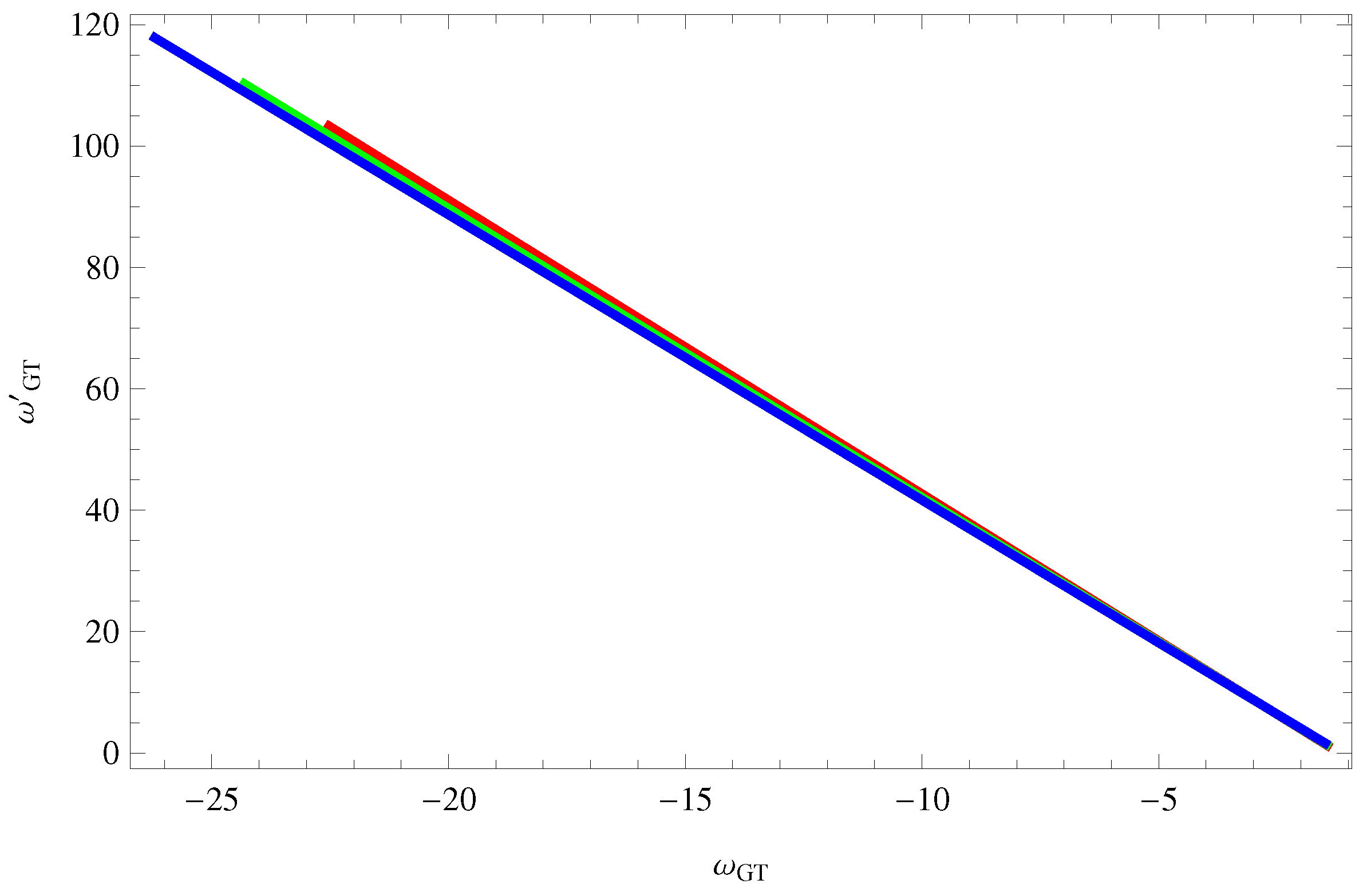
3.1. Cosmic Diagnostics Parameters for Conserved EMT
3.2. Cosmic Diagnostics Parameters for Non-Conserved EMT
4. Concluding Remarks
Author Contributions
Funding
Conflicts of Interest
References
- Capozziello, S.; De Laurentis, M.; Luongo, O.; Ruggeri, A.C. Cosmographic constraints and cosmic fluids. Galaxies 2013, 1, 216–260. [Google Scholar] [CrossRef]
- Susskind, L. The world as a hologram. J. Math. Phys. 1995, 36, 6377–6396. [Google Scholar] [CrossRef]
- Cohen, A.G.; Kaplan, D.B.; Nelson, A.E. Effective field theory, black holes, and the cosmological constant. Phys. Rev. Lett. 1999, 82, 4971. [Google Scholar] [CrossRef]
- Li, M. A model of holographic dark energy. Phys. Lett. B 2004, 603, 1–5. [Google Scholar] [CrossRef]
- Karami, K.; Khaledian, M.S. Reconstructing f(R) modified gravity from ordinary and entropy-corrected versions of the holographic and new agegraphic dark energy models. J. High Energy Phys. 2011, 3, 86. [Google Scholar] [CrossRef]
- Houndjo, M.J.S.; Piattella, O.F. Reconstructing f(R,T) gravity from holographic dark energy. Int. J. Mod. Phys. D 2012, 21, 1250024. [Google Scholar] [CrossRef]
- Daouda, M.H.; Rodrigues, M.E.; Houndjo, M.J.S. Static anisotropic solutions in f(T) theory. Eur. Phys. J. C 2012, 72, 1890. [Google Scholar] [CrossRef]
- Jawad, A.; Pasqua, A.; Chattopadhyay, S. Holographic reconstruction of f(G) gravity for scale factors pertaining to emergent, logamediate and intermediate scenarios. Eur. Phys. J. Plus 2013, 128, 156. [Google Scholar] [CrossRef]
- Sharif, M.; Zubair, M. Cosmology of holographic and new agegraphic f(R,T) models. J. Phys. Soc. Jpn. 2013, 82, 064001. [Google Scholar] [CrossRef]
- Fayaz, V.; Hossienkhani, H.; Amirabadi, M.; Azimi, N. Anisotropic cosmological models in f(R,T) gravity according to holographic and new agegraphic dark energy. Astrophys. Space Sci. 2014, 353, 301–309. [Google Scholar] [CrossRef]
- Horava, P.; Minic, D. Probable values of the cosmological constant in a holographic theory. Phys. Rev. Lett. 2000, 85, 1610. [Google Scholar] [CrossRef] [PubMed]
- Thomas, S. Holography stabilizes the vacuum energy. Phys. Rev. Lett. 2002, 89, 081301. [Google Scholar] [CrossRef] [PubMed]
- Hsu, S.D.H. Entropy bounds and dark energy. Phys. Lett. B 2004, 594, 13–16. [Google Scholar] [CrossRef]
- Guberina, B.; Horvat, H.; Nikolić, H. Non-saturated holographic dark energy. J. Cosmol. Astropart. Phys. 2007, 1, 012. [Google Scholar] [CrossRef]
- Wang, B.; Abdalla, E.; Atrio-Barandela, F.; Pavon, D. Dark matter and dark energy interactions: Theoretical challenges, cosmological implications and observational signatures. Rep. Prog. Phys. 2016, 79, 096901. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.; Wang, Y.; Li, M. Holographic dark energy. Phys. Rep. 2017, 699, 1–57. [Google Scholar] [CrossRef]
- Moradpour, H. Implications, consequences and interpretations of generalized entropy in the cosmological setups. Int. J. Theor. Phys. 2016, 55, 4176–4184. [Google Scholar] [CrossRef]
- Wen, W.Y. Thermodynamic metric of deformed Schwarzschild black holes. Int. J. Mod. Phys. D 2017, 26, 1750106. [Google Scholar] [CrossRef]
- Moradpour, H.; Bonilla, A.; Abreu, E.M.C.; Neto, A.J. Accelerated cosmos in a nonextensive setup. Phys. Rev. D 2017, 96, 123504. [Google Scholar] [CrossRef]
- Jahmori, A.S.; Moosavi, S.A.; Moradpour, H.; Graca, J.M.; Lobo, I.P.; Salako, I.G.; Jawad, A. Generalized entropy formalism and a new holographic dark energy model. Phys. Lett. B 2018, 780, 21–24. [Google Scholar] [CrossRef]
- Moradpour, H.; Moosavi, S.A.; Lobo, I.P.; Graca, J.P.; Jawad, A.; Salako, I.G. Thermodynamic approach to holographic dark energy and the Rényi entropy. Eur. Phys. J. C 2018, 78, 829. [Google Scholar] [CrossRef]
- Biró, T.S.; Ván, P. Zeroth law compatibility of nonadditive thermodynamics. Phys. Rev. E 2011, 83, 061187. [Google Scholar] [CrossRef] [PubMed]
- Majhi, A. Non-extensive statistical mechanics and black hole entropy from quantum geometry. Phys. Lett. B 2017, 775, 32–36. [Google Scholar] [CrossRef]
- Tsallis, C. Possible generalization of Boltzmann-Gibbs statistics. J. Stat. Phys. 1988, 52, 479–487. [Google Scholar] [CrossRef]
- Tsallis, C.; Citro, L.J.L. Black hole thermodynamical entropy. Eur. Phys. J. C 2013, 73, 2487. [Google Scholar] [CrossRef]
- Czinner, V.G.; Iguchi, H. A zeroth law compatible model to Kerr black hole thermodynamics. Universe 2017, 3, 14. [Google Scholar] [CrossRef]
- Komatsu, N. Cosmological model from the holographic equipartition law with a modified Rényi entropy. Eur. Phys. J. C 2017, 77, 229. [Google Scholar] [CrossRef]
- Tavayef, M.; Sheykhi, A.; Bamba, K.; Moradpour, H. Tsallis holographic dark energy. Phys. Lett. B 2018, 781, 195–200. [Google Scholar] [CrossRef]
- Harko, T.; Lobo, F.S.; Nojiri, S.I.; Odintsov, S.D. f(R,T) gravity. Phys. Rev. D 2011, 84, 024020. [Google Scholar] [CrossRef]
- Sharif, M.; Ikram, A. Energy Conditions in f(,T) Gravity. Eur. Phys. J. C 2016, 76, 640. [Google Scholar] [CrossRef]
- Sharif, M.; Ikram, A. Stability analysis of some reconstructed cosmological models in f(,T) gravity. Phys. Dark Universe 2017, 17, 11–19. [Google Scholar] [CrossRef]
- Bhatti, M.Z.U.; Sharif, M.; Yousaf, Z.; Ilyas, M. Role of f(G,T) gravity on the evolution of relativistic stars. Int. J. Mod. Phys. D 2017, 27, 1850044. [Google Scholar] [CrossRef]
- Shamir, M.F.; Ahmad, M. Noether symmetry approach in f(,T) gravity. Eur. Phys. J. C 2017, 77, 55. [Google Scholar] [CrossRef]
- Jamil, M.; Saridakis, E.N. New agegraphic dark energy in Horava-Lifshitz cosmology. J. Cosmol. Astropart. Phys. 2010, 1007, 028. [Google Scholar] [CrossRef]
- Jawad, A.; Chattopadhyay, S. Cosmological analysis of F() models via pilgrim dark energy. Astrophys. Space Sci. 2015, 357, 37. [Google Scholar] [CrossRef]
- Sharif, M.; Nazir, K. Cosmological evolution of generalized ghost pilgrim dark energy in f(T) gravity. Astrophys. Space Sci. 2015, 360, 57. [Google Scholar] [CrossRef]
- Sharif, M.; Nazir, K. Cosmological analysis of reconstructed (T,T) models. Eur. Phys. J. C 2018, 78, 77. [Google Scholar] [CrossRef]
- Kleidis, K.; Oikonomou, V.K. Unification of late-and early-time acceleration, with that of the intermediate eras, by scalar fields. Astrophys. Space Sci. 2017, 362, 74. [Google Scholar] [CrossRef]
- Ghaffari, S.; Moradpour, H.; Lobo, I.P.; Graca, J.M.; Bezerra, V.B. Tsallis holographic dark energy in the Brans-Dicke cosmology. Eur. Phys. J. C 2018, 78, 706. [Google Scholar] [CrossRef]
- Ghaffari, S.; Dehghani, M.H.; Sheykhi, A. Holographic dark energy in the DGP braneworld with Granda-Oliveros cutoff. Phys. Rev. D 2014, 89, 123009. [Google Scholar] [CrossRef]
- Sharif, M.; Ikram, A. Stability analysis of Einstein universe in f(,T) gravity. Int. J. Mod. Phys. D 2017, 26, 1750084. [Google Scholar] [CrossRef]
- Aviles, A.; Bravetti, A.; Capozziello, S.; Luongo, O. Cosmographic reconstruction of f(T) cosmology. Phys. Rev. D 2013, 87, 064025. [Google Scholar] [CrossRef]
- Capozziello, S.; Luongo, O.; Saridakis, E.N. Transition redshift in f(T) cosmology and observational constraints. Phys. Rev. D 2015, 91, 124037. [Google Scholar] [CrossRef]
- Capozziello, S.; Luongo, O.; Pincak, R.; Ravanpak, A. Cosmic acceleration in non-flat f(T) cosmology. Gen. Relativ. Gravit. 2018, 50, 53. [Google Scholar] [CrossRef]
- Caldwell, R.; Linder, E.V. Limits of quintessence. Phys. Rev. Lett. 2005, 95, 141301. [Google Scholar] [CrossRef] [PubMed]
- Sahni, V.; Saini, T.D.; Starobinsky, A.A.; Alam, U. Statefinder-a new geometrical diagnostic of dark energy. J. Exp. Theor. Phys. Lett. 2003, 77, 201–206. [Google Scholar] [CrossRef]
- Aghanim, N.; Akrami, Y.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; Bartolo, N.; Basak, S.; et al. Planck 2018 results. VI. Cosmological parameters. arXiv, 2018; arXiv:1807.06209. [Google Scholar]
- Aviles, A.; Gruber, C.; Luongo, O.; Quevedo, H. Cosmography and constraints on the equation of state of the Universe in various parametrizations. Phys. Rev. D 2012, 86, 123516. [Google Scholar] [CrossRef]
- Dunsby, P.K.S.; Luongo, O. On the theory and applications of modern cosmography. Int. J. Geom. Meth. Mod. Phys. 2016, 13, 1630002. [Google Scholar] [CrossRef]

© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sharif, M.; Saba, S. Tsallis Holographic Dark Energy in f(G,T) Gravity. Symmetry 2019, 11, 92. https://doi.org/10.3390/sym11010092
Sharif M, Saba S. Tsallis Holographic Dark Energy in f(G,T) Gravity. Symmetry. 2019; 11(1):92. https://doi.org/10.3390/sym11010092
Chicago/Turabian StyleSharif, Muhammad, and Saadia Saba. 2019. "Tsallis Holographic Dark Energy in f(G,T) Gravity" Symmetry 11, no. 1: 92. https://doi.org/10.3390/sym11010092
APA StyleSharif, M., & Saba, S. (2019). Tsallis Holographic Dark Energy in f(G,T) Gravity. Symmetry, 11(1), 92. https://doi.org/10.3390/sym11010092





