A Forecasting Model Based on Multi-Valued Neutrosophic Sets and Two-Factor, Third-Order Fuzzy Fluctuation Logical Relationships
Abstract
1. Introduction
2. Preliminaries
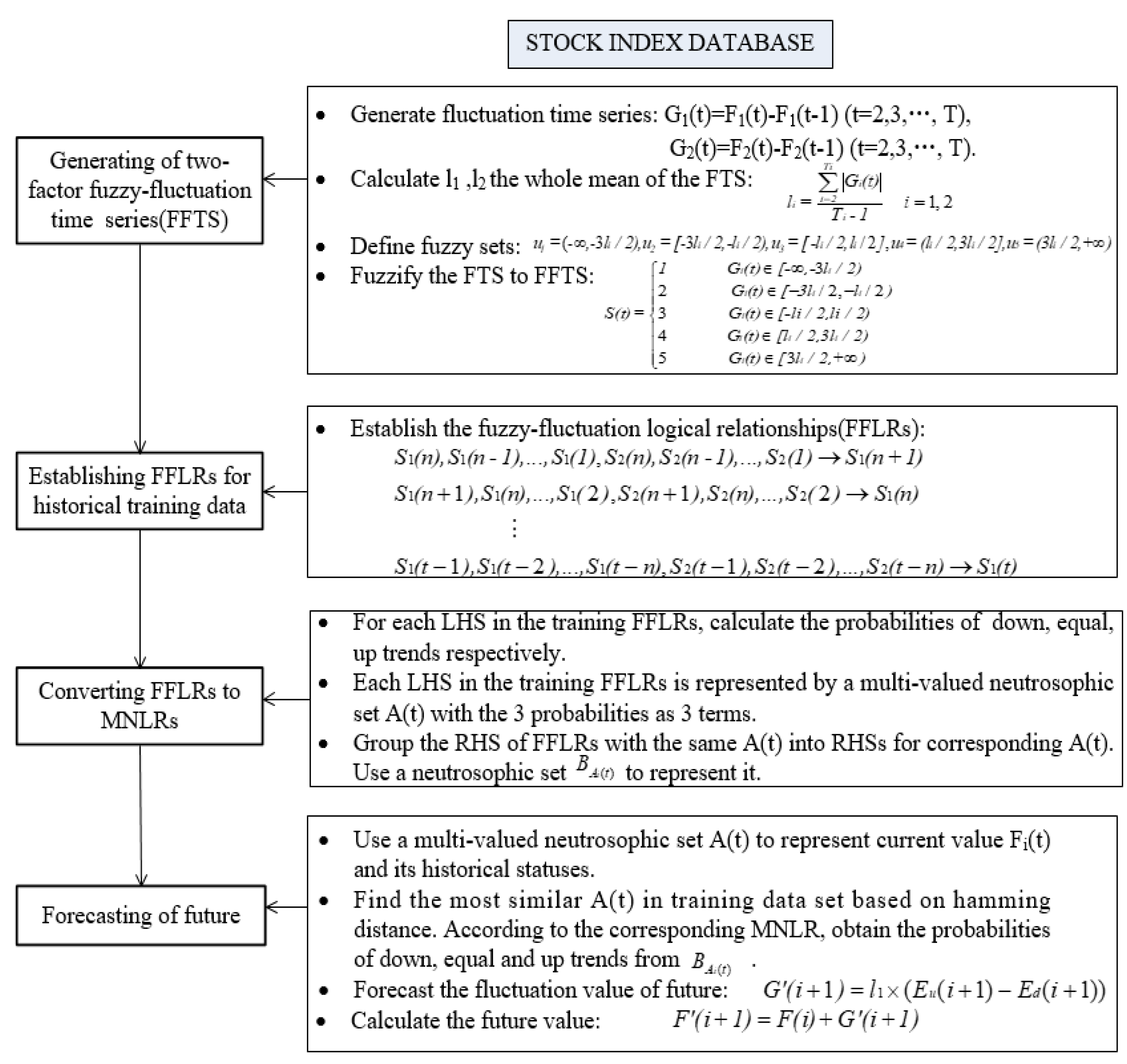
3. A Novel Forecasting Model Based on Multi-Valued Neutrosophic Logical Relationships
4. Empirical Analysis
4.1. Forecasting the Taiwan Stock Exchange Capitalization Weighted Stock Index
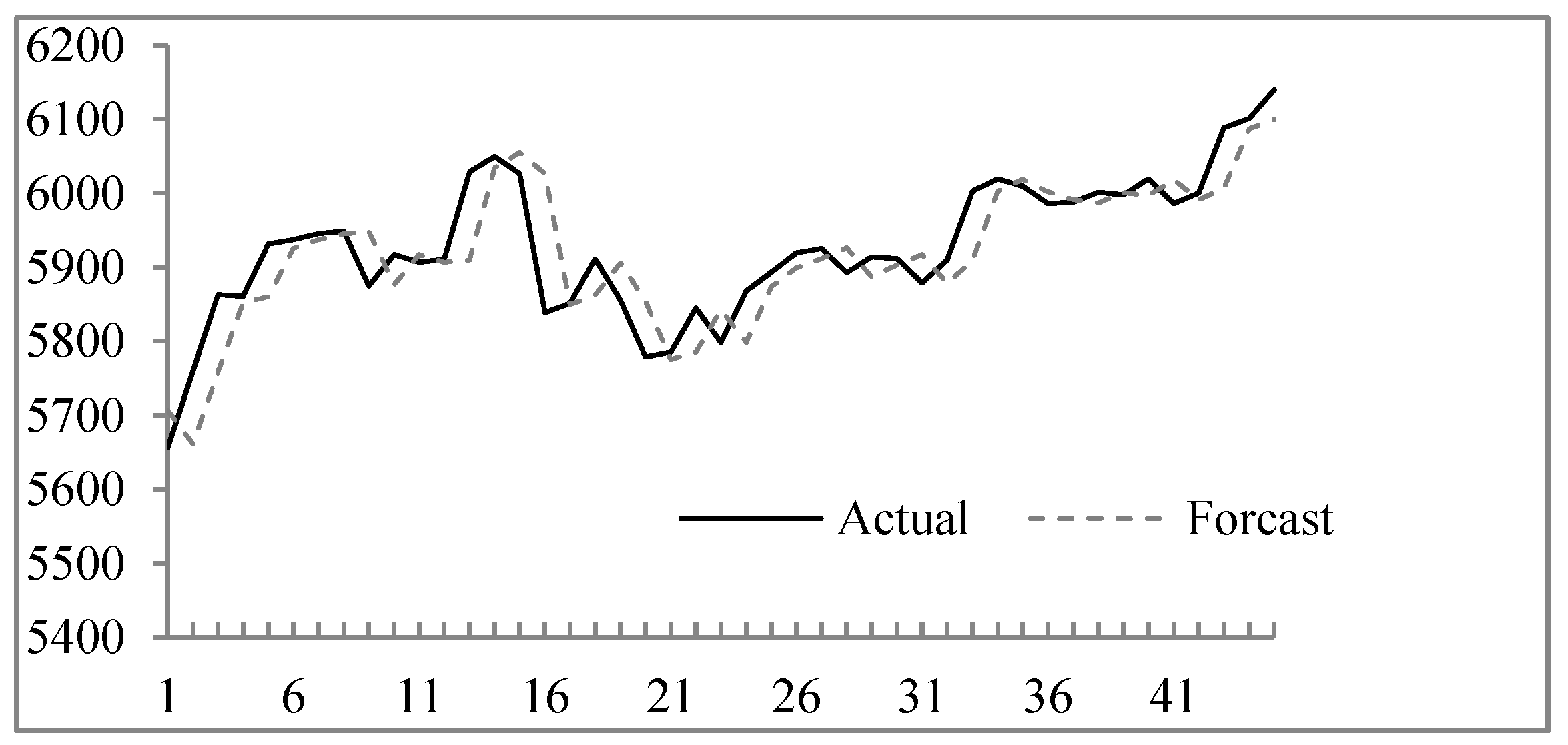
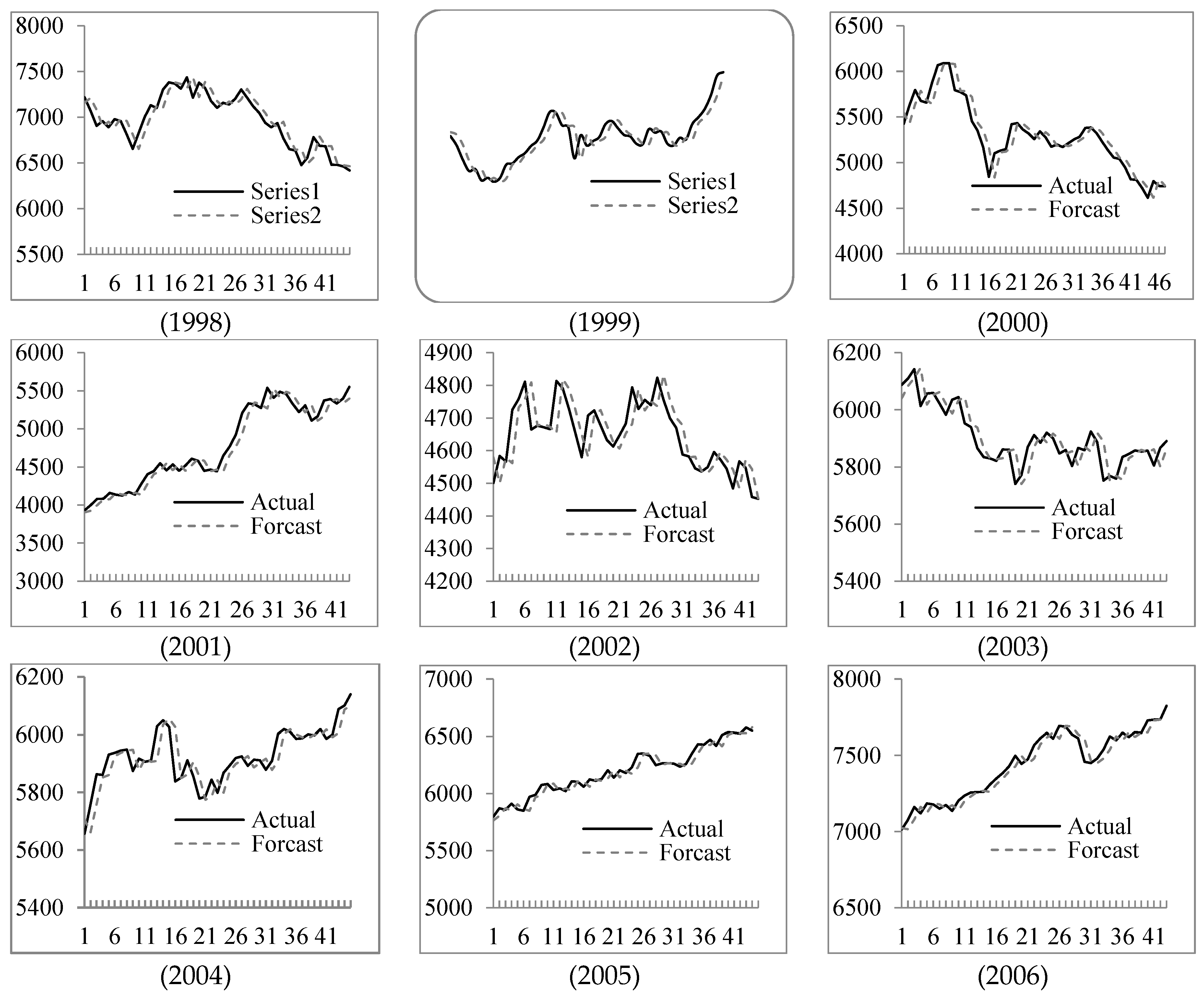
4.2. Forecasting the Shanghai Stock Exchange Composite Index
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
| Date (YYYY/MM/DD) | TAIEX | Fluctuation | Fuzzified | Date (YYYY/MM/DD) | TAIEX | Fluctuation | Fuzzified | Date (YYYY/MM/DD) | TAIEX | Fluctuation | Fuzzified |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 2004/1/2 | 6041.56 | - | - | 2004/4/16 | 6818.2 | 81.41 | 4 | 2004/7/23 | 5373.85 | -14.11 | 3 |
| 2004/1/5 | 6125.42 | 83.86 | 4 | 2004/4/19 | 6779.18 | −39.02 | 2 | 2004/7/26 | 5331.71 | −42.14 | 2 |
| 2004/1/6 | 6144.01 | 18.59 | 3 | 2004/4/20 | 6799.97 | 20.79 | 3 | 2004/7/27 | 5398.61 | 66.9 | 4 |
| 2004/1/7 | 6141.25 | −2.76 | 3 | 2004/4/21 | 6810.25 | 10.28 | 3 | 2004/7/28 | 5383.57 | −15.04 | 3 |
| 2004/1/8 | 6169.17 | 27.92 | 3 | 2004/4/22 | 6732.09 | −78.16 | 2 | 2004/7/29 | 5349.66 | −33.91 | 2 |
| 2004/1/9 | 6226.98 | 57.81 | 4 | 2004/4/23 | 6748.1 | 16.01 | 3 | 2004/7/30 | 5420.57 | 70.91 | 4 |
| 2004/1/12 | 6219.71 | −7.27 | 3 | 2004/4/26 | 6710.7 | −37.4 | 2 | 2004/8/2 | 5350.4 | −70.17 | 2 |
| 2004/1/13 | 6210.22 | −9.49 | 3 | 2004/4/27 | 6646.8 | −63.9 | 2 | 2004/8/3 | 5367.22 | 16.82 | 3 |
| 2004/1/14 | 6274.97 | 64.75 | 4 | 2004/4/28 | 6574.75 | −72.05 | 2 | 2004/8/4 | 5316.87 | −50.35 | 2 |
| 2004/1/15 | 6264.37 | −10.6 | 3 | 2004/4/29 | 6402.21 | −172.54 | 1 | 2004/8/5 | 5427.61 | 110.74 | 5 |
| 2004/1/16 | 6269.71 | 5.34 | 3 | 2004/4/30 | 6117.81 | −284.4 | 1 | 2004/8/6 | 5399.16 | −28.45 | 3 |
| 2004/1/27 | 6384.63 | 114.92 | 5 | 2004/5/3 | 6029.77 | −88.04 | 2 | 2004/8/9 | 5399.45 | 0.29 | 3 |
| 2004/1/28 | 6386.25 | 1.62 | 3 | 2004/5/4 | 6188.15 | 158.38 | 5 | 2004/8/10 | 5393.73 | −5.72 | 3 |
| 2004/1/29 | 6312.65 | −73.6 | 2 | 2004/5/5 | 5854.23 | −333.92 | 1 | 2004/8/11 | 5367.34 | −26.39 | 3 |
| 2004/1/30 | 6375.38 | 62.73 | 4 | 2004/5/6 | 5909.79 | 55.56 | 4 | 2004/8/12 | 5368.02 | 0.68 | 3 |
| 2004/2/2 | 6319.96 | −55.42 | 2 | 2004/5/7 | 6040.26 | 130.47 | 5 | 2004/8/13 | 5389.93 | 21.91 | 3 |
| 2004/2/3 | 6252.23 | −67.73 | 2 | 2004/5/10 | 5825.05 | −215.21 | 1 | 2004/8/16 | 5352.01 | −37.92 | 2 |
| 2004/2/4 | 6241.39 | −10.84 | 3 | 2004/5/11 | 5886.36 | 61.31 | 4 | 2004/8/17 | 5342.49 | −9.52 | 3 |
| 2004/2/5 | 6268.14 | 26.75 | 3 | 2004/5/12 | 5958.79 | 72.43 | 4 | 2004/8/18 | 5427.75 | 85.26 | 4 |
| 2004/2/6 | 6353.35 | 85.21 | 4 | 2004/5/13 | 5918.09 | −40.7 | 2 | 2004/8/19 | 5602.99 | 175.24 | 5 |
| 2004/2/9 | 6463.09 | 109.74 | 5 | 2004/5/14 | 5777.32 | -140.77 | 1 | 2004/8/20 | 5622.86 | 19.87 | 3 |
| 2004/2/10 | 6488.34 | 25.25 | 3 | 2004/5/17 | 5482.96 | -294.36 | 1 | 2004/8/23 | 5660.97 | 38.11 | 4 |
| 2004/2/11 | 6454.39 | −33.95 | 2 | 2004/5/18 | 5557.68 | 74.72 | 4 | 2004/8/26 | 5813.39 | 152.42 | 5 |
| 2004/2/12 | 6436.95 | −17.44 | 3 | 2004/5/19 | 5860.58 | 302.9 | 5 | 2004/8/27 | 5797.71 | −15.68 | 3 |
| 2004/2/13 | 6549.18 | 112.23 | 5 | 2004/5/20 | 5815.33 | −45.25 | 2 | 2004/8/30 | 5788.94 | −8.77 | 3 |
| 2004/2/16 | 6565.37 | 16.19 | 3 | 2004/5/21 | 5964.94 | 149.61 | 5 | 2004/8/31 | 5765.54 | −23.4 | 3 |
| 2004/2/17 | 6600.47 | 35.1 | 4 | 2004/5/24 | 5942.08 | −22.86 | 3 | 2004/9/1 | 5858.14 | 92.6 | 4 |
| 2004/2/18 | 6605.85 | 5.38 | 3 | 2004/5/25 | 5958.38 | 16.3 | 3 | 2004/9/2 | 5852.85 | −5.29 | 3 |
| 2004/2/19 | 6681.52 | 75.67 | 4 | 2004/5/26 | 6027.27 | 68.89 | 4 | 2004/9/3 | 5761.14 | −91.71 | 2 |
| 2004/2/20 | 6665.54 | −15.98 | 3 | 2004/5/27 | 6033.05 | 5.78 | 3 | 2004/9/6 | 5775.99 | 14.85 | 3 |
| 2004/2/23 | 6665.89 | 0.35 | 3 | 2004/5/28 | 6137.26 | 104.21 | 5 | 2004/9/7 | 5846.83 | 70.84 | 4 |
| 2004/2/24 | 6589.23 | −76.66 | 2 | 2004/5/31 | 5977.84 | −159.42 | 1 | 2004/9/8 | 5846.02 | −0.81 | 3 |
| 2004/2/25 | 6644.28 | 55.05 | 4 | 2004/6/1 | 5986.2 | 8.36 | 3 | 2004/9/9 | 5842.93 | −3.09 | 3 |
| 2004/2/26 | 6693.25 | 48.97 | 4 | 2004/6/2 | 5875.67 | −110.53 | 1 | 2004/9/10 | 5846.19 | 3.26 | 3 |
| 2004/2/27 | 6750.54 | 57.29 | 4 | 2004/6/3 | 5671.45 | −204.22 | 1 | 2004/9/13 | 5928.22 | 82.03 | 4 |
| 2004/3/1 | 6888.43 | 137.89 | 5 | 2004/6/4 | 5724.89 | 53.44 | 4 | 2004/9/14 | 5919.77 | −8.45 | 3 |
| 2004/3/2 | 6975.26 | 86.83 | 4 | 2004/6/7 | 5935.82 | 210.93 | 5 | 2004/9/15 | 5871.07 | −48.7 | 2 |
| 2004/3/3 | 6932.17 | −43.09 | 2 | 2004/6/8 | 5986.76 | 50.94 | 4 | 2004/9/16 | 5891.05 | 19.98 | 3 |
| 2004/3/4 | 7034.1 | 101.93 | 5 | 2004/6/9 | 5965.7 | −21.06 | 3 | 2004/9/17 | 5818.39 | −72.66 | 2 |
| 2004/3/5 | 6943.68 | −90.42 | 2 | 2004/6/10 | 5867.51 | −98.19 | 2 | 2004/9/20 | 5864.54 | 46.15 | 4 |
| 2004/3/8 | 6901.48 | −42.2 | 2 | 2004/6/14 | 5735.07 | −132.44 | 1 | 2004/9/21 | 5949.26 | 84.72 | 4 |
| 2004/3/9 | 6973.9 | 72.42 | 4 | 2004/6/15 | 5574.08 | −160.99 | 1 | 2004/9/22 | 5970.18 | 20.92 | 3 |
| 2004/3/10 | 6874.91 | −98.99 | 2 | 2004/6/16 | 5646.49 | 72.41 | 4 | 2004/9/23 | 5937.25 | −32.93 | 3 |
| 2004/3/11 | 6879.11 | 4.2 | 3 | 2004/6/17 | 5560.16 | −86.33 | 2 | 2004/9/24 | 5892.21 | −45.04 | 2 |
| 2004/3/12 | 6800.24 | −78.87 | 2 | 2004/6/18 | 5664.35 | 104.19 | 5 | 2004/9/27 | 5849.22 | −42.99 | 2 |
| 2004/3/15 | 6635.98 | −164.26 | 1 | 2004/6/21 | 5569.29 | −95.06 | 2 | 2004/9/29 | 5809.75 | −39.47 | 2 |
| 2004/3/16 | 6589.72 | −46.26 | 2 | 2004/6/22 | 5556.54 | −12.75 | 3 | 2004/9/30 | 5845.69 | 35.94 | 4 |
| 2004/3/17 | 6577.98 | −11.74 | 3 | 2004/6/23 | 5729.3 | 172.76 | 5 | 2004/10/1 | 5945.35 | 99.66 | 4 |
| 2004/3/18 | 6787.03 | 209.05 | 5 | 2004/6/24 | 5779.09 | 49.79 | 4 | 2004/10/4 | 6077.96 | 132.61 | 5 |
| 2004/3/19 | 6815.09 | 28.06 | 3 | 2004/6/25 | 5802.55 | 23.46 | 3 | 2004/10/5 | 6081.01 | 3.05 | 3 |
| 2004/3/22 | 6359.92 | −455.17 | 1 | 2004/6/28 | 5709.84 | −92.71 | 2 | 2004/10/6 | 6060.61 | −20.4 | 3 |
| 2004/3/23 | 6172.89 | −187.03 | 1 | 2004/6/29 | 5741.52 | 31.68 | 3 | 2004/10/7 | 6103 | 42.39 | 4 |
| 2004/3/24 | 6213.56 | 40.67 | 4 | 2004/6/30 | 5839.44 | 97.92 | 4 | 2004/10/8 | 6102.16 | −0.84 | 3 |
| 2004/3/25 | 6156.73 | −56.83 | 2 | 2004/7/1 | 5836.91 | −2.53 | 3 | 2004/10/11 | 6089.28 | −12.88 | 3 |
| 2004/3/26 | 6132.62 | −24.11 | 3 | 2004/7/2 | 5746.7 | −90.21 | 2 | 2004/10/12 | 5979.56 | −109.72 | 1 |
| 2004/3/29 | 6474.11 | 341.49 | 5 | 2004/7/5 | 5659.78 | −86.92 | 2 | 2004/10/13 | 5963.07 | −16.49 | 3 |
| 2004/3/30 | 6494.71 | 20.6 | 3 | 2004/7/6 | 5733.57 | 73.79 | 4 | 2004/10/14 | 5831.07 | −132 | 1 |
| 2004/3/31 | 6522.19 | 27.48 | 3 | 2004/7/7 | 5727.78 | −5.79 | 3 | 2004/10/15 | 5820.82 | −10.25 | 3 |
| 2004/4/1 | 6523.49 | 1.3 | 3 | 2004/7/8 | 5713.39 | −14.39 | 3 | 2004/10/18 | 5772.12 | −48.7 | 2 |
| 2004/4/2 | 6545.54 | 22.05 | 3 | 2004/7/9 | 5777.72 | 64.33 | 4 | 2004/10/19 | 5807.79 | 35.67 | 4 |
| 2004/4/5 | 6682.73 | 137.19 | 5 | 2004/7/12 | 5758.74 | −18.98 | 3 | 2004/10/20 | 5788.34 | −19.45 | 3 |
| 2004/4/6 | 6635.54 | −47.19 | 2 | 2004/7/13 | 5685.57 | −73.17 | 2 | 2004/10/21 | 5797.24 | 8.9 | 3 |
| 2004/4/7 | 6646.74 | 11.2 | 3 | 2004/7/14 | 5623.65 | −61.92 | 2 | 2004/10/22 | 5774.67 | −22.57 | 3 |
| 2004/4/8 | 6672.86 | 26.12 | 3 | 2004/7/15 | 5542.8 | −80.85 | 2 | 2004/10/26 | 5662.88 | −111.79 | 1 |
| 2004/4/9 | 6620.36 | −52.5 | 2 | 2004/7/16 | 5502.14 | −40.66 | 2 | 2004/10/27 | 5650.97 | −11.91 | 3 |
| 2004/4/12 | 6777.78 | 157.42 | 5 | 2004/7/19 | 5489.1 | −13.04 | 3 | 2004/10/28 | 5695.56 | 44.59 | 4 |
| 2004/4/13 | 6794.33 | 16.55 | 3 | 2004/7/20 | 5325.68 | −163.42 | 1 | 2004/10/29 | 5705.93 | 10.37 | 3 |
| 2004/4/14 | 6880.18 | 85.85 | 4 | 2004/7/21 | 5409.13 | 83.45 | 4 | ||||
| 2004/4/15 | 6736.79 | −143.39 | 1 | 2004/7/22 | 5387.96 | −21.17 | 3 |
| Date (YYYY/MM/DD) | Dow Jones | Fluctuation | Fuzzified | Date (YYYY/MM/DD) | Dow Jones | Fluctuation | Fuzzified | Date (YYYY/MM/DD) | Dow Jones | Fluctuation | Fuzzified |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 2004/1/2 | 10,409.85 | - | - | 2004/4/19 | 10,437.85 | −14.12 | 3 | 2004/7/27 | 10,085.14 | 123.22 | 5 |
| 2004/1/5 | 10,544.07 | 134.22 | 5 | 2004/4/20 | 10,314.5 | −123.35 | 1 | 2004/7/28 | 10,117.07 | 31.93 | 4 |
| 2004/1/6 | 10,538.66 | −5.41 | 3 | 2004/4/21 | 10,317.27 | 2.77 | 3 | 2004/7/29 | 10,129.24 | 12.17 | 3 |
| 2004/1/7 | 10,529.03 | −9.63 | 3 | 2004/4/22 | 10,461.2 | 143.93 | 5 | 2004/7/30 | 10,139.71 | 10.47 | 3 |
| 2004/1/8 | 10,592.44 | 63.41 | 4 | 2004/4/23 | 10,472.84 | 11.64 | 3 | 2004/8/2 | 10,179.16 | 39.45 | 4 |
| 2004/1/9 | 10,458.89 | −133.55 | 1 | 2004/4/26 | 10,444.73 | −28.11 | 2 | 2004/8/3 | 10,120.24 | −58.92 | 2 |
| 2004/1/12 | 10,485.18 | 26.29 | 3 | 2004/4/27 | 10,478.16 | 33.43 | 4 | 2004/8/4 | 10,126.51 | 6.27 | 3 |
| 2004/1/13 | 10,427.18 | −58 | 2 | 2004/4/28 | 10,342.6 | −135.56 | 1 | 2004/8/5 | 9963.03 | −163.48 | 1 |
| 2004/1/14 | 10,538.37 | 111.19 | 5 | 2004/4/29 | 10,272.27 | −70.33 | 2 | 2004/8/6 | 9815.33 | −147.7 | 1 |
| 2004/1/15 | 10,553.85 | 15.48 | 3 | 2004/4/30 | 10,225.57 | −46.7 | 2 | 2004/8/9 | 9814.66 | −0.67 | 3 |
| 2004/1/16 | 10,600.51 | 46.66 | 4 | 2004/5/3 | 10,314 | 88.43 | 5 | 2004/8/10 | 9944.67 | 130.01 | 5 |
| 2004/1/27 | 10,609.92 | 9.41 | 3 | 2004/5/4 | 10,317.2 | 3.2 | 3 | 2004/8/11 | 9938.32 | −6.35 | 3 |
| 2004/1/28 | 10,468.37 | −141.55 | 1 | 2004/5/5 | 10,310.95 | −6.25 | 3 | 2004/8/12 | 9814.59 | −123.73 | 1 |
| 2004/1/29 | 10,510.29 | 41.92 | 4 | 2004/5/6 | 10,241.26 | −69.69 | 2 | 2004/8/13 | 9825.35 | 10.76 | 3 |
| 2004/1/30 | 10,488.07 | −22.22 | 3 | 2004/5/7 | 10,117.34 | −123.92 | 1 | 2004/8/16 | 9954.55 | 129.2 | 5 |
| 2004/2/2 | 10,499.18 | 11.11 | 3 | 2004/5/10 | 9990.02 | −127.32 | 1 | 2004/8/17 | 9972.83 | 18.28 | 3 |
| 2004/2/3 | 10,505.18 | 6 | 3 | 2004/5/11 | 10,019.47 | 29.45 | 4 | 2004/8/18 | 10,083.15 | 110.32 | 5 |
| 2004/2/4 | 10,470.74 | −34.44 | 2 | 2004/5/12 | 10,045.16 | 25.69 | 3 | 2004/8/19 | 10,040.82 | −42.33 | 2 |
| 2004/2/5 | 10,495.55 | 24.81 | 3 | 2004/5/13 | 10,010.74 | −34.42 | 2 | 2004/8/20 | 10,110.14 | 69.32 | 4 |
| 2004/2/6 | 10,593.03 | 97.48 | 5 | 2004/5/14 | 10,012.87 | 2.13 | 3 | 2004/8/23 | 10,073.05 | −37.09 | 2 |
| 2004/2/9 | 10,579.03 | −14 | 3 | 2004/5/17 | 9906.91 | −105.96 | 1 | 2004/8/26 | 10,173.41 | 100.36 | 5 |
| 2004/2/10 | 10,613.85 | 34.82 | 4 | 2004/5/18 | 9968.51 | 61.6 | 4 | 2004/8/27 | 10,195.01 | 21.6 | 3 |
| 2004/2/11 | 10,737.7 | 123.85 | 5 | 2004/5/19 | 9937.71 | −30.8 | 2 | 2004/8/30 | 10,122.52 | −72.49 | 2 |
| 2004/2/12 | 10,694.07 | −43.63 | 2 | 2004/5/20 | 9937.64 | −0.07 | 3 | 2004/8/31 | 10,173.92 | 51.4 | 4 |
| 2004/2/13 | 10,627.85 | −66.22 | 2 | 2004/5/21 | 9966.74 | 29.1 | 4 | 2004/9/1 | 10,168.46 | −5.46 | 3 |
| 2004/2/16 | 10,627.85 | 0 | 3 | 2004/5/24 | 9958.43 | −8.31 | 3 | 2004/9/2 | 10,290.28 | 121.82 | 5 |
| 2004/2/17 | 10,714.88 | 87.03 | 5 | 2004/5/25 | 10,117.62 | 159.19 | 5 | 2004/9/3 | 10,260.2 | −30.08 | 2 |
| 2004/2/18 | 10,671.99 | −42.89 | 2 | 2004/5/26 | 10,109.89 | −7.73 | 3 | 2004/9/6 | 10,260.2 | 0 | 3 |
| 2004/2/19 | 10,664.73 | −7.26 | 3 | 2004/5/27 | 10,205.2 | 95.31 | 5 | 2004/9/7 | 10,341.16 | 80.96 | 4 |
| 2004/2/20 | 10,619.03 | −45.7 | 2 | 2004/5/28 | 10,188.45 | −16.75 | 3 | 2004/9/8 | 10,313.36 | −27.8 | 2 |
| 2004/2/23 | 10,609.62 | −9.41 | 3 | 2004/5/31 | 10,188.45 | 0 | 3 | 2004/9/9 | 10,289.1 | −24.26 | 3 |
| 2004/2/24 | 10,566.37 | −43.25 | 2 | 2004/6/1 | 10,202.65 | 14.2 | 3 | 2004/9/10 | 10,313.07 | 23.97 | 3 |
| 2004/2/25 | 10,601.62 | 35.25 | 4 | 2004/6/2 | 10,262.97 | 60.32 | 4 | 2004/9/13 | 10,314.76 | 1.69 | 3 |
| 2004/2/26 | 10,580.14 | −21.48 | 3 | 2004/6/3 | 10,195.91 | −67.06 | 2 | 2004/9/14 | 10,318.16 | 3.4 | 3 |
| 2004/2/27 | 10,583.92 | 3.78 | 3 | 2004/6/4 | 10,242.82 | 46.91 | 4 | 2004/9/15 | 10,231.36 | −86.8 | 1 |
| 2004/3/1 | 10,678.14 | 94.22 | 5 | 2004/6/7 | 10,391.08 | 148.26 | 5 | 2004/9/16 | 10,244.49 | 13.13 | 3 |
| 2004/3/2 | 10,591.48 | −86.66 | 1 | 2004/6/8 | 10,432.52 | 41.44 | 4 | 2004/9/17 | 10,284.46 | 39.97 | 4 |
| 2004/3/3 | 10,593.11 | 1.63 | 3 | 2004/6/9 | 10,368.44 | −64.08 | 2 | 2004/9/20 | 10,204.89 | −79.57 | 2 |
| 2004/3/4 | 10,588 | −5.11 | 3 | 2004/6/10 | 10,410.1 | 41.66 | 4 | 2004/9/21 | 10,244.93 | 40.04 | 4 |
| 2004/3/5 | 10,595.55 | 7.55 | 3 | 2004/6/14 | 10,334.73 | −75.37 | 2 | 2004/9/22 | 10,109.18 | −135.75 | 1 |
| 2004/3/8 | 10,529.48 | −66.07 | 2 | 2004/6/15 | 10,380.43 | 45.7 | 4 | 2004/9/23 | 10,038.9 | −70.28 | 2 |
| 2004/3/9 | 10,456.96 | −72.52 | 2 | 2004/6/16 | 10,379.58 | −0.85 | 3 | 2004/9/24 | 10,047.24 | 8.34 | 3 |
| 2004/3/10 | 10,296.89 | −160.07 | 1 | 2004/6/17 | 10,377.52 | −2.06 | 3 | 2004/9/27 | 9988.54 | −58.7 | 2 |
| 2004/3/11 | 10,128.38 | −168.51 | 1 | 2004/6/18 | 10,416.41 | 38.89 | 4 | 2004/9/29 | 10,136.24 | 147.7 | 5 |
| 2004/3/12 | 10,240.08 | 111.7 | 5 | 2004/6/21 | 10,371.47 | −44.94 | 2 | 2004/9/30 | 10,080.27 | −55.97 | 2 |
| 2004/3/15 | 10,102.89 | −137.19 | 1 | 2004/6/22 | 10,395.07 | 23.6 | 3 | 2004/10/1 | 10,192.65 | 112.38 | 5 |
| 2004/3/16 | 10,184.67 | 81.78 | 5 | 2004/6/23 | 10,479.57 | 84.5 | 5 | 2004/10/4 | 10,216.54 | 23.89 | 3 |
| 2004/3/17 | 10,300.3 | 115.63 | 5 | 2004/6/24 | 10,443.81 | −35.76 | 2 | 2004/10/5 | 10,177.68 | −38.86 | 2 |
| 2004/3/18 | 10,295.78 | −4.52 | 3 | 2004/6/25 | 10,371.84 | −71.97 | 2 | 2004/10/6 | 10,239.92 | 62.24 | 4 |
| 2004/3/19 | 10,186.6 | −109.18 | 1 | 2004/6/28 | 10,357.09 | −14.75 | 3 | 2004/10/7 | 10,125.4 | −114.52 | 1 |
| 2004/3/22 | 10,064.75 | −121.85 | 1 | 2004/6/29 | 10,413.43 | 56.34 | 4 | 2004/10/8 | 10,055.2 | −70.2 | 2 |
| 2004/3/23 | 10,063.64 | −1.11 | 3 | 2004/6/30 | 10,435.48 | 22.05 | 3 | 2004/10/11 | 10,081.97 | 26.77 | 3 |
| 2004/3/24 | 10,048.23 | −15.41 | 3 | 2004/7/1 | 10,334.16 | −101.32 | 1 | 2004/10/12 | 10,077.18 | −4.79 | 3 |
| 2004/3/25 | 10,218.82 | 170.59 | 5 | 2004/7/2 | 10,282.83 | −51.33 | 2 | 2004/10/13 | 10,002.33 | −74.85 | 2 |
| 2004/3/26 | 10,212.97 | −5.85 | 3 | 2004/7/5 | 10,282.83 | 0 | 3 | 2004/10/14 | 9894.45 | −107.88 | 1 |
| 2004/3/29 | 10,329.63 | 116.66 | 5 | 2004/7/6 | 10,219.34 | −63.49 | 2 | 2004/10/15 | 9933.38 | 38.93 | 4 |
| 2004/3/30 | 10,381.7 | 52.07 | 4 | 2004/7/7 | 10,240.29 | 20.95 | 3 | 2004/10/18 | 9956.32 | 22.94 | 3 |
| 2004/3/31 | 10,357.7 | −24 | 3 | 2004/7/8 | 10,171.56 | −68.73 | 2 | 2004/10/19 | 9897.62 | −58.7 | 2 |
| 2004/4/1 | 10,373.33 | 15.63 | 3 | 2004/7/9 | 10,213.22 | 41.66 | 4 | 2004/10/20 | 9886.93 | −10.69 | 3 |
| 2004/4/2 | 10,470.59 | 97.26 | 5 | 2004/7/12 | 10,238.22 | 25 | 3 | 2004/10/21 | 9865.76 | −21.17 | 3 |
| 2004/4/5 | 10,558.37 | 87.78 | 5 | 2004/7/13 | 10,247.59 | 9.37 | 3 | 2004/10/22 | 9757.81 | −107.95 | 1 |
| 2004/4/6 | 10,570.81 | 12.44 | 3 | 2004/7/14 | 10,208.8 | −38.79 | 2 | 2004/10/26 | 9888.48 | 130.67 | 5 |
| 2004/4/7 | 10,480.15 | −90.66 | 1 | 2004/7/15 | 10,163.16 | −45.64 | 2 | 2004/10/27 | 10,002.03 | 113.55 | 5 |
| 2004/4/8 | 10,442.03 | −38.12 | 2 | 2004/7/16 | 10,139.78 | −23.38 | 3 | 2004/10/28 | 10,004.54 | 2.51 | 3 |
| 2004/4/9 | 10,442.03 | 0 | 3 | 2004/7/19 | 10,094.06 | −45.72 | 2 | 2004/10/29 | 10,027.47 | 22.93 | 3 |
| 2004/4/12 | 10,515.56 | 73.53 | 4 | 2004/7/20 | 10,149.07 | 55.01 | 4 | ||||
| 2004/4/13 | 10,381.28 | −134.28 | 1 | 2004/7/21 | 10,046.13 | −102.94 | 1 | ||||
| 2004/4/14 | 10,377.95 | −3.33 | 3 | 2004/7/22 | 10,050.33 | 4.2 | 3 | ||||
| 2004/4/15 | 10,397.46 | 19.51 | 3 | 2004/7/23 | 9962.22 | −88.11 | 1 | ||||
| 2004/4/16 | 10,451.97 | 54.51 | 4 | 2004/7/26 | 9961.92 | −0.3 | 3 |
| MNLRs | MNLRs | MNLRs |
|---|---|---|
| {(0.00,0.33), (0.50,0.00), (0.50,0.67)} → (0,0.5,0.5) | {(0.00,0.33), (0.83,0.33), (0.17,0.33)} → (0,1,0) | {(0.17,0.00), (0.83,0.67), (0.00,0.33)} → (0.25,0.75,0) |
| {(0.00,0.00), (0.17,0.33), (0.83,0.67)} → (0,0.5,0.5) | {(0.17,0.17), (0.67,0.50), (0.17,0.33)} → (0,0.75,0.25) | {(0.17,0.00), (0.67,0.83), (0.17,0.17)} → (0.17,0.83,0) |
| {(0.50,0.00), (0.17,0.17), (0.33,0.83)} → (0.5,0.5,0) | {(0.17,0.17), (0.33,0.83), (0.50,0.00)} → (0.2,0.7,0.1) | {(0.33,0.00), (0.67,0.67), (0.00,0.33)} → (0.5,0.5,0) |
| {(0.00,0.00), (0.17,0.50), (0.83,0.50)} → (0.5,0.5,0) | {(0.33,0.17), (0.50,0.50), (0.17,0.33)} → (0.5,0.5,0) | {(0.00,0.33), (0.83,0.50), (0.17,0.17)} → (0.5,0.5,0) |
| {(0.00,0.00), (0.33,0.50), (0.67,0.50)} → (0,1,0) | {(0.17,0.33), (0.33,0.67), (0.50,0.00)} → (0,1,0) | {(0.17,0.17), (0.67,0.67), (0.17,0.17)} → (0.2,0.7,0.1) |
| {(0.00,0.00), (0.33,0.33), (0.67,0.67)} → (0.5,0.5,0) | {(0.50,0.00), (0.33,0.67), (0.17,0.33)} → (0,0.5,0.5) | {(0.00,0.50), (0.83,0.33), (0.17,0.17)} → (1,0,0) |
| {(0.33,0.00), (0.33,0.33), (0.33,0.67)} → (0,0,1) | {(0.50,0.00), (0.17,0.83), (0.33,0.17)} → (1,0,0) | {(0.33,0.17), (0.50,0.67), (0.17,0.17)} → (0.25,0.41,0.33) |
| {(0.17,0.00), (0.17,0.67), (0.67,0.33)} → (0,0.5,0.5) | {(0.17,0.33), (0.67,0.33), (0.17,0.33)} → (0,1,0) | {(0.50,0.17), (0.33,0.67), (0.17,0.17)} → (1,0,0) |
| {(0.50,0.17), (0.17,0.17), (0.33,0.67)} → (0.5,0.5,0) | {(0.33,0.33), (0.50,0.33), (0.17,0.33)} → (0,1,0) | {(0.50,0.17), (0.50,0.50), (0.00,0.33)} → (1,0,0) |
| {(0.17,0.17), (0.17,0.50), (0.67,0.33)} → (1,0,0) | {(0.33,0.33), (0.17,0.67), (0.50,0.00)} → (0.5,0.5,0) | {(0.67,0.17), (0.17,0.67), (0.17,0.17)} → (0,1,0) |
| {(0.00,0.17), (0.33,0.33), (0.67,0.50)} → (0,0,1) | {(0.50,0.33), (0.33,0.33), (0.17,0.33)} → (1,0,0) | {(0.50,0.33), (0.50,0.33), (0.00,0.33)} → (0,0.5,0.5) |
| {(0.00,0.33), (0.17,0.33), (0.83,0.33)} → (1,0,0) | {(0.50,0.50), (0.17,0.33), (0.33,0.17)} → (0.5,0.5,0) | {(0.17,0.50), (0.67,0.50), (0.17,0.00)} → (0.25,0.5,0.25) |
| {(0.17,0.00), (0.67,0.17), (0.17,0.83)} → (0,0.5,0.5) | {(0.33,0.17), (0.67,0.33), (0.00,0.50)} → (0,1,0) | {(0.33,0.50), (0.50,0.50), (0.17,0.00)} → (0,1,0) |
| {(0.00,0.33), (0.67,0.00), (0.33,0.67)} → (0,0,1) | {(0.00,0.00), (1.00,0.67), (0.00,0.33)} → (0,0.25,0.75) | {(0.17,0.67), (0.67,0.33), (0.17,0.00)} → (1,0,0) |
| {(0.00,0.67), (0.33,0.00), (0.67,0.33)} → (0,1,0) | {(0.33,0.00), (0.33,1.00), (0.33,0.00)} → (0,0.25,0.75) | {(0.33,0.67), (0.50,0.33), (0.17,0.00)} → (0,1,0) |
| {(0.00,0.33), (0.17,0.50), (0.83,0.17)} → (0.5,0.5,0) | {(0.17,0.17), (0.83,0.50), (0.00,0.33)} → (0,0.83,0.17) | {(0.50,0.50), (0.50,0.33), (0.00,0.17)} → (0.17,0.67,0.17) |
| {(0.00,0.17), (0.33,0.67), (0.67,0.17)} → (0.5,0.5,0) | {(0.33,0.17), (0.67,0.50), (0.00,0.33)} → (0,0.5,0.5) | {(0.67,0.33), (0.33,0.50), (0.00,0.17)} → (0,1,0) |
| {(0.00,0.17), (0.67,0.33), (0.33,0.50)} → (0,0.5,0.5) | {(0.33,0.33), (0.67,0.33), (0.00,0.33)} → (0,0.5,0.5) | {(0.00,0.17), (1.00,0.83), (0.00,0.00)} → (0.125,0.75,0.125) |
| {(0.00,0.17), (0.50,0.50), (0.50,0.33)} → (0.5,0.5,0) | {(0.50,0.17), (0.50,0.50), (0.00,0.33)} → (0,0,1) | {(0.00,0.33), (1.00,0.67), (0.00,0.00)} → (0.5,0.5,0) |
| {(0.33,0.00), (0.50,0.33), (0.17,0.67)} → (0,1,0) | {(0.33,0.17), (0.33,0.83), (0.33,0.00)} → (0,0.5,0.5) | {(0.17,0.33), (0.83,0.67), (0.00,0.00)} → (0,1,0) |
| {(0.00,0.67), (0.50,0.00), (0.50,0.33)} → (1,0,0) | {(0.17,0.67), (0.50,0.33), (0.33,0.00)} → (0,1,0) | {(0.17,0.50), (0.83,0.50), (0.00,0.00)} → (0.67,0.17,0.17) |
| {(0.50,0.00), (0.17,0.50), (0.33,0.50)} → (0,0,1) | {(0.67,0.33), (0.33,0.33), (0.00,0.33)} → (0,0.5,0.5) | {(0.50,0.50), (0.50,0.50), (0.00,0.00)} → (0,0.5,0.5) |
| {(0.17,0.17), (0.17,0.67), (0.67,0.17)} → (0,0.5,0.5) | {(0.00,0.00), (0.67,0.67), (0.33,0.33)} → (0,0.25,0.75) | {(0.67,0.67), (0.33,0.33), (0.00,0.00)} → (0,1,0) |
| {(0.17,0.17), (0.50,0.33), (0.33,0.50)} → (0,1,0) | {(0.00,0.33), (0.50,0.50), (0.50,0.17)} → (0.5,0.5,0) | {(0.00,0.67), (0.67,0.00), (0.33,0.33)} → (0.5,0.5,0) |
| {(0.17,0.33), (0.17,0.50), (0.67,0.17)} → (1,0,0) | {(0.33,0.00), (0.33,0.83), (0.33,0.17)} → (0.5,0.5,0) | {(0.17,0.00), (0.50,0.33), (0.33,0.67)} → (0,1,0) |
| {(0.17,0.00), (0.50,0.67), (0.33,0.33)} → (0.25,0.625,0.125) | {(0.00,0.33), (0.67,0.50), (0.33,0.17)} → (0,0.5,0.5) | {(0.00,0.17), (0.50,0.33), (0.50,0.50)} → (0,0,1) |
| {(0.00,0.17), (0.33,0.83), (0.67,0.00)} → (0,0.25,0.75) | {(0.00,0.17), (0.67,0.67), (0.33,0.17)} → (0.25,0.625,0.125) | {(0.00,0.17), (0.50,0.50), (0.50,0.33)} → (0,0,1) |
| {(0.33,0.00), (0.67,0.33), (0.00,0.67)} → (0,1,0) | {(0.33,0.33), (0.50,0.33), (0.17,0.33)} → (0,1,0) | {(0.00,0.00), (0.67,0.50), (0.33,0.50)} → (0.5,0.5,0) |
| {(0.00,0.33), (0.67,0.33), (0.33,0.33)} → (0,1,0) | {(0.00,0.00), (0.83,0.83), (0.17,0.17)} → (0.5,0.5,0) | {(0.00,0.00), (0.83,0.50), (0.17,0.50)} → (0.33,0.33,0.33) |
| {(0.17,0.17), (0.50,0.50), (0.33,0.33)} → (0,1,0) | {(0.33,0.33), (0.50,0.50), (0.17,0.17)} → (0,1,0) | {(0.17,0.00), (0.33,0.83), (0.50,0.17)} → (0,0,1) |
| {(0.00,0.67), (0.67,0.00), (0.33,0.33)} → (0.5,0.5,0) | {(0.33,0.67), (0.50,0.17), (0.17,0.17)} → (1,0,0) | {(0.17,0.00), (0.67,0.50), (0.17,0.50)} → (0.25,0.5,0.25) |
| {(0.33,0.17), (0.33,0.50), (0.33,0.33)} → (0,0.5,0.5) | {(0.17,0.00), (0.50,1.00), (0.33,0.00)} → (0,1,0) | {(0.33,0.00), (0.50,0.50), (0.17,0.50)} → (0,1,0) |
| {(0.17,0.00), (0.50,0.33), (0.33,0.67)} → (0,1,0) | {(0.17,0.00), (0.83,0.67), (0.00,0.33)} → (0.25,0.75,0) | {(0.33,0.33), (0.17,0.50), (0.50,0.17)} → (0,0,1) |
| {(0.00,0.17), (0.50,0.33), (0.50,0.50)} → (0,0,1) | {(0.17,0.00), (0.67,0.83), (0.17,0.17)} → (0.17,0.83,0) | {(0.00,0.00), (0.83,0.67), (0.17,0.33)} → (0.125,0.5625,0.3125) |
| {(0.00,0.17), (0.50,0.50), (0.50,0.33)} → (0,0,1) | {(0.33,0.00), (0.67,0.67), (0.00,0.33)} → (0.5,0.5,0) | {(0.17,0.00), (0.83,0.50), (0.00,0.50)} → (0.25,0.25,0.5) |
| {(0.00,0.00), (0.67,0.50), (0.33,0.50)} → (0.5,0.5,0) | {(0.00,0.33), (0.83,0.50), (0.17,0.17)} → (0.5,0.5,0) | {(0.00,0.00), (1.00,0.83), (0.00,0.17)} → (0.5,0.5,0) |
| {(0.00,0.00), (0.83,0.50), (0.17,0.50)} → (0.33,0.33,0.33) | {(0.17,0.17), (0.67,0.67), (0.17,0.17)} → (0.2,0.7,0.1) | {(0.17,0.00), (0.83,0.83), (0.00,0.17)} → (0,0.75,0.25) |
| {(0.17,0.00), (0.33,0.83), (0.50,0.17)} → (0,0,1) | {(0.00,0.50), (0.83,0.33), (0.17,0.17)} → (1,0,0) | {(0.00,0.17), (1.00,0.67), (0.00,0.17)} → (0.5,0.5,0) |
| {(0.17,0.00), (0.67,0.50), (0.17,0.50)} → (0.25,0.5,0.25) | {(0.33,0.17), (0.50,0.67), (0.17,0.17)} → (0.25,0.41,0.33) | {(0.00,0.33), (0.83,0.67), (0.17,0.00)} → (0.25,0.5,0.25) |
| {(0.33,0.00), (0.50,0.50), (0.17,0.50)} → (0,1,0) | {(0.50,0.17), (0.33,0.67), (0.17,0.17)} → (1,0,0) | {(0.17,0.17), (0.67,0.83), (0.17,0.00)} → (0,0.81,0.19) |
| {(0.33,0.33), (0.17,0.50), (0.50,0.17)} → (0,0,1) | {(0.50,0.17), (0.50,0.50), (0.00,0.33)} → (1,0,0) | {(0.17,0.33), (0.83,0.50), (0.00,0.17)} → (0.375,0.625,0) |
| {(0.00,0.00), (0.83,0.67), (0.17,0.33)} → (0.125,0.5625,0.3125) | {(0.67,0.17), (0.17,0.67), (0.17,0.17)} → (0,1,0) | {(0.33,0.17), (0.50,0.83), (0.17,0.00)} → (0,1,0) |
| {(0.17,0.00), (0.83,0.50), (0.00,0.50)} → (0.25,0.25,0.5) | {(0.50,0.33), (0.50,0.33), (0.00,0.33)} → (0,0.5,0.5) | {(0.33,0.17), (0.67,0.67), (0.00,0.17)} → (0.75,0.25,0) |
| {(0.00,0.33), (0.50,0.00), (0.50,0.67)} → (0,0.5,0.5) | {(0.00,0.33), (0.83,0.33), (0.17,0.33)} → (0,1,0) | {(0.33,0.33), (0.67,0.50), (0.00,0.17)} → (0,1,0) |
| {(0.00,0.00), (0.17,0.33), (0.83,0.67)} → (0,0.5,0.5) | {(0.17,0.17), (0.67,0.50), (0.17,0.33)} → (0,0.75,0.25) | {(0.50,0.17), (0.33,0.83), (0.17,0.00)} → (0,0.5,0.5) |
| {(0.50,0.00), (0.17,0.17), (0.33,0.83)} → (0.5,0.5,0) | {(0.17,0.17), (0.33,0.83), (0.50,0.00)} → (0.2,0.7,0.1) | {(0.50,0.33), (0.50,0.67), (0.00,0.00)} → (0.1,0.7,0.2) |
| {(0.00,0.00), (0.17,0.50), (0.83,0.50)} → (0.5,0.5,0) | {(0.33,0.17), (0.50,0.50), (0.17,0.33)} → (0.5,0.5,0) | {(0.17,0.67), (0.83,0.33), (0.00,0.00)} → (1,0,0) |
| {(0.00,0.00), (0.33,0.50), (0.67,0.50)} → (0,1,0) | {(0.17,0.33), (0.33,0.67), (0.50,0.00)} → (0,1,0) | {(0.33,0.67), (0.67,0.33), (0.00,0.00)} → (0,0.5,0.5) |
| {(0.33,0.00), (0.33,0.33), (0.33,0.67)} → (0,0,1) | {(0.50,0.00), (0.17,0.83), (0.33,0.17)} → (1,0,0) | {(0.17,0.00), (0.50,1.00), (0.33,0.00)} → (0,1,0) |
| {(0.17,0.00), (0.17,0.67), (0.67,0.33)} → (0,0.5,0.5) | {(0.17,0.33), (0.67,0.33), (0.17,0.33)} → (0,1,0) | {(0.50,0.17), (0.17,0.33), (0.33,0.50)} → (0,0.5,0.5) |
| {(0.50,0.17), (0.17,0.17), (0.33,0.67)} → (0.5,0.5,0) | {(0.33,0.33), (0.50,0.33), (0.17,0.33)} → (0,1,0) | {(0.17,0.33), (0.50,0.33), (0.33,0.33)} → (0.5,0.5,0) |
| {(0.17,0.17), (0.17,0.50), (0.67,0.33)} → (1,0,0) | {(0.33,0.33), (0.17,0.67), (0.50,0.00)} → (0.5,0.5,0) | {(0.33,0.17), (0.33,0.50), (0.33,0.33)} → (0,0.5,0.5) |
| {(0.00,0.17), (0.33,0.33), (0.67,0.50)} → (0,0,1) | {(0.50,0.33), (0.33,0.33), (0.17,0.33)} → (1,0,0) | {(0.00,0.00), (0.33,0.33), (0.67,0.67)} → (0.5,0.5,0) |
| {(0.00,0.33), (0.17,0.33), (0.83,0.33)} → (1,0,0) | {(0.50,0.50), (0.17,0.33), (0.33,0.17)} → (0.5,0.5,0) | {(0.50,0.00), (0.33,0.67), (0.17,0.33)} → (0,0.5,0.5) |
| {(0.17,0.00), (0.67,0.17), (0.17,0.83)} → (0,0.5,0.5) | {(0.33,0.17), (0.67,0.33), (0.00,0.50)} → (0,1,0) | {(0.33,0.67), (0.50,0.17), (0.17,0.17)} → (1,0,0) |
| {(0.00,0.33), (0.67,0.00), (0.33,0.67)} → (0,0,1) | {(0.00,0.00), (1.00,0.67), (0.00,0.33)} → (0,0.25,0.75) | |
| {(0.00,0.67), (0.33,0.00), (0.67,0.33)} → (0,1,0) | {(0.33,0.00), (0.33,1.00), (0.33,0.00)} → (0,0.25,0.75) | |
| {(0.00,0.33), (0.17,0.50), (0.83,0.17)} → (0.5,0.5,0) | {(0.17,0.17), (0.83,0.50), (0.00,0.33)} → (0,0.83,0.17) | |
| {(0.00,0.17), (0.33,0.67), (0.67,0.17)} → (0.5,0.5,0) | {(0.33,0.17), (0.67,0.50), (0.00,0.33)} → (0,0.5,0.5) | |
| {(0.00,0.17), (0.67,0.33), (0.33,0.50)} → (0,0.5,0.5) | {(0.33,0.33), (0.67,0.33), (0.00,0.33)} → (0,0.5,0.5) | |
| {(0.00,0.17), (0.50,0.50), (0.50,0.33)} → (0.5,0.5,0) | {(0.50,0.17), (0.50,0.50), (0.00,0.33)} → (0,0,1) | |
| {(0.33,0.00), (0.50,0.33), (0.17,0.67)} → (0,1,0) | {(0.33,0.17), (0.33,0.83), (0.33,0.00)} → (0,0.5,0.5) | |
| {(0.00,0.67), (0.50,0.00), (0.50,0.33)} → (1,0,0) | {(0.17,0.67), (0.50,0.33), (0.33,0.00)} → (0,1,0) | |
| {(0.50,0.00), (0.17,0.50), (0.33,0.50)} → (0,0,1) | {(0.67,0.33), (0.33,0.33), (0.00,0.33)} → (0,0.5,0.5) | |
| {(0.17,0.17), (0.17,0.67), (0.67,0.17)} → (0,0.5,0.5) | {(0.00,0.00), (0.67,0.67), (0.33,0.33)} → (0,0.25,0.75) | |
| {(0.17,0.17), (0.50,0.33), (0.33,0.50)} → (0,1,0) | {(0.00,0.33), (0.50,0.50), (0.50,0.17)} → (0.5,0.5,0) | |
| {(0.50,0.17), (0.17,0.33), (0.33,0.50)} → (0,0.5,0.5) | {(0.17,0.33), (0.50,0.33), (0.33,0.33)} → (0.5,0.5,0) | |
| {(0.17,0.33), (0.17,0.50), (0.67,0.17)} → (1,0,0) | {(0.33,0.00), (0.33,0.83), (0.33,0.17)} → (0.5,0.5,0) | |
| {(0.17,0.00), (0.50,0.67), (0.33,0.33)} → (0.25,0.625,0.125) | {(0.00,0.33), (0.67,0.50), (0.33,0.17)} → (0,0.5,0.5) | |
| {(0.00,0.17), (0.33,0.83), (0.67,0.00)} → (0,0.25,0.75) | {(0.00,0.17), (0.67,0.67), (0.33,0.17)} → (0.25,0.625,0.125) | |
| {(0.33,0.00), (0.67,0.33), (0.00,0.67)} → (0,1,0) | {(0.33,0.33), (0.50,0.33), (0.17,0.33)} → (0,1,0) | |
| {(0.00,0.33), (0.67,0.33), (0.33,0.33)} → (0,1,0) | {(0.00,0.00), (0.83,0.83), (0.17,0.17)} → (0.5,0.5,0) | |
| {(0.17,0.17), (0.50,0.50), (0.33,0.33)} → (0,1,0) | {(0.33,0.33), (0.50,0.50), (0.17,0.17)} → (0,1,0) |
References
- Robinson, P.M. Time Series with Long Memory; Oxford University Press: New York, NY, USA, 2003. [Google Scholar]
- Brown, R.G. Smoothing, Forecasting and Prediction of Discrete Time Series; Courier Corporation: Chelmsford, MA, USA, 2004; p. 127. [Google Scholar]
- Box, G.E.P.; Jenkins, G.M.; Reinsel, G.C. Time Series Analysis: Forecasting and Control (Revised Edition). J. Mark. Res. 1994, 14, 199–201. [Google Scholar]
- Song, Q.; Chissom, B.S. Fuzzy time series and its models. Fuzzy Sets Syst. 1993, 3, 269–277. [Google Scholar] [CrossRef]
- Song, Q.; Chissom, B.S. Forecasting enrollments with fuzzy time series—Part I. Fuzzy Sets Syst. 1993, 54, 1–9. [Google Scholar] [CrossRef]
- Schuster, A. On the Periodicities of Sunspots. Philos. Trans. R. Soc. Lond. 1906, 206, 69–100. [Google Scholar] [CrossRef]
- Yule, G.U. On a Method of Investigating Periodicities in Disturbed Series, with Special Reference to Wolfer’s Sunspot Numbers. Philos. Trans. R. Soc. Lond. 1927, 226, 267–298. [Google Scholar] [CrossRef]
- Chen, S.-M. Forecasting enrollments based on fuzzy time series. Fuzzy Sets Syst. 1996, 81, 311–319. [Google Scholar] [CrossRef]
- Chen, S.-M. Forecasting enrollments based on high-order fuzzy time series. J. Cybern. 2002, 33, 1–16. [Google Scholar] [CrossRef]
- Chen, S.-M.; Chung, N.Y. Forecasting enrollments using high-order fuzzy time series and genetic algorithms. Int. J. Intell. Syst. 2006, 21, 485–501. [Google Scholar] [CrossRef]
- Aladag, C.H.; Yolcu, U.; Egrioglu, E. A high order fuzzy time series forecasting model based on adaptive expectation and artificial neural networks. Math. Comput. Simul. 2010, 81, 875–882. [Google Scholar] [CrossRef]
- Chen, M.Y. A high-order fuzzy time series forecasting model for internet stock trading. Future Gener. Comput. Syst. 2014, 37, 461–467. [Google Scholar] [CrossRef]
- Zhang, R.; Ashuri, B.; Deng, Y. A novel method for forecasting time series based on fuzzy logic and visibility graph. Adv. Data Anal. Classif. 2017, 11, 759–783. [Google Scholar] [CrossRef]
- Zhang, R.; Ashuri, B.; Shyr, Y.; Deng, Y. Forecasting Construction Cost Index based on visibility graph: A network approach. Physica A 2018, 493, 239–252. [Google Scholar] [CrossRef]
- Chen, S.-M.; Hwang, J.R. Temperature prediction using fuzzy time series. IEEE Trans. Syst. Man Cybern. 2000, 30, 263–275. [Google Scholar] [CrossRef] [PubMed]
- Lee, L.W.; Wang, L.H.; Chen, S.-M.; Leu, Y.H. Handling forecasting problems based on two-factors high-order fuzzy time series. IEEE Trans. Fuzzy Syst. 2006, 14, 468–477. [Google Scholar] [CrossRef]
- Guan, S.; Zhao, A. A Two-Factor Autoregressive Moving Average Model Based on Fuzzy Fluctuation Logical Relationships. Symmetry 2017, 9, 207. [Google Scholar] [CrossRef]
- Wang, N.Y.; Chen, S.-M. Temperature prediction and TAIFEX forecasting based on automatic clustering techniques and two-factors high-order fuzzy time series. Exp. Syst. Appl. 2009, 36, 2143–2154. [Google Scholar] [CrossRef]
- Singh, P.; Borah, B. An effective neural network and fuzzy time series-based hybridized model to handle forecasting problems of two factors. Knowl. Inf. Syst. 2014, 38, 669–690. [Google Scholar] [CrossRef]
- Smarandache, F. A Unifying Field in Logics: Neutrosophic Logic. Neutrosophy, Neutrosophic Set, Neutrosophic Probability, 3rd ed; American Research Press: Rehoboth, DE, USA, 1999; Volume 8, pp. 489–503. [Google Scholar]
- Haibin, W.A.; Smarandache, F.; Zhang, Y.; Sunderraman, R. Single Valued Neutrosophic Sets. Available online: https://www.researchgate.net/publication/262047656_Single_valued_neutrosophic_sets (accessed on 20 June 2018).
- Wang, H.; Madiraju, P.; Zhang, Y.; Sunderraman, R. Interval Neutrosophic Sets. Mathematics 2004, 1, 274–277. [Google Scholar]
- Ye, J. Similarity measures between interval neutrosophic sets and their applications in multicriteria decision-making. J. Intell. Fuzzy Syst. 2014, 26, 165–172. [Google Scholar]
- Wang, J.Q.; Yang, Y.; Li, L. Multi-criteria decision-making method based on single-valued neutrosophic linguistic Maclaurin symmetric mean operators. Neural Comput. Appl. 2016, 4, 1–19. [Google Scholar] [CrossRef]
- Wang, J.Q.; Li, X.E. TODIM method with multi-valued neutrosophicsets. Control Decis. 2015, 30, 1139–1142. [Google Scholar]
- Peng, H.G.; Zhang, H.Y.; Wang, J.Q. Probability multi-valued neutrosophic sets and its application in multi-criteria group decision-making problems. Neural Comput. Appl. 2016, 1–21. [Google Scholar] [CrossRef]
- Peng, J.J.; Wang, J.Q.; Yang, W.E. A multi-valued neutrosophic qualitative flexible approach based on likelihood for multi-criteria decision-making problems. Int. J. Syst. Sci. 2017, 48, 425–435. [Google Scholar] [CrossRef]
- Ji, P.; Zhang, H.Y.; Wang, J.Q. A projection-based TODIM method under multi-valued neutrosophic environments and its application in personnel selection. Neural Comput. Appl. 2017, 29, 1–14. [Google Scholar] [CrossRef]
- Liang, R.X.; Wang, J.Q.; Zhang, H.Y. A multi-criteria decision-making method based on single-valued trapezoidal neutrosophic preference relations with complete weight information. Neural Comput. Appl. 2017, 1–16. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, H.Y.; Wang, J.Q. Frank Choquet Bonferroni Mean Operators of Bipolar Neutrosophic Sets and Their Application to Multi-criteria Decision-Making Problems. Int. J. Fuzzy Syst. 2018, 20, 13–28. [Google Scholar] [CrossRef]
- Garg, H.; Nancy. An improved score function for ranking neutrosophic sets and its application to decision-making process. Int. J. Uncertain. Quantif. 2016, 6, 377–385. [Google Scholar]
- Garg, H.; Nancy. Non-linear programming method for multi-criteria decision making problems under interval neutrosophic set environment. Appl. Intell. 2017, 2, 1–15. [Google Scholar] [CrossRef]
- Garg, H.; Nancy. Linguistic single-valued neutrosophic prioritized aggregation operators and their applications to multiple-attribute group decision-making. J. Ambient Intell. Humaniz. Comput. 2018, 1, 1–23. [Google Scholar] [CrossRef]
- Majumdar, P.; Samanta, S.K. On similarity and entropy of neutrosophic sets. J. Intell. Fuzzy Syst. 2013, 26, 1245–1252. [Google Scholar]
- Şahin, R. Cross-entropy measure on interval neutrosophic sets and its applications in multicriteria decision making. Neural Comput. Appl. 2015, 28, 1177–1187. [Google Scholar] [CrossRef]
- Ye, J. Improved cosine similarity measures of simplified neutrosophic sets for medical diagnoses. Artif. Intell. Med. 2015, 63, 171–179. [Google Scholar] [CrossRef] [PubMed]
- Garg, H.; Nancy. Some New Biparametric Distance Measures on Single-Valued Neutrosophic Sets with Applications to Pattern Recognition and Medical Diagnosis. Information 2018, 8, 162. [Google Scholar] [CrossRef]
- Guan, H.; Guan, S.; Zhao, A. Forecasting Model Based on Neutrosophic Logical Relationship and Jaccard Similarity. Symmetry 2017, 9, 191. [Google Scholar] [CrossRef]
- TAIEX Data Resources. Available online: https://wenku.baidu.com/view/02bd0b43e2bd960590c677ba.html (accessed on 20 June 2018).
- Dow Jones Data Resources. Available online: https://wenku.baidu.com/view/6c4cf886767f5acfa0c7cd26.html (accessed on 20 June 2018).
- Huarng, K.; Yu, T.H.K.; Hsu, Y.W. A multivariate heuristic model for fuzzy time-series forecasting. IEEE Trans. Syst. Man Cybern. B 2007, 37, 836–846. [Google Scholar] [CrossRef]
- Chen, S.-M.; Kao, P.Y. TAIEX forecasting based on fuzzy time series, particle swarm optimization techniques and support vector machines. Inf. Sci. 2013, 247, 62–71. [Google Scholar] [CrossRef]
- Cheng, S.H.; Chen, S.-M.; Jian, W.S. Fuzzy time series forecasting based on fuzzy logical relationships and similarity measures. Inf. Sci. 2016, 327, 272–287. [Google Scholar] [CrossRef]
- Chen, S.-M.; Manalu, G.M.T.; Pan, J.S.; Liu, H.C. Fuzzy forecasting based on two-factors second-order fuzzy-trend logical relationship groups and particle swarm optimization techniques. IEEE Trans. Cybern. 2013, 43, 1102–1117. [Google Scholar] [CrossRef] [PubMed]
- Chen, S.-M.; Chang, Y.C. Multi-variable fuzzy forecasting based on fuzzy clustering and fuzzy rule in terpolation techniques. Inf. Sci. 2010, 180, 4772–4783. [Google Scholar] [CrossRef]
- Chen, S.-M.; Chen, C.D. TAIEX forecasting based on fuzzy time series and fuzzy variation groups. IEEE Trans. Fuzzy Syst. 2011, 19, 1–12. [Google Scholar] [CrossRef]
- Yu, T.H.K.; Huarng, K.H. A neural network-based fuzzy time series model to improve forecasting. Expert Syst. Appl. 2010, 37, 3366–3372. [Google Scholar] [CrossRef]
- SHSECI Data Resources. Available online: http://vdisk.weibo.com/s/zvrAajMjlVK6F (accessed on 20 June 2018).

| Date (YYYY/MM/DD) | Actual | Forecast | (Forecast − Actual)2 | Date (YYYY/MM/DD) | Actual | Forecast | (Forecast − Actual)2 |
|---|---|---|---|---|---|---|---|
| 2004/11/1 | 5656.17 | 5708.72 | 2761.50 | 2004/12/2 | 5867.95 | 5798.62 | 4806.65 |
| 2004/11/2 | 5759.61 | 5678.51 | 6576.48 | 2004/12/3 | 5893.27 | 5867.95 | 641.10 |
| 2004/11/3 | 5862.85 | 5781.95 | 9575.93 | 2004/12/6 | 5919.17 | 5926.79 | 58.01 |
| 2004/11/4 | 5860.73 | 5862.85 | 10,658.50 | 2004/12/7 | 5925.28 | 5908.00 | 298.68 |
| 2004/11/5 | 5931.31 | 5860.73 | 4981.54 | 2004/12/8 | 5892.51 | 5925.28 | 1073.87 |
| 2004/11/8 | 5937.46 | 5908.97 | 811.94 | 2004/12/9 | 5913.97 | 5896.98 | 288.70 |
| 2004/11/9 | 5945.2 | 5959.80 | 213.29 | 2004/12/10 | 5911.63 | 5880.45 | 971.99 |
| 2004/11/10 | 5948.49 | 5945.20 | 10.82 | 2004/12/13 | 5878.89 | 5933.97 | 3034.30 |
| 2004/11/11 | 5874.52 | 5970.83 | 0.25 | 2004/12/14 | 5909.65 | 5901.23 | 70.82 |
| 2004/11/12 | 5917.16 | 5874.52 | 1818.17 | 2004/12/15 | 6002.58 | 5931.99 | 4982.31 |
| 2004/11/15 | 5906.69 | 5917.16 | 109.62 | 2004/12/16 | 6019.23 | 6024.92 | 32.43 |
| 2004/11/16 | 5910.85 | 5906.69 | 17.31 | 2004/12/17 | 6009.32 | 6019.23 | 98.21 |
| 2004/11/17 | 6028.68 | 5902.36 | 15,956.97 | 2004/12/20 | 5985.94 | 5998.15 | 149.03 |
| 2004/11/18 | 6049.49 | 6062.20 | 161.46 | 2004/12/21 | 5987.85 | 6008.28 | 417.57 |
| 2004/11/19 | 6026.55 | 6083.01 | 3187.36 | 2004/12/22 | 6001.52 | 6010.19 | 13.49 |
| 2004/11/22 | 5838.42 | 6004.21 | 27,484.83 | 2004/12/23 | 5997.67 | 6023.86 | 686.15 |
| 2004/11/23 | 5851.1 | 5844.01 | 50.32 | 2004/12/24 | 6019.42 | 6020.01 | 0.35 |
| 2004/11/24 | 5911.31 | 5856.69 | 2983.77 | 2004/12/27 | 5985.94 | 6019.42 | 1120.91 |
| 2004/11/25 | 5855.24 | 5888.97 | 1137.41 | 2004/12/28 | 6000.57 | 6008.28 | 59.51 |
| 2004/11/26 | 5778.65 | 5846.75 | 4637.49 | 2004/12/29 | 6088.49 | 6022.91 | 4300.15 |
| 2004/11/29 | 5785.26. | 5771.05 | 201.84 | 2004/12/30 | 6100.86 | 6080.00 | 435.18 |
| 2004/11/30 | 5844.76 | 5776.88 | 4607.58 | 2004/12/31 | 6139.69 | 6092.37 | 2239.27 |
| 2004/12/1 | 5798.62 | 5850.35 | 2675.59 | RMSE | 53.01 | ||
| n | 1 | 3 | 5 | 7 | 9 |
|---|---|---|---|---|---|
| RMSE | 54.65 | 53.01 | 55.60 | 55.06 | 54.43 |
| Year | 1998 | 1999 | 2000 | 2001 | 2002 | 2003 | 2004 | 2005 | 2006 |
|---|---|---|---|---|---|---|---|---|---|
| RMSE | 115.45 | 105.49 | 129.11 | 113.69 | 67.12 | 53.6 | 53.01 | 53.49 | 51.90 |
| Methods | RMSE |
|---|---|
| Huarng et al.’s method [41] | 73.57 |
| Chen and Kao’s method [42] | 58.17 |
| Cheng et al.’s method [43] | 54.24 |
| Chen et al.’s method [44] | 56.16 |
| Chen and Chang’s method [45] | 60.48 |
| Chen and Chen’s method [46] | 61.94 |
| Yu and Huarng’s method [47] | 55.91 |
| The proposed method | 53.01 |
| Year | 2004 | 2005 | 2006 | 2007 | 2008 | 2009 | 2010 | 2011 | 2012 | 2013 | 2014 | 2015 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| RMSE | 13.54 | 9.03 | 37.68 | 108.17 | 50.88 | 50.22 | 46.08 | 28.1 | 25.36 | 20.19 | 52.79 | 55.80 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guan, H.; He, J.; Zhao, A.; Dai, Z.; Guan, S. A Forecasting Model Based on Multi-Valued Neutrosophic Sets and Two-Factor, Third-Order Fuzzy Fluctuation Logical Relationships. Symmetry 2018, 10, 245. https://doi.org/10.3390/sym10070245
Guan H, He J, Zhao A, Dai Z, Guan S. A Forecasting Model Based on Multi-Valued Neutrosophic Sets and Two-Factor, Third-Order Fuzzy Fluctuation Logical Relationships. Symmetry. 2018; 10(7):245. https://doi.org/10.3390/sym10070245
Chicago/Turabian StyleGuan, Hongjun, Jie He, Aiwu Zhao, Zongli Dai, and Shuang Guan. 2018. "A Forecasting Model Based on Multi-Valued Neutrosophic Sets and Two-Factor, Third-Order Fuzzy Fluctuation Logical Relationships" Symmetry 10, no. 7: 245. https://doi.org/10.3390/sym10070245
APA StyleGuan, H., He, J., Zhao, A., Dai, Z., & Guan, S. (2018). A Forecasting Model Based on Multi-Valued Neutrosophic Sets and Two-Factor, Third-Order Fuzzy Fluctuation Logical Relationships. Symmetry, 10(7), 245. https://doi.org/10.3390/sym10070245





