Three Classes of Fractional Oscillators
Abstract
1. Introduction
- Establishing three equivalent 2-order differential equations respectively corresponding to three classes of fractional oscillators.
- Presenting the analytical representations, in the closed form, of equivalent masses, equivalent dampings, equivalent damping ratios, equivalent natural frequencies, and equivalent frequency ratios, for each class of fractional oscillators.
- Proposing the analytic expressions, in the closed form by using elementary functions, of the free, impulse, step, frequency, and sinusoidal responses to three classes of fractional oscillators.
- Revealing the power laws of equivalent mass and equivalent damping for each class of fractional oscillators.
- Representing some of the generalized Mittag-Leffler functions by using elementary functions.
2. Preliminaries
2.1. Brief of Linear Oscillations of Order 2
2.1.1. Simple Oscillation Model
2.1.2. Responses
2.1.3. Spectra of Three Excitations
2.1.4. Generalization of Linear Oscillators
2.2. Three Classes of Fractional Oscillators
2.3. Equivalence of Functions in the Sense of Fourier Transform
3. Problem Statement and Research Thoughts
3.1. Problem Statement
3.2. Research Thoughts
4. Equivalent Systems of Three Classes of Fractional Oscillators
4.1. Equivalent System for Fractional Oscillators in Class I
4.1.1. Equivalent Oscillation Equation of Fractional Oscillators in Class I
4.1.2. Equivalent Mass of Fractional Oscillators in Class I
4.1.3. Equivalent Damping of Fractional Oscillators of Class I
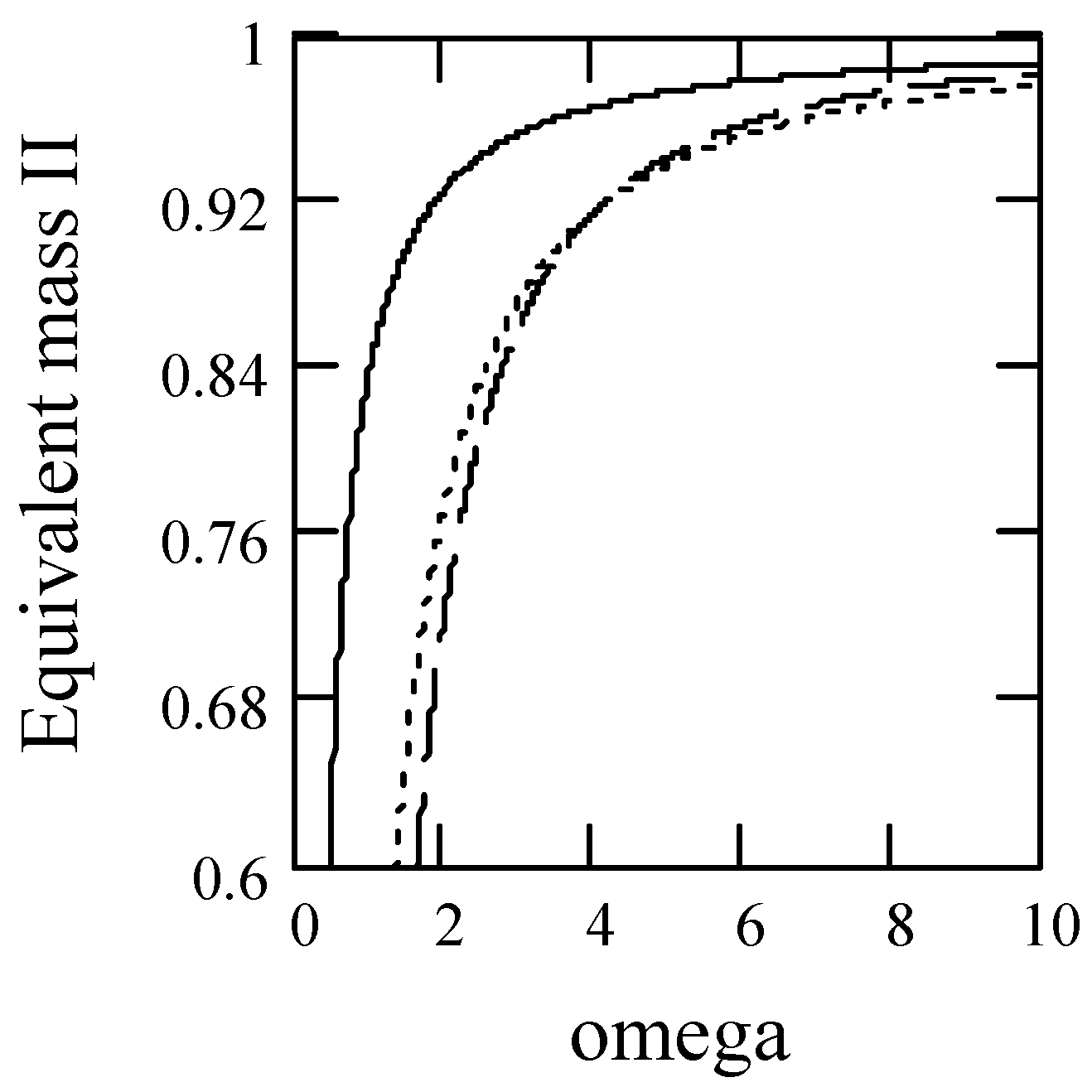
4.2. Equivalent Oscillation System for Fractional Oscillators of Class II Type
4.2.1. Equivalent Oscillation Equation of Fractional Oscillators in Class II
4.2.2. Equivalent Mass of Fractional Oscillators of Class II
4.2.3. Equivalent Damping of Fractional Oscillators in Class II
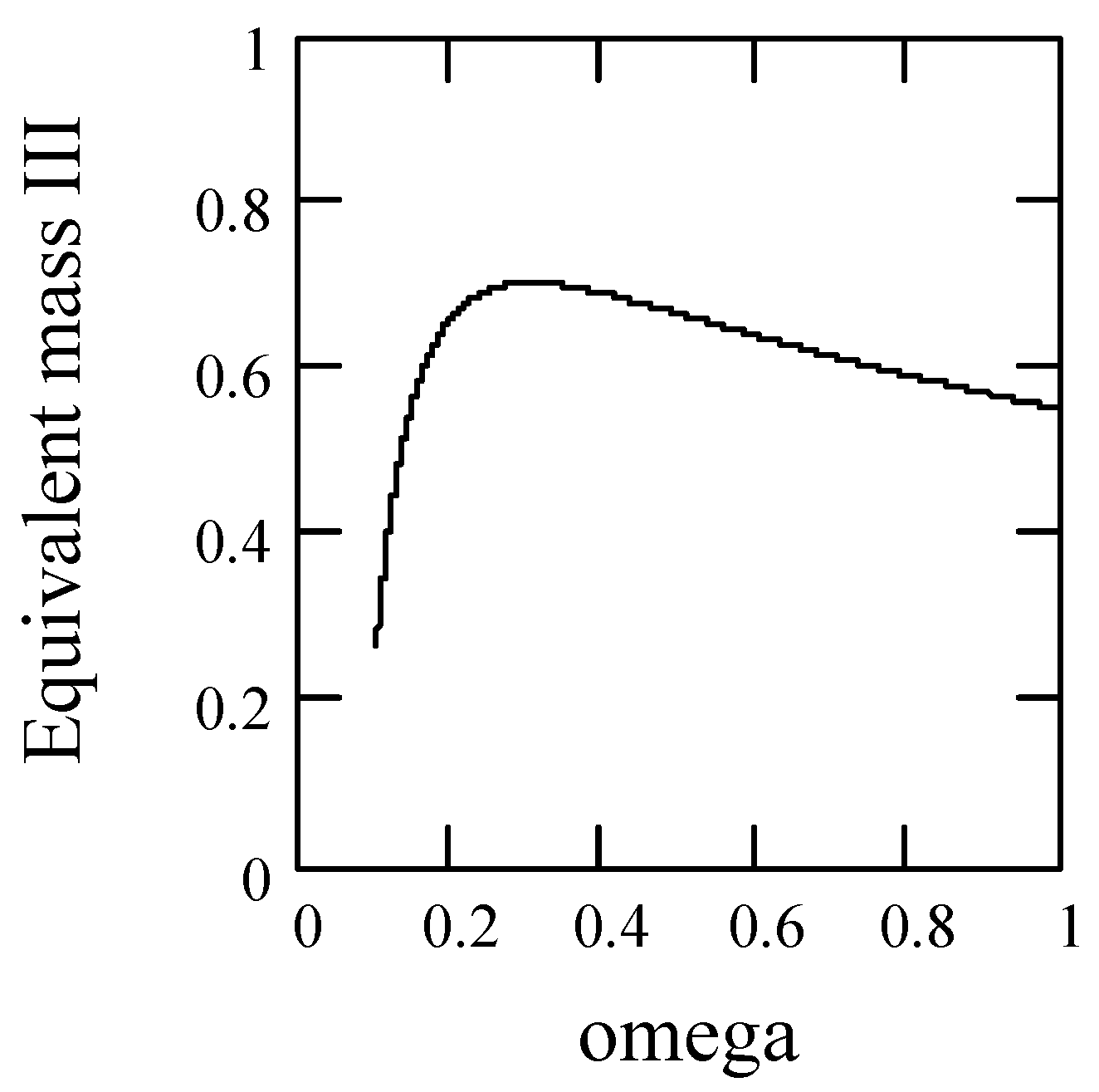
4.3. Equivalent Oscillation System for Fractional Oscillators of Class III
4.3.1. Equivalent Oscillation Equation of Fractional Oscillators in Class III
4.3.2. Equivalent Mass of Fractional Oscillators in Class III
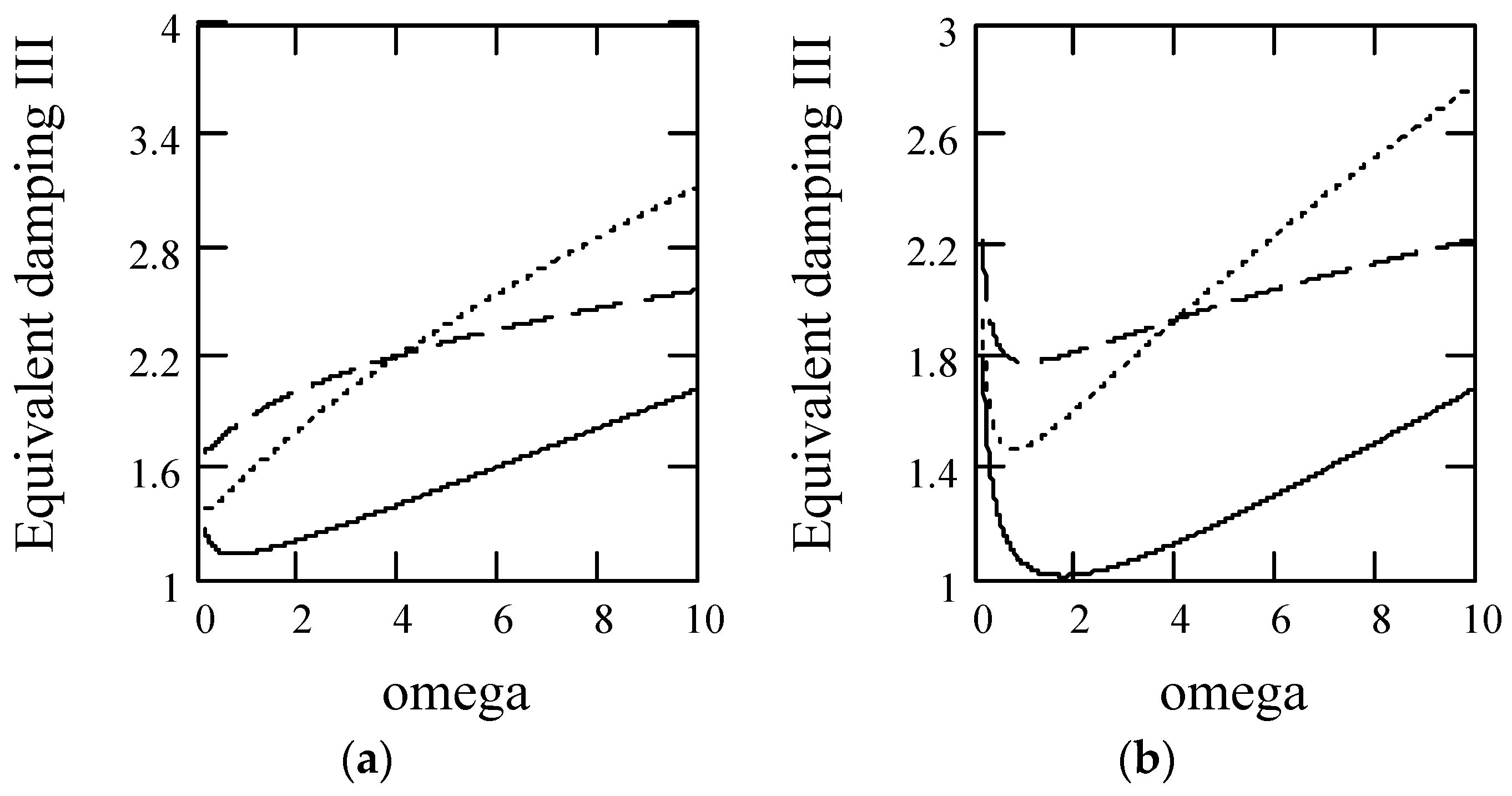
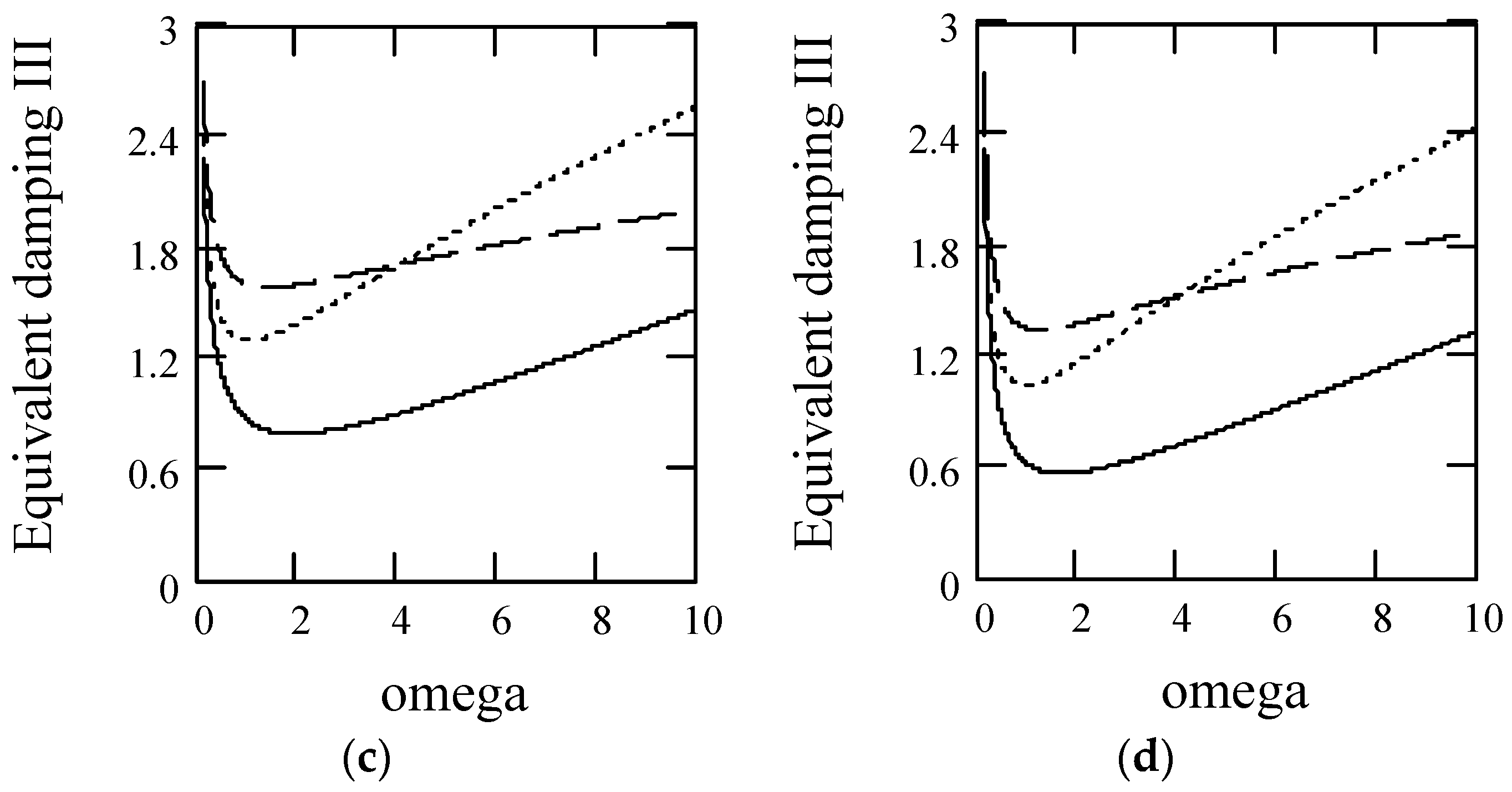
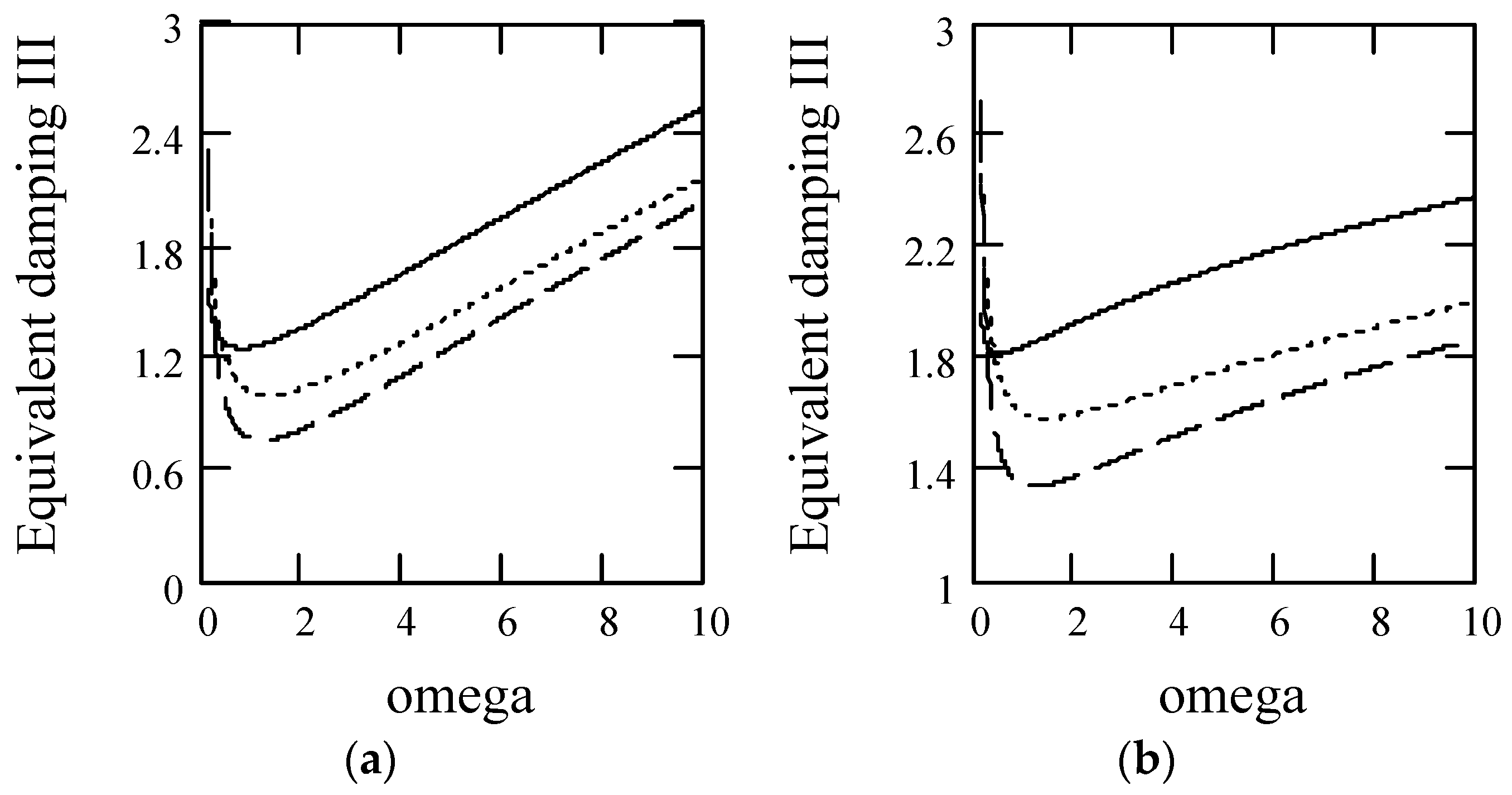
4.3.3. Equivalent Damping of Fractional Oscillators in Class III
4.4. Summary
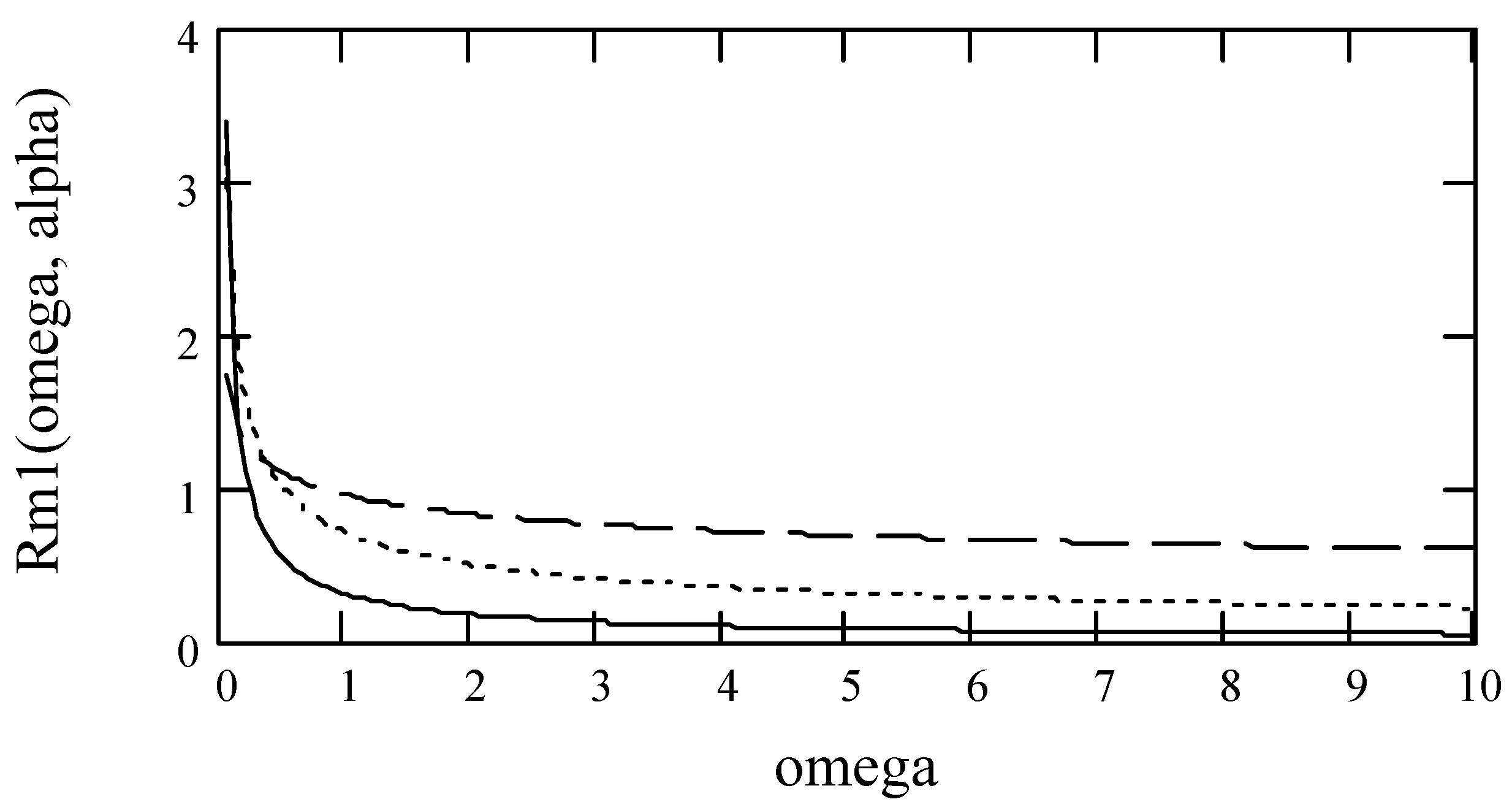
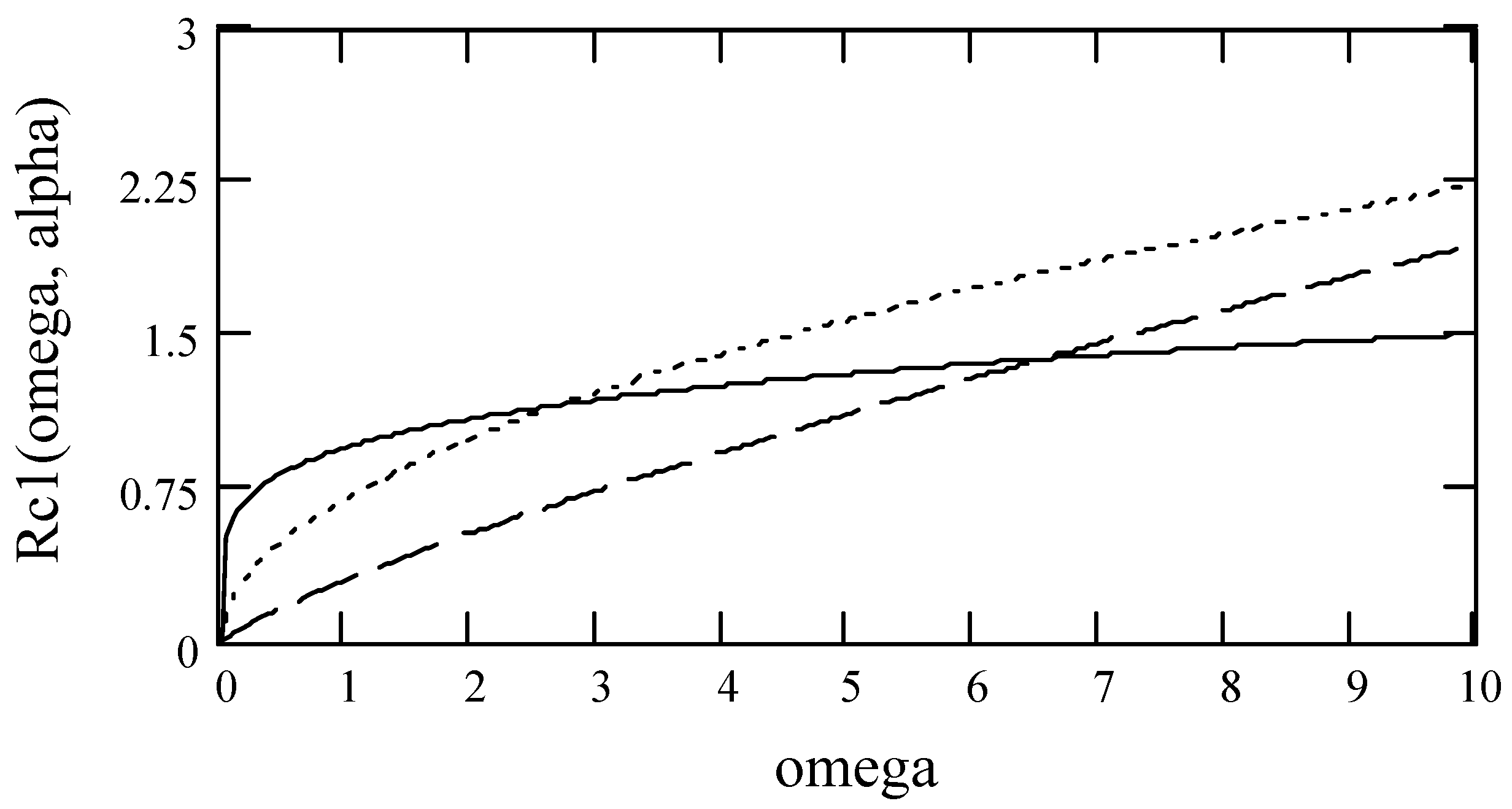
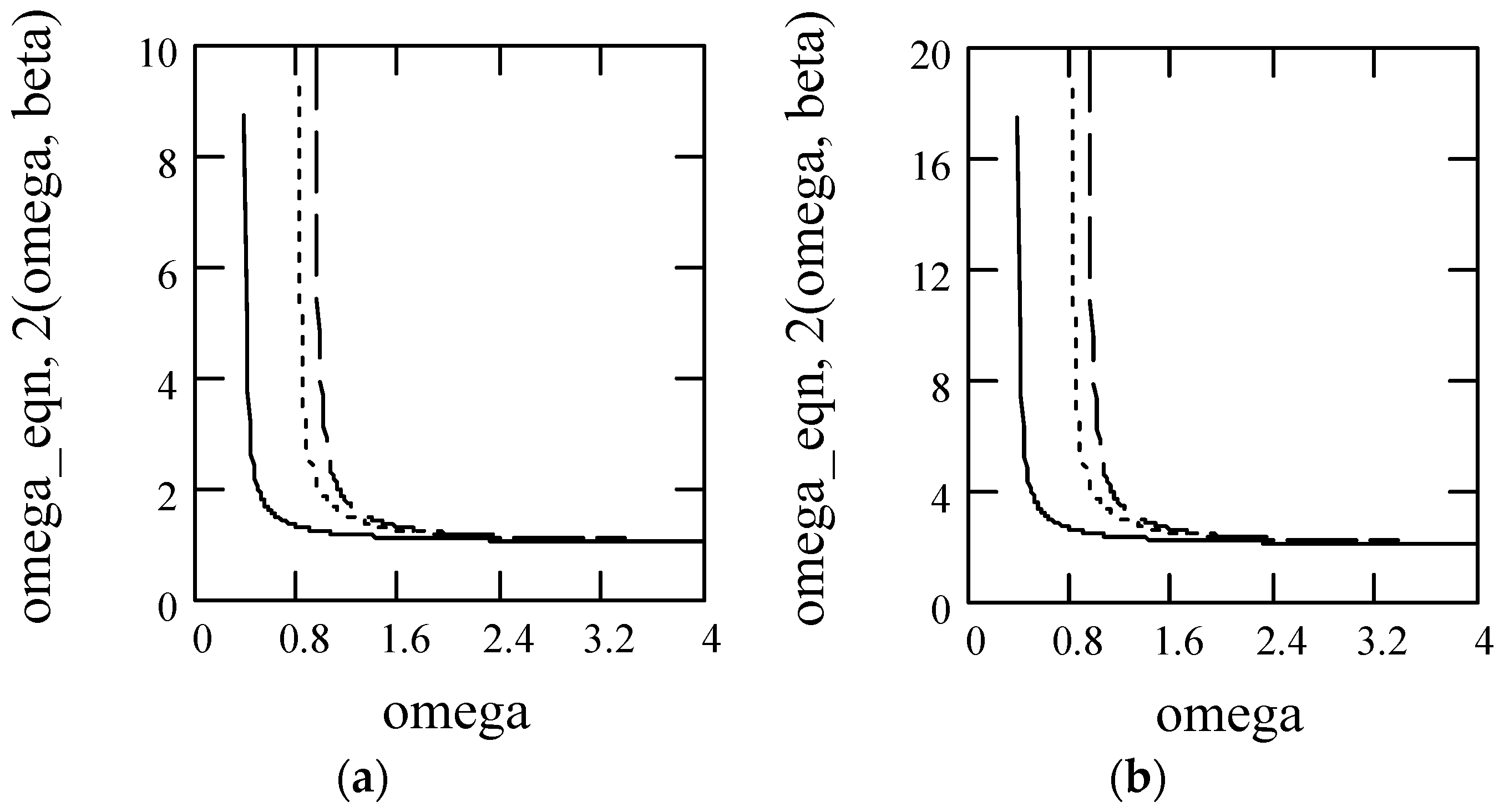
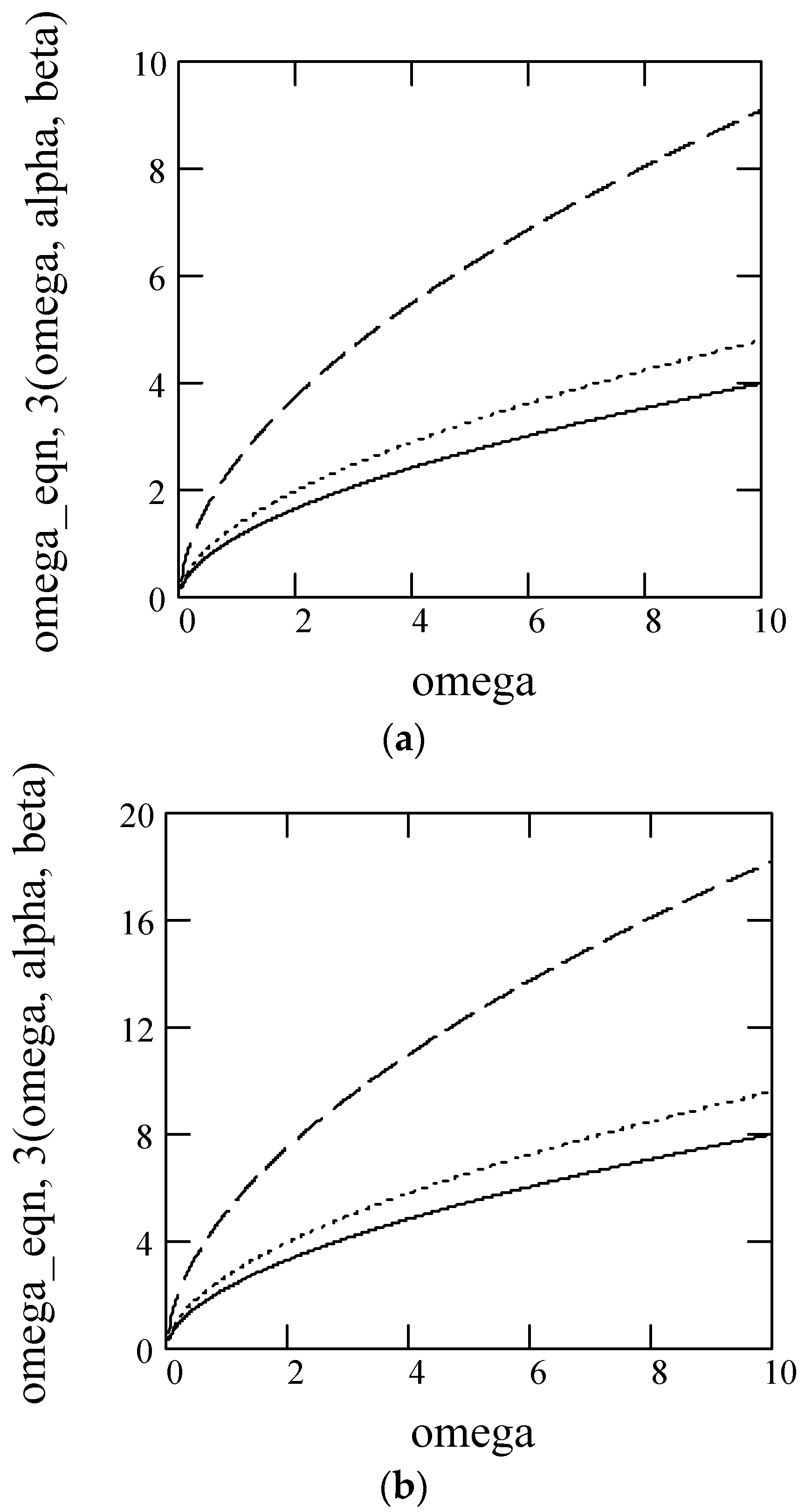
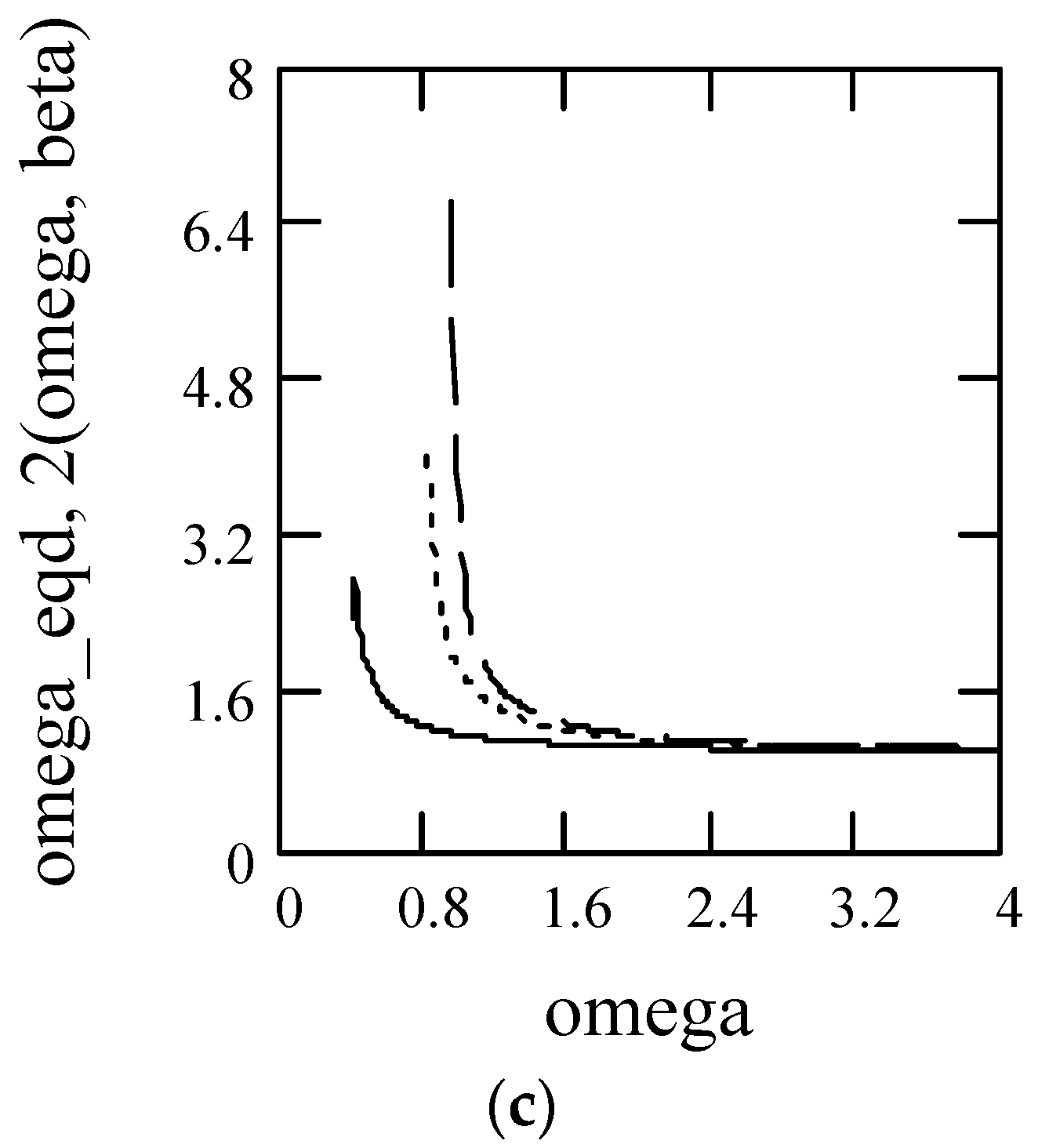
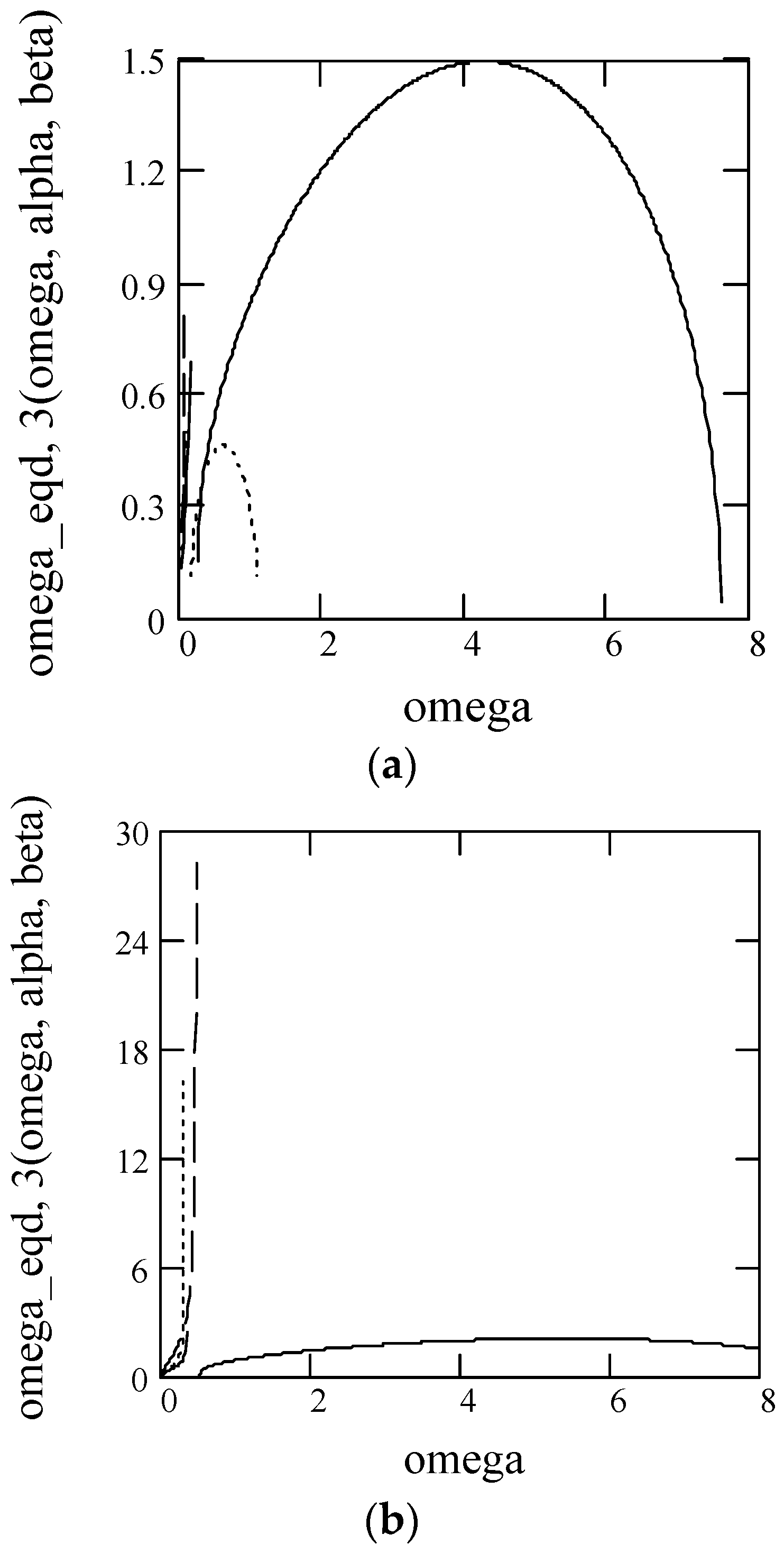
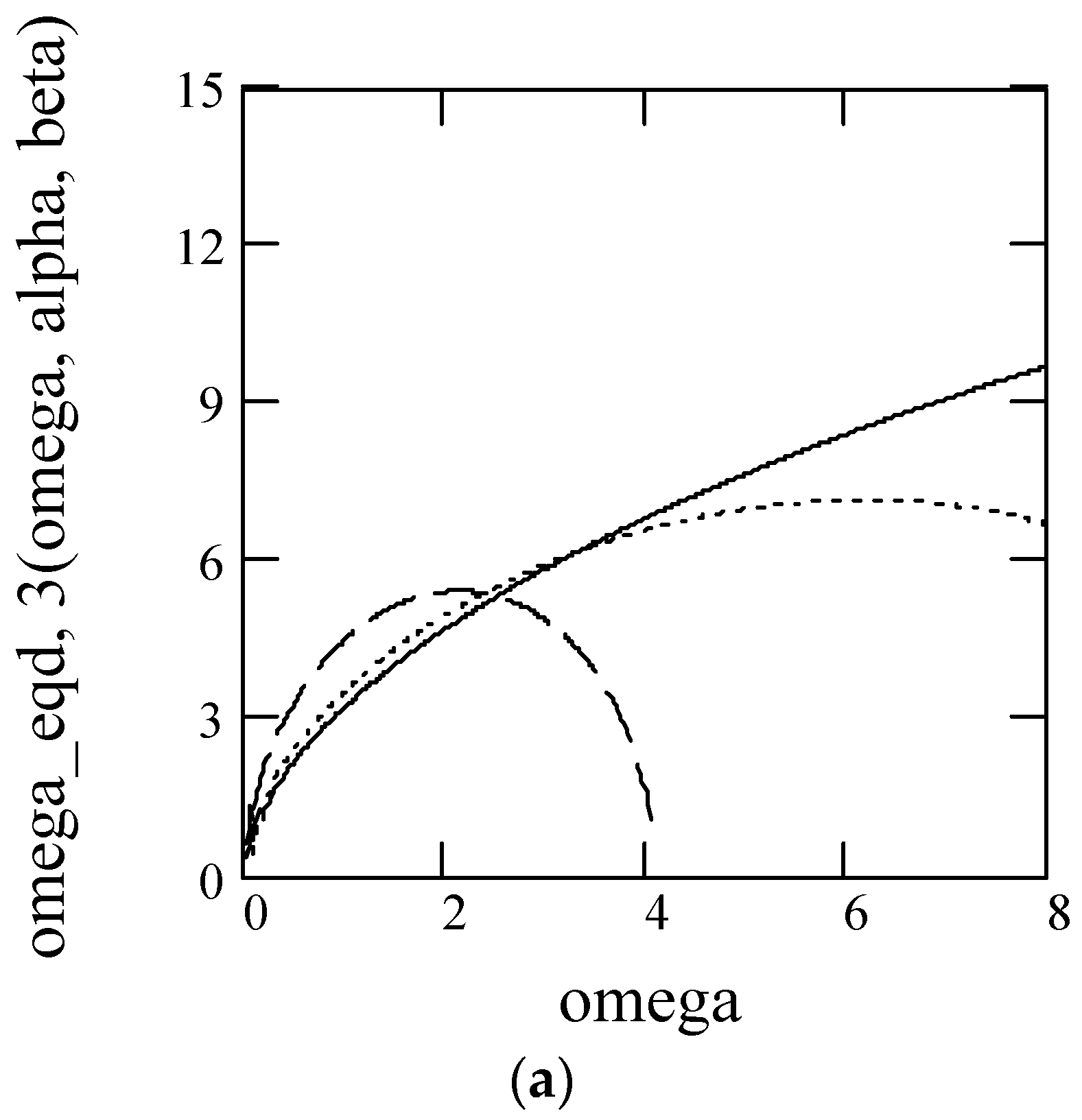
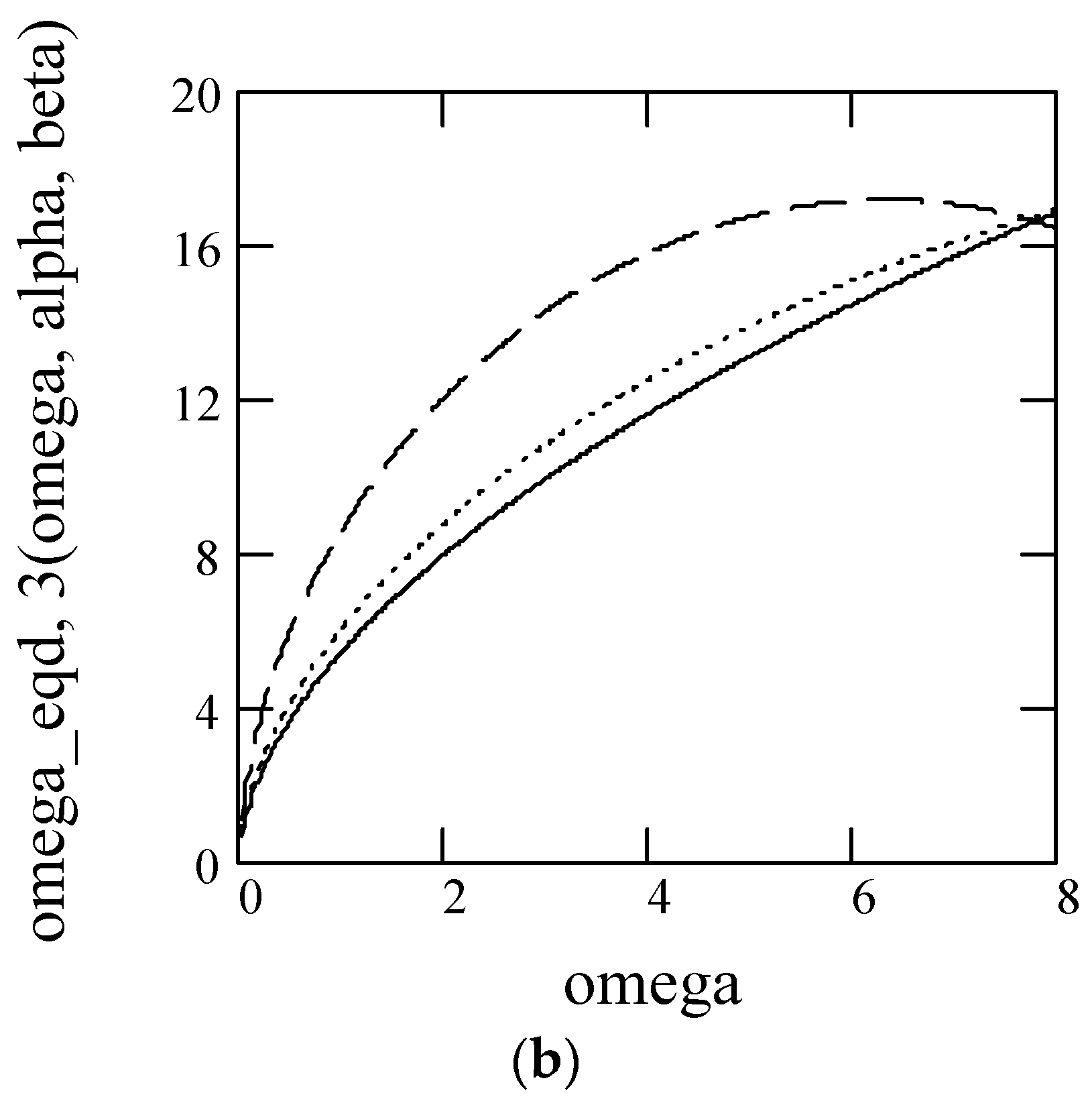
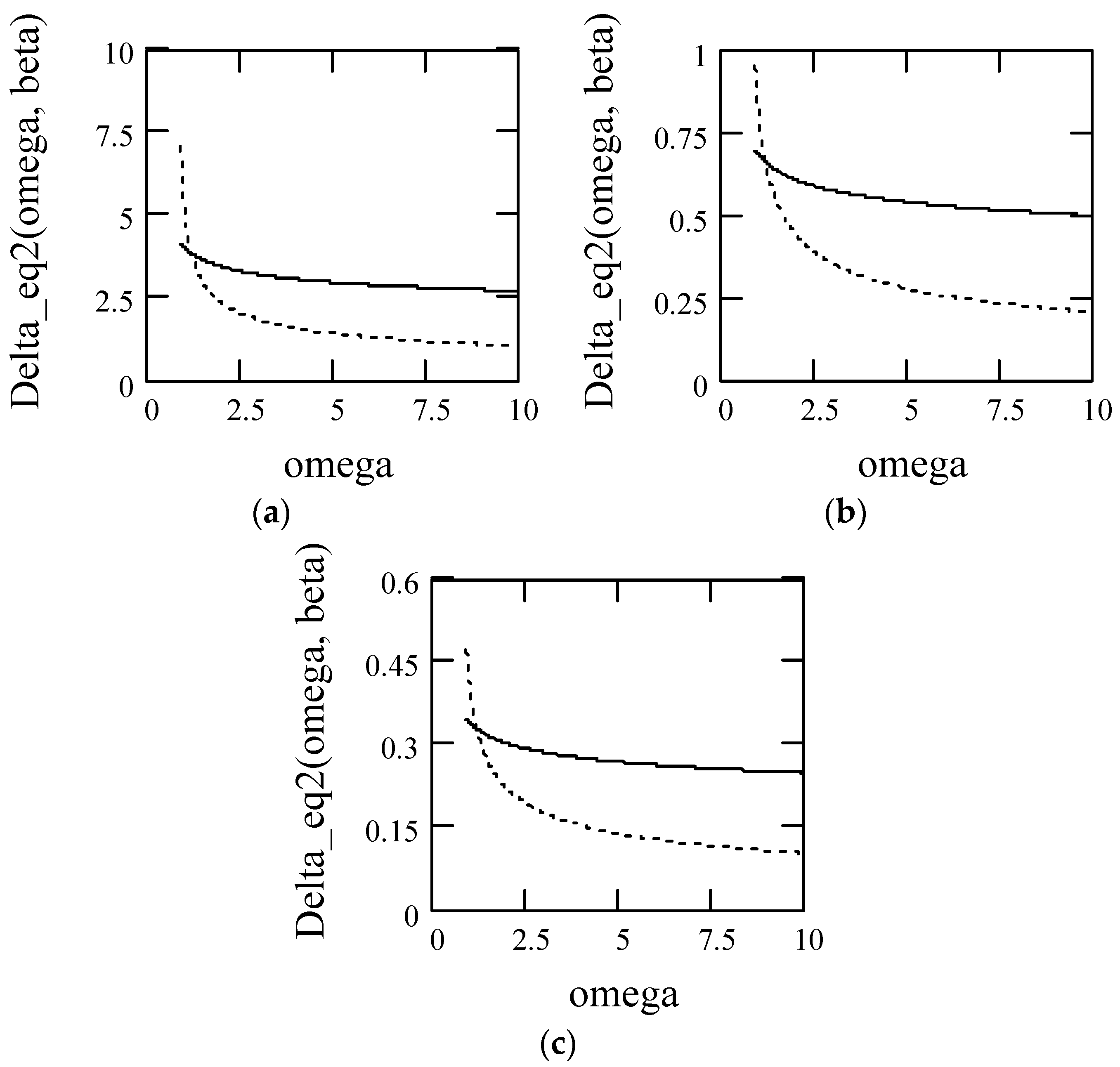
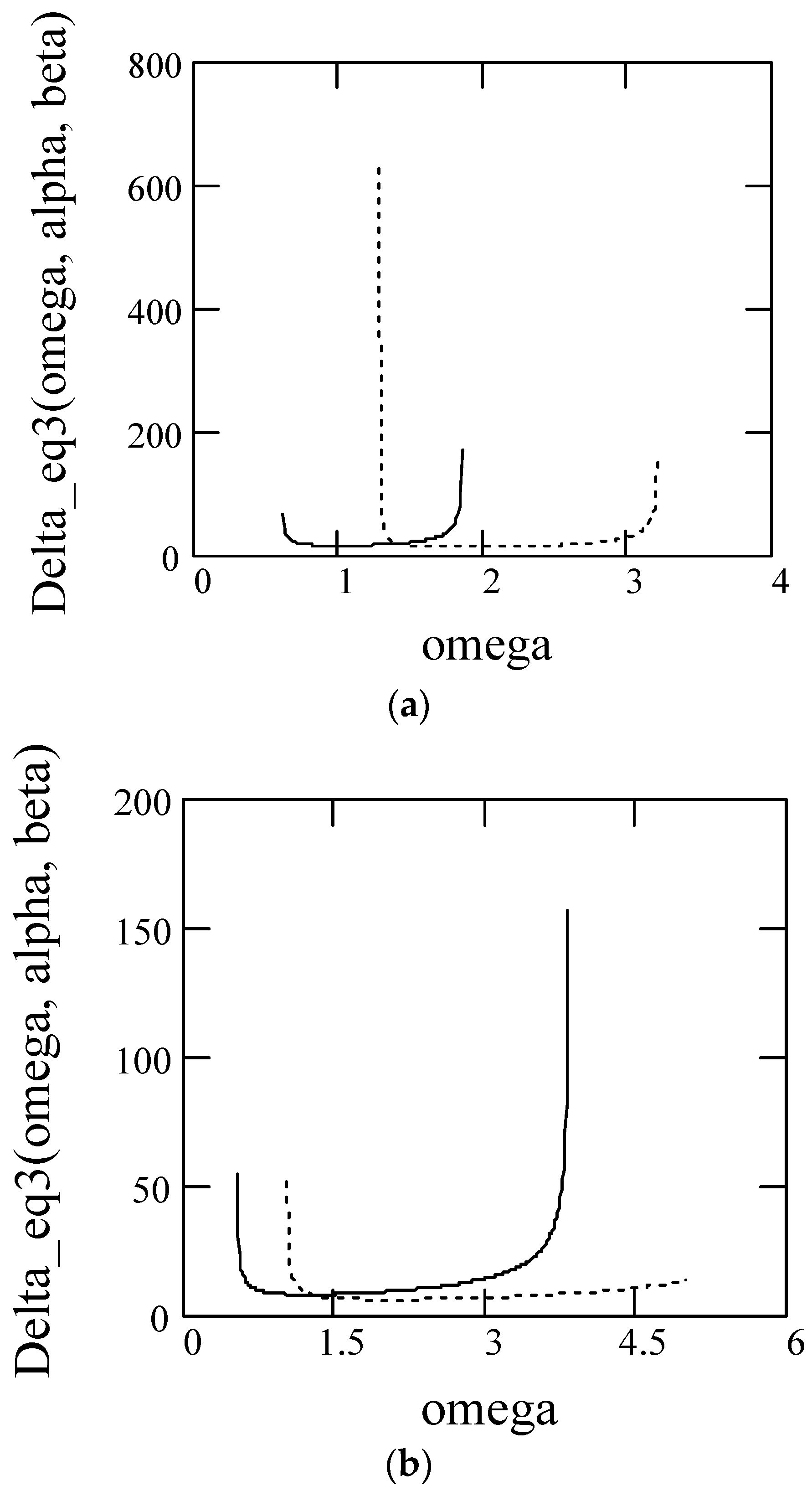
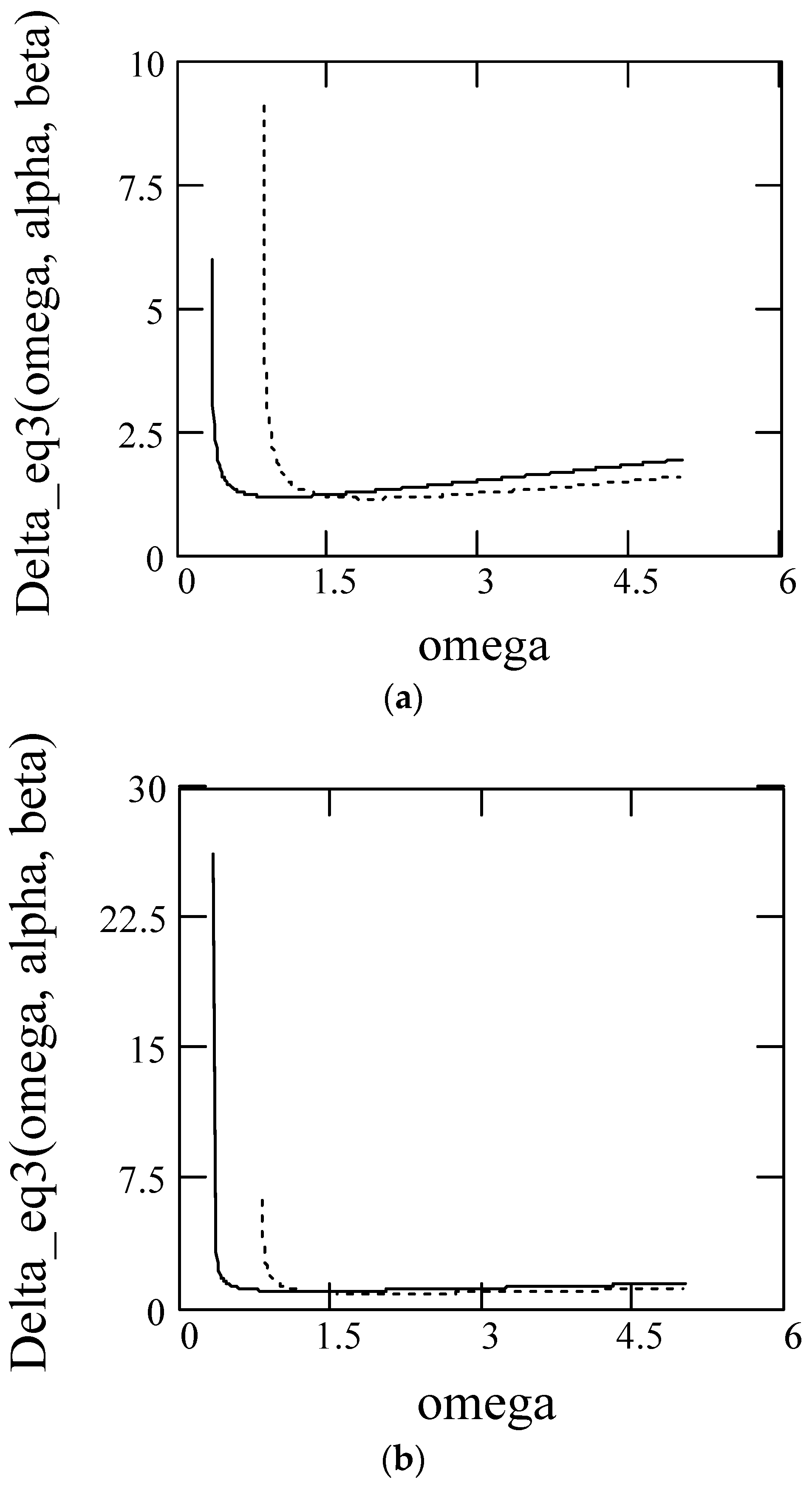
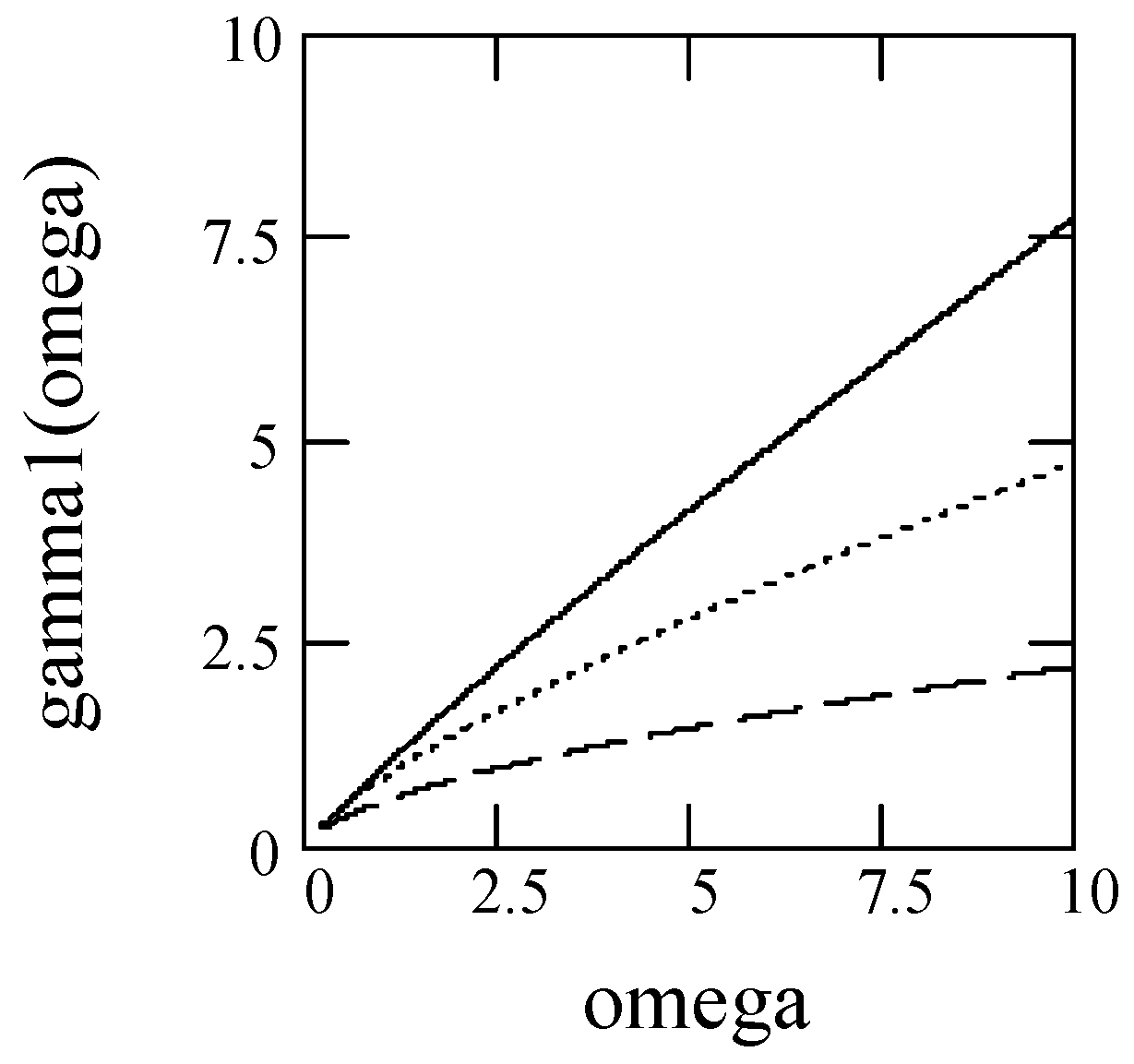
5. Equivalent Natural Frequencies and Damping Ratio of Three Classes of Fractional Oscillators
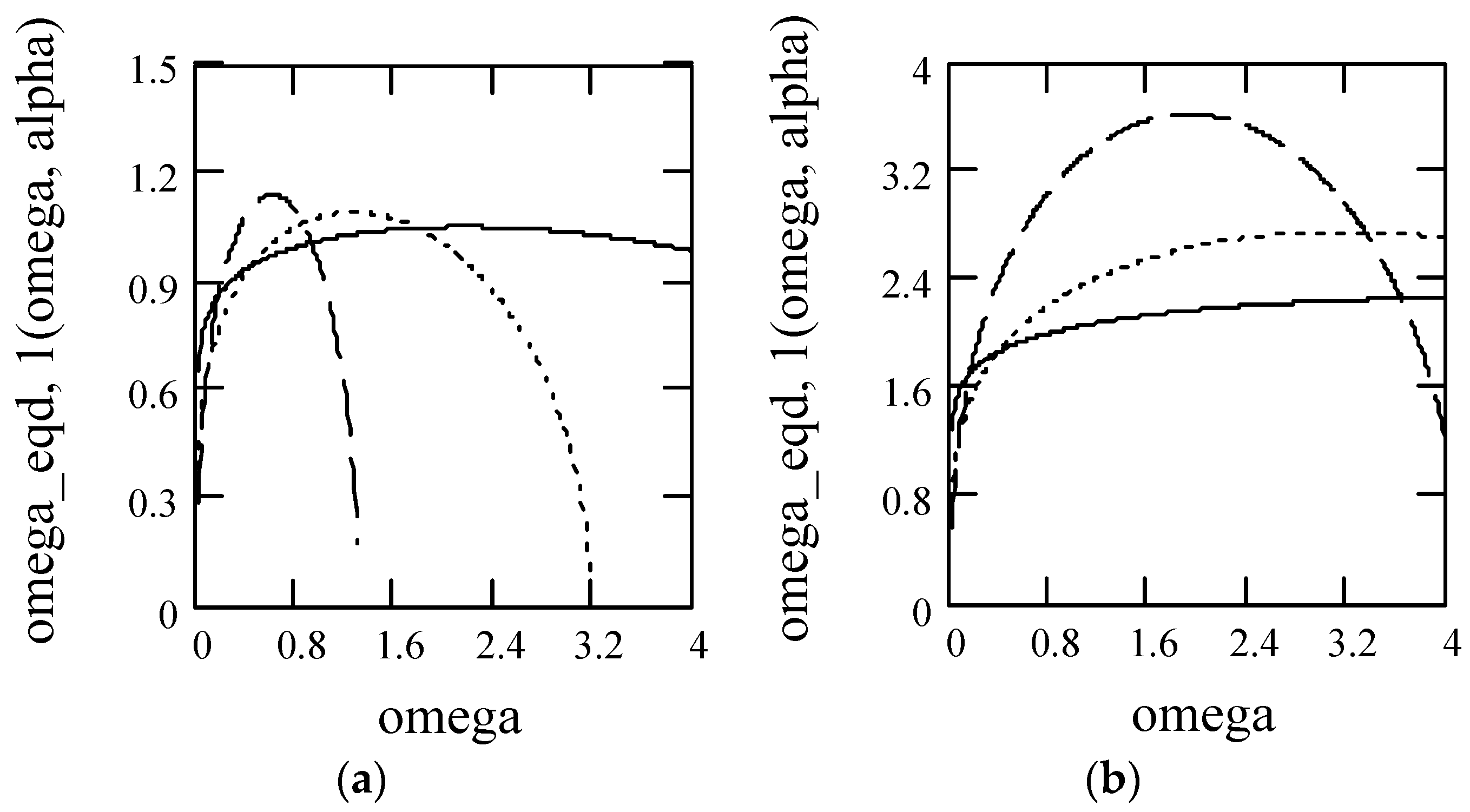
5.1. Equivalent Natural Frequency I
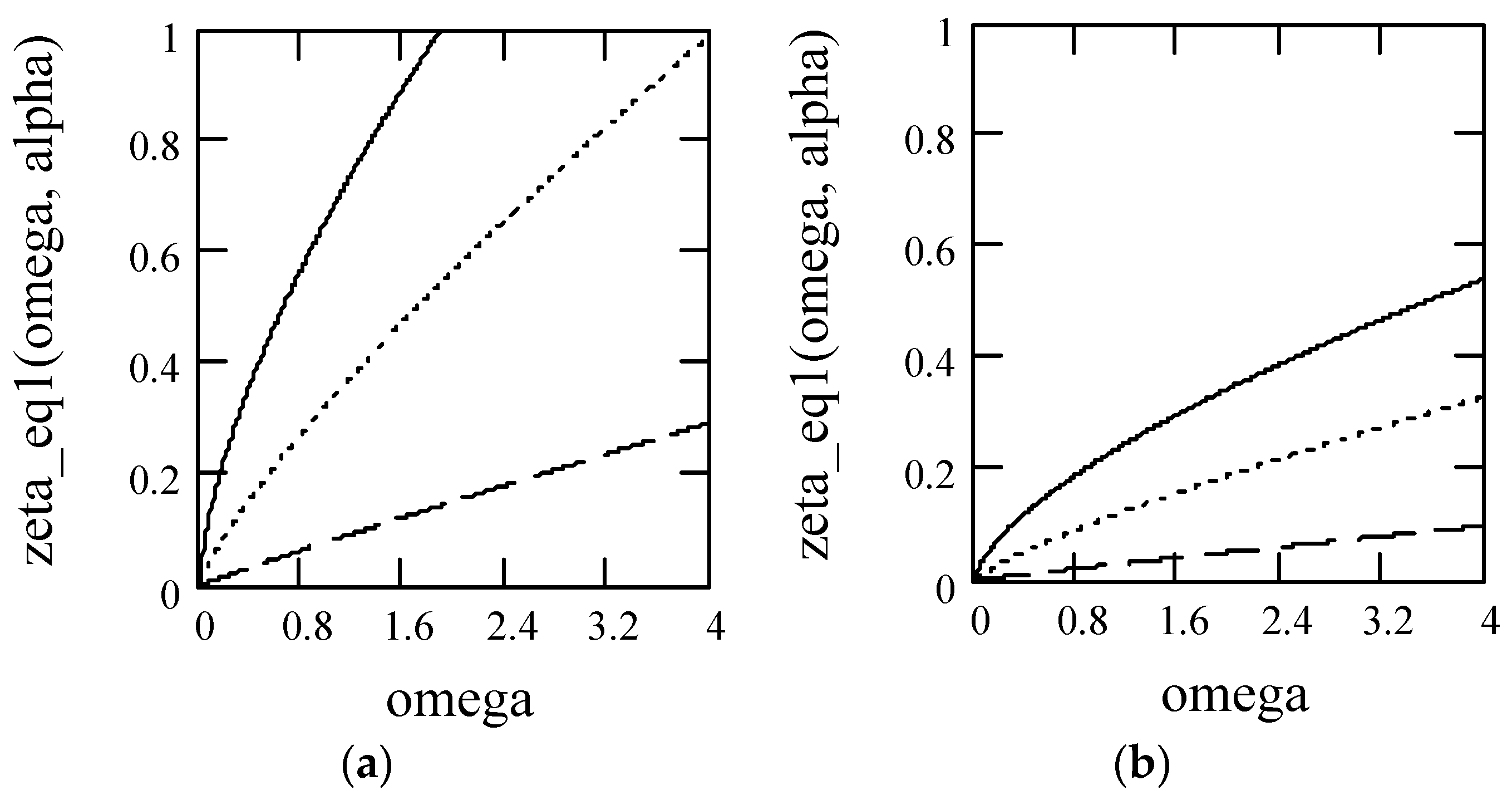
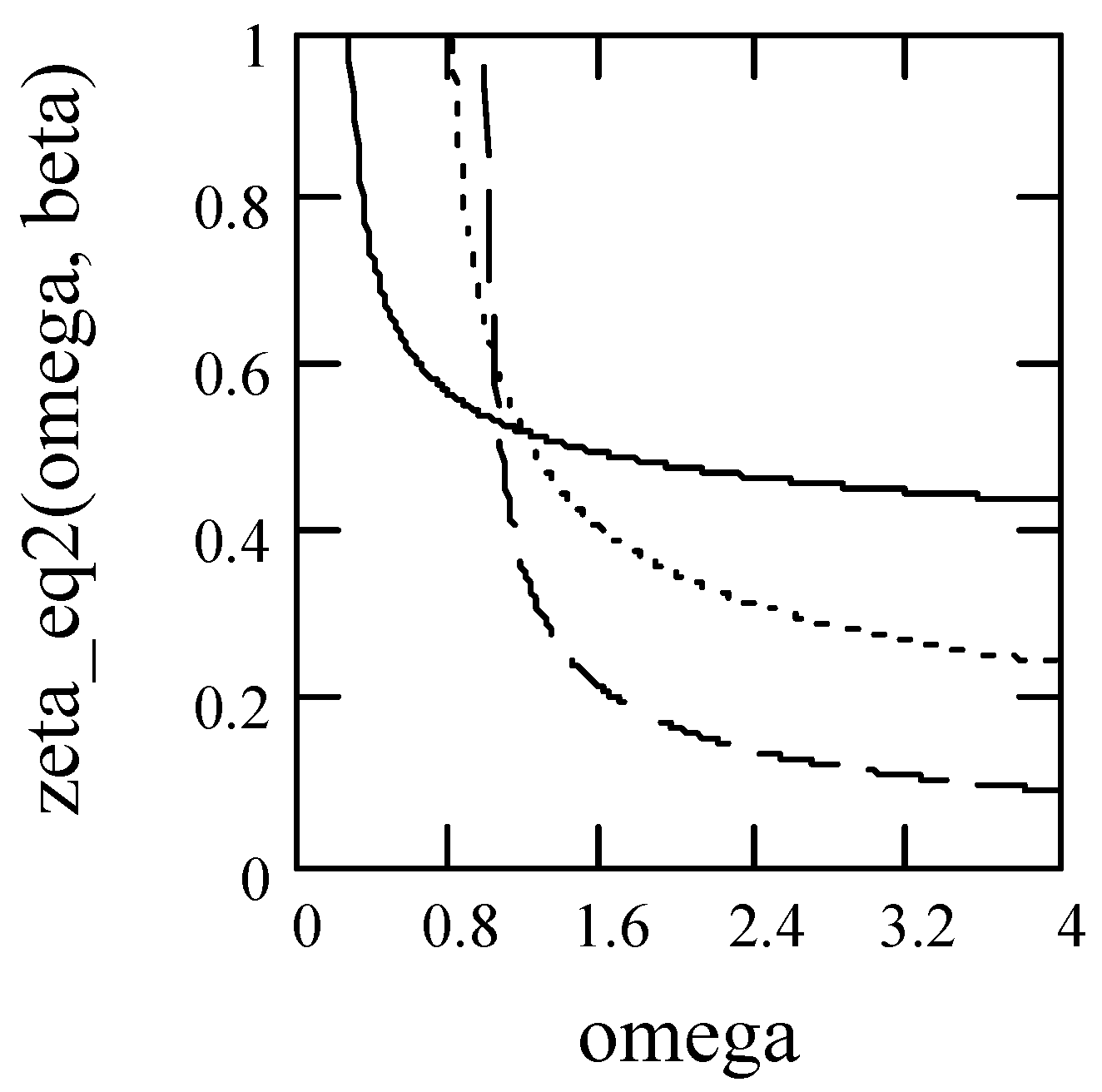
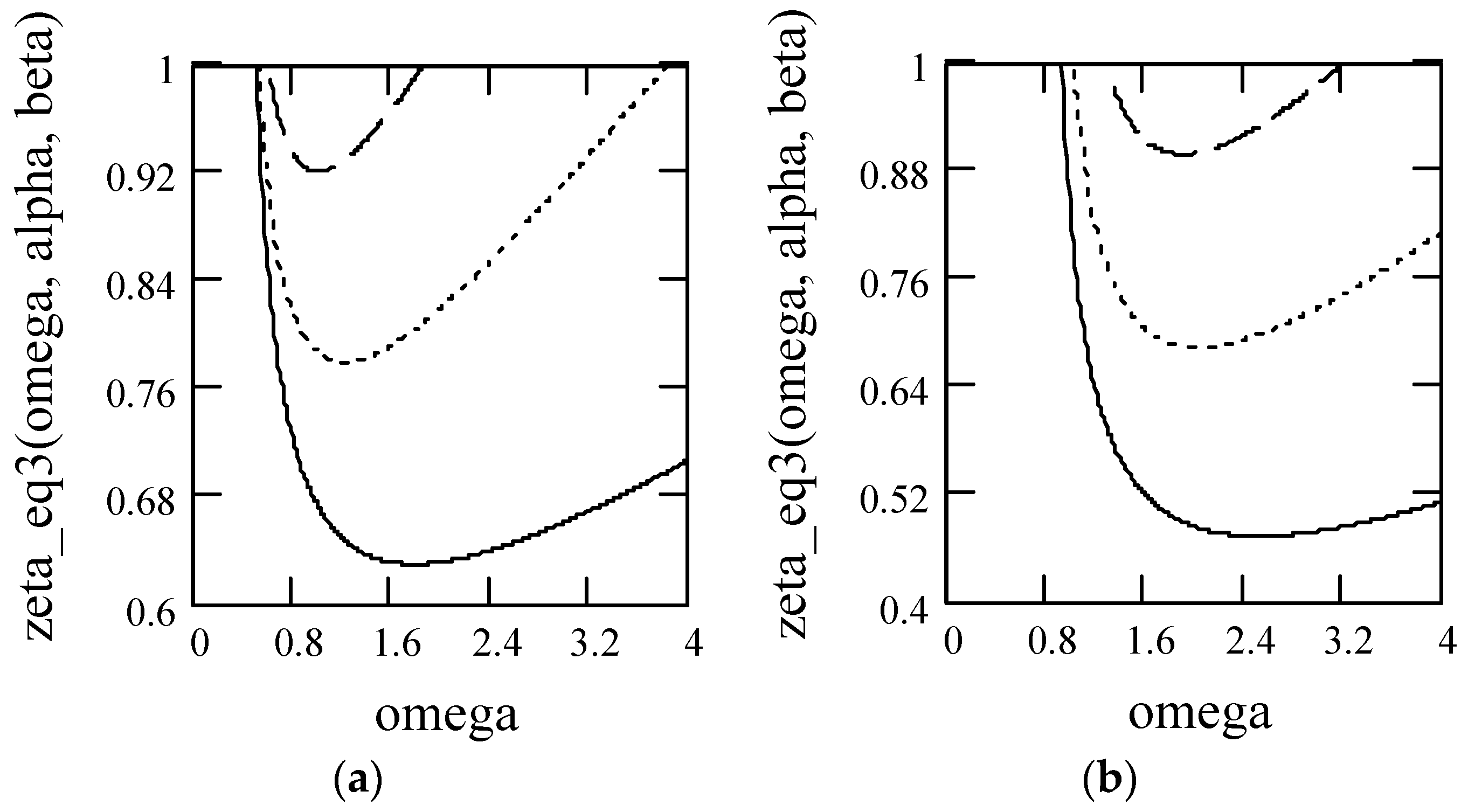
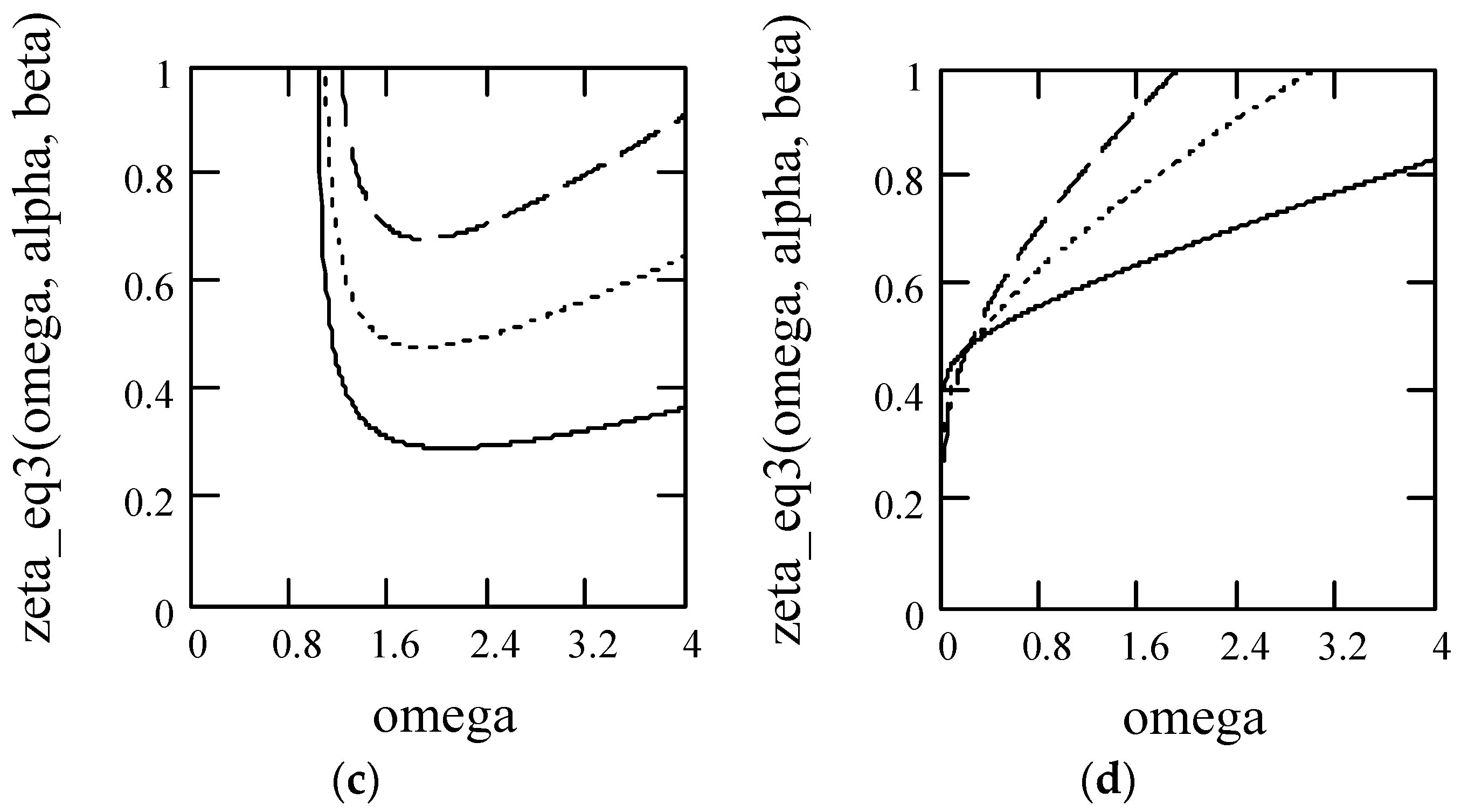
5.2. Equivalent Damping Ratio
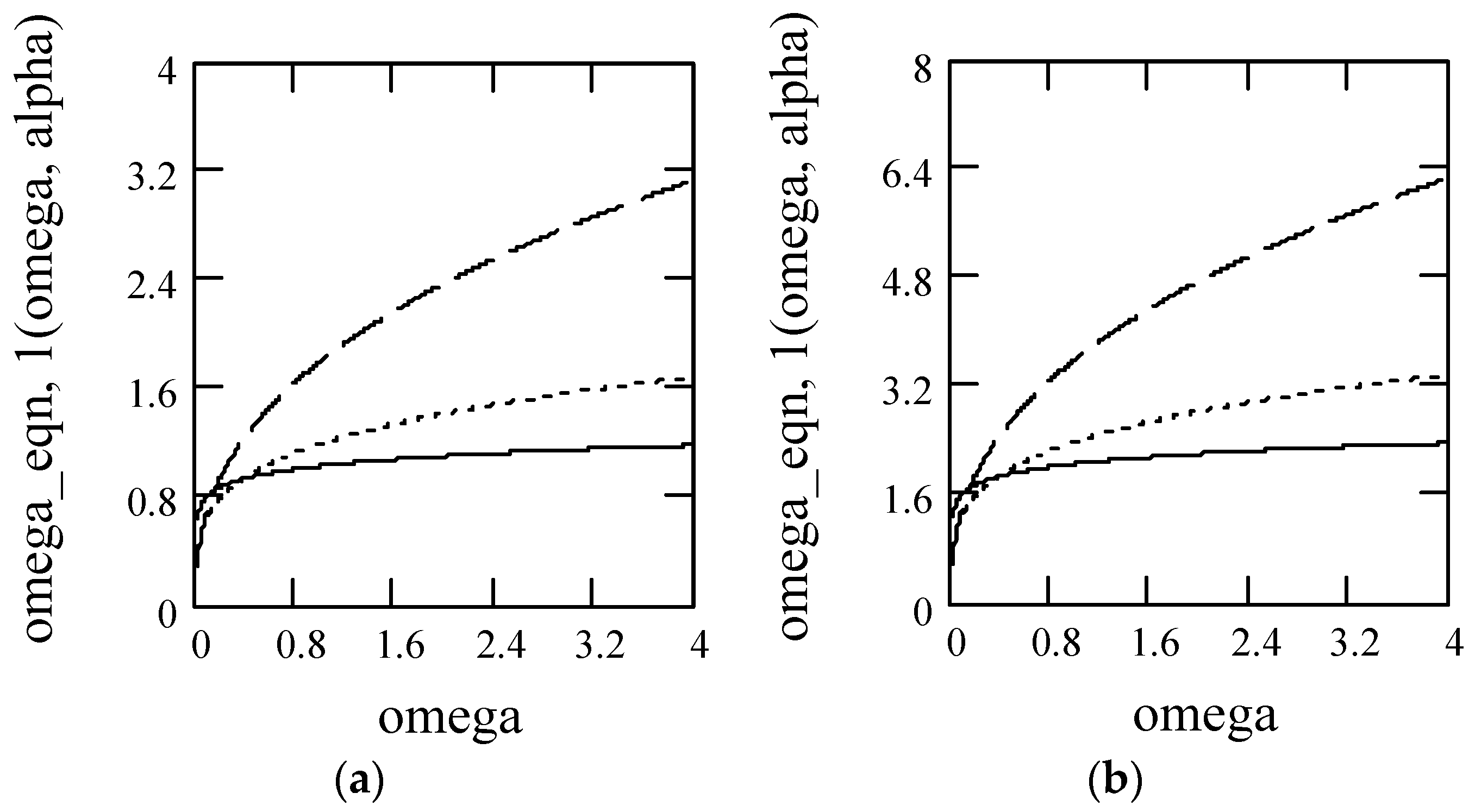
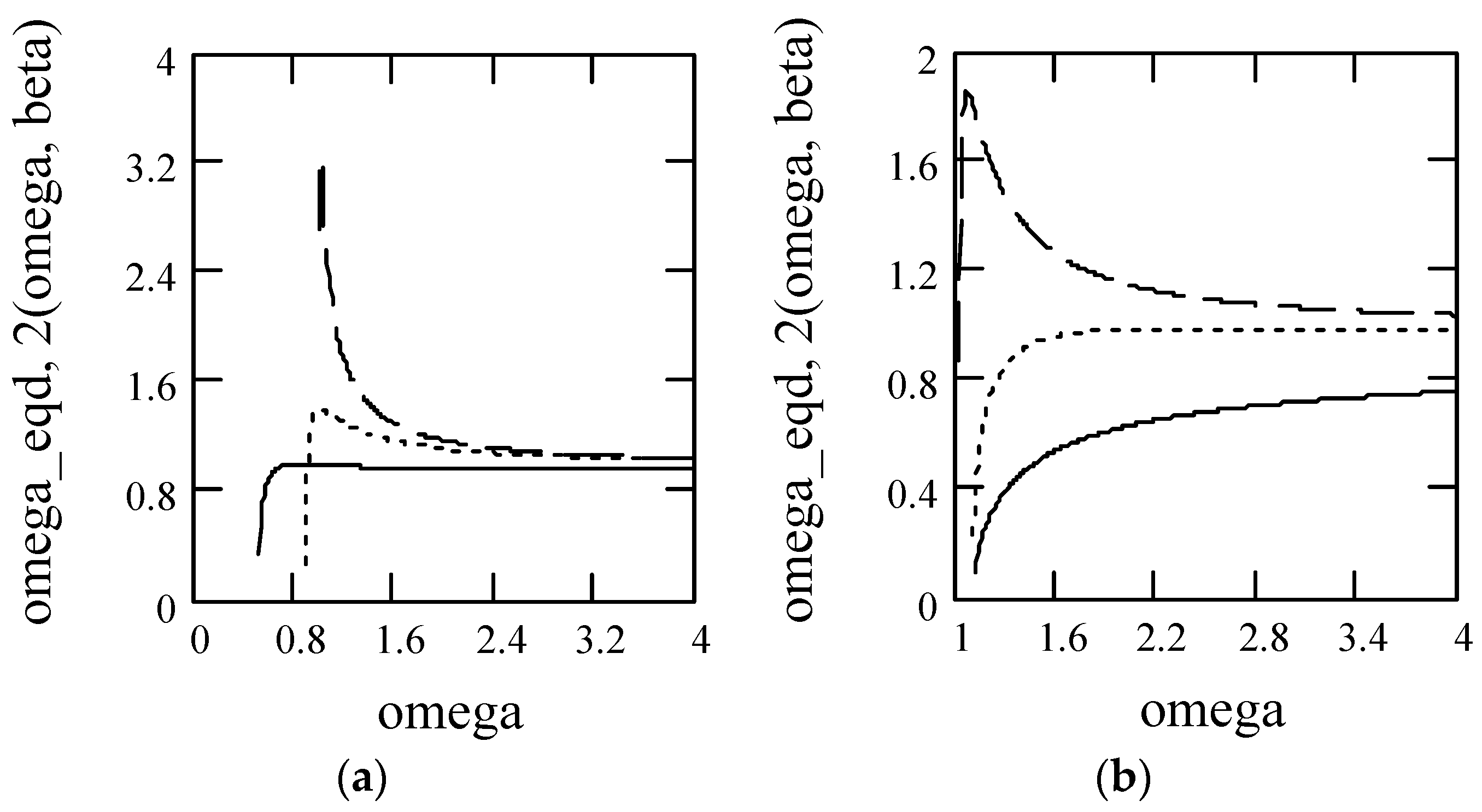
5.3. Equivalent Natural Frequency II
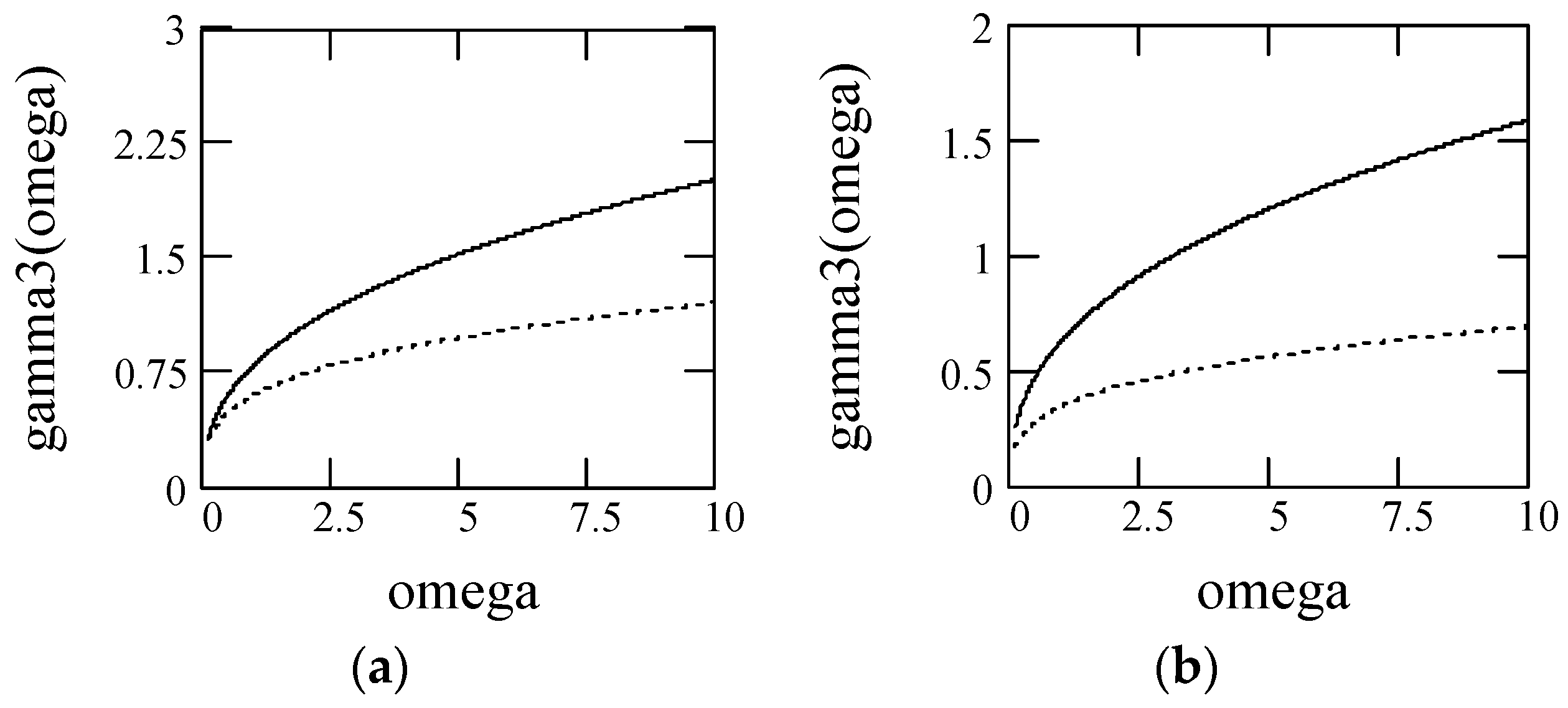
5.4. There Exists Infinity of Natural Frequencies of a Fractional Oscillator
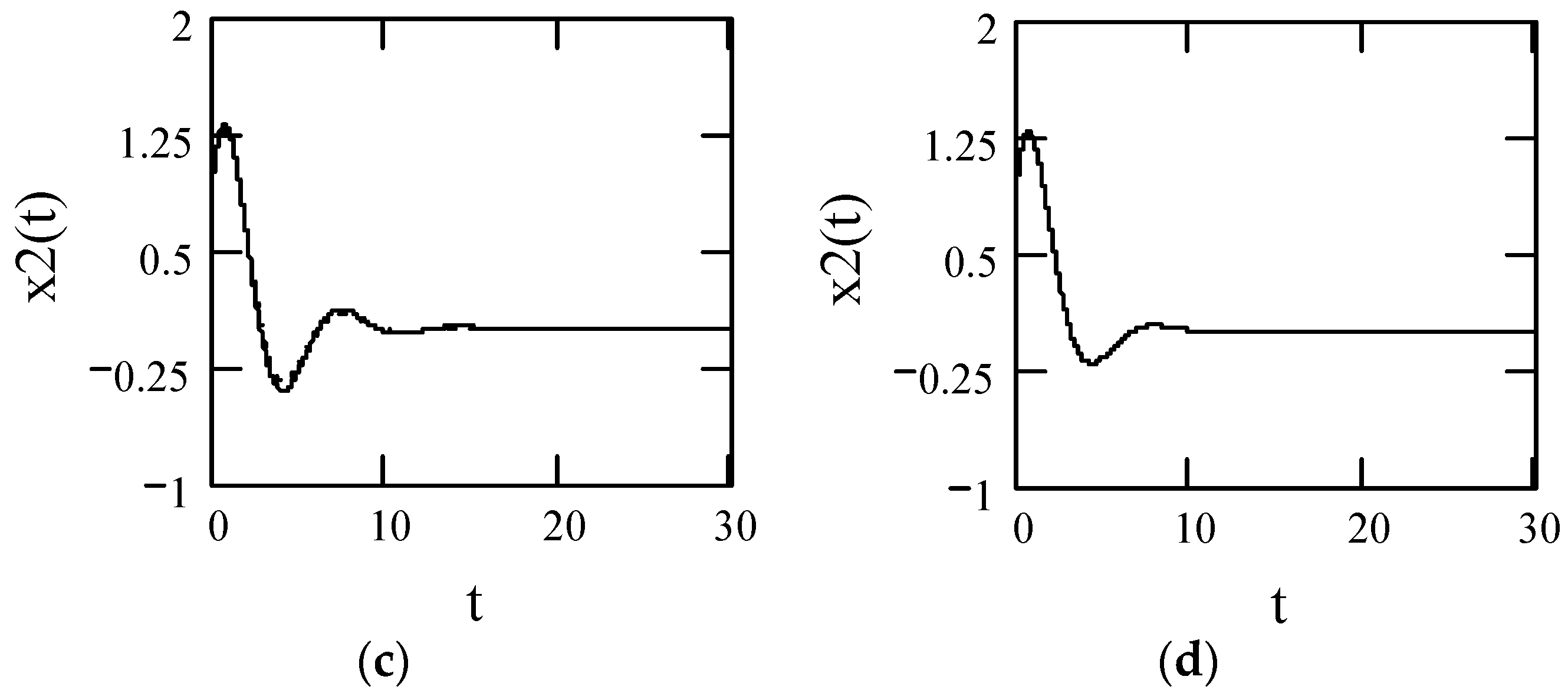
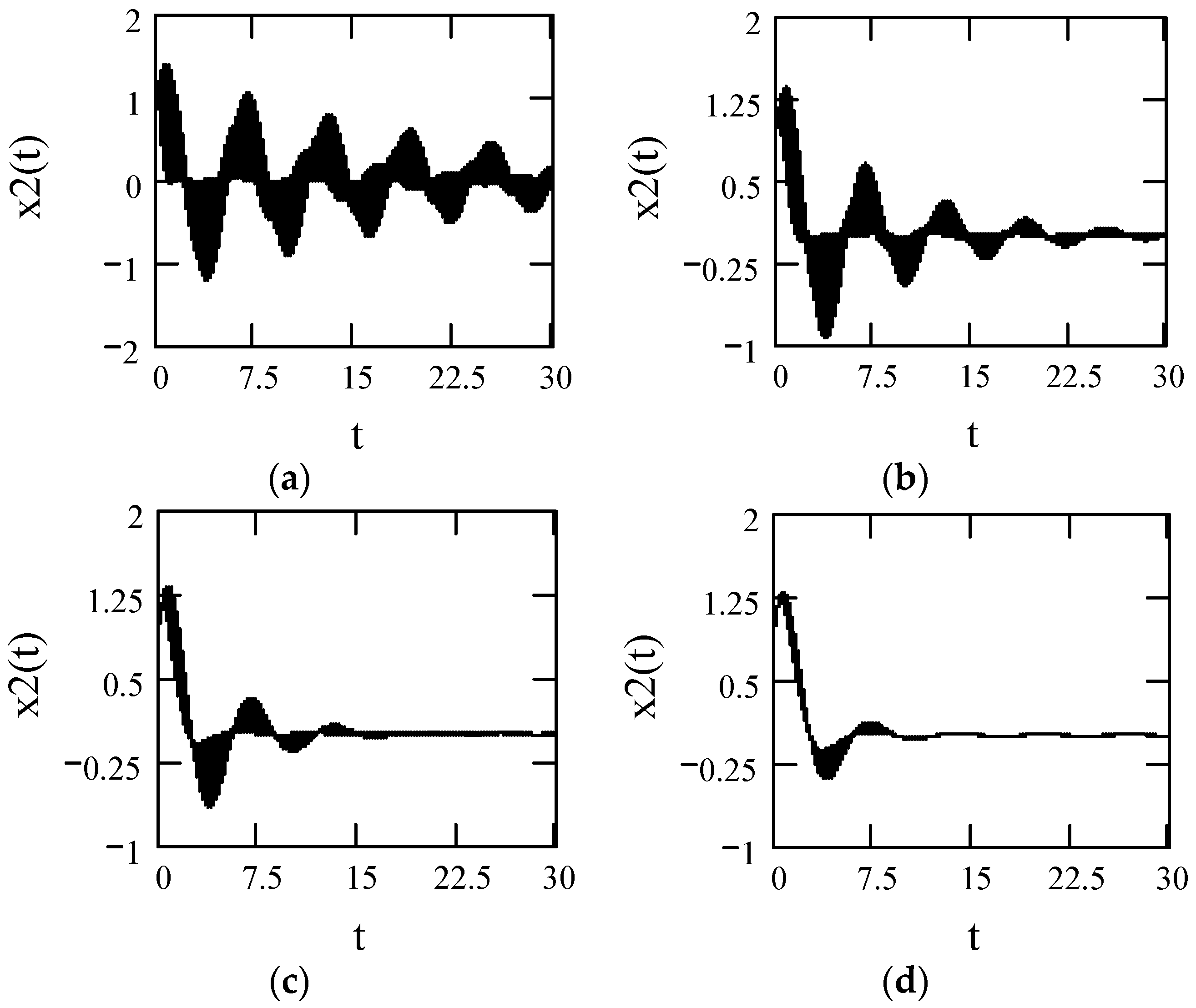
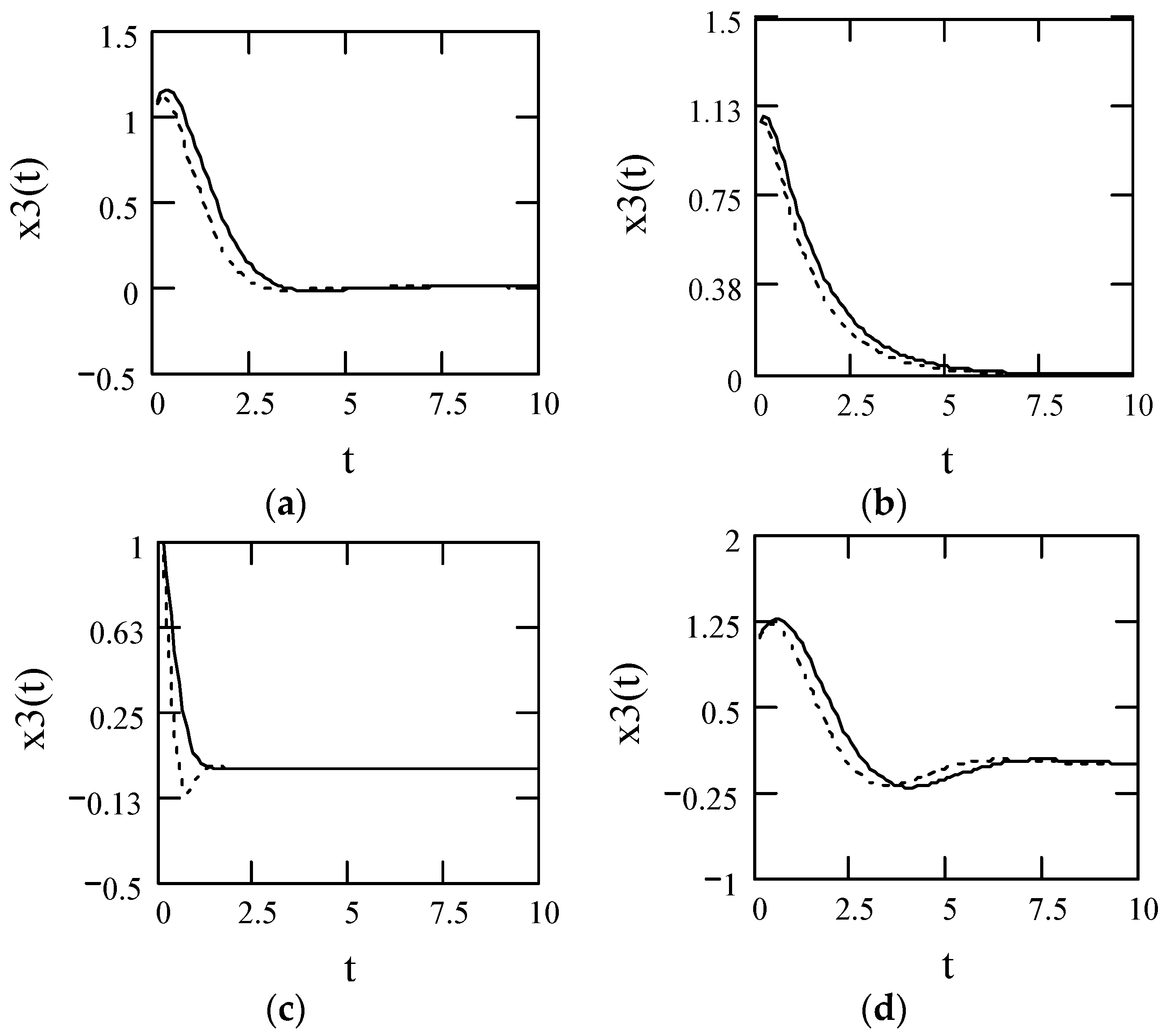
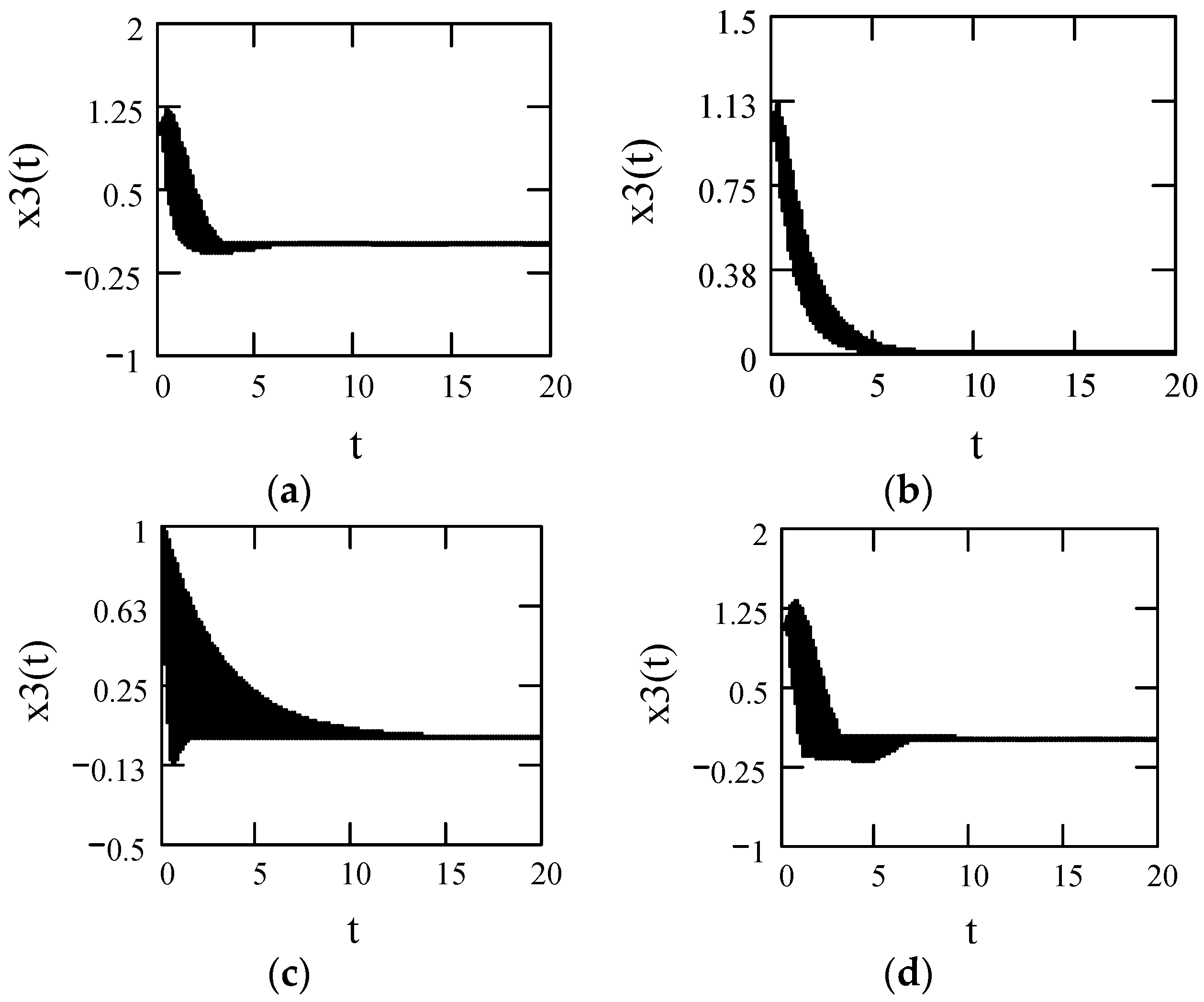
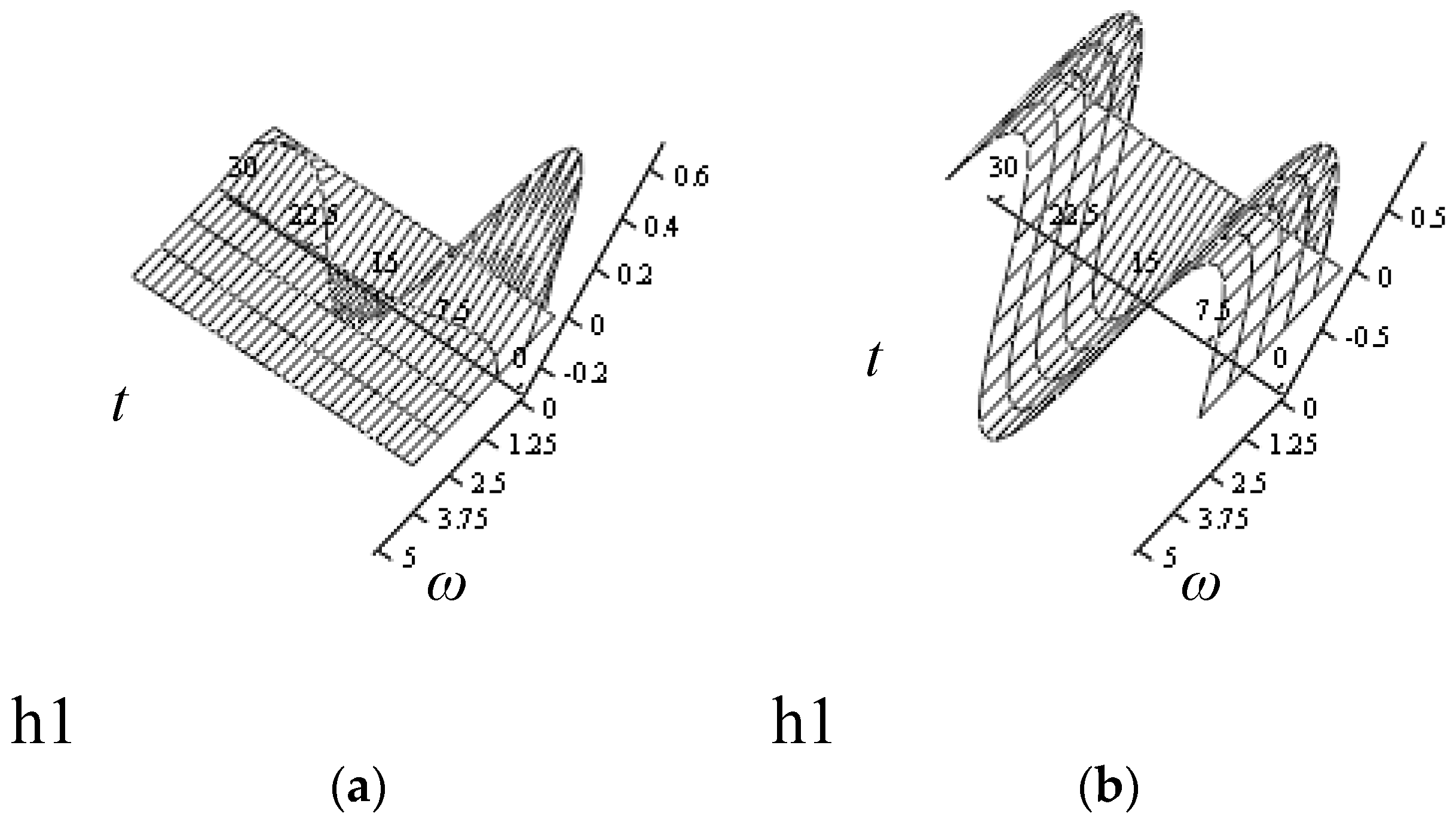
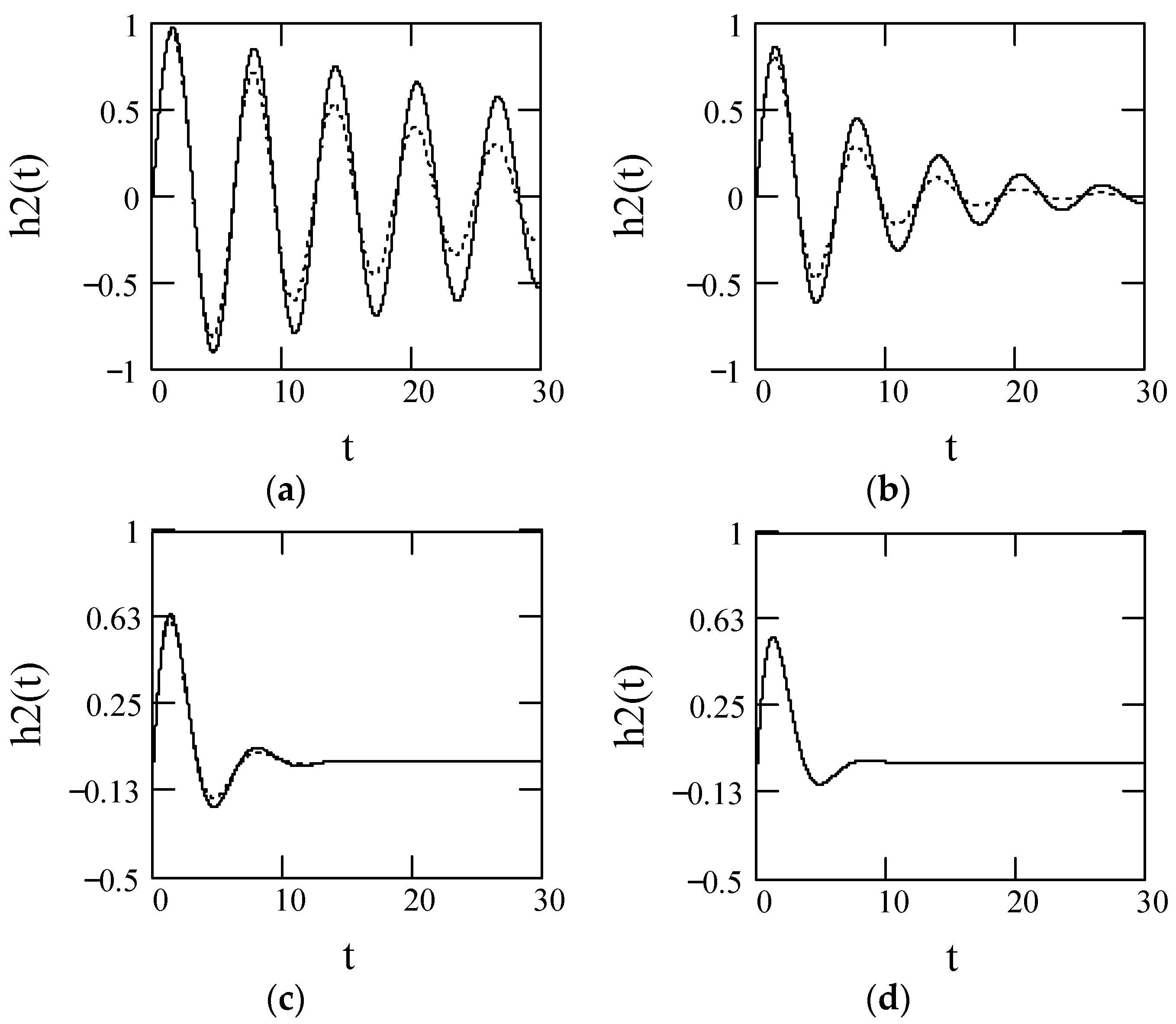
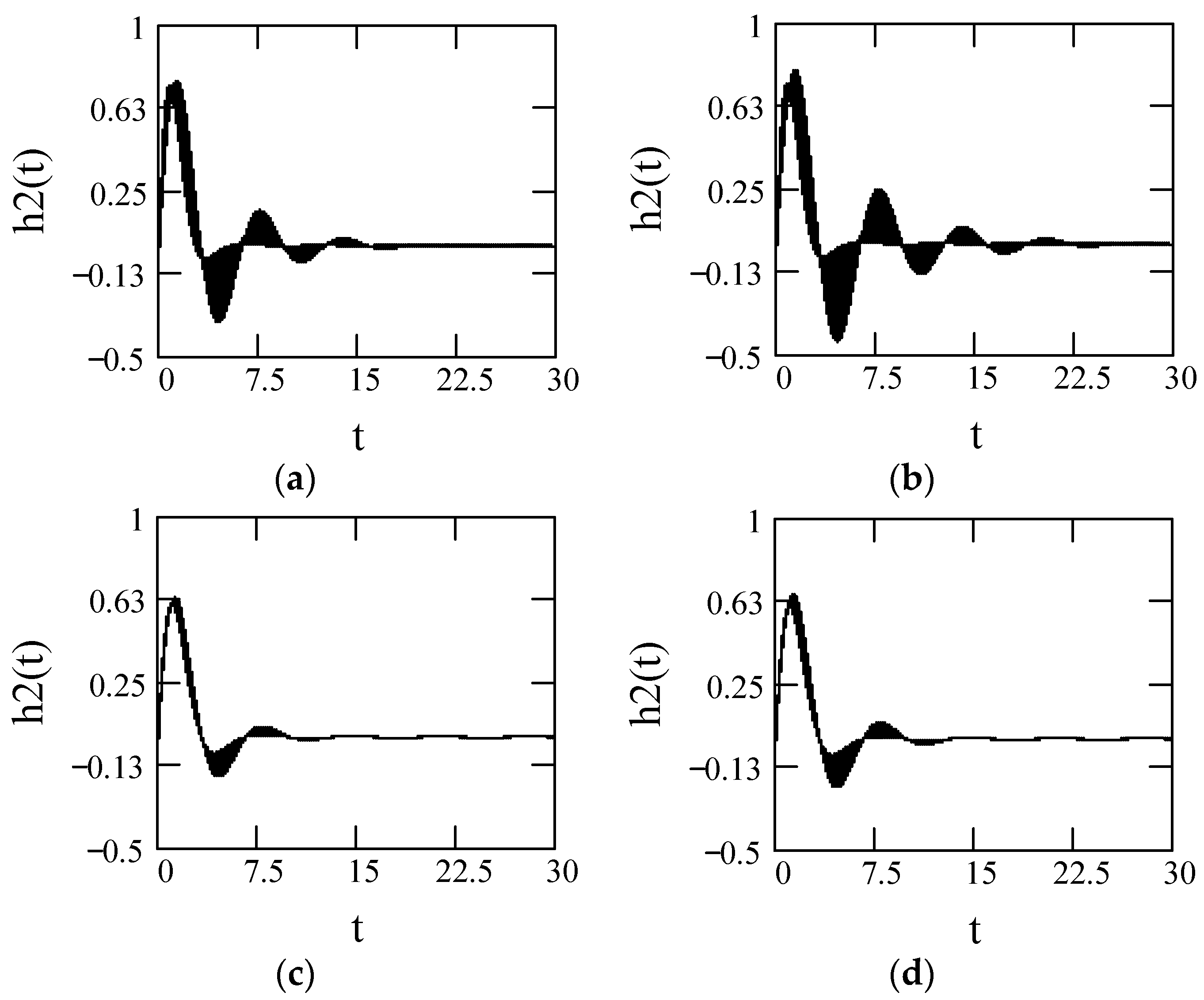
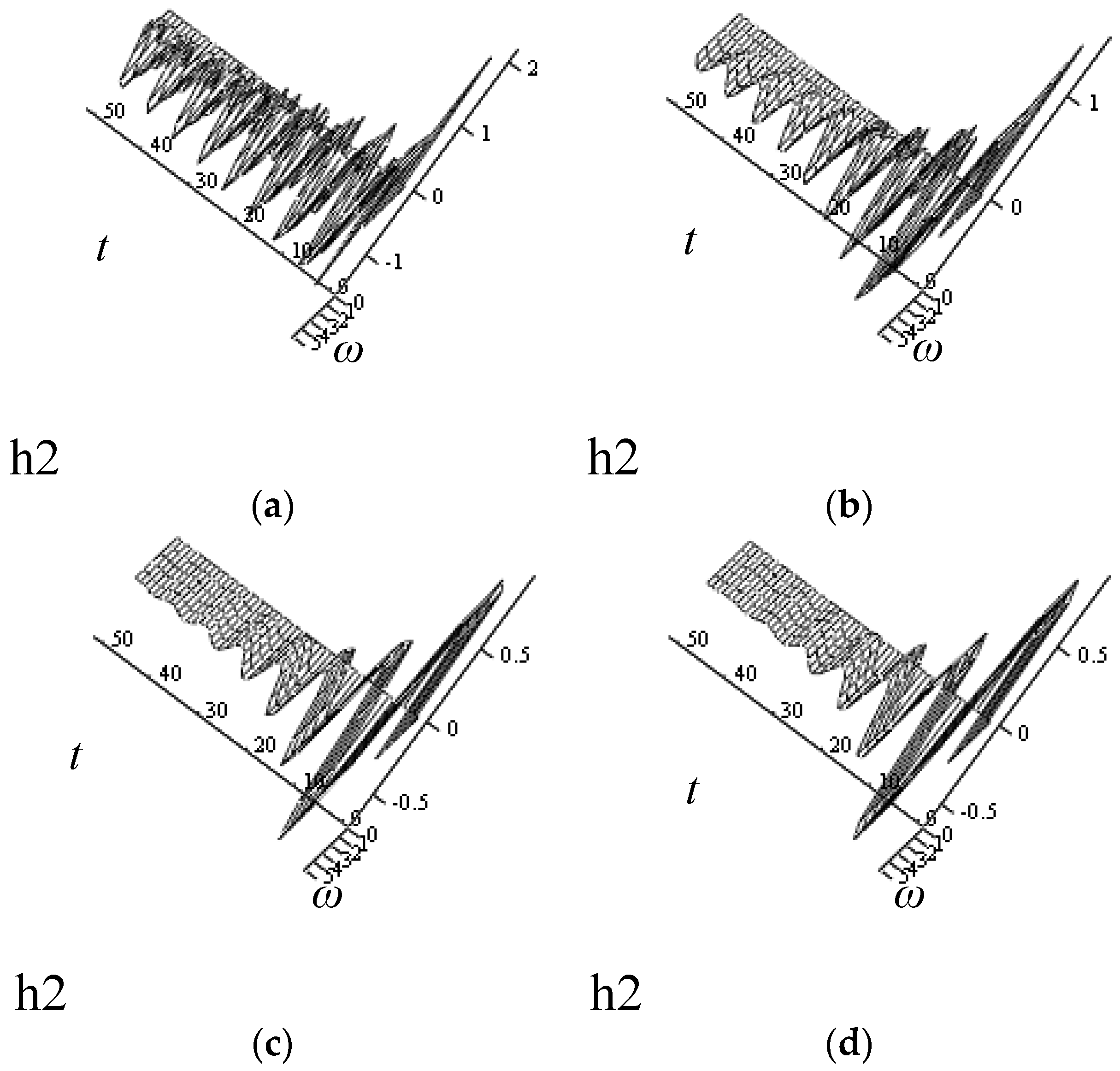
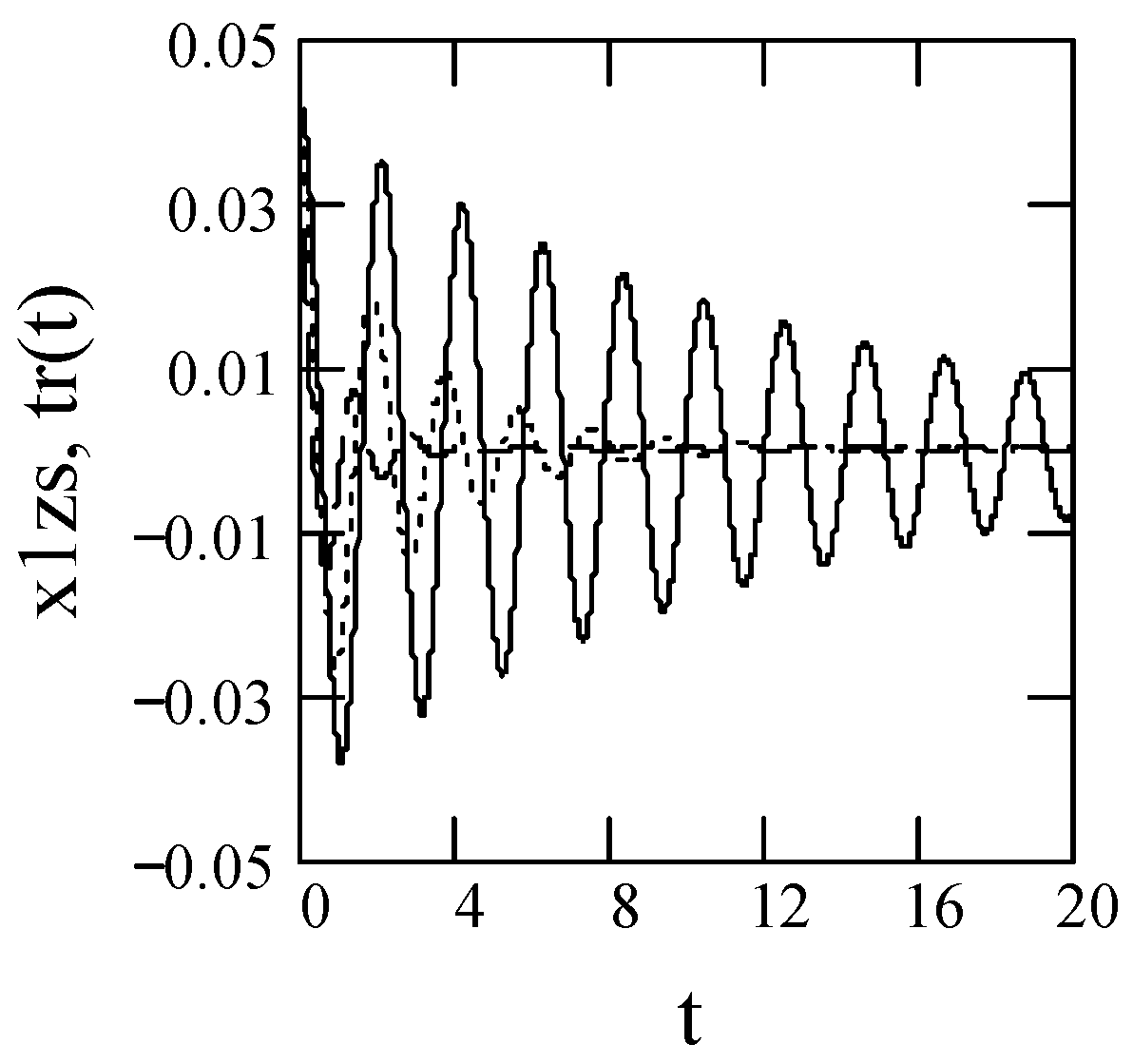
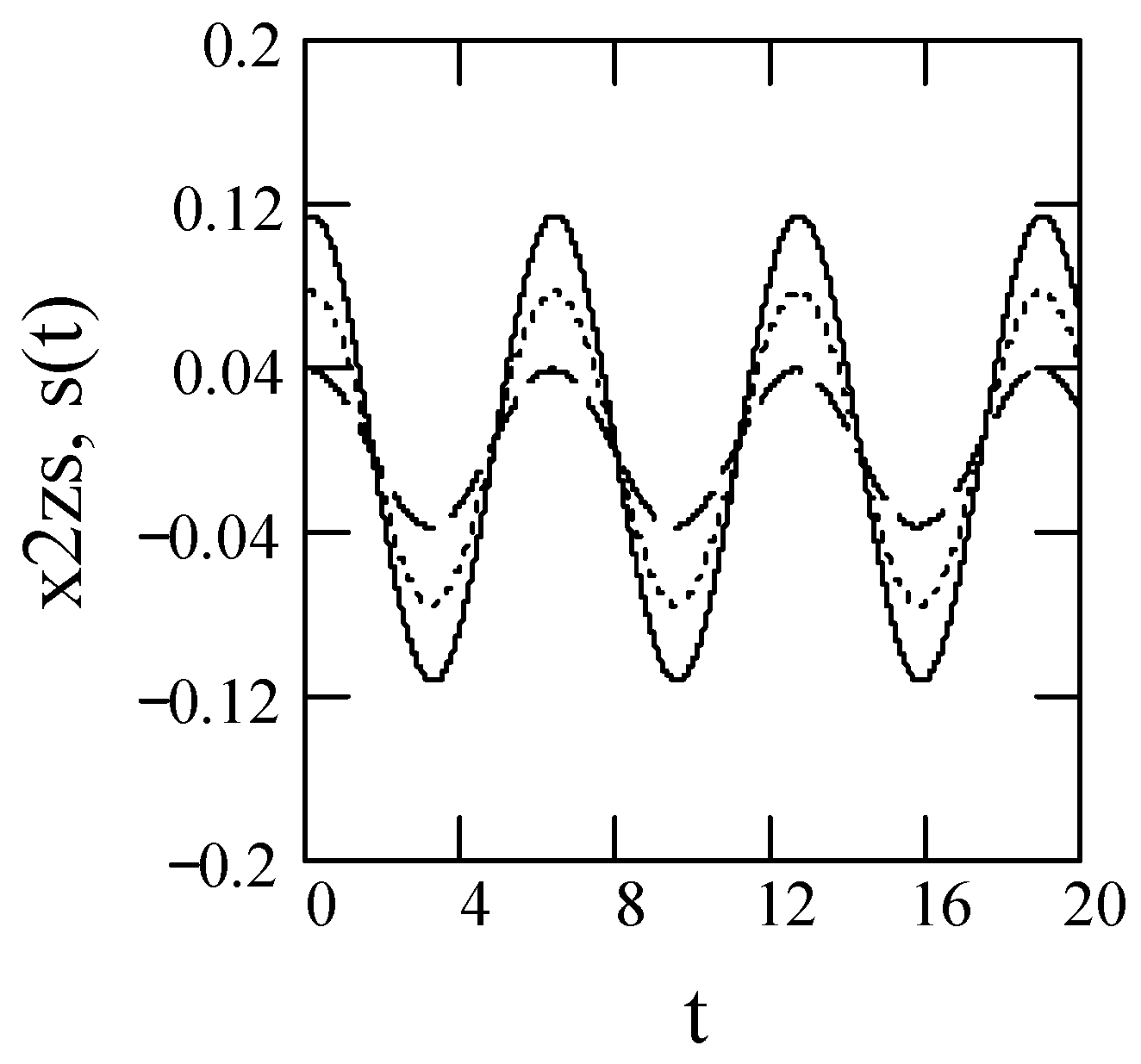
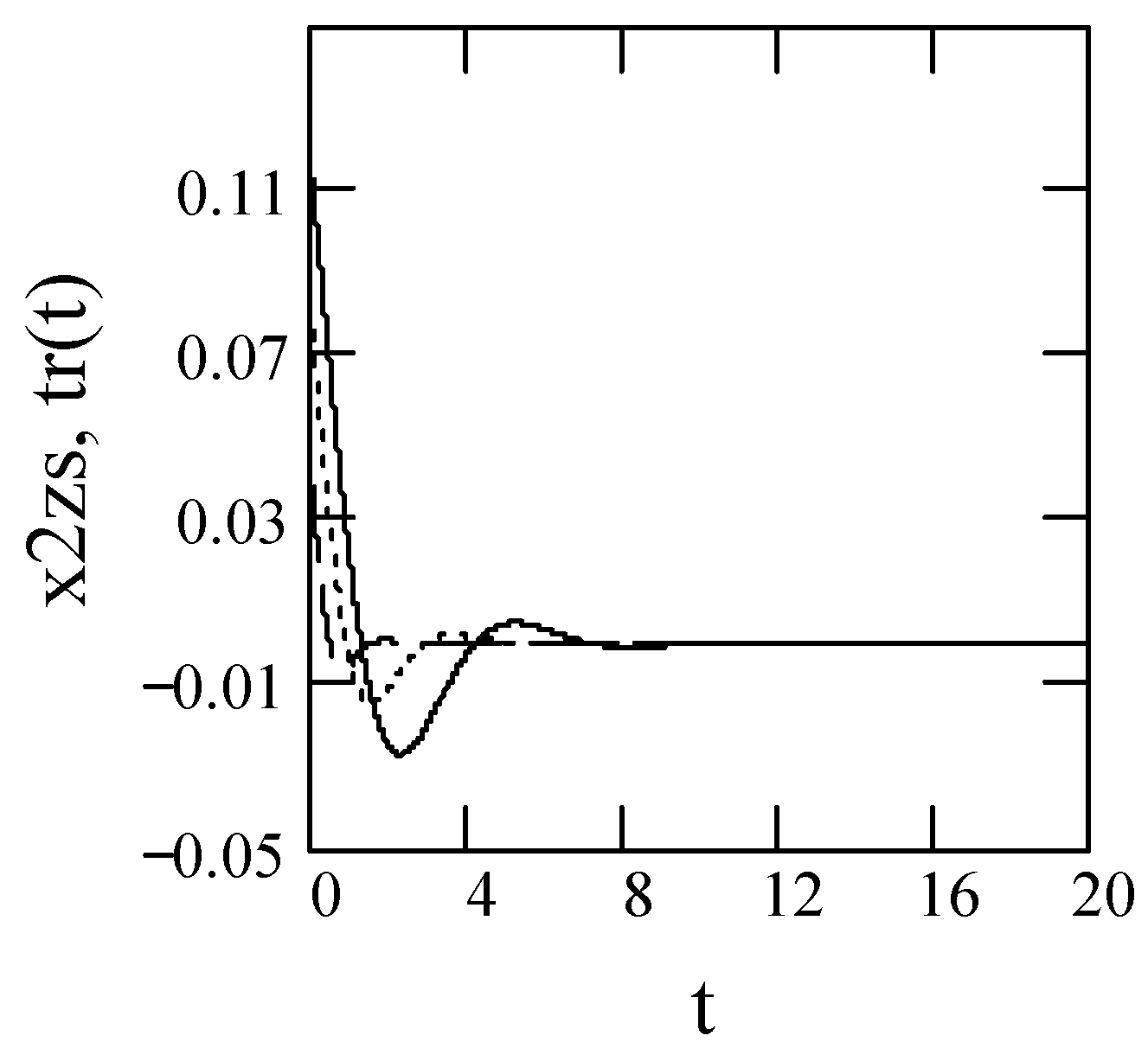
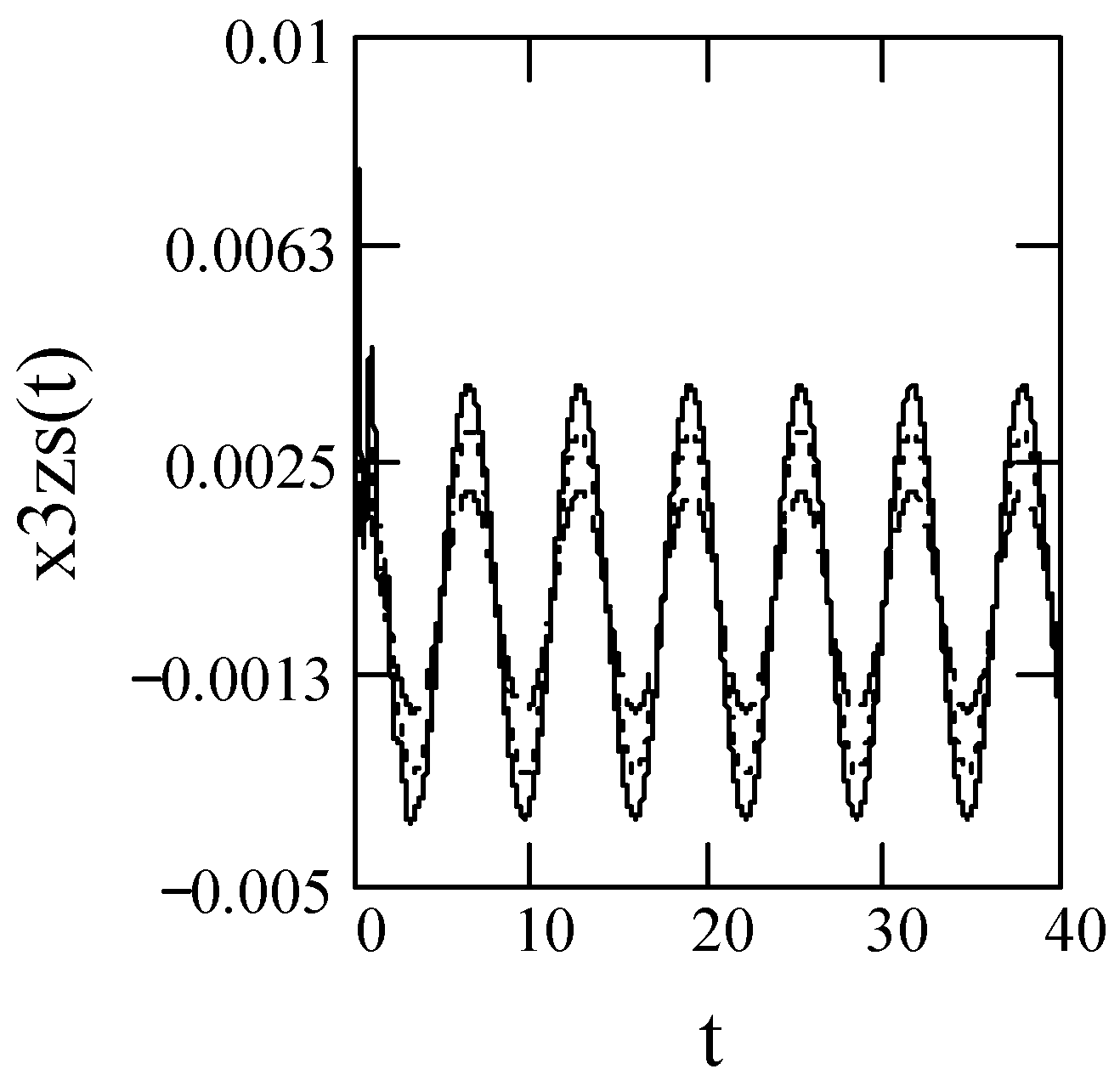
6. Free Responses to Three Classes of Fractional Oscillators
- First, proposing the free responses to three classes of fractional oscillators using the way of solving conventional oscillators.
- Then, since the responses to conventional oscillators are represented by elementary functions while those to fractional ones are expressed by special functions, such as the Mittag-Leffler function and its generalizations, we shall present novel representation to a certain special functions by elementary ones.
- Finally, analytic expressions of the logarithmic decrements, which are useful in practice, of three classes of fractional oscillators are proposed.
6.1. General Form of Free Responses
6.2. Free Response to Fractional Oscillators in Class I
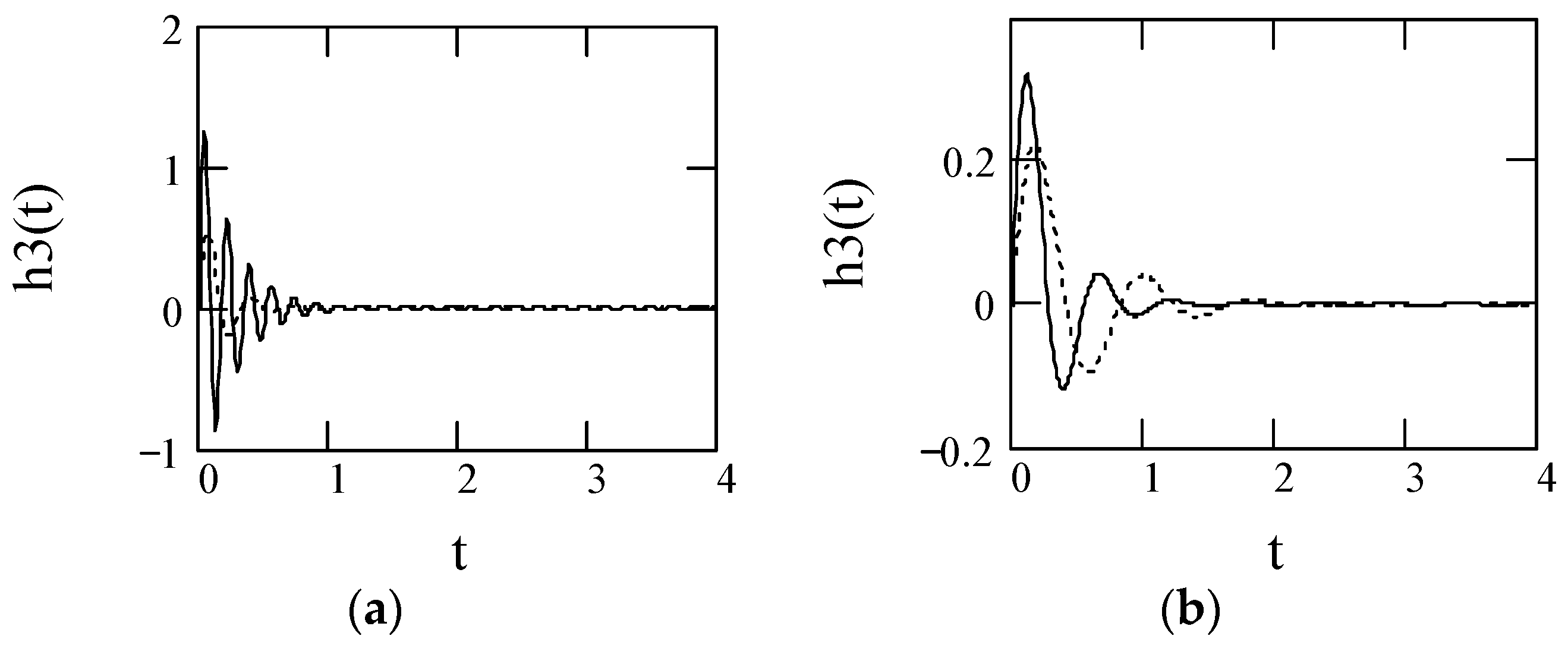
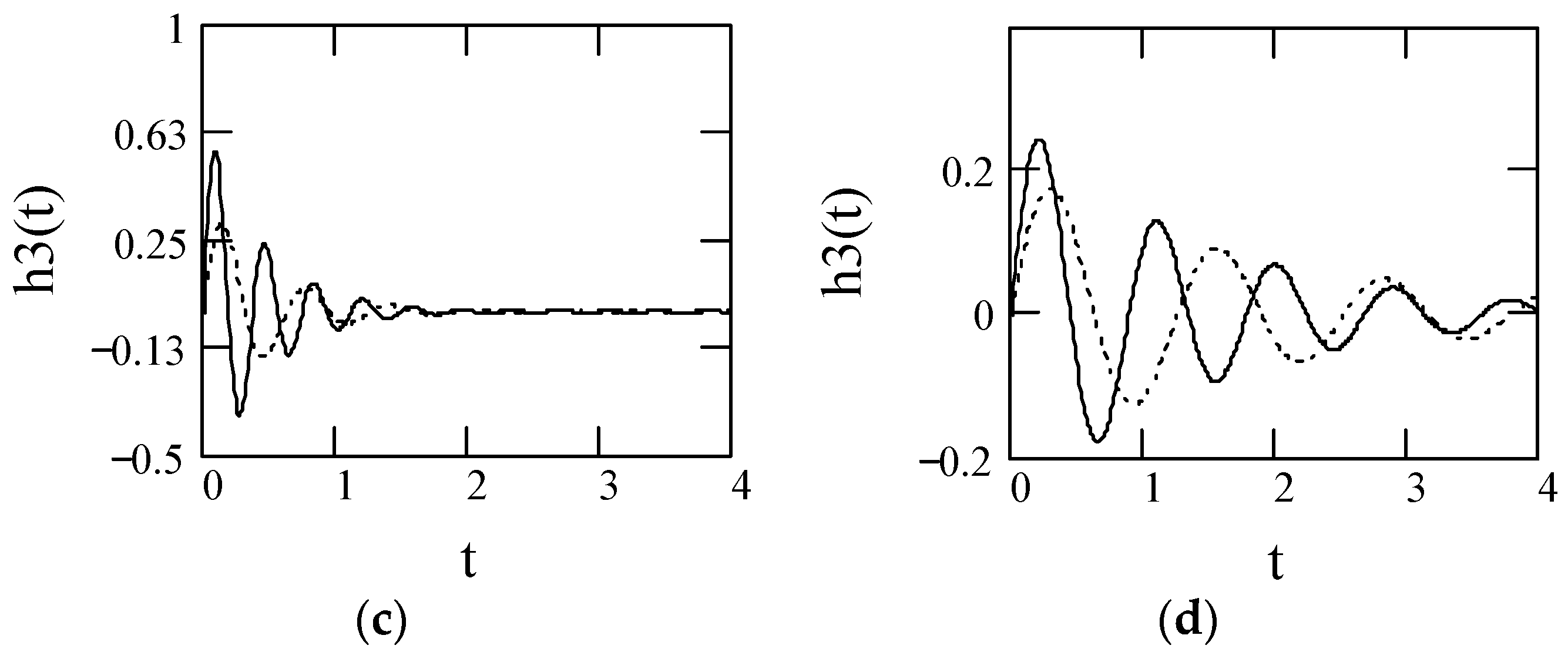
6.3. Free Response to Fractional Oscillators in Class II
6.4. Free Response to Fractional Oscillators in Class III
6.5. Application to Representing Generalized Mittag-Leffler Function (1)
7. Impulse Responses to Three Classes of Fractional Oscillators
7.1. General Form of Impulse Responses
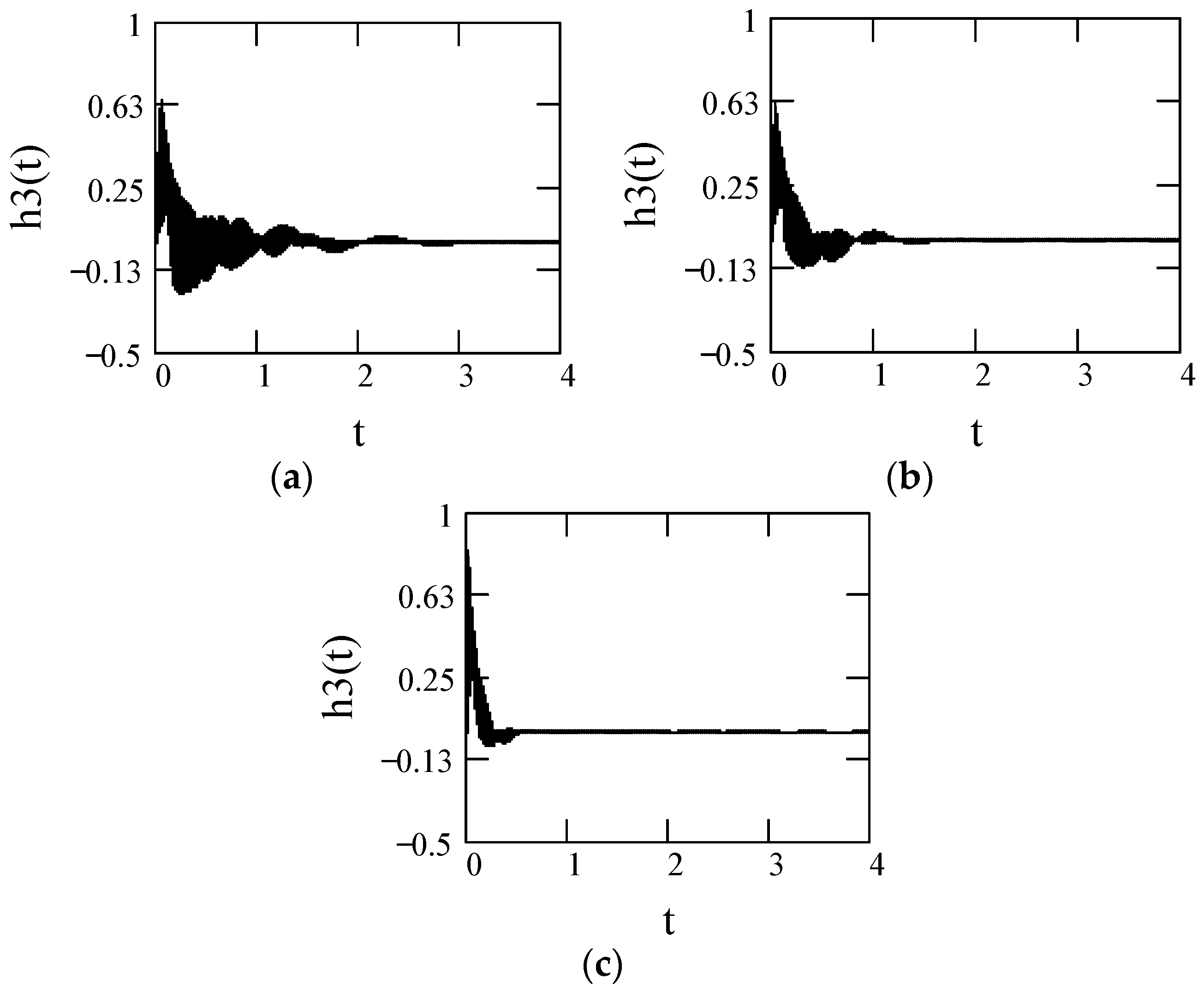
7.2. Impulse Response to Fractional Oscillators in Class I
7.3. Impulse Response to Fractional Oscillators in Class II
7.4. Impulse Response to Fractional Oscillators in Class III
7.5. Application to Represetenting Generalized Mittag-Leffler Function (2)
8. Step Responses to Three Classes of Fractional Oscillators
8.1. General Form of Step Responses
8.2. Step Response to a Fractional Oscillator in Class I
8.3. Step Response to a Fractional Oscillator in Class II
8.4. Step Response to a Fractional Oscillator in Class III
8.5. Application to Represetenting Mittag-Leffler Function (3)
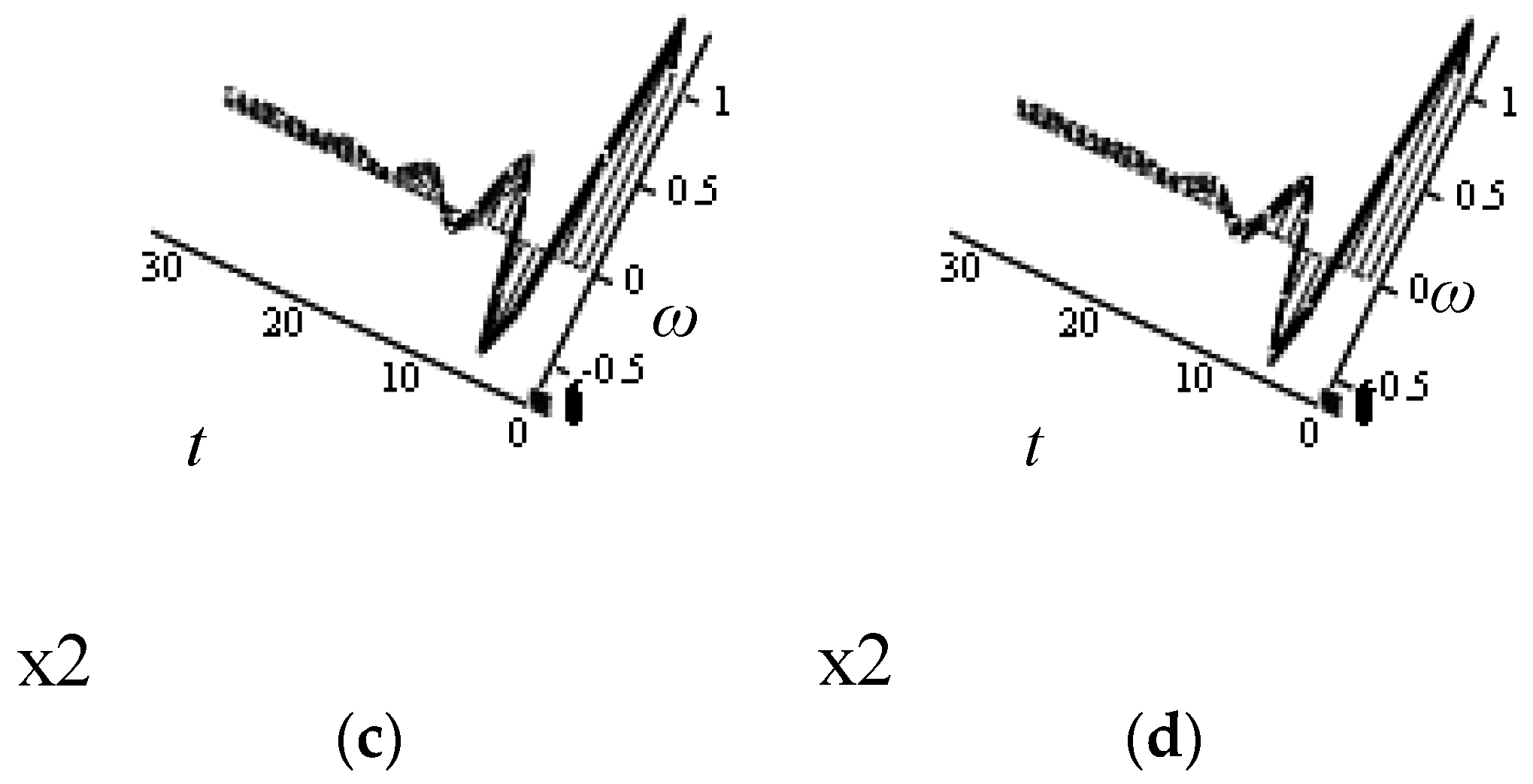
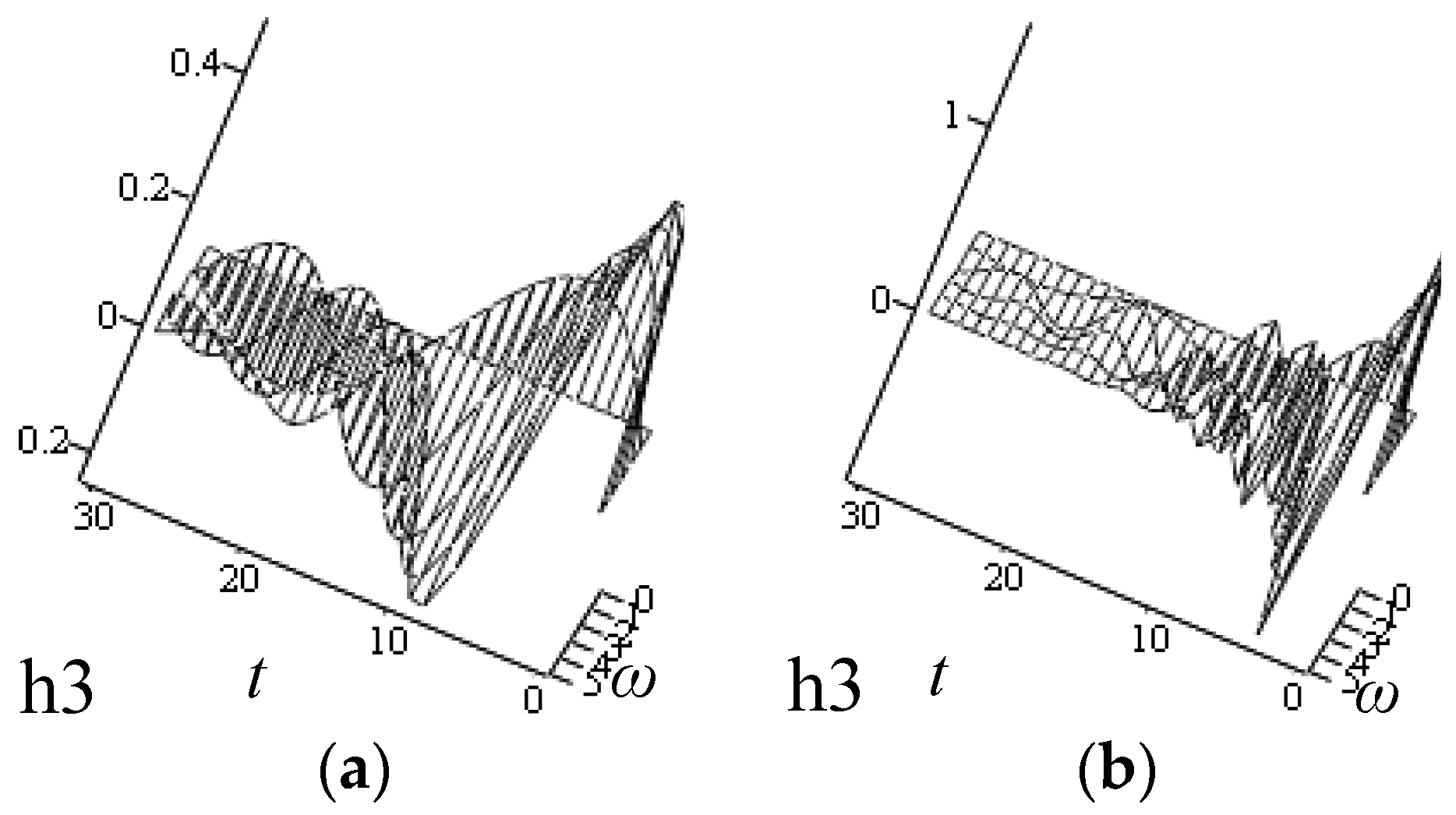
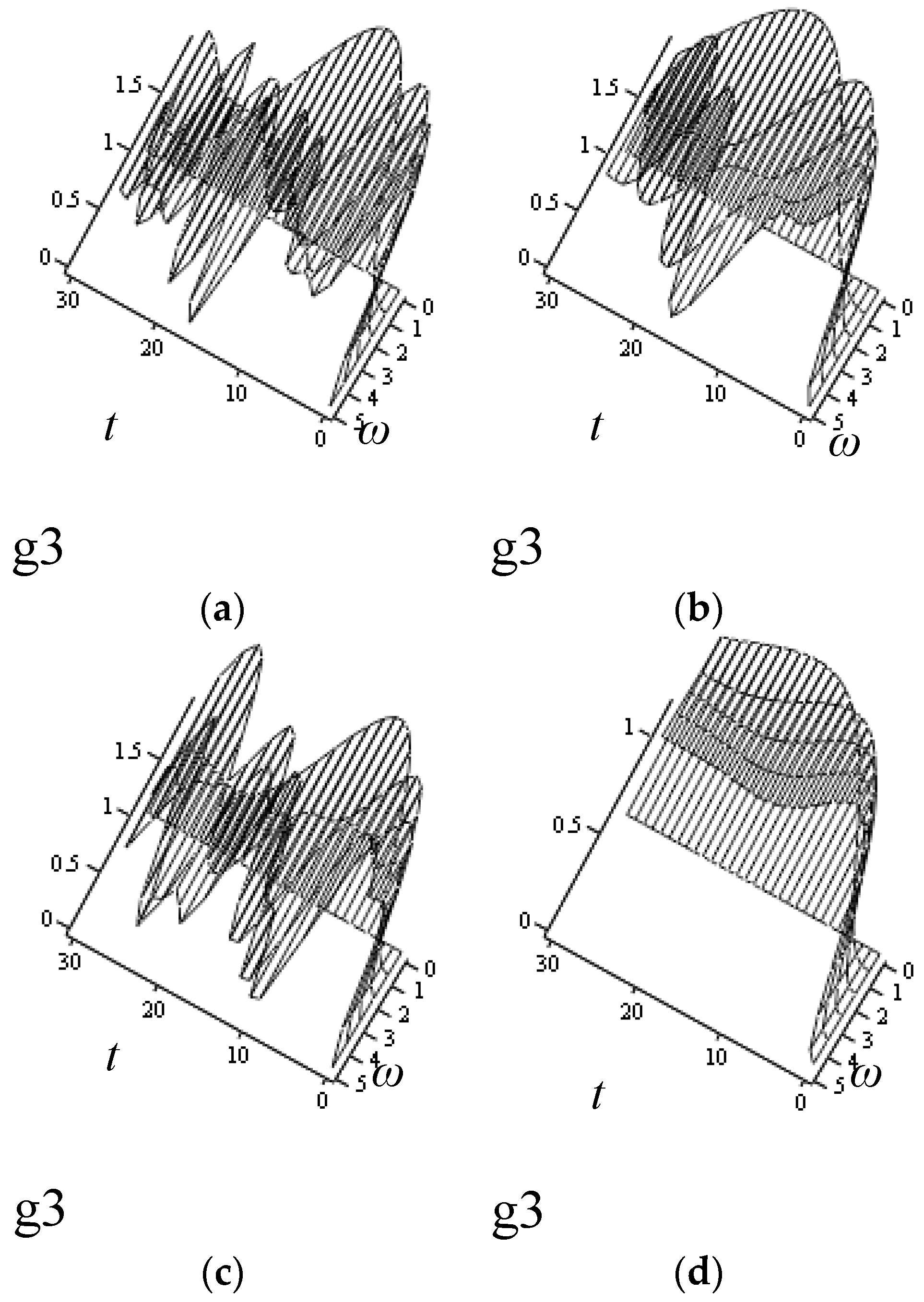
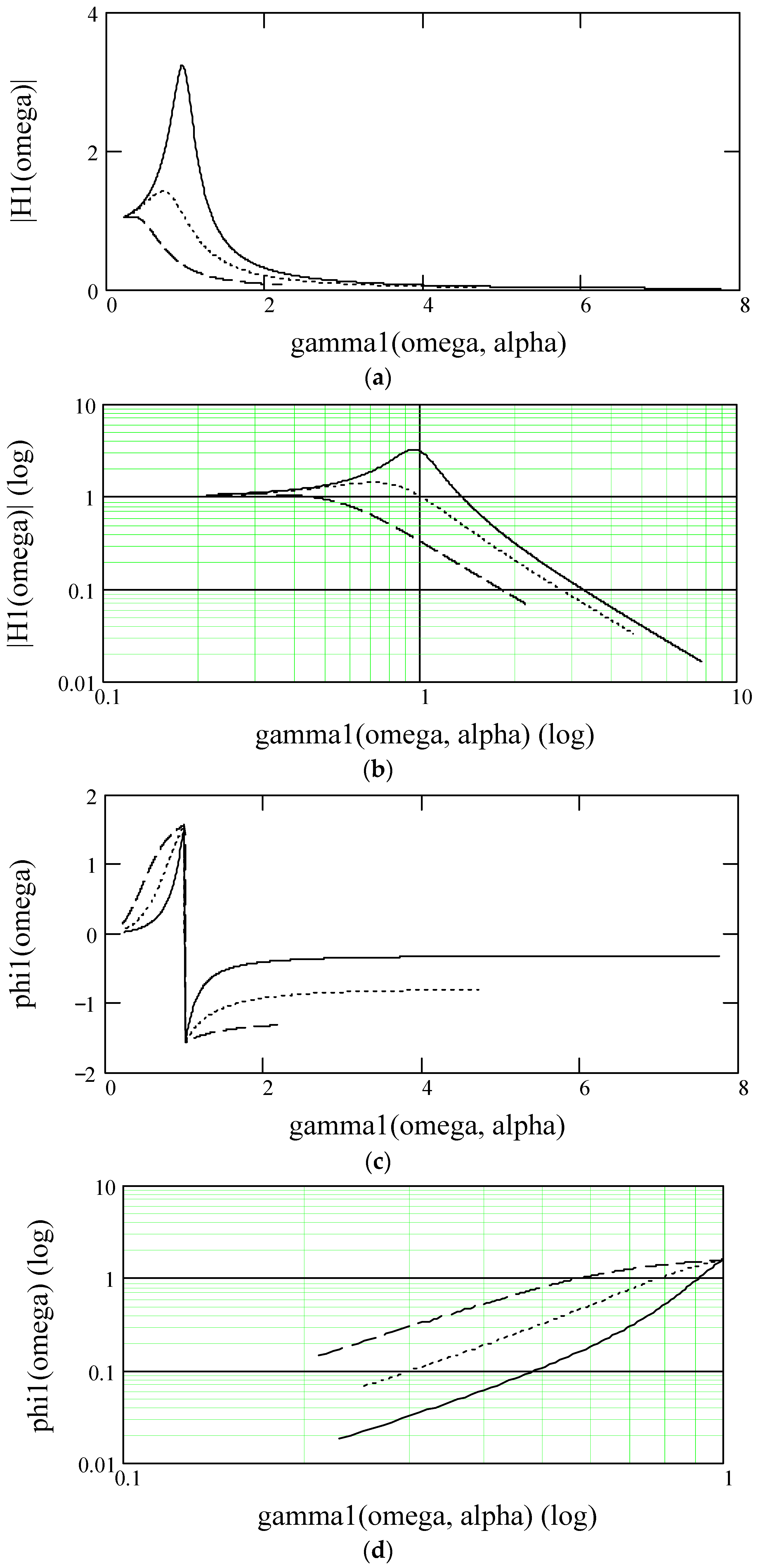
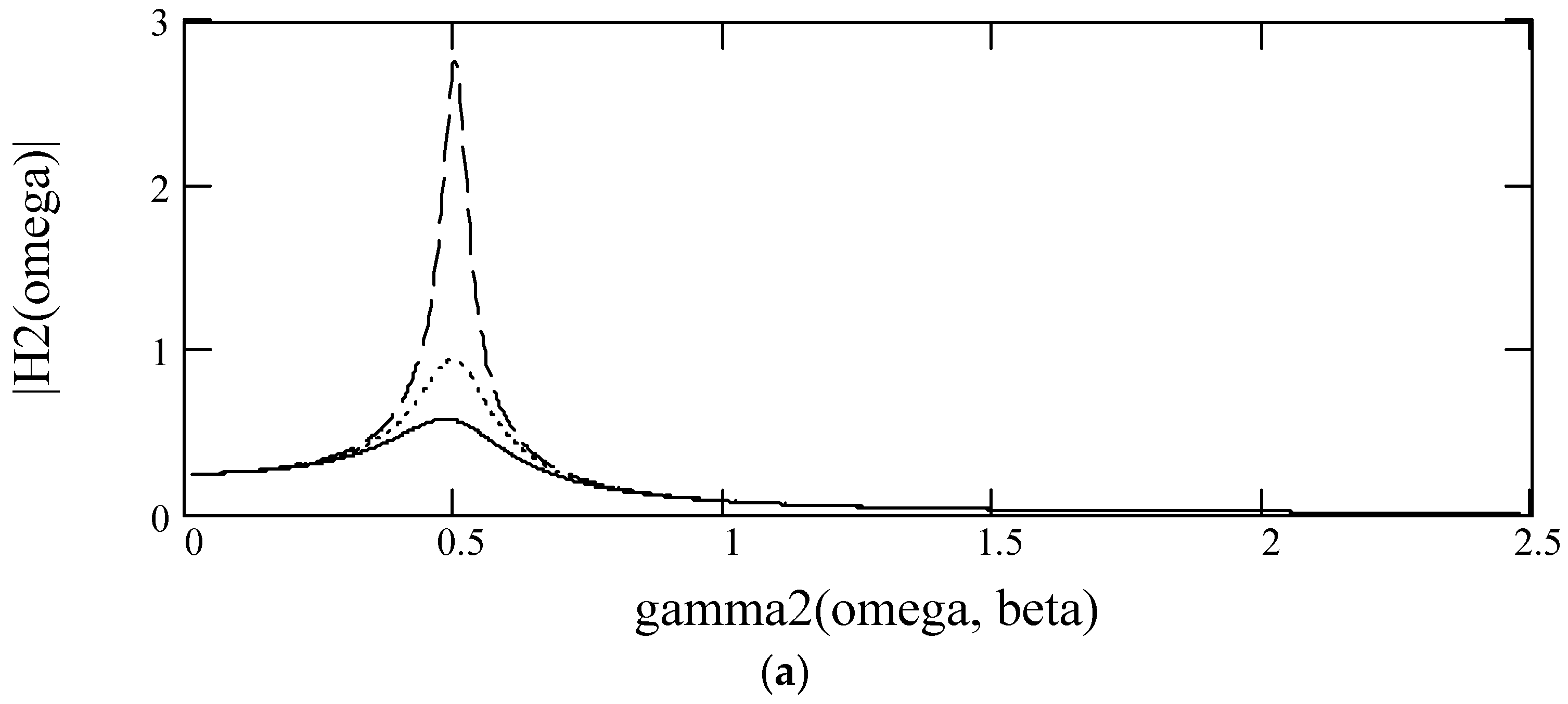
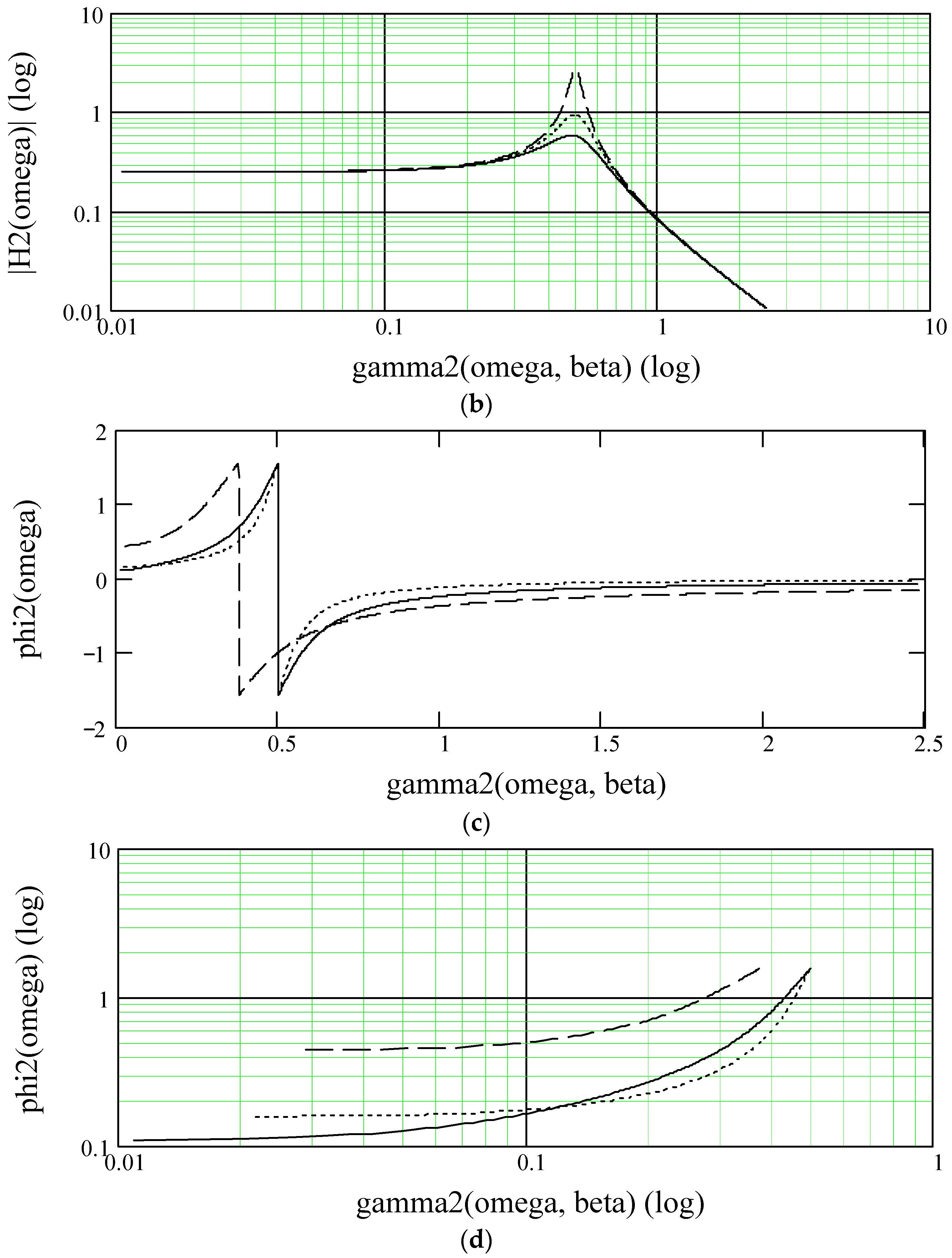
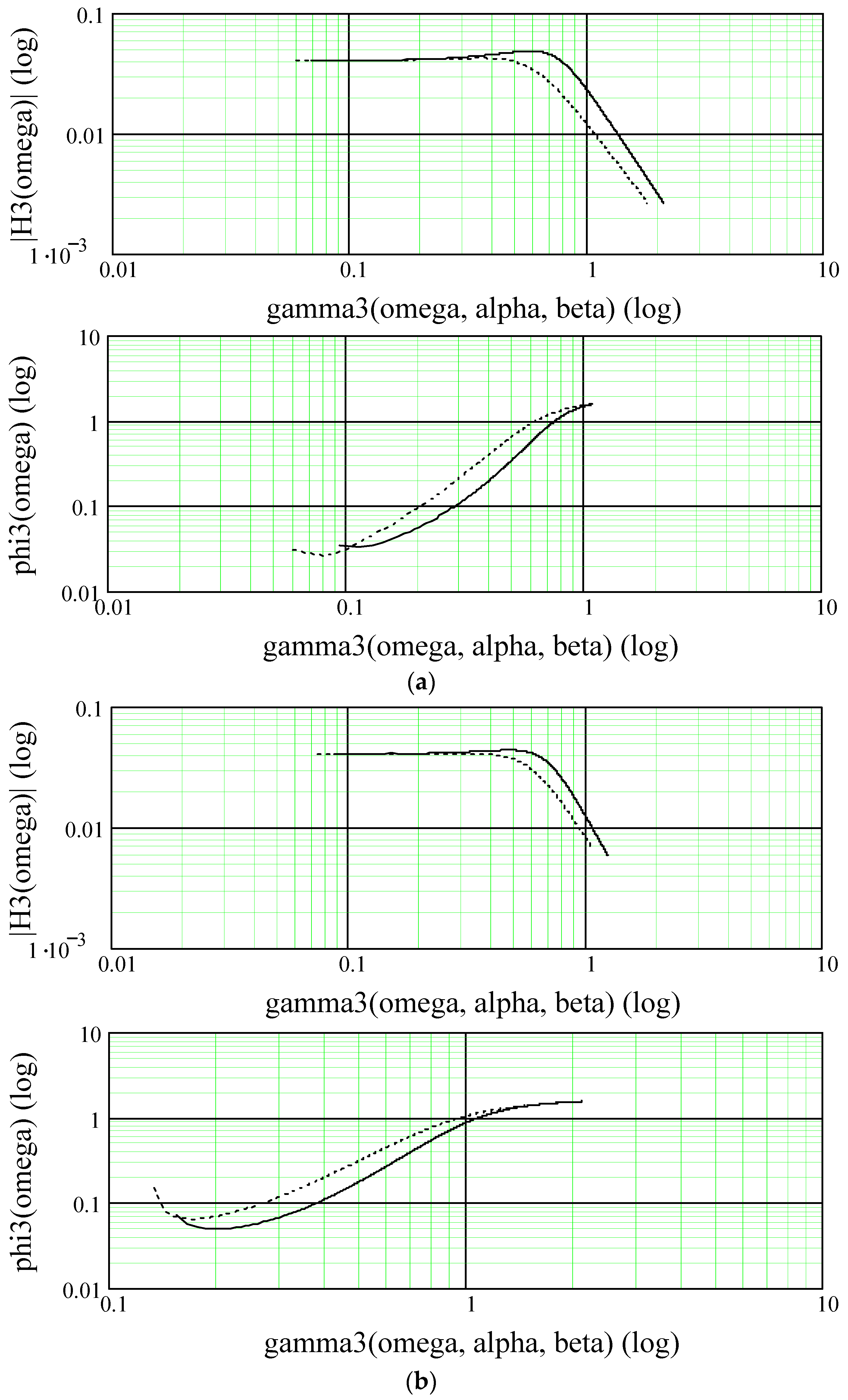
9. Frequency Responses to Three Classes of Fractional Oscillators
9.1. General Form of Frequency Responses to Three Classes of Fractional Oscillators
9.2. Frequency Response to a Fractional Oscillator in Class I
9.3. Frequency Response to a Fractional Oscillator in Class II
9.4. Frequency Response to a Fractional Oscillator in Class III
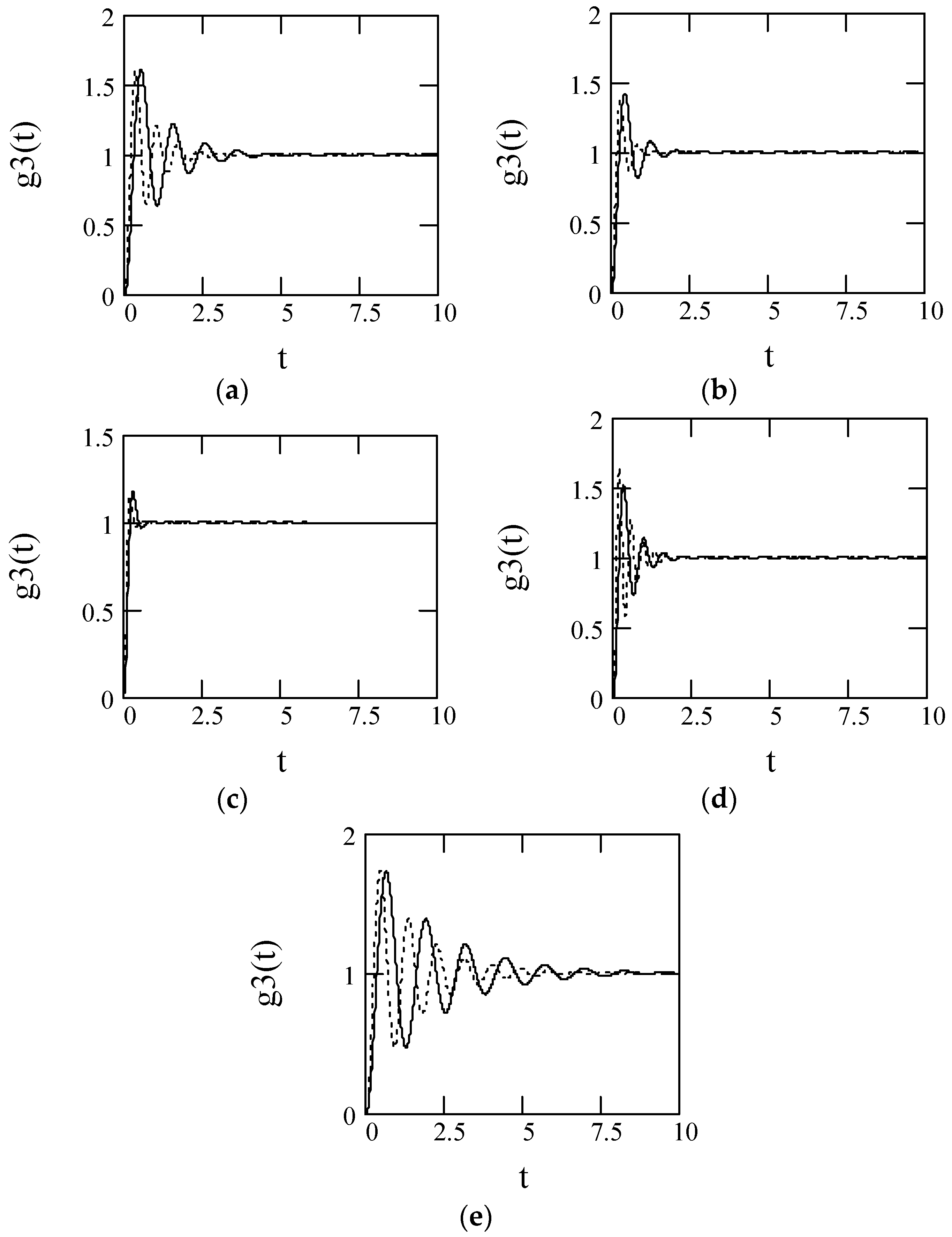
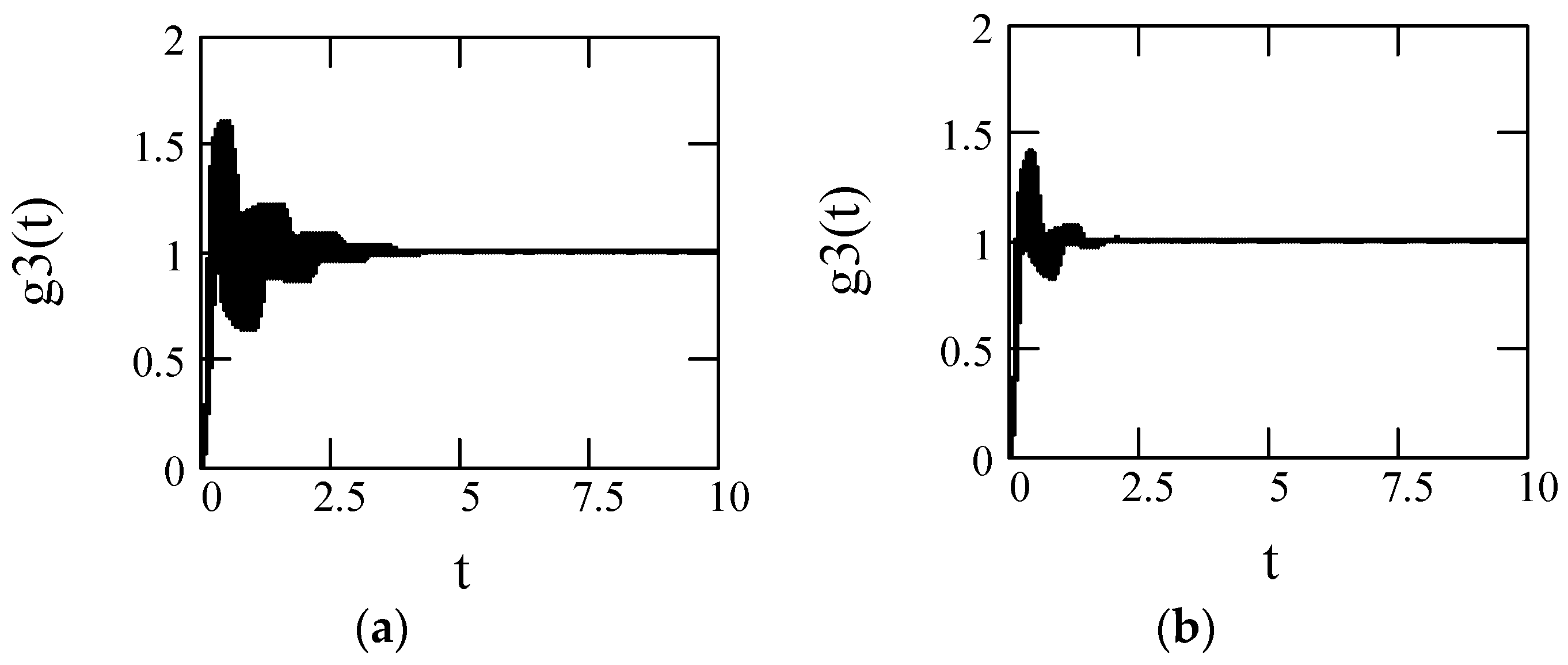
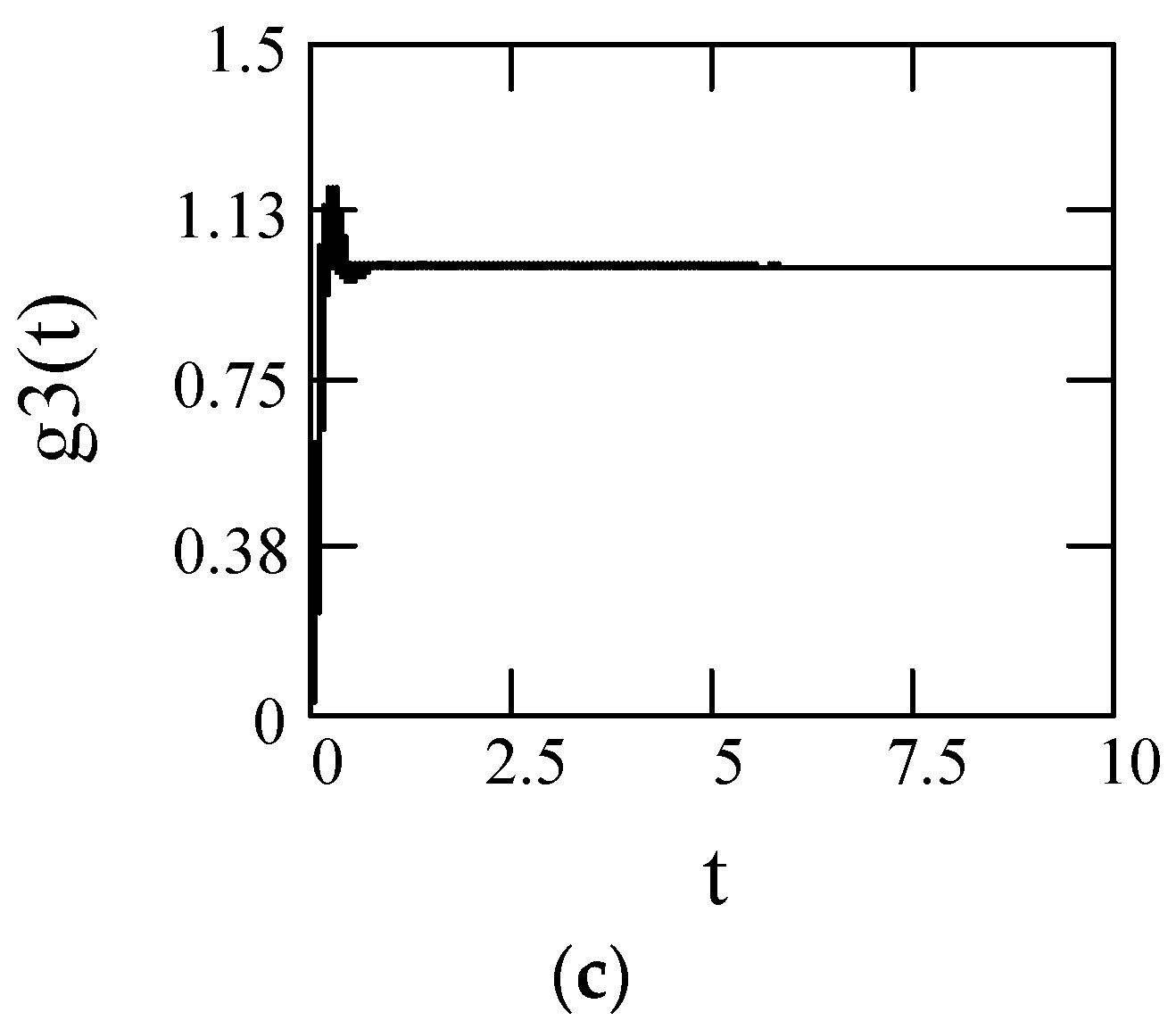
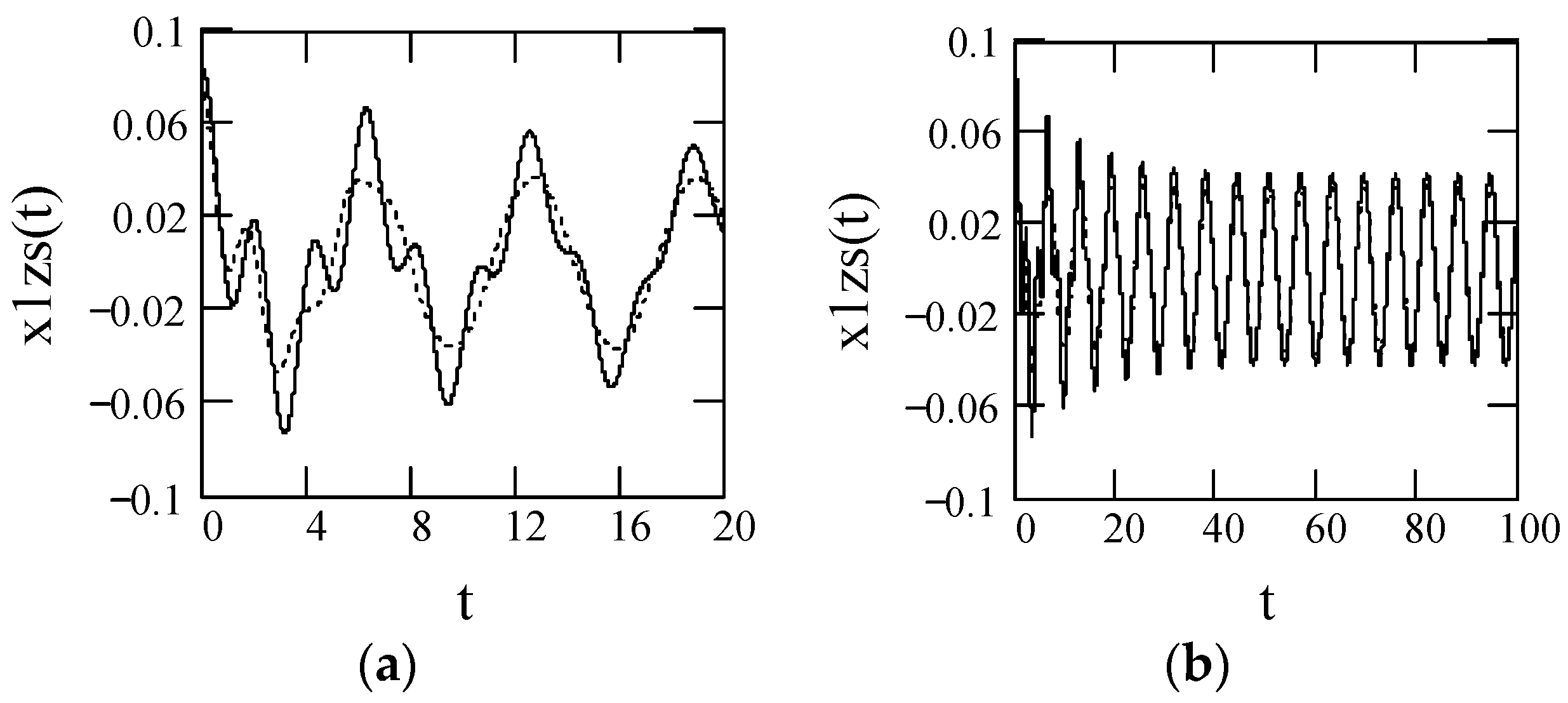
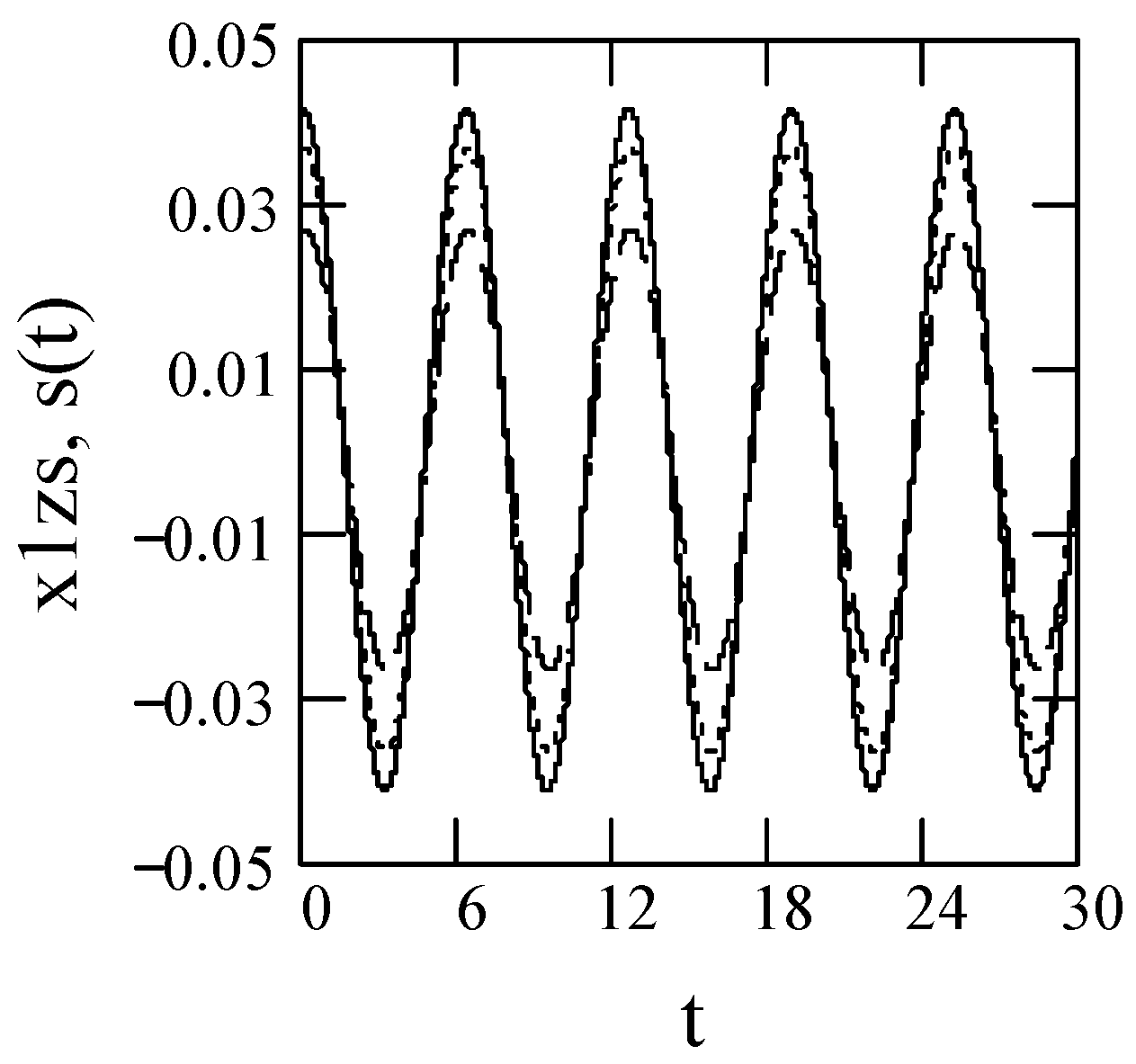
10. Sinusoidal Responses of Three Classes of Fractional Oscillators
10.1. Stating Problem
10.2. Stating Research Thought
10.3. General Form of Sinusoidal Responses to Three Classes of Fractional Oscillators
10.4. Sinusoidal Response to Fractional Oscillators in Class I
10.5. Sinusoidal Response to Fractional Oscillators in Class II
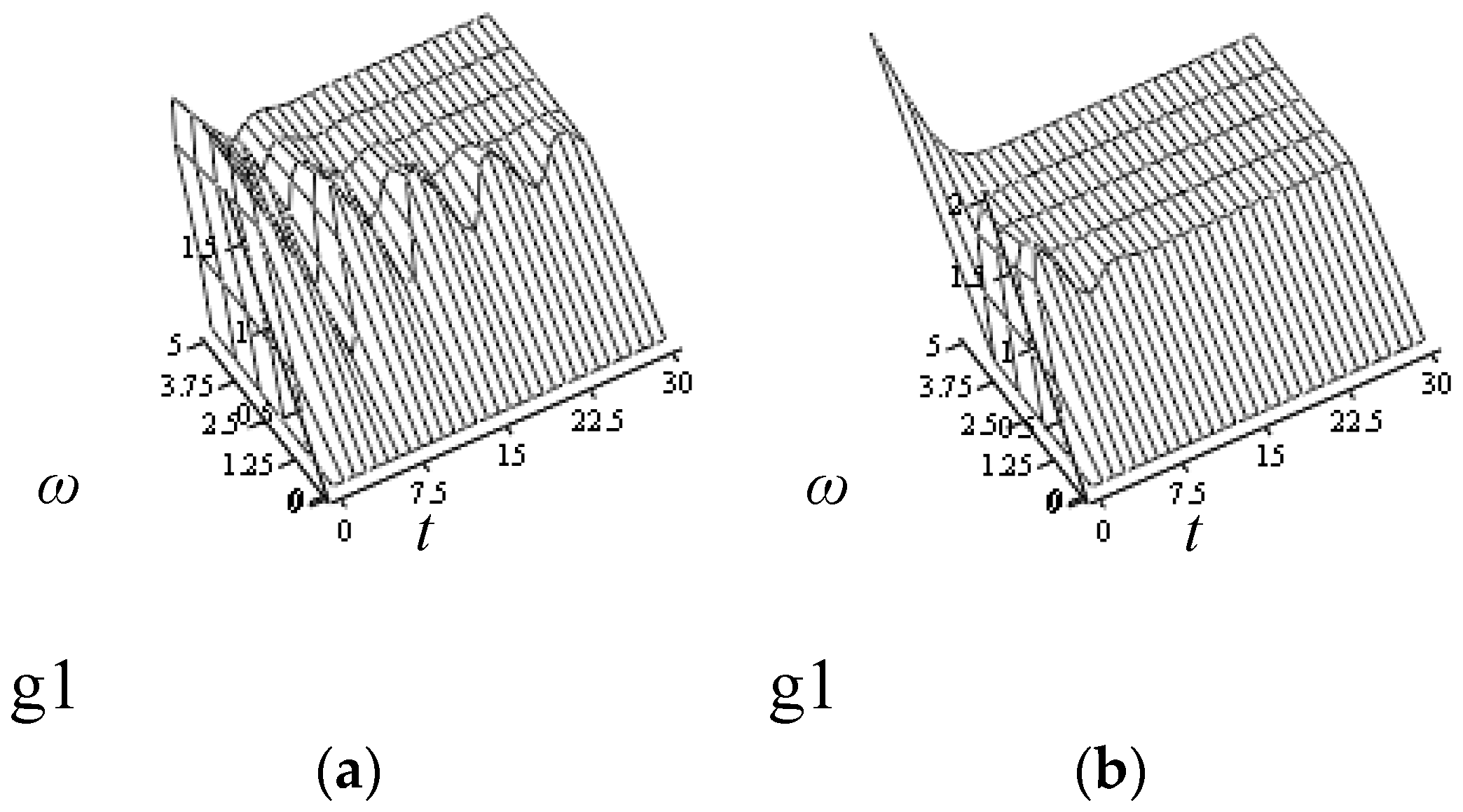
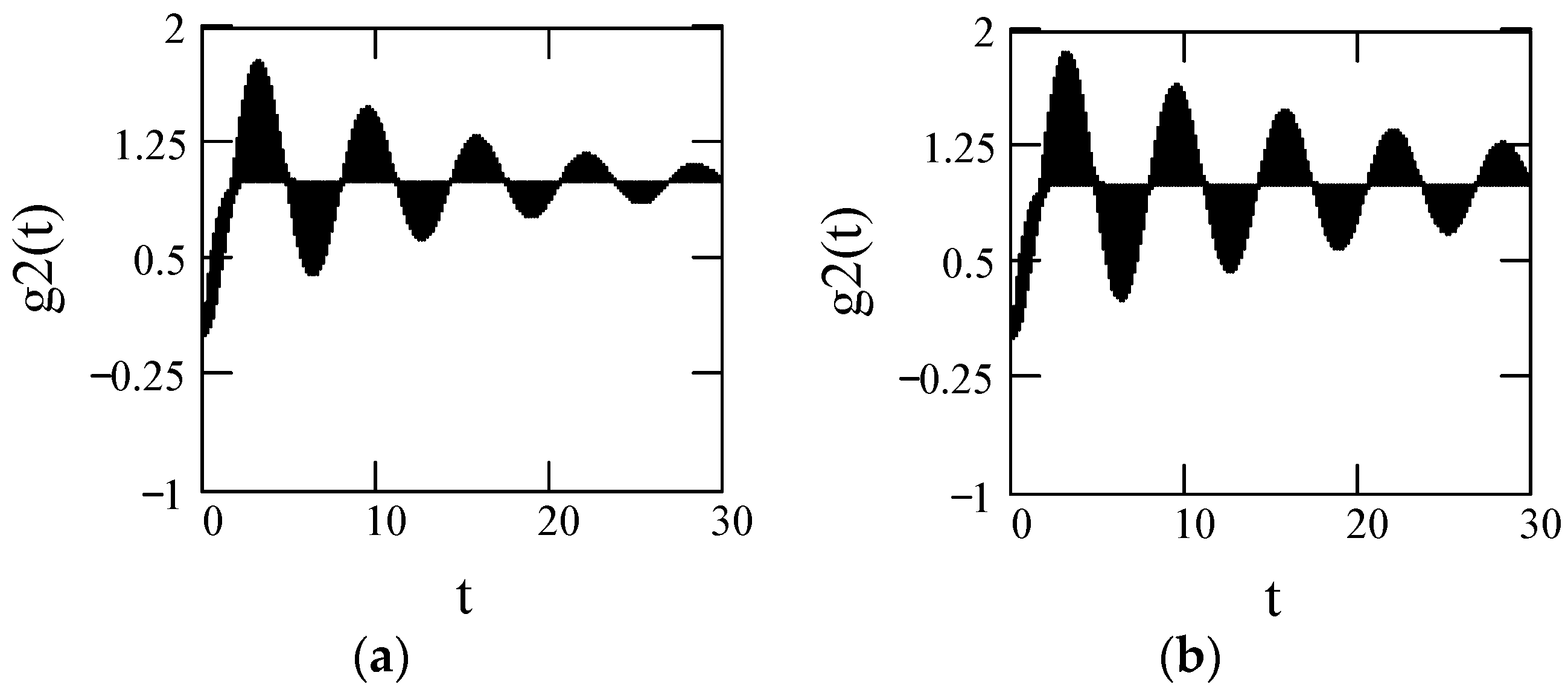
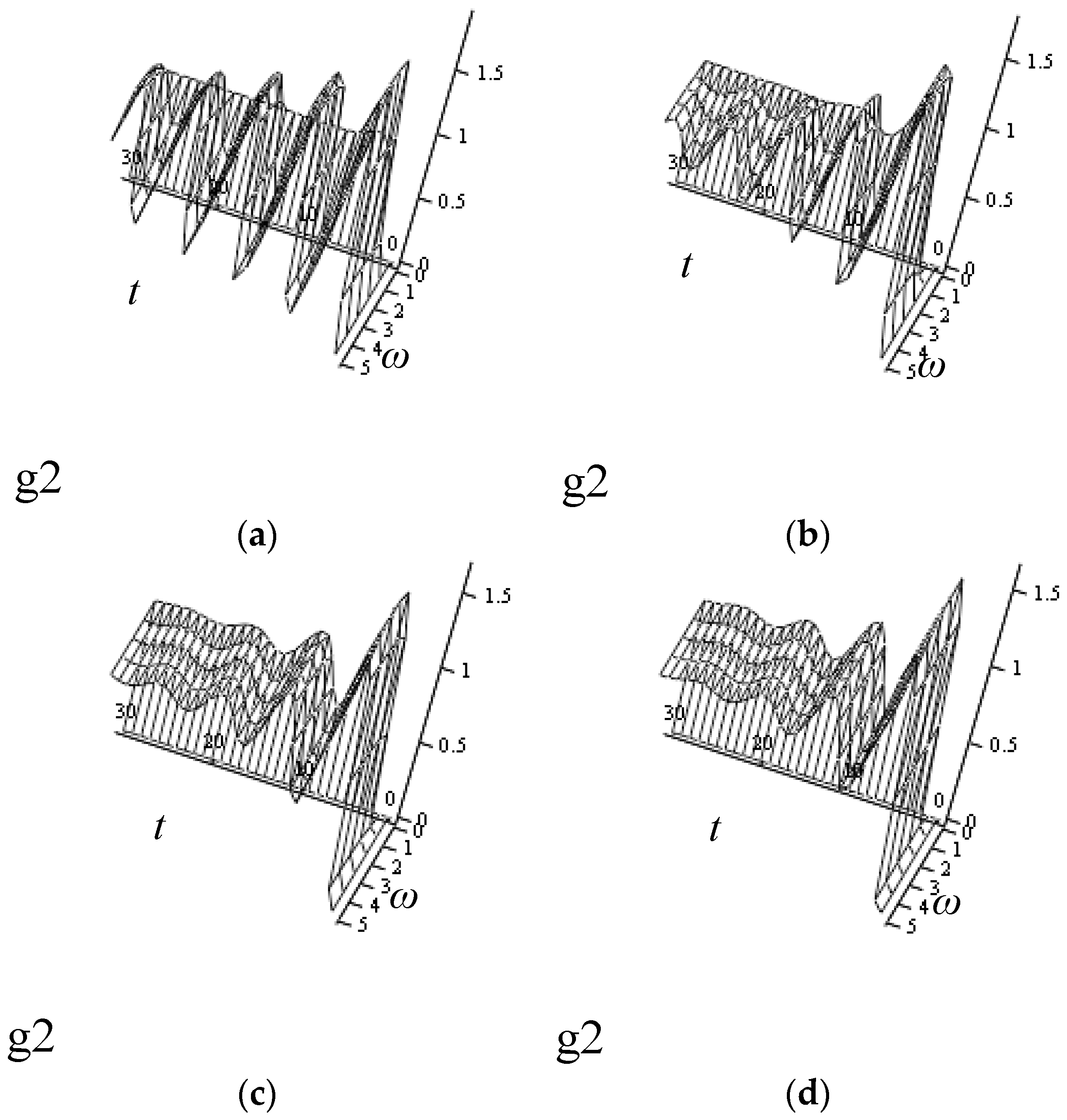
10.6. Sinusoidal Response to Fractional Oscillators in Class III
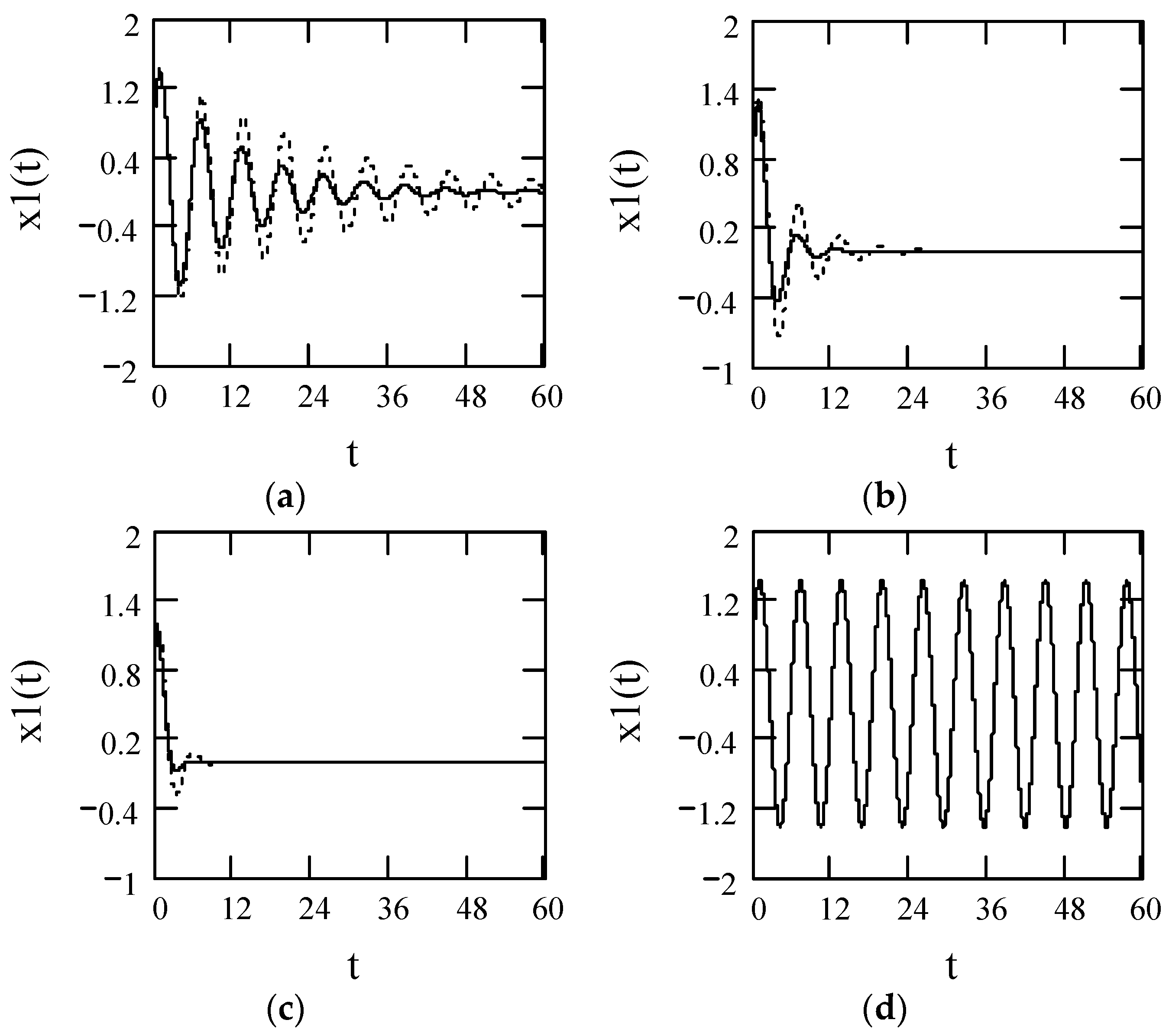
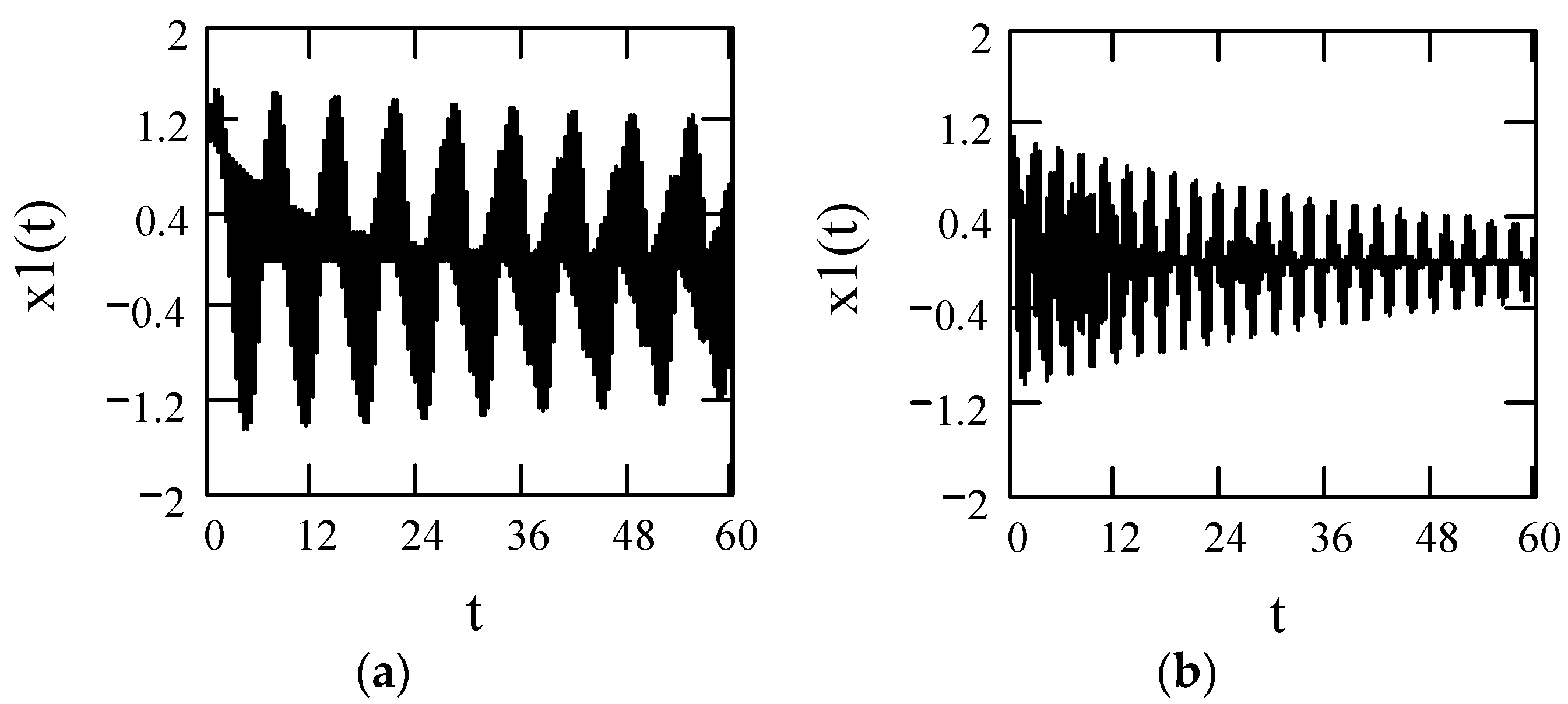
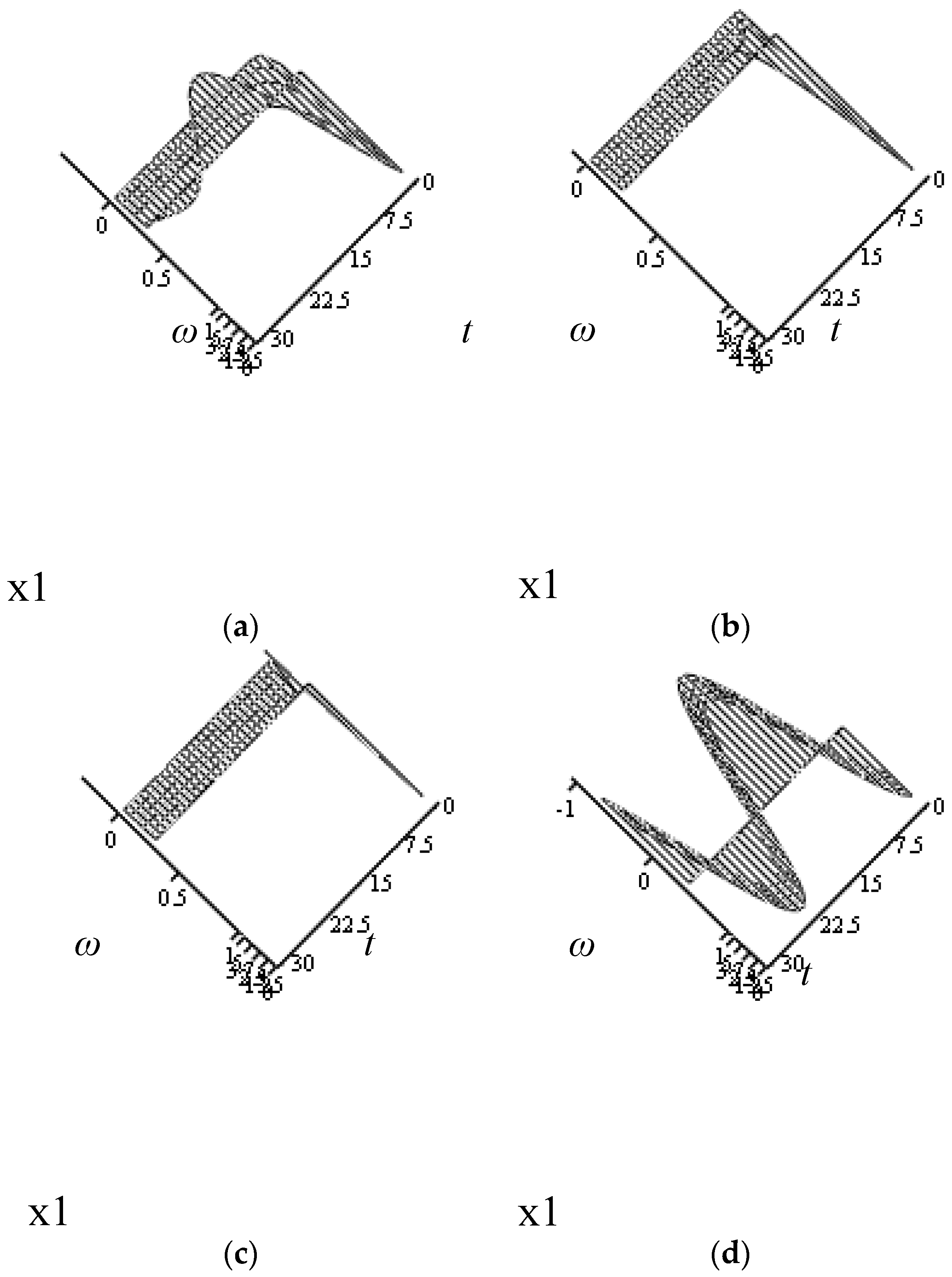
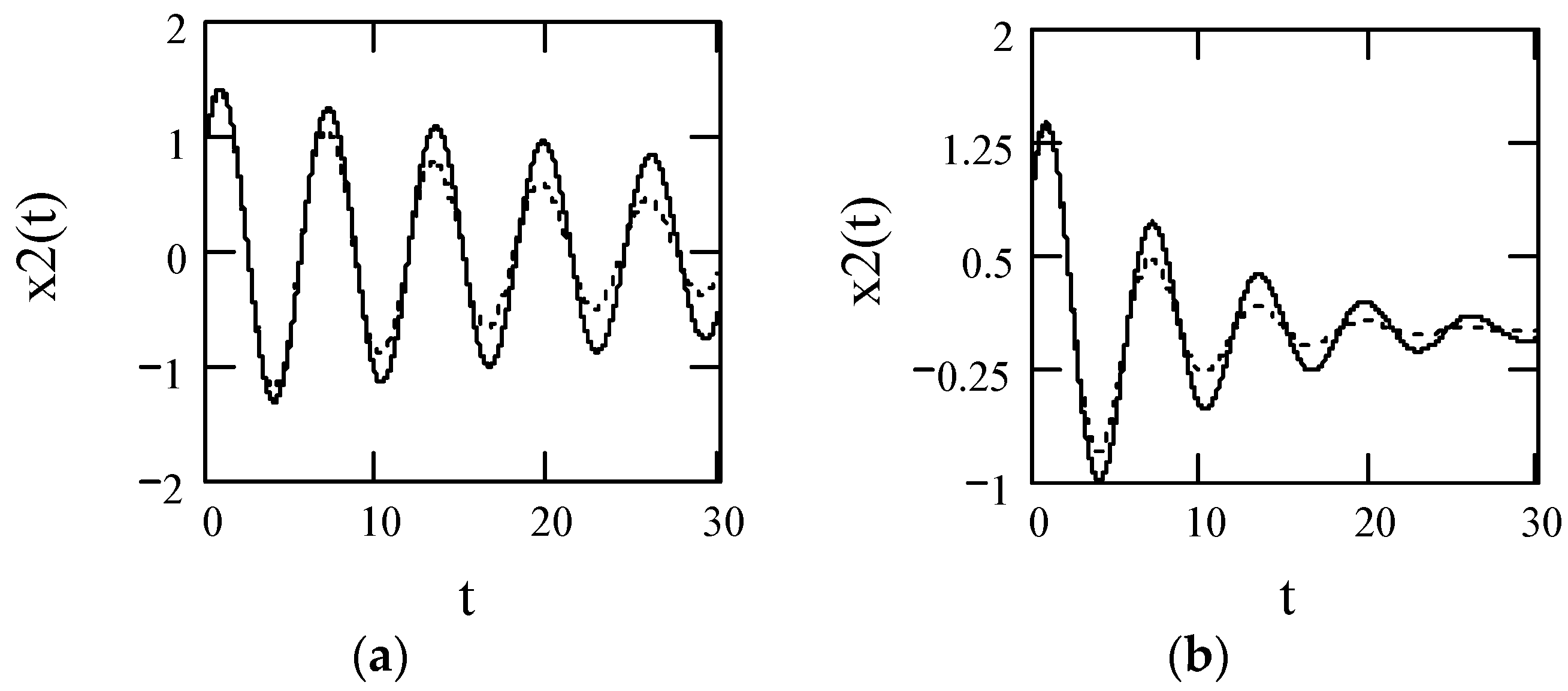
11. Discussion
12. Conclusions
Acknowledgments
Conflicts of Interest
References
- Timoshenko, S.P. Vibration Problems in Engineering, 2nd ed.; Fifth Printing; D. Van Nostrand Company, Inc.: New York, NY, USA, 1955. [Google Scholar]
- Timoshenko, S.P. Mechanical Vibrations; D. Van Nostrand Company, Inc.: New York, NY, USA, 1955. [Google Scholar]
- Den Hartog, J.P. Mechanical Vibrations; McGraw-Hill: New York, NY, USA, 1956. [Google Scholar]
- Harris, C.M. Shock and Vibration Handbook, 5th ed.; McGraw-Hill: New York, NY, USA, 2002. [Google Scholar]
- Palley, O.M.; Bahizov, Г.B.; Voroneysk, E.Я. Handbook of Ship Structural Mechanics; Translated from Russian by Xu, B.H., Xu, X., Xu, M.Q.; National Defense Industry Publishing House: Beijing, China, 2002. (In Chinese) [Google Scholar]
- Rayleigh, J.W.S.B., III. The Theory of Sound; Macmillan & Co., Ltd.: London, UK, 1877; Volume 1. [Google Scholar]
- Lalanne, C. Mechanical Vibration and Shock, 2nd ed.; Wiley: New York, NY, USA, 2013; Volumes 1–5. [Google Scholar]
- Nilsson, J.W.; Riedel, S.A. Electronic Circuits, 9th ed.; Prentice Hall: Upper Saddle River, NJ, USA, 2011. [Google Scholar]
- Diaz, H.F.; Markgraf, V. El Niño and the Southern Oscillation Multiscale Variability and Global and Regional Impacts; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Hurrell, J.W.; Kushnir, Y.; Ottersen, G.; Visbeck, M. The North Atlantic Oscillation: Climatic Significance and Environmental Impact; John Wiley & Sons: New York, NY, USA, 2003; Volume 134. [Google Scholar]
- Xu, L.; Chen, Z.; Hu, K.; Stanley, H.E.; Ivanov, P.C. Spurious detection of phase synchronization in coupled nonlinear oscillators. Phys. Rev. E 2006, 73, 065201. [Google Scholar] [CrossRef] [PubMed]
- Fourier, J. Thkorie Analytique de la Chaleur; Didot: Paris, France, 1822. [Google Scholar]
- Bernoulli, D. Hydrodynamica, Sive de Viribus et Motibus Fluidorum Commentarii. Opus Academicum ab Auctore, dum Petropoli Ageret, Congestum; Argentorati, Sumptibus Johannis Reinholdi Dulseckeri, Typis Joh. Deckeri, Typographi Basiliensis: ETH-Bibliothek Zürich, Zürich, Switzerland, 1738. [Google Scholar] [CrossRef]
- Timoshenko, S.P. On the transverse vibrations of bars of uniform cross-section. Philos. Mag. 1922, 43, 125–131. [Google Scholar] [CrossRef]
- Searle, J.H.C. The Effect of rotatory inertia on the vibration of bars. Philos. Mag. 1907, 14, 35–60. [Google Scholar] [CrossRef]
- Steinmetz, C.P. Theory and Calculation of Alternating Current Phenomena; McGraw-Hill: New York, NY, USA, 1897. [Google Scholar]
- Steinmetz, C.P. Engineering Mathematics: A Series of Lectures Delivered at Union College; McGraw-Hill: New York, NY, USA, 1917. [Google Scholar]
- Li, M.; Lim, S.C.; Cattani, C.; Scalia, M. Characteristic roots of a class of fractional oscillators. Adv. High Energy Phys. 2013, 2013, 853925. [Google Scholar] [CrossRef]
- Li, M.; Lim, S.C.; Chen, S.Y. Exact solution of impulse response to a class of fractional oscillators and its stability. Math. Probl. Eng. 2011, 2011, 657839. [Google Scholar] [CrossRef]
- Lim, S.C.; Li, M.; Teo, L.P. Langevin equation with two fractional orders. Phys. Lett. A 2008, 372, 6309–6320. [Google Scholar] [CrossRef]
- Lim, S.C.; Li, M.; Teo, L.P. Locally self-similar fractional oscillator processes. Fluct. Noise Lett. 2007, 7, L169–L179. [Google Scholar] [CrossRef]
- Lim, S.C.; Teo, L.P. The fractional oscillator process with two indices. J. Phys. A Stat. Mech. Appl. 2009, 42, 065208. [Google Scholar] [CrossRef]
- West, B.J.; Geneston, E.L.; Grigolini, P. Maximizing information exchange between complex networks. Phys. Rep. 2008, 468, 1–99. [Google Scholar] [CrossRef]
- Duan, J.-S. The periodic solution of fractional oscillation equation with periodic input. Adv. Math. Phys. 2013, 2013, 869484. [Google Scholar] [CrossRef]
- Mainardi, F. Fractional relaxation-oscillation and fractional diffusion-wave phenomena. Chaos Solitons Fractals 1996, 7, 1461–1477. [Google Scholar] [CrossRef]
- Zurigat, M. Solving fractional oscillators using Laplace homotopy analysis method. Ann. Univ. Craiova Math. Comput. Sci. Ser. 2011, 38, 1–11. [Google Scholar]
- Blaszczyk, T.; Ciesielski, M. Fractional oscillator equation—Transformation into integral equation and numerical solution. Appl. Math. Comput. 2015, 257, 428–435. [Google Scholar] [CrossRef]
- Blaszczyk, T.; Ciesielski, M.; Klimek, M.; Leszczynski, J. Numerical solution of fractional oscillator equation. Appl. Math. Comput. 2011, 218, 2480–2488. [Google Scholar] [CrossRef]
- Al-rabtah, A.; Ertürk, V.S.; Momani, S. Solutions of a fractional oscillator by using differential transform method. Comput. Math. Appl. 2010, 59, 1356–1362. [Google Scholar] [CrossRef]
- Drozdov, A.D. Fractional oscillator driven by a Gaussian noise. Phys. A Stat. Mech. Appl. 2007, 376, 237–245. [Google Scholar] [CrossRef]
- Stanislavsky, A.A. Fractional oscillator. Phys. Rev. E 2004, 70, 051103. [Google Scholar] [CrossRef] [PubMed]
- Achar, B.N.N.; Hanneken, J.W.; Clarke, T. Damping characteristics of a fractional oscillator. Phys. A Stat. Mech. Appl. 2004, 339, 311–319. [Google Scholar] [CrossRef]
- Achar, B.N.N.; Hanneken, J.W.; Clarke, T. Response characteristics of a fractional oscillator. Phys. A Stat. Mech. Appl. 2002, 309, 275–288. [Google Scholar] [CrossRef]
- Achar, B.N.N.; Hanneken, J.W.; Enck, T.; Clarke, T. Dynamics of the fractional oscillator. Phys. A Stat. Mech. Appl. 2001, 297, 361–367. [Google Scholar] [CrossRef]
- Tofighi, A. The intrinsic damping of the fractional oscillator. Phys. A Stat. Mech. Appl. 2003, 329, 29–34. [Google Scholar] [CrossRef]
- Ryabov, Y.E.; Puzenko, A. Damped oscillations in view of the fractional oscillator equation. Phys. Rev. B 2002, 66, 184201. [Google Scholar] [CrossRef]
- Ahmad, W.E.; Elwakil, A.S.R. Fractional-order Wien-bridge oscillator. Electron. Lett. 2001, 37, 1110–1112. [Google Scholar] [CrossRef]
- Uchaikin, V.V. Fractional Derivatives for Physicists and Engineers; Springe: Berlin Heidelberg, 2013; Volumes II. [Google Scholar]
- Duan, J.-S.; Wang, Z.; Liu, Y.-L.; Qiu, X. Eigenvalue problems for fractional ordinary differential equations. Chaos Solitons Fractals 2013, 46, 46–53. [Google Scholar] [CrossRef]
- Lin, L.-F.; Chen, C.; Zhong, S.-C.; Wang, H.-Q. Stochastic resonance in a fractional oscillator with random mass and random frequency. J. Stat. Phys. 2015, 160, 497–511. [Google Scholar] [CrossRef]
- Duan, J.-S. A modified fractional derivative and its application to fractional vibration equation. Appl. Math. Inf. Sci. 2016, 10, 1863–1869. [Google Scholar] [CrossRef]
- Alkhaldi, H.S.; Abu-Alshaikh, I.M.; Al-Rabadi, A.N. Vibration control of fractionally-damped beam subjected to a moving vehicle and attached to fractionally-damped multi-absorbers. Adv. Math. Phys. 2013, 2013, 232160. [Google Scholar] [CrossRef]
- Dai, H.; Zheng, Z.; Wang, W. On generalized fractional vibration equation. Chaos Solitons Fractals 2017, 95, 48–51. [Google Scholar] [CrossRef]
- Ren, R.; Luo, M.; Deng, K. Stochastic resonance in a fractional oscillator driven by multiplicative quadratic noise. J. Stat. Mech. Theory Exp. 2017, 2017, 023210. [Google Scholar] [CrossRef]
- Xu, Y.; Li, Y.; Liu, D.; Jia, W.; Huang, H. Responses of Duffing oscillator with fractional damping and random phase. Nonlinear Dyn. 2013, 74, 745–753. [Google Scholar] [CrossRef]
- He, G.; Tian, Y.; Wang, Y. Stochastic resonance in a fractional oscillator with random damping strength and random spring stiffness. J. Stat. Mech. Theory Exp. 2013, 2013, P09026. [Google Scholar] [CrossRef]
- Leung, A.Y.T.; Guo, Z.; Yang, H.X. Fractional derivative and time delay damper characteristics in Duffing-van der Pol oscillators. Commun. Nonlinear Sci. Numer. Simul. 2013, 18, 2900–2915. [Google Scholar] [CrossRef]
- Chen, L.C.; Zhuang, Q.Q.; Zhu, W.Q. Response of SDOF nonlinear oscillators with lightly fractional derivative damping under real noise excitations. Eur. Phys. J. Spec. Top. 2011, 193, 81–92. [Google Scholar] [CrossRef]
- Deü, J.-F.; Matignon, D. Simulation of fractionally damped mechanical systems by means of a Newmark-diffusive scheme. Comput. Math. Appl. 2010, 59, 1745–1753. [Google Scholar] [CrossRef]
- Drăgănescu, G.E.; Bereteu, L.; Ercuţa, A.; Luca, G. Anharmonic vibrations of a nano-sized oscillator with fractional damping. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 922–926. [Google Scholar] [CrossRef]
- Rossikhin, Y.A.; Shitikova, M.V. New approach for the analysis of damped vibrations of fractional oscillators. Shock Vib. 2009, 16, 365–387. [Google Scholar] [CrossRef]
- Xie, F.; Lin, X. Asymptotic solution of the van der Pol oscillator with small fractional damping. Phys. Scr. 2009, 2009, 014033. [Google Scholar] [CrossRef]
- Gomez-Aguilar, J.F.; Rosales-Garcia, J.J.; Bernal-Alvarado, J.J.; Cordova-Fraga, T.; Guzman-Cabrera, R. Fractional mechanical oscillators. Rev. Mex. Fis. 2012, 58, 348–352. [Google Scholar]
- Liu, Q.X.; Liu, J.K.; Chen, Y.M. An analytical criterion for jump phenomena in fractional Duffing oscillators. Chaos Solitons Fractals 2017, 98, 216–219. [Google Scholar] [CrossRef]
- Leung, A.Y.T.; Yang, H.X.; Guo, Z.J. The residue harmonic balance for fractional order van der Pol like oscillators. J. Sound Vib. 2012, 331, 1115–1126. [Google Scholar] [CrossRef]
- Kavyanpoor, M.; Shokrollahi, S. Challenge on solutions of fractional Van Der Pol oscillator by using the differential transform method. Chaos Solitons Fractals 2017, 98, 44–45. [Google Scholar] [CrossRef]
- Xiao, M.; Zheng, W.X.; Cao, J. Approximate expressions of a fractional order Van der Pol oscillator by the residue harmonic balance method. Math. Comput. Simul. 2013, 89, 1–12. [Google Scholar] [CrossRef]
- Chen, L.; Wang, W.; Li, Z.; Zhu, W. Stationary response of Duffing oscillator with hardening stiffness and fractional derivative. Int. J. Non-Linear Mech. 2013, 48, 44–50. [Google Scholar] [CrossRef]
- Wen, S.-F.; Shen, Y.-J.; Yang, S.-P.; Wang, J. Dynamical response of Mathieu-Duffing oscillator with fractional-order delayed feedback. Chaos Solitons Fractals 2017, 94, 54–62. [Google Scholar] [CrossRef]
- Liao, H. Optimization analysis of Duffing oscillator with fractional derivatives. Nonlinear Dyn. 2015, 79, 1311–1328. [Google Scholar] [CrossRef]
- Abu-Gurra, S.; Ertürk, V.S.; Momani, S. Application of the modified differential transform method to fractional oscillators. Kybernetes 2011, 40, 751–761. [Google Scholar] [CrossRef]
- Ikeda, T.; Harata, Y.; Hiraoka, R. Intrinsic localized modes of 1/2-order subharmonic oscillations in nonlinear oscillator arrays. Nonlinear Dyn. 2015, 81, 1759–1777. [Google Scholar] [CrossRef]
- Department of Mathematics, Fudan University. Vibration Theory; Shanghai Scientific & Technical Publishing House: Shanghai, China, 1960. (In Chinese) [Google Scholar]
- Andronov, A.A.; Victor, A.A.; Hayijin, С.Э. Oscillation Theory; Translated from Russian by Gao, W.B., Yang, R.W., Xiao, Z.Y.; Science Press: Beijing, China, 1974. (In Chinese) [Google Scholar]
- Gabel, R.A.; Roberts, R.A. Signals and Linear Systems; John Wiley & Sons: New York, NY, USA, 1973. [Google Scholar]
- Zheng, J.L.; Ying, Q.Y.; Yang, W.L. Signals and Systems, 2nd ed.; Higher Education Press: Beijing, China, 2000; Volume 1. (In Chinese) [Google Scholar]
- Gelfand, I.M.; Vilenkin, K. Generalized Functions; Academic Press: New York, NY, USA, 1964; Volume 1. [Google Scholar]
- Griffel, D.H. Applied Functional Analysis; John Wiley & Sons: New York, NY, USA, 1981. [Google Scholar]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; John Wiley: New York, NY, USA, 1993. [Google Scholar]
- Klafter, J.; Lim, S.C.; Metzler, R. Fractional Dynamics: Recent Advances; World Scientific: Singapore, 2012. [Google Scholar]
- Lavoie, J.L.; Osler, T.J.; Tremblay, R. Fractional derivatives and special functions. SIAM Rev. 1976, 18, 240–268. [Google Scholar] [CrossRef]
- Uchaikin, V.V. Fractional Derivatives for Physicists and Engineers; Springer: Berlin Heidelberg, 2013; Volume I. [Google Scholar]
- Mathai, A.M.; Haubold, H.J. Special Functions for Applied Scientists; Springer: New York, NY, USA, 2008. [Google Scholar]
- Gorenflo, R.; Kilbas, A.A.; Mainardi, F.; Rogosin, S.V. Mittag-Leffler Functions: Related Topics and Applications, Theory and Applications; Monographs in Mathematics; Springer: Berlin Heidelberg, 2014. [Google Scholar]
- Erdelyi, A.; Magnus, W.; Oberhettinger, F.; Tricomi, F.G. Higher Transcendental Functions; McGraw Hill: New York, NY, USA, 1955; Volume I. [Google Scholar]
- Papoulis, A. The Fourier Integral and Its Applications; McGraw-Hill Inc.: New York, NY, USA, 1962. [Google Scholar]
- Chung, W.S.; Jung, M. Fractional damped oscillators and fractional forced oscillators. J. Korean Phys. Soc. 2014, 64, 186–191. [Google Scholar] [CrossRef]
- Korotkin, A.I. Added Masses of Ship Structures, Fluid Mechanics and Its Applications; Springer: Berlin Heidelberg, 2009; Volume 88. [Google Scholar]
- Jin, X.D.; Xia, L.J. Ship Hull Vibration; The Press of Shanghai Jiaotong University: Shanghai, China, 2011. (In Chinese) [Google Scholar]
- Nakagawa, K.; Ringo, M. Engineering Vibrations; Translated from Japanese by Xia, S.R.; Shanghai Science and Technology Publishing House: Shanghai, China, 1981. (In Chinese) [Google Scholar]
- Kaslik, E.; Sivasundaram, S. Non-existence of periodic solutions in fractional-order dynamical systems and a remarkable difference between integer and fractional-order derivatives of periodic functions. Nonlinear Anal. Real World Appl. 2012, 13, 1489–1497. [Google Scholar] [CrossRef]
- Gabaix, X.; Gopikrishnan, P.; Plerou, V.; Stanley, H.E. A theory of power-law distributions in financial market fluctuations. Nature 2003, 423, 267–270. [Google Scholar] [CrossRef] [PubMed]
- Stanley, H.E. Phase transitions: Power laws and universality. Nature 1995, 378, 554. [Google Scholar] [CrossRef]
- Levy, C.; Pinchas, M. Maximum likelihood estimation of clock skew in IEEE 1588 with fractional Gaussian noise. Math. Probl. Eng. 2015, 2015, 174289. [Google Scholar] [CrossRef]
- Pinchas, M. Symbol error rate for non-blind adaptive equalizers applicable for the SIMO and fGn case. Math. Probl. Eng. 2014, 2014, 606843. [Google Scholar] [CrossRef]
- Toma, C. Wavelets-computational aspects of Sterian-realistic approach to uncertainty principle in high energy physics: A transient approach. Adv. High Energy Phys. 2013, 2013, 735452. [Google Scholar] [CrossRef]
- Bakhoum, E.G.; Toma, C. Transient aspects of wave propagation connected with spatial coherence. Math. Probl. Eng. 2013, 2013, 691257. [Google Scholar] [CrossRef]
- Cattani, C.; Ciancio, A.; Laserra, E.; Bochicchio, I. On the fractal distribution of primes and prime-indexed primes by the binary image analysis. Phys. A Stat. Mech. Appl. 2016, 460, 222–229. [Google Scholar] [CrossRef]
- Mardani, A.; Heydari, M.H.; Hooshmandasl, M.R.; Cattani, C. A meshless method for solving time fractional advection-diffusion equation with variable coefficients. Comput. Math. Appl. 2017, in press. [Google Scholar] [CrossRef]










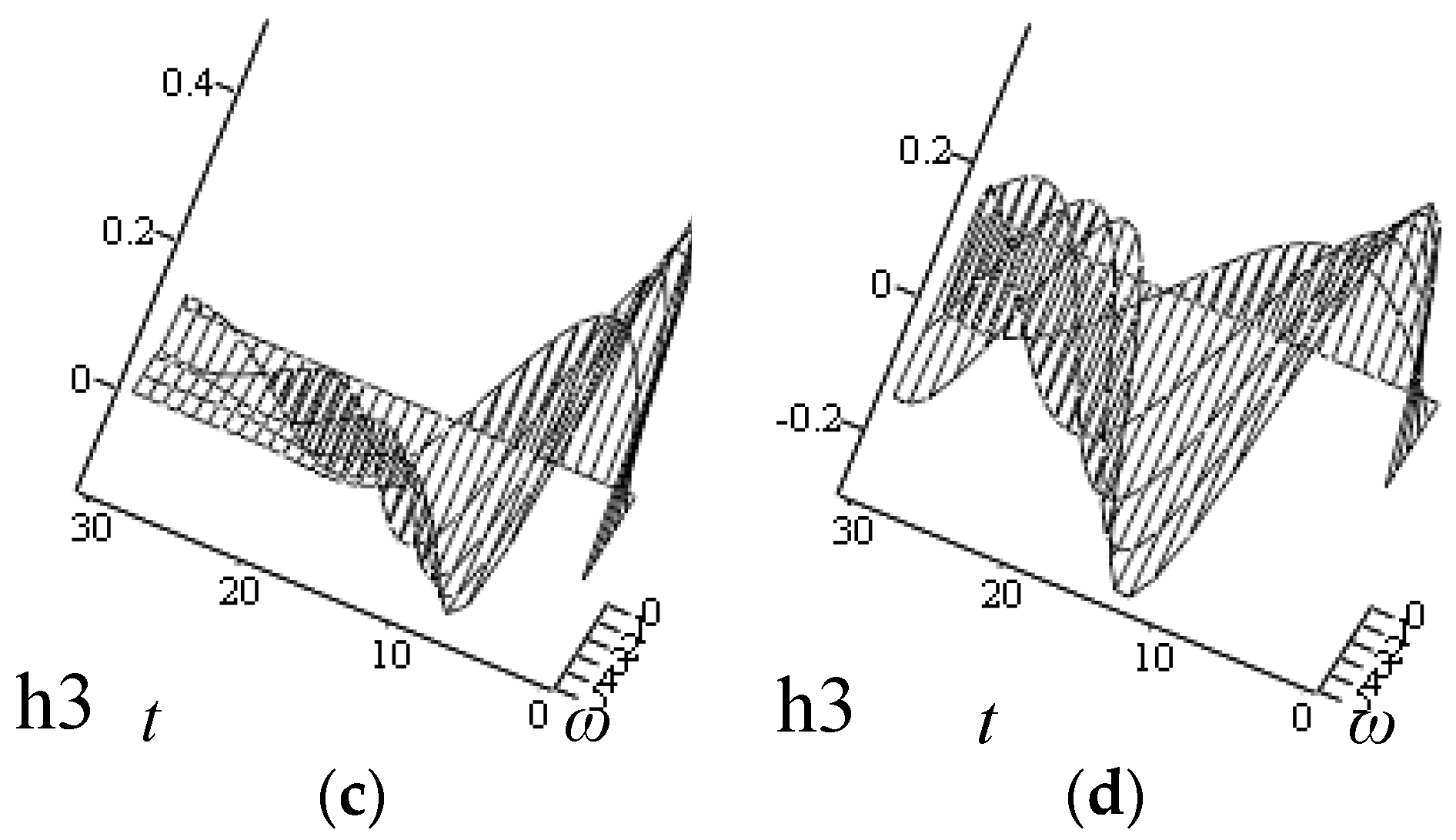
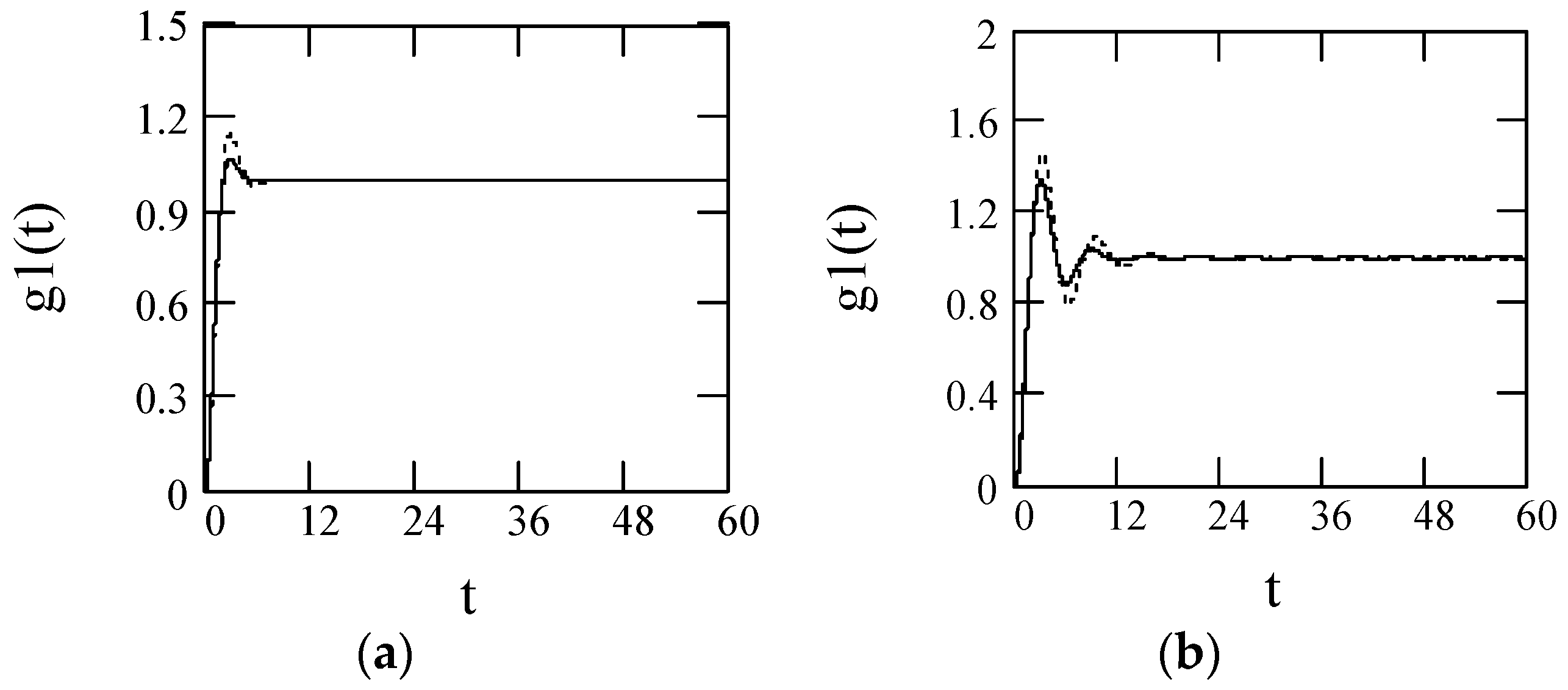
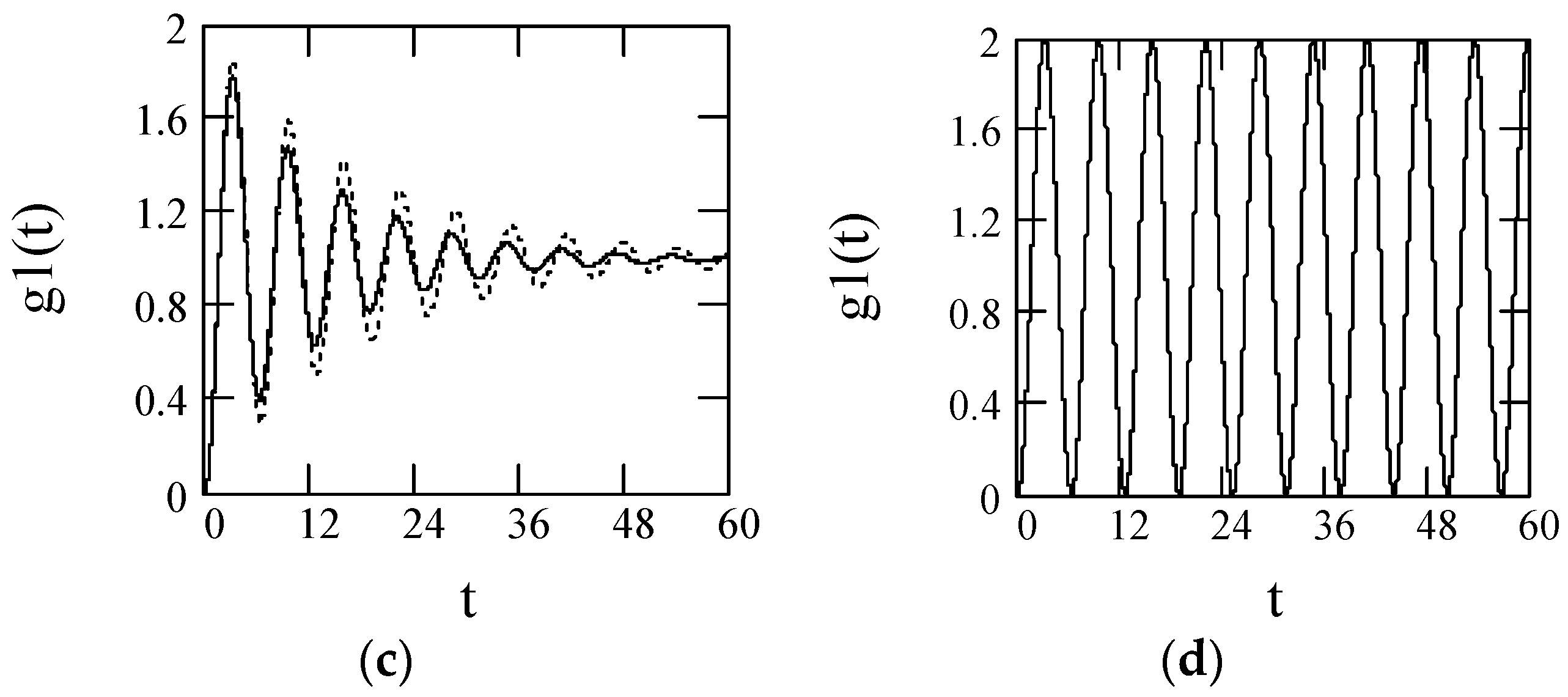
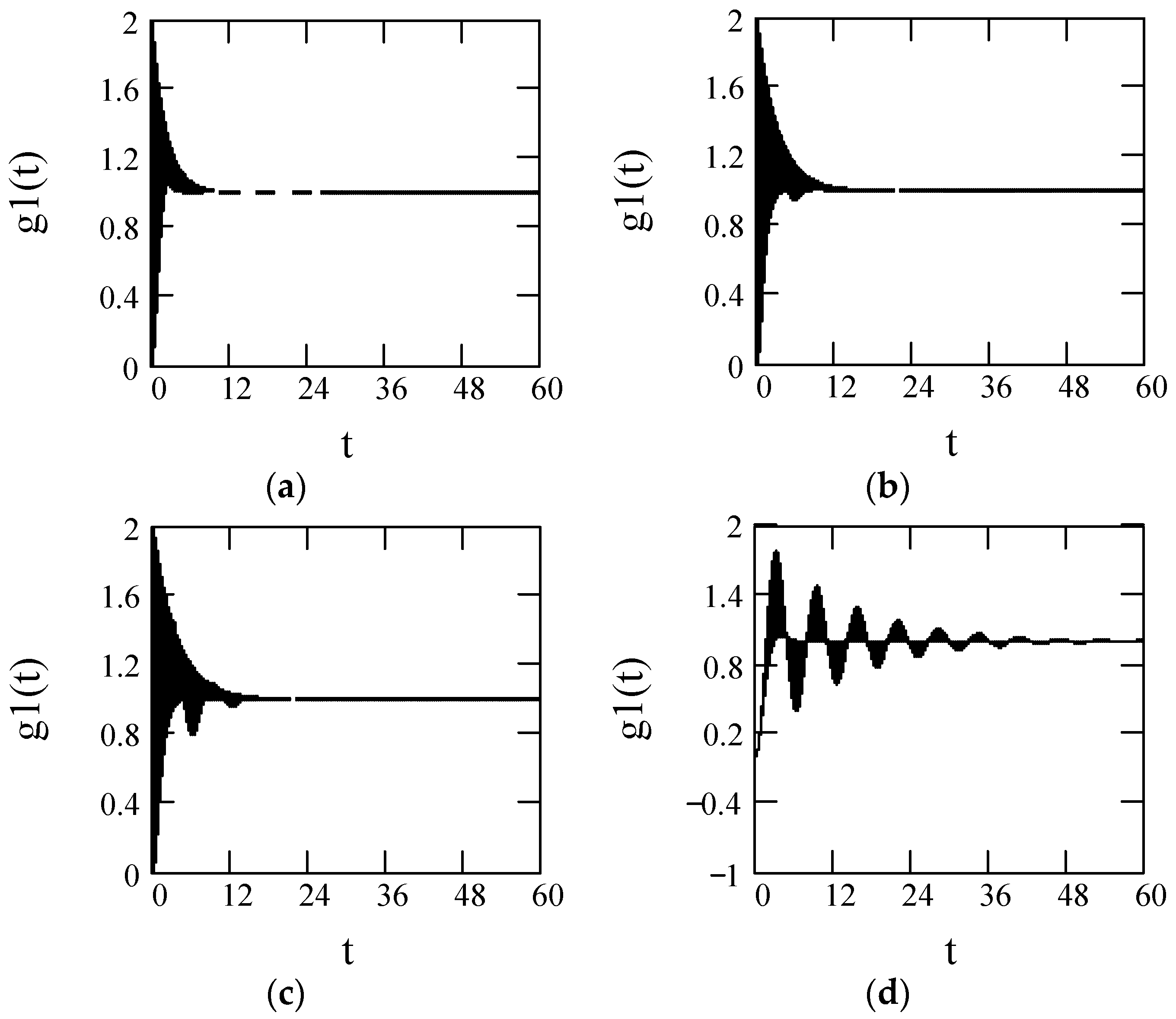

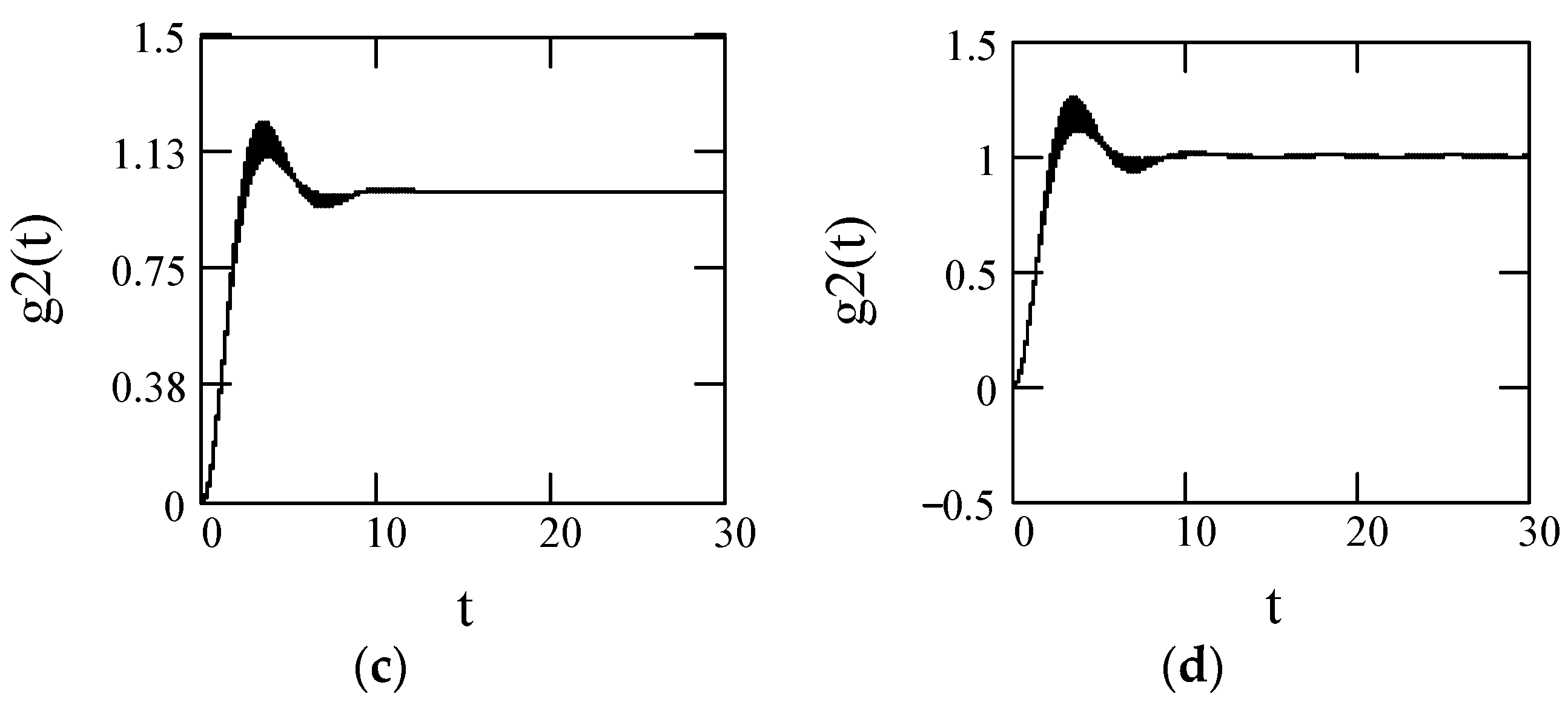




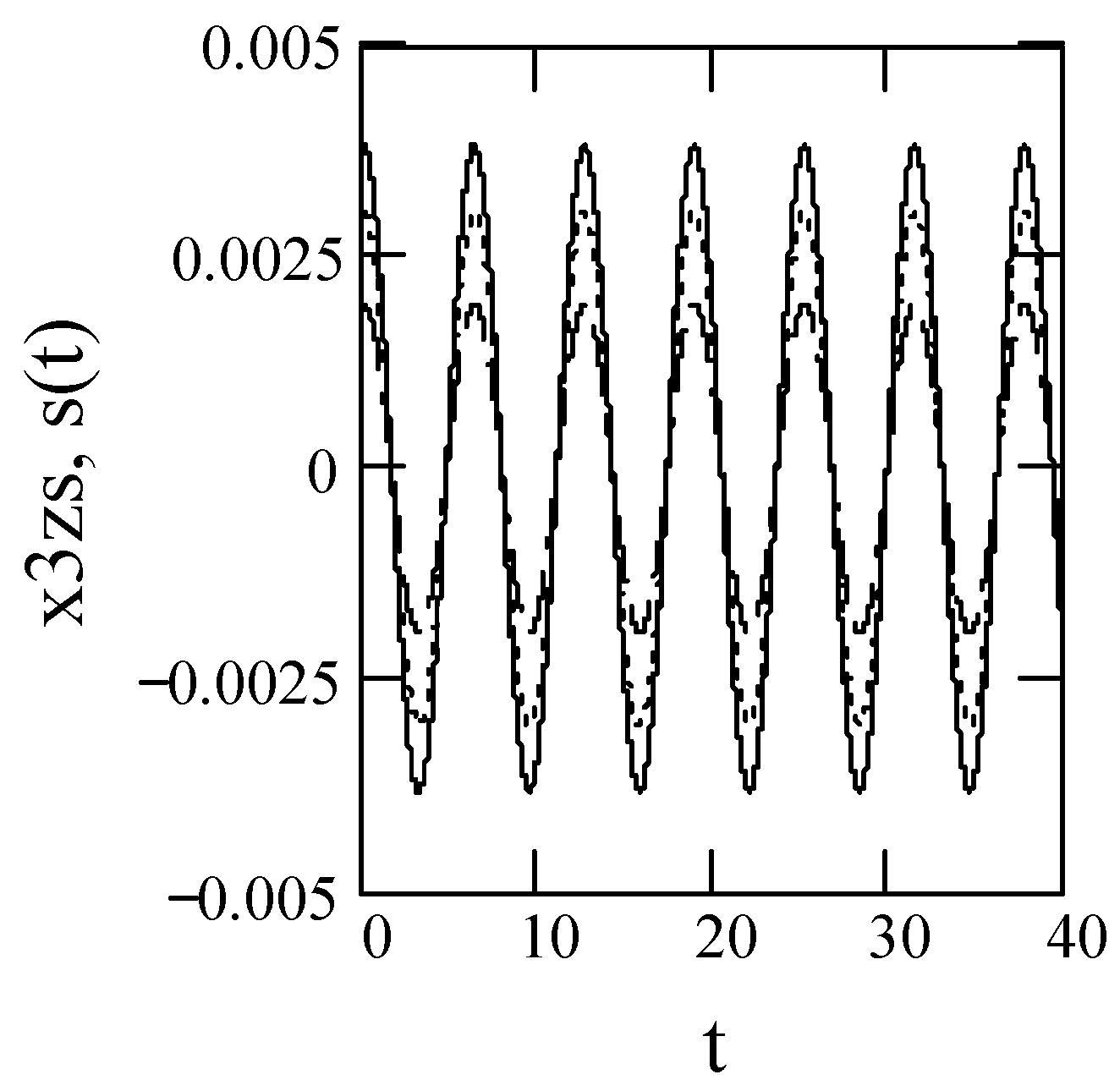
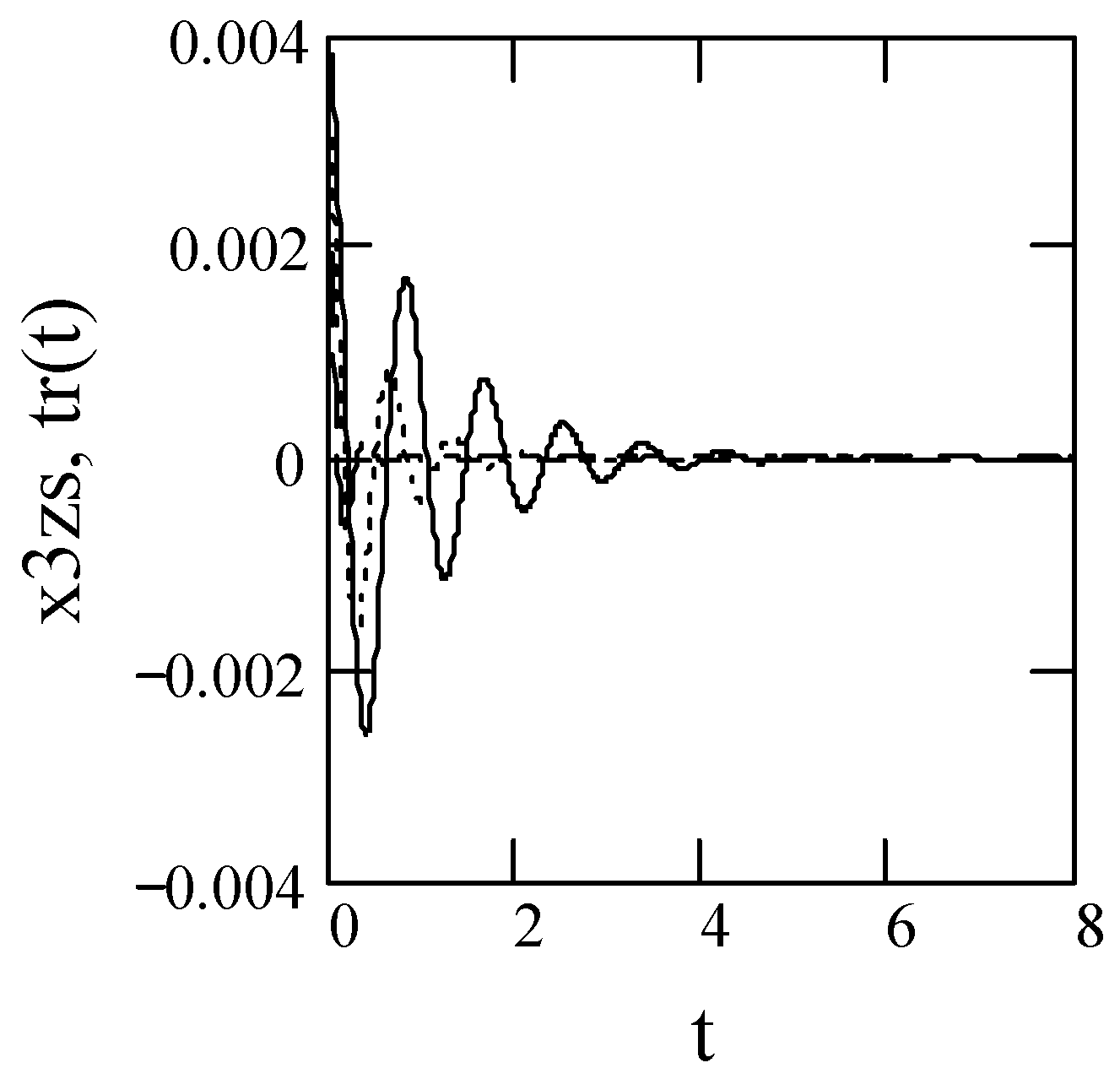
© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, M. Three Classes of Fractional Oscillators. Symmetry 2018, 10, 40. https://doi.org/10.3390/sym10020040
Li M. Three Classes of Fractional Oscillators. Symmetry. 2018; 10(2):40. https://doi.org/10.3390/sym10020040
Chicago/Turabian StyleLi, Ming. 2018. "Three Classes of Fractional Oscillators" Symmetry 10, no. 2: 40. https://doi.org/10.3390/sym10020040
APA StyleLi, M. (2018). Three Classes of Fractional Oscillators. Symmetry, 10(2), 40. https://doi.org/10.3390/sym10020040




