Dispersion Interactions between Neutral Atoms and the Quantum Electrodynamical Vacuum
Abstract
1. Introduction
2. Atom-Field Interaction Hamiltonian: Minimal and Multipolar Coupling
3. Effective Hamiltonians
4. Vacuum Fluctuations
5. The Van Der Waals and Casimir–Polder Dispersion Interaction between Two Neutral Ground-State Atoms
6. The Three-Body Casimir–Polder Interaction
7. Two- and Three-Body Dispersion Interactions as a Consequence of Vacuum Field Fluctuations
7.1. Dressed Field Energy Densities
7.2. Vacuum Field Correlations
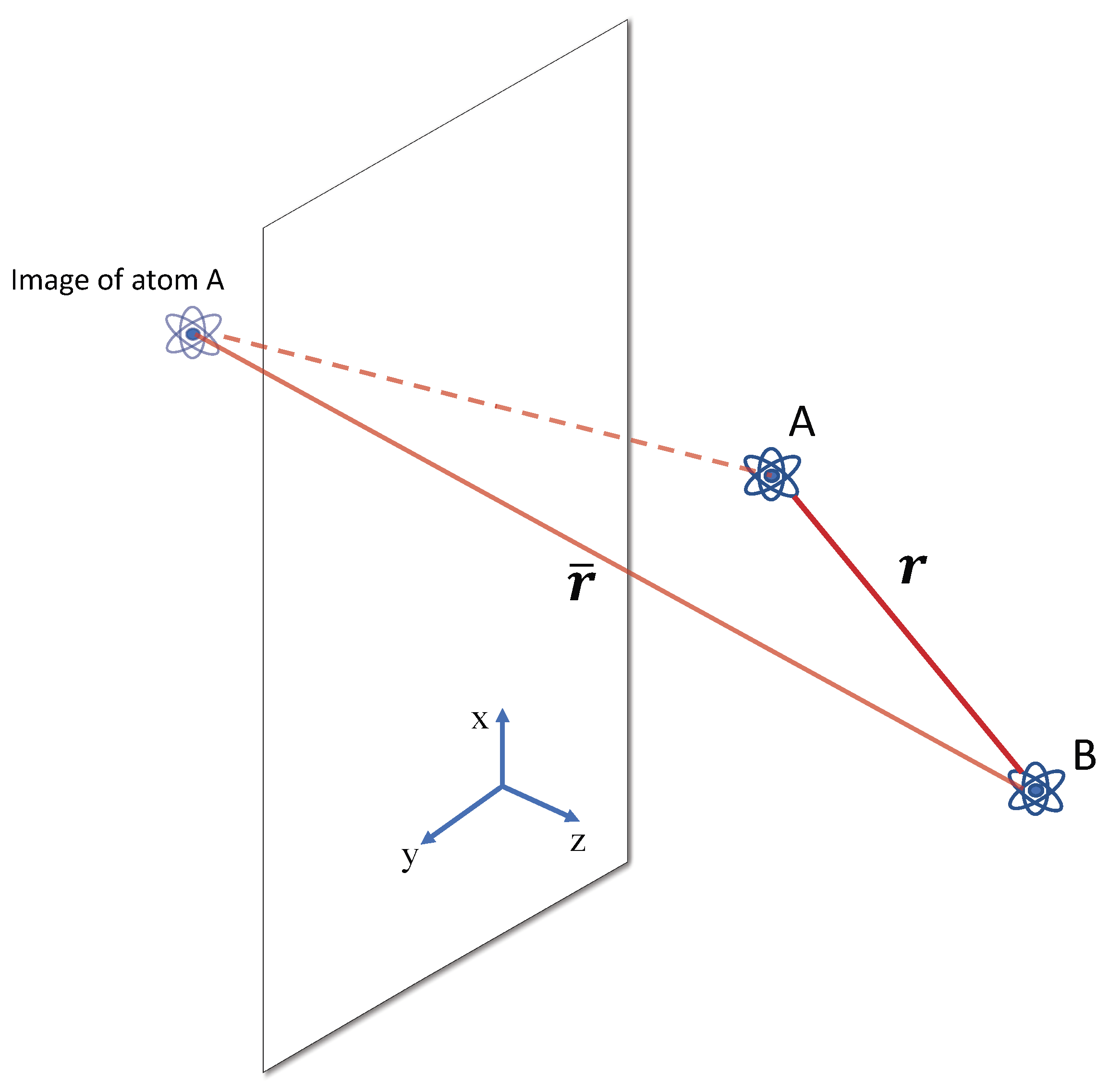
8. Casimir–Polder Forces between Atoms Nearby Macroscopic Boundaries
9. Casimir–Polder and Resonance Interactions between Uniformly Accelerated Atoms
10. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Casimir, H.B.G.; Polder, D. The Influence of Retardation on the London–van der Waals Forces. Phys. Rev. 1948, 73, 360. [Google Scholar] [CrossRef]
- Milonni, P.W. The Quantum Vacuum. An Introduction to Quantum Electrodynamics; Academic Press: San Diego, CA, USA, 1994. [Google Scholar]
- Power, E.A. Casimir–Polder potential from first principles. Eur. J. Phys. 2001, 22, 453. [Google Scholar] [CrossRef]
- Barnett, S.M.; Aspect, A.; Milonni, P.W. On the quantum nature of the Casimir–Polder interaction. J. Phys. B 2000, 33, L143. [Google Scholar] [CrossRef]
- Axilrod, B.M.; Teller, E. Interaction of the van der Waals type between three atoms. J. Chem. Phys. 1943, 11, 299. [Google Scholar] [CrossRef]
- Aub, M.R.; Zienau, S. Studies on the retarded interaction between neutral atoms I. Three-body London–van der Waals interaction of neutral atoms. Proc. R. Soc. A 1960, 257, 464. [Google Scholar] [CrossRef]
- Milton, K.A.; Abalo, E.; Parashar, P.; Shajesh, K.V. Three-body Casimir–Polder interactions. Nuovo Cimento C 2013, 36, 183. [Google Scholar] [CrossRef]
- Brevik, M.; Marachevsky, V.N.; Milton, K.A. Identity of the van der Waals Force and the Casimir Effect and the Irrelevance of These Phenomena to Sonoluminescence. Phys. Rev. Lett. 1999, 82, 3948. [Google Scholar] [CrossRef]
- Barcellona, P.; Passante, R. A microscopic approach to Casimir and Casimir–Polder forces between metallic bodies. Ann. Phys. 2015, 355, 282. [Google Scholar] [CrossRef]
- Buhmann, S.Y.; Welsch, D.-K. Dispersion forces in macroscopic quantum electrodynamics. Prog. Quantum Electron. 2007, 31, 51. [Google Scholar] [CrossRef]
- Scheel, S.; Buhmann, S.Y. Macroscopic quantum electrodynamics—Concepts and applications. Acta Phys. Slov. 2008, 58, 675. [Google Scholar] [CrossRef]
- Compagno, G.; Passante, R.; Persico, F. Atom-Field Interactions and Dressed Atoms; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Power, E.A. Introductory Quantum Electrodynamics; Longmans: London, UK, 1964. [Google Scholar]
- Power, E.A.; Thirunamachandran, T. On the nature of the Hamiltonian for the interaction of radiation with atoms and molecules: (e/mc)p·A, −μ·E, and all that. Am. J. Phys. 1978, 46, 370. [Google Scholar] [CrossRef]
- Craig, D.P.; Thirunamachandran, T. Molecular Quantum Electrodynamics; Dover Publ.: Mineola, NY, USA, 1998. [Google Scholar]
- London, F. Zur Theorie und Systematik der Molekularkräfte. Z. Phys. 1930, 63, 245. [Google Scholar] [CrossRef]
- Power, E.A.; Zienau, S. On the radiative contribution to the van der Waals Force. Nuovo Cim. 1957, 6, 7. [Google Scholar] [CrossRef]
- Power, E.A.; Zienau, S. Coulomb gauge in non-relativistic quantum electrodynamics and the shape of spectral lines. Philos. Trans. R. Soc. A 1959, 251, 427. [Google Scholar] [CrossRef]
- Woolley, R.G. Molecular quantum electrodynamics. Proc. R. Soc. Lond. A 1971, 321, 557. [Google Scholar] [CrossRef]
- Cohen-Tannoudji, C.; Dupont-Roc, J.; Grynberg, G. Photons and Atoms: Introduction to Quantum Electrodynamics; Wiley: New York, NY, USA, 1989. [Google Scholar]
- Atkins, P.W.; Woolley, R.G. The interaction of molecular multipoles with the electromagnetuc field in the canonical formulation of non-covariant quantum electrodynamics. Proc. R. Soc. Lond. A 1970, 319, 549. [Google Scholar] [CrossRef]
- Babiker, M.; Loudon, R. Derivation of the Power–Zienau–Woolley Hamiltonian in quantum electrodynamics by gauge transformation. Proc. R. Soc. Lond. A 1983, 385, 439. [Google Scholar] [CrossRef]
- Andrews, D.L.; Jones, G.A.; Salam, A.; Woolley, R.G. Perspective: Quantum Hamiltonians for optical interactions. J. Chem. Phys. 2018, 148, 040901. [Google Scholar] [CrossRef]
- Salam, A. Molecular Quantum Electrodynamics: Long-Range Intermolecular Interactions; Wiley: Hoboken, NJ, USA, 2010. [Google Scholar]
- Bykov, V.P. Radiation of Atoms in a Resonant Environment; World Scientific: Singapore, 1993. [Google Scholar]
- Biswas, A.K.; Compagno, G.; Palma, G.M.; Passante, R.; Persico, F. Virtual photons and causality in the dynamics of a pair of two-level atoms. Phys. Rev. A 1990, 42, 4291. [Google Scholar] [CrossRef]
- Compagno, G.; Palma, G.M.; Passante, R.; Persico, F. Relativistic causality and quantum-mechanical states in the Fermi problem. Chem. Phys. 1995, 198, 19. [Google Scholar] [CrossRef]
- Buhmann, S.Y. Dispersion Forces I: Macroscopic Quantum Electrodynamics and Ground-State Casimir, Casimir–Polder and van der Waals forces; Springer: Berlin, Germany, 2012. [Google Scholar]
- Buhmann, S.Y. Dispersion Forces II: Many-Body Effects, Excited Atoms, Finite Temperature and Quantum Friction; Springer: Berlin, Germany, 2012. [Google Scholar]
- Salam, A. Molecular quantum electrodynamics in the Heisenberg picture: A field theoretic viewpoint. Int. Rev. Phys. Chem. 2008, 27, 405. [Google Scholar] [CrossRef]
- Salam, A. Non-Relativistic QED Theory of the Van Der Waals Dispersion Interaction; Springer: Cham, Switzerland, 2016. [Google Scholar]
- Passante, R.; Power, E.A.; Thirunamachandran, T. Radiation-molecule coupling using dynamic polarizabilities: Application to many-body forces. Phys. Lett. A 1998, 249, 77. [Google Scholar] [CrossRef]
- Passante, R.; Power, E.A. The Lamb shift in non-relativistic quantum electrodynamics. Phys. Lett. A 1987, 122, 14. [Google Scholar] [CrossRef]
- Craig, D.P.; Power, E.A. The asymptotic Casimir–Polder potential from second-order perturbation theory and its generalization for anisotropic polarizabilities. Int. J. Quantum Chem. 1969, 3, 903. [Google Scholar] [CrossRef]
- Casimir, H.B.G. On the attraction between two perfectly conducting plates. Proc. Kon. Ned. Akad. Wet. 1948, 51, 793. [Google Scholar]
- Power, E.A.; Thirunamachandran, T. Casimir–Polder potential as an interaction between induced dipoles. Phys. Rev. A 1993, 48, 4761. [Google Scholar] [CrossRef]
- Power, E.A.; Thirunamachandran, T. Quantum electrodynamics in a cavity. Phys. Rev. A 1982, 25, 2473. [Google Scholar] [CrossRef]
- Ford, L.H.; Svaiter, N.F. Vacuum energy density near fluctuating boundaries. Phys. Rev. D 1998, 58, 065007. [Google Scholar] [CrossRef]
- Bartolo, N.; Passante, R. Electromagnetic-field fluctuations near a dielectric-vacuum boundary and surface divergences in the ideal conductor limit. Phys. Rev. A 2012, 86, 012122. [Google Scholar] [CrossRef]
- Bartolo, N.; Butera, S.; Lattuca, M.; Passante, R.; Rizzuto, L.; Spagnolo, S. Vacuum Casimir energy densities and field divergences at boundaries. J. Phys. Condens. Matter 2015, 27, 214015. [Google Scholar] [CrossRef]
- Butera, S.; Passante, R. Field Fluctuations in a One-Dimensional Cavity with a Mobile Wall. Phys. Rev. Lett. 2013, 111, 060403. [Google Scholar] [CrossRef] [PubMed]
- Armata, F.; Passante, R. Vacuum energy densities of a field in a cavity with a mobile boundary. Phys. Rev. D 2015, 91, 025012. [Google Scholar] [CrossRef]
- Armata, F.; Butera, S.; Fiscelli, G.; Incardone, R.; Notararigo, V.; Palacino, R.; Passante, R.; Rizzuto, L.; Spagnolo, S. Effect of boundaries on vacuum field fluctuations and radiation-mediated interactions between atoms. J. Phys. Conf. Ser. 2017, 880, 012064. [Google Scholar] [CrossRef]
- Shahmoon, E. Van der Waals and Casimir–Polder dispersion forces. In Forces of the Quantum Vacuum. An Introduction to Casimir Physics; Simpson, W.M.R., Leonhardt, U., Eds.; World Scientific Publ. Co.: Singapore, 2015; p. 61. [Google Scholar]
- Margenau, H. Van der Waals forces. Rev. Mod. Phys. 1939, 11, 1. [Google Scholar] [CrossRef]
- Babb, J.F. Casimir effects in atomic, molecular, and optical physics. In Advances in Atomic, Molecular, and Optical Physics; Arimondo, E., Berman, P.R., Lin, C.C., Eds.; Elsevier Inc.: London, UK, 2010; Volume 59, p. 1. [Google Scholar]
- Spagnolo, S.; Passante, R.; Rizzuto, L. Field fluctuations near a conducting plate and Casimir–Polder forces in the presence of boundary conditions. Phys. Rev. A 2006, 73, 062117. [Google Scholar] [CrossRef]
- Verwey, E.J.W.; Overbeek, J.T. Theory of the Stability of Lyophobic Colloids; Dover Publ.: Mineola, NY, USA, 1999. [Google Scholar]
- Przybytek, M.; Jeziorski, B.; Cencek, W.; Komasa, J.; Mehl, J.B.; Szalewicz, K. Onset of Casimir–Polder Retardation in a Long-Range Molecular Quantum State. Phys. Rev. Lett. 2012, 108, 183201. [Google Scholar] [CrossRef] [PubMed]
- Béguin, L.; Vernier, A.; Chicireanu, R.; Lahaye, T.; Browaeys, A. Direct measurement of the van der Waals Interaction between Two Rydberg Atoms. Phys. Rev. Lett. 2013, 110, 263201. [Google Scholar] [CrossRef]
- McLachlan, A.D. Retarded dispersion forces in dielectrics at finite temperatures. Proc. R. Soc. Lond. A 1963, 274, 80. [Google Scholar] [CrossRef]
- Boyer, T.H. Temperature dependence of Van der Waals forces in classical electrodynamics with classical electromagnetic zero-point radiation. Phys. Rev. A 1975, 11, 1650. [Google Scholar] [CrossRef]
- Goedecke, G.H.; Wood, R.C. Casimir–Polder interaction at finite temperature. Phys. Rev. A 1999, 11, 2577. [Google Scholar] [CrossRef]
- Barton, G. Long-range Casimir–Polder-Feinberg-Sucher intermolecular potential at nonzero temperature. Phys. Rev. A 2001, 64, 032102. [Google Scholar] [CrossRef]
- Power, E.A.; Thirunamachandran, T. Quantum electrodynamics with nonrelativistic sources. V. Electromagnetic field correlations and intermolecular interactions between molecules in either ground or excited states. Phys. Rev. A 1993, 47, 2593. [Google Scholar] [CrossRef]
- Rizzuto, L.; Passante, R.; Persico, F. Dynamical Casimir–Polder energy between an excited- and a ground-state atom. Phys. Rev. A 2004, 70, 012107. [Google Scholar] [CrossRef]
- Berman, P.R. Interaction energy of nonidentical atoms. Phys. Rev. A 2015, 91, 042127. [Google Scholar] [CrossRef]
- Donaire, M.; Guérout, R.; Lambrecht, A. Quasiresonant van der Waals Interaction between nonidentical atoms. Phys. Rev. Lett. 2015, 115, 033201. [Google Scholar] [CrossRef]
- Milonni, P.W.; Rafsanjani, S.M.H. Distance dependence of two-atom dipole interactions with one atom in an excited state. Phys. Rev. A 2015, 92, 062711. [Google Scholar] [CrossRef]
- Barcellona, P.; Passante, R.; Rizzuto, L.; Buhmann, S.Y. Van der Waals interactions between excited atoms in generic environments. Phys. Rev. A 2016, 94, 012705. [Google Scholar] [CrossRef]
- Power, E.A.; Thirunamachandran, T. Dispersion interactions between atoms involving electric quadrupole polarizabilities. Phys. Rev. A 1996, 53, 1567. [Google Scholar] [CrossRef]
- Salam, A.; Thirunamachandran, T. A new generalization of the Casimir–Polder potential to higher electric multipole polarizabilities. J. Chem. Phys. 1996, 104, 5094. [Google Scholar] [CrossRef]
- Salam, A. A general formula obtained from induced moments for the retarded van derWaals dispersion energy shift between two molecule with arbitrary electric multipole polarizabilities: I. Ground state interactions. J. Phys. B 2006, 39, S651. [Google Scholar] [CrossRef]
- Jenkins, J.K.; Salam, A.; Thirunamachandran, T. Retarded dispersion interaction energies between chiral molecules. Phys. Rev. A 1994, 50, 4767. [Google Scholar] [CrossRef] [PubMed]
- Salam, A. On the effect of a radiation field in modifying the intermolecular interaction between two chiral molecules. J. Chem. Phys. 2006, 124, 014302. [Google Scholar] [CrossRef] [PubMed]
- Barcellona, P.; Passante, R.; Rizzuto, L.; Buhmann, S.Y. Dynamical Casimir–Polder interaction between a chiral molecule and a surface. Phys. Rev. A 2016, 93, 032508. [Google Scholar] [CrossRef]
- Power, E.A.; Thirunamachandran, T. The non-additive dispersion energies for N molecules: A quantum electrodynamical theory. Proc. R. Soc. Lond. A 1985, 401, 167. [Google Scholar] [CrossRef]
- Salam, A. Higher-order electric multipole contributions to retarded non-additive three-body dispersion interaction energies between atoms: Equilateral triangle and collinear configurations. J. Chem. Phys. 2013, 139, 244105. [Google Scholar] [CrossRef] [PubMed]
- Salam, A. Dispersion potential between three-bodies with arbitrary electric multipole polarizabilities: Molecular QED theory. J. Chem. Phys. 2014, 140, 044111. [Google Scholar] [CrossRef]
- Buhmann, S.Y.; Salam, A. Three-Body Dispersion Potentials Involving Electric Octupole Coupling. Symmetry 2018, 10, 343. [Google Scholar] [CrossRef]
- Milonni, P.W. Casimir forces without the vacuum radiation field. Phys. Rev. A 1982, 25, 1315. [Google Scholar] [CrossRef]
- Milonni, P.W. Different ways of looking at the electromagnetic vacuum. Phys. Scr. 1988, T21, 102. [Google Scholar] [CrossRef]
- Power, E.A. Zero-point energy and the Lamb shift. Am. J. Phys. 1966, 34, 516. [Google Scholar] [CrossRef]
- Compagno, G.; Passante, R.; Persico, F. The role of the cloud of virtual photons in the shift of the ground state energy of a hydrogen atom. Phys. Lett. A 1983, 98, 253. [Google Scholar] [CrossRef]
- Passante, R.; Compagno, G.; Persico, F. Cloud of virtual photons in the ground state of the hydrogen atom. Phys. Rev. A 1985, 31, 2827. [Google Scholar] [CrossRef]
- Passante, R.; Rizzuto, L.; Spagnolo, S. Vacuum local and global electromagnetic self-energies for a point-like and an extended field source. Eur. Phys. J. C 2013, 73, 2419. [Google Scholar] [CrossRef]
- NIST Handbook of Mathematical Functions; Olver, F.W.J., Lozier, D.W., Boisvert, R.F., Clark, C.W., Eds.; NIST and Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Passante, R.; Power, E.A. Electromagnetic-energy-density distribution around a ground-state hydrogen atom and connection with van der Waals forces. Phys. Rev. A 1987, 35, 188. [Google Scholar] [CrossRef]
- Compagno, G.; Palma, G.M.; Passante, R.; Persico, F. Atoms dressed and partially dressed by the zero-point fluctuations of the electromagnetic field. J. Phys. B 1995, 28, 1105. [Google Scholar] [CrossRef]
- Feinberg, G.; Sucher, J. General theory of the van der Waals Interaction: A model-independent Approach. Phys. Rev. A 1970, 2, 2395. [Google Scholar] [CrossRef]
- Passante, R.; Persico, F. Virtual photons and three-body forces. J. Phys. B 1999, 32, 19. [Google Scholar] [CrossRef]
- Compagno, G.; Persico, F.; Passante, R. Interference in the virtual photon clouds of two hydrogen atoms. Phys. Lett. A 1985, 112, 215. [Google Scholar] [CrossRef]
- Hinds, E.A.; Sandoghdar, V. Cavity QED level shifts of simple atoms. Phys. Rev. A 1991, 43, 398. [Google Scholar] [CrossRef] [PubMed]
- Messina, R.; Passante, R.; Rizzuto, L.; Spagnolo, S.; Vasile, R. Casimir–Polder forces, boundary conditions and fluctuations. J. Phys. A 2008, 41, 164031. [Google Scholar] [CrossRef]
- Barton, G. Frequency shifts near an interface: Inadequacy of two-level atomic models. J. Phys. B 1974, 29, 1871. [Google Scholar] [CrossRef]
- Passante, R.; Rizzuto, R.; Spagnolo, S.; Petrosky, T.Y.; Tanaka, S. Harmonic oscillator model for the atom–surface Casimir–Polder interaction energy. Phys. Rev. A 2012, 85, 062109. [Google Scholar] [CrossRef]
- Ciccarello, F.; Karpov, E.; Passante, R. Exactly solvable model of two three-dimensional harmonic oscillators interacting with the quantum electromagnetic field: The far-zone Casimir–Polder potential. Phys. Rev. A 2005, 72, 052106. [Google Scholar] [CrossRef]
- Born, M.; Wolf, E. Principles of Optics; Pergamon Press: Oxford, UK, 1980. [Google Scholar]
- McLone, R.R.; Power, E.A. On the Interaction between two identical neutral dipole systems, one in an excited state and the other in the ground state. Mathematika 1964, 11, 91. [Google Scholar] [CrossRef]
- Passante, R.; Persico, F.; Rizzuto, L. Spatial correlations of vacuum fluctuations and the Casimir–Polder potential. Phys. Lett. A 2003, 316, 29. [Google Scholar] [CrossRef]
- Cirone, M.; Passante, R. Vacuum field correlations and the three-body Casimir–Polder potential. J. Phys. B 1996, 29, 1871. [Google Scholar] [CrossRef]
- Cirone, M.; Passante, R. Dressed zero-point field correlations and the non-additive three-body van der Waals potential. J. Phys. B 1997, 30, 5579. [Google Scholar] [CrossRef]
- Cirone, M.A.; Mostowski, J.; Passante, R.; Rza̧żewsi, K. The concept of vacuum in nonrelativistic QED. Recent. Res. Devel. Physics 2001, 2, 131. [Google Scholar]
- Passante, R.; Persico, F.; Rizzuto, L. Vacuum field correlations and three-body Casimir–Polder potential with one excited atom. J. Mod. Opt. 2005, 52, 1957. [Google Scholar] [CrossRef]
- Power, E.A.; Thirunamachandran, T. Dispersion forces between molecules with one or both molecules excited. Phys. Rev. A 1995, 51, 3660. [Google Scholar] [CrossRef]
- Power, E.A.; Thirunamachandran, T. Two- and three-body dispersion forces with one excited molecule. Chem. Phys. 1995, 198, 5. [Google Scholar] [CrossRef]
- Passante, R.; Persico, F.; Rizzuto, L. Causality, non-locality and three-body Casimir–Polder energy between three ground-state atoms. J. Phys. B 2006, 39, S685. [Google Scholar] [CrossRef]
- Passante, R.; Persico, F.; Rizzuto, L. Nonlocal field correlations and dynamical Casimir–Polder forces between one excited- and two ground-state atoms. J. Phys. B 2007, 40, 1863. [Google Scholar] [CrossRef]
- Rizzuto, L.; Passante, R.; Persico, F. Nonlocal Properties of Dynamical Three-Body Casimir–Polder Forces. Phys. Rev. Lett. 2007, 98, 240404. [Google Scholar] [CrossRef]
- Vasile, R.; Passante, R. Dynamical Casimir–Polder force between an atom and a conducting wall. Phys. Rev. A 2008, 78, 032108. [Google Scholar] [CrossRef]
- Shresta, S.; Hu, B.L.; Phillips, N.G. Moving atom-field interaction: Correction to the Casimir–Polder effect from coherent backaction. Phys. Rev. A 2003, 68, 062101. [Google Scholar] [CrossRef]
- Hu, B.L.; Roura, A.; Shresta, S. Vacuum fluctuations and moving atoms/detectors: From the Casimir–Polder to the Unruh–Davies–DeWitt–Fulling effect. J. Opt. B Quantum Semiclass. Opt. 2004, 6, S698. [Google Scholar] [CrossRef]
- Messina, M.; Vasile, R.; Passante, R. Dynamical Casimir–Polder force on a partially dressed atom near a conducting wall. Phys. Rev. A 2010, 82, 062501. [Google Scholar] [CrossRef]
- Messina, R.; Passante, R.; Rizzuto, L.; Spagnolo, S.; Vasile, R. Dynamical Casimir–Polder potentials in non-adiabatic conditions. Phys. Scr. 2014, T160, 014032. [Google Scholar] [CrossRef]
- Haakh, H.R.; Henkel, C.; Spagnolo, S.; Rizzuto, L.; Passante, R. Dynamical Casimir–Polder interaction between an atom and surface plasmons. Phys. Rev. A 2014, 89, 022509. [Google Scholar] [CrossRef]
- Armata, F.; Vasile, R.; Barcellona, P.; Buhmann, S.Y.; Rizzuto, L.; Passante, R. Dynamical Casimir–Polder force between an excited atom and a conducting wall. Phys. Rev. A 2016, 94, 042511. [Google Scholar] [CrossRef]
- Haakh, H.R.; Scheel, S. Modified and controllable dispersion interaction in a one-dimensional waveguide geometry. Phys. Rev. A 2015, 91, 052707. [Google Scholar] [CrossRef]
- Dung, H.T. Interatomic dispersion potential in a cylindrical system: Atoms being off axis. J. Phys. B 2016, 49, 165502. [Google Scholar] [CrossRef]
- Weeraddana, D.; Premaratne, M.; Gunapala, S.D.; Andrews, D.L. Controlling resonance energy transfer in nanostructure emitters by positioning near a mirror. J. Chem. Phys. 2017, 147, 074117. [Google Scholar] [CrossRef] [PubMed]
- Fiscelli, G.; Rizzuto, L.; Passante, R. Resonance energy transfer between two atoms in a conducting cylindrical waveguide. Phys. Rev. A 2018, 98, 013849. [Google Scholar] [CrossRef]
- Passante, R.; Spagnolo, S. Casimir–Polder interatomic potential between two atoms at finite temperature and in the presence of boundary conditions. Phys. Rev. A 2007, 76, 042112. [Google Scholar] [CrossRef]
- Incardone, R.; Fukuta, T.; Tanaka, S.; Petrosky, T.; Rizzuto, L.; Passante, R. Enhanced resonant force between two entangled identical atoms in a photonic crystal. Phys. Rev. A 2014, 89, 062117. [Google Scholar] [CrossRef]
- Notararigo, V.; Passante, R.; Rizzuto, L. Resonance interaction energy between two entangled atoms in a photonic bandgap environment. Sci. Rep. 2018, 8, 5193. [Google Scholar] [CrossRef]
- Bordag, M.; Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. Advances in Casimir Physics; Oxford Science Publications: Oxford, UK, 2009. [Google Scholar]
- Kittel, C. Introduction to Solid State Physics; John Wiley & Sons: Hoboken, NJ, USA, 2004. [Google Scholar]
- Lifshits, E.M. The theory of molecular attractive fiorces between solids. Sov. Phys. JETP 1956, 2, 73. [Google Scholar]
- Lifshits, E.M.; Pitaevskii. Landau and Lifshits Course of Theoretical Physics, Vol. 9: Statistical Physics, Part 2; Pergamon Press: Oxford, UK, 1980. [Google Scholar]
- Babiker, M.; Barton, G. Quantum frequency shifts near a plasma surface. J. Phys. A 1976, 9, 129. [Google Scholar] [CrossRef]
- McLachlan, A.D. Van der Waals forces between an atom and a surface. Mol. Phys. 1964, 7, 381. [Google Scholar] [CrossRef]
- Cho, M.; Silbey, R.J. Suppression and enhancement of van der Waals interactions. J. Chem. Phys. 1996, 104, 8730. [Google Scholar] [CrossRef]
- Marcovitch, M.; Diamant, H. Enhanced dispersion interaction in confined geometry. Phys. Rev. Lett. 2005, 95, 223203. [Google Scholar] [CrossRef] [PubMed]
- Matloob, R.; Loudon, R.; Barnett, S.M.; Jeffers, J. Electromagnetic field quantization in absorbing dielectrics. Phys. Rev. A 1995, 52, 4823. [Google Scholar] [CrossRef]
- Gruner, T.; Welsch, D.-G. Green-function approach to the radiation-field quantization for homogeneous and inhomogeneous Kramers-Kronig dielectrics. Phys. Rev. A 1996, 53, 1818. [Google Scholar] [CrossRef]
- Dung, H.T.; Knöll, L.; Welsch, D.-G. Three-dimensional quantization of the electromagnetic field in dispersive and absorbing inhomogeneous dielectrics. Phys. Rev. A 1998, 57, 3931. [Google Scholar] [CrossRef]
- Buhmann, S.Y.; Butcher, D.T.; Scheel, S. Macroscopic quantum electrodynamics in nonlocal and nonreciprocal media. New J. Phys. 2012, 65, 032813. [Google Scholar] [CrossRef]
- Scheel, S. The Casimir stress in real materials. In Forces of the Quantum Vacuum. An Introduction to Casimir Physics; Simpson, W.M.R., Leonhardt, U., Eds.; World Scientific Publ. Co.: Singapore, 2015; p. 107. [Google Scholar]
- Dung, H.T.; Knöll, L.; Welsch, D.-G. Intermolecular energy transfer in the presence of dispersing and absorbing media. Phys. Rev. A 2002, 14, 083034. [Google Scholar] [CrossRef]
- Unruh, W.G. Notes on black-hole evaporation. Phys. Rev. D 1976, 14, 870. [Google Scholar] [CrossRef]
- Fulling, S.A. Nonuniqueness of canonical field quantization in Riemannian space-time. Phys. Rev. D 1973, 7, 2850. [Google Scholar] [CrossRef]
- Davies, P.C.W. Scalar production in Schwarzschild and Rindler metrics. J. Phys. A 1973, 8, 609. [Google Scholar] [CrossRef]
- Crispino, L.C.B.; Higuchi, A.; Matsas, G.E.A. The Unruh effect and its applications. Rev. Mod. Phys. 2008, 80, 787. [Google Scholar] [CrossRef]
- Audretsch, G.; Müller, R. Radiative energy shifts of an accelerated two-level system. Phys. Rev. A 1995, 52, 629. [Google Scholar] [CrossRef] [PubMed]
- Passante, R. Radiative level shifts of an accelerated hydrogen atom and the Unruh effect in quantum electrodynamics. Phys. Rev. A 1998, 57, 1590. [Google Scholar] [CrossRef]
- Audretsch, G.; Müller, R. Spontaneous excitation of an accelerated atom: The contributions of vacuum fluctuations and radiation reaction. Phys. Rev. A 1994, 50, 1755. [Google Scholar] [CrossRef] [PubMed]
- Zhu, A.; Yu, H. Fulling-Davies-Unruh effect and spontaneous excitation of an accelerated atom interacting with a quantum scalar field. Phys. Lett. B 2006, 645, 459. [Google Scholar] [CrossRef]
- Calogeracos, A. Spontaneous excitation of an accelerated atom: (i) acceleration of infinite duration (the Unruh effect), (ii) acceleration of finite duration. Res. Phys. 2016, 6, 377. [Google Scholar] [CrossRef]
- Moore, G.T. Quantum theory of the electromagnetic field in a variable-length one-dimensional cavity. J. Math. Phys. 1970, 11, 2679. [Google Scholar] [CrossRef]
- Dodonov, V.V. Current status of the dynamical Casimir effect. Phys. Scr. 2010, 82, 038105. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Klimov, A.B. Generation and detection of photons in a cavity with a resonantly oscillating boundary. Phys. Rev. A 1996, 53, 2664. [Google Scholar] [CrossRef]
- Mundarain, D.F.; Maia Neto, P.A.M. Quantum radiation in a plane cavity with moving mirrors. Phys. Rev. A 1998, 57, 1379. [Google Scholar] [CrossRef]
- Law, C.K. Effective Hamiltonian for the radiation in a cavity with a moving mirror and a time-varying dielectric medium. Phys. Rev. A 1994, 49, 433. [Google Scholar] [CrossRef]
- Law, C.K. Interaction between a moving mirror and radiation pressure: A Hamiltonian formulation. Phys. Rev. A 1995, 51, 2537. [Google Scholar] [CrossRef] [PubMed]
- Dalvit, D.A.R.; Maia Neto, P.A.; Mazzitelli, D. Fluctuations, dissipation and the dynamical Casimir effect. In Casimir Physics; Dalvit, D., Milonni, P., Roberts, D., Rosa, F., Eds.; Springer: Berlin, Germany, 2011; p. 419. [Google Scholar]
- Barton, G. On van der Waals friction. I. Between two atoms. New J. Phys. 2010, 10, 113044. [Google Scholar] [CrossRef]
- Barton, G. On van der Waals friction. II: Between atom and half-space. New J. Phys. 2010, 10, 113045, Corrigendum in New J. Phys. 2012, 14, 079502, doi:10.1088/1367-2630/14/7/07950. [Google Scholar] [CrossRef]
- Intravaia, F.; Mkrtchian, V.E.; Buhmann, S.Y.; Scheel, S.; Dalvit, D.A.R.; Henkel, C. Friction forces on atoms after acceleration. J. Phys. Condens. Matter 2015, 27, 214020. [Google Scholar] [CrossRef] [PubMed]
- Rizzuto, L.; Spagnolo, S. Lamb shift of a uniformly accelerated hydrogen atom in the presence of a conducting plate. Phys. Rev. A 2009, 79, 062110. [Google Scholar] [CrossRef]
- Rizzuto, L. Casimir–Polder interaction between an accelerated two-level system and an infinite plate. Phys. Rev. A 2007, 76, 062114. [Google Scholar] [CrossRef]
- Schützhold, R.; Schaller, G.; Habs, D. Signatures of the Unruh Effect from Electrons Accelerated by Ultrastrong Laser Fields. Phys. Rev. Lett. 2006, 97, 121302. [Google Scholar] [CrossRef]
- Steinhauer, J. Observation of quantum Hawking radiation and its entanglement in an analogue black hole. Nature Phys. 2016, 12, 959. [Google Scholar] [CrossRef]
- Noto, A.; Passante, R. Van der Waals interaction energy between two atoms moving with uniform acceleration. Phys. Rev. D 2013, 88, 025041. [Google Scholar] [CrossRef]
- Dicke, R.H. Coherence in spontaneous radiation processes. Phys. Rev. 1954, 93, 99. [Google Scholar] [CrossRef]
- Rindler, W. Relativity. Special, General, and Cosmological; Oxford Univ. Press: Oxford, UK, 2006. [Google Scholar]
- Birrell, N.D.; Davies, P.C.W. Quantum Fields in Curved Space; Cambridge Univ. Press: Cambridge, UK, 1982. [Google Scholar]
- Dalibard, J.; Dupont-Roc, J.; Cohen-Tannoudji, C. Vacuum fluctuations and radiation reaction: Identification of their respective contributions. J. Phys. (Fr.) 1982, 43, 1617. [Google Scholar] [CrossRef]
- Dalibard, J.; Dupont-Roc, J.; Cohen-Tannoudji, C. Dynamics of a small system coupled to a reservoir: reservoir fluctuations and self-reaction. J. Phys. (Fr.) 1984, 45, 637. [Google Scholar] [CrossRef]
- Menezes, G.; Svaiter, N.F. Radiative processes of uniformly accelerated entangled atoms. Phys. Rev. A 2016, 93, 052117. [Google Scholar] [CrossRef]
- Zhou, W.; Yu, H. Spontaneous excitation of a uniformly accelerated atom coupled to vacuum Dirac field fluctuations. Phys. Rev. A 2012, 86, 033841. [Google Scholar] [CrossRef]
- Marino, J.; Noto, A.; Passante, R. Thermal and Nonthermal Signatures of the Unruh Effect in Casimir–Polder Forces. Phys. Rev. Lett. 2014, 113, 020403. [Google Scholar] [CrossRef]
- Noto, A.; Marino, J.; Passante, R. A fourth–order statistical method for the calculation of dispersion Casimir–Polder interactions. 2018. in preparation. [Google Scholar]
- Rizzuto, L.; Lattuca, M.; Marino, J.; Noto, A.; Spagnolo, S.; Zhou, W.; Passante, R. Nonthermal effects of acceleration in the resonance interaction between two uniformly accelerated atoms. Phys. Rev. A 2016, 94, 012121. [Google Scholar] [CrossRef]
- Zhou, W.; Passante, R.; Rizzuto, L. Resonance interaction energy between two accelerated identical atoms in a coaccelerated frame and the Unruh effect. Phys. Rev. D 2016, 94, 105025. [Google Scholar] [CrossRef]
- Zhou, W.; Passante, R.; Rizzuto, L. Resonance Dipole–Dipole Interaction between Two Accelerated Atoms in the Presence of Reflecting Plane Boundary. Symmetry 2018, 10, 185. [Google Scholar] [CrossRef]
- Menezes, G.; Kiefer, C.; Marino, J. Thermal and nonthermal scaling of the Casimir–Polder interaction in a black hole spacetime. Phys. Rev. D 2016, 95, 085014. [Google Scholar] [CrossRef]
- Zhou, W.; Yu, Y. Resonance interatomic energy in a Schwarzschild spacetime. Phys. Rev. D 2017, 96, 045018. [Google Scholar] [CrossRef]
- Zhou, W.; Yu, Y. Boundarylike behaviors of the resonance interatomic energy in a cosmic string spacetime. Phys. Rev. D 2018, 97, 045007. [Google Scholar] [CrossRef]
- Senitzky, I.R. Radiation-reaction and vacuum-field effects in Heisenberg-picture quantum electrodynamics. Phys. Rev. Lett. 1973, 31, 955. [Google Scholar] [CrossRef]
- Milonni, P.W.; Ackerhalt, J.R.; Smith, W.A. Interpretation of radiative corrections in spontaneous emission. Phys. Rev. Lett. 1973, 31, 958. [Google Scholar] [CrossRef]
- Milonni, P.W. Semiclassical and quantum-electrodynamical approaches in nonrelativistic radiation theory. Phys. Rep. 1976, 25, 1. [Google Scholar] [CrossRef]
- Adler, R.J.; Casey, B.; Jacob, O.C. Vacuum catastrophe: An elementary exposition of the cosmological constant problem. Am. J. Phys. 1995, 63, 620. [Google Scholar] [CrossRef]
- Cree, S.S.; Davis, T.M.; Ralph, T.C.; Wang, Q.; Zhu, Z.; Unruh, W.G. Can the fluctuations of the quantum vacuum solve the cosmological constant problem? Phys. Rev. D 2018, 98, 063506. [Google Scholar] [CrossRef]
- Solá, J. Cosmological constant and vacuum energy: Old and new ideas. J. Phys. Conf. Ser. 2013, 453, 012015. [Google Scholar] [CrossRef]
- Carroll, S.M. Spacetime and Geometry: An Introduction to General Relativity; Pearson Education Limited: Harlow, UK, 2014. [Google Scholar]

© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Passante, R. Dispersion Interactions between Neutral Atoms and the Quantum Electrodynamical Vacuum. Symmetry 2018, 10, 735. https://doi.org/10.3390/sym10120735
Passante R. Dispersion Interactions between Neutral Atoms and the Quantum Electrodynamical Vacuum. Symmetry. 2018; 10(12):735. https://doi.org/10.3390/sym10120735
Chicago/Turabian StylePassante, Roberto. 2018. "Dispersion Interactions between Neutral Atoms and the Quantum Electrodynamical Vacuum" Symmetry 10, no. 12: 735. https://doi.org/10.3390/sym10120735
APA StylePassante, R. (2018). Dispersion Interactions between Neutral Atoms and the Quantum Electrodynamical Vacuum. Symmetry, 10(12), 735. https://doi.org/10.3390/sym10120735




