Hesitant Picture 2-Tuple Linguistic Aggregation Operators Based on Archimedean T-Norm and T-Conorm and Their Use in Decision-Making
Abstract
1. Introduction
2. Preliminaries
- (1)
- if SC(b) < SC(e), then b < e,
- (2)
- if SC(b) > SC(e), then b > e,
- (3)
- when SC(b) = SC(e), and
- (a)
- AC(b) = AC(e), then b = e,
- (b)
- AC(b) < AC(e), then b < e,
- (c)
- AC(b) > AC(e), then b > e.
3. Operational Laws of Hesitant Picture 2-Tuple Linguistic Sets
- .
- .
- .
- If , , then .
- .
- .
- .
- If , , then .
- Addition operation
- Multiplication operation
- Scalar-multiplication operation
- Exponential operation
- (1)
- (2)
- (3)
- (4)
- (1)
- (2)
- (3)
- (4)
- (1)
- (2)
- (3)
- (4)
- (5)
- (6)
- (1)
- (2)
- (3)
- (4)
- (5)
- Notice that
- (6)
- Based onthe following formulas are obtained:By using the above equations, we can obtain .
4. Some Aggregation Operators of HP2TLSs Based on Archimedean T-norm and T-conorm
- (1)
- (Idempotency): Let be some HP2TLSs, if all are identical, i.e., , for all i, thenProof.Let and , where , for all , then we have□
- (2)
- (Boundedness): Let be some HP2TLSs, if and , thenwhereProof.Since , and are two increasing functions, and are two decreasing functions, then we have:In the same way, we can obtain , . According to Definition 3, 4, and 5, we can verify that . Similarity, can be proved. Thus, the following inequality holds:□
- (3)
- (Monotonicity): Let and be two HP2TLSs, and , . If any and satisfy , then
5. A Novel Method of Solving MADM Problems
6. Application of the Designed Method and Discussion
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Park, J.H.; Kwun, Y.C.; Son, M.J. A generalized intuitionistic fuzzy soft set theoretic approach to decision making problems. Int. J. Fuzzy Logic Intell. Syst. 2011, 11, 71–76. [Google Scholar] [CrossRef]
- Xiao, G.Q.; Li, K.L.; Li, K.Q. Reporting/most influential objects in uncertain databases based on probabilistic reverse top-k queries. Inf. Sci. 2017, 405, 207–226. [Google Scholar] [CrossRef]
- Xiao, G.Q.; Li, K.L.; Zhou, X.; Li, K.Q. Efficient monochromatic and bichromatic probabilistic reverse top-k query processing for uncertain big data. J. Comput. Syst. Sci. 2017, 89, 92–113. [Google Scholar] [CrossRef]
- Shenbaga, T.S. A study on the customer satisfaction towards online shopping. Sumedha J. Manag. 2015, 4, 4–15. [Google Scholar]
- Trong, V.H.; Tan, N.; Khanh, V.; Gim, G. Evaluating factors influencing consumer satisfaction towards online shopping in viet nam. J. Emerg. Trends Comput. Inf. Sci. 2014, 5, 67–71. [Google Scholar]
- Akusok, A.; Miche, Y.; Hegedus, J.; Rui, N.; Lendasse, A. A two-stage methodology using K-NN and false-positive minimizing ELM for nominal data classification. Cognit. Comput. 2014, 6, 432–445. [Google Scholar] [CrossRef]
- Czubenko, M.; Kowalczuk, Z.; Ordys, A. Autonomous driver based on an intelligent system of decision-making. Cognit. Comput. 2015, 7, 569–581. [Google Scholar] [CrossRef] [PubMed]
- Meng, F.Y.; Chen, X.H. Correlation coefficients of hesitant fuzzy sets and their application based on fuzzy measures. Cognit. Comput. 2015, 7, 445–463. [Google Scholar] [CrossRef]
- Yang, J.; Gong, L.Y.; Tang, Y.F.; Yan, J.; He, H.B.; Zhang, L.Q.; Li, G. An improved SVM-based cognitive diagnosis algorithm for operation states of distribution grid. Cognit. Comput. 2015, 7, 582–593. [Google Scholar] [CrossRef]
- Herrera, F.; Herrera-Viedma, E. Choice functions and mechanisms for linguistic preference relations. Eur. J. Oper. Res. 2000, 120, 144–161. [Google Scholar] [CrossRef]
- Herrera, F.; Herrera-Viedma, E. Linguistic decision analysis: Steps for solving decision problems under linguistic information. Fuzzy Sets Syst. 2000, 115, 67–82. [Google Scholar] [CrossRef]
- Herrera, F.; Herrera-Viedma, E.; Verdegay, J.L. A model of consensus in group decision making under linguistic assessments. Fuzzy Sets Syst. 1996, 78, 73–87. [Google Scholar] [CrossRef]
- Wu, Q.; Wang, F.; Zhou, L.G.; Chen, H.Y. Method of multiple attribute group decision making based on 2-dimension interval type-2 fuzzy aggregation operators with multi-granularity linguistic information. Int. J. Fuzzy Syst. 2017, 19, 1880–1903. [Google Scholar] [CrossRef]
- Xu, Z.S. A method based on linguistic aggregation operators for group decision making with linguistic preference relations. Inf. Sci. 2014, 166, 19–30. [Google Scholar] [CrossRef]
- Atanassov, K.T. Intuitionistic fuzzy sets. Fuzzy Sets Syst. 2012, 20, 87–96. [Google Scholar] [CrossRef]
- Kim, T.; Sotirova, E.; Shannon, A.; Atanassova, V.; Atanassov, K.; Jang, L. Interval valued intuitionistic fuzzy evaluations for analysis of a student’s knowledge in university e-learning courses. Int. J. Fuzzy Logic Intell. Syst. 2018, 18, 190–195. [Google Scholar] [CrossRef]
- Qin, J.D.; Liu, X.W.; Pedrycz, W. A multiple attribute interval type-2 fuzzy group decision making and its application to supplier selection with extended LINMAP method. Soft Comput. 2017, 21, 3207–3226. [Google Scholar] [CrossRef]
- Xia, M.M.; Xu, Z.S. Hesitant fuzzy information aggregation in decision making. Int. J. Approx. Reason. 2011, 52, 395–407. [Google Scholar] [CrossRef]
- Xia, M.M.; Xu, Z.S.; Chen, N. Some hesitant fuzzy aggregation operators with their application in group decision making. Group Decis. Negot. 2013, 22, 259–279. [Google Scholar] [CrossRef]
- Zadeh, L.A. Fuzzy Sets, Fuzzy logic, & Fuzzy Systems. Fuzzy Sets 1996, 8, 394–432. [Google Scholar]
- Martínez, L.; Rodriguez, R.M.; Herrera, F. The 2-Tuple Linguistic Model-Computing with Words in Decision Making; Springer Publishing Company: New York, NY, USA, 2015. [Google Scholar]
- Chen, Z.C.; Liu, P.H.; Pei, Z. An approach to multiple attribute group decision making based on linguistic intuitionistic fuzzy numbers. Int. J. Comput. Intell. Syst. 2015, 8, 747–760. [Google Scholar] [CrossRef]
- Wang, J.Q.; Li, H.B. Multi-criteria decision-making method based on aggregation operators for intuitionistic linguistic fuzzy numbers. Control Decis. 2010, 25, 1571–1574. [Google Scholar]
- Herrera, F.; Martinez, L. A 2-tuple fuzzy linguistic representation model for computing with words. IEEE Trans. Fuzzy Syst. 2000, 8, 746–752. [Google Scholar]
- Herrera, F.; Martinez, L. A model based on linguistic 2-tuples for dealing with multigranular hierarchical linguistic contexts in multi-expert decision-making. IEEE Trans. Syst. Man Cybern. Part B Cybern. 2000, 31, 227–234. [Google Scholar] [CrossRef] [PubMed]
- Wang, R.; Li, Y.L. Picture hesitant fuzzy set and its application to multiple criteria decision-making. Symmetry 2018, 10. [Google Scholar] [CrossRef]
- Cuong, B.C. Picture fuzzy sets-first results: Part 1. J. Comput. Sci. Cybern. 2014, 30, 409–420. [Google Scholar]
- Cuong, B.C.; Prhong, P.H.; Smarandache, F. Smarandache. Standard neutrosophic soft theory: Some first results. Neutrosophic Sets Syst. 2016, 12, 80–91. [Google Scholar]
- Thão, N.X.; Dinh, N.V. Rough picture fuzzy set and picture fuzzy topologies. J. Comput. Sci. Cybern. 2015, 31, 245–253. [Google Scholar]
- Wei, G.W. Some cosine similarity measures for picture fuzzy sets and their applications to strategic decision making. Informatica 2017, 28, 547–564. [Google Scholar] [CrossRef]
- Le, H.S.; Viet, P.V.; Hai, P.V. Picture inference system: A new fuzzy inference system on picture fuzzy set. Appl. Intell. 2017, 46, 652–669. [Google Scholar]
- Dutta, P.; Ganju, S. Application of interval valued picture fuzzy set in medical diagnosis. In Proceedings of the 19th International Conference on Knowledge Discovery and Fuzzy Systems, Mumbai, India, 7–8 February 2017. [Google Scholar]
- Wei, G.W. Picture 2-tuple linguistic Bonferroni mean operators and their application to multiple attribute decision making. Int. J. Fuzzy Syst. 2017, 19, 997–1010. [Google Scholar] [CrossRef]
- Nie, R.X.; Wang, J.Q.; Wang, T.L. A hybrid outranking method for greenhouse gas emissions’ institution selection with picture 2-tuple linguistic information. Comput. Appl. Math. 2018. [Google Scholar] [CrossRef]
- Wei, G.W.; Alsaadi, F.E.; Hayat, T.; Alsaedi, A. Picture 2-tuple linguistic aggregation operators in multiple attribute decision making. Soft Comput. 2018, 22, 989–1002. [Google Scholar] [CrossRef]
- Zhang, X.Y.; Wang, J.Q.; Hu, J.H. On novel operational laws and aggregation operators of picture 2-tuple linguistic information for MCDM problems. Int. J. Fuzzy Syst. 2018, 20, 958–969. [Google Scholar] [CrossRef]
- Beg, I.; Rashid, T. Group decision making using intuitionistic hesitant fuzzy sets. Int. J. Fuzzy Logic Intell. Syst. 2014, 14, 181–187. [Google Scholar] [CrossRef]
- Wei, G.W. A method for multiple attribute group decision making based on the ET-WG and ET-OWG operators with 2-tuple linguistic information. Exp. Syst. Appl. 2010, 37, 7895–7900. [Google Scholar] [CrossRef]
- Xu, Y.J.; Wang, H.M. Approaches based on 2-tuple linguistic power aggregation operators for multiple attribute group decision making under linguistic environment. Soft Comput. 2011, 11, 3988–3997. [Google Scholar] [CrossRef]
- Ge, S.N.; Wei, C.P. A hesitant fuzzy language decision making method based on 2-tuple. Oper. Res. Manag. Sci. 2017, 26, 108–114. (In Chinese) [Google Scholar]
- Wang, L.D.; Wang, Y.J.; Liu, X.D. Prioritized aggregation operators and correlated aggregation operators for hesitant 2-tuple linguistic variables. Symmetry 2018, 10. [Google Scholar] [CrossRef]
- Klir, G.J.; Yuan, B. Fuzzy sets and fuzzy logic: Theory and applications. Prent. Hall 1995, 1, 283–287. [Google Scholar]
- Nguyen, H.T.; Walker, E.A. A First Course in Fuzzy Logic; CRC Press: Boca Raton, FL, USA, 1997. [Google Scholar]
- Liu, P.D. The aggregation operators based on Archimedean t-conorm and t-norm for single-valued neutrosophic numbers and their application to decision making. Int. J. Fuzzy Syst. 2016, 18, 849–863. [Google Scholar] [CrossRef]
- Xia, M.M.; Xu, Z.S.; Zhu, B. Some issues on intuitionistic fuzzy aggregation operators based on Archimedean t-conorm and t-norm. Knowl. Based Syst. 2012, 31, 78–88. [Google Scholar] [CrossRef]
- Liu, P.D.; Chen, S.M. Heronian aggregation operators of intuitionistic fuzzy numbers based on the Archimedean t-norm and t-conorm. In Proceedings of the 2016 International Conference on Machine Learning & Cybernetics, Jeju, Korea, 10–13 July 2016; pp. 686–691. [Google Scholar]
- Yu, D. Group decision making under interval-valued multiplicative intuitionistic fuzzy environment based on Archimedean t-conorm and t-norm. Int. J. Intell. Syst. 2015, 30, 590–616. [Google Scholar] [CrossRef]
- Zhang, Z.M.; Wu, C. Some interval-valued hesitant fuzzy aggregation operators based on Archimedean t-norm and t-conorm with their application in multi-criteria decision making. Int. J. Intell. Syst. 2014, 27, 2737–2748. [Google Scholar]
- Tao, Z.F.; Chen, H.Y.; Zhou, L.G.; Liu, J.P. On new operational laws of 2-tuple linguistic information using Archimedean t-norm and s-norm. Knowl. Based Syst. 2014, 66, 156–165. [Google Scholar] [CrossRef]
- Herrera, F.; Martinez, L. An approach for combining linguistic and numerical information based on the 2-tuple fuzzy linguistic representation model in decision-making. Int. J. Uncertain. Fuzziness Knowl. Based Syst. 2000, 8, 539–562. [Google Scholar] [CrossRef]
- Yang, W.; Chen, Z.P. New aggregation operators based on the Choquet integral and 2-tuple linguistic information. Expert Syst. Appl. 2012, 39, 2662–2668. [Google Scholar] [CrossRef]
- Zhang, W.C.; Xu, Y.J.; Wang, H.M. A consensus reaching model for 2-tuple linguistic multiple attribute group decision making with incomplete weight information. Int. J. Syst. Sci. 2016, 47, 389–405. [Google Scholar] [CrossRef]
- Zhang, Z.M. Archimedean operations-based interval-valued hesitant fuzzy ordered weighted operators and their application to multi-criteria decision making. Br. J. Math. Comput. Sci. 2016, 17, 1–34. [Google Scholar] [CrossRef] [PubMed]
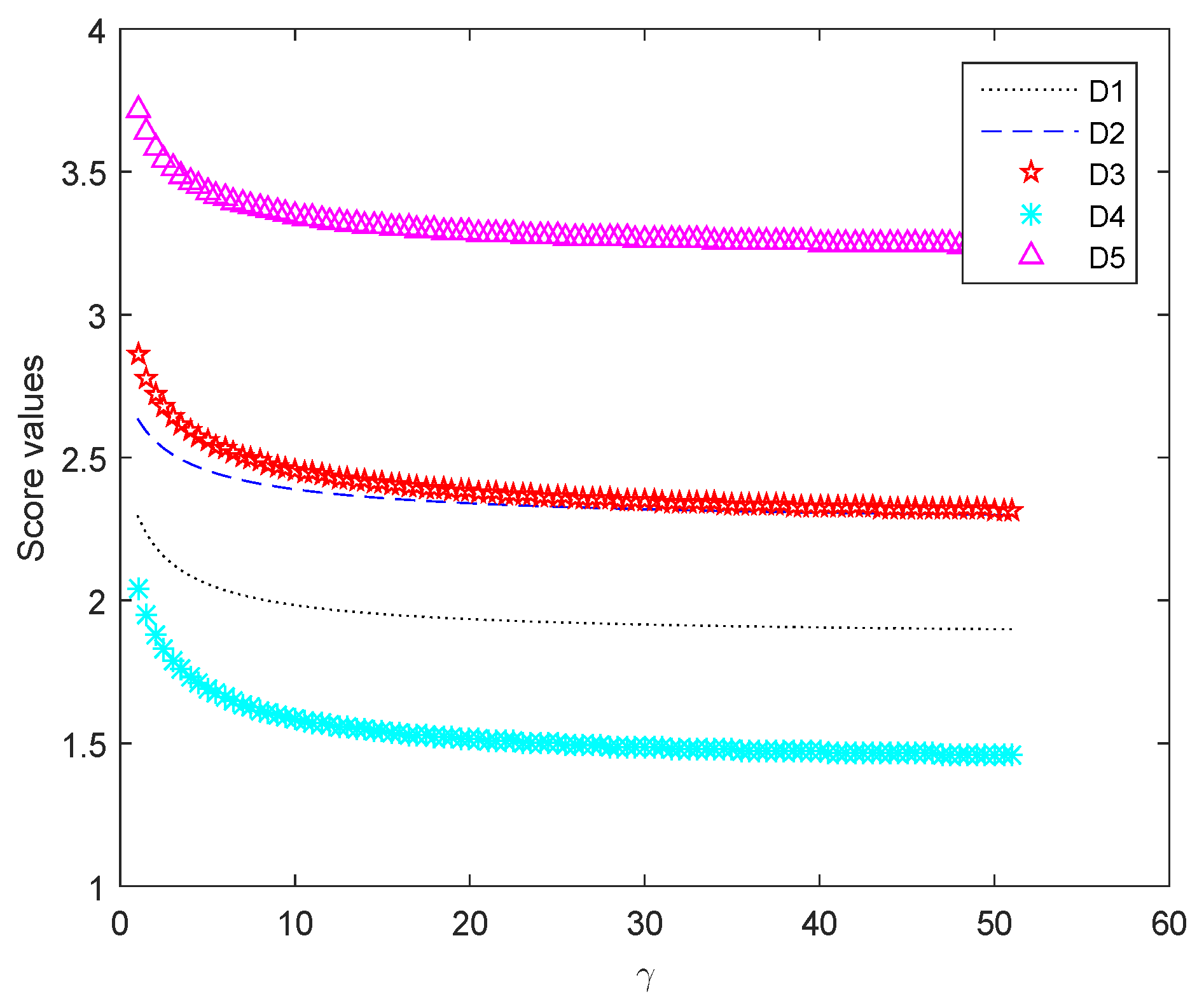
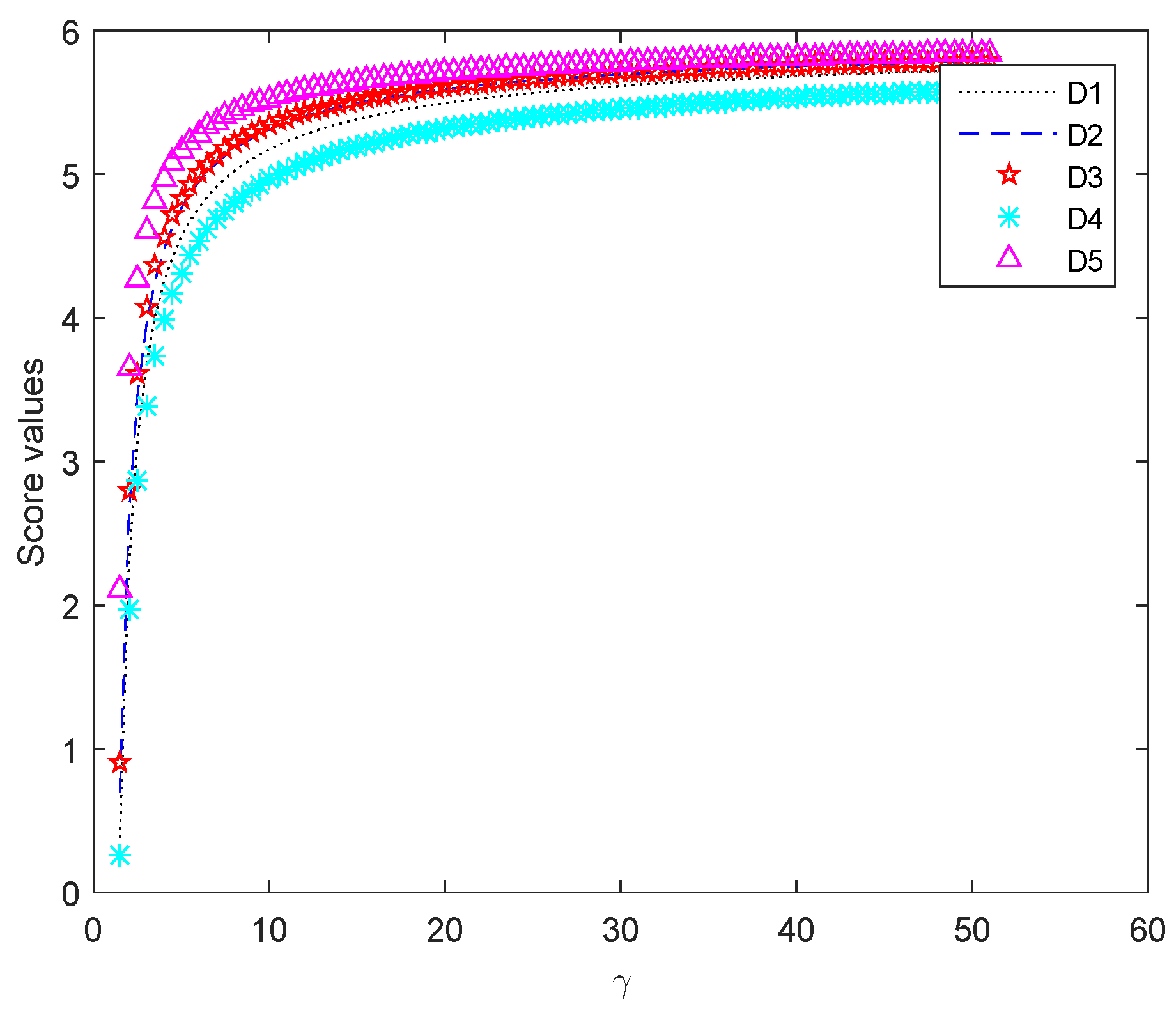
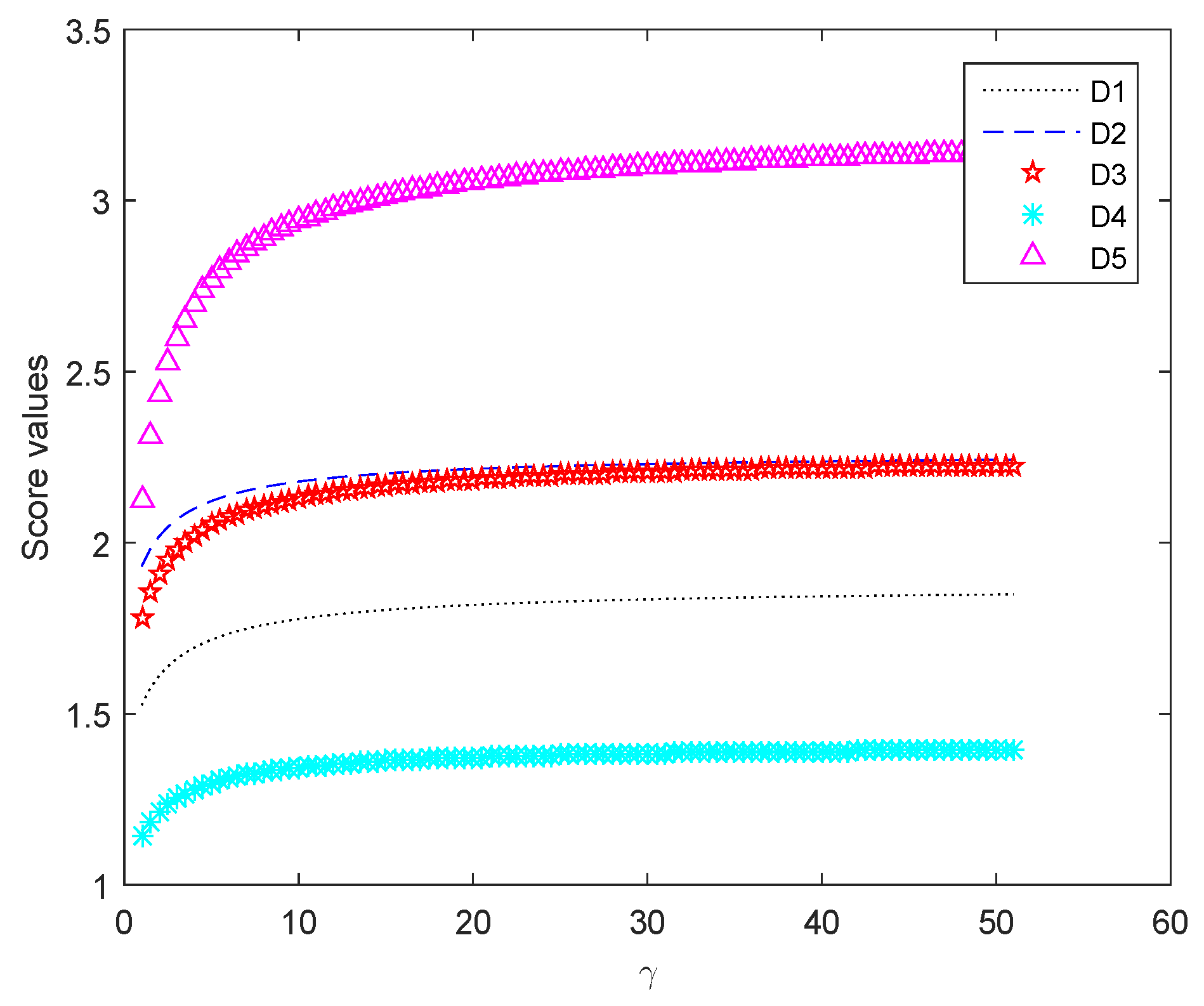
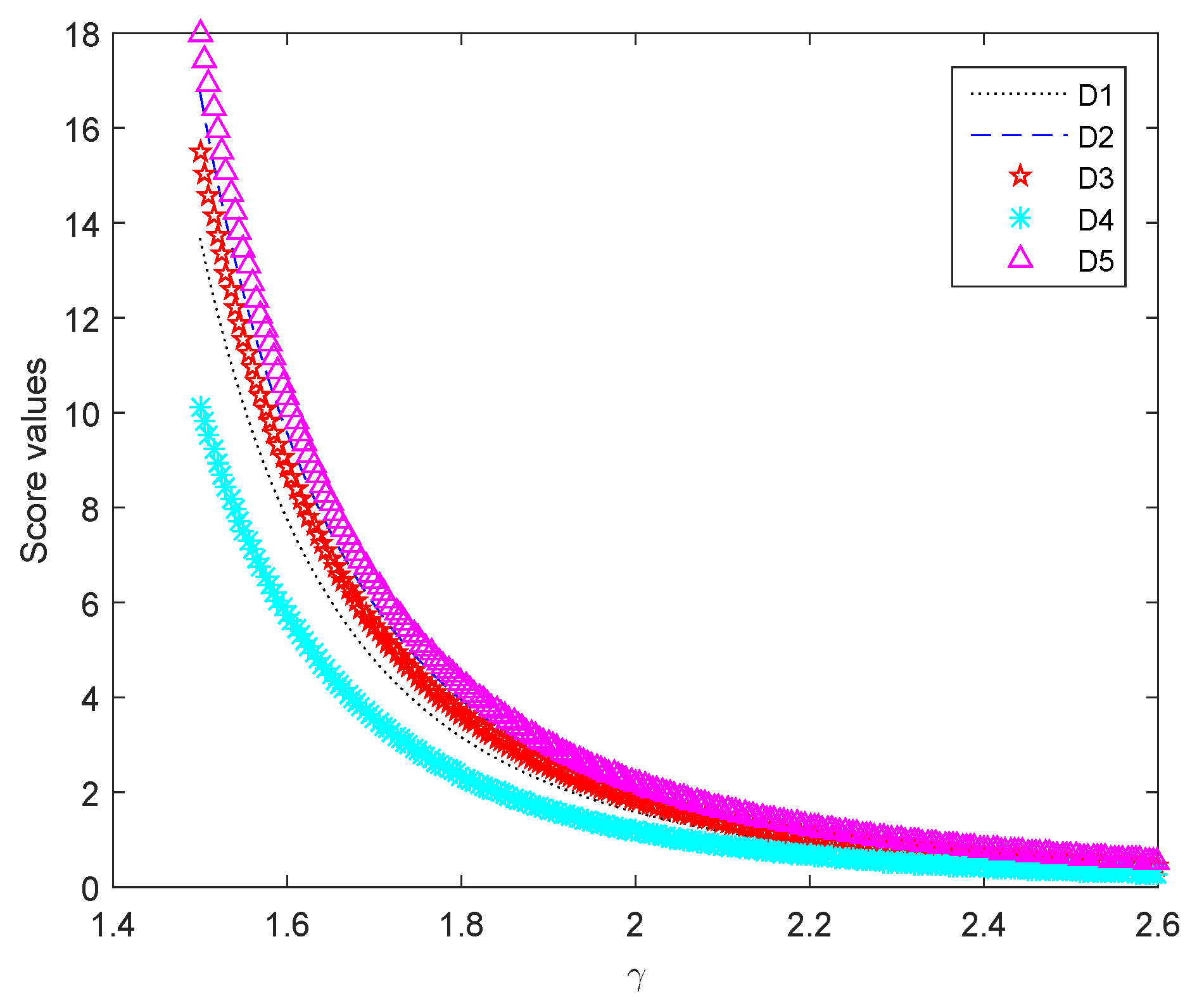
| } | ||
| HP2TLWA Operator | EHP2TLWA Operator | |
| HHP2TLWA Operator () | FHP2TLWA Operator () | |
| HP2TLWG Operator | EHP2TLWG Operator | |
| HHP2TLWA Operator () | FHP2TLWA Operator () | |
| HP2TLWA Operator | EHP2TLWA Operator | HHP2TLWA Operator () | FHP2TLWA Operator () | |
|---|---|---|---|---|
| 2.2969 | 2.1888 | 2.1888 | 2.2462 | |
| 2.6355 | 2.5578 | 2.5574 | 2.5999 | |
| 2.8581 | 2.7213 | 2.7213 | 2.7910 | |
| 2.0440 | 1.8793 | 1.8793 | 1.9658 | |
| 3.7158 | 3.5839 | 3.5839 | 3.6514 | |
| Ranking |
| HP2TLWA Operator | EHP2TLWA Operator | HHP2TLWA Operator () | FHP2TLWA Operator () | |
|---|---|---|---|---|
| 1.5257 | 1.6329 | 1.6112 | 1.5805 | |
| 1.9307 | 2.0435 | 2.0188 | 1.9892 | |
| 1.7824 | 1.9052 | 1.9081 | 1.8435 | |
| 1.1420 | 1.2177 | 1.2141 | 1.1804 | |
| 2.1235 | 2.2435 | 2.4362 | 2.1812 | |
| Ranking |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Wang, L.; Wang, H.; Feng, X. Hesitant Picture 2-Tuple Linguistic Aggregation Operators Based on Archimedean T-Norm and T-Conorm and Their Use in Decision-Making. Symmetry 2018, 10, 629. https://doi.org/10.3390/sym10110629
Wang Y, Wang L, Wang H, Feng X. Hesitant Picture 2-Tuple Linguistic Aggregation Operators Based on Archimedean T-Norm and T-Conorm and Their Use in Decision-Making. Symmetry. 2018; 10(11):629. https://doi.org/10.3390/sym10110629
Chicago/Turabian StyleWang, Yanjun, Lidong Wang, Huijuan Wang, and Xinghua Feng. 2018. "Hesitant Picture 2-Tuple Linguistic Aggregation Operators Based on Archimedean T-Norm and T-Conorm and Their Use in Decision-Making" Symmetry 10, no. 11: 629. https://doi.org/10.3390/sym10110629
APA StyleWang, Y., Wang, L., Wang, H., & Feng, X. (2018). Hesitant Picture 2-Tuple Linguistic Aggregation Operators Based on Archimedean T-Norm and T-Conorm and Their Use in Decision-Making. Symmetry, 10(11), 629. https://doi.org/10.3390/sym10110629





