Inexact Two-Stage Stochastic Programming for Water Resources Allocation under Considering Demand Uncertainties and Response—A Case Study of Tianjin, China
Abstract
:1. Introduction
2. Methodology
3. Case Study
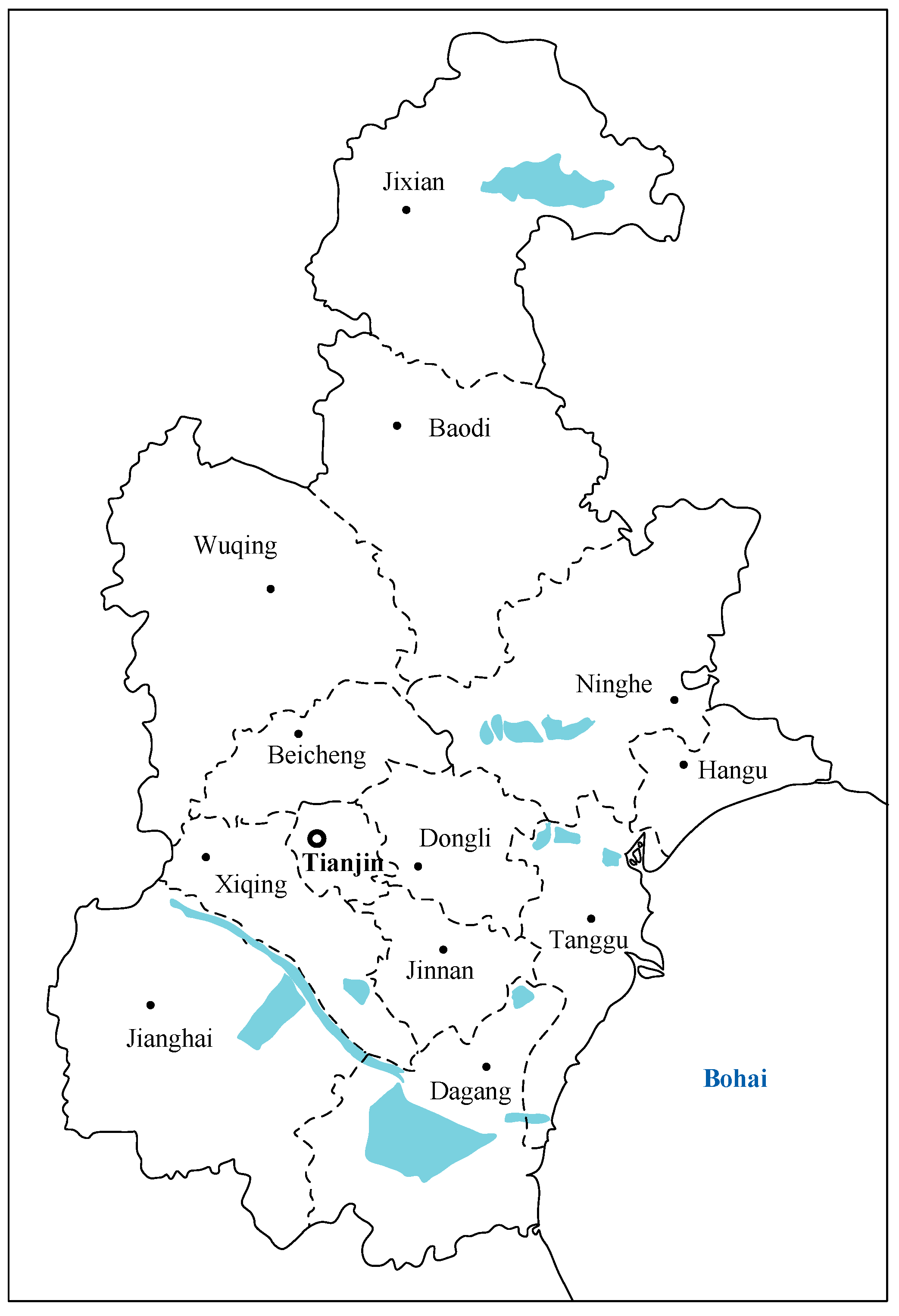
3.1. Overview of Water Supply and Demand in Tianjin
3.2. Model Formulation
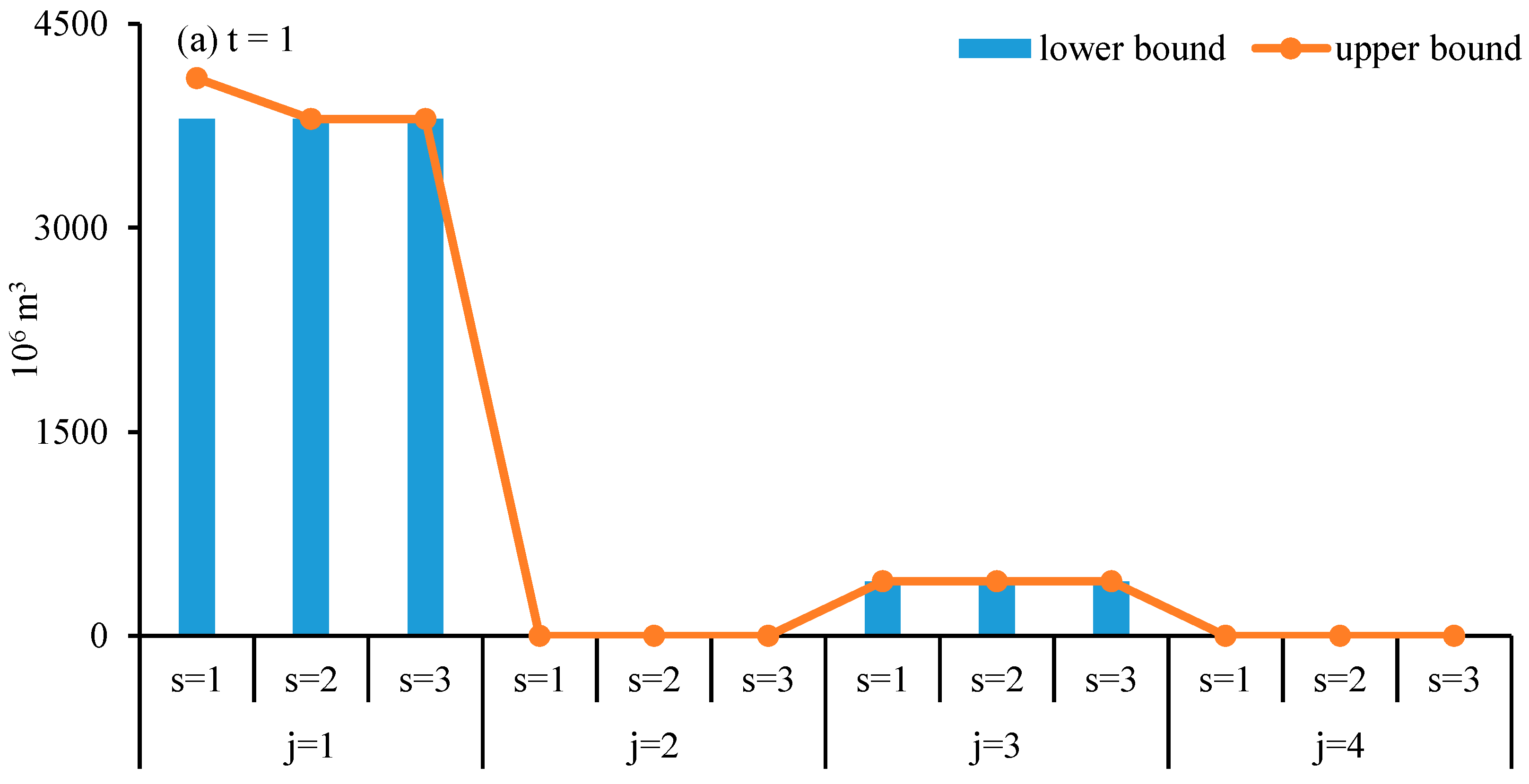
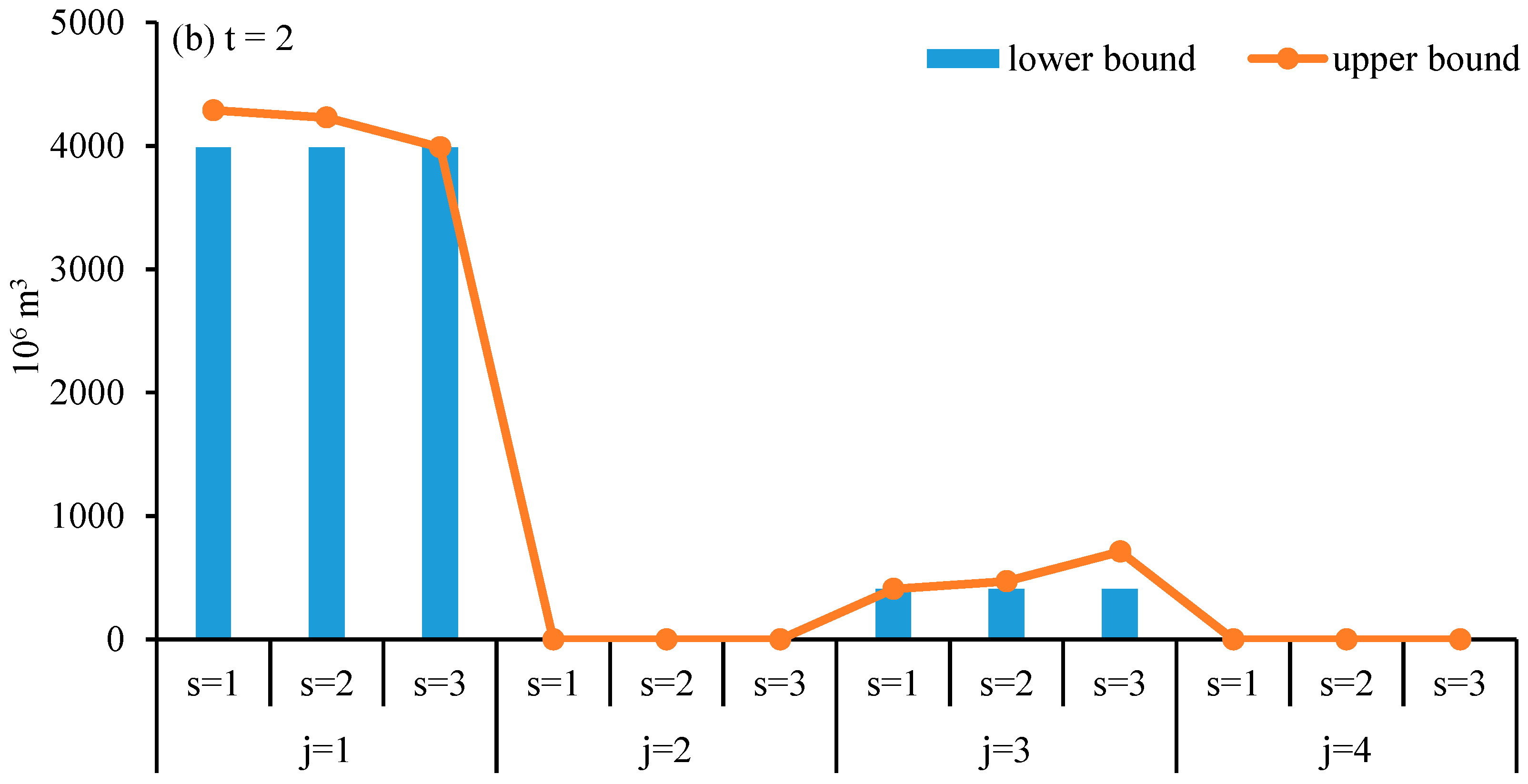
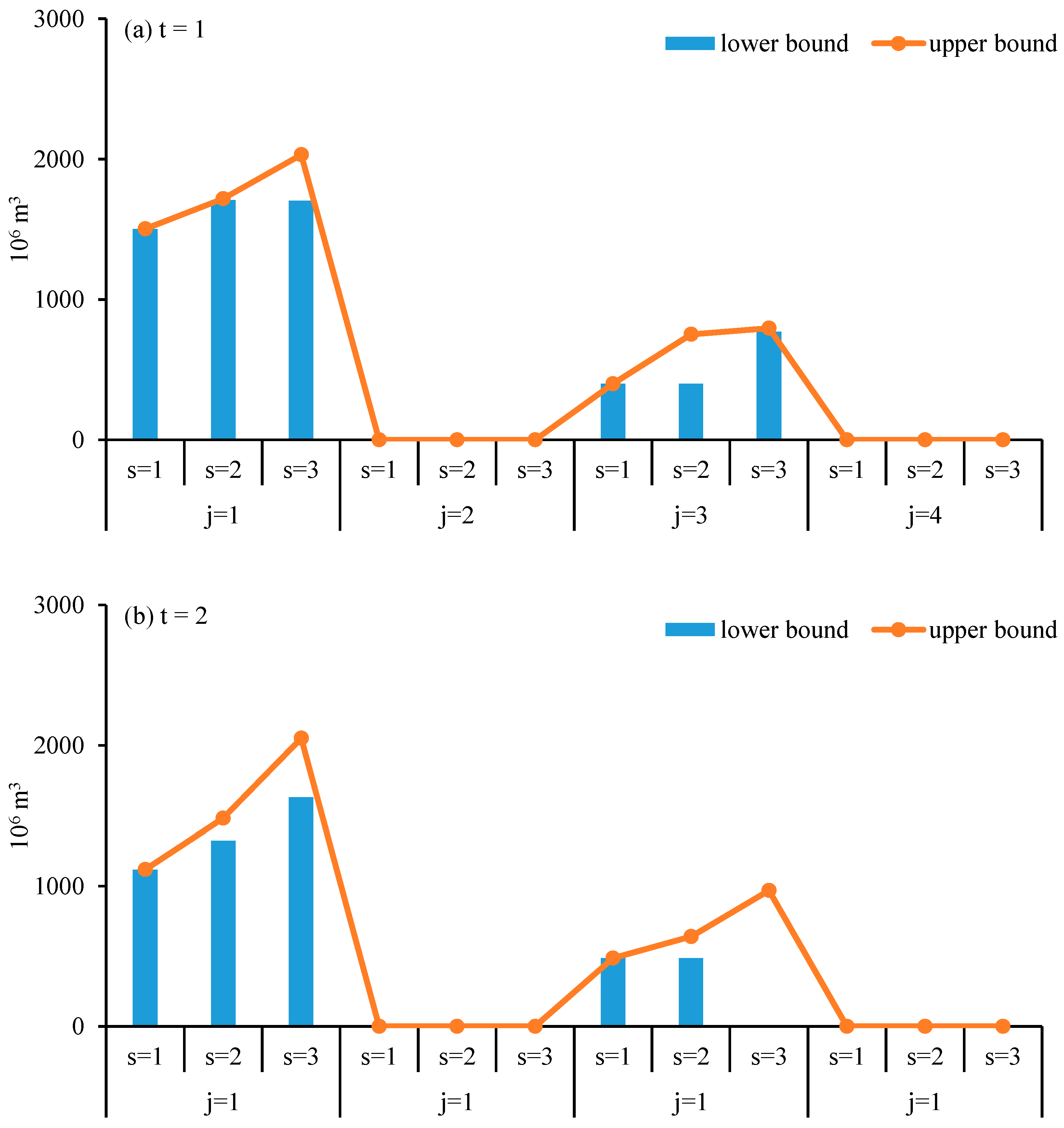
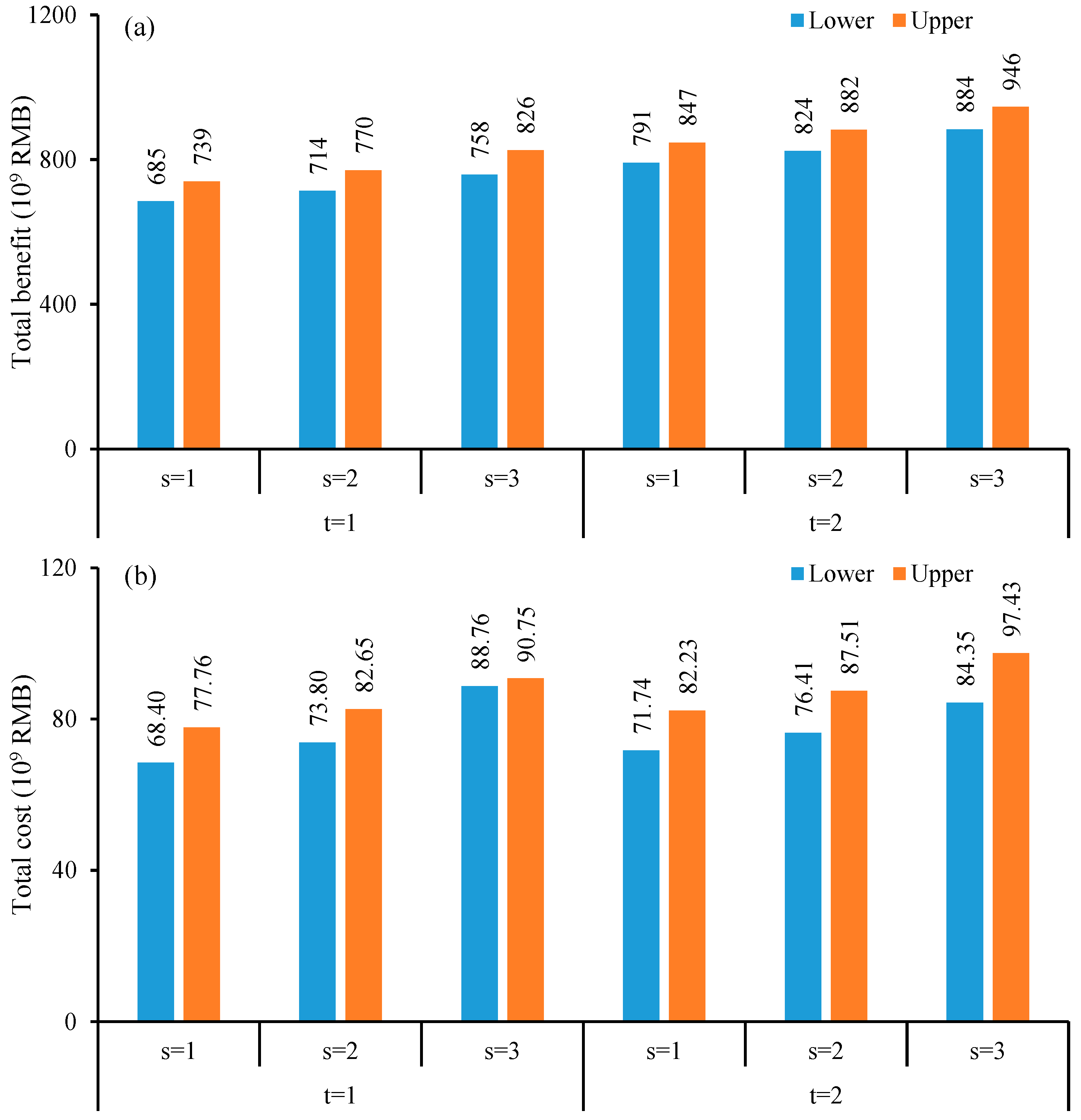
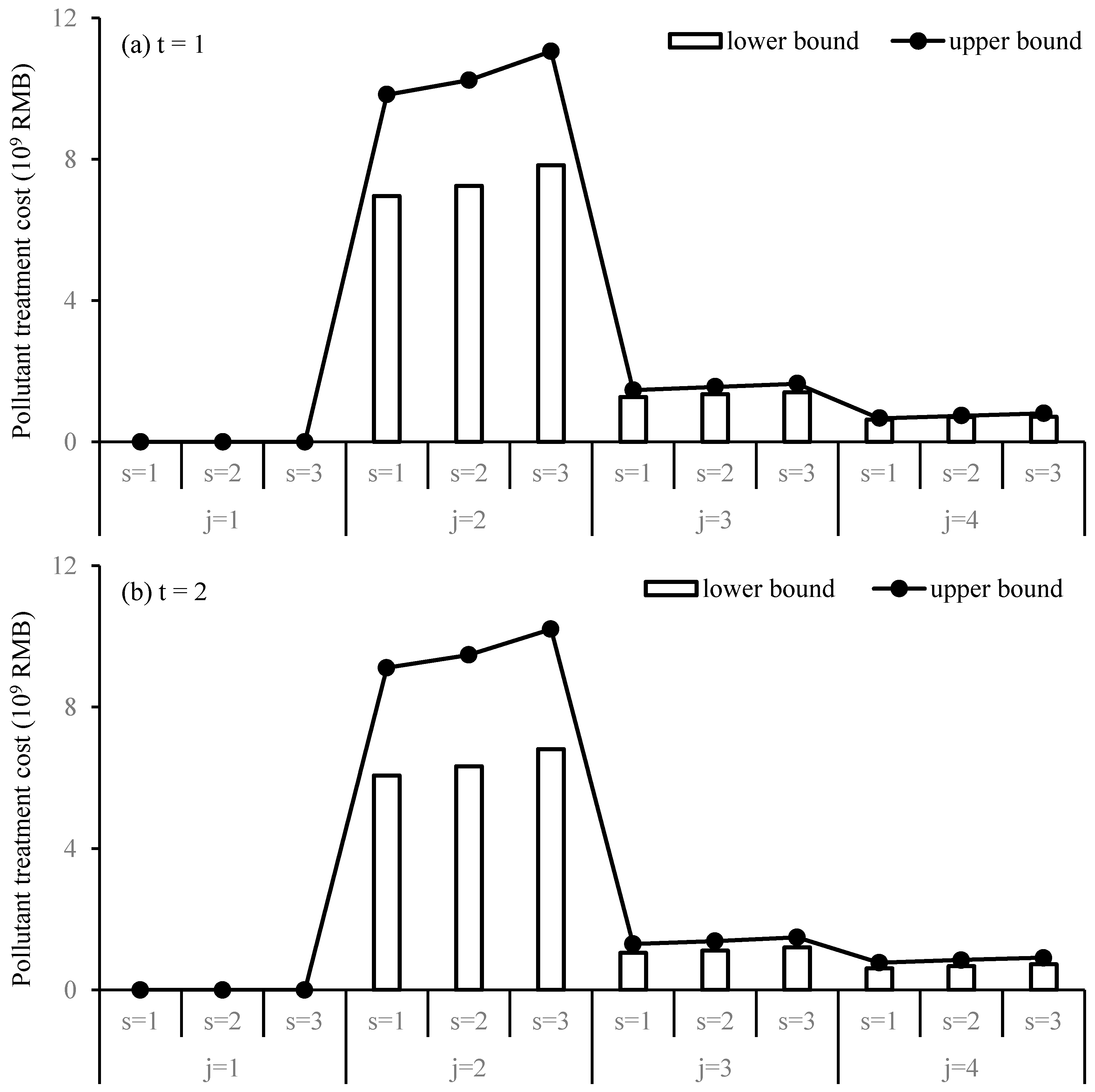
4. Results Analysis and Discussion
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Tumeizi, A.; Hammad, A.A. Traditional water distribution for irrigation in the Middle Ease: Practices and environmental impacts. J. Water Clim. Chang. 2016. [Google Scholar] [CrossRef]
- Wang, Y.Y.; Huang, G.H.; Wang, S.; Li, W. A stochastic programming with imprecise probabilities model for planning water resources systems under multiple uncertainties. Stoch. Environ. Res. Risk Assess. 2016, 30, 2169–2178. [Google Scholar] [CrossRef]
- Kondili, E.; Kaldellis, J.K.; Papapostolou, C. A novel systemic approach to water resources optimisation in areas with limited water resources. Desalination 2010, 250, 297–301. [Google Scholar] [CrossRef]
- Davijani, M.H.; Banihabib, M.E.; Anvar, A.N.; Hashemi, S.R. Multi-objective optimization model for the allocation of water resources in arid regions based on the maximization of socioeconomic efficiency. Water Resour. Manag. 2016, 30, 927–946. [Google Scholar] [CrossRef]
- He, G.; Yan, J.; Sha, J.; Song, C.; Zhong, S. Exploration of an optimal policy for water resources management including the introduction of advanced sewage treatment technologies in Zaozhuang City, China. Water 2016, 8, 608. [Google Scholar] [CrossRef]
- Hong, S.; Xia, J.; Chen, J.; Wan, L.; Ning, L.; Shi, W. Multi-object approach and its application to adaptive water management under climate change. J. Geogr. Sci. 2017, 27, 259–274. [Google Scholar] [CrossRef]
- Xie, Y.L.; Xia, D.H.; Huang, G.H.; Li, W.; Xu, Y. A multistage stochastic robust optimization model with fuzzy probability distribution for water supply management under uncertainty. Stoch. Environ. Res. Risk Assess. 2017, 31, 125–143. [Google Scholar] [CrossRef]
- Rezaei, F.; Safavi, H.R.; Zekri, M. A hybrid fuzzy-based multi-objective PSO algorithm for conjunctive water use and optimal multi-crop pattern planning. Water Resour. Manag. 2017, 31, 1139–1155. [Google Scholar] [CrossRef]
- Li, X.; Kang, S.; Niu, J.; Du, T.; Tong, L.; Li, S.; Ding, R. Applying uncertain programming model to improve regional farming economic benefits and water productivity. Agric. Water Manag. 2017, 179, 352–365. [Google Scholar] [CrossRef]
- Puy, A.; Muneepeerakul, R.; Balbo, A. Size and stochasticity in irrigated social-ecological systems. Sci. Rep. 2017. [Google Scholar] [CrossRef] [PubMed]
- Zahiri, B.; Torabi, S.A.; Tavakkoli-Moghaddam, R. A novel multi-stage possibilistic stochastic programming approach (with an application in relief distribution planning). Inf. Sci. 2017, 385–386, 225–249. [Google Scholar] [CrossRef]
- Xu, J.; Huang, G.; Li, Z.; Chen, J. A two-stage fuzzy chance-constrained water management model. Environ. Sci. Pollut. Res. 2017. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Y.; Huang, G.; Wang, S.; Zhai, Y.; Xin, X. Water resources management under dual uncertainties: A factorial fuzzy two-stage stochastic programming approach. Stoch. Environ. Res. Risk Assess. 2016, 30, 795–811. [Google Scholar] [CrossRef]
- Birge, J.R.; Louveaux, F.V. Introduction to Stochastic Programming; Springer: New York, NY, USA, 1997. [Google Scholar]
- Huang, G.H.; Loucks, D.P. An inexact two-stage stochastic programming model for water resources management under uncertainty. Civ. Eng. Environ. Syst. 2000, 17, 95–118. [Google Scholar] [CrossRef]
- Niu, G.; Li, Y.P.; Huang, G.H.; Liu, J.; Fan, Y.R. Crop planning and water resource allocation for sustainable development of an irrigation region in China under multiple uncertainties. Agric. Water Manag. 2016, 166, 53–69. [Google Scholar] [CrossRef]
- Li, M.; Guo, P.; Singh, V.; Zhao, J. Irrigation water allocation using an inexact two-stage quadratic programming with fuzzy input under climate change. J. Am. Water Resour. Assoc. 2016. [Google Scholar] [CrossRef]
- Xin, X.; Huang, G.H.; Sun, W.; Zhou, Y. Factorial two-stage irrigation system optimization model. J. Irrig. Drain. Eng. 2016, 142. [Google Scholar] [CrossRef]
- Wang, B.; Cai, Y.; Yin, X.; Tan, Q.; Hao, Y. An integrated approach of system dynamics, orthogonal experimental design and inexact optimization for supporting water resources management under uncertainty. Water Resour. Manag. 2017, 31, 1665–1694. [Google Scholar] [CrossRef]
- Ahmed, S.; Tawarmalani, M.; Sahinidis, N.V. A finite branch-and-bound algorithm for two-stage stochastic integer programs. Math. Program. Ser. A 2004, 100, 355–377. [Google Scholar] [CrossRef]
- Huang, G.H. IPWM: An interval-parameter water quality management model. Eng. Optim. 1996, 26, 79–103. [Google Scholar] [CrossRef]
- Ji, L.; Huang, G.H.; Huang, L.C.; Xie, Y.L.; Niu, D.X. Inexact stochastic risk-aversion optimal day-ahead dispatch model for electricity system management with wind power under uncertainty. Energy 2016, 109, 920–932. [Google Scholar] [CrossRef]
- Zeng, X.; Li, Y.; Huang, G.H.; Yu, L.Y. Inexact mathematical modeling for the identification of water trading policy under uncertainty. Water 2014, 6, 229–252. [Google Scholar] [CrossRef]
- Tianjin Statistic Bureau. Tianjin Statistical Yearbook 2015; China Statistics Press: Beijing, China, 2016. [Google Scholar]
- Tianjin Municipality’s Water Conservancy Bureau. Tianjin Water Resources Bulletin 2015; Tianjin Municipality’s Water Conservancy Bureau: Tianjin, China, 2016. [Google Scholar]
- Tianjin Municipal Government. Tianjin General Planning (2015~2030); Tianjin Municipal Government: Tianjin, China, 2016.
- Xie, Y.L.; Huang, G.H.; Li, W.; Li, J.B.; Li, Y.F. An inexact two-stage stochastic programming model for water resources management in Nansihu Lake Basin, China. J. Environ. Manag. 2013, 127, 188–205. [Google Scholar] [CrossRef] [PubMed]
| Sectors | t = 1 | t = 2 | ||||
|---|---|---|---|---|---|---|
| Low | Medium | High | Low | Medium | High | |
| j = 1 | [5700, 5985] | [5900, 6195] | [6100, 6405] | [5800, 6090] | [6000, 6300] | [6300, 6615] |
| j = 2 | [2400, 2520] | [2500, 2625] | [2700, 2835] | [2500, 2625] | [2600, 2730] | [2800, 2940] |
| j = 3 | [2300, 2415] | [2450, 2573] | [2650, 2783] | [2400, 2520] | [2550, 2678] | [2750, 2888] |
| j = 4 | [1900, 1995] | [2100, 2205] | [2300, 2415] | [2000, 2100] | [2200, 2310] | [2400, 2520] |
| Sectors | Net Benefit for Water Consumption | Penalty Cost for Unsatisfied Demand | ||
|---|---|---|---|---|
| t = 1 | t = 2 | t = 1 | t = 2 | |
| j = 1 | [22.5, 23.5] | [23.5, 24.5] | [32.5, 33.5] | [36.5, 39.0] |
| j = 2 | [220, 225] | [248, 251] | [305, 310] | [322, 328] |
| j = 3 | [2.8, 3.0] | [3.5, 3.8] | [5.0, 6.2] | [5.5, 6.9] |
| j = 4 | [11.5, 12.0] | [13.2, 14.0] | [20.5, 22.0] | [23.8, 25.4] |
| Period | Water Resources | j = 1 | j = 2 | j = 3 | j = 4 |
|---|---|---|---|---|---|
| t = 1 | i = 1 | 3.80 | 0 | 0.40 | 0 |
| i = 2 | 1.50 | 0 | 0.40 | 0 | |
| i = 3 | 0.59 | 1.98 | 0.84 | 1.59 | |
| i = 4 | 0 | 0.12 | 0.11 | 0 | |
| i = 5 | 0 | 0.35 | 0.35 | 0.35 | |
| t = 2 | i = 1 | 3.99 | 0 | 0.41 | 0 |
| i = 2 | 1.12 | 0 | 0.48 | 0 | |
| i = 3 | 0.83 | 2.11 | 0.75 | 1.56 | |
| i = 4 | 0 | 0 | 0.06 | 0.07 | |
| i = 5 | 0 | 0.37 | 0.37 | 0.37 |
| t = 1 | t = 2 | |||||
|---|---|---|---|---|---|---|
| s = 1 | s = 2 | s = 3 | s = 1 | s = 2 | s = 3 | |
| Transferred water (i = 3) | ||||||
| j = 1 | [595, 680] | [595, 685] | 595 | [832, 917] | 832 | 832 |
| j = 2 | 1980 | 1980 | [1980, 2088] | 2110 | 2110 | [2110, 2260] |
| j = 3 | [837, 885] | [837, 1047] | [837, 840] | [748, 896] | [748, 998] | 748 |
| j = 4 | 1589 | 1589 | [1589, 1777] | 1559 | 1559 | [1559, 1660] |
| Sea water desalination (i = 4) | ||||||
| j = 1 | 0 | 0 | 0 | 0 | 0 | 0 |
| j = 2 | 119 | 119 | [119, 239] | 0 | 0 | 0 |
| j = 3 | 115 | 115 | [115, 229] | 59 | 59 | [59, 118] |
| j = 4 | 0 | 0 | 0 | 67 | 67 | 134 |
| Reused water (i = 5) | ||||||
| j = 1 | 0 | 0 | 0 | 0 | 0 | 0 |
| j = 2 | [350, 486] | [452, 593] | [537, 700] | [425, 568] | [527, 675] | [729, 740] |
| j = 3 | [610, 700] | [414, 700] | [350, 700] | 740 | 740 | [557, 740] |
| j = 4 | [350, 457] | [554, 673] | [590, 700] | [402, 517] | [604, 731] | 740 |
| Sectors | t = 1 | t = 2 | |||||
|---|---|---|---|---|---|---|---|
| s = 1 | s = 2 | s = 3 | s = 1 | s = 2 | s = 3 | ||
| Unsatisfied water demand (106 m3) | j = 1 | 0 | 0 | [0, 204.73] | 0 | 0 | 0 |
| j = 2 | 0 | 0 | 0 | 0 | 0 | 0 | |
| j = 3 | 0 | 0 | [59, 109.18] | 0 | 0 | 0 | |
| j = 4 | 0 | 0 | [0, 165.14] | 0 | 0 | [0, 37] | |
| Penalty cost (million RMB) | j = 1 | 0 | 0 | [0, 6654] | 0 | 0 | 0 |
| j = 2 | 0 | 0 | 0 | 0 | 0 | 0 | |
| j = 3 | 0 | 0 | [326, 546] | 0 | 0 | 0 | |
| j = 4 | 0 | 0 | [0, 3385] | 0 | 0 | [0, 951] | |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ji, L.; Sun, P.; Ma, Q.; Jiang, N.; Huang, G.-H.; Xie, Y.-L. Inexact Two-Stage Stochastic Programming for Water Resources Allocation under Considering Demand Uncertainties and Response—A Case Study of Tianjin, China. Water 2017, 9, 414. https://doi.org/10.3390/w9060414
Ji L, Sun P, Ma Q, Jiang N, Huang G-H, Xie Y-L. Inexact Two-Stage Stochastic Programming for Water Resources Allocation under Considering Demand Uncertainties and Response—A Case Study of Tianjin, China. Water. 2017; 9(6):414. https://doi.org/10.3390/w9060414
Chicago/Turabian StyleJi, Ling, Ping Sun, Qiang Ma, Na Jiang, Guo-He Huang, and Yu-Lei Xie. 2017. "Inexact Two-Stage Stochastic Programming for Water Resources Allocation under Considering Demand Uncertainties and Response—A Case Study of Tianjin, China" Water 9, no. 6: 414. https://doi.org/10.3390/w9060414
APA StyleJi, L., Sun, P., Ma, Q., Jiang, N., Huang, G.-H., & Xie, Y.-L. (2017). Inexact Two-Stage Stochastic Programming for Water Resources Allocation under Considering Demand Uncertainties and Response—A Case Study of Tianjin, China. Water, 9(6), 414. https://doi.org/10.3390/w9060414








