Analysis of Unsteady Internal Flow and Its Induced Structural Response in a Circulating Water Pump
Abstract
1. Introduction
2. Numerical Simulation Methods
2.1. Governing Equation
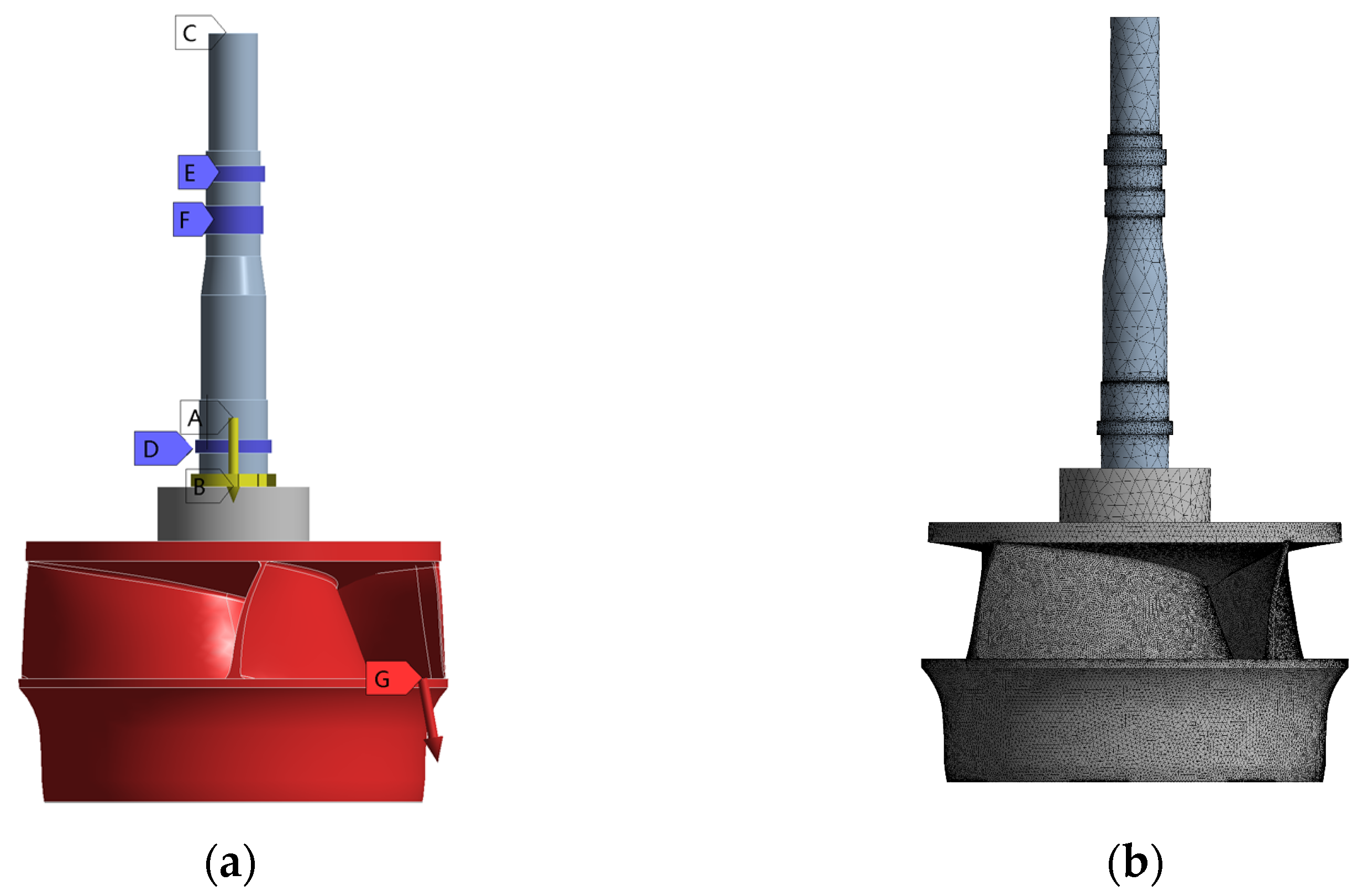
2.2. Research Model
2.3. Numerical Simulation
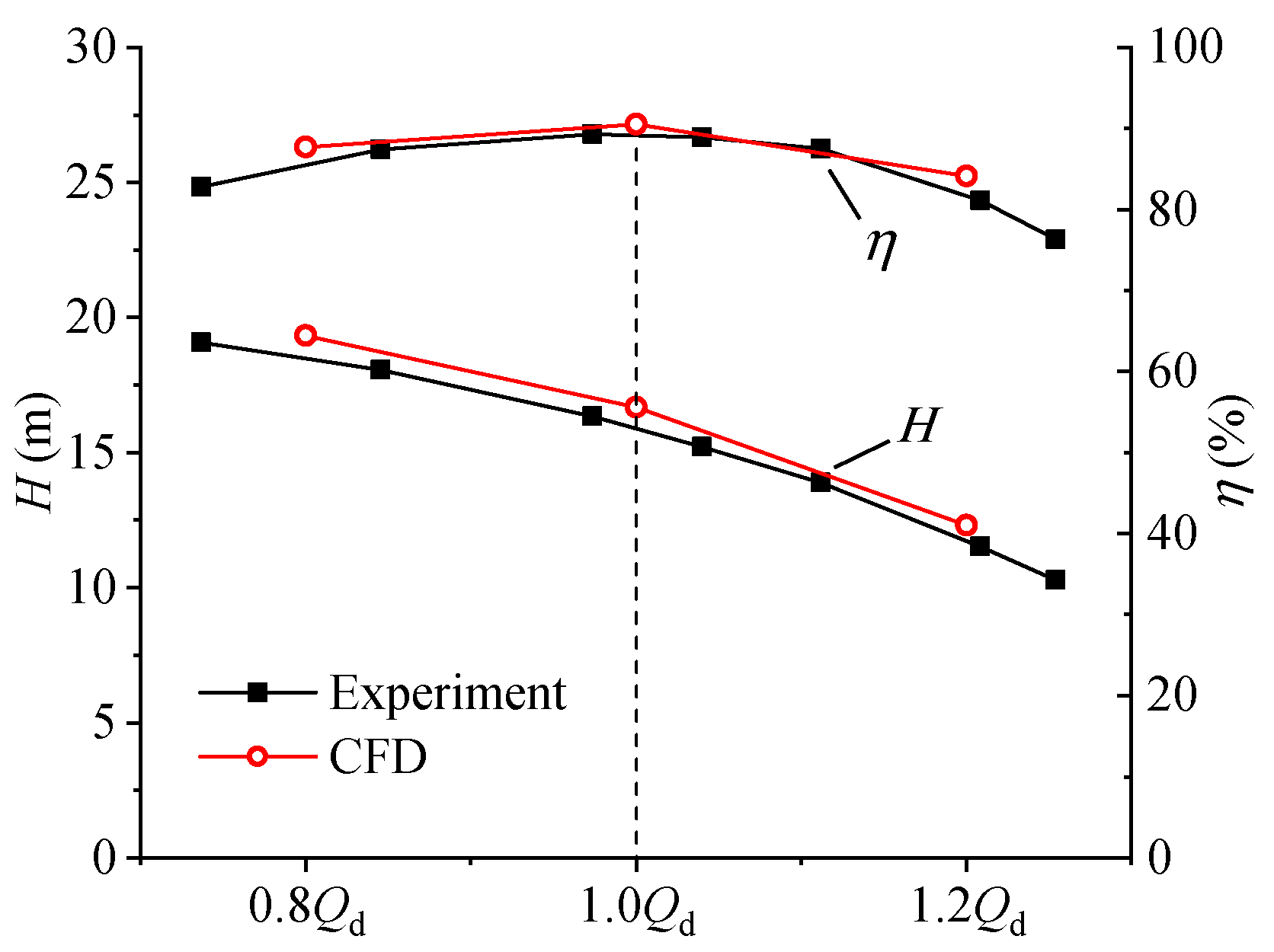
2.4. Experimental Verification
3. Internal Flow Analysis
3.1. Unsteady Flow
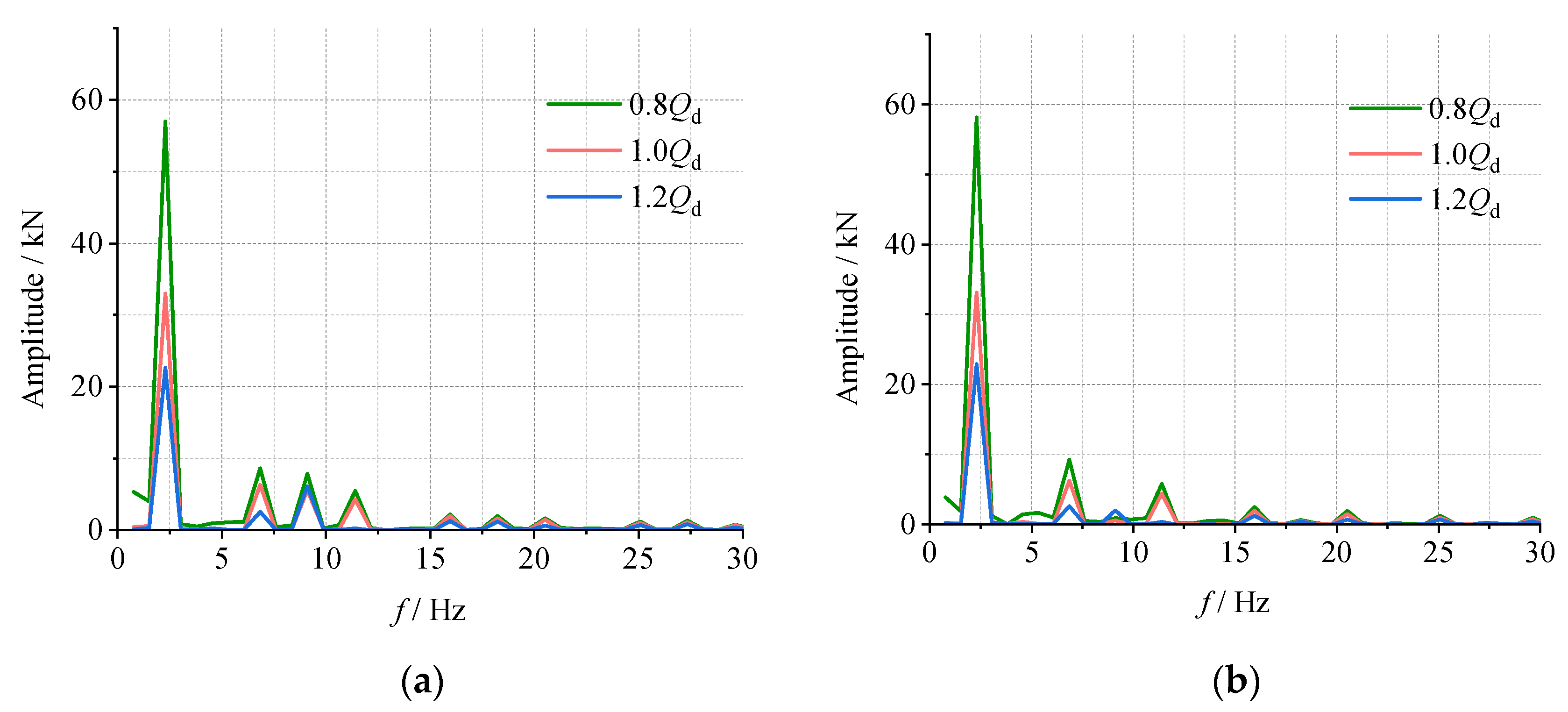
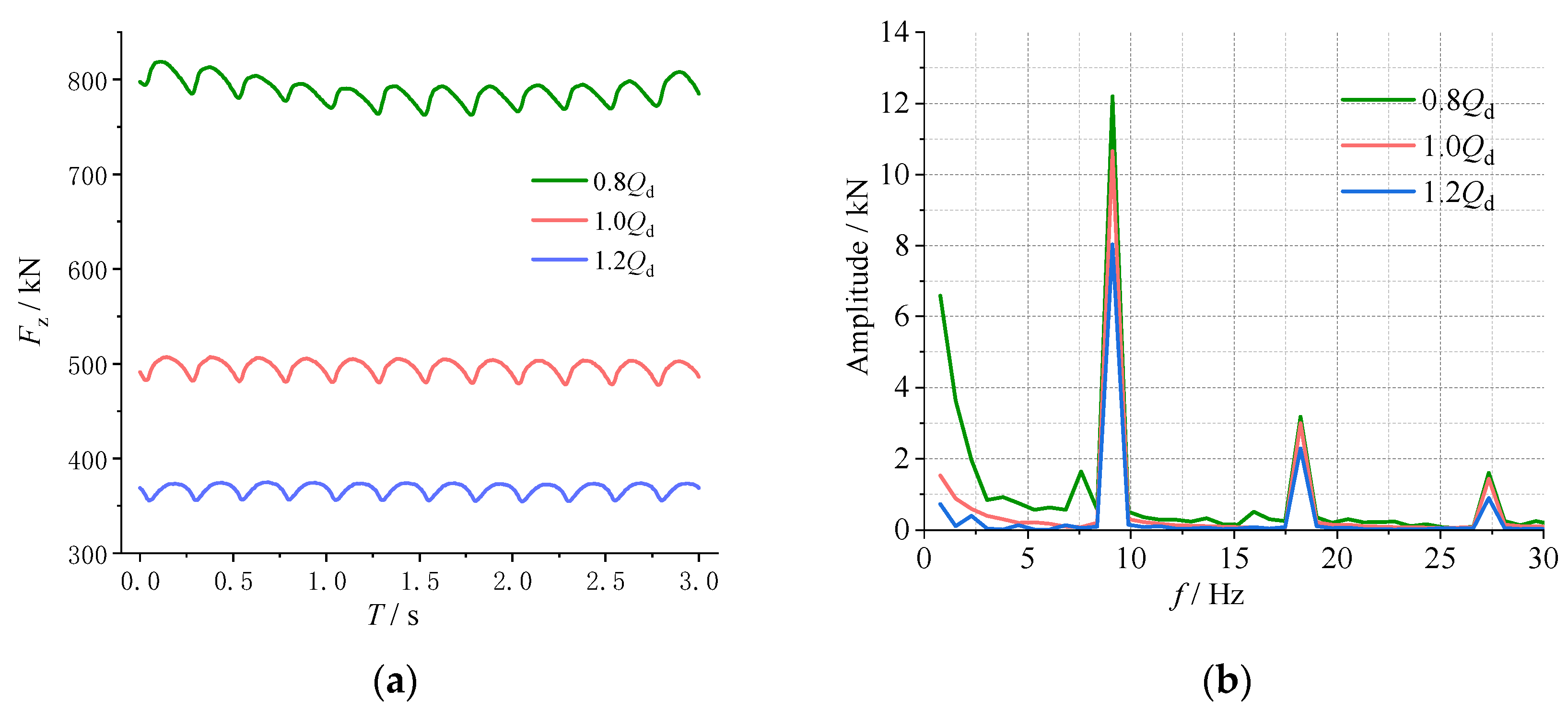
3.2. Unsteady Flow-Induced Forces
4. Structural Response Analysis of Rotation System
4.1. Finite Element Method
4.2. Modal Analysis
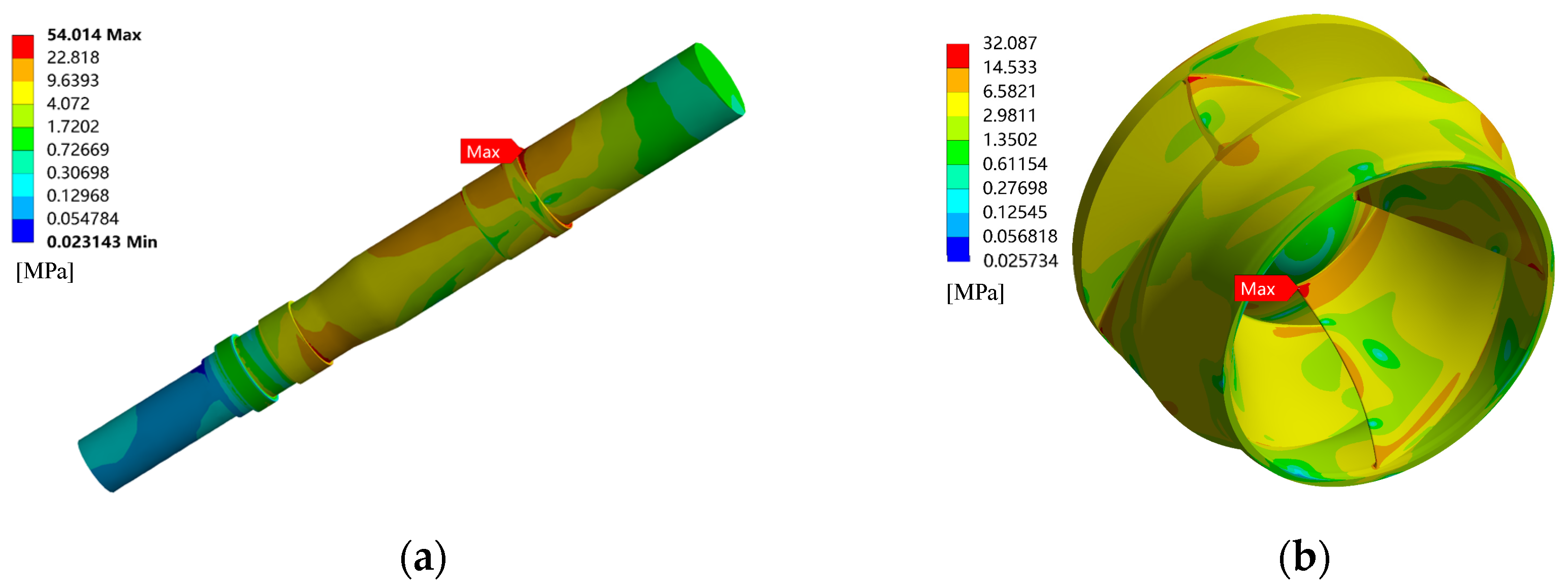
4.3. Stress Analysis
5. Conclusions
- (1)
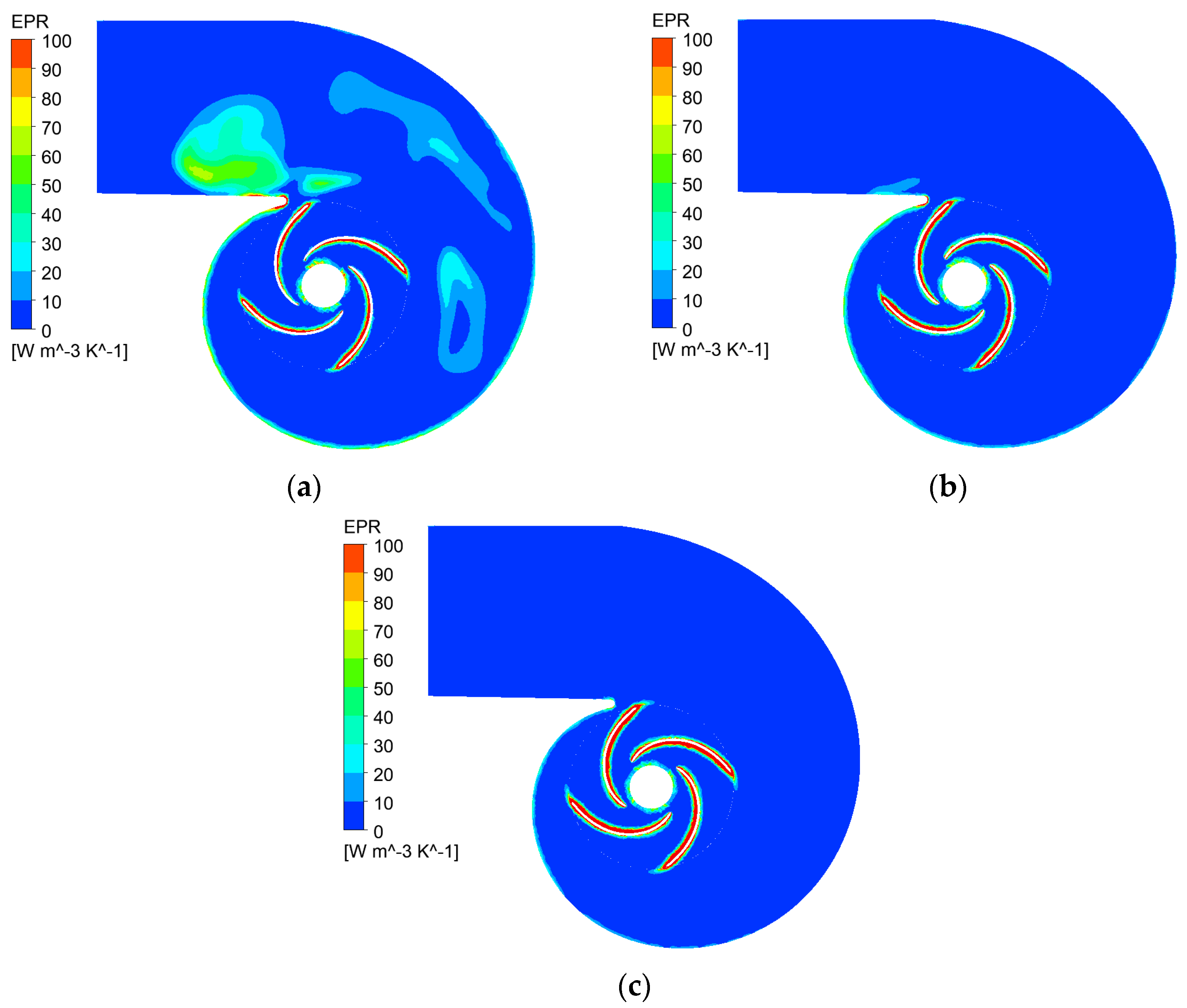
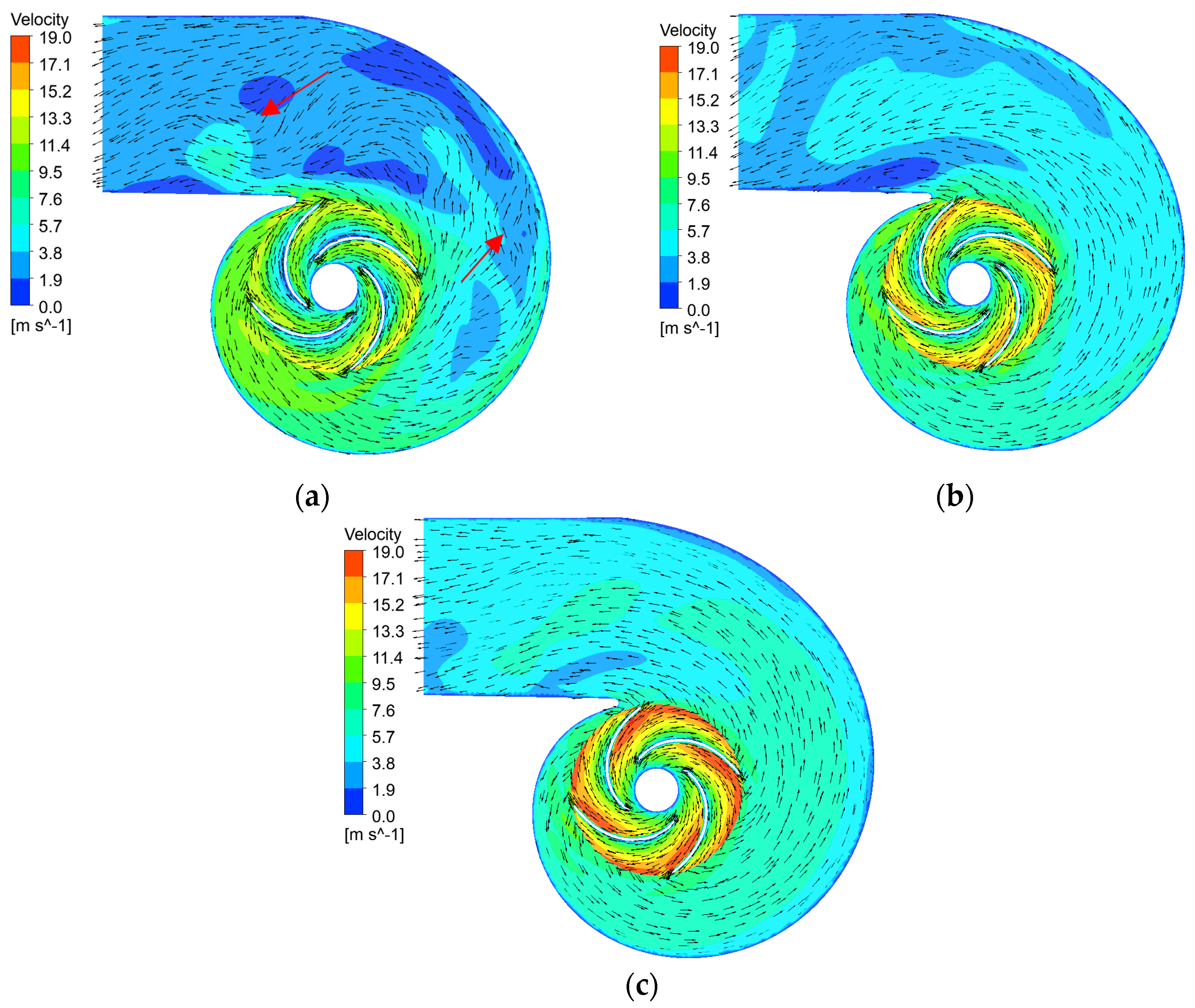
- The flow rate has a significant impact on the ratio of energy loss of the CPW. Under the part-load condition, the volute has severe energy loss due to the unsteady flow, while under the over-load condition, the impeller suffers from significant flow losses due to large velocity gradients. Additionally, the asymmetrical pressure distribution within the volute is a primary factor in causing radial force imbalance.
- (2)
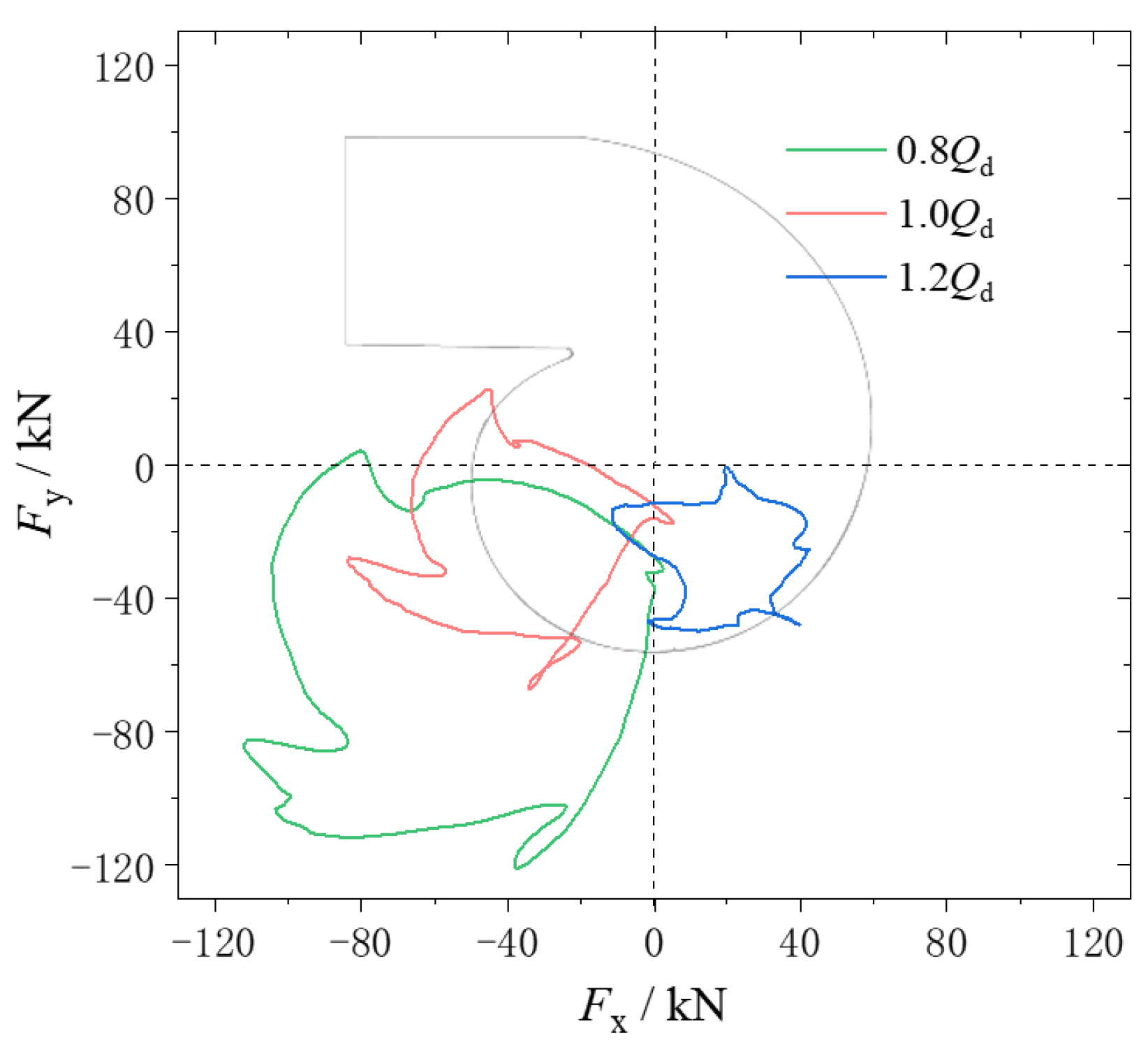
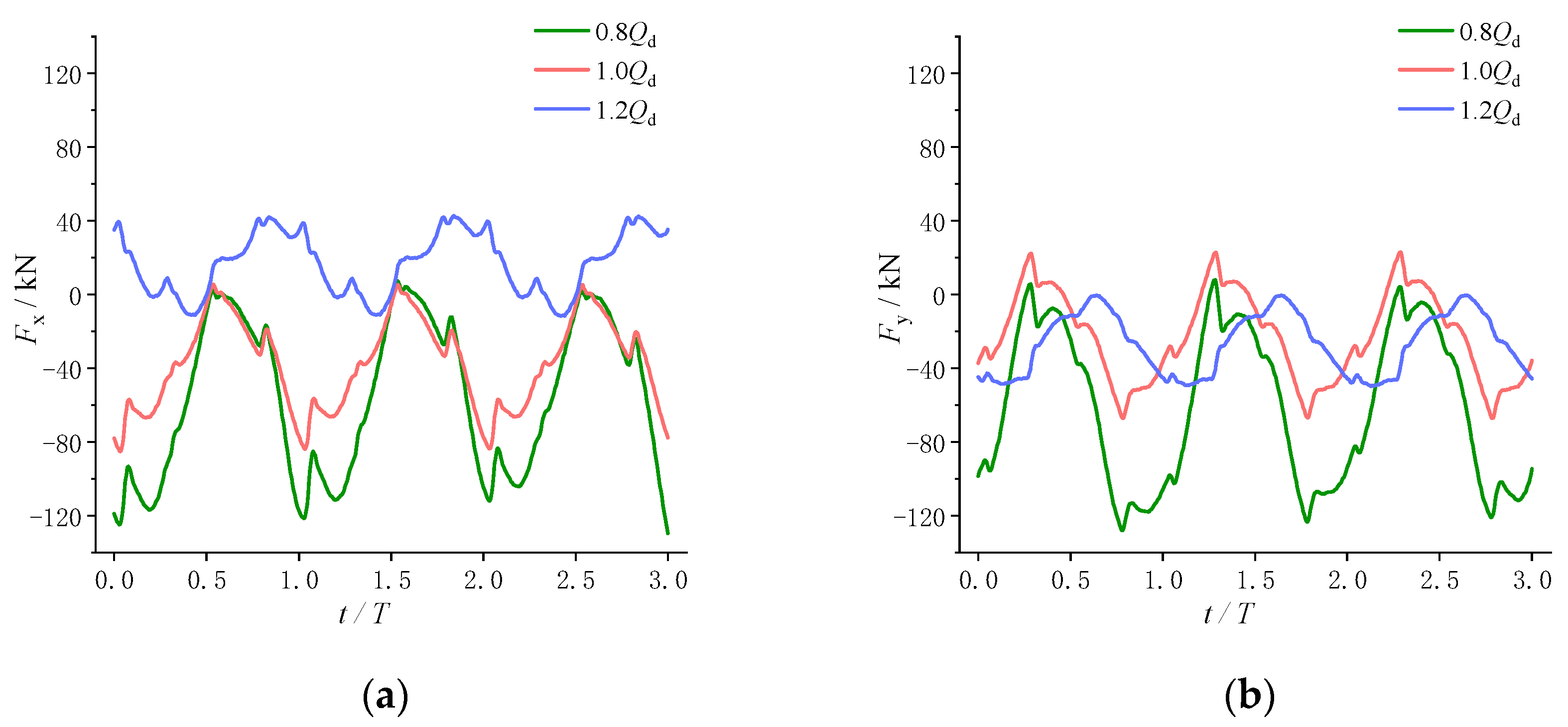
- The average radial force of the CWP under all conditions is lower than the axial force, but the fluctuation amplitude of the radial force is significantly higher. As the flow rate increases, both the radial and axial forces of the CWP show a decreasing trend. Therefore, increasing the flow rate is an effective approach for enhancing the stability of the CWP.
- (3)
- The FEM results indicate that the main force position on the rotation system is at the bearing and the root of the impeller blade leading edge. Additionally, the maximum stress position of the pump shaft varies at different flow rates, with a close relationship between the ratio of the radial force and the axial force.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Danish; Ulucak, R.; Erdogan, S. The effect of nuclear energy on the environment in the context of globalization: Consumption vs production-based CO2 emissions. Nucl. Eng. Technol. 2022, 54, 1312–1320. [Google Scholar] [CrossRef]
- Mahmood, N.; Wang, Z.; Zhang, B. The role of nuclear energy in the correction of environmental pollution: Evidence from Pakistan. Nucl. Eng. Technol. 2020, 52, 1327–1333. [Google Scholar] [CrossRef]
- Mathew, M.D. Nuclear energy: A pathway towards mitigation of global warming. Prog. Nucl. Energy 2022, 143, 104080. [Google Scholar] [CrossRef]
- Xia, L.; Liu, D.; Zhou, L.; Wang, F.; Wang, P. Optimal number of circulating water pumps in a nuclear power plant. Nucl. Eng. Des. 2015, 288, 35–41. [Google Scholar] [CrossRef]
- Ma, Y.Y.; Yan, S.; Yang, Z.G.; Qi, G.S.; He, X.Y. Failure analysis on circulating water pump of duplex stainless steel in 1000 MW ultra-supercritical thermal power unit. Eng. Fail. Anal. 2015, 47, 162–177. [Google Scholar] [CrossRef]
- Bing, H.; Tan, L.; Cao, S.; Lu, L. Prediction method of impeller performance and analysis of loss mechanism for mixed-flow pump. Sci. China Technol. Sci. 2012, 55, 1988–1998. [Google Scholar] [CrossRef]
- Wu, X.F.; Tian, X.; Tan, M.G.; Liu, H.L. Multi-Parameter Optimization and Analysis on Performance of a Mixed Flow Pump. J. Appl. Fluid Mech. 2020, 13, 199–209. [Google Scholar] [CrossRef]
- Bing, H.; Cao, S.; Tan, L.; Zhu, B. Effects of meridional flow passage shape on hydraulic performance of mixed-flow pump impellers. Chin. J. Mech. Eng. 2013, 26, 469–475. [Google Scholar] [CrossRef]
- Lei, T.; Zhiyi, Y.; Yun, X.; Yabin, L.; Shuliang, C. Role of blade rotational angle on energy performance and pressure fluctuation of a mixed-flow pump. Proc. Inst. Mech. Eng. Part A J. Power Energy 2017, 231, 227–238. [Google Scholar] [CrossRef]
- Ji, L.; Li, W.; Shi, W.; Tian, F.; Agarwal, R. Effect of blade thickness on rotating stall of mixed-flow pump using entropy generation analysis. Energy 2021, 236, 121381. [Google Scholar] [CrossRef]
- Kim, S.; Kim, Y.I.; Kim, J.H.; Choi, Y.S. Three-objective optimization of a mixed-flow pump impeller for improved suction performance and efficiency. Adv. Mech. Eng. 2019, 11, 1687814019898969. [Google Scholar] [CrossRef]
- Wang, M.; Li, Y.; Yuan, J.; Meng, F.; Appiah, D.; Chen, J. Comprehensive Improvement of Mixed-Flow Pump Impeller Based on Multi-Objective Optimization. Processes 2020, 8, 905. [Google Scholar] [CrossRef]
- Zhang, W.; Yu, Z.Y.; Zhu, B.S. Numerical Study of Pressure Fluctuation in a Gas- Liquid Two-Phase Mixed-Flow Pump. Energies 2017, 10, 634. [Google Scholar] [CrossRef]
- Zheng, Y.; Chen, Y.; Mao, X.; Wang, H.; Shi, W.; Kan, K.; Zhang, Y. Pressure pulsation characteristics and its impact on flow-induced noise in mixed-flow pump. Trans. Chin. Soc. Agric. Eng. 2015, 31, 67–73. [Google Scholar]
- Shen, X.; Zhang, D.; Xu, B.; Zhao, R.; Jin, Y.; Chen, J.; Shi, W. Numerical and experimental investigation of the pressure fluctuation in a mixed-flow pump under low flow conditions. Proc. Inst. Mech. Eng. Part A J. Power Energy 2020, 234, 46–57. [Google Scholar] [CrossRef]
- Zhang, H.; Meng, F.; Zheng, Y.; Li, Y. The Cavitation-Induced Pressure Fluctuations in a Mixed-Flow Pump under Impeller Inflow Distortion. Machines 2021, 9, 326. [Google Scholar] [CrossRef]
- Van Esch, B.P.M. Performance and Radial Loading of a Mixed-Flow Pump Under Non-Uniform Suction Flow. J. Fluids Eng. 2009, 131, 051101. [Google Scholar] [CrossRef]
- Hao, Y.; Tan, L. Symmetrical and unsymmetrical tip clearances on cavitation performance and radial force of a mixed flow pump as turbine at pump mode. Renew. Energy 2018, 127, 368–376. [Google Scholar] [CrossRef]
- Li, Y.; Sun, D.; Meng, F.; Zheng, Y.; Zhong, Y. Study Regarding the Influence of Blade Rotation Angle Deviations on the Hydraulic Pulsation Characteristics of a Mixed-Flow Pump. J. Mar. Sci. Eng. 2023, 11, 530. [Google Scholar] [CrossRef]
- Shah, S.R.; Jain, S.V.; Patel, R.N.; Lakhera, V.J. CFD for Centrifugal Pumps: A Review of the State-of-the-Art. Procedia Eng. 2013, 51, 715–720. [Google Scholar] [CrossRef]
- Shi, L.; Zhang, W.; Jiao, H.; Tang, F.; Wang, L.; Sun, D.; Shi, W. Numerical simulation and experimental study on the comparison of the hydraulic characteristics of an axial-flow pump and a full tubular pump. Renew. Energy 2020, 153, 1455–1464. [Google Scholar] [CrossRef]
- Al-Azawy, M.G.; Turan, A.; Revell, A. Assessment of turbulence models for pulsatile flow inside a heart pump. Comput. Methods Biomech. Biomed. Eng. 2016, 19, 271–285. [Google Scholar] [CrossRef] [PubMed]
- Fu, Q.; Yang, D.; Zhang, J.; Zhu, R.; Shi, W. Study on Unsteady Flow Characteristics of Cooling Water Pump for Nuclear Power Plant Equipment under Low Flow Rate Conditions. Water 2023, 15, 3780. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Xie, C.; Zhang, C.; Fu, T.; Zhang, T.; Feng, A.; Jin, Y. Numerical Analysis and Model Test Verification of Energy and Cavitation Characteristics of Axial Flow Pumps. Water 2022, 14, 2853. [Google Scholar] [CrossRef]
- Chen, K.; Zhang, F.; Liu, R.; Adu-Pokua, K.A.; Yuan, S.; Hong, Q. Experimental and Numerical Evaluation of Affinity Law of Single-Stage and Multistage Side Channel Pumps at Variable Rotating Speeds. J. Fluids Eng. 2023, 145, 101201. [Google Scholar] [CrossRef]
- Chang, L.; Yang, C.; Su, X.; Dai, X.; Xu, Q.; Guo, L. Investigations on affinity law under gas–liquid conditions in multistage radial and mixed-flow multiphase pumps. Int. J. Fluid Eng. 2024, 1, 013503. [Google Scholar] [CrossRef]
- Zhou, L.; Hang, J.; Bai, L.; Krzemianowski, Z.; El-Emam, M.A.; Yasser, E.; Agarwal, R. Application of entropy production theory for energy losses and other investigation in pumps and turbines: A review. Appl. Energy 2022, 318, 119211. [Google Scholar] [CrossRef]
- Wu, D.; Zhu, Z.; Ren, Y.; Gu, Y.; Zhou, P. Influence of blade profile on energy loss of sewage self-priming pump. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 470. [Google Scholar] [CrossRef]
- Hou, H.; Zhang, Y.; Zhou, X.; Zuo, Z.; Chen, H. Optimal hydraulic design of an ultra-low specific speed centrifugal pump based on the local entropy production theory. Proc. Inst. Mech. Eng. Part A J. Power Energy 2019, 233, 715–726. [Google Scholar] [CrossRef]
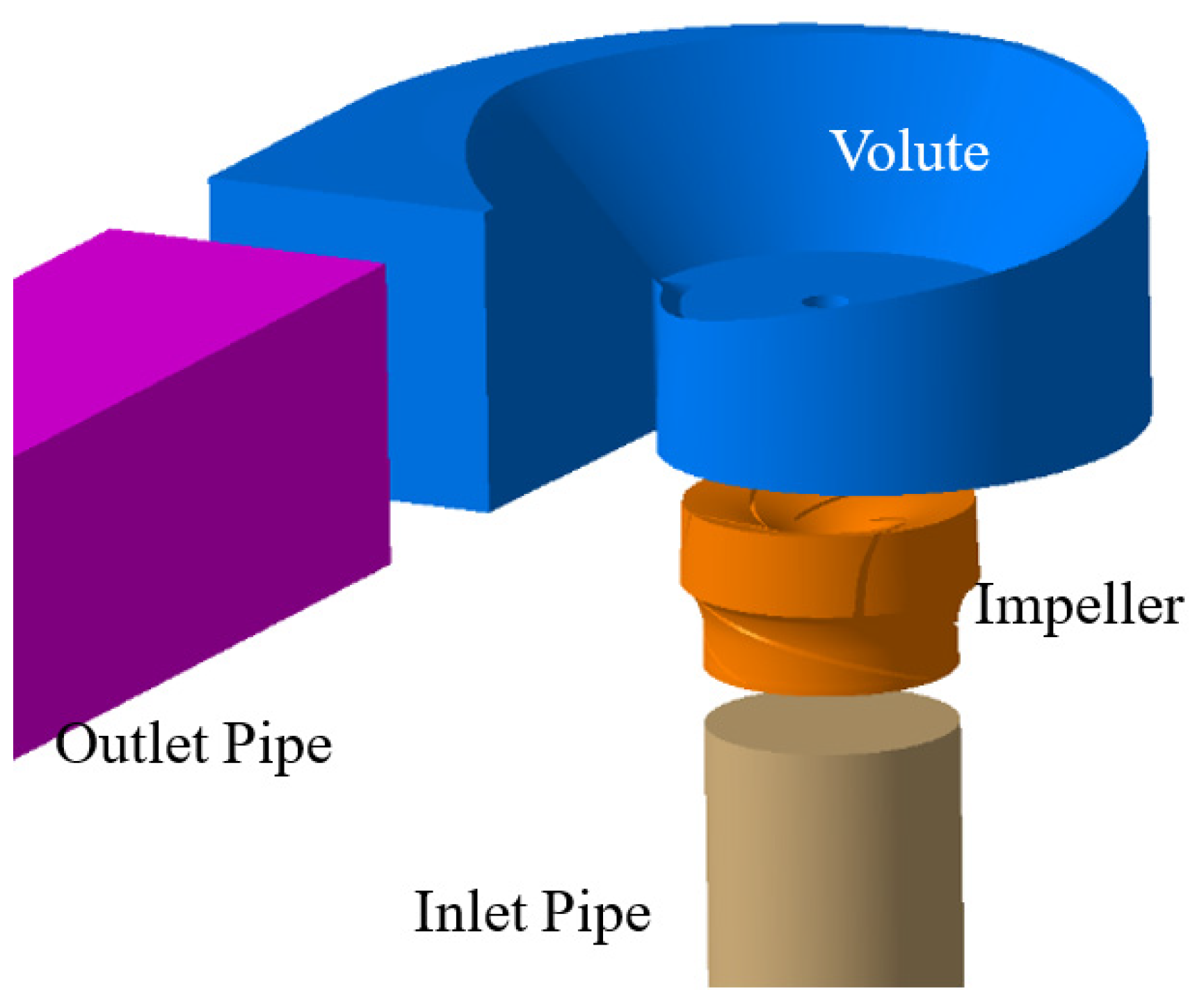
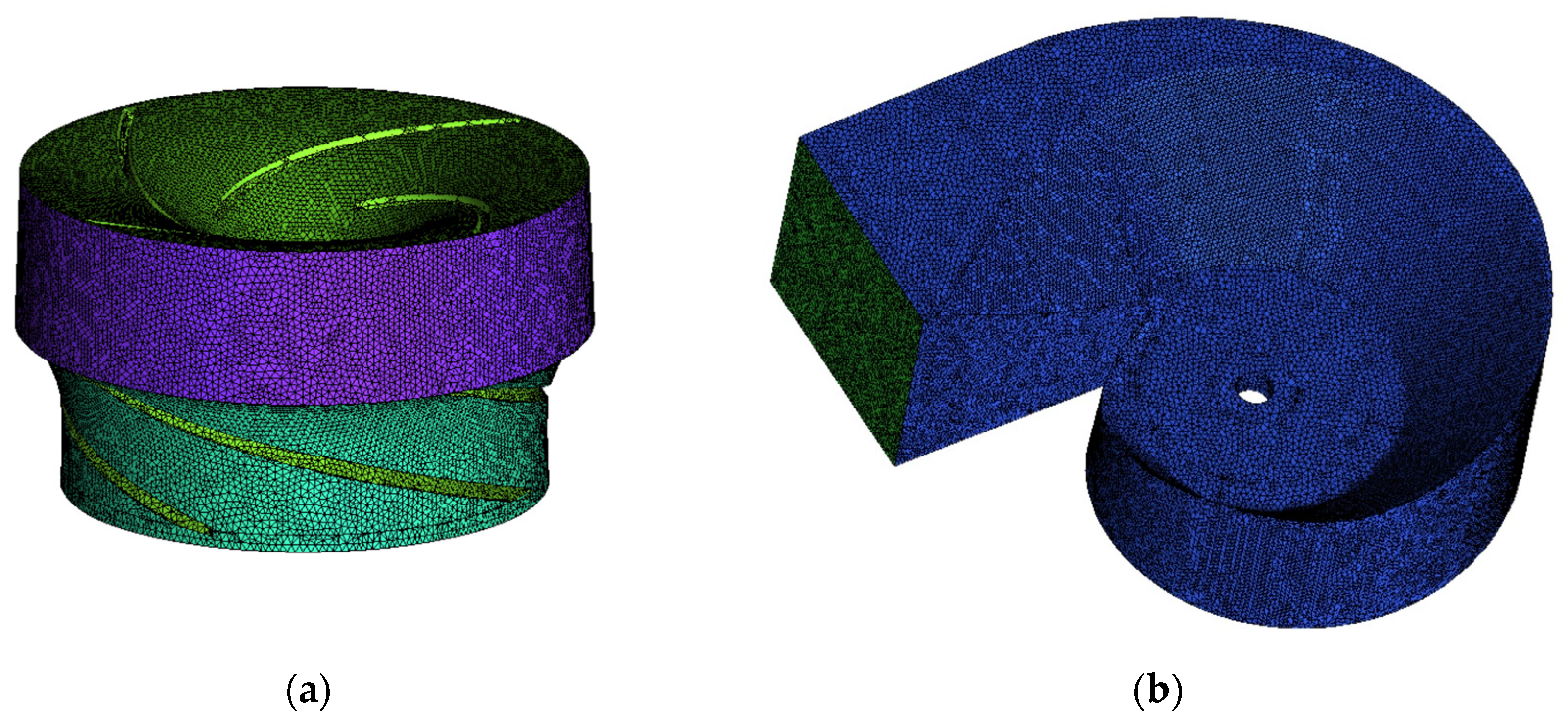
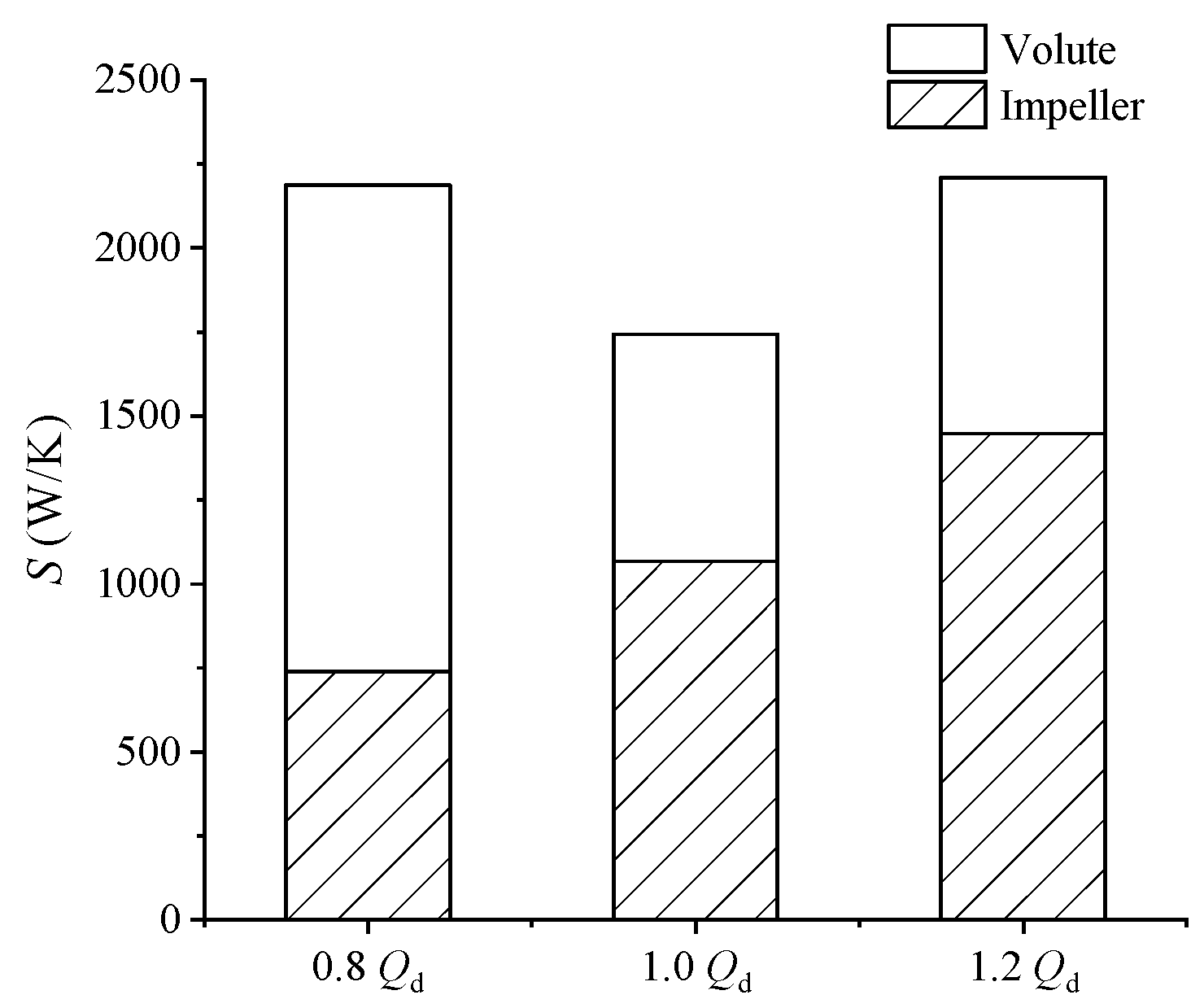




| NO. | Total Grid Number | Impeller Grid Number | Volute Grid Number | Head/m |
|---|---|---|---|---|
| 1 | 4,067,240 | 1,251,821 | 1,808,665 | 17.18 |
| 2 | 7,084,856 | 2,616,115 | 3,061,987 | 16.67 |
| 3 | 10,834,149 | 3,635,343 | 5,702,062 | 16.59 |
| Order | Natural Frequency/Hz | Critical Speed/rpm |
|---|---|---|
| 1 | 25.3 | 1379.6 |
| 2 | 26.5 | 2072.9 |
| 3 | 36.0 | 2160.4 |
| 4 | 74.4 | 3596.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, J.; Yao, X.; Zheng, H.; Yan, X.; Liu, H.; Wu, T. Analysis of Unsteady Internal Flow and Its Induced Structural Response in a Circulating Water Pump. Water 2024, 16, 1294. https://doi.org/10.3390/w16091294
Lu J, Yao X, Zheng H, Yan X, Liu H, Wu T. Analysis of Unsteady Internal Flow and Its Induced Structural Response in a Circulating Water Pump. Water. 2024; 16(9):1294. https://doi.org/10.3390/w16091294
Chicago/Turabian StyleLu, Jinqi, Xueliang Yao, Haixia Zheng, Xiaowei Yan, Houlin Liu, and Tianxin Wu. 2024. "Analysis of Unsteady Internal Flow and Its Induced Structural Response in a Circulating Water Pump" Water 16, no. 9: 1294. https://doi.org/10.3390/w16091294
APA StyleLu, J., Yao, X., Zheng, H., Yan, X., Liu, H., & Wu, T. (2024). Analysis of Unsteady Internal Flow and Its Induced Structural Response in a Circulating Water Pump. Water, 16(9), 1294. https://doi.org/10.3390/w16091294





