Comprehension of Seismic-Induced Groundwater Level Rise in Unsaturated Sandy Layer Based on Soil–Water–Air Coupled Finite Deformation Analysis
Abstract
1. Introduction
2. Analysis Conditions
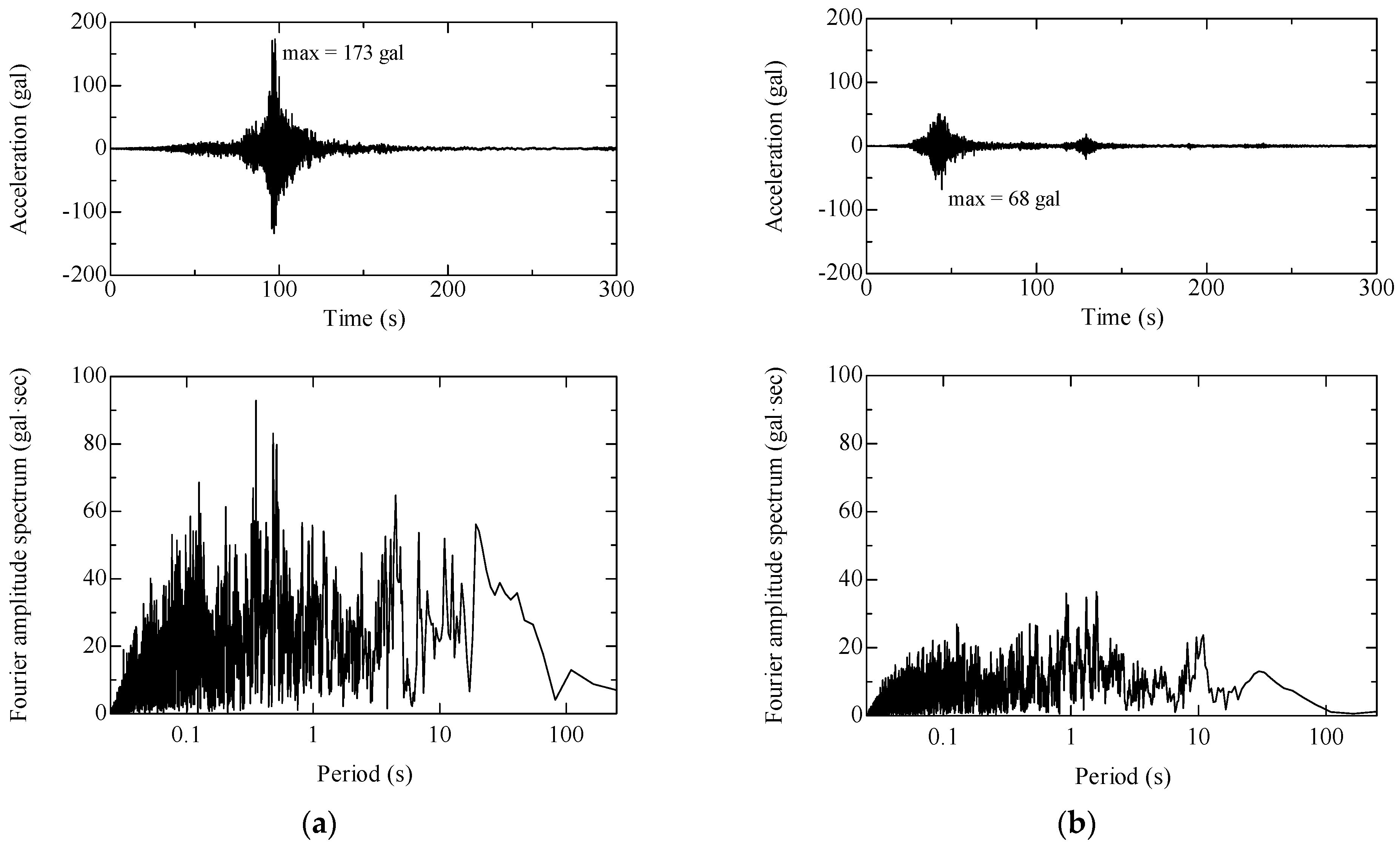
3. Results and Discussion
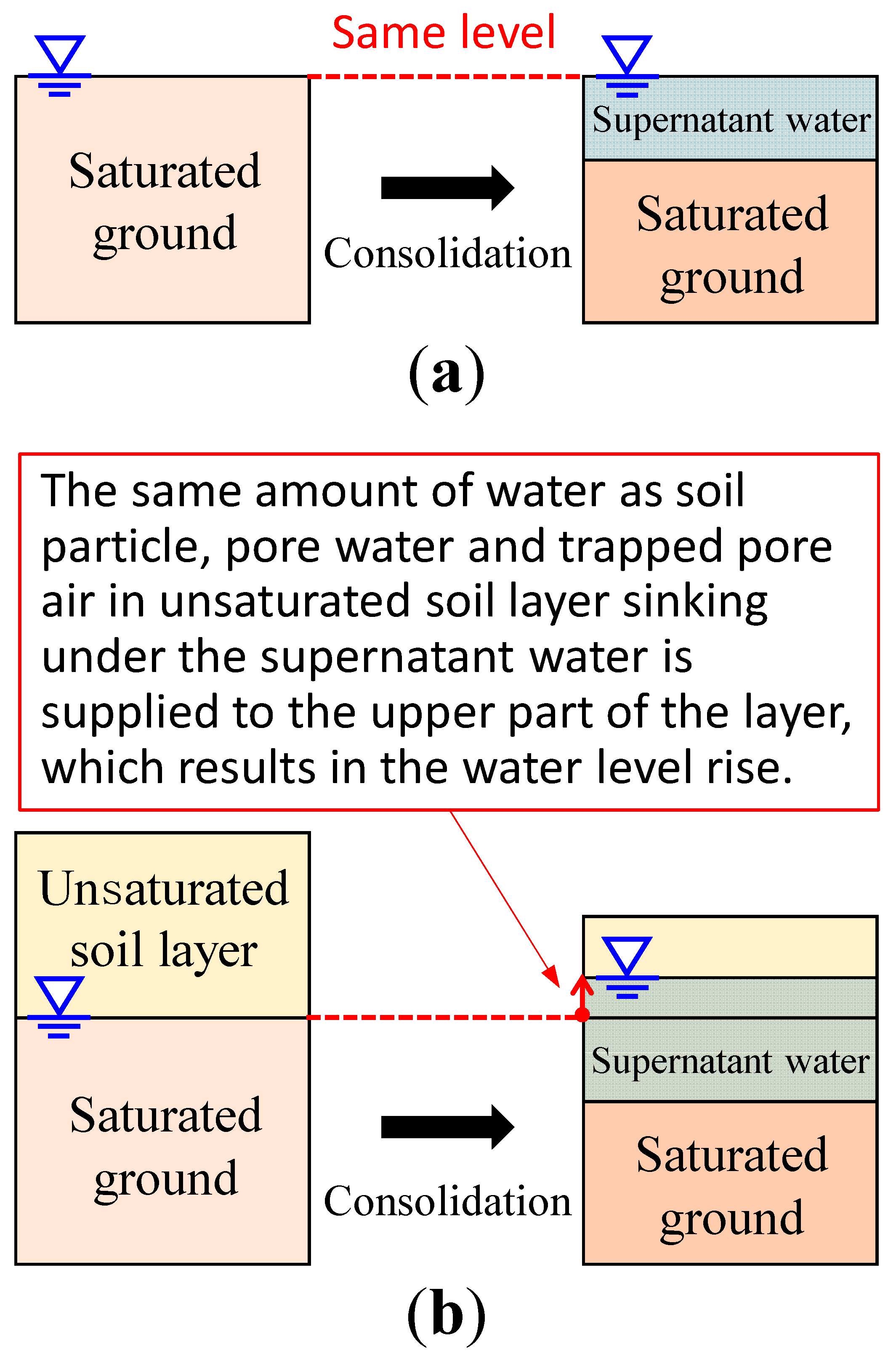
4. Case Studies on Mechanism of Groundwater Level Rise
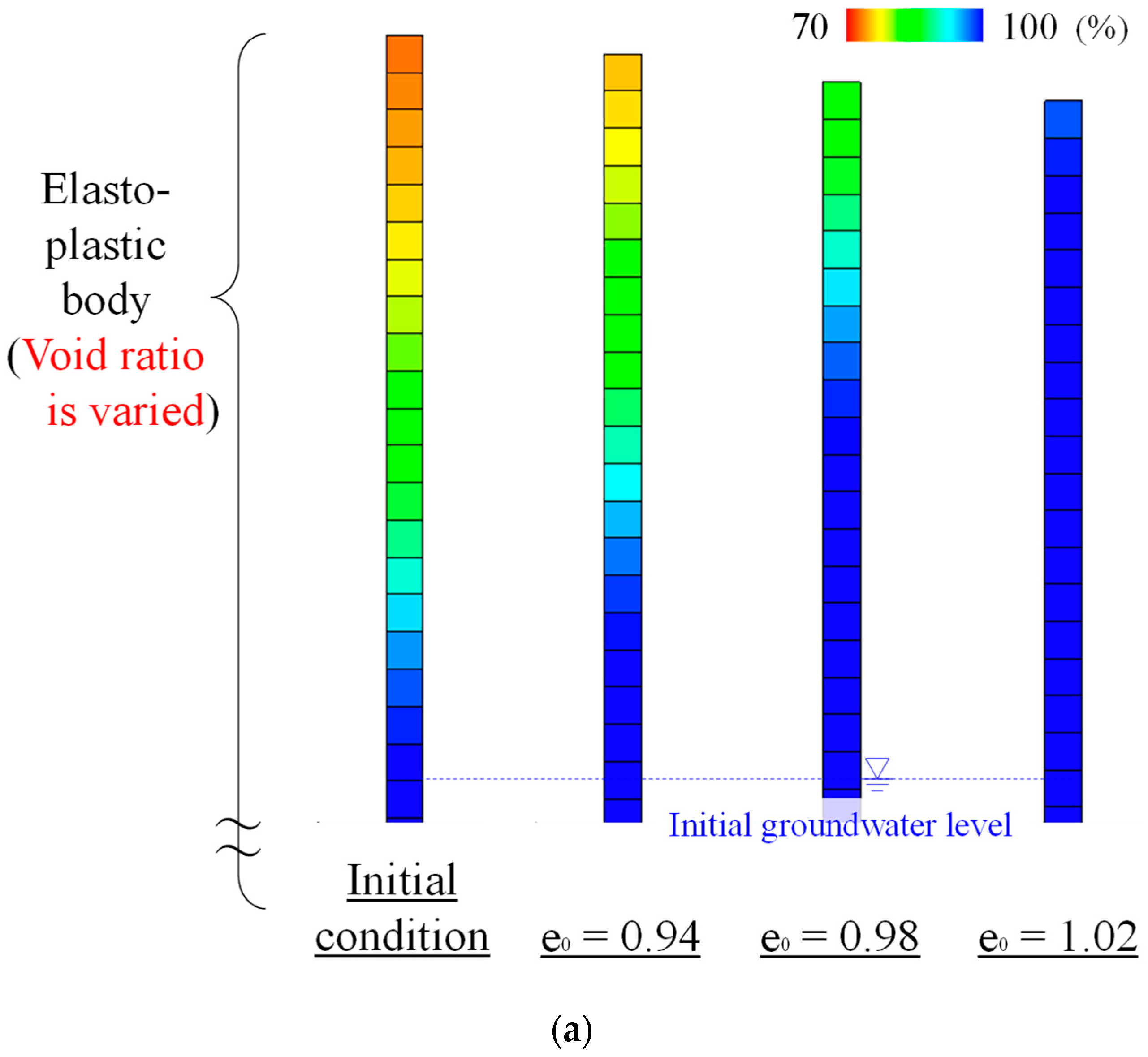
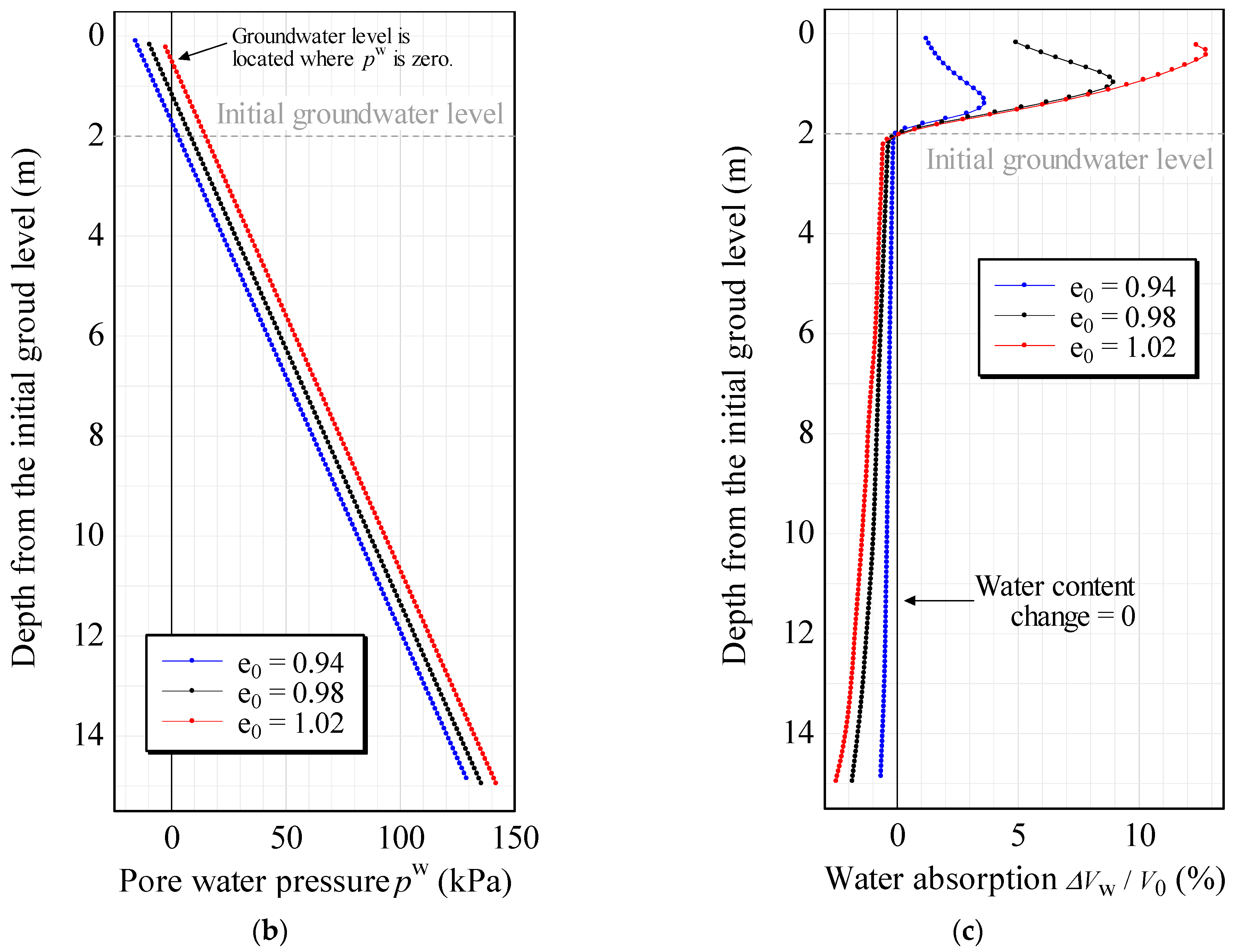
4.1. Case Where Void Ratio of the Entire Sand Layer Varies
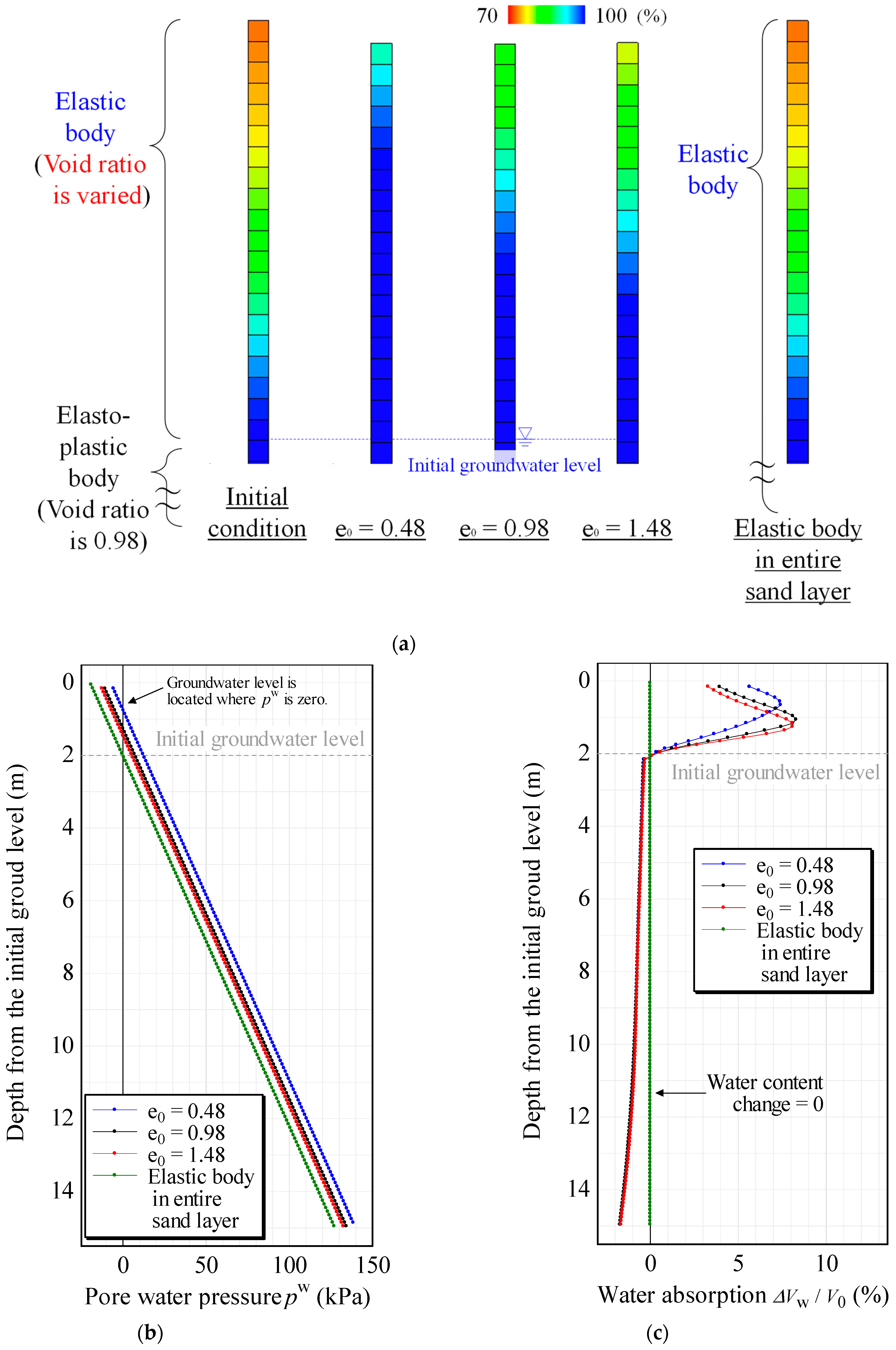
4.2. Case Where Void Ratio of the Unsaturated Sand Layer Modeled as Elastic Body Varies
5. Conclusions
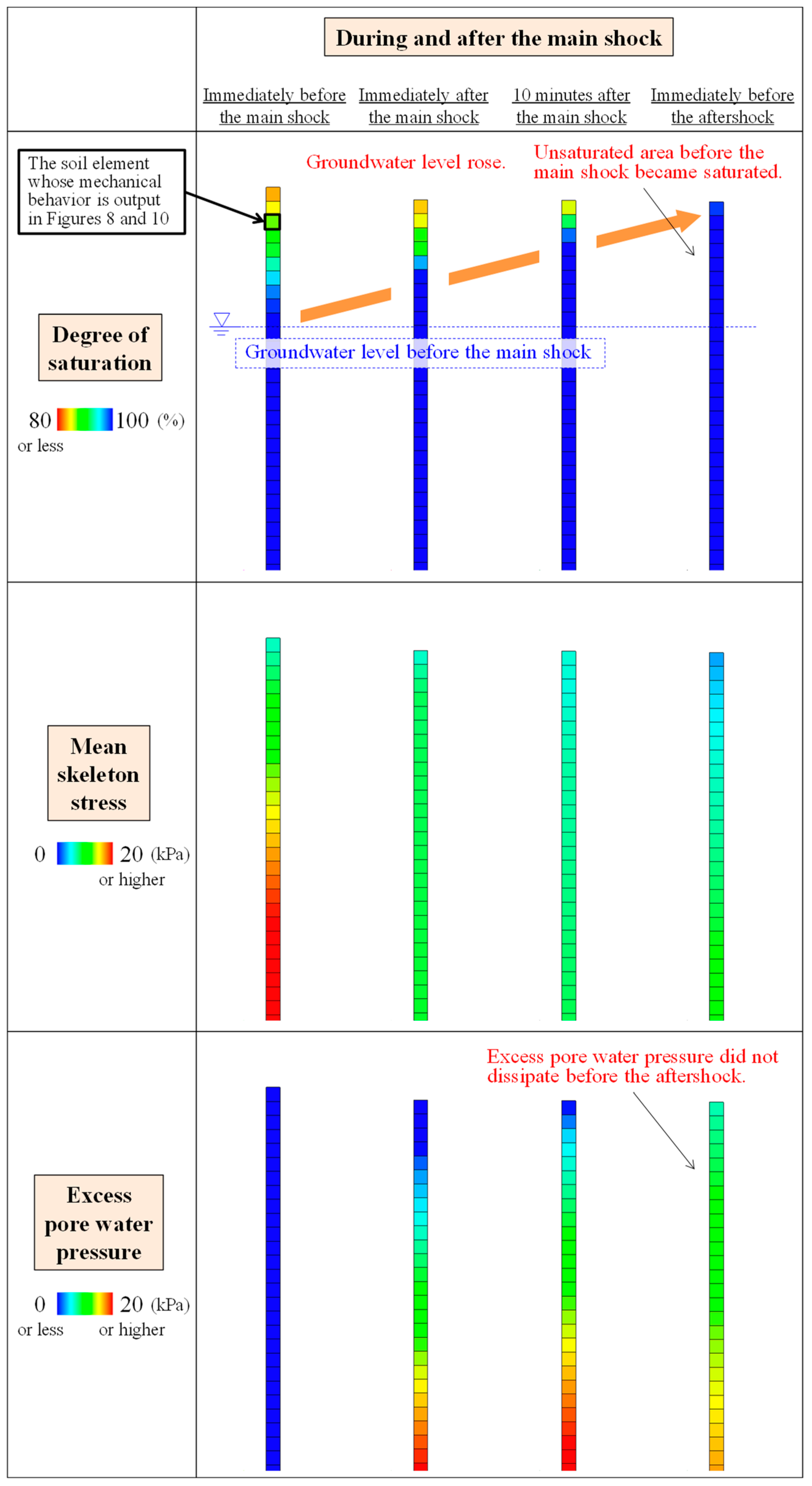
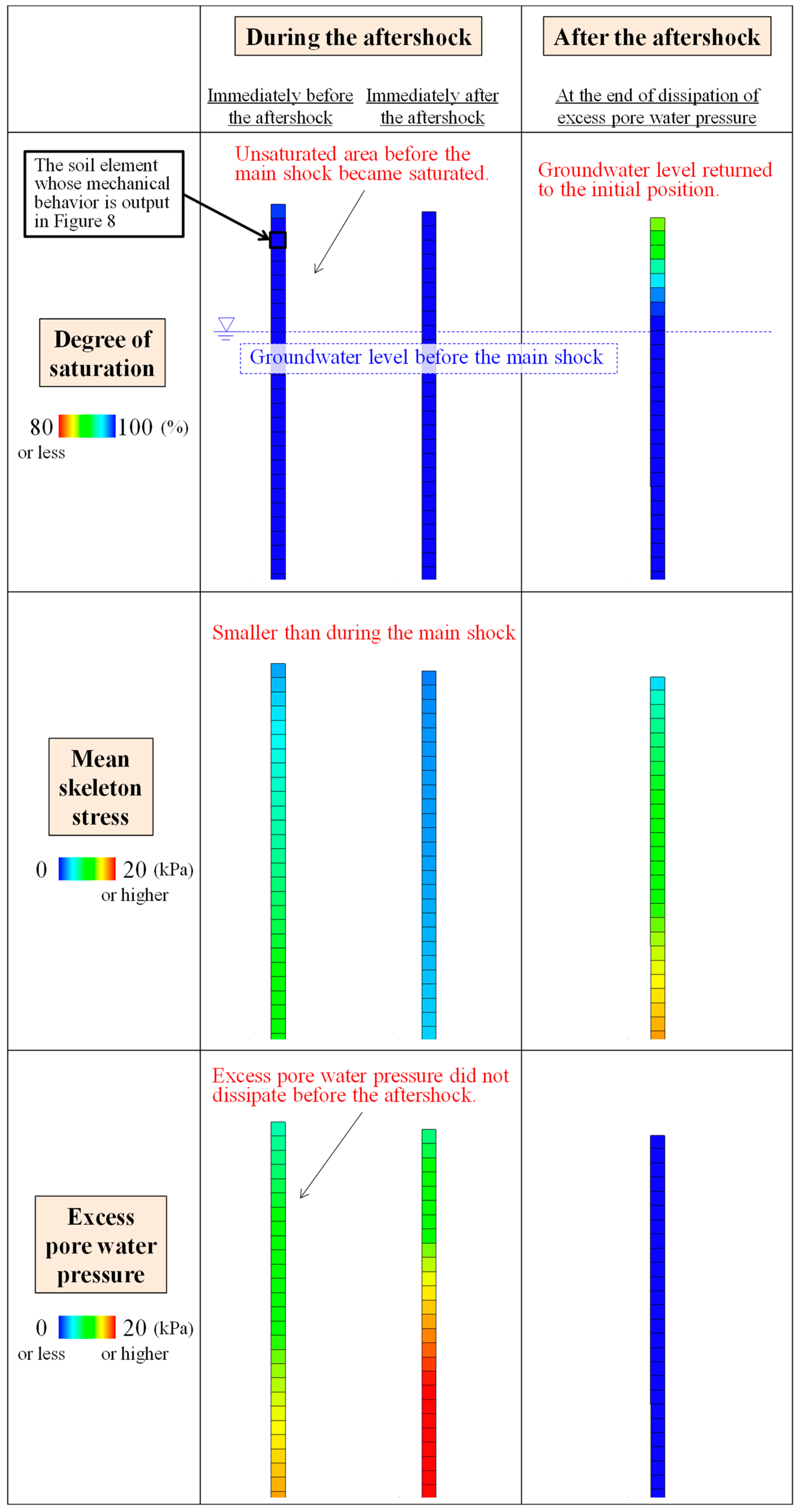
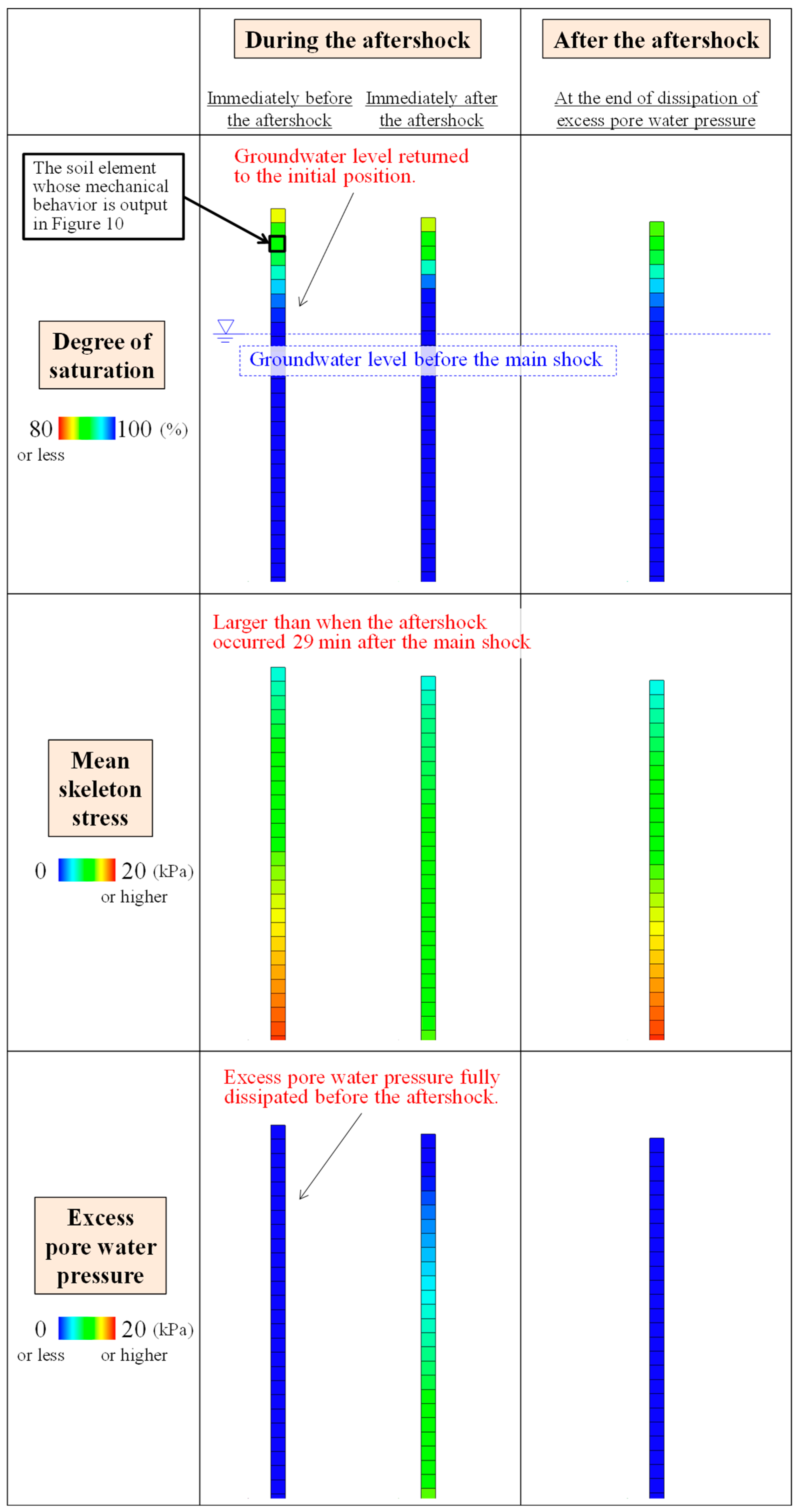
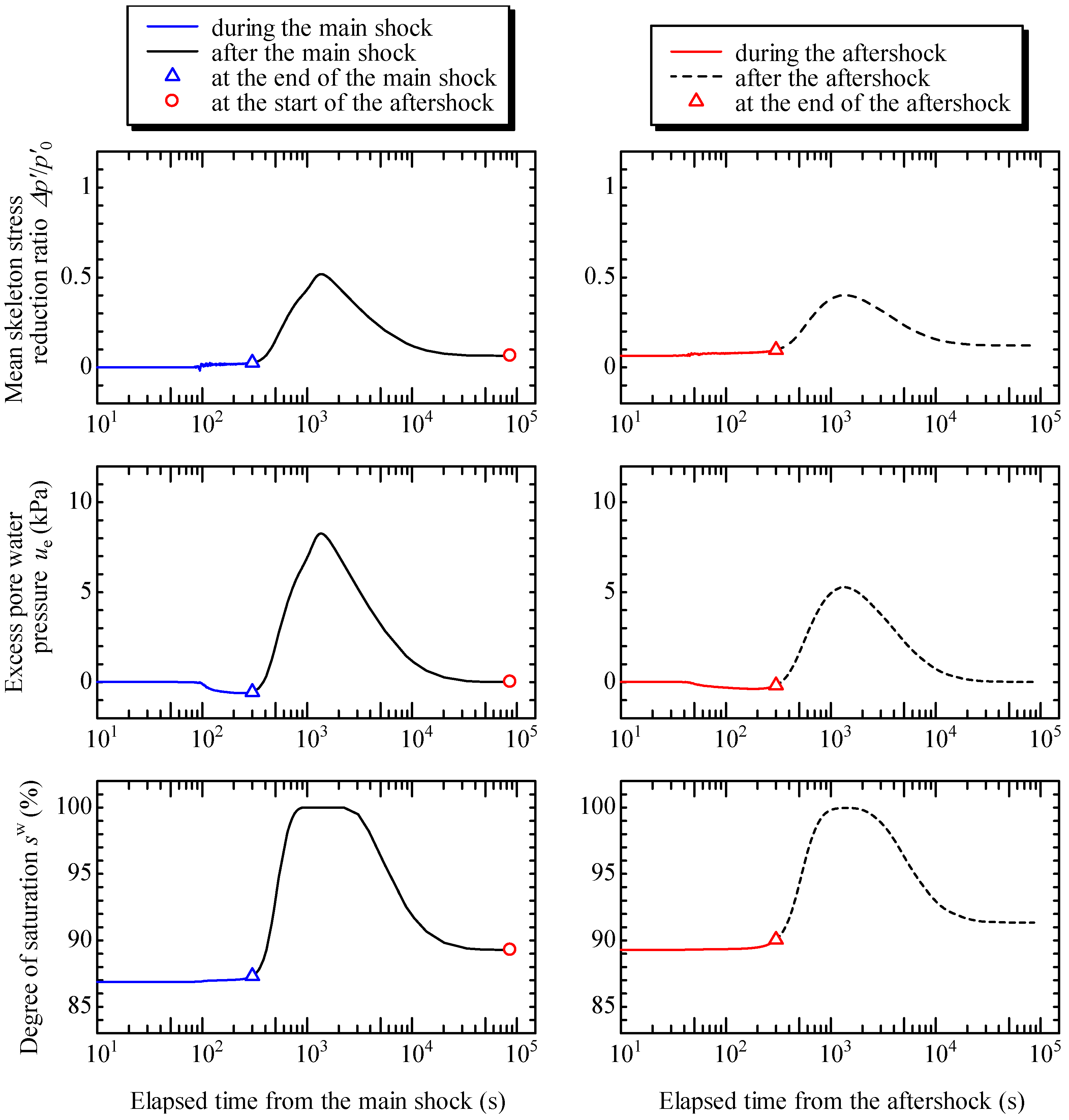
- In the saturated sand layer below the groundwater level, seismic external forces caused positive excess pore water pressure accompanied by negative dilatancy (plastic volume compression) due to seismic cyclic shear without immediate drainage.
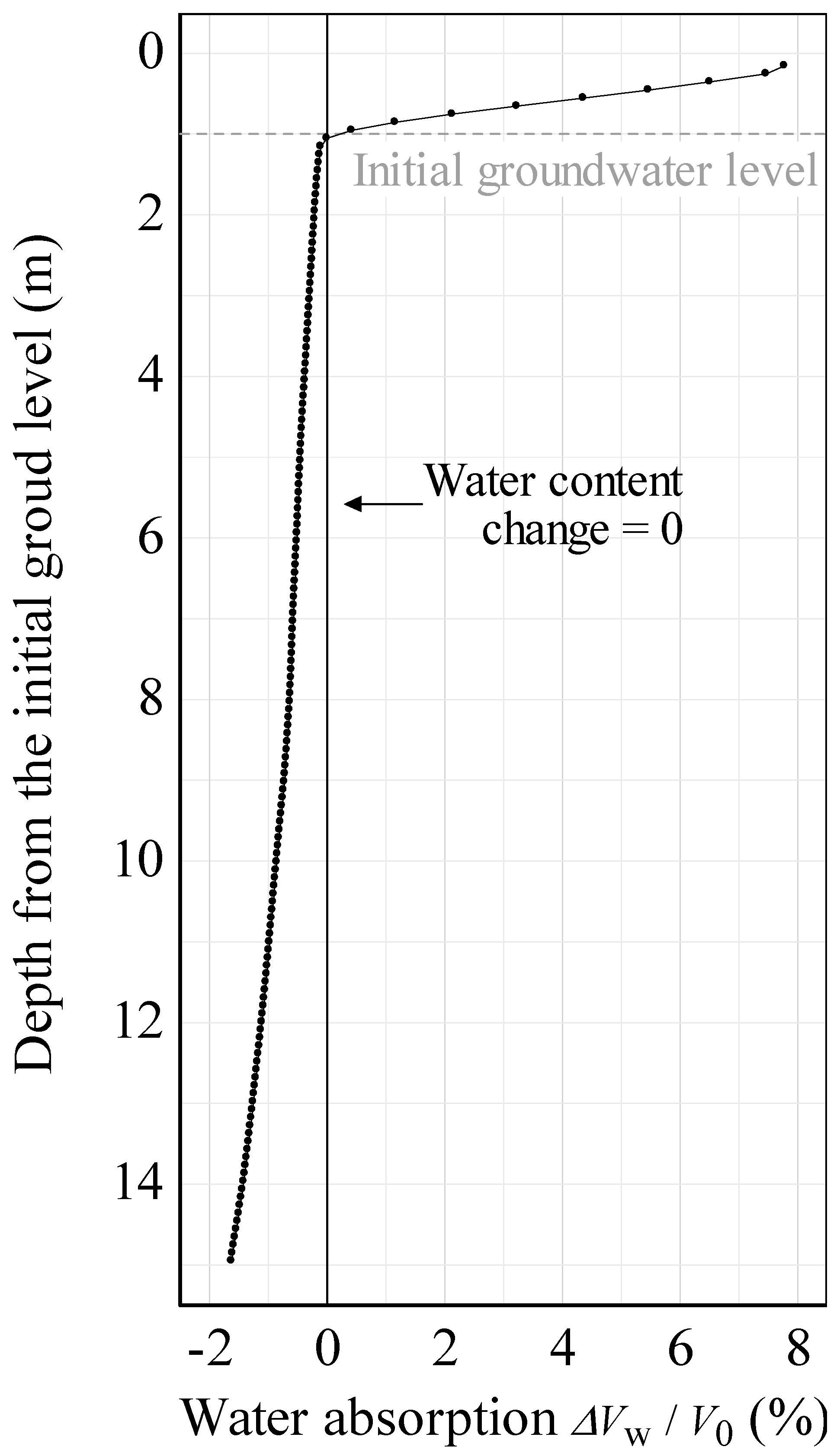
- The main shock leads to consolidation drainage between the main shock and aftershock, supplying water from the saturated sand layer to the unsaturated sand layer above the groundwater level. In other words, the groundwater level rises between the main shock and aftershock, expanding the saturated area.
- Aftershock increases the possibility of liquefaction in the expanded saturated area.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Outline of the Analysis Method
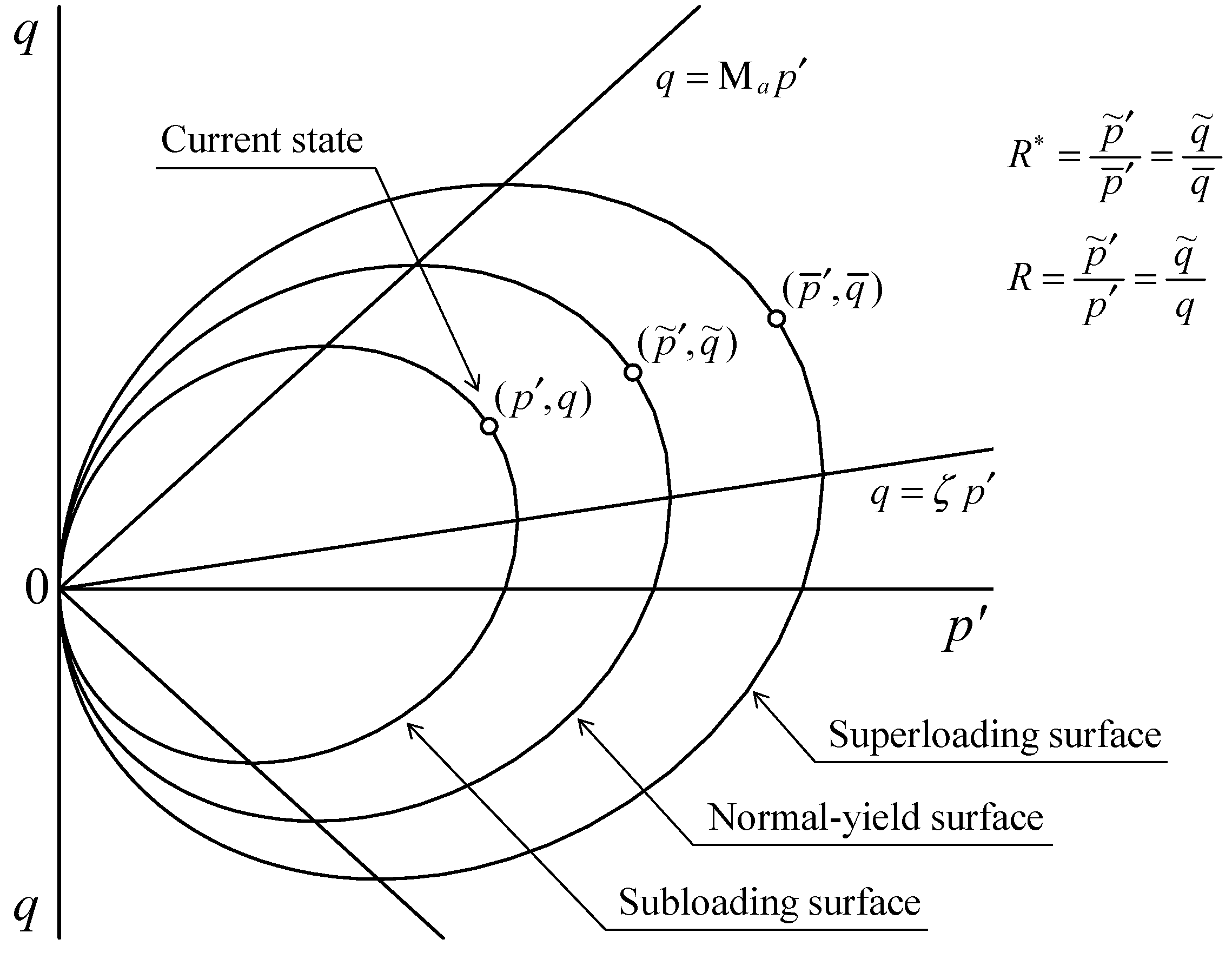
Appendix B. The Super/Subloading Yield Surface (SYS) Cam-Clay Model
Appendix B.1. Quantified Expression of Structure, Overconsolidation, Anisotropy, and Their Respective Evolution Rules
Appendix B.2. The Associated Flow Rule and the Constitutive Equation
References
- National Research Institute for Earth Science and Disaster Resilience. NIED K-NET, KiK-Net; National Research Institute for Earth Science and Disaster Resilience: Ibaraki, Japan, 2019. [CrossRef]
- Ueda, K.; Izawa, J.; Murono, Y.; Iai, S. Analytical study on the influence of aftershocks on the liquefaction behavior of ground. J. JSCE (A1) 2014, 70, I_578–I_585. (In Japanese) [Google Scholar] [CrossRef]
- Morikawa, Y.; Bao, X.; Zhang, F.; Taira, A.; Sakaguchi, H. Why an aftershock with a maximum acceleration of 25 gal could make ground liquefied in the 2011 Great East Japan Earthquake. In Proceedings of the 6th International Workshop on New Frontiers in Computational Geotechnics, Takayama, Japan, 21–23 May 2012; pp. 117–122. [Google Scholar]
- Yasuda, S.; Harada, K.; Ishikawa, K.; Kanemaru, Y. Characteristics of liquefaction in Tokyo Bay area by the 2011 Great East Japan Earthquake. Soils Found. 2012, 52, 793–810. [Google Scholar] [CrossRef]
- Urayasu City Liquefaction Countermeasure Technology Consideration Investigating Committee. The Second Committee. Available online: https://www.city.urayasu.lg.jp/shisei/johokoukai/shingikai/shichoukoushotsu/1002796/1002934.html (accessed on 15 November 2023). (In Japanese).
- Koizumi, N.; Kano, Y.; Kitagawa, Y.; Sato, T.; Takahashi, M.; Nishimura, S.; Nishida, R. Groundwater anomalies associated with the 1995 Hyogo-ken Nanbu Earthquake. J. Phys. Earth 1996, 44, 373–380. [Google Scholar] [CrossRef]
- Chia, Y.; Wang, Y.S.; Chiu, J.J.; Liu, C.W. Changes of groundwater level due to the 1999 Chi-Chi earthquake in the Choshui River alluvial fanin Taiwan. BSSA 2001, 91, 1062–1068. [Google Scholar] [CrossRef]
- Hosono, T.; Yamada, C.; Manga, M.; Wang, C.-Y.; Tanimizu, M. Stable isotopes show that earthquakes enhance permeability and release water from mountains. Nat. Commun. 2020, 11, 2776. [Google Scholar] [CrossRef] [PubMed]
- Biot, M.A. General theory of three-dimensional consolidation. J. Appl. Phys. 1941, 12, 155–164. [Google Scholar] [CrossRef]
- Manga, M.; Wang, C.-Y. Earthquake Hydrology. Treatise Geophys. 2015, 4, 305–328. [Google Scholar] [CrossRef]
- Noda, T.; Yoshikawa, T. Soil-water-air coupled finite deformation analysis based on a rate-type equation of motion incorporating the SYS Cam-clay model. Soils Found. 2015, 55, 45–62. [Google Scholar] [CrossRef]
- Asaoka, A.; Noda, T.; Yamada, E.; Kaneda, K.; Nakano, M. An elastoplastic description of two distinct volume change mechanisms of soils. Soils Found. 2002, 42, 47–57. [Google Scholar] [CrossRef]
- Yoshikawa, T.; Noda, T.; Kodaka, T.; Takaine, T. Analysis of the effect of groundwater level on the seismic behavior of an unsaturated embankment on clayey ground. Soil Dyn. Earthq. Eng. 2016, 85, 217–230. [Google Scholar] [CrossRef]
- Ravichandran, N. Fully coupled finite element model for dynamics of partially saturated soils. Soil Dyn. Earthq. Eng. 2009, 29, 1294–1304. [Google Scholar] [CrossRef]
- Khoei, A.; Mohammadnejad, T. Numerical modeling of multiphase fluid flow in deforming porous media: A comparison between two- and three-phase models for seismic analysis of earth and rockfill dams. Comput. Geotech. 2011, 38, 142–166. [Google Scholar] [CrossRef]
- Matsumaru, T.; Uzuoka, R. Three-phase seepage-deformation coupled analysis about unsaturated embankment damaged by earthquake. Int. J. Geomech. 2016, 16, C4016006. [Google Scholar] [CrossRef]
- Zhang, B.; Muraleetharan, K.K. Liquefaction of level ground unsaturated sand deposits using a validated fully coupled analysis procedure. Int. J. Geomech. 2018, 18, 04018118. [Google Scholar] [CrossRef]
- Chiba Prefecture. CHIBA INFORMATION MAP. Available online: https://map.pref.chiba.lg.jp/pref-chiba/Portal (accessed on 15 November 2023). (In Japanese).
- Ishikawa, K.; Yasuda, S. Study of sand boiling characteristics along Tokyo Bay during the 2011 Tohoku-Pacific Ocean Earthquake. J. JSCE (A1) 2012, 68, I_274–I_281. (In Japanese) [Google Scholar] [CrossRef]
- Nakai, K.; Noda, T.; Asaoka, A. Severe and nonuniform liquefaction damage of reclaimed ground contributed by interference between body waves and stratigraphic irregularity-induced surface waves. Earthq. Spectra, 2024; in press. [Google Scholar] [CrossRef]
- Noda, T.; Asaoka, A.; Nakano, M. Soil-water coupled finite deformation analysis based on a rate-type equation of motion incorporating the SYS Cam-clay model. Soils Found. 2008, 48, 771–790. [Google Scholar] [CrossRef]
- Lysmer, J.; Kuhlemeyer, R.L. Finite dynamic model for infinite media. ASCE 1969, 95, 859–878. [Google Scholar] [CrossRef]
- Ishikawa, K.; Yasuda, S. Effect of lowering the ground water table as the countermeasure against liquefaction-induced damage to houses. J. JAEE 2017, 15, 7_205–7_219. (In Japanese) [Google Scholar] [CrossRef][Green Version]
- Jommi, C. Remarks on the constitutive modelling of unsaturated soils. In Experimental Evidence and Theoretical Approaches in Unsaturated Soils; CRC Press/Balkema: Boca Raton, FL, USA, 2000; pp. 139–153. [Google Scholar]
- van Genuchten, M.T. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Mualem, Y. A new model for predicting the hydraulic conductivity of unsaturated porous media. Water Resour. Res. 1976, 12, 513–522. [Google Scholar] [CrossRef]
- Muskat, M. The Flow of Homogeneous Fluid through Porous Media; Mcgraw-Hill: New York, NY, USA, 1937; pp. 69–74. [Google Scholar]
- Yamamoto, T.; Nakai, T.; Maruki, Y.; Kodaka, T.; Kishida, K.; Ohnishi, Y. Health assessment of the slopes along the roads introducing the long-term degradation concept. Jpn. Geotech. J. 2009, 4, 21–33. (In Japanese) [Google Scholar] [CrossRef][Green Version]
- Yamamizu, F.; Kasahara, K.; Suzuki, H.; Ikawa, T.; Adachi, I. Seismic reflection profiling around the Shimohsa deep-well. Programme Abstr. Seismol. Soc. Jpn. 1993, 2, 261. (In Japanese) [Google Scholar]
- Midorikawa, S. Prediction of isoseismal map in the Kanto plain due to hypothetical earthquake. J. Struct. Eng. 1987, 33B, 43–48. (In Japanese) [Google Scholar]
- Christian, J.T. Undrained stress distribution by numerical method. J. Soil Mech. Found. Div. 1968, 94, 1333–1345. [Google Scholar] [CrossRef]
- Akai, K.; Tamura, T. Numerical analysis of multi-dimensional consolidation accompanied with elasto-plastic constitutive equation. Proc. JSCE 1978, 269, 95–104. (In Japanese) [Google Scholar] [CrossRef]
- Wilson, E.L.; Farhoomand, I.; Bathe, K.J. Nonlinear dynamic analysis of complex structures. Int. J. Earthq. Eng. Struct. Dyn. 1973, 1, 241–252. [Google Scholar] [CrossRef]
- Roscoe, K.H.; Burland, J.B. On the generalized stress-strain behaviour of ‘wet’ clay. In Engineering Plasticity; Cambridge University Press: Cambridge, UK, 1968; pp. 535–609. [Google Scholar]
- Sekiguchi, H.; Ohta, H. Induced anisotropy and time dependency in clays. In Proceedings of the 9th International Conference on Soil Mechanics and Foundation Engineering, Specialty Session 9, Tokyo, Japan, 10–15 July 1977; pp. 229–238. [Google Scholar]
- Asaoka, A.; Nakano, M.; Noda, T. Superloading yield surface concept for highly structured soil behavior. Soils Found. 2000, 40, 99–110. [Google Scholar] [CrossRef]
- Hashiguchi, K. Plastic constitutive equations of granular materials. In Proceedings of the US-Japan Seminar on Continuum Mechanics and Statistical Approaches in the Mechanics of Granular Materials, Sendai, Japan, 5–9 June 1978; pp. 321–329. [Google Scholar]
- Hashiguchi, K. Subloading surface model in unconventional plasticity. Int. J. Solids Struct. 1989, 25, 917–945. [Google Scholar] [CrossRef]
- Hashiguchi, K.; Chen, Z.P. Elastoplastic constitutive equations of soils with the subloading surface and the rotational hardening. Int. J. Numer. Anal. Meth. Geomech. 1998, 22, 197–227. [Google Scholar] [CrossRef]
- Green, A.E.; Naghdi, P.M. A general theory of an elastic-plastic continuum. Arch. Ration. Mech. Anal. 1965, 18, 251–281. [Google Scholar] [CrossRef]

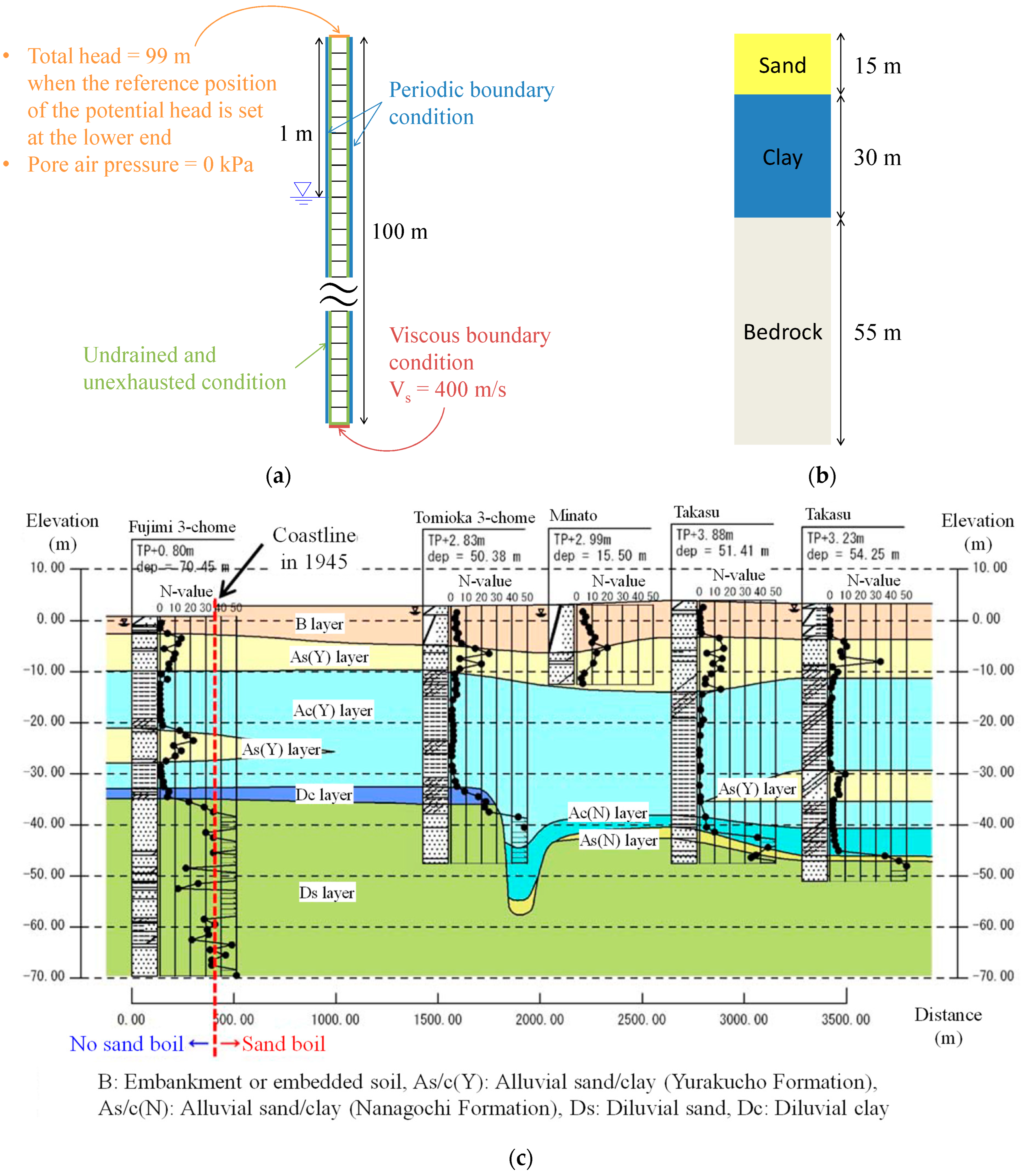



| Sand | Clay | Bedrock | ||
|---|---|---|---|---|
| Elastoplastic parameters | ||||
| Specific volume at q = 0 and p′ = 98.1 kPa on NCL | 2.0 | 3.02 | 2.0 | |
| Critical state constant | 1.4 | 1.4 | 1.5 | |
| Compression index | 0.1 | 0.242 | 0.005 | |
| Swelling index | 0.0025 | 0.02 | 0.0005 | |
| Poisson’s ratio | 0.2 | 0.1 | 0.1 | |
| Evolution parameters | ||||
| Degradation parameter of overconsolidated state | 8.0 | 20.0 | 0.3 | |
| Degradation parameter of structure | 8.0 | 0.65 | 0.05 | |
| Degradation parameter of structure | 1.0 | 1.0 | 1.0 | |
| Degradation parameter of structure | 1.0 | 1.0 | 1.0 | |
| Degradation parameter of structure | 1.0 | 0.4 | 0.5 | |
| Evolution parameter of rotational hardening | 10.0 | 0.2 | 0.2 | |
| Limit of rotational hardening | 0.44 | 1.0 | 0.7 | |
| Initial values | ||||
| Void ratio | 0.98 | 2.35 | 0.21 | |
| Degree of structure | 3.04 | 21.75 | 100.0 | |
| Overconsolidation ratio | Distributed | Distributed | Distributed | |
| Stress ratio | 0.545 | 0.545 | 0.545 | |
| Degree of anisotropy | 0.0 | 0.3 | 1.0 |
| Sand | Clay | Bedrock | ||
|---|---|---|---|---|
| Soil–water characteristic | ||||
| Maximum degree of saturation % | 100.0 | - | - | |
| Minimum degree of saturation % | 60.0 | - | - | |
| van Genuchten parameter kPa−1 | 0.15 | - | - | |
| van Genuchten parameter () | 2.0 | - | - | |
| Saturated coefficient of water permeability m/s | 6.17 × 10−5 | 1.0 × 10−9 | 1.0 × 10−8 | |
| Dry coefficient of air permeability m/s | 3.40 × 10−3 | - | - | |
| Physical property | ||||
| Soil particle density g/cm3 | 2.787 | 2.690 | 2.650 | |
| Bulk modulus of water kPa | 2.19 × 106 | 2.19 × 106 | 2.19 × 106 | |
| Specific gas constant of air m2/s2/K | 287.04 | 287.04 | 287.04 | |
| Absolute temperature K | 293.15 | 293.15 | 293.15 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yoshikawa, T.; Noda, T. Comprehension of Seismic-Induced Groundwater Level Rise in Unsaturated Sandy Layer Based on Soil–Water–Air Coupled Finite Deformation Analysis. Water 2024, 16, 452. https://doi.org/10.3390/w16030452
Yoshikawa T, Noda T. Comprehension of Seismic-Induced Groundwater Level Rise in Unsaturated Sandy Layer Based on Soil–Water–Air Coupled Finite Deformation Analysis. Water. 2024; 16(3):452. https://doi.org/10.3390/w16030452
Chicago/Turabian StyleYoshikawa, Takahiro, and Toshihiro Noda. 2024. "Comprehension of Seismic-Induced Groundwater Level Rise in Unsaturated Sandy Layer Based on Soil–Water–Air Coupled Finite Deformation Analysis" Water 16, no. 3: 452. https://doi.org/10.3390/w16030452
APA StyleYoshikawa, T., & Noda, T. (2024). Comprehension of Seismic-Induced Groundwater Level Rise in Unsaturated Sandy Layer Based on Soil–Water–Air Coupled Finite Deformation Analysis. Water, 16(3), 452. https://doi.org/10.3390/w16030452







