Steady-State and Dynamic Simulation for Wastewater Treatment Plant Management: Case Study of Maghnia City, North-West Algeria
Abstract
1. Introduction
2. Materials and Methods
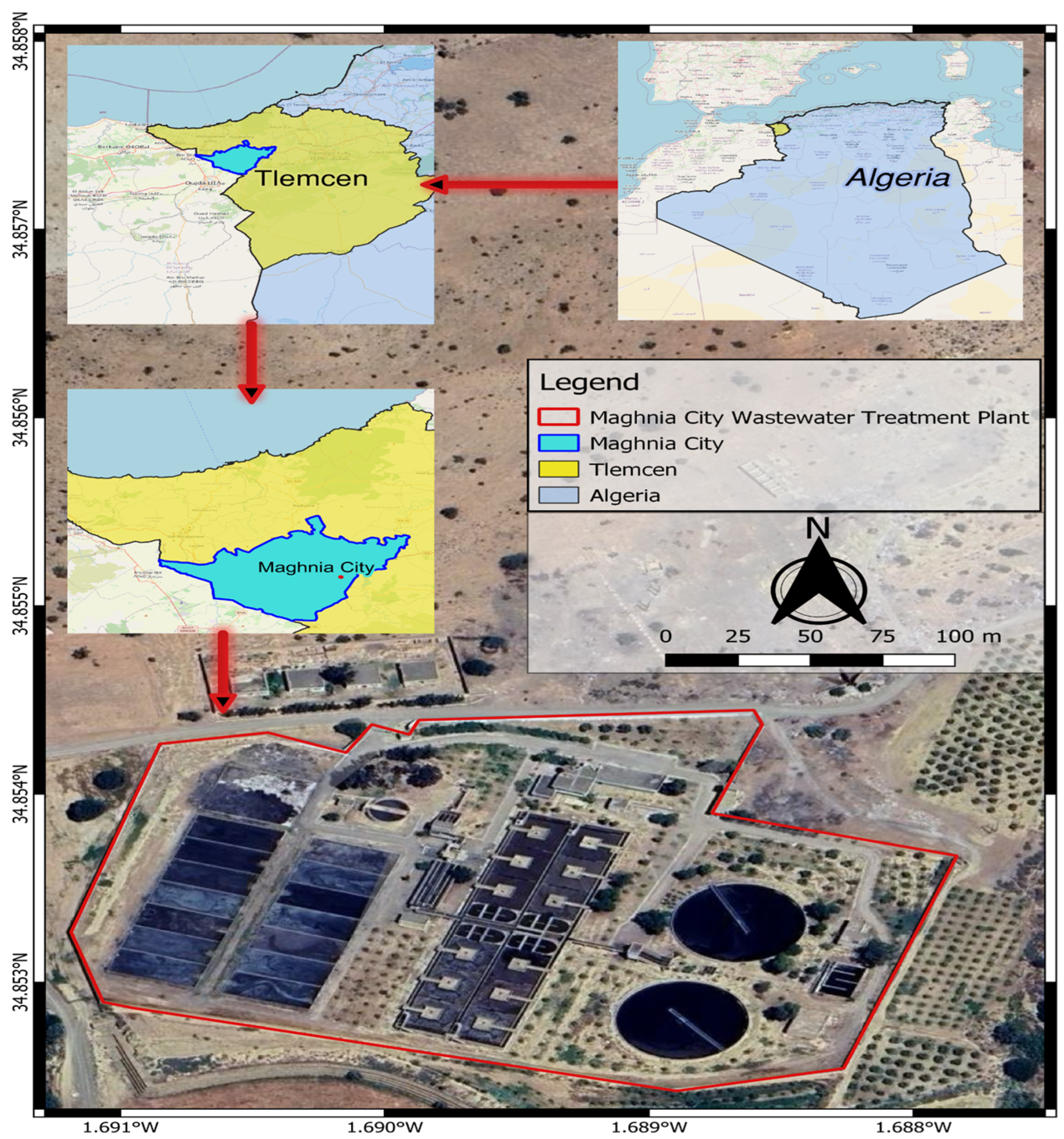
2.1. Study Area
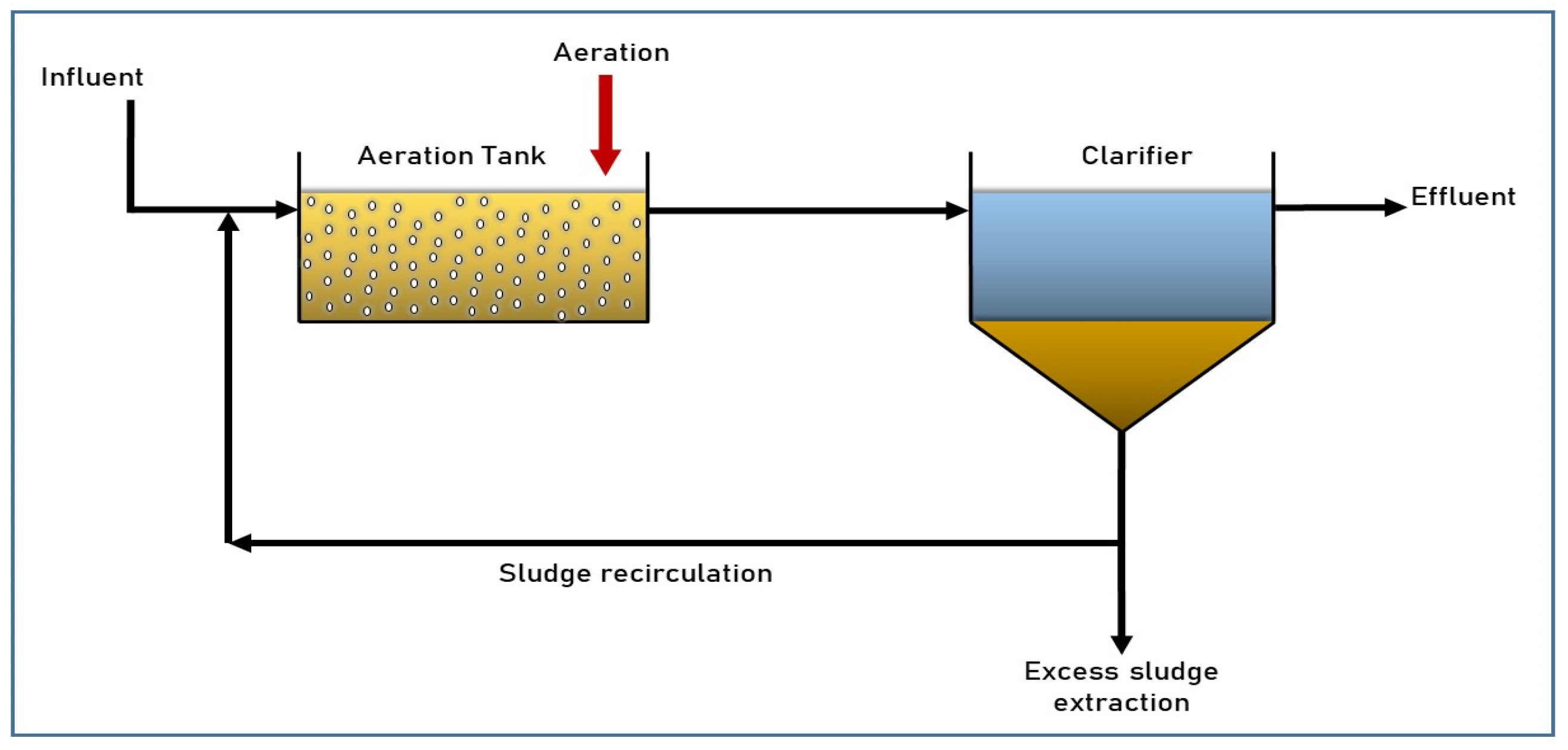
2.2. Description of Maghnia City Wastewater Treatment Plant
2.3. Monitored Parameters and Analytical Methods
2.4. Methodology
2.4.1. Presentation of the Software GPS-X Version 8
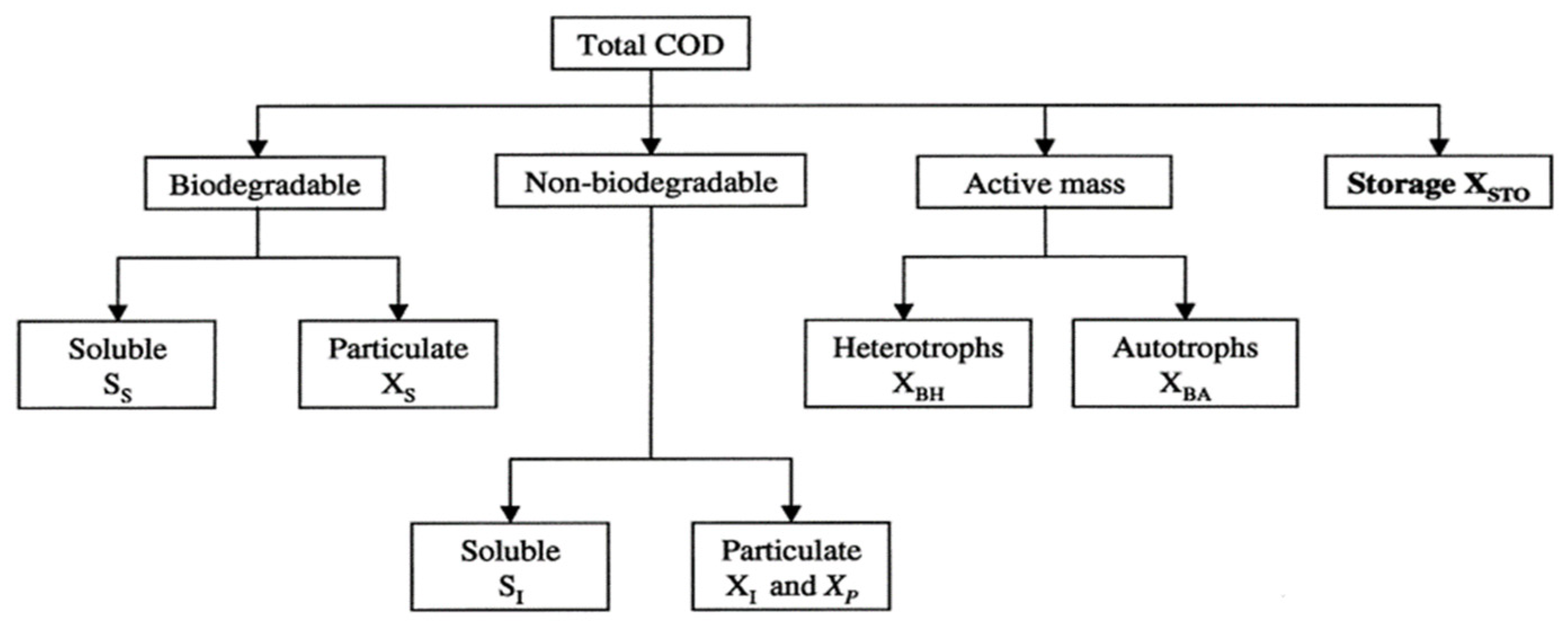
2.4.2. The ASM1 Model
2.4.3. Calibration of the Model
- Enhance the clarity of the mathematical response to COD output and dynamic variables by using a range of values for μ–max H [43] while maintaining default values of other parameters to reduce the dynamism.
- Ones μ–max H is obtained, adjusting parameters related to XBH, such as YH and bH, is required for a complete calibration [31].
- Discrepancies between predicted and observed values are identified and adjustments are made in parameter values until achieving a precise match. The objective of the model calibration is to establish a correlation between the model’s prediction and the experimental results.
3. Results & Discussion
3.1. Characterization of Influent Wastewater
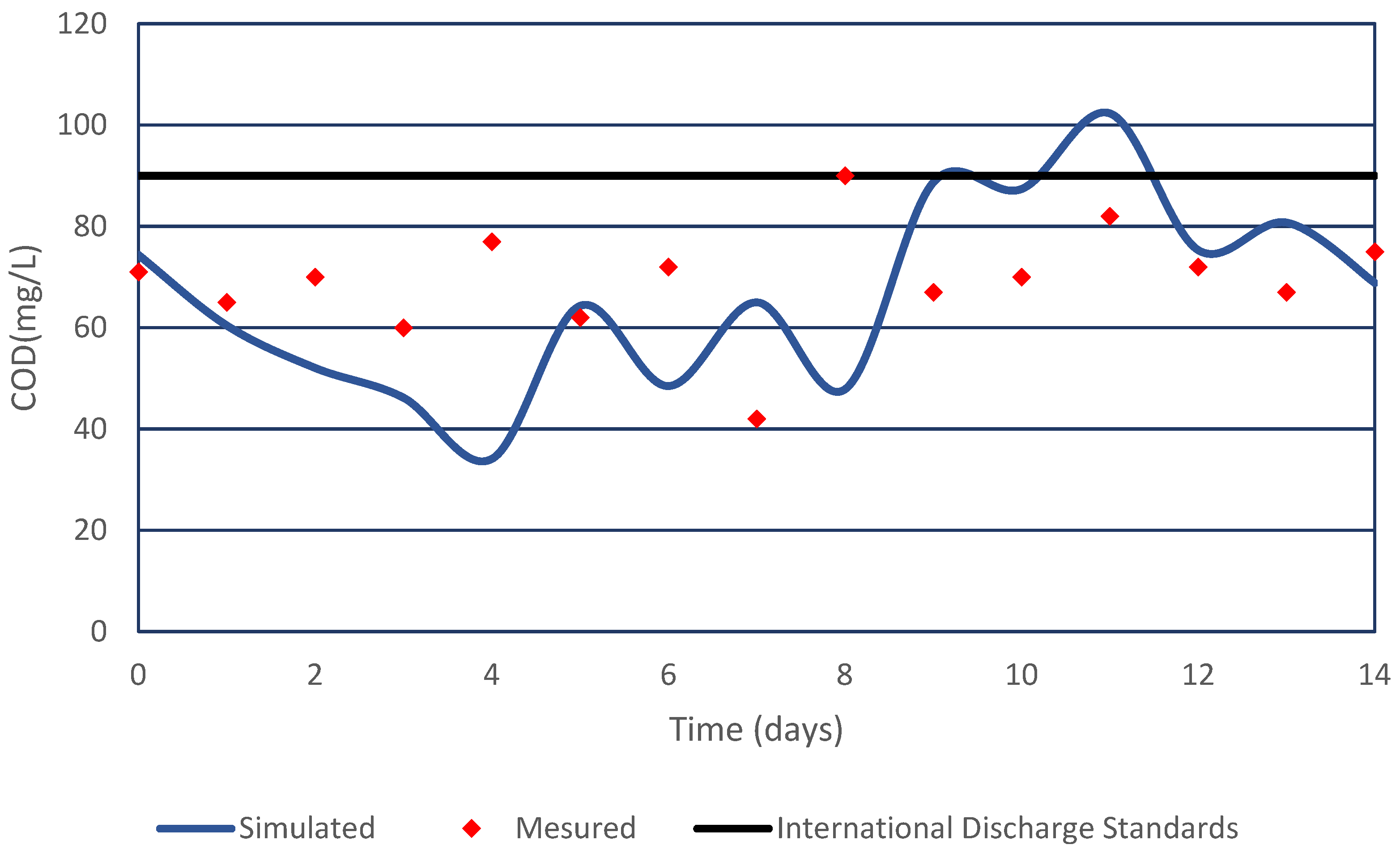
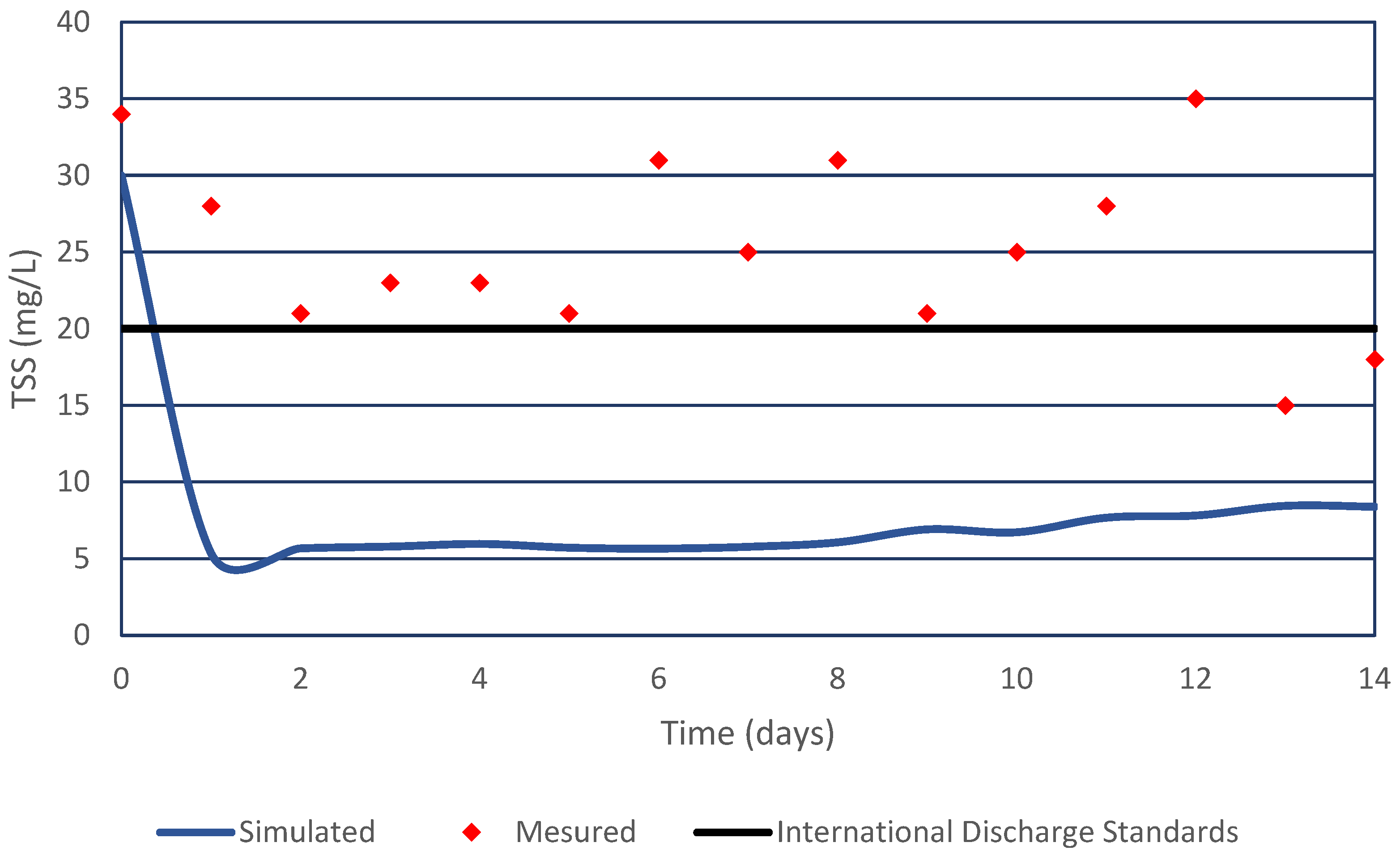
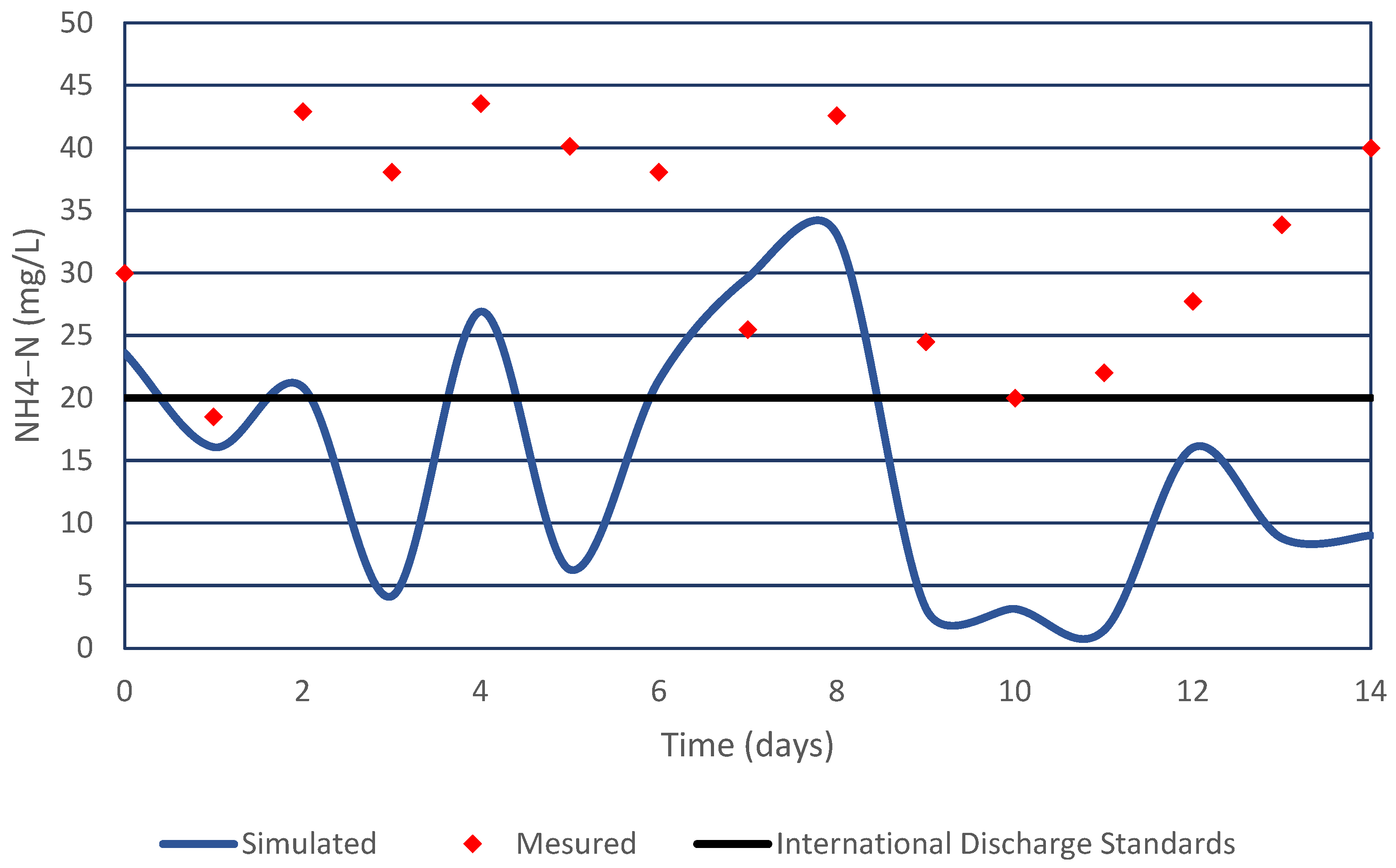
3.2. Model Calibration
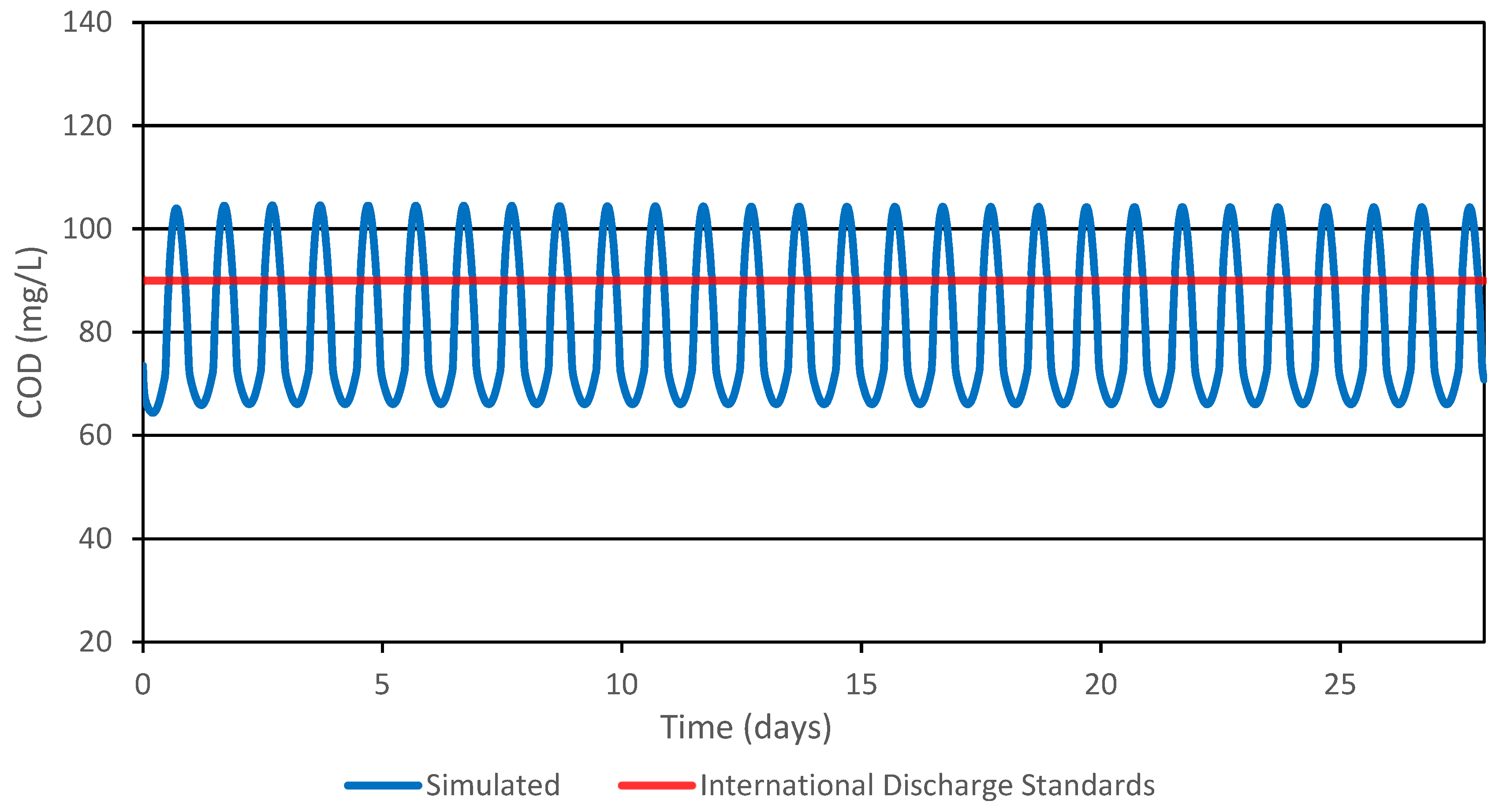
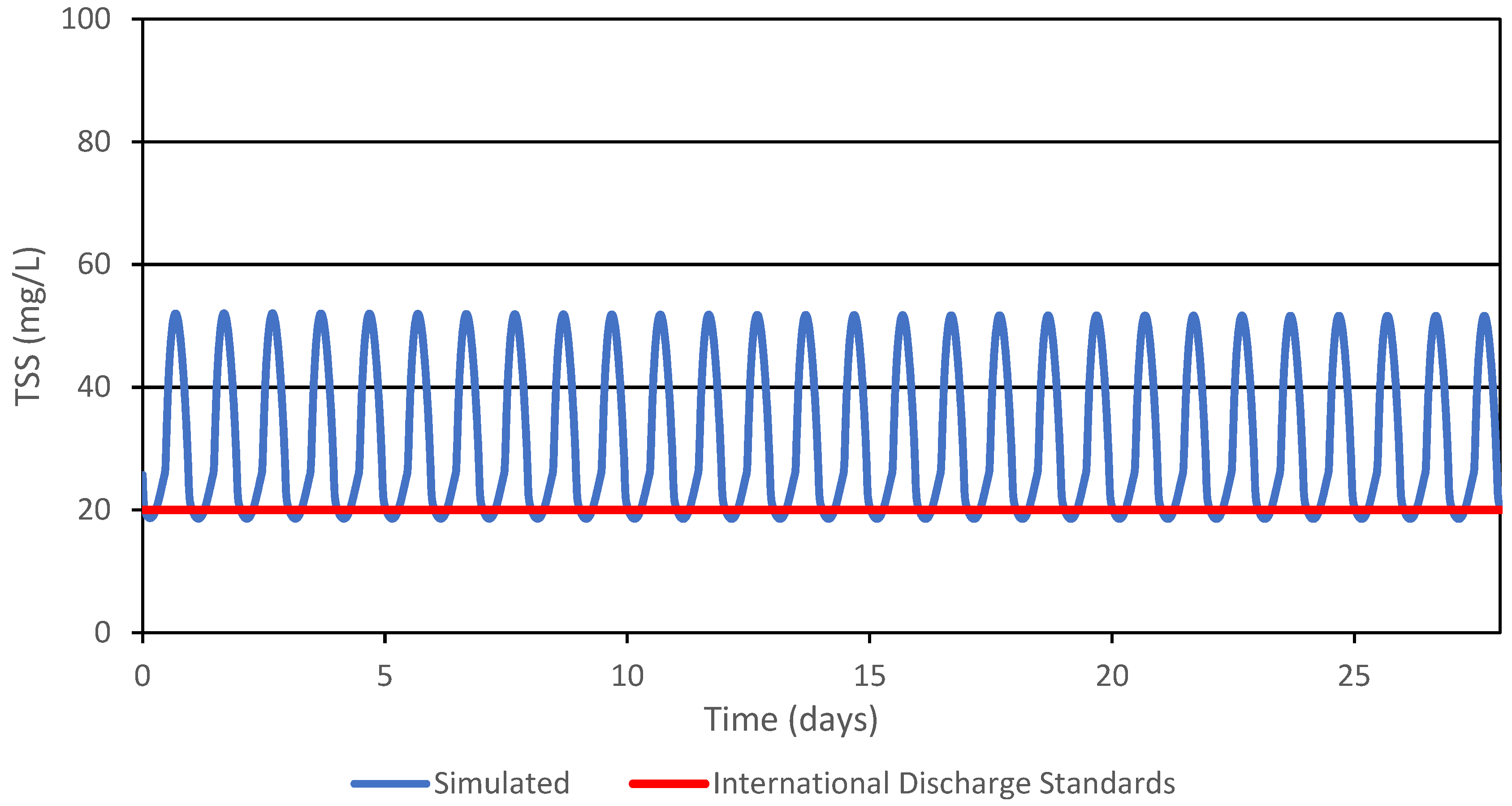
3.3. Dynamic Simulation Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Nomenclature | |
| bA | decay coefficient for autotrophic biomass (d−1); |
| bH | decay coefficient for heterotrophic biomass (d−1); |
| DO | dissolved oxygen (mg/L); |
| fp | fraction of biomass leading to particulate products; |
| iXB | nitrogen fraction in biomass; |
| iXP | nitrogen fraction in products from biomass; |
| kh | hydrolysis rate constant (d−1); |
| KOH | oxygen half-saturation coefficient for heterotrophic biomass (mg/L); |
| Ks | half-saturation coefficient for readily biodegradable substrate (mg/L); |
| Q | influent flow rate (m3/d); |
| substrate utilization rate (mg/(L d)); | |
| r(ξ) | conversion vector of the variable ξ (mg/(L d)); |
| Si | soluble inert organic matter (mg/L); |
| SND | soluble biodegradable organic nitrogen (mg/L); |
| SNH | ammonia nitrogen (mg/L); |
| SNO | nitrate and nitrite nitrogen (mg/L); |
| Ss | readily biodegradable substrate (mg/L); |
| SS,in | influent readily biodegradable substrate (mg/L); |
| t | time (d); |
| T | temperature (°C); |
| V | reactor volume (L); |
| XBA | active autotrophic biomass (mg/L); |
| XBH | active heterotrophic biomass (mg/L); |
| XBH,in | influent active heterotrophic biomass (mg/L); |
| Xi | particulate inert organic matter (mg/L); |
| XND | particulate biodegradable organic nitrogen (mg/L); |
| XP | particulate products arising from biomass decay (mg/L); |
| XS | slowly biodegradable substrate (mg/L); |
| XS,in | influent slowly biodegradable substrate (mg/L); |
| YA | growth yield of autotrophic biomass; |
| YH | growth yield of heterotrophic biomass. |
| Greek symbols | |
| ξ | vector of reactor and effluent concentration (mg/L); |
| ξin | vector of influent concentration (mg/L); |
| μ–max H | maximum specific growth rate for heterotrophic biomass (d − 1); |
| ρ(ξ) | vector of reaction kinetics (mg/(L d)); |
| ρj | process rate (mg/(L d)); |
| Θ | hydraulic residence time, HRT (d); |
| νij | stoichiometric coefficient; |
| ηg | correction factor of µH under anoxic conditions; |
| ηh | correction factor for hydrolysis under anoxic conditions. |
References
- McHarg, A.M. Optimisation of Municipal Wastewater Biological Nutrient Removal Using Computer Simulation. Ph.D. Thesis, University of Ottawa, Ottawa, ON, Canada, 2002. [Google Scholar]
- Chachuat, B.; Latifi, A.; Roche, N. Methodologie d’Optimisation Dynamique et de Commande Optimale des Petites Stations d’Epuration a Boues Activees; Institut National Polytechnique de Lorraine (INPL): Vandoeuvre-lés-Nancy, France, 2001. [Google Scholar]
- Barnett, M.W.; Takacs, I.; Stephenson, J.; Gall, B.; Perdeus, M. Dynamic Modeling. Water Environ. Technol. 1995, 7, 41–44. [Google Scholar]
- Revollar, S.; Vilanova, R.; Vega, P.; Francisco, M.; Meneses, M. Wastewater Treatment Plant Operation: Simple Control Schemes with a Holistic Perspective. Sustainability 2020, 12, 768. [Google Scholar] [CrossRef]
- Han, H.-G.; Qiao, J.-F. Prediction of Activated Sludge Bulking Based on a Self-Organizing RBF Neural Network. J. Process Control 2012, 22, 1103–1112. [Google Scholar] [CrossRef]
- Olsson, G.; Newell, B. Wastewater Treatment Systems: Modelling, Diagnosis and Control. Water Intell. Online 2015, 4, 9781780402864. [Google Scholar] [CrossRef]
- Gujer, W.; Henze, M. Activated Sludge Modelling and Simulation. Water Sci. Technol. 1991, 23, 1011–1023. [Google Scholar] [CrossRef]
- Henze, M.; Gujer, W.; Mino, T.; Van Loosdrecht, M. Activated Sludge Models ASM1, ASM2, ASM2d and ASM3; IWA Scientific and Technical Report No. 9; IWA 2000 Publishing: London, UK, 2006. [Google Scholar]
- Jeppsson, U. Modelling Aspects of Wastewater Treatment Processes. Ph.D. Thesis, Department of Industrial Electrical Engineering and Automation, Lund Institute of Technology, Lund, Sweden, 1996. [Google Scholar]
- Nuhoglu, A.; Keskinler, B.; Yildiz, E. Mathematical Modelling of the Activated Sludge Process—The Erzincan Case. Process Biochem. 2005, 40, 2467–2473. [Google Scholar] [CrossRef]
- Van Loosdrecht, M.C.M.; Lopez-Vazquez, C.M.; Meijer, S.C.F.; Hooijmans, C.M.; Brdjanovic, D. Twenty-Five Years of ASM1: Past, Present and Future of Wastewater Treatment Modelling. J. Hydroinform. 2015, 17, 697–718. [Google Scholar] [CrossRef]
- Baek, S.H.; Jeon, S.K.; Pagilla, K. Mathematical Modeling of Aerobic Membrane Bioreactor (MBR) Using Activated Sludge Model No. 1 (ASM1). J. Ind. Eng. Chem. 2009, 15, 835–840. [Google Scholar] [CrossRef]
- Elshorbagy, W.E.; Shawaqfah, M. Development of an ASM1 Dynamic Simulation Model for an Activated Sludge Process in United Arab Emirates. Desalination Water Treat. 2015, 54, 15–27. [Google Scholar] [CrossRef]
- Mohammadi, F.; Rahimi, S.; Bina, B.; Amin, M.M. Modeling of Activated Sludge with ASM1 Model, Case Study on Wastewater Treatment Plant of South of Isfahan. Curr. World Environ. 2015, 10, 96–105. [Google Scholar] [CrossRef]
- Lahdhiri, A.; Lesage, G.; Hannachi, A.; Heran, M. Steady-State Methodology for Activated Sludge Model 1 (ASM1) State Variable Calculation in MBR. Water 2020, 12, 3220. [Google Scholar] [CrossRef]
- STOWA. Methoden voor Influentkarakterisering—Inventarisatie en Richtlijnen; STOWA Report 80-96; STOWA: Utrecht, The Netherlands, 1996. [Google Scholar]
- Sollfrank, U.; Gujer, W. Characterisation of Domestic Wastewater for Mathematical Modelling of the Activated Sludge Process. Water Sci. Technol. 1991, 23, 1057–1066. [Google Scholar] [CrossRef]
- Stokes, L.; Takács, I.; Watson, B.; Watts, J.B. Dynamic Modelling of an ASP Sewage Works—A Case Study. Water Sci. Technol. 1993, 28, 151–161. [Google Scholar] [CrossRef]
- Weijers, S.R.; Kok, J.J.; Preisig, H.A.; Buunen, A.; Wouda, T.W.M. Parameter Identifiablity in the IAWQ Model No. 1 for Modelling Activated Sludge Plants for Enhanced Nitrogen Removal. Comput. Chem. Eng. 1996, 20, S1455–S1460. [Google Scholar] [CrossRef]
- Schütze, M.R.; Butler, D.; Beck, M.B. Modelling, Simulation and Control of Urban Wastewater Systems; Springer: London, UK, 2002; ISBN 978-1-4471-1105-4. [Google Scholar]
- Hauduc, H.; Gillot, S.; Rieger, L.; Ohtsuki, T.; Shaw, A.; Takács, I.; Winkler, S. Activated Sludge Modelling in Practice: An International Survey. Water Sci. Technol. 2009, 60, 1943–1951. [Google Scholar] [CrossRef] [PubMed]
- ONS: Office National des Statistiques. Available online: https://www.ons.dz/spip.php?rubrique127 (accessed on 17 June 2023).
- Medejerab, A.; Henia, L. Variations spatio-temporelles de la sècheresse climatique en Algérie nord-occidentale. Courr. Savoir 2011, 11, 71–79. [Google Scholar]
- ONA. 2013 National Sanitation Office. Available online: http://ona-dz.org/cgi-sys/suspendedpage.cgi (accessed on 17 June 2023).
- APHA. Standard Methods for the Examination of Water and Wastewater, 22nd ed.; Rice, E.W., Baird, R.B., Eaton, A.D., Clesceri, L.S., Eds.; American Public Health Association (APHA): Washington, DC, USA, 2012. [Google Scholar]
- Hydromantis Water and Wastewater Treatment Modeling and Simulation Software|Hydromantis. Available online: https://www.hydromantis.com/ (accessed on 17 June 2023).
- Mu’azu, N.D.; Alagha, O.; Anil, I. Systematic Modeling of Municipal Wastewater Activated Sludge Process and Treatment Plant Capacity Analysis Using GPS-X. Sustainability 2020, 12, 8182. [Google Scholar] [CrossRef]
- Hydromantis, G.-X.; Environmental Software Solutions Inc. Hydromantis GPS-X Technical Reference; Environmental Software Solutions Inc.: Hamilton, ON, Canada, 2017; Available online: https://www.hydromantis.com/help/GPS-X/docs/8.0/Technical/index.html (accessed on 11 June 2023).
- Fehlberg, E. Klassische Runge-Kutta-Formeln fünfter und siebenter Ordnung mit Schrittweiten-Kontrolle. Computing 1969, 4, 93–106. [Google Scholar] [CrossRef]
- Henze, M.; Grady, C.P.L.; Gujer, W.; Marais, G.V.R.; Matsuo, T. A General Model for Single-Sludge Wastewater Treatment Systems. Water Res. 1987, 21, 505–515. [Google Scholar] [CrossRef]
- Costa, C. A Comprehensive View of the ASM1 Dynamic Model: Study on a Practical Case. Water 2022, 14, 1046. [Google Scholar] [CrossRef]
- Costa, C.; Domínguez, J.; Autrán, B.; Márquez, M.C. Dynamic Modeling of Biological Treatment of Leachates from Solid Wastes. Environ. Model. Assess. 2018, 23, 165–173. [Google Scholar] [CrossRef]
- Takacs, I. A Dynamic Model of the Clarification-Thickening Process. Water Res. 1991, 25, 1263–1271. [Google Scholar] [CrossRef]
- Petersen, B.; Gernaey, K.; Henze, M.; Vanrolleghem, P.A. Calibration of Activated Sludge Models: A Critical Review of Experimental Designs. In Biotechnology for the Environment: Wastewater Treatment and Modeling, Waste Gas Handling; Agathos, S.N., Reineke, W., Eds.; Focus on Biotechnology; Springer: Dordrecht, The Netherlands, 2003; pp. 101–186. ISBN 978-94-017-0932-3. [Google Scholar]
- Henze, M.; Grady, L., Jr.; Gujer, W.; Marais, G.; Matsuo, T. Activated Sludge Model No 1. Wat. Sci. Technol. 1987, 29, 183–193. [Google Scholar]
- Siegrist, H.; Tschui, M. Interpretation of Experimental Data with Regard to the Activated Sludge Model No.1 and Calibration of the Model for Municipal Wastewater Treatment Plants. Water Sci. Technol. 1992, 25, 167–183. [Google Scholar] [CrossRef]
- Maurer, M.; Gujer, W. Dynamic Modelling of Enhanced Biological Phosphorus and Nitrogen Removal in Activated Sludge Systems. Water Sci. Technol. 1998, 38, 203–210. [Google Scholar] [CrossRef]
- Van Veldhuizen, H. Modelling Biological Phosphorus and Nitrogen Removal in a Full Scale Activated Sludge Process. Water Res. 1999, 33, 3459–3468. [Google Scholar] [CrossRef]
- Gaudy, A.F.; Jrand Gaudy, E.T. Biological Concepts for Design and Operation of the Activated Sludge Process; US Environmental Protection Agency Water Pollution Research Series, Report No. 17090, FQJ, 09/71; US EPA: Washington, DC, USA, 1971. [Google Scholar]
- Dupont, R.; Sinkjær, O. Optimisation of W ASTEW A TER treatment plants by means of computer models. Water Sci. Technol. 1994, 30, 181–190. [Google Scholar] [CrossRef]
- Kristensen, G.H.; Jansen, J.L.C.; Jorgensen, P.E. Batch Test Procedures as Tools for Calibration of the Activated Sludge Model—A Pilot Scale Demonstration. Water Sci. Technol. 1998, 37, 235–242. [Google Scholar] [CrossRef]
- Henze, M.; Gujer, W.; Mino, T.; Matsuo, T.; Wentzel, M.C.; Marais, G.V.R.; Van Loosdrecht, M.C.M. Activated Sludge Model No.2d, ASM2D. Water Sci. Technol. 1999, 39, 165–182. [Google Scholar] [CrossRef]
- Sharifi, S.; Murthy, S.; Takács, I.; Massoudieh, A. Probabilistic Parameter Estimation of Activated Sludge Processes Using Markov Chain Monte Carlo. Water Res. 2014, 50, 254–266. [Google Scholar] [CrossRef]
- Spérandio, M.; Espinosa, M.C. Modelling an Aerobic Submerged Membrane Bioreactor with ASM Models on a Large Range of Sludge Retention Time. Desalination 2008, 231, 82–90. [Google Scholar] [CrossRef]
- Sin, G.; Kaelin, D.; Kampschreur, M.J.; Takács, I.; Wett, B.; Gernaey, K.V.; Rieger, L.; Siegrist, H.; Van Loosdrecht, M.C.M. Modelling Nitrite in Wastewater Treatment Systems: A Discussion of Different Modelling Concepts. Water Sci. Technol. 2008, 58, 1155–1171. [Google Scholar] [CrossRef] [PubMed]
- Power, M. The Predictive Validation of Ecological and Environmental Models. Ecol. Model. 1993, 68, 33–50. [Google Scholar] [CrossRef]
- Surampalli, R. Nitrification, Denitrification and Phosphorus Removal in Sequential Batch Reactors. Bioresour. Technol. 1997, 61, 151–157. [Google Scholar] [CrossRef]
- Choubert, J.M. Analyse et Optimisation du Traitement de l’Azote par Boues Activées à Basse Température. Ph.D. Thesis, Université Louis Pasteur, Strasbourg, France, 2002. [Google Scholar]
- Choubert, J.-M.; Racault, Y.; Grasmick, A.; Beck, C.; Heduit, A. Nitrogen Removal from Urban Wastewater by Activated Sludge Process Operated over the Conventional Carbon Loading Rate Limit at Low Temperature. Water SA 2005, 31, 503–510. [Google Scholar] [CrossRef][Green Version]
- Marquot, A.; Stricker, A.-E.; Racault, Y. ASM1 Dynamic Calibration and Long-Term Validation for an Intermittently Aerated WWTP. Water Sci. Technol. 2006, 53, 247–256. [Google Scholar] [CrossRef]
- Elawwad, A.; Matta, M.; Abo-Zaid, M.; Abdel-Halim, H. Plant-Wide Modeling and Optimization of a Large-Scale WWTP Using BioWin’s ASDM Model. J. Water Process Eng. 2019, 31, 100819. [Google Scholar] [CrossRef]
- Barker, P.S.; Dold, P.L. General Model for Biological Nutrient Removal Activated-Sludge Systems: Model Presentation. Water Environ. Res. 1997, 69, 969–984. [Google Scholar] [CrossRef]
- Henze, M.; Van Loosdrecht, M.C.M.; Ekama, G.A.; Brdjanovic, D. Biological Wastewater Treatment: Principles, Modelling and Design; IWA Publishing: London, UK, 2008; ISBN 978-1-78040-186-7. [Google Scholar]
- Makinia, J.; Rosenwinkel, K.-H.; Spering, V. Comparison of Two Model Concepts for Simulation of Nitrogen Removal at a Full-Scale Biological Nutrient Removal Pilot Plant. J. Environ. Eng. 2006, 132, 476–487. [Google Scholar] [CrossRef]
- Vanrolleghem, P.; Insel, G.; Petersen, B.; Sin, G.; Pauw, D.; Nopens, I.; Dovermann, H.; Weijers, S.; Gernaey, K. A Comprehensive Model Calibration Procedure for Activated Sludge Models; Water Environment Federation: Alexandria, VA, USA, 2003. [Google Scholar] [CrossRef]
- Nelson, M.I.; Sidhu, H.S. Analysis of the Activated Sludge Model (Number 1). Appl. Math. Lett. 2009, 22, 629–635. [Google Scholar] [CrossRef]
- Nguyen, D.H.; Latifi, M.A.; Lesage, F.; Mulholland, M. Dynamic Simulation and Optimization of Wastewater Treatment Plants. In Proceedings of the 2013 International Conference on Process Control (PC), Strbske Pleso, Slovakia, 18–21 June 2013; pp. 407–414. [Google Scholar]
- Dairi, S.; Yassine, D.; Yahia, H.; Mrad, D. Dynamic Simulation for Wastewater Treatment Plants Management: Case of Souk-Ahras Region, North-Eastern Algeria. J. Water Land Dev. 2017, 34, 221. [Google Scholar] [CrossRef]
- World Health Organization (WHO). Guidelines for the Safe Use of Wastewater, Excreta and Greywater, Volume 4: Excreta and Greywater Use in Agriculture; WHO: Geneva, Switzerland, 2006. [Google Scholar]
- Fenu, A.; Guglielmi, G.; Jimenez, J.; Spèrandio, M.; Saroj, D.; Lesjean, B.; Brepols, C.; Thoeye, C.; Nopens, I. Activated Sludge Model (ASM) Based Modelling of Membrane Bioreactor (MBR) Processes: A Critical Review with Special Regard to MBR Specificities. Water Res. 2010, 44, 4272–4294. [Google Scholar] [CrossRef] [PubMed]


| Parameters | Unit | MCWWTP |
|---|---|---|
| Population | inhabitants | 150,000 |
| Average daily flow rate | m3.d−1 | 29,400 |
| Flow to discharge in case of rain | m3.h−1 | 30,312 |
| peak flow | m3.h−1 | 3266 |
| BOD load | kg.d−1 | 9614 |
| Suspended Solids | kg.d−1 | 17,640 |
| Recirculation Flow RAS | m3.h−1 | 1300 |
| i | Component | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| j | Process | SI | SS | XI | XS | XBH | XBA | XP | SO | SNO | SNH | SD | XND | SALK |
| 1-Aerobic growth of heterotrophs | 1 | |||||||||||||
| 2-Anoxic growth of heterotrophs | 1 | |||||||||||||
| 3-Aerobic growth of autotrophs | 1 | |||||||||||||
| 4-Decay of heterotrophs | 1 fP | 1 | fP | fP | ||||||||||
| 5-Decay of autotrophs | 1 fP | 1 | fP | fP | ||||||||||
| 6-Ammonification of soluble organic nitrogen | 1 | 1 | ||||||||||||
| 7-Hydrolysis of entrapped organics | 1 | 1 | ||||||||||||
| 8-Hydrolysis of entrapped organics nitrogen | 1 | 1 | ||||||||||||
| Parameters | Symbol | Unit | Default Value |
|---|---|---|---|
| Theoretical maximum sedimentation rate. | °F | m.j−1 | 712 |
| Maximum effective sedimentation rate. | m.j−1 | 340 | |
| Sedimentation parameter for highly concentrated suspensions. | m3.g−1 | 4.26 × 10−4 | |
| Sedimentation parameter for weakly concentrated suspensions. | m3.g−1 | 5.0 × 10−3 | |
| Unsettled fraction of incidental solids. | - | 5.0 × 10−4 | |
| Limit concentration of suspended solids. | g.m−3 | 3000 |
| Parameter | Unit | Min | Q1 | Median | Q3 | Max | Mean | SD |
|---|---|---|---|---|---|---|---|---|
| Influent Values | ||||||||
| TSS | mg/L | 76.00 | 210.00 | 261.00 | 335.00 | 583.00 | 280.44 | 123.84 |
| BOD | mgO2/L | 170.00 | 360.00 | 460.00 | 592.00 | 850.00 | 467.52 | 152.96 |
| COD | mgCOD/L | 190.00 | 555.50 | 653.00 | 918.00 | 1403.00 | 719.04 | 271.67 |
| NH4-N | mgN/L | 25.36 | 49.67 | 52.00 | 60.11 | 79.74 | 54.31 | 10.27 |
| NO3-N | mgN/L | 0.14 | 0.23 | 0.32 | 0.52 | 2.70 | 0.51 | 0.52 |
| NO2-N | mgN/L | 0.15 | 0.28 | 0.40 | 0.49 | 0.96 | 0.40 | 0.17 |
| PO4-P | mg/L | 7.30 | 10.40 | 11.90 | 14.90 | 21.50 | 12.65 | 3.28 |
| Temp | °C | 13.00 | 20.00 | 26.50 | 29.50 | 32.00 | 24.85 | 5.72 |
| PH | - | 7.05 | 7.37 | 7.57 | 7.95 | 8.21 | 7.63 | 0.33 |
| Effluent Values | ||||||||
| TSS | mg/L | 13.00 | 21.00 | 24.50 | 28.00 | 35.00 | 24.39 | 5.45 |
| BOD | mgO2/L | 4.00 | 20.00 | 23.50 | 26.50 | 36.00 | 22.68 | 8.40 |
| COD | mgCOD/L | 42.00 | 61.50 | 70.00 | 72.75 | 90.00 | 67.71 | 11.33 |
| NH4-N | mgN/L | 18.50 | 25.48 | 32.58 | 40.03 | 57.08 | 33.54 | 10.12 |
| NO3-N | mgN/L | 0.02 | 0.03 | 0.06 | 0.08 | 2.40 | 0.23 | 0.62 |
| NO2-N | mgN/L | 0.02 | 0.04 | 0.05 | 0.07 | 0.57 | 0.10 | 0.14 |
| PO4-P | mg/L | 2.10 | 5.63 | 10.05 | 17.70 | 34.80 | 13.03 | 9.40 |
| Temp | °C | 13.00 | 19.38 | 26.25 | 29.63 | 32.00 | 24.68 | 5.84 |
| PH | - | 6.77 | 7.35 | 7.69 | 7.96 | 8.19 | 7.62 | 0.39 |
| Parameter Fraction | Symbol | Ratio | Value gCOD/m3 | Reference |
|---|---|---|---|---|
| Soluble biodegradable substrate | SS | 0.32 | 230.10 | [49,50] |
| Soluble inert substrate | SI | 0.056 | 40.26 | [36] |
| Particulate biodegradable substrate | XS | 0.574 | 412.72 | Own Study [XS = TCOD − (SS + SI + XI)] |
| Particulate inert substrate | XI | 0.05 | 35.95 | [49,50] |
| Parameters | Symbol | Unit | Range | Default Values | Calibrated Values | References |
|---|---|---|---|---|---|---|
| Stoichiometric Parameters | ||||||
| Yield for heterotrophic biomass | YH | g COD/g COD | (0.57–0.67) | 0.67 | 0.66 | [52] |
| Yield for Autotrophic biomass | YA | g COD/g COD | (0.15–0.24) | 0.24 | 0.24 | [53] |
| volatile suspended solids/total suspended solids | VSS/TSS | g VSS/g TSS | - | 0.70 | 0.80 | [52] |
| particulate COD to total COD | XCOD/VSS1 | g COD/g VSS | - | 1.48 | 1.3 | [54] |
| Kinetic Parameters | ||||||
| Maximum specific growth rate for heterotrophic biomass | μ–max H | d−1 | (0.6–13.2) | 6 | 3.2 | [52] |
| Heterotrophic decay coefficient | bH | d−1 | (0.3–1.2) | 0.62 | 0.66 | [30] |
| Half saturation constant | Ks | mg3 COD/L | (10–40) | 20 | 20 | [30] |
| Parameter | Unit | Measurement | Simulation | RMSE |
|---|---|---|---|---|
| COD | mg/L | 67.71 | 70.25 | 0.037 |
| TSS | mg/L | 24.39 | 20.23 | 0.17 |
| NH4-N | mg/L | 33.54 | 21.03 | 0.37 |
| Parameter | Unit | Measurement | Simulation | RMSE |
|---|---|---|---|---|
| COD | mg/L | 66.75 | 70.44 | 0.23 |
| TSS | mg/L | 8.15 | 25.06 | 0.67 |
| NH4-N | mg/L | 14.54 | 32.27 | 0.56 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tiar, S.M.; Bessedik, M.; Abdelbaki, C.; ElSayed, N.B.; Badraoui, A.; Slimani, A.; Kumar, N. Steady-State and Dynamic Simulation for Wastewater Treatment Plant Management: Case Study of Maghnia City, North-West Algeria. Water 2024, 16, 269. https://doi.org/10.3390/w16020269
Tiar SM, Bessedik M, Abdelbaki C, ElSayed NB, Badraoui A, Slimani A, Kumar N. Steady-State and Dynamic Simulation for Wastewater Treatment Plant Management: Case Study of Maghnia City, North-West Algeria. Water. 2024; 16(2):269. https://doi.org/10.3390/w16020269
Chicago/Turabian StyleTiar, Sidi Mohamed, Madani Bessedik, Chérifa Abdelbaki, Nadia Badr ElSayed, Abderrahim Badraoui, Amaria Slimani, and Navneet Kumar. 2024. "Steady-State and Dynamic Simulation for Wastewater Treatment Plant Management: Case Study of Maghnia City, North-West Algeria" Water 16, no. 2: 269. https://doi.org/10.3390/w16020269
APA StyleTiar, S. M., Bessedik, M., Abdelbaki, C., ElSayed, N. B., Badraoui, A., Slimani, A., & Kumar, N. (2024). Steady-State and Dynamic Simulation for Wastewater Treatment Plant Management: Case Study of Maghnia City, North-West Algeria. Water, 16(2), 269. https://doi.org/10.3390/w16020269








