1. Introduction
The increase in the impervious area of the watershed due to urbanization causes a decrease in soil infiltration, an increase in direct runoff and flood peak, and an increase in nonpoint source pollution, thereby distorting the water circulation system. As an alternative measure to this, low-impact development (LID) practices, eco-friendly plans to efficiently manage urban stormwater by infiltrating and storing rainwater, have been proposed. As technical measures of the LID practices, vegetation reservoirs, water permeable pavement, retention ponds, grassed swales, rainwater tanks, constructed wetlands, and green roofs have been developed and applied. Among them, the term green roof refers to the greening of buildings so that plants can grow on the roof of a building.
It is often difficult to secure green spaces in a city center that has already been developed, but utilizing green roofs is a practice that makes it possible to create green spaces in the urban core. Green roofs are generally divided into the following four layers: the drainage layer, a filter that prevents the soil losses, the substrate layer, and vegetation [
1]. Green roofs have various application effects such as mitigating stormwater and heat island phenomena, saving energy for cooling and heating in buildings, and improving urban landscapes. Thanks to the advantages of green roofs, interest in the application of green roofs has been increasing domestically and internationally. Studies on monitoring changes in the quantity and quality of runoff water according to the installation of a green roof [
2,
3] and modeling studies to predict and evaluate the stormwater reduction efficiency [
4,
5,
6] have also been widely carried out.
Models commonly employed to simulate green roofs are EPA’s SWMM, SWMS-2D SWAP and HYDRUS [
7]. Among them, HYDRUS is a soil physics model for simulating the movement of water, heat, and solutes in porous media with various dimensions and shapes [
8]. Several studies have been conducted on the modeling of the runoff response of green roofs using HYDRUS [
4,
8,
9,
10,
11,
12].
Hilten [
4] carried out pioneering work in the USA that used HYDRUS-1D for green roof runoff simulations and showcased its capability for the first time. Similar efforts were continued in Hawaii, USA, by Li and Babcock [
8] to derive practical regression equations that predict the hydrologic performance of green roofs to be used for design purposes. More recently, Yang and Davidson [
11] have studied the aging effect of green roofs in the USA and found improvements in retention and detention performance with aging, showing that HYDRUS-1D can replicate the conditions.
In other parts of the world, Fietosa and Wilkinson [
9] studied stormwater response regarding green roofs in New Zealand for different depths using HYDRUS-1D. Peczkowski et al. [
10] investigated the efficiency of runoff reduction by green roofs in Poland for variable substrate thicknesses using HYDRUS-1D. Wang et al. [
12] explored the hydrological response of green roofs in the USA with different capacities in regard to water storage layers and water storage on the soil surface through simulated rainfall experiments and HYDRUS-1D. Research around the world was seen to be maturing, accounting for various aspects of green roofs in mitigating stormwater runoff.
Many factors influence the capacity of green roofs to reduce urban runoff, such as evaporation and transpiration, initial moisture conditions, rainfall intensity, and the hydraulic properties of the soils. Of these factors, evapotranspiration is an important mechanism for soil moisture removal in regard to restoring its retention capacity [
13]. HYDRUS-1D allows users to input evapotranspiration estimates that have been pre-determined outside [
9]. Therefore, users are faced with the difficulty of choosing a particular evapotranspiration method. However, there is no study on the comparison of possible estimation methods for evapotranspiration from green roofs.
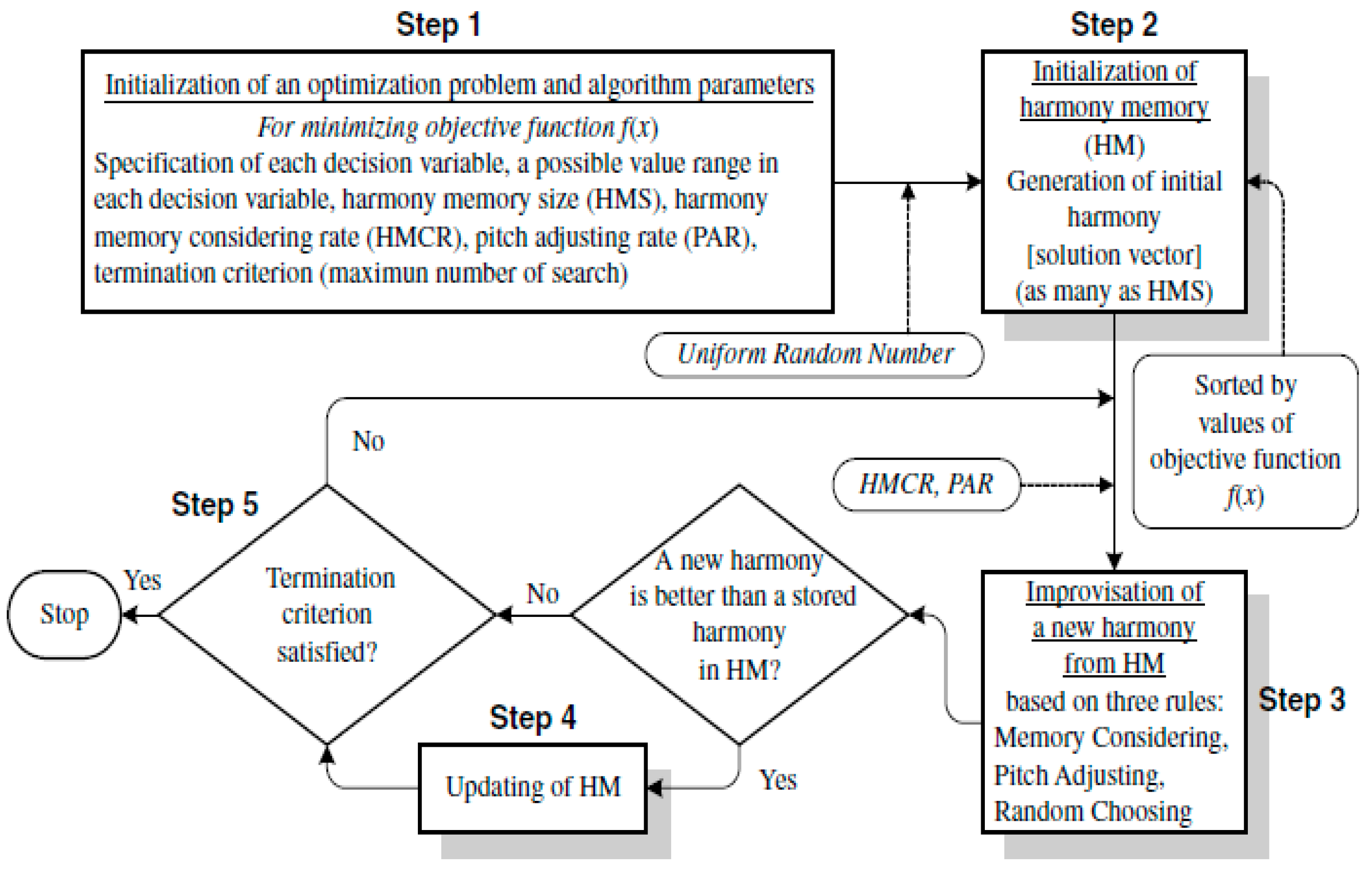
Estimating the parameters of the model is another essential task in accurately simulating the effectiveness of green roofs prior to installation [
4]. Since the HYDRUS-1D model itself includes an optimization module for hydraulic parameters (
, many studies using this module [
14,
15,
16] have been performed. However, its performance was not compared against other competitive optimization schemes for alternative considerations. According to the foregoing discussions, major difficulties and challenges for the modeler’s considering HYDRUS include finding a way to account for the important loss mechanisms of evapotranspiration from green roofs and, also, establishing how model parameters can be fitted. In this study, we aimed to find appropriate evapotranspiration and parameter optimization schemes for HYDRUS-1D, a commonly used modeling tool for green roofs. A comparative study of this sort in the context of modeling green roof runoff has not been conducted previously and is important in regard to guiding modelers who may be interested in using HYDRUS-1D for an accurate prediction of runoff from a green roof, thus improving knowledge over the cases discussed in the literature.
For evapotranspiration methods, two simple evapotranspiration schemes are considered. They are the Hargreaves method and Blaney–Criddle method, which have been applied many times in the literature [
17,
18]. As for optimizations, HYDRUS’ own inverse solution module is compared against the harmony search algorithm, the excellence of which in regard to optimization has also been recognized in various engineering fields [
19] as a possible alternative.
4. Conclusions
In this study, not only were the Hargreaves formula and the Blaney–Criddle method compared, but the HYDRUS’ own optimization module (inverse solution) and the optimization method using the harmony search algorithm were compared and analyzed to find evapotranspiration and optimization schemes that have better applicability for green roof runoff modeling.
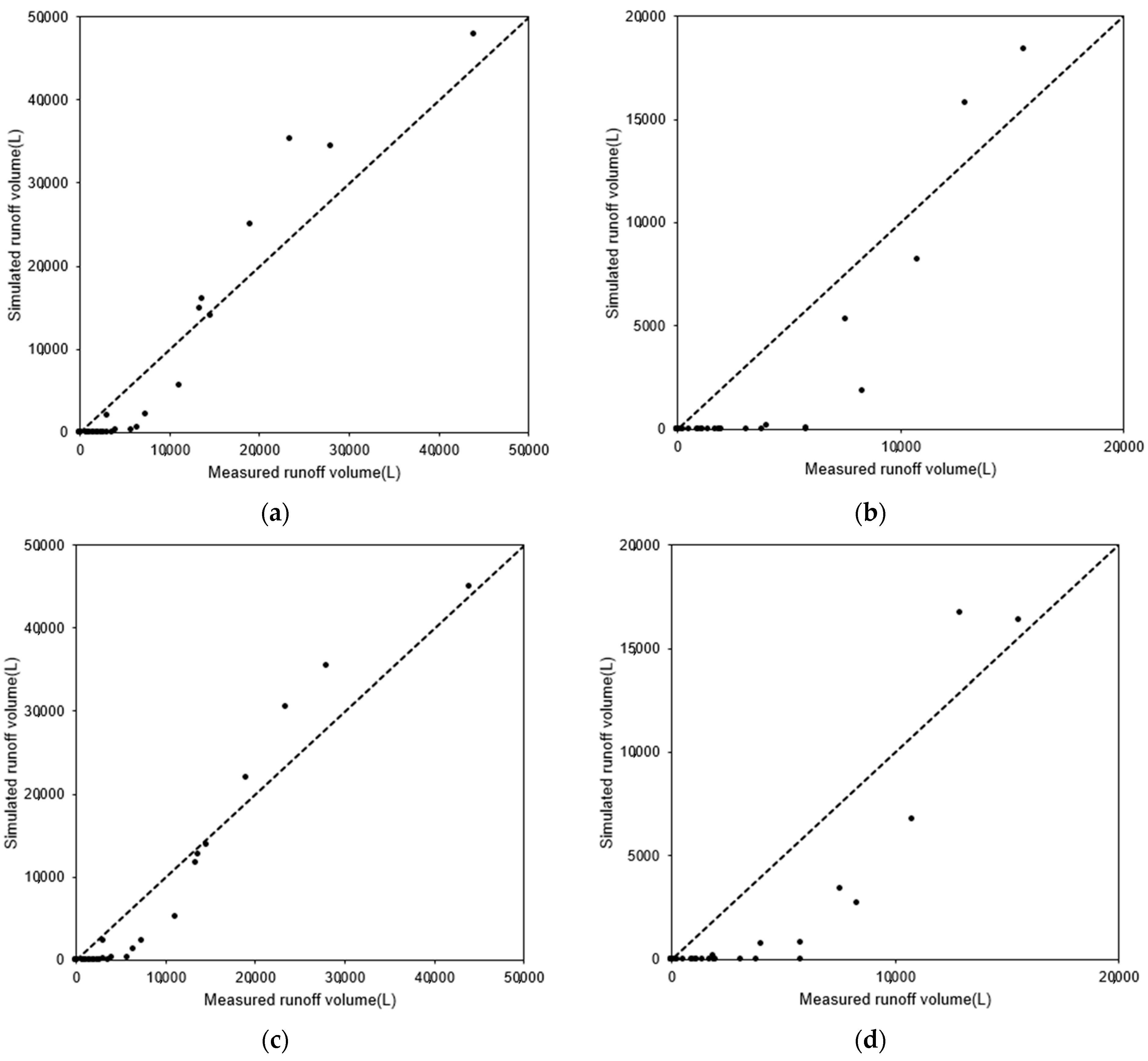
From the results of the applicability evaluation, it was found that the applicability when using the Blaney–Criddle method was better than the one using the Hargreaves method for both optimization methods—HYDRUS’ own optimization module and the harmony search algorithm. In particular, the applicability results when using the Blaney–Criddle method in HYDRUS’ own optimization module were much better (R
2 = 0.82) than the applicability results when using the Hargreaves formula (R
2 = 0.46). This is in resemblance to the findings in Limos et al. [
29], where the Blaney–Criddle evapotranspiration calculation method was found to be the most applicable in green roof runoff modeling. HYDRUS’ own optimization module had shown a good applicability evaluation index; however, except for the objective function specified by itself, it is limited by the aspect that other useful performance statistics are unable to be designated as objective functions.
In the case of using the harmony search algorithm, both the NSE (0.9) and R
2 (0.95) values were higher compared to the ones (NSE = 0.73; R
2 = 0.82) obtained by HYDRUS-1D’s own optimization module. This seems to be due to a limitation of HYDRUS-1D’s optimization module, as other useful objective functions, such as NSE and R
2, which may improve its performance, cannot be specified. In contrast, the harmony search algorithm gives the flexibility to the users to choose the applicability evaluation indices so that the parameters can be optimized for the best possible performance. The harmony search algorithm has been successfully applied in green infrastructure modeling [
40,
41]. Therefore, in this study, the harmony search algorithm was proposed as an alternative parameter optimization method for predicting green roof runoff when using HYDRUS-1D, which is expected to be useful for future studies experimenting with various evaluation indices as objective functions.
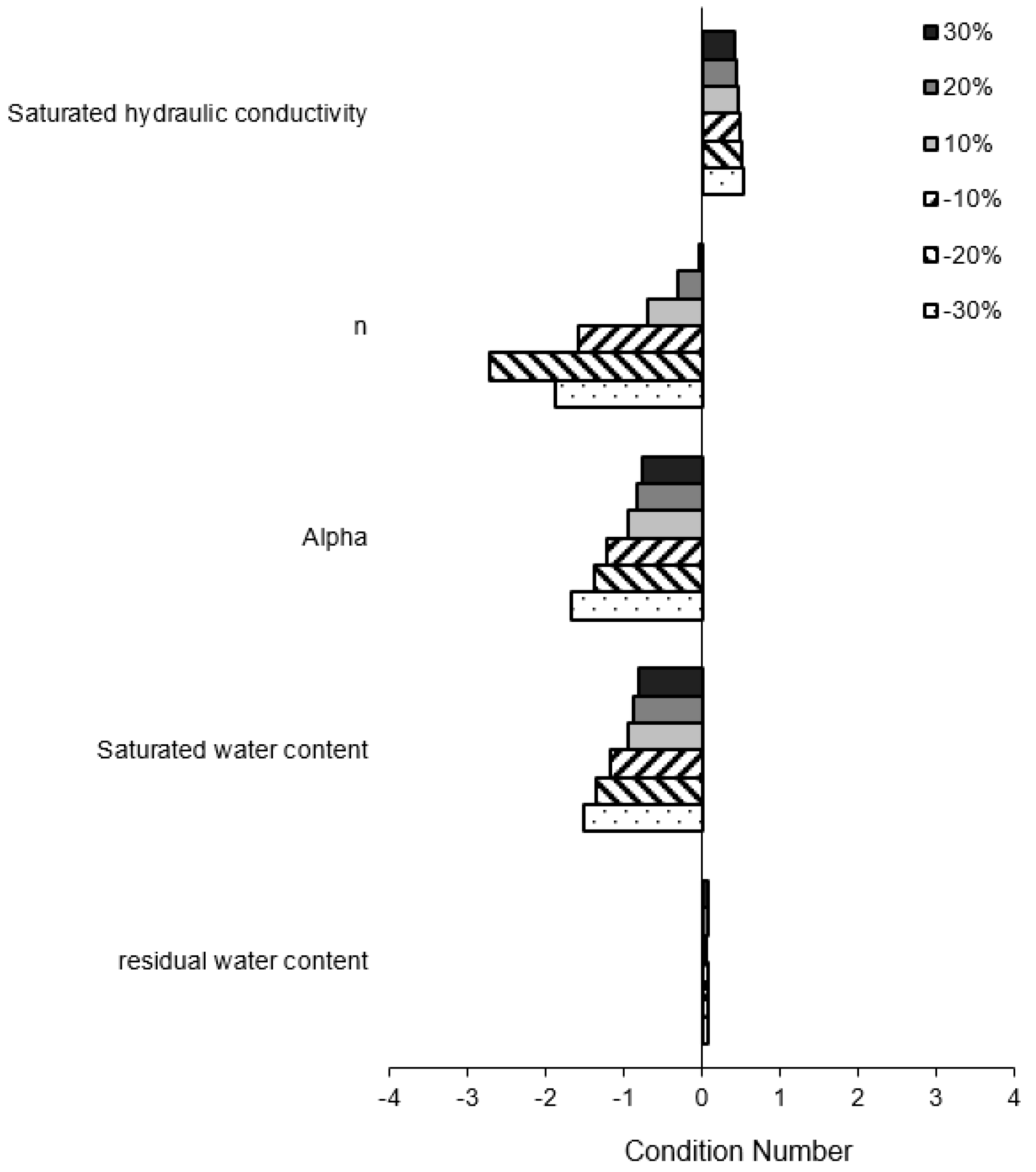
Lastly, the sensitivity analysis in the form of condition numbers showed that the parameters of n, alpha, and saturation water content were noticeably larger than other parameters, emphasizing the importance of these parameters in obtaining the good fit of the runoff volume predicted by the HYDRUS-1D model. This was similarly found in other studies [
32,
33].
Overall, the findings in this study should help potential users considering using HYDRUS-1D to model green roof performance for stormwater mitigation in selecting evapotranspiration and optimization es, which are important for accurately predicting green roof runoff responses to various rainfall conditions.