Irrotational Flow over Ogee Spillway Crest: New Solution Method and Flow Geometry Analysis
Abstract
1. Introduction

2. Governing Equations
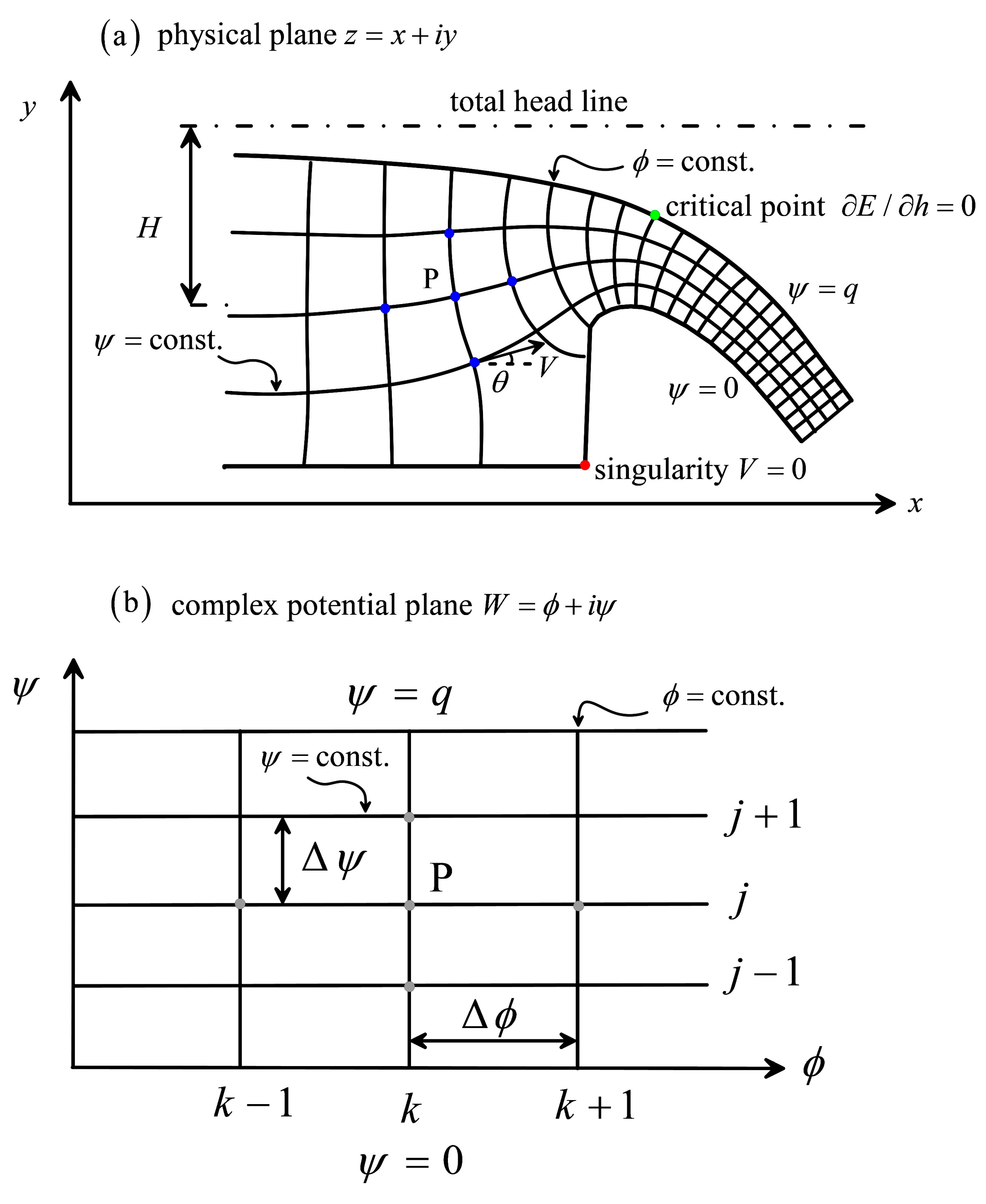
2.1. Complex Potential Plane
2.2. Free Surface
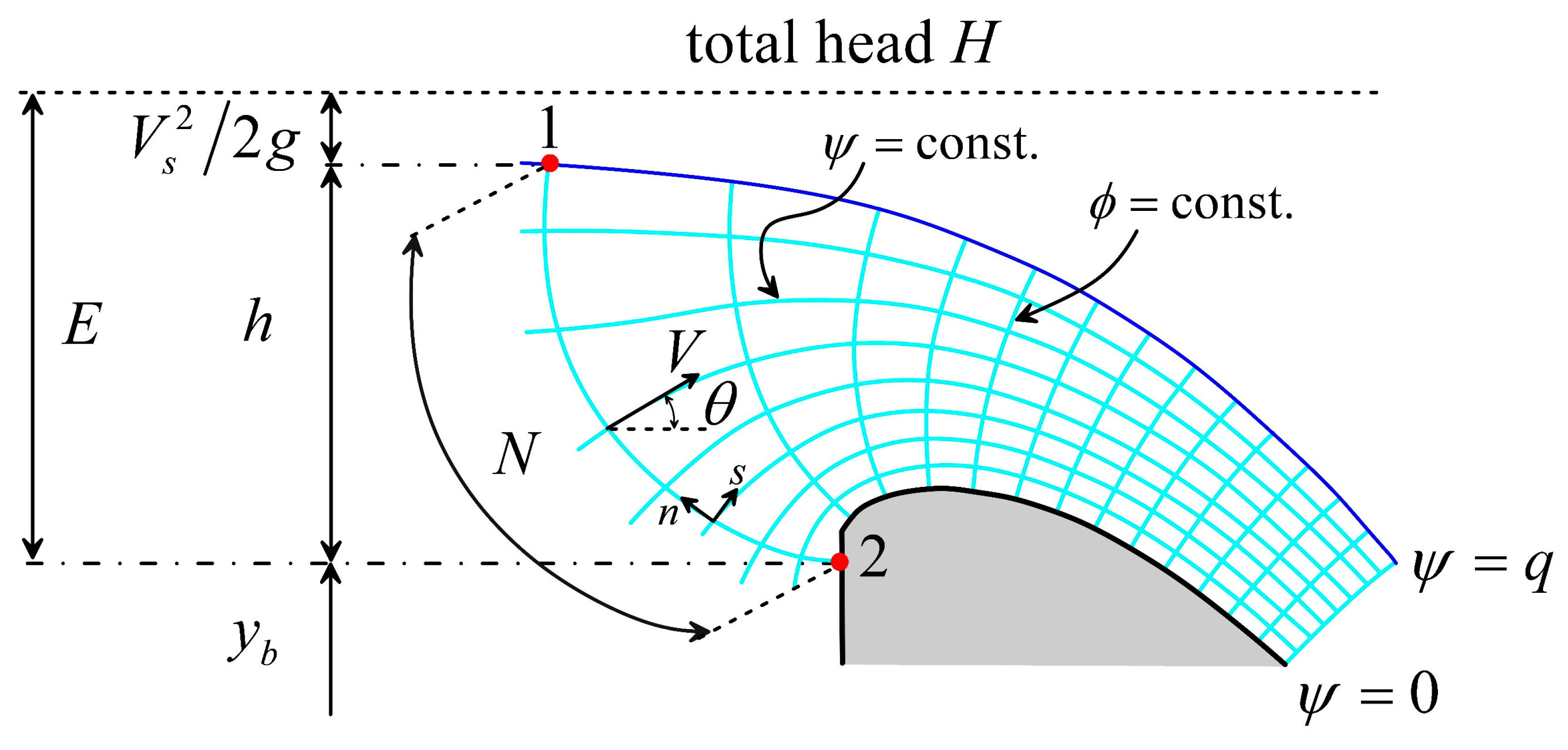
2.3. Critical Flow
2.4. Flow Curvature
2.5. Singularity
2.6. Flow Net Geometry and Jaeger’s Approximation
3. Solution Method
3.1. Iterative Solution
3.2. Solution Checks
3.3. Post-Processing of Results
- The first one consists of a 6th-order polynomial fitting R(k, j) = R(k,1) + ∂R/∂n(k,1)n(k,1) + … to the function R(n) within the lower third of the streamlines used to model the flow, forcing the polynomial to satisfy the bed value Rb = R(k,1) at n = 0. This process represents for each equipotential line the near-bed variation in the radius of streamline curvature analytically; thus, it can be adopted as a discrete approximation of the truncated Taylor series Equation (35). The value of K follows from Equation (37).
- The second consists of performing the linear fitting of Equation (38) to each equipotential using all the streamlines characterizing the flow, forcing it to satisfy the bed value Rb = R(k,1) at n = 0. This gives an average K value along each equipotential in a least-squares sense.
4. Results
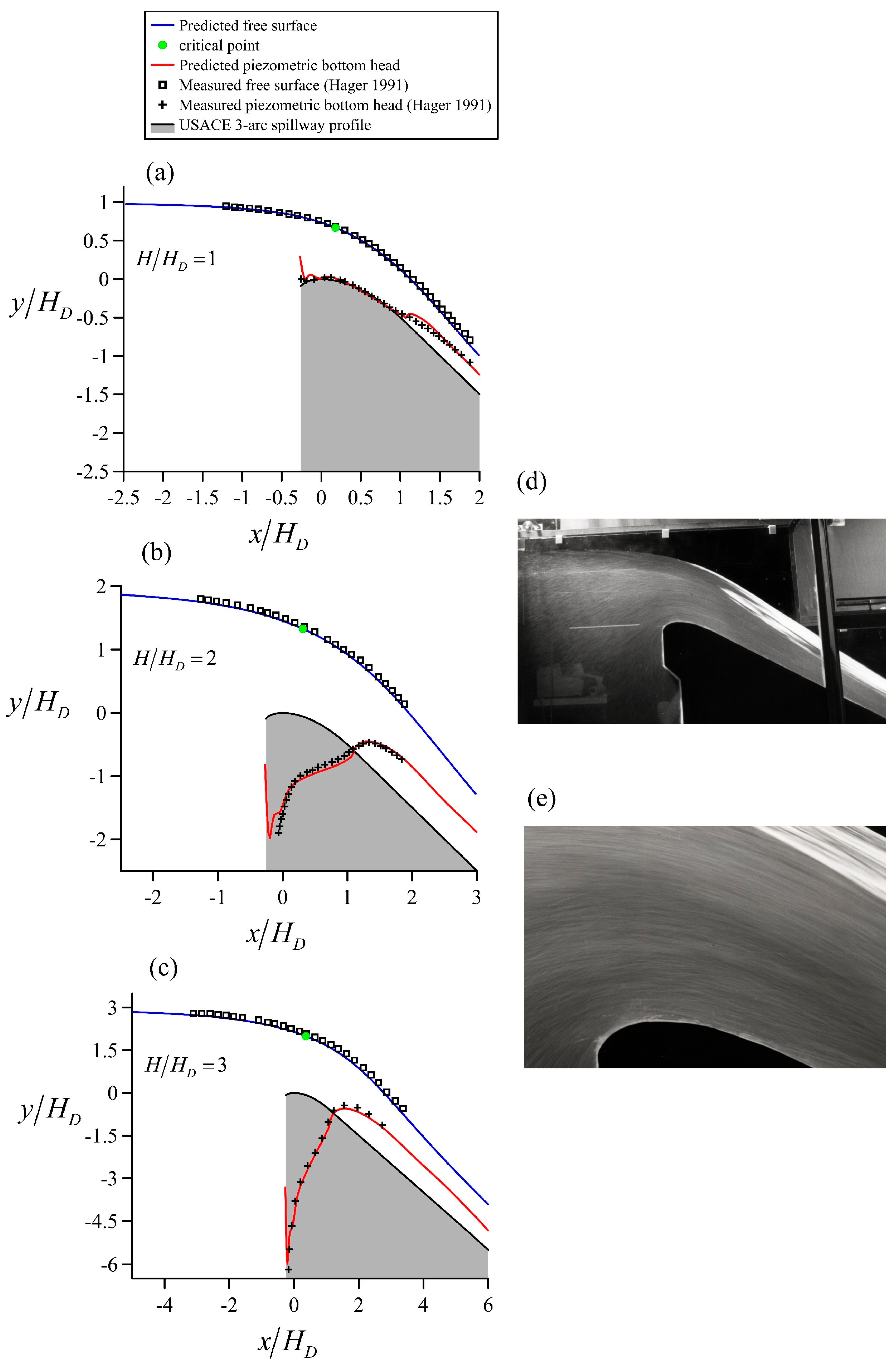
4.1. Free Surface and Bottom Pressure Head
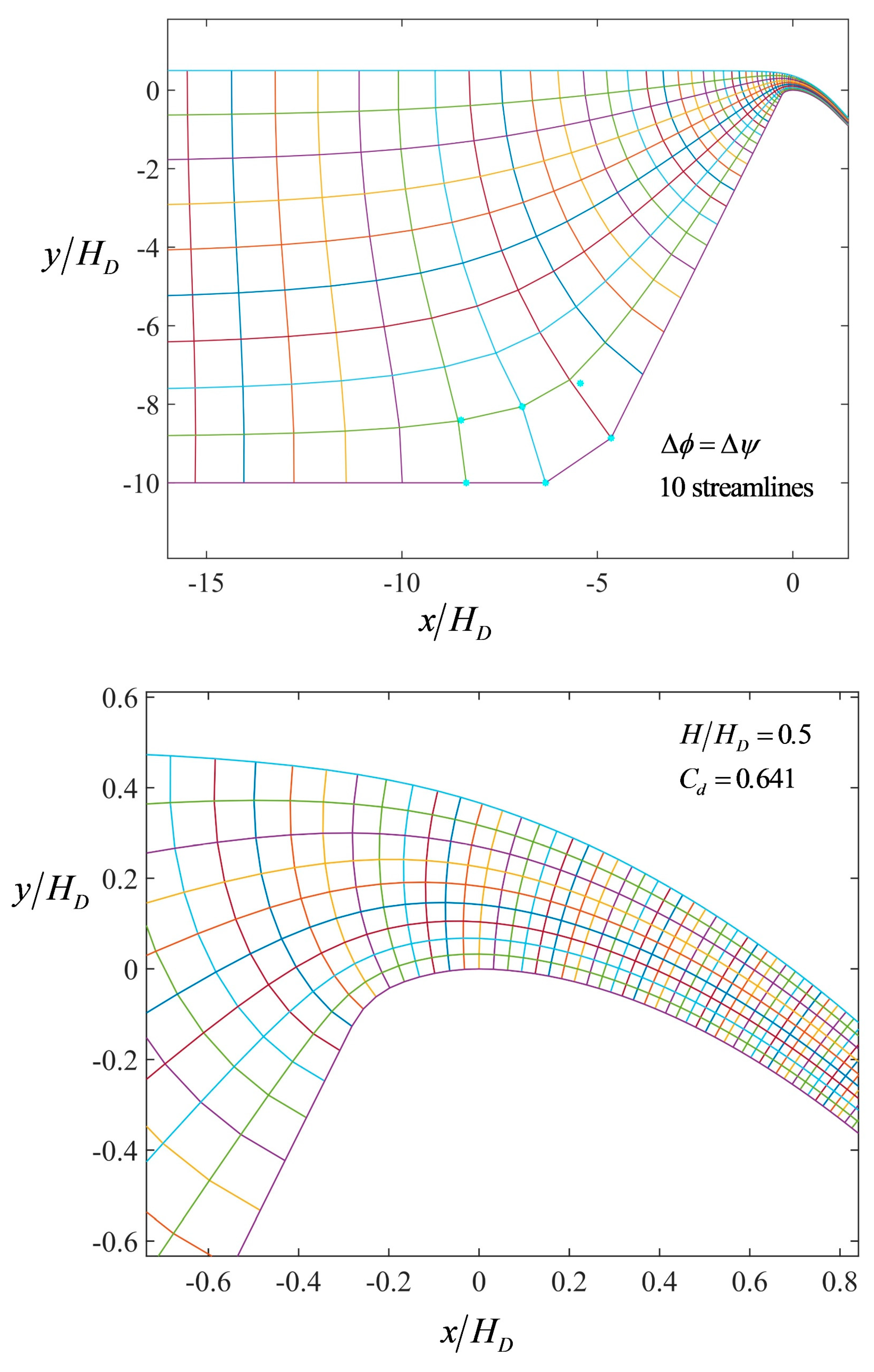
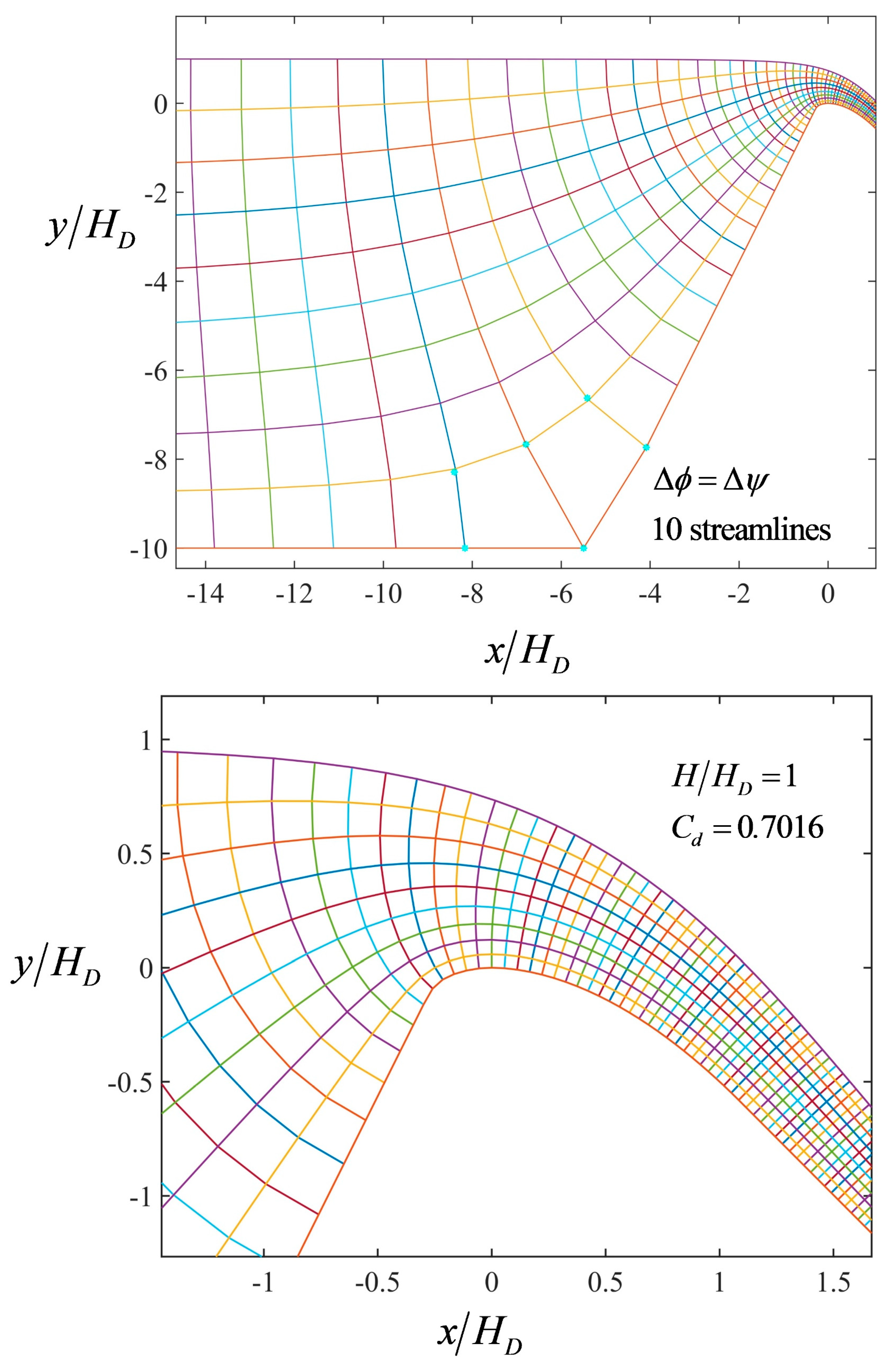
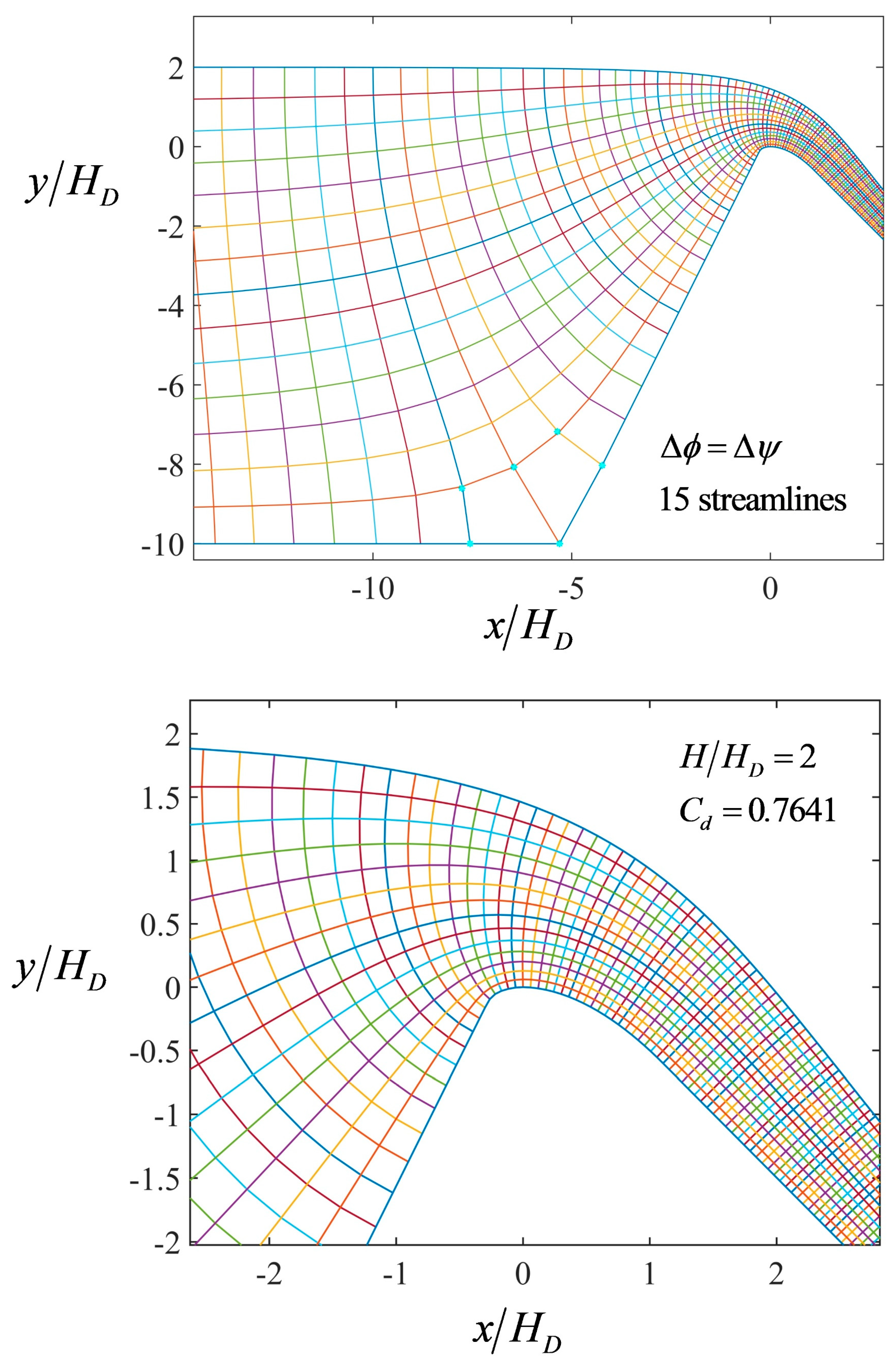
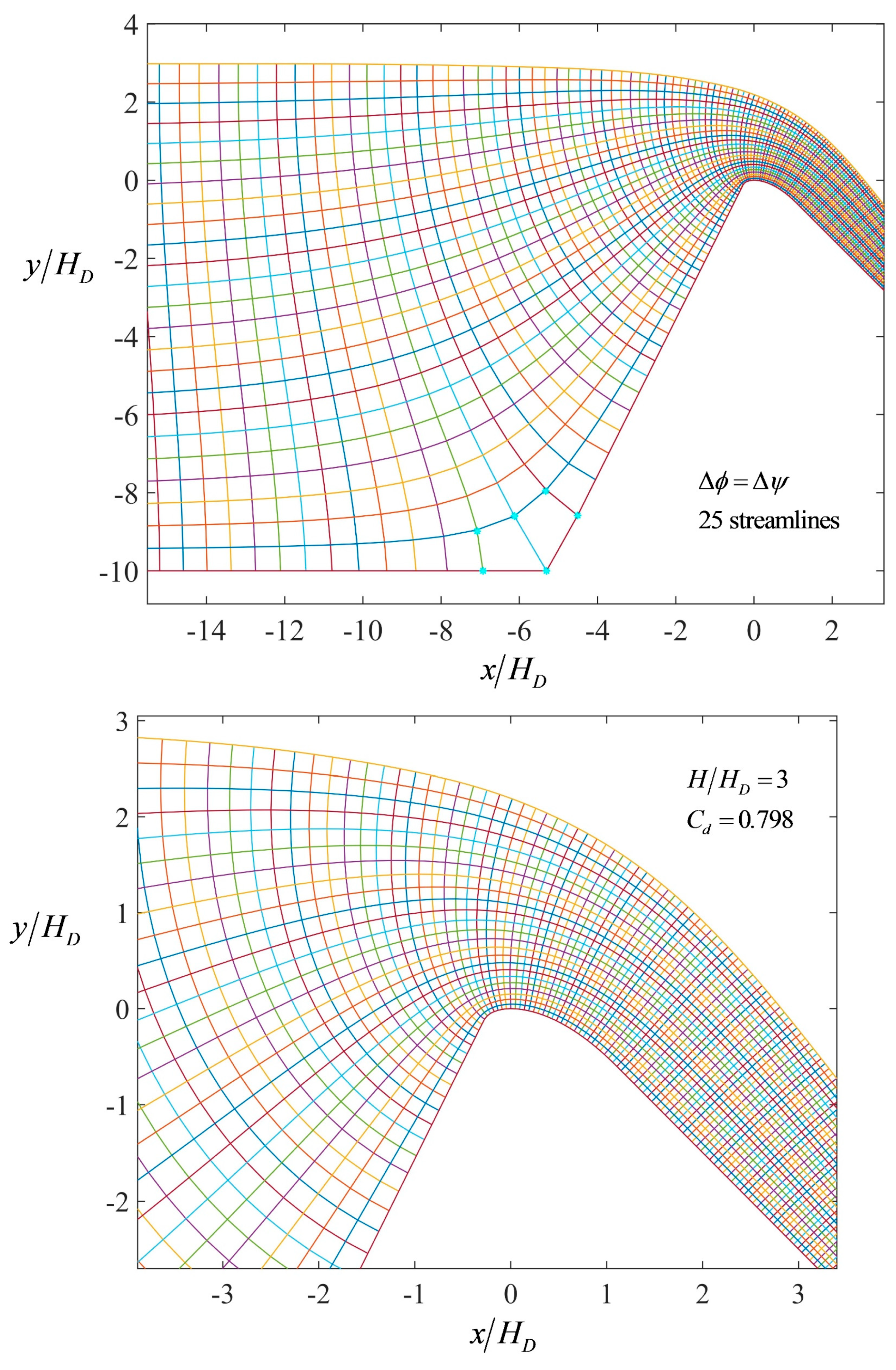
4.2. Flow Net
4.3. Velocity Field
4.4. Flow Geometry
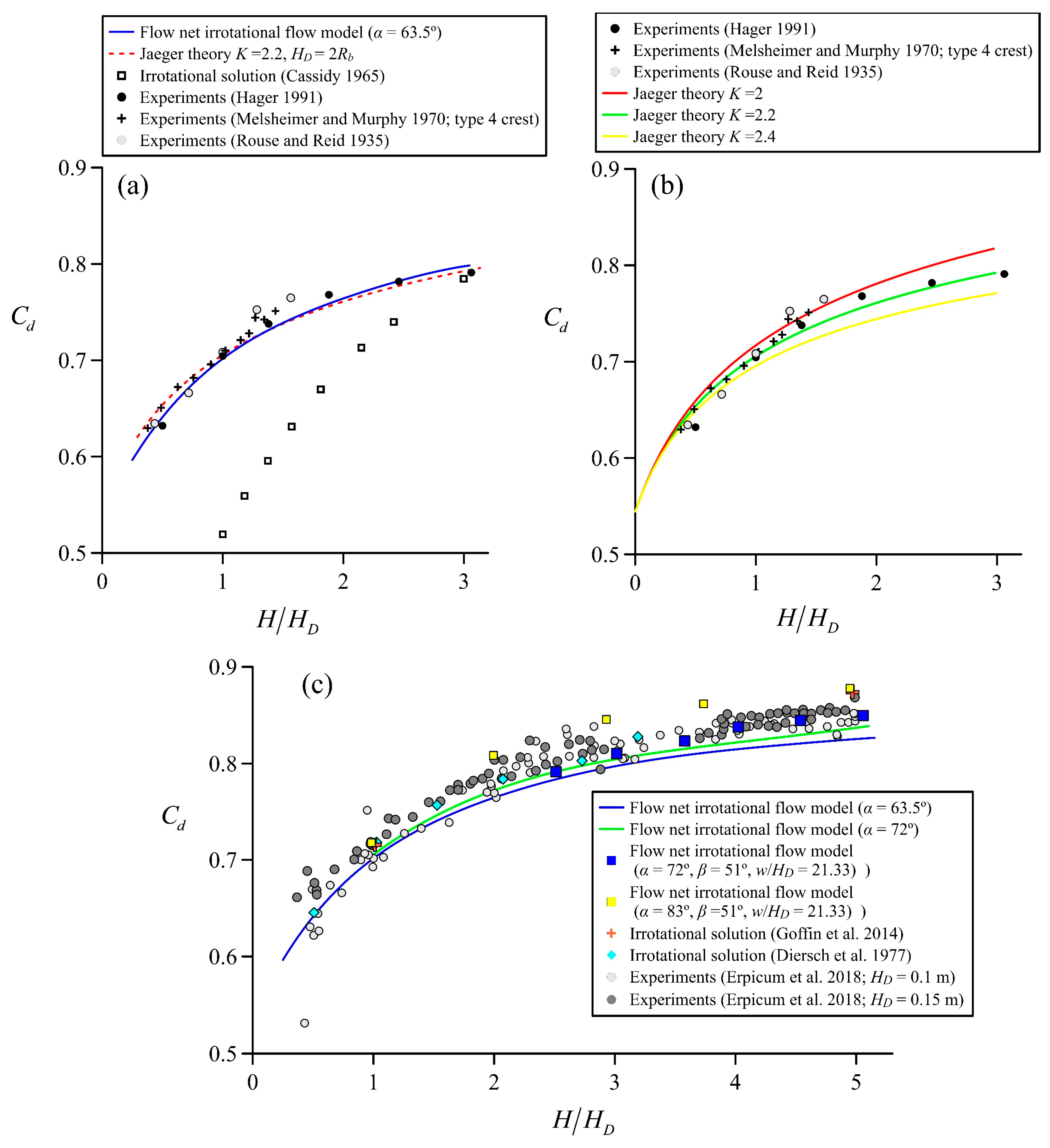
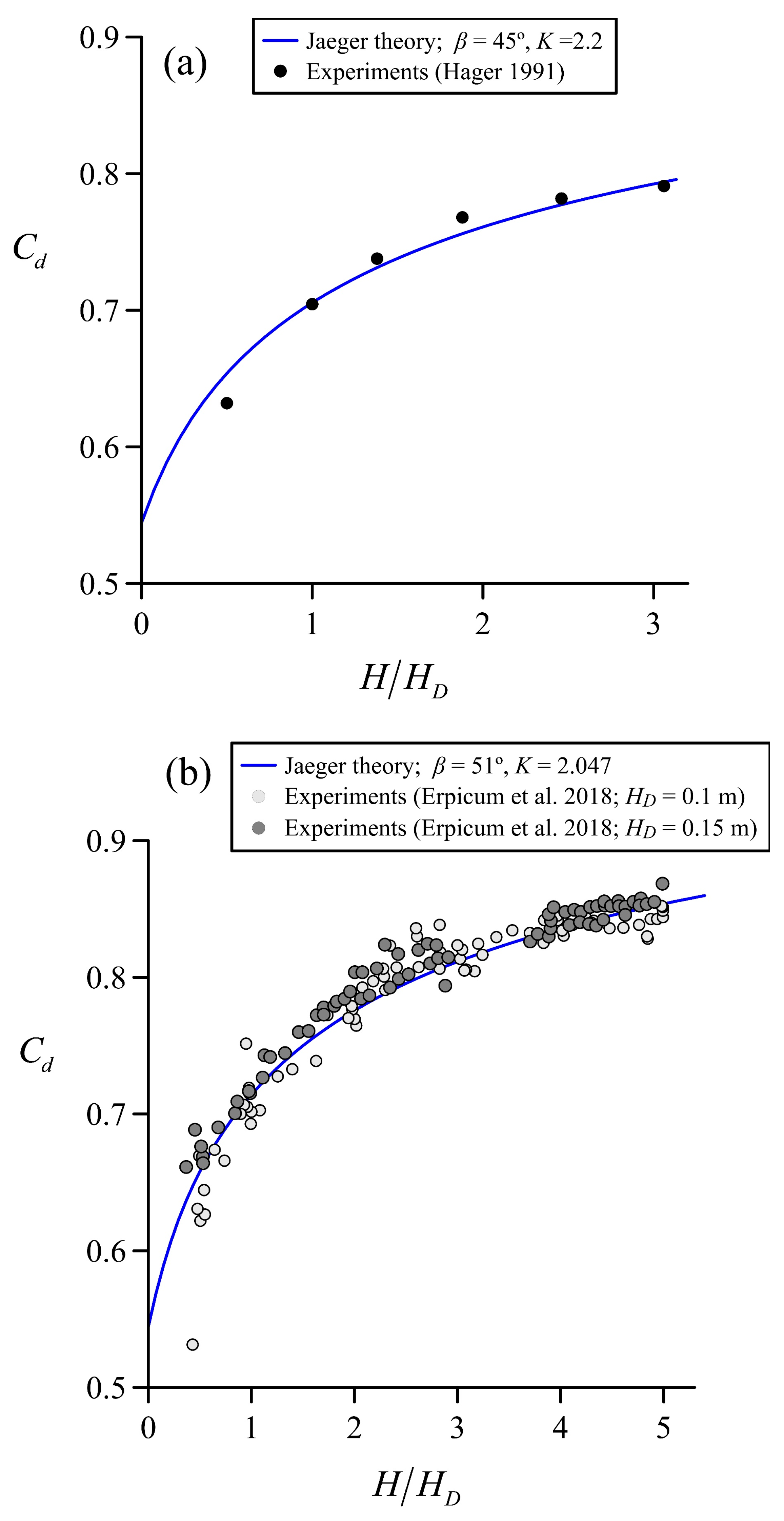
4.5. Discharge Coefficient
5. Limitations of the Proposed Method and Practical Utility
- -
- Complex flows involving turbulence and hydraulic jumps are excluded, like those which may appear at low weirs for high tailwater levels. It is the same with lateral contraction effects at abutments and piers, which are not considered in this study.
- -
- According to Vermeyen [72], under ideal entrance conditions, e.g., no lateral flow contraction when approaching the spillway, H/HD can reach a value of five on a spillway designed using the recommendation of USBR. However, under this flow condition, the nappe is unstable, and separation can occur from very small surface disturbances. Erpicum et al.’s [12] experiments conducted with WES profiles showed that at up to H/HD = 5 the flow is attached to the spillway surface, while separation occurs at H/HD = 5.5 [12]. The proposed method assumes that the flow is perfectly attached to the spillway surface, thus it cannot be applied for H/HD > 5, which was the upper limit adopted in our study.
- -
- For a standard ogee spillway, the design charts of USACE are available for limited operational flow conditions, e.g., up to H/HD = 1.5. However, in many circumstances, one may need data beyond that limit. For example, in dams experiencing the requirement to pass increased discharges because of a revised probable maximum flood, operational conditions may be much above H/HD = 1.5. This means that it may be necessary to investigate the safety conditions of the dam by estimating spillway operation for high overflow conditions, e.g., those up to H/HD = 5 [13,72]. Such operation above the design head is not well known and requires investigation. For instance, it has been shown in this work that the chute slope has a notable impact on Cd for heads 3 < H/HD < 5. To our best knowledge, this has never been previously reported.
- -
- Real spillway crests at existing dams may not correspond to standard crest shapes. For practical construction reasons (simplifications by approximate geometries or deviations during execution) or simply because the dam commissioning date was far before the proposal of the currently recommended ogee crest profile, the actual profiles of spillways rarely correspond exactly to the theoretical profile [12,13]. The dams operated by Electricité de France (EDF) are no exception to this observation, particularly for the upstream quadrant of the spillways, as illustrated by Erpicum et al. [13] for 27 real dams in France. This great heterogeneity of the upstream quadrant profiles can have a significant impact on spillway flow features. It is well known that the geometry of the upstream quadrant is of paramount importance in spillway operation [68], with a significant impact on the flow features. For these dams, design charts are not available, and the proposed theoretical method can be used to generate a fast analysis of the operational conditions using the real spillway profile.
- -
- Finally, the proposed model may be used to propose new designs for the overflow crest of a spillway considering cavitation conditions [2], given that the bottom pressure distribution is easily obtained.
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hager, W.H.; Schleiss, A. Constructions hydrauliques: Ecoulements Stationnaires (Hydraulic Structures: Steady Flows); Traité de génie civil, 15; Presses Polytechniques Universitaires Romandes: Lausanne, Switzerland, 2009. (In French) [Google Scholar]
- Hager, W.H.; Schleiss, A.J.; Boes, R.M.; Pfister, M. Hydraulic Engineering of Dams; CRC Press: London, UK, 2021. [Google Scholar]
- Creager, W.P. Engineering for Masonry Dams; Wiley and Sons: New York, NY, USA, 1917. [Google Scholar]
- USACE. Hydraulic Design Criteria; U.S. Army Waterways Experiment Station: Vicksburg, MI, USA, 1987. [Google Scholar]
- USACE. Hydraulic Design of Spillways; U.S. Army Corps of Engineers: Washington, DC, USA, 1990. [Google Scholar]
- Melsheimer, E.S.; Murphy, T.E. Investigations of Various Shapes of the Upstream Quadrant of the Crest of a High Spillway: Hydraulic Laboratory Investigation; U.S. Army Engineer Waterways Experiment Station: Vicksburg, MS, USA, 1970. [Google Scholar]
- Murphy, T.E. Spillway Crest Design; U.S. Army Engineer Waterways Experiment Station: Vicksburg, MS, USA, 1973. [Google Scholar]
- Maynord, S.T. General Spillway Investigation: Hydraulic Model Investigation; U.S. Army Engineer Waterways Experiment Station: Vicksburg, MS, USA, 1985. [Google Scholar]
- Reese, A.J.; Maynord, S.T. Design of Spillway Crests. J. Hydraul. Eng. 1987, 113, 476–490. [Google Scholar] [CrossRef]
- Knapp, F.H. Ausfluss, Überfall und Durchfluss im Wasserbau (Outflow, Overflow and Throughflow in Hydraulic Engineering); Braun: Karlsruhe, Germany, 1960. (In German) [Google Scholar]
- Hager, W.H. Continuous crest profile for standard spillway. J. Hydraul. Eng. 1987, 113, 1453–1457. [Google Scholar] [CrossRef]
- Erpicum, S.; Blancher, B.; Vermeulen, J.; Peltier, Y.; Archambeau, P.; Dewals, B.; Pirotton, M. Experimental study of Ogee crested weir operation above the design head and influence of the upstream quadrant geometry. In Proceedings of the 7th International Symposium on Hydraulic Structures, Aachen, Germany, 15 May 2018. [Google Scholar]
- Erpicum, S.; Pirotton, M.; Blancher, B.; Vermeulen, J. Influence de la géométrie du quadrant amont et comportement hydraulique sous forte charge des seuils profilés standards. La Houille Blanche 2019, 105, 40–47. [Google Scholar] [CrossRef]
- Peltier, Y.; Dewals, B.; Archambeau, P.; Pirotton, M.; Erpicum, S. Pressure and Velocity on an Ogee Spillway Crest Operating at High Head Ratio: Experimental Measurements and Validation. J. Hydro-Environ. Res. 2018, 19, 128–136. [Google Scholar] [CrossRef]
- Erpicum, S.; Crookston, B.; Bombardelli, F.; Bung, D.; Felder, S.; Mulligan, S.; Oertel, M.; Palermo, M. Hydraulic structures engineering: An evolving science in a changing world. Wiley Interdiscip. Rev. Water 2021, 8, e1505. [Google Scholar] [CrossRef]
- Rouse, H. The Distribution of Hydraulic Energy in Weir Flow in Relation to Spillway Design. Master’s Thesis, MIT, Boston, MA, USA, 1932. [Google Scholar]
- Escande, L. Détermination pratique du profile optimum d’un barrage déversoir: Tracé des piles par les méthodes aérodynamiques. Application à un ouvrage déterminé (Practical determination of the optimum weir profile: Pier shape using aerodynamic methods, application to prototype structure). Sci. Ind. 1993, 17, 467–474. (In French) [Google Scholar]
- Ishihara, T.; Iwasa, Y.; Ihda, K. Basic studies on hydraulic performances of overflow spillways and diversion weirs. Bull. Disaster Prev. Res. Inst. 1960, 33, 1–30. [Google Scholar]
- Sivakumaran, N.S.; Hosking, R.J.; Tingsanchali, T. Steady shallow flow over a spillway. J. Fluid Mech. 1981, 111, 411–420. [Google Scholar] [CrossRef]
- Vallentine, H.R. Applied Hydrodynamics; Butterworths: London, UK, 1969. [Google Scholar]
- Castro-Orgaz, O.; Hager, W.H. Non-Hydrostatic Free Surface Flows; Advances in Geophysical and Environmental Mechanics and Mathematics; Springer: Berlin/Heidelberg, Germany, 2017; 696p. [Google Scholar] [CrossRef]
- Katopodes, N.D. Free Surface Flow: Computational Methods; Butterworth-Heinemann: Oxford, UK, 2019. [Google Scholar]
- Rouse, H. Fluid Mechanics for Hydraulic Engineers; McGraw-Hill: New York, NY, USA, 1938. [Google Scholar]
- Castro-Orgaz, O. Hydraulics of developing chute flow. J. Hydraul. Res. 2009, 47, 185–194. [Google Scholar] [CrossRef]
- Ikegawa, M.; Washizu, K. Finite element method applied to analysis of flow over a spillway crest. Int. J. Numer. Methods Eng. 1973, 6, 179–189. [Google Scholar] [CrossRef]
- Nakayama, T.; Ikegawa, M. Finite element analysis of flow over a weir. Comput. Struct. 1984, 19, 129–135. [Google Scholar] [CrossRef]
- Henderson, H.C.; Kok, M.; De Koning, W.L. Computer-aided spillway design using the boundary element method and non-linear programming. Int. J. Numer. Methods Fluids 1991, 13, 625–641. [Google Scholar] [CrossRef]
- Ding, D.Y.; Liu, M.L. Mathematical model of flow over a spillway dam. In Proceedings of the 13th International Congress on Large Dams, New Delhi, India, 29 October–2 November 1979; Volume Q50, pp. 959–976. [Google Scholar]
- Ding, D.Y. A numerical method of flow over spillway dam with unknown energy head. Acta Mech. Sin. 1985, 17, 300–308. (In Chinese) [Google Scholar]
- Guo, Y.; Wen, X.; Wu, C.; Fang, D. Numerical modelling of spillway flow with free drop and initially unknown discharge. J. Hydraul. Res. 1998, 36, 785–801. [Google Scholar]
- Tu, R. Free Boundary Potential Flow Using Finite Elements. Ph.D. Thesis, University of Arizona, Department of Civil Engineering and Engineering Mechanics, Tucson, AZ, USA, 1971. [Google Scholar]
- Cheng, A.H.-D.; Liggett, J.A.; Liu, P.L.-F. Boundary calculations of sluice and spillway flows. J. Hydraul. Div. 1981, 107, 1163–1178. [Google Scholar] [CrossRef]
- Liggett, J.A. The Boundary Element Method. Engineering Applications of Computational Hydraulics; Pitman Publisher: Boston, MA, USA, 1982; Chapter 8; Volume I. [Google Scholar]
- Lai, C.; Hromadka, T.D. Modeling complex two-dimensional flows by the complex-variable boundary-element method. In Proceedings of the International Symposium on Refined Flow Modelling and Turbulence Measurements, Iowa City, IA, USA, 16–18 September 1985; G-26-1/10;. University of Iowa: Iowa City, IA, USA, 1985; Volume II. [Google Scholar]
- Barbier, C. Computer algebra and transputers applied to the finite element method. Ph.D. Thesis, Durham University, Durham, UK, 1992. [Google Scholar]
- Castro-Orgaz, O.; Hager, W.H. Analytical determination of irrotational flow profiles in open-channel transitions. Water 2023, 15, 4217. [Google Scholar] [CrossRef]
- Thom, A.; Apelt, C. Field Computations in Engineering and Physics; Van Nostrand: London, UK, 1961. [Google Scholar]
- Cassidy, J.J. Spillway Discharge at Other than Design Head; State University of Iowa: Iowa City, IA, USA, 1964. [Google Scholar]
- Cassidy, J.J. Irrotational flow over spillways of finite height. J. Eng. Mech. Div. 1965, 91, 155–173. [Google Scholar] [CrossRef]
- Diersch, H.J.; Schirmer, A.; Busch, K.F. Analysis of flows with initially unknown discharge. J. Hydraul. Div. 1977, 103, 213–232. [Google Scholar] [CrossRef]
- Escande, L. Sur L’emploi des Barrages Déversoirs à Seuil Creager Déprimé (On the Use of Creager-Crested Dams). Chapitre VII in Nouveaux Compléments d’Hydraulique; Nº280; Publications Scientifiques et Techniques du ministère de L’Air: Paris, France, 1953. (In French) [Google Scholar]
- Jaeger, C. Engineering Fluid Mechanics; Blackie and Son: Edinburgh, UK, 1956. [Google Scholar]
- Fawer, C. Etude de quelques écoulements permanents à filets courbes (Study of some steady flows with curved streamlines). Ph.D. Thesis, Université de Lausanne, La Concorde, Lausanne, Switzerland, 1937. (In French). [Google Scholar]
- Jaeger, C. Remarques sur quelques écoulements le long des lits à pente variant graduellement (Remarks on some flows along bottoms of gradually varied slope). Schweiz. Bauztg. 1939, 114, 231–234. (In French) [Google Scholar]
- Sananes, F. Étude de l’écoulement sur un seuil circulaire muni d’une fente aspiratrice (Study of flow over a circular sill with an air-entraining slot). Comptes Rendus Académie Sci. 1955, 241, 1262–1264. [Google Scholar]
- Sananes, F. Étude du décollement de la veine dans l’écoulement au-dessus d’un seuil déversant (Study of flow separation above a weir). Comptes Rendus L’académie Sci. 1958, 246, 1971–1973. [Google Scholar]
- Castro-Orgaz, O. Curvilinear flow over round-crested weirs. J. Hydraul. Res. 2008, 46, 543–547. [Google Scholar] [CrossRef]
- Castro-Orgaz, O.; Giraldez, J.V.; Ayuso, J.L. Critical flow over circular crested weirs. J. Hydraul. Eng. 2008, 134, 1661–1664. [Google Scholar] [CrossRef]
- Castro-Orgaz, O.; Giraldez, J.V.; Ayuso, J.L. Higher order critical flow condition in curved streamline flow. J. Hydraul. Res. 2008, 46, 849–853. [Google Scholar] [CrossRef]
- Stilmant, F.; Erpicum, S.; Peltier, Y.; Archambeau, P.; Dewals, B.; Pirotton, M. Flow at an Ogee Crest Axis for a Wide Range of Head Ratios: Theoretical Model. Water 2022, 14, 2337. [Google Scholar] [CrossRef]
- Castro-Orgaz, O. Weakly undular hydraulic jump: Effects of friction. J. Hydraul. Res. 2010, 48, 453–465. [Google Scholar] [CrossRef]
- Milne-Thomson, L.M. Theoretical Hydrodynamics; MacMillan: London, UK, 1962. [Google Scholar]
- Montes, J.S. Hydraulics of Open Channel Flow; ASCE Press: Reston VA, USA, 1998. [Google Scholar]
- Chow, V.T. Open Channel Hydraulics; McGraw-Hill: New York, NY, USA, 1959. [Google Scholar]
- Henderson, F.M. Open Channel Flow; MacMillan: New York, NY, USA, 1966. [Google Scholar]
- Castro-Orgaz, O.; Hager, W.H. Shallow Water Hydraulics; Springer: Berlin/Heidelberg, Germany, 2019; 563p. [Google Scholar] [CrossRef]
- Bos, M.G. Discharge Measurement Structures; Publication 20; International Institute for Land Reclamation and Improvement (ILRI): Wageningen, The Netherlands, 1976. [Google Scholar]
- Varoḡlu, E.; Finn, W.D. Variable domain finite element analysis of free surface gravity flow. Comput. Fluids 1978, 6, 103–114. [Google Scholar] [CrossRef]
- Bettess, P.; Bettess, J. Analysis of free surface flows using isoparametric finite elements. Int. J. Numer. Methods Eng. 1983, 19, 1675–1689. [Google Scholar] [CrossRef]
- Castro-Orgaz, O.; Hager, W.H. Comment to Flow at an ogee crest axis for a wide range of head ratios: Theoretical model. Water 2024, 16, 231. [Google Scholar] [CrossRef]
- Montes, J.S. Flow over round crested weirs. L’Energ. Elettr. 1970, 47, 155–164. [Google Scholar]
- Southwell, R.V.; Vaisey, G. Relaxation methods applied to engineering problems XII: Fluid motions characterized by free streamlines. Philos. Trans. R. Soc. Lond. A 1946, 240, 117–161. [Google Scholar]
- Hay, N.; Markland, E. The determination of the discharge over weirs by the electrolytic tank. Proc. Inst. Civ. Eng. 1958, 10, 59–86. [Google Scholar] [CrossRef]
- Ganguli, M.K.; Roy, S.K. On the standardisation of the relaxation treatment of systematic pressure computations for overflow spillway discharge. Irrig. Power 1952, 9, 187–204. [Google Scholar]
- Hager, W.H. Experiments on standard spillway flow. Proc. Inst. Civ. Eng. 1991, 91, 399–416. [Google Scholar]
- Selby, S.M. Standard Mathematical Tables; CRC: Cleveland, OH, USA, 1973. [Google Scholar]
- Savage, B.M.; Johnson, M.C. Flow over ogee spillway: Physical and Numerical Model Case Study. J. Hydraul. Eng. 2001, 127, 640–649. [Google Scholar] [CrossRef]
- Rouse, H.; Reid, L. Model research on spillway crests. Civ. Eng. 1935, 5, 10–14. [Google Scholar]
- Goffin, L.; Erpicum, S.; Dewals, B.J.; Pirotton, M.; Archambeau, P. How to simulate quickly and efficiently a flow over a spillway? In Proceedings of the 5th International Junior Researcher and Engineer Workshop on Hydraulic Structures, Spa, Belgium, 28–30 August 2014. [Google Scholar]
- Castro-Orgaz, O.; Cantero-Chinchilla, F.N.; Hager, W.H. High-order shallow water expansions in free surface flows: Application to steady overflow processes. Ocean. Eng. 2022, 250, 110717. [Google Scholar] [CrossRef]
- Castro-Orgaz, O.; Gamero-Ojeda, P.P.; Cantero-Chinchilla, F.N.; Morales de Luna, T.; Chanson, H. Application of high-level Green Naghdi theory to sill-controlled flows. Environ. Fluid Mech. 2024, 24, 19–56. [Google Scholar] [CrossRef]
- Vermeyen, T.B. Uncontrolled Ogee Crest Research; US Department of the Interior, Bureau of Reclamation: Washington, DC, USA, 1992; Available online: www.usbr.gov/tsc/techreferences/hydraulics_lab/pubs/PAP/PAP-0609.pdf (accessed on 11 December 2017).
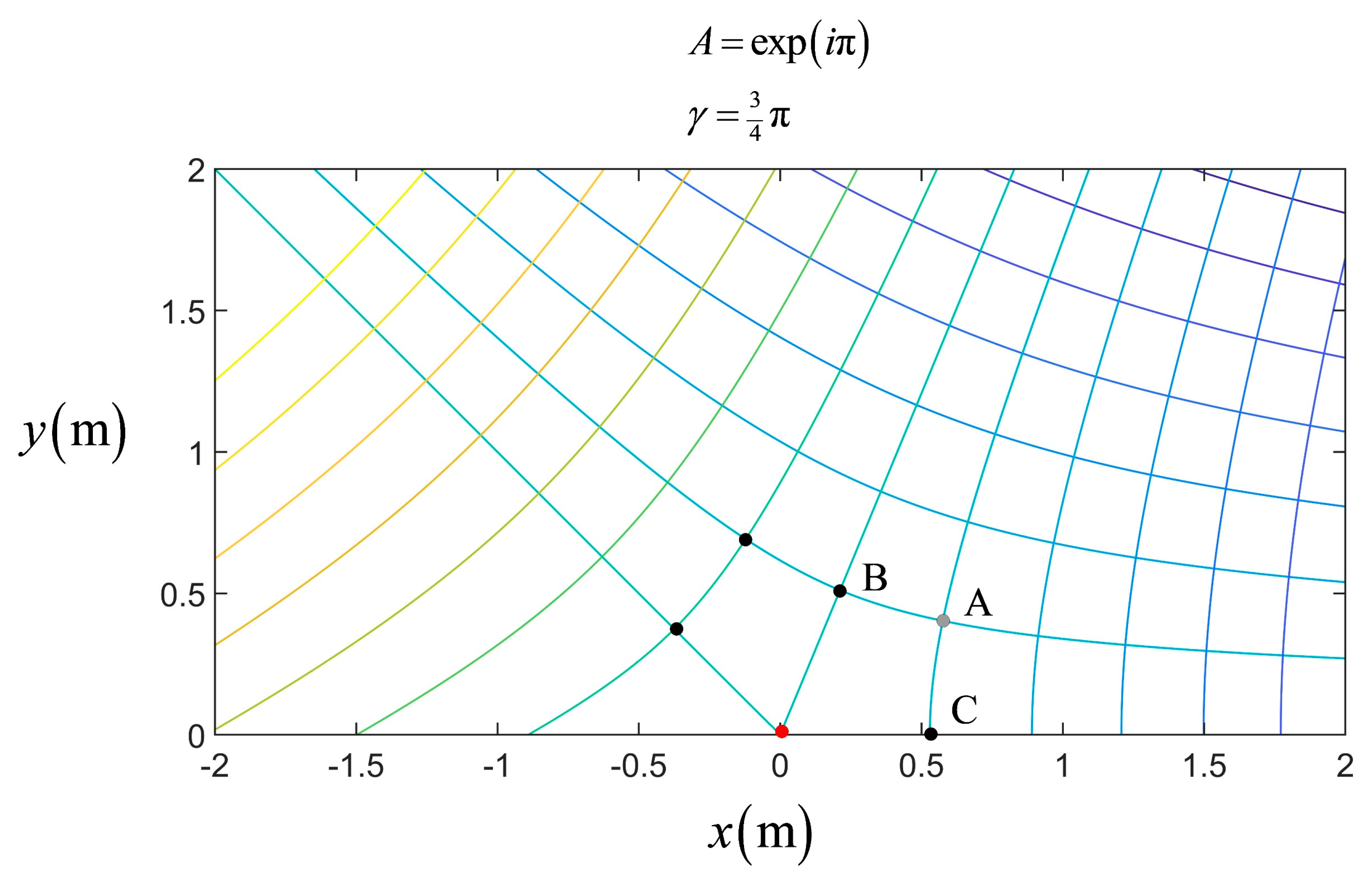


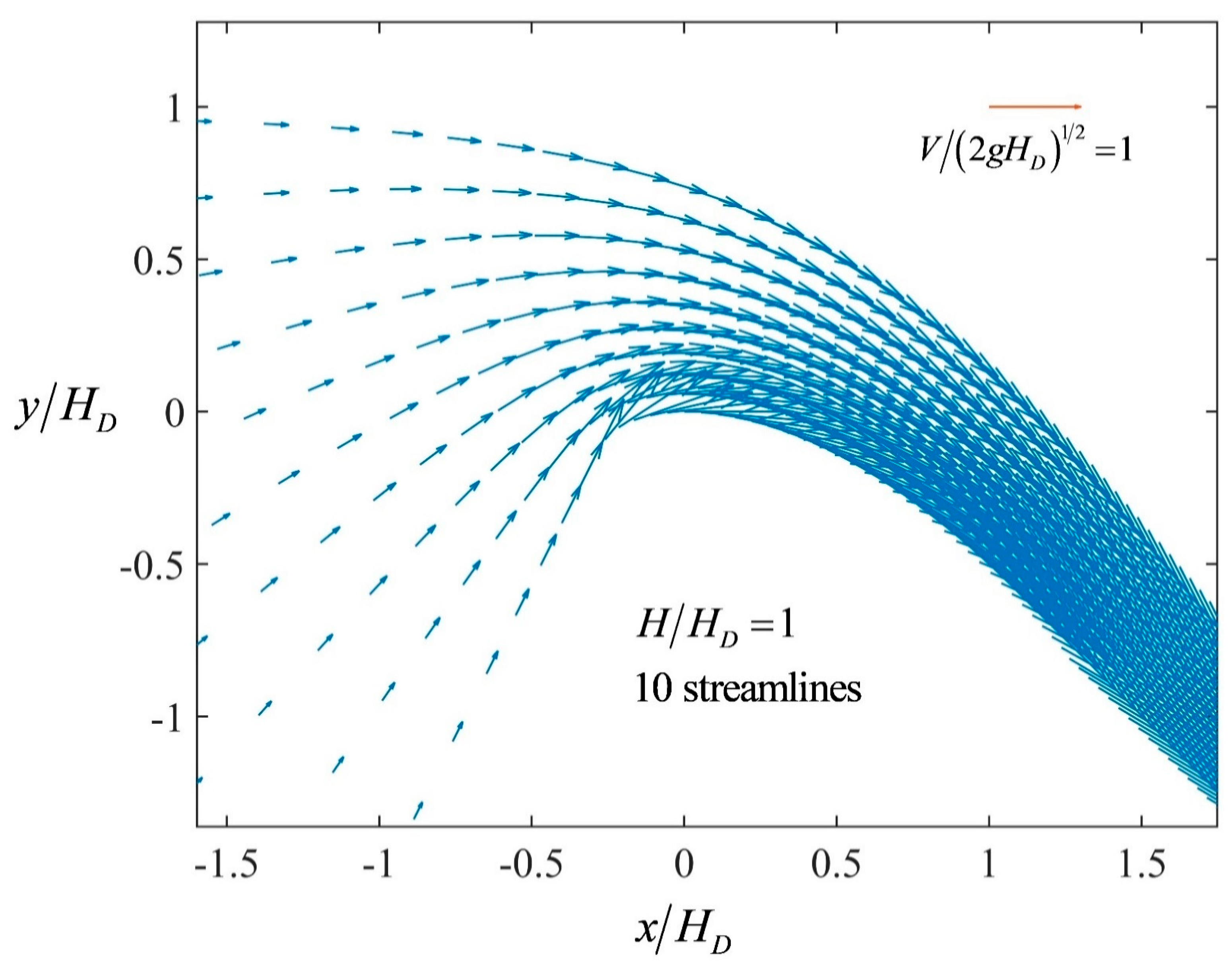

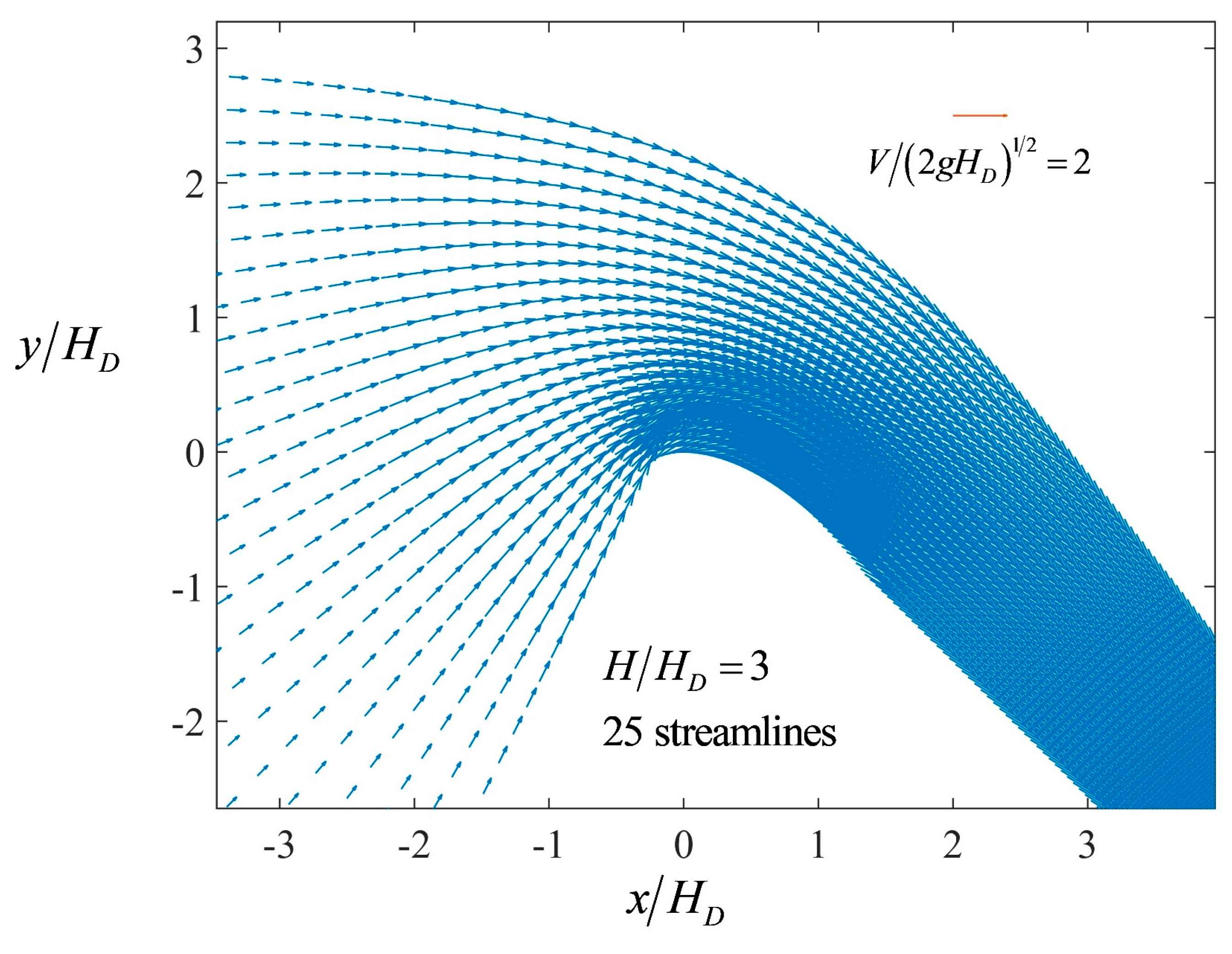
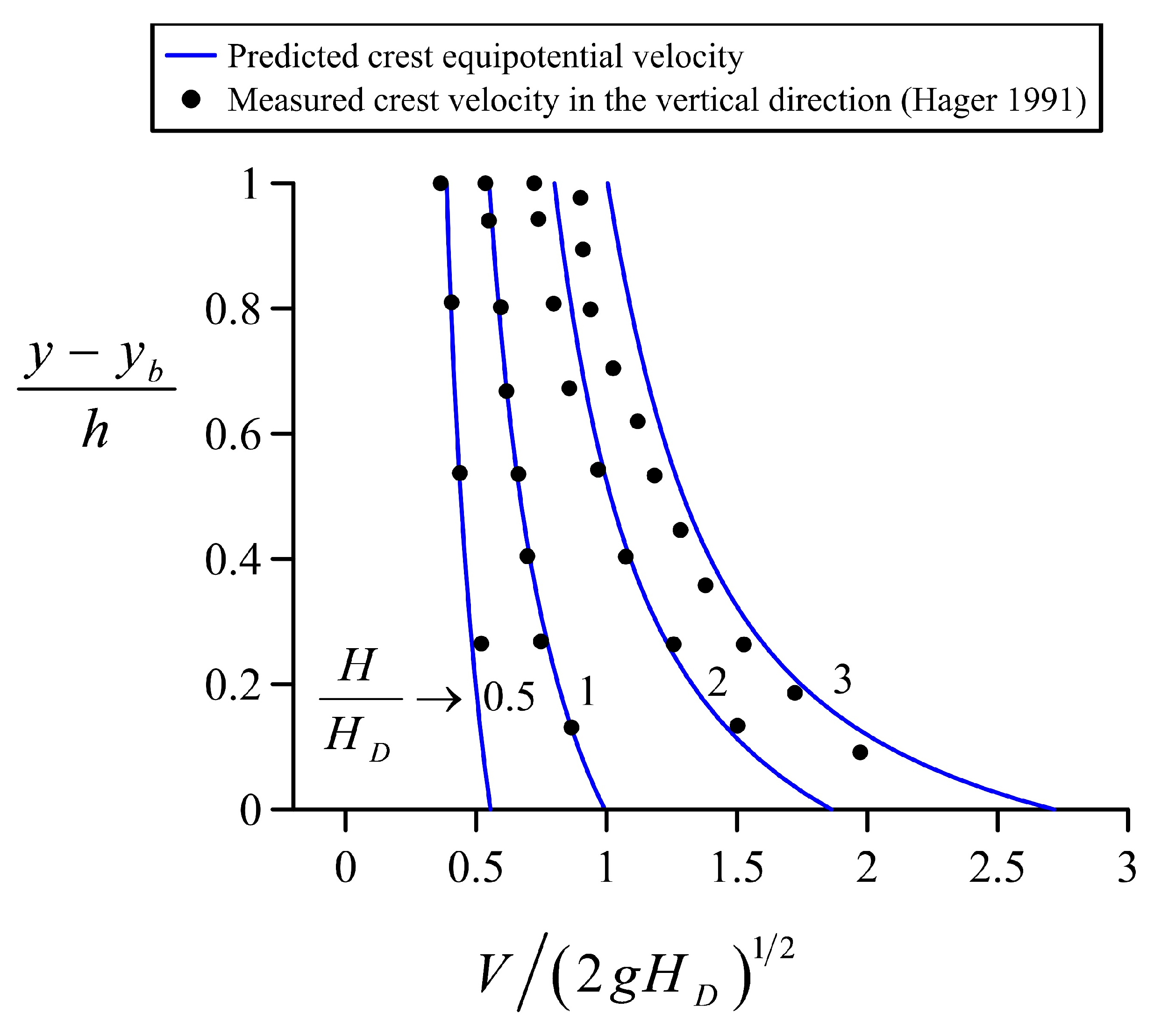


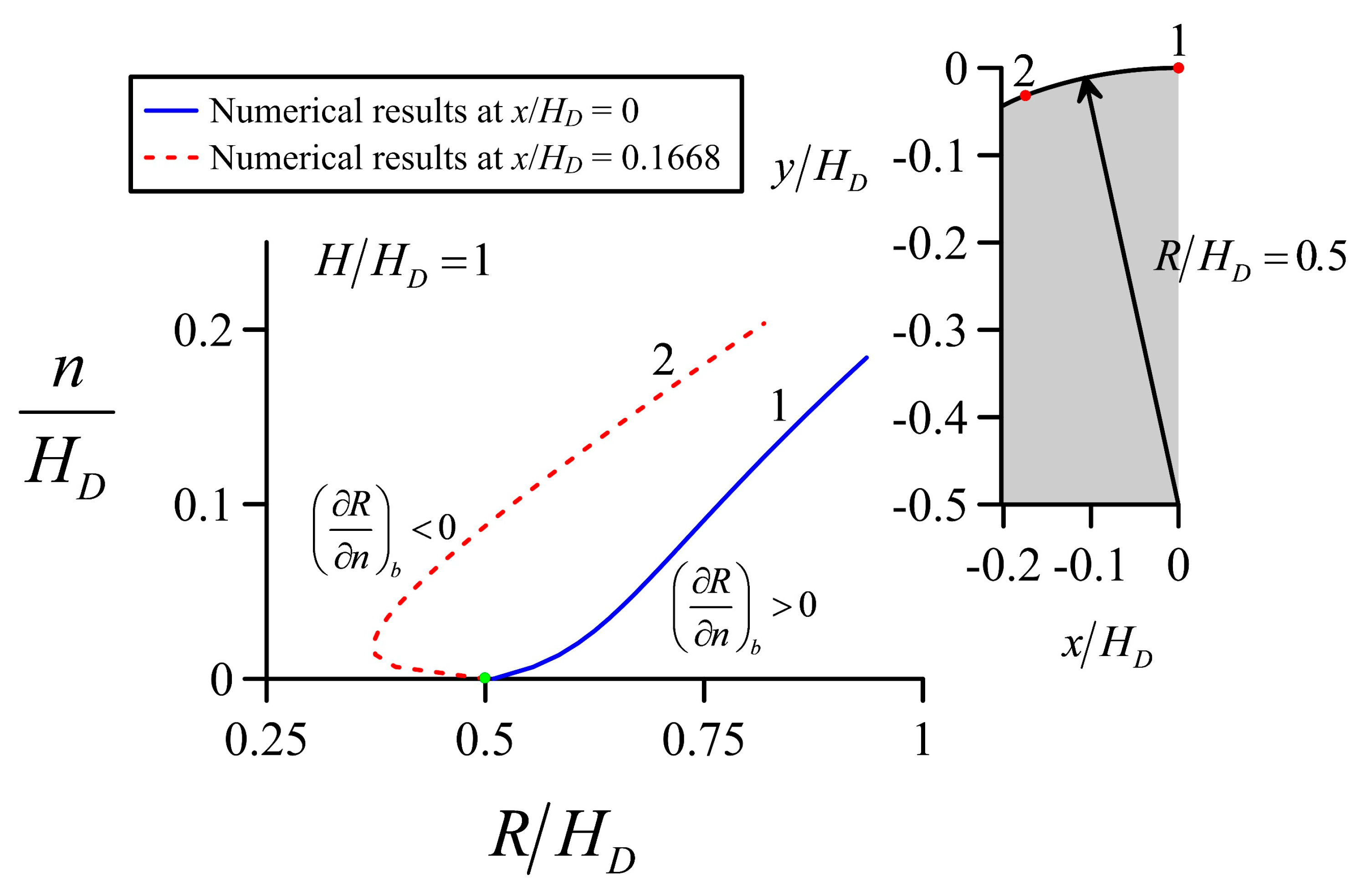


| Point | O1 | O2 | O3 | 1 | 2 | 3 | 4 |
|---|---|---|---|---|---|---|---|
| x/HD | 0 | −0.105 | −0.2418 | 0 | −0.1750 | −0.2760 | −0.2818 |
| y/HD | −0.5 | −0.219 | −0.1360 | 0 | −0.0316 | −0.1153 | −0.1360 |
| Number of Streamlines | Number of Equipotentials | Mass-Conservation Ratio | Cd | Computation Time |
|---|---|---|---|---|
| % | s | |||
| 15 | 126 | 0.2273 | 0.7087 | 1.2550 |
| 20 | 160 | 0.5904 | 0.7081 | 1.8231 |
| 25 | 210 | 0.3327 | 0.7090 | 3.1694 |
| 30 | 252 | 0.4973 | 0.7093 | 5.2612 |
| 35 | 294 | 0.3281 | 0.7088 | 10.0412 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Castro-Orgaz, O.; Hager, W.H.; Guo, Y.; Erpicum, S.; Cantero-Chinchilla, F.N. Irrotational Flow over Ogee Spillway Crest: New Solution Method and Flow Geometry Analysis. Water 2024, 16, 2720. https://doi.org/10.3390/w16192720
Castro-Orgaz O, Hager WH, Guo Y, Erpicum S, Cantero-Chinchilla FN. Irrotational Flow over Ogee Spillway Crest: New Solution Method and Flow Geometry Analysis. Water. 2024; 16(19):2720. https://doi.org/10.3390/w16192720
Chicago/Turabian StyleCastro-Orgaz, Oscar, Willi H. Hager, Yakun Guo, Sebastien Erpicum, and Francisco Nicolás Cantero-Chinchilla. 2024. "Irrotational Flow over Ogee Spillway Crest: New Solution Method and Flow Geometry Analysis" Water 16, no. 19: 2720. https://doi.org/10.3390/w16192720
APA StyleCastro-Orgaz, O., Hager, W. H., Guo, Y., Erpicum, S., & Cantero-Chinchilla, F. N. (2024). Irrotational Flow over Ogee Spillway Crest: New Solution Method and Flow Geometry Analysis. Water, 16(19), 2720. https://doi.org/10.3390/w16192720









