1. Introduction
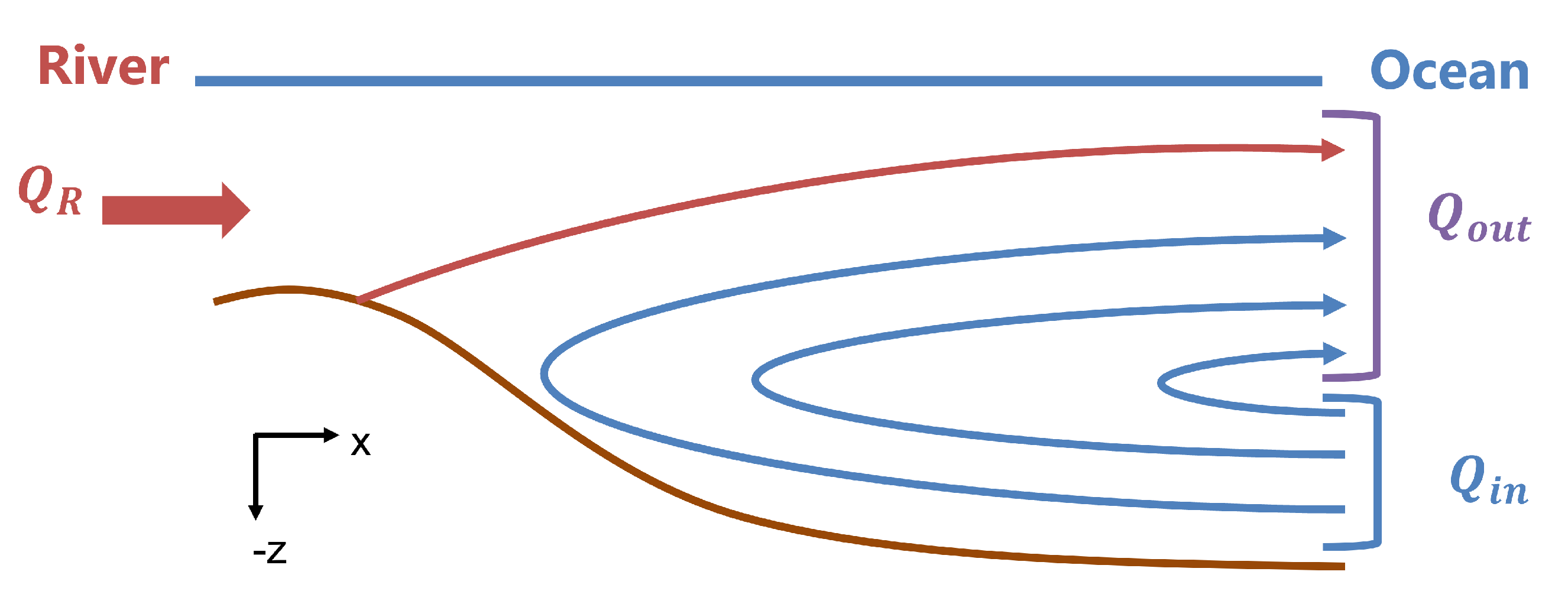
Estuarine exchange flow, driven directly by longitudinal density gradients [
1] and indirectly by tidally asymmetric flows [
2], provides the foundational circulation and transport for estuaries and fjords (
Figure 1). These flows carry freshwater seaward, typically in an upper surface layer, with dense saline waters replacing those outflows at depth. This exchange produces flushing and dispersion of river-sourced scalars. Sinking particles, whether they are biotic or abiotic, can result in trapping and retention within the estuary [
2], forming the estuarine turbidity maximum (ETM), a localized maximum of suspended particulate matter concentration in an estuary. While naturally occurring, ETMs are frequently associated with deteriorated ecological and physical conditions, including ecological dead zones due to either decreased light or increased organic matter decomposition, naval navigation challenges, and other water quality problems [
3]. This study aims to investigate this sinking particle trapping phenomenon and how it relates to ETMs by looking at both a sinking tracer in isolation and sinking detritus within a planktonic ecosystem.
ETMs have been the subject of modeling studies for several decades. In 1978, ref. [
4] used an idealized numerical model to investigate ETM formation and found it to be a function of settling velocity and the strength of the estuarine circulation. In 1980, researchers began to realize that ETMs could also be tidally caused [
5]. Later studies found that there may be seasonal effects driving ETMs, especially for sediments deposited during large flooding events from winter storms [
6]. While some estuaries develop ETMs from bathymetry or lateral trapping processes, most basic ETM dynamics can be described using a constant settling velocity [
4,
5,
7]. A summary is provided in the review in [
3], where one of their concluding thoughts is that more fundamental research in suspended particulate matter (SPM) dynamics is needed to be able to classify estuaries by their ETMs. Further, one of the main remaining questions those authors found in their review is, “How do the fast dynamics of SPM in the water column and the slow dynamics of the bottom pool interact to determine ETM locations and variability, and what processes govern the dynamics of the mobile bottom pool?” [
3]. The analyses presented in this paper are motivated in part by this call for additional research on ETMs and how sinking particles contribute to these accumulations.
Sinking detritus can lead to hypoxic (low-oxygen) regions of the estuary, in addition to ETMs. For example, the Hood Canal section of the Puget Sound has seasonal periods of low dissolved oxygen [
8], leading to fish kills and other ecosystem impacts [
9]. One of the causes of hypoxic regions is the sinking of dead organic material that originally grew in the high-irradiance surface layer and is then decomposed at depth by aerobic microorganisms that use up the oxygen. Field studies have detected patchiness in dissolved oxygen levels in the depths of the Hood Canal [
10]. An investigation of the interaction of detritus sinking with estuarine exchange flow will help to develop an understanding of the physical and biological processes that lead to oxygen depletion in the Hood Canal and other similar fjordic and estuarine systems. While we do not study the formation of hypoxia directly, it serves as a motivation for this work, which we hope will inform the further study of sinking decaying matter that leads to hypoxic regions via biological oxygen demand.
In order to capture dynamics similar to those in Puget Sound, we simulate a partially mixed estuarine system, with a density-driven circulation dominating the along-estuary net transport, which is reflective of the fjord-like systems of the Puget Sound region [
2,
9]. The exchange flow increases the longitudinal dispersion of passive tracers but traps sinking particles that enter the lower layer [
2]. This study aims to investigate this sinking particle trapping phenomena and how it relates to ETMs/sinking particle/detritus accumulation, as well as to look to the future to understand how physical changes to the estuary will alter these ETMs.
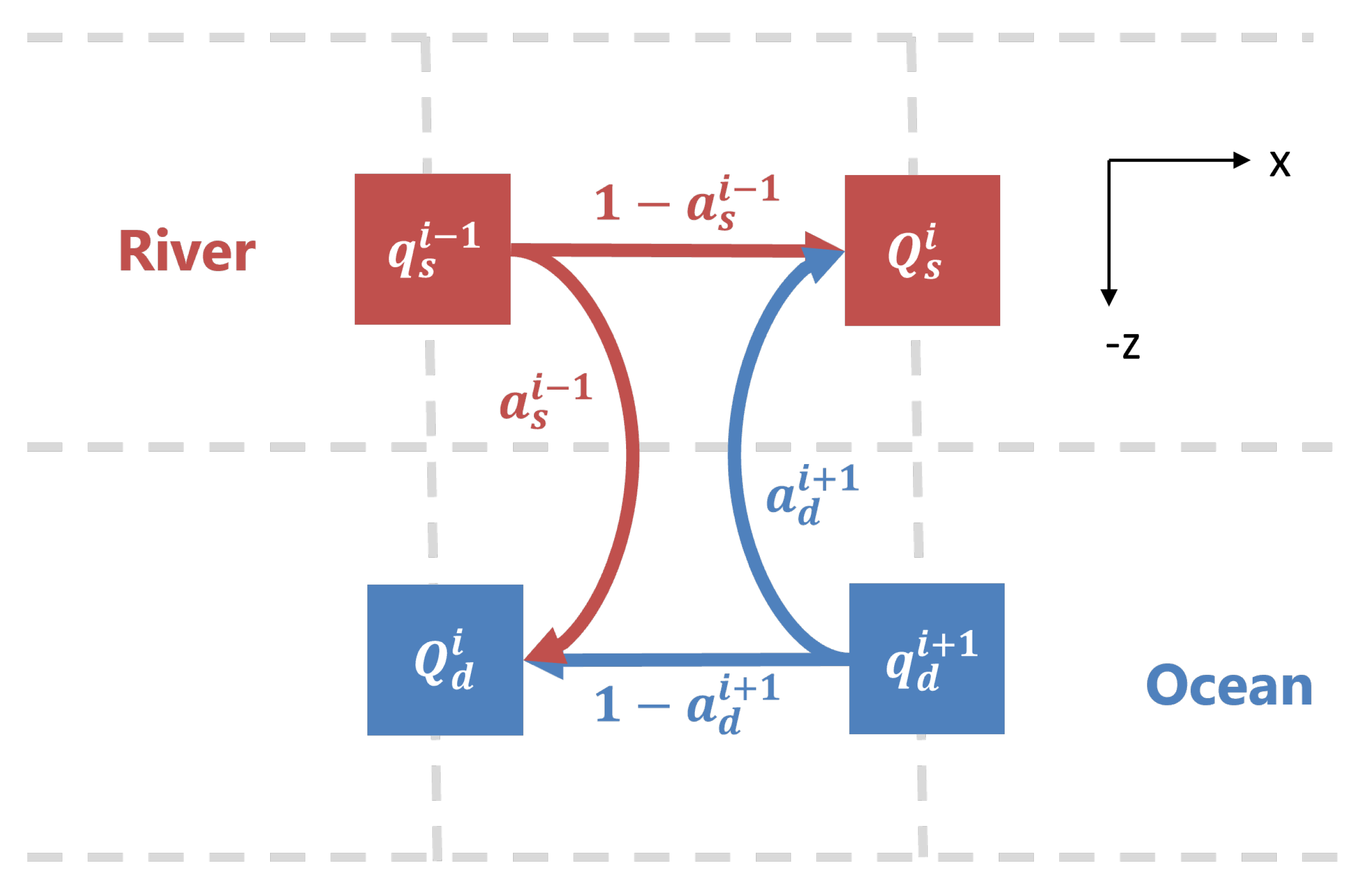
The tool of this study is a numerical model of a two-layer estuary that divides the estuary into longitudinal compartments. This model was originally used by [
11] to investigate residence times in the Salish Sea estuary by advecting a passive, non-sinking tracer. We expanded the box model in [
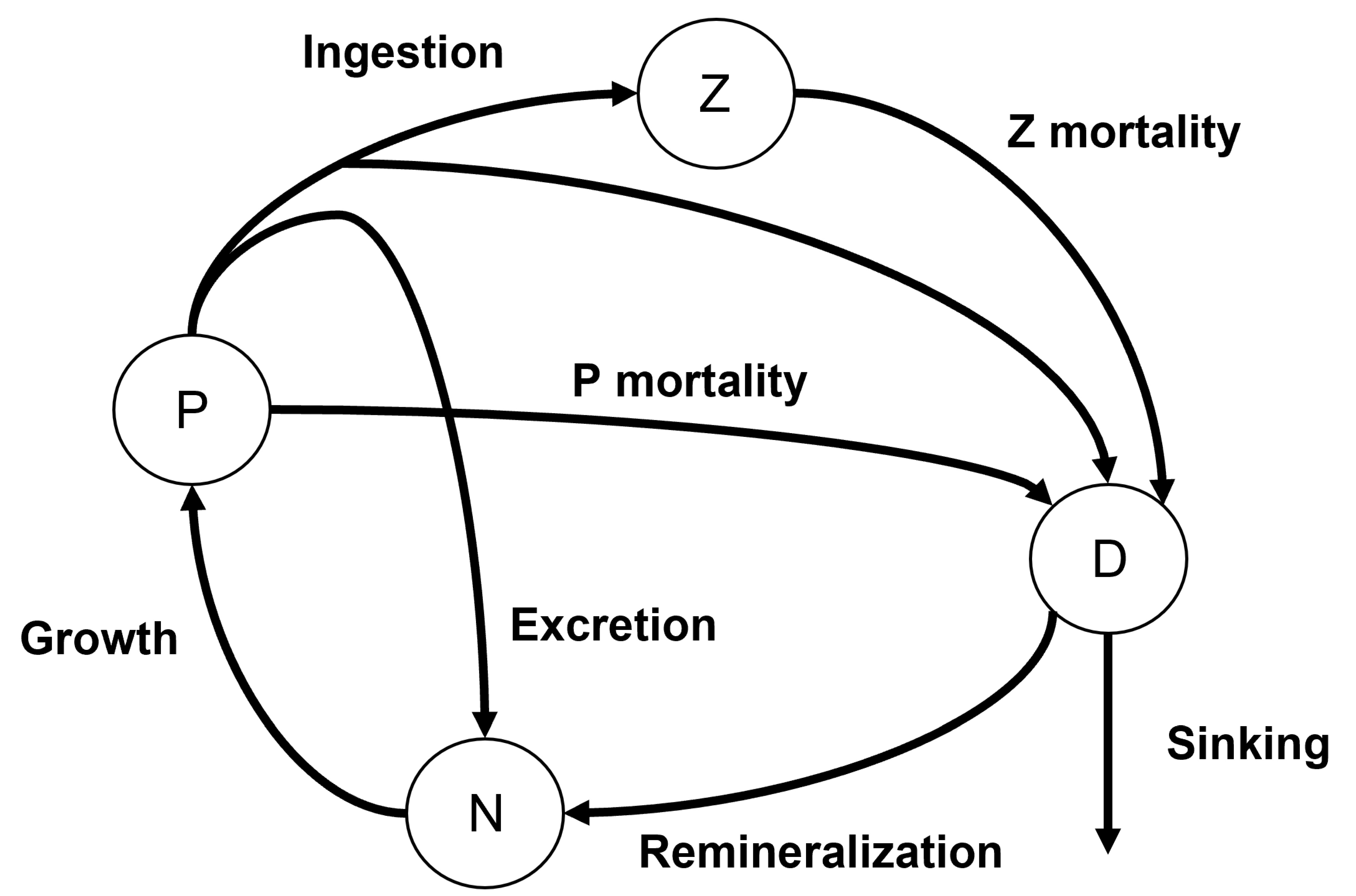
11] with a sinking tracer to understand the relationship between particle sinking and exchange flow. First, we explore the implications of varying parameters in this model for estuarine turbidity maximum/sinking particle accumulation in a system inspired by, but not strictly tied to, the Puget Sound. We next assess the planktonic ecosystem impacts of sinking detritus with the Peter–Parker Model, which adds a biological component that tracks nutrients, phytoplankton, zooplankton, and detritus (NPZD) [
12] concentrations through time with the Total Exchange Flow (TEF) box model [
11]. Then, we break apart the mechanisms leading to these accumulations by using a timescale analysis and compare the accumulations of systems with and without biology. Lastly, we characterize the flow of the model with a dimensionless parameter that summarizes the dynamics of the sinking tracer and can be used across systems.
3. Results: Effects of Sinking Speed on Tracer or Detritus Accumulation
We first present concentration results from a sinking tracer in the TEF box model (Equation (
1)) without any biological considerations (Variation 1 above). We then present the effects of detritus sinking as it interacts with the ecosystem with results from the Peter–Parker Model (Variation 2 above).
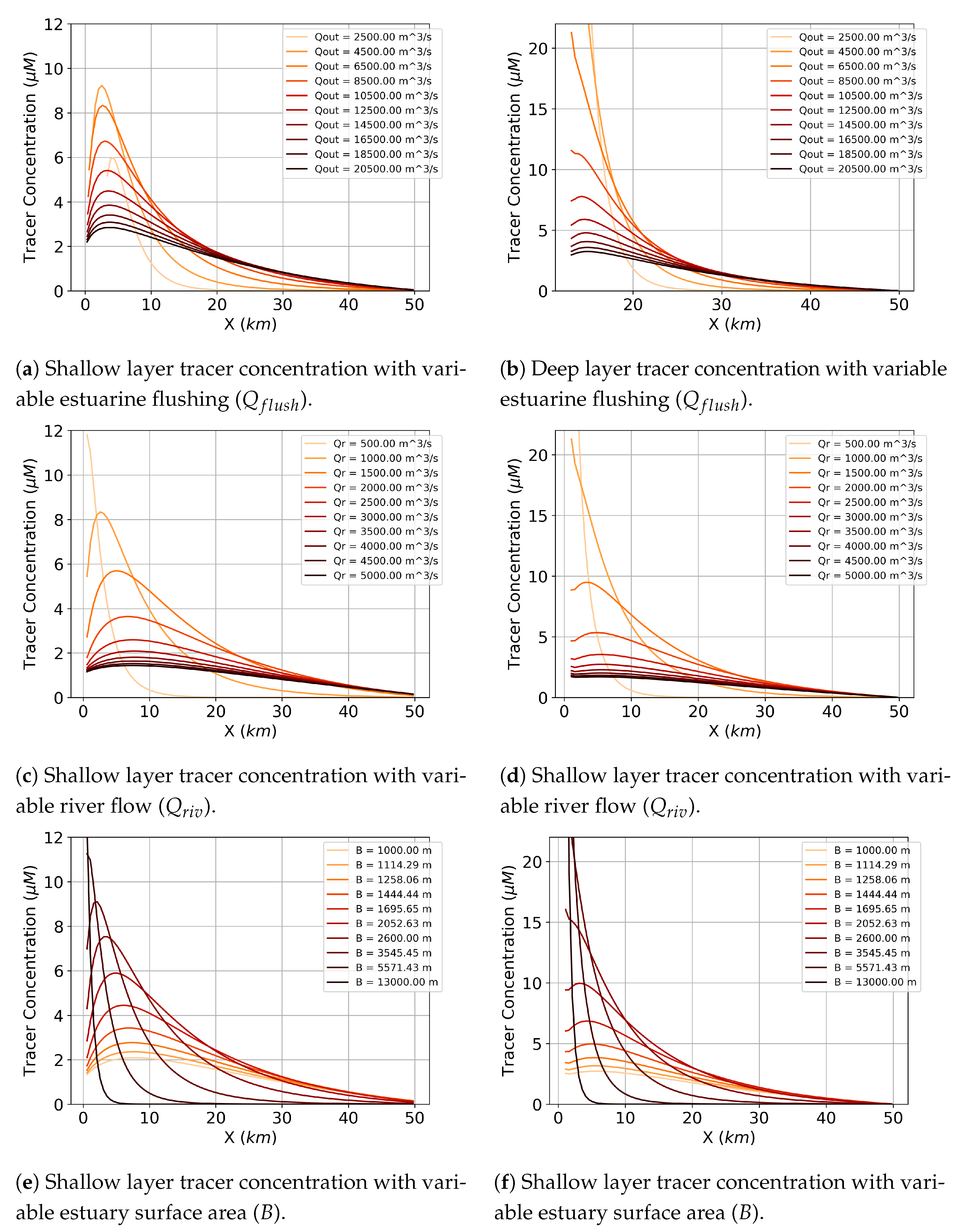
The tracer concentration profiles results for varying sinking while holding all other parameters constant (
Figure 6) indicate that there is accumulation of the sinking tracer near the river (
) in both the shallow and deep layer for certain sinking speeds. We define accumulation as when the concentration surpasses the river input value of 1
M. Without sinking,
; the tracer advects out or mixes down from its box and does not get above the river input concentration. Interestingly, the peak concentration increases with increased sinking rate to reach an inflection point at around
in both the shallow and the deep layer, after which the peak grows unbounded at
(does not reach steady state within the 200 days of simulation time). In the deep layer, there is accumulation regardless of sinking speed, but the amount increases and becomes unbounded above the same sinking speed as the shallow layer. The deep layer always has a higher peak concentration than the shallow layer. Also, the locations of these peak concentrations shift toward the river with increasing sinking speed in both layers.
Why do sinking particles (
Figure 6) lead to accumulation in the estuary? Following one particle starting in the shallow layer of the box model (
Figure 2), as it sinks out of the shallow layer into the deep, it then is pushed back towards the river (
). This happening to a group of particles leads to high concentration in the deep layer up-estuary (
). Some of that accumulated concentration is moved back into the shallow layer via efflux. The balance between the sinking and efflux creates a type of vertical circular eddy motion, which maintains the tracer concentration at a higher value than the river input of 1
M in both the shallow and deep layer at
. The peak also occurs near
because that is where there is increased vertical mixing activity via efflux (
Figure 4). When sinking passes a certain threshold of around 15
, the effects of the efflux are diminished in the shallow layer as the efflux is no longer sufficient to balance the sinking flux anywhere in the estuary and the system grows unbounded (does not reach steady state within the simulation time of 200 days).
For large settling velocities, the peak concentration is constrained spatially by the up-estuary boundary condition at
and the mass conservation of the system: as tracer is not allowed to leave the system, it is pushed against
with higher sinking speeds, resulting in narrower peaks. For sinking speeds less than 15
, steady state is reached, and the peak appears downstream of the boundary. Note that 15
is quite low; marine snow can be 36
[
20]. If we compare the sinking speed to a ratio of the sinking flux to the vertical mixing flux,
, where
is the sinking flux (defined in Equation (
6)) and
is the vertical flux (arrow pointing from deep to shallow in
Figure 2), sinking speeds greater than 15
correspond to when the ratio of settling flux to vertical flux is greater than 0.5. Thus, this balance of sinking and vertical flux is determining when the system accumulates unboundedly: the sinking flux must be at most 50% of the vertical flux to reach steady state.
Peak concentrations move closer to the river () because that is where the sinking tracer concentration is being sourced. So, the faster a particle sinks, the closer it will sink to its source because it is sinking faster than the estuarine flow, which otherwise would move it further from its source. This is further investigated with timescales in the following section.
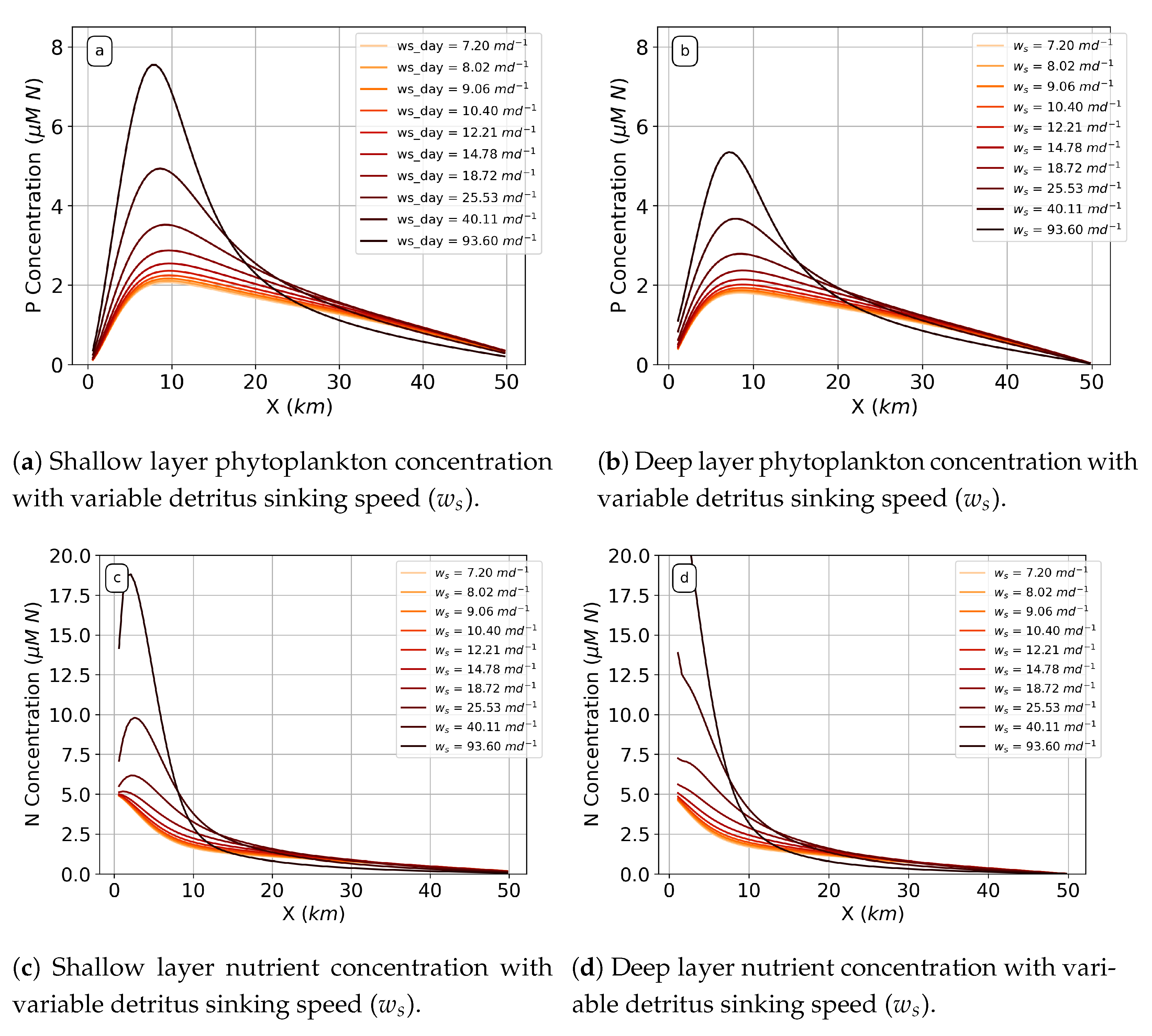
When incorporating a biological model to evaluate sinking detritus, we also see this phenomenon with the peak detritus concentration in the estuary (
Figure 7). Increasing detritus sinking speed leads to an increased detritus peak concentration that moves closer to the river end of the estuary (
). Also, the detritus grows unbounded (does not reach steady state) with a sinking speed above
.
Increasing the sinking rate (
) of detritus from the shallow to the deep layer leads to an increase in phytoplankton (
P) concentration in both the shallow and deep layer (
Figure 8a,b) for all sinking speeds.
P concentration reaches a maximum in both the shallow and deep layer at around
and the location of this peak does not significantly change with increasing sinking speed, contrary to the peak detritus concentration. Nutrient concentration follows a similar pattern: increasing detritus sinking speed leads to increased nutrient concentrations with peaks that do not move with increased sinking speed. Peak nutrient concentration occurs between 0 and 5 km.
Increased detritus influences phytoplankton and nutrient peak concentration because more detritus accumulating in one spot of the estuary leads to more nutrients available to the phytoplankton via remineralization. As long as the location of the nutrients does not change, which is the case with increasing detritus sinking speed and constant remineralization rate (
Figure 8c,d), the location of the phytoplankton will not change. Increasing nutrient concentration does not change the rate of nutrient uptake by the phytoplankton and, thus, does not change the location of the peak. But their peaks themselves increase with the increase in detritus/nutrient supply.
As demonstrated above, the passive tracer accumulates indefinitely for sinking speeds greater than 15
due to the balance of the sinking and vertical mixing flux. With biology, however, there are additional fluxes keeping the concentration from growing indefinitely (growth, grazing, remineralization, etc.). Thus, detritus and nutrients grow unboundedly for sinking speeds greater than 40
in the deep layer, but phytoplankton does not grow unboundedly regardless of sinking speed. This is because there is a carrying capacity represented in the Michaelis–Menten phytoplankton growth expression (Equation (
8)), which restricts unlimited phytoplankton growth even with unlimited nutrients
, where
is half-saturation
N uptake by
P. We explore the interplay of these physical–biological interactions in the following timescales section.
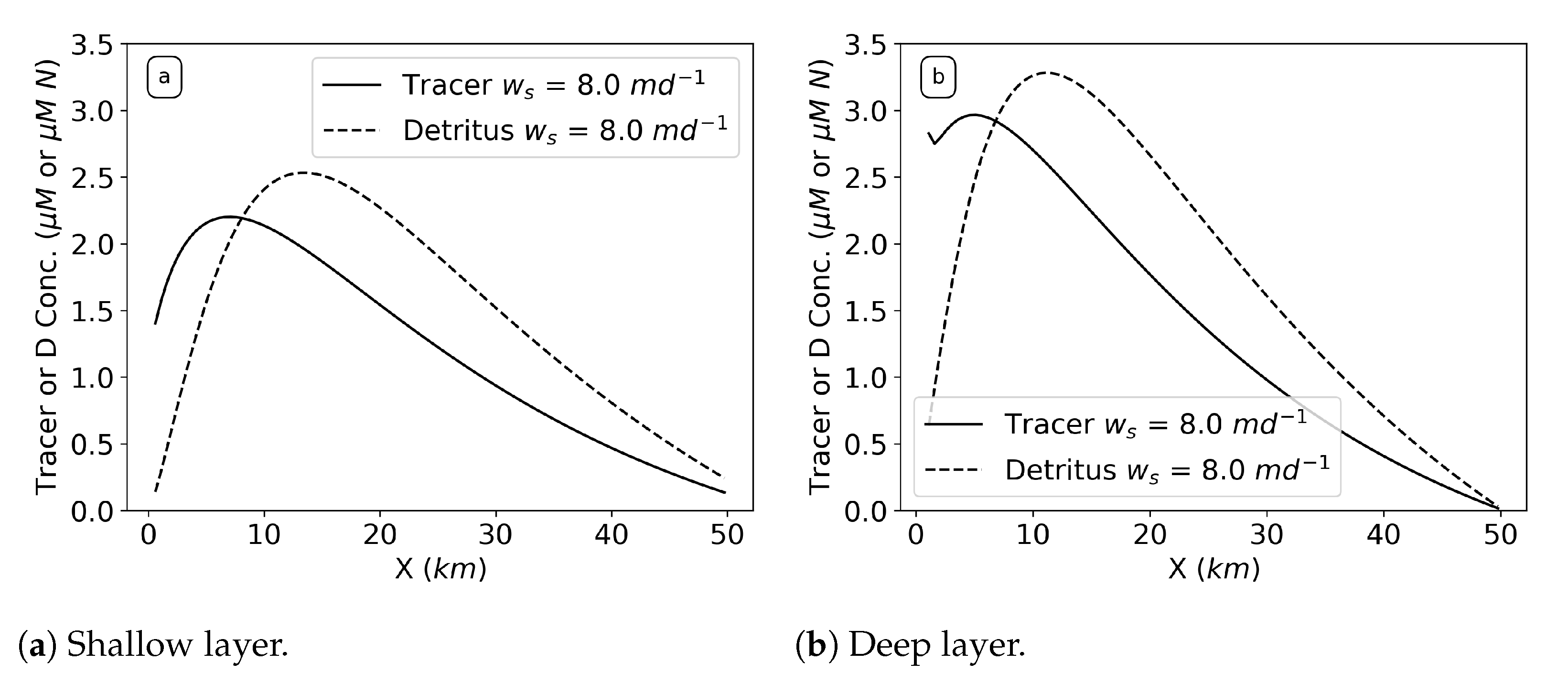
Interestingly, the peak detritus location moves closer to the river with higher detritus sinking speeds even though we only source nutrients in the river, not detritus. Phytoplankton will follow nutrients due to its nutrient-dependent growth rate, and zooplankton will then follow the phytoplankton. Detritus concentration is high where concentrations of phytoplankton and zooplankton are high. Where the detritus is formed via biological processes is then the source of the sinking tracer, as in
Figure 6, and, as occurs with the sinking tracer, the interaction of the detritus sinking and exchange flow leads to accumulation.
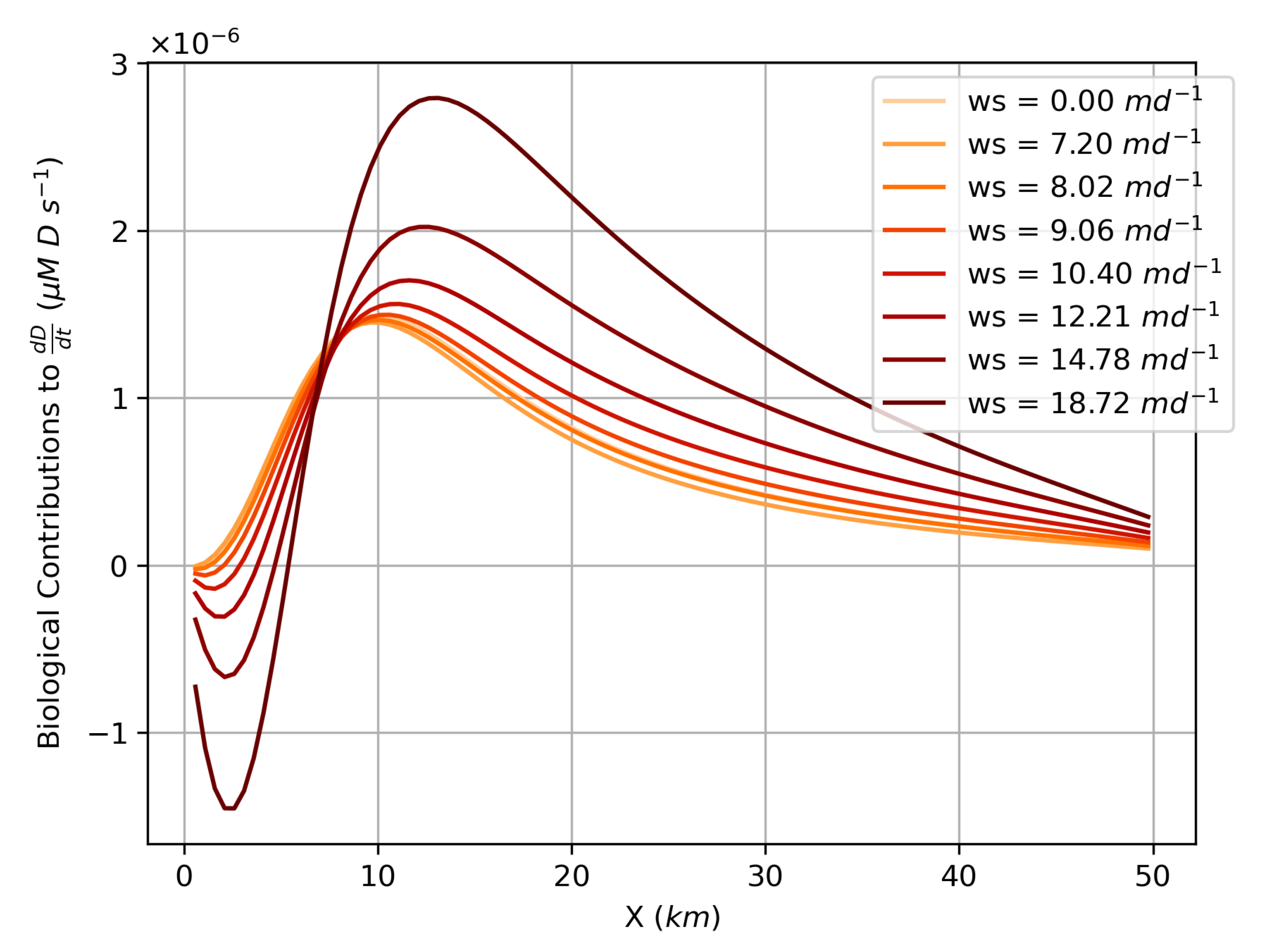
Figure 9 shows the biological contribution to
, which includes sources from zooplankton “messy eating”, phytoplankton and zooplankton mortality, and sinks from remineralization (Equations (
7) and (
11)). This demonstrates that the source for the detritus is shifted 8–15 km downstream of the river mouth and distributed over a broader reach of the estuary, as compared to the river-sourced sinking tracer considered in the previous section. Regardless, the peak detritus is positioned based on a balance between efflux and sinking, which occurs near
, especially for larger sinking speeds. This results in a location for the peak detritus concentration that moves upstream as sinking increases. This is further explored with the timescale analysis in the following section.
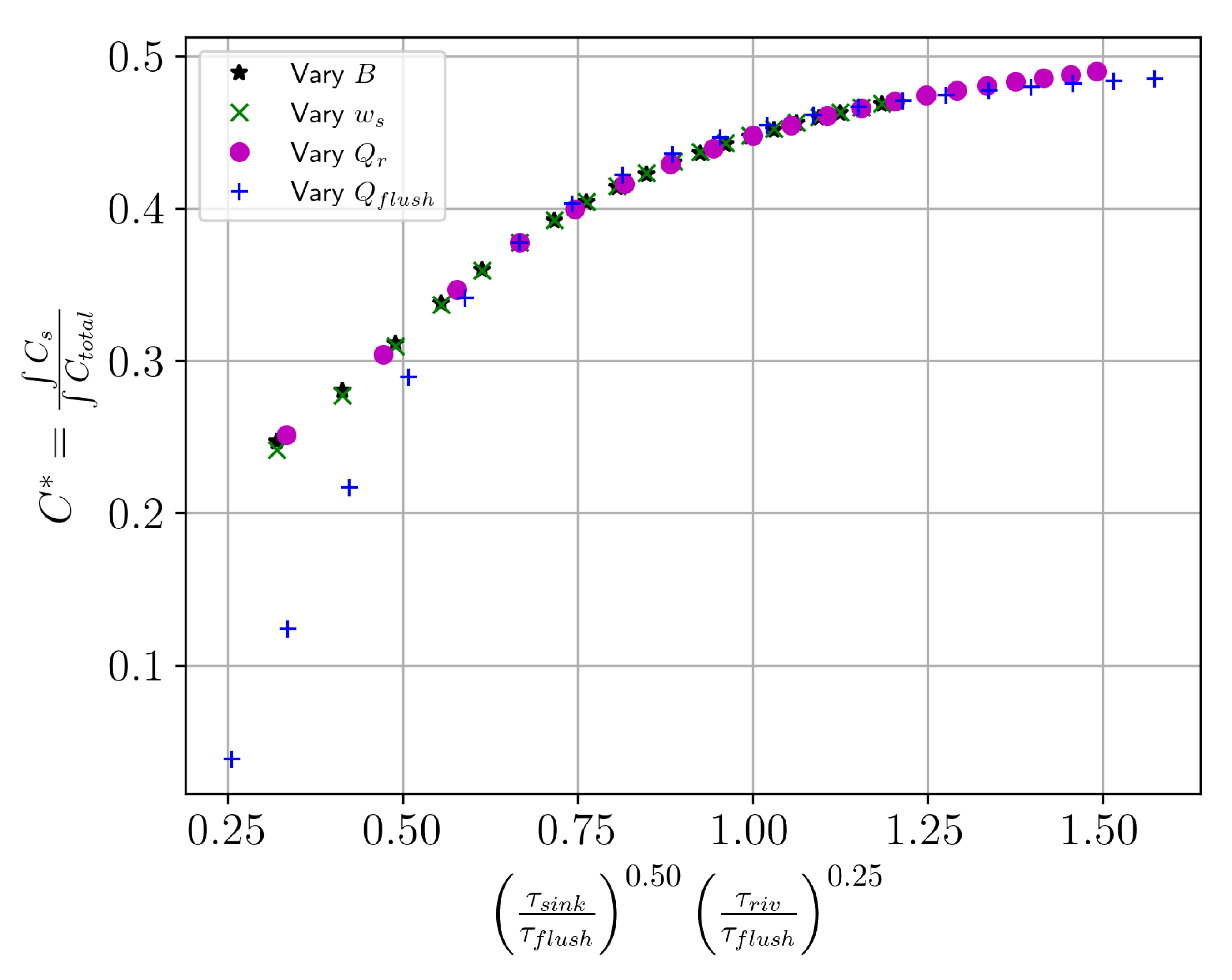
5. Summarizing the Behavior with a Dimensionless Group
Through some further evaluations of varying sinking speed (
), estuarine flushing rate (
), estuary width (
B), and river flow rate (
), we found that increasing sinking speed and width and decreasing flushing rate and river flow lead to increased ETM magnitude, but only up to certain values of each parameter before the system does not reach a steady state (see
Appendix A for additional details). Through dimensional analysis, we obtained the following dimensionless group:
using general timescales
,
, and
, where
is total estuary volume and
h is depth. We validated this group by using the estuary length (
L) as a constant parameter and dimensionless concentration
, which represents the integrated shallow layer concentration normalized to the total integrated concentration (shallow+deep) (
Figure 14).
focuses on the strength of the concentration peak in the estuary while taking away the x-dependence of the ETMs. The dimensionless concentration
increases as the shallow and total integrated concentrations approach each other. Thus,
alone does not tell us the location of the peak concentration, but allows us to compare the state of concentration in the estuary. We performed this dimensionless analysis on both the sinking tracer and sinking detritus studies and found the outcomes to be similar, so we present the results from the tracer study only here to reduce redundancies.
Figure 14 shows that varying
,
B,
, or
will result in the same state in the estuary,
. This dimensionless group connects three timescales, which represent three fluxes in the system: the estuarine flux, the sinking flux, and the river flux. The ratio of sinking to exchange flow and the ratio of river flow to exchange flow are the two drivers of the system, with the former having a stronger exponential dependence than the latter. As such, increasing flushing has a similar effect as reducing sinking and increasing flushing has a similar effect to reducing river flow. These relationships are not one to one, so increasing flushing has a stronger effect than decreasing river flow or sinking. Flushing has this extra complexity because of how the flow is defined (Equation (
3)): the in- and outflow (
and
) depend on a fraction of the river flow that varies with the salinity difference taken at different locations in the estuary. Thus, unlike estuary width, sinking speed, and river flow, this parameter varies in space and cannot be combined with the others as cleanly.
This parameter informs the relative importance of particle sinking, estuary surface area, estuary flow, and river flow for accumulation of scalars in an estuary. As discussed above, particle sinking influences accumulation because of the interplay of sinking and vertical mixing. Varying estuarine flushing () rate also leads to accumulation because, when the overall advection scheme is slower, the effects of the sinking tracers are more pronounced. In other words, if the estuary is flushing slower, the sinking tracer has more time to accumulate in the bottom layer. Thus, it is able to efflux back upwards with more ease and present more accumulation in both the shallow and deep layers. Accumulation occurs with varying estuarine surface area (B) due to similar logic as with reduced flushing rate; when the river flow is held constant but the estuary is larger, the effects of the river on the longitudinal exchange in the estuary are reduced. Thus, the sinking tracer has more time to accumulate in both layers. Lastly, accumulation occurs with decreased river flow () for the same reason as altering estuary size and flushing; when the river is faster, the tracer has less time to settle and accumulate.
6. Conclusions
We would like to highlight several key findings from this work that establish the differences between ETM dynamics when considering a sinking tracer versus sinking detritus in an estuary. In both cases, the concentration of sinking tracer or detritus accumulates in an estuary because the sinking of the tracer is in balance with the vertical mixing (efflux) of the system at some location along the estuary. The peak concentration of a river-sourced sinking tracer moves towards the river with increased sinking speed because it comes into balance with the efflux further upstream and creates a narrower vertical recirculation. The peak concentration of sinking detritus in a system where nutrients are sourced in the river moves towards the river with increased sinking speed as well, but the peak is displaced down-estuary. This shift in the position of the ETM is a result of the biological processes that govern the creation of detritus, which is tied to the peaks of phytoplankton and zooplankton. This shift in the peak is created by a temporal lag for nutrients to turn into detritus in the biological model. As nutrients are taken up by phytoplankton, which are, in turn, consumed by zooplankton, down-estuary transport in the surface layer means that the source of detritus (whether from messy eating or mortality) is shifted downstream from the river by a distance related to the surface-layer advection and the characteristic cycling timescale of the ecosystem.
Accumulation increases until a distinct sinking speed for both sinking tracer and detritus, after which the peak does not reach a steady state. The sinking speed after which this happens is smaller for sinking tracer than detritus. The peak accumulates unboundedly after the ratio of the sinking flux to the vertical flux surpasses 0.5 due to the retention of mass at the upstream boundary and the rapid recycling of a sinking tracer between the lower and upper layers (a balance between efflux and settling). It is interesting to note that the other components of the ecological model (e.g., phytoplankton) maintain a steady state at the same sinking speed due to the other ecological controls on each component (e.g., grazing by zooplankton).
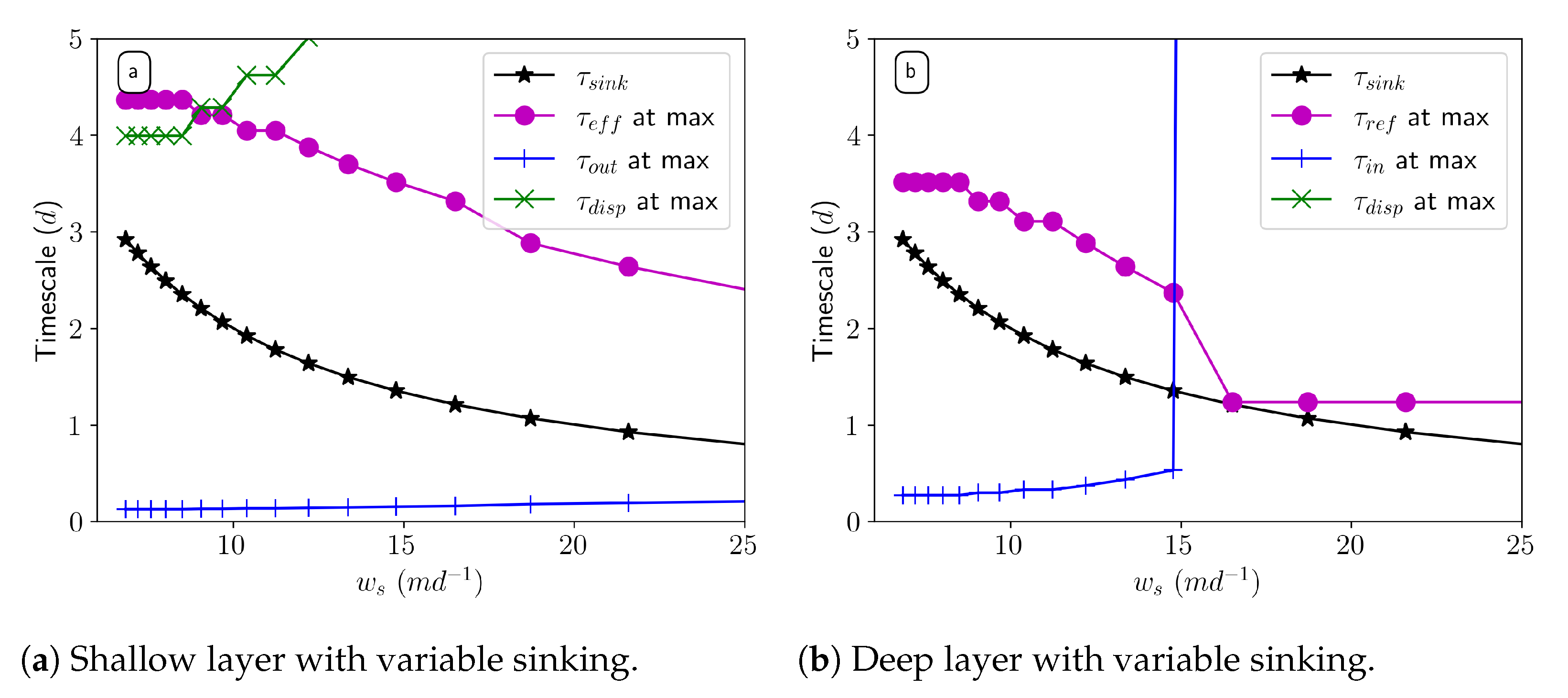
We break apart the contributions of the processes in our model by analyzing timescales. The fastest timescale indicates the process that is most influential on the concentration profiles. Longitudinal mixing is the shortest timescale for the sinking tracer in the shallow layer. In the deep layer, the shortest timescale switches from longitudinal exchange to vertical mixing and sinking at the same point at which the system switches from steady state to unbounded. With detritus, zooplankton mortality and longitudinal mixing are the fastest timescales in the shallow layer. In the deep layer, zooplankton mortality is also the fastest timescale up to a certain sinking speed and the physical timescales follow similar dynamics as the sinking tracer; this indicates that adding biology to the system influences the processes that determine the concentration in the shallow layer.
The peak concentration occurs at a different location in the estuary at the same sinking speed because of the time lag of biological processes. This time lag is about 1 day for steady-state cases, but it devolves for cases which grow unboundedly because they all peak up-estuary at , where vertical mixing activity is the strongest.
Lastly, sinking tracer concentration depends on four different variables, which were summarized in a single nondimensional parameter (
Section 5). This parameter allows us to directly compare the impacts of the individual attributes of the estuary on ETMs. For instance, given an estuary of a certain size and river flow rate, we can determine how fast a particle needs to sink to accumulate.