Adsorption of a Multicomponent Pharmaceutical Wastewater on Charcoal-Based Activated Carbon: Equilibrium and Kinetics
Abstract
1. Introduction
- Treatment of high-strength multicomponent pharmaceutical wastewater under batch conditions along with the optimization of the adsorption process by studying the effects of pH, contact time, and initial concentration of organics;
- Investigating the adsorption mechanism by analysis of dynamic and equilibrium behaviour using experimental data and various kinetic and isotherm models; and
- Developing an isotherm and kinetic model with potential application for predicting a continuous flow adsorption system.
2. Materials and Methods
2.1. Preparation of Wastewater
2.2. Preparation and Characterization of the Activated Carbon
2.3. Batch Adsorption Experiments
2.4. Analytical Technique
2.5. Error Analysis
3. Results and Discussion
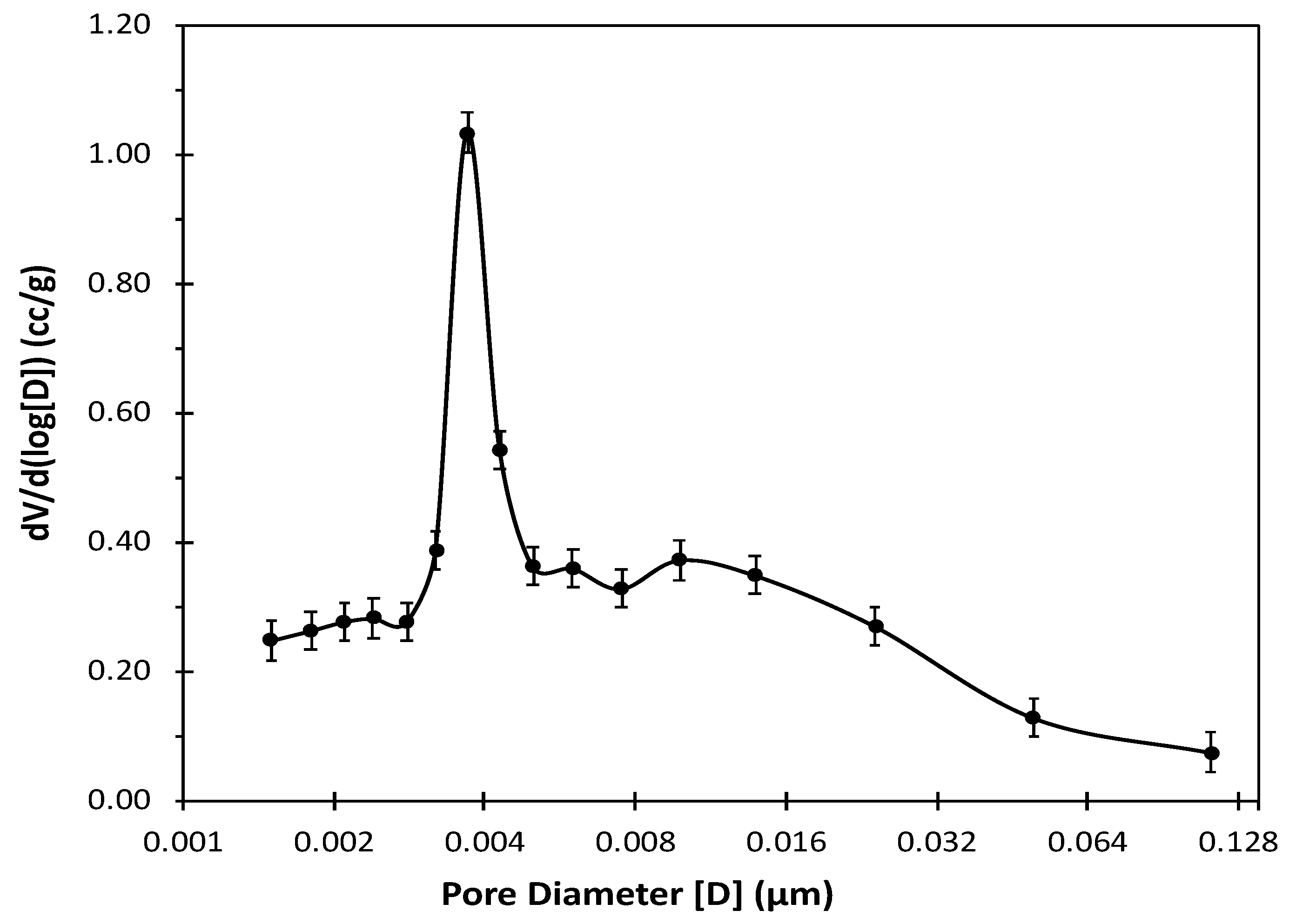
3.1. Surface Properties of the GAC
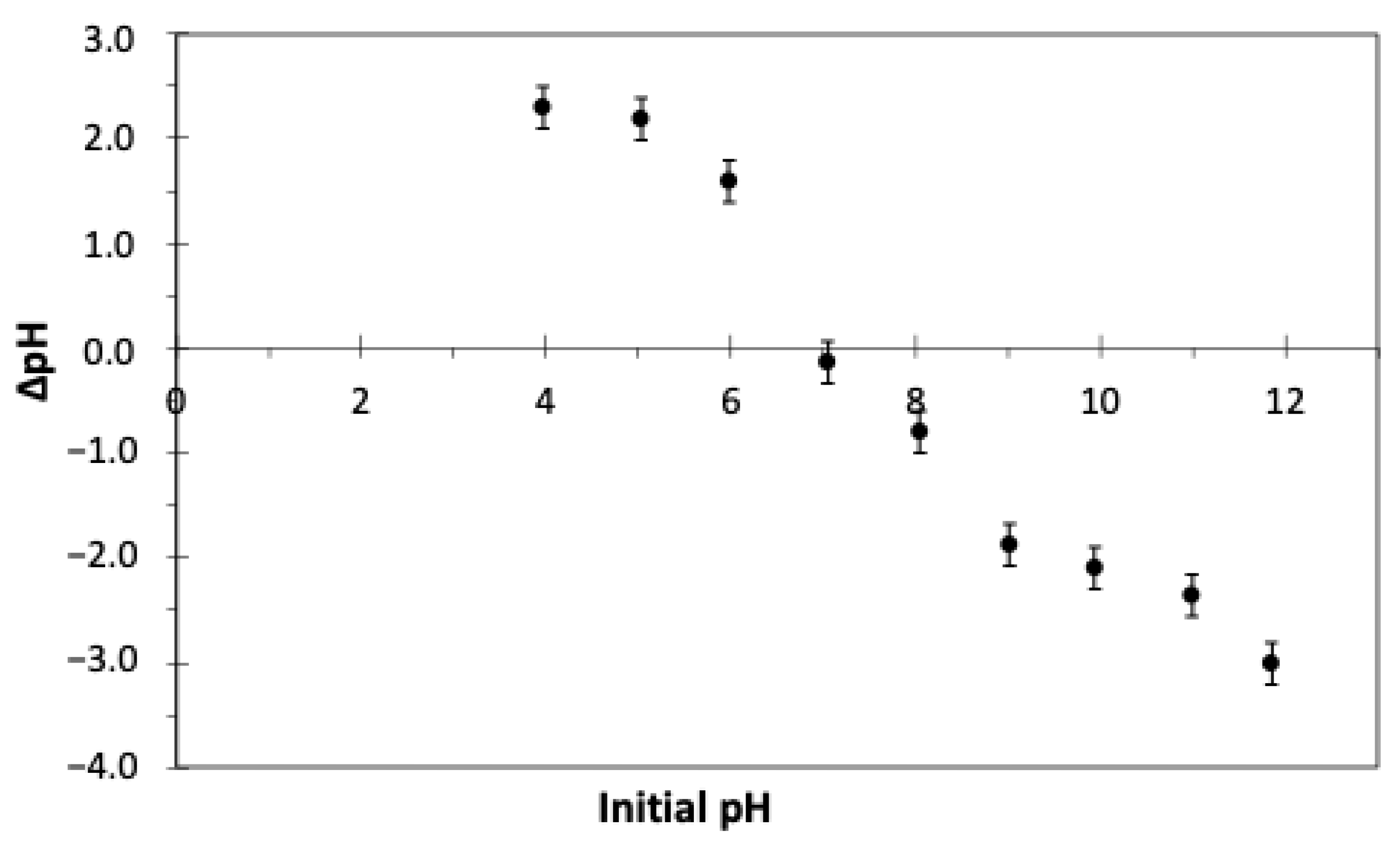
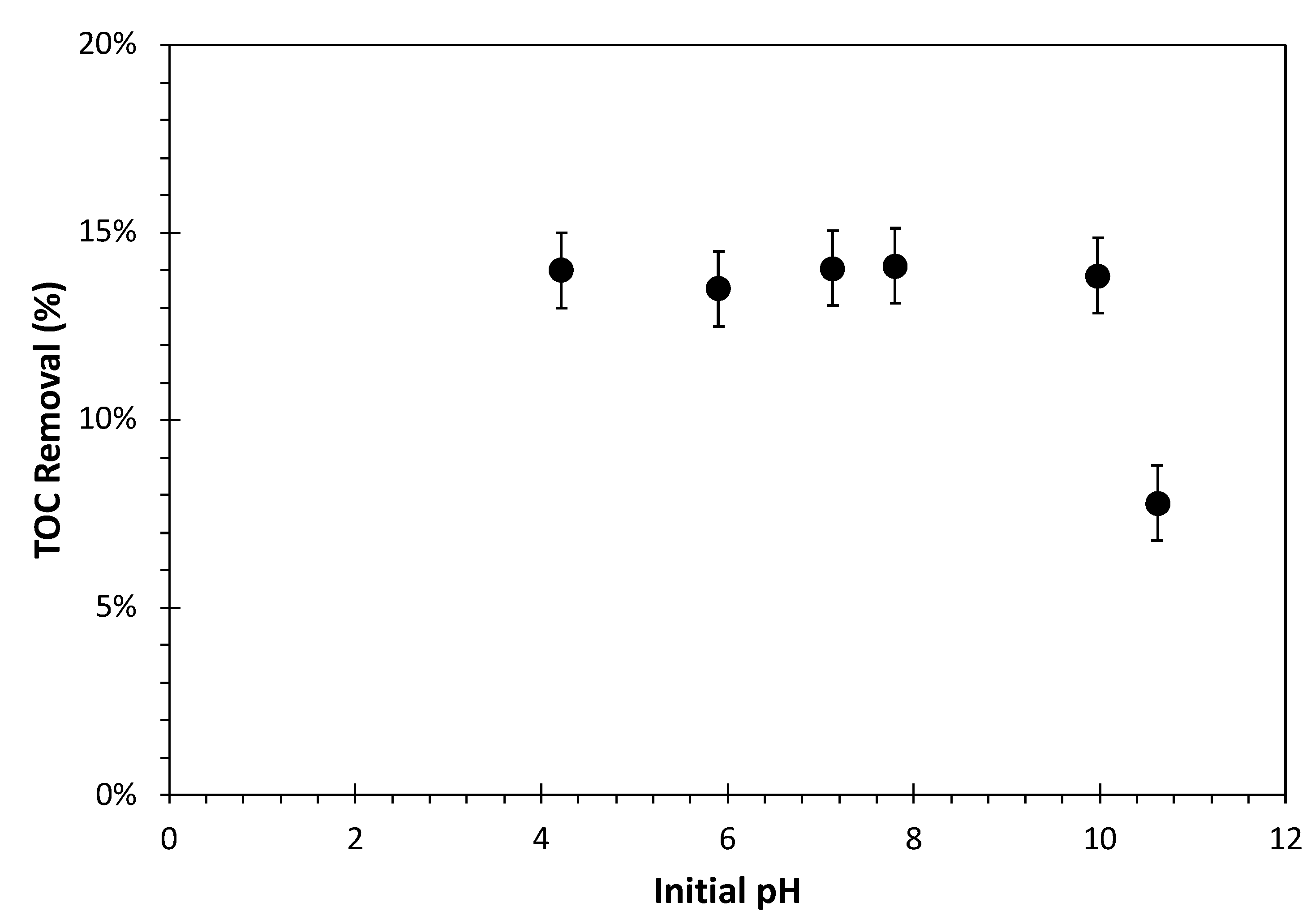
3.2. Effect of pH
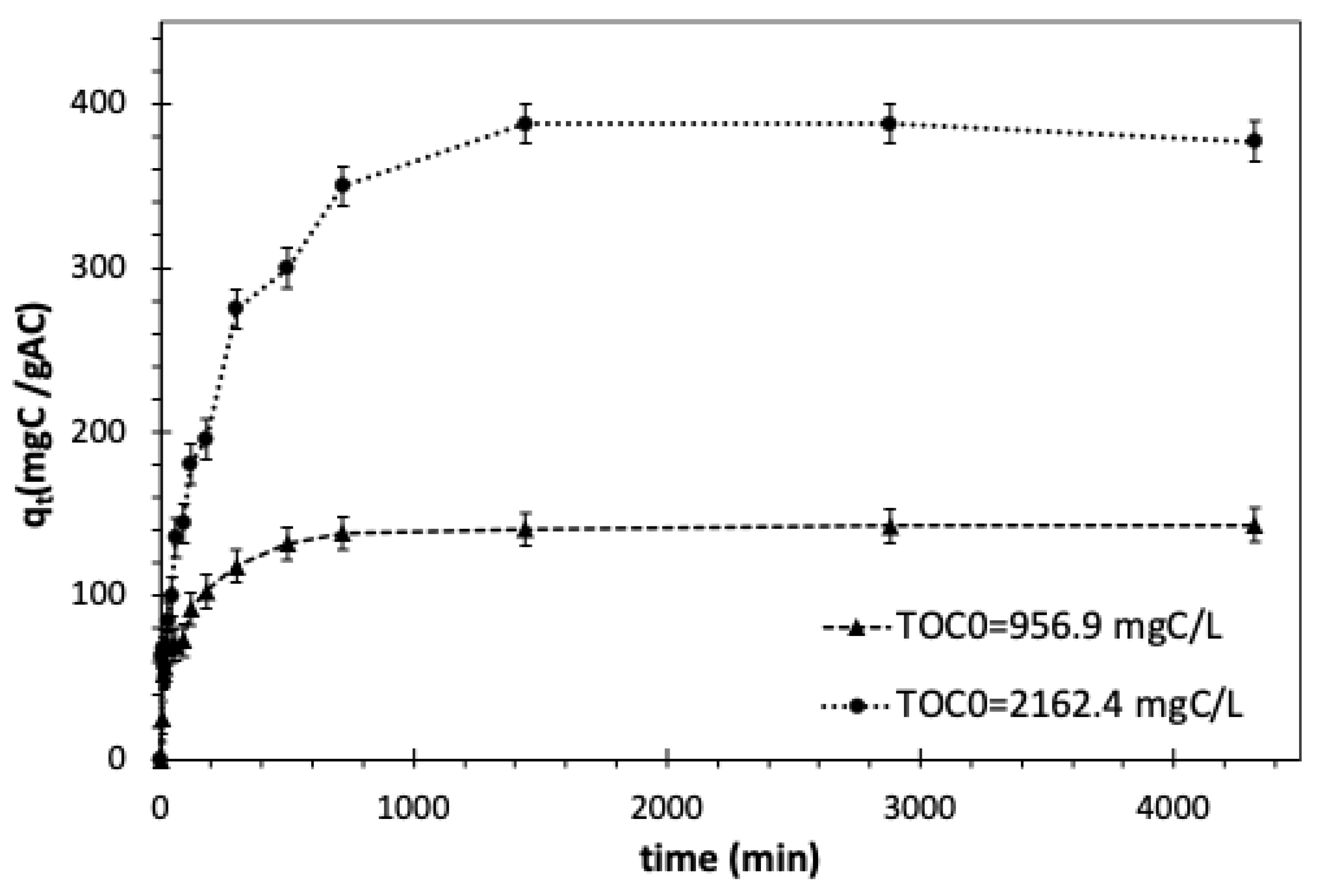
3.3. Effect of Contact Time
3.4. Effect of Initial Concentration on Adsorption
3.5. Adsorption Kinetic Studies
| Model | Correlation | Estimated Parameters | Error Analysis | |||
|---|---|---|---|---|---|---|
| ARE | R2 (%) | |||||
| W-M 1 | (9) | 3.66 | 66.5 | 9.90 | ||
| PFO 2 | (10) | 84.2 | 4.66 | |||
| Langmuir | (11) | 84.2 | 4.66 | |||
| PSO 3 | (12) | 99.9 | 2.29 | |||
3.6. Adsorption Isotherms
3.6.1. Langmuir Isotherm
3.6.2. Freundlich Isotherm
3.6.3. Dubinin-Radushkevich (D-R) Isotherm
3.6.4. Langmuir-Freundlich Isotherm
3.6.5. Brunauer-Emmett-Teller Isotherm
3.6.6. Fritz-Schlunder Isotherm
3.6.7. Selection of the Best Isotherm
4. Conclusions
- From the textural and surface analysis, commercial GAC is characterized by a narrow mesoporous structure, showing an average pore diameter of 3.63 nm and making it a proper candidate for removing pharmaceuticals as most have a molecular size of less than 2 nm. In addition, the zero net charge on the studied GAC was measured to be at a pH of 7.1.
- The findings from varying contact times and initial concentrations indicated that higher initial concentrations and longer content times resulted in greater pharmaceutical adsorption. This can be attributed to an increased driving force and sufficient time for the adsorbates to diffuse and bind to the activated carbon surface, leading to an enhanced adsorption capacity. The removal of pharmaceuticals remained unaffected by the change in the solution’s pH at values below 10. Beyond that, a substantial drop in the removal was observed due to the repulsion that exists between the negatively charged compounds and the surface of activated carbon. Consequently, no pH adjustment is required to favour the removal, which is beneficial in scale-up cost as no raw material for pH adjustment is needed.
- The dynamic studies showed that the Pseudo-second order reaction kinetic model provided the most accurate results over the entire adsorption time with an adsorption rate of for synthetic wastewater with initial TOC of . This model indicates that the removal of pharmaceuticals is mainly dependent on the rate at which the pharmaceuticals bind to the surface of the activated carbon. Furthermore, the multilinearity obtained by the Weber and Morris model confirmed the intraparticle diffusion, as well as the adsorption rate, determined the rate of pharmaceutical removal with the diffusion effect more dominant at the initial stages of the adsorption.
- Among the six investigated isotherm models explaining adsorption equilibrium behaviour, the Langmuir-Freundlich model provided the most accurate prediction of the experimental data, which could be inferred as multilayer adsorption and heterogeneous adsorption surface. The studied adsorption system was estimated to have a maximum adsorption capacity of 522.3 mgC/gAC following heterogeneity intensity of 4.70 and equilibrium constant equal to .
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Acronyms | |
| AC | Activated Carbon |
| ARE | Average Relative Error |
| BET | Brunauer–Emmett–Teller model |
| BJH | Barrett–Joyner–Halenda model |
| COD | Chemical Oxygen Demand |
| D-R | Dubinin-Radushkevich isotherm model |
| DCM | Dichloromethane |
| EPA | Environmental Protection Agency |
| F-test | Statistical Fisher test |
| GAC | Granular Activated Carbon |
| GHS | Globally Harmonized System |
| HK | Horvath-Kawazoe model |
| IUPAC | International Union of Pure and Applied Chemistry |
| PFO | Pseudo-First Order kinetic model |
| PPCP | Pharmaceuticals and Personal Care Products |
| PSO | Pseudo-Second Order kinetic model |
| PZC | Point of Zero Charge |
| RN | Residual Norm error |
| SPWW | Synthetic Pharmaceutical Wastewater |
| TN | Total Nitrogen |
| TOC | Total Organic Carbon |
| VOC | Volatile Organic Compound |
| W-M | Weber and Morris kinetic model |
| Greek letters | |
| Dubinin-Radushkevich isotherm constant | |
| Polanyi potential | |
| Special symbols | |
| b1 | BET isotherm constants |
| b2 | BET isotherm constants |
| C0 | Initial concentration |
| Ce | Concentration at equilibrium |
| WM | Weber and Morris constant |
| df | Degree of freedom |
| E | Energy of adsorption |
| K1 | Fritz-Schlunder isotherm constant |
| K2 | Fritz-Schlunder isotherm constant |
| KAd | Adsorption rate constant |
| KD | Desorption rate constant |
| KF | Freundlich isotherm constant |
| KL | Langmuir isotherm constant |
| KLF | Langmuir-Freundlich isotherm constant |
| KPFO | Pseudo-first order rate constant |
| KPSO | Pseudo-second order rate constant |
| KWM | Weber and Morris coefficient |
| m | Mass dry of activated carbon [g] |
| n | Langmuir-Freundlich isotherm power |
| nF | Freundlich isotherm power |
| p | Fritz-Schlunder isotherm power |
| P0 | Nitrogen saturation pressure at 77 K (kPa) |
| q | Activated carbon capacity |
| qe | Equilibrium adsorption capacity |
| qm | Maximum monolayer adsorption capacity |
| qs | Maximum adsorption capacity |
| R | Universal gas constant |
| R2 | Coefficient of determination |
| RL | Separation factor |
| s | Fritz-Schlunder isotherm power |
| T | Temperature () |
| t | Time (min) |
| TOC0 | Initial total organic carbon |
| V | Volume of solution (L) |
References
- Ghafoori, S.; Shah Kiran, K.; Mehrvar, M.; Chan, P.K. Pharmaceutical Wastewater Treatment Using Granular Activated Carbon and UV/H2O2 Processes: Experimental Analysis and Modelling. Can. J. Chem. Eng. 2014, 92, 1163–1173. [Google Scholar] [CrossRef]
- Johnson, M.B.; Mehrvar, M. Treatment of Actual Winery Wastewater by Fenton-like Process: Optimization to Improve Organic Removal, Reduce Inorganic Sludge Production and Enhance Co-Treatment at Municipal Wastewater Treatment Facilities. Water 2021, 14, 39. [Google Scholar] [CrossRef]
- Gadipelly, C.; Pérez-González, A.; Yadav, G.D.; Ortiz, I.; Ibáñez, R.; Rathod, V.K.; Marathe, K.V. Pharmaceutical Industry Wastewater: Review of the Technologies for Water Treatment and Reuse. Ind. Eng. Chem. Res. 2014, 53, 11571–11592. [Google Scholar] [CrossRef]
- Methanol Institute Methanol Safe Handling Technical Bulletin. Available online: https://www.methanol.org/safe-handling/ (accessed on 21 February 2024).
- Al-Dawery, S.K. Adsorption of Methanol from Methanol–Water Mixture by Activated Carbon and Its Regeneration Using Photo-Oxidation Process. Desalination Water Treat. 2016, 57, 3065–3073. [Google Scholar] [CrossRef]
- Han, Y.; Zhao, J.; Guo, X.; Jiao, T. Removal of Methanol from Water by Capacitive Deionization System Combined with Functional Nanoporous Graphene Membrane. Chemosphere 2023, 311, 137011. [Google Scholar] [CrossRef] [PubMed]
- Shestakova, M.; Sillanpää, M. Removal of Dichloromethane from Ground and Wastewater: A Review. Chemosphere 2013, 93, 1258–1267. [Google Scholar] [CrossRef] [PubMed]
- Teimoori, S.; Hassani, A.H.; Panahi, M.; Mansouri, N. A Review, Methods for Removal and Adsorption of Volatile Organic Compounds from Environmental Matrixes. Anal. Methods Environ. Chem. J. 2020, 3, 34–58. [Google Scholar] [CrossRef]
- Su, Y.; Fu, K.; Zheng, Y.; Ji, N.; Song, C.; Ma, D.; Lu, X.; Han, R.; Liu, Q. Catalytic Oxidation of Dichloromethane over Pt-Co/HZSM-5 Catalyst: Synergistic Effect of Single-Atom Pt, Co3O4, and HZSM-5. Appl. Catal. B Environ. 2021, 288, 119980. [Google Scholar] [CrossRef]
- Kyzas, G.Z.; McKay, G.; Al-Musawi, T.J.; Salehi, S.; Balarak, D. Removal of Benzene and Toluene from Synthetic Wastewater by Adsorption onto Magnetic Zeolitic Imidazole Framework Nanocomposites. Nanomaterials 2022, 12, 3049. [Google Scholar] [CrossRef]
- Ravindran, C.; Panayam Parambil Kunnathulli, A.; Kunhikrishnan Maniath, J. Polyvinyl Alcohol-Polyvinylpyrrolidone-Hydroxy Apatite (PVA-PVP-Hap) Membrane for Effective Removal of Benzene from Aqueous Solutions:-Kinetic, Isotherm, and Thermodynamic Studies. Mater. Today Proc. 2022, 66, 2422–2430. [Google Scholar] [CrossRef]
- US EPA National Primary Drinking Water Regulations. Available online: https://www.epa.gov/ground-water-and-drinking-water/national-primary-drinking-water-regulations (accessed on 25 April 2023).
- Mohajerani, M.; Mehrvar, M.; Ein-Mozaffari, F. Optimization of Aqueous P-Aminophenol Degradation by External-Loop Airlift Sonophotoreactor Using Response Surface Methodology. Chem. Eng. Res. Des. 2012, 90, 1221–1234. [Google Scholar] [CrossRef]
- Koyuncu, H.; Kul, A.R. Removal of Aniline from Aqueous Solution by Activated Kaolinite: Kinetic, Equilibrium and Thermodynamic Studies. Colloids Surf. A Physicochem. Eng. Asp. 2019, 569, 59–66. [Google Scholar] [CrossRef]
- Li, N.; Zhang, F.; Wang, H.; Hou, S. Catalytic Degradation of 4-Nitrophenol in Polluted Water by Three-Dimensional Gold Nanoparticles/Reduced Graphene Oxide Microspheres. Eng. Sci. 2019, 7, 72–79. [Google Scholar] [CrossRef]
- Sun, Y.; Wang, Y.; Peng, Z.; Liu, Y. Treatment of High Salinity Sulfanilic Acid Wastewater by Bipolar Membrane Electrodialysis. Sep. Purif. Technol. 2022, 281, 119842. [Google Scholar] [CrossRef]
- Karimi-Maleh, H.; Darabi, R.; Karimi, F.; Karaman, C.; Shahidi, S.A.; Zare, N.; Baghayeri, M.; Fu, L.; Rostamnia, S.; Rouhi, J.; et al. State-of-Art Advances on Removal, Degradation and Electrochemical Monitoring of 4-Aminophenol Pollutants in Real Samples: A Review. Environ. Res. 2023, 222, 115338. [Google Scholar] [CrossRef] [PubMed]
- Mowla, A.; Mehrvar, M.; Dhib, R. Combination of Sonophotolysis and Aerobic Activated Sludge Processes for Treatment of Synthetic Pharmaceutical Wastewater. Chem. Eng. J. 2014, 255, 411–423. [Google Scholar] [CrossRef]
- Shojaee Nasirabadi, P.; Saljoughi, E.; Mousavi, S.M. Membrane Processes Used for Removal of Pharmaceuticals, Hormones, Endocrine Disruptors and Their Metabolites from Wastewaters: A Review. Desalination Water Treat. 2016, 57, 24146–24175. [Google Scholar] [CrossRef]
- Jahani, R.; Dhib, R.; Mehrvar, M. Photochemical Degradation of Aqueous Artificial Sweeteners by UV/H2O2 and Their Biodegradability Studies. J. Chem. Technol. Biotechnol. 2020, 95, 2509–2521. [Google Scholar] [CrossRef]
- Wang, J.; Zhuan, R. Degradation of Antibiotics by Advanced Oxidation Processes: An Overview. Sci. Total Environ. 2020, 701, 135023. [Google Scholar] [CrossRef] [PubMed]
- Alfonso-Muniozguren, P.; Serna-Galvis, E.A.; Bussemaker, M.; Torres-Palma, R.A.; Lee, J. A Review on Pharmaceuticals Removal from Waters by Single and Combined Biological, Membrane Filtration and Ultrasound Systems. Ultrason. Sonochem. 2021, 76, 105656. [Google Scholar] [CrossRef]
- Martínez, F.; Molina, R.; Rodríguez, I.; Pariente, M.I.; Segura, Y.; Melero, J.A. Techno-Economical Assessment of Coupling Fenton/Biological Processes for the Treatment of a Pharmaceutical Wastewater. J. Environ. Chem. Eng. 2018, 6, 485–494. [Google Scholar] [CrossRef]
- Schneider, I.; Abbas, A.; Bollmann, A.; Dombrowski, A.; Knopp, G.; Schulte-Oehlmann, U.; Seitz, W.; Wagner, M.; Oehlmann, J. Post-Treatment of Ozonated Wastewater with Activated Carbon and Biofiltration Compared to Membrane Bioreactors: Toxicity Removal In Vitro and in Potamopyrgus Antipodarum. Water Res. 2020, 185, 116104. [Google Scholar] [CrossRef]
- Della-Flora, A.; Wilde, M.L.; Thue, P.S.; Lima, D.; Lima, E.C.; Sirtori, C. Combination of Solar Photo-Fenton and Adsorption Process for Removal of the Anticancer Drug Flutamide and Its Transformation Products from Hospital Wastewater. J. Hazard. Mater. 2020, 396, 122699. [Google Scholar] [CrossRef]
- Ricky, R.; Shanthakumar, S. Phycoremediation Integrated Approach for the Removal of Pharmaceuticals and Personal Care Products from Wastewater—A Review. J. Environ. Manag. 2022, 302, 113998. [Google Scholar] [CrossRef] [PubMed]
- Asheghmoalla, M.; Mehrvar, M. Integrated and Hybrid Processes for the Treatment of Actual Wastewaters Containing Micropollutants: A Review on Recent Advances. Processes 2024, 12, 339. [Google Scholar] [CrossRef]
- Al-Zghoul, T.M.; Al-Qodah, Z.; Al-Jamrah, A. Performance, Modeling, and Cost Analysis of Chemical Coagulation-Assisted Solar Powered Electrocoagulation Treatment System for Pharmaceutical Wastewater. Water 2023, 15, 980. [Google Scholar] [CrossRef]
- Jayawardhana, Y.; Mayakaduwa, S.S.; Kumarathilaka, P.; Gamage, S.; Vithanage, M. Municipal Solid Waste-Derived Biochar for the Removal of Benzene from Landfill Leachate. Env. Geochem. Health 2019, 41, 1739–1753. [Google Scholar] [CrossRef] [PubMed]
- Feng, X.; Tian, M.; He, C.; Li, L.; Shi, J.-W.; Yu, Y.; Cheng, J. Yolk-Shell-like Mesoporous CoCrOx with Superior Activity and Chlorine Resistance in Dichloromethane Destruction. Appl. Catal. B Environ. 2020, 264, 118493. [Google Scholar] [CrossRef]
- Shahid, M.K.; Kashif, A.; Fuwad, A.; Choi, Y. Current Advances in Treatment Technologies for Removal of Emerging Contaminants from Water—A Critical Review. Coord. Chem. Rev. 2021, 442, 213993. [Google Scholar] [CrossRef]
- Parsa, Z.; Dhib, R.; Mehrvar, M. Dynamic Modelling, Process Control, and Monitoring of Selected Biological and Advanced Oxidation Processes for Wastewater Treatment: A Review of Recent Developments. Bioengineering 2024, 11, 189. [Google Scholar] [CrossRef]
- Lin, Y.P.; Dhib, R.; Mehrvar, M. Nonlinear System Identification for Aqueous PVA Degradation in a Continuous UV/H2O2 Tubular Photoreactor. Ind. Eng. Chem. Res. 2021, 60, 1302–1315. [Google Scholar] [CrossRef]
- Patiño-Ruiz, D.; Rehmann, L.; Mehrvar, M.; Quiñones-Bolaños, E.; Herrera, A. Synthesis of FeO@SiO2–DNA Core–Shell Engineered Nanostructures for Rapid Adsorption of Heavy Metals in Aqueous Solutions. RSC Adv. 2020, 10, 39284–39294. [Google Scholar] [CrossRef]
- Zamiri, M.A.; Niu, C.H. Development and Characterization of Novel Activated Carbons Based on Reed Canary Grass. Ind. Crops Prod. 2022, 187, 115316. [Google Scholar] [CrossRef]
- De Andrade, J.R.; Oliveira, M.F.; Da Silva, M.G.C.; Vieira, M.G.A. Adsorption of Pharmaceuticals from Water and Wastewater Using Nonconventional Low-Cost Materials: A Review. Ind. Eng. Chem. Res. 2018, 57, 3103–3127. [Google Scholar] [CrossRef]
- Mansour, F.; Al-Hindi, M.; Yahfoufi, R.; Ayoub, G.M.; Ahmad, M.N. The Use of Activated Carbon for the Removal of Pharmaceuticals from Aqueous Solutions: A Review. Rev. Environ. Sci. Biotechnol. 2018, 17, 109–145. [Google Scholar] [CrossRef]
- Jatmiko, T.H. Optimization of Production Activated Carbon for Removal of Pharmaceuticals Wastewater Using Taguchi Method and Grey Relational Analysis. J. Ris. Teknol. Pencegah. Pencemaran Ind. 2020, 11, 11–18. [Google Scholar] [CrossRef]
- Pirvu, F.; Covaliu-Mierlă, C.I.; Paun, I.; Paraschiv, G.; Iancu, V. Treatment of Wastewater Containing Nonsteroidal Anti-Inflammatory Drugs Using Activated Carbon Material. Materials 2022, 15, 559. [Google Scholar] [CrossRef] [PubMed]
- Mestre, A.S.; Viegas, R.M.C.; Mesquita, E.; Rosa, M.J.; Carvalho, A.P. Engineered Pine Nut Shell Derived Activated Carbons for Improved Removal of Recalcitrant Pharmaceuticals in Urban Wastewater Treatment. J. Hazard. Mater. 2022, 437, 129319. [Google Scholar] [CrossRef]
- Hu, W.; Niu, Y.; Dong, K.; Wang, D. Removal of Sulfamethoxazole from Aqueous Solution onto Bagasse-Derived Activated Carbon: Response Surface Methodology, Isotherm and Kinetics Studies. J. Mol. Liq. 2022, 347, 118141. [Google Scholar] [CrossRef]
- Hörsing, M.; Andersen, H.R.; Grabic, R.; Jansen, J.L.C.; Ledin, A. Sorption of 71 Pharmaceuticals to Powder Activated Carbon for Improved Wastewater Treatment. Clean Technol. 2022, 4, 296–308. [Google Scholar] [CrossRef]
- Zhang, Y.; Dou, B.; Liu, X.; Fan, H.; Geng, C.; Liu, X.; Chang, J.; Hao, Q.; Hu, X.; Yang, Y.; et al. Experimental and Theoretical Study of the Adsorption of Mixed Low Carbon Alcohols and Acids from Fischer Tropsch Synthesis Wastewater by Activated Carbon. Fuel 2023, 339, 126928. [Google Scholar] [CrossRef]
- Eljaiek-Urzola, M.; Guardiola-Meza, L.; Ghafoori, S.; Mehrvar, M. Treatment of Mature Landfill Leachate Using Hybrid Processes of Hydrogen Peroxide and Adsorption in an Activated Carbon Fixed Bed Column. J. Environ. Sci. Health Part A 2018, 53, 238–243. [Google Scholar] [CrossRef] [PubMed]
- Kim, S.H.; Shon, H.K.; Ngo, H.H. Adsorption Characteristics of Antibiotics Trimethoprim on Powdered and Granular Activated Carbon. J. Ind. Eng. Chem. 2010, 16, 344–349. [Google Scholar] [CrossRef]
- Awwad, M.; Al-Rimawi, F.; Dajani, K.J.K.; Khamis, M.; Nir, S.; Karaman, R. Removal of Amoxicillin and Cefuroxime Axetil by Advanced Membranes Technology, Activated Carbon and Micelle–Clay Complex. Environ. Technol. 2015, 36, 2069–2078. [Google Scholar] [CrossRef] [PubMed]
- Al-Dawery, S.K.; Aljundi, I.H. Methanol Removal from Methanol-Water Mixture Using Activated Sludge, Air Stripping and Adsorption Process: Comparative Study. J. Eng. Sci. Technol. 2015, 10, 1615–1627. [Google Scholar]
- Kennedy, A.M.; Reinert, A.M.; Knappe, D.R.U.; Ferrer, I.; Summers, R.S. Full- and Pilot-Scale GAC Adsorption of Organic Micropollutants. Water Res. 2015, 68, 238–248. [Google Scholar] [CrossRef] [PubMed]
- Sgroi, M.; Anumol, T.; Roccaro, P.; Vagliasindi, F.G.A.; Snyder, S.A. Modeling Emerging Contaminants Breakthrough in Packed Bed Adsorption Columns by UV Absorbance and Fluorescing Components of Dissolved Organic Matter. Water Res. 2018, 145, 667–677. [Google Scholar] [CrossRef]
- Krahnstöver, T.; Santos, N.; Georges, K.; Campos, L.; Antizar-Ladislao, B. Low-Carbon Technologies to Remove Organic Micropollutants from Wastewater: A Focus on Pharmaceuticals. Sustainability 2022, 14, 11686. [Google Scholar] [CrossRef]
- Aljeboree, A.M.; Alshirifi, A.N. Adsorption of Pharmaceuticals as Emerging Contaminants from Aqueous Solutions on to Friendly Surfaces Such as Activated Carbon: A Review. J. Pharm. Sci. Res 2018, 10, 2252–2257. [Google Scholar]
- Metcalf, L.; Eddy, H.; Tchobanoglous, G. Wastewater Engineering: Treatment, Disposal, and Reuse, 4th ed.; Tchobanoglous, G., Stensel, H.D., Burton, F.L., Eds.; McGraw-Hill: New York, NY, USA, 1991; ISBN 0-07-124140-X. [Google Scholar]
- Rodgers, M.; Healy, M.G.; Mulqueen, J. Organic Carbon Removal and Nitrification of High Strength Wastewaters Using Stratified Sand Filters. Water Res. 2005, 39, 3279–3286. [Google Scholar] [CrossRef]
- Vanerkar, A.P.; Satyanarayan, S.; Dharmadhikari, D.M. Enhancement of Organic Removals in High Strength Herbal Pharmaceutical Wastewater. Environ. Technol. 2005, 26, 389–396. [Google Scholar] [CrossRef]
- Wang, J.; Guo, X. Adsorption Kinetic Models: Physical Meanings, Applications, and Solving Methods. J. Hazard. Mater. 2020, 390, 122156. [Google Scholar] [CrossRef] [PubMed]
- Largitte, L.; Pasquier, R. A Review of the Kinetics Adsorption Models and Their Application to the Adsorption of Lead by an Activated Carbon. Chem. Eng. Res. Des. 2016, 109, 495–504. [Google Scholar] [CrossRef]
- Ayawei, N.; Ebelegi, A.N.; Wankasi, D. Modelling and Interpretation of Adsorption Isotherms. J. Chem. 2017, 2017, 3039817. [Google Scholar] [CrossRef]
- Behjoee, M.; Zamiri, M.A.; Tabil, L.G.; Baik, O.-D.; Niu, C.H. Equilibrium, Thermodynamics, and Regeneration and Reuse of Biosorbents Developed from Pelletized Agricultural Residues for Gas Dehydration. Energy Fuels 2023, 37, 1451–1463. [Google Scholar] [CrossRef]
- Priya, V.S.; Philip, L. Biodegradation of Dichloromethane Along with Other VOCs from Pharmaceutical Wastewater. Appl. Biochem. Biotechnol. 2013, 169, 1197–1218. [Google Scholar] [CrossRef] [PubMed]
- Ghafoori, S.; Mowla, A.; Jahani, R.; Mehrvar, M.; Chan, P.K. Sonophotolytic Degradation of Synthetic Pharmaceutical Wastewater: Statistical Experimental Design and Modeling. J. Environ. Manag. 2015, 150, 128–137. [Google Scholar] [CrossRef]
- Gupta, A.; Garg, A. Adsorption and Oxidation of Ciprofloxacin in a Fixed Bed Column Using Activated Sludge Derived Activated Carbon. J. Environ. Manag. 2019, 250, 109474. [Google Scholar] [CrossRef]
- Kaya, Y.; Bacaksiz, A.M.; Bayrak, H.; Gönder, Z.B.; Vergili, I.; Hasar, H.; Yilmaz, G. Treatment of Chemical Synthesis-Based Pharmaceutical Wastewater in an Ozonation-Anaerobic Membrane Bioreactor (AnMBR) System. Chem. Eng. J. 2017, 322, 293–301. [Google Scholar] [CrossRef]
- Ganzenko, O.; Trellu, C.; Papirio, S.; Oturan, N.; Huguenot, D.; van Hullebusch, E.D.; Esposito, G.; Oturan, M.A. Bioelectro-Fenton: Evaluation of a Combined Biological—Advanced Oxidation Treatment for Pharmaceutical Wastewater. Environ. Sci. Pollut. Res. 2018, 25, 20283–20292. [Google Scholar] [CrossRef]
- Ng, K.K.; Shi, X.; Tang, M.K.Y.; Ng, H.Y. A Novel Application of Anaerobic Bio-Entrapped Membrane Reactor for the Treatment of Chemical Synthesis-Based Pharmaceutical Wastewater. Sep. Purif. Technol. 2014, 132, 634–643. [Google Scholar] [CrossRef]
- Gupta, R.; Sati, B.; Gupta, A. Treatment and Recycling of Wastewater from Pharmaceutical Industry. In Advances in Biological Treatment of Industrial Wastewater and their Recycling for a Sustainable Future, Applied Environmental Science and Engineering for a Sustainable Future; Singh, R.L., Singh, R.P., Eds.; Applied Environmental Science and Engineering for a Sustainable Future; Springer: Singapore, 2019; pp. 267–302. ISBN 9789811314681. [Google Scholar]
- Wang, K.; Liu, S.; Zhang, Q.; He, Y. Pharmaceutical Wastewater Treatment by Internal Micro-electrolysis–Coagulation, Biological Treatment and Activated Carbon Adsorption. Environ. Technol. 2009, 30, 1469–1474. [Google Scholar] [CrossRef] [PubMed]
- Kavitha, R.V.; Murthy, V.K.; Makam, R.; Asith, K. a Physico-Chemical Analysis of Effluents from Pharmaceutical Industry and Its Efficiency Study. Int. J. Eng. Res. Appl. 2012, 2, 103–110. [Google Scholar]
- Wu, Z.; Cong, Y.; Zhou, M.; Tan, T. P-Nitrophenol Abatement by the Combination of Electrocatalysis and Activated Carbon. Chem. Eng. J. 2005, 106, 83–90. [Google Scholar] [CrossRef]
- Obiri-Nyarko, F.; Kwiatkowska-Malina, J.; Kumahor, S.K.; Malina, G. Evaluating Low-Cost Permeable Adsorptive Barriers for the Removal of Benzene from Groundwater: Laboratory Experiments and Numerical Modelling. J. Contam. Hydrol. 2022, 250, 104054. [Google Scholar] [CrossRef]
- Rineksa, G.; Bustomy, A.; Park, D.-H.; Gozan, M. Isotherm Coefficient for Benzene and Toluene Adsorption on Granular Activated Carbon for Preparation of Biobarrier. IOP Conf. Ser. Earth Environ. Sci. 2022, 1111, 012049. [Google Scholar] [CrossRef]
- Melaphi, K.; Sadare, O.O.; Simate, G.S.; Wagenaar, S.; Moothi, K. Adsorptive Removal of BTEX Compounds from Wastewater Using Activated Carbon Derived from Macadamia Nut Shells. WSA 2023, 49, 36–45. [Google Scholar] [CrossRef]
- Zeinali, F.; Ghoreyshi, A.A.; Najafpour, G. Removal of toluene and dichloromethane from aqueous phase by granular activated carbon (GAC). Chem. Eng. Commun. 2012, 199, 203–220. [Google Scholar] [CrossRef]
- Alhooshani, K.R. Adsorption of Chlorinated Organic Compounds from Water with Cerium Oxide-Activated Carbon Composite. Arab. J. Chem. 2019, 12, 2585–2596. [Google Scholar] [CrossRef]
- Mangotra, A.; Singh, S.K.; Mohan, A. Preparation, Characterization and Assessment of Low Cost Green Adsorbent Prepared from Coconut Shell for Removal of Toxic Dichloromethane. Ann. Biol. 2023, 39, 41–48. [Google Scholar]
- Chen, C.; Geng, X.; Huang, W. Adsorption of 4-Chlorophenol and Aniline by Nanosized Activated Carbons. Chem. Eng. J. 2017, 327, 941–952. [Google Scholar] [CrossRef]
- Li, H.; Liu, L.; Cui, J.; Cui, J.; Wang, F.; Zhang, F. High-Efficiency Adsorption and Regeneration of Methylene Blue and Aniline onto Activated Carbon from Waste Edible Fungus Residue and Its Possible Mechanism. RSC Adv. 2020, 10, 14262–14273. [Google Scholar] [CrossRef]
- Celarek, M. Sorptive Removal of 4-Aminophenol from Water Using a Polymeric Sorbent. Master’s Thesis, University of Waterloo, Waterloo, ON, Canada, 2017. [Google Scholar]
- Abdul Ameer, A.M. Removal of Mixture of Phenolic Compounds from Aqueous Solution by Tire Char Adsorption. IOP Conf. Ser. Mater. Sci. Eng. 2019, 518, 062011. [Google Scholar] [CrossRef]
- Mishra, P.; Singh, K.; Dixit, U.; Agarwal, A.; Ahmad Bhat, R. Effective Removal of 4-Aminophenol from Aqueous Environment by Pea (Pisum sativum) Shells Activated with Sulfuric Acid: Characterization, Isotherm, Kinetics and Thermodynamics. J. Indian Chem. Soc. 2022, 99, 100528. [Google Scholar] [CrossRef]
- Al-Amrani, W.A.; Hanafiah, M.A.K.M.; Lim, P.-E. Influence of Hydrophilicity/Hydrophobicity on Adsorption/Desorption of Sulfanilic Acid Using Amine-Modified Silicas and Granular Activated Carbon. DWT 2022, 249, 109–118. [Google Scholar] [CrossRef]
- Brunauer, S.; Emmett, P.H.; Teller, E. Adsorption of Gases in Multimolecular Layers. J. Am. Chem. Soc. 1938, 60, 309–319. [Google Scholar] [CrossRef]
- Dubinin, M.M. The Potential Theory of Adsorption of Gases and Vapors for Adsorbents with Energetically Nonuniform Surfaces. Chem. Rev. 1960, 60, 235–241. [Google Scholar] [CrossRef]
- de Boer, J.H.; Linsen, B.G.; van der Plas, T.; Zondervan, G.J. Studies on Pore Systems in Catalysts: VII. Description of the Pore Dimensions of Carbon Blacks by the t Method. J. Catal. 1965, 4, 649–653. [Google Scholar] [CrossRef]
- Ravikovitch, P.I.; Haller, G.L.; Neimark, A.V. Density Functional Theory Model for Calculating Pore Size Distributions: Pore Structure of Nanoporous Catalysts. Adv. Colloid Interface Sci. 1998, 76–77, 203–226. [Google Scholar] [CrossRef]
- Rivera-Utrilla, J.; Bautista-Toledo, I.; Ferro-García, M.A.; Moreno-Castilla, C. Activated Carbon Surface Modifications by Adsorption of Bacteria and Their Effect on Aqueous Lead Adsorption. J. Chem. Tech. Biotech. 2001, 76, 1209–1215. [Google Scholar] [CrossRef]
- Órfão, J.J.M.; Silva, A.I.M.; Pereira, J.C.V.; Barata, S.A.; Fonseca, I.M.; Faria, P.C.C.; Pereira, M.F.R. Adsorption of a Reactive Dye on Chemically Modified Activated Carbons—Influence of pH. J. Colloid Interface Sci. 2006, 296, 480–489. [Google Scholar] [CrossRef] [PubMed]
- Ehiomogue, P.; Ahuchaogu, I.; Ahaneku, E. A Review of Bioreremediation of Hydrocarbon Contaminated Soils in Niger Delta Area of Nigeria. Poljopr. Teh. 2021, 46, 23–39. [Google Scholar] [CrossRef]
- Qureshi, U.A.; Hameed, B.H.; Ahmed, M.J. Adsorption of Endocrine Disrupting Compounds and Other Emerging Contaminants Using Lignocellulosic Biomass-Derived Porous Carbons: A Review. J. Water Process Eng. 2020, 38, 101380. [Google Scholar] [CrossRef]
- Kalam, S.; Abu-Khamsin, S.A.; Kamal, M.S.; Patil, S. Surfactant Adsorption Isotherms: A Review. ACS Omega 2021, 6, 32342–32348. [Google Scholar] [CrossRef] [PubMed]
- Marković, D.D.; Lekić, B.M.; Rajaković-Ognjanović, V.N.; Onjia, A.E.; Rajaković, L.V. A New Approach in Regression Analysis for Modeling Adsorption Isotherms. Sci. World J. 2014, 2014, 930879. [Google Scholar] [CrossRef] [PubMed]
- MATLAB and Curve Fitting Toolbox. Version 9.14 (R2023a); The MathWorks Inc.: Natick, MA, USA, 2023; Available online: https://www.mathworks.com (accessed on 12 December 2023).
- Podder, M.S.; Majumder, C.B. Sequestering of As(III) and As(V) from Wastewater Using a Novel Neem Leaves/MnFe 2 O 4 Composite Biosorbent. Int. J. Phytoremediation 2016, 18, 1237–1257. [Google Scholar] [CrossRef] [PubMed]
- Pauletto, P.S.; Lütke, S.F.; Dotto, G.L.; Salau, N.P.G. Adsorption Mechanisms of Single and Simultaneous Removal of Pharmaceutical Compounds onto Activated Carbon: Isotherm and Thermodynamic Modeling. J. Mol. Liq. 2021, 336, 116203. [Google Scholar] [CrossRef]
- Allen, S.J.; Gan, Q.; Matthews, R.; Johnson, P.A. Comparison of Optimised Isotherm Models for Basic Dye Adsorption by Kudzu. Bioresour. Technol. 2003, 88, 143–152. [Google Scholar] [CrossRef] [PubMed]
- Hashem, A.; Al-Anwar, A.; Nagy, N.M.; Hussein, D.M.; Eisa, S. Isotherms and Kinetic Studies on Adsorption of Hg(II) Ions onto Ziziphus Spina-Christi L. from Aqueous Solutions. Green Process. Synth. 2016, 5, 213–224. [Google Scholar] [CrossRef]
- Revellame, E.D.; Fortela, D.L.; Sharp, W.; Hernandez, R.; Zappi, M.E. Adsorption Kinetic Modeling Using Pseudo-First Order and Pseudo-Second Order Rate Laws: A Review. Clean. Eng. Technol. 2020, 1, 100032. [Google Scholar] [CrossRef]
- Foo, K.Y.; Hameed, B.H. Insights into the Modeling of Adsorption Isotherm Systems. Chem. Eng. J. 2010, 156, 2–10. [Google Scholar] [CrossRef]
- Kletting, P.; Glatting, G. Model Selection for Time-Activity Curves: The Corrected Akaike Information Criterion and the F-Test. Z. Med. Phys. 2009, 19, 200–206. [Google Scholar] [CrossRef] [PubMed]
- Yu, J.; Yang, F.-C.; Hung, W.-N.; Liu, C.-L.; Yang, M.; Lin, T.-F. Prediction of Powdered Activated Carbon Doses for 2-MIB Removal in Drinking Water Treatment Using a Simplified HSDM Approach. Chemosphere 2016, 156, 374–382. [Google Scholar] [CrossRef] [PubMed]
- Connelly, A. BET Surface Area—Andy Connelly. Available online: https://andyjconnelly.wordpress.com/2017/03/13/bet-surface-area/ (accessed on 27 September 2022).
- Sing, K.S.W.; Everett, D.H.; Haul, R.A.W.; Moscou, L.; Pierotti, R.A.; Rouquerol, J.; Siemieniewska, T. Reporting Physisorption Data for Gas/Solid Systems with Special Reference to the Determination of Surface Area and Porosity (Recommendations 1984). Pure Appl. Chem. 1985, 57, 603–619. [Google Scholar] [CrossRef]
- Sotelo, J.L.; Rodríguez, A.R.; Mateos, M.M.; Hernández, S.D.; Torrellas, S.A.; Rodríguez, J.G. Adsorption of Pharmaceutical Compounds and an Endocrine Disruptor from Aqueous Solutions by Carbon Materials. J. Environ. Sci. Health Part B 2012, 47, 640–652. [Google Scholar] [CrossRef] [PubMed]
- Sumanjit; Rani, S.; Mahajan, R.K. Equilibrium, Kinetics and Thermodynamic Parameters for Adsorptive Removal of Dye Basic Blue 9 by Ground Nut Shells and Eichhornia. Arab. J. Chem. 2016, 9, S1464–S1477. [Google Scholar] [CrossRef]
- Çalışkan, E.; Göktürk, S. Adsorption Characteristics of Sulfamethoxazole and Metronidazole on Activated Carbon. Sep. Sci. Technol. 2010, 45, 244–255. [Google Scholar] [CrossRef]
- Sajid, M.; Bari, S.; Saif Ur Rehman, M.; Ashfaq, M.; Guoliang, Y.; Mustafa, G. Adsorption Characteristics of Paracetamol Removal onto Activated Carbon Prepared from Cannabis Sativum Hemp. Alex. Eng. J. 2022, 61, 7203–7212. [Google Scholar] [CrossRef]
- Al-Qodah, Z.; Shawaqfeh, A.T.; Lafi, W.K. Two-Resistance Mass Transfer Model for the Adsorption of the Pesticide Deltamethrin Using Acid Treated Oil Shale Ash. Adsorption 2007, 13, 73–82. [Google Scholar] [CrossRef]
- Baccar, R.; Sarrà, M.; Bouzid, J.; Feki, M.; Blánquez, P. Removal of Pharmaceutical Compounds by Activated Carbon Prepared from Agricultural By-Product. Chem. Eng. J. 2012, 211–212, 310–317. [Google Scholar] [CrossRef]
- Mohammed, R.R.; Ketabachi, M.R.; McKay, G. Combined Magnetic Field and Adsorption Process for Treatment of Biologically Treated Palm Oil Mill Effluent (POME). Chem. Eng. J. 2014, 243, 31–42. [Google Scholar] [CrossRef]
- Kaur, H.; Bansiwal, A.; Hippargi, G.; Pophali, G.R. Effect of Hydrophobicity of Pharmaceuticals and Personal Care Products for Adsorption on Activated Carbon: Adsorption Isotherms, Kinetics and Mechanism. Environ. Sci. Pollut. Res. 2018, 25, 20473–20485. [Google Scholar] [CrossRef] [PubMed]
- Gao, J.; Kong, D.; Wang, Y.; Wu, J.; Sun, S.; Xu, P. Production of Mesoporous Activated Carbon from Tea Fruit Peel Residues and Its Evaluation of Methylene Blue Removal from Aqueous Solutions. BioResources 2013, 8, 2145–2160. [Google Scholar] [CrossRef]
- Song, X.; Zhang, Y.; Yan, C.; Jiang, W.; Chang, C. The Langmuir Monolayer Adsorption Model of Organic Matter into Effective Pores in Activated Carbon. J. Colloid Interface Sci. 2013, 389, 213–219. [Google Scholar] [CrossRef] [PubMed]
- Lyubchik, S.; Lyubchik, A.; Lygina, O.; Lyubchik, S.; Fonsec, I. Comparison of the Thermodynamic Parameters Estimation for the Adsorption Process of the Metals from Liquid Phase on Activated Carbons. In Thermodynamics—Interaction Studies—Solids, Liquids and Gases; Moreno Pirajn, J.C., Ed.; InTech: London, UK, 2011; p. 932. ISBN 978-953-307-563-1. [Google Scholar]
- Álvarez-Torrellas, S.; Peres, J.A.; Gil-Álvarez, V.; Ovejero, G.; García, J. Effective Adsorption of Non-Biodegradable Pharmaceuticals from Hospital Wastewater with Different Carbon Materials. Chem. Eng. J. 2017, 320, 319–329. [Google Scholar] [CrossRef]
- Batool, F.; Akbar, J.; Iqbal, S.; Noreen, S.; Bukhari, S.N.A. Study of Isothermal, Kinetic, and Thermodynamic Parameters for Adsorption of Cadmium: An Overview of Linear and Nonlinear Approach and Error Analysis. Bioinorg. Chem. Appl. 2018, 2018, 1–11. [Google Scholar] [CrossRef]
- Yaneva, Z.L.; Koumanova, B.K.; Georgieva, N.V. Linear and Nonlinear Regression Methods for Equilibrium Modelling of p-Nitrophenol Biosorption by Rhizopus Oryzae: Comparison of Error Analysis Criteria. J. Chem. 2013, 2013, 1–10. [Google Scholar] [CrossRef]
- Ramadoss, R.; Subramaniam, D. Removal of Divalent Nickel from Aqueous Solution Using Blue-Green Marine Algae: Adsorption Modeling and Applicability of Various Isotherm Models. Sep. Sci. Technol. 2019, 54, 943–961. [Google Scholar] [CrossRef]
- Brião, G.D.V.; Hashim, M.A.; Chu, K.H. The Sips Isotherm Equation: Often Used and Sometimes Misused. Sep. Sci. Technol. 2023, 58, 884–892. [Google Scholar] [CrossRef]
- Girish, C.R. Various Isotherm Models for Multicomponent Adsorption: A Review. Int. J. Civ. Eng. Technol. 2017, 8, 80–86. [Google Scholar]
- Shimizu, S.; Matubayasi, N. Cooperative Sorption on Porous Materials. Langmuir 2021, 37, 10279–10290. [Google Scholar] [CrossRef] [PubMed]
- Hamdaoui, O.; Naffrechoux, E. Modeling of Adsorption Isotherms of Phenol and Chlorophenols onto Granular Activated carbonPart II. Models with More than Two Parameters. J. Hazard. Mater. 2007, 147, 401–411. [Google Scholar] [CrossRef] [PubMed]
- Bolong, N.; Ismail, A.F.; Salim, M.R.; Matsuura, T. A Review of the Effects of Emerging Contaminants in Wastewater and Options for Their Removal. Desalination 2009, 239, 229–246. [Google Scholar] [CrossRef]
- Brião, G.D.V.; Da Silva, M.G.C.; Vieira, M.G.A.; Chu, K.H. Correlation of Type II Adsorption Isotherms of Water Contaminants Using Modified BET Equations. Colloid Interface Sci. Commun. 2022, 46, 100557. [Google Scholar] [CrossRef]
- Al-Ghouti, M.A.; Da’ana, D.A. Guidelines for the Use and Interpretation of Adsorption Isotherm Models: A Review. J. Hazard. Mater. 2020, 393, 122383. [Google Scholar] [CrossRef] [PubMed]
- Isiuku, B.O.; Okonkwo, P.C.; Emeagwara, C.D. Batch Adsorption Isotherm Models Applied in Single and Multicomponent Adsorption Systems—A Review. J. Dispers. Sci. Technol. 2021, 42, 1879–1897. [Google Scholar] [CrossRef]
- Al-Qodah, Z.; Al-Shannag, M.; Hudaib, B.; Bani-Salameh, W. Enhancement of Dairy Wastewater Treatment Efficiency in Batch Chemical-Assisted Solar-Powered Electrocoagulation-Adsorption System. Case Stud. Chem. Environ. Eng. 2024, 9, 100760. [Google Scholar] [CrossRef]
- Al-Qodah, Z.; Al-Zghoul, T.M.; Jamrah, A. The Performance of Pharmaceutical Wastewater Treatment System of Electrocoagulation Assisted Adsorption Using Perforated Electrodes to Reduce Passivation. Env. Sci. Pollut. Res. 2024, 31, 20434–20448. [Google Scholar] [CrossRef]




| Compound | Structure | Properties | Manufacturer |
|---|---|---|---|
| Methanol (CH3OH) |  | MW: 32.04 (g/mol) Log KOW: −0.77 pKa: 15.3 size: 0.36 nm Purity: 99.8% | VWR Chemicals BDH, Edmonton, AB, Canada |
| Benzene (C6H6) |  | MW: 78.11 (g/mol) Log KOW: 2.13 Size: 0.53 nm Purity: 99% | EMD Chemicals, Etobicoke, ON, Canada |
| Methylene chloride (CH2Cl2) |  | MW: 84.93 (g/mol) Log KOW: 1.25 Size: 0.33 nm Purity: 99.8% | EMD Chemicals, Etobicoke, ON, Canada |
| Aniline (C6H5NH2) |  | MW: 93.13 (g/mol) Log KOW: 0.90 pKa: 4.60 Purity: 100% | J.T. Baker, Mississauga, ON, Canada |
| 4-Aminophenol (C6H4OHNH2) | 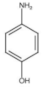 | MW: 109.13 (g/mol) Log KOW: 7.40 pK1: 5.48 pK2: 10.46 Purity: 98% | Alfa Aesar, Mississauga, ON, Canada |
| Sulfanilic Acid (C6H4NH2SO3H) | 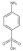 | MW: 173.19 (g/mol) Log KOW: −2.16 pKa: 3.25 Purity: 98+% | Thermo Scientific Chemicals, Mississauga, ON, Canada |
| Parameters | Range in Open Literature | Range in This Study | References |
|---|---|---|---|
| pH | 0.34–14 | 2.9–12.5 | [27,62,63] |
| 80–17,000 | 997.3–2162.4 | [27,64,65] | |
| 128–65,000 | 2500–7100 | [63,66,67] | |
| 8–4000 | 50.2–109.5 | [27,62,64] | |
| 100–8200 | 1250–2500 | [43,59,68] | |
| 20–1000 | 200–400 | [69,70,71] | |
| 10–12,700 | 300–600 | [72,73,74] | |
| 20–200 | 85–170 | [14,75,76] | |
| 50–3000 | 75–150 | [77,78,79] | |
| 50–1000 | 400–800 | [16,60,80] |
| Parameters | Measured Value |
|---|---|
| Total pore volume (cm3/gAC) | 0.6924 |
| Micropore volume (cm3/gAC) | 0.2042 |
| Mesopore volume (cm3/gAC) | 0.4883 |
| SBET (m2/gAC) | 763.0 |
| Average pore diameter (nm)—BJH method | 3.631 |
| Average pore diameter (nm)—HK method | 3.675 |
| Particle size (mesh) | 4–12 |
| Isotherm | Parameters | Error Analysis | |||
|---|---|---|---|---|---|
| ARE | R2 (%) | ||||
| Langmuir | 47.00 | 8.69 | 47.3 | 58.8 | |
| 1.07 | |||||
| Freundlich | 2.82 | 1.04 | 98.9 | 6.65 | |
| 4.89 | |||||
| Langmuir-Freundlich | 522.3 | 5.78 | 99.3 | 4.02 | |
| 4.70 | |||||
| Dubinin-Radushkevich (D-R) | 678.5 | 1.18 | 98.8 | 7.83 | |
| 1.65 | |||||
| Fritz-Schlunder | 1.68 | 98.9 | |||
| 2.80 | |||||
| 5.01 | |||||
| −4.77 | |||||
| Brunauer-Emmett-Teller | 16.38 | 4.32 | 89.3 | 6.50 | |
| 9.54 | |||||
| b2 | |||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Asheghmoalla, M.; Mehrvar, M. Adsorption of a Multicomponent Pharmaceutical Wastewater on Charcoal-Based Activated Carbon: Equilibrium and Kinetics. Water 2024, 16, 2086. https://doi.org/10.3390/w16152086
Asheghmoalla M, Mehrvar M. Adsorption of a Multicomponent Pharmaceutical Wastewater on Charcoal-Based Activated Carbon: Equilibrium and Kinetics. Water. 2024; 16(15):2086. https://doi.org/10.3390/w16152086
Chicago/Turabian StyleAsheghmoalla, Mina, and Mehrab Mehrvar. 2024. "Adsorption of a Multicomponent Pharmaceutical Wastewater on Charcoal-Based Activated Carbon: Equilibrium and Kinetics" Water 16, no. 15: 2086. https://doi.org/10.3390/w16152086
APA StyleAsheghmoalla, M., & Mehrvar, M. (2024). Adsorption of a Multicomponent Pharmaceutical Wastewater on Charcoal-Based Activated Carbon: Equilibrium and Kinetics. Water, 16(15), 2086. https://doi.org/10.3390/w16152086








