Hydrogeophysical Evaluation of the Karst Aquifer near the Western Edge of the Ring of Cenotes, Yucatán Peninsula
Abstract
1. Introduction
1.1. Study Area
1.2. Geological and Hydrogeological Settings
2. Materials and Methods
2.1. ERT Measurements
2.2. Inverse Modeling of Pseudosections
2.3. Estimation of Effective Porosity by Archie’s Law
2.4. Hydrogeology Evaluation
3. Results and Discussion
3.1. Inverted True Resistivity 2D Sections
3.2. Effective Porosity Sections
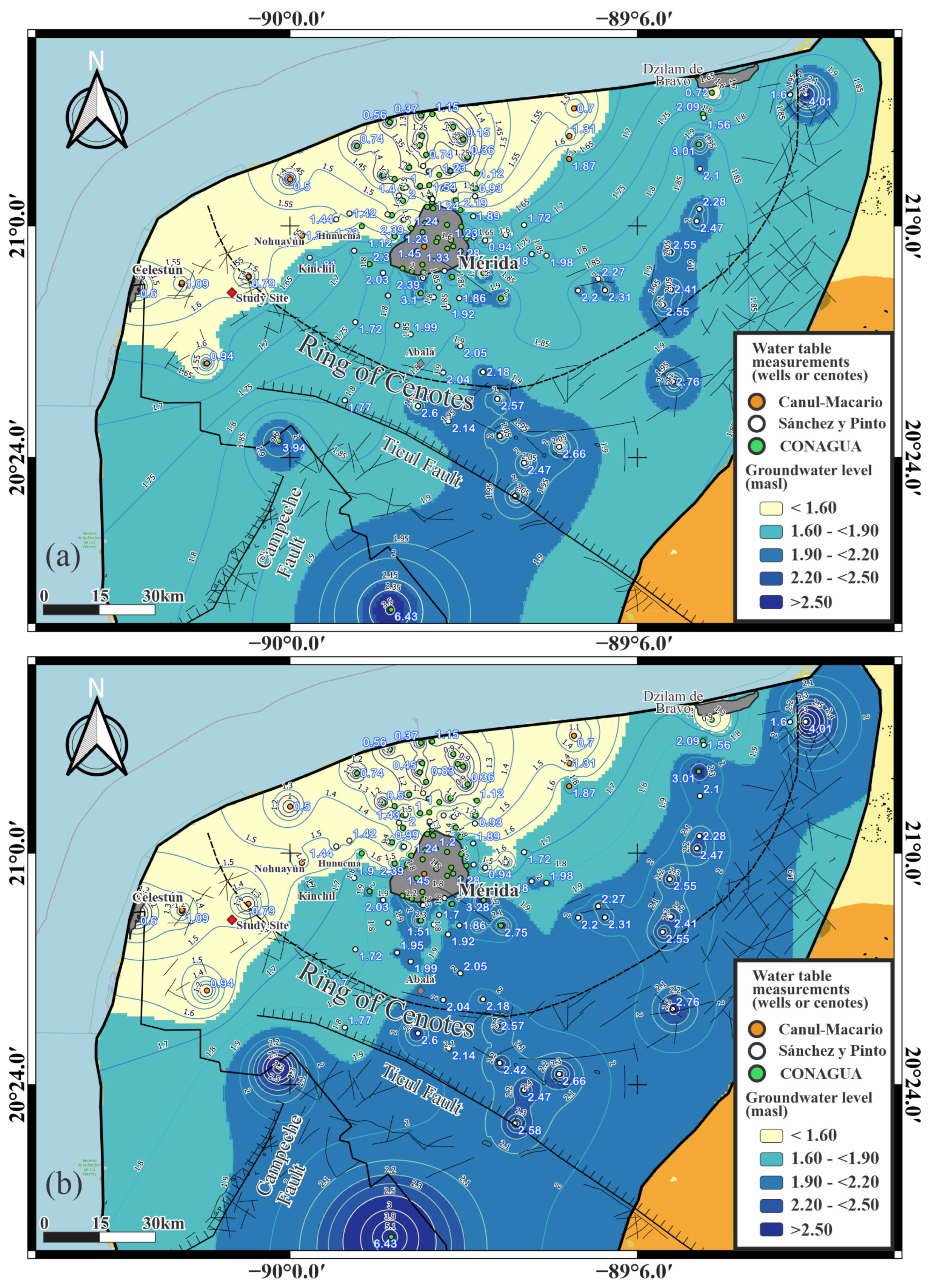
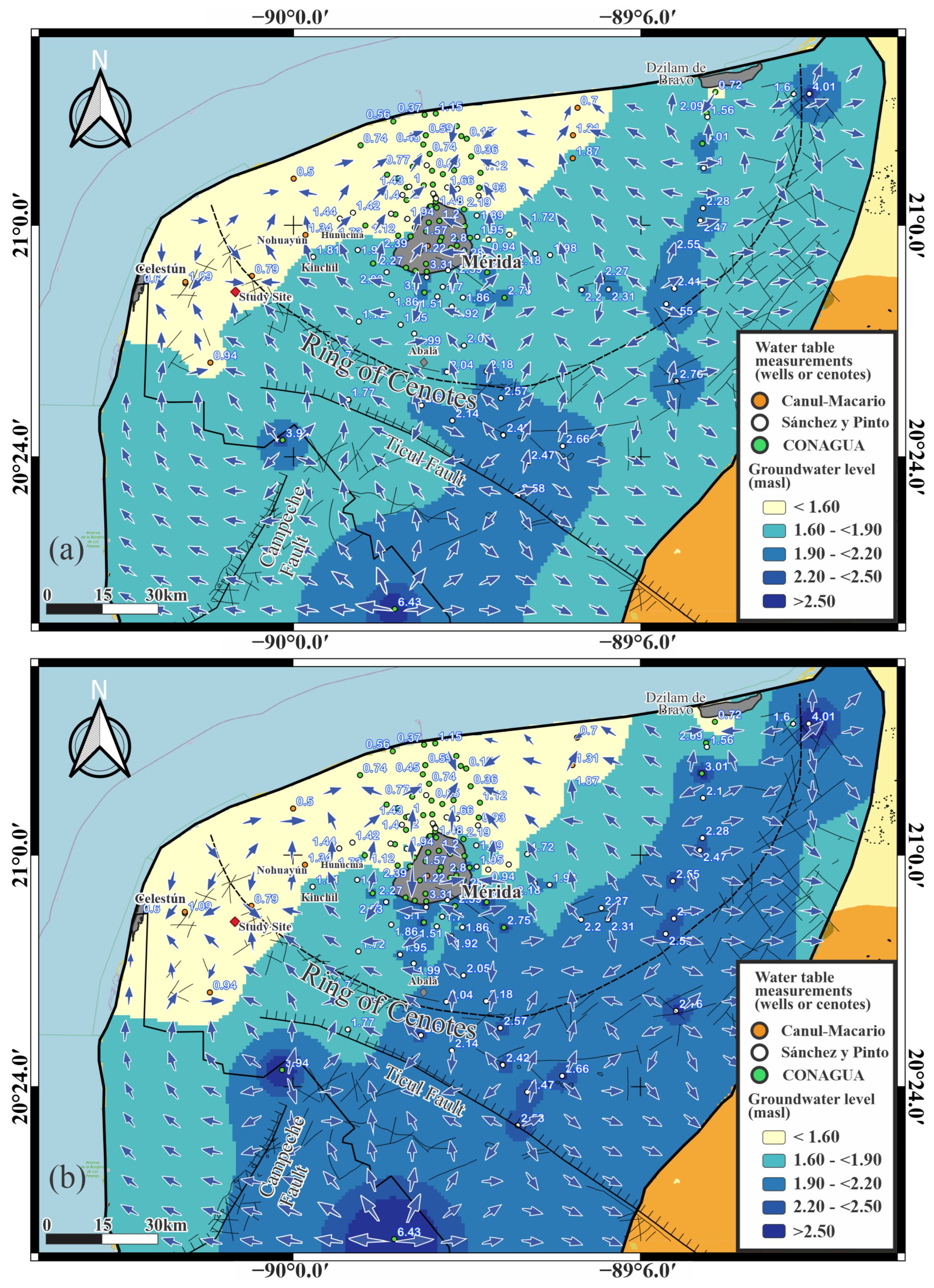
3.3. Groundwater Flows
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1. Details of the Numerical Inversion of Apparent Resistivity Sections

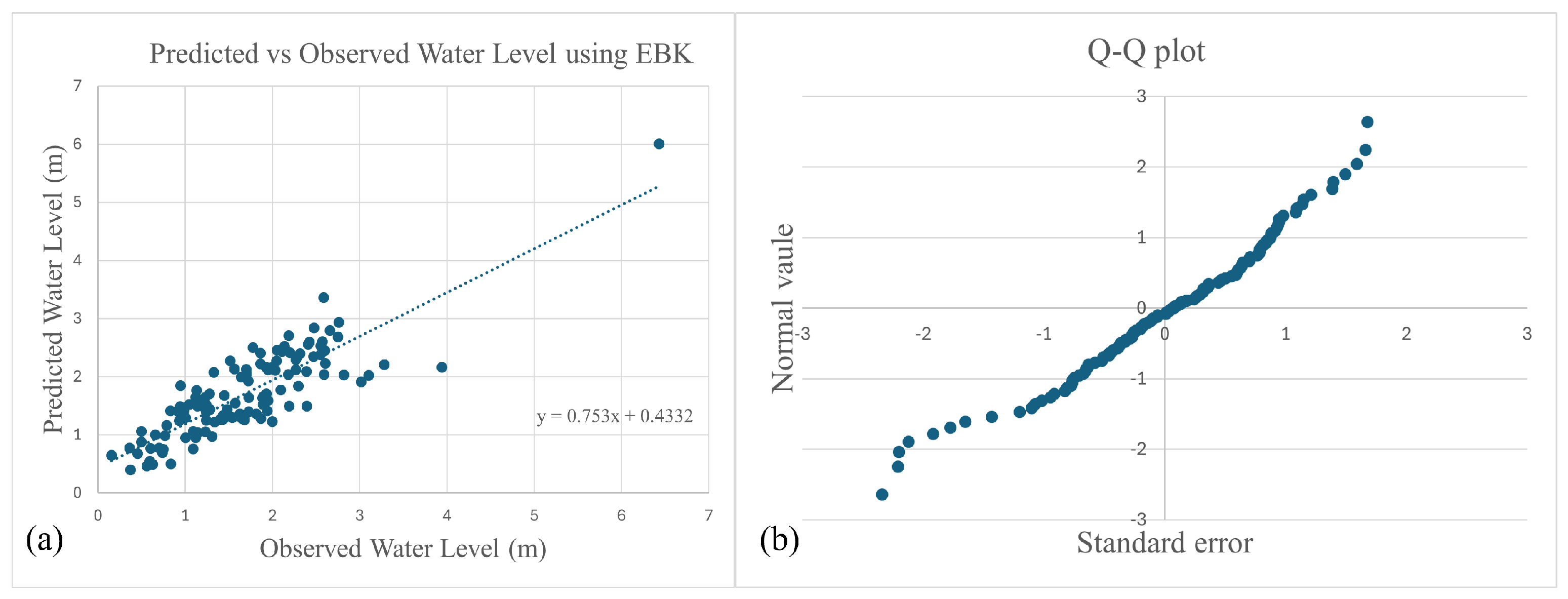
Appendix A.2. Additional Information on Interpolation Methods
| Subset size | 23 |
| Overlap factor | 1 |
| Number of simulations | 100 |
| Output surface type | Prediction |
| Transformation | Empirical |
| Semivariogram type | K-Bessel Detrended |
| Neighborhood type | Standard Circular |
| Maximum neighbors | 15 |
| Minimum neighbors | 10 |
| Sector type | 1 |
| Angles | 0 |
| Radius | 10,000 |
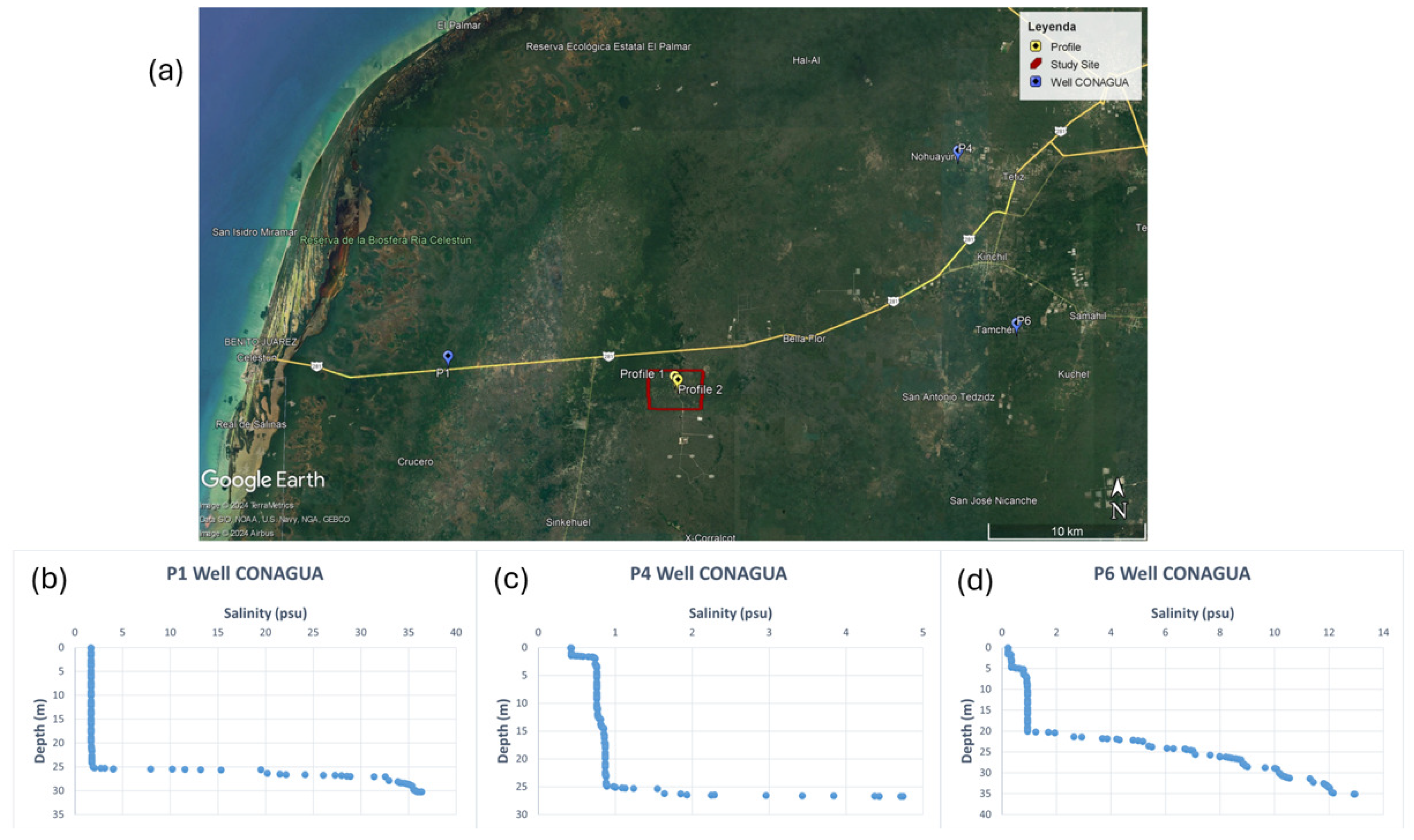
Appendix A.3. CONAGUA Monitoring Wells
References
- Torres-Díaz, M.C.; Basulto-Solís, Y.Y.; Cortés-Esquivel, J.; García-Uitz, K.; Koh-Sosa, A.; Puerto-Romero, F.; Pacheco-Ávila, J.G. Vulnerability and risk assessment of groundwater pollution in Yucatan. Ecosistemas Recur. Agropecu. 2014, 1, 189–203. [Google Scholar]
- Aguilar-Duarte, Y.; Bautista, F.; Mendoza, M.E.; Frausto, O.; Ihl, T.; Delgado, C. IVAKY: Índice de la vulnerabilidad del acuífero kárstico Yucateco a la contaminación [IVAKY: Index of the vulnerability of the Yucatecan karst aquifer to contamination]. Rev. Mex. Ing. Química 2016, 15, 913–933. [Google Scholar] [CrossRef]
- Moreno-Gómez, M.; Martínez-Salvador, C.; Moulahoum, A.W.; Liedl, R.; Stefan, C.; Pacheco, J. First Steps into and Integrated Karst Aquifer Vulnerability Approach (IKAV). Groundwater Vulnerability Analysis of the Yucatan Karst, Mexico. Water 2019, 11, 1610. [Google Scholar] [CrossRef]
- Batllori, E.; Canto, S. Vulnerabilidad intrínseca a la contaminación del acuífero kárstico en Yucatán, considerando las anomalías gravimétricas de Bouguer [Intrinsic vulnerability to pollution of the Yucatán karst aquifer, as determined by means of Bouguer gravimetric anomalies]. Boletín Soc. Geológica Mex. 2022, 74, A130921. [Google Scholar] [CrossRef]
- Bauer-Gottwein, P.; Gondwe, B.R.N.; Charvet, G.; Marin, L.E.; Rebolledo-Vieyra, M.; Merediz-Alonso, G. Review: The Yucatán Peninsula karst aquifer, Mexico. Hydrogeol. J. 2011, 19, 507–524. [Google Scholar] [CrossRef]
- Canul-Macario, C.; Salles, P.; Espriú, A.H.; Pacheco-Castro, R. Empirical relationships of groundwater head-salinity response to variations of sea level and vertical recharge in coastal confined karst aquifers. Hydrogeol. J. 2020, 28, 1679–1694. [Google Scholar] [CrossRef]
- Marin, L.E.; Steinich, B.; Pacheco, J.; Escolero, O.A. Hydrogeology of a contaminated sole-source karst aquifer, Mérida, Yucatán, México. Geofísica Int. 2000, 39, 359–365. [Google Scholar] [CrossRef]
- Pacheco, J.; Cabrera, A. Groundwater Contamination by Nitrates in the Yucatan Peninsula, Mexico. Hydrogeol. J. 2012, 5, 47–53. [Google Scholar] [CrossRef]
- Polanco-Rodríguez, A.G.; López, M.I.R.; Casillas, A.D.; León, J.A.A.; Banik, S.D. Impact of pesticides in karst groundwater. Review of recent trends in Yucatan, Mexico. Groundw. Sustain. Dev. 2018, 7, 20–29. [Google Scholar] [CrossRef]
- Arcega-Cabrera, F.; Sickman, J.O.; Fargher, L.; Herrera-Silveira, J.; Lucero, D.; Oceguera-Vargas, I.; Lamas-Cosío, E.; Robledo-Ardila, P.A. Groundwater Quality in the Yucatan Peninsula: Insights from Stable Isotope and Metal Analysis. Groundwater 2021, 59, 878–891. [Google Scholar] [CrossRef]
- Martínez-Salvador, C.; Moreno-Gómez, M.; Liedl, R. Estimating Pollutant Residence Time and NO3 Concentrations in the Yucatan Karst Aquifer; Considerations for an Integrated Karst Aquifer Vulnerability Methodology. Water 2019, 11, 1431. [Google Scholar] [CrossRef]
- Perry, E.; Velazquez-Oliman, G.; Marin, L. The Hydrogeochemistry of the Karst Aquifer System of the Northern Yucatan Peninsula, Mexico. Int. Geol. Rev. 2002, 44, 191–221. [Google Scholar] [CrossRef]
- Kresic, N.; Mikszewski, A. Hydrogeological Conceptual Site Models; Taylor & Francis Group: Abingdon, UK, 2012. [Google Scholar]
- Rubin, Y.; Hubbard, S. Stochastic Forward and Inverse Modeling: The Hydrogeophysical Challenge: In Hydrogeophysics; Water Science and Technology Library, Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar] [CrossRef]
- Binley, A.; Hubbard, S.S.; Huisman, J.A.; Revil, A.; Robinson, D.A.; Singha, K.; Slater, L.D. The emergence of hydrogeophysics for improved understanding of subsurface processes over multiple scales. Water Resour. Res. 2015, 51, 3837–3866. [Google Scholar] [CrossRef]
- Gómez-Nicolás, M.; Rebolledo-Vieyra, M.; Canto-Lugo, E.; Huerta-Quintanilla, R.; Ochoa-Sandoval, P. Connectivity in a karst system using electrical resistivity tomography and network theory. Groundwater 2017, 56, 732–741. [Google Scholar] [CrossRef]
- Andrade-Gómez, L.; Rebolledo-Vieyra, M.; Andrade, J.L.; López, P.Z.; Estrada-Contreras, J. Karstic aquifer structure from geoelectrical modeling in the Ring of Sinkholes, Mexico. Hydrogeol. J. 2019, 27, 2365–2376. [Google Scholar] [CrossRef]
- Zamora-Luria, J.C.; Perera-Burgos, J.A.; González-Calderon, A.; Marin-Stillman, L.E.; Leal-Bautista, R.M. Control of fracture networks on a coastal karstic aquifer: A case study from northeastern Yucatán Peninsula (Mexico). Hydrogeol. J. 2020, 28, 2765–2777. [Google Scholar] [CrossRef]
- Villela-y-Mendoza, A.; Perez-Flores, M.A.; Ochoa-Tinajero, L.E.; Vargas-Huitzil, E. Applying resistivity (dipole-dipole, Schlumberger, and Wenner) joint inversion to detect endokarst features in Quintana Roo, México. J. S. Am. Earth Sci. 2021, 106, 103041. [Google Scholar] [CrossRef]
- Polanco-Rodríguez, A.G.; Navarro-Alberto, J.A.; Solorio-Sánchez, J.; Mena-Rejón, G.J.; Marrufo-Gómez, J.; Del Valls-Casillas, T.A. Contamination by organochlorine pesticides in the aquifer of the Ring of Cenotes in Yucatán, México. Water Environ. J. 2014, 29, 140–150. [Google Scholar] [CrossRef]
- Derriene, M.; Arcega-Cabrera, F.; Velazquez-Tavera, N.L.; Kantún-Manzano, C.A.; Cappela-Vizcaino, S. Sources and distribution of organic matter along the Ring of Cenotes, Yucatan, Mexico: Sterol markers and statistical approaches. Sci. Total Environ. 2015, 511, 223–229. [Google Scholar] [CrossRef]
- Hildebrand, A.R.; Pilkington, M.; Connors, M.; Ortiz-Aleman, C.; Chavez, R.E. Size and structure of the Chicxulub crater revealed by horizontal gravity gradients and cenotes. Nature 1995, 376, 415–417. [Google Scholar] [CrossRef]
- Urrutia-Fucugauchi, J.; Camargo-Zanoguera, A.; Pérez-Cruz, L.; Pérez-Cruz, G. The Chicxulub multi-ring impact crater, Yucatán Carbonate platform, Gulf of Mexico. Geofís. Int. 2011, 50, 99–127. [Google Scholar] [CrossRef]
- Butterlin, J. Reconocimiento geológico preliminar del territorio de Quintana Roo [Preliminary geological reconnaissance of the territory of Quintana Roo]. BoletÍn Asoc. Mex. Geólogos Pet. 1958, 10, 531–564. [Google Scholar]
- Ward, W.C.; Keller, G.; Stinnesbeck, W.; Adatte, T. Yucatán subsurface stratigraphy: Implications and constraints for the Chicxulub impact. Geology 1995, 23, 873–876. [Google Scholar] [CrossRef]
- García-Gil, G.; Graniel-Castro, E. Geología. Biodivers. Desarro. Hum. Yucatán 2000, 10, 531–564. [Google Scholar]
- Perry, E.C.; Swift, J.; Gamboa, J.; Reeve, A.; Sanbon, R.; Marín, L.E.; Villasuso, M. Geological and environmental aspects of surface cementation, north coast, Yucatan, Mexico. Geology 1989, 17. [Google Scholar] [CrossRef]
- Batllori-Sampedro, E.; González-Piedra, J.I.; Diáz-Sosa, J.; Febles-Patrón, J.L. Caracterización hidrológica de la región costera noroccidental del estado de Yucatán, México [Hydrological characterization of the northwestern coastal region of the state of Yucatán, México]. Investig. Geogr. 2006, 59, 74–92. [Google Scholar]
- Perry, E.; Marin, L.; McClain, J.; Velazquez, G. Ring of Cenotes (sinkholes), northwest Yucatan, Mexico: Its hydrogeologic characteristics and possible association with the Chicxulub impact crater. Geology 1995, 23, 17–20. [Google Scholar] [CrossRef]
- Steinich, B.; Marin, L.E. Determination of flow characteristics in the aquifer of the Northwestern Peninsula of Yucatan, Mexico. J. Hydrol. 1997, 191, 315–331. [Google Scholar] [CrossRef]
- Hanshaw, B.B.; Black, W. Chemical mass-wasting of the northern Yucatan Peninsula by groundwater dissolution. Geology 1980, 8, 222–224. [Google Scholar] [CrossRef]
- Pope, K.O.; Ocampo, A.C.; Duller, C.E. Surficial geology of the Chicxulub impact crater, Yucatan, Mexico. Earth Moon Planets 1993, 63, 93–104. [Google Scholar] [CrossRef]
- Pérez-Ceballos, R.; Pacheco-Ávila, J.; Euán-Avila, J.I.; Hernández-Arana, H. Regionalization Based on Water Chemistry and Physicochemical Traits in the Ring of Cenotes, Yucatan, Mexico. J. Cave Karst Stud. 2012, 74, 90–102. [Google Scholar] [CrossRef]
- Pérez-Ceballos, R.; Canul-Macario, C.; Pacheco-Castro, R.; Pacheco-Avila, J.; Euán-Ávila, J.; Merino-Ibarra, M. Regional Hydrogeochemical Evolution of Groundwater in the Ring of Cenotes, Yucatán (Mexico): An Inverse Modelling Approach. Water 2021, 13, 614. [Google Scholar] [CrossRef]
- Marin, L.E. Field Investigations and Numerical Simulation of Groundwater Flow in the Karstic Aquifer of Northwestern Yucatan, Mexico. Ph.D. Thesis, Northern Illinois University, DeKalb, IL, USA, 1990. [Google Scholar]
- Marin, L.E.; Perry, E.C. The hydrogeology and contamination potential of northwestern Yucatán, Mexico. Geofís. Int. 1994, 33, 619–623. [Google Scholar] [CrossRef]
- Escolero, O.A.; Marin, L.E.; Steinich, B.; Pacheco, J. Delimitation of a hydrogeological reserve for a city within a karstic aquifer: The Merida, Yucatan example. Landsc. Urban Plan. 2000, 51, 53–62. [Google Scholar] [CrossRef]
- González-Herrera, R.; Sánchez-y-Pinto, I.; Gamboa-Vargas, J. Groundwater-flow modeling in the Yucatan karstic aquifer, Mexico. Hydrogeol. J. 2002, 10, 539–552. [Google Scholar] [CrossRef]
- Escobar-Sanchez, J.E.; Urrutia-Fuccugauchi, J. Chicxulub crater post-impact hydrothermal activity—Evidence from Paleocene carbonates in the Santa Elena boreholes. Geofís. Int. 2010, 49, 97–106. [Google Scholar] [CrossRef]
- Simpson, S.L.; Osinski, G.R.; Longstaffe, F.J.; Schmieder, M.; Kring, D.A. Hydrothermal alteration associated with the Chicxulub impact crater upper peak-ring breccias. Earth Planet. Sci. Lett. 2020, 547. [Google Scholar] [CrossRef]
- Monroy-Ríos, E. Advancements in Our Understanding of the Yucatán Platform: Sedimentary Geology and Geochemestry, Speleogenesis, Chicxulub Ring of Cenotes, and Tectonic Stability. Ph.D. Thesis, Northwestern University, Evanston, IL, USA, 2020. [Google Scholar]
- Reynolds, J.M. An Introduction to Applied and Environment Geophysics, 2nd ed.; Wiley-Blackwell: Hoboken, NJ, USA, 2011. [Google Scholar]
- Advances Geosciences Inc. The SuperSting with Swift Automatic Resistivity and Ip System —Instructor Manual; Advances Geosciences: Austin, TX, USA, 2006. [Google Scholar]
- Narayan, S.; Dusseault, M.B.; Nobes, D.C. Inversion techniques applied to resistivity inverse problems. Inverse Probl. 1994, 10, 669–686. [Google Scholar] [CrossRef]
- Sharma, S.; Verma, G.K. Inversion of Electrical Resistivity Data: A Review. Int. J. Environ. Ecol. Geol. Geophys. Eng. 2015, 9, 400–406. [Google Scholar] [CrossRef]
- Aster, R.C.; Borchers, B.; Thurber, C.H. Parameter Estimation and Inverse Problems; Elsevier Academic Press: Amsterdam, The Netherlands, 2005. [Google Scholar]
- Advances Geosciences Inc. Instruction Manual for EarthImager 2D Version 2.4.2—Resistivity and IP Inversion Software; Advances Geosciences: Austin, TX, USA, 2014. [Google Scholar]
- Cejudo, E.; Ortega-Almazán, P.J.; Ortega-Camacho, D.; Acosta-González, G. Hydrochemistry and water isotopes of a deep sinkhole in north Quintana Roo, Mexico. J. S. Am. Earth Sci. 2022, 116, 103846. [Google Scholar] [CrossRef]
- Verwer, K.; Eberli, G.P.; Weger, R.J. Effect of pore structure on electrical resistivity in carbonates. AAPG Bull. 2011, 95, 175–190. [Google Scholar] [CrossRef]
- Archie, G.E. The Electrical Resistivity Log as an Aid in Determining Some Reservoir Characteristics. Trans. AIME. Soc. Pet. Eng. 1942, 146, 54–67. [Google Scholar] [CrossRef]
- Sen, P.N.; Kenyon, W.E.; Takezaki, H.; Petricola, M.J. Formation factor of carbonate rocks with microporosity: Model calculations. J. Pet. Sci. Eng. 1997, 17, 345–352. [Google Scholar] [CrossRef]
- Azar, J.H.; Javaherian, A.; Pishvaie, M.R.; Nabi-Bidhendi, M. An approach to defining tortuosity and cementation factor in carbonate reservoir rocks. J. Pet. Sci. Eng. 2008, 60, 125–131. [Google Scholar] [CrossRef]
- Brunet, P.; Clément, R.; Bouvier, C. Monitoring soil water content and deficit using Electrical Resistivity Tomography (ERT)—A case study in the Cevennes area, France. J. Hydrol. 2010, 380, 146–153. [Google Scholar] [CrossRef]
- Niwas, S.; Celik, M. Equation estimation of porosity and hydraulic conductivity of Ruhrtal aquifer in Germany using near surface geophysics. J. Appl. Geophys. 2012, 84, 77–85. [Google Scholar] [CrossRef]
- Whitman, D.; Yeboah-Forson, A. Electrical resistivity and porosity structure of the upper Biscayne Aquifer in Miami-Dade County, Florida. J. Hydrol. 2015, 531, 781–791. [Google Scholar] [CrossRef]
- Kazakis, N.; Vargemezis, G.; Voudouris, K.S. Estimation of hydraulic parameters in a complex porous aquifer system using geoelectrical methods. Sci. Total Environ. 2016, 550, 742–750. [Google Scholar] [CrossRef]
- Trejo-Corzo, J.A. Modelación geoestadística de la porosidad y la conductividad hidráulica de un acuífero kárstico costero [Geostatistical modeling of porosity and hydraulic conductivity in a coastal karst aquifer]. Master’s Thesis, Unidad de Ciencias del Agua—Centro de Investigación Científica de Yucatán, Quintana Roo, Mexico, 2022. [Google Scholar]
- Shahmohammadi-Kalalagh, S.; Taran, F. Evaluation of the classical statistical, deterministic and geostatistical interpolation methods for estimating the groundwater level. Int. J. Energy Water Resour. 2021, 5, 33–42. [Google Scholar] [CrossRef]
- Li, Y.; Hernandez, J.H.; Aviles, M.; Knappett, P.S.; Giardino, J.R.; Miranda, R.; Puy, M.J.; Padilla, F.; Morales, J. Empirical Bayesian Kriging method to evaluate inter-annual water-table evolution in the Cuenca Alta del Río Laja aquífer, Guanajuato, México. J. Hydrol. 2020, 582, 124517. [Google Scholar] [CrossRef]
- Krivoruchko, K.; Gribov, A. Evaluation of empirical Bayesian kriging. Spat. Stat. 2019, 32, 100368. [Google Scholar] [CrossRef]
- Fetter, C.W. Applied Hydrogeology, 4 ed.; Prentice Hall: Upper Saddle River, NJ, USA, 2001. [Google Scholar]
- Estrada-Medina, H.; Jiménez-Osornio, J.J.; Álvarez-Rivera, O.; Barrientos-Medina, R.C. The karst of Yucatan: Its origin, morphology and biology. Acta Univ. 2019, 29. [Google Scholar] [CrossRef]
- Kong, J.; Shen, C.J.; Xin, P.; Song, Z.; Li, L.; Barry, D.A.; Jeng, D.S.; Stagnitti, F.; Lockington, D.A.; Parlange, J.Y. Capillary effect on water table fluctuations in unconfined aquifers. Water Resour. Res. 2012, 49, 3064–3069. [Google Scholar] [CrossRef]
- Cejudo, E.; Acosta-González, G.; Ortega-Camacho, D.; Perera-Burgos, J.A.; Leal-Bautista, R.M. Caracterización hidroquímica y geofísica de una descarga de agua subterránea en Quintana Roo, México [Hydrochemical and geophysical characterization of a groundwater discharge in Quintana Roo, Mexico]. Ecosistemas Recur. Agropecu. 2022, 9, 1–13. [Google Scholar] [CrossRef]
- CONAGUA. Medición Piezométrica en el Acuífero Costero (litoral Poniente) de la Península de Yucatán, Estado de Yucatán [Piezometric Measurement in the Coastal Aquifer (Western Coast) of the Yucatán Peninsula, State of Yucatán]. 2002. Available online: https://sigagis.conagua.gob.mx/rp20/ (accessed on 13 July 2024).
- Lucia, F.J. Carbonate Reservoir Characterization—An Integrated Approach, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Zhang, S.; Lu, P.; Cantrell, D.; Zaretskiy, Y.; Jobe, D.; Agar, S.M. Improved quantification of the porosity-permeability relationship of limestones using petrographical texture. Pet. Geosci. 2018, 24, 440–448. [Google Scholar] [CrossRef]
- Legrande, H.E.; Stringfield, V.T. Development and Distribution of Permeability in Carbonate Aquifers. Water Resour. Res. 1971, 7, 1284–1294. [Google Scholar] [CrossRef]
- Buckley, D.K.; Macdonald, D.; Villasuso, P.M.; Graniel, C.E.; Vázquez, M.J.; Virgilio, J.M. Geophysical logging of a karstic limestone aquifer for hydrogeological purposes at Merida, Yucatan, Mexico. Br. Geol. Survey. Tech. Rep. WD 1994, 94, 194/4C. [Google Scholar]
- Canul-Macario, C.; Salles, P.; Hernández-Espriú, J.A.; González-Herrera, R. Simulación numérica de flujo y transporte de solutos de la porción noroeste del acuífero cárstico Península de Yucatán [Numerical simulation of flow and solute transport of the northwestern portion of the Yucatán Peninsula karstic aquifer]. Química Calid. Contam. Agua 2018, 1, 53–60. [Google Scholar]
- Sánchez y Pinto, I.A. Modelo Numérico del Flujo Subterráneo de la Porción Acuífera N-NW del Estado de Yucatán: Implicaciones Hidrogeológicas [Numerical Model of the Groundwater Flow of the N-NW Aquifer Portion of the State of Yucatán: Hydrogeological Implications]. Master’s Thesis, Universidad Autonoma de Chihuahua, Chihuahua, Mexico, 1996. [Google Scholar]
- Costall, A.R.; Harris, B.D.; Teo, B.; Schaa, R.; Wagner, F.M.; Pigois, J.P. Groundwater Throughflow and Seawater Intrusion in High Quality Coastal Aquifers. Sci. Rep. 2020, 10, 9866. [Google Scholar] [CrossRef]
- Back, W.; Hanshaw, B. Hydrogeochemistry of the Northern of the Yucatan Peninsula, Mexico with a Section on Mayan Water Practices. In Field Seminar on Water and Carbonate Rocks of the Yucatan Peninsula, Mexico; Weidie, A.E., Ed.; New Orleans Geological Society: New Orleans, LA, USA, 1974; pp. 53–54. [Google Scholar]
- Bear, J. Dynamics of Fluids in Porous Media; Dover Publications, Inc.: Garden City, NY, USA, 1988. [Google Scholar]
- Rousseau-Gueutin, P.; Love, A.J.; Vasseur, G.; Robinson, N.I.; Simmons, C.T.; de Marsily, G. Time to reach near-steady state in large aquifers. Water Resour. Res. 2013, 49, 6893–6908. [Google Scholar] [CrossRef]
- Escolero, O.; Marin, L.E.; Domínguez-Mariani, E.; Torres-Onofre, S. Dynamic of the freshwater-saltwater interface in a karstic aquifer under extraordinary recharge action: The Merida Yucatan case study. Environ. Geol. 2007, 51, 719–723. [Google Scholar] [CrossRef]
- Graniel-Castro, E.; Yam-Caamal, J. Efectos del Huracán Wilma al acuífero de la Península de Yucatán, México [Effects of Hurricane Wilma on the aquifer of the Yucatán Peninsula]. Tecnología Ciencias Agua 2014, 5, 141–147. [Google Scholar]
- Kovacs, S.E.; Reinhardt, E.G.; Stastna, M.; Coutino, A.; Werner, C.; Collins, S.V.; Devos, F.; Maillot, C.L. Hurricane Ingrid and Tropical Storm Hanna’s effects on the salinity of the coastal aquifer, Quintana Roo, Mexico. J. Hydrol. 2017, 551, 703–704. [Google Scholar] [CrossRef]
- Vera, I.; no Tapia, I.M.; Enriquez, C. Effects of drought and subtidal sea-level variability on salt intrusion in a coastal karst aquifer. Mar. Freshw. Res. 2012, 63, 485–493. [Google Scholar] [CrossRef]
- Escobedo-Cen, I.D. Modelación Regional en Estado Estacionario del Acuífero Noreste de Quintana Roo [Regional Modeling in Steady State of the Northeast Aquifer of Quintana Roo]. Master’s Thesis, Unidad de Ciencias del Agua—Centro de Investigación Científica de Yucatán, Quintana Roo, Mexico, 2021. [Google Scholar]
- Rotzoll, K.; Fletcher, C.H. Assessment of groundwater inundation as a consequence of sea-level rise. Nat. Clim. Chang. 2013, 3, 477–481. [Google Scholar] [CrossRef]
- Shrestha, S.; Neupane, S.; Mohanasundaram, S.; Pandey, V.P. Mapping groundwater resiliency under climate change scenarios: A case study of Kathmandu Valley, Nepal. Environ. Res. 2020, 183, 109149. [Google Scholar] [CrossRef]
- Steinich, B.; Olimán, G.V.; Marín, L.E.; Perry, E. Determination of the groundwater divide in the karst aquifer of Yucatán, México, combining geochemical and hydrogeological data. Geofís. Int. 1996, 35, 153–159. [Google Scholar] [CrossRef]
- Töth, J. A Theoretical Analysis of Groundwater Flow in Small Drainage Basins. J. Geophys. Res. 1963, 68, 4795–4812. [Google Scholar] [CrossRef]
- Freeze, R.A.; Witherspoon, P.A. Theoretical Analysis of Regional Groundwater Flow. 2. Effect of Water-Table Configuration and Subsurface Permeability Variation. Water Resour. Res. 1967, 3, 623–634. [Google Scholar] [CrossRef]
- Back, W.; Hanshaw, B.B. Comparison of Chemical Hydrogeology of the Carbonate Peninsulas of Florida and Yucatan. J. Hydrol. 1970, 10, 330–368. [Google Scholar] [CrossRef]
- Jouves, J.; Viseur, S.; Arfib, B.; Baudement, C.; Camus, H.; Collon, P.; Guglielmi, Y. Speleogenesis, geometry, and topology of caves: A quantitative study of 3D karst conduits. Geomorphology 2017, 298, 86–106. [Google Scholar] [CrossRef]
- Kovács, A. Quantitative classification of carbonate aquifers based on hydrodynamic behaviour. Hydrogeol. J. 2021, 29, 33–52. [Google Scholar] [CrossRef]
- Batllori-Sampedro, E.A.; Canto-Mendiburu, S.N. Relationship between the Map of Bouguer Anomalies and the Geohydrological Characteristics of the Karst Aquifer of Yucatán. A Review on the Water Vulnerability. J. Earth Environ. Sci. 2024, 3. [Google Scholar] [CrossRef]


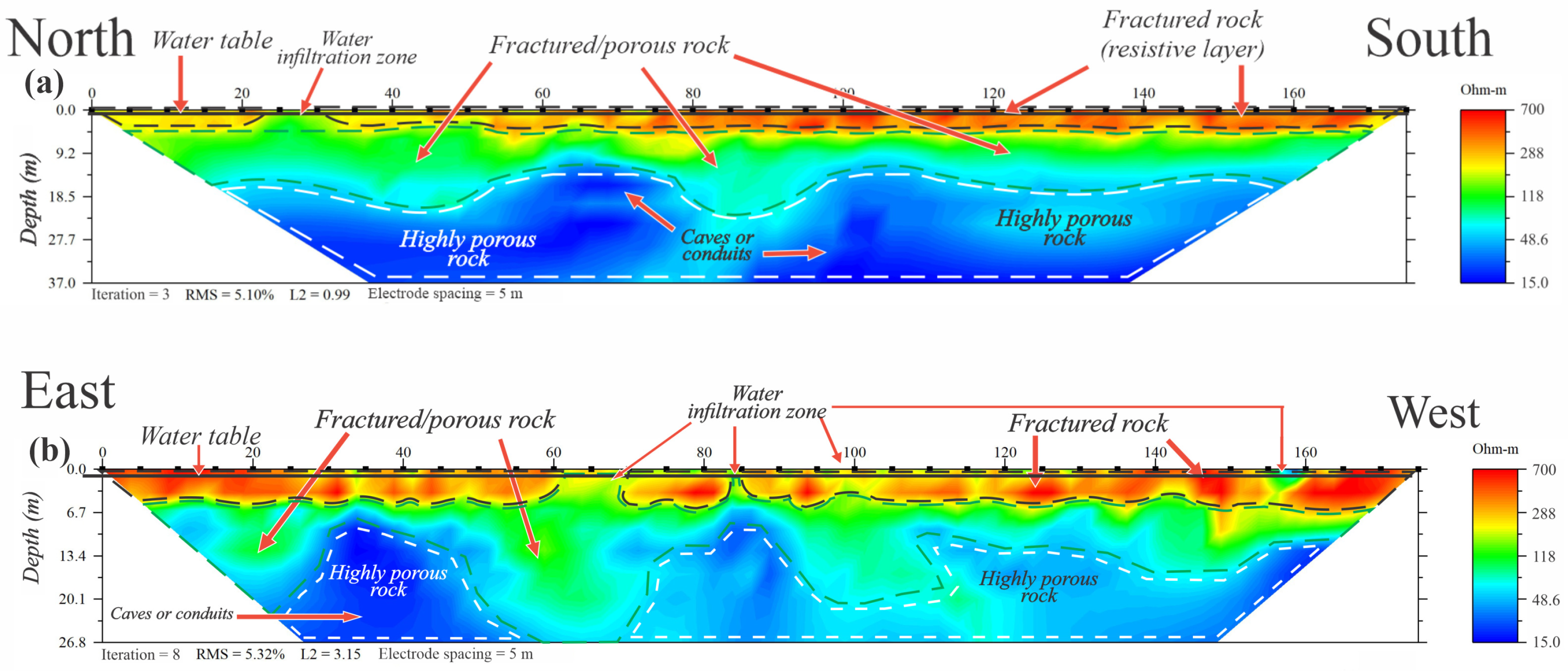
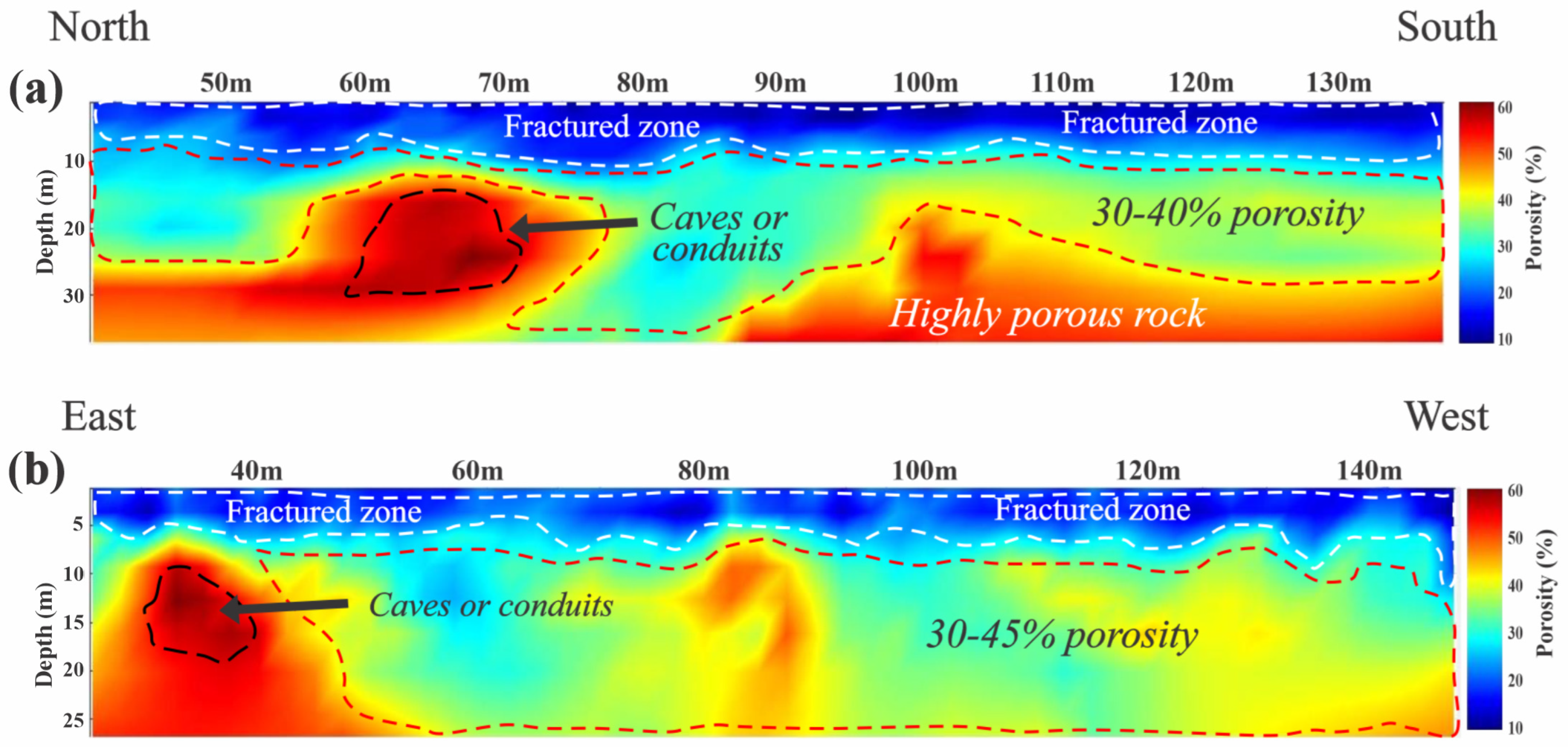

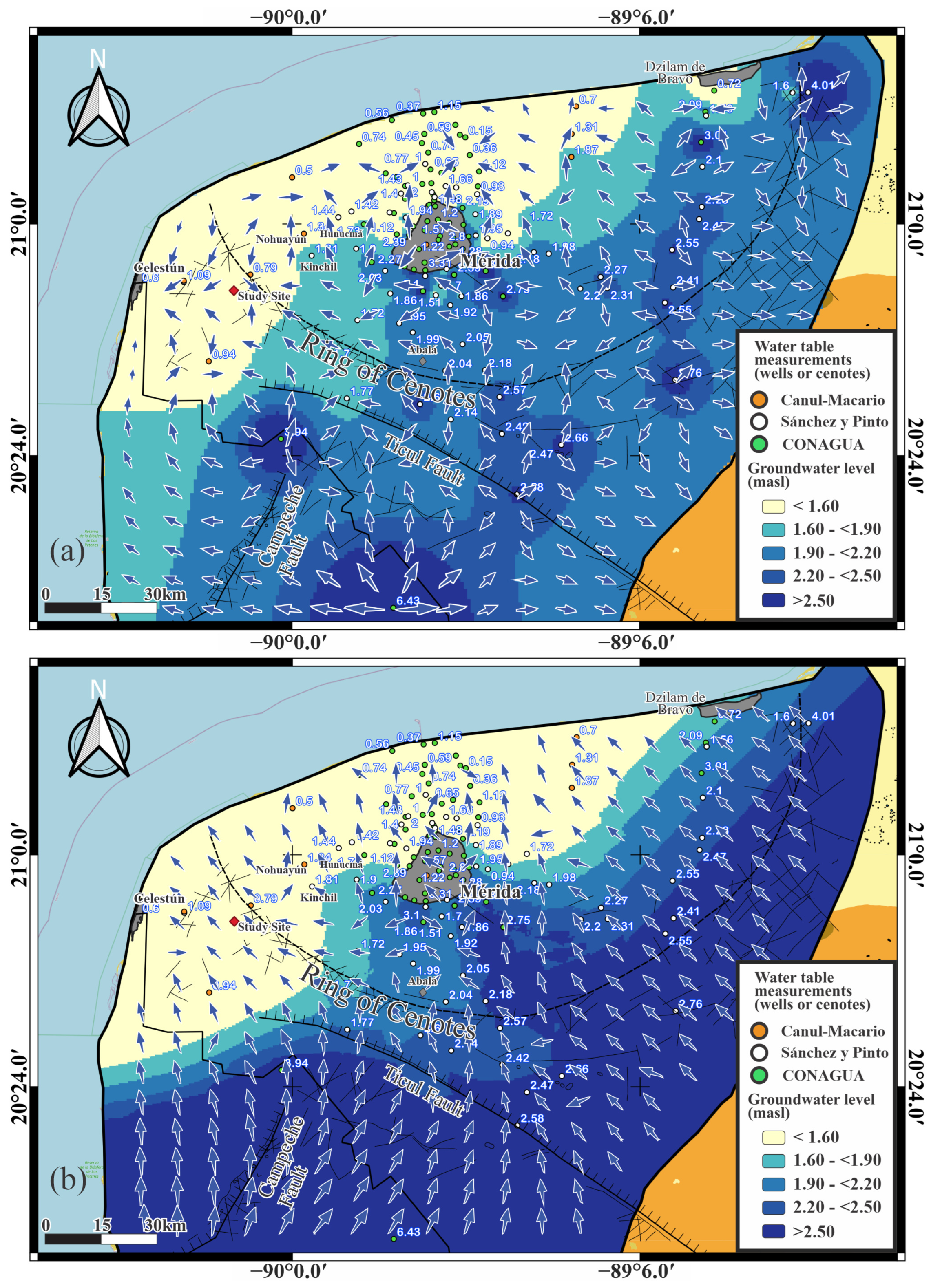
| Rock Type | Resistivity Values (m) |
|---|---|
| Unsaturated limestone | ≥1000 |
| Fractured limestone saturated with freshwater | 20–1000 |
| Fractured limestone saturated with brackish water | 10–20 |
| Fractured limestone saturated with saltwater | ≤10 |
| Caverns or conduits saturated with freshwater | ≥5 |
| Caverns or conduits saturated with saltwater | ≤5 |
| Profile | Iteration | RMS | L2 |
|---|---|---|---|
| T1 DD | 4 | 5.09% | 0.66 |
| T1 W | 2 | 1.91% | 0.41 |
| T1 DD-W | 3 | 5.10% | 0.99 |
| T2 DD | 8 | 5.23% | 3.15 |
| T2 W | 3 | 2.17% | 0.52 |
| T2 DD-W | 8 | 5.32% | 3.15 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Perera-Burgos, J.A.; Alvarado-Izarraras, L.G.; Mixteco-Sánchez, J.C.; Canul-Macario, C.; Acosta-González, G.; González-Calderón, A.; Hernández-Anguiano, J.H.; Li, Y. Hydrogeophysical Evaluation of the Karst Aquifer near the Western Edge of the Ring of Cenotes, Yucatán Peninsula. Water 2024, 16, 2021. https://doi.org/10.3390/w16142021
Perera-Burgos JA, Alvarado-Izarraras LG, Mixteco-Sánchez JC, Canul-Macario C, Acosta-González G, González-Calderón A, Hernández-Anguiano JH, Li Y. Hydrogeophysical Evaluation of the Karst Aquifer near the Western Edge of the Ring of Cenotes, Yucatán Peninsula. Water. 2024; 16(14):2021. https://doi.org/10.3390/w16142021
Chicago/Turabian StylePerera-Burgos, Jorge Adrián, Luis Gerardo Alvarado-Izarraras, Juan Carlos Mixteco-Sánchez, César Canul-Macario, Gilberto Acosta-González, Alfredo González-Calderón, Jesús Horacio Hernández-Anguiano, and Yanmei Li. 2024. "Hydrogeophysical Evaluation of the Karst Aquifer near the Western Edge of the Ring of Cenotes, Yucatán Peninsula" Water 16, no. 14: 2021. https://doi.org/10.3390/w16142021
APA StylePerera-Burgos, J. A., Alvarado-Izarraras, L. G., Mixteco-Sánchez, J. C., Canul-Macario, C., Acosta-González, G., González-Calderón, A., Hernández-Anguiano, J. H., & Li, Y. (2024). Hydrogeophysical Evaluation of the Karst Aquifer near the Western Edge of the Ring of Cenotes, Yucatán Peninsula. Water, 16(14), 2021. https://doi.org/10.3390/w16142021










