Risk-Based Inspection and Rehabilitation Planning of Service Connections in Intermittent Water Supply Systems for Leakage Management in Arid Regions
Abstract
:1. Introduction
2. Materials and Methods
2.1. Study Area and Baseline Data
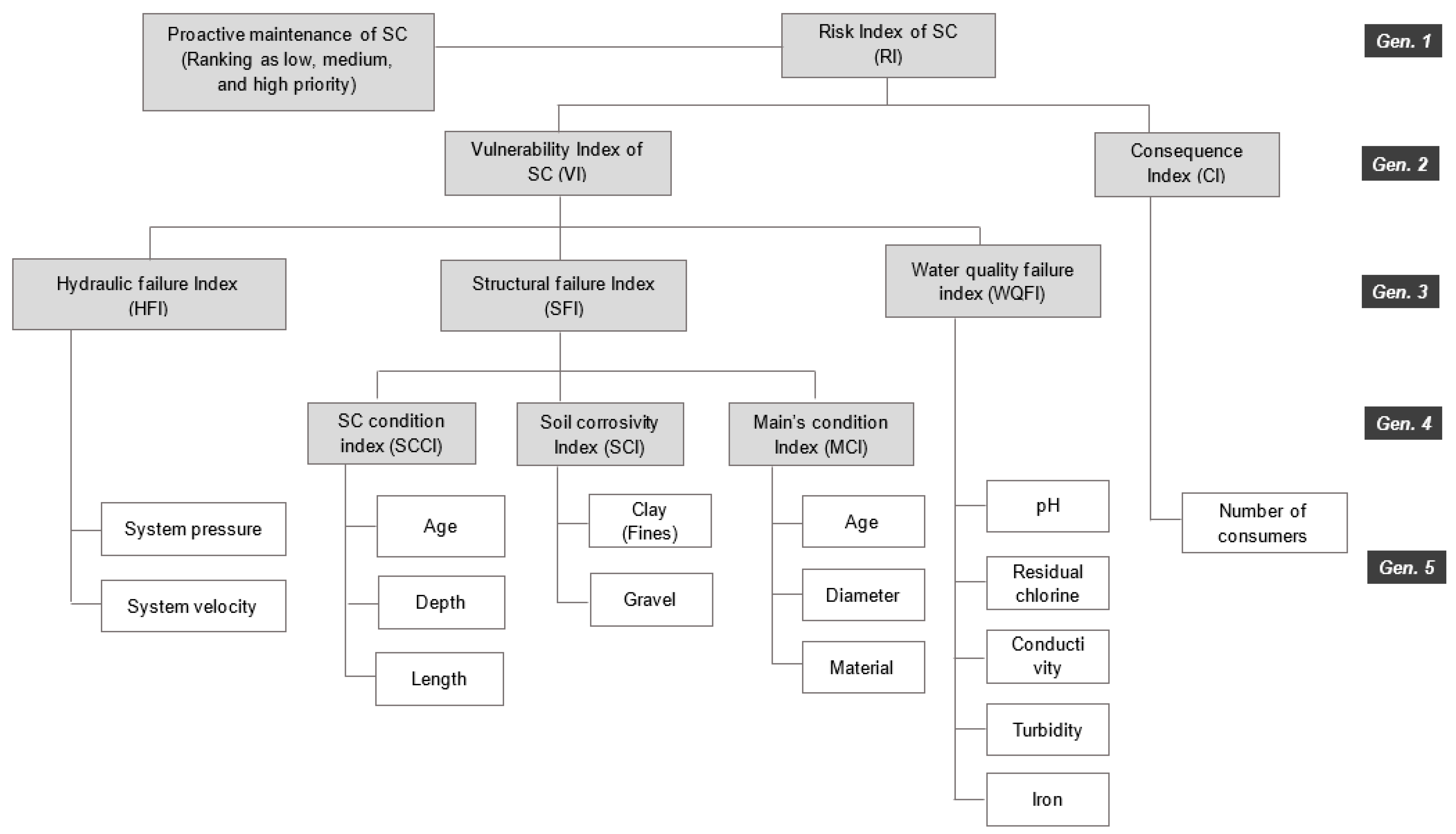
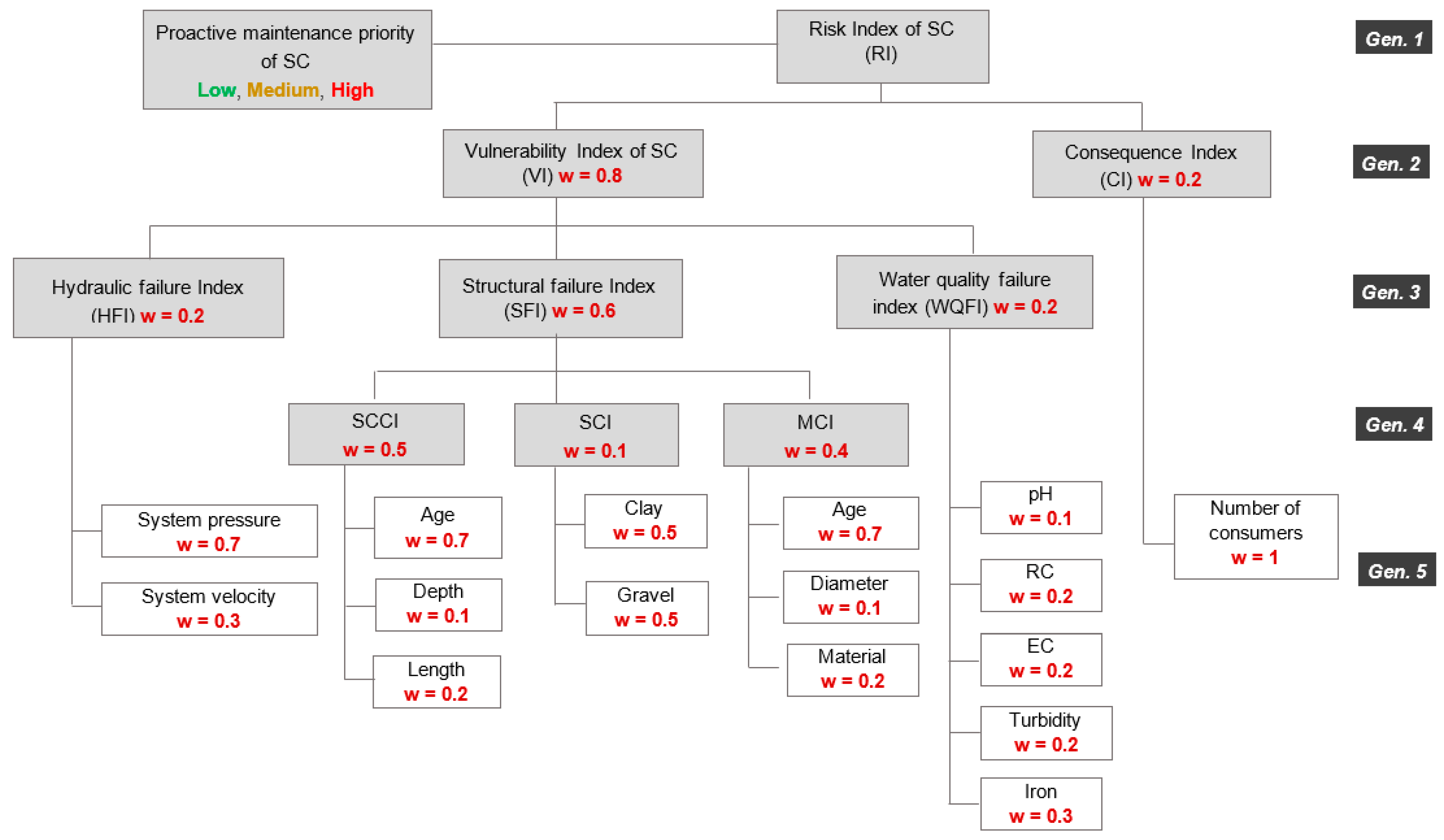
2.2. Risk-Based Inspection and Rehabilitation Methodology
2.3. Vulnerability of Service Connections
2.3.1. Hydraulic Failure
2.3.2. Structural Failure
2.3.3. Water Quality Failure
2.4. Consequence
2.5. Risk Index
2.6. Weighting and Aggregation Process
2.6.1. Fuzzy Analytic Hierarchy Process
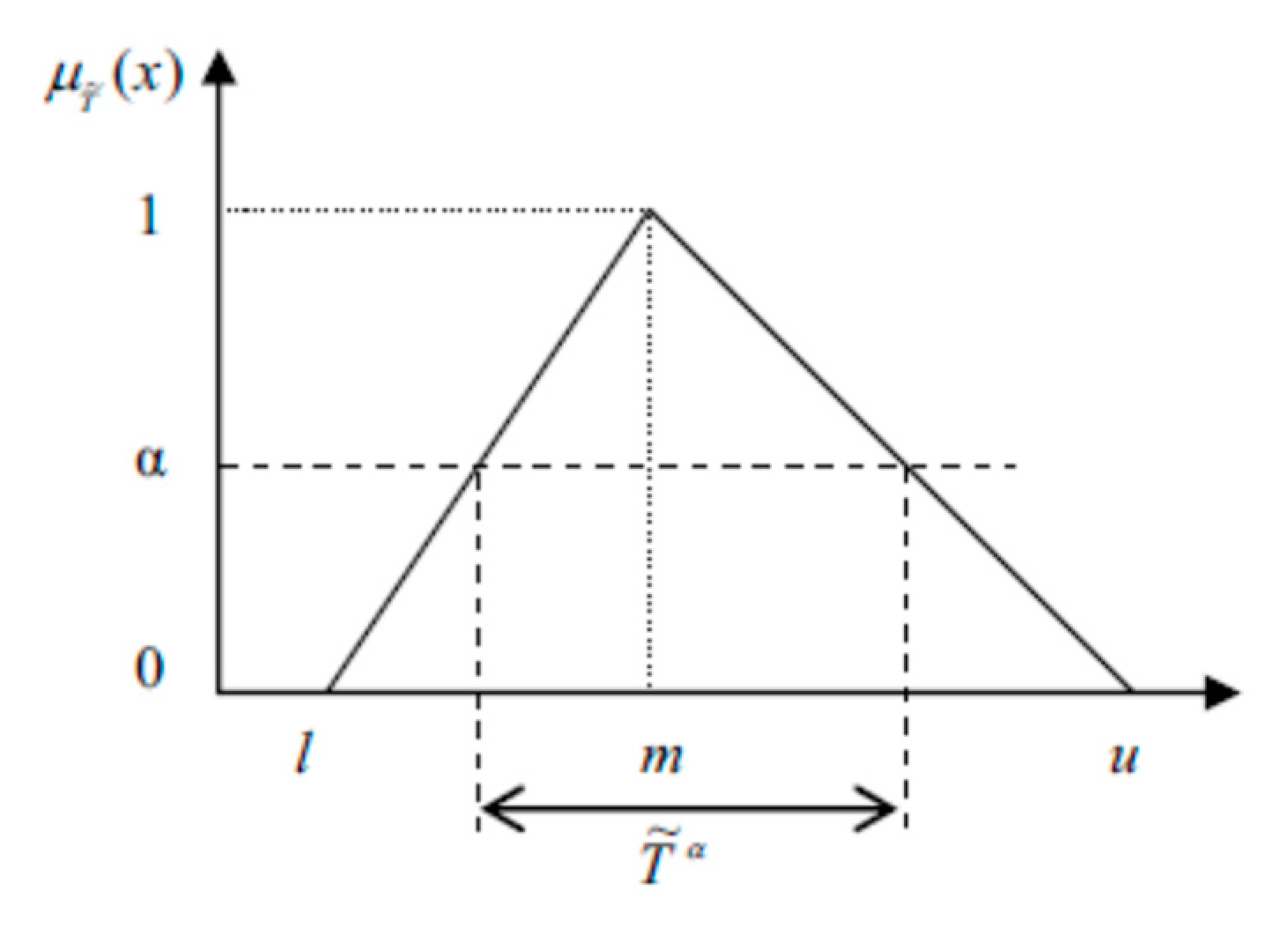
- Step 1:
- Pairwise comparison matrix.
- Step 2:
- Consistency check.
- Step 3:
- Fuzzy weights estimation.
- Step 4:
- Combined judgment of all DMs.
2.6.2. Aggregation Method
2.7. Risk Prioritization Using Spatial Mapping
3. Results
3.1. Hydraulic Capacity
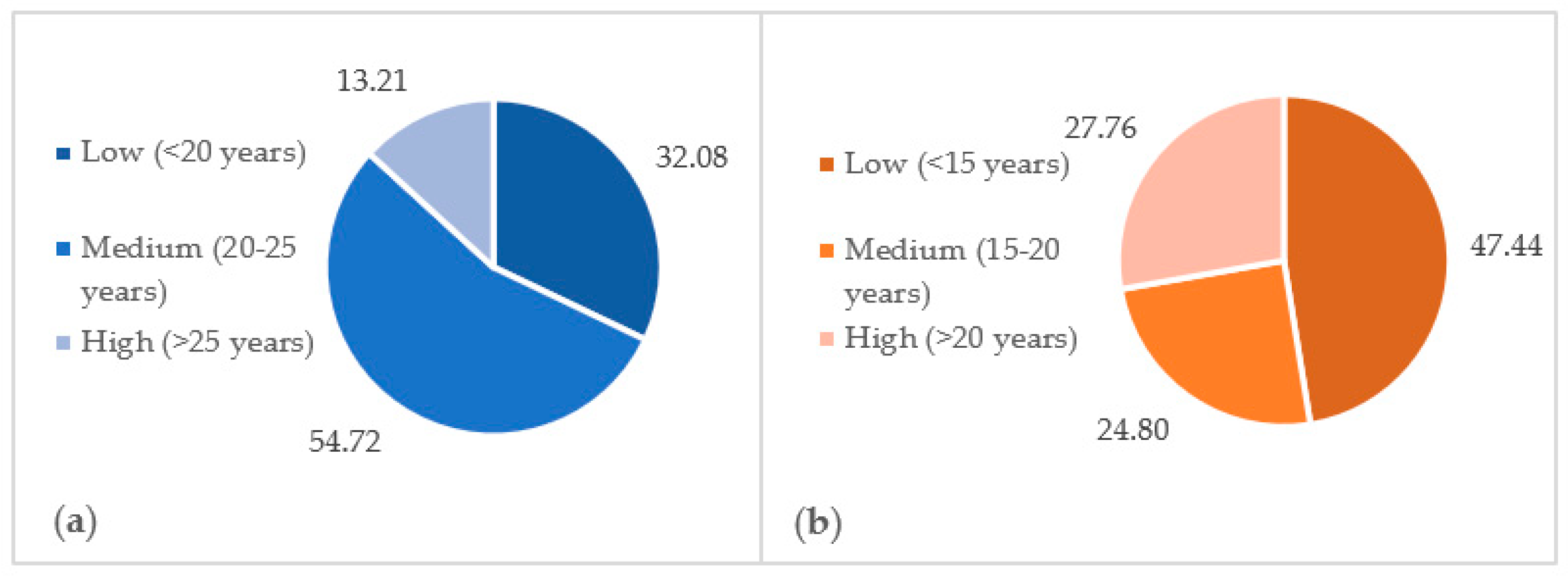
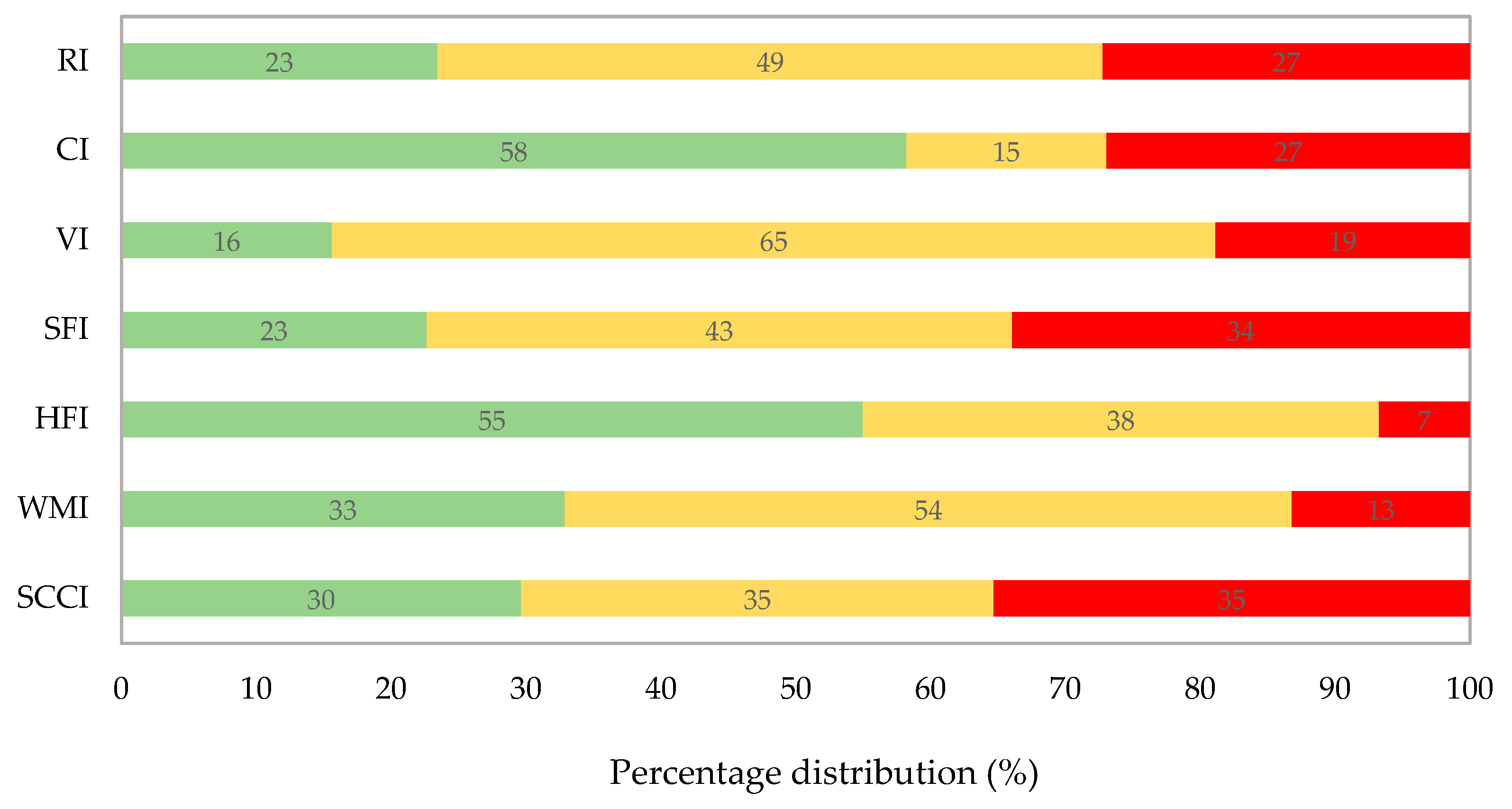
3.2. Performance, Condition, and Risk Indices
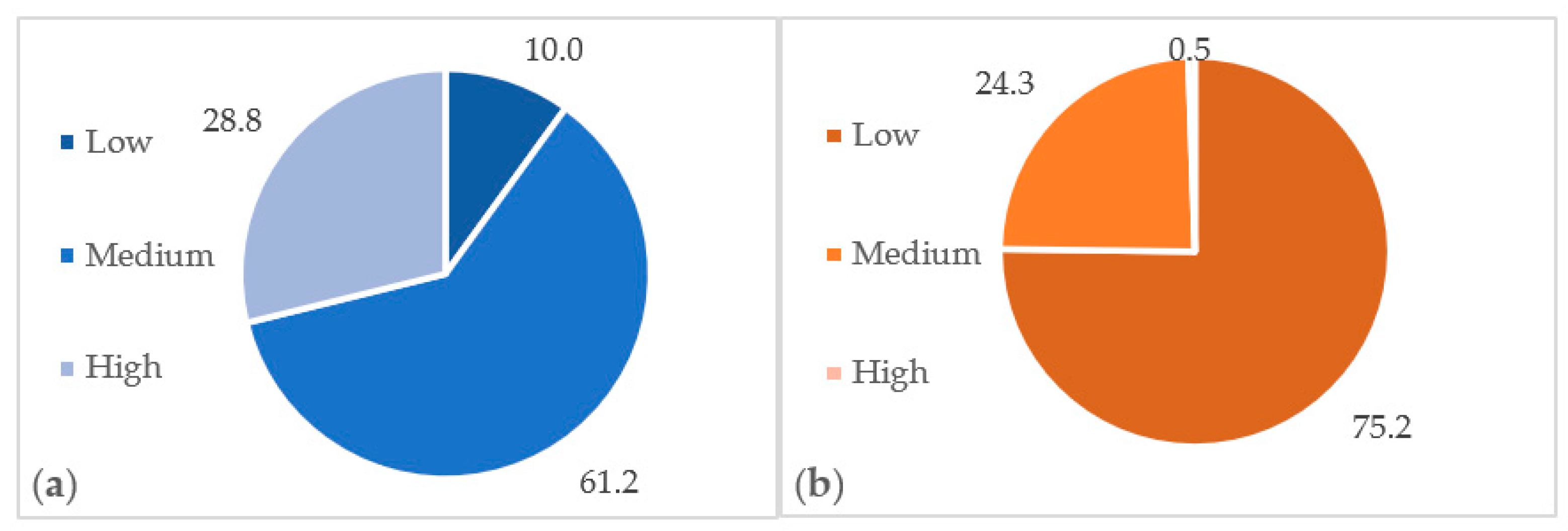
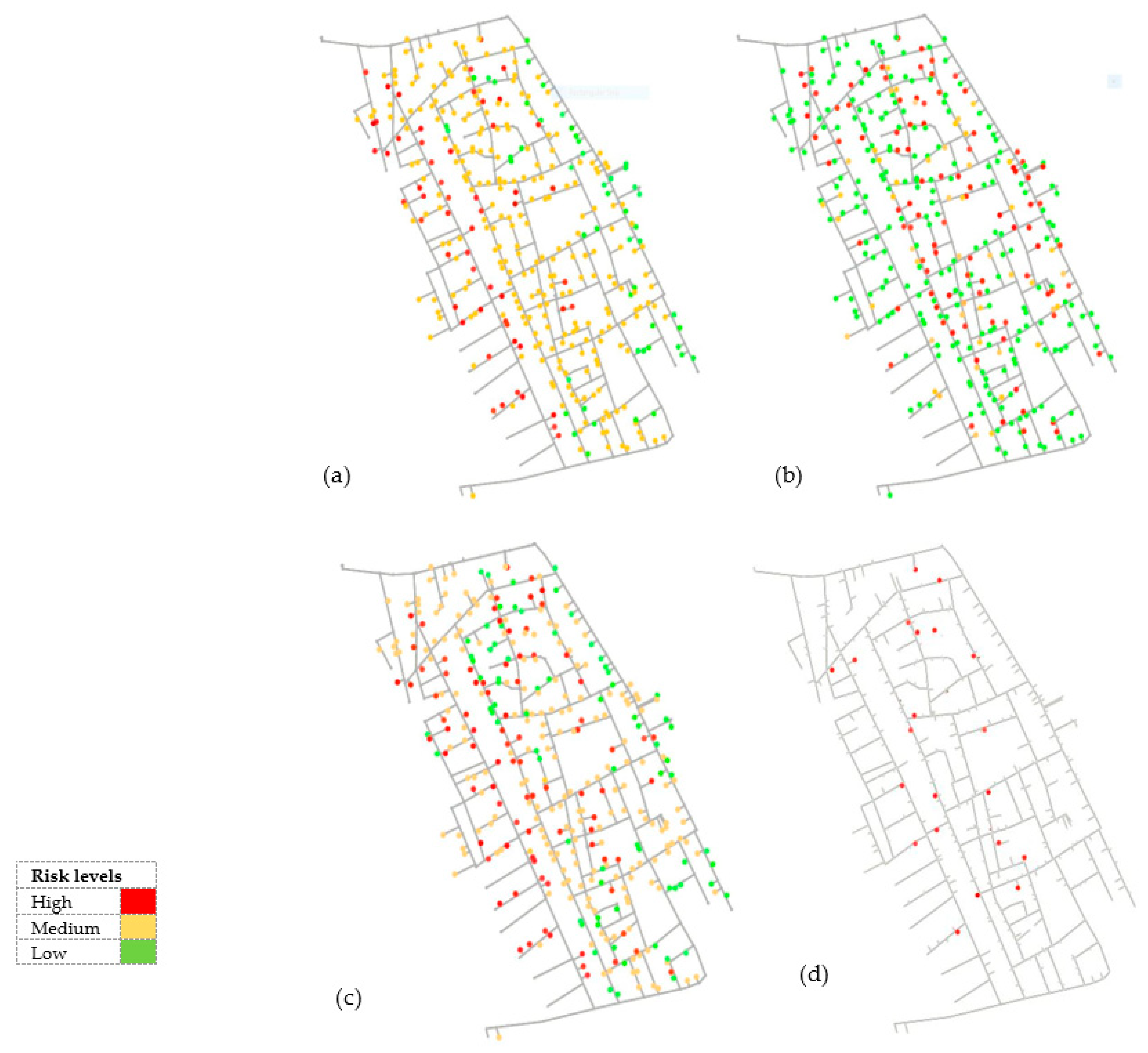
3.3. Risk-Based Prioritization of Service Connections
3.4. Model Validation
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- World Resources Institute. Water Stress by Country. Available online: http://www.wri.org/resources/charts-graphs/water-stress-country (accessed on 17 August 2022).
- Ahmed, I.; Nazzal, Y.; Zaidi, F.K.; Al-Arifi, N.S.N.; Ghrefat, H.; Naeem, M. Hydrogeological vulnerability and pollution risk mapping of groundwater in the Saq and overlying aquifers using the DRASTIC model and GIS techniques. Environ. Earth Sci. 2015, 74, 1303–1318. [Google Scholar] [CrossRef]
- Haider, H. Performance assessment framework for groundwater treatment plants in Arid Environments: A case of Buraydah, Saudi Arabia. Environ. Monit. Assess. 2017, 189, 544. [Google Scholar] [CrossRef] [PubMed]
- Global Water Market. Meeting the World’s Water and Wastewater Needs Until 2020. In Global Water Market Volume 4: Middle East and Africa. Saudi Arabia; Global Water Intelligence: Oxford, UK, 2017; pp. 1379–1385. [Google Scholar]
- Hashwa, F.; Tokajian, S. Intermittent Water Supply and Domestic Water Quality in the Middle East. In Water in the Middle East and North Africa; Springer: Berlin/Heidelberg, Germany, 2004; Chapter 2; pp. 157–166. [Google Scholar] [CrossRef]
- Haider, H.; Al-Salamah, I.S.; Ghazaw, Y.M.; Abdel-Maguid, R.H.; Shafiquzzaman, M.; Ghumman, A.R. Framework to establish economic level of leakage for intermittent water supplies in arid environments. J. Water Resour. Plan. Manag. 2019, 145, 05018018. [Google Scholar] [CrossRef]
- Granit, I. The Impact of Water Insecurity on GuIf Countries’ SociaI Contracts: A Call to Action. Available online: https://gulfif.org/the-impact-of-water-insecurity-on-guif-countries-sociai-contracts-a-call-to-action/ (accessed on 17 October 2022).
- Andey, S.P.; Kelkar, P.S. Performance of water distribution systems during intermittent versus continuous water supply. J. Am. Water Works Assoc. 2007, 99, 99–106. [Google Scholar] [CrossRef]
- Hussein, M.H.; Magram, S.F. Domestic water quality in Jeddah, Saudi Arabia. J. King Abdulaziz Univ. Eng. Sci. 2012, 23, 207–223. [Google Scholar] [CrossRef]
- Erickson, J.J.; Smith, C.D.; Goodridge, A.; Nelson, K.L. Water quality effects of intermittent water supply in Arraiján, Panama. Water Res. 2017, 114, 338–350. [Google Scholar] [CrossRef]
- Agathokleous, A.; Christodoulou, S. The impact of intermittent water supply policies on urban water distribution networks. Procedia Eng. 2016, 162, 204–211. [Google Scholar] [CrossRef]
- Charalambous, B.; Laspidou, C. Dealing with the Complex Interrelation of Intermittent Supply and Water Losses; IWA Publishing: London, UK, 2017. [Google Scholar]
- Tokajian, S.; Hashwa, F. Water quality problems associated with intermittent water supply. Water Sci. Technol. 2003, 47, 229–234. [Google Scholar] [CrossRef]
- Avvedimento, S.; Todeschini, S.; Manenti, S.; Creaco, E. Comparison of Techniques for Maintaining Adequate Disinfectant Residuals in a Full-Scale Water Distribution Network. Water 2022, 14, 1029. [Google Scholar] [CrossRef]
- Haider, H.; Alkhowaiter, M.H.; Shafiquzzaman, M.; Alresheedi, M.; AlSaleem, S.S.; Ghumman, A.R. Source to Tap Risk Assessment for Intermittent Water Supply Systems in Arid Regions: An Integrated FTA—Fuzzy FMEA Methodology. Environ. Manag. 2021, 67, 324–341. [Google Scholar] [CrossRef]
- Accurate Leak and Line. Available online: https://www.accurateleak.com/the-importance-of-pvc-pipes/ (accessed on 15 September 2022).
- InspectAPedia. Available online: https://inspectapedia.com/plumbing/Plastic_Pipe_Leaks.php#Causes (accessed on 22 September 2022).
- Monfared, Z.; Molavi, N.M.; Bayat, A. A review of water quality factors in water main failure prediction models. Water Pract. Technol. 2022, 17, 60–74. [Google Scholar] [CrossRef]
- Hunaidi, Q.; Chu, W.T. Acoustical characteristics of leak signals in plastic water distribution pipes. Appl. Acoust. 1999, 58, 235–254. [Google Scholar] [CrossRef] [Green Version]
- Rajakumar, A.G.; Cornelio, A.A.; Mohan Kumar, M.S. Leak management in district metered areas with internal-pressure reducing valves. Urban Water J. 2020, 17, 714–722. [Google Scholar] [CrossRef]
- Ghorpade, A.; Sinha, A.K.; Kalbar, P.P. Drivers for Intermittent Water Supply in India: Critical Review and Perspectives. Front. Water 2021, 3, 696630. [Google Scholar] [CrossRef]
- Valis, D.; Hasilová, K.; Forbelská, M.; Pietrucha-Urbanik, K. Modelling water distribution network failures and deterioration. In Proceedings of the 2017 IEEE International Conference on Industrial Engineering and Engineering Management (IEEM), Singapore, 10–13 December 2017; IEEE: New York, NY, USA, 2017; pp. 924–928. [Google Scholar] [CrossRef]
- Cheung, P.B.; Reis, L.F.R.; Carrijo, I.B. Multi-Objective Optimization to the Rehabilitation of a Water Distribution Network. In Advances Water Supply Manage; CRC Press: New York, NY, USA, 2003; pp. 315–325. ISBN 9780203833667. [Google Scholar]
- Giustolisi, O.; Laucelli, D.; Savic, D.A. Development of rehabilitation plans for water mains replacement considering risk and cost-benefit assessment. Civ. Eng. Environ. Syst. 2006, 23, 175–190. [Google Scholar] [CrossRef]
- Nafi, A.; Werey, C.; Llerena, P. Water pipe renewal using a multiobjective optimization approach. Can. J. Civ. Eng. 2008, 35, 87–94. [Google Scholar] [CrossRef]
- Jin, X.; Zhang, J.; Gao, J.L.; Wu, W.Y. Multi-objective optimization of water supply network rehabilitation with non-dominated sorting genetic algorithm-II. J. Zhejiang Univ. -Sci. A 2008, 9, 391–400. [Google Scholar] [CrossRef]
- D’Ercole, M.; Righetti, M.; Raspati, G.S.; Bertola, P.; Maria Ugarelli, R. Rehabilitation planning of water distribution network through a reliability—Based risk assessment. Water 2018, 10, 277. [Google Scholar] [CrossRef]
- Marzouk, M.; Hamid, S.A.; El-Said, M. A methodology for prioritizing water mains rehabilitation in Egypt. HBRC J. 2015, 11, 114–128. [Google Scholar] [CrossRef] [Green Version]
- Kabir, G.; Tesfamariam, S.; Francisque, A.; Sadiq, R. Evaluating risk of water mains failure using a Bayesian belief network model. Eur. J. Oper. Res. 2015, 240, 220–234. [Google Scholar] [CrossRef]
- Nickel Institute. Available online: https://www.dtkhydronet.com/post/leakages-in-water-distribution-networks (accessed on 25 October 2022).
- Aliaxis. Available online: https://aliaxis.com/the-benefits-of-plastic-pipe-systems/ (accessed on 17 September 2022).
- Haider, H.; Ghumman, A.R.; Al-Salamah, I.S.; Thabit, H. Assessment framework for natural groundwater contamination in arid regions: Development of indices and wells ranking system using fuzzy VIKOR method. Water 2020, 12, 423. [Google Scholar] [CrossRef] [Green Version]
- Van Zyl, J.; Clayton, C.R.I. The effect of pressure on leakage in water distribution systems. In Proceedings of the Institution of Civil Engineers-Water Management; Thomas Telford Ltd.: London, UK, 2007; Volume 160, pp. 109–114. [Google Scholar] [CrossRef]
- USEPA. Available online: https://www.epa.gov/water-research/epanet (accessed on 13 January 2022).
- Berardi, L.; Giustolisi, O.; Kapelan, Z.; Savic, D.A. Development of pipe deterioration models for water distribution systems using EPR. J. Hydroinform. 2008, 10, 113–126. [Google Scholar] [CrossRef] [Green Version]
- Hu, Y.; Hubble, D.W. Factors contributing to the failure of asbestos cement water mains. Can. J. Civ. Eng. 2007, 34, 608–621. [Google Scholar] [CrossRef]
- Alzabeebee, S.; Chapman, D.; Jefferson, I.; Faramarzi, A. The response of buried pipes to UK standard traffic loading. Proc. Inst. Civ. Eng.-Geotech. Eng. 2017, 170, 38–50. [Google Scholar] [CrossRef] [Green Version]
- Sadiq, R.; Veitch, B.; Husain, T.; Bose, N. Prioritising Environmental Effects Monitoring (EEM) Programs: A Risk-Based Strategy. In Offshore Oil and Gas Environmental Effects Monitoring Workshop: Approaches and Technologies; University of Tasmania: Hobart, Australia, 2003; pp. 71–86. [Google Scholar]
- Ketrane, R.; Yahiaoui, C. Scale precipitation on HDPE pipe by degassing of CO2 dissolved in water. AQUA—Water Infrastruct. Ecosyst. Soc. 2021, 70, 1204–1216. [Google Scholar] [CrossRef]
- Mounce, S.R.; Mounce, R.B.; Jackson, T.; Austin, J.; Boxall, J.B. Pattern matching and associative artificial neural networks for water distribution system time series data analysis. J. Hydroinform. 2014, 16, 617–632. [Google Scholar] [CrossRef] [Green Version]
- Francisque, A.; Tesfamariam, S.; Kabir, G.; Haider, H.; Reeder, A.; Sadiq, R. Water mains renewal planning framework for small to medium sized water utilities: A life cycle cost analysis approach. Urban Water J. 2017, 14, 493–501. [Google Scholar] [CrossRef]
- McGhee, T.J. Water Supply and Sewerage, 6th ed.; McGraw-Hill Publishing Company: New York, NY, USA, 2007. [Google Scholar]
- UN Habitat. Buraydah City Profile, Ministry of Municipal and Rural Affairs and United Nations Human Settlements Programme; UN Habitat: Riyadh, Saudi Arabia, 2019. [Google Scholar]
- Haider, H.; Ghumman, A.R.; Al-Salamah, I.S.; Ghazaw, Y.; Abdel-Maguid, R.H. Sustainability evaluation of rainwater harvesting-based flood risk management strategies: A multilevel decision-making framework for arid environments. Arab. J. Sci. Eng. 2019, 44, 8465–8488. [Google Scholar] [CrossRef]
- Wang, C.; Chou, M.; Pang, C. Applying Fuzzy Analytic Hierarchy Process for Evaluating Service Quality of Online Auction. Int. J. Comput. Inf. Eng. 2012, 6, 586–593. [Google Scholar] [CrossRef]
- Utilities. Available online: https://www.utilities-me.com/water/11332-special-report-preventing-leaks (accessed on 20 October 2022).
- Totsuka, N.; Trifunovic, N.; Vairavamoorthy, K. Intermittent urban water supply under water starving situations. In People-Centred Approaches to Water and Environmental Sanitation, Proceedings of the 30th WEDC International Conference, Vientiane, Laos, 25–29 October 2004; Godfrey, S., Ed.; Loughborough University: Loughborough, UK; pp. 505–512. Available online: https://repository.lboro.ac.uk/articles/conference_contribution/Intermittent_urban_water_supply_under_water_starving_situations/9595109/ (accessed on 2 November 2022).
- Hawle. Available online: https://www.hawle.com/en/hawle-knowledge/how-to/leaks-repair/ (accessed on 22 October 2022).
- Okwori, E.; Pericault, Y.; Ugarelli, R.; Viklander, M.; Hedström, A. Data-driven asset management in urban water pipe networks: A proposed conceptual framework. J. Hydroinform. 2021, 23, 1014–1029. [Google Scholar] [CrossRef]

| No. | Parameter | Units | Low (1,2,4) | Medium (3,5,7) | High (6,8,10) | Range | Polarity |
|---|---|---|---|---|---|---|---|
| 1.0 | Hydraulic | ||||||
| 1.1 | Pressure | m | 0.5–5 | 5–15 | >15 | 0.5–20 | Positive |
| 1.2 | Velocity | m/s | <0.2 | 0.2–1 | >1 | <1 | Positive |
| 2.0 | Service connection | ||||||
| 2.1 | Age | Years | <15 | 15–20 | >20 | 6–26 | Positive |
| 2.2 | Depth | m | >2 | 2–1.5 | <1.5 | 1.5 | Negative |
| 2.3 | Length | m | <3 | 3–10 | >10 | 1–62 | Positive |
| 3.0 | Water main | ||||||
| 3.1 | Age | Years | ≤20 | 20–25 | >25 | 20–27 | Positive |
| 3.2 | Material | - | Plastic | Steel | Cement | - | - |
| 3.3 | Diameter | m | <150 | 150–250 | >250 | 110–225 | Positive |
| 4.0 | Soil corrosivity | ||||||
| 4.1 | Clay | % | <20 | 20–40 | ≥40 | 2 | Positive |
| 4.2 | Gravel | % | <8 | 8–30 | ≥30 | 4 | Positive |
| 5.0 | Water Quality | ||||||
| 5.1 | Turbidity | NTU | <1 | 1–5 | >5 | 0.08–0.215 | Positive |
| 5.2 | PH | -- | >8 | 7–8 | <7 | 7.75–7.76 | Negative |
| 5.3 | Electrical conductivity | MS/cm | <1000 | 1000–1200 | >1200 | 1040–1117 | Positive |
| 5.4 | Free residual chlorine | mg/L | 0.3–0.8 | 0.1–0.3 OR 0.8–1.2 | >1.2 OR <0.1 | 0.18–0.19 | Positive/Negative |
| 5.5 | Iron | mg/L | <0.05 | 0.05–0.3 | >0.3 | 0.005–0.02 | Positive |
| 6.0 | Consequence | ||||||
| 6.1 | Number of consumers served | Person | <8 | 8–12 | >12 | 1–114 | Positive |
| Score | Vulnerability/Consequence/Risk Index | Color-Coding Scheme |
|---|---|---|
| 0–3.5 | Low | |
| 3.6–5.5 | Medium | |
| >5.5 | High |
| Linguistic Term | Fuzzy Number | TFN (l, m, u) | Linguistic Term | Fuzzy Number | TFN (l, m, u) |
|---|---|---|---|---|---|
| Extreme unimportance | 1/9, 1/9, 1/9 | 1, 2, 3 | |||
| 1/9, 1/8, 1/7 | Moderate importance | 2, 3, 4 | |||
| Very unimportant | 1/8, 1/7, 1/6 | 3, 4, 5 | |||
| 1/7, 1/6, 1/5 | Essential importance | 4, 5, 6 | |||
| Essential unimportance | 1/6, 1/5, 1/4 | 5, 6, 7 | |||
| 1/5, 1/4, 1/3 | Very vital importance | 6, 7, 8 | |||
| Moderate unimportance | 1/4, 1/3, 1/2 | 7, 8, 9 | |||
| 1/3, 1/2, 1 | Extreme importance | 9, 9, 9 | |||
| Equally importance | 1 | 1, 1, 1 | - | - | - |
| n | 1 | 2 | 3 | 4 | 5 | 6 | 8 | 8 | 9 | 10 |
| RI | 0 | 0 | 0.58 | 0.90 | 1.12 | 1.24 | 1.32 | 1.41 | 1.45 | 1.49 |
| Code | Age (Year) | PS 1 | Fuzzy Score | Depth (m) | PS | Fuzzy Score | Length (m) | PS | Fuzzy Score | SCCI | Priority | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Wi | 0.62 | 0.69 | 0.73 | 0.12 | 0.12 | 0.13 | 0.15 | 0.19 | 0.22 | l | m | u | Crisp | |||||||
| SC6 | 22 | H 2 | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 18 | H | 6 | 8 | 10 | 5.02 | 7.65 | 10.4 | 7.67 | HIGH |
| SC7 | 22 | H | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 5.5 | M | 3 | 5 | 7 | 4.55 | 7.07 | 9.74 | 7.1 | HIGH |
| SC8 | 23 | H | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 10.8 | H | 6 | 8 | 10 | 5.02 | 7.65 | 10.4 | 7.67 | HIGH |
| SC9 | 19 | M 3 | 3 | 5 | 7 | 1.5 | M | 3 | 5 | 7 | 7.7 | M | 3 | 5 | 7 | 2.68 | 5 | 7.55 | 5.04 | MEDIUM |
| SC10 | 21 | H | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 10 | M | 3 | 5 | 7 | 4.55 | 7.07 | 9.74 | 7.1 | HIGH |
| SC11 | 22 | H | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 14 | H | 6 | 8 | 10 | 5.02 | 7.65 | 10.4 | 7.67 | HIGH |
| SC12 | 23 | H | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 13.2 | H | 6 | 8 | 10 | 5.02 | 7.65 | 10.4 | 7.67 | HIGH |
| SC17 | 21 | H | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 7 | M | 3 | 5 | 7 | 4.55 | 7.07 | 9.74 | 7.1 | HIGH |
| SC18 | 17 | M | 3 | 5 | 7 | 1.5 | M | 3 | 5 | 7 | 6.1 | M | 3 | 5 | 7 | 2.68 | 5 | 7.55 | 5.04 | MEDIUM |
| SC19 | 21 | H | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 11.9 | H | 6 | 8 | 10 | 5.02 | 7.65 | 10.4 | 7.67 | HIGH |
| SC20 | 23 | H | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 11.8 | H | 6 | 8 | 10 | 5.02 | 7.65 | 10.4 | 7.67 | HIGH |
| SC21 | 14 | L 4 | 1 | 2 | 4 | 1.5 | M | 3 | 5 | 7 | 12.1 | H | 6 | 8 | 10 | 1.9 | 3.5 | 6.01 | 3.65 | MEDIUM |
| SC22 | 21 | H | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 4 | M | 3 | 5 | 7 | 4.55 | 7.07 | 9.74 | 7.1 | HIGH |
| SC23 | 24 | H | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 3.8 | M | 3 | 5 | 7 | 4.55 | 7.07 | 9.74 | 7.1 | HIGH |
| SC24 | 20 | M | 3 | 5 | 7 | 1.5 | M | 3 | 5 | 7 | 4 | M | 3 | 5 | 7 | 2.68 | 5 | 7.55 | 5.04 | MEDIUM |
| SC25 | 14 | L | 1 | 2 | 4 | 1.5 | M | 3 | 5 | 7 | 2.7 | L | 1 | 2 | 4 | 1.13 | 2.35 | 4.7 | 2.54 | LOW |
| SC26 | 21 | H | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 3 | M | 3 | 5 | 7 | 4.55 | 7.07 | 9.74 | 7.1 | HIGH |
| SC27 | 20 | M | 3 | 5 | 7 | 1.5 | M | 3 | 5 | 7 | 2.5 | L | 1 | 2 | 4 | 2.38 | 4.43 | 6.9 | 4.5 | MEDIUM |
| SC28 | 15 | M | 3 | 5 | 7 | 1.5 | M | 3 | 5 | 7 | 11.4 | H | 6 | 8 | 10 | 3.14 | 5.57 | 8.2 | 5.61 | HIGH |
| SC31 | 19 | M | 3 | 5 | 7 | 1.5 | M | 3 | 5 | 7 | 13.3 | H | 6 | 8 | 10 | 3.14 | 5.57 | 8.2 | 5.61 | HIGH |
| SC32 | 20 | M | 3 | 5 | 7 | 1.5 | M | 3 | 5 | 7 | 6.4 | M | 3 | 5 | 7 | 2.68 | 5 | 7.55 | 5.04 | MEDIUM |
| SC34 | 17 | M | 3 | 5 | 7 | 1.5 | M | 3 | 5 | 7 | 3.5 | M | 3 | 5 | 6 | 2.68 | 5 | 7.33 | 5 | MEDIUM |
| SC36 | 24 | H | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 11 | H | 6 | 8 | 10 | 5.02 | 7.65 | 10.4 | 7.67 | HIGH |
| SC37 | 12 | L | 1 | 2 | 4 | 1.5 | M | 3 | 5 | 7 | 49.2 | H | 6 | 8 | 10 | 1.9 | 3.5 | 6.01 | 3.65 | MEDIUM |
| SC38 | 22 | H | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 7.6 | M | 3 | 5 | 7 | 4.55 | 7.07 | 9.74 | 7.1 | HIGH |
| SC39 | 16 | M | 3 | 5 | 7 | 1.5 | M | 3 | 5 | 7 | 2.9 | L | 1 | 2 | 4 | 2.38 | 4.43 | 6.9 | 4.5 | MEDIUM |
| SC40 | 23 | H | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 16.5 | H | 6 | 8 | 10 | 5.02 | 7.65 | 10.4 | 7.67 | HIGH |
| SC44 | 15 | M | 3 | 5 | 7 | 1.5 | M | 3 | 5 | 7 | 6.3 | M | 3 | 5 | 7 | 2.68 | 5 | 7.55 | 5.04 | MEDIUM |
| SC45 | 13 | L | 1 | 2 | 4 | 1.5 | M | 3 | 5 | 7 | 2.8 | L | 1 | 2 | 4 | 1.13 | 2.35 | 4.7 | 2.54 | LOW |
| SC46 | 6 | L | 1 | 2 | 4 | 1.5 | M | 3 | 5 | 7 | 1 | L | 1 | 2 | 4 | 1.13 | 2.35 | 4.7 | 2.54 | LOW |
| SC47 | 23 | H | 6 | 8 | 10 | 1.5 | M | 3 | 5 | 7 | 3 | M | 3 | 5 | 7 | 4.55 | 7.07 | 9.74 | 7.1 | HIGH |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Haider, H.; Almutlaq, M.A.; Alodah, A.; Ghumman, A.R.; AlSalamah, I.S.; Ghazaw, Y.M.; Shafiquzzaman, M. Risk-Based Inspection and Rehabilitation Planning of Service Connections in Intermittent Water Supply Systems for Leakage Management in Arid Regions. Water 2022, 14, 3994. https://doi.org/10.3390/w14243994
Haider H, Almutlaq MA, Alodah A, Ghumman AR, AlSalamah IS, Ghazaw YM, Shafiquzzaman M. Risk-Based Inspection and Rehabilitation Planning of Service Connections in Intermittent Water Supply Systems for Leakage Management in Arid Regions. Water. 2022; 14(24):3994. https://doi.org/10.3390/w14243994
Chicago/Turabian StyleHaider, Husnain, Muath Abdullah Almutlaq, Abdullah Alodah, Abdul Razzaq Ghumman, Ibrahim Saleh AlSalamah, Yousry Mahmoud Ghazaw, and Md. Shafiquzzaman. 2022. "Risk-Based Inspection and Rehabilitation Planning of Service Connections in Intermittent Water Supply Systems for Leakage Management in Arid Regions" Water 14, no. 24: 3994. https://doi.org/10.3390/w14243994





