Al-Impregnated Granular Activated Carbon for Removal of Fluoride from Aqueous Solution: Batch and Fixed-Bed Column Study
Abstract
1. Introduction
2. Materials and Methods
2.1. Chemicals and Materials
2.2. Preparation and Characterization of Material
2.3. Batch Study
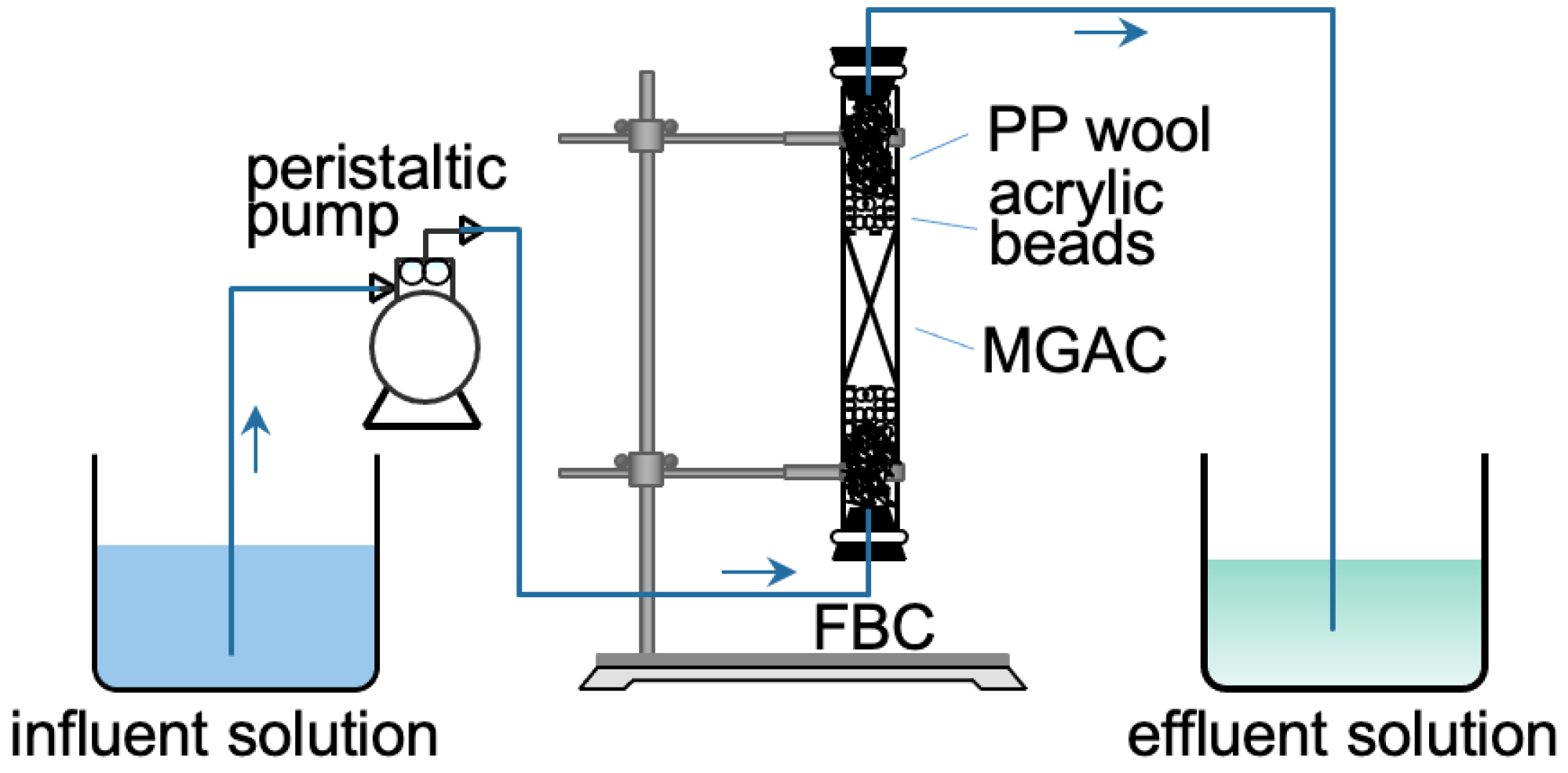
2.4. Column Study
2.4.1. Experimental Procedure
2.4.2. Determination of Breakthrough Curves
3. Results
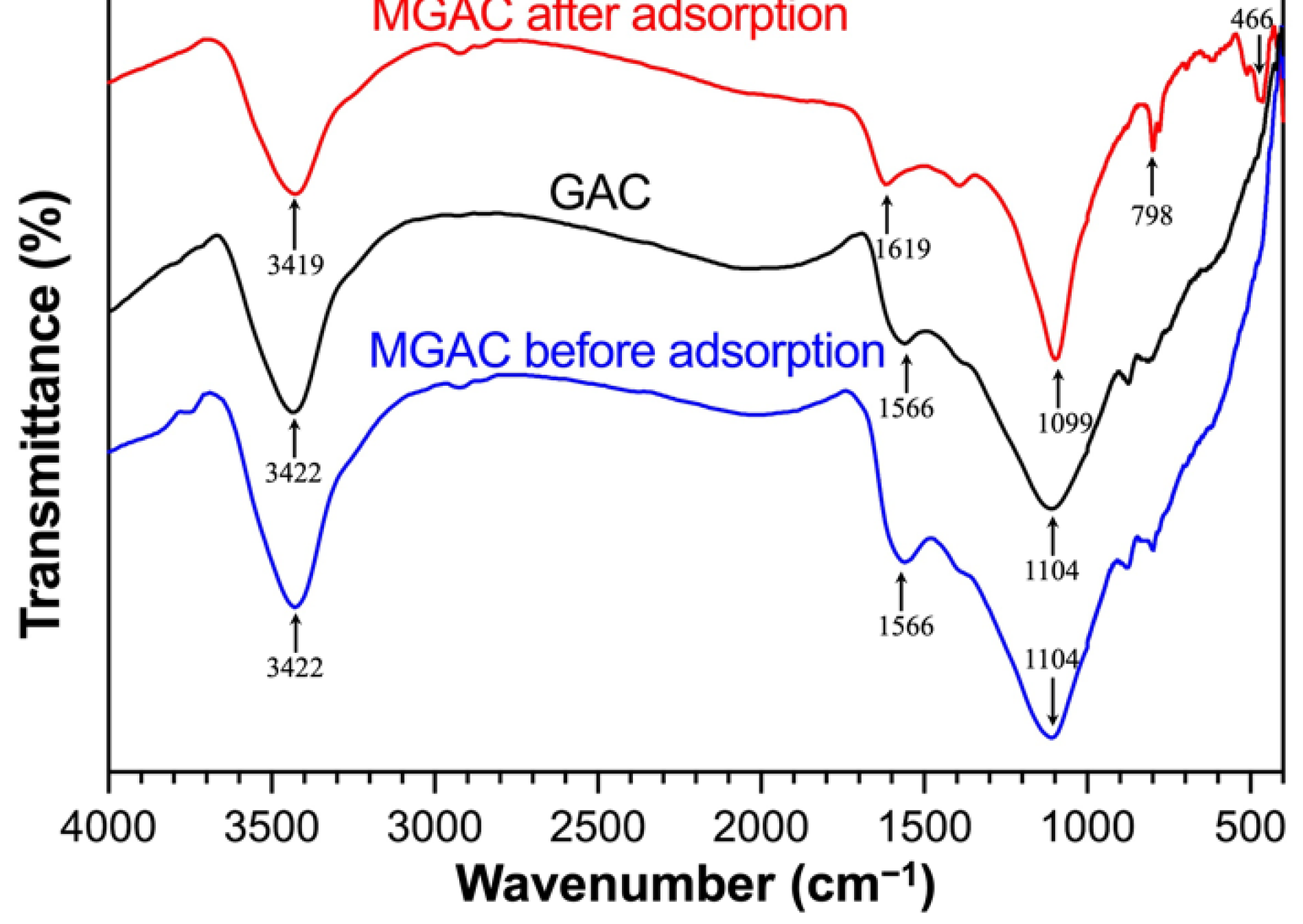
3.1. Characterization
3.2. Isotherm and Kinetic Study
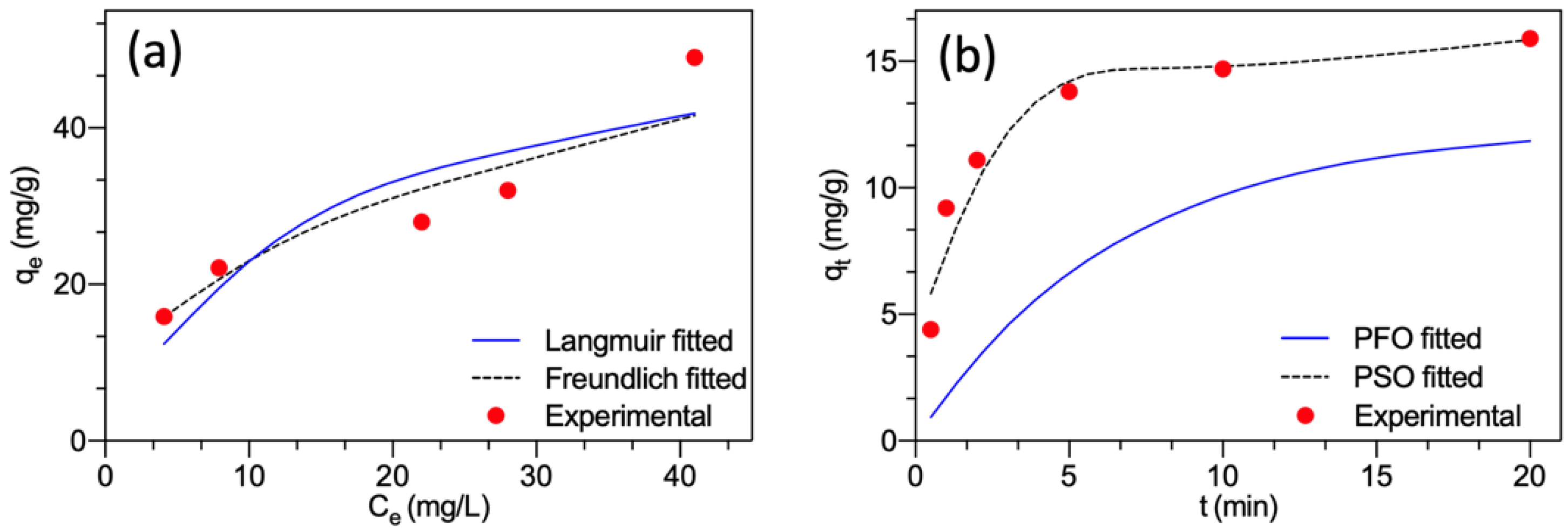
3.2.1. Isotherm Study
3.2.2. Kinetic Study
3.3. Fixed-Bed Column Adsorption
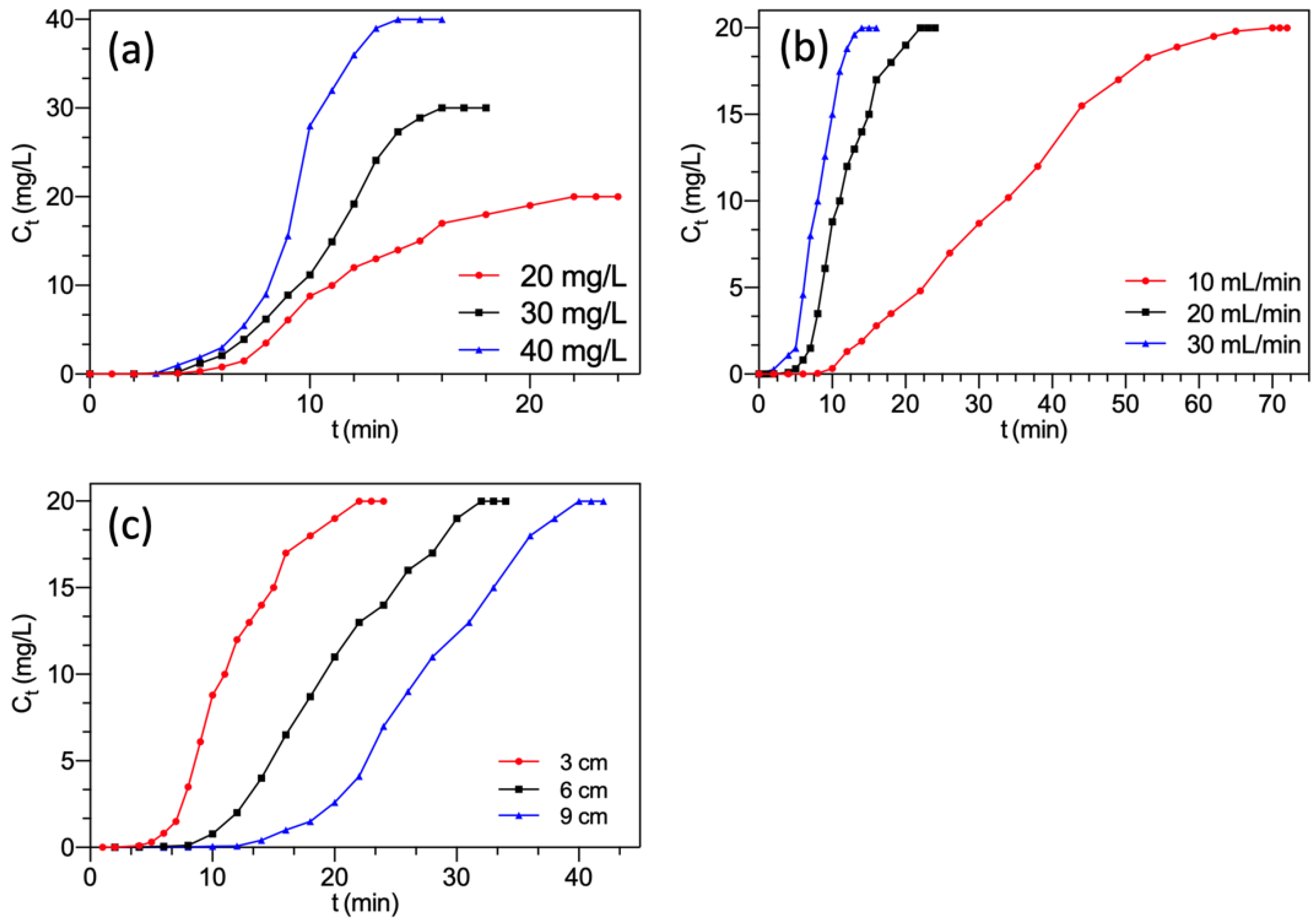
3.3.1. Effect of Fluoride Concentration
3.3.2. Effect of Flow Rate
3.3.3. Effect of Bed Height
3.4. Breakthrough Curve Modelling
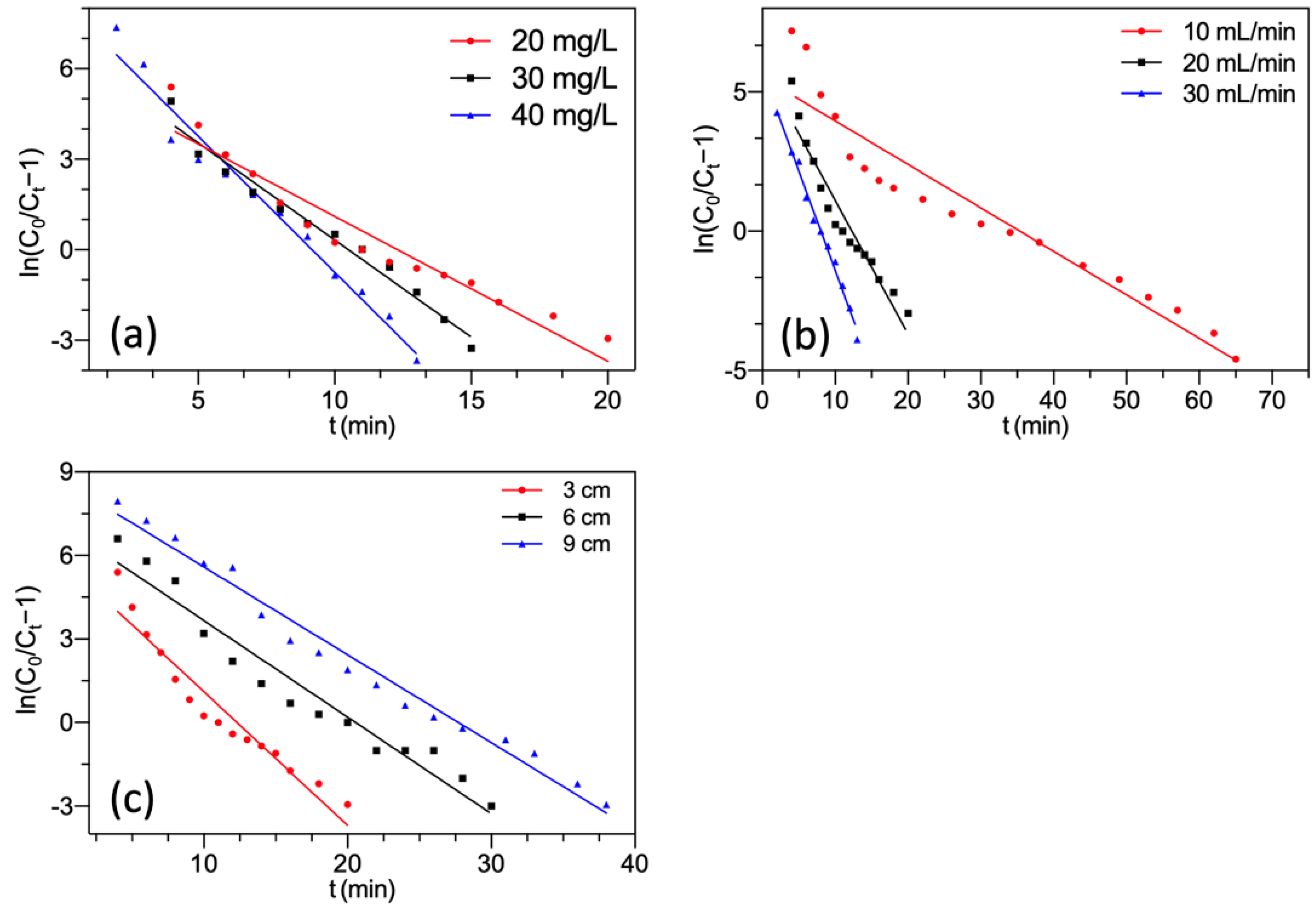
3.4.1. Thomas Model
3.4.2. Yoon-Nelson Model
3.4.3. Yan Model
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dong, Q.; Yang, D.; Luo, L.; He, Q.; Cai, F.; Cheng, S.; Chen, Y. Engineering porous biochar for capacitive fluorine removal. Sep. Purif. Technol. 2020, 257, 117932. [Google Scholar] [CrossRef]
- Fan, C.; Yin, N.; Cai, X.; Du, X.; Wang, P.; Liu, X.; Li, Y.; Chang, X.; Du, H.; Ma, J.; et al. Stabilization of fluorine-contaminated soil in aluminum smelting site with biochar loaded iron-lanthanide and aluminum-lanthanide bimetallic materials. J. Hazard. Mater. 2022, 426, 128072. [Google Scholar] [CrossRef] [PubMed]
- Brown, W.; Gregory, T.; Chow, L. Effects of Fluoride on Enamel Solubility and Cariostasis. Caries Res. 1977, 11, 118–141. [Google Scholar] [CrossRef] [PubMed]
- Nell, J.; Livanos, G. Effects of fluoride concentration in seawater on growth and fluoride accumulation by Sydney rock oyster (Saccostrea commercialis) and flat oyster (Ostrea angasi) spat. Water Res. 1988, 22, 749–753. [Google Scholar] [CrossRef]
- Kanduti, D.; Sterbenk, P.; Artnik, A. Fluoride: A Review of Use and Effects on Health. Mater. Socio Medica 2016, 28, 133–137. [Google Scholar] [CrossRef]
- WHO. Guidelines for Drinking-Water Quality, 4th ed.; Incorporating the 1st Addendum; WHO: Geneva, Switzerland, 2017; Volume 24, 631p, Available online: https://www.who.int/publications/i/item/9789241549950 (accessed on 7 May 2021).
- Bhatnagar, A.; Kumar, E.; Sillanpaa, M. Fluoride removal from water by adsorption—A review. Chem. Eng. J. 2011, 171, 811–840. [Google Scholar] [CrossRef]
- He, J.; Yang, Y.; Wu, Z.; Xie, C.; Zhang, K.; Kong, L.; Liu, J. Review of fluoride removal from water environment by adsorption. J. Environ. Chem. Eng. 2020, 8, 104516. [Google Scholar] [CrossRef]
- Gago, C.; Romar, A.; Marcos, M.L.F.; Álvarez, E. Fluorine sorption by soils developed from various parent materials in Galicia (NW Spain). J. Colloid Interface Sci. 2012, 374, 232–236. [Google Scholar] [CrossRef]
- Wang, Z.; Gu, X.; Zhang, Y.; Zhang, X.; Ngo, H.H.; Liu, Y.; Jiang, W.; Tan, X.; Wang, X.; Zhang, J. Activated nano-Al2O3 loaded on polyurethane foam as a potential carrier for fluorine removal. J. Water Process. Eng. 2021, 44, 102444. [Google Scholar] [CrossRef]
- Kumari, U.; Behera, S.K.; Meikap, B.C. A novel acid modified alumina adsorbent with enhanced defluoridation property: Kinetics, isotherm study and applicability on industrial wastewater. J. Hazard. Mater. 2019, 365, 868–882. [Google Scholar] [CrossRef]
- Iwar, R.T.; Iorhemen, O.T.; Katibi, K.K. Novel aluminium (hydr) oxide-functionalized activated carbon derived from Raffia palm (Raphia hookeri) shells: Augmentation of its adsorptive properties for efficient fluoride uptake in aqueous media. Environ. Chem. Ecotoxicol. 2021, 3, 142–154. [Google Scholar] [CrossRef]
- Mullick, A.; Neogi, S. Acoustic cavitation induced synthesis of zirconium impregnated activated carbon for effective fluoride scavenging from water by adsorption. Ultrason. Sonochem. 2018, 45, 65–77. [Google Scholar] [CrossRef]
- Bhatnagar, A.; Hogland, W.; Marques, M.; Sillanpää, M. An overview of the modification methods of activated carbon for its water treatment applications. Chem. Eng. J. 2013, 219, 499–511. [Google Scholar] [CrossRef]
- Shao, Y.; Li, J.; Fang, X.; Yang, Z.; Qu, Y.; Yang, M.; Tan, W.; Li, G.; Wang, H. Chemical modification of bamboo activated carbon surface and its adsorption property of simultaneous removal of phosphate and nitrate. Chemosphere 2021, 287, 132118. [Google Scholar] [CrossRef]
- Li, Y.-H.; Wang, S.; Cao, A.; Zhao, D.; Zhang, X.; Xu, C.; Luan, Z.; Ruan, D.; Liang, J.; Wu, D.; et al. Adsorption of fluoride from water by amorphous alumina supported on carbon nanotubes. Chem. Phys. Lett. 2001, 350, 412–416. [Google Scholar] [CrossRef]
- Mullick, A.; Neogi, S. Ultrasound assisted synthesis of Mg-Mn-Zr impregnated activated carbon for effective fluoride adsorption from water. Ultrason. Sonochemistry 2019, 50, 126–137. [Google Scholar] [CrossRef]
- Roy, S.; Das, P.; Sengupta, S.; Manna, S. Calcium impregnated activated charcoal: Optimization and efficiency for the treatment of fluoride containing solution in batch and fixed bed reactor. Process. Saf. Environ. Prot. 2017, 109, 18–29. [Google Scholar] [CrossRef]
- Ahmad, A.A.; Hameed, B.H. Fixed-bed adsorption of reactive azo dye onto granular activated carbon prepared from waste. J. Hazard. Mater. 2010, 175, 298–303. [Google Scholar] [CrossRef]
- Chen, J.; Yang, R.; Zhang, Z.; Wu, D. Removal of fluoride from water using aluminum hydroxide-loaded zeolite synthesized from coal fly ash. J. Hazard. Mater. 2021, 421, 126817. [Google Scholar] [CrossRef]
- Pang, T.; Marken, F.; Zhang, D.; Shen, J. Investigating the role of dissolved inorganic and organic carbon in fluoride removal by membrane capacitive deionization. Desalination 2022, 528, 115618. [Google Scholar] [CrossRef]
- Saravanan, L.; Subramanian, S. Surface chemical studies on the competitive adsorption of poly(ethylene glycol) and ammonium poly(methacrylate) onto alumina. J. Colloid Interface Sci. 2005, 284, 363–377. [Google Scholar] [CrossRef] [PubMed]
- Wahab, M.A.; Jellali, S.; Jedidi, N. Ammonium biosorption onto sawdust: FTIR analysis, kinetics and adsorption isotherms modeling. Bioresour. Technol. 2010, 101, 5070–5075. [Google Scholar] [CrossRef] [PubMed]
- Hamid, Y.; Liu, L.; Usman, M.; Naidu, R.; Haris, M.; Lin, Q.; Ulhassan, Z.; Hussain, M.I.; Yang, X. Functionalized biochars: Synthesis, characterization, and applications for removing trace elements from water. J. Hazard. Mater. 2022, 437, 129337. [Google Scholar] [CrossRef] [PubMed]
- Kumari, U.; Behera, S.K.; Siddiqi, H.; Meikap, B.C. Facile method to synthesize efficient adsorbent from alumina by nitric acid activation: Batch scale defluoridation, kinetics, isotherm studies and implementation on industrial wastewater treatment. J. Hazard. Mater. 2020, 381, 120917. [Google Scholar] [CrossRef] [PubMed]
- De Franco, M.A.E.; de Carvalho, C.B.; Bonetto, M.M.; de Pelegrini Soares, R.; Féris, L.A. Diclofenac removal from water by adsorption using activated carbon in batch mode and fixed-bed column: Isotherms, thermodynamic study and breakthrough curves modeling. J. Clean. Prod. 2018, 181, 145–154. [Google Scholar] [CrossRef]
- Freundlich, H.M.F. Over the adsorption in solution. J. Phys. Chem. 1906, 57, 385–471. [Google Scholar]
- Das, L.; Sengupta, S.; Das, P.; Bhowal, A.; Bhattacharjee, C. Experimental and Numerical modeling on dye adsorption using pyrolyzed mesoporous biochar in Batch and fixed-bed column reactor: Isotherm, thermodynamics, mass transfer, kinetic analysis. Surf. Interfaces 2021, 23, 100985. [Google Scholar] [CrossRef]
- Chen, C.; Chen, Z.; Shen, J.; Kang, J.; Zhao, S.; Wang, B.; Chen, Q.; Li, X. Dynamic adsorption models and artificial neural network prediction of mercury adsorption by a dendrimer-grafted polyacrylonitrile fiber in fixed-bed column. J. Clean. Prod. 2021, 310, 127511. [Google Scholar] [CrossRef]
- Bacelo, H.; Santos, S.C.; Ribeiro, A.; Boaventura, R.A.; Botelho, C.M. Antimony removal from water by pine bark tannin resin: Batch and fixed-bed adsorption. J. Environ. Manag. 2021, 302, 114100. [Google Scholar] [CrossRef]
- Singh, A.; Kumar, D.; Gaur, J.P. Continuous metal removal from solution and industrial effluents using Spirogyra biomass-packed column reactor. Water Res. 2012, 46, 779–788. [Google Scholar] [CrossRef]
- Nur, T.; Loganathan, P.; Nguyen, T.; Vigneswaran, S.; Singh, G.; Kandasamy, J. Batch and column adsorption and desorption of fluoride using hydrous ferric oxide: Solution chemistry and modeling. Chem. Eng. J. 2014, 247, 93–102. [Google Scholar] [CrossRef]
- Bishayee, B.; Ruj, B.; Nandi, S.; Chatterjee, R.P.; Mallick, A.; Chakraborty, P.; Nayak, J.; Chakrabortty, S. Sorptive elimination of fluoride from contaminated groundwater in a fixed bed column: A kinetic model validation based study. J. Indian Chem. Soc. 2021, 99, 100302. [Google Scholar] [CrossRef]
- Han, X.; Zhang, Y.; Zheng, C.; Yu, X.; Li, S.; Wei, W. Enhanced Cr(VI) removal from water using a green synthesized nanocrystalline chlorapatite: Physicochemical interpretations and fixed-bed column mathematical model study. Chemosphere 2020, 264, 128421. [Google Scholar] [CrossRef]
- Madan, S.S.; De, B.S.; Wasewar, K.L. Adsorption performance of packed bed column for benzylformic acid removal using CaO2 nanoparticles. Chem. Data Collect. 2019, 23, 100267. [Google Scholar] [CrossRef]
- Chatterjee, S.; Mondal, S.; De, S. Design and scaling up of fixed bed adsorption columns for lead removal by treated laterite. J. Clean. Prod. 2018, 177, 760–774. [Google Scholar] [CrossRef]
- Yanyan, L.; Kurniawan, T.A.; Zhu, M.; Ouyang, T.; Avtar, R.; Othman, M.H.D.; Mohammad, B.T.; Albadarin, A.B. Removal of acetaminophen from synthetic wastewater in a fixed-bed column adsorption using low-cost coconut shell waste pretreated with NaOH, HNO3, ozone, and/or chitosan. J. Environ. Manag. 2018, 226, 365–376. [Google Scholar] [CrossRef]
- Kumari, U.; Mishra, A.; Siddiqi, H.; Meikap, B. Effective defluoridation of industrial wastewater by using acid modified alumina in fixed-bed adsorption column: Experimental and breakthrough curves analysis. J. Clean. Prod. 2020, 279, 123645. [Google Scholar] [CrossRef]
- Bai, S.; Li, J.; Ding, W.; Chen, S.; Ya, R. Removal of boron by a modified resin in fixed bed column: Breakthrough curve analysis using dynamic adsorption models and artificial neural network model. Chemosphere 2022, 296, 134021. [Google Scholar] [CrossRef]
- Cruz-Olivares, J.; Perez-Alonso, C.; Barrera-Díaz, C.; Ureña-Nuñez, F.; Chaparro-Mercado, M.; Bilyeu, B. Modeling of lead (II) biosorption by residue of allspice in a fixed-bed column. Chem. Eng. J. 2013, 228, 21–27. [Google Scholar] [CrossRef]
- Cruz, M.A.P.; Guimarães, L.C.M.; Júnior, E.F.D.C.; Rocha, S.D.F.; Mesquita, P.D.L. Adsorption of crystal violet from aqueous solution in continuous flow system using bone char. Chem. Eng. Commun. 2019, 207, 372–381. [Google Scholar] [CrossRef]
- Manjunath, S.V.; Kumar, M. Simultaneous removal of antibiotic and nutrients via Prosopis juliflora activated carbon column: Performance evaluation, effect of operational parameters and breakthrough modeling. Chemosphere 2021, 262, 127820. [Google Scholar] [CrossRef] [PubMed]
- Ang, T.N.; Young, B.R.; Taylor, M.; Burrell, R.; Aroua, M.K.; Baroutian, S. Breakthrough analysis of continuous fixed-bed adsorption of sevoflurane using activated carbons. Chemosphere 2019, 239, 124839. [Google Scholar] [CrossRef] [PubMed]
- Banerjee, K.; Ramesh, S.T.; Gandhimathi, R. A novel agricultural waste adsorbent, watermelon shell for the removal of copper from aqueous solutions. Iran. J. Energy Environ. 2012, 3, 143–156. [Google Scholar] [CrossRef]
- Aksu, Z.; Gönen, F. Biosorption of phenol by immobilized activated sludge in a continuous packed bed: Prediction of breakthrough curves. Process. Biochem. 2004, 39, 599–613. [Google Scholar] [CrossRef]
- Chen, S.; Yue, Q.; Gao, B.; Li, Q.; Xu, X.; Fu, K. Adsorption of hexavalent chromium from aqueous solution by modified corn stalk: A fixed-bed column study. Bioresour. Technol. 2012, 113, 114–120. [Google Scholar] [CrossRef]
- Kumar, P.A.; Chakraborty, S. Fixed-bed column study for hexavalent chromium removal and recovery by short-chain polyaniline synthesized on jute fiber. J. Hazard. Mater. 2009, 162, 1086–1098. [Google Scholar] [CrossRef]
- Du, Z.; Zheng, T.; Wang, P. Experimental and modelling studies on fixed bed adsorption for Cu(II) removal from aqueous solution by carboxyl modified jute fiber. Powder Technol. 2018, 338, 952–959. [Google Scholar] [CrossRef]
- Gupta, A.; Garg, A. Adsorption and oxidation of ciprofloxacin in a fixed bed column using activated sludge derived activated carbon. J. Environ. Manag. 2019, 250, 109474. [Google Scholar] [CrossRef]
- Talat, M.; Mohan, S.; Dixit, V.; Singh, D.K.; Hasan, S.H.; Srivastava, O.N. Effective removal of fluoride from water by coconut husk activated carbon in fixed bed column: Experimental and breakthrough curves analysis. Groundw. Sustain. Dev. 2018, 7, 48–55. [Google Scholar] [CrossRef]
- Mohan, S.; Singh, D.K.; Kumar, V.; Hasan, S.H. Effective removal of fluoride ions by rGO/ZrO2 nanocomposite from aqueous solution: Fixed bed column adsorption modelling and its adsorption mechanism. J. Fluor. Chem. 2017, 194, 40–50. [Google Scholar] [CrossRef]
- Bai, B.; Xu, X.; Li, C.; Xing, J.; Wang, H.; Suo, Y. Magnetic Fe3O4@Chitosan Carbon Microbeads: Removal of Doxycycline from Aqueous Solutions through a Fixed Bed via Sequential Adsorption and Heterogeneous Fenton-Like Regeneration. J. Nanomater. 2018, 2018, 5296410. [Google Scholar] [CrossRef]
- Yan, G.; Viraraghavan, T.; Chen, M. A New Model for Heavy Metal Removal in a Biosorption Column. Adsorpt. Sci. Technol. 2001, 19, 25–43. [Google Scholar] [CrossRef]
- De Franco, M.A.E.; de Carvalho, C.B.; Bonetto, M.M.; de Pelegrini Soares, R.; Féris, L.A. Removal of amoxicillin from water by adsorption onto activated carbon in batch process and fixed bed column: Kinetics, isotherms, experimental design and breakthrough curves modelling. J. Clean. Prod. 2017, 161, 947–956. [Google Scholar] [CrossRef]
- Ye, Y.; Yang, J.; Jiang, W.; Kang, J.; Hu, Y.; Ngo, H.H.; Guo, W.; Liu, Y. Fluoride removal from water using a magnesia-pullulan composite in a continuous fixed-bed column. J. Environ. Manag. 2018, 206, 929–937. [Google Scholar] [CrossRef]
- Reck, I.M.; Paixão, R.M.; Bergamasco, R.; Vieira, M.F.; Vieira, A.M.S. Investigation of Moringa oleifera seeds as effective and low-cost adsorbent to remove yellow dye tartrazine in fixed-bed column. Sep. Sci. Technol. 2018, 55, 13–25. [Google Scholar] [CrossRef]



| C0 (mg/L) | Q (mL/min) | Z (cm) | w (g) | VF (mL) | tb (min) | ts (min) | mads (mg) | mtotal (mg) | qs (mg/g) | R (%) |
|---|---|---|---|---|---|---|---|---|---|---|
| 20 | 20 | 3 | 1.5 | 430 | 7.0 | 21.5 | 4.62 | 8.60 | 3.08 | 53.7% |
| 30 | 20 | 3 | 1.5 | 312 | 5.4 | 15.6 | 6.24 | 9.36 | 4.16 | 66.6% |
| 40 | 20 | 3 | 1.5 | 270 | 4.6 | 13.5 | 7.27 | 10.8 | 4.84 | 67.3% |
| 20 | 10 | 3 | 1.5 | 650 | 12.5 | 65.0 | 6.53 | 13.0 | 4.35 | 50.2% |
| 20 | 30 | 3 | 1.5 | 393 | 5.0 | 13.1 | 4.58 | 7.86 | 3.05 | 58.2% |
| 20 | 20 | 6 | 3.0 | 630 | 11.3 | 31.5 | 7.78 | 12.6 | 2.59 | 61.8% |
| 20 | 20 | 9 | 4.5 | 790 | 18.0 | 39.5 | 10.80 | 15.8 | 2.40 | 68.3% |
| C0 (mg/L) | Q (mL/min) | Z (cm) | KTh (mL/(min·mg)) | q0 (mg/g) | R2 |
|---|---|---|---|---|---|
| 20 | 20 | 3 | 0.0240 | 3.28 | 0.929 |
| 30 | 20 | 3 | 0.0215 | 4.20 | 0.976 |
| 40 | 20 | 3 | 0.0225 | 4.89 | 0.973 |
| 20 | 10 | 3 | 0.00781 | 4.72 | 0.910 |
| 20 | 30 | 3 | 0.0357 | 3.22 | 0.991 |
| 20 | 20 | 6 | 0.0172 | 5.50 | 0.949 |
| 20 | 20 | 9 | 0.0158 | 7.39 | 0.980 |
| C0 (mg/L) | Q (mL/min) | Z (cm) | KYN (min−1) | τ (min) | R2 | qY (mg/g) |
|---|---|---|---|---|---|---|
| 20 | 20 | 3 | 0.480 | 12.3 | 0.929 | 3.28 |
| 30 | 20 | 3 | 0.644 | 10.5 | 0.976 | 4.20 |
| 40 | 20 | 3 | 0.902 | 9.17 | 0.973 | 4.89 |
| 20 | 10 | 3 | 0.156 | 35.4 | 0.910 | 4.72 |
| 20 | 30 | 3 | 0.714 | 8.04 | 0.991 | 3.22 |
| 20 | 20 | 6 | 0.343 | 20.6 | 0.949 | 2.75 |
| 20 | 20 | 9 | 0.315 | 27.7 | 0.980 | 2.46 |
| C0 (mg/L) | Q (mL/min) | Z (cm) | a | qYa (mg/g) | R2 |
|---|---|---|---|---|---|
| 20 | 20 | 3 | 5.00 | 3.01 | 0.993 |
| 30 | 20 | 3 | 5.44 | 3.97 | 0.958 |
| 40 | 20 | 3 | 5.51 | 4.59 | 0.959 |
| 20 | 10 | 3 | 3.88 | 3.76 | 0.972 |
| 20 | 30 | 3 | 4.27 | 2.87 | 0.913 |
| 20 | 20 | 6 | 4.77 | 5.08 | 0.978 |
| 20 | 20 | 9 | 5.05 | 7.26 | 0.952 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Z.; Zheng, S.; Zhang, D. Al-Impregnated Granular Activated Carbon for Removal of Fluoride from Aqueous Solution: Batch and Fixed-Bed Column Study. Water 2022, 14, 3554. https://doi.org/10.3390/w14213554
Liu Z, Zheng S, Zhang D. Al-Impregnated Granular Activated Carbon for Removal of Fluoride from Aqueous Solution: Batch and Fixed-Bed Column Study. Water. 2022; 14(21):3554. https://doi.org/10.3390/w14213554
Chicago/Turabian StyleLiu, Zheng, Sijie Zheng, and Daolong Zhang. 2022. "Al-Impregnated Granular Activated Carbon for Removal of Fluoride from Aqueous Solution: Batch and Fixed-Bed Column Study" Water 14, no. 21: 3554. https://doi.org/10.3390/w14213554
APA StyleLiu, Z., Zheng, S., & Zhang, D. (2022). Al-Impregnated Granular Activated Carbon for Removal of Fluoride from Aqueous Solution: Batch and Fixed-Bed Column Study. Water, 14(21), 3554. https://doi.org/10.3390/w14213554





