Turning Pools in Stepped Fishways: Biological Assessment via Fish Response and CFD Models
Abstract
1. Introduction
2. Materials and Methods
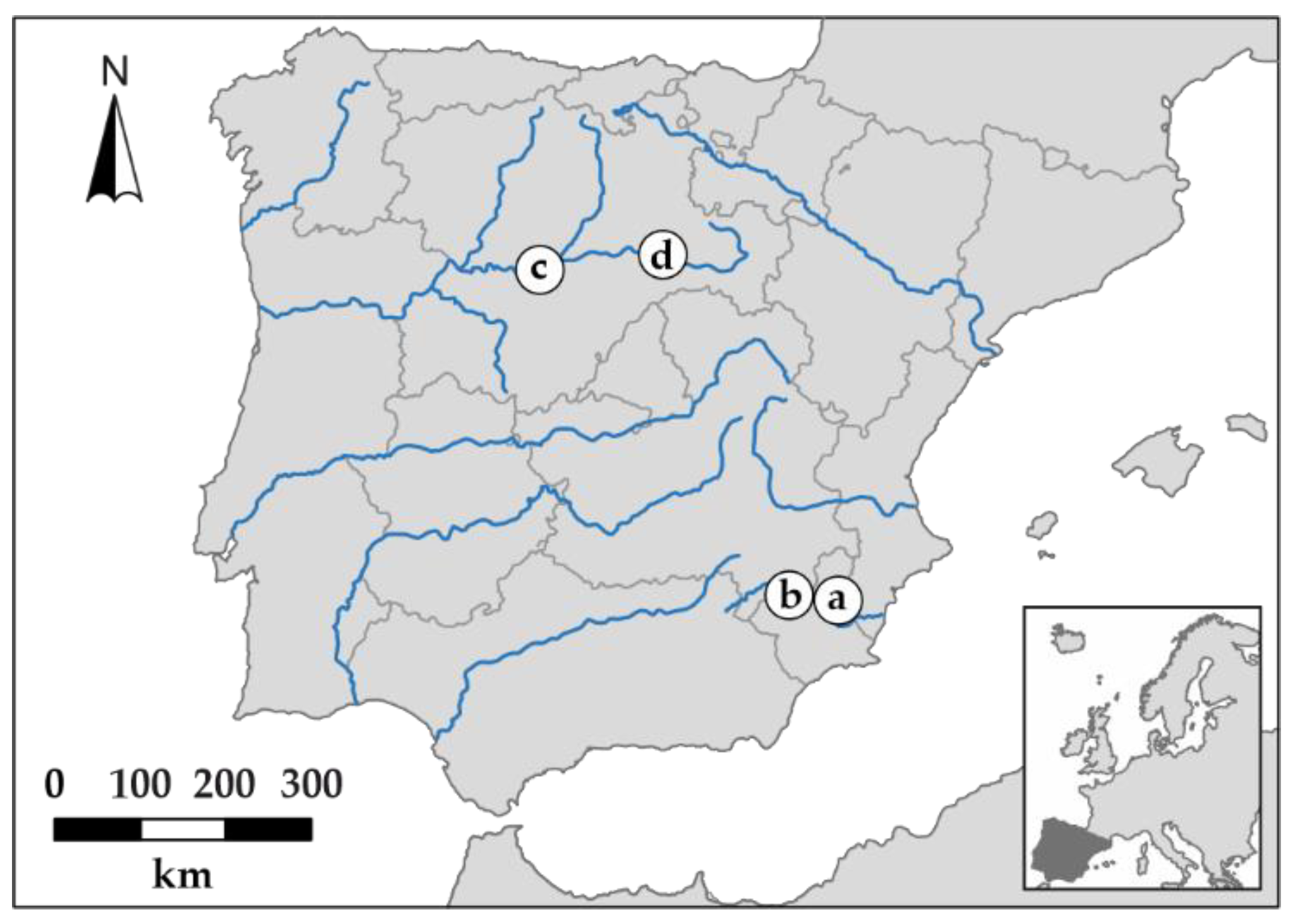
2.1. Study Sites
2.2. 3D Numerical Model
2.2.1. CFD Methods
2.2.2. Mesh, Boundary Conditions, and Time Sensitivity Analysis
2.2.3. CFD Data Treatment and Hydraulic Variables
2.3. Fish Response
2.3.1. Fish Collection and Tagging
2.3.2. Data Collection
- Only those fish with attempts (i.e., at least with one record on the first antenna) were included.
- For transit time calculation, only ascent events with success were taken into account (a successful event was considered when a fish reached the uppermost antenna).
- Transit time in each section was calculated as the time taken from the first detection on the most downstream antenna to the first detection on the most upstream antenna (from antenna 1 to 2 for turning pools and from antenna 2 to 3 for straight sections).
- To avoid the possible effect of fish with multiple events (mainly in free trials related to fish that used the fishway as a daily habitat), when a fish had more than one successful event, only the one with the fastest transit time was selected, resulting in one event per fish.
- To make possible the comparisons among both fishway sections (straight section vs. turning pool) and different field test cases, data of transit time were relativized by the water height difference between antennas, resulting in a transit time per meter of height ascended (min/m).
2.3.3. Data Analysis
3. Results
3.1. 3D Numerical Models
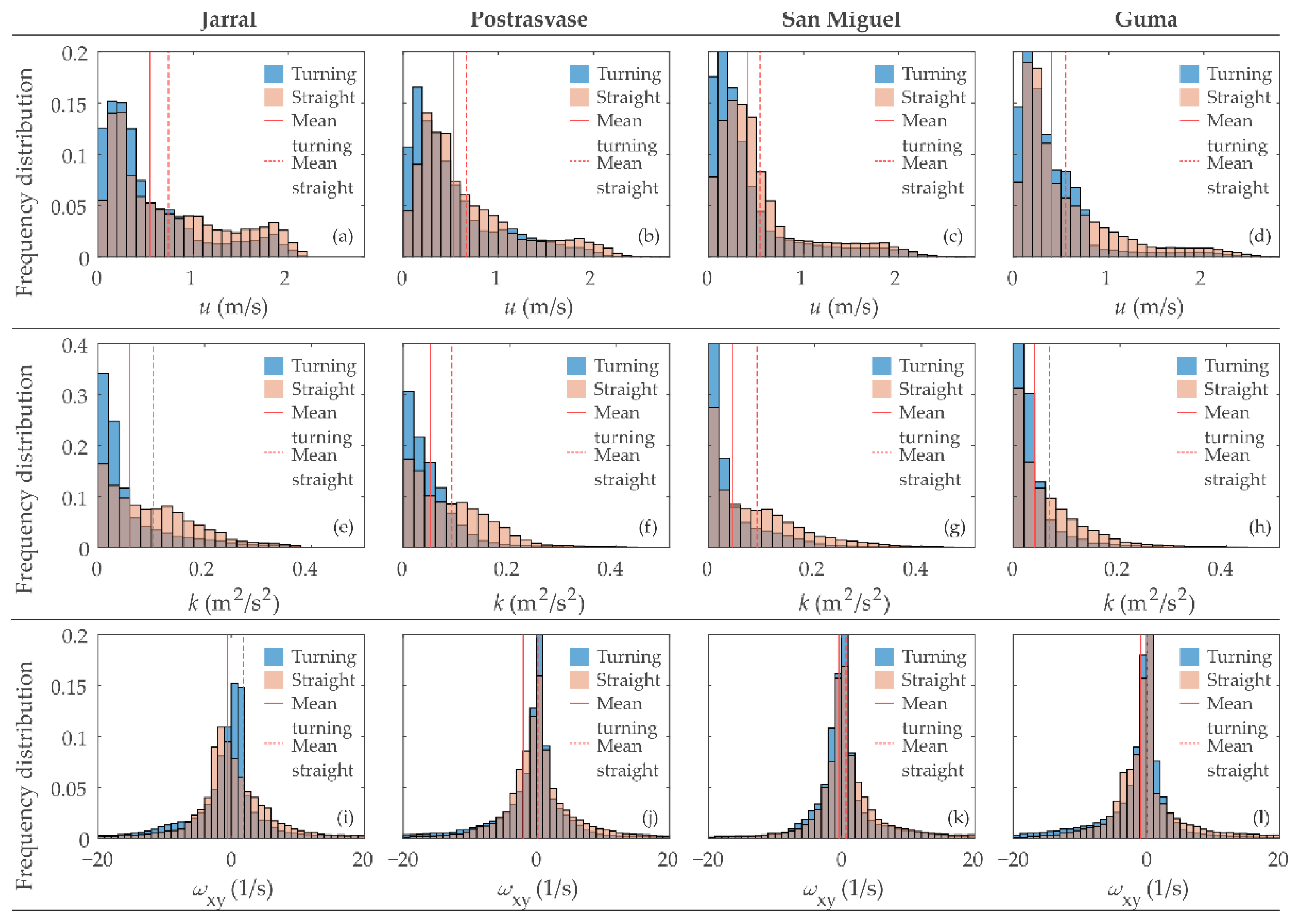
3.2. Fish Response
4. Discussion
4.1. Numerical Models
4.2. Fish Responses
5. Summary and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1. Velocity Contours
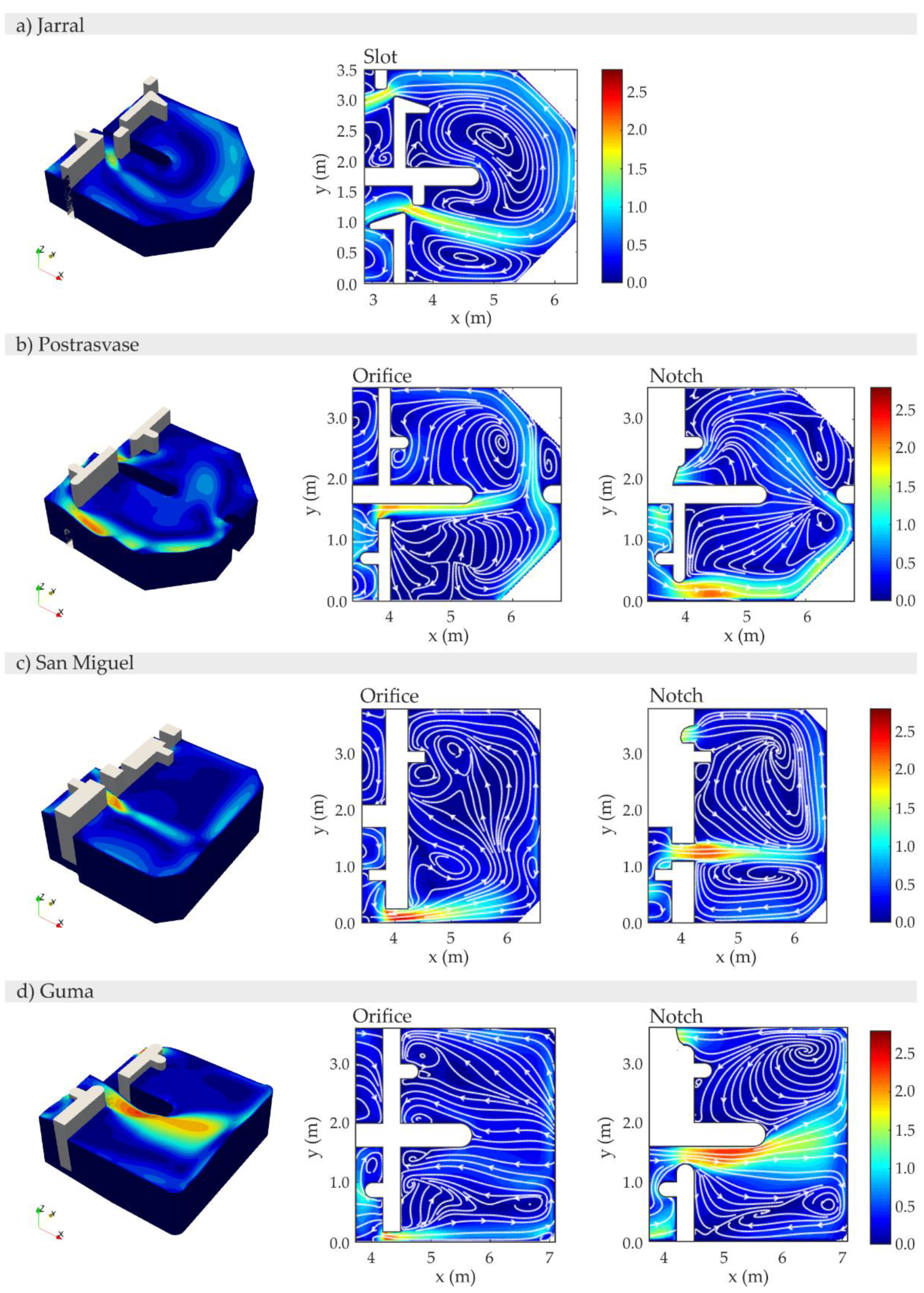
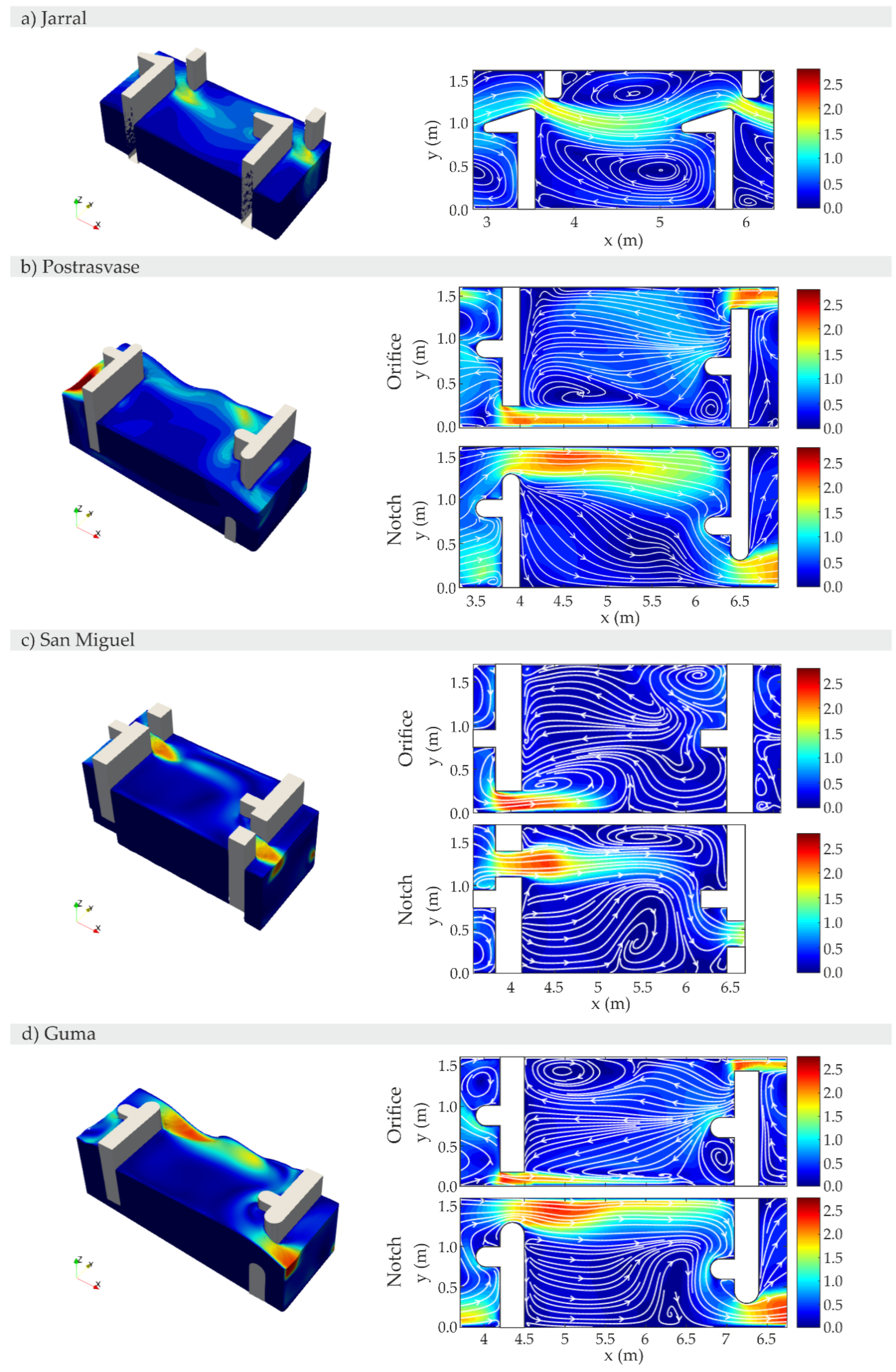
Appendix A.2. Turbulent Kinetic Energy Contours
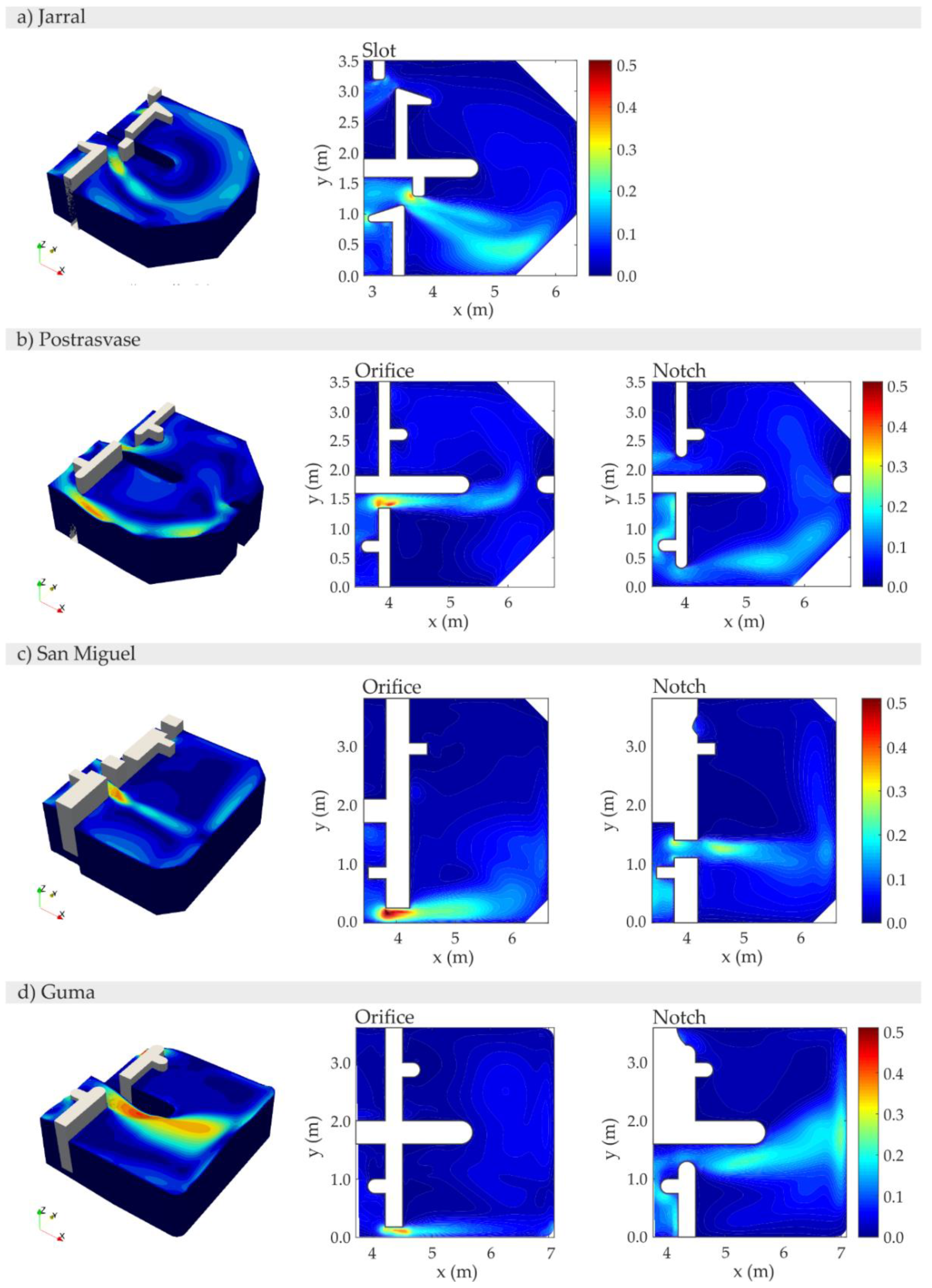
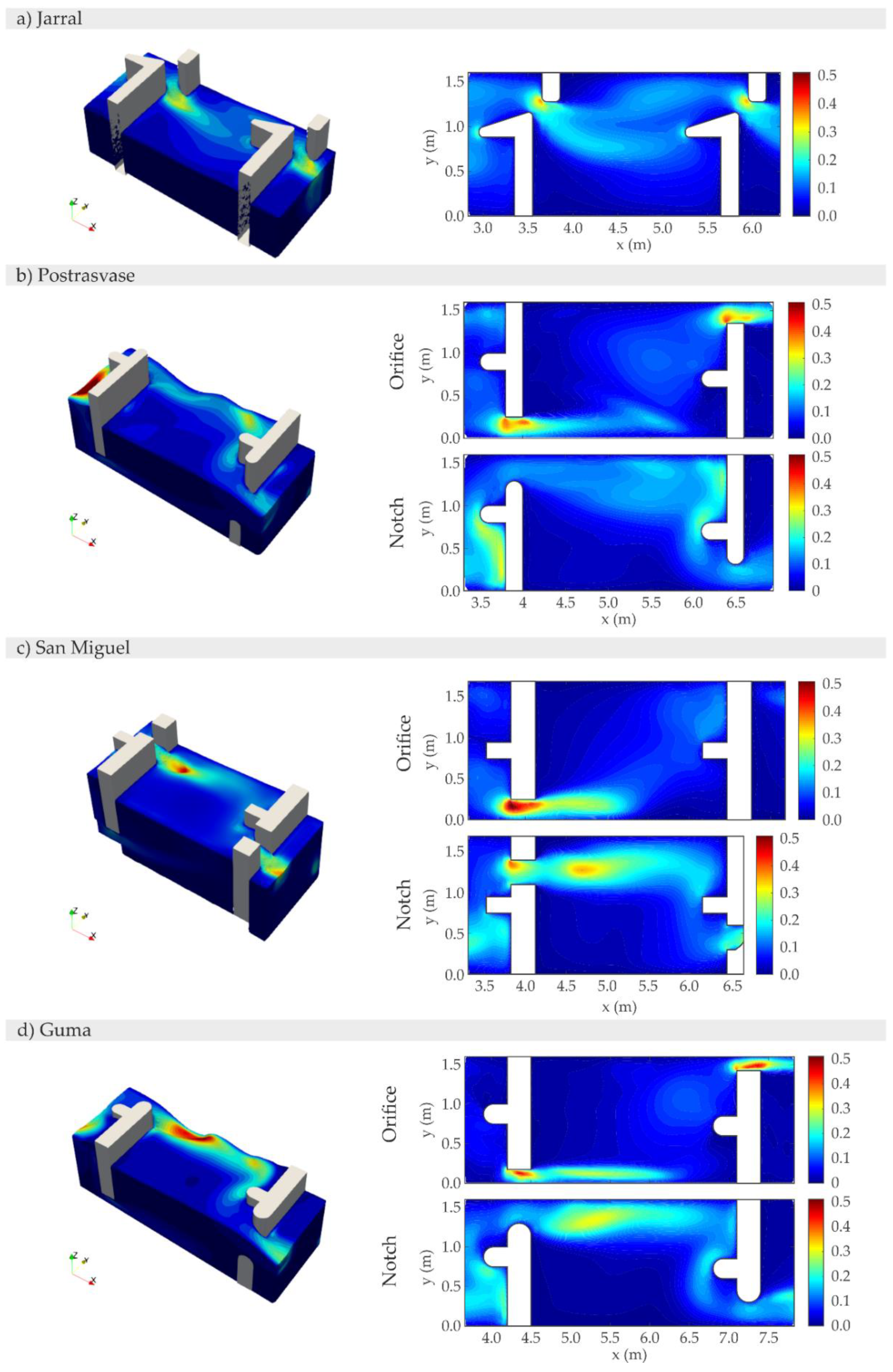
Appendix A.3. Vorticity Contours
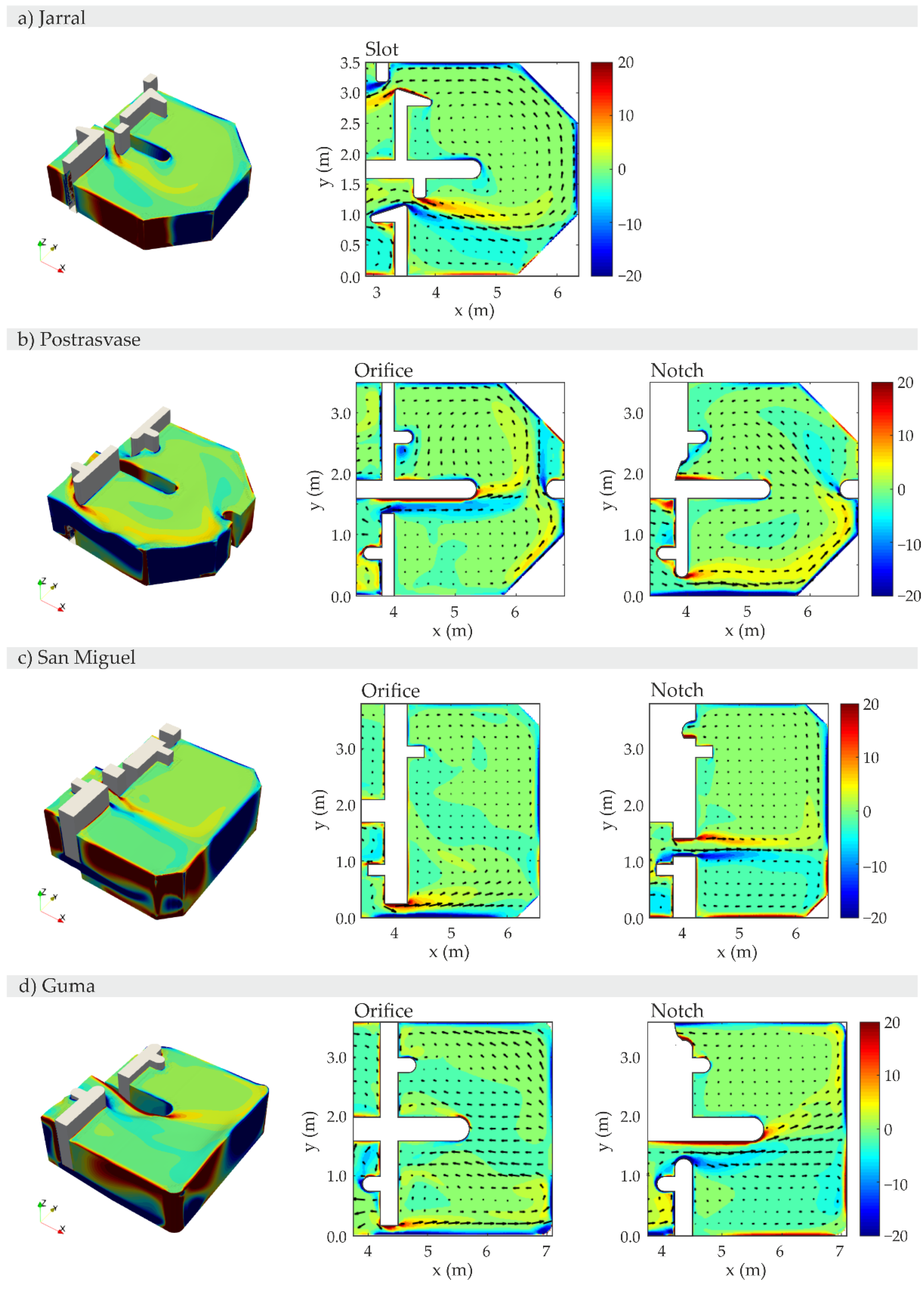

Appendix B
Appendix B.1. Velocity (m/s)
Appendix B.2. Turbulence kinetic energy definition (m2/s2 = J/kg)
Appendix B.3. Vorticity definition (s−1)
References
- Clay, C.H.; Eng, P. Design of Fishways and Other Fish. Facilities; Apple Academic Press: Palm Bay, FL, USA, 2017. [Google Scholar]
- Silva, A.T.; Lucas, M.C.; Castro-Santos, T.; Katopodis, C.; Baumgartner, L.J.; Thiem, J.D.; Aarestrup, K.; Pompeu, P.S.; O’Brien, G.C.; Braun, D.C.; et al. The future of fish passage science, engineering, and practice. Fish. Fish. 2018, 19, 340–362. [Google Scholar] [CrossRef]
- FAO/DVWK Fish Passes: Design, Dimensions, and Monitoring; FAO: Rome, Italy, 2002; ISBN 9251048940.
- Santos, J.M.; Silva, A.; Katopodis, C.; Pinheiro, P.; Pinheiro, A.; Bochechas, J.; Ferreira, M.T. Ecohydraulics of pool-type fishways: Getting past the barriers. Ecol. Eng. 2012, 48, 38–50. [Google Scholar] [CrossRef]
- Larinier, M. Pool Fishways, Pre-Barrages and Natural Bypass Channels. Bull. Fr. Pêche Piscic. 2002, 364, 54–82. [Google Scholar] [CrossRef]
- Martínez de Azagra, A. Escalas Para Peces; ETSIIAA; Universidad de Valladolid: Palencia, Spain, 1999. [Google Scholar]
- Rajaratnam, N.; Katopodis, C.; Solanki, S. New designs for vertical slot fishways. Can. J. Civ. Eng. 1992, 19, 402–414. [Google Scholar] [CrossRef]
- Fuentes-Pérez, J.F.; Sanz-Ronda, F.J.; Paredes, A.M.D.A.; García-Vega, A. Modeling Water-Depth Distribution in Vertical-Slot Fishways under Uniform and Nonuniform Scenarios. J. Hydraul. Eng. 2014, 140, 06014016. [Google Scholar] [CrossRef]
- Fuentes-Pérez, J.F.; Sanz-Ronda, F.J.; De Azagra, A.M.; García-Vega, A. Non-uniform hydraulic behavior of pool-weir fishways: A tool to optimize its design and performance. Ecol. Eng. 2016, 86, 5–12. [Google Scholar] [CrossRef]
- Rajaratnam, N.; Van Der Vinne, G.; Katopodis, C. Hydraulics of Vertical Slot Fishways. J. Hydraul. Eng. 1986, 112, 909–927. [Google Scholar] [CrossRef]
- Puertas, J.; Pena, L.; Teijeiro, T. Experimental Approach to the Hydraulics of Vertical Slot Fishways. J. Hydraul. Eng. 2004, 130, 10–23. [Google Scholar] [CrossRef]
- Rajaratnam, N.; Katopodis, C.; Wu, S.; Sabur, M.A. Hydraulics of Resting Pools for Denil Fishways. J. Hydraul. Eng. 1997, 123, 632–638. [Google Scholar] [CrossRef]
- Marriner, B.A.; Baki, A.B.M.; Zhu, D.Z.; Thiem, J.D.; Cooke, S.J.; Katopodis, C. Field and numerical assessment of turning pool hydraulics in a vertical slot fishway. Ecol. Eng. 2014, 63, 88–101. [Google Scholar] [CrossRef]
- Marriner, B.A.; Baki, A.B.; Zhu, D.Z.; Cooke, S.J.; Katopodis, C. The hydraulics of a vertical slot fishway: A case study on the multi-species Vianney-Legendre fishway in Quebec, Canada. Ecol. Eng. 2016, 90, 190–202. [Google Scholar] [CrossRef]
- Fish, U.S.; Service, W. Fish Passage Engineering Design Criteria; USFWS Northeast Reg.: Hadley, MA, USA, 2017.
- Cooke, S.J.; Cech, J.J.; Glassman, D.M.; Simard, J.; Louttit, S.; Lennox, R.J.; Cruz-Font, L.; O’Connor, C.M. Water resource development and sturgeon (Acipenseridae): State of the science and research gaps related to fish passage, entrainment, impingement and behavioural guidance. Rev. Fish. Biol. Fish. 2020, 30, 219–244. [Google Scholar] [CrossRef]
- Wang, R.; David, L.; Larinier, M. Contribution of experimental fluid mechanics to the design of vertical slot fish passes. Knowl. Manag. Aquat. Ecosyst. 2010, 396, 2. [Google Scholar] [CrossRef]
- Silva, A.T.; Katopodis, C.; Santos, J.M.; Ferreira, M.T.; Pinheiro, A.N. Cyprinid swimming behaviour in response to turbulent flow. Ecol. Eng. 2012, 44, 314–328. [Google Scholar] [CrossRef]
- Fuentes-Pérez, J.F.; Eckert, M.; Tuhtan, J.A.; Ferreira, M.T.; Kruusmaa, M.; Branco, P. Spatial preferences of Iberian barbel in a vertical slot fishway under variable hydrodynamic scenarios. Ecol. Eng. 2018, 125, 131–142. [Google Scholar] [CrossRef]
- Bravo-Córdoba, F.J.; Valbuena-Castro, J.; García-Vega, A.; Fuentes-Pérez, J.F.; Ruiz-Legazpi, J.; Sanz-Ronda, F.J. Fish passage assessment in stepped fishways: Passage success and transit time as standardized metrics. Ecol. Eng. 2021, 162, 106172. [Google Scholar] [CrossRef]
- Bunt, C.M.; Cooke, S.J.; McKinley, R.S. Assessment of the Dunnville Fishway for Passage of Walleyes from Lake Erie to the Grand River, Ontario. J. Great Lakes Res. 2000, 26, 482–488. [Google Scholar] [CrossRef]
- Pon, L.B.; Cooke, S.J.; Hinch, S.G. Passage Efficiency and Migration Behaviour of Salmonid Fishes at the Seton Dam Fishway; Department of Forest Sciences: Vancouver, BC, Canada, 2006. [Google Scholar]
- Silva, A.T.; Hatry, C.; Thiem, J.D.; Gutowsky, L.F.G.; Hatin, D.; Zhu, D.Z.; Dawson, J.W.; Katopodis, C.; Cooke, S.J. Behaviour and Locomotor Activity of a Migratory Catostomid during Fishway Passage. PLoS ONE 2015, 10, e0123051. [Google Scholar] [CrossRef] [PubMed]
- Thiem, J.D.; Dawson, J.W.; Hatin, D.; Danylchuk, A.J.; Dumont, P.; Gleiss, A.C.; Wilson, R.P.; Cooke, S.J. Swimming activity and energetic costs of adult lake sturgeon during fishway passage. J. Exp. Biol. 2016, 219, 2534–2544. [Google Scholar] [CrossRef] [PubMed]
- Thiem, J.; Binder, T.; Dawson, J.; Dumont, P.; Hatin, D.; Katopodis, C.; Zhu, D.; Cooke, S.; Thiem, J. Behaviour and passage success of upriver-migrating lake sturgeon Acipenser fulvescens in a vertical slot fishway on the Richelieu River, Quebec, Canada. Endanger. Species Res. 2011, 15, 1–11. [Google Scholar] [CrossRef]
- Noonan, M.J.; Grant, J.W.; Jackson, C.D. A quantitative assessment of fish passage efficiency. Fish. Fish. 2011, 13, 450–464. [Google Scholar] [CrossRef]
- Bunt, C.M.; Castro-Santos, T.; Haro, A. Reinforcement and Validation of the Analyses and Conclusions Related to Fishway Evaluation Data from Bunt Performance of Fish Passage Structures at Upstream Barriers to Migratio. River Res. Appl. 2016, 32, 2125–2137. [Google Scholar] [CrossRef]
- Castro-Santos, T.; Cotel, A.; Webb, P. Fishway Evaluations for Better Bioengineering: An Integrative Approach A Framework for Fishway. Am. Fish. Soc. Symp. 2009, 69, 557–575. [Google Scholar]
- Bravo-Córdoba, F.J.; Sanz-Ronda, F.J.; Ruiz-Legazpi, J.; Valbuena-Castro, J.; Makrakis, S. Vertical slot versus submerged notch with bottom orifice: Looking for the best technical fishway type for Mediterranean barbels. Ecol. Eng. 2018, 122, 120–125. [Google Scholar] [CrossRef]
- Córdoba, F.J.B.; Sanz-Ronda, F.; Navas-Pariente, A.; Valbuena-Castro, J. Comportamiento del barbo ibérico (Luciobarbus bocagei) en una escala de peces de vertedero sumergido con orificio de fondo. Cuad. Soc. Esp. Cienc. For. 2019, 45, 231–240. [Google Scholar] [CrossRef]
- Sanz-Ronda, F.J.; Bravo-Córdoba, F.J.; Sánchez-Pérez, A.; García-Vega, A.; Valbuena-Castro, J.; Fernandes-Celestino, L.; Torralva, M.; Oliva-Paterna, F.J. Passage Performance of Technical Pool-Type Fishways for Potamodromous Cyprinids: Novel Experiences in Semiarid Environments. Water 2019, 11, 2362. [Google Scholar] [CrossRef]
- Ubbink, O. Numerical Prediction of Two Fluid Systems with Sharp Interfaces; University of London: London, UK, 1997. [Google Scholar]
- Hirt, C.; Nichols, B. Volume of fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Fuentes-Pérez, J.; Silva, A.; Tuhtan, J.; García-Vega, A.; Carbonell-Baeza, R.; Musall, M.; Kruusmaa, M. 3D modelling of non-uniform and turbulent flow in vertical slot fishways. Environ. Model. Softw. 2018, 99, 156–169. [Google Scholar] [CrossRef]
- Barton, A.F.; Keller, R.J.; Katopodis, C. Verification of a numerical model for the prediction of low slope vertical slot fishway hydraulics. Australas. J. Water Resour. 2009, 13, 53–60. [Google Scholar] [CrossRef]
- Bombač, M.; Novak, G.; Rodic, P.; Četina, M. Numerical and physical model study of a vertical slot fishway. J. Hydrol. Hydromech. 2014, 62, 150–159. [Google Scholar] [CrossRef]
- Cea, L.; Pena, L.; Puertas, J.; Vázquez-Cendón, M.E.; Peña, E. Application of Several Depth-Averaged Turbulence Models to Simulate Flow in Vertical Slot Fishways. J. Hydraul. Eng. 2007, 133, 160–172. [Google Scholar] [CrossRef]
- Greenshields, C.J. The Open Source CFD Toolbox User Guide; OpenFOAM Foundation Ltd.: London, UK, 2015. [Google Scholar]
- Quaresma, A.L.; Romão, F.; Branco, P.; Ferreira, M.T.; Pinheiro, A.N. Multi slot versus single slot pool-type fishways: A modelling approach to compare hydrodynamics. Ecol. Eng. 2018, 122, 197–206. [Google Scholar] [CrossRef]
- Fuentes-Pérez, J.F.; García-Vega, A.; Sanz-Ronda, F.J.; Paredes, A.M.D.A. Villemonte’s approach: A general method for modeling uniform and non-uniform performance in stepped fishways. Knowl. Manag. Aquat. Ecosyst. 2017, 418, 23. [Google Scholar] [CrossRef]
- Doadrio, I. Atlas y Libro Rojo de los Peces Continentales de España; Ministerio de Medio Ambiente: Madrid, Spain, 2002; ISBN 84-8014-313-4.
- Rodriguez-Ruiz, A.; Granado-Lorencio, C. Spawning period and migration of three species of cyprinids in a stream with Mediterranean regimen (SW Spain). J. Fish. Biol. 1992, 41, 545–556. [Google Scholar] [CrossRef]
- García-Vega, A.; Fuentes-Pérez, J.F.; Bravo-Córdoba, F.J.; Ruiz-Legazpi, J.; Valbuena-Castro, J.; Sanz-Ronda, F.J. Pre-reproductive movements of potamodromous cyprinids in the Iberian Peninsula: When environmental variability meets semipermeable barriers. Hydrobiology 2021, 1–22. [Google Scholar] [CrossRef]
- Sanz-Ronda, F.J.; Ruiz-Legazpi, J.; Bravo-Córdoba, F.J.; Makrakis, S.; Castro-Santos, T. Sprinting performance of two Iberian fish: Luciobarbus bocagei and Pseudochondrostoma duriense in an open channel flume. Ecol. Eng. 2015, 83, 61–70. [Google Scholar] [CrossRef]
- Ruiz-Legazpi, J.; Sanz-Ronda, F.J.; Bravo-córdoba, F.J.; Fuentes-Pérez, J.F.; Castro-Santos, T. Influence of environmental and biometric factors on the swimming capacity of the Iberian barbel (Luciobarbus bocageiSteindachner, 1864), an endemic po-tamodromous cyprinid of the Iberian Peninsula. Limnetica 2018, 37, 251–265. [Google Scholar] [CrossRef]
- Tarrade, L.; Texier, A.; David, L.; Larinier, M. Topologies and measurements of turbulent flow in vertical slot fishways. Hydrobiology 2008, 609, 177–188. [Google Scholar] [CrossRef]
- Liao, J.C. A review of fish swimming mechanics and behaviour in altered flows. Philos. Trans. R. Soc. B Biol. Sci. 2007, 362, 1973–1993. [Google Scholar] [CrossRef] [PubMed]
- Towler, B.; Mulligan, K.; Haro, A. Derivation and application of the energy dissipation factor in the design of fishways. Ecol. Eng. 2015, 83, 208–217. [Google Scholar] [CrossRef]
- Alexandre, C.; Quintella, B.; Silva, A.; Mateus, C.; Romão, F.; Branco, P.; Ferreira, M.; Almeida, P. Use of electromyogram telemetry to assess the behavior of the Iberian barbel (Luciobarbus bocagei Steindachner, 1864) in a pool-type fishway. Ecol. Eng. 2013, 51, 191–202. [Google Scholar] [CrossRef]
- Pavlov, D.; Lupandin, A.; Skorobogatov, M. The Effects of Flow Turbulence on the Behavior and Distribution of Fish. J. Ich-thyol. 2000, 20, 232–261. [Google Scholar]
- Tritico, H.M.; Cotel, A.J. The effects of turbulent eddies on the stability and critical swimming speed of creek chub (Semotilus atromaculatus). J. Exp. Biol. 2010, 213, 2284–2293. [Google Scholar] [CrossRef] [PubMed]
- Liao, J.C. Neuromuscular control of trout swimming in a vortex street: Implications for energy economy during the Karman gait. J. Exp. Biol. 2004, 207, 3495–3506. [Google Scholar] [CrossRef] [PubMed]
- Haro, A.; Castro-Santos, T. Passage of American Shad: Paradigms and Realities. Mar. Coast. Fish. 2012, 4, 252–261. [Google Scholar] [CrossRef]
- White, L.J.; Harris, J.H.; Keller, R.J. Movement of three non-salmonid fish species through a low-gradient vertical-slot fishway. River Res. Appl. 2011, 27, 499–510. [Google Scholar] [CrossRef]
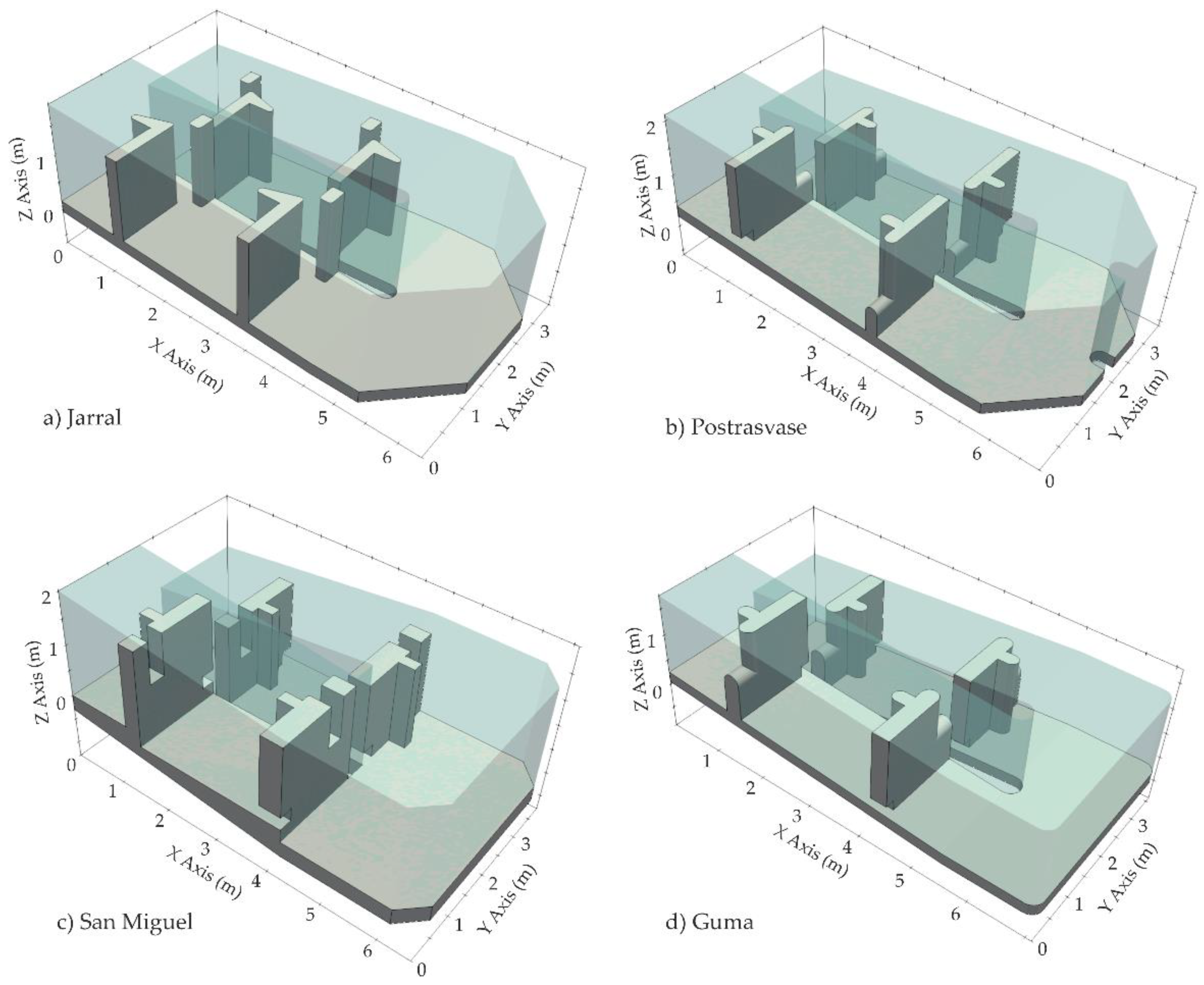
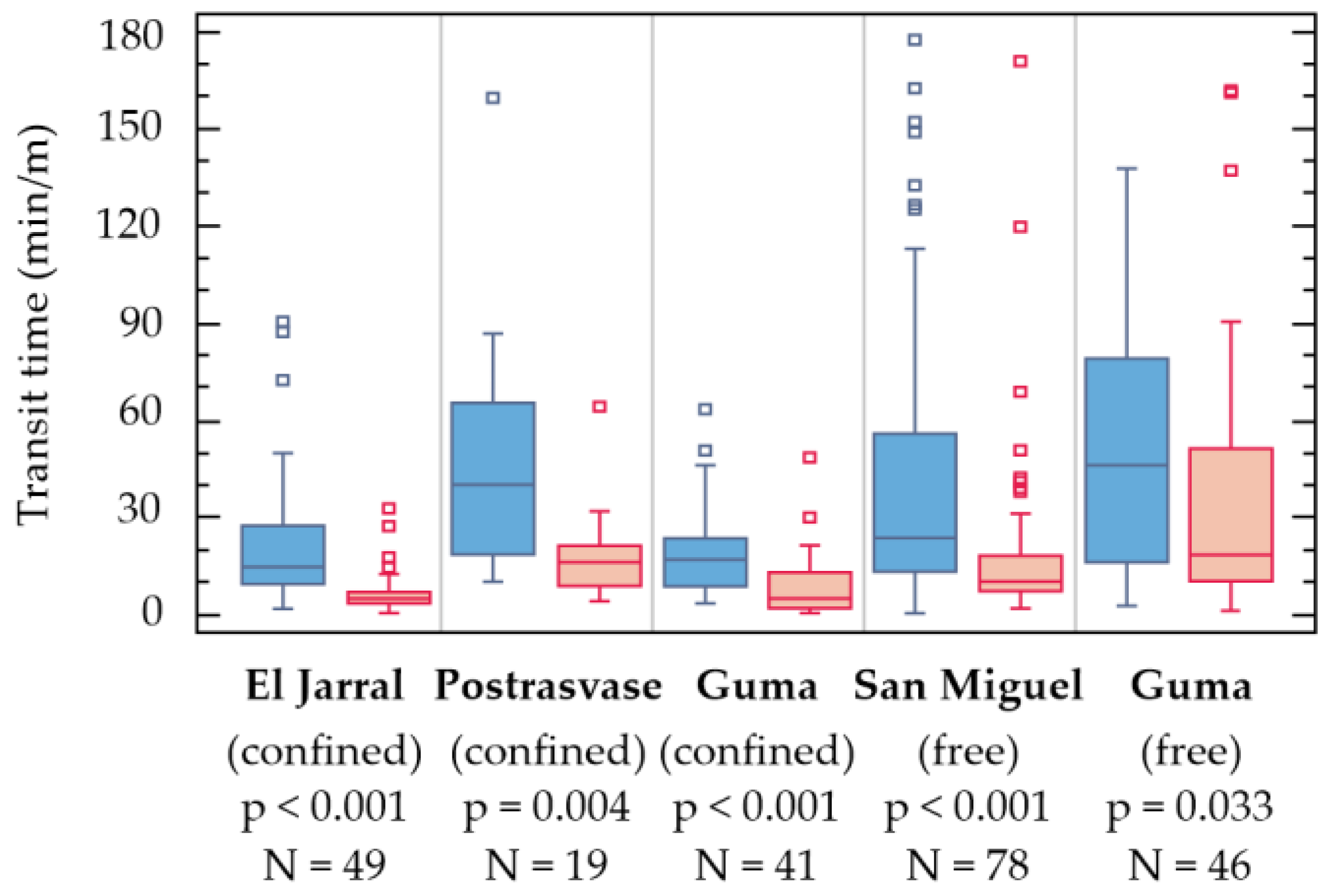
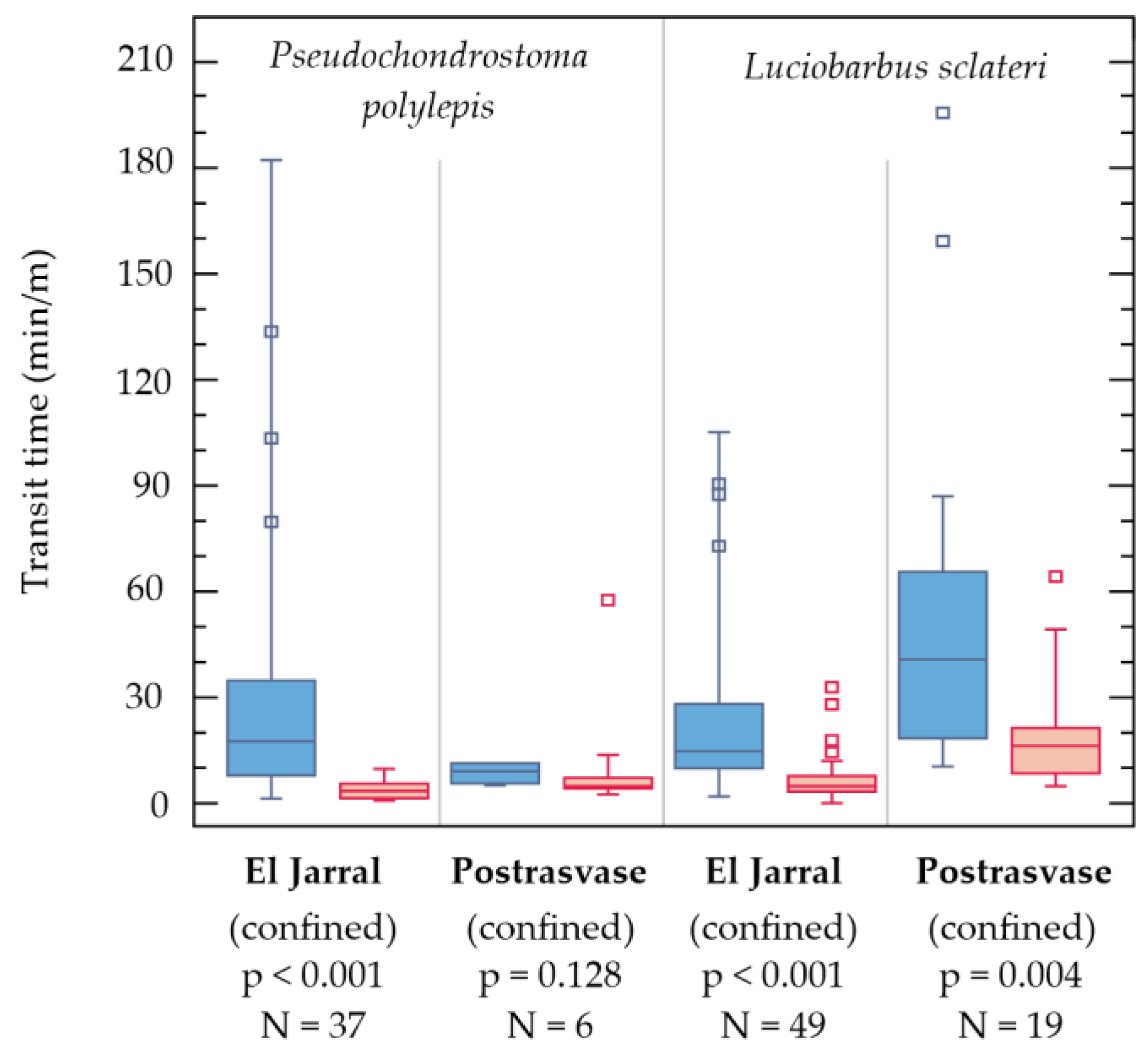
| Variables | El Jarral (VS) | Postrasvase (SNBO) | San Miguel (SNBO) | Guma (SNBO) |
|---|---|---|---|---|
| Pool dimension (length × width) | 2.10 × 1.60 m | 2.40 × 1.60 m | 2.40 × 1.70 m | 2.60 × 1.60 m |
| Slope | 6.52% | 7.31% | 9.39% | 8.77% |
| Width of the slot/notch 1 | 0.21 m | 0.31 m | 0.30 m | 0.30 m |
| Bottom orifice size (length × width) 1 | NA | 0.20 × 0.25 m | 0.25 × 0.25 m | 0.18 × 0.18 m |
| Sill height 1 | NA | 0.50 m | 0.85 m | 0.80 m |
| Test Case | Fishway Type | Trial Condition | Species | N | FL (mm) | |
|---|---|---|---|---|---|---|
| Mean ± C.I. | Range | |||||
| El Jarral | VS | Confined | L. sclateri P. polylepis | 49 37 | 204 ± 10 151 ± 5 | 135–282 135–198 |
| Postrasvase | SNBO | Confined | L. sclateri P. polylepis | 19 6 | 185 ± 43 160 ± 29 | 128–437 128–196 |
| Guma | SNBO | Confined | L. bocagei | 41 | 180 ± 10 | 128–277 |
| Guma | SNBO | Free | L. bocagei | 46 | 157 ± 12 | 88–293 |
| San Miguel | SNBO | Free | L. bocagei | 78 | 267 ± 16 | 145–470 |
| Name | Q (m3/s) | Curve | Straight | ||||||
|---|---|---|---|---|---|---|---|---|---|
| h0 (m) | ΔH (m) | umax (m/s) | VPD (W/m3) | h0 (m) | ΔH (m) | umax (m/s) | VPD (W/m3) | ||
| Jarral | 0.30 | 0.90 | 0.15 | 2.174 | 58.535 | 0.90 | 0.15 | 2.198 | 147.492 |
| Postrasvase | 0.31 | 1.00 | 0.20 | 2.450 | 73.128 | 0.98 | 0.20 | 2.739 | 162.143 |
| San Miguel | 0.28 | 1.31 | 0.25 | 2.775 | 59.837 | 1.30 | 0.25 | 2.642 | 132.740 |
| Guma | 0.25 | 1.17 | 0.224 | 2.820 | 48.869 | 1.15 | 0.25 | 2.792 | 115.288 |
| Species | Trial Conditions | Test Cases | Passage Success in Turning Pool 1 | Transit Time Turning Pool | Transit Time Straight Section |
|---|---|---|---|---|---|
| Barbel | Confined | Jarral | 98.3% a | 15.1 (9.7–27.7) a | 5.1 (3.5–7.6) a |
| Postrasvase | 86.7% a | 40.4 (18.3–65.9) b | 16.6 (8.8–21.5) b | ||
| Guma | 100% a | 16.7 (8.6–24.0) a | 5.2 (2.1–13.1) a | ||
| Free | San Miguel | 92.2% a | 23.8 (13.5–56.3) a | 10.6 (7.1–18.7) a | |
| Guma | 96.1% a | 46.5 (16.1–79.3) a | 18.9 (10.4–51.6) b | ||
| Nase | Confined | Jarral | 100% a | 17.9 (7.9–35.2) a | 3.2 (1.4–5.8) a |
| Postrasvase | 87.5% a | 8.9 (5.4–11.5) a | 4.7 (4.4–6.9) a |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bravo-Córdoba, F.J.; Fuentes-Pérez, J.F.; Valbuena-Castro, J.; Martínez de Azagra-Paredes, A.; Sanz-Ronda, F.J. Turning Pools in Stepped Fishways: Biological Assessment via Fish Response and CFD Models. Water 2021, 13, 1186. https://doi.org/10.3390/w13091186
Bravo-Córdoba FJ, Fuentes-Pérez JF, Valbuena-Castro J, Martínez de Azagra-Paredes A, Sanz-Ronda FJ. Turning Pools in Stepped Fishways: Biological Assessment via Fish Response and CFD Models. Water. 2021; 13(9):1186. https://doi.org/10.3390/w13091186
Chicago/Turabian StyleBravo-Córdoba, Francisco Javier, Juan Francisco Fuentes-Pérez, Jorge Valbuena-Castro, Andrés Martínez de Azagra-Paredes, and Francisco Javier Sanz-Ronda. 2021. "Turning Pools in Stepped Fishways: Biological Assessment via Fish Response and CFD Models" Water 13, no. 9: 1186. https://doi.org/10.3390/w13091186
APA StyleBravo-Córdoba, F. J., Fuentes-Pérez, J. F., Valbuena-Castro, J., Martínez de Azagra-Paredes, A., & Sanz-Ronda, F. J. (2021). Turning Pools in Stepped Fishways: Biological Assessment via Fish Response and CFD Models. Water, 13(9), 1186. https://doi.org/10.3390/w13091186









