1. Introduction
Embankment dams, constructed with locally excavated earth or rockfill represent 78% of the total number of existing dams worldwide [
1]. Embankment dams comprising of coarse rockfill materials in more than 50% of the dam volume are defined as rockfill dams and represent 13% of the worldwide dam population [
1]. A rockfill dam structure generally consists of an impervious element, filter zones, support fill and some means of controlling the development of phreatic surface and seepage through the dam structure.
The issue of dam safety has gained much attention in the recent past. Stringent measures are being put in place by the respective national dam safety authorities to ensure safety of dams. Although there exists significant amount of accumulated scientific literature within the research discipline of embankment dams in general, technical literature describing throughflow behavior of rockfill dams is scarce. This article aims at adding to the research discipline of rockfill dam safety. Dam safety assessment is a complex task, as it is influenced by multitudes of internal and external factors [
2]. It is essential to determine the most common causes of dam failures to identify probable factors which commonly contribute to dam instability. Statistics from the International Commission on Large Dams (ICOLD) state overtopping as the main cause of embankment dam failure appearing as the primary factor in 31% of the total number of failures, and is further involved in another 18% of failures as a secondary agent [
3]. Hence, equipping embankment dams with defense mechanisms against unanticipated overtopping or leakage events is of importance from a dam safety perspective. This includes safeguarding against accidental throughflow conditions arising when the core of a rockfill dam is overtopped, resulting in turbulent flow within the downstream dam shoulder, as shown in
Figure 1b.
The rockfill toe structure situated within the downstream slope of rockfill dams can be considered as an integral part of a defense mechanism installed to protect the dam structure under normal seepage situation as described by
Figure 1a, as well as under accidental overtopping situations leading to extreme throughflow conditions as shown in
Figure 1b. In fact, some previous studies into embankment dam failures describe the downstream toe as a critical location for failure initiation under throughflow scenarios [
4,
5,
6,
7,
8]. Furthermore, findings of Toledo and Morera [
9] and Moran and Toledo [
10] suggest that rockfill toes may be used as effective protection against throughflow in rockfill dams. Furthermore, Moran et al. [
11] present a procedure for the design of external toe protection for rockfill embankments.
A recent investigation conducted by Ravindra [
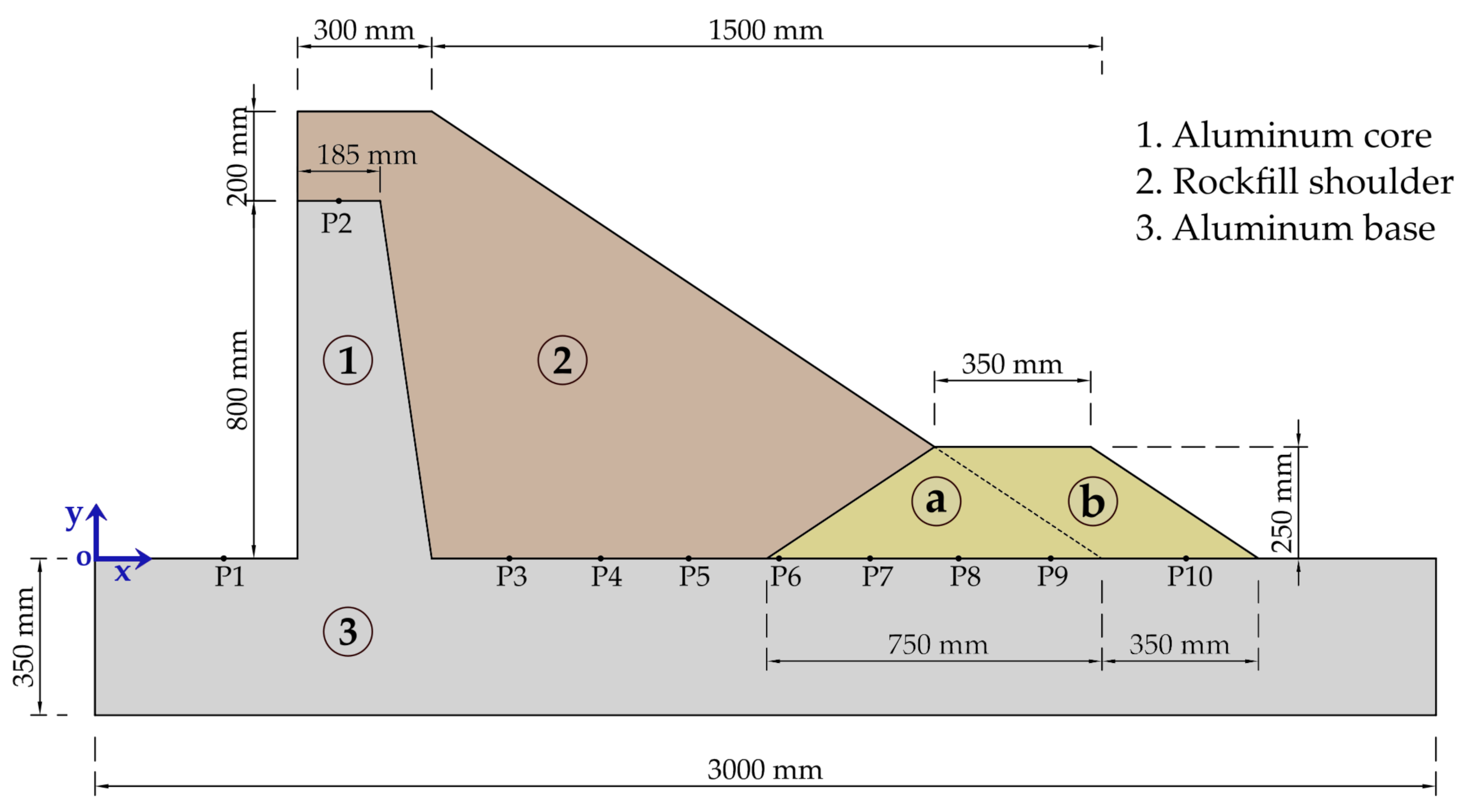
12] studied the effects of various configurations of rockfill toes on throughflow development within hydraulic scale models of rockfill dams (
Figure 2). Findings from the experimental studies, presented by Kiplesund et al. [
13], highlight the fact that toe configuration can have significant impact on the development and progression of phreatic surfaces within rockfill dam models subjected to incremental overtopping scenarios. The toe configurations were also found to influence the stability of the downstream slope. The study gave valuable insight into the significance of rockfill toes with regard to rockfill dam safety. The present study builds numerical models on the data accumulated through the experimental investigations [
12,
13].
The present study is a part of an ongoing research program into dam overtopping and throughflow.
Figure 3 visualizes the modeling strategies of this overarching research program from full-scale to model-scale investigations, as well as numerical modeling efforts. The full-scale part embraces consideration of the design of existing dams [
14,
15], as well as analysis of data accumulated from full-scale tests [
16]. The hydraulic scale models that relate to the numerical modeling of the present study are those presented by Kiplesund et al. [
13]. These considered the scaling of previous hydraulic scale models for investigating riprap erosion protection on the downstream slope of embankment dams [
17,
18,
19]. The combined application of the different modeling strategies is for enhanced applicability and relevance of the research for full-scale dam cases.
The overarching goal of the present part of the research program is to evaluate the hydraulic response of rockfill dams exposed to accidental throughflow scenarios (
Figure 1b) and to study the effects of rockfill toes on throughflow hydraulic properties of rockfill dams. For this purpose, a numerical model is developed for replicating results from physical model tests considering turbulence of the flow. Hence, an important aspect of the present study is the implementation of a geotechnical software [
20] commonly employed in dam engineering for practical applications as well as in research [
21,
22]. However, the modeling usually assumes laminar flow or Darcy flow conditions, suitable for cases as in
Figure 1a. Thus, the present study aims at investigating the ability of a tool provided within such software [
23] to model turbulent or non-Darcy flow commonly encountered in the physical rockfill dam models. This has a relevance when proceeding to numerical models of real dam cases considering non-Darcy flow for the accidental overtopping situation. Moreover, numerical modeling of the effect of various toe configurations on flow through rockfill dams has not been looked into in the past. The datasets gathered through the previously mentioned physical modeling investigations [
12,
13] are used to calibrate numerical models employing the numerical seepage software SEEP/W [
20] with a non-Darcy tool. The aim is to predict the development of throughflow within rockfill dam structures and to numerically model the effect of a drainage component within the downstream dam slope on non-linear throughflow development.
2. Background
Flow through porous media is generally characterized as either Darcy or non-Darcy type based on flow properties. The linear Darcy flow theory is widely implemented in soil mechanics and is described by the following equation:
where the velocity of flow,
v, is described by a linear relationship between hydraulic conductivity,
k, and the hydraulic gradient,
i.
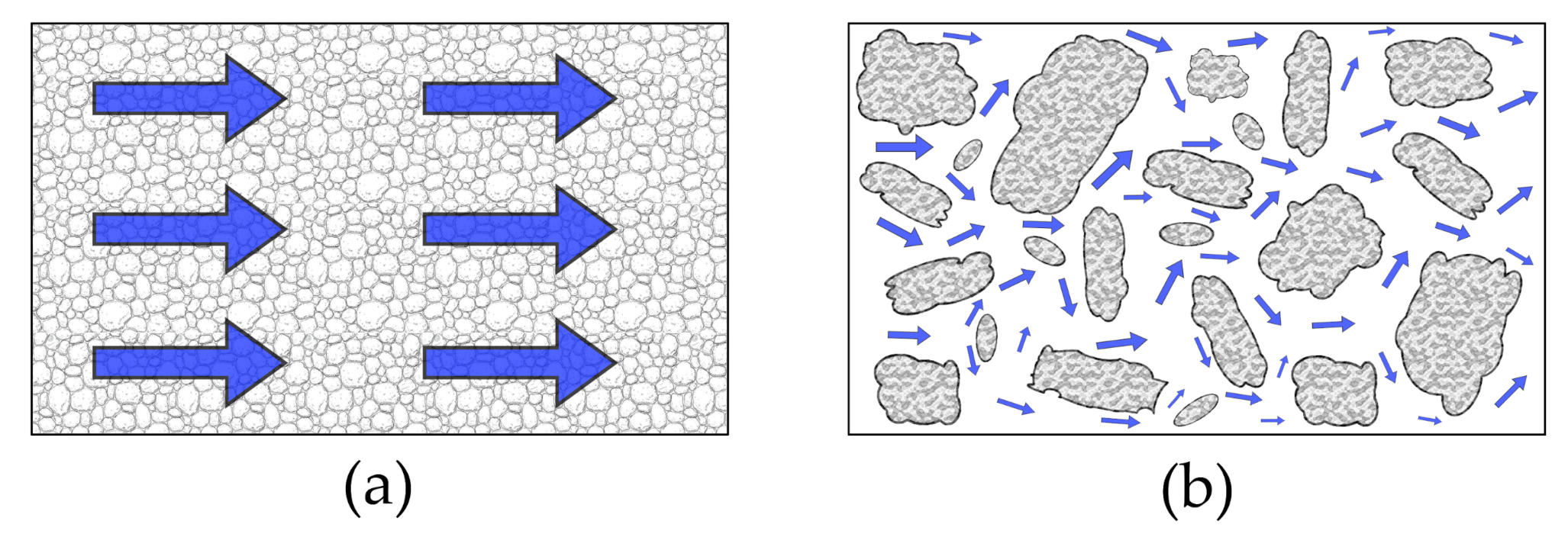
Darcy’s law is only valid at low velocities, i.e., laminar flow. At higher velocities, the inertial forces distort the streamlines and turbulent flow occurs, removing the linear relationship. In rockfill material, the voids are of a magnitude that turbulent flow is expected [
16]. An illustration is made in
Figure 4. Where Darcy’s law is applicable, flow is evenly distributed and laminar. In the rockfill case, the voids are bigger and velocities vary along with the grains redirecting the flow.
Non-Darcian or turbulent flow through porous media is generally represented as a power-law function:
where,
a and
b represent empirical coefficients to be determined experimentally. Coefficient
a depends on the properties of fluid and porous media such as porosity, particle shape, particle size, roughness, tortuosity of void structure and viscosity of fluid. Parameter
b is dependent upon the state of flow or the level of flow turbulence [
8].
Until recently, the performance of the general non-linear flow law of the form presented in Equation (
2) was verified only through experimental studies conducted in permeameters. Past studies have investigated non-linear flow through rockfill medium through elaborate permeameter experimental testing conducted on rockfill with sizes ranging from
mm to 240 mm [
8,
24,
25,
26,
27,
28,
29]. Several empirical relationships describing the non-linear
i-
v flow properties have also been proposed as a result of these investigations. Although a considerable number of investigations have investigated non-Darcian flow through rockfill material in permeameters of varying sizes, experimental validation of these past findings in rockfill embankments exposed to throughflow conditions can be stated as quintessential for validation of past research findings in terms of relevance of application in rockfill dam engineering. A recent study put forth by Ravindra et al. [
16] has made attempts at validating some of the widely employed non-linear flow equations from the past and have also further proposed a new equation applicable for non-linear flow through homogeneous rockfill dams.
Dealing with soil or rockfill, which is generally heterogeneous and discontinuous in nature, approximate solutions are normally pursued [
30]. The finite element method is a powerful tool for approximating complex field problems. The domain in which the analysis is being conducted is divided into finite elements creating a mesh. For each node in the mesh, the field variable is explicitly calculated through a mass balance approach. The functions that define how the field variable varies in the domain are controlled through the material properties. The mass balance approach for the utilized software relevant for this study can be summarized by the following general equation [
20]:
where
is the stored mass in the control volume, the inflow and outflow terms,
and
, represent flow in and out of the control volume and
is the source term, with dot-notation representing rates.
For seepage problems, the governing differential equation utilized by the software in a 2D case is defined by:
where
k is the hydraulic conductivity in x- and y-direction,
H is the total head,
Q is the mass source or sink term. The right side of the equation is the change in volumetric water content,
, with respect to time. The simulations in this study are conducted in a steady-state condition, which yields that there are no time-dependant variables and the right side of Equation (
4) becomes zero.
The finite element method then entails that the governing differential equation must be satisfied at every node; assembled in matrix form, this can be summarized as the finite element equation:
where
K represents the global element matrix, defining each element’s geometry and material properties;
h is the primary unknown vector consisting of the total head at each node. Lastly,
q is the resultant vector also called the nodal flow vector, defined by the boundary conditions. This system of equations is iteratively solved so that each element in addition to the whole domain satisfies the governing equation.
To account for non-linearity of the flow, or non-Darcy flow, an added feature is usually required. Professional packages are available that employ a flux approach where the nonlinear nature of Equation (
2) is relegated to an apparent hydraulic conductivity term,
, by rearranging the equations as follows with the hydraulic gradient expressed in vector notation as
and velocity expressed as a flux vector,
[
23]:
where the apparent hydraulic conductivity,
can be expressed in terms of total head as [
23]:
where
is the form drag constant,
k is the hydraulic conductivity of the porous media,
is fluid density,
g is gravitational acceleration and
is the dynamic viscosity of fluid.
It can be seen from Equation (
7) that the apparent conductivity will decrease with increasing velocity, providing non-linear behavior of the rockfill material. Definition of the parameters that govern the non-linear behavior are based on the closed form equation for hydraulic conductivity derived by Van Genuchten [
31]. The input parameters include, saturated and residual water content,
,
n,
l,
and fluid temperature. Originally developed for agrophysical purposes, the equation builds on the soil water retention curve, which can be established through laboratory testing, to find the relative conductivity between saturated and unsaturated material.
Listing some parameters found for clay to sandstone soils,
n-values ranges from 1.2 to 10 [
31]. In a later study, typical values are presented as 1.2 for fine soils and 2.7 for coarse soil [
32]. For the
-parameter variation lies between 0.01 and 1 for fine material including clay [
33,
34]. There exist multiple studies with varying values for the form drag constant,
, and there are no input limitations in the add-in of the software used [
20]. As a selected limitation for the present study, the drag constant can vary between 0.5 and 1.5 for coarse granular material [
35]. The
l-parameter represents the inter connectivity and tortuosity of the voids in the material, with values ranging from −1 to 2 in different solutions [
32].
Several past studies available in the international literature perform the function of defining turbulent flow through uniform and homogeneous rockfill materials [
8,
16,
24,
25,
26,
27,
28,
29]. However, the validity of these equations as applied to zoned rockfill structures comprising several different materials with varying properties has not been investigated. This can be attributed to the fact that hydraulic throughflow properties in zoned rockfill dam models can be very complex and deriving general results/relationships to describe such behavior can be challenging. Hence, numerical modeling can be considered as a well suited method for investigating such complex hydraulic aspects in rockfill dams. This study aims at employing a numerical model to obtain a representative description of flow through rockfill dam models with two individual zones. This can form a strong launchpad for further developments to the model which can help improve our capabilities to model complex hydraulic behaviors within large scale rockfill dams.
5. Discussion
The results from the numerical analysis demonstrate that turbulent non-Darcy flow through rockfill dam structures can be modelled with good calibration metrics. However, some challenges with the numerical modeling work were encountered. This section discusses these challenges and aims at putting forth recommendations and insights that can potentially supplement further research in this regard.
5.1. Boundaries
The upstream boundary condition is simplified by assuming that the discharge is evenly distributed along the corresponding water level (see
Figure 8). Thus, the velocity profile will be homogeneously distributed along the face. This can be stated as a simplification. However, due to the low entry velocities at the entry surface, the variability in the velocities with depth (0.2 m high crest) can be considered as insignificant. Additionally, the effect of this simplification on the results of the study are deemed to be minimal.
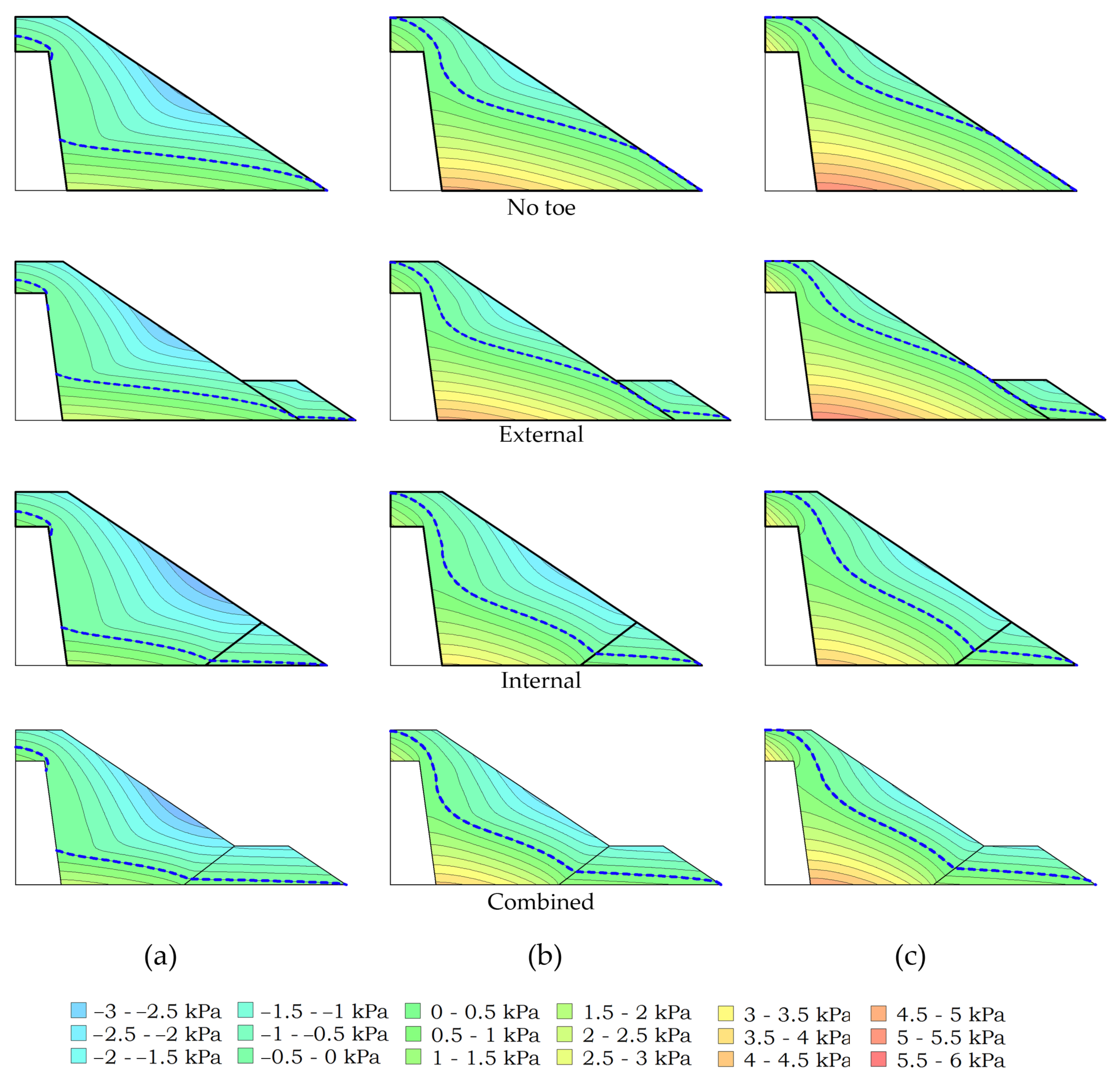
Definition of a drainage boundary with a potential seepage face results in that water is able to escape the domain along the boundary. In the numerical model, the dam surface is a drainage boundary, including the surface at the crest. Thus, for the high upstream phreatic line, as occurs for the highest discharges, a minor amount of water exits the dam at the crest where the inflow enters the dam, as shown in
Figure 11. The effect was observed in the numerical model results for discharges,
m
/s and higher. To ensure that drainage out of the system does not distort the results in the downstream dam shoulder, data were extracted to calculate the water loss at the highest applied discharge,
m
/s. Considering the external toe case as an example, the total loss was computed to be
m
/s. Furthermore, for the no toe configuration, the total loss was calculated as
m
/s. The magnitude of losses was therefore deemed negligible for all models. This further entails that the same applies to lower applied discharges,
m
/s.
5.2. Pressure Development
Investigating the pressure development within the dam models, for
m
/s, the numerical results for the no toe and external toe models show somewhat lower pressure values than physical modeling observations. The reasoning for this could be that the selected parameter set and successive numerical results were better fitted to the higher discharges. However, for both the internal toe and combined toe cases, the fit is similar for all the discharges, and even best for
m
/s in the case of the combined toe. Furthermore, the observed discrepancy does not affect the general outcome of the study relating to investigating the different toe configurations. Moreover, it is of value to be able to use the same material properties for all the models and discharges. Comparison of the numerical and physical model results in
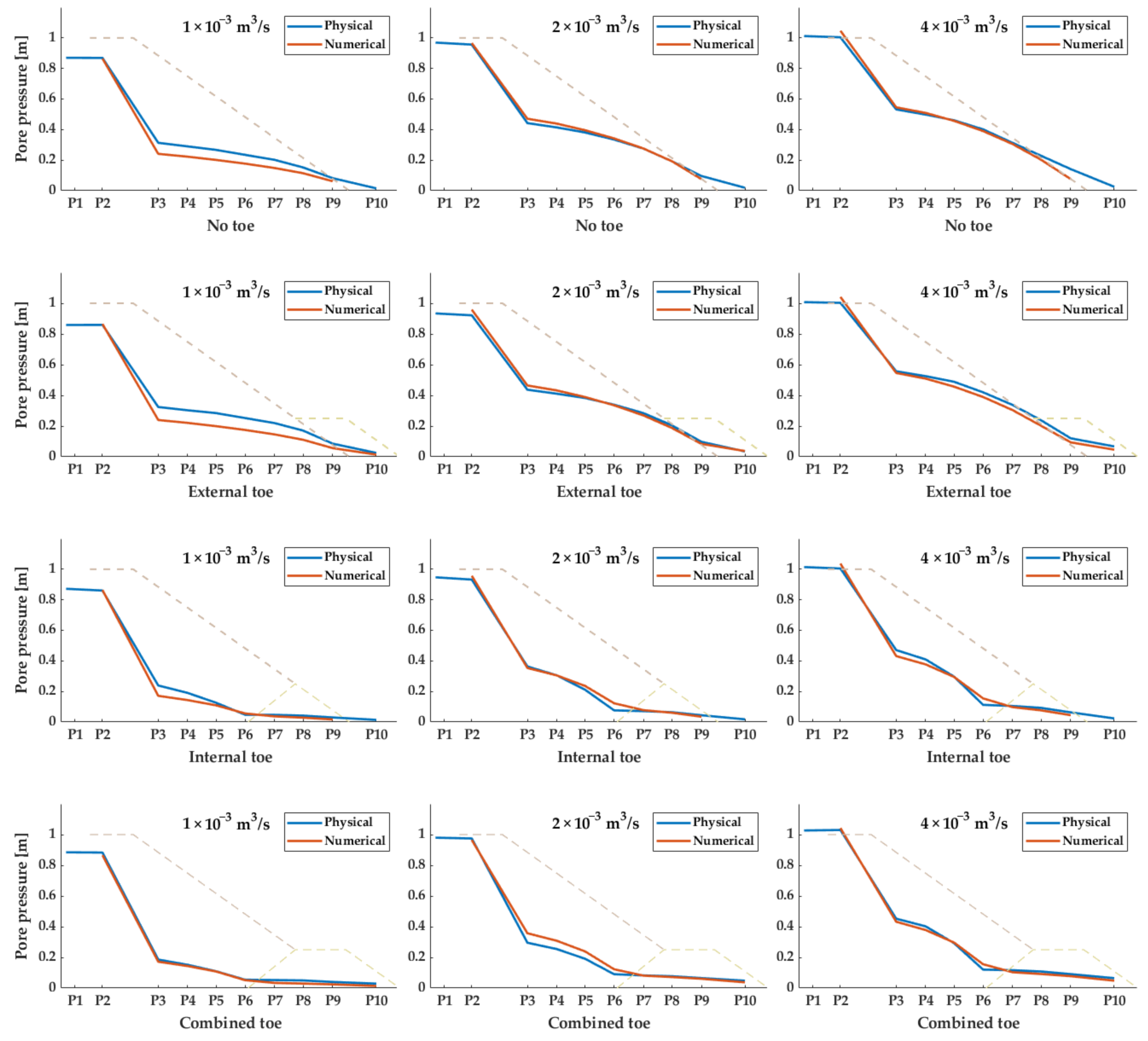
Figure 10 is a validation of the parameter set used and supports further investigations using the numerical model.
The numerical results for the internal and combined toe configurations are in good agreement with the physical results. However, for location P6, close to the interface of the rockfill material and the toe, the pore pressure is slightly lower for the physical models for discharges m/s and larger. This can be due to increased permeability at this location in the physical model, explained by the methodology adopted for construction of the dam. The toe is first placed in its position in layers and the dam is built adjacent to the toe. When compacting the dam layers, the shell material in contact with the toe needs to be carefully tamped, so as to not cause filling of the voids or disturbing the shape of the toe. This can cause the interface and surrounding area to have greater permeability than the rest of the dam. For the numerical model, the material properties are defined for the respective region, and the interface has no effect outside of the contact. A similar limitation lies at the crest of the dam, which can be seen as the numerical results yielding higher pressure values at P2 for increasing discharge. Construction of the physical model requires a metal mesh at the face in order to withhold the materials from sliding into the upstream reservoir. This causes some of the finer material to slide through, as well as diminishing the compactability. Ultimately this can have an increasing effect on the permeability compared to the main body of the shell, which is not represented in the numerical model, as it remains completely homogeneous. These effects are important to note when proceeding to real dam cases, considering that the method of construction introduces regions of different permeability.
From
Table 4, the physical model results show the relative reduction in pore pressure decreases with increasing discharge. The same overall trend can be seen for the numerical results. However, the results for
m
/s, do not agree with that trend. These peculiarities can be linked to issues with the input boundary. For
m
/s, the water level was measured at 0.99 m at P1, meaning that the mesh size used, of 0.02 m, is split for this edge.
A consequence of lowering the phreatic surface, due to introduction of a high permeability zone such as the toe installations, is increased flow velocities within the dam structure. Increased velocity can have a detrimental effect on stability through internal erosion processes, if it is not accounted for in the dam design. To investigate the velocity increase, the numerical model was used, extracting data at a horizontal line from the top of the core into the dam. Comparing the internal toe configuration to no toe, the data showed a 10% average increase in velocity fluxes for m/s.
5.3. Calibration
The parameter set that was obtained for the dam and the toe structure was arrived at through trial and error. When using the non-Darcy add-in, there are multiple physical properties and fitting parameters that affect the flow patterns and subsequent phreatic line developments. The critical component being the hydraulic conductivity, defining the permeability of the dam. Minute adjustments of the hydraulic conductivity will have great influence on the phreatic line and pore pressure development. Upon setting an agreeable hydraulic conductivity, the Van Genuchten fitting parameters and tortuosity parameters were dialed in.
The -parameter will alter the fit at the upstream end of the dam, showing influence on the early sensor locations. It was found that increasing the -value in general raised the phreatic surfaces.
The l-parameter had significant influence on the flow pattern through the downstream dam shell and the toe structure. It was found that, when decreasing the l-parameter flow, velocity vectors were seen to a larger degree above the phreatic lines. This effect has the largest influence on the toe, where there is high inter-connectivity of the voids. The l-parameter was also found to affect the pressure in the main body of the dam.
Some limitations on the parameters are hard to estimate, as the user interface of the non-Darcy add-in of the software used [
20] does not limit any input, but incorrect values will cause failure to find a solution during analysis. For example, the
n-parameter is said to have a limit yet, with increasing hydraulic conductivity, the limit changes. In this sense, the add-in calibration can be slightly cryptic, prolonging the process. The calibration process aimed at finding a parameter set best fitting P2-P10, as the phreatic lines on top of the core remained largely unaffected by toe design in the physical model. However, for internal and no toe configurations, the last measurement points, P9 and P10 were affected by the end of the domain.
In examining the available literature regarding the Van Genuchten input parameters, it should be clarified that the described parameters are detailed for soils of different compositions. Another limitation to bring up is that the apparent conductivity used in the non-Darcy add-in is designed for groundwater aquifers, and is valid when velocities remain low to intermediate [
23]. This could pose issues with upscaling the model to larger dams where velocities can be considerably larger. There is no available research utilizing the non-Darcy add-in, which further adds some uncertainty.
Equifinality of parameters is an additional point of discussion for the non-Darcy modeling. As calibration is done on a trial and error basis, with multiple fitting parameters, the results could possibly be reproduced with another parameter-set.
5.4. Application and Future Recommendations
The study demonstrates how numerical models can be useful for deeper apprehension of the results from physical tests. The numerical model enables detailed investigation of flow through the dam structure at every specific location, not just discreet positions determined by, e.g., installed pressure sensor locations. Moreover, the numerical model has the advantageous possibility of investigating different parameters at specific locations that the physical test cannot, such as velocity. In addition, through a calibrated numerical model, one can experiment with modifications to the physical model which are more resource intensive than modifications to numerical models. Hence, prior to customization of the physical model, it is highly recommended to utilize the numerical model for planning of future experiments. The numerical model requires very little resources for alteration of the design, which can then be used to design the changes and hypothesize the results of alterations to the physical model.
It is important to proceed from physical scale models to full scale dam cases, preferably with relevant data for calibration and validation. In general, to optimize the calibration process, it is recommended to investigate the usage of optimization algorithms to find optimally fitted parameter sets. With adoption of an optimization algorithm or machine learning method, higher precision calibrations could be achieved, but one must be cautious of unrealistic parameters. Before expanding the numerical model to other cases, it is recommended to verify the numerical model on a prototype rockfill dam with well-documented throughflow data. Calibrating the model to a full-scale embankment dam can provide verification of the applicability and validity of the non-Darcy add-in within prototype dams.