Modeling and Solving of Joint Flood Control Operation of Large-Scale Reservoirs: A Case Study in the Middle and Upper Yangtze River in China
Abstract
1. Introduction
2. Reservoirs Joint Flood Control Optimal Operation Model
2.1. Objective Function
2.2. Constraints
2.3. Optimization Algorithm
2.3.1. Dynamic Programming (DP)
2.3.2. Progressive Optimality Algorithm (POA)
2.3.3. Particle Swarm Optimization (PSO)
3. Study Area
3.1. Introduction of Study Area
3.2. Data of Study Area
3.2.1. Data of Reservoir
3.2.2. Data of Flood Control Point
3.2.3. Muskingum Parameters of the Basin
4. Application
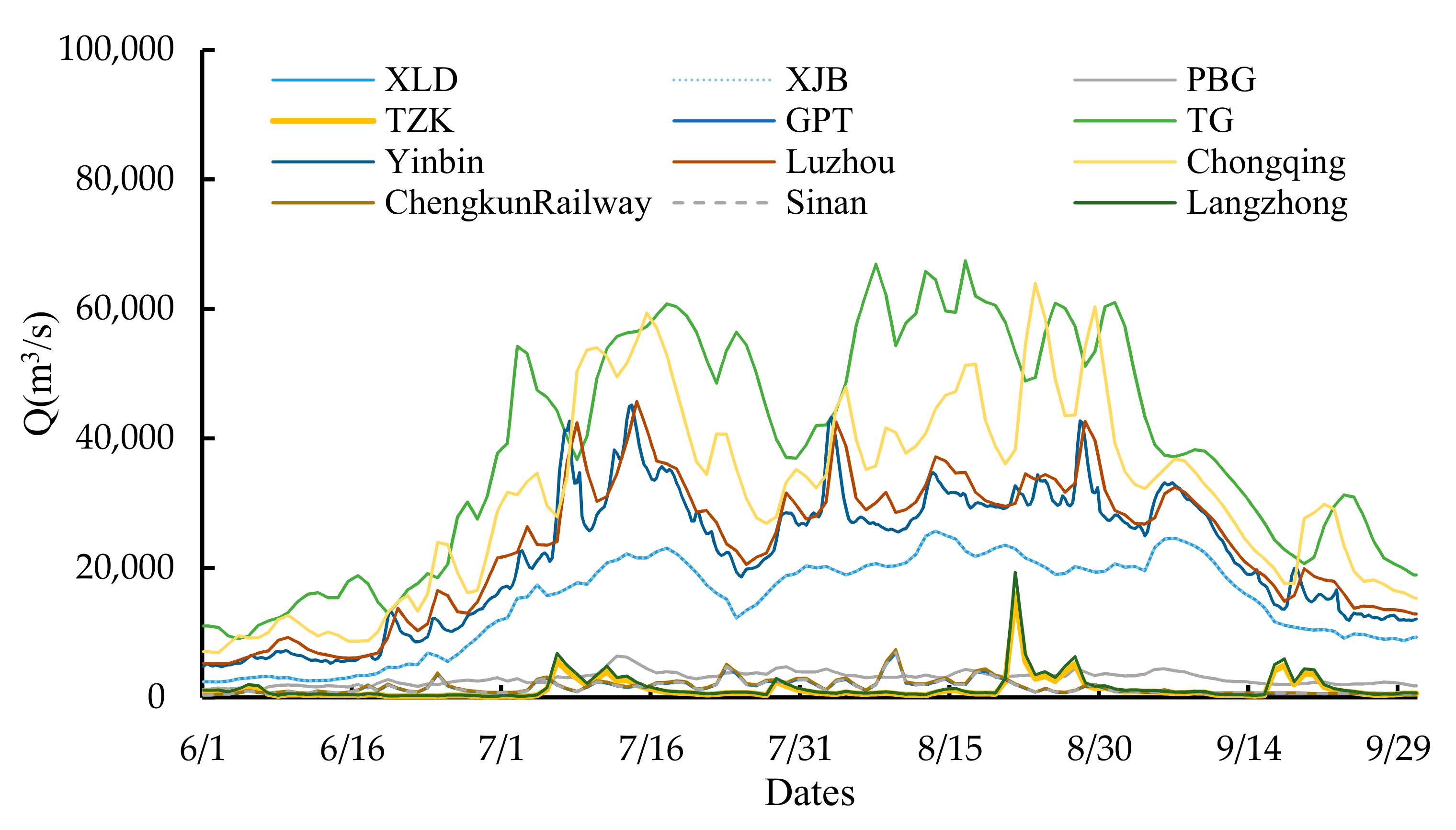
4.1. Design Flood Hydrographs
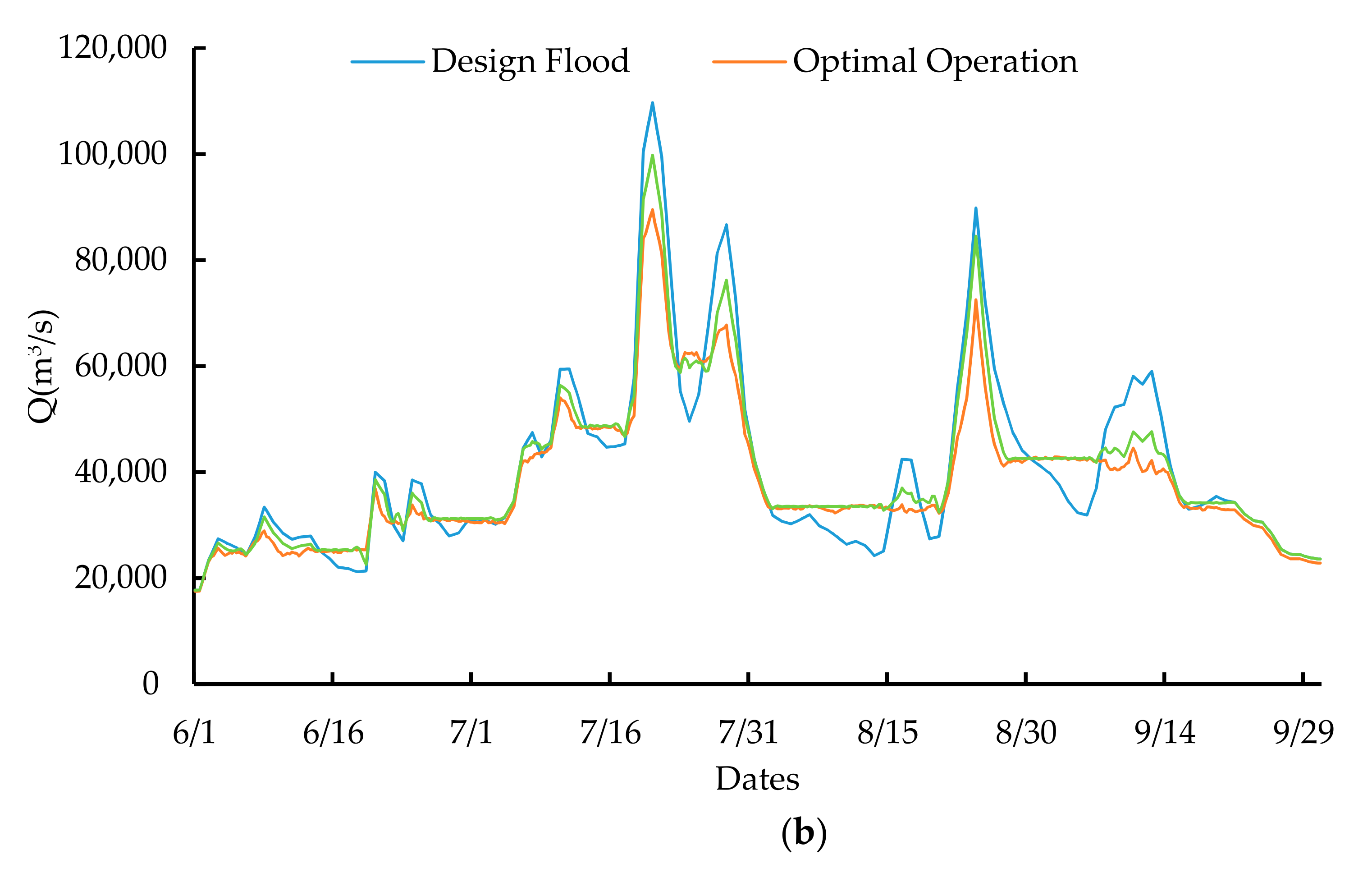
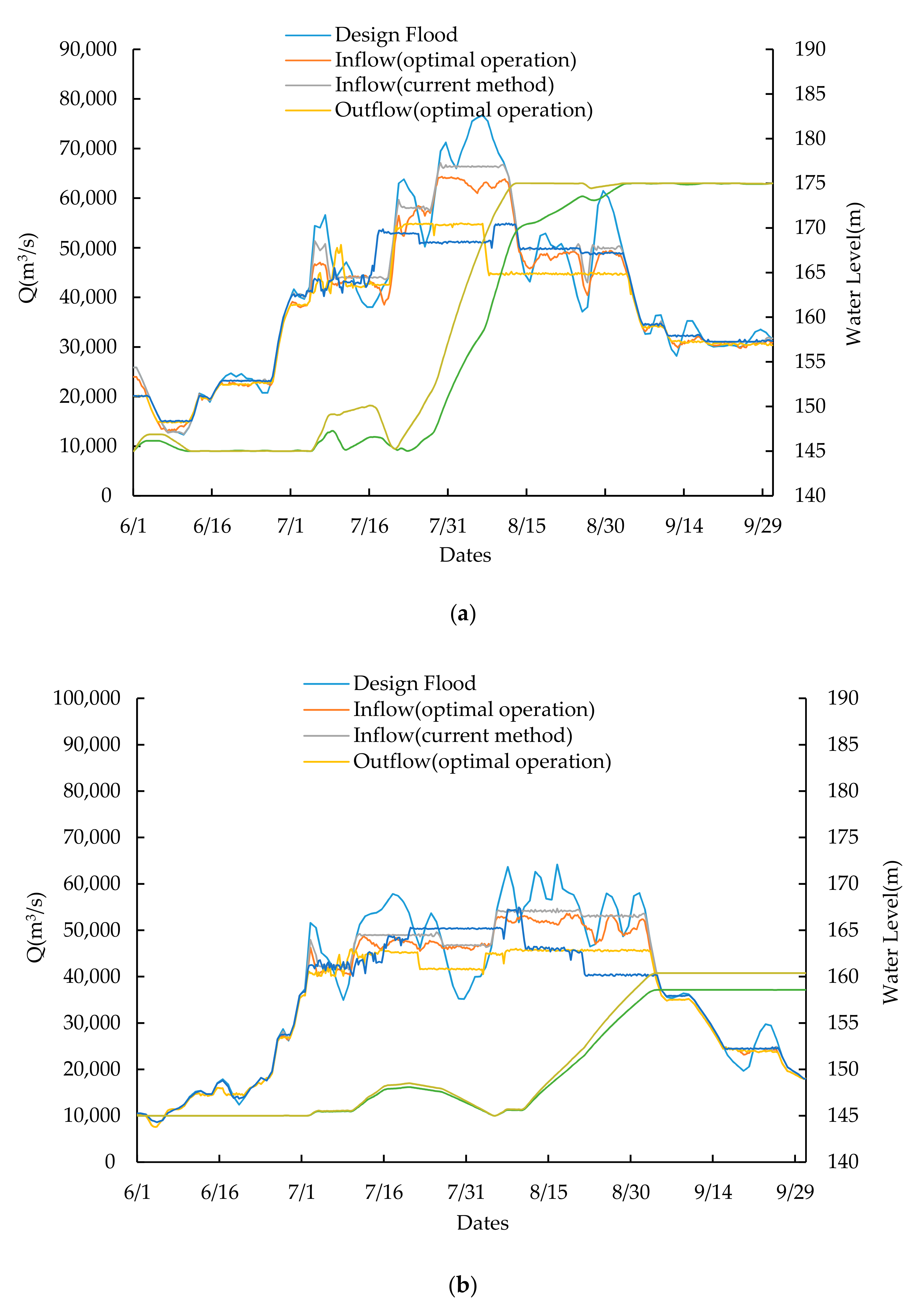
4.2. Optimal Reservoir Operation Results
4.2.1. Influence on the Inflow Flood of TG
4.2.2. Operation Results of Jingjiang and Chenglingji
4.2.3. Operation Results of TG
5. Conclusions and Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Chen, C.; Yuan, Y.; Yuan, X. An Improved NSGA-III Algorithm for Reservoir Flood Control Operation. Water Resour. Manag. 2017, 31, 4469–4483. [Google Scholar] [CrossRef]
- Zhang, W.; Liu, P.; Chen, X.; Wang, L.; Ai, X.; Feng, M.; Liu, D.; Liu, Y. Optimal Operation of Multi-reservoir Systems Considering Time-lags of Flood Routing. Water Resour. Manag. 2015, 30, 523–540. [Google Scholar] [CrossRef]
- Zhou, Y.; Guo, S.; Liu, P.; Xu, C. Joint operation and dynamic control of flood limiting water levels for mixed cascade reservoir systems. J. Hydrol. 2014, 519, 248–257. [Google Scholar] [CrossRef]
- Qin, H.; Zhou, J.; Lu, Y.; Li, Y.; Zhang, Y. Multi-objective Cultured Differential Evolution for Generating Optimal Trade-offs in Reservoir Flood Control Operation. Water Resour. Manag. 2010, 24, 2611–2632. [Google Scholar] [CrossRef]
- Reddy, M.J.; Kumar, D.N. Optimal Reservoir Operation Using Multi-Objective Evolutionary Algorithm. Water Resour. Manag. 2006, 20, 861–878. [Google Scholar] [CrossRef]
- Liu, X.; Chen, L.; Zhu, Y.; Singh, V.P.; Qu, G.; Guo, X. Multi-objective reservoir operation during flood season considering spillway optimization. J. Hydrol. 2017, 552, 554–563. [Google Scholar] [CrossRef]
- Yazdi, J.; Neyshabouri, S.A.A.S. Optimal design of flood-control multi-reservoir system on a watershed scale. Nat. Hazards 2012, 63, 629–646. [Google Scholar] [CrossRef]
- Xiaocong, H.; Lisheng, Z.; Linyun, L. Study on flood control operation of Jinsha River cascade reservoirs combined with the Three Gorges along the Yangtze River. IOP Conf. Ser.: Mater. Sci. Eng. 2017, 199, 12032. [Google Scholar] [CrossRef]
- Lee, S.Y.; Hamlet, A.F.; Fitzgerald, C.J.; Burges, S.J. Optimized Flood Control in the Columbia River Basin for a Global Warming Scenario. J. Water Resour. Plan. Manag. 2009, 135, 440–450. [Google Scholar] [CrossRef]
- Chou, F.N.-F.; Wu, C.-W. Stage-wise optimizing operating rules for flood control in a multi-purpose reservoir. J. Hydrol. 2015, 521, 245–260. [Google Scholar] [CrossRef]
- Guo, S.; Chen, J.; Li, Y.; Liu, P.; Li, T. Joint Operation of the Multi-Reservoir System of the Three Gorges and the Qingjiang Cascade Reservoirs. Energies 2011, 4, 1036–1050. [Google Scholar] [CrossRef]
- Chang, L.-C.; Chang, F.-J. Multi-objective evolutionary algorithm for operating parallel reservoir system. J. Hydrol. 2009, 377, 12–20. [Google Scholar] [CrossRef]
- Li, X.; Guo, S.; Liu, P.; Chen, G. Dynamic control of flood limited water level for reservoir operation by considering inflow uncertainty. J. Hydrol. 2010, 391, 124–132. [Google Scholar] [CrossRef]
- Ouyang, S.; Zhou, J.; Li, C.; Liao, X.; Wang, H. Optimal design for flood limit water level of cascade reservoirs. Water Resour. Manag. 2015, 29, 445–457. [Google Scholar] [CrossRef]
- Chen, J.; Guo, S.; Li, Y.; Liu, P.; Zhou, Y. Joint Operation and Dynamic Control of Flood Limiting Water Levels for Cascade Reservoirs. Water Resour. Manag. 2013, 27, 749–763. [Google Scholar] [CrossRef]
- Unver, O.I.; Mays, L.W. Model for real-time optimal flood control operation of a reservoir system. Water Resour. Manag. 1990, 4, 21–46. [Google Scholar] [CrossRef]
- Wei, C.C.; Hsu, N.S. Multireservoir Flood-Control Optimization with Neural-Based Linear Channel Level Routing Under Tidal Effects. Water Resour. Manag. 2008, 22, 1625–1647. [Google Scholar] [CrossRef]
- Xin-Yu, W.; Ping-An, Z.; Xuan, C.; Li, D.; Ben-You, J. Computer Simulation of Flood Scheduling in Large Scale Flood Control Systems. Procedia Eng. 2012, 29, 3267–3275. [Google Scholar] [CrossRef][Green Version]
- Zhou, C.; Sun, N.; Chen, L.; Ding, Y.; Zhou, J.; Zha, G.; Luo, G.; Dai, L.; Yang, X. Optimal operation of cascade reservoirs for flood control of multiple areas downstream: A case study in the upper Yangtze river basin. Water 2018, 10, 1250. [Google Scholar] [CrossRef]
- Moridi, A.; Yazdi, J. Optimal Allocation of Flood Control Capacity for Multi-Reservoir Systems Using Multi-Objective Optimization Approach. Water Resour. Manag. 2017, 31, 4521–4538. [Google Scholar] [CrossRef]
- Zhou, Y.; Guo, S.; Chang, L.-C.; Liu, P.; Chen, A.B. Methodology that improves water utilization and hydropower generation without increasing flood risk in mega cascade reservoirs. Energy 2018, 143, 785–796. [Google Scholar] [CrossRef]
- Hall, W.A.; Butcher, W.S.; Esogbue, A. Optimization of the Operation of a Multiple-Purpose Reservoir by Dynamic Programming. Water Resour. Res. 1968, 4, 471–477. [Google Scholar] [CrossRef]
- Jiang, Z.; Qin, H.; Ji, C.; Feng, Z.; Zhou, J. Two Dimension Reduction Methods for Multi-Dimensional Dynamic Programming and Its Application in Cascade Reservoirs Operation Optimization. Water 2017, 9, 634. [Google Scholar] [CrossRef]
- Jiang, Z.; Ji, C.; Qin, H.; Feng, Z. Multi-stage progressive optimality algorithm and its application in energy storage operation chart optimization of cascade reservoirs. Energy 2018, 148, 309–323. [Google Scholar] [CrossRef]
- Nanda, J.; Bijwe, P.R. Optimal Hydrothermal Scheduling with Cascaded Plants Using Progressive Optimality Algorithm. IEEE Trans. Power Appar. Syst. 1981, 100, 2093–2099. [Google Scholar] [CrossRef]
- Liu, P.; Cai, X.; Guo, S. Deriving multiple near-optimal solutions to deterministic reservoir operation problems. Water Resour. Res. 2011, 47, 2168–2174. [Google Scholar] [CrossRef]
- Windsor, J.S. Optimization model for the operation of flood control systems. Water Resour. Res. 1973, 9, 1219–1226. [Google Scholar] [CrossRef]
- Brandao, J.L.B. Performance of the Equivalent Reservoir Modelling Technique for Multi-Reservoir Hydropower Systems. Water Resour. Manag. 2010, 24, 3101–3114. [Google Scholar] [CrossRef]
- Chuanwen, J.; Bompard, E. A self-adaptive chaotic particle swarm algorithm for short term hydroelectric system scheduling in deregulated environment. Energy Convers. Manag. 2005, 46, 2689–2696. [Google Scholar] [CrossRef]
- Ostadrahimi, L.; Marino, M.A.; Afshar, A. Multi-reservoir Operation Rules: Multi-swarm PSO-based Optimization Approach. Water Resour. Manag. 2011, 26, 407–427. [Google Scholar] [CrossRef]
- Wang, W.; Lin, Q. Optimal Reservoir Operation Using PSO with Adaptive Random Inertia Weight. In Proceedings of the 2010 International Conference on Artificial Intelligence and Computational Intelligence, Sanya, China, 23–24 October 2010. [Google Scholar]
- Kumphon, B. Genetic Algorithms for Multi-objective Optimization: Application to a Multi-reservoir System in the Chi River Basin, Thailand. Water Resour. Manag. 2013, 27, 4369–4378. [Google Scholar] [CrossRef]
- Moreira, R.M.M. Simulation–Optimization Modeling: A Survey and Potential Application in Reservoir Systems Operation. Water Resour. Manag. 2010, 24, 1107–1138. [Google Scholar]
- Reis, L.F.R.; Walters, G.A.; Savic, D.; Chaudhry, F.H. Multi-Reservoir Operation Planning Using Hybrid Genetic Algorithm and Linear Programming (GA-LP): An Alternative Stochastic Approach. Water Resour. Manag. 2005, 19, 831–848. [Google Scholar] [CrossRef]
- Reddy, M.J.; Kumar, D.N. Multiobjective Differential Evolution with Application to Reservoir System Optimization. J. Comput. Civ. Eng. 2007, 21, 136–146. [Google Scholar] [CrossRef]
- Qian, W.; Li, A. Adaptive differential evolution algorithm for multiobjective optimization problems. Appl. Math. Comput. 2008, 201, 431–440. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, X.; Liu, P.; Lei, X.; Li, Z.; Gong, W.; Duan, Q.; Wang, H. Assessing the weighted multi-objective adaptive surrogate model optimization to derive large-scale reservoir operating rules with sensitivity analysis. J. Hydrol. 2017, 544, 613–627. [Google Scholar] [CrossRef]
- Sun, P.; Wang, L.P.; Jiang, Z.Q.; Ji, C.M.; Zhang, Y.K. Application of two multi-dimensional dynamic programming algorithms in optimization of cascade reservoirs operation. J. Hydraul. Eng. 2014, 45, 1327–1335. [Google Scholar]
- Zong, H.; Li, C.; Zhou, J. Research and application for short-time cascaded hydroelectric scheduling based on progressive optimality algorithm. Hydroelectr. Energy 2003, 21, 46–48. [Google Scholar]
- Wen, X.; Zhou, J.; He, Z.; Wang, C. Long-Term Scheduling of Large-Scale Cascade Hydropower Stations Using Improved Differential Evolution Algorithm. Water 2018, 10, 383. [Google Scholar] [CrossRef]
- Azamathulla, H.M.; Wu, F.C.; Ghani, A.A.; Narulkar, S.M.; Zakaria, N.A.; Chang, C.K. Comparison between genetic algorithm and linear programming approach for real time operation. J. Hydro-Environ. Res. 2008, 2, 172–181. [Google Scholar] [CrossRef]
- Gill, M.A. Flood routing by the Muskingum method. J. Hydrol. 1978, 36, 353–363. [Google Scholar] [CrossRef]
- Tung, Y.K. River Flood Routing by Nonlinear Muskingum Method. J. Hydraul. Eng. 1985, 111, 1447–1460. [Google Scholar] [CrossRef]
- Jia, B.; Zhong, P.; Wan, X.; Xu, B.; Chen, J. Decomposition–coordination model of reservoir group and flood storage basin for real-time flood control operation. Hydrol. Res. 2015, 46, 11–25. [Google Scholar] [CrossRef]
- Xubao, Q. Research for Optimal Flood Dispatch Model for Reservoir Based on POA. Water Resour. Power 2008, 4, 35–37. [Google Scholar]
- Afshar, M.H. Large scale reservoir operation by Constrained Particle Swarm Optimization algorithms. J. Hydro-Environ. Res. 2012, 6, 75–87. [Google Scholar] [CrossRef]
- He, Y.; Xu, Q.; Yang, S.; Liao, L. Reservoir flood control operation based on chaotic particle swarm optimization algorithm. Appl. Math. Model. 2014, 38, 4480–4492. [Google Scholar] [CrossRef]
- Tayfur, G. Modern Optimization Methods in Water Resources Planning, Engineering and Management. Water Resour. Manag. 2017, 31, 3205–3233. [Google Scholar] [CrossRef]
- Fang, H.; Han, D.; He, G.J.; Chen, M. Flood management selections for the Yangtze River midstream after the Three Gorges Project operation. J. Hydrol. 2012, 432–433, 1–11. [Google Scholar] [CrossRef]
- Gang, Z. Preliminary study on the causes and countermeasures of the 1954 and 1998 extraordinary Yangtze river floods. J. Catastrophol. 1999, 4, 43–44. [Google Scholar]
- Huang, W.C.; Hsieh, C.L. Real-time reservoir flood operation during typhoon attacks. Water Resour. Res. 2010, 46, 759–768. [Google Scholar] [CrossRef]
- Yue, S.; Ouarda, T.B.M.J.; Bobée, B.; Legendre, P.; Bruneau, P. Approach for Describing Statistical Properties of Flood Hydrograph. J. Hydrol. Eng. 2002, 7, 147–153. [Google Scholar] [CrossRef]
- Faulkner, D.S.; Barber, S. Performance of the Revitalised Flood Hydrograph method. J. Flood Risk Manag. 2009, 2, 254–261. [Google Scholar] [CrossRef]
- Pramanik, N.; Panda, R.K.; Sen, D. Development of design flood hydrographs using probability density functions. Hydrol. Process. 2010, 24, 415–428. [Google Scholar] [CrossRef]
- Yi, X.; Guo, S.; Pan, L.; Yan, B.; Lu, C. Design Flood Hydrograph Based on Multicharacteristic Synthesis Index Method. J. Hydrol. Eng. 2009, 14, 1359–1364. [Google Scholar]
- Chen, L.; Guo, S.; Yan, B.; Liu, P.; Fang, B. A new seasonal design flood method based on bivariate joint distribution of flood magnitude and date of occurrence. Hydrol. Sci. J. 2010, 55, 1264–1280. [Google Scholar] [CrossRef]
- Li, J.B.; Guo, Y.C.; Zhao, Z.H.; Shuai, H.; Xie, L. Impact of the Three Gorges Reservoir Flood Prevention Dispatching Tohydrologic Information and Disaster Information in Dongting Lake Area. Adv. Mater. Res. 2012, 518, 3652–3661. [Google Scholar] [CrossRef]
- Liu, X.; Qu, G.; Guo, X.; Yue, H. Study on the Three Gorges reservoir flood control operation chart considering comprehensive utilization requirements. IOP Conf. Ser. Earth Environ. Sci. 2019, 304, 042040. [Google Scholar] [CrossRef]
- Yan, Z. Thinking on the flood disaster in the middle reaches of the Yangtze river. Earth Sci. Front. 2000, 7, 87–93. [Google Scholar]
- Zhenzhen, W.; Wei, S. Great Flood Water and Disaster Years of Dongting Lake. J. Wuhan Univ. Hydraul. Electr. Eng. 1995, 1, 155–156. [Google Scholar]
- Hu, H.; Tian, Z.; Sun, L.; Wen, J.; Liang, Z.; Dong, G.; Liu, J. Synthesized trade-off analysis of flood control solutions under future deep uncertainty: An application to the central business district of Shanghai. Water Res. 2019, 166, 115067. [Google Scholar] [CrossRef]
- Krzysztofowicz, R.; Duckstein, L. Preference criterion for flood control under uncertainty. Water Resour. Res. 1979, 15, 513–520. [Google Scholar] [CrossRef]
- Chen, L.; Singh, V.P.; Lu, W.; Zhang, J.; Zhou, J.; Guo, S. Streamflow forecast uncertainty evolution and its effect on real-time reservoir operation. J. Hydrol. 2016, 540, 712–726. [Google Scholar] [CrossRef]
- Vermuyten, E.; Meert, P.; Wolfs, V.; Willems, P. Model uncertainty reduction for real-time flood control by means of a flexible data assimilation approach and reduced conceptual models. J. Hydrol. 2018, 564, 490–500. [Google Scholar] [CrossRef]
- Chen, J.; Zhong, P.-A.; Zhao, Y.-F. Research on a layered coupling optimal operation model of the Three Gorges and Gezhouba cascade hydropower stations. Energy Convers. Manag. 2014, 86, 756–763. [Google Scholar] [CrossRef]

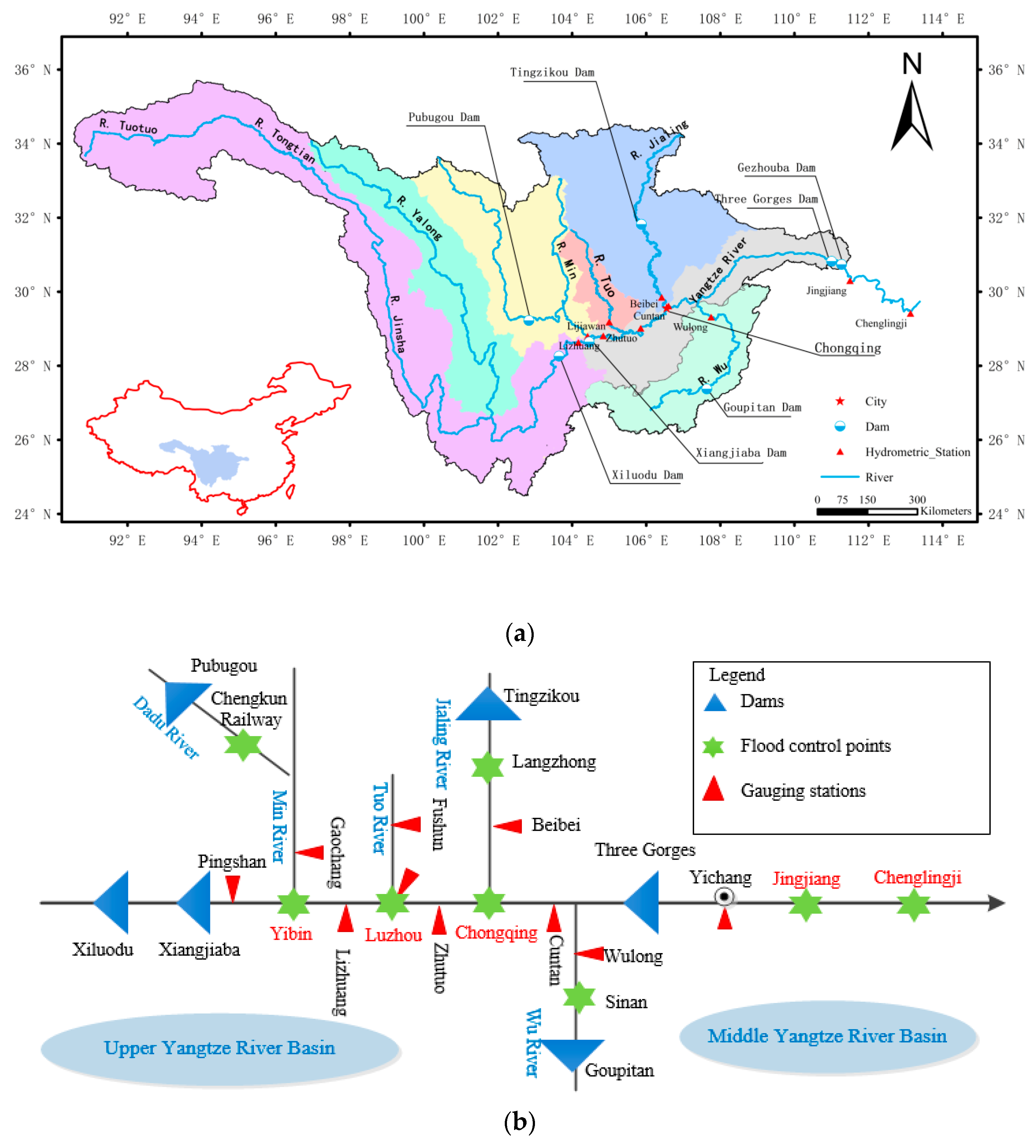


| Reservoir | Location | Drainage Area (104 km2) | Normal Water Level (m) | Flood Control Water Level (m) | Total Storage Capacity (Billion m3) | Flood Control Storage Capacity (Billion m3) |
|---|---|---|---|---|---|---|
| XLD | Jinsha River | 45.4 | 600 | 560 | 12.7 | 4.7 |
| XJB | Jinsha River | 45.9 | 380 | 370 | 5.2 | 0.9 |
| PBG | Min River | 6.8 | 850 | 836 | 5.4 | 1.1 |
| TZK | Jialing River | 6.1 | 458 | 447 | 4.1 | 14.4 |
| GPT | Wu River | 4.3 | 630 | 626 | 6.4 | 0.4 |
| TG | Yangtze River | 100 | 175 | 145 | 39.3 | 22.1 |
| Upper Section | Lower Section | Number of Segments | C0 | C1 | C2 |
|---|---|---|---|---|---|
| XLD | XJB | 0 | - | - | - |
| XJB | Yibin | 1 | 0.283 | 0.498 | 0.219 |
| PBG | Chengkun railway | 1 | 0.291 | 0.476 | 0.233 |
| Chengkun railway | Yibin | 3 | 0.283 | 0.498 | 0.219 |
| Yibin | Luzhou | 3 | 0.290 | 0.430 | 0.280 |
| Luzhou | Chongqing | 2 | 0.240 | 0.420 | 0.340 |
| TZK | Langzhong | 1 | 0.293 | 0.467 | 0.240 |
| Langzhong | Chongqing | 3 | 0.243 | 0.417 | 0.340 |
| Chongqing | TG | 8 | 0.187 | 0.430 | 0.383 |
| GPT | Sinan | 0 | - | - | - |
| Sinan | TG | 8 | 0.187 | 0.430 | 0.383 |
| Category | Parameters | Return Period (year) | ||||||
|---|---|---|---|---|---|---|---|---|
| EX | CV | CV/CS | 10,000 | 1000 | 100 | 50 | 20 | |
| flood peak | 52,000 | 0.21 | 4 | 113,000 | 98,800 | 83,700 | 79,000 | 72,300 |
| 7-day volumes | 275 | 0.19 | 3.5 | 547.2 | 486.8 | 420.8 | 400 | 368.5 |
| 15-day volumes | 524 | 0.19 | 3 | 1022 | 911.8 | 796.5 | 757 | 702.2 |
| 30-day volumes | 935 | 0.18 | 3 | 1767 | 1590 | 1393 | 1330 | 1234 |
| Typical Flood Hydrograph | Return Period | Design Value | Current Method | Optimal Method | Comparison | ||
|---|---|---|---|---|---|---|---|
| Inflow | Reduction | Inflow | Reduction | ||||
| 1954 | 1000 | 76,676 | 70,693 | 5983 | 65,886 | 10,790 | 4807 (80%) |
| 100 | 67,025 | 61,456 | 5570 | 59,914 | 7112 | 1542 (27%) | |
| 50 | 63,720 | 58,327 | 5394 | 56,822 | 6898 | 1505 (28%) | |
| 1968 | 1000 | 96,390 | 86,911 | 9479 | 78,094 | 18,296 | 8817 (93%) |
| 100 | 83,916 | 74,855 | 9061 | 69,812 | 14,104 | 5043 (56%) | |
| 50 | 79,834 | 70,886 | 8947 | 66,278 | 13,555 | 4608 (51%) | |
| 1980 | 1000 | 104,369 | 100,250 | 4119 | 90,369 | 14,000 | 9881 (239%) |
| 100 | 79,661 | 71,532 | 8129 | 67,531 | 12,130 | 4001 (49%) | |
| 50 | 75,730 | 67,643 | 8087 | 63,920 | 11,810 | 3723 (46%) | |
| 1981 | 1000 | 111,200 | 102,116 | 9084 | 97,494 | 13,706 | 4621 (51%) |
| 100 | 97,092 | 89,195 | 7896 | 86,505 | 10,586 | 2690 (34%) | |
| 50 | 92,922 | 84,406 | 8515 | 81,830 | 11,091 | 2576 (30%) | |
| 1982 | 1000 | 92,040 | 82,497 | 9543 | 75,844 | 16,196 | 6654 (70%) |
| 100 | 80,417 | 71,275 | 9142 | 70,712 | 9705 | 563 (6%) | |
| 50 | 76,523 | 67,396 | 9127 | 66,885 | 9638 | 511 (6%) | |
| 1988 | 1000 | 80,106 | 73,462 | 6644 | 68,683 | 11,423 | 4780 (72%) |
| 100 | 69,773 | 63,759 | 6014 | 61,329 | 8444 | 2430 (40%) | |
| 50 | 66,360 | 60,527 | 5833 | 58,139 | 8221 | 2388 (41%) | |
| 1996 | 1000 | 78,501 | 72,972 | 5529 | 69,848 | 8653 | 3125 (57%) |
| 100 | 68,555 | 62,813 | 5742 | 61,171 | 7384 | 1642 (29%) | |
| 50 | 65,185 | 59,570 | 5614 | 58,017 | 7167 | 1553 (28%) | |
| 1998 | 1000 | 77,125 | 72,560 | 4565 | 68,931 | 8194 | 3629 (79%) |
| 100 | 67,438 | 63,126 | 4312 | 61,567 | 5871 | 1559 (36%) | |
| 50 | 64,168 | 59,984 | 4184 | 58,585 | 5583 | 1399 (33%) | |
| 1999 | 1000 | 90,153 | 80,476 | 9677 | 72,982 | 17,171 | 7494 (77%) |
| 100 | 78,643 | 69,232 | 9411 | 64,598 | 14,045 | 4634 (49%) | |
| 50 | 74,787 | 65,427 | 9360 | 61,015 | 13,772 | 4412 (47%) | |
| 2010 | 1000 | 109,705 | 101,932 | 7773 | 96,770 | 12,935 | 5162 (66%) |
| 100 | 96,408 | 87,967 | 8441 | 82,567 | 13,841 | 5400 (64%) | |
| 50 | 91,089 | 82,820 | 8269 | 77,942 | 13,146 | 4877 (59%) | |
| Average Value | 1000 | 91,250 | 83,313 | 7936 | 77,939 | 13,311 | 5375 (68%) |
| 100 | 78,751 | 71,287 | 7464 | 68,493 | 10,258 | 2794 (37%) | |
| 50 | 74,890 | 67,491 | 7399 | 64,863 | 10,027 | 2628 (36%) | |
| Typical Flood Hydrograph | Return Period | Without Operations | Current Method | Optimal Method | Comparison | ||
|---|---|---|---|---|---|---|---|
| Diversion | Reduction | Diversion | Reduction | ||||
| 1954 | 1000 | 23.7 | 2.93 | 20.77 | 0.51 | 23.18 | 2.41 (11%) |
| 100 | 8.4 | 0 | 8.4 | 0 | 8.4 | 0 (0%) | |
| 50 | 4.78 | 0 | 4.78 | 0 | 4.78 | 0 (0%) | |
| 1968 | 1000 | 56.45 | 9.72 | 46.73 | 7.58 | 48.87 | 2.13 (5%) |
| 100 | 24.02 | 0 | 24.02 | 0 | 24.02 | 0 (0%) | |
| 50 | 16.96 | 0 | 16.96 | 0 | 16.96 | 0 (0%) | |
| 1980 | 1000 | 24.83 | 22.18 | 2.64 | 17.52 | 7.3 | 4.65 (176%) |
| 100 | 11.07 | 0 | 11.07 | 0 | 11.07 | 0 (0%) | |
| 50 | 8.51 | 0 | 8.51 | 0 | 8.51 | 0 (0%) | |
| 1981 | 1000 | 38.12 | 2.34 | 35.78 | 2.15 | 35.97 | 0.19 (1%) |
| 100 | 18.48 | 0 | 18.48 | 0 | 18.48 | 0 (0%) | |
| 50 | 14.02 | 0 | 14.02 | 0 | 14.02 | 0 (0%) | |
| 1982 | 1000 | 25.99 | 4.13 | 21.85 | 3.18 | 22.81 | 0.95 (4%) |
| 100 | 11.31 | 0 | 11.31 | 0 | 11.31 | 0 (0%) | |
| 50 | 8.26 | 0 | 8.26 | 0 | 8.26 | 0 (0%) | |
| 1988 | 1000 | 26.7 | 1.04 | 25.65 | 0 | 26.7 | 1.04 (4%) |
| 100 | 10.51 | 0 | 10.51 | 0 | 10.51 | 0 (0%) | |
| 50 | 5.86 | 0 | 5.86 | 0 | 5.86 | 0 (0%) | |
| 1996 | 1000 | 42.12 | 20.09 | 22.02 | 17.6 | 24.51 | 2.49 (11%) |
| 100 | 15.9 | 0 | 15.9 | 0 | 15.9 | 0 (0%) | |
| 50 | 9.7 | 0 | 9.7 | 0 | 9.7 | 0 (0%) | |
| 1998 | 1000 | 29.38 | 17.62 | 11.76 | 16.17 | 13.21 | 1.45 (12%) |
| 100 | 12.91 | 0 | 12.91 | 0 | 12.91 | 0 (0%) | |
| 50 | 6.02 | 0 | 6.02 | 0 | 6.02 | 0 (0%) | |
| 1999 | 1000 | 37.47 | 15.87 | 21.59 | 14.3 | 23.16 | 1.56 (7%) |
| 100 | 15.23 | 0 | 15.23 | 0 | 15.23 | 0 (0%) | |
| 50 | 9.35 | 0 | 9.35 | 0 | 9.35 | 0 (0%) | |
| 2010 | 1000 | 29.38 | 3 | 26.38 | 2.07 | 27.31 | 0.92 (3%) |
| 100 | 17.22 | 0 | 17.22 | 0 | 17.22 | 0 (0%) | |
| 50 | 13.36 | 0 | 13.36 | 0 | 13.36 | 0 (0%) | |
| Average | 1000 | 33.41 | 9.89 | 23.52 | 8.11 | 25.30 | 1.78 (8%) |
| 100 | 14.51 | 0 | 14.51 | 0 | 14.51 | 0 (0%) | |
| 50 | 9.68 | 0 | 9.68 | 0 | 9.68 | 0 (0%) | |
| Typical Flood Hydrograph | Return Period | Without Operations | Current Method | Optimal Method | Comparison | ||
|---|---|---|---|---|---|---|---|
| Diversion | Reduction | Diversion | Reduction | ||||
| 1954 | 1000 | 6.48 | 5.53 | 0.94 | 5.42 | 1.05 | 0.1 (11%) |
| 100 | 3.34 | 1.87 | 1.47 | 1.7 | 1.64 | 0.17 (12%) | |
| 50 | 2.42 | 1.59 | 0.83 | 0 | 2.42 | 1.59 (191%) | |
| 1968 | 1000 | 11.14 | 9.56 | 1.57 | 9.38 | 1.75 | 0.18 (10%) |
| 100 | 7.58 | 3.64 | 3.94 | 3.18 | 4.39 | 0.45 (11%) | |
| 50 | 5.84 | 3.47 | 2.37 | 0 | 5.84 | 3.47 (146%) | |
| 1980 | 1000 | 9.58 | 6.51 | 3.06 | 6.16 | 3.42 | 0.35 (10%) |
| 100 | 3.82 | 2.44 | 1.37 | 2.29 | 1.53 | 0.15 (13%) | |
| 50 | 1.88 | 1.83 | 0.04 | 0 | 1.88 | 1.83 (4600%) | |
| 1981 | 1000 | 9.31 | 7.08 | 2.23 | 6.83 | 2.48 | 0.25 (10%) |
| 100 | 4.42 | 2.42 | 1.99 | 2.19 | 2.22 | 0.22 (12%) | |
| 50 | 3.33 | 1.96 | 1.36 | 0 | 3.33 | 1.96 (144%) | |
| 1982 | 1000 | 5.25 | 5.17 | 0.08 | 5.16 | 0.08 | 0 (0%) |
| 100 | 2.89 | 2.14 | 0.75 | 2.05 | 0.84 | 0.08 (12%) | |
| 50 | 2.04 | 1.61 | 0.42 | 0 | 2.04 | 1.61 (385%) | |
| 1988 | 1000 | 5.84 | 4.92 | 0.91 | 4.82 | 1.01 | 0.1 (11%) |
| 100 | 2.89 | 1.23 | 1.66 | 1.04 | 1.85 | 0.19 (12%) | |
| 50 | 1.87 | 0.98 | 0.89 | 0 | 1.87 | 0.98 (110%) | |
| 1996 | 1000 | 7.18 | 6.9 | 0.28 | 6.86 | 0.31 | 0.03 (10%) |
| 100 | 5.7 | 2.49 | 3.21 | 2.12 | 3.57 | 0.36 (12%) | |
| 50 | 4.64 | 2.3 | 2.33 | 0 | 4.64 | 2.3 (99%) | |
| 1998 | 1000 | 9.09 | 4.66 | 4.43 | 4.15 | 4.94 | 0.5 (10%) |
| 100 | 6.53 | 2.48 | 4.04 | 2.01 | 4.51 | 0.46 (12%) | |
| 50 | 5.17 | 2.89 | 2.27 | 0 | 5.17 | 2.89 (127%) | |
| 1999 | 1000 | 7.93 | 7.24 | 0.69 | 7.16 | 0.76 | 0.07 (11%) |
| 100 | 4.72 | 2.31 | 2.4 | 2.04 | 2.68 | 0.27 (12%) | |
| 50 | 3.82 | 1.91 | 1.91 | 0 | 3.82 | 1.91 (100%) | |
| 2010 | 1000 | 4.42 | 4.18 | 0.24 | 4.15 | 0.27 | 0.02 (11%) |
| 100 | 2.13 | 1.35 | 0.78 | 1.26 | 0.87 | 0.09 (10%) | |
| 50 | 1.58 | 1.02 | 0.56 | 0 | 1.58 | 1.02 (182%) | |
| Average | 1000 | 7.622 | 6.175 | 1.443 | 6.009 | 1.607 | 0.16 (12%) |
| 100 | 4.402 | 2.237 | 2.161 | 1.988 | 2.41 | 0.25 (9%) | |
| 50 | 3.259 | 1.956 | 1.298 | 0 | 3.259 | 1.96 (151%) | |
| Typical Flood Hydrograph | Return Period | Current Method | Optimal Method | Comparison |
|---|---|---|---|---|
| 1954 | 1000 | 172.56 | 172.54 | 0.02 |
| 100 | 165.58 | 162.69 | 2.89 | |
| 50 | 160.38 | 158.57 | 1.81 | |
| 1968 | 1000 | 175 | 175 | 0 |
| 100 | 166.24 | 164.05 | 2.19 | |
| 50 | 163.27 | 161.21 | 2.06 | |
| 1980 | 1000 | 175 | 175 | 0 |
| 100 | 168.45 | 166.44 | 2.01 | |
| 50 | 165.54 | 163.21 | 2.33 | |
| 1981 | 1000 | 170.45 | 170.22 | 0.23 |
| 100 | 171.14 | 170.71 | 0.43 | |
| 50 | 166.98 | 166.24 | 0.74 | |
| 1982 | 1000 | 171.51 | 171.51 | 0 |
| 100 | 165.83 | 165.38 | 0.45 | |
| 50 | 162.77 | 162.68 | 0.09 | |
| 1988 | 1000 | 174.81 | 172.86 | 1.95 |
| 100 | 165.37 | 163.23 | 2.14 | |
| 50 | 164 | 158.67 | 5.33 | |
| 1996 | 1000 | 175 | 175 | 0 |
| 100 | 169.56 | 167.64 | 1.92 | |
| 50 | 161.35 | 159.16 | 2.19 | |
| 1998 | 1000 | 175 | 175 | 0 |
| 100 | 166.68 | 165.5 | 1.18 | |
| 50 | 160.3 | 158.65 | 1.65 | |
| 1999 | 1000 | 175 | 175 | 0 |
| 100 | 167.83 | 165.92 | 1.91 | |
| 50 | 161.76 | 160.02 | 1.74 | |
| 2010 | 1000 | 173.94 | 172.97 | 0.97 |
| 100 | 167.52 | 166.3 | 1.22 | |
| 50 | 162.03 | 160.08 | 1.95 | |
| Average | 1000 | 173.96 | 173.58 | 0.38 |
| 100 | 167.08 | 165.5 | 1.58 | |
| 50 | 162.65 | 160.93 | 1.72 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zha, G.; Zhou, J.; Yang, X.; Fang, W.; Dai, L.; Wang, Q.; Ding, X. Modeling and Solving of Joint Flood Control Operation of Large-Scale Reservoirs: A Case Study in the Middle and Upper Yangtze River in China. Water 2021, 13, 41. https://doi.org/10.3390/w13010041
Zha G, Zhou J, Yang X, Fang W, Dai L, Wang Q, Ding X. Modeling and Solving of Joint Flood Control Operation of Large-Scale Reservoirs: A Case Study in the Middle and Upper Yangtze River in China. Water. 2021; 13(1):41. https://doi.org/10.3390/w13010041
Chicago/Turabian StyleZha, Gang, Jianzhong Zhou, Xin Yang, Wei Fang, Ling Dai, Quansen Wang, and Xiaoling Ding. 2021. "Modeling and Solving of Joint Flood Control Operation of Large-Scale Reservoirs: A Case Study in the Middle and Upper Yangtze River in China" Water 13, no. 1: 41. https://doi.org/10.3390/w13010041
APA StyleZha, G., Zhou, J., Yang, X., Fang, W., Dai, L., Wang, Q., & Ding, X. (2021). Modeling and Solving of Joint Flood Control Operation of Large-Scale Reservoirs: A Case Study in the Middle and Upper Yangtze River in China. Water, 13(1), 41. https://doi.org/10.3390/w13010041




