Assessment of the Physically-Based Hydrus-1D Model for Simulating the Water Fluxes of a Mediterranean Cropping System
Abstract
1. Introduction
2. Materials and Methods
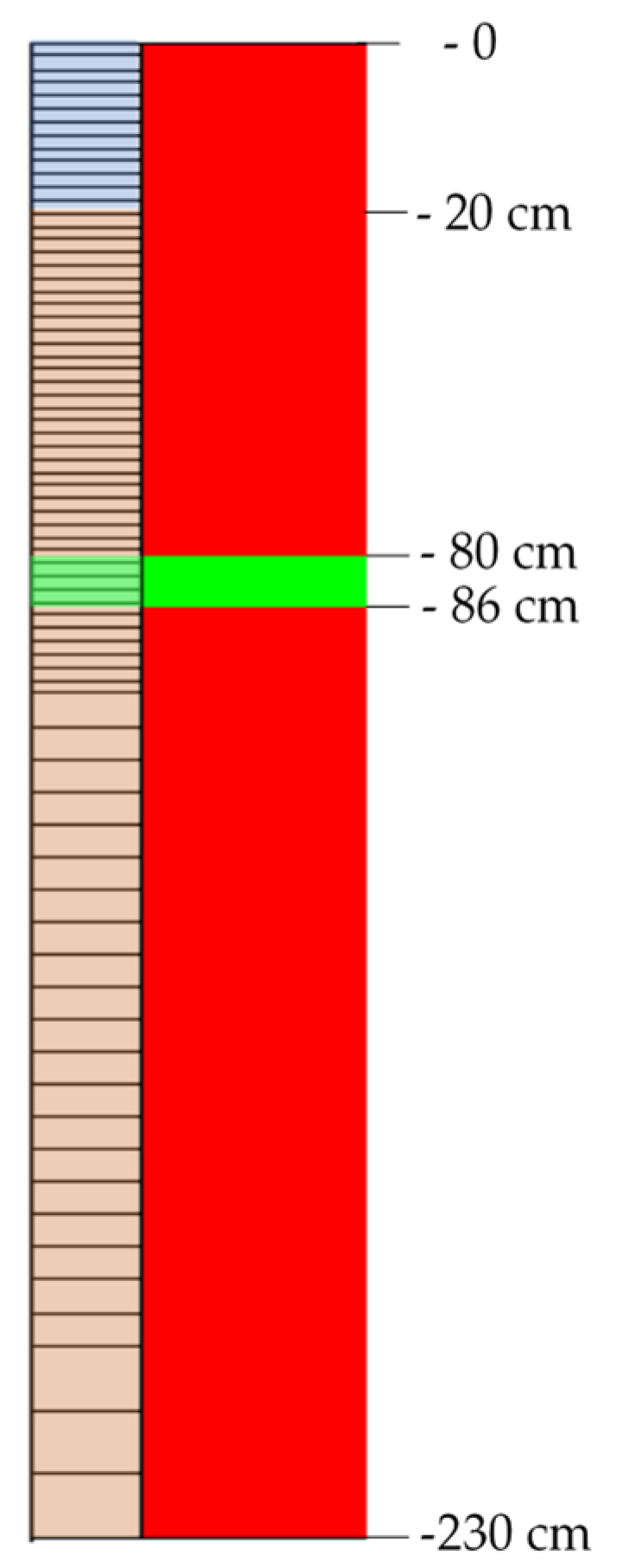
2.1. Experimental Site
2.2. Modelling Approach
2.3. Hydraulic Conductivity Measurements
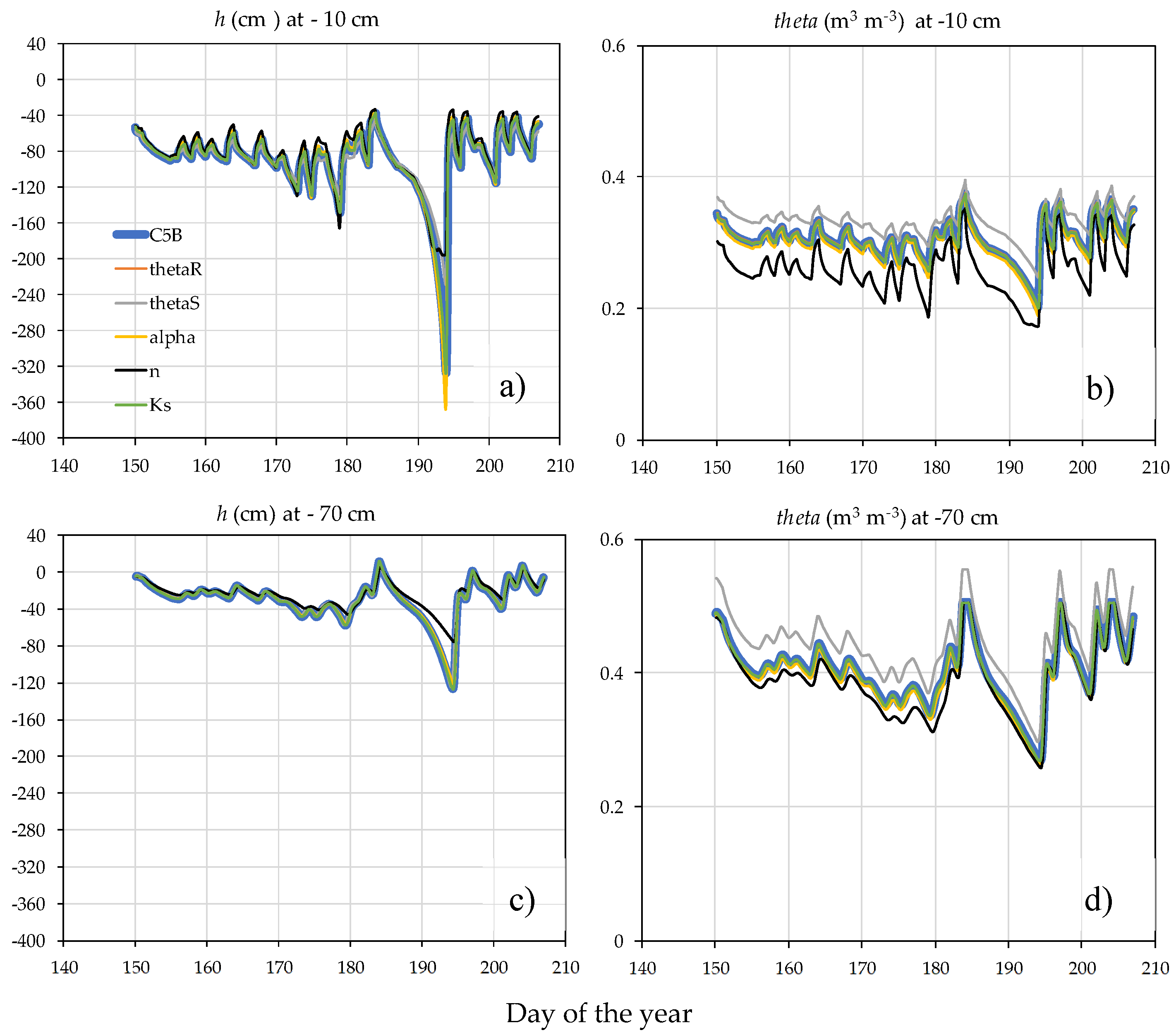
2.4. Parametrization Evaluation
3. Results
3.1. Field Meterological Characterization
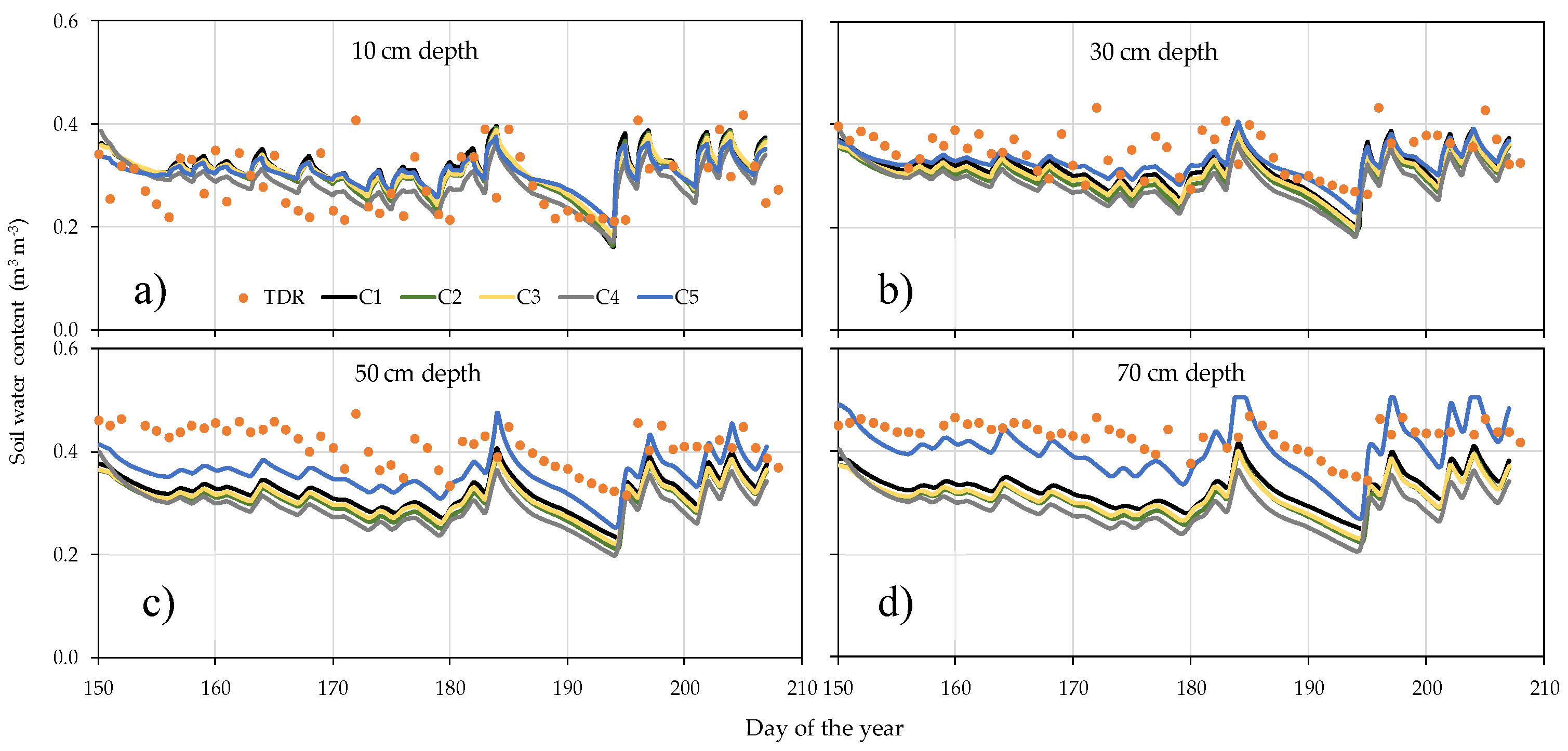
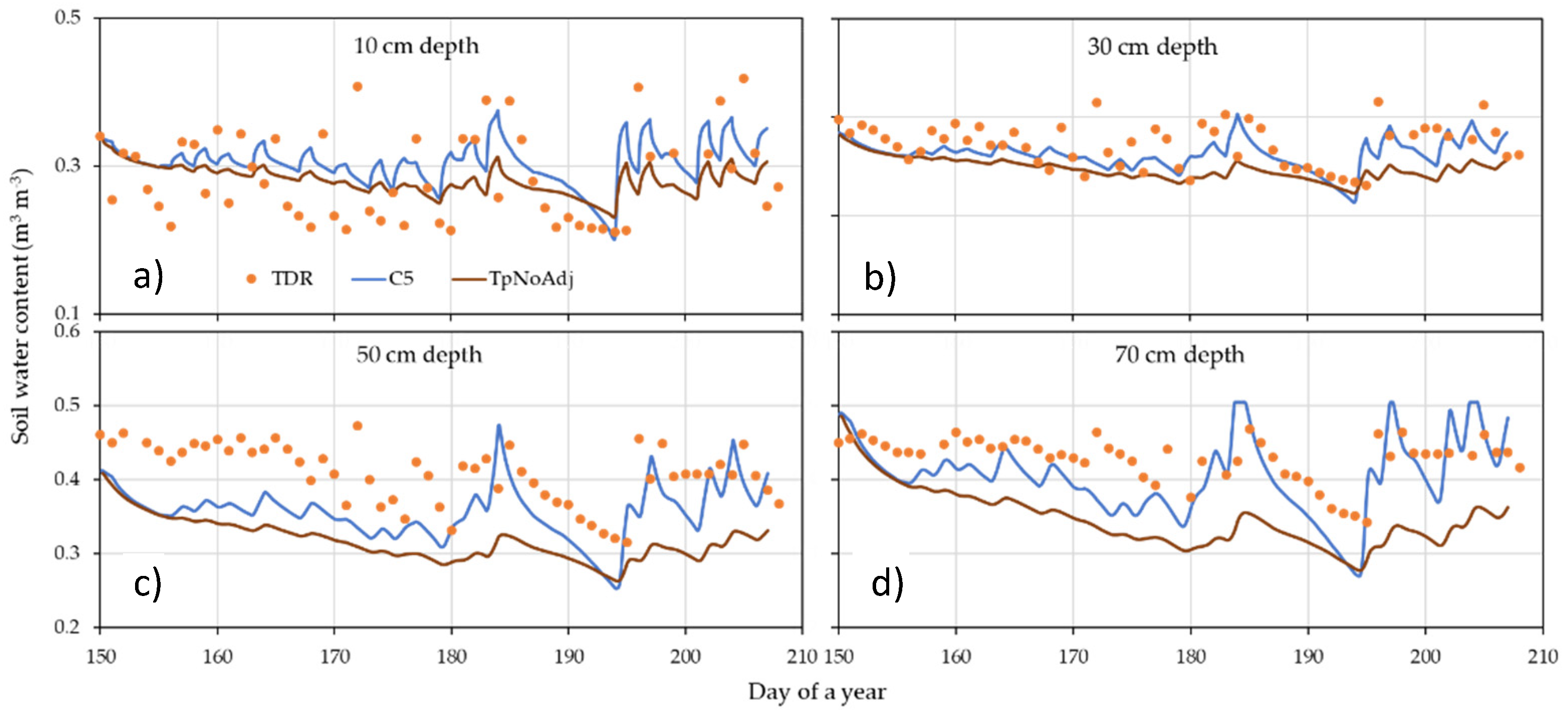
3.2. Calibration of Hydrus-1D
3.3. Saturated Hydraulic Conductivity Measurement Impact
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Ventrella, D.; Charfeddine, M.; Giglio, L.; Castellini, M. Application of DSSAT models for an agronomic adaptation strategy under climate change in Southern of Italy: Optimum sowing and transplanting time for winter durum wheat and tomato. Ital. J. Agron. 2012, 7, e16. [Google Scholar] [CrossRef]
- Autovino, D.; Rallo, G.; Provenzano, G. Predicting soil and plant water status dynamic in olive orchards under different irrigation systems with Hydrus-2D: Model performance and scenario analysis. Agr. Water Manag. 2018, 203, 225–235. [Google Scholar] [CrossRef]
- Kader, M.A.; Nakamura, K.; Senge, M.; Mojid, M.A.; Kawashima, S. Numerical simulation of water- and heat-flow regimes of mulched soil in rain-fed soybean field in central Japan. Soil Till. Res. 2019, 191, 142–155. [Google Scholar] [CrossRef]
- Pinheiro, E.A.R.; de Jong van Lier, Q.; Inforsato, L.; Šimůnek, J. Measuring full-range soil hydraulic properties for the prediction of crop water availability using gamma-ray attenuation and inverse modeling. Agr. Water Manag. 2019, 216, 294–305. [Google Scholar] [CrossRef]
- Silva Ursulino, B.; Maria Gico Lima Montenegro, S.; Paiva Coutinho, A.; Hugo Rabelo Coelho, V.; Cezar dos Santos Araújo, D.; Cláudia Villar Gusmão, A.; Martins dos Santos Neto, S.; Lassabatere, L.; Angulo-Jaramillo, R. Modelling soil water dynamics from soil hydraulic parameters estimated by an alternative method in a tropical experimental basin. Water 2019, 11, 1007. [Google Scholar] [CrossRef]
- Di Prima, S.; Castellini, M.; Abou Najm, M.R.; Stewart, R.D.; Angulo-Jaramillo, R.; Winiarski, T.; Lassabatere, L. Experimental assessment of a new comprehensive model for single ring infiltration data. J. Hydrol. 2019, 573, 937–951. [Google Scholar] [CrossRef]
- Šimůnek, J.; van Genuchten, M.T.; Šejna, M. Recent developments and applications of the HYDRUS computer software packages. Vadose Zone J. 2016, 15. [Google Scholar] [CrossRef]
- Ventrella, D.; Moahanty, B.P.; Šimůnek, J.; Losavio, N.; van Genuchten, M.T. Use of HYDRUS-1D for simulating water and chloride transport in a bare clayey soil in presence of shallow groundwater. Soil Sci. 2000, 165, 624–631. [Google Scholar] [CrossRef]
- Han, M.; Zhao, C.; Feng, G.; Yan, Y.; Sheng, Y. Evaluating the effects of mulch and irrigation amount on soil water distribution and root zone water balance using HYDRUS-2D. Water 2015, 7, 2622–2640. [Google Scholar] [CrossRef]
- Yang, Q.; Zuo, H.; Xiao, X.; Wang, S.; Chen, B.; Chen, J. Modelling the effects of plastic mulch on water, heat and CO2 fluxes over cropland in an arid region. J. Hydrol. 2012, 452–453, 102–118. [Google Scholar] [CrossRef]
- Zhang, H.; Huang, G.; Xu, X.; Xiong, Y.; Huang, Q. Estimating evapotranspiration of processing tomato under plastic mulch using the SIMDualKc model. Water 2018, 10, 1088. [Google Scholar] [CrossRef]
- Ghazouani, H.; Autovino, D.; Rallo, G.; Rallo, G.; Provenzano, G. Using HYDRUS-2D model to assess the optimal drip lateral depth for Eggplant crop in a sandy loam soil of central Tunisia. Ital. J. Agrometeorol. 2016, 1079, 47–58. [Google Scholar]
- Castellini, M.; Iovino, M. Pedotransfer functions for estimating soil water retention curve of Sicilian soils. Arch. Agron. Soil Sci. 2019, 65, 1401–1416. [Google Scholar] [CrossRef]
- Schaap, M.G.; Leij, F.J.; van Genuchten, M.T. Rosetta: A computer program for estimating soil hydraulic parameters with hierarchical pedotransfer functions. J. Hydrol. 2001, 251, 163–176. [Google Scholar] [CrossRef]
- Van Genuchten, M.T. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Topp, G.C.; Davis, J.L.; Annan, A.P. Electromagnetic determination of soil water content: Measurements in coaxial transmission lines. Water Resour. Res. 1980, 16, 574–582. [Google Scholar] [CrossRef]
- Allen, R.G.; Pereira, L.S.; Raes, D.; Smith, M. Crop evapotranspiration—Guidelines for computing crop water requirements. In FAO Irrigation and Drainage; Paper 56; Food and Agriculture Organization: Rome, Italy, 1998; p. 15. [Google Scholar]
- Šimůnek, J.; Šejna, M.; Saito, H.; Sakai, M.; Van Genuchten, M.T. The HYDRUS-1D Software Package for Simulating the One-Dimensional Movement of Water, Heat, and Multiple Solutes in Variably-Saturated Media; Version 4.17; Department of Environmental Sciences University of California Riverside: Riverside, CA, USA, 2013. [Google Scholar]
- Feddes, R.A.; Kowalik, P.J.; Zaradny, H. Simulation of field water use and crop yield. In Simulation of Field Water Use and Crop Yield. Simul. Monogr.; Pudoc: Wageningen, The Netherlands, 1978; p. 188. [Google Scholar]
- Wind, G.P. Capillary conductivity data estimated by a simple method. In Water in the Unsaturated Zone Proc Wageningen Symp; Institute for Land and Water Management Research: Amsterdam, The Netherlands, 1969. [Google Scholar]
- Klute, A.; Dirksen, C. Hydraulic conductivity and diffusivity: Laboratory methods. In Methods of Soil Analysis: Part 1—Physical and Mineralogical Methods; American Society of Agronomy-Soil Science Society of America: South Segoe Road, MA, USA, 1986; pp. 687–734. [Google Scholar]
- Bagarello, V.; Castellini, M.; Iovino, M. Comparison of unconfined and confined unsaturated hydraulic conductivity. Geoderma 2007, 137, 394–400. [Google Scholar] [CrossRef]
- Halbertsma, J.M.; Veerman, G.J. A new calculation procedure and simple set-up for the evaporation method to determine soil hydraulic functions. In Report. 88; Wageningen: Amsterdam, The Netherlands, 1994; p. 21. [Google Scholar]
- Castellini, M.; Di Prima, S.; Iovino, M. An assessment of the BEST procedure to estimate the soil water retention curve: A comparison with the evaporation method. Geoderma 2018, 320, 82–94. [Google Scholar] [CrossRef]
- Coelho, E.F.; Or, D. Root distribution and water uptake patterns of corn under surface and subsurface drip irrigation. Plant Soil 1999, 206, 123–136. [Google Scholar] [CrossRef]
- Braud, I.; De Condappa, D.; Soria, J.M.; Haverkamp, R.; Angulo-Jaramillo, R.; Galle, S.; Vauclin, M. Use of scaled forms of the infiltration equation for the estimation of unsaturated soil hydraulic properties (the Beerkan method). Eur. J. Soil Sci. 2005, 56, 361–374. [Google Scholar] [CrossRef]
- Lassabatere, L.; Angulo-Jaramillo, R.; Soria Ugalde, J.M.; Cuenca, R.; Braud, I.; Haverkamp, R. Beerkan estimation of soil transfer parameters through infiltration experiments—BEST. Soil Sci. Soc. Am. J. 2006, 70, 521. [Google Scholar] [CrossRef]
- Stewart, R.D.; Abou Najm, M.R. A Comprehensive Model for Single Ring Infiltration I: Initial Water Content and Soil Hydraulic Properties. Soil Sci. Soc. Am. J. 2018, 82, 548–557. [Google Scholar] [CrossRef]
- Bagarello, V.; Di Prima, S.; Iovino, M. Estimating saturated soil hydraulic conductivity by the near steady-state phase of a Beerkan infiltration test. Geoderma 2017, 303, 70–77. [Google Scholar] [CrossRef]
- Fila, G.; Bellocchi, G.; Acutis, M.; Donatelli, M. Irene: A software to evaluate model performance. Eur. J. Agron. 2003, 18, 369–372. [Google Scholar] [CrossRef]
- Kobayashi, K.; Salam, M.U. Comparing simulated and measured values using mean squared deviation and its components. Agron J. 2000, 92, 345–352. [Google Scholar] [CrossRef]
- Greenwood, D.J.; Neeteson, J.J.; Draycott, A. Response of potatoes to N fertilizer: Dynamic model. Plant Soil 1985, 85, 185–203. [Google Scholar] [CrossRef]
- Willmott, C.J.; Wicks, D.E. An Empirical method for the spatial interpolation of monthly precipitation within California. Phys. Geogr. 1980, 1, 59–73. [Google Scholar] [CrossRef]
- Loague, K.; Green, R.E. Statistical and graphical methods for evaluating solute transport models: Overview and application. J. Contam. Hydrol. 1991, 7, 51–73. [Google Scholar] [CrossRef]
- Shaeffer, D.L. A model evaluation methodology applicable to environmental assessment models. Ecol. Model. 1980, 8, 275–295. [Google Scholar] [CrossRef]
- Ventrella, D.; Castellini, M.; Di Giacomo, E.; Giglio, L. Valutazione di diversi metodi di misura per il monitoraggio del contenuto idrico del suolo. In Proceedings of the XXXVII Conference of Italian Society of Agronomy, Il Contributo della Ricerca Agronomica all’Innovazione dei Sistemi Colturali Mediterranei, Catania, Italy, 13–14 September 2007; Cosentino, S.D., Tuttobene, R., Eds.; pp. 249–250. [Google Scholar]
- Bagarello, V.; Baiamonte, G.; Castellini, M.; Di Prima, S.; Iovino, M. A comparison between the single ring pressure infiltrometer and simplified falling head techniques. Hydrol. Process. 2014, 28, 4843–4853. [Google Scholar] [CrossRef]
- Fiorentino, C.; Ventrella, D.; Giglio, L.; Giacomo, E.D.; Lopez, R. Land use cover mapping of water melon and cereals in southern Italy. Ital. J. Agron. 2010, 5, 185–192. [Google Scholar] [CrossRef]
- Rallo, G.; Provenzano, G.; Castellini, M.; Sirera, À.P. Application of EMI and FDR Sensors to assess the fraction of transpirable soil water over an olive grove. Water 2018, 10, 168. [Google Scholar] [CrossRef]
- Liang, X.; Liakos, V.; Wendroth, O.; Vellidis, G. Scheduling irrigation using an approach based on the van Genuchten model. Agr. Water Manag. 2016, 176, 170–179. [Google Scholar] [CrossRef]
- Vereecken, H.; Huisman, J.A.; Bogena, H.; Vanderborght, J.; Vrugt, J.A.; Hopmans, J.W. On the value of soil moisture measurements in vadose zone hydrology: A review. Water Resour. Res. 2008, 44, W00D06. [Google Scholar] [CrossRef]
- Pirastru, M.; Niedda, M.; Castellini, M. Effects of maquis clearing on the properties of the soil and on the near-surface hydrological processes in a semi-arid Mediterranean environment. J. Agric. Eng. 2014, 45, 176. [Google Scholar] [CrossRef]
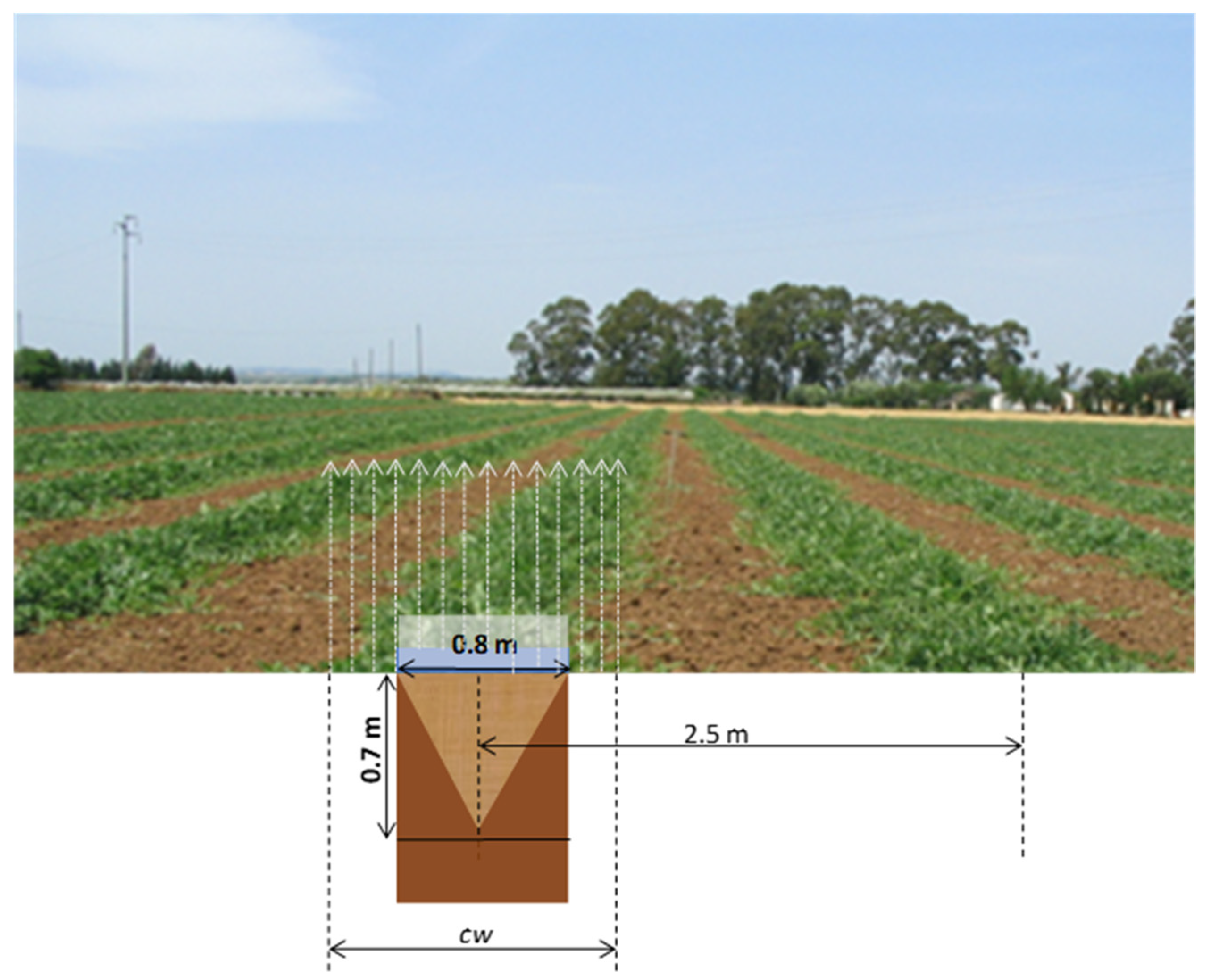
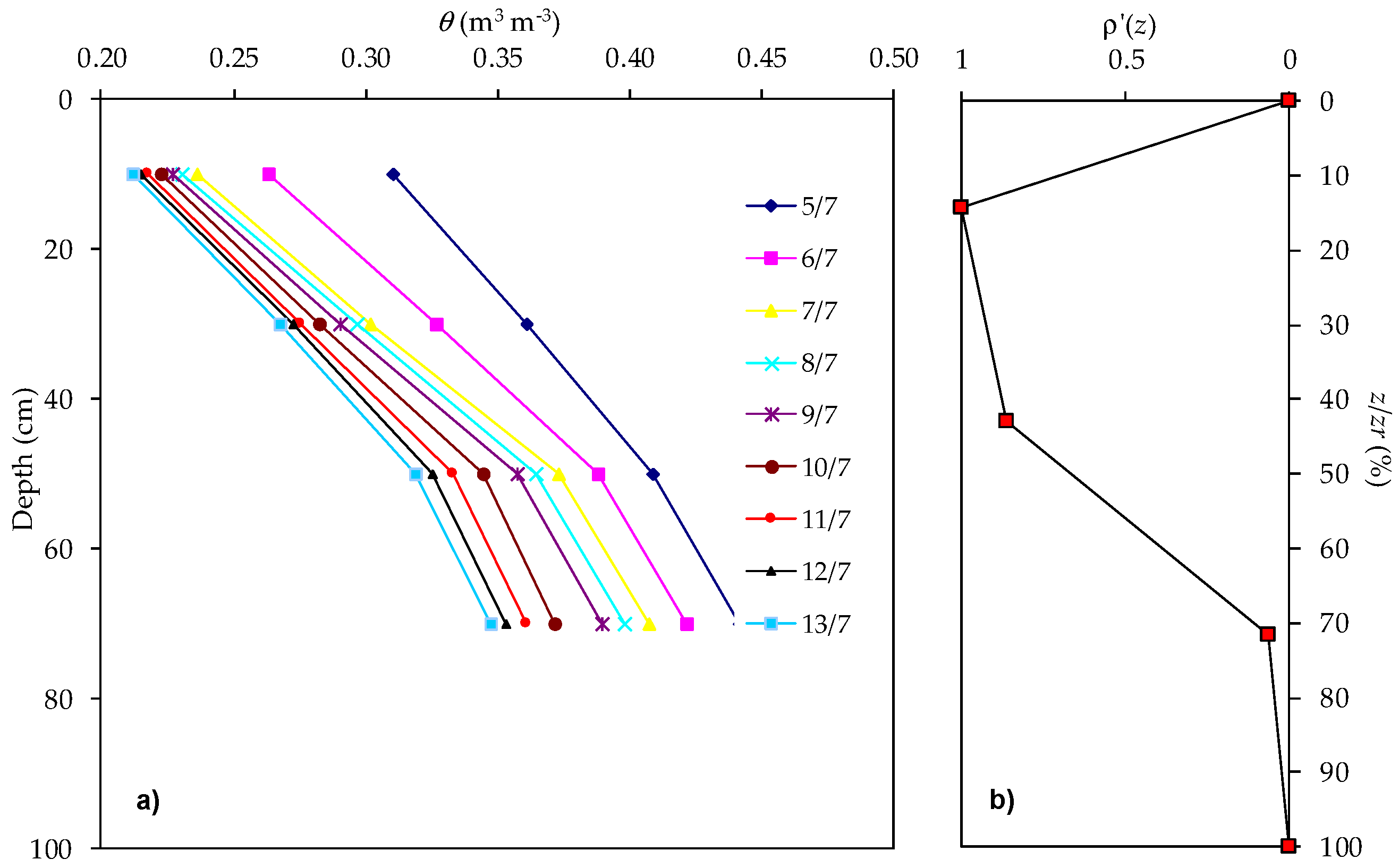
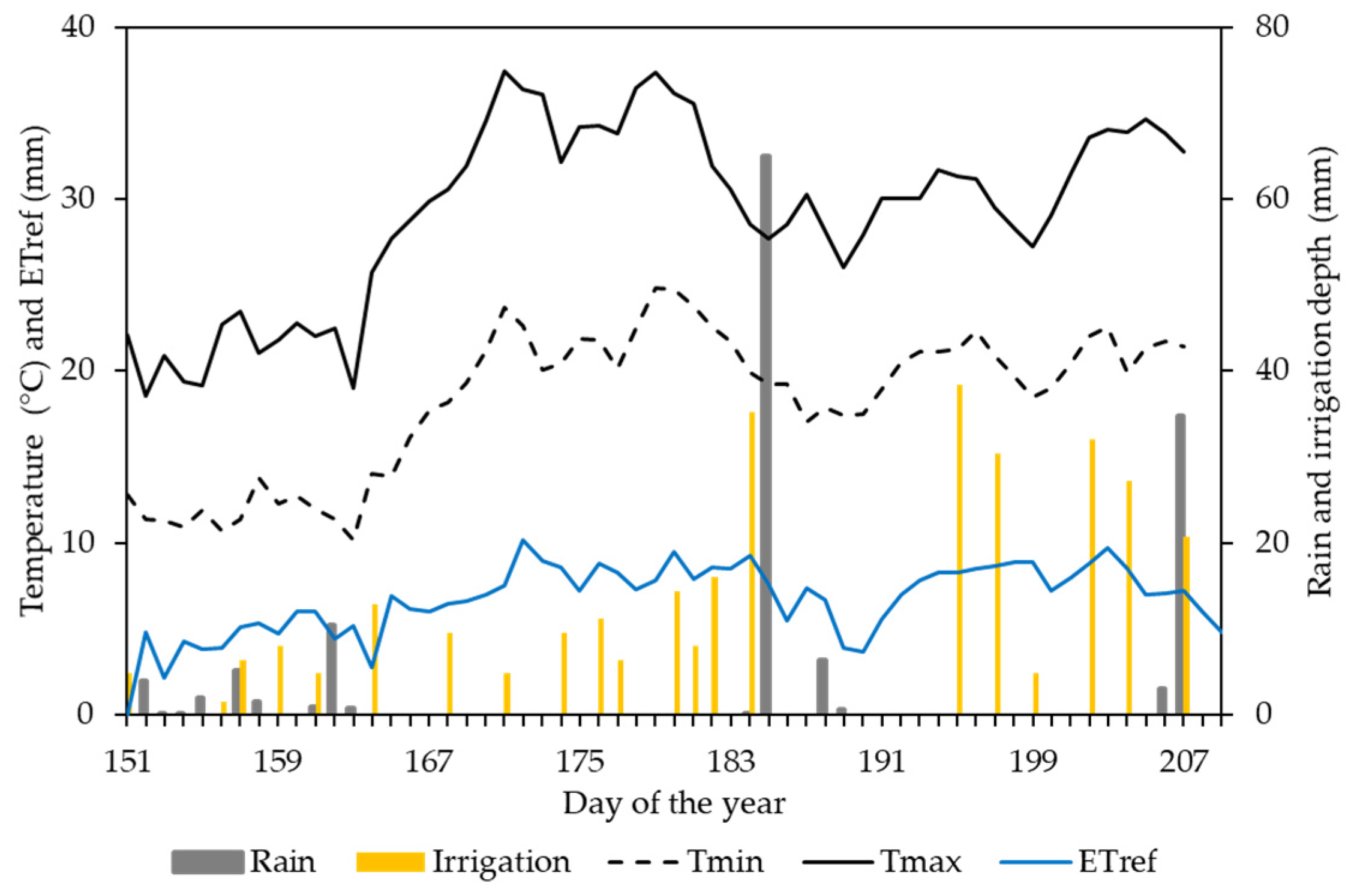

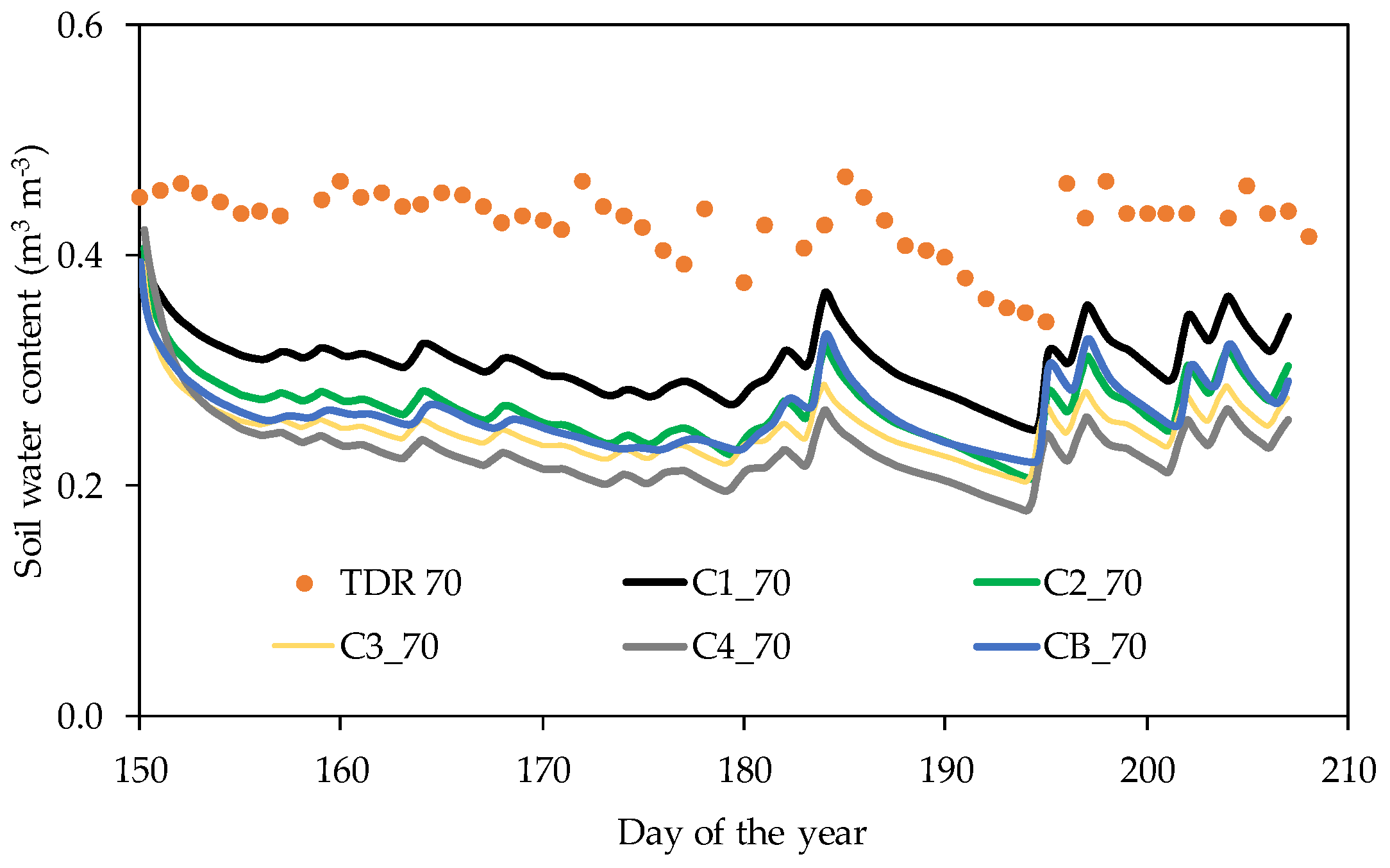
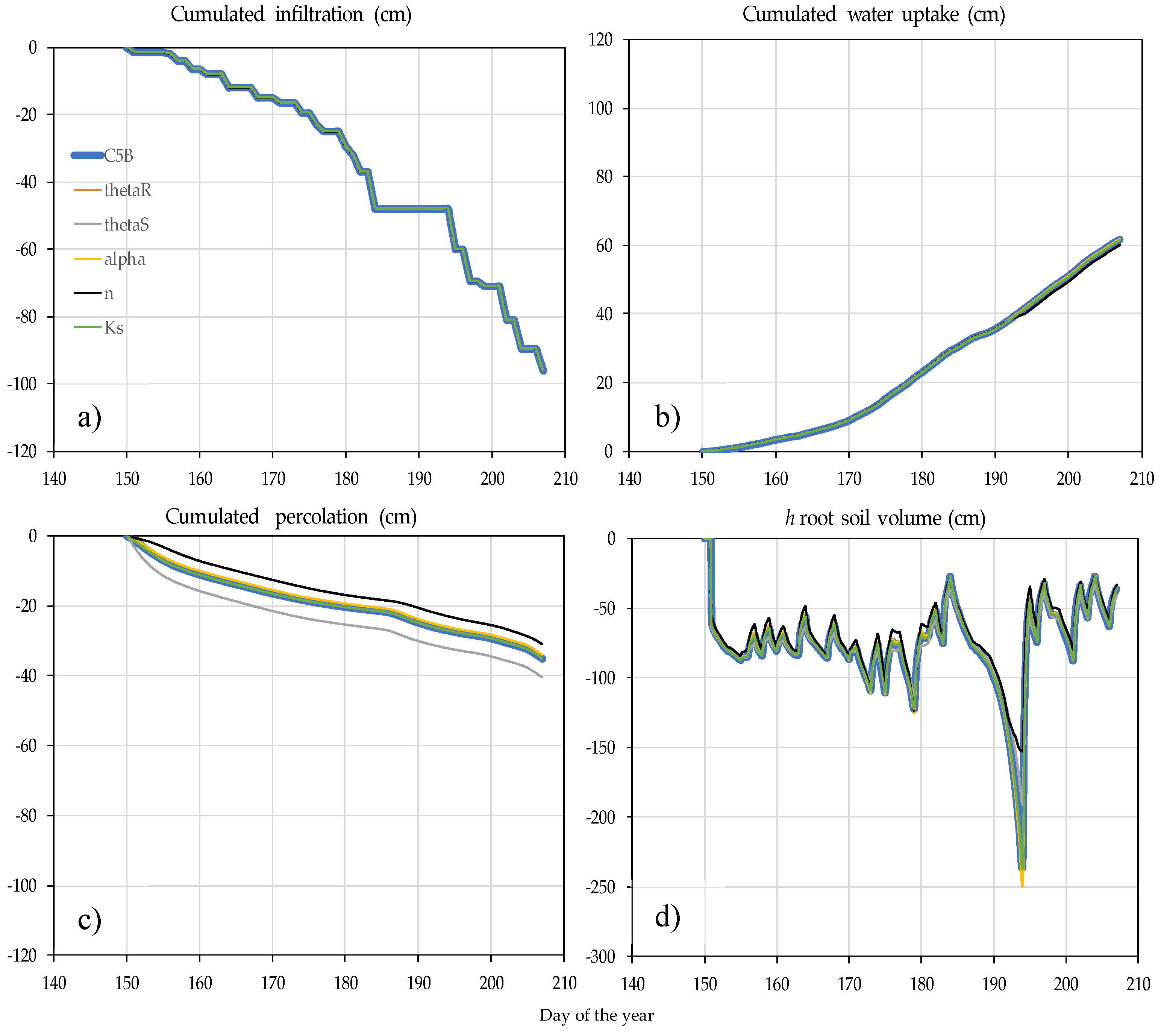
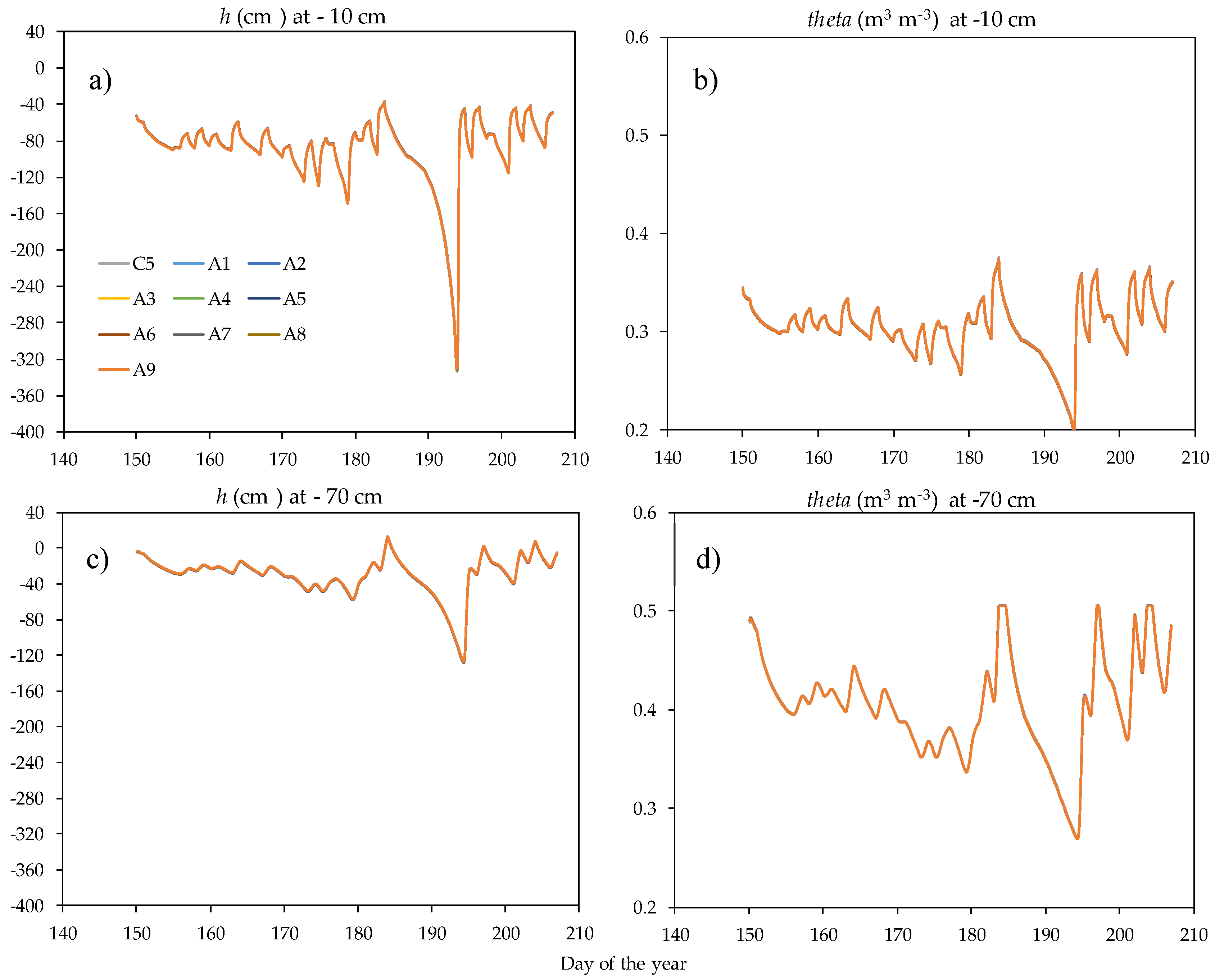
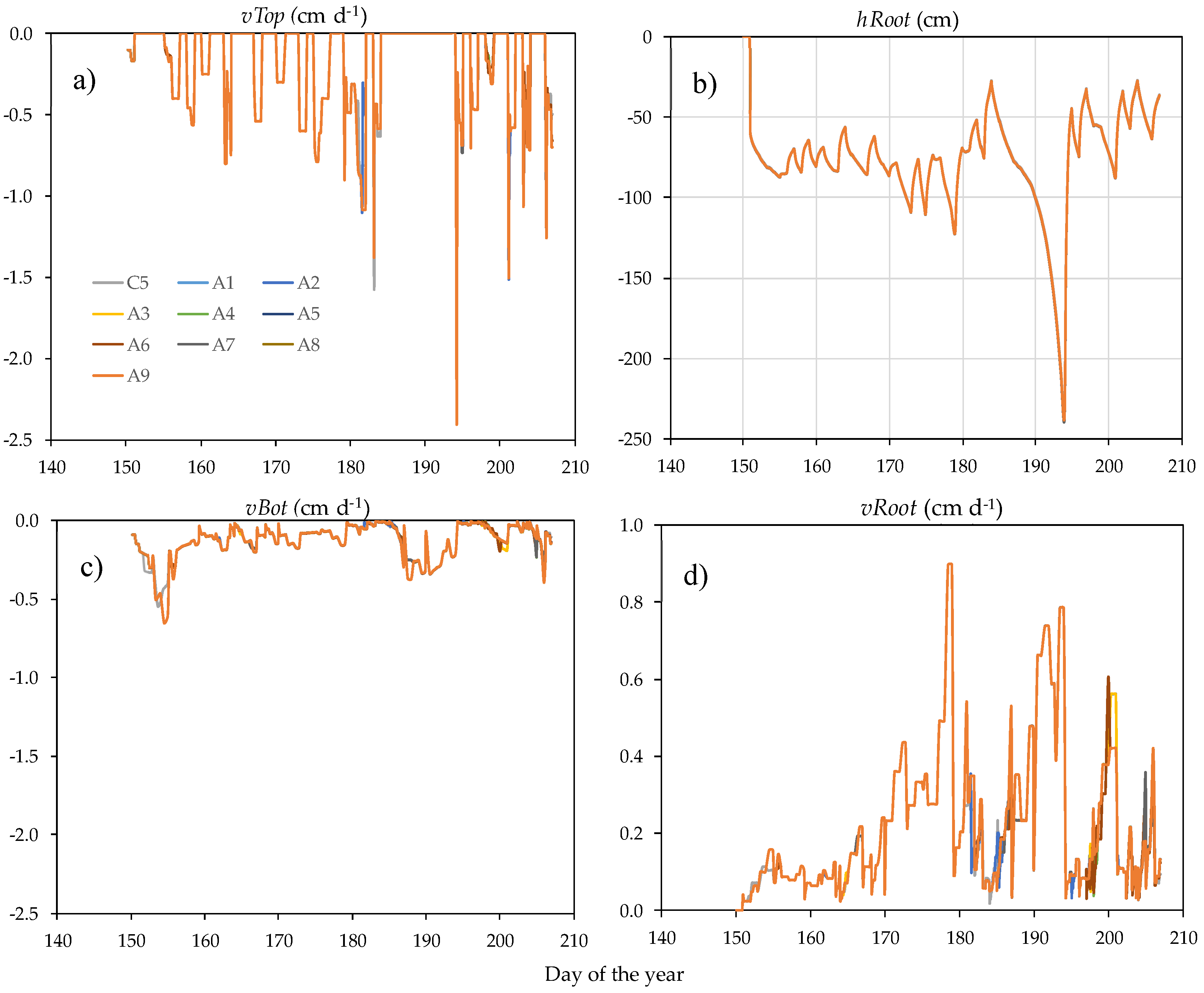
| Parameter | Unit | First Layer 0–20 cm | Second Layer 20–40 cm |
|---|---|---|---|
| pH | 8.23 | 8.15 | |
| Electrical conductivity | dS m−1 | 0.366 | 0.4632 |
| Total organic carbon | g kg−1 | 10.0 | 9.7 |
| Total nitrogen | g kg−1 | 0.99 | 0.99 |
| Sand | % | 16.48 | 16.33 |
| Silt | % | 36.10 | 37.32 |
| Clay | % | 47.43 | 46.35 |
| Dry bulk density | g cm−3 | 1.27 | 1.36 |
| Field capacity | cm3 cm−3 | 0.37 | 0.38 |
| Permanent wilting point | cm3 cm−3 | 0.19 | 0.20 |
| Approach | Layer (cm) | θr | θs | α | n | Ks |
|---|---|---|---|---|---|---|
| C1 | 0–20 | 0.0911 | 0.4554 | 0.0204 | 1.2546 | 11.36 |
| 21–230 | 0.0910 | 0.4577 | 0.0193 | 1.2667 | 10.76 | |
| C2 | 0–20 | 0.0992 | 0.5015 | 0.0185 | 1.3257 | 24.74 |
| 21–230 | 0.0955 | 0.4738 | 0.0172 | 1.325 | 15.75 | |
| C3 | 0–20 | 0.0901 | 0.4986 | 0.021 | 1.2525 | 37.84 |
| 21–230 | 0.0890 | 0.4714 | 0.0177 | 1.2536 | 20.10 | |
| C4 | 0–20 | 0.0722 | 0.4916 | 0.0076 | 1.378 | 30.75 |
| 21–230 | 0.0706 | 0.4694 | 0.0061 | 1.392 | 18.05 | |
| C5 | 0–230 | 0.1784 | 0.5049 | 0.0522 | 1.324 | 116.41 |
| Approach | RMSE * | rRMSE | ME | IA | CD | RM | AE | R2 | a | b | SE | RI |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| C1 | 0.064 | 0.169 | −1.74 | 0.622 | 3.28 | 0.157 | 0.060 | 0.81 | 0.105 | 0.858 | 0.022 | 3.95 |
| C2 | 0.079 | 0.209 | −3.19 | 0.548 | 4.76 | 0.199 | 0.075 | 0.81 | 0.128 | 0.825 | 0.023 | 2.18 |
| C3 | 0.073 | 0.194 | −2.60 | 0.569 | 4.11 | 0.184 | 0.070 | 0.82 | 0.102 | 0.895 | 0.022 | 3.41 |
| C4 | 0.093 | 0.247 | −4.85 | 0.493 | 6.44 | 0.237 | 0.090 | 0.78 | 0.156 | 0.770 | 0.024 | 1.00 |
| C5 | 0.035 | 0.093 | 0.16 | 0.814 | 1.43 | 0.067 | 0.028 | 0.80 | 0.097 | 0.798 | 0.023 | 4.45 |
| Perfect fit | 0 | 0 | 1 | 1 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 5 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ventrella, D.; Castellini, M.; Di Prima, S.; Garofalo, P.; Lassabatère, L. Assessment of the Physically-Based Hydrus-1D Model for Simulating the Water Fluxes of a Mediterranean Cropping System. Water 2019, 11, 1657. https://doi.org/10.3390/w11081657
Ventrella D, Castellini M, Di Prima S, Garofalo P, Lassabatère L. Assessment of the Physically-Based Hydrus-1D Model for Simulating the Water Fluxes of a Mediterranean Cropping System. Water. 2019; 11(8):1657. https://doi.org/10.3390/w11081657
Chicago/Turabian StyleVentrella, Domenico, Mirko Castellini, Simone Di Prima, Pasquale Garofalo, and Laurent Lassabatère. 2019. "Assessment of the Physically-Based Hydrus-1D Model for Simulating the Water Fluxes of a Mediterranean Cropping System" Water 11, no. 8: 1657. https://doi.org/10.3390/w11081657
APA StyleVentrella, D., Castellini, M., Di Prima, S., Garofalo, P., & Lassabatère, L. (2019). Assessment of the Physically-Based Hydrus-1D Model for Simulating the Water Fluxes of a Mediterranean Cropping System. Water, 11(8), 1657. https://doi.org/10.3390/w11081657







