Tidal Flats Morphodynamics: A new Conceptual Model to Predict Their Evolution over a Medium-Long Period

Abstract

The morphological evolution of tidal flats has been widely investigated in recent years as it represents a very important topic which is highly related to the climate-driven environmental changes. The period over which geomorphological changes can be noted is a multi-year up to pluri-decennial time scale, defined as medium-long period. This work presents a new conceptual model which is able to predict and estimate a limit depth between an erosion condition and a no-erosion condition for tidal flats. The domains of applicability are shallow and confined basins, where tidal flats are characterized by near-horizontal topography, as occurs inside lagoons. The theoretical approach provides a general equation which relates the limit depth of tidal flats to current velocity and critical erosion shear stress. The procedure, followed through to its development, takes into account the important role of the bottom friction dissipation in wind wave generation process for shallow water. The relationship between tidal flat depth, current velocity and critical shear stress is provided in three different configurations, depending on the direction of the wave motion compared to the current. The limit depth compared to the measured depth can suggest if tidal flats tend or not towards an erosion state over a medium-long period. In this sense, the conceptual model provides a relevant contribution to the comprehension of morphodynamics of these important environments. This approach has been validated with its application to a real context and the results are provided in the paper.

1. Introduction

Tidal flat landforms in coastal and estuarine systems include a great variety of environments, which are constantly shaped by complex physical and biological factors [1,2]. As transitional environments, tidal flats are periodically submerged and exposed under tide and wave driven processes which deeply affect the sediment transport mechanisms [3,4,5,6,7,8,9]. Moreover, sediment supply and fluvial inflow as well as organic and anthropic activities can alter their morphology [10,11,12,13]. According to Friedrichs [5], sediment transport mechanisms induced by tides and waves also involve the gradual morphological transition beyond low tide level. For this reason, in this paper the term “tidal flats” is used in its dynamic meaning, which includes both the actual tidal-flats, in the strict sense, and the sub-tidal flats.

Due to their particular nature, tidal flats are fundamental to estuarine and lagoon morphodynamics and their ecological and socio-economic importance is widely recognized [14,15,16]. At the same time, the fragile balance of these environments can interfere with human-induced changes, such as shoreline protection intervention or dredging operations for the maintenance of waterways navigability. Within this context, the possible configurations of tidal flats over a medium-long period, become an essential element for the management and sustainability of coastal areas [17]. The term “medium-long” refers to a multi-year up to a pluri-decennial time scale over which the geomorphological changes can be noted.

Many studies have investigated morphological changes over a medium-long period occurring in coastal lagoon and tidal flat environments, in order to assess the erosional/accretionary pattern, and the relative sediment budget. These studies consider geomatics techniques based on historical cartography, photogrammetry, topography [18,19,20,21,22] or on the satellite image processing [23]. Direct measurements and monitoring of bed level changes in tidal flats are quite difficult to carry out, due to the complexity of the environment and the lack of appropriate measuring equipment and methods [24]. Only recent studies have provided detailed quantification of high-resolution short-term (from daily to seasonal time scale) bed level dynamics, coupled with hydrodynamic data [24,25,26,27].

In order to improve understanding and predicting of how a tidal flat can evolve over a medium-long time scale, theoretical and numerical approaches have been developed, which need monitoring and measurement data in order to be applied. This research is based on a proper definition of an equilibrium pattern which can lead to a stable morphology [4,5,7,28,29,30,31]. In fact, tides, wind and wave climate operate over a wide range of scales, from that of a storm, to the semidiurnal or diurnal cycle to seasonal, annual or decade long periods. In this sense, the concept of quasi-equilibrium [4] or dynamic equilibrium [5,28,29,30] is preferred as opposed to an unrealistic static condition in which no sediment is transported. Equivalently, this means that erosion is balanced by deposition at each point of the tidal flat, when considered over a suitably long period [4,30].

Starting from this assumption, the cross-shore profile shapes of tidal flats exposed to the sea, typical of estuarine environments, have been widely investigated through experimental observations [32,33,34], and numerical solutions [4,5,17,35,36,37]. Given the complexity of the processes involved, the results obtained are generally only qualitative and strongly conditioned by the necessary simplifications that have been made.

Among the first studies, Friedrichs and Aubrey [38] proposed the dynamic equilibrium theory, based on a simplified analytical approach, useful to explain the morphological responses of intertidal systems. This method assumes that the cross-shore profile of a tidal flat is stable when the maximum bed shear stress during a tidal cycle is uniform over space, ensuring no net deposition or erosion anywhere in the system. Taking into account annual or longer time scales, the profile approximates a concave shape if it is purely wave-dominated, while it results convex if the tide is prevailing in the overall balance. If a shorter timescale is considered, tidal flat morphology can evolve dynamically between these two configurations depending on the bed shear stress variability.

Subsequent and more complex models [4,8,35,39] have been used to simulate the mudflat profile shapes in the 2D-vertical plane, finding results which qualitatively agree with those of Friedrichs and Aubrey [38]. Hu et al. [28] have extended the dynamic equilibrium theory by explicitly taking into account a spatiotemporal bed shear stress variation to predict tidal flat morphodynamics. In this sense, the developed model overcomes the limit of the original analytical solution of Friedrichs and Aubrey [38] that is based on the assumption of a uniform bed shear stress distribution that may not occur in reality.

A similar choice of a reference shear stress for long-term modeling is also followed by Mariotti and Fagherazzi [40] who proposed a dynamic model to study the morphological evolution of fetch-limited basins or lagoons. The analyzed configuration is typical of tidal flats which are sheltered from the sea by barrier islands. In these cases, tidal flats are inherently horizontal with a very small slope and such that they can be characterized in a sufficient area by a uniform depth value. Moreover, the wave motion is locally generated here and it presents lower periods and wave heights [41,42,43,44,45]. Mariotti and Fagherazzi [46] feature the non–monotonic relationship between wave shear stress and depth, which represents the focus point of a previous conceptual model, which was proposed by Fagherazzi et al. [47]. This non-monotonic distribution of the bed shear stress with depth is then compared to a critical erosion threshold for cohesive sediments and a fixed deposition rate. The conceptual approach has been subsequently improved taking into account the effects of a collinear current shear stress and different sediment inputs [48]. Starting from similar assumptions some numerical applications to the Venice Lagoon have been conducted with the aim to describe the morphological evolutionary trend through hypsometry, which corresponds to frequency area distribution related to bottom elevation [18,49].

A recent line of research deals with the role played both by bottom friction and wave period on the wave bottom shear stress, which is fundamental in coastal morphodynamics [45,50]. It has been numerically shown that the bottom friction dissipation can severely limit the wave field with the consequence that the relative curve of bed shear stress loses the bell shape, tending instead to decrease monotonically with the water depth [45]. In this sense, the wave motion is able to induce sediment transport only when very strong winds are considered, which are however rare. Experimental findings [29] support this outcome, that necessarily leads to reviewing the processes that drive sediment transport over a tidal cycle or a longer time scale, in order to identify the conditions for the morphodynamic equilibrium of tidal flats. For these reasons, the conceptual model briefly recalled can be revised and extended.

In the present paper a new theoretical approach is proposed with the aim of providing a reasonable morphodynamic trend over a medium-long period for tidal flats in sheltered environments. This method gives the equation to derive a limit depth value that can suggest if the tidal flat tends, or not towards an erosion state. The maximum shear stress caused both by the wave motion and the current field is compared to the critical threshold of erosion which represents the resistance of the bottom to sediment mobilization. The wave shear stress is numerically computed by means of a generation process with a morphologically representative wind speed and a proper representation of bottom dissipation. The relationship between tidal flat depth, current velocity and critical shear stress is provided in three different configurations, depending on the direction of the wave motion compared to the current.

With the aim of validating the new conceptual model, an application to the Marano and Grado lagoon in the Northern Adriatic Sea (Italy) has been conducted, starting from two bathymetries detected 45 years apart and numerical data obtained from a previous application by means of a spectral–morphodynamic coupled model [51].

2. Tidal Flat Morphodynamics: Some Preliminary Considerations

Within lagoons and sheltered areas, tidal currents are undoubtedly dominant for sediment transport close to tidal inlets and inside the main channels, where higher current velocities can profoundly incise the bed [52,53,54,55,56,57,58]. On the other hand, wave motion is decisive in the resuspension of sediments from the tidal flats bottom, where shallow depths limit current velocities and the corresponding values of shear stress [3,9,40,48,51,54,55]. Even the small waves generated by local moderate winds can contribute to morphological stability in the long-term period, the wave orbital motion being able to penetrate down to the water depth [3,7,9].

The generation process of wind waves on shallow depths leads to quite different characteristics from offshore waves developed on deep waters, as the friction dissipation severely restricts the maximum value that wave heights and periods can reach [3,45]. This particular aspect has been verified both experimentally and numerically and it has led to a set of equations formulated for wave forecasting in shallow water [43,59]. However, the growth curves, extensively used in many applications [40,46,55,60], may not be exhaustive in order to correctly interpret the wave field. In fact, they have been determined by adopting a low friction factor, generally representative of very smooth, homogenous and flat beds.

In the presence of a more irregular bottom, characterized by bedforms (uneven granulometry with coexistence of sand and mud, and vegetation), the wave friction factor can considerably increase. This result was achieved by the authors in a previous study [45], performing a numerical generation process by means of the third generation spectral model SWAN [61], based on the complete wave action balance equation.

A regular computational grid has been chosen to reproduce the generation process in a microtidal limited-fetch environment, where the tidal flats are characterized by near-horizontal topography, such the Marano and Grado or the Venice lagoons mapped in Figure 1.

Several configurations of depth, wind speed and bed roughness have been set to establish the role played by bottom friction and to identify the spectral approach that better reproduces the energy dissipation of locally generated waves. The comparison between the available formulations in SWAN, has outlined that the Madsen model [62] is more suitable to be applied in such coastal and transitional environments. The previously mentioned study by Pascolo et al. [45] has verified that an equivalent roughness of a few centimeters is sufficient to considerably limit the wave orbital velocity U_w computed at the bottom.

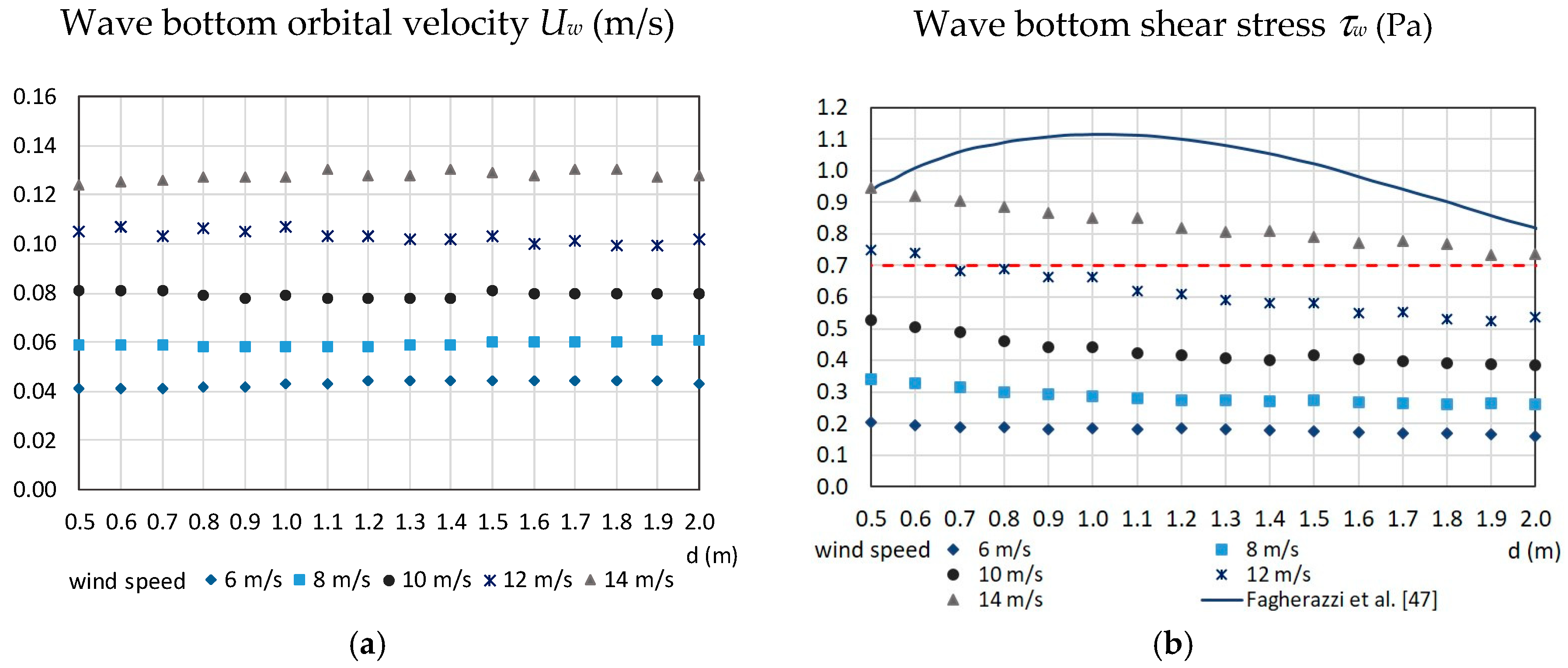
As depicted in Figure 2a for different wind speed values, these wave velocities tend to follow an almost constant trend on the tidal flat depths, herein indicated as d, commonly included in the range 0.5–2 m below still water level.

Pascolo et al. [45] have also shown that the shallow water generation process is substantially independent from the fetch length, as the fully developed sea state can be reached on very short distances, less than 5 km.

The more relevant consequence, decisive for morphodynamic processes, is the considerable reduction of the wave bottom shear stress acting on the sediment grains. The wave bottom shear stress depends on the wave velocity according to the following quadratic law:

$${\tau }_w=\frac{1}{2}\rho f_w{U}_w^2,$$

(1)

where ρ is the water density, f_w is the friction factor and U_w the maximum wave bottom orbital velocity. The friction factor is evaluated by means of the Soulsby formulation [65]:

$$f_w=1.39{\left(\frac{A}{z_0}\right)}^{-0.52},$$

(2)

A = U_wT/2π being one half of the horizontal orbital excursion, T the wave period, z₀ = K_N/30, and K_N the equivalent Nikuradse roughness. This last parameter is generally taken as a function of the median diameter if the bed is composed of coarse grains, while for fine cohesive grains or mud-beds, K_N can be set equal to a few millimeters as an approximation of the mud protrusions height [66].

Figure 2b highlights how the bed shear stress computed assuming K_N = 0.005 m, tends to be quite flat and homogeneous for the analyzed depths. These trends are very different from the non-monotonic curve suggested by Fagherazzi et al. [47] for a wind speed of 8 m/s, as already discussed by Pascolo et al. [45]. The reason could be attributable to the assumptions of a K_N value equal to 5 × 10⁻⁵ m and above all monochromatic wind waves, generated with an imposed constant wave period.

The dashed line refers to a critical shear stress for cohesive sediments of 0.7 Pa evaluated as a mean value for the tidal flats of the Venice lagoon [63,64]. The same value has been adopted successfully in several applications in some coastal environments of the North Adriatic Sea [47,48,51,54,67]. It can be noticed that the non-monotonic curve derived by Fagherazzi et al. [47] exceeds the critical shear stress over the whole analyzed domain. On the other hand, the wave stress component on rougher bed, is sufficient to resuspend sediments from the bottom only for high wind speeds and therefore extreme events. This means that the current velocity becomes truly decisive in triggering the transport process in presence of moderate winds, which are considered representative for the morphological evolution over timescale longer than that of a storm [5,40]. The new conceptual model, which is proposed in the present paper, is precisely based on these assumptions.

3. Conceptual Model

The conceptual model starts from the computation of the maximum total bed shear stress due to the non-linear interaction of wave and current shear stresses, as suggested by Soulsby [65]:

$${\tau }_{max}=\sqrt{{\left({\tau }_m{+\tau }_w\cos \phi \right)}^2+{\left({\tau }_w\sin \phi \right)}^2},$$

(3)

where the mean bottom shear stress τ_m and the current contribution τ_c are respectively equal to:

$${\tau }_m{=\tau }_c\left[1+1.2{\left(\frac{{\tau }_w}{{\tau }_c+{\tau }_w}\right)}^{3.2}\right],$$

(4)

$${\tau }_c= \frac{\rho {gn}^2{U}_c{}^2}{d^{7/3}},$$

(5)

U_c being the absolute value of the current velocity, φ the angle between the wave motion direction and the current, g the gravity acceleration, n the Manning coefficient, and d the water depth.

The wave bed shear stress component τ_w, is computed through the Equations (1) and (2), where the wave friction factor derives from an equivalent Nikuradse roughness of 0.005 m and the wave bottom velocity is the result of the numerical generation process achieved performing SWAN in stationary mode on a regular grid. In

Table 1. Summary of the source terms used in SWAN simulation for the wind wave generation process and their relative formulation references and set parameters.

Source Term	Formula	Parameters
Wind input: linear growth	Cavaleri and Malanotte-Rizzoli [68]	-
Wind input: exponential growth	Janssen [69]	-
White capping	Janssen [69]	cds1 = 4.5 δ = 0.5	rate of dissipation dependency on wave number
Bottom friction dissipation	Madsen [62]	kn = 0.05 m	equivalent roughness height
Surf breaking	Battjes and Janssen [70]	γ = 0.78	breaker index

, a summary has been reported of the main applied source terms and their relative formulation references and set parameters. The wind speed range, chosen for the wave generation, is from 6 m/s to 14 m/s as deduced from data collected inside the Marano and Grado lagoon [51]. This range is coherent with values observed inside the Venice lagoon [47,48,54], the Virginia Coast Reserve in Virginia [55] and the Willapa Bay in Washington State [46], taken as examples.

Once the wave bottom shear stress has been determined on all the considered tidal flats depths, the maximum bed shear stress is computed adding the current contribution by means of Equation (5) for different current velocity values. In the present study the Manning coefficient n, representative of the bed resistance to the flow field inside tidal channels and over tidal flats, is taken equal to 0.0285 s/m^1/3. This value has been calibrated in a previous hydrodynamic modeling study of the Marano and Grado lagoon [51]. The current velocity is assumed variable in an appropriate range, taking into account that generally it rarely exceeds 0.25 m/s on the tidal flats in shallow and confined basins [3,9,46,48].

Figure 3 shows the effects of the combination of waves and current under three different scenarios between the flow direction and the wave rays, and suggests current velocities which lead to maximum shear stress greater than the chosen critical threshold. The wave component in this case is relative to a wind speed of 10 m/s.

The analyzed configurations refer to the limit conditions of respectively collinearity and orthogonality between waves and current, with the addition of the middle angle of 45°. The presence of the flow field enhances the bottom shear stress, shifting the wave component upwards. When a collinearity is considered, even low velocities of the order of 0.10–0.20 m/s can give a shear stress component with magnitude comparable to the wave motion. The existence of an angle between the two components attenuates the resultant forcing until considerably reducing it in a condition close to the orthogonality, for which the current contribution is much lower.

From another point of view, it is possible to compute the depth at which the maximum bottom shear stress achieves the assigned critical threshold, at varying currents. In this way, the conceptual model provides a depth value which can be compared to the actual depth of a tidal flat in order to determine if it tends to deepen or to remain stable. The procedure followed to establish this limit depth value is illustrated in the flow chart in Figure 4.

The asterisked variables inside the rectangles represent the values which the conceptual model requires as input. The maximum total bed shear stress can be computed at varying depth once the current velocity U_c*, the wind speed U_wind* and the angle φ* between the resultant wind waves and tidal current, have been established. The depth d* corresponds to the value for which this maximum shear stress is equal to the critical erosion threshold τ_crit*. In this manner, a limit depth separates two conditions: an erosion condition for depths lower than d* and a no-erosion condition for depths greater than d*. The conceptual model provides the theoretical curve of this limit depth d_lim(U_c, U_wind*, φ*, τ_crit*) at varying current velocities and for different assigned values of U_wind*, φ*, τ_crit*.

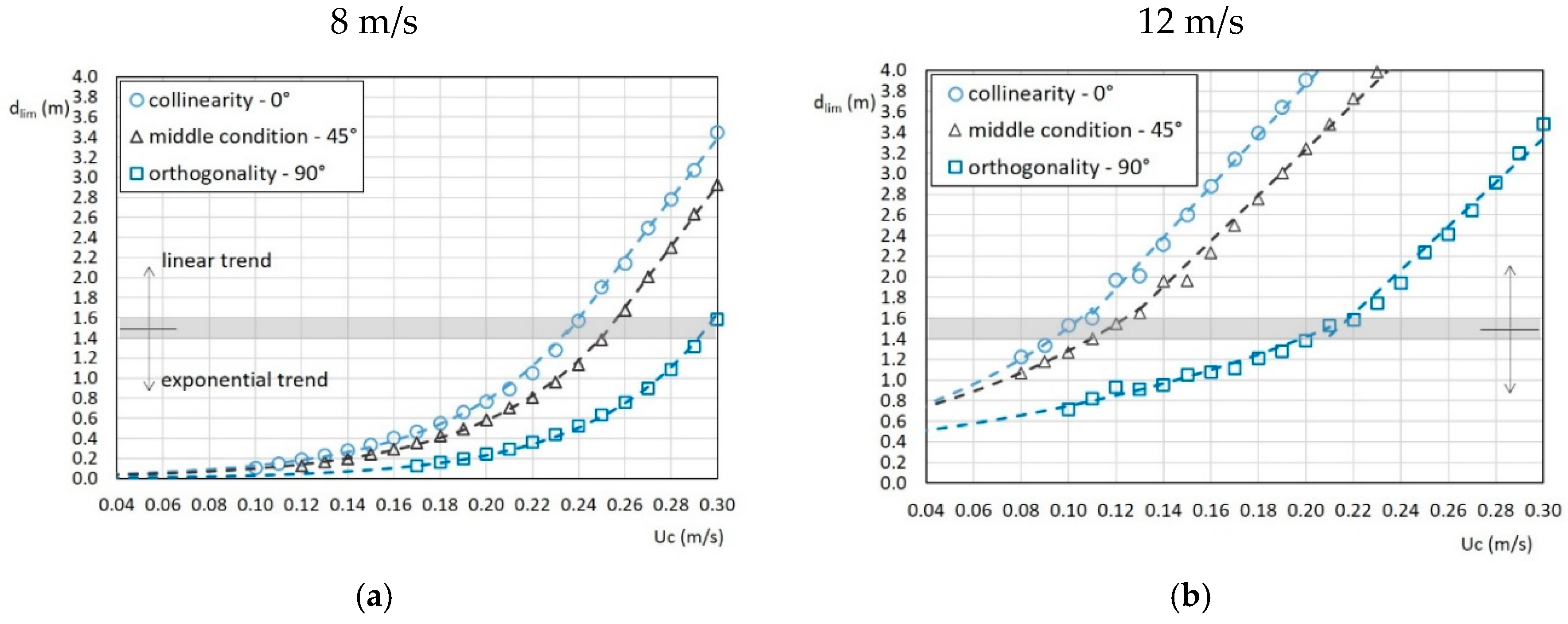
For a better comprehension of this procedure, first the example with a wind speed of 10 m/s and a critical shear stress of 0.7 Pa is followed. Figure 5a shows the discrete values of the limit depth in the three cases of vector composition between waves and current, i.e., for angles of respectively 0°, 45°, and 90°.

Observing the dashed lines, which represent the regression curves of the obtained points, two different trends can be clearly recognized: the former exponential and the latter linear. The transition between these two conditions is placed around a depth of about 1.5 m.

The applicability of the conceptual model becomes clearer from Figure 5b where the collinearity curve is taken as an example. The curve subdivides the plane into two zones: the lower represents conditions for which depths tend to deepen; the upper one indicates depths which can be considered dynamically stable or under a depositional trend if sediment supply is available. This latter condition, for which the bed tends to fill up, can go on until it reaches the limit depth on the curve, given the current speed value U_c*. Therefore, the continuous arrows direction shows the tendency of the flat bed to deepen, while the dotted arrows suggest the depth can be reduced by a potential deposition.

This procedure has been performed by computing the total bed shear stress for different wind speeds and comparing it with some values of the erosion critical shear stress, among those proposed in literature [63,64,65,66]. In this manner, many curves representative of the limit depths between erosion conditions and not, have been obtained. In all these cases, the three angles of 0°, 45° and 90° between waves and current directions have been analyzed.

Figure 6 shows the results for a critical shear stress equal to 0.7 Pa and a wind speed respectively of 8 m/s and 12 m/s.

Both the wind speeds of 6 m/s and 14 m/s cannot be considered representative for a morphological evolution over a medium-long period, the former being too low while the latter is quite high but rare.

As shown by the grey band, the exponential trends for depths lower than about 1.5 m and the linear ones for greater values are confirmed, suggesting substantial independence from the wind conditions, at least among those examined.

The regression curves can be expressed in a dimensional form by the following equations:

$$d_{lim\_exp}{= A}_1\exp \left(B_1{U}_c\right),$$

(6)

$$d_{lim\_lin}{=A}_2{U}_c+B_2,$$

(7)

d_{lim_exp} and d_{lim_lin} being respectively the limit depth given by the exponential and the linear trends, for which the relative coefficients A_i and B_i with i = 1, 2 can be computed by means of the least squares method.

These dimensional equations provide a quantitative estimate of the limit depth for a critical shear stress of 0.7 Pa and for the analysed wind speeds. In

Table 2. Summary of the parameters entering in the Equations (6) and (7) for the specified wind speed and angles between the flow direction and the wave motion.

Wind Speed	Parameters		Collinearity 0°	Middle Condition 45°	Orthogonality 90°
8 m/s	exponential	A1 (m)	0.0203	0.0163	0.0047
		B1 (s/m)	18.213	17.841	19.522
	linear	A2 (m)	29.966	30.441	25.115
		B2 (s/m)	−5.5993	−6.2116	−5.9576
10 m/s	exponential	A1 (m)	0.1245	0.1137	0.0348
		B1 (s/m)	13.674	12.551	13.776
	linear	A2 (m)	28.526	28.699	27.688
		B2 (s/m)	−3.7127	−4.374	−6.0564
12 m/s	exponential	A1 (m)	0.4853	0.5103	0.3933
		B1 (s/m)	11.338	9.2039	6.4084
	linear	A2 (m)	24.685	22.104	21.241
		B2 (s/m)	−1.0692	−1.1855	−3.0313

the values of the coefficients for the different cases are summarized.

Both the exponential and the linear trends are also found if the critical erosion shear stress is left as varying while the wind speed remains set equal to 10 m/s. This substantial independence also from the erosion threshold, as well as from the wind speed, corroborates the general validity of the approach.

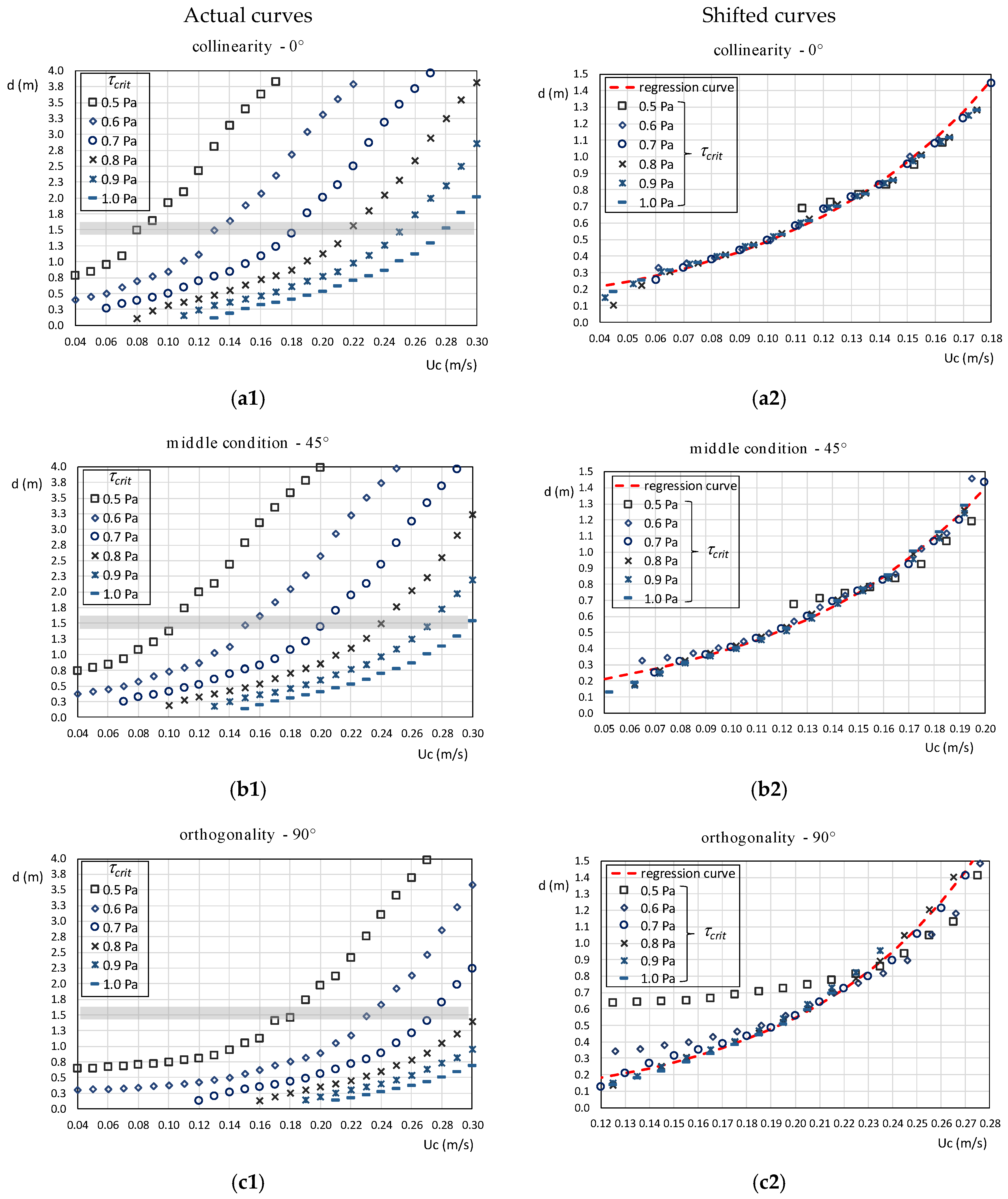
The results are presented in two forms as discussed below. The curves indicated as “actual” in Figure 7(a1,b1,c1) refer to water depths derived by imposing the maximum bed shear stress equal to different critical thresholds, here taken from 0.5 Pa up to 1.0 Pa.

Observing the arrangement of these curves, it is noted that they can be horizontally shifted towards each other until an almost perfect overlapping is obtained as shown in Figure 7(a2,b2,c2), with the exception of the orthogonal configuration for which there are some discrepancies. These curves are indicated as “shifted”.

The amount by which the curves are shifted can be related to the corresponding value of the critical shear stress. These quantities have therefore been fit through polynomial curves in order to provide a general equation that can give a quantitative estimate of the limit depth of erosion for a tidal flat inside shallow lagoons, if the current velocity and the erosion threshold are known.

By limiting the analysis only to the exponential component, the limit depth can be so expressed as:

$$d_{lim\_exp}{=A}_1\exp \left[B_1\left(U_c+{C\tau }_{crit}^2+{D\tau }_{crit}+E\right)\right]$$

(8)

C, D and E being the coefficients deriving from the polynomial regression, the values of which are reported in

Table 3. Summary of the parameters within the Equations (8) for the specified wind speed and angle between the flow direction and the wave motion.

Wind Speed	Parameters	Collinearity 0°	Middle Condition 45°	Orthogonality 90°
10 m/s	A1 (m)	0.1245	0.1137	0.0348
	B1 (s/m)	13.674	12.551	13.776
	C (s·m−1·Pa−2)	0.2759	0.3750	0.4625
	D (s·m−1·Pa)	−0.7851	−0.9602	−1.0703
	E (m/s)	0.4149	0.4893	0.5234

depending on the angle between the wave rays and the flow direction.

To test the applicability of the present conceptual model to a real context, the Marano and Grado lagoon is taken as a case study, since the topographical differences of various portions of its tidal flats, evaluated over a period of 45 years, are available. The 1964 hydrographic map edited by the Magistrato alle Acque of Venice [71] has been compared to the more recent bathymetric data surveyed in 2009 [72], making it possible to carry out morphological evaluations over the long term.

4. Study Site

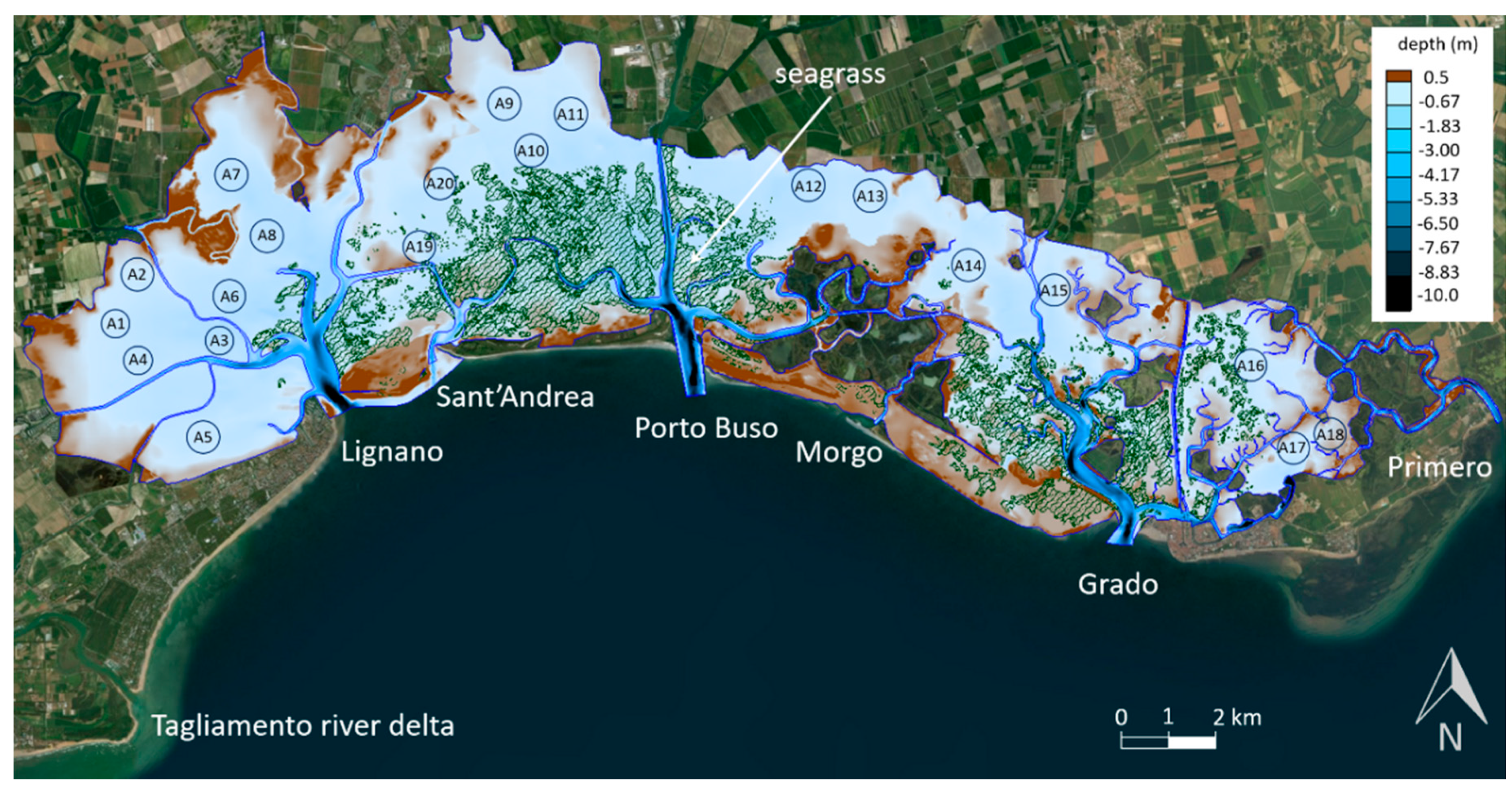
The Marano and Grado lagoon is depicted in Figure 8. Together with the Venice lagoon, it is one of the two most important coastal systems in the North East of Italy and is located between the low Friuli Venezia Giulia plain and the Northern Adriatic Sea.

Its extension roughly covers an area of 160 km² and it is bordered by the delta systems of the two main rivers that cross Friuli Venezia Giulia: the Tagliamento on the west and the Isonzo on the east. On the sea side, the lagoon area is bounded by a series of barrier islands alternating with six tidal inlets. The shoreline develops from the western tidal inlet of Lignano to the eastern inlet of Primero for an overall length of about 32 km.

Petti et al. [51] have recently investigated the sediment transport processes of the Marano and Grado lagoon over an annual period, by means of a morphodynamic spectral coupled model. This study required the analyses of a large amount of wind and tide data to define a representative sequence of waves and water levels. The mean tidal range, given by the difference between the mean high water level (MHWL) and the mean low water level (MLWL) is about 0.76 m as resulting from the zero-crossing technique, carried out over 24 years of recording of the Grado tide gauge [51]. The mean range increases up to about 1.05 m during the spring tides and it is reduced to 0.22 m in the neap tides [20,73]. The annual average tidal signal, depicted in Figure 9, consists of three main astronomical components, the two principal semidiurnal, solar and lunar, and the lunar diurnal. It has a resultant periodicity of 12.5 days and is consistent with the tidal ranges specified above [51].

In order to derive also an annual average anemometric characterization, the extensive field of wind data collected inside the lagoon area has been subdivided into appropriate classes. These classes, of both wind speed and direction, have been combined with the tide sequence as shown in Figure 9. The so determined hydrodynamic conditions have been used to perform the morphodynamic numerical simulations of the lagoon bottom evolution over a period of an average year.

The comparison between the final and initial bed levels confirms that the interaction between tidal currents and waves governs the sediment transport mechanism inside the entire lagoon area. In particular, the most of the erosion areas are concentrated precisely on the tidal flats and near the saltmarshes, due to the shallower depths. The wind speed of 10 m/s, corresponding to the 90th percentile value as deduced from the duration curve, contributes significantly to cohesive sediment resuspension from tidal flats. The following transport inside internal channels provides 75% of their annual filling, as deduced from the simulated deposited volumes [51]. These results are in agreement with the flattening trend of the bottom topography which has been observed in the last decades with a net loss of fine sediments and saltmarshes surface [20].

The tidal flat composition is prevalently characterized by fine and cohesive sediments, even if sand content progressively increases towards the tidal inlets. An extended vegetal cover by seagrass is present in the central lagoon [74], and in the eastern portion along the main channel departing from the Grado inlet, as depicted in Figure 8. Seagrass has an important stabilizing effect on sediment resuspension from the bottom, as roots increase the critical erosion shear stress that can reach quite considerable values [63], while vegetation biomass favors mineral sediment trapping and promotes belowground organic production [36]. Moreover, the presence of vegetation can greatly affect the hydrodynamic field, decreasing both wave heights and current velocities, and consequently the corresponding bed shear stress.

On the contrary, some areas in the two extreme lateral portion of the lagoon, respectively on the west and east sides, are characterized by a greater mobility of the cohesive sediments due to several factors, among them shellfish farming. Trawl nets and dredges produce disturbances which lead to sediment resuspension comparable to that induced by the wave motion during storm conditions [75,76]. The different resistance degree of the bed to erosion has been taken into account in the morphodynamic modelling, setting a spatially variable critical threshold by the comparison with the morphologies of the Venice lagoon, for which measured values are available.

On the basis of these considerations, twenty tidal flats picked out in Figure 8 by the circles and an identification number have been chosen as test cases for the developed conceptual model. Their arrangement is as distributed as possible throughout the overall lagoon area, except for the portion covered by seagrass as it deeply affects the morphodynamic balance, which therefore cannot be considered representative for a medium-long period evolution.

5. Results

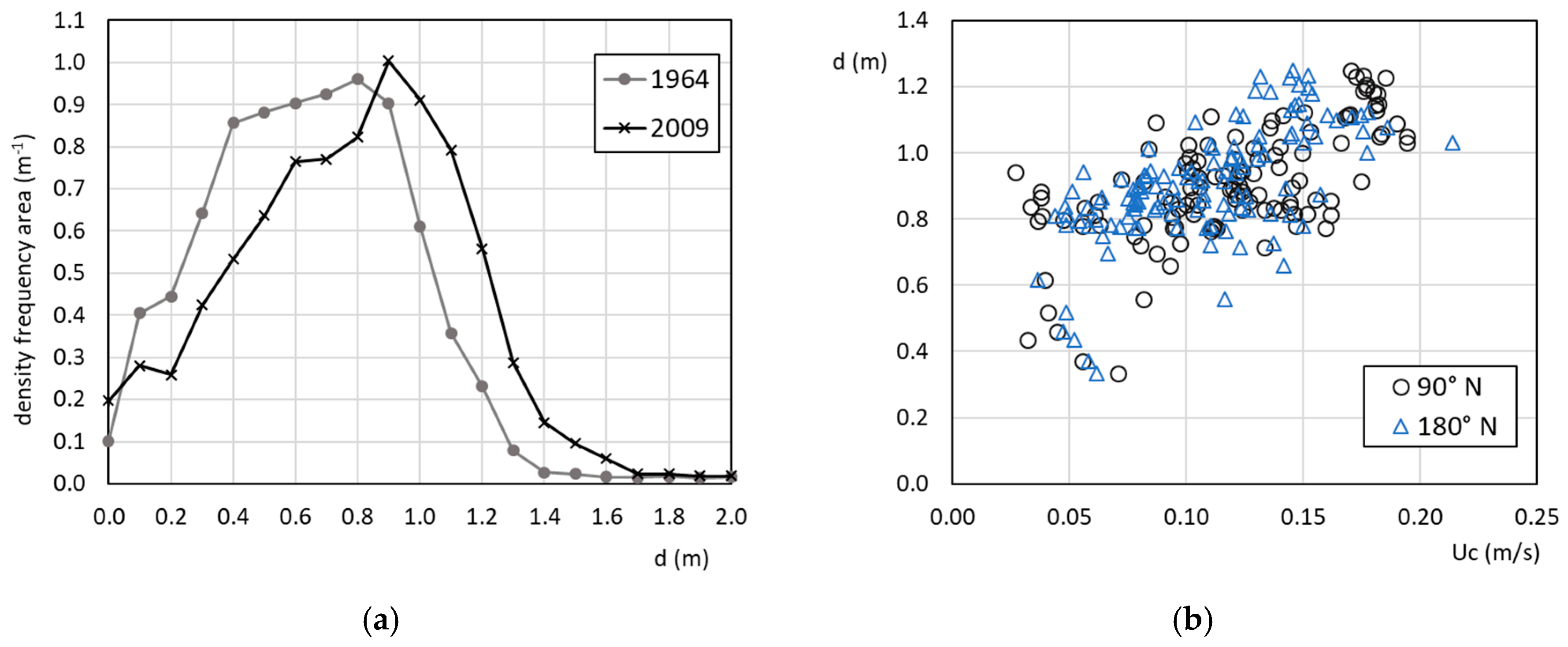
The morphological evolution of the Marano and Grado lagoon in 45 years can be shown by the comparison between two different hypsometric distributions. These curves have been obtained respectively from the digitalized and reconstructed bathymetry from 1964 and the one deriving from the more recent survey of 2009. The area subtended by the curve up to a given depth represents the percentage of the lagoon surface that was at a greater or equal depth. The shifting between the two curves reported in Figure 10a, underlines the deepening trend of the lagoon with a loss of area at depths shallower than 0.8 m compared to a consistent increase of greater depths from 0.9 m up to about 1.7 m.

The peak of the tidal flat distributions has remained fairly constant, moving in the small range of 0.8–0.9 m, which in fact characterizes the average depth of the greatest number of points taken as samples in the selected areas. Nowadays, more than 80% of the lagoon area is represented by tidal flats up to 2 m deep, while only the tidal channels are deeper, and occupy 5% of the global area. In detail, only 25% of the tidal flats are shallower than 0.5 m and the remaining part is subtidal, confined up to 1.5 m, rarely reaching 2.0 m. In the western and the central part of the lagoon, tidal flats extend as intertidal talus of the fringing salt marshes and gently deepen up to becoming subtidal, towards the secondary channels; flat beds around the bifurcation between main and secondary channels, as in Lignano and Porto Buso, are always subtidal. In the eastern part of the lagoon, influenced by the Grado inlet, tidal flats are variably distributed according to the complex morphological systems, consisting of saltmarshes, islands, fish farms and channels.

In order to apply the new conceptual model, the hydrodynamic conditions are needed for each of the analyzed points, taken as samples in the selected areas. In particular, the model requires the representative current velocity value, its vector composition with the wind direction and the critical erosion shear stress. These features have been deduced from the morphodynamic application of Petti et al. [51]. As the current magnitude varies during a tidal cycle, the maximum value simulated during wind events of 10 m/s blowing from respectively 90° N and 180° N has been chosen, according to other applications in similar contexts [5,40,55]. These values are plotted in Figure 10b together with the corresponding depth, and they are all less than 0.25 m/s. This result confirms the choices made in the development of the model. The list of the preliminary parameters necessary for the use of the conceptual model within each area is shown in

Table 4. The list of the preliminary parameters for the conceptual model within each area, referring to the wind condition which leads to the maximum value of the bed shear stress.

Lagoon Portion	Area	τcrit (Pa)	Uc (m/s)	Wind Direction	Wave-Current Angle
West	A1	0.7	0.097	90° N	0°
	A2	0.7	0.092	90° N	0°
	A3	0.7	0.177	90° N	0°
	A4	0.5	0.122	90° N	0°
	A5	0.5	0.145	90° N	0°
	A6	0.7	0.178	90° N	0°
	A7	0.7	0.132	180° N	45°
	A8	0.7	0.177	180° N	0°
Central	A9	0.7	0.117	180° N	0°
	A10	0.7	0.132	90° N	45°
	A11	0.7	0.125	180° N	0°
	A12	0.7	0.187	90° N	0°
	A13	0.7	0.149	90° N	0°
	A14	0.7	0.131	90° N	45°
	A15	0.7	0.130	180° N	0°
	A19	0.7	0.144	180° N	0°
	A20	0.7	0.112	180° N	0°
East	A16	0.5	0.053	180° N	0°
	A17	0.5	0.056	180° N	45°
	A18	0.5	0.051	180° N	45°

.

They refer to the wind and current conditions which lead to the maximum value of the bed shear stress and they are obtained as average values on the set of selected points.

The wave-current angle has been established according to the wind direction and that of the tidal flow at the maximum velocity instant and it has been associated with one of the three examined configurations of 0°, 45° or 90°. Given that the orthogonality between waves and current leads to much lower values of bed shear stress compared to the other two configurations, it does not figure among the chosen settings.

Most areas are characterized by a critical shear stress of 0.7 Pa with the exception of the tidal flats belonging to A4 and A5 located in the western portion and to A16–A18 in the eastern part of the lagoon, which have a shear stress of 0.5 Pa, as justified above.

Both the wind directions contribute to the morphological evolution of the lagoon, and not only the direction that maximizes the fetch length or that is collinear with the current field, as is usually assumed in similar studies [48,49]. These particular features depend on the generation process of wind waves in shallow and confined waters and on the bi-dimensional nature of the flow field. In light of this, it is important to consider an omnidirectional distribution of winds [51] and also to check the vector composition in relation to the current, as the present conceptual model allows us to do.

The summarized data can be combined through the Equation (8), taking into account the different parameters specified in Table 3 according to the wave-current configuration. The conceptual model provides the limit depth between the erosion state and the no-erosion state, as highlighted in Figure 5b. In this way, it is possible to compare the conceptual erosion state with the measured one, making it possible to validate the model. The results are reported in

Table 5. Validation of the conceptual model. Symbol x is used when the difference between the depths are negative, suggesting an erosion state. The compared depths are those measured in 1964 (d₁₉₆₄) and 2009 (d₂₀₀₉) and the limit depth (d_lim) provided by the conceptual model.

Area	Depth (m)			Erosion State
	d1964	d2009	dlim	d1964 − d2009	d1964 − dlim	d2009 − dlim
A1	0.83	0.84	0.47
A2	0.83	0.77	0.44
A3	0.79	1.20	1.42	x	x	x
A4	0.75	0.90	>1.5	x	x	x
A5	0.83	0.83	>1.5		x	x
A6	1.05	1.17	1.42	x	x	x
A7	1.05	1.07	0.61
A8	0.91	1.08	1.41	x	x	x
A9	0.82	0.83	0.62
A10	0.96	0.98	0.60
A11	0.94	0.83	0.69
A12	0.80	1.08	>1.5	x	x	x
A13	0.77	0.89	0.96	x	x	x
A14	0.63	0.85	0.60	x
A15	0.54	0.79	0.74	x	x
A19	0.45	0.92	0.90	x	x
A20	0.67	0.96	0.58	x
A16	0.69	0.86	0.90	x	x	x
A17	0.23	0.81	0.84	x	x	x
A18	0.26	0.46	0.78	x	x	x

.

The measured erosion state can be defined by the comparison between the 1964 and the 2009 depths, respectively indicated as d₁₉₆₄ and d₂₀₀₉. If the difference d₁₉₆₄ − d₂₀₀₉ is negative, an erosion trend will have been verified over the 45 years. The limit depths provided by the conceptual model have been compared to both the measured depth in 1964 and that surveyed in 2009. Similarly, a negative difference d₁₉₆₄ − d_lim means that a deepening trend should have happened over the 45 years, while the difference d₂₀₀₉ − d_lim suggests if the depth measured in 2009 is still under an erosion trend or not. In all these cases, the symbol “x” is used to identify an erosion condition.

The agreement between the measured erosion states and those predicted by the conceptual model is substantial over almost all the selected areas, referring to both the erosion trend or not. This correspondence corroborates and validates the described procedure and the determined analytical solution making it therefore suitable to predict the medium-long term morphological evolution of tidal flats in sheltered coastal environments.

It is noticeable that the stable areas are all located along the northern internal edge of the lagoon, and they are characterized by an average depth close to the interval of 0.8–0.9 m, which effectively corresponds to the peaks of density frequency area distributions in Figure 10a. At the same time the current velocities do not exceed 0.13 m/s, which is a rather low value, thus ensuring both dynamic stability and the possibility for sediments to be deposited if available. Zones A12 and A13 are an exception because, despite having the same bottom resistance, the erosion processes are triggered by higher current velocities. The southern portion of the lagoon is characterized by the presence of fishing valleys which are bounded by embankments and for this reason hydraulically impermeable. This condition produces an effect similar to that of a cross-section narrowing on the flow between the eastern and the western part of the lagoon, with a consequent increasing of current velocities near the zones A12 and A13.

In the western part of the lagoon area connected to the Lignano inlet, an important deepening of the seabed toward depths approaching 1.5 m can be recognized. In particular, tidal flats A3, A6 and A8 are close to main channels characterized by higher values of depth and current velocities, while A4 and A5 are concession areas for shellfish farming since 2006. This intended use can make the bottom more exposed to erosive mechanisms, due to the destabilization of sediment structures by fishing gear, which cause an increased likelihood of renewed suspension during natural storms [75,76]. The mismatch between the measured and theoretical state for the tidal flat A5 can be ascribed to its less intensive use, which therefore does not significantly alter the critical shear stress. In fact, if a 0.7 Pa is taken as threshold, the resulting limit depth would be equal to 0.91 m which is much closer to the real depth.

Also the tidal flats in the eastern lagoon area tend to reach a depth of around 0.8–0.9 m, but on the contrary in this case there is a significant erosive tendency with respect to the bathymetric data of 1964. This condition happens even though the Primero basin is sheltered, with very small extensions on all the directions and shallower depths than the rest of the lagoon, leading to limited wave field and a maximum current velocities range which does not exceed 0.06 m/s, as can be seen from Table 4. This case represents an example of how important it is to consider the strong interaction between local wind waves and current, whose respective low values are sufficient, only if taken together, to overcome the bed resistance.

Two of the examined areas, A14 and A20, located in the central area outside the surface covered by seagrass, have deepened over time, despite the conceptual model missing this trend, providing limit depths very similar to the original ones. The reason can be sought in a different bottom critical shear stress or hydrodynamic condition, since area A20 is close to a channel that over time has been filled and has disappeared.

6. Discussion

The considerations and results presented in the previous section corroborate the procedure followed to define the conceptual model. The proposed approach is able to adequately address the question of estimating a limit depth for the morphological evolution of tidal flats, and it can suggest if they undergo an erosion trend or not over a medium-long period.

The model can be applied to shallow and confined basins, such as the coastal lagoon taken as a case study; in these contexts, tidal flats are characterized by a very small slope and they can be considered sufficiently uniform in depth. Their depth variation is related to the distribution of the total bed shear stress resulting from the complex interaction between currents and local wind waves. A methodology widely used to investigate the hydrodynamic and morphodynamic processes, consists in applying a dedicated numerical modelling. In particular, this is usually done by coupling different modules, as in the study of Petti et al. [51]: a hydrodynamic model to reproduce the flow field, a wind wave model, and a morphodynamic model to compute the bed evolution. This approach is certainly more complete because it takes into account the variability of the phenomena both on the vertical section and on the horizontal plane. However, it requires great computational effort especially in very large domains, and it needs proper calibration and validation processes to be performed. Moreover, from a morphodynamic point of view, it is possible to provide an estimation of the evolution trend only for reduced time scales, at most an annual period or just over.

In order to predict tidal flats morphology for medium-long periods, such as the 45 years considered in the analyzed case study, more simplified approaches are needed. This is the focus of many analytical methods and theories, developed around the dynamic equilibrium of the cross-shore profile shapes, as reported in the introduction. The present model starts from similar assumptions but it provides a quantitative estimation in the morphological evolution of tidal flats, and not only a qualitative one as in the dynamic model by Friedrichs and Aubrey [38]. Moreover, it extends the study proposed by Fagherazzi et al. [47], considering the variability of the wind speed and the current velocity, and also adding their vector composition through the angle φ. In this sense, the conceptual model takes into account the effects on the bottom shear stress induced by the interaction between waves and current velocities on the horizontal plane. Recent outcomes for the generation process in shallow water show that it is deeply affected by the role played by bed roughness and the variability of the wave period [45,50]. The consequence is an important reduction in the main wave parameters, above all in the wave orbital velocity and in the resulting wave bottom shear stress which tends to assume low values, especially on the tidal flat shallow depths. In the present model, the evaluation of the total maximum bed shear stress takes into account all of these issues.

The wind speed, the current velocity, the angle between their directions and the critical erosion shear stress are required as input values by the model. These parameters can be quite easily deduced from direct measurements, literature suggestions or as results from more simplified numerical modeling. More details about the choice of these parameters are given below.

Wave motion is undoubtedly the dominant factor in tidal flats evolution, because the wave-induced bed shear stress is often the main factor that controls the suspended-sediment concentration [7]. However, current velocity cannot be neglected as it can drag and move away sediments, which would otherwise only be resuspended. To evaluate erosion mechanisms over the medium-long period, it is important to choose morphologically representative values of both wind speed and current velocity to be used in the conceptual model, similarly to Fagherazzi et al. [47].

In particular, the required values should account for both the characteristic magnitude of the hydrodynamic forcing as well as the duration in which this forcing is strong enough [29]. The astronomical tide component does not vary significantly in time, giving a current field that periodically repeats alternating flood and ebb phases. In this sense, the tidal velocity oscillates from a minimum to a maximum value over every cycle. On the other hand, the wave motion is strongly linked to wind speed and its frequency, i.e., lower but more frequent wind speeds generate a weak wave motion, as opposed to stronger but extreme events. A useful scale widely adopted for evaluating coastal morphologies [5,30] is the 90th percentile velocity, in analogy to the procedure followed in the application to the case study of the Marano and Grado lagoon.

Generally, the tidal flow direction is linked to level changes inside the basin and to the tidal channel geometry, while the wave direction can be taken substantially coincident to that of the wind, as only sheltered environments and locally generated waves are herein considered. These evidences suggest the proper angle between current velocities and waves to be set.

The last input parameter is the critical threshold of bed resistance, which is a key factor to interpreting the morphological evolution of tidal flats. In fact, erosion processes are triggered when the resultant bed shear stress exceeds the critical erosion shear stress [45,46]. However, this important parameter does not have a unique definition in literature, because it can take on very different values within a wide range both from a spatial and a temporal point of view [63,64]. In this sense, it is the most sensitive parameter which can also lead to quite important differences in the results provided by the conceptual model, as can be observed in Figure 7.

Equation (8), which is derived from the depicted curves, represents the main outcome of the conceptual model and its validity is general if within the limits recommended for its application, i.e., shallow depths and confined environments. The investigated ranges of both tidal flat depths and current velocities are in fact quite short and they are compatible with the typical values of many shallow lagoons. This is the case for example of the lagoons cited in the present paper [46,47,51,55], which have all been the subject of morphodynamic studies to understand the relative role of waves and current motion. In these contexts, the average depth of tidal flats is around 1 m where current velocities are rather low and within the range 0.1–0.3 m/s.

Furthermore, the equation is the result of the regression of the points below the grey band that establishes the upper limit of the exponential part, as outlined in Figure 5, Figure 6 and Figure 7. This band indicates a different relationship between the limit depth and the maximum bed shear stress and it can reflect the prevailing of waves or current in the erosion mechanism. In particular, for intermediate wind speeds both wind waves and tidal currents are relevant in the erosive process on shallow depths, even if the major role is however played by the wave motion, as the current velocities are quite low. Depths greater than about a few meters are reached only inside the lagoon channels where the flow is more concentrated and it is able to engrave the seabed. In these conditions, where the hydrodynamics are more energetic, the current is certainly the dominant forcing, but for this reason they are not suitable for the conceptual model.

A further consideration concerns the dynamic equilibrium, which is defined as the balance condition between erosion and deposition, so that no net sediment transport occurs. If the erosion mechanism is relatively simple to define by comparing it with the critical stress for sediment resuspension, this is not the same for the deposition process. In fact, this latter term is associated not only with a relative shear stress, but also with the sediments supply, which can have a strong variability both over time and in space [63,64]. For these reasons and given the complexity and the uncertainty in quantifying the deposition rate, the herein presented conceptual model does not distinguish a morphologically stable condition from a deposition one.

7. Conclusions

In this paper a new simple conceptual model has been proposed in order to investigate the dynamic equilibrium of a tidal flat over a medium-long period. The theoretical approach provides a quantitative estimation of a limit depth, which separates an erosion condition from a no-erosion condition for tidal flats in sheltered basins. These contexts are generally characterized by shallow and quite flat bottoms, continuously reshaped by the currents deriving from the periodic sea water level variations, and the local wind waves. In particular, the wave field is deeply affected by bottom friction dissipations, differently from the offshore generation process, leading to much lower bed shear stress.

In this sense, the contribution of the tidal current becomes fundamental in triggering the erosion processes and the subsequent transport of sediments, until the limit depth, for which this mechanism stops, is reached. If deposition and sediment supply are not considered, this limit depth corresponds to the condition that makes the assigned erosion threshold equal to the maximum shear stress resulting from the interaction between waves and current.

The limit depth is expressed by means of a dimensional equation which requires as input the values of the critical shear stress, the current velocity and the angle between the flow and the wind direction. This equation is related to an assigned wind speed and it gives an exponential trend of the limit depth at varying current velocity, up to depth values of about 1.5 m. For values greater than this, the dependence becomes linear and it involves current velocities higher than 0.2–0.3 m/s, that exceed the range considered in the development of the present conceptual model. In this sense, its application is suitable to provide a realistic estimation of the limit depth for the morphological evolution of a tidal flats belonging to shallow and limited-fetch environments.

It has been furthermore verified that the vector composition of the current flow in relation to the wave rays can deeply affect the erosion mechanism, configuring itself as an important additional variable that must be taken into account. To this purpose, three angle configurations between the waves and the current direction have been considered: collinearity, orthogonality and a middle condition. In particular, this last case which considers a generic angle of 45° does not actually lead to a condition so different from the collinear case, while the curve of the limit depth tends to significantly decrease if orthogonality is realized.

To validate the conceptual model, its application to a real context has been performed. The Marano and Grado lagoon has been taken as a case study, starting from hydrodynamic data deriving from a previous numerical modeling and two bathymetries surveyed 45 years apart. The comparison between the measured erosion states and the ones theoretically predicted, gives encouraging results in support of the usefulness of this new conceptual model. As a next step, the authors plan to determine the analytical expression of the limit erosion depth for a tidal flat, in a dimensionless form, to generalize its applicability as far as possible.