Investigation into Complex Boundary Solutions of Water Filling Process in Pipeline Systems
Abstract
:1. Introduction
2. Numerical Modeling and Meshing
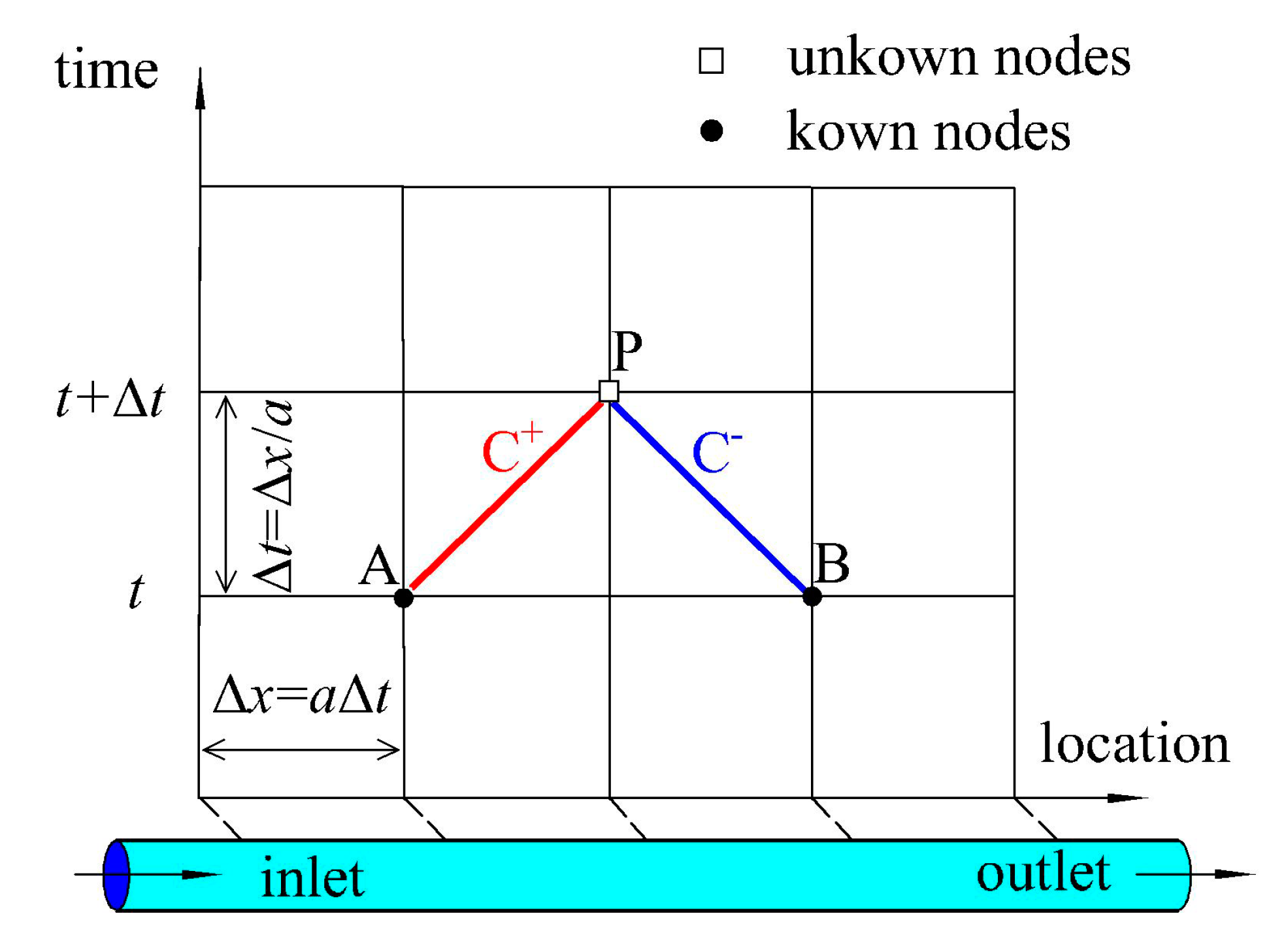
2.1. Method of Characteristics
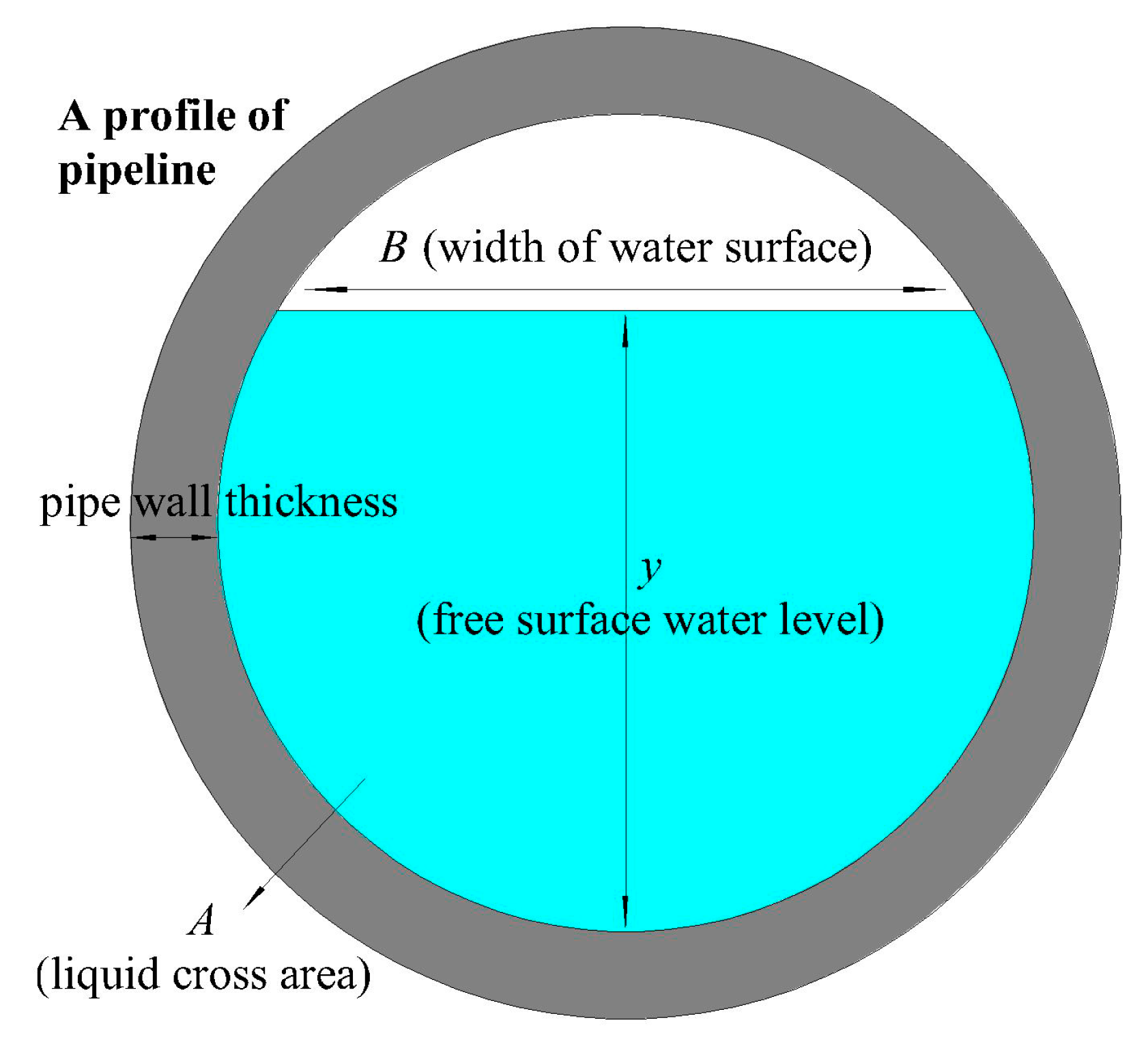
2.1.1. Stage 1: Free Surface Pipe Flow
2.1.2. Stage 2: Pressured Pipe Flow
2.1.3. Combination Method
2.2. Boundary Conditions
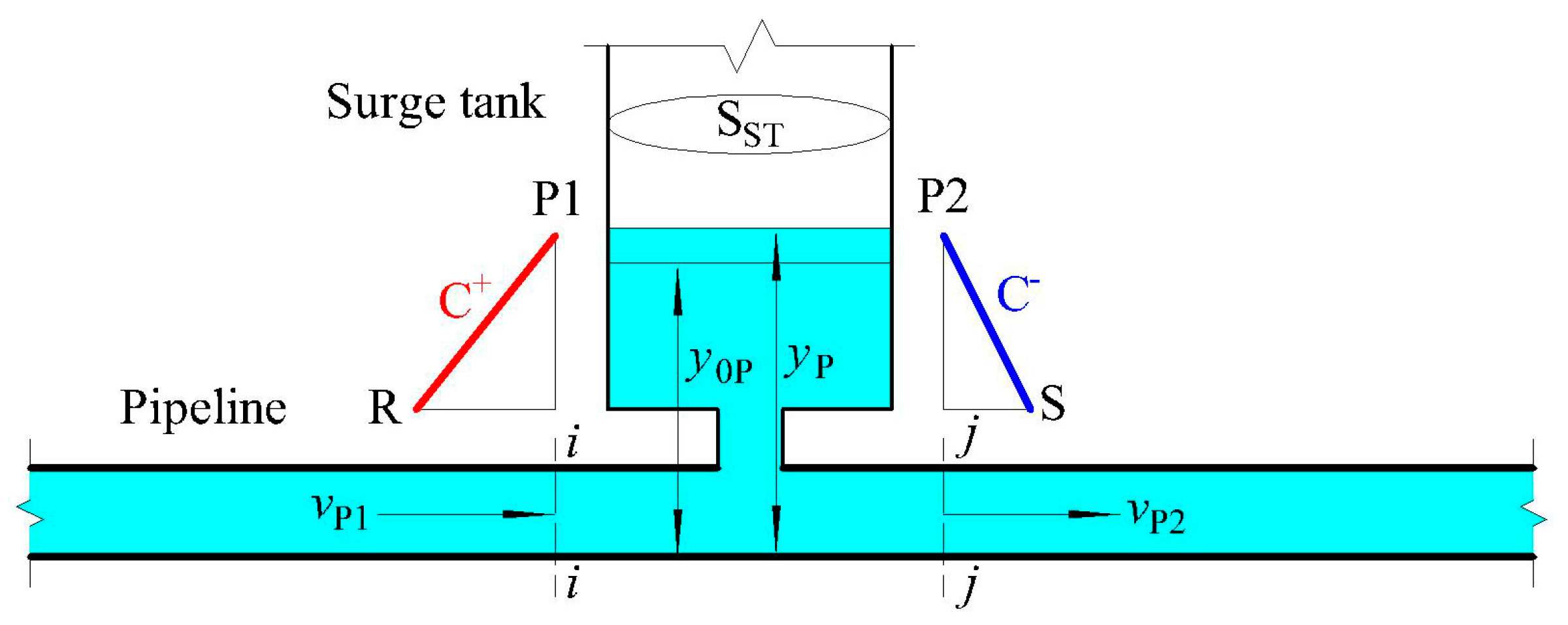
2.2.1. Surge Tank Boundary Model
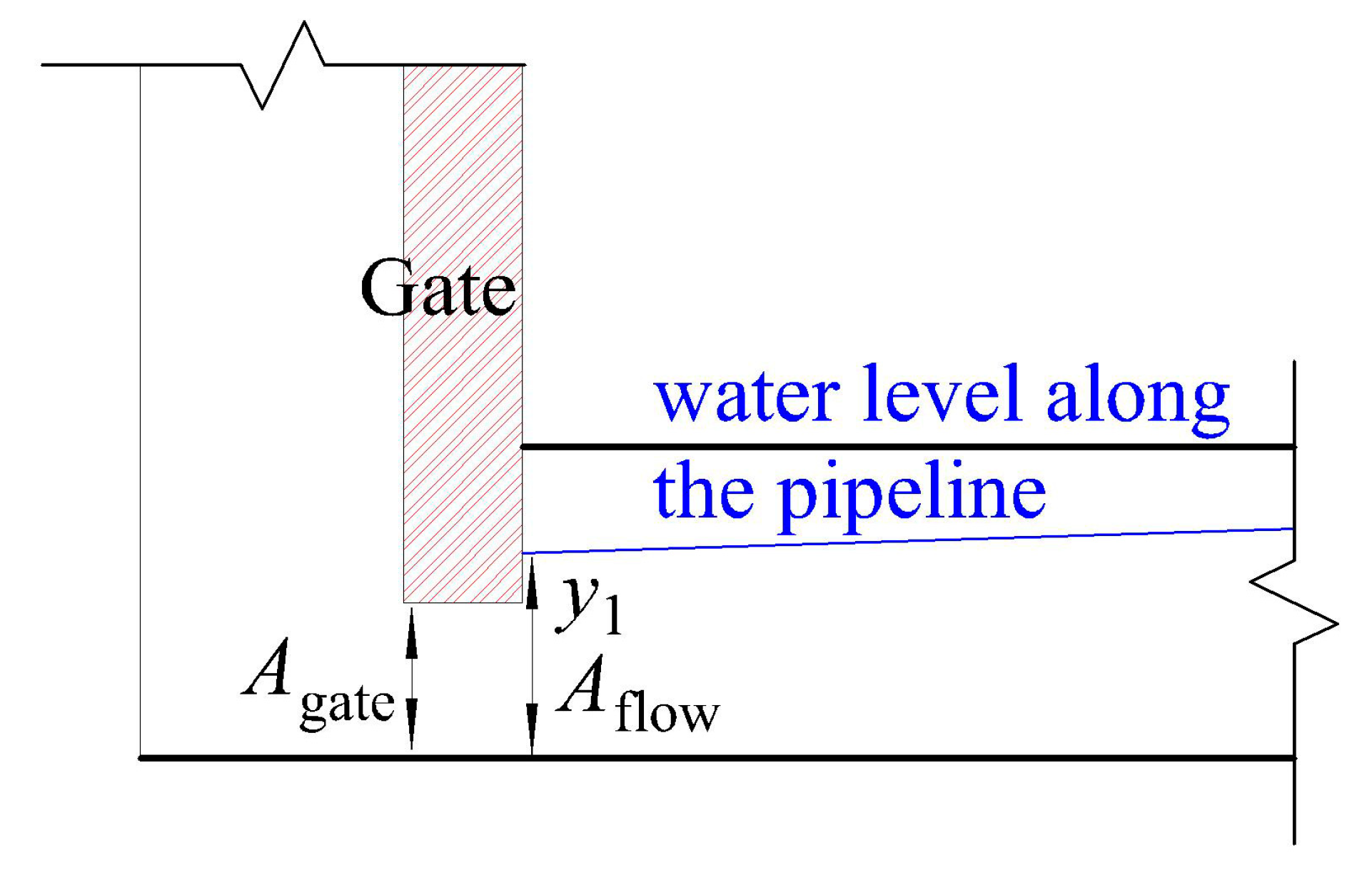
2.2.2. Upstream and Downstream Boundary Models
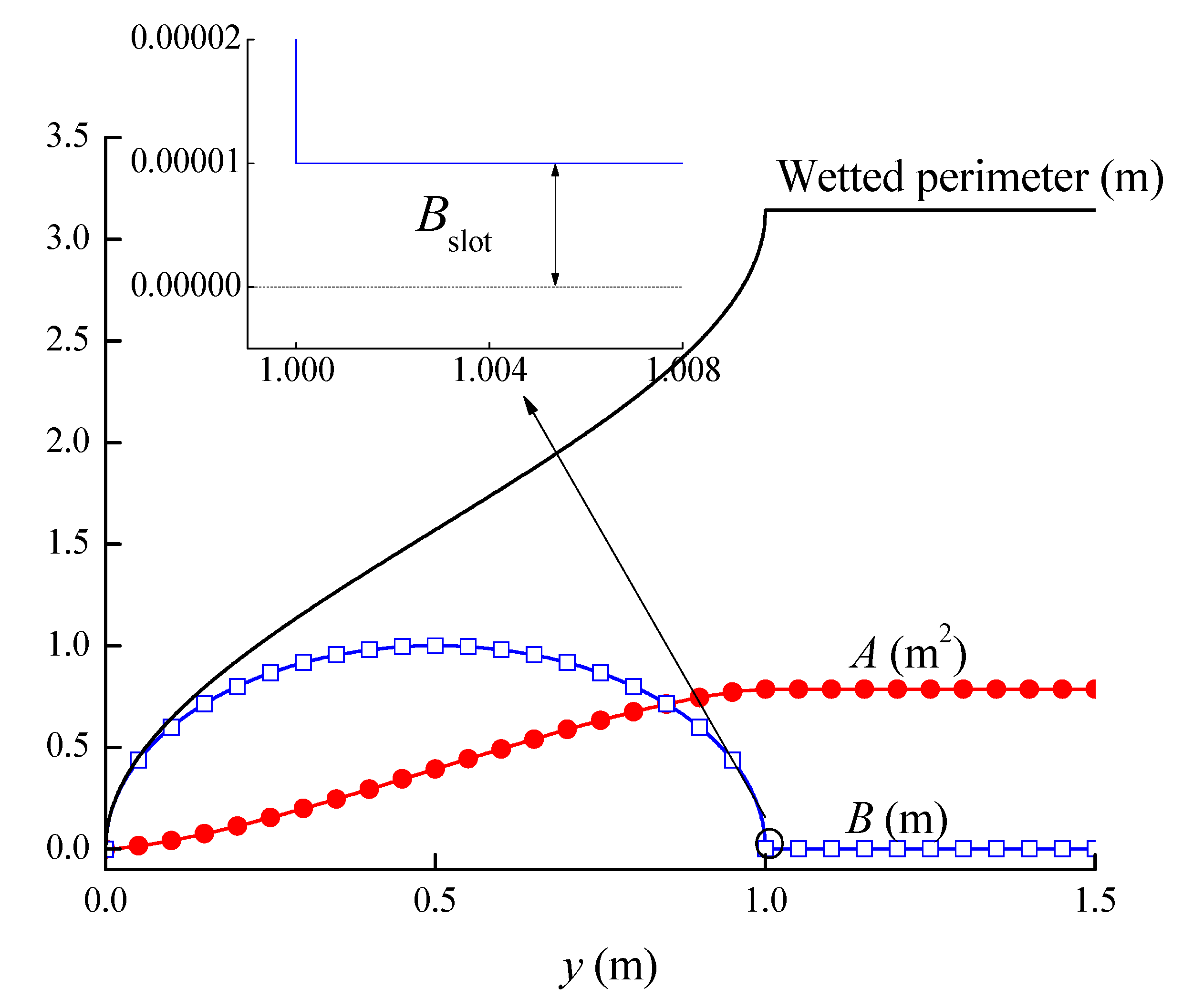
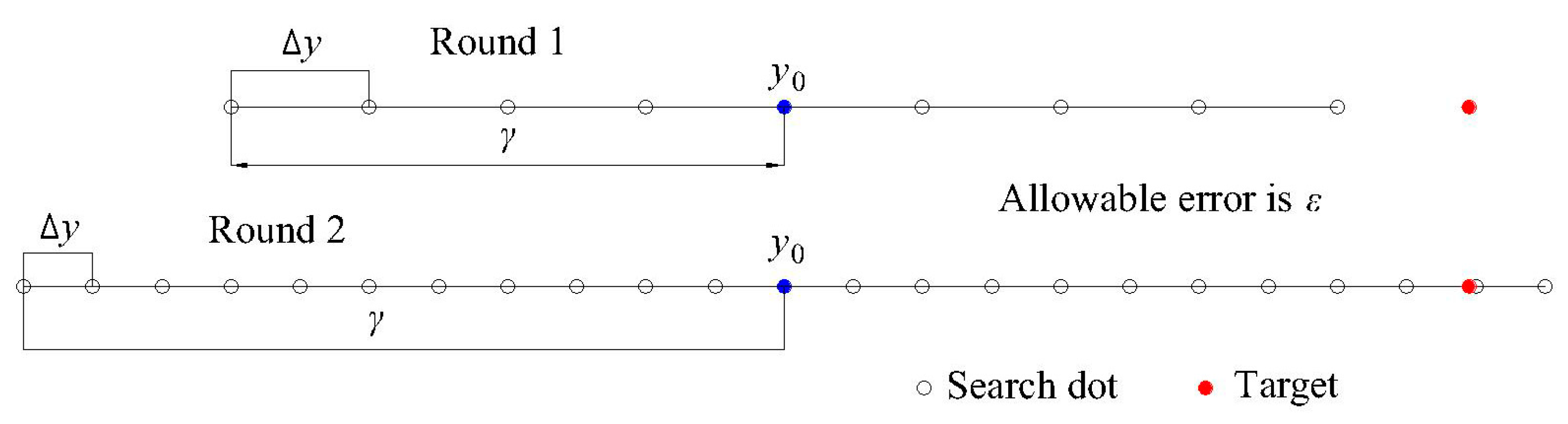
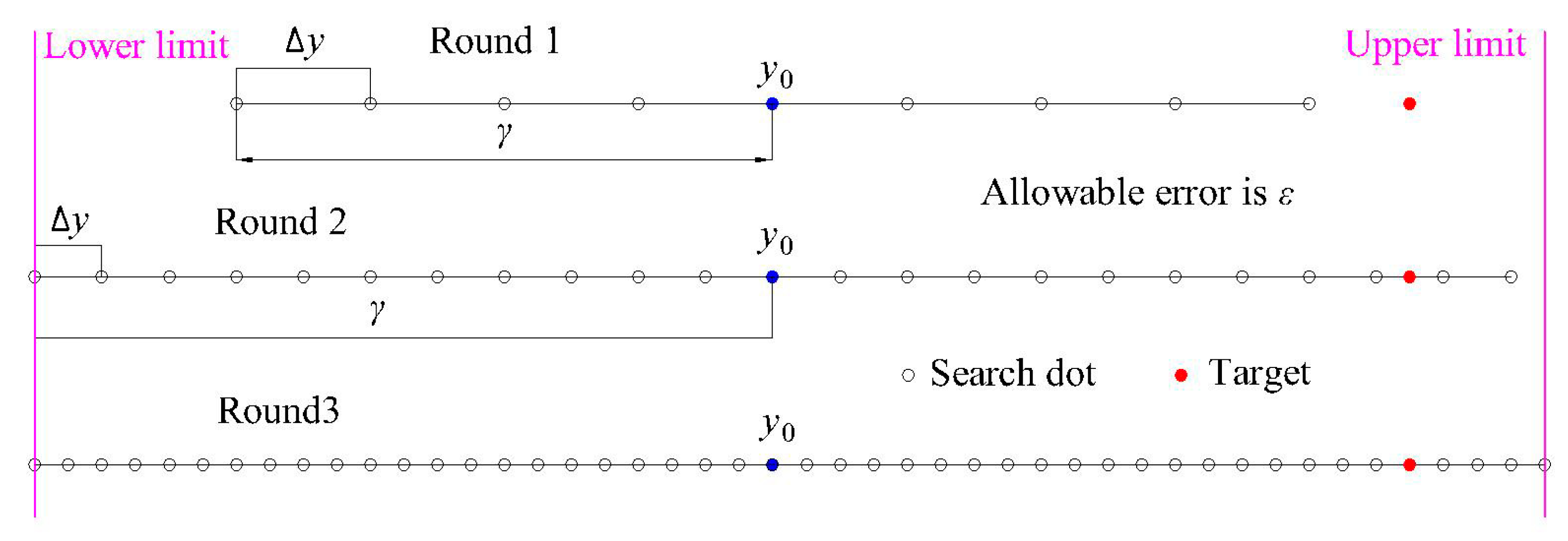
2.3. Proposed Double Forward Method
3. Application in a Typical Filling Process
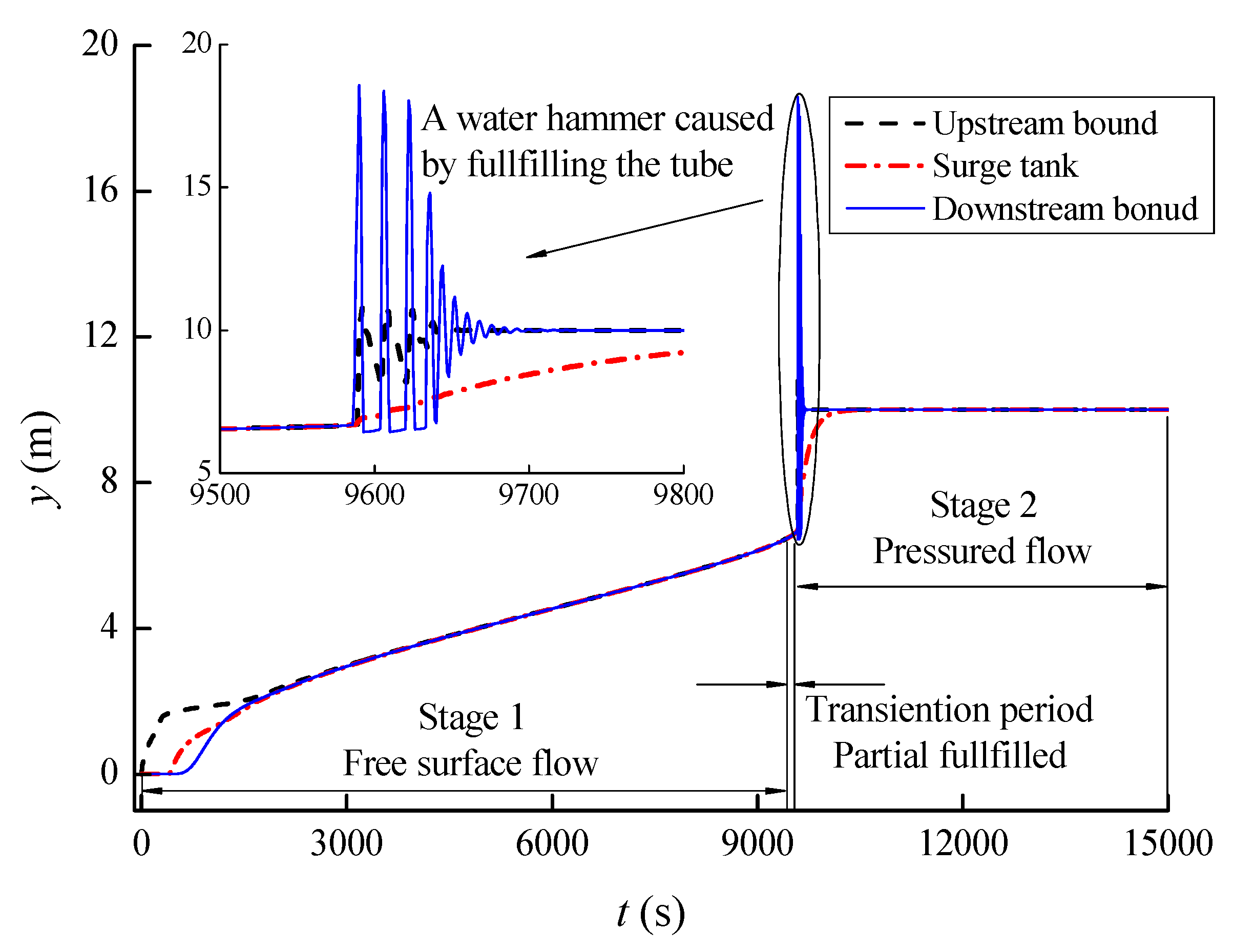
3.1. Free Surface Flow Stage
3.2. Pressured Flow Stage
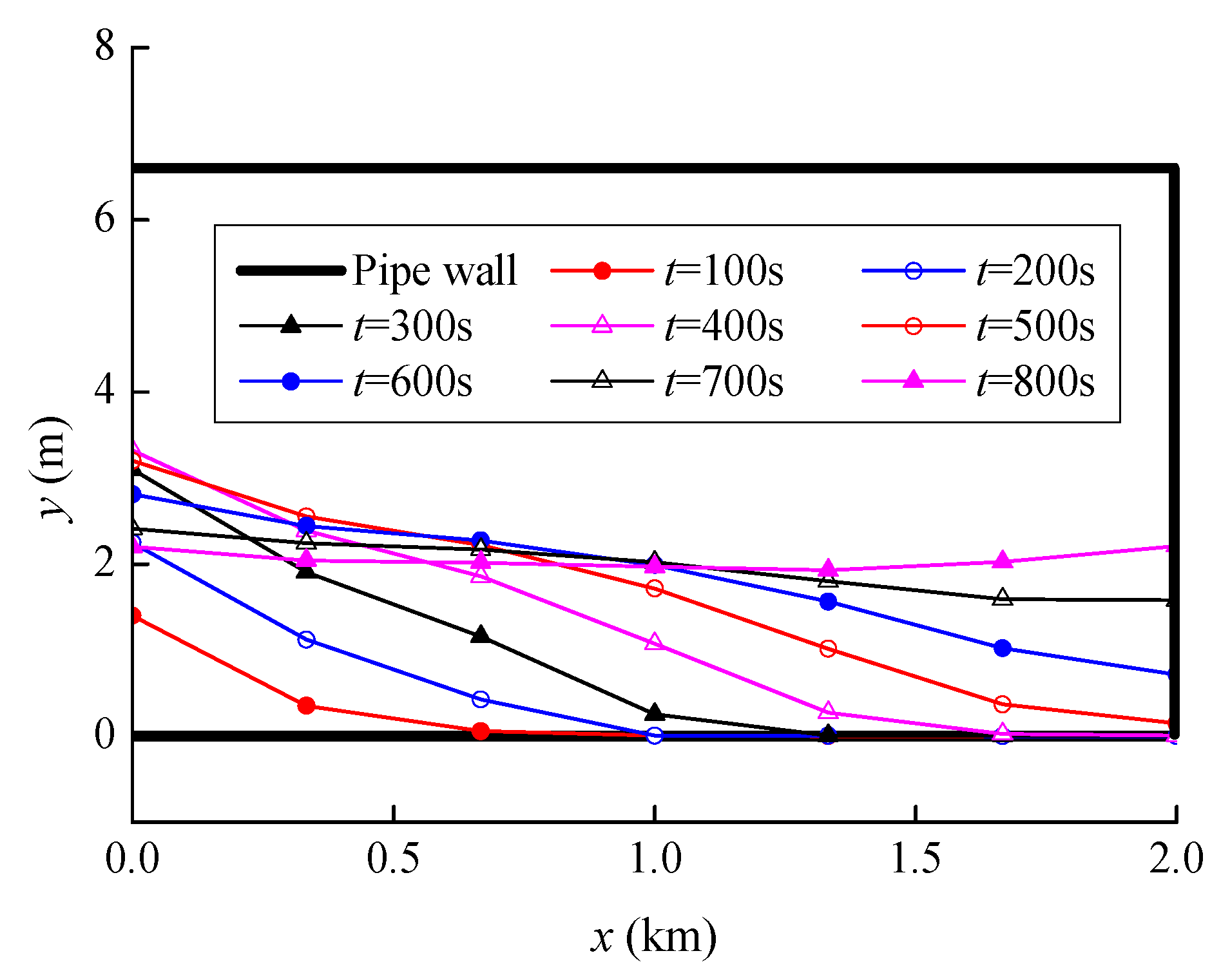
3.3. Validation
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| t | time (s) |
| ∆t | time step (s) |
| v | flow velocity (m/s) |
| g | acceleration of gravity (m/s2) |
| c | wave speed in free surface flow (m/s) |
| y | water level (m) |
| S | energy gradient |
| q | inlet discharge from the transverse direction within a unit length (m3/s) |
| A | flow-filled cross area (m2) |
| B | water surface width (m) |
| H | pressure head (m) |
| Q | discharge (m3/s) |
| x | distance along pipe from inlet (m) |
| ∆x | length of segment (m) |
| a | speed of pressure wave in pressured flow (m/s) |
| f | Darcy friction factor |
| D | main pipe diameter (m) |
| Cd | discharge coefficient |
| τ | valve opening ratio |
| ∆y | water level step (m) |
| ε | allowable error (m3/s) |
| α | forward coefficient |
| β | forward coefficient |
| γ | searching range (m) |
| DFM | double forward method |
| MOC | method of characteristics |
References
- Boulos, P.F.; Karney, B.W.; Wood, D.J.; Lingireddy, S. Hydraulic transient guidelines for protecting water distribution systems. J. Am. Water Work Assoc. 2005, 97, 111–124. [Google Scholar] [CrossRef]
- Arnold, J.G.; Srinivasan, R.; Muttiah, R.S.; Williams, J.R. Large area hydrologic modeling and assessment—Part 1: Model development. J. Am. Water Resour. Assoc. 1998, 34, 73–89. [Google Scholar] [CrossRef]
- Fang, H.Q.; Chen, L.; Dlakavu, N.; Shen, Z.Y. Basic Modeling and simulation tool for analysis of hydraulic transients in hydroelectric power plants. IEEE Trans. Energy Convers. 2008, 23, 834–841. [Google Scholar] [CrossRef]
- Bergant, A.; Simpson, A.R.; Vitkovsky, J. Developments in unsteady pipe flow friction modelling. J. Hydraul. Res. 2001, 39, 249–257. [Google Scholar] [CrossRef]
- Bergant, A.; Simpson, A.R.; Tijsseling, A.S. Water hammer with column separation: A historical review. J. Fluids Struct. 2006, 22, 135–171. [Google Scholar] [CrossRef]
- Martins, N.M.C.; Delgado, J.N.; Ramos, H.M.; Covas, D.I.C. Maximum transient pressures in a rapidly filling pipeline with entrapped air using a CFD model. J. Hydraul. Res. 2017, 55, 506–519. [Google Scholar] [CrossRef]
- Lingireddy, S.; Wood, D.J.; Zloczower, N. Pressure surges in pipeline systems resulting from air releases. J. Am. Water Works Assn. 2004, 7, 88–94. [Google Scholar] [CrossRef]
- Martin, C.S. Proceedings of the 2nd International Conference on Pressure Surges; The Bedford Press: London, UK, 1976; pp. F2/15–F2/28. [Google Scholar]
- Triki, A. Water-hammer control in pressurized-pipe flow using a branched polymeric penstock. J. Pipeline Syst. Eng. Pract. 2017, 8, 04017024. [Google Scholar] [CrossRef]
- Tian, W.X.; Su, G.H.; Wang, G.P.; Qiu, S.Z.; Xia, Z.J. Numerical simulation and optimization on valve-induced water hammer characteristics for parallel pump feedwater system. Ann. Nucl. Eng. 2008, 35, 2280–2287. [Google Scholar] [CrossRef]
- Kim, H.; Kim, S.; Kim, Y.; Kim, J. Optimization of operation parameters for direct spring loaded pressure relief valve in a pipeline system. J. Pressure Vessel Technol. 2018, 140, 051603. [Google Scholar] [CrossRef]
- Kim, H.; Baek, D.; Kim, S. Exploring integrated impact of temperature and flow velocity on chlorine decay for a pilot-scale water distribution system. Desalin. Water Treat. 2019, 138, 265–269. [Google Scholar] [CrossRef]
- Apollonio, C.; Balacco, G.; Fontana, N.; Giugni, M.; Marini, G.; Piccinni, A.F. Hydraulic transients caused by air expulsion during rapid filling of undulating pipelines. Water 2016, 8, 25. [Google Scholar] [CrossRef]
- Balacco, G.; Apollonio, C.; Piccinni, A.F. Experimental analysis of air valve behaviour during hydraulic transients. J. Appl. Water Eng. Res. 2015, 3, 3–11. [Google Scholar] [CrossRef]
- Chaudhry, M.; Hussaini, M. Second-order accurate explicit finite-difference schemes for waterhammer analysis. J. Fluids Eng. 1985, 107, 523–529. [Google Scholar] [CrossRef]
- Kochupillai, J.; Ganesan, N.; Padmanabhan, C. A new finite element formulation based on the velocity of flow for water hammer problems. Int. J. Press. Vessels Pip. 2005, 82, 1–14. [Google Scholar] [CrossRef]
- Izquierdo, J.; Iglesias, P.L. Mathematical modelling of hydraulic transients in simple systems. Math. Comput. Modell. 2002, 35, 801–812. [Google Scholar] [CrossRef]
- Karadzic, U.; Bulatovic, V.; Bergant, A. Valve-Induced water hammer and column separation in a pipeline apparatus. Strojniski Vestn. J. Mech. Eng. 2014, 60, 742–754. [Google Scholar] [CrossRef]
- Zhang, B.; Wan, W.; Shi, M. Experimental and numerical simulation of water hammer in gravitational pipe flow with continuous air entrainment. Water 2018, 10, 928. [Google Scholar] [CrossRef]
- Coronado-Hernandez, O.E.; Fuertes-Miquel, V.S.; Besharat, M.; Ramos, H.M. Experimental and numerical analysis of a water emptying pipeline using different air valves. Water 2017, 9, 98. [Google Scholar] [CrossRef]
- Wan, W.; Li, F. Sensitivity analysis of operational time differences for a pump–valve system on a water hammer response. J. Pressure Vessel Technol. 2016, 138, 011303. [Google Scholar] [CrossRef]
- Karney, B.W.; McInnis, D. Efficient calculation of transient flow in simple pipe networks. J. Hydraul. Eng. 1992, 118, 1014–1030. [Google Scholar] [CrossRef]
- Wylie, E.B.; Streeter, V.L. Fluid Transients; McGraw-Hill International Book Co.: New York, NY, USA, 1978. [Google Scholar]
- Wan, W.; Huang, W. Investigation on complete characteristics and hydraulic transient of centrifugal pump. J. Mech. Sci. Technol. 2011, 25, 2583–2590. [Google Scholar] [CrossRef]
- Wan, W.; Zhang, B. Investigation of water hammer protection in water supply pipeline systems using an intelligent self-controlled surge tank. Energies 2018, 11, 1450. [Google Scholar] [CrossRef]
- Wan, W.; Huang, W. Investigation of fluid transients in centrifugal pump integrated system with multi-channel pressure vessel. J. Pressure Vessel Technol. 2013, 135, 9. [Google Scholar] [CrossRef]
- Hou, Q.; Zhang, L.X.; Tijsseling, A.S.; Kruisbrink, A.C.H. Rapid filling of pipelines with the SPH particle method. In International Conference on Advances in Computational Modeling and Simulation; Lixiang, Z., Ed.; Elsevier Science Bv: Amsterdam, The Netherlands, 2012; pp. 38–43. [Google Scholar]
- Balacco, G.; Fontana, N.; Apollonio, C.; Giugni, M.; Marini, G.; Piccinni, A.F. Pressure surges during filling of partially empty undulating pipelines. ISH J. Hydraul. Eng. 2018, 1–9. [Google Scholar] [CrossRef]
- Chaudhry, M.H. Applied Hydraulic Transients; Van Nostrand Reinhold: New York, NY, USA, 1979. [Google Scholar]
- Vakil, A.; Firoozabadi, B. Investigation of Valve-Closing Law on the Maximum Head Rise of a Hydropower Plant. Sci. Iranica Trans. B-Mech. Eng. 2009, 16, 222–228. [Google Scholar]
- Pepa, D.; Ursoniu, C.; Gillich, G.R.; Campian, C.V. Water hammer effect in the spiral case and penstock of Francis turbines. In International Conference on Applied Sciences; Lemle, L.D., Jiang, Y., Eds.; Iop Publishing Ltd.: Bristol, UK, 2017. [Google Scholar]
- Wan, W.; Huang, W. Water hammer simulation of a series pipe system using the MacCormack time marching scheme. Acta Mech. 2018, 229, 3143–3160. [Google Scholar] [CrossRef]



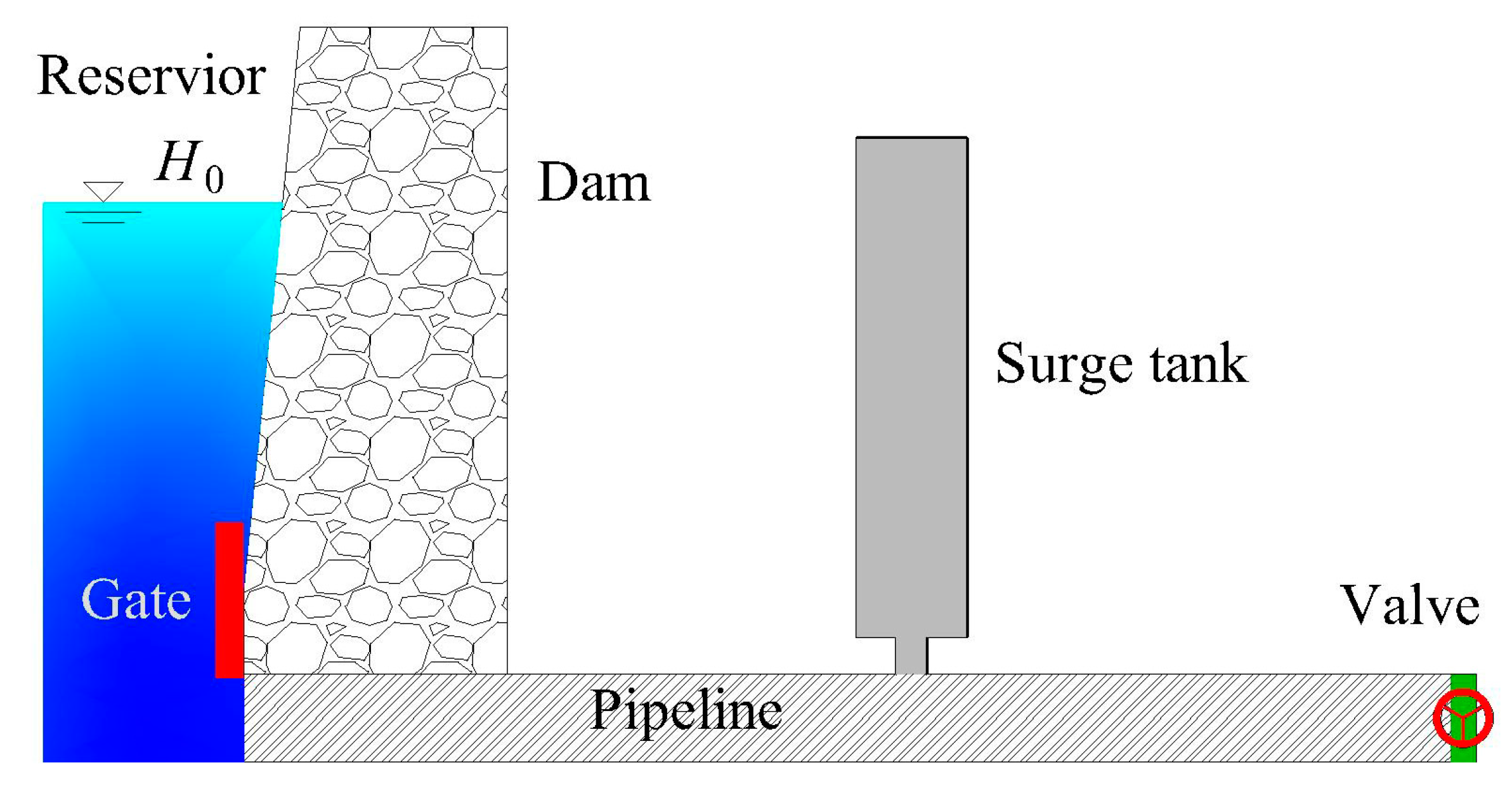




| Reservoir | Dam | Gate | Pipeline | Surge Tank | Valve | ||
|---|---|---|---|---|---|---|---|
| Water level | Height | Nominal area | Cross area | Length | Cross area | Water level | Height |
| 10 m | 15 m | 25 m2 | 35 m2 | 2000 m | 100 m2 | 10 m | 15 m |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, B.; Wan, W.; Fan, L. Investigation into Complex Boundary Solutions of Water Filling Process in Pipeline Systems. Water 2019, 11, 641. https://doi.org/10.3390/w11040641
Zhang B, Wan W, Fan L. Investigation into Complex Boundary Solutions of Water Filling Process in Pipeline Systems. Water. 2019; 11(4):641. https://doi.org/10.3390/w11040641
Chicago/Turabian StyleZhang, Boran, Wuyi Wan, and Leilei Fan. 2019. "Investigation into Complex Boundary Solutions of Water Filling Process in Pipeline Systems" Water 11, no. 4: 641. https://doi.org/10.3390/w11040641
APA StyleZhang, B., Wan, W., & Fan, L. (2019). Investigation into Complex Boundary Solutions of Water Filling Process in Pipeline Systems. Water, 11(4), 641. https://doi.org/10.3390/w11040641






