A New Method to Estimate Reference Crop Evapotranspiration from Geostationary Satellite Imagery: Practical Considerations
Abstract
1. Introduction
2. Material and Methods
2.1. Used Datasets
2.2. The T-ABL Model
2.3. LSA SAF Reference Evapotranspiration Data
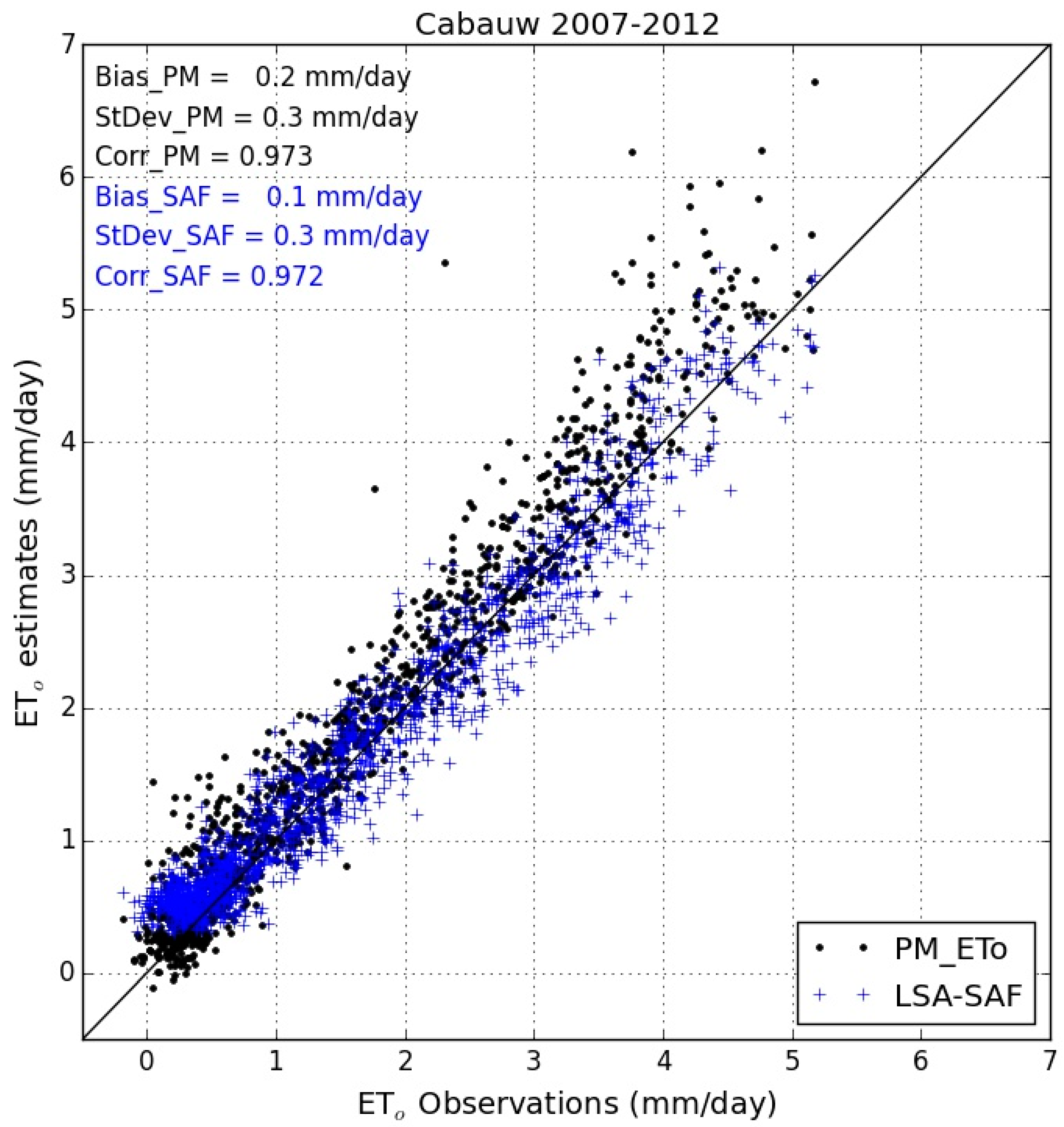
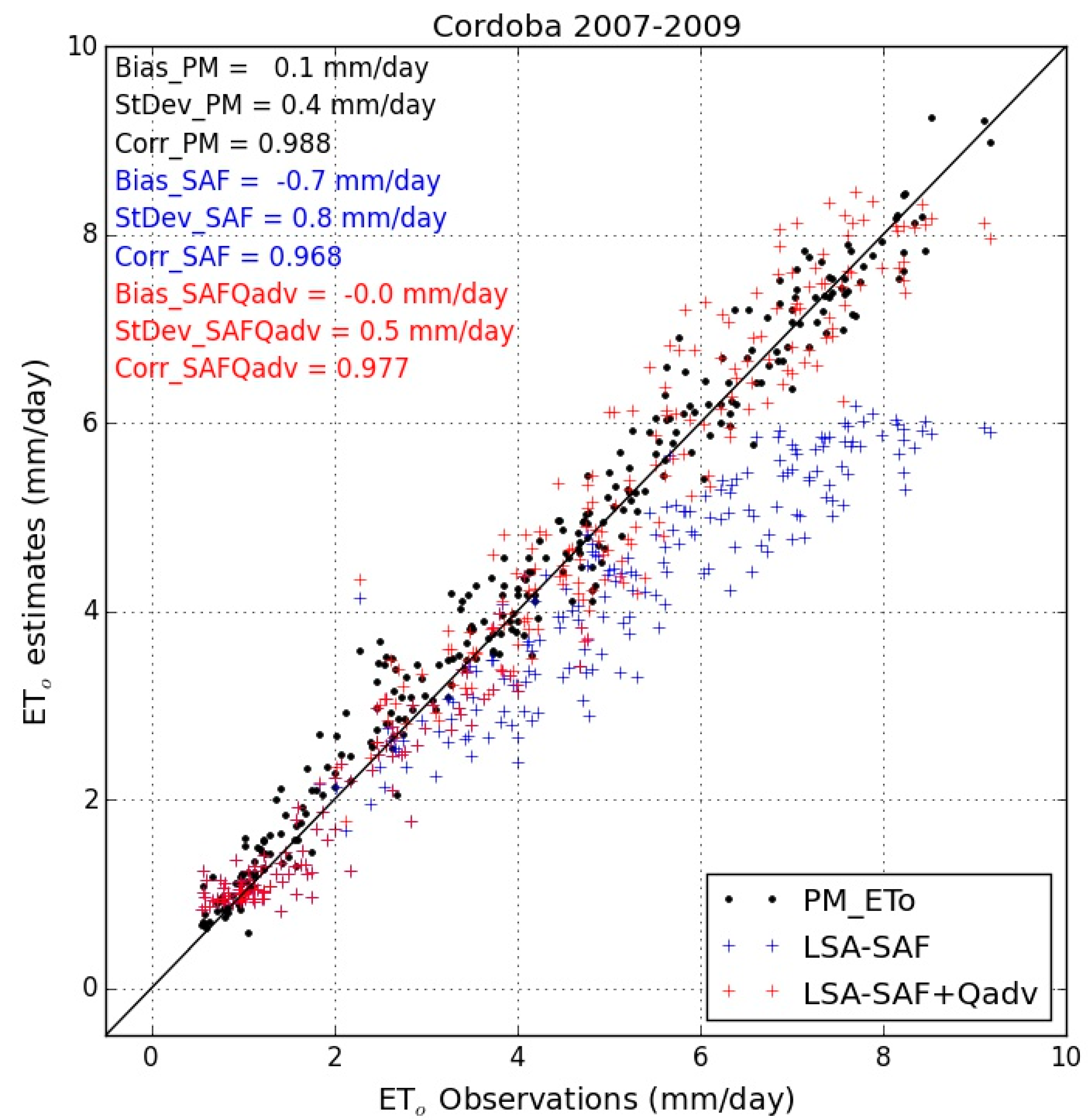
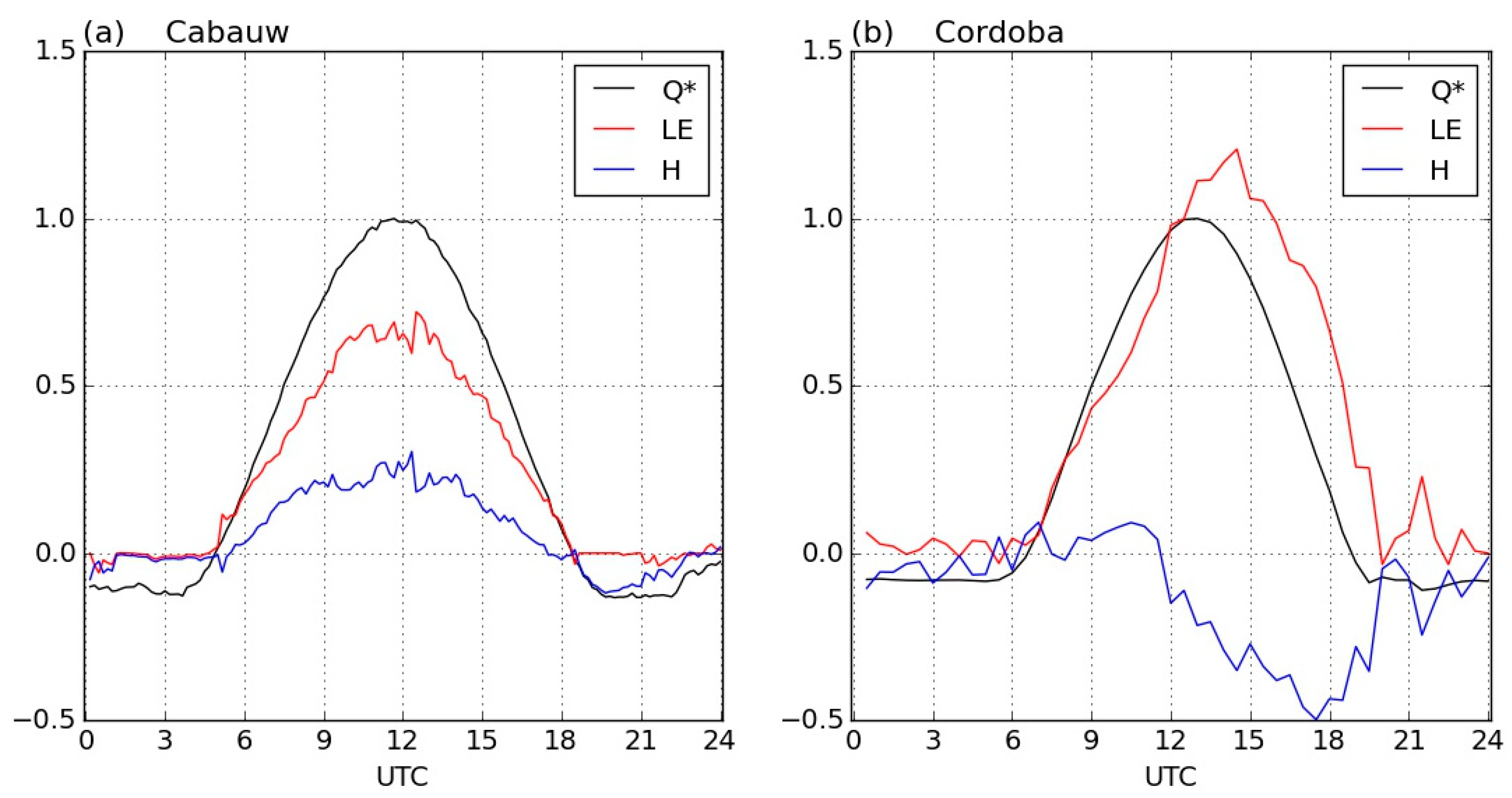
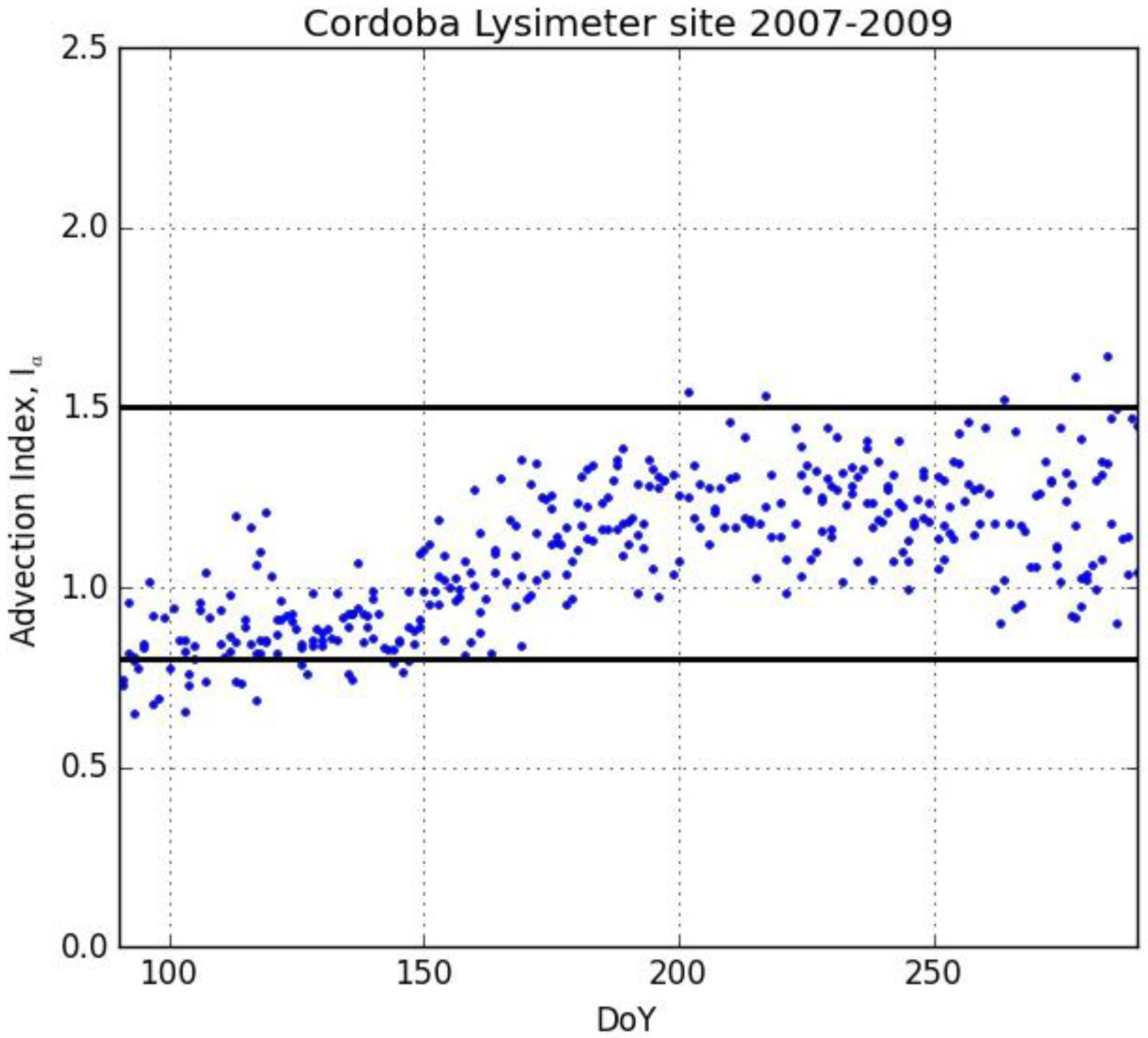
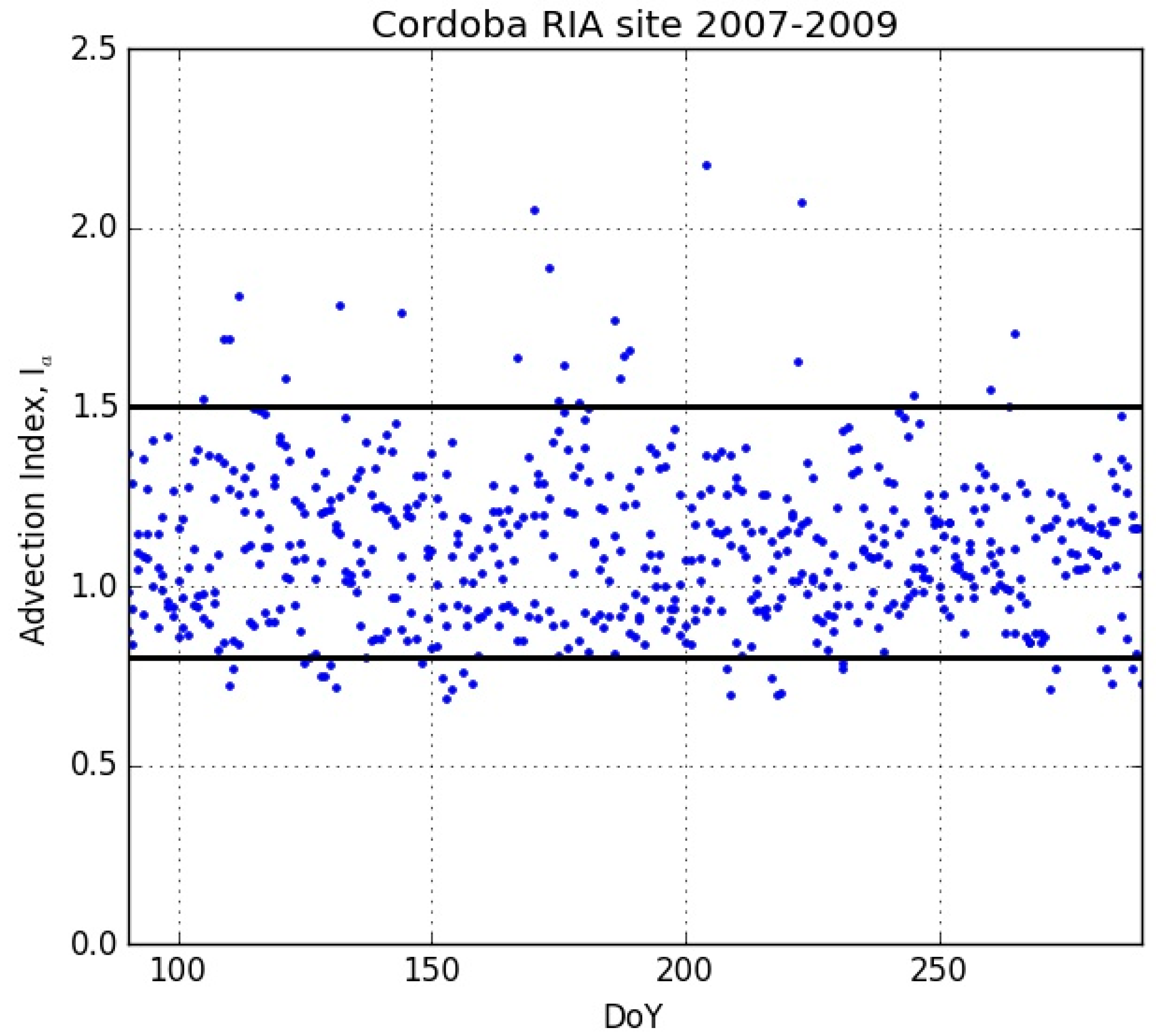
3. Results
4. Discussion
5. Concluding Remarks
Author Contributions
Funding
Acknowledgements
Conflicts of Interest
Annex—Terminology
| Name/Symbol | Meaning |
| ETo | Reference crop as defined in [1] and [2]; local advection effects are excluded. |
| ETc | Crop water requirement as defined in [1], and obtained via kc.ETo, with kc being a crop factor. Note that [1] introduced ETo and ETc to avoid the use of potential evapotranspriation, since the latter was generally used in as a maximum ET, without specifying the crop of surface. |
| PM-ETo | Version of the Penman-Monteith equation for estimating ETo, introduced by [1]. |
| LSA SAF ETo | Estimated using Equation (1) derived from thermodynamics and PBL-physics in [4] and validated in [5]. |
| METREF | The LSA SAF ETo estimates, as specified in the LSA SAF catalogue. |
| T-ABL | The model to estimate ETo derived from thermodynamics and atmospheric boundary layer (ABL) physics, leading to Equation (1). |
| Q* | Net radiation, i.e., the sum of the down-welling short and long wave radiation reaching the surface minus reflected (short and long wave) and the emitted (long wave) radiation. |
| Rs | Global radiation, or down-welling short wave radiation reaching the surface |
| Rext | Incoming shortwave radiation at the top of the atmosphere, often denoted as the extra-terrestrial radiation. |
References
- Allen, R.G.; Pereira, L.A.; Raes, D.; Smith, M. Crop Evapotranspiration—Guidelines for Computing Crop Water Requirements; FAO Irrigation and Drainage Paper 56; FAO: Rome, Italy, 1998; p. 293. [Google Scholar]
- Pereira, L.S.; Perrier, A.; Allen, R.G.; Alves, I. Evapotranspiration: Concepts and future trends. J. Irrig. Drain. 1999, 125, 45–51. [Google Scholar] [CrossRef]
- Allen, R.G.; Pruitt, W.O.; Wright, J.L.; Howell, T.A.; Ventura, F.; Snyder, R.; Itenfisu, D.; Steduto, P.; Berengena, J.; Baselga, J.; et al. A recommendation on standardized surface resistance for hourly calculation of reference ETo by the FAO56 Penman-Monteith method. Agric. Water Manag. 2006, 81, 1–22. [Google Scholar] [CrossRef]
- De Bruin, H.A.R.; Trigo, I.F.; Bosveld, F.C.; Meirink, J.F. A thermodynamically based model for actual evapotranspiration of an extensive grass field close to FAO reference suitable for remote sensing application. J. Hydrometeor. 2016, 17, 1373–1382. [Google Scholar] [CrossRef]
- Trigo, I.F.; de Bruin, H.; Beyrich, F.; Bosveld, F.C.; Gavilán, P.; Groh, J.; López-Urrea, R. Validation of reference evapotranspiration from Meteosat Second Generation (MSG) observations. Agric. For. Meteorol. 2018, 259, 271–285. [Google Scholar] [CrossRef]
- Schmidt, W. Strahlung und Verdunstung an freienWasserflächen; ein Beitrag zum Wärmehaushalt des Weltmeers und zum Wasserhaushalt der Erde (Radiation and evaporation over open water surfaces; a contribution to the heat budget of the world ocean and to the water budget of the Earth). Ann. Calender Hydrogr. Marit. Meteorol. 1915, 43, 111–124. [Google Scholar]
- Slatyer, R.O.; McIlroy, I.C. Evaporation and the principles of its measurement. In Practical Micrometeorology; CSIRO (Australia); UNESCO: Paris, France, 1961. [Google Scholar]
- De Bruin, H.A.R. A model for the Priestley-Taylor parameter. J. Appl. Meteorol. 1983, 22, 572–578. [Google Scholar] [CrossRef]
- Monteith, J.L. Accommodation between transpiring vegetation and the convective boundary layer. J. Hydrol. 1995, 166, 251–263. [Google Scholar] [CrossRef]
- McNaughton, K.G.; Spriggs, T.W. A mixed-layer model for regional evaporation. Bound.-Layer Meteorol. 1986, 34, 243–262. [Google Scholar] [CrossRef]
- McNaughton, K.G.; Jarvis, P.G. Predicting effects of vegetation changes on transpiration and evaporation. In Water Deficits and Plant Growth; Kozlowski, T.T., Ed.; Academic Press: New York, NY, USA, 1983; Volume 7, pp. 1–47. [Google Scholar]
- Temesgen, B.; Allen, R.G.; Jensen, D.T. Adjusting temperature parameters to reflect well-watered conditions. J. Irrig. Drain. Eng. 1999, 125, 26–33. [Google Scholar] [CrossRef]
- Geiger, B.; Meurey, C.; Lajas, D.; Franchistéguy, L.; Carrer, D.; Roujean, J.L. Near real-time provision of downwelling shortwave radiation estimates derived from satellite observations. Meteorol. Appl. 2008, 15, 411–420. [Google Scholar] [CrossRef]
- Greuell, W.; Meirink, J.F.; Wang, P. Retrieval and validationof global, direct, and diffuse irradiance derived from SEVIRI satellite observations. J. Geophys. Res. Atmos. 2013, 118, 2340–2361. [Google Scholar] [CrossRef]
- Monna, W.; Bosveld, F. In Higher Spheres: 40 Years of Observations at the Cabauw Site. 2013, p. 56. Available online: http://publicaties.minienm.nl/documenten/in-higher-spheres-40-years-of-observations-at-the-cabauw-site (accessed on 21 February 2019).
- Berengena, J.; Gavilán, P. Reference Evapotranspiration Estimation in a Highly Advective Semiarid Environment. J. Irrig. Drain. Eng. 2005, 131, 147–163. [Google Scholar] [CrossRef]
- Cruz-Blanco, M.; Gavilán, P.; Santos, C.; Lorite, I.J. Assessment of reference evapotranspiration using remote sensing and forecasting tools under semi-arid conditions. Int. J. Appl. Earth Obs. Geoinf. 2014, 33, 280–289. [Google Scholar] [CrossRef]
- Castellvi, F.; Gavilan, P.; Gonzalez-Dugo, M.P. Combining the bulk transfer formulation and surface renewal analysis for estimating the sensible heat flux without involving the parameter kB−1. Water Resour. Res. 2014, 50, 8179–8190. [Google Scholar] [CrossRef]
- De Bruin, H.A.R.; Holtslag, A.A.M. A simple parameterization of the surface fluxes of sensible and latent heat during daytime compared with the Penman-Monteith concept. J. Appl. Meteorol. 1982, 21, 1610–1621. [Google Scholar] [CrossRef]
- De Bruin, H.A.R. From Penman to Makkink. In Proceedings and Information: TNO Committee on Hydrological Research N°39, Den Haag, The Netherlands, 25 March 1987; Hooghart, J.C., Ed.; The Netherlands Organization for Applied Scientific Research TNO: Den Haag, The Netherlands, 1987; pp. 5–31. [Google Scholar]
- Pinker, R.T.; Tarpley, J.D.; Laszlo, I.; Mitchell, K.E.; Houser, P.R.; Wood, E.F.; Schaake, J.C.; Robock, A.; Lohmann, D.; Cosgrove, B.A.; et al. Surface radiation budgets in support of the GEWEX Continental-Scale International Project (GCIP) and the GEWEX Americas Prediction Project (GAPP), including the North American Land Data Assimilation System (NLDAS) project. J. Geophys. Res. 2003, 108, 8844. [Google Scholar] [CrossRef]
- Dee, D.P.; Simmons, A.J.; Berrisford, P.; Poli, P.; Kobayashi, S.; Andrae, U.; Balmaseda, M.A.; Balsamo, G.; Bauer, P.; Bechtold, P.; et al. The ERA-Interim reanalysis: configuration and performance of the data assimilation system. Q. J. R. Meteorol. Soc. 2011, 137, 553–597. [Google Scholar] [CrossRef]
- De Bruin, H.A.R.; Trigo, I.F.; Gavilán, P.; Martínez-Cob, A.; González-Dugo, M.P. Reference crop evapotranspiration estimated from geostationary satellite imagery. In Proceedings of the Remote Sensing and Hydrology, Jackson Hole, WY, USA, 27–30 September 2010; pp. 111–114. [Google Scholar]
- Makkink, G.F. Testing the Penman formula by means of lysimeters. J. Inst. Water Eng. 1957, 11, 277–288. [Google Scholar]
- Hargreaves, G.H.; Samani, Z. A Reference crop evapotranspiration from temperature. Appl. Eng. Agric. 1985, 1, 96–99. [Google Scholar] [CrossRef]
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
de Bruin, H.A.R.; Trigo, I.F. A New Method to Estimate Reference Crop Evapotranspiration from Geostationary Satellite Imagery: Practical Considerations. Water 2019, 11, 382. https://doi.org/10.3390/w11020382
de Bruin HAR, Trigo IF. A New Method to Estimate Reference Crop Evapotranspiration from Geostationary Satellite Imagery: Practical Considerations. Water. 2019; 11(2):382. https://doi.org/10.3390/w11020382
Chicago/Turabian Stylede Bruin, Henk A. R., and Isabel F. Trigo. 2019. "A New Method to Estimate Reference Crop Evapotranspiration from Geostationary Satellite Imagery: Practical Considerations" Water 11, no. 2: 382. https://doi.org/10.3390/w11020382
APA Stylede Bruin, H. A. R., & Trigo, I. F. (2019). A New Method to Estimate Reference Crop Evapotranspiration from Geostationary Satellite Imagery: Practical Considerations. Water, 11(2), 382. https://doi.org/10.3390/w11020382





