Overtopping Failure of a Reinforced Tailings Dam: Laboratory Investigation and Forecasting Model of Dam Failure
Abstract
:1. Introduction
2. Experimental
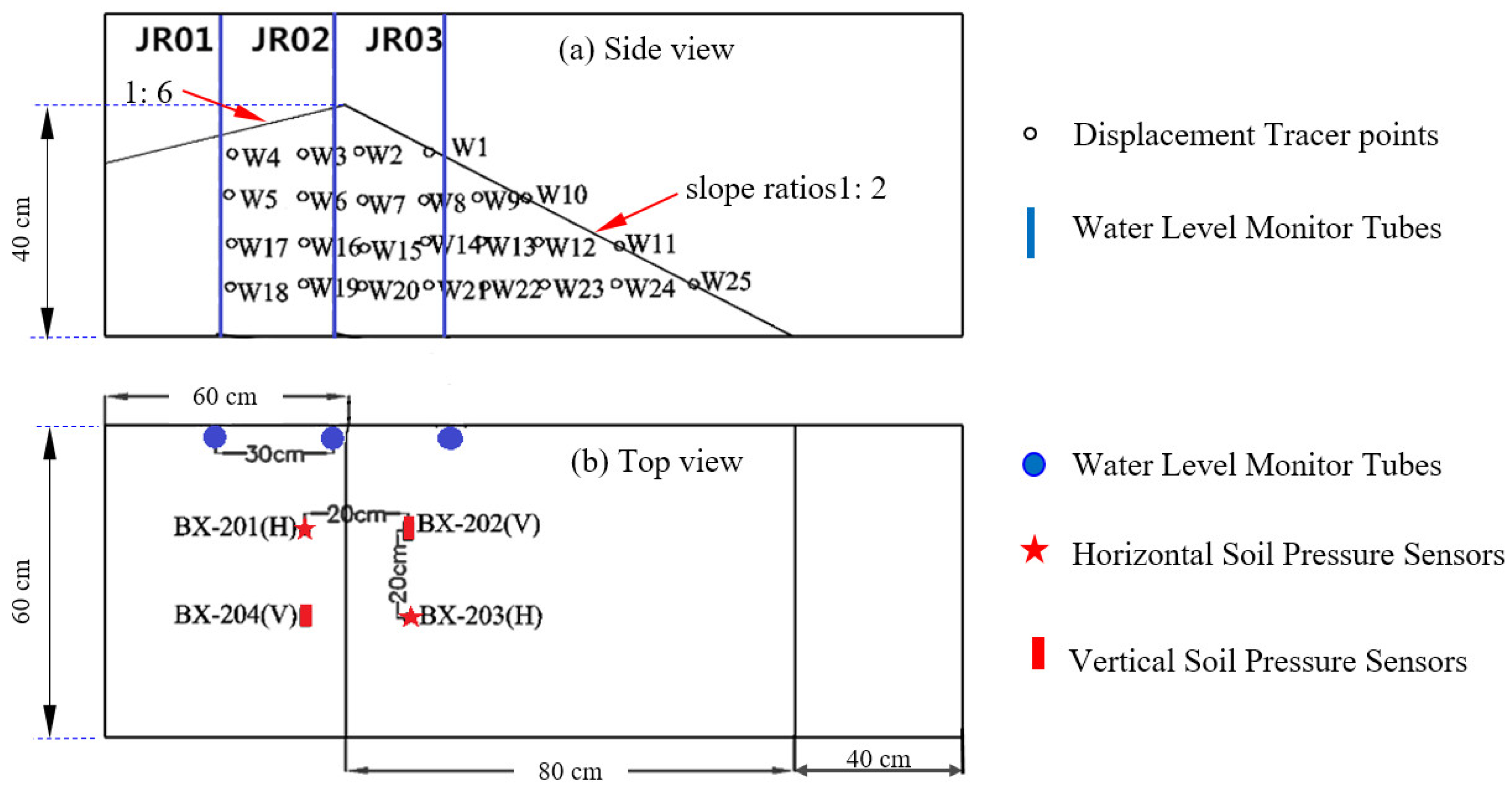
2.1. Experimental Facilities
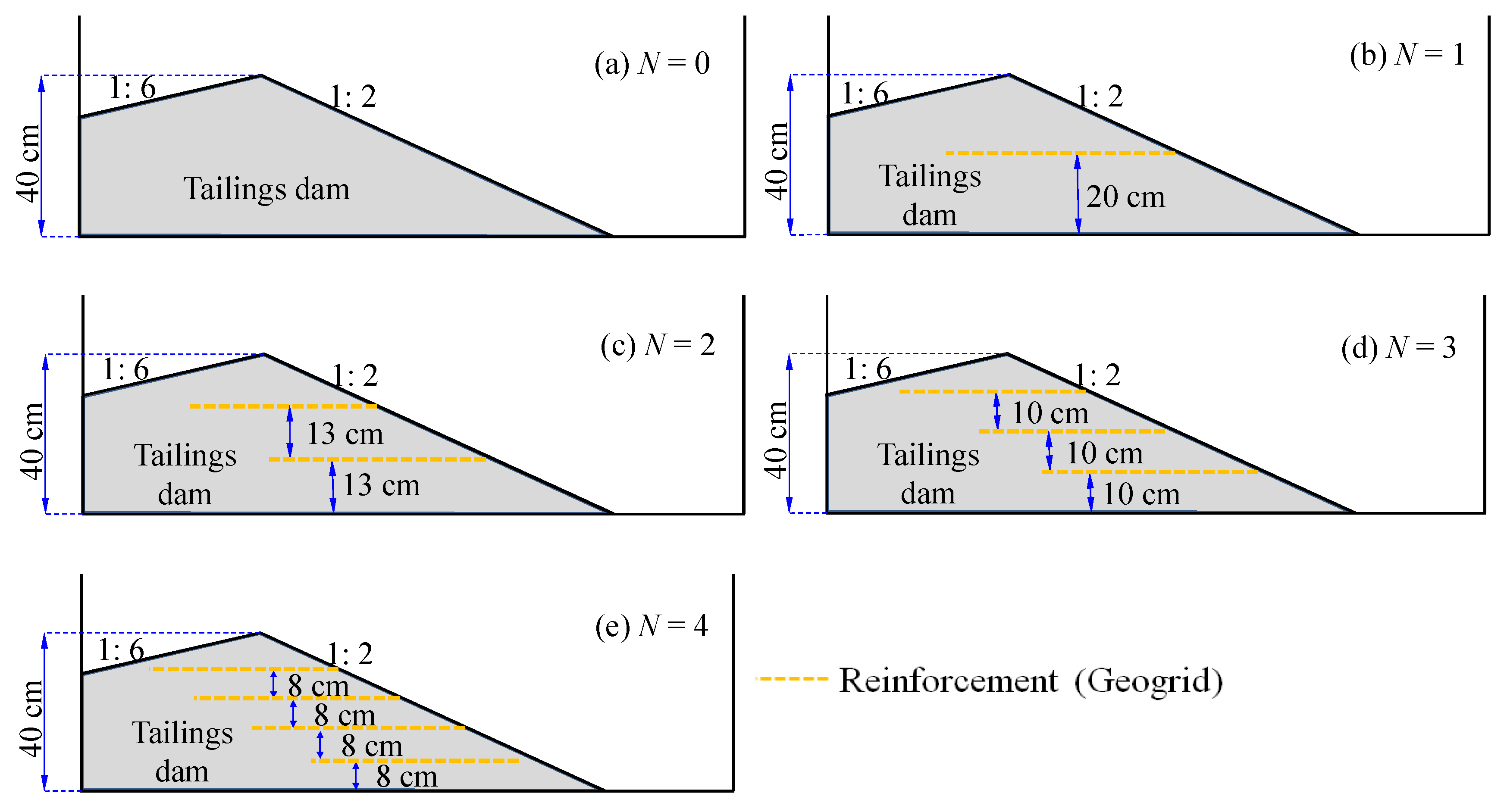
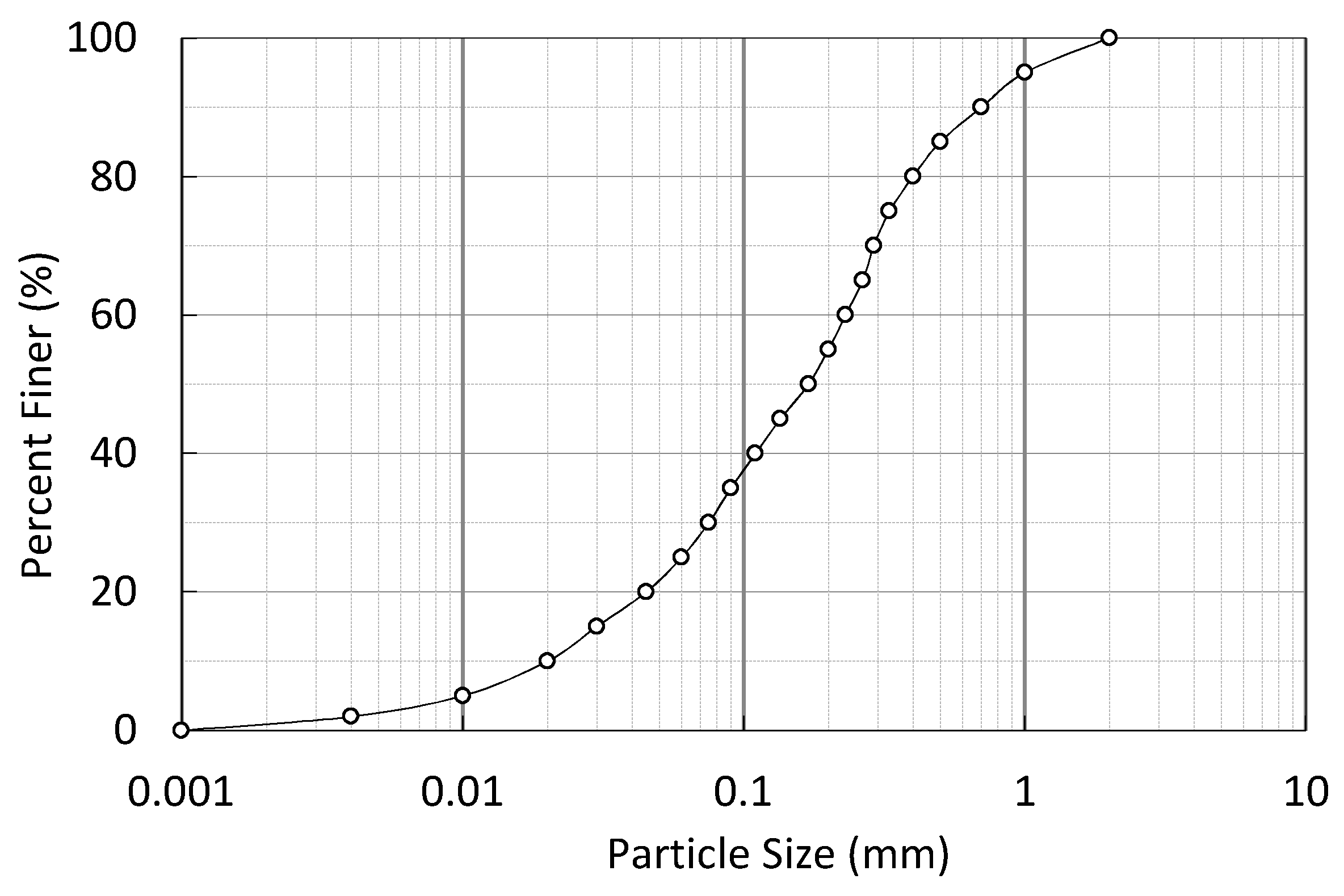
2.2. Experimental Materials and Procedures
- The tailings with 10% water content were prepared according to the test requirements (as shown in Figure 5a).
- Layer compaction method for construction tailings dam, each layer was 8 cm, the compactness of each layer is 85% (as shown in Figure 5b).
- Lay down the reinforcement and sensors (as shown in Figure 5c).
- The displacement monitoring mark were placed on the side of the dam every 10 cm according to the drawn grid lines (as shown in Figure 5d).
- Install the camera, and calibrate these equipment after their placement in the tailings dam.
3. Results and Discussion
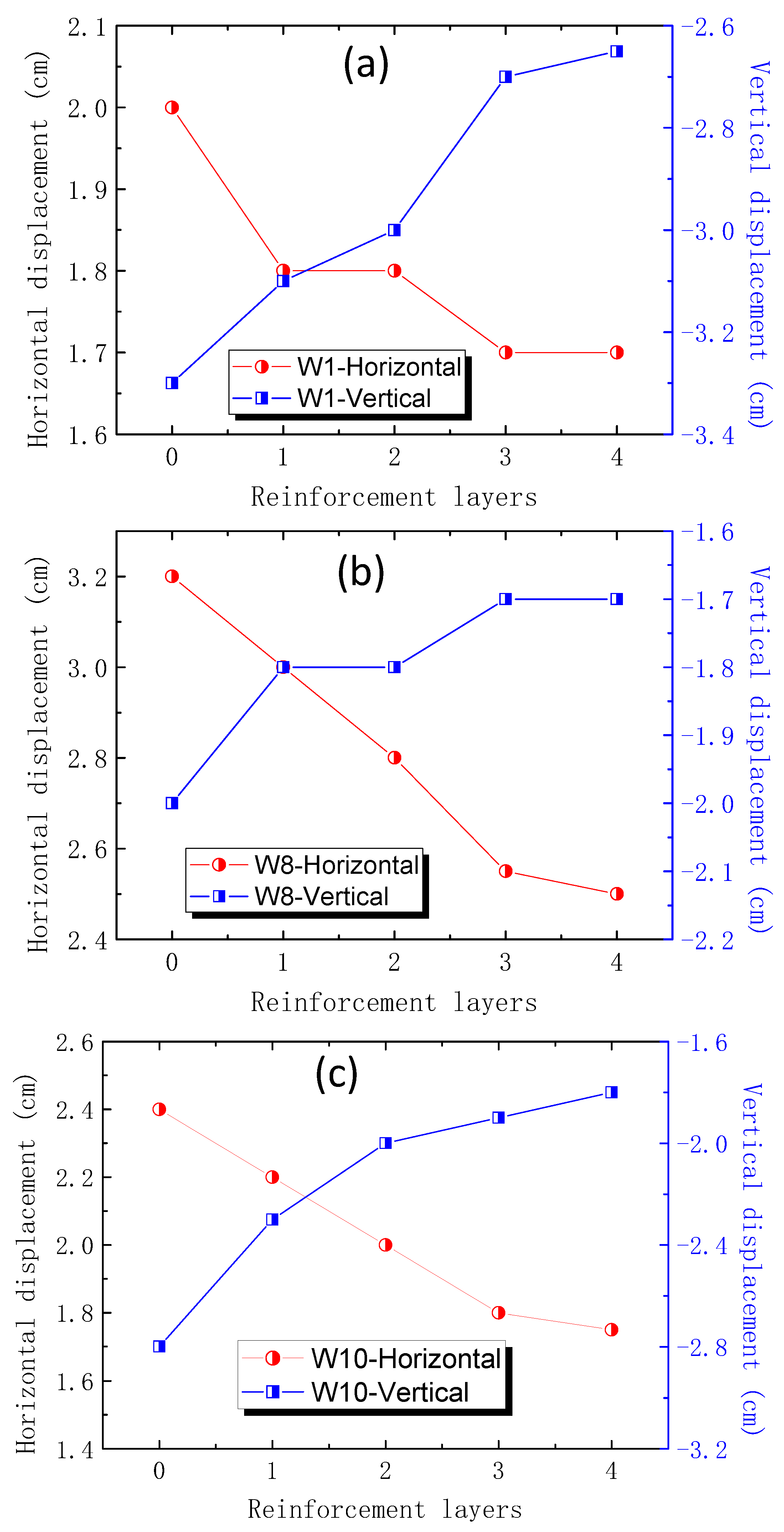
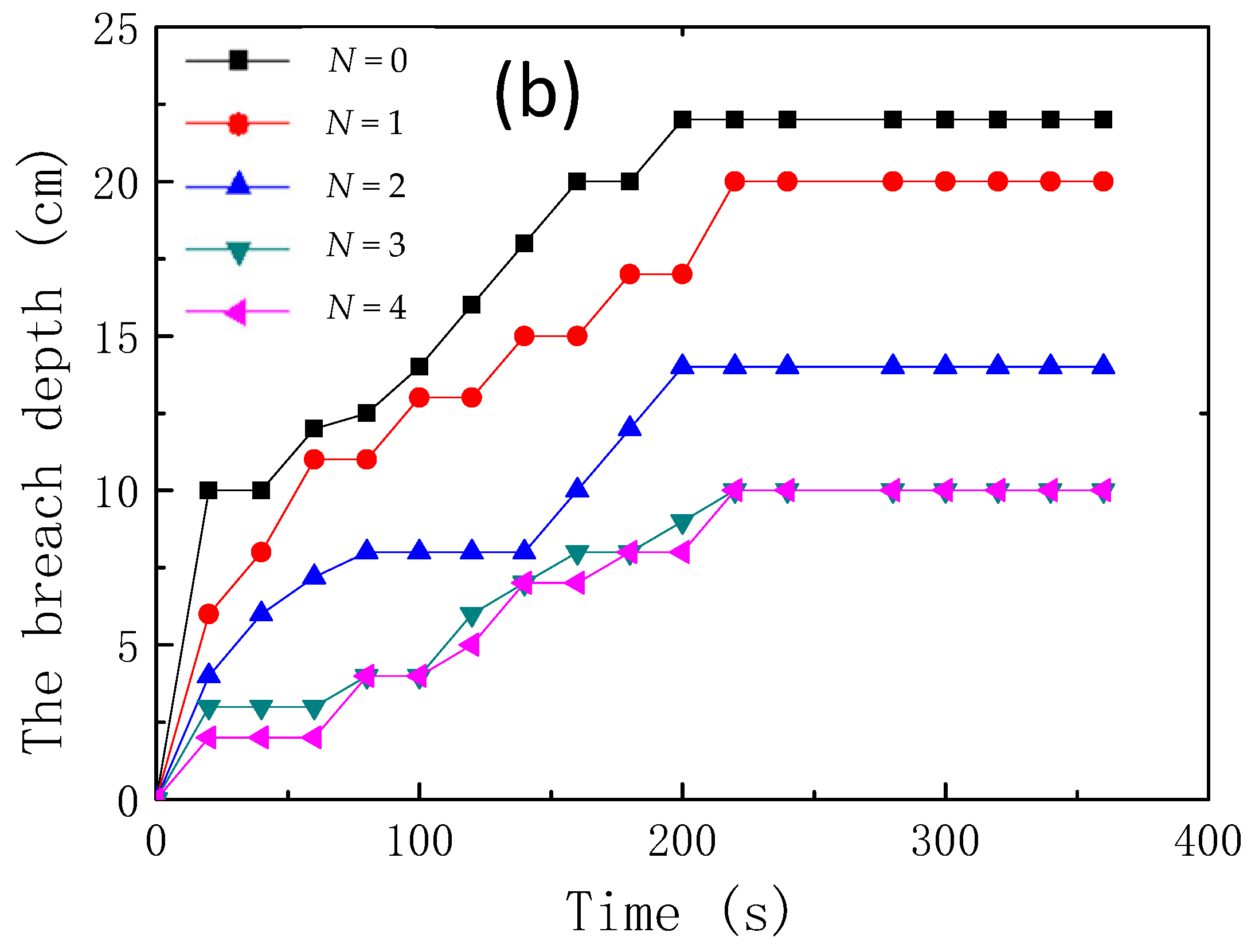
3.1. Reinforcement Density on Dam Displacement
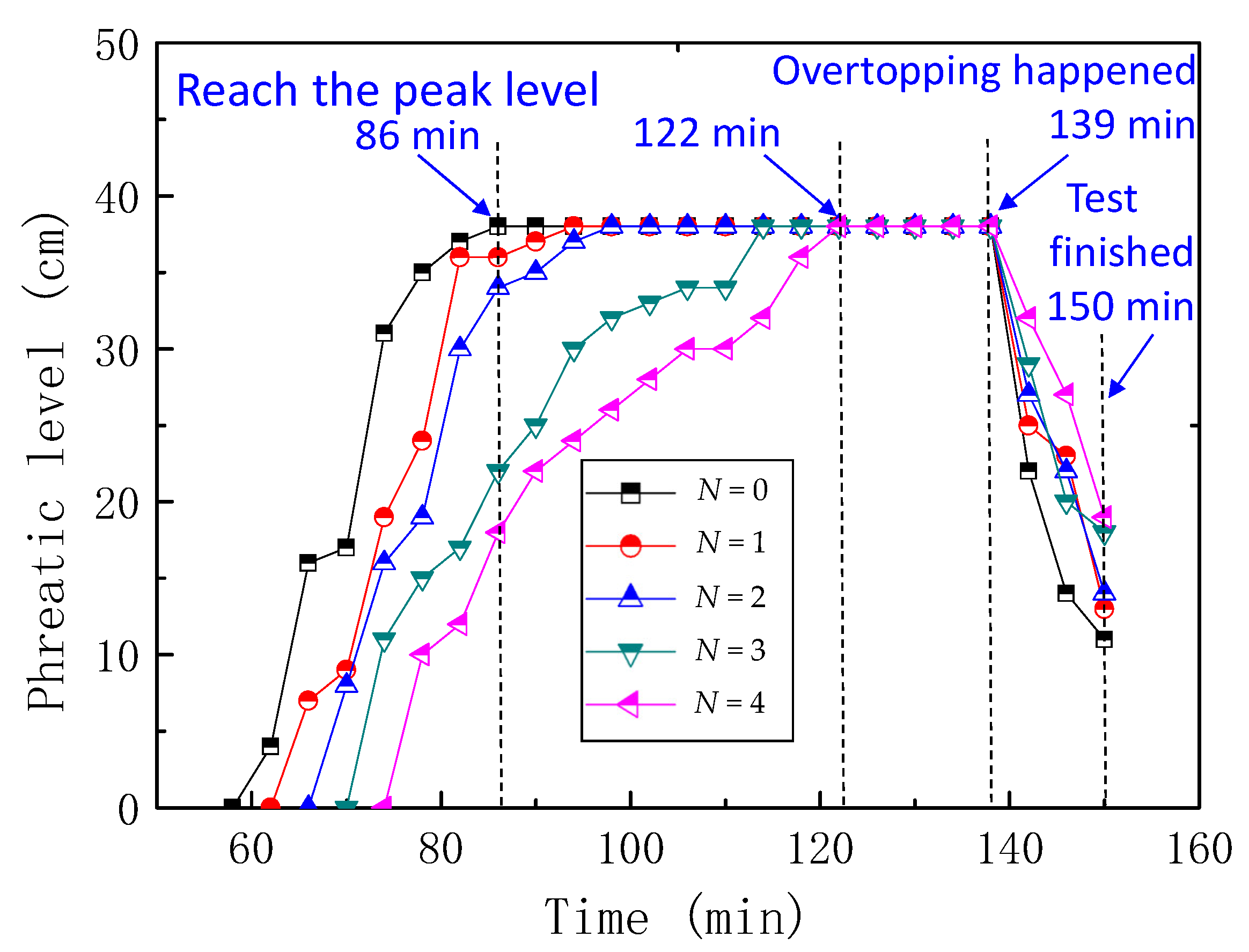
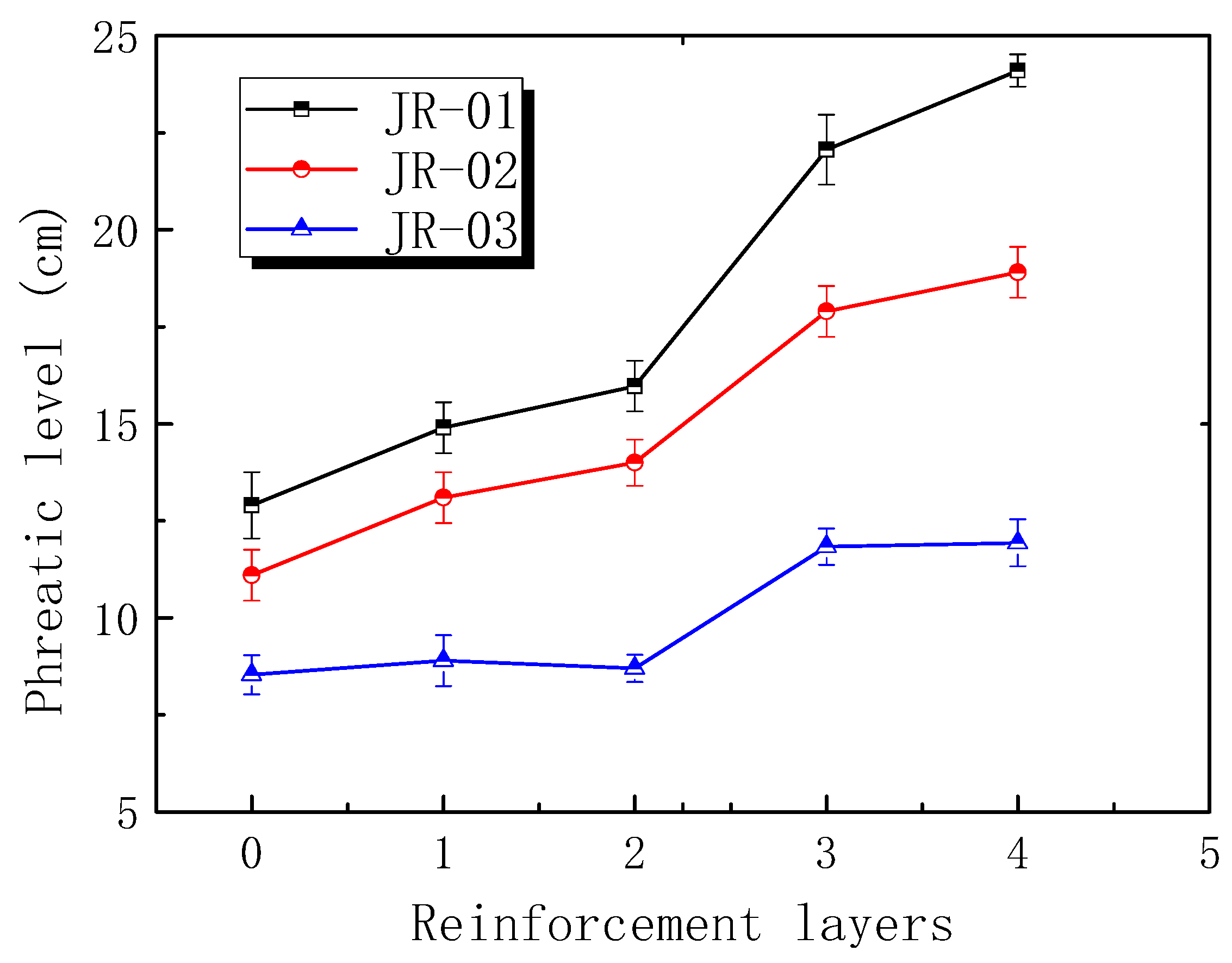
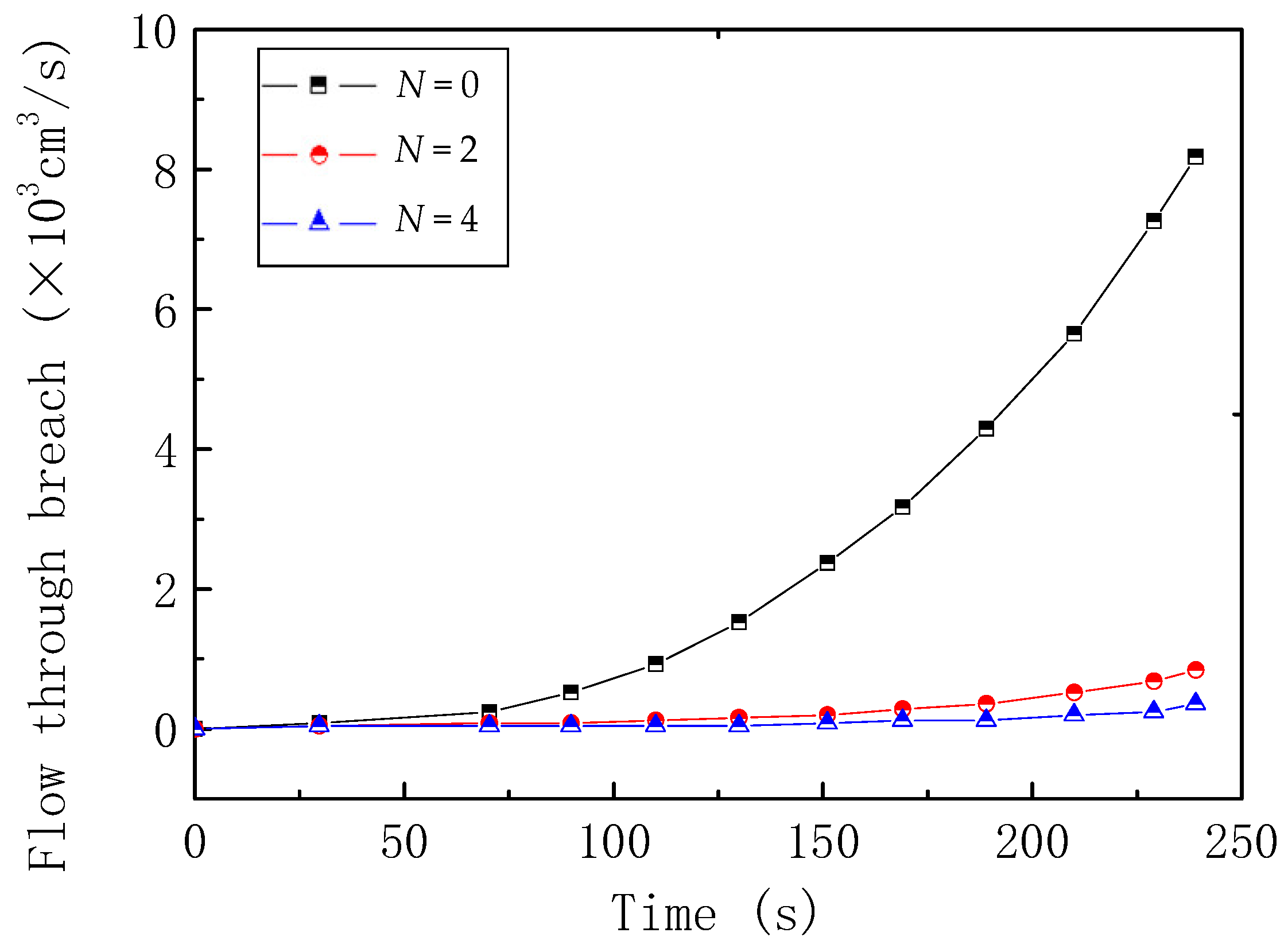
3.2. Reinforcement Layers and Flooding Time on the Phreatic Level
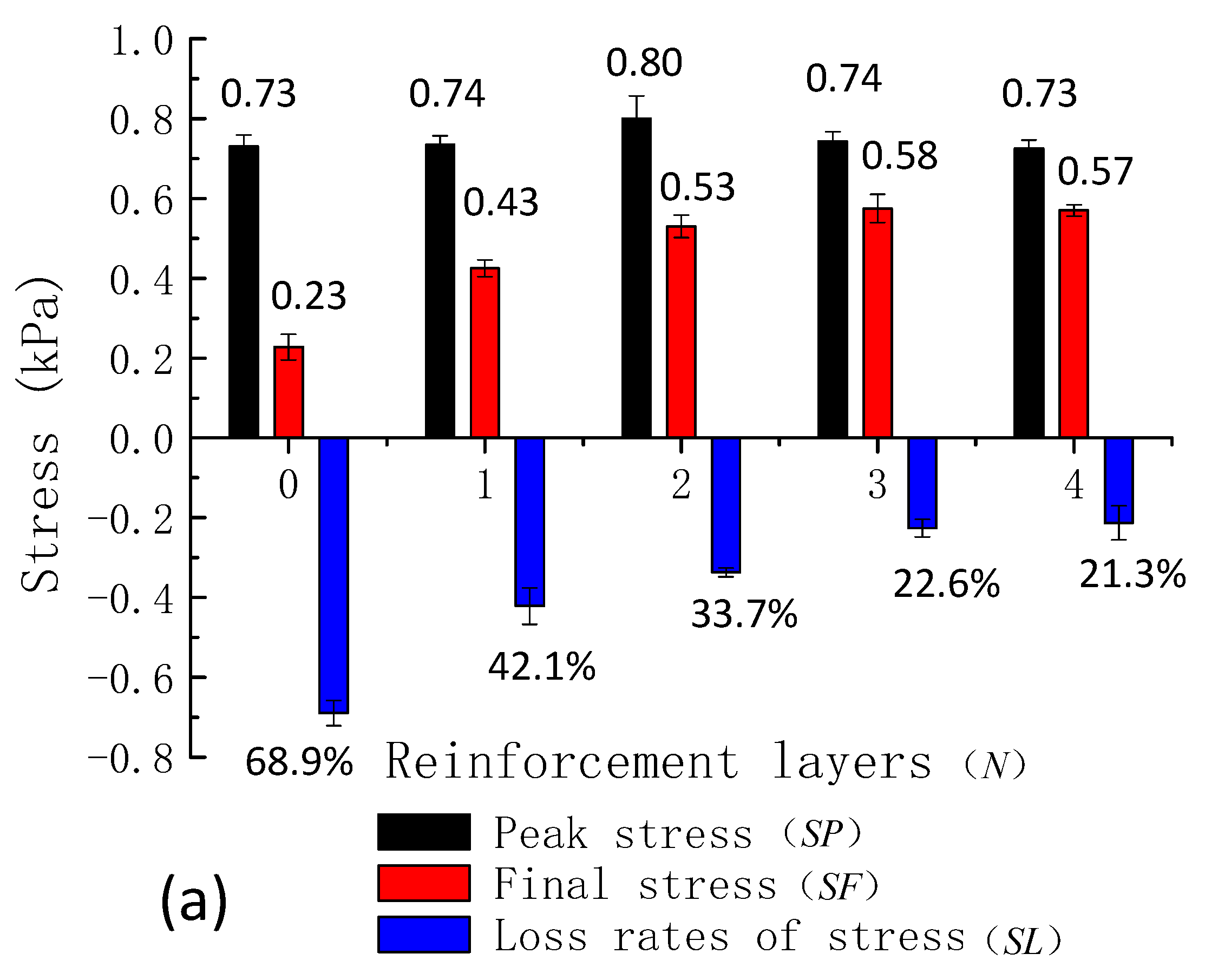
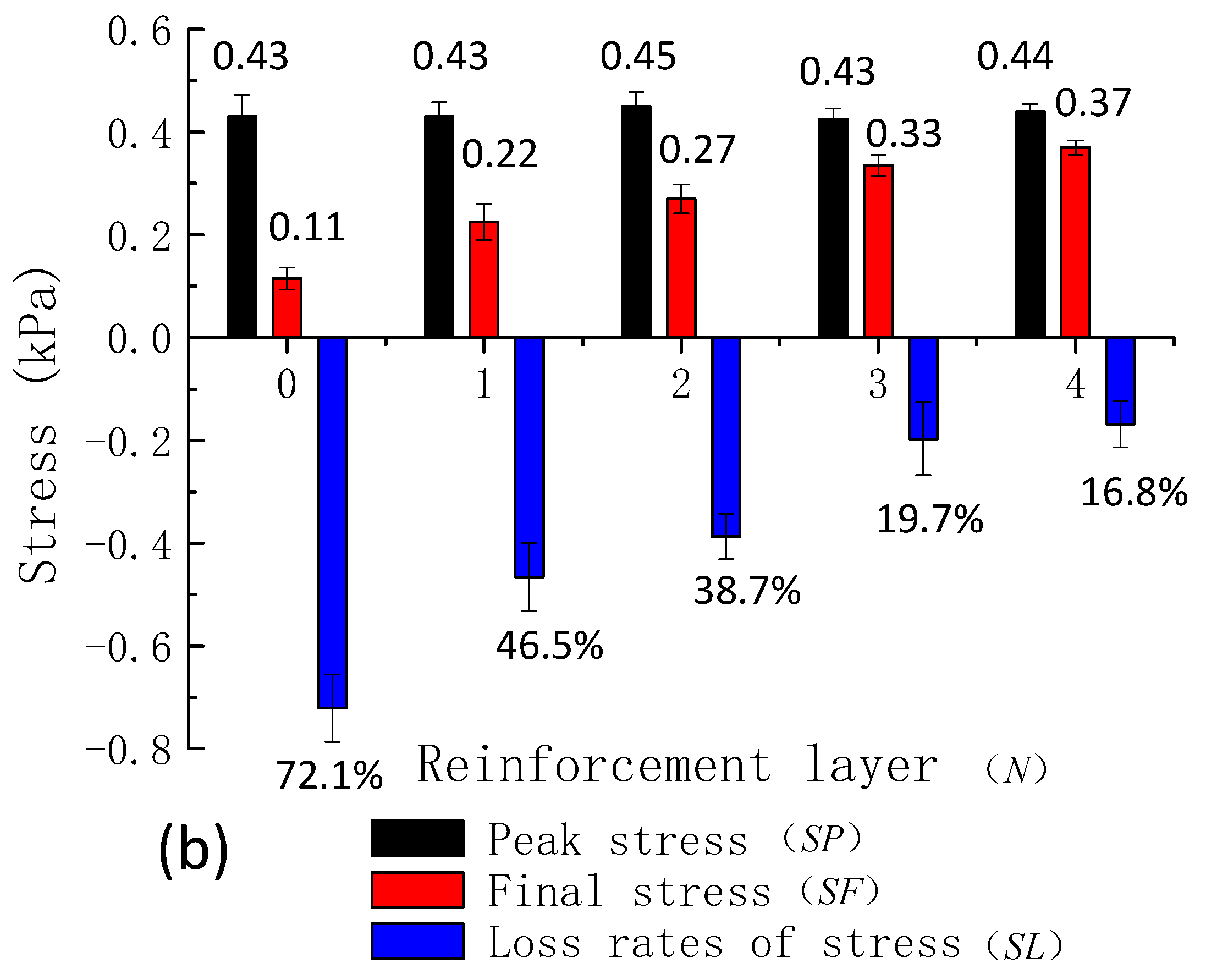
3.3. Stress Change during Overtopping
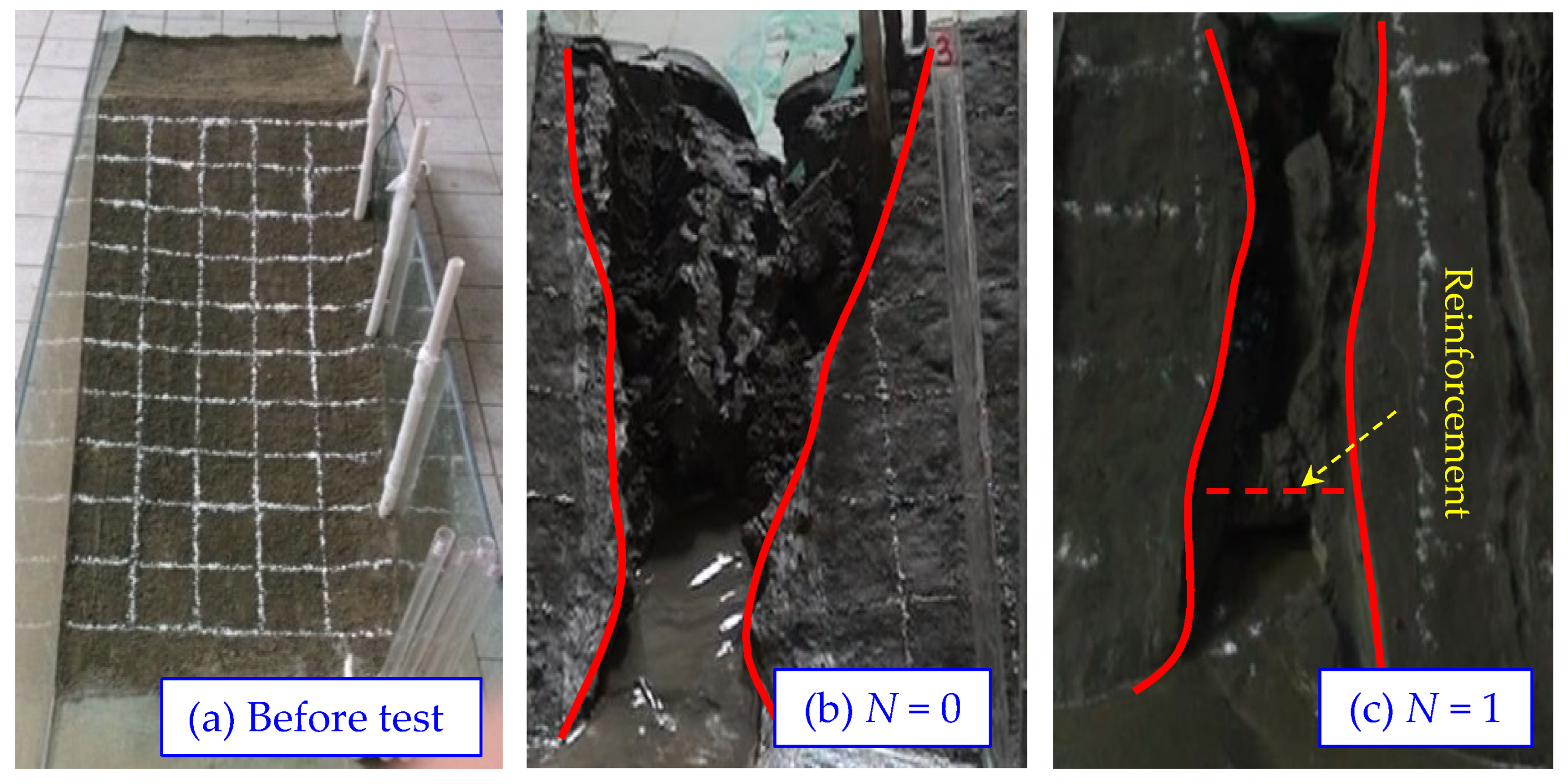
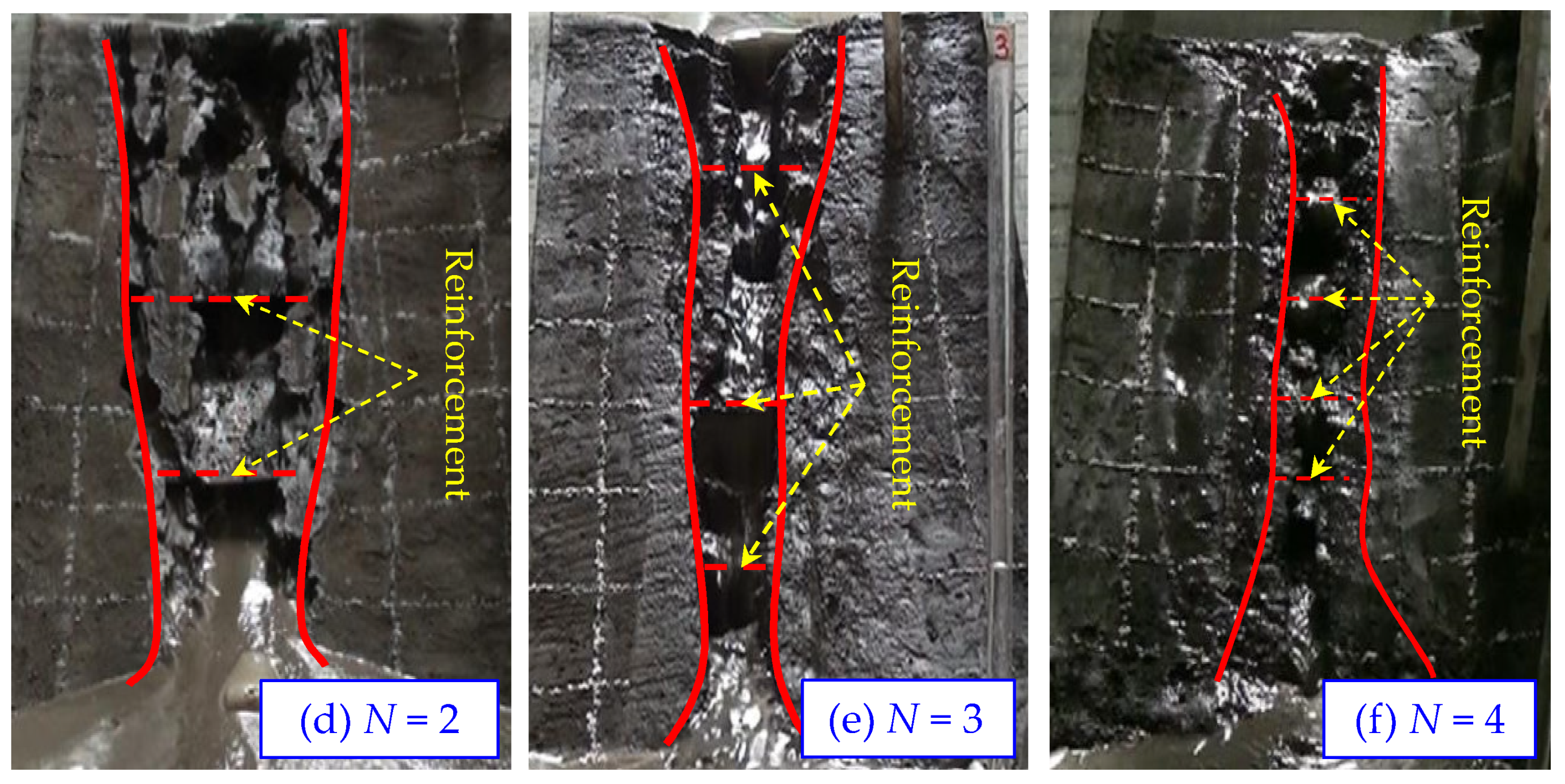
3.4. Analysis on Evolution of the Overtopping Failure Process
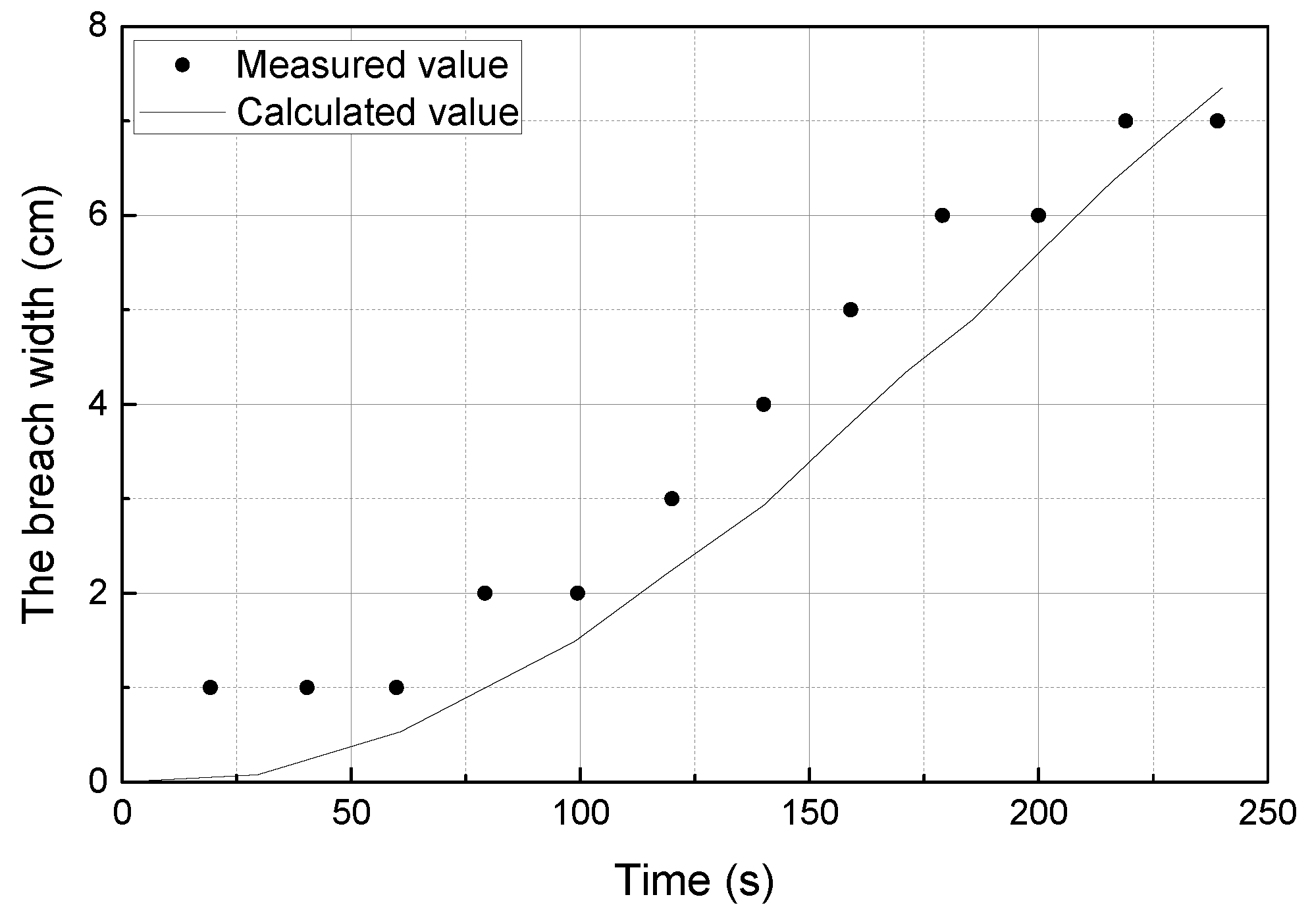
4. Prediction Model of Overtopping Failure
5. Conclusions
- The reinforcement layers significantly affected the phreatic level. As the number of reinforcement layers increased, the rate of the rise of the phreatic level was slowing down, but the final phreatic level became higher.
- The number of reinforcement layers had an impact on the final shape of dam breach after overtopping erosion. The final breach was shaped as a ladder with four reinforcement layers and an hourglass without reinforcement.
- The reinforcement layers could improve the anti-erosion capability of tailings dam. With the increase of reinforcement layers, the size of breach and the loss rate of stress were reduced significantly.
- Based on the erosion principle, a mathematical model including the number of reinforcement layers was proposed to predict the width and flow of the tailings dam breach.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ozcan, N.; Ulusay, R.; Isik, N. A study on geotechnical characterization and stability of downstream slope of a tailings dam to improve its storage capacity (Turkey). Environ. Earth Sci. 2013, 69, 1871–1890. [Google Scholar] [CrossRef]
- Reid, C.; Becaert, V.; Aubertin, M.; Rosenbaum, R.; Deschenes, L. Life cycle assessment of mine tailings management in Canada. J. Clean Prod. 2009, 17, 471–479. [Google Scholar] [CrossRef]
- Harder, L.F.; Stewart, J.P. Failure of Tapo Canyon tailings dam. J. Perform. Constr. Facil. 1996, 10, 109–114. [Google Scholar] [CrossRef]
- McDermott, R.K.; Sibley, J.M. The Aznalcollar tailings dam accident a case study. Miner. Res. Eng. 2000, 9, 101–118. [Google Scholar] [CrossRef]
- Blight, G.E. Destructive mudflows as a consequence of tailings dyke failures. Proc. Inst. Civ. Eng. Geotech. Eng. 1997, 125, 9–18. [Google Scholar] [CrossRef]
- Fourie, A.; Blight, G.E.; Papageorgiou, G. Static liquefaction as a possible explanation for the Merriespruit tailings dam failure. Can. Geotech. J. 2001, 38, 707–719. [Google Scholar] [CrossRef]
- Villavicencio, G.; Espinace, R.; Palma, J. Failures of sand tailings dams in a highly seismic country. Can. Geotech. J. 2014, 51, 449–464. [Google Scholar] [CrossRef]
- Sun, E.J.; Zhang, X.K.; Li, Z.X.; Wang, Y.H. Tailings dam flood overtopping failure evolution pattern. Procedia Eng. 2012, 28, 356–362. [Google Scholar] [CrossRef]
- Hahn, W.; Hanson, G.J.; Cook, K.R. Breach morphology observations of embankment overtopping tests. Water Res. 2000, 1–10. [Google Scholar] [CrossRef]
- Coleman, S.E.; Andrews, D.P.; Webby, M.G. Overtopping breaching of noncohesive homogeneous embankments. J. Hydraul. Eng. 2002, 128, 829–838. [Google Scholar] [CrossRef]
- Hanson, G.J.; Cook, K.R.; Hunt, S.L. Physical modeling of overtopping erosion and breach formation of cohesive embankments. Trans. ASAE 2005, 48, 1783–1794. [Google Scholar] [CrossRef]
- Tørum, A.; Kuhnen, F.; Menze, A. On berm breakwaters. Stability, scour, overtopping. Coast. Eng. 2003, 49, 209–238. [Google Scholar] [CrossRef]
- Hanson, G.J.; Cook, K.R.; Hahn, W.; Britton, S.L. Observed erosion processes during embankment overtopping tests. In Proceedings of the ASAE Annual Meeting, Las Vegas, NV, USA, 27–30 July 2003; pp. 11–17. [Google Scholar] [CrossRef]
- Yang, Y.; Cao, S.Y.; Yang, K.J.; Li, W.P. Experimental study of breach process of landslide dams by overtopping and its initiation mechanisms. J. Hydrodyn. 2015, 27, 872–883. [Google Scholar] [CrossRef]
- Yuan, S.U.; Tang, H.W.; Li, L.; Pan, Y.; Amini, F. Combined wave and surge overtopping erosion failure model of HPTRM levees: Accounting for grass-mat strength. Ocean Eng. 2015, 109, 256–269. [Google Scholar] [CrossRef]
- Mohamed, M.M.A.; El-Ghorab, E.A.S. Investigating scale effects on breach evolution of overtopped sand embankments. Water Sci. 2016, 30, 84–95. [Google Scholar] [CrossRef]
- Mohamed, M.M.A. Overtopping breach peak outflow approximation of embankment dam by using Monte Carlo method. In Proceedings of the Joint Conference on Water Resource Engineering and Water Resources Planning and Management, Minneapolis, MN, USA, 30 July–2 August 2000. [Google Scholar] [CrossRef]
- He, Z.G.; Hu, P.; Zhao, L.; Wu, G.F.; Pähtz, T. Modeling of Breaching Due to Overtopping Flow and Waves Based on Coupled Flow and Sediment Transport. Water 2015, 7, 4283–4304. [Google Scholar] [CrossRef]
- Mulder, J.P.M.; Hommes, S.; Horstman, E.M. Implementation of coastal erosion management in the Netherlands. Ocean Coast. Manag. 2011, 54, 888–897. [Google Scholar] [CrossRef]
- Van Rijn, L.C. Coastal erosion and control. Ocean Coast. Manag. 2011, 54, 867–887. [Google Scholar] [CrossRef]
- Alessio, G.; Eleni, D.; Stuart, P.; Giorgio, S.; Kees, D.H. A Regional Application of Bayesian Modeling for Coastal Erosion and Sand Nourishment Management. Water 2019, 11, 61. [Google Scholar] [CrossRef]
- Carlsten, S.; Johansson, S.; Wörman, A. Radar techniques for indicating internal erosion in embankment dams. J. Appl. Geophys. 1995, 33, 143–156. [Google Scholar] [CrossRef]
- Ran, Q.H.; Wang, F.; Li, P.; Ye, S.; Tang, H.L.; Gao, J.H. Effect of rainfall moving direction on surface flow and soil erosion processes on slopes with sealing. J. Hydrol. 2018, 567, 478–488. [Google Scholar] [CrossRef]
- Wu, Y.Y.; Ouyang, W.; Hao, Z.C.; Lin, C.Y.; Liu, H.B.; Wang, Y.D. Assessment of soil erosion characteristics in response to temperature and precipitation in a freeze-thaw watershed. Geoderma 2018, 328, 56–65. [Google Scholar] [CrossRef]
- Callaghan, D.P.; Nielsen, P.; Short, A.; Ranasinghe, R. Statistical simulation of wave climate and extreme beach erosion. Coast. Eng. 2008, 55, 375–390. [Google Scholar] [CrossRef]
- Serpa, D.; Nunes, J.P.; Santos, J.; Sampaio, E.; Jacinto, R.; Veiga, S.; Lima, J.C.; Moreira, M.; Corte-Real, J.; Keizer, J.J.; et al. Impacts of climate and land use changes on the hydrological and erosion processes of two contrasting Mediterranean catchments. Sci. Total Environ. 2015, 538, 64–77. [Google Scholar] [CrossRef] [PubMed]
- Stefanidis, S.; Stathis, D. Effect of Climate Change on Soil Erosion in a Mountainous Mediterranean Catchment (Central Pindus, Greece). Water 2018, 10, 1469. [Google Scholar] [CrossRef]
- Simonneaux, V.; Cheggour, A.; Deschamps, C.; Mouillot, F.; Cerdan, O.; Le Bissonnais, Y. Land use and climate change effects on soil erosion in a semi-arid mountainous watershed (High Atlas, Morocco). J. Arid Environ. 2015, 122, 64–75. [Google Scholar] [CrossRef]
- Teng, H.; Liang, Z.; Chen, S.; Liu, Y.; Rossel, R.A.V.; Chappell, A.; Yu, W.; Shi, Z. Current and future assessments of soil erosion by water on the Tibetan Plateau based on RUSLE and CMIP5 climate models. Sci. Total Environ. 2018, 635, 673–686. [Google Scholar] [CrossRef]
- Wang, T.; Li, P.; Hou, J.M.; Li, Z.B.; Ren, Z.P.; Cheng, S.D.; Xu, G.C.; Su, Y.Y.; Wang, F.C. Response of the Meltwater Erosion to Runoff Energy Consumption on Loessal Slopes. Water 2018, 10, 1522. [Google Scholar] [CrossRef]
- Sujatha, E.R.; Sridhar, V. Spatial Prediction of Erosion Risk of a Small Mountainous Watershed Using RUSLE: A Case-Study of the Palar Sub-Watershed in Kodaikanal, South India. Water 2018, 10, 1608. [Google Scholar] [CrossRef]
- Deng, S.S.; Xia, J.Q.; Zhou, M.R.; Lin, F.F. Coupled modeling of bed deformation and bank erosion in the Jingjiang Reach of the middle Yangtze River. J. Hydrol. 2019, 568, 221–233. [Google Scholar] [CrossRef]
- Choi, C.E.; Cui, Y.F.; Kelvin, Y.K.A.; Liu, H.M.; Wang, J.; Liu, D.Z.; Wang, H. Case Study: Effects of a Partial-Debris Dam on Riverbank Erosion in the Parlung Tsangpo River, China. Water 2018, 10, 250. [Google Scholar] [CrossRef]
- Zhang, X.K.; Sun, E.J.; Li, Z.X. Experimental Study on Evolution Law of Tailings Dam Flood Overtopping. China Saf. Sci. J. 2011, 21, 118–124. [Google Scholar] [CrossRef]
- Liu, L.; Zhang, H.W.; Zhong, D.Y.; Miao, R.Z. Research on tailings dam break due to overtopping. J. Hydraul. Eng. 2014, 45, 675–681. [Google Scholar] [CrossRef]
- Festugato, L.; Consoli, N.C.; Fourie, A. Cyclic shear behaviour of fibre-reinforced mine tailings. Geosynth. Int. 2015, 22, 196–206. [Google Scholar] [CrossRef]
- Li, L.; Mitchell, R. Effects of reinforcing elements on the behavior of weakly cemented sands. Can. Geotech. J. 1988, 25, 389–395. [Google Scholar] [CrossRef]
- Wei, Z.A.; Yin, G.Z.; Li, G.Z.; Wang, J.G.; Wan, L.; Shen, L.Y. Reinforced terraced fields method for fine tailings disposal. Miner. Eng. 2009, 22, 1053–1059. [Google Scholar] [CrossRef]
- Yin, G.; Wei, Z.; Wang, J.G.; Wan, L.; Shen, L. Interaction characteristics of geosynthetics with fine tailings in pullout test. Geosynth. Int. 2008, 15, 428–436. [Google Scholar] [CrossRef]
- James, M.; Aubertin, M. The use of waste rock inclusions to improve the seismic stability of tailings impoundments. In Proceedings of the GeoCongress 2012: State of the Art and Practice in Geotechnical Engineering, Oakland, CA, USA, 25–29 March 2012; pp. 4166–4175. [Google Scholar] [CrossRef]
- Liu, J.; Bai, Y.X.; Li, D.; Wang, Q.Y.; Qian, W.; Wang, Y.; Debi Prasanna, K.; Wei, J.H. An Experimental Study on the Shear Behaviors of Polymer-Sand Composite Materials after Immersion. Polymers 2018, 10, 924. [Google Scholar] [CrossRef]
- Consoli, N.C.; Nierwinski, H.P.; da Silva, A.P.; Sosnoski, J. Durability and strength of fiber-reinforced compacted gold tailings-cement blends. Geotext. Geomembr. 2017, 45, 98–102. [Google Scholar] [CrossRef]
- Zhu, X.; Huang, X.M. Laboratory simulating test and field settlement observation of reinforced embankment. Chin. J. Geotech. Eng. 2002, 24, 386–388. [Google Scholar] [CrossRef]
- Zhang, H.W.; Zhang, J.H.; Bu, H.L. Discuss the probability formula of bed load transport. South-to-North Water Transf. Water Sci. 2011, 9, 140–145. [Google Scholar] [CrossRef]
- Singh, V.P. Dam Breach Modeling Technology; Kluwer Academic Publisher: Dordrecht, The Netherlands, 1996; Volume 17, pp. 181–200. [Google Scholar]



| Type | Grid Size (mm) | Tensile Strength (kN·m−1) | Elongation at Break (%) | ||
|---|---|---|---|---|---|
| Vertical | Horizontal | Longitudinal | Transverse | ||
| JT1101 | 25 | 25 | 6.5 | 6.5 | 12.7 |
| Index | Values |
|---|---|
| Specific gravity | 2.10 |
| Moisture content (%) | 12.5 |
| Porosity | 0.024 |
| Modulus of compression (MPa) | 17.6 |
| Coefficient of compressibility (MPa−1) | 0.058 |
| Permeability coefficient (×10−6 m·s−1) | 5.86 |
| Cohesion (kPa) | 7.52 |
| Internal friction angle (Φ/°) | 31.2 |
| Index | Values | ||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Time (s) | 0 | 20 | 40 | 60 | 80 | 100 | 120 | 140 | 160 | 180 | 200 | 220 | 240 | 280 | 300 | 320 | |
| Breach Depth (cm) | N = 0 | 0 | 10 | 10 | 12 | 12.5 | 14 | 16 | 18 | 20 | 20 | 22 | 22 | 22 | 22 | 22 | 22 |
| N = 1 | 0 | 6 | 8 | 11 | 11 | 13 | 13 | 15 | 15 | 17 | 17 | 20 | 20 | 20 | 20 | 20 | |
| N = 2 | 0 | 4 | 6 | 7.2 | 8 | 8 | 8 | 8 | 10 | 12 | 14 | 14 | 14 | 14 | 14 | 14 | |
| N = 3 | 0 | 3 | 3 | 3 | 4 | 4 | 6 | 7 | 8 | 8 | 9 | 10 | 10 | 10 | 10 | 10 | |
| N = 4 | 0 | 2 | 2 | 2 | 4 | 4 | 5 | 7 | 7 | 8 | 8 | 10 | 10 | 10 | 10 | 10 | |
| Reinforcement Layers (N) | 0 | 1 | 2 | 3 | 4 |
|---|---|---|---|---|---|
| Ct (×10−4 kg/cm3) | 38.62 | 13.51 | 9.92 | 6.10 | 5.59 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jing, X.; Chen, Y.; Williams, D.J.; Serna, M.L.; Zheng, H. Overtopping Failure of a Reinforced Tailings Dam: Laboratory Investigation and Forecasting Model of Dam Failure. Water 2019, 11, 315. https://doi.org/10.3390/w11020315
Jing X, Chen Y, Williams DJ, Serna ML, Zheng H. Overtopping Failure of a Reinforced Tailings Dam: Laboratory Investigation and Forecasting Model of Dam Failure. Water. 2019; 11(2):315. https://doi.org/10.3390/w11020315
Chicago/Turabian StyleJing, Xiaofei, Yulong Chen, David J. Williams, Marcelo L. Serna, and Hengwei Zheng. 2019. "Overtopping Failure of a Reinforced Tailings Dam: Laboratory Investigation and Forecasting Model of Dam Failure" Water 11, no. 2: 315. https://doi.org/10.3390/w11020315
APA StyleJing, X., Chen, Y., Williams, D. J., Serna, M. L., & Zheng, H. (2019). Overtopping Failure of a Reinforced Tailings Dam: Laboratory Investigation and Forecasting Model of Dam Failure. Water, 11(2), 315. https://doi.org/10.3390/w11020315







