Modeling and Dynamic-Simulating the Water Distribution of a Fixed Spray-Plate Sprinkler on a Lateral-Move Sprinkler Irrigation System
Abstract
:1. Introduction
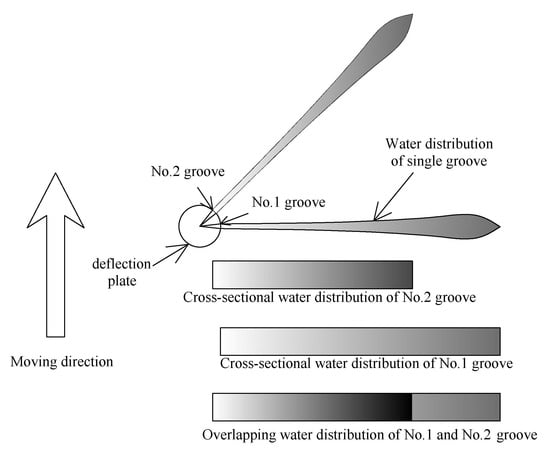
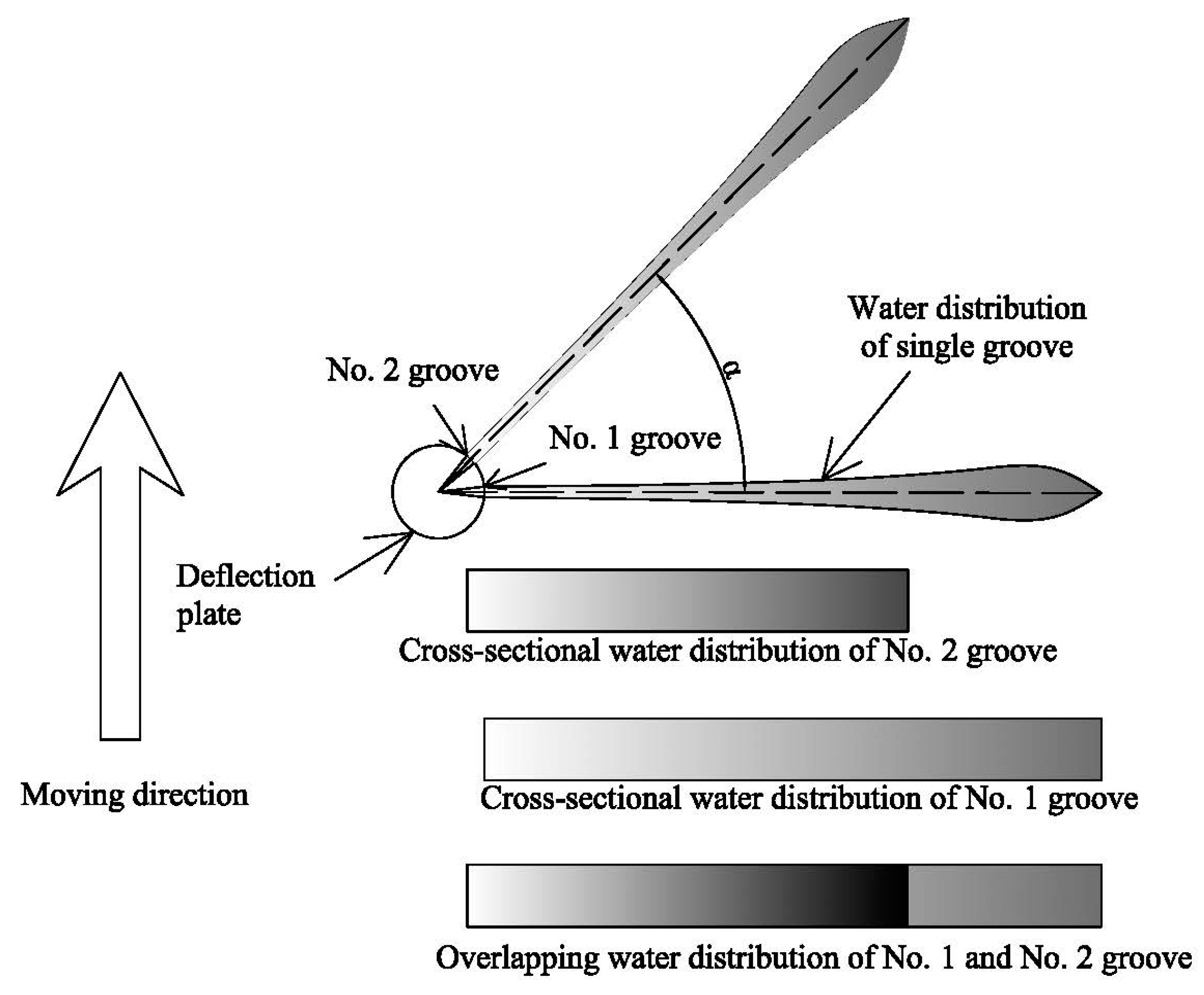
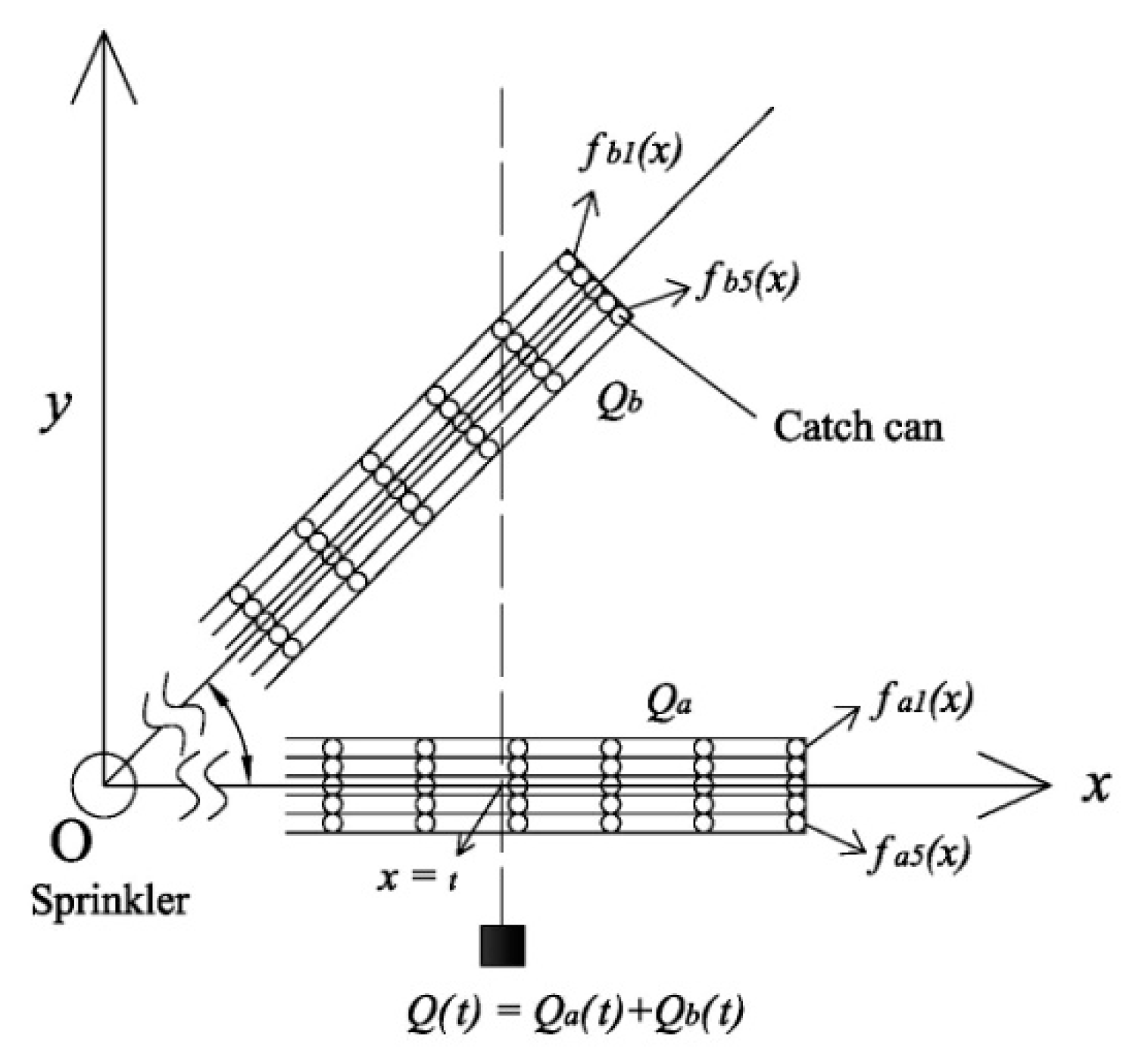
2. Calculation Theory
2.1. Theoretical Basis
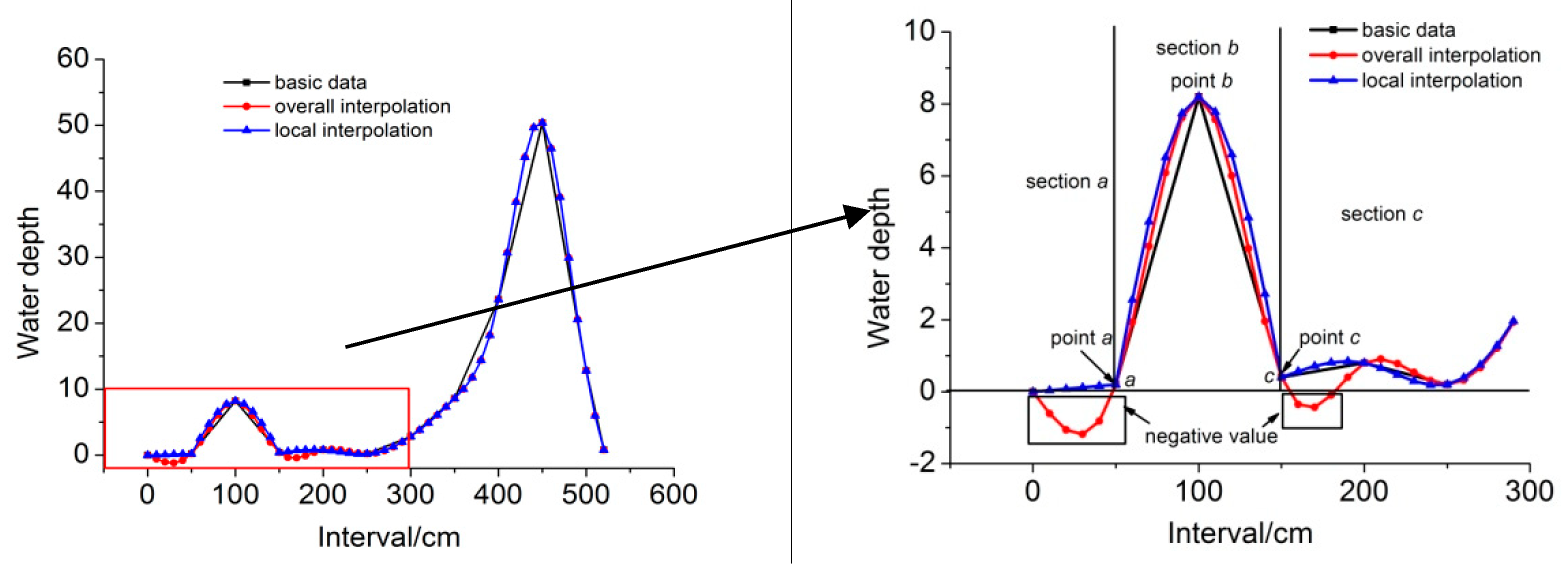
2.2. Software Development
3. Validation and Application of the Theory
3.1. Verification Experiment
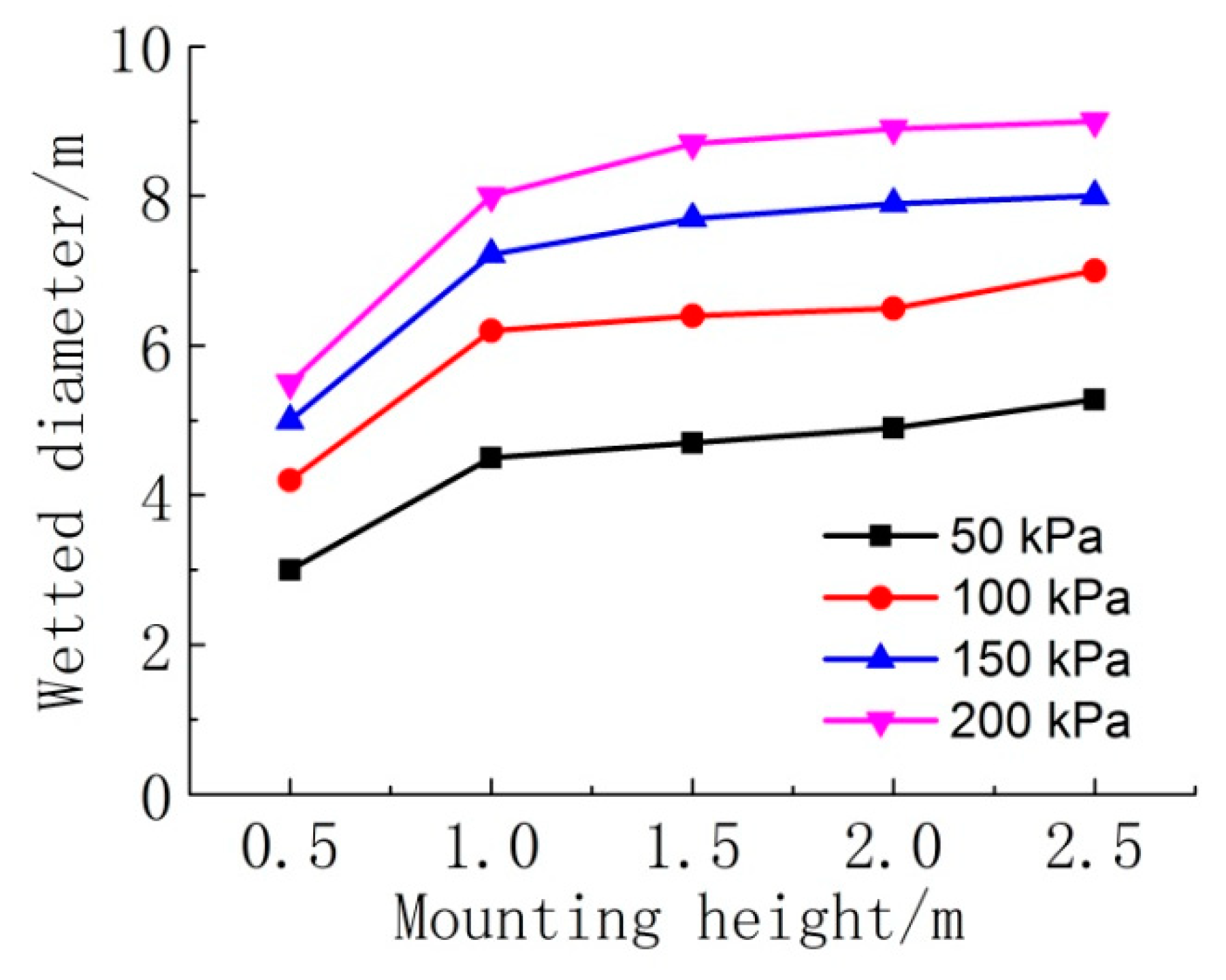
3.2. Application of Theory
4. Results
4.1. Verification of the Theory
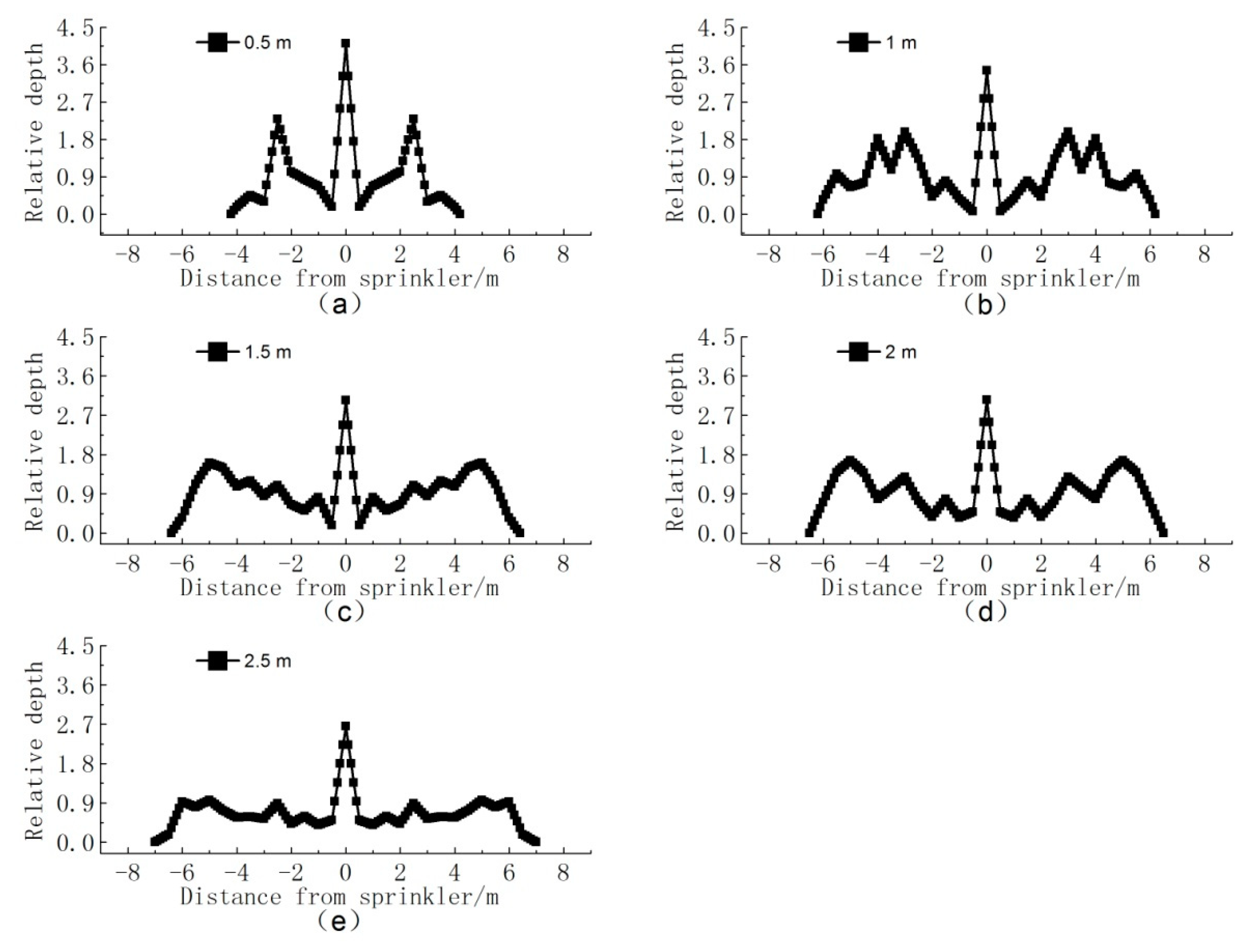
4.2. Calculated Water Distribution Characteristics of Single Sprinkler in Motion
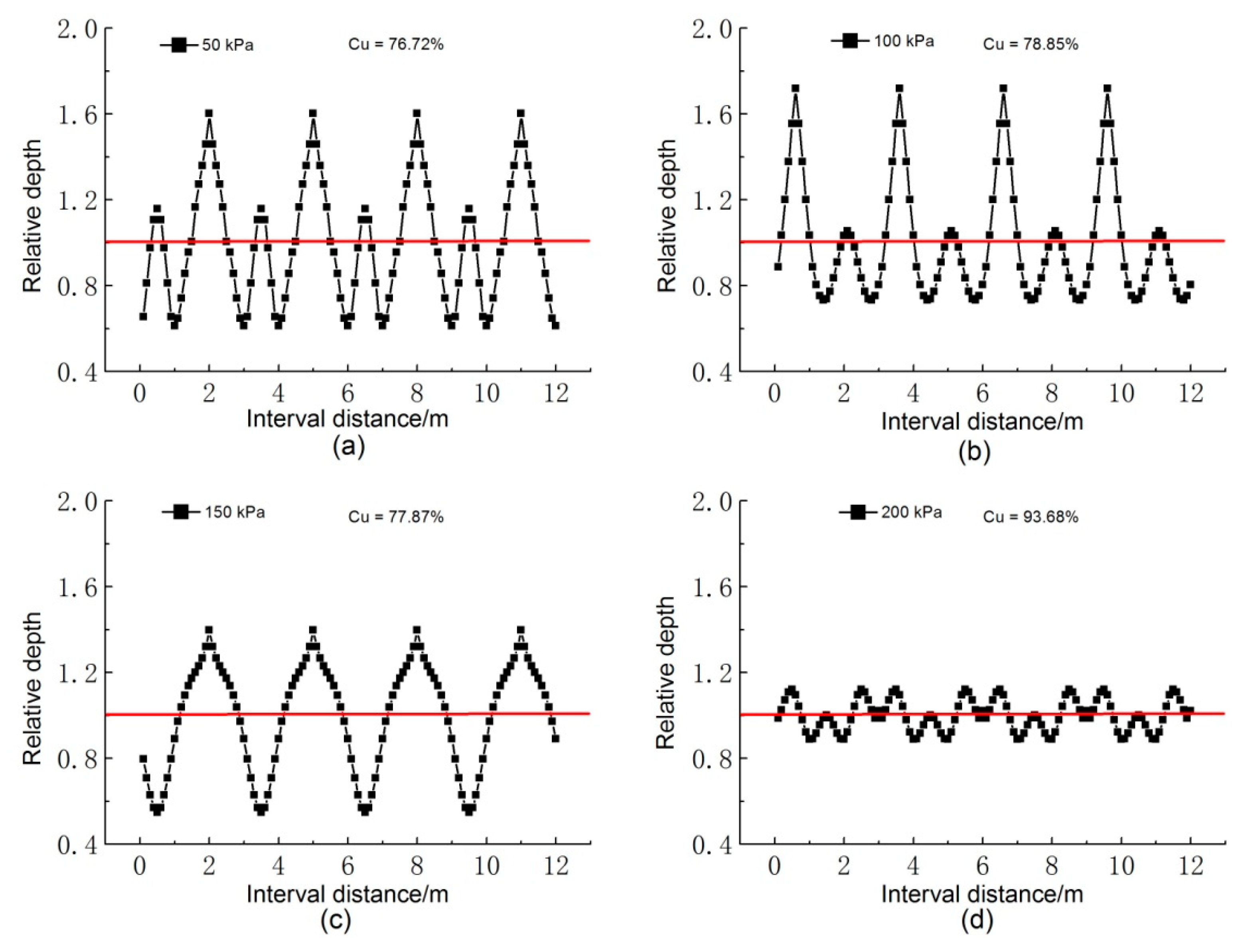
4.3. Christiansen Uniformity
5. Discussion
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Clemmens, A.J.; Dedrick, A.R. Irrigation Techniques and Evaluations Management of Water Use in Agriculture; Springer: Berlin/Heidelberg, Germany, 1994; pp. 64–103. [Google Scholar]
- Kincaid, D.C. Application rates from center pivot irrigation with current sprinkler types. Appl. Eng. Agric. 2005, 21, 605–610. [Google Scholar] [CrossRef]
- Playán, E.; Mateos, L. Modernization and optimization of irrigation systems to increase water productivity. Agric. Water Manag. 2006, 80, 100–116. [Google Scholar] [CrossRef] [Green Version]
- Musick, J.T.; Pringle, F.B.; Walker, J.D. Sprinkler and furrow irrigation trends—Texas High Plains. Appl. Eng. Agric. 1988, 4, 46–52. [Google Scholar] [CrossRef]
- Hanson, B.; Orloff, S. Rotator nozzles more uniform than spray nozzles on center-pivot sprinklers. Calif. Agric. 1996, 50, 32–35. [Google Scholar] [CrossRef]
- Hills, D.J.; Barragan, J. Application uniformity for fixed and rotating spray plate sprinklers. Appl. Eng. Agric. 1998, 14, 33–36. [Google Scholar] [CrossRef]
- Faci, J.M.; Salvador, R.; Playán, E.; Sourell, H. Comparison of fixed and rotating spray plate sprinklers. J. Irrig. Drain. Eng. 2001, 127, 224–233. [Google Scholar] [CrossRef]
- Playán, E.; Garrido, S.; Faci, J.M.; Galan, A. Characterizing pivot sprinklers using an experimental irrigation machine. Agric. Water Manag. 2004, 70, 177–193. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.; Sun, B.; Fang, H.; Zhu, D.; Yang, L.; Li, Z. Experimental and simulation investigation on the kinetic energy dissipation rate of a fixed spray-plate sprinkler. Water 2018, 10, 1365. [Google Scholar] [CrossRef]
- Sayyadi, H.; Nazemi, A.H.; Sadraddini, A.A.; Delirhasannia, R. Characterising droplets and precipitation profiles of a fixed spray-plate sprinkler. Biosyst. Eng. 2014, 119, 13–24. [Google Scholar] [CrossRef]
- Carrión, P.; Tarjuelo, J.; Montero, J. SIRIAS: A simulation model for sprinkler irrigation. Irrig. Sci. 2001, 20, 73–84. [Google Scholar] [CrossRef]
- Playán, E.; Zapata, N.; Faci, J.M.; Tolosa, D. Assessing sprinkler irrigation uniformity using a ballistic simulation model. Agric. Water Manag. 2006, 84, 89–100. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Bai, G.; Yan, H. Development and validation of a modified model to simulate the sprinkler water distribution. Comput. Electron. Agric. 2015, 111, 38–47. [Google Scholar] [CrossRef]
- Ouazaa, S.; Burguete, J.; Paniagua, M.P.; Salvador, R.; Zapata, N. Simulating water distribution patterns for fixed spray plate sprinkler using the ballistic theory. Span. J. Agric Res. 2014, 12, 850–863. [Google Scholar] [CrossRef] [Green Version]
- Christiansen, J.E. Irrigation by sprinkling. University of California Agricultural Experiment Station. Bulletin 1942, 670, 124. [Google Scholar]
- Tarjuelo, J.M.; Montero, J.; Honrubia, F.T.; Ortiz, J.J.; Ortega, J.F. Analysis of uniformity of sprinkle irrigation in a semi-arid area. Agric. Water Manag. 1999, 40, 315–331. [Google Scholar] [CrossRef]
- Louie, M.J.; Selker, J.S. Sprinkler head maintenance effects on water application uniformity. J. Irrig. Drain. Eng. 2000, 126, 142–148. [Google Scholar] [CrossRef]
- Silva, L.L. The effect of spray head sprinklers with different deflector plates on irrigation uniformity, runoff and sediment yield in a Mediterranean soil. Agric. Water Manag. 2006, 85, 243–252. [Google Scholar] [CrossRef]
- Marjang, N.; Merkley, G.P.; Shaban, M. Center-pivot uniformity analysis with variable container spacing. Irrig. Sci. 2012, 30, 149–156. [Google Scholar] [CrossRef]
- Dogan, E.; Kirnak, H.; Dogan, Z. Effect of varying the distance of collectors below a sprinkler head and travel speed on measurements of mean water depth and uniformity for a linear move irrigation sprinkler system. Biosyst. Eng. 2008, 99, 190–195. [Google Scholar] [CrossRef]
- Faria, L.C.; Nörenberg, B.G.; Colombo, A.; Dukes, M.D.; Timm, L.C.; Beskow, S.; Caldeira, T.L. Irrigation distribution uniformity analysis on a lateral-move irrigation system. Irrig. Sci. 2019, 37, 195–206. [Google Scholar] [CrossRef]
- Mateos, L. Assessing whole-field uniformity of stationary sprinkler irrigation systems. Irrig. Sci. 1998, 18, 73–81. [Google Scholar] [CrossRef]
- Zhang, L.; Merkley, G.P.; Pinthong, K. Assessing whole-field sprinkler irrigation application uniformity. Irrig. Sci. 2013, 31, 87–105. [Google Scholar] [CrossRef]
- Zhang, L.; Hui, X.; Chen, J. Effects of terrain slope on water distribution and application uniformity for sprinkler irrigation. Int. J. Agric. Biol. Eng. 2018, 11, 120–125. [Google Scholar] [CrossRef]
- Fukui, Y.; Nakanishi, K.; Okamura, S. Computer evaluation of sprinkler irrigation uniformity. Irrig. Sci. 1980, 2, 23–32. [Google Scholar] [CrossRef]
- Omary, M.; Sumner, H. Modeling water distribution for irrigation machine with small spray nozzles. J. Irrig. Drain. Eng. 2001, 127, 156–160. [Google Scholar] [CrossRef]
- Clark, G.A.; Srinivas, K.; Rogers, D.H.; Stratton, R. Measured and simulated uniformity of low drift nozzle sprinklers. Trans. ASAE 2003, 46, 321. [Google Scholar] [CrossRef]
- Gat, Y.L.; Molle, B. Model of water application under pivot sprinkler. I: Theoretical grounds. J. Irrig. Drain. Eng. 2000, 126, 343–347. [Google Scholar] [CrossRef]
- Liu, J.; Zhu, X.; Yuan, S.; Fordjour, A. Modeling the application depth and water distribution uniformity of a linearly moved irrigation system. Water 2019, 11, 827. [Google Scholar] [CrossRef]
- Ge, M.S.; Wu, P.; Zhu, D.; Ames, D.P. Comparison between sprinkler irrigation and natural rainfall based on droplet diameter. Span. J. Agric. Res. 2016, 14, 1201. [Google Scholar] [CrossRef]
- McKinley, S.; Levine, M. Cubic spline interpolation. College Redwoods 1998, 45, 1049–1060. [Google Scholar]
- Sarfraz, M.; Butt, S.; Hussain, M.Z. Visualization of shaped data by a rational cubic spline interpolation. Comput. Graph. 2001, 25, 833–845. [Google Scholar] [CrossRef]
- Yan, H.J.; Jin, H.Z.; Qian, Y.C. Characterizing center pivot irrigation with fixed spray plate sprinklers. Sci. China Technol. Sci. 2010, 53, 1398–1405. [Google Scholar] [CrossRef]
- Vories, E.D.; Von Bernuth, R.D. Single nozzle sprinkler performance in wind. Trans. ASAE 1986, 29, 1325–1330. [Google Scholar] [CrossRef]
- Seginer, I.; Kantz, D.; Nir, D. The distortion by wind of the distribution patterns of single sprinklers. Agric. Water Manag. 1991, 19, 341–359. [Google Scholar] [CrossRef]

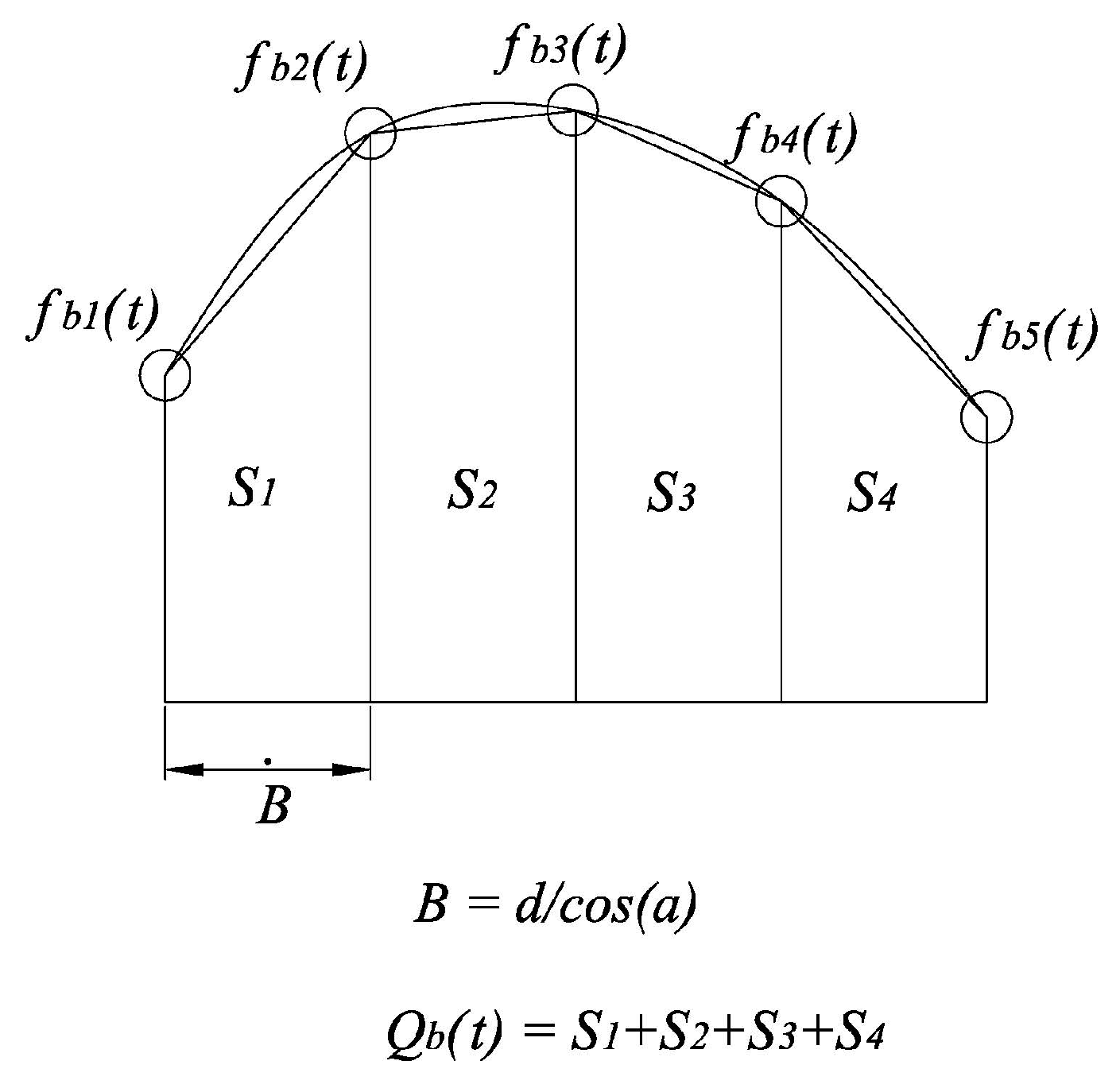

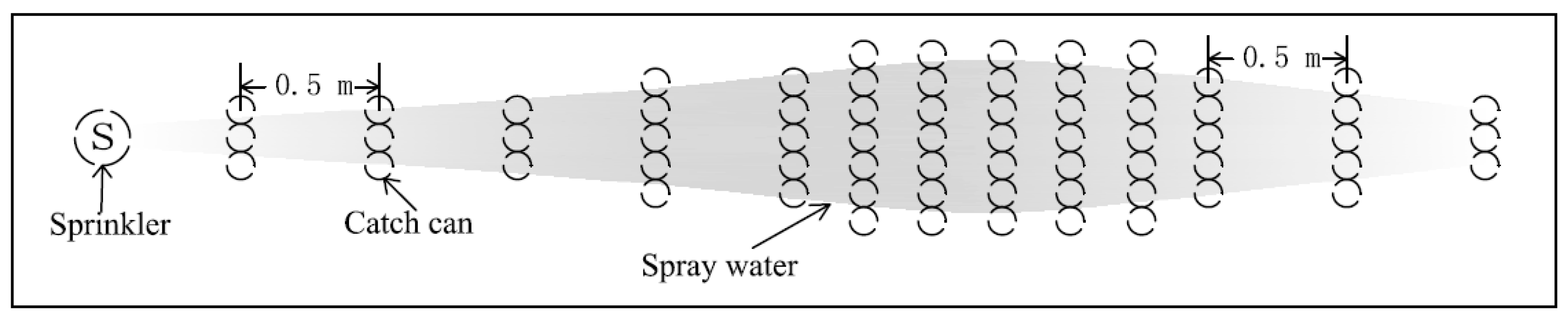

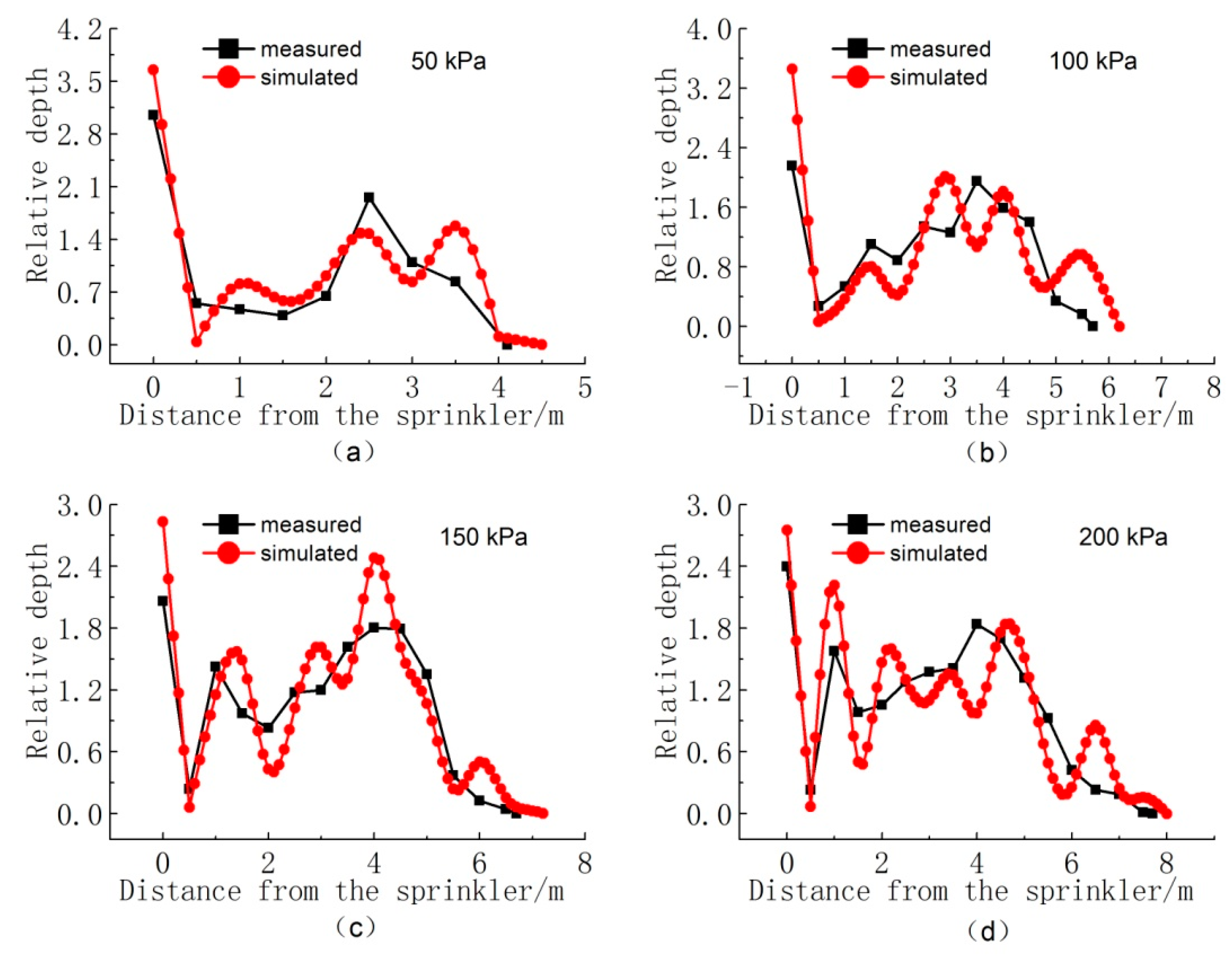
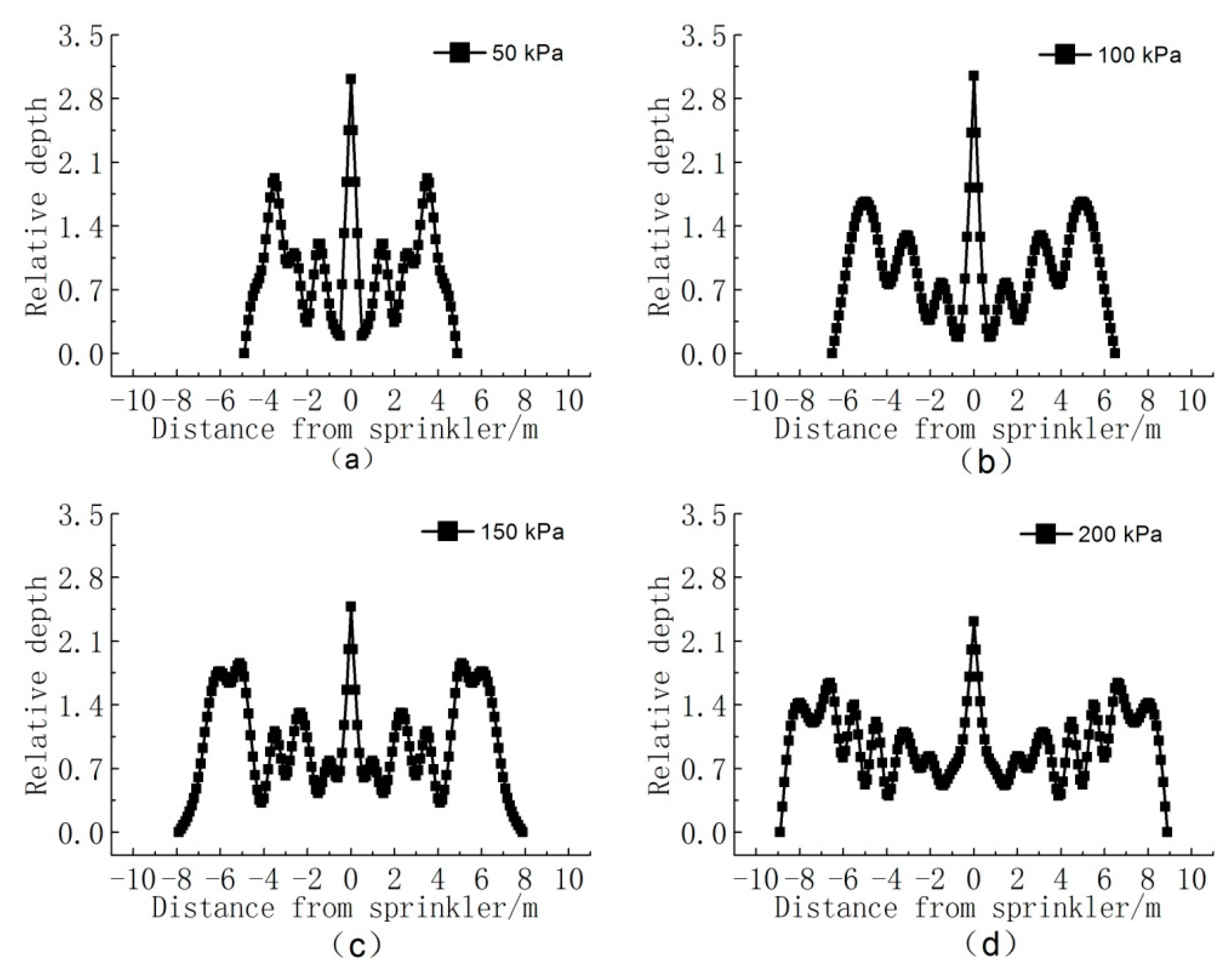

| Row | X-Coordinate Value | Y-Coordinate Value |
|---|---|---|
| 1 | m × L | |
| 2 | m × L | |
| 3 | m × L | |
| n | m × L |
| Distance from Sprinkler (m) | Application Rate (mm·h−1) | ||||
|---|---|---|---|---|---|
| Row 1 | Row 2 | Row 3 | Row 4 | Row 5 | |
| 0 | 0 | 0 | 0 | 0 | 0 |
| 0.5 | 0 | 0.8 | 10.8 | 0.8 | 0 |
| 1 | 0 | 0.8 | 8.8 | 0.7 | 0 |
| 2 | 0 | 1.2 | 9.4 | 0.9 | 0 |
| 3 | 0 | 0.8 | 10.4 | 0.8 | 0 |
| 3.5 | 0 | 0.8 | 9.8 | 0.6 | 0 |
| 4 | 0 | 1.2 | 13.6 | 1.4 | 0 |
| 4.5 | 0 | 1.6 | 10.4 | 1.8 | 0 |
| 5 | 0 | 0.2 | 10.8 | 0.4 | 0 |
| 5.2 | 0 | 0.2 | 0.2 | 0.2 | 0 |
| Level | Factors | ||
|---|---|---|---|
| Working Pressure (kPa) | Sprinkler Spacing (m) | Mounting Height (m) | |
| 1 | 50 | 2 | 0.5 |
| 2 | 100 | 3 | 1 |
| 3 | 150 | 4 | 1.5 |
| 4 | 200 | _ | 2 |
| 5 | _ | _ | 2.5 |
| Working Pressure (kPa) | Jet Distance (m) | ||
|---|---|---|---|
| Measured | Calculated | Difference | |
| 50 | 4.0 | 4.5 | −12.50% |
| 100 | 5.7 | 6.2 | −8.77% |
| 150 | 6.7 | 7.22 | −7.76% |
| 200 | 7.7 | 8 | −3.90% |
| Factors | Mean Square | F | Sig | Significance |
|---|---|---|---|---|
| Mounting height | 945.522 | 10.685 | 0.000 | *** |
| Sprinkler spacings | 308.043 | 3.466 | 0.039 | ** |
| Working pressure | 1379.039 | 15.519 | 0.000 | *** |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Guo, J.; Sun, B.; Fang, H.; Zhu, D.; Wang, H. Modeling and Dynamic-Simulating the Water Distribution of a Fixed Spray-Plate Sprinkler on a Lateral-Move Sprinkler Irrigation System. Water 2019, 11, 2296. https://doi.org/10.3390/w11112296
Zhang Y, Guo J, Sun B, Fang H, Zhu D, Wang H. Modeling and Dynamic-Simulating the Water Distribution of a Fixed Spray-Plate Sprinkler on a Lateral-Move Sprinkler Irrigation System. Water. 2019; 11(11):2296. https://doi.org/10.3390/w11112296
Chicago/Turabian StyleZhang, Yisheng, Jinjun Guo, Bin Sun, Hongyuan Fang, Delan Zhu, and Huiliang Wang. 2019. "Modeling and Dynamic-Simulating the Water Distribution of a Fixed Spray-Plate Sprinkler on a Lateral-Move Sprinkler Irrigation System" Water 11, no. 11: 2296. https://doi.org/10.3390/w11112296
APA StyleZhang, Y., Guo, J., Sun, B., Fang, H., Zhu, D., & Wang, H. (2019). Modeling and Dynamic-Simulating the Water Distribution of a Fixed Spray-Plate Sprinkler on a Lateral-Move Sprinkler Irrigation System. Water, 11(11), 2296. https://doi.org/10.3390/w11112296