The Effect of Landscape Interventions on Groundwater Flow and Surface Runoff in a Watershed in the Upper Reaches of the Blue Nile
Abstract
1. Introduction
2. Materials and Methods
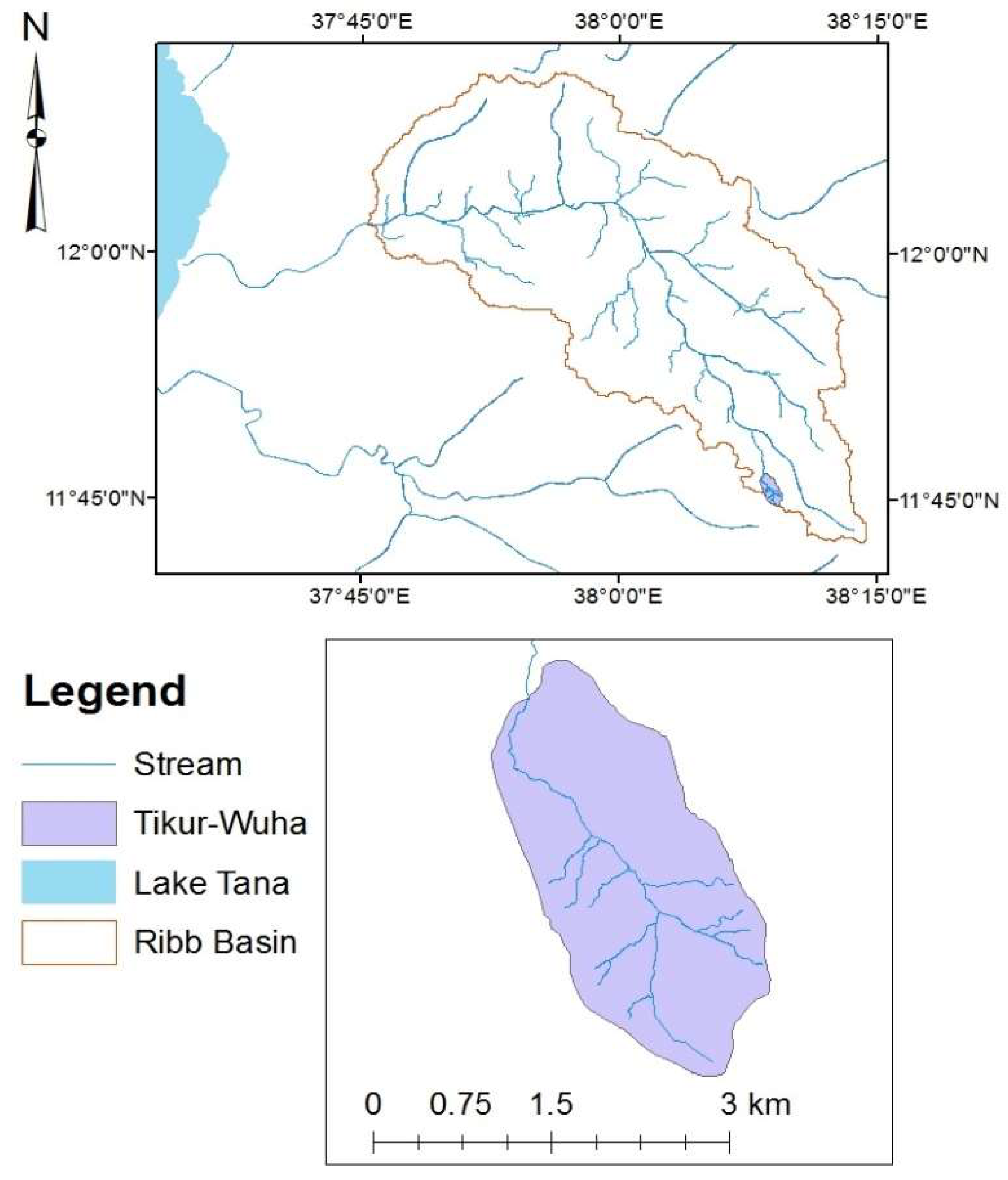
2.1. Description of the Study Area
2.2. Data Collection
2.3. Methods
2.3.1. Groundwater Flow Separation
2.3.2. Hydrological Modeling for Groundwater Flow Separation
3. Results
3.1. Preciptation
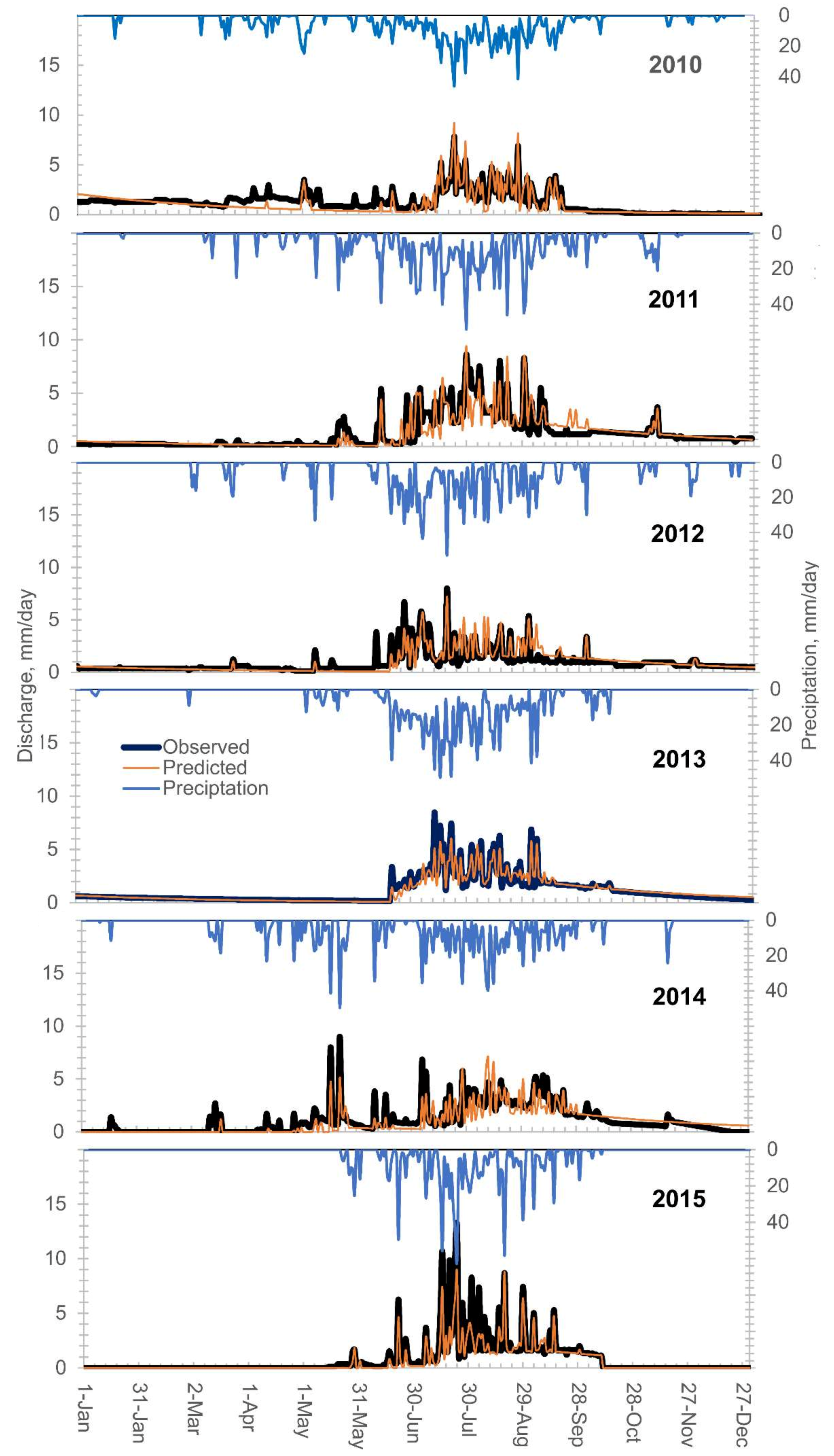
3.2. Discharge at the Outlet
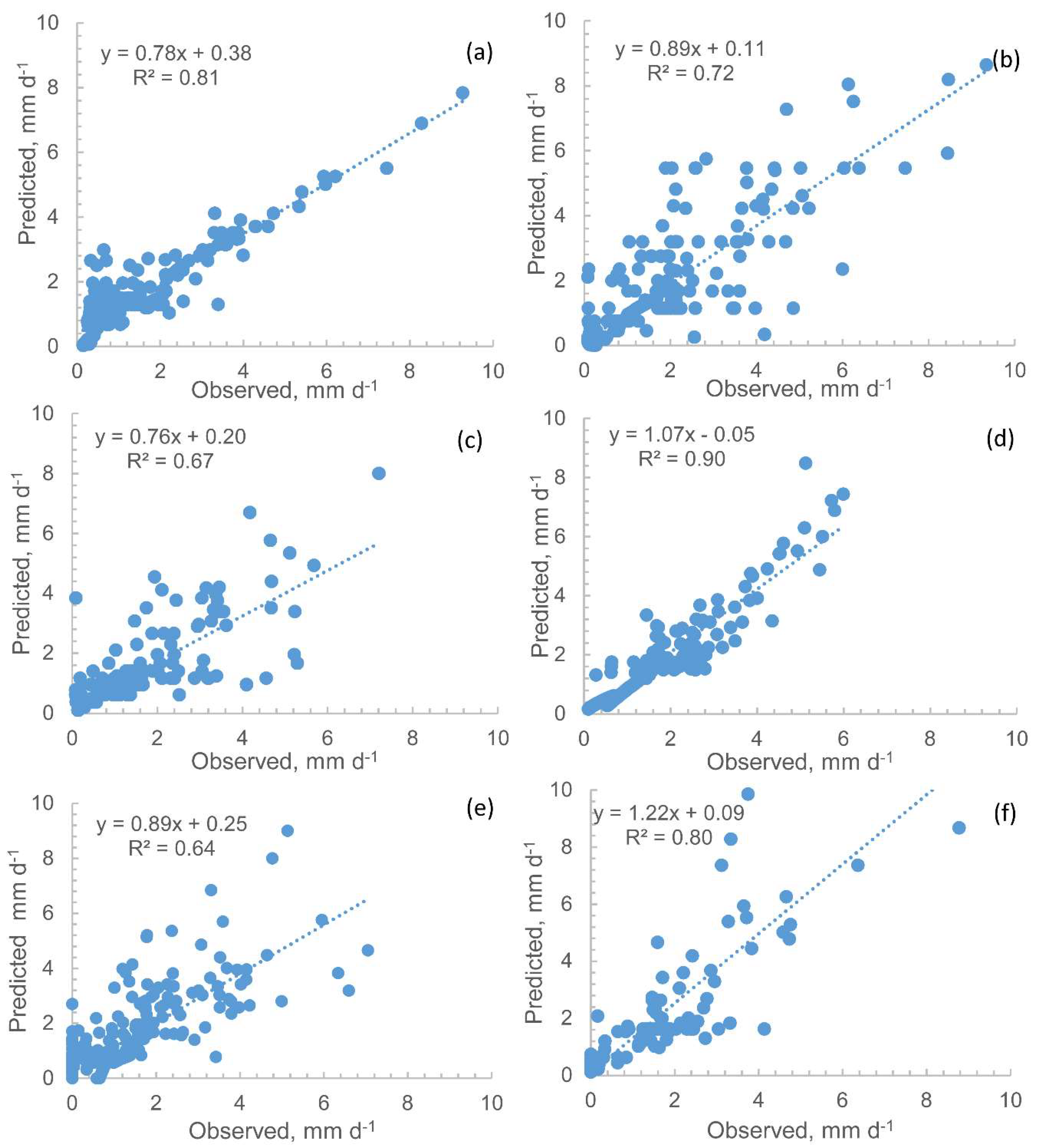
3.3. Assessing Changes in the Hydrology Watershed with the Parameter Efficient Distributed Model
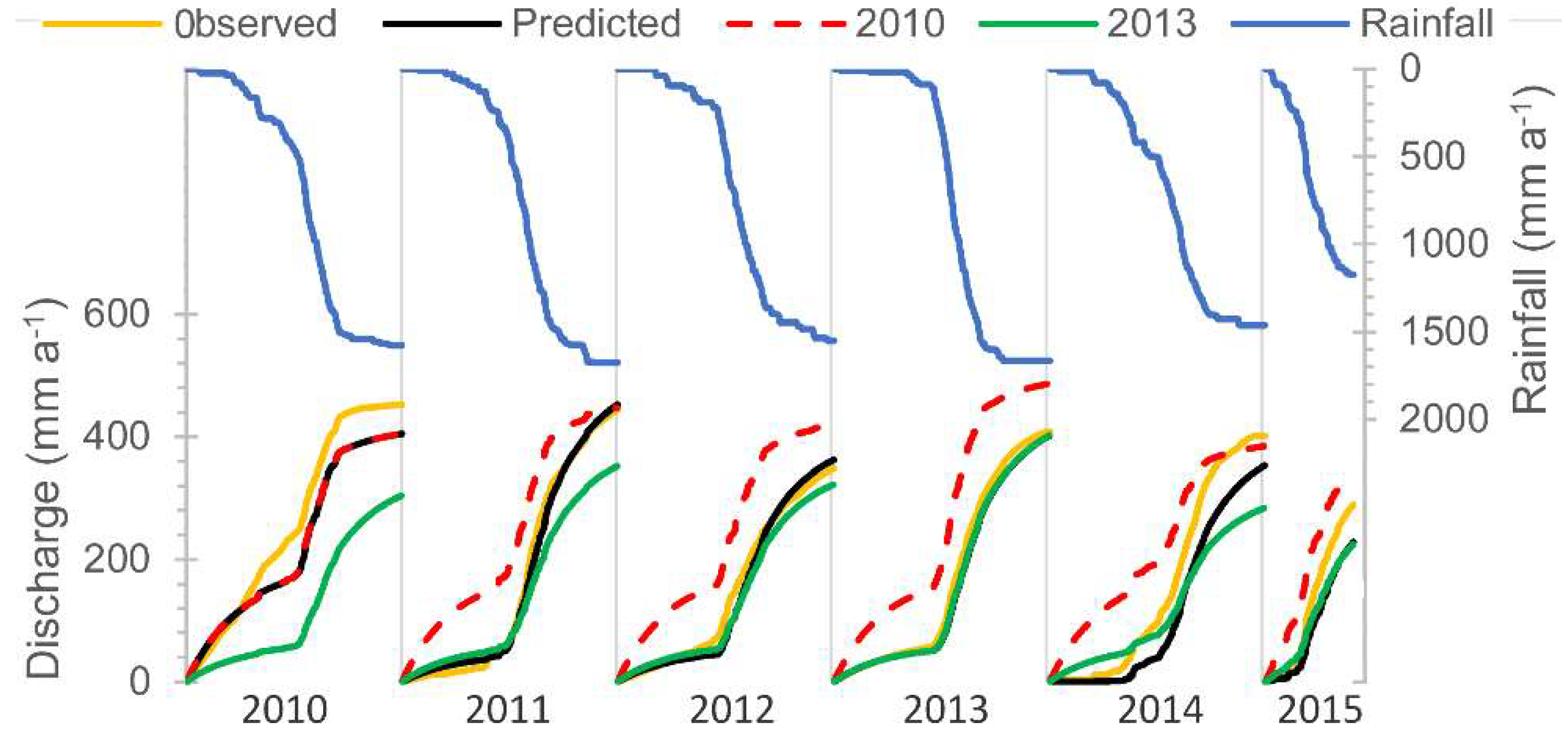
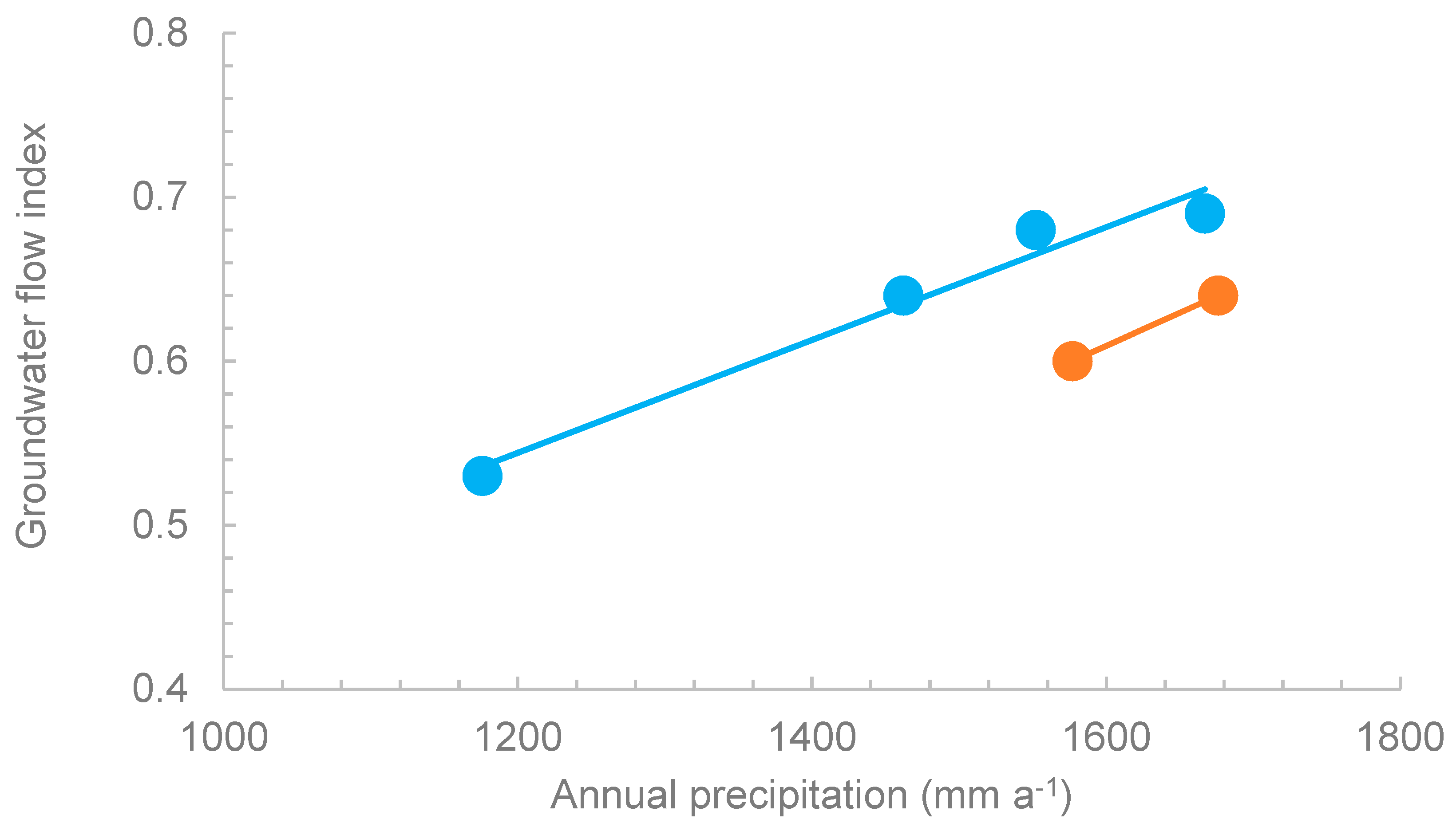
3.4. Groundwater Flow Index
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Kaur, J. Involvement of Women in the Management of Selected Natural Resources in Kandi Area of Punjab; Diss. Punjab Agricultural University: Ludhiana, India, 2016. [Google Scholar]
- Xing, M.A. Response of hydrological processes to land-cover and climate changes in Kejie watershed, south-west China. Hydrol. Proc. Int. J. 2009, 23, 1179–1191. [Google Scholar] [CrossRef]
- Schilling, K.E.; Libra, R.D. Increased baseflow in Iowa over the second half of the 20th century. JAWRA J. Am. Water Resour. Assoc. 2003, 39, 851–860. [Google Scholar] [CrossRef]
- Bruijnzeel, L.A. Hydrological functions of tropical forests: Not seeing the soil for the trees? Agric. Ecosyst. Environ. 2004, 104, 185–228. [Google Scholar] [CrossRef]
- Line, D.E.; White, N.M. Effects of development on runoff and pollutant export. Water Environ. Res. 2007, 79, 185–190. [Google Scholar] [CrossRef] [PubMed]
- Worqlul, A.W.; Collick, A.S.; Rossiter, D.G.; Langan, S.; Steenhuis, T.S. Assessment of surface water irrigation potential in the Ethiopian highlands: The Lake Tana Basin. Catena 2015, 129, 76–85. [Google Scholar] [CrossRef]
- Brodie, R.S.; Hostetler, S.; Slatter, E. Comparison of daily percentiles of streamflow and rainfall to investigate stream–aquifer connectivity. J. Hydrol. 2008, 349, 56–67. [Google Scholar] [CrossRef]
- Welderufael, W.A.; Woyessa, Y.E. Stream flow analysis and comparison of methods for base flow separation: Case study of the Modder River basin in central South Africa. Interim Interdiscip. J. 2009, 8, 107–119. [Google Scholar]
- Eckhardt, K. A comparison of baseflow indices, which were calculated with seven different baseflow separation methods. J. Hydrol. 2008, 352, 168–173. [Google Scholar] [CrossRef]
- Wittenberg, H. Effects of season and man-made changes on baseflow and flow recession: Case studies. Hydrol. Proc. 2003, 17, 2113–2123. [Google Scholar] [CrossRef]
- Liu, D.; Chang, J.; Tian, F.; Huang, Q.; Meng, X. Analysis of baseflow index based hydrological model in Upper Wei River basin on the Loess Plateau in China. Remote Sens. GIS Hydrol. Water Resour. 2015, 368, 403–408. [Google Scholar] [CrossRef][Green Version]
- Miller, M.P.; Buto, S.G.; Susong, D.D.; Rumsey, C.A. The importance of base flow in sustaining surface water flow in the Upper Colorado River Basin. Water Resour. 2016, 52, 3547–3562. [Google Scholar] [CrossRef]
- Gonzales, A.L.; Nonner, J.; Heijkers, J.; Uhlenbrook, S. Comparison of different base flow separation methods in a lowland catchment. Hydrol. Earth Syst. Sci. 2009, 13, 2055–2068. [Google Scholar] [CrossRef]
- Abiy, A.Z.; Demissie, S.S.; MacAlister, C.; Dessu, S.B.; Melesse, A.M. Groundwater Recharge and Contribution to the Tana Sub-basin, Upper Blue Nile Basin, Ethiopia. In Landscape Dynamics, Soils and Hydrological Processes in Varied Climates; Springer: Cham, Switzerland, 2016; pp. 463–481. [Google Scholar] [CrossRef]
- Rittenburg, R.A.; Squires, A.L.; Boll, J.; Brooks, E.S.; Easton, Z.M.; Seenhuis, T.S. Agricultural BMP Effectiveness and Dominant Hydrological Flow Paths: Concepts and a Review. JAWRA J. Am. Water Resour. Assoc. 2015, 51, 305–329. [Google Scholar] [CrossRef]
- Zhang, Y.K.; Schilling, K.E. Increasing streamflow and baseflow in Mississippi River since the 1940s: Effect of land use change. J. Hydrol. 2006, 324, 412–422. [Google Scholar] [CrossRef]
- Dagnew, C.D.; Guzman, C.D.; Zegeye, A.D.; Tebebu, T.Y.; Getaneh, M.; Abate, S.; Zimale, F.A.; Ayana, E.K.; Tilahun, S.A.; Steenhuis, T.S. Impact of conservation practices on runoff and soil loss in the sub-humid Ethiopian Highlands: The Debre Mawi watershed. J. Hydrol. Hydromech. 2015, 63, 210–219. [Google Scholar] [CrossRef]
- Potter, K.W. Hydrological impacts of changing land management practices in a moderate-sized agricultural catchment. Water Resour. Res. 1991, 27, 845–855. [Google Scholar] [CrossRef]
- Juckem, P.F.; Hunt, R.J.; Anderson, M.P.; Robertson, D.M. Effects of climate and land management change on streamflow in the driftless area of Wisconsin. J. Hydrol. 2008, 355, 123–130. [Google Scholar] [CrossRef]
- Price, K.; Jackson, C.R. Effects of forest conversion on baseflow in the southern Appalachians: A cross-landscape comparison of synoptic measurements. In Proceedings of the Georgia Water Resources Conference, Athens, Greece, 27–29 March 2007. [Google Scholar]
- Schilling, K.E.; Jha, M.K.; Zhang, Y.-K.; Gassman, P.W.; Wolter, C.F. Impact of land use and land cover change on the water balance of a large agricultural watershed: Historical effects and future directions. J. Water Resour. 2008, 44. [Google Scholar] [CrossRef]
- Huang, X.D.; Shi, Z.H.; Fang, N.F.; Li, X. Influences of land use change on baseflow in mountainous watersheds. Forests 2016, 7, 16. [Google Scholar] [CrossRef]
- Abate, M.; Nyssen, J.; Moges, M.M.; Enku, T.; Zimale, F.A.; Tilahun, S.A.; Adgo, E.; Steenhuis, T.S. Long-term landscape changes in the lake tana basin as evidenced by delta development and floodplain aggradation, Ethiopia. Land Degrad. Devel. 2016, 28, 1820–1830. [Google Scholar] [CrossRef]
- Tebebu, T.Y.; Bayabil, H.K.; Stoof, C.R.; Giri, S.K.; Gessess, A.A.; Tilahun, S.A.; Steenhuis, T.S. Characterization of degraded soils in the humid Ethiopian highlands. Land Degrad. Devel. 2017, 28, 1891–1901. [Google Scholar] [CrossRef]
- Fenta, A.A.; Yasuda, H.; Shimizu, K.; Haregeweyn, N. Response of streamflow to climate variability and changes in human activities in the semiarid highlands of northern Ethiopia. Reg. Environ. Chang. 2017, 17, 1229–1240. [Google Scholar] [CrossRef]
- Lanckriet, S.; Araya, T.; Cornelis, W.; Verfaillie, E.; Poesen, J.; Govaerts, B.; Bauer, H.; Deckers, J.; Haile, M.; Nyssen, J. Impact of conservation agriculture on catchment runoff and soil loss under changing climate conditions in May Zeg-zeg (Ethiopia). J. Hydrol. 2012, 475, 336–349. [Google Scholar] [CrossRef]
- Tebebu, T.Y.; Steenhuis, T.S.; Dagnew, D.C.; Guzman, C.D.; Bayabil, H.K.; Zegeye, A.D.; Collick, A.S.; Langan, S.; MacAlister, C.; Langendoen, E.J.; et al. Improving efficacy of landscape interventions in the (sub) humid Ethiopian highlands by improved understanding of runoff processes. Front. Earth Sci. 2015, 3, 49. [Google Scholar] [CrossRef]
- Gebreegziabher, T.; Nyssen, J.; Govaerts, B.; Getnet, F.; Behailu, M.; Haile, M.; Deckers, J. Contour furrows for in situ soil and water conservation, Tigray, Northern Ethiopia. Soil Tillage Res. 2009, 103, 257–264. [Google Scholar] [CrossRef]
- Akale, A.; Dagnew, D.; Belete, M.; Tilahun, S.; Mekuria, W.; Steenhuis, T. Impact of soil depth and topography on the effectiveness of conservation practices on discharge and soil loss in the Ethiopian highlands. Land 2017, 6, 78. [Google Scholar] [CrossRef]
- Guzman, C.D.; Zimale, F.A.; Tebebu, T.Y.; Bayabil, H.K.; Tilahun, S.A.; Yitaferu, B.; Rientjes, T.H.; Steenhuis, T.S. Modeling discharge and sediment concentrations after landscape interventions in a humid monsoon climate: The Anjeni watershed in the highlands of Ethiopia. Hydrol. Proc. 2017, 31, 1239–1257. [Google Scholar] [CrossRef]
- Melaku, N.D.; Renschler, C.S.; Flagler, J.; Bayu, W.; Klik, A. Integrated impact assessment of soil and water conservation structures on runoff and sediment yield through measurements and modeling in the Northern Ethiopian highlands. Catena 2018, 169, 140–150. [Google Scholar] [CrossRef]
- Ayele, G.K.; Addisie, M.B.; Langendoen, E.J.; Tegegne, N.H.; Tilahun, S.A.; Moges, M.A.; Nicholson, C.F.; Steenhuis, T.S. Evaluating erosion control practices in an actively gullying watershed in the highlands of Ethiopia. Earth Surf. Proc. Landf. 2018, 43, 2835–2843. [Google Scholar] [CrossRef]
- Adgo, E.; Teshome, A.; Mati, B. Impacts of long-term soil and water conservation on agricultural productivity: The case of Anjenie watershed, Ethiopia. Agric. Water Manag. 2013, 117, 55–61. [Google Scholar] [CrossRef]
- Adimassu, Z.; Langan, S.; Johnston, R.; Mekuria, W.; Amede, T. Impacts of Soil and Water Conservation Practices on Crop Yield, Run-off, Soil Loss and Nutrient Loss in Ethiopia: Review and Synthesis. Environ. Manag. 2017, 59, 87–101. [Google Scholar] [CrossRef] [PubMed]
- Mhiret, D.A.; Dagnew, D.C.; Guzman, C.D.; Alemie, T.C.; Zegeye, A.D.; Tebebu, T.Y.; Langendoen, E.; Zaitchik, B.F.; Tilahun, S.A.; Steenhuis, T.S. The Debre Mawi Watershed: A nine-year study on the benefits and risks of soil and water conservation practices in the humid highlands of Ethiopia. [submitted].
- Mhiret, D.A.; Dagnew, D.C.; Tilahun, S.A.; Zaitchik, B.F.; Steenhuis, T.S. Impact of conservation practices, gullies, and eucalyptus trees on discharge and sediment loss in a degraded watershed in the humid Ethiopian highlands. [submitted].
- Enku, T.; Melesse, A.; Ayana, E.; Tilahun, S.; Abate, M.; Steenhuis, T. Response of Groundwater table to Eucalyptus Plantations in a Tropical Monsoon Climate, Lake Tana Basin, Ethiopia. In Proceedings of the EGU General Assembly Conference Abstracts, Vienna, Austria, 23–28 April 2017; Volume 19, p. 4652. [Google Scholar]
- Haregeweyn, N.; Tsunekawa, A.; Tsubo, M.; Meshesha, D.; Adgo, E.; Poesen, J.; Schütt, B. Analyzing the hydrologic effects of region-wide land and water development interventions: A case study of the Upper Blue Nile basin. Reg. Environ. Change 2016, 16, 951–966. [Google Scholar] [CrossRef]
- Addisie, M.B.; Ayele, G.K.; Gessess, A.A.; Tilahun, S.A.; Zegeye, A.D.; Moges, M.M.; Schmitter, P.; Langendoen, E.J.; Steenhuis, T.S. Gully head retreat in the sub-humid Ethiopian highlands: The Ene-Chilala catchment. Land Degrad. Dev. 2017, 28, 1579–1588. [Google Scholar] [CrossRef]
- Lemann, T.; Zeleke, G.; Amsler, C.; Giovanoli, L.; Suter, H.; Roth, V. Modelling the effect of soil and water conservation on discharge and sediment yield in the upper Blue Nile basin, Ethiopia. Appl. Geogr. 2016, 73, 89–101. [Google Scholar] [CrossRef]
- Betrie, G.D.; Mohamed, Y.A.; van Griensven, A.; Srinivasan, R. Sediment management modelling in the Blue Nile Basin using SWAT model. Hydrol. Earth Syst. Sci. 2011, 15, 807. [Google Scholar] [CrossRef]
- Akale, A.T.; Dagnew, D.C.; Giri, S.; Belete, M.A.; Tilahun, S.A.; Mekuria, W.; Steenhuis, T.S. Groundwater quality in an upland agricultural watershed in the sub-humid Ethiopian highlands. J. Water Resour. Prot. 2017, 9, 1199. [Google Scholar] [CrossRef]
- Kebede, S.; Travi, Y.; Alemayehu, T.; Ayenew, T. Groundwater recharge, circulation and geochemical evolution in the source region of the Blue Nile River, Ethiopia. Appl. Geochem. 2005, 20, 1658–1676. [Google Scholar] [CrossRef]
- Amhara Design and Supervision Works Enterprise (ADSWE). Soil Map of Amhara Region, Land Use and Administration Work Process; Amhara Design and Supervision Works Enterprise (ADSWE): Bahir Dar, Ethiopia, 2012. [Google Scholar]
- Partington, D.; Brunner, P.; Simmons, C.T.; Werner, A.D.; Therrien, R.; Maier, H.R.; Dandy, G.C. Evaluation of outputs from automated baseflow separation methods against simulated baseflow from a physically based, surface water-groundwater flow model. J. Hydrol. 2012, 458, 28–39. [Google Scholar] [CrossRef]
- Zhang, R.; Li, Q.; Chow, T.L.; Li, S.; Danielescu, S. Baseflow separation in a small watershed in New Brunswick, Canada, using a recursive digital filter calibrated with the conductivity mass balance method. Hydrol. Proc. 2012, 27, 259–2665. [Google Scholar] [CrossRef]
- Ladouche, B.; Probst, A.; Viville, D.; Idir, S.; Baqué, D.; Loubet, M.; Probst, J.L.; Bariac, T. Hydrograph separation using isotopic, chemical and hydrological approaches (Strengbach catchment, France). J. Hydrol. 2001, 242, 255–274. [Google Scholar] [CrossRef]
- Joerin, C.; Beven, K.J.; Iorgulescu, I.; Musy, A. Uncertainty in hydrograph separations based on geochemical mixing models. J. Hydrol. 2002, 255, 90–106. [Google Scholar] [CrossRef]
- Arnold, J.G.; Muttiah, R.S.; Srinivasan, R.; Allen, P.M. Regional estimation of base flow and groundwater recharge in the Upper Mississippi river basin. J. Hydrol. 2000, 227, 21–40. [Google Scholar] [CrossRef]
- Ahmad, S.; Simonovic, S.P. An artificial neural network model for generating hydrograph from hydro-meteorological parameters. J. Hydrol. 2005, 315, 236–251. [Google Scholar] [CrossRef]
- Beven, K.; Binley, A. The future of distributed models: Model calibration and uncertainty prediction. Hydrol. Proc. 1992, 6, 279–298. [Google Scholar] [CrossRef]
- Tilahun, S.A.; Guzman, C.D.; Zegeye, A.D.; Engda, T.A.; Collick, A.S.; Rimmer, A.; Steenhuis, T.S. An efficient semi-distributed hillslope erosion model for the sub-humid Ethiopian Highlands. Hydrol. Earth Syst. Sci. 2013, 17, 1051–1063. [Google Scholar] [CrossRef]
- Moges, M.A.; Schmitter, P.; Tilahun, S.A.; Langan, S.; Dagnew, D.C.; Akale, A.T.; Steenhuis, T.S. Suitability of watershed models to predict distributed hydrologic response in the Awramba watershed in lake Tana basin. Land Degrad. Dev. 2017, 28, 1386–1397. [Google Scholar] [CrossRef]
- Zimale, F.A.; Moges, M.A.; Alemu, M.L.; Ayana, E.K.; Demissie, S.S.; Tilahun, S.A.; Steenhuis, T.S. Budgeting suspended sediment fluxes in tropical monsoonal watersheds with limited data: The Lake Tana basin. Journal of Hydrology and Hydromechanics. J. Hydrol. Hydromech. 2018, 66, 65–78. [Google Scholar] [CrossRef]
- Steenhuis, T.S.; Collick, A.S.; Easton, Z.M.; Leggesse, E.S.; Bayabil, H.K.; White, E.D.; Ahmed, A.A. Predicting discharge and sediment for the Abay (Blue Nile) with a simple model. Hydrol. Proc. 2009, 23, 3728–3737. [Google Scholar] [CrossRef]
- Steenhuis, T.S.; van der Molen, W.H. The Thornthwaite-Mather Procedure as a Simple Engineering Method to Predict Recharge. J. Hydrol. 1986, 84, 221–229. [Google Scholar] [CrossRef]
- Enku, T.; Melesse, A.M. A simple temperature method for the estimation of evapotranspiration. Hydrol. Proc. 2014, 28, 2945–2960. [Google Scholar] [CrossRef]
- Bayabil, H.K.; Tilahun, S.A.; Collick, A.S.; Yitaferu, B.; Steenhuis, T.S. Are runoff processes ecologically or topographically driven in the (sub) humid Ethiopian highlands? The case of the Maybar watershed. J. Ecohydrol. 2010, 3, 457–466. [Google Scholar] [CrossRef]
- Moges, M.A.; Zimale, F.A.; Alemu, M.L.; Ayele, G.K.; Dagnew, D.C.; Tilahun, S.A.; Steenhuis, T.S. Sediment concentration rating curves for a monsoonal climate: Upper Blue Nile Basin. Soil 2016, 2, 337–349. [Google Scholar] [CrossRef]
- Adem, A.A.; Aynalem, D.W.; Addis, G.G.; Tilahun, S.A.; Mekuria, W.; Belete, M.A.; Steenhuis, T.S. Runoff and soil loss of an uphill reforested and a downstream conserved agricultural Ethiopian faulted highland watershed. 2019; [submitted]. [Google Scholar]
- Tesemma, Z.K.; Mohamed, Y.A.; Steenhuis, T.S. Trends in rainfall and runoff in the Blue Nile Basin: 1964–2003. Hydrol. Proc. 2010, 25, 3747–3758. [Google Scholar] [CrossRef]
- Dagnew, D.C.; Guzman, C.D.; Zegeye, A.D.; Akal, A.T.; Moges, M.A.; Tigist, T.Y.; Mekuria, W.; Ayana, E.K.; Tilahun, S.A.; Steenhuis, T.S. Sediment loss patterns in the sub-humid Ethiopian highlands. Land Degrad. Dev. 2016, 28, 1795–1805. [Google Scholar] [CrossRef]
- Opolot, E.; Araya, T.; Nyssen, J.; Al-Barri, B.; Verbist, K.; Cornelis, W.M. Evaluating in Situ Water and Soil Conservation Practices with a Fully Coupled, Surface/Subsurface Process-Based Hydrological Model in Tigray, Ethiopia. Land Degrad. Dev. 2013, 27, 1840–1852. [Google Scholar] [CrossRef]
- Collick, A.S.; Easton, Z.M.; Ashagrie, T.; Biruk, B.; Tilahun, S.; Adgo, E.; Awulachew, S.B.; Zeleke, G.; Steenhuis, T.S. A simple semi-distributed water balance model for the Ethiopian highlands. Hydrol. Proc. 2009, 23, 3718–3727. [Google Scholar] [CrossRef]
- Tilahun, S.A.; Mukundan, R.; Demisse, B.A.; Engda, T.A.; Guzman, C.D.; Tarakegn, B.C.; Easton, Z.M.; Collick, A.S.; Zegeye, A.D.; Schneiderman, E.M.; et al. A saturation excess erosion model. Trans. ASABE 2013, 56, 681–695. [Google Scholar] [CrossRef]
- Malvicini, C.F.; Steenhuis, T.S.; Walter, M.T.; Parlange, J.-Y.; Walter, M.F. Evaluation of spring flow in the uplands of Matalom, Leyte, Philippines. Adv. Water Resour. 2005, 28, 1083–1090. [Google Scholar] [CrossRef]
- Haregeweyn, N.; Tsunekawa, A.; Poesen, J.; Tsubo, M.; Meshesha, D.T.; Fenta, A.A.; Nyssen, J.; Adgo, E. Comprehensive assessment of soil erosion risk for better land use planning in river basins: Case study of the Upper Blue Nile River. Sci. Total Environ. 2017, 574, 95–108. [Google Scholar] [CrossRef]

| Month | Rainfall (mm month−1) | Runoff (mm month−1) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 2010 | 2011 | 2012 | 2013 | 2014 | 2015 | 2010 | 2011 | 2012 | 2013 | 2014 | 2015 | |
| January | 23 | 5 | 0 | 10 | 13 | 0 | 41 | 8 | 12 | 17 | 3 | |
| February | 0 | 0 | 0 | 0 | 0 | 0 | 38 | 5 | 11 | 12 | 0 | |
| March | 58 | 47 | 95 | 9 | 64 | 0 | 36 | 4 | 14 | 11 | 8 | |
| April | 108 | 48 | 16 | 0 | 100 | 0 | 50 | 5 | 11 | 8 | 9 | |
| May | 115 | 127 | 77 | 68 | 240 | 73 | 41 | 19 | 13 | 7 | 46 | 7 |
| June | 151 | 193 | 266 | 218 | 83 | 157 | 33 | 22 | 45 | 24 | 27 | 23 |
| July | 461 | 500 | 481 | 672 | 302 | 444 | 79 | 110 | 71 | 102 | 60 | 95 |
| August | 435 | 458 | 319 | 458 | 403 | 344 | 81 | 125 | 62 | 93 | 96 | 91 |
| September | 168 | 165 | 163 | 166 | 197 | 147 | 39 | 51 | 38 | 61 | 84 | 59 |
| October | 22 | 31 | 42 | 68 | 26 | 11 | 8 | 40 | 28 | 40 | 36 | 14 |
| November | 26 | 102 | 77 | 0 | 35 | 0 | 3 | 35 | 26 | 22 | 23 | |
| December | 9 | 0 | 16 | 0 | 0 | 0 | 2 | 23 | 18 | 13 | 7 | |
| Total | 1577 | 1676 | 1552 | 1667 | 1462 | 1176 | 453 | 445 | 348 | 410 | 401 | 290 |
| Statistical Parameter | 2010 | 2011 | 2012 | 2013 | 2014 | 2015 |
|---|---|---|---|---|---|---|
| Nash | 0.80 | 0.72 | 0.67 | 0.90 | 0.63 | 0.73 |
| R2 | 0.81 | 0.72 | 0.67 | 0.90 | 0.64 | 0.80 |
| Proportional Area | 2010 | 2011 | 2012 | 2013 | 2014 | 2015 |
|---|---|---|---|---|---|---|
| Saturated Area, A1 | 0.05 | 0.05 | 0.05 | 0.05 | 0.05 | 0.05 |
| Degraded Area, A2 | 0.06 | 0.1 | 0.07 | 0.05 | 0.1 | 0.08 |
| Hillside, A3 | 0.15 | 0.29 | 0.26 | 0.22 | 0.35 | 0.28 |
| Storage max (mm) | ||||||
| Saturated area, Smax1 | 120 | 120 | 120 | 120 | 120 | 120 |
| Degraded area, Smax2 | 25 | 25 | 25 | 25 | 25 | 25 |
| Hillside, Smax3 | 100 | 100 | 100 | 100 | 100 | 100 |
| Initial moisture (mm) | ||||||
| Saturated area | 25 | 25 | 25 | 25 | 25 | 25 |
| Degraded area | 10 | 10 | 10 | 10 | 10 | 10 |
| Hillside | 50 | 50 | 50 | 50 | 50 | 50 |
| Sub-surface flow parameters | ||||||
| Maximum groundw. stor, BSmax (mm) | 250 | 250 | 250 | 250 | 250 | 250 |
| Initial groundw. stor (mm) | 180 | 45 | 50 | 60 | 0 | 0 |
| Base flow half-life (t1/2) (day) | 60 | 60 | 60 | 60 | 60 | 60 |
| Interflow (τ*) (day) | 10 | 10 | 10 | 10 | 10 | 10 |
| Year | Observed Flow (mm. a−1) | Simulated Total Flow (mm. a−1) | Subsurface Flow (mm. a−1) | Evaporation (mm. a−1) | GWFI |
|---|---|---|---|---|---|
| 2010 | 452 | 461 | 275 | 208 | 0.60 |
| 2011 | 445 | 453 | 289 | 264 | 0.64 |
| 2012 | 348 | 362 | 247 | 228 | 0.68 |
| 2013 | 410 | 402 | 279 | 147 | 0.69 |
| 2014 | 402 | 353 | 227 | 327 | 0.64 |
| 2015 | 290 | 226 | 121 | 134 | 0.53 |
| Average | 391 | 376 | 240 | 218 | 0.63 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akale, A.T.; Dagnew, D.C.; Moges, M.A.; Tilahun, S.A.; Steenhuis, T.S. The Effect of Landscape Interventions on Groundwater Flow and Surface Runoff in a Watershed in the Upper Reaches of the Blue Nile. Water 2019, 11, 2188. https://doi.org/10.3390/w11102188
Akale AT, Dagnew DC, Moges MA, Tilahun SA, Steenhuis TS. The Effect of Landscape Interventions on Groundwater Flow and Surface Runoff in a Watershed in the Upper Reaches of the Blue Nile. Water. 2019; 11(10):2188. https://doi.org/10.3390/w11102188
Chicago/Turabian StyleAkale, Adugnaw T., Dessalegn C. Dagnew, Mamaru A. Moges, Seifu A. Tilahun, and Tammo S. Steenhuis. 2019. "The Effect of Landscape Interventions on Groundwater Flow and Surface Runoff in a Watershed in the Upper Reaches of the Blue Nile" Water 11, no. 10: 2188. https://doi.org/10.3390/w11102188
APA StyleAkale, A. T., Dagnew, D. C., Moges, M. A., Tilahun, S. A., & Steenhuis, T. S. (2019). The Effect of Landscape Interventions on Groundwater Flow and Surface Runoff in a Watershed in the Upper Reaches of the Blue Nile. Water, 11(10), 2188. https://doi.org/10.3390/w11102188







