On the Difference of River Resistance Computation between the
k
−
ε
Model and the Mixing Length Model
Abstract
:1. Introduction
2. Computational Methods of Resistance Factors by Using Different Models
- Solve the turbulence equations to obtain the vertical distribution of ;
- Average over the depth to obtain ; and
- Calculate the resistance factor or by the ratio of to .
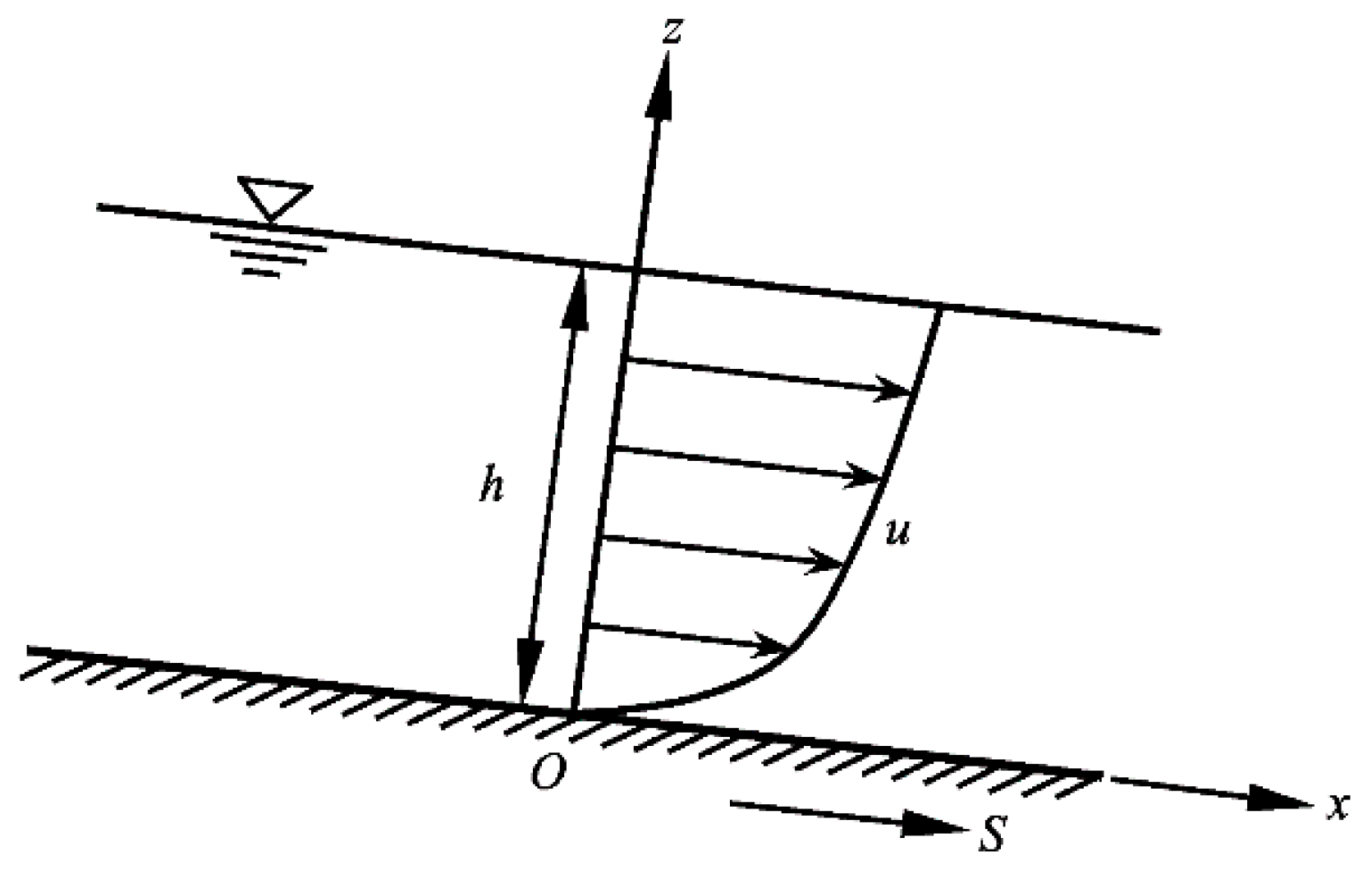
2.1. Prandtl Mixing Length Model
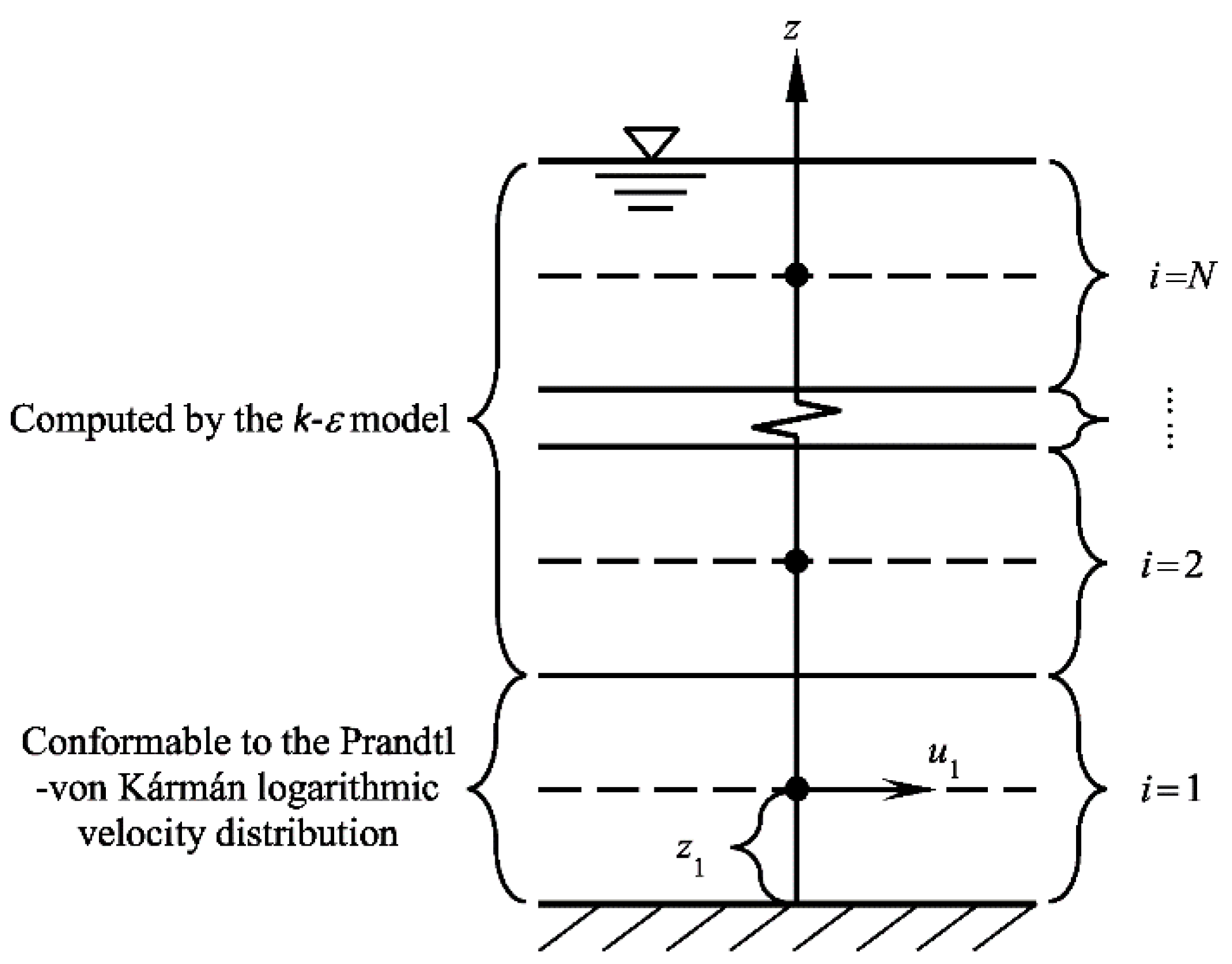
2.2. Model
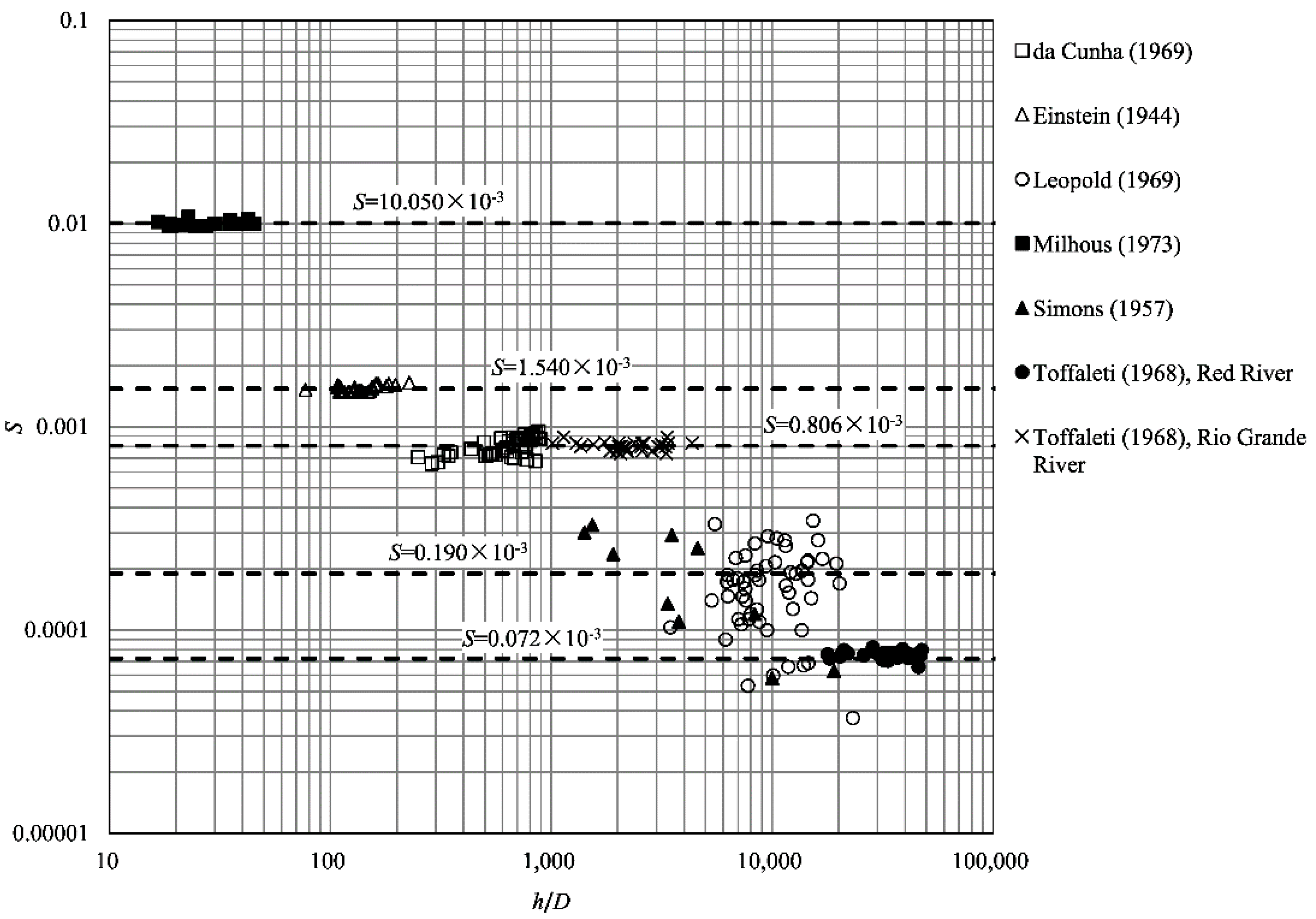
3. Determination of Equivalent Bed-Roughness
4. Data, Results, and Analyses
4.1. Data Sources and Range
4.2. Analysis of Influential Factors
4.3. Computational Results and Analyses
- (a)
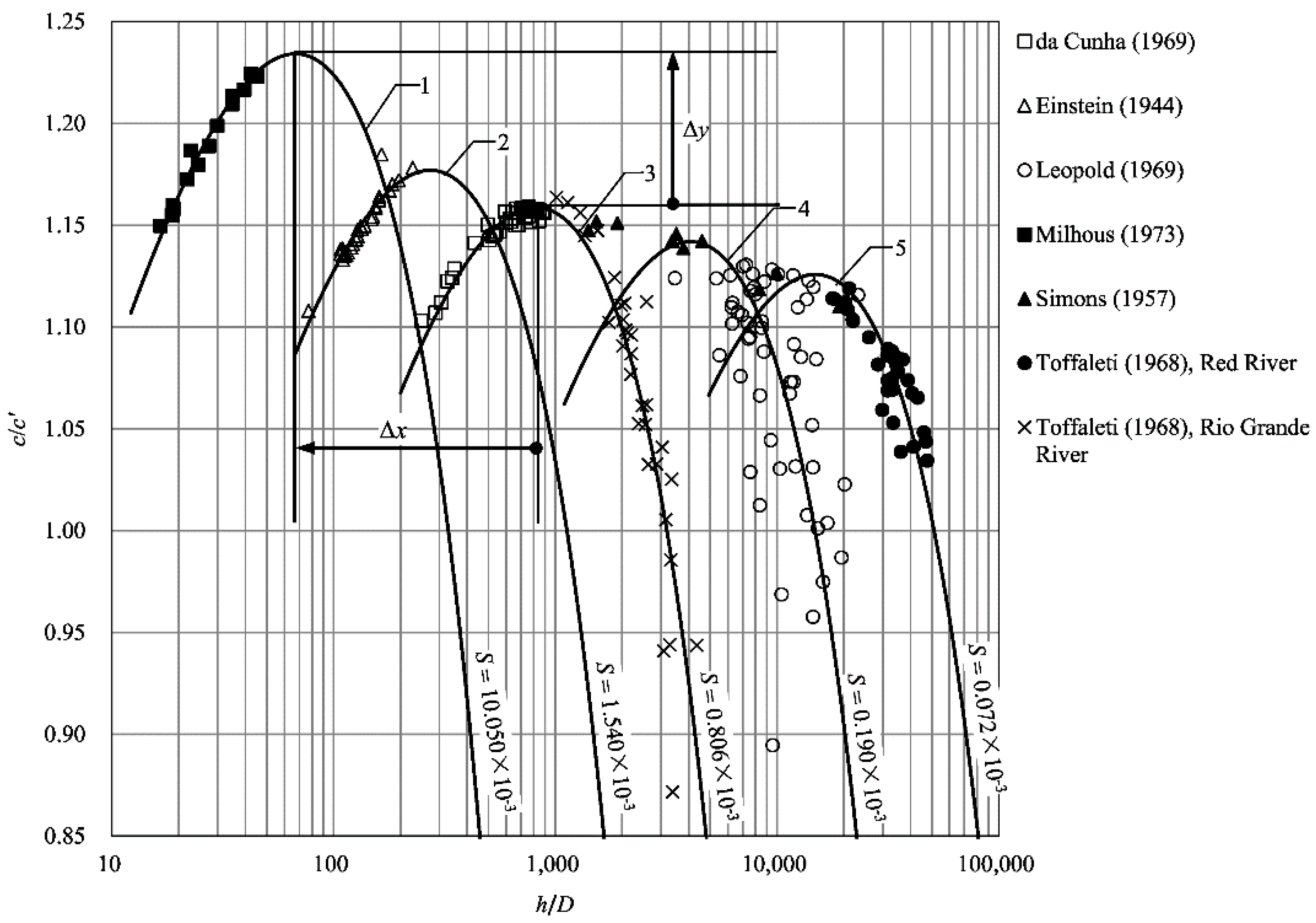
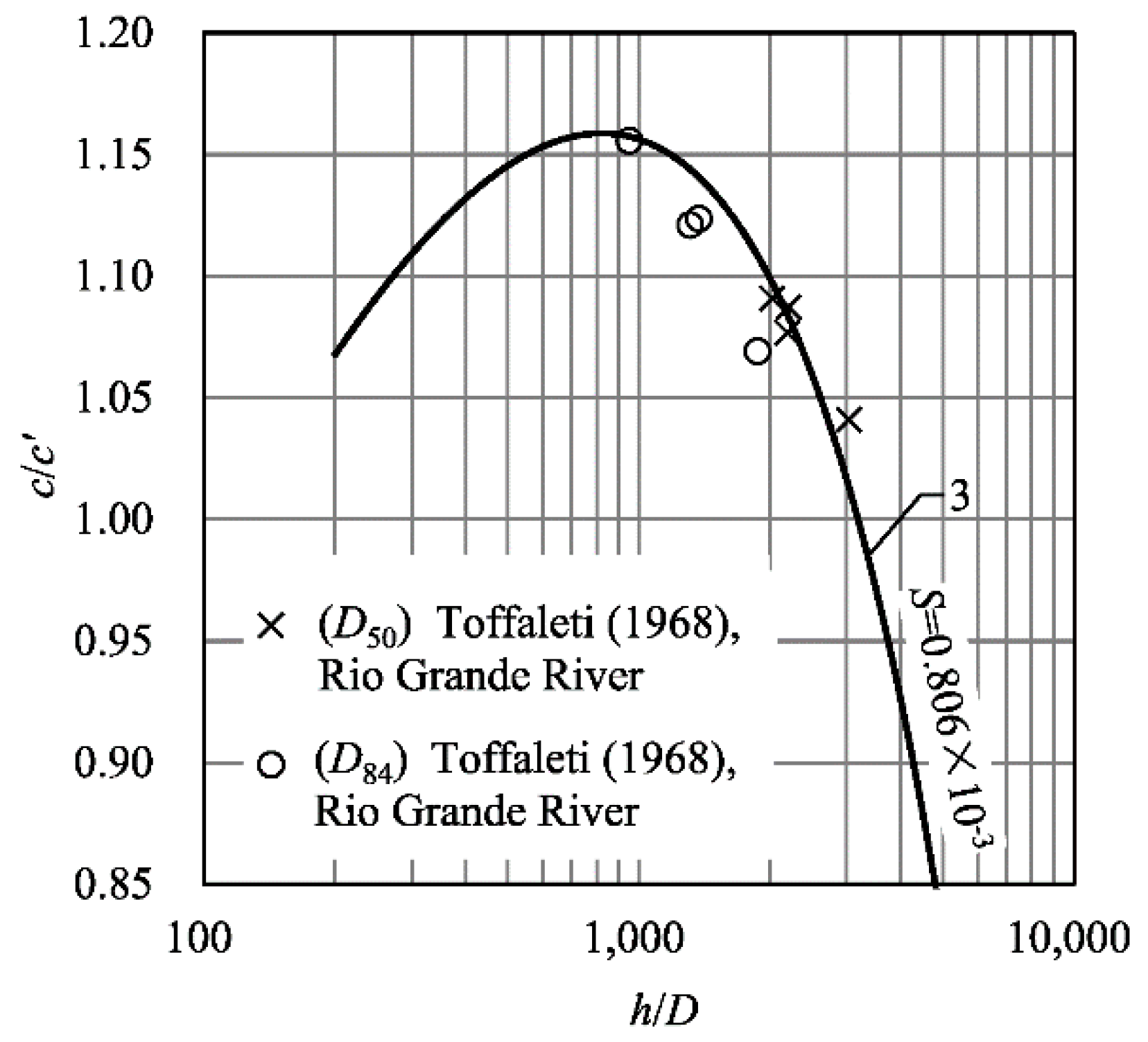
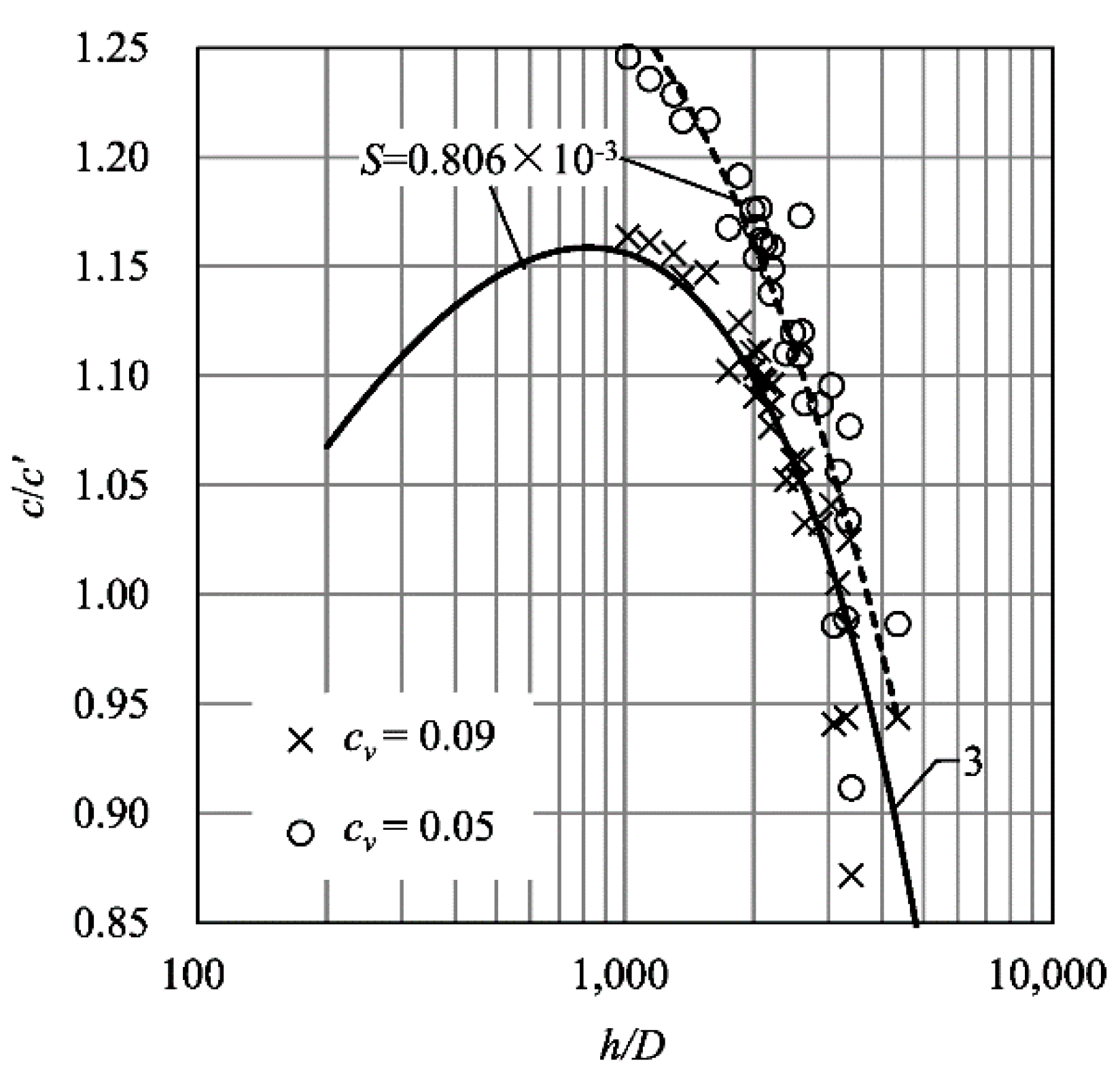
- For a specific , first increases and then decreases with the increment of ; the variation pattern of versus is shown as a convex upward curve;
- (b)
- The peak value of the convex upward curve increases with the increment of ; Figure 9b, along with Equation (49), indicates that the peak value is approximately linear with ; and
- (c)
- The abscissa of the peak value decreases with the increment of ; Figure 9a, along with Equation (48), indicates that the abscissa of the peak value is approximately linear with as well.
- Calculate the resistance factor corresponding to the Prandtl mixing length model by employing Equation (3) (see Section 2.1);
- Covert the resistance factor from the one corresponding to the Prandtl mixing length model into the one corresponding to the model by employing Equation (50);
- Substitute instead of into Equation (5) to calculate the depth-averaged velocity ; and
- Calculate the flow discharge as .
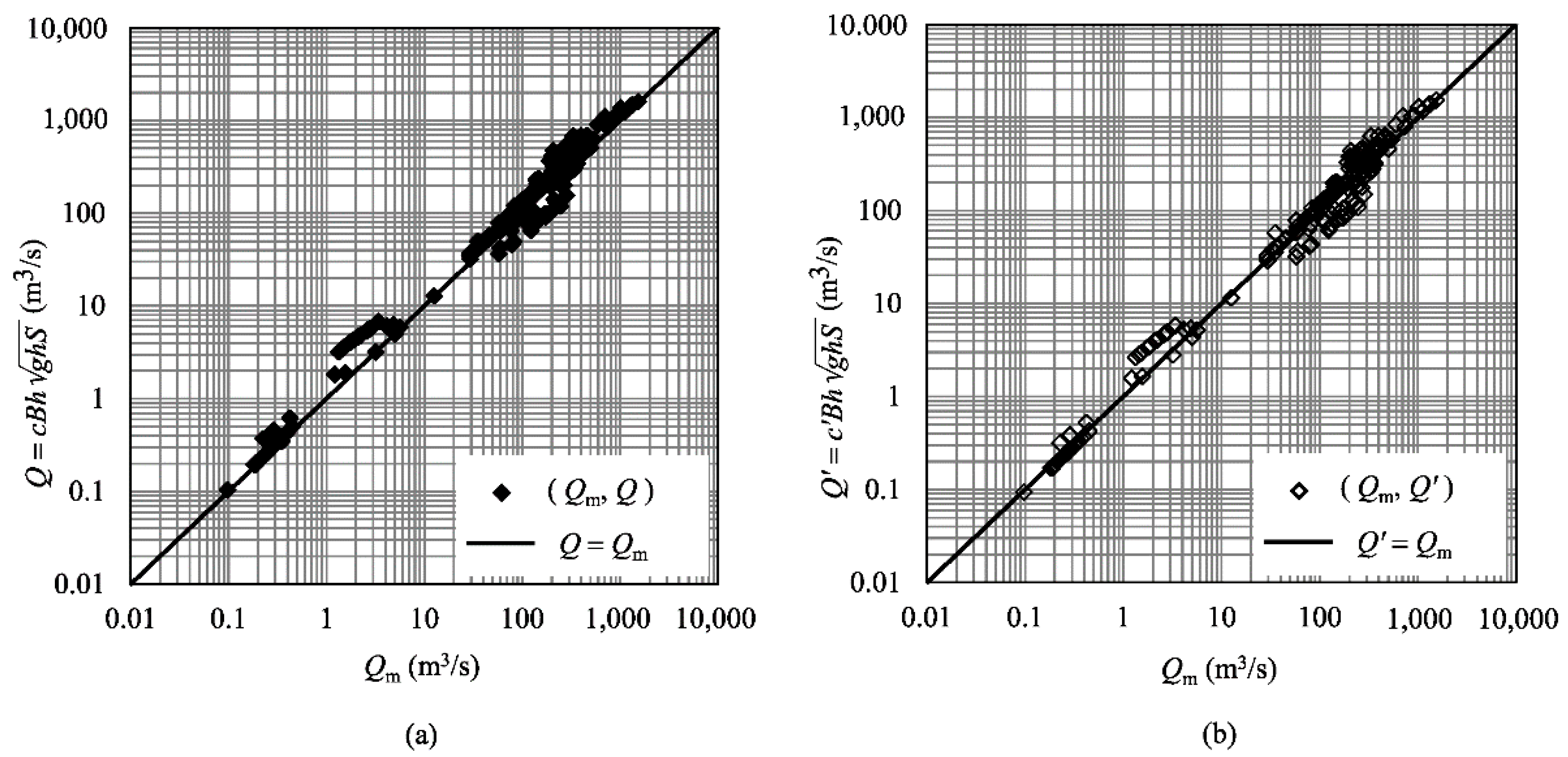
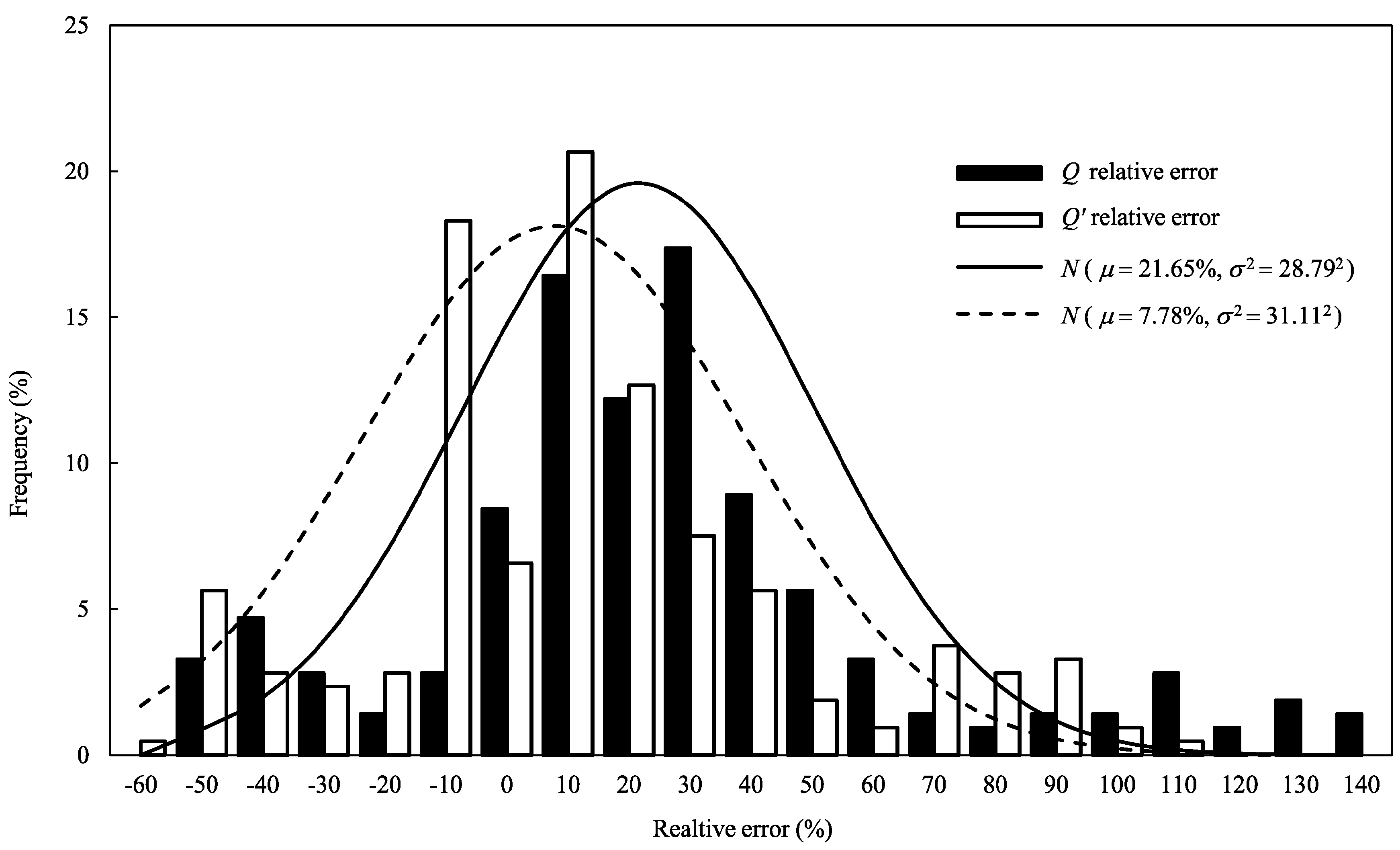
4.4. Discharge Verification
5. Discussion
5.1. Influence of the Selection of the Characteristic Bed Material Diameter
5.2. Influence of the Coefficients in the Model
6. Conclusions
- (a)
- The resistance factors corresponding to these two models are not identical. A new expression Equation (50), has been derived to quantify it. The difference of these two models, evaluated by the ratio , first increases and then decreases with the increment of the relative water depth . This variation pattern can be represented by a convex upward curve, of which the peak value increases and the abscissa of the peak value decreases with the increment of the bed slope .
- (b)
- Both turbulence models can generate reasonable results with acceptable errors with respect to the flow discharges in natural rivers. Each model has its own advantages and disadvantages. Within the range of the collected natural rivers, the mean relative error of the computed flow discharges corresponding to the Prandtl mixing length model is less than that corresponding to the model; in contrast, the error distribution corresponding to the former is more scattered than that corresponding to the latter. Furthermore, the flow discharges computed with these two models can be converted into each other by taking into account Equation (55).
- (c)
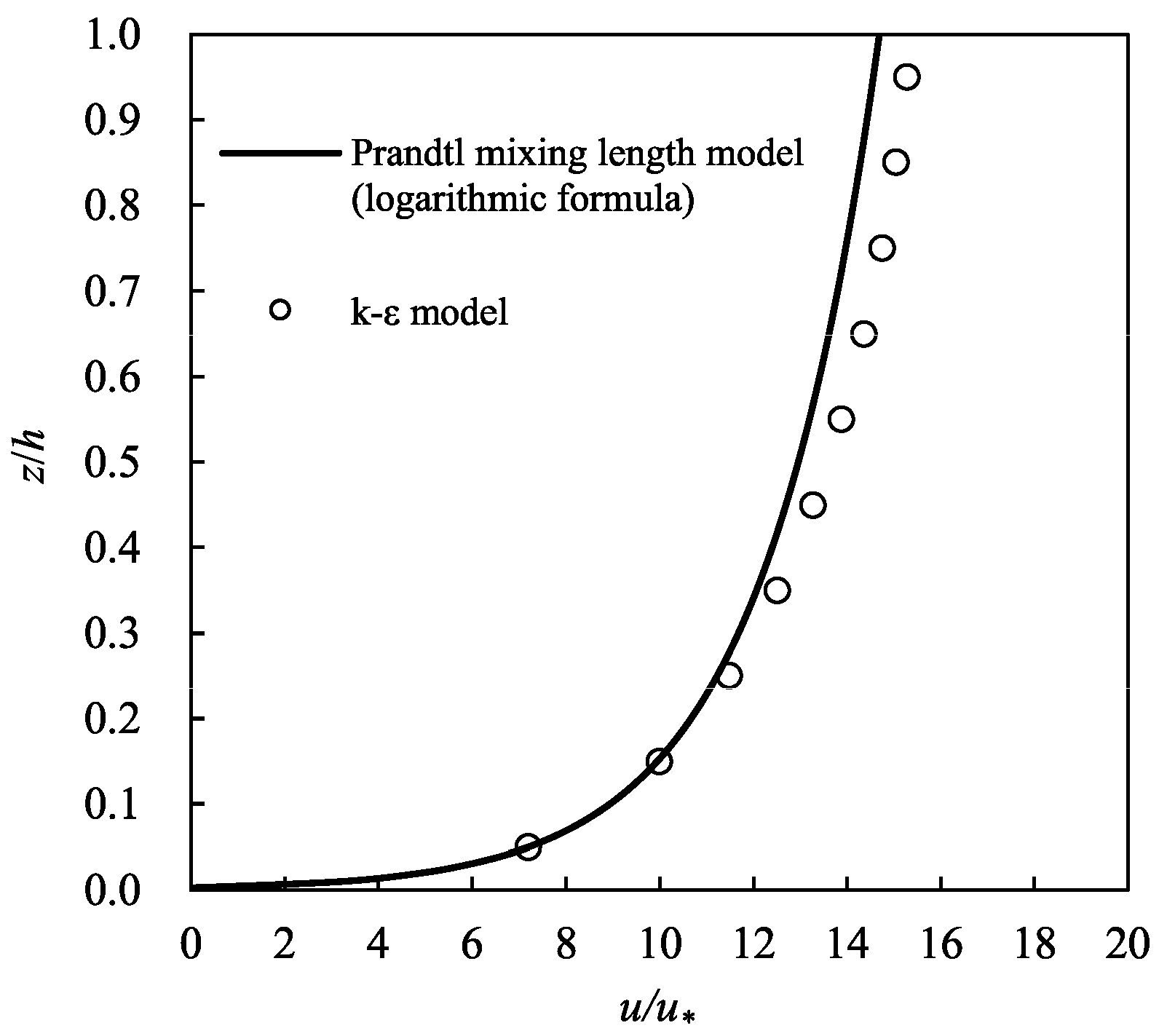
- The velocity distribution patterns corresponding to these two models within the range of are approximately consistent, whereas the difference displays to some extent within the range of .
- (d)
- For river computation issues, the simulation results corresponding to the model and the Prandtl mixing length model are not exactly identical, although both can well simulate the flow. Accounting for that, the difference between the river resistance characteristics reflected by these two models should be considered.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Yalin, M.S.; da Silva, A.M.F. Fluvial Process; International Association of Hydraulic Engineering and Research (IAHR): Delft, The Netherlands, 2001. [Google Scholar]
- Zhang, Z.X.; Dong, Z.N. Viscous Fluid Mechanics, 2nd ed.; Tsinghua University Press: Beijing, China, 2011. (In Chinese) [Google Scholar]
- Yalin, M.S. Mechanics of Sediment Transport; Pergamon: Oxford, UK, 1972. [Google Scholar]
- Nezu, I.; Nakagawa, H. Turbulence in Open-Channel Flows; A.A. Balkema: Rotterdam, The Netherlands, 1993. [Google Scholar]
- Wu, W.; Rodi, W.; Wenka, T. 3D numerical modeling of flow and sediment transport in open channels. J. Hydraul. Eng. 2000, 126, 4–15. [Google Scholar] [CrossRef]
- Zhang, M.L.; Shen, Y.M.; Zhu, L.Y. Depth-averaged two-dimensional numerical simulation for curved open channels with vegetation. J. Hydraul. Eng. 2008, 39, 794–800. (In Chinese) [Google Scholar]
- Thi, H.T.N.; Jungkyu, A.; Sung, W.P. Numerical and physical investigation of the performance of turbulence modeling schemes around a scour hole downstream of a fixed bed protection. Water 2018, 10, 103. [Google Scholar] [CrossRef]
- Wang, X.L.; Wang, Q.S.; Zhou, Z.Y.; Ao, X.F. Three-dimensional turbulent numerical simulation of Bingham fluid in the goaf grouting of the south-to-north water transfer project. J. Hydraul. Eng. 2013, 44, 1295–1302. (In Chinese) [Google Scholar]
- Wibron, E.; Ljung, A.-L.; Lundström, T.S. Computational fluid dynamics modeling and validating experiments of airflow in a data center. Energies 2018, 11, 644. [Google Scholar] [CrossRef]
- Wookyung, K.; Volodymyr, S.; Dmitriy, M.; Vladimir, M. Simulations of blastwave and fireball occurring due to rupture of high-pressure hydrogen tank. Safety 2017, 3, 16. [Google Scholar] [CrossRef]
- Vesselin, K.K.; Luca, S.; Giacomo, F. A modified version of the RNG k–ε turbulence model for the scale-resolving simulation of internal combustion engines. Energies 2018, 10, 2116. [Google Scholar] [CrossRef]
- Liu, F.; Qian, H.; Zheng, X.; Zhang, L.; Liang, W. Numerical study on the urban ventilation in regulating microclimate and pollutant dispersion in urban street canyon: A case study of Nanjing new region, China. Atmosphere 2017, 8, 164. [Google Scholar] [CrossRef]
- Rodi, W. Turbulence Model and Their Application in Hydraulics—A State-of-the-Art Review, 3rd ed.; A.A. Balkema: Rotterdam, The Netherlands, 1993. [Google Scholar]
- Nezu, I.; Rodi, W. Open-channel flow measurements with a laser Doppler anemometer. J. Hydraul. Eng. 1986, 112, 335–355. [Google Scholar] [CrossRef]
- Toffaleti, F.B. A Procedure for Computation of the Total River Sand Discharge and Detailed Distribution, Bed to Surface; US Army Corps of Engineers: Washington, DC, USA, 1968.
- Meftah, M.B.; Serio, F.D.; Malcangio, D.; Mossa, M. Resistance and boundary shear in a partly obstructed channel flow. In River Flow 2016; Constantinescu, G., Garcia, M., Hanes, D., Eds.; Taylor & Francis Group: London, UK, 2016; pp. 795–801. [Google Scholar]
- Rijn, L.C.V. Sediment transport, part III: Bed forms and alluvial roughness. J. Hydraul. Eng. 1984, 110, 1733–1754. [Google Scholar] [CrossRef]
- Shao, X.J. Introduction to River Mechanics; Tsinghua University Press: Beijing, China, 2013. (In Chinese) [Google Scholar]
- Da Cunha, L.V. River Mondego, Portugal; 1969. In Brownlie, W.R. Compilation of Alluvial Channel Data: Laboratory and Field; California Institute of Technology: Pasadena, CA, USA, 1981. [Google Scholar]
- Einstein, H.A. Bed-Load Transportation in Mountain Creek; US Department of Agriculture, Soil Conservation Service: Washington, DC, USA, 1944. [Google Scholar]
- Leopold, L.B. Sediment Transport Data for Various U.S. Rivers; 1969. In Brownlie, W.R. Compilation of Alluvial Channel Data: Laboratory and Field; California Institute of Technology: Pasadena, CA, USA, 1981. [Google Scholar]
- Milhous, R.T. Sediment Transport in a Gravel-Bottomed Stream. Ph.D. Thesis, Oregon State University, Corvallis, OR, USA, 1973. [Google Scholar]
- Simons, D.B. Theory of Design of Stable Channels in Alluvial Materials. Ph.D. Thesis, Colorado State University, Fort Collins, CO, USA, 1957. [Google Scholar]


| No. | Data Sources | Q (m3/s) | h (m) | S (×10−3) | D (mm) | Number of Groups |
|---|---|---|---|---|---|---|
| 1 | da Cunha (1969) | 29.00∼159.99 | 0.55∼1.97 | 0.66∼0.95 | 2.2 | 44 |
| 2 | Einstein (1944) | 0.10∼0.45 | 0.07∼0.21 | 1.48∼1.65 | 0.9 | 29 |
| 3 | Leopold (1969) | 83.33∼499.30 | 0.96∼4.11 | 0.04∼0.35 | 0.14∼0.81 | 55 |
| 4 | Milhous (1973) | 1.33∼3.40 | 0.37∼0.53 | 9.70∼10.80 | 8.20∼27.00 | 14 |
| 5 | Simons (1957) | 1.22∼29.42 | 0.80∼2.59 | 0.06∼0.33 | 0.10∼0.72 | 10 |
| 6 | Toffaleti (1968), Red River | 190.28∼1537.56 | 3.00∼7.38 | 0.07∼0.08 | 0.09∼0.22 | 30 |
| 7 | Toffaleti (1968), Rio Grande River | 35.11∼282.31 | 0.33∼1.06 | 0.74∼0.89 | 0.21∼0.36 | 31 |
| Range | 0.10∼1537.56 | 0.07∼7.38 | 0.04∼10.80 | 0.10∼27.00 | 213 (total) |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dai, W.; Ding, M.; Zhang, H.
On the Difference of River Resistance Computation between the
Dai W, Ding M, Zhang H.
On the Difference of River Resistance Computation between the
Dai, Wenhong, Mengjiao Ding, and Haitong Zhang.
2018. "On the Difference of River Resistance Computation between the
Dai, W., Ding, M., & Zhang, H.
(2018). On the Difference of River Resistance Computation between the





