Long Term Hydrodynamic Effects in a Semi-Arid Mediterranean Multilayer Aquifer: Campo de Cartagena in South-Eastern Spain
Abstract
:1. Introduction
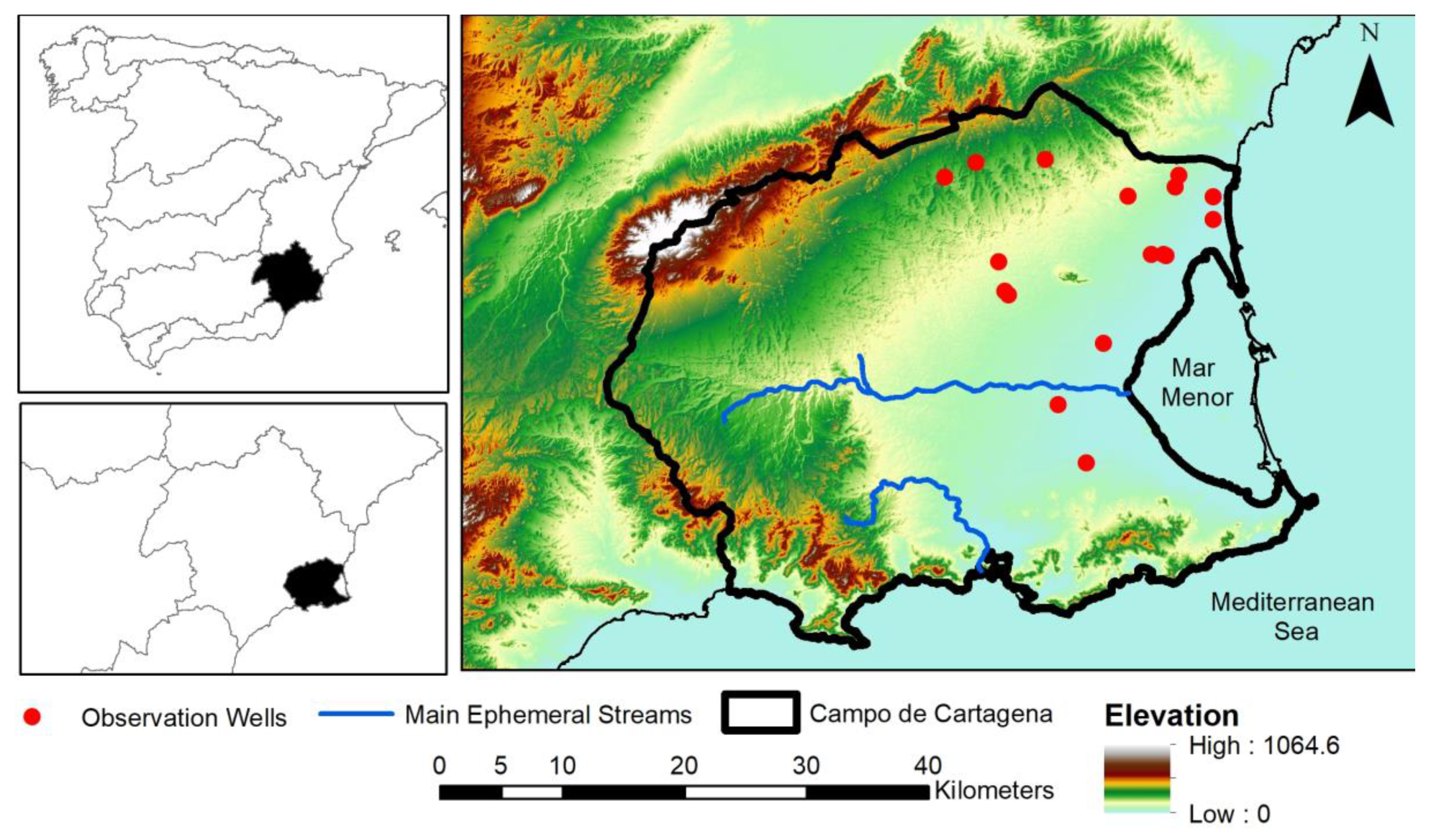
2. Case Study Area
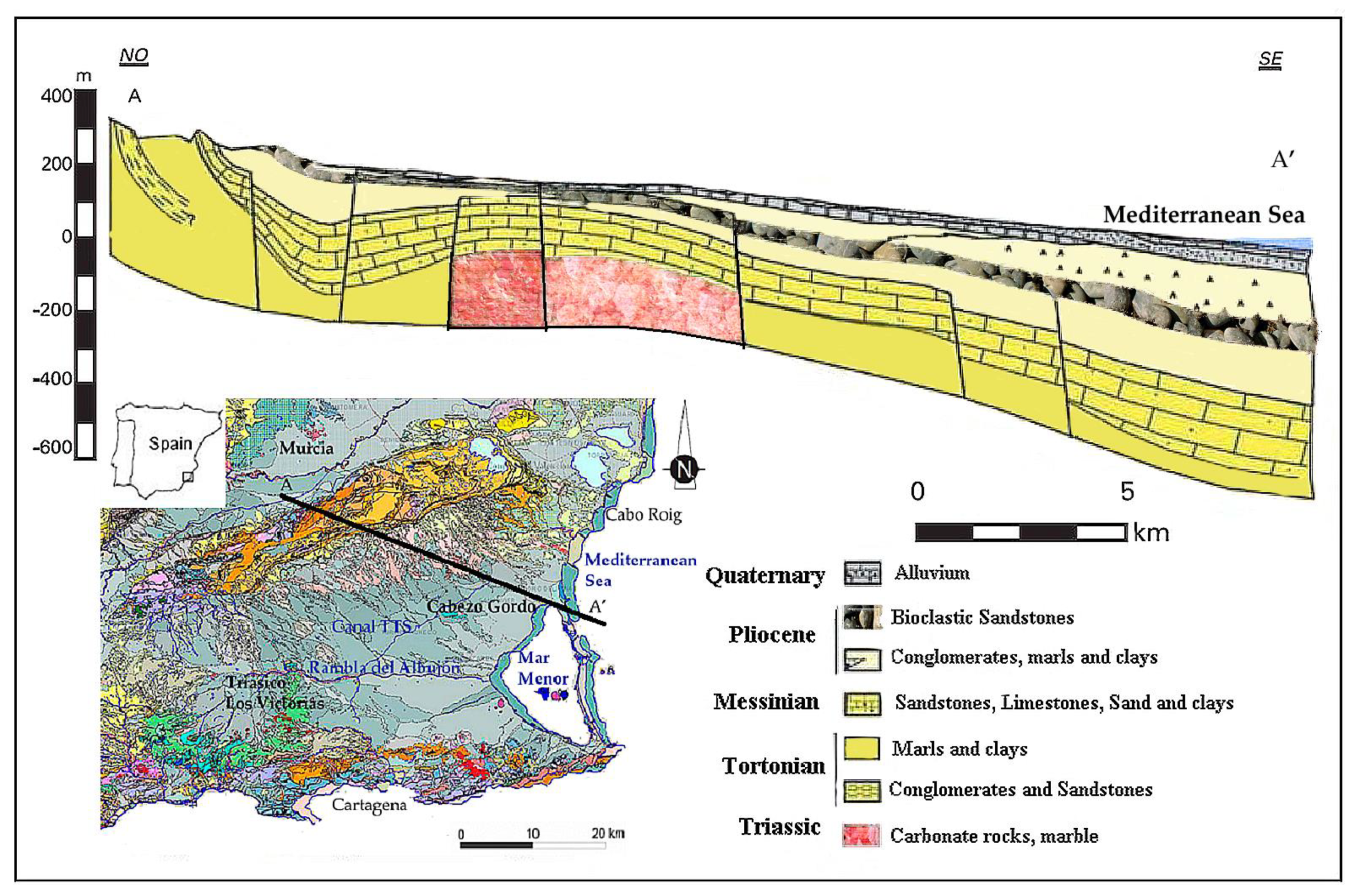
2.1. Geology
2.2. Historical Evolution of Anthropogenic Activities
3. Materials and Methods
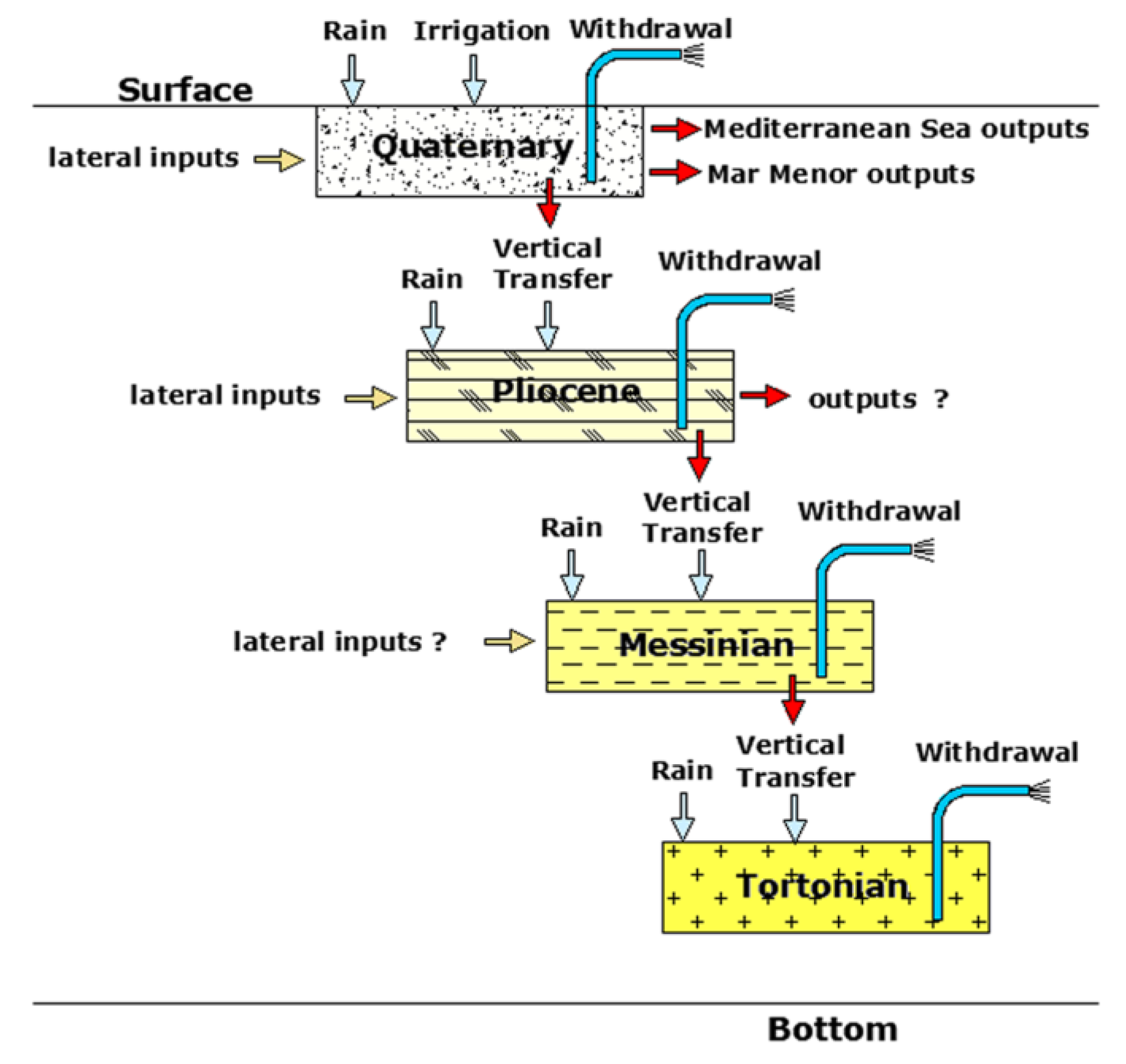
3.1. Numerical Model
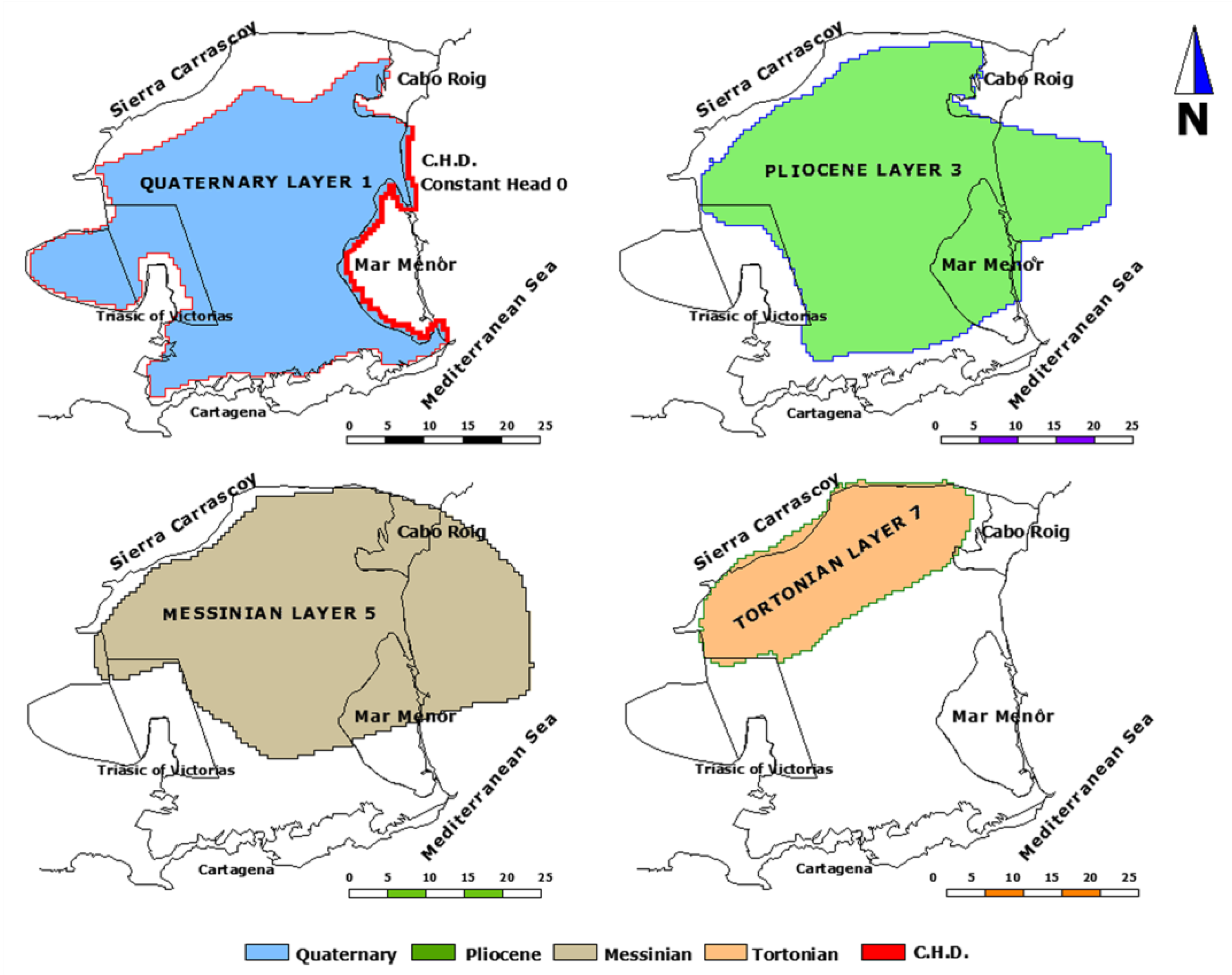
3.2. Model Setup and Geometry
3.3. Hydrodynamic Parameters
3.4. Boundary Conditions and External Forcings
3.4.1. Piezometric Data
3.4.2. Recharge
3.4.3. Withdrawals
3.4.4. Intra-Borehole Aquifer Cross-Contamination
3.4.5. Lateral Groundwater Discharge
3.5. Model Evaluation Criteria
4. Results
4.1. Calibration of the Model
4.1.1. Calibration of the Model in the Steady State
4.1.2. Calibration and Validation of the Model in Transient State
4.2. Results for the Steady State
4.3. Results for the Transient State
4.4. Sensitivity Analysis
5. Discussion
6. Conclusions
- The water balances generated and analyzed in the present study indicate the importance of irrigation returns in the Campo de Cartagena’s multilayer aquifer system—and generally, in similar systems that are linked to intensive agriculture in semi-arid zones. Slight drops in recharge significantly unbalanced the system’s ability to meet the intended exploitation.
- There is hydraulic communication between aquifer layers, partly caused by intrusions in aquitards such as the Cabezo Gordo horst, by contact with the Triassic of Los Victorias or Sierra Cartagena-La Unión, and by the existence of more than 2000 wells and boreholes, 600 of which cross several aquifers and thus generate direct vertical hydraulic communication.
- The vertical hydraulic communication through the wells was calculated at 40.01 Mm3/yr. If the total flow transfer across the aquifer is 84.68 Mm3/yr, this implies that 47.23% of the total flow takes place through vertical communication. The remaining 52.77% takes place through discontinuities, contacts and infiltration between layers. The model considered the fact that the Pliocene and Messinian aquifer layers lie under the Mar Menor lagoon and the Mediterranean Sea, as well as the fact that there is no communication between those masses of water and the inferior confined aquifers in that zone.
- Total present withdrawal in the Campo de Cartagena multilayer aquifer system is evaluated to be 91 Mm3/yr. As groundwater was the only source of water before the beginning of the TSWT, withdrawal values introduced into the model for its calibration were higher. The water balance is clearly favorable to the recovery of water levels in the Quaternary aquifer layer, as there is both water balance in the Pliocene aquifer layer and overexploitation in the Messinian aquifer layer.
- The average value for the annual global recharge was established as approximately 123 Mm3/yr for the period studied. However, the study of recharge and pumping of Quaternary-layer water for private desalination plants deserves further investigation.
- In the Triassic areas of Los Victorias and Cabezo Gordo, heavy falls in the piezometric levels occurred, even after the drying of the cells caused by the strong hydraulic gradients where communication between aquifers occurred. Such zones constitute carbonate aquifers, whose interrelations with the multilayer aquifer are difficult to model.
- This historical study was carried out by fitting the entire aquifer system into a numerical model, and it provided valuable information to stakeholders for sustainable management of the aquifer in the future. The water-balance values that the model provided were similar to the figures from other studies, and the recovery of piezometric levels in the upper aquifer, as observed in recent decades, was corroborated.
- Two desirable studies to carry out in the future include an analysis of possible pumping using scavenger wells near the coast, which would reduce the discharge of the Quaternary layer to the Mar Menor, and a study regarding transferring flow from the Quaternary to the Messinian so as to recharge the latter and minimize discharge to the Mar Menor.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Leduc, C.; Pulido-Bosch, A.; Remini, B. Anthropization of groundwater resources in the Mediterranean region: Processes and challenges. Hydrogeol. J. 2017, 25, 1529–1547. [Google Scholar] [CrossRef]
- Baudron, P.; Alonso-Sarria, P.; García-Aróstegui, J.L.; Cánovas-García, L.; Martínez-Vicente, D.; Moreno-Brotons, J. Identifying the origin of groundwater samples in a multi-layer aquifer system with Random Forest classification. J. Hidrol. 2013, 499, 303–315. [Google Scholar] [CrossRef]
- Jiménez-Martínez, J.; García-Aróstegui, J.L.; Hunink, J.E.; Contreras, S.; Baudron, P.; Candela, L. The role of groundwater in highly human-modified hydrosystems: A review of impacts and mitigation options in the Campo de Cartagena-Mar Menor coastal plain (SE Spain). Environ. Rev. 2016, 24, 377–392. [Google Scholar] [CrossRef]
- García-Aróstegui, J.L.; Marín, F.; Martínez, D. Informe integral sobre el estado ecológico del Mar Menor. Hidrogeología. 2016. Available online: http://www.canalmarmenor.es/documents/575990/603406/Informe+Integral+sobre+el+estado+ecol%C3%B3gico+del+Mar+Menor (accessed on 10 November 2017).
- Molina, J.L.; Bromley, J.; Garcia-Arostegui, J.L.; Sullivan, C.; Benavente, J. Integrated Water Resources Management of Overexploited Hydrogeological Systems using Object-Oriented Bayesian Networks. Environ. Model. Softw. 2010, 25, 383–397. [Google Scholar] [CrossRef]
- Custodio, E.; Andreu-Rodes, J.M.; Aragón, R.; Estrela, T.; Ferrer, J.; García-Aróstegui, J.L.; Manzano, M.; Rodríguez-Hernández, L.; Sahuquillo, A.; Villar, A. Groundwater intensive use and mining in south-eastern peninsular Spain: Hydrogeological, economic and social aspects. Sci. Total Environ. 2016, 559, 302–316. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Baudron, P.; Barbecot, F.; García-Aróstegui, J.L.; Leduc, C.; Travi, I.; Martínez-Vicente, D. Impacts of human activities on recharge in a multilayered semiarid aquifer (Campo de Cartagena, SE Spain). Hydrol. Process. 2014, 28, 2223–2236. [Google Scholar] [CrossRef]
- Baudron, P.; Cockenpot, S.; López-Castejon, F.; Radakovitch, O.; Gilabert, J.; Mayer, A.; García-Aróstegui, J.L.; Martínez-Vicente, D.; Leduc, C.; Claude, C. Combining radon, short-lived radium isotopes and hydrodynamic modeling to assess submarine groundwater discharge from an anthropized semiarid watershed to a Mediterranean lagoon (Mar Menor, SE Spain). J. Hydrol. 2015, 525, 55–71. [Google Scholar] [CrossRef]
- Senent-Alonso, M.; Martínez-Vicente, D.; Cabezas, F.; García-Aróstegui, J.L.; Baudron, P. Aproximación mediante modelización matemática a la evaluación de las descargas del acuífero cuaternario del Campo de Cartagena al Mar Menor (Murcia). In El Mar Menor. Estado Actual del Conocimiento Científico, 1st ed.; Fundación Instituto Euromediterráneo del Agua, Ed.; Fundación Instituto Euromediterráneo del Agua: Murcia, Spain, 2009; pp. 109–130. ISBN 978–984-936326-8-7. [Google Scholar]
- García-Aróstegui, J.L.; Jiménez-Martínez, J.; Baudron, P.; Martínez-Vicente, D.; Guerra, J. Geometría del acuífero del Campo de Cartagena e implicaciones en el funcionamiento hidrogeológico. In Nuevas Aportaciones al Conocimiento de los Acuíferos Costeros, 1st ed.; Instituto Geológico y Minero de España, Ed.; Instituto Geológico y Minero de España: Madrid, Spain, 2012; pp. 439–449. ISBN 978–984-7840-874-0. [Google Scholar]
- Jiménez-Martínez, J.; Skaggs, T.H.; Van Genuchten, M.T.; Candela, L. A root zone modelling approach to estimating groundwater recharge from irrigated areas. J. Hydrol. 2009, 367, 138–149. [Google Scholar] [CrossRef]
- Jiménez-Martinez, J.; Candela, L.; García-Aróstegui, J.L.; Aragón, R. A 3D geological model of Campo de Cartagena, SE Spain: Hydrogeological implications. Geol. Acta 2012, 10, 49–62. [Google Scholar]
- March, H.; Saurí, D.; Rico-Amorós, A.M. The end of scarcity? Water desalination as the new cornucopia for Mediterranean Spain. J. Hydrol. 2014, 519, 2642–2651. [Google Scholar] [CrossRef] [Green Version]
- Aparicio, J.; Candela, L.; Alfranca, O.; García-Aróstegui, J.L. Economic evaluation of small desalination plants from brackish aquifers. Application to Campo de Cartagena (SE Spain). Desalination 2017, 411, 38–44. [Google Scholar] [CrossRef]
- Lacombe, S.; Sudicky, E.A.; Frape, S.K.; Unger, J.A. Influence of leaky boreholes on cross-formational groundwater flow and contaminant transport. Waterloo Centre for Groundwater Research. Ontario. Canada. Water Resour. Res. 1995, 31, 1871–1882. [Google Scholar] [CrossRef]
- Timms, W.; Acworth, I. Origin, lithology and weathering characteristics of Upper Tertiary—Quaternary clay aquitard units on the Lower Murrumbidgee alluvial fan. Aust. J. Earth Sci. 2002, 49, 525–537. [Google Scholar] [CrossRef]
- Santi, P.M.; McCray, J.E.; Martens, J.L. Investigating cross-contamination of aquifers. Hydrogeol. J. 2006, 14, 51–68. [Google Scholar] [CrossRef]
- Jiménez-Martínez, J.; Aravena, J.; Candela, L. The Role of Leaky Boreholes in the Contamination of a Regional Confined Aquifer. A Case Study: The Campo de Cartagena Region, Spain. Water Air Soil Poll. 2011, 215, 311–327. [Google Scholar] [CrossRef]
- McMillan, L.A.; Rivett, M.O.; Tellam, J.H.; Dumble, P.; Sharp, H. Influence of vertical flows in wells on groundwater sampling. J. Contam. Hydrol. 2014, 169, 50–61. [Google Scholar] [CrossRef] [PubMed]
- Khadri, S.F.R.; Pande, C. Groundwater flow modeling for calibrating steady state using MODFLOW software: A case study of Mahesh River basin, India. Model. Earth Syst. Environ. 2016, 2, 39. [Google Scholar] [CrossRef]
- McDonald, M.G.; Harbaugh, A.W. A Modular Three-Dimensional Finite-Difference Ground-Water Flow Model; U.S. Geological Survey Open-File Report 83-875; USGS Survey: Reston, VA, USA, 1984.
- Candela, L.; Elorza, F.J.; Tamoh, K.; Jiménez-Martinez, J.; Aureli, A. Groundwater modeling with limited data sets: The Chari-Logone area. Lake Chad Basin, Chad. Hydrol. Process. 2014, 28, 3714–3727. [Google Scholar] [CrossRef]
- Castejón-Porcel, G.; Espín-Sánchez, D.; Ruiz-Álvarez, V.; García-Marín, R.; Moreno-Muñoz, D. Runoff Water as a Resource in the Campo de Cartagena (Region of Murcia): Current Possibilities for Use and Benefits). Water 2018, 10, 456. [Google Scholar] [CrossRef]
- Garcia-Pintado, J.; Martinez-Mena, M.; Barbera, G.G.; Albaladejo, J.; Castillo, V.M. Anthropogenic nutrient sources and loads from a Mediterranean catchment into a coastal lagoon: Mar Menor, Spain. Sci. Total Environ. 2007, 373, 220–239. [Google Scholar] [CrossRef] [PubMed]
- Segura Basin Management Plan, 2015–2021. Available online: https://www.chsegura.es/chs/planificacionydma/planificacion15-21/ (accessed on 11 October 2017).
- Cabezas, F. Balance hídrico del Mar menor. In El Mar Menor. Estado Actual del Conocimiento Científico, 1st ed.; Fundación Instituto Euromediterráneo del Agua, Ed.; Fundación Instituto Euromediterráneo del Agua: Murcia, Spain, 2009; pp. 167–206. ISBN 978–984-936326-8-7. [Google Scholar]
- Rubio, J.M. La cuenca artesiana del Campo de Cartagena. Revista Minera, Metalúrgica y de Ingeniería 1928, LXXIX, 229–231, 433–444, 457–460. [Google Scholar]
- Senent-Aparicio, J.; Pérez-Sánchez, J.; García-Aróstegui, J.L.; Bielsa-Artero, A.; Domingo-Pinillos, J.C. Evaluating Groundwater Management Sustainability under Limited Data Availability in Semiarid Zones. Water 2015, 7, 4305–4322. [Google Scholar] [CrossRef] [Green Version]
- Alcalá, F.J.; Custodio, E. Natural uncertainty of spatial average aquifer recharge through atmospheric chloride mass balance in continental Spain. J. Hydrol. 2015, 524, 642–661. [Google Scholar] [CrossRef]
- IGME. Estudio Hidrogeológico del Campo de Cartagena. 1991. Available online: http://info.igme.es/SidPDF%5C067000%5C228%5CTomo%201%20Memoria%5C67228_0001.pdf (accessed on 25 September 2017).
- Alcon, F.; de Miguel, M.D.; Burton, M. Duration analysis of adoption of drip irrigation technology in southeastern Spain. Technol. Forecast. Soc. Chang. 2011, 78, 991–1001. [Google Scholar] [CrossRef]
- Cox, M.E.; James, A.; Hawke, A.; Raiber, M. Groundwater Visualisation System (GVS): A software framework for integrated display and interrogation of conceptual hydrogeological models, data and time-series animation. J. Hydrol. 2013, 491, 56–72. [Google Scholar] [CrossRef]
- Harbaugh, A.W.; Banta, E.R.; Hill, M.C.; McDonald, M.G. Modflow-2000, the U.S. Geological Survey Modular Ground-Water Model-User Guide to Modularization Concepts and the Ground-Water Flow Process; Open-File Report; U.S. Geological Survey: Reston, VA, USA, 2000; p. 134.
- Panagopoulos, G. Aplication of Modflow for simulating groundwater flow in the Trifilia karst aquifer. Greece. Environ. Earth Sci. 2012, 67, 1877–1889. [Google Scholar] [CrossRef]
- Gebreyohannes, T.; De Smedt, F.; Walraevens, K.; Gebresilassie, S.; Hussien, A.; Hagos, M.; Amare, K.; Deckers, J.; Gebrehiwot, K. Regional groundwater flow modeling of the Geba basin, Northern Ethiopia. Hydrogeol. J. 2017, 25, 639–655. [Google Scholar] [CrossRef]
- Mohamed, M.; Al-Suwaidi, N.; Ebraheem, A.; Al Mulla, M. Groundwater modeling as a precursor tool for water resources sustainability in Khatt area, UAE. Environ. Earth. Sci. 2016, 75, 400. [Google Scholar] [CrossRef]
- Dupuy de Lome, E.; Gorostízaga, J.; Novo y Chicarro, P. Informe del Instituto Geológico sobre alumbramientos de aguas subterráneas por cuenta del estado; Boletín Oficial de Minas y Metalurgia: Madrid, Spain, 1917; pp. 55–68. [Google Scholar]
- Baudron, P.; Barbecot, F.; Gillon, M.; García-Aróstegui, J.L.; Travi, Y.; Leduc, C.; Gomariz-Castillo, F.; Martínez-Vicente, D. Assessing modern agricultural recharge to a semiarid unconfined aquifer using bomb peak 14C and reconstituted irrigation water 3H. Radiocarbon 2013, 55, 1480–1492. [Google Scholar] [CrossRef]
- Nordbotten, J.M.; Celia, M.A.; Bachu, S. Analytical solutions for leakage rates through abandoned wells. Water Resour. Res. 2004, 40. [Google Scholar] [CrossRef] [Green Version]
- Yao, Y.Y.; Zheng, C.M.; Liu, J.; Cao, G.L.; Xiao, H.L.; Li, H.T.; Li, W.P. Conceptual and numerical models for groundwater flow in an arid inland river basin. Hydrol. Process. 2015, 29, 2034–2050. [Google Scholar] [CrossRef]
- Rey, J.; Martínez, J.; Barberá, G.G.; García-Aróstegui, J.L.; García-Pintado, J.; Martínez-Vicente, D. Geophysical characterization of the complex dynamics of groundwater and seawater exchange in a highly stressed aquifer system linked to a coastal lagoon (SE Spain). Environ. Earth Sci. 2013, 70, 1877–1889. [Google Scholar] [CrossRef]
- Ebrahimi, H.; Ghazavi, R.; Karimi, H. Estimation of Groundwater Recharge from the Rainfall and Irrigation in an Arid Environment Using Inverse Modeling Approach and RS. Water Resour. Manag. 2016, 30, 1939–1951. [Google Scholar] [CrossRef]

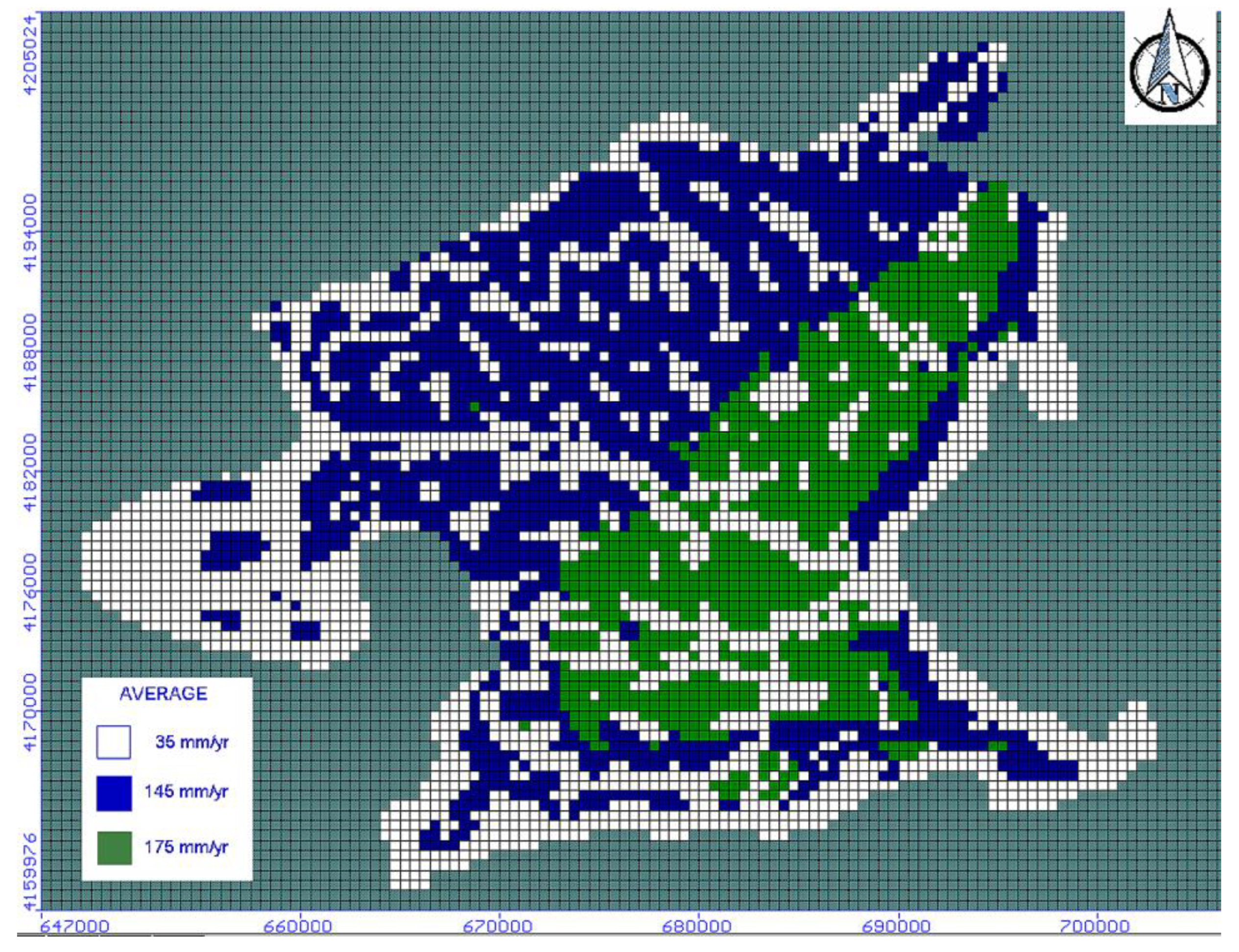
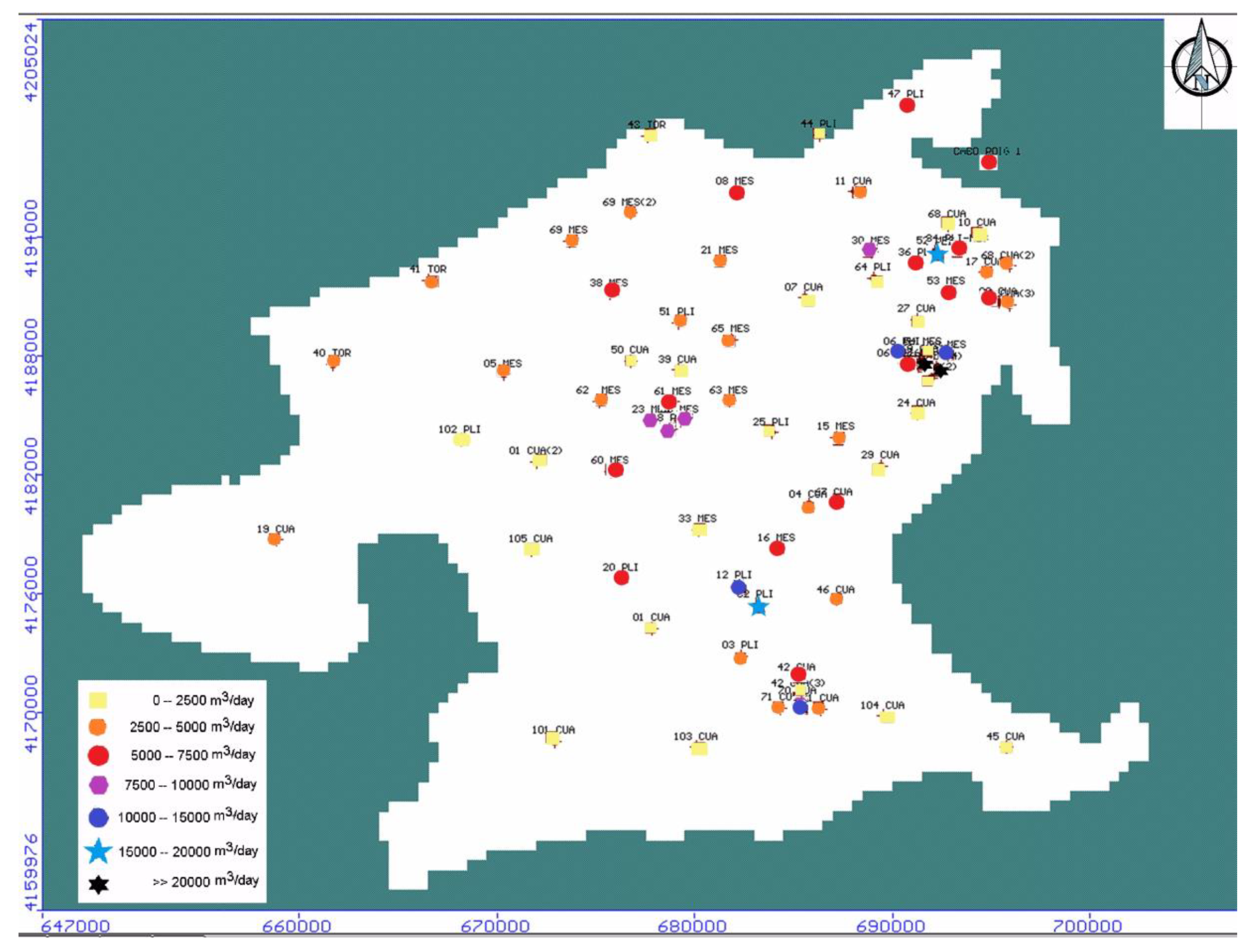
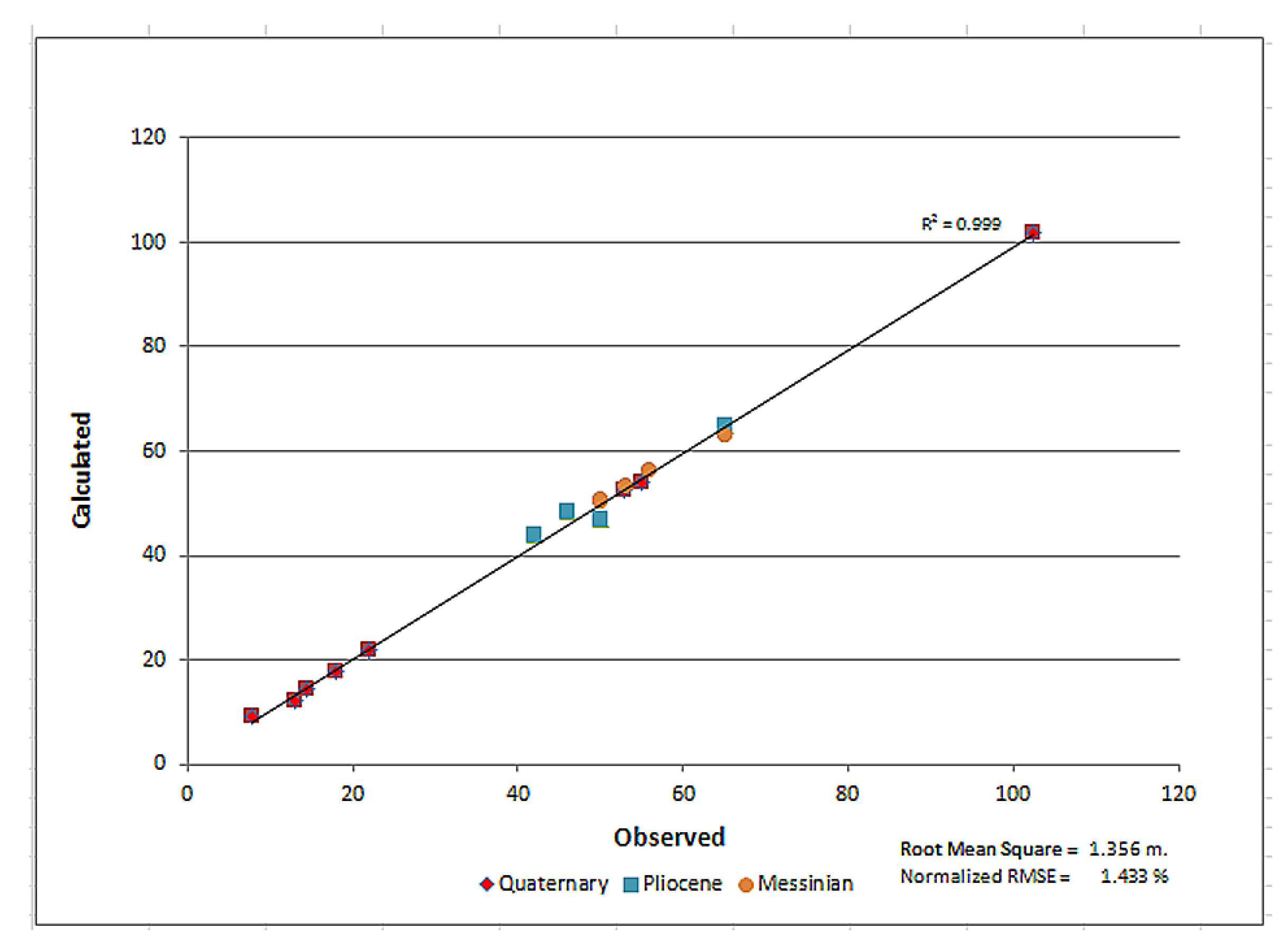
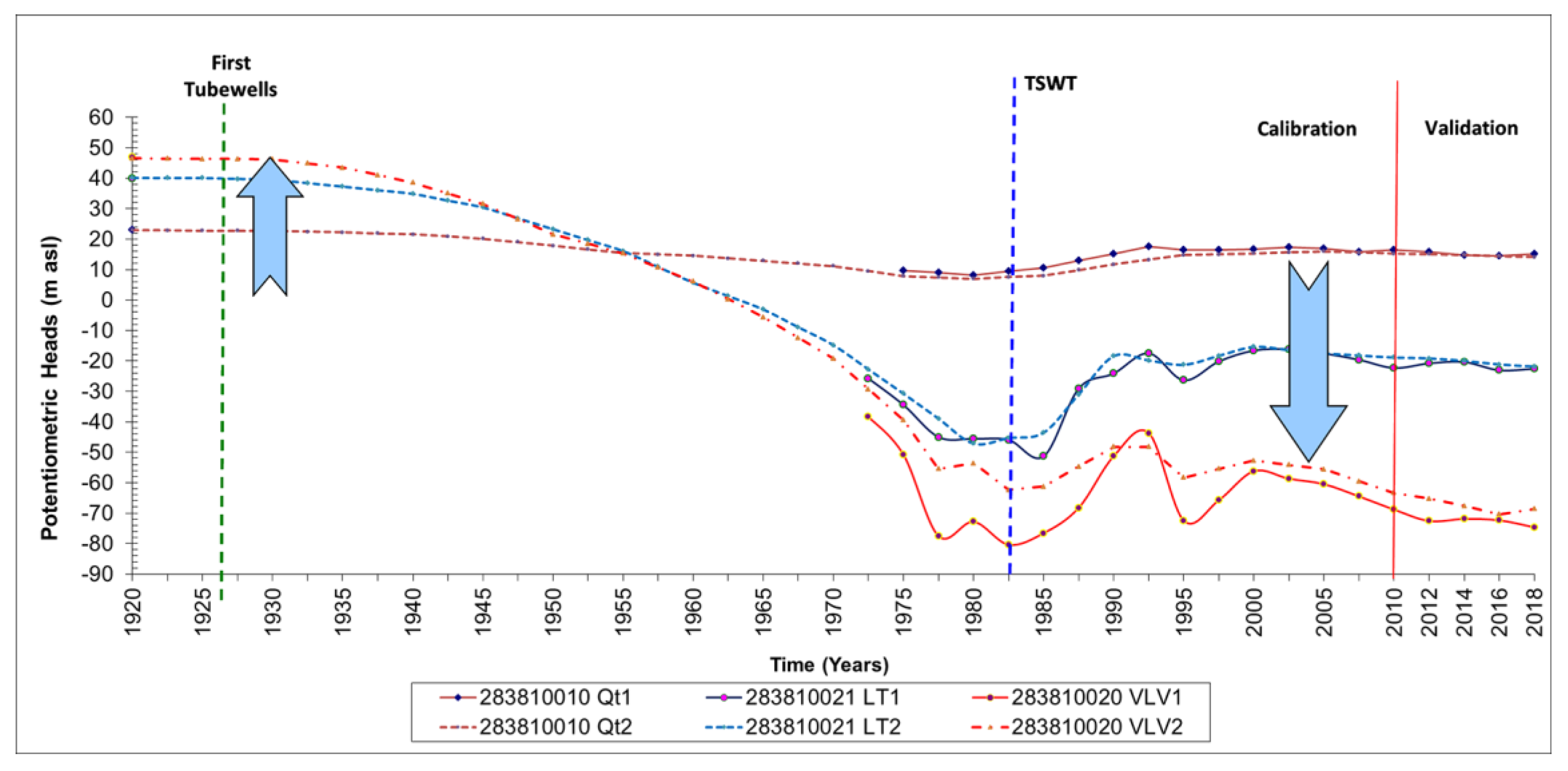

| Description | 1980 | 1991 | 1998 | 2004 | 2010 | 2015 |
|---|---|---|---|---|---|---|
| Irrigated Area (ha) | 14,521 | 23,911 | 34,328 | 39,254 | 42,253 | 43,071 |
| Irrigated Demand (Mm3/yr) | 94.39 | 155.42 | 223.14 | 223.75 | 270.50 | 258.40 |
| Irrigated Rate (m3/ha/yr) | 6500 | 6500 | 6500 | 5700 | 6500 | 6000 |
| Layers | Type | Total Surface | Outcrops Surface | Elevation | Thickness | Hydraulic Conductivity | Specific Storage | Specific Yield | Effective Porosity |
|---|---|---|---|---|---|---|---|---|---|
| (Inland Surface) | Top/Bottom | Average | Average | Average | Average | Average | |||
| km2 | km2 | m | m | m/day | m−1 | ||||
| 1 | Unconfined | 1187 (1135) | 1135 | 0/−55 | 55 | 1.5 × 102 | 4.0 × 10−3 | 0.200 | 0.230 |
| 2 | Confined | - | - | −55/−160 | 105 | 1.0 × 10−5 | 1.0 × 10−7 | 6.00 × 10−5 | 0.015 |
| 3 | Confined | 1265 (945) | 110 | −160/−225 | 65 | 5.5 × 102 | 4.2× 10−3 | 0.022 | 0.250 |
| 4 | Confined | - | - | −225/−325 | 100 | 1.0 × 10−5 | 1.0 × 10−7 | 6.00 × 10−5 | 0.015 |
| 5 | Confined | 1309 (851) | 63 | −325/−470 | 145 | 4.0 × 102 | 1.0 × 10−5 | 0.020 | 0.250 |
| 6 | Confined | - | - | −470/−610 | 140 | 1.0 × 10−5 | 1.0 × 10−7 | 6.00 × 10−5 | 0.015 |
| 7 | Confined | 460 (460) | 46 | −610/−725 | 115 | 2.0 × 102 | 3.5 × 10−5 | 0.035 | 0.230 |
| 8 | Confined | - | - | −725/−2000 | 1275 | 1.0 × 10−6 | 1.0 × 10−7 | 6.00 × 10−5 | 0.015 |
| Layers | K (m/day) (Min/Max) | Ss (1/m) (Min/Max) | P (Mm3/yr) (Average) |
|---|---|---|---|
| Quaternary | 0.01/40 | 1.2 × 10−3/2.4 × 10−3 | 22.2 |
| Pliocene | 0.01/35 | 2.0 × 10−4/3.4 × 10−4 | 24.6 |
| Messinian | 0.01/4 | 2.0 × 10−4/2.4 × 10−4 | 42.1 |
| Tortonian | 0.01/3.5 | 3.2 × 10−4 | 3.1 |
| Marls Aquitard | 3 × 10−5 | 5 × 10−7 |
| Layers | Inputs | Outputs | |||||
|---|---|---|---|---|---|---|---|
| R | V | L | MMD | MD | V | L | |
| Quaternary | 39.85 | 0 | 8.50 | 28.50 | 4.50 | 12.40 | 2.95 |
| Pliocene | 2.90 | 12.40 | 2.20 | 0 | 0 | 6.65 | 10.85 |
| Messinian | 2.65 | 6.65 | 0.50 | 0 | 0 | 1.55 | 8.25 |
| Tortonian | 1.60 | 1.55 | 0 | 0 | 0 | 0 | 3.15 |
| Σ | 47.00 | 20.60 | 11.20 | 28.50 | 4.50 | 20.60 | 25.20 |
| Layers | Inputs | Outputs | Global Balance | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| R | I | V | L | W | MMD | MD | L | V | ||
| Quaternary | 39.85 | 73.05 | 0 | 3.30 | 22.67 | 34.80 | 5.50 | 1.00 | 44.53 | +7.70 |
| Pliocene | 2.90 | 0 | 44.53 | 2.40 | 24.51 | 0 | 0 | 0.35 | 25.90 | −0.93 |
| Messinian | 2.65 | 0 | 25.90 | 7.65 | 40.21 | 0 | 0 | 0 | 2.00 | −6.01 |
| Tortonian | 1.60 | 0 | 2.00 | 0 | 3.07 | 0 | 0 | 0 | 0 | +0.53 |
| ∑ | 47.00 | 73.05 | 72.43 | 12.25 | 90.46 | 34.80 | 5.50 | 1.35 | 72.43 | +1.29 |
| Designation Transfer | Global Vertical Transfer (1) | Hydro-Geological Vertical Transfer (2) | Transfer in Areas With Boreholes (1-2) | Transfer in Areas Without Boreholes (3) | Transfer Through Boreholes (model) (1-2-3) | Modified Transfer Through Boreholes, IGME (1991) (Estimated) | Transfer Through Boreholes, IGME (1991) (Estimated) |
|---|---|---|---|---|---|---|---|
| Quaternary-Pliocene | 44.53 | 10.28 | 34.25 | 10.50 | 23.75 | 15.77 | 23.65 |
| Pliocene- Messinian | 25.90 | 4.64 | 21.26 | 5.45 | 15.81 | 18.92 | 28.38 |
| Messinian-Tortonian | 2.00 | 1.45 | 0.55 | 0.10 | 0.45 | 1.38 | 0 |
| ∑ | 16.37 | 40.01 | 36.07 | 52.03 |
| Concepts | IGME [30] | CHS [25] | Jiménez-Martínez et al. (2016) [3] | This Study | ||||
|---|---|---|---|---|---|---|---|---|
| Quaternary | Others | Global | Quaternary | Global | Quaternary | Others | ||
| Inputs | Net infiltration | 46 | 4 | 76 | 46 | - | 40 | 7 |
| Irrigation returns | 23 | 0 | 18 | 66 | - | 73 | 0 | |
| To other aquifers | - | - | - | - | - | 3 | 57 | |
| Total | 69 | 4 | 94 | 112 | 112 | 116 | 64 | |
| Outputs | Pumps | 2 | 19 | 88 | - | 77 | 23 | 68 |
| Lateral to sea (mainly Mar Menor) | 5 | 0 | 6 | 68 | 68 | 40 | 0 | |
| To other aquifers | 0 | 0 | 46 | 0 | 44 | 1 | ||
| Losses | 0 | 0 | 0 | 2 | 2 | 1 | 1 | |
| Total | 54 | 19 | 94 | 116 | 147 | 108 | 70 | |
| Balance | 15 | −15 | 0 | −4 | −35 | 8 | −6 | |
| Observations | This positive balance translated into elevations in the Quaternary’s piezometric level, thus causing drainage problems in the lower areas. | This was balanced. | This was balanced on average. There was overexploitation of the lower aquifers. The Triassic of Victories was not incorporated. | A positive balance in the Quaternary caused a rise in piezometric levels. The rest of the system was in balance, with slight overexploitation in the Messinian. | ||||
| Calculation procedures | Piezometry measured in 1998, with a gradient of 3 per thousand, 48 m2/day and 29 km of front. | Accepted the IGME value [30]. | The 60–40 distribution of the recharge hydrological model was according to previous articles. | The procedures were according to the hydrogeological flow model of the entire aquifer system. | ||||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Domingo-Pinillos, J.C.; Senent-Aparicio, J.; García-Aróstegui, J.L.; Baudron, P. Long Term Hydrodynamic Effects in a Semi-Arid Mediterranean Multilayer Aquifer: Campo de Cartagena in South-Eastern Spain. Water 2018, 10, 1320. https://doi.org/10.3390/w10101320
Domingo-Pinillos JC, Senent-Aparicio J, García-Aróstegui JL, Baudron P. Long Term Hydrodynamic Effects in a Semi-Arid Mediterranean Multilayer Aquifer: Campo de Cartagena in South-Eastern Spain. Water. 2018; 10(10):1320. https://doi.org/10.3390/w10101320
Chicago/Turabian StyleDomingo-Pinillos, Juan Carlos, Javier Senent-Aparicio, José Luis García-Aróstegui, and Paul Baudron. 2018. "Long Term Hydrodynamic Effects in a Semi-Arid Mediterranean Multilayer Aquifer: Campo de Cartagena in South-Eastern Spain" Water 10, no. 10: 1320. https://doi.org/10.3390/w10101320
APA StyleDomingo-Pinillos, J. C., Senent-Aparicio, J., García-Aróstegui, J. L., & Baudron, P. (2018). Long Term Hydrodynamic Effects in a Semi-Arid Mediterranean Multilayer Aquifer: Campo de Cartagena in South-Eastern Spain. Water, 10(10), 1320. https://doi.org/10.3390/w10101320







