Effect of Teleconnection Patterns on Changes in Water Temperature in Polish Lakes
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Lakes
2.2. Data
2.3. Analysis
3. Results
3.1. Correlations
3.1.1. NAO
3.1.2. AO
3.1.3. EA
3.1.4. EAWR
3.1.5. SCAND
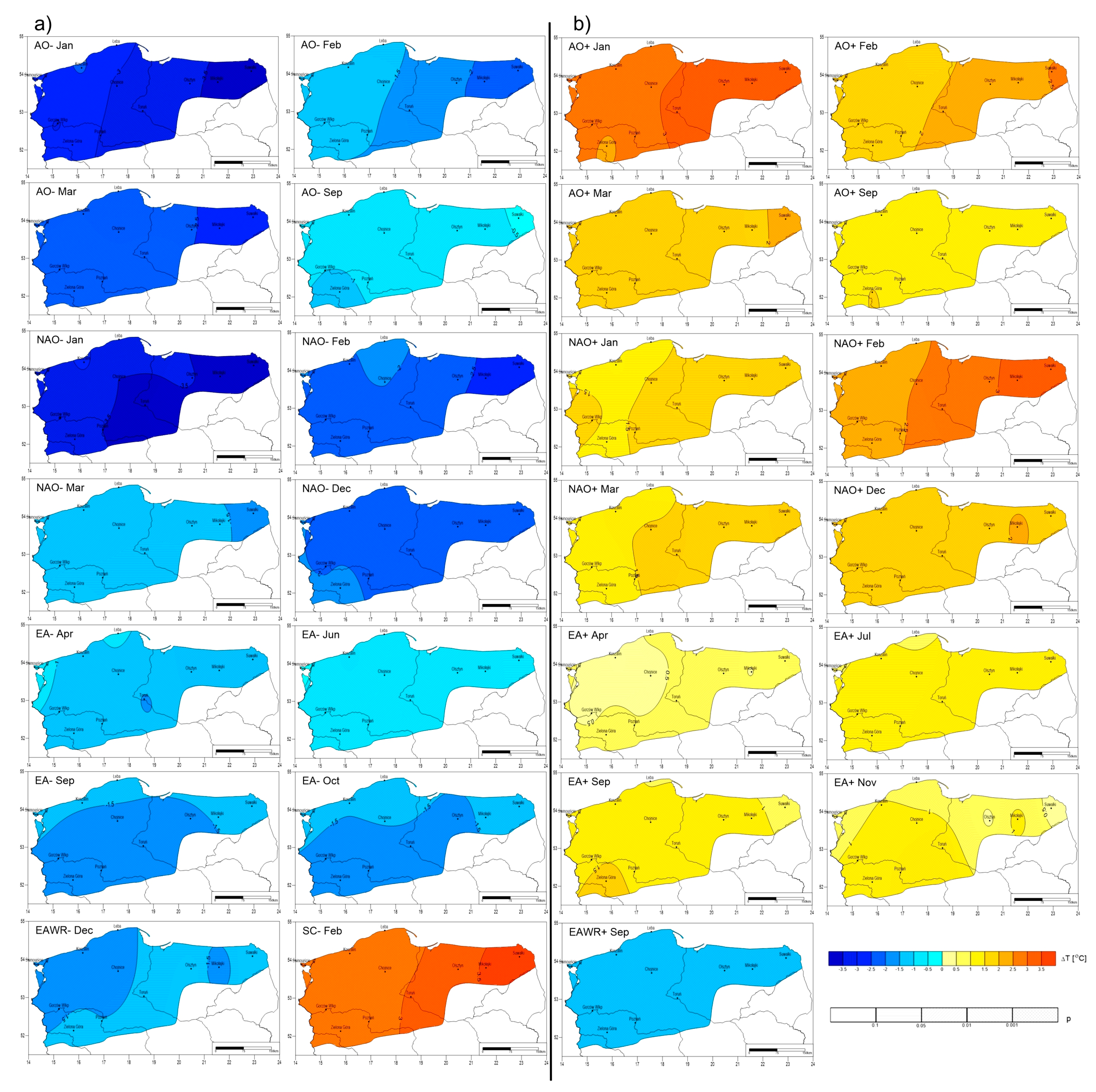
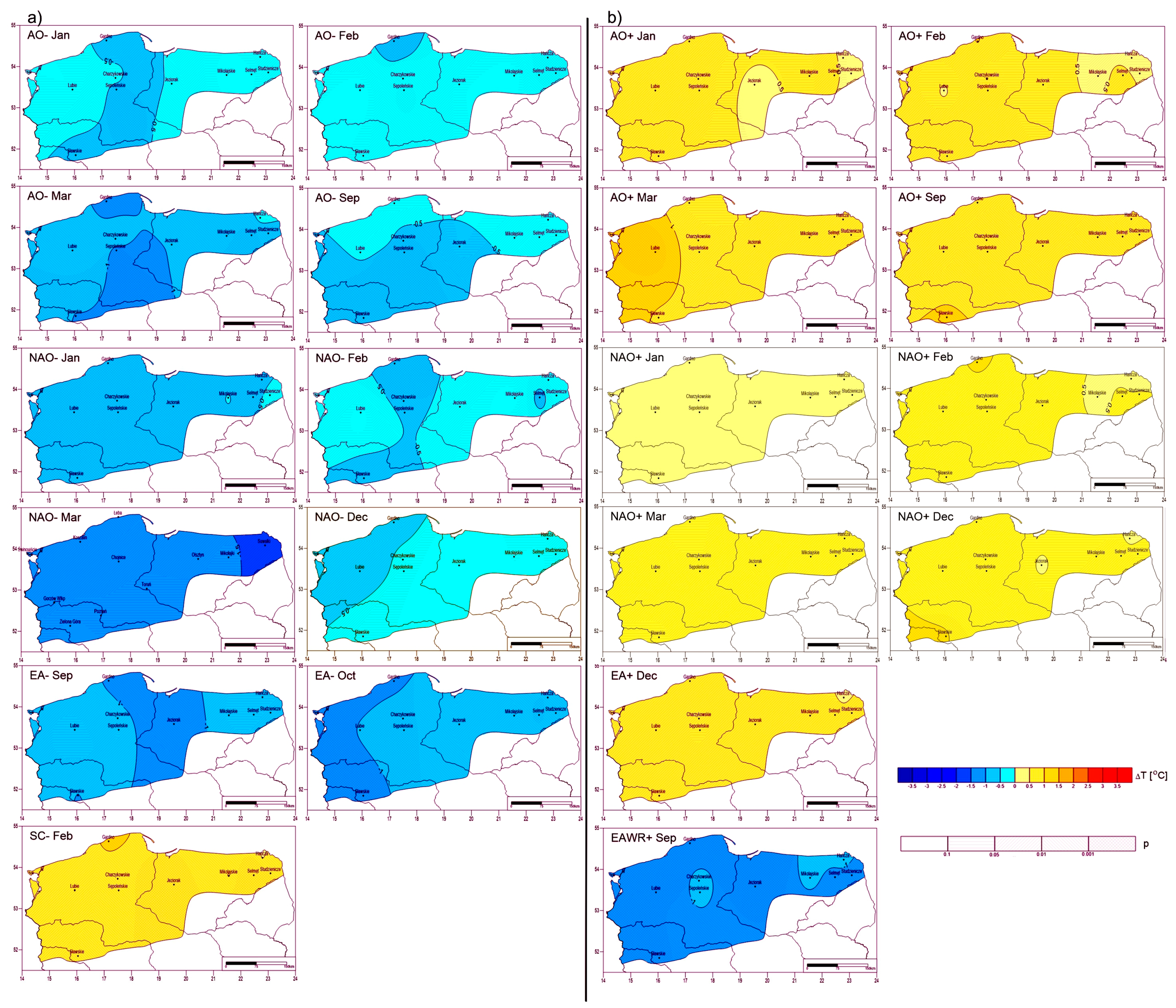
3.2. Differences in Air and Lake Water Temperature in Comparison to Mean Values in Different Phases of Macroscale Types of Atmospheric Circulation
3.2.1. NAO
3.2.2. AO
3.2.3. EA
3.2.4. EAWR
3.2.5. SCAND
4. Discussion and Summary
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Wrzesiński, D.; Ptak, M. Water level changes in Polish lakes during 1976–2010. J. Geogr. Sci. 2016, 26, 83–101. [Google Scholar] [CrossRef]
- Magee, M.R.; Wu, C.H. Effects of changing climate on ice cover in three morphometrically different lakes. Hydrol. Process. 2017, 31, 308–323. [Google Scholar] [CrossRef]
- Magee, M.R.; Wu, C.H.; Robertson, D.M.; Lathrop, R.C.; Hamilton, D.P. Trends and abrupt changes in 104 years of ice cover and water temperature in a dimictic lake in response to air temperature, wind speed, and water clarity drivers. Hydrol. Earth Syst. Sci. 2016, 20, 1681–1702. [Google Scholar] [CrossRef]
- Maher, O.A.; Uvo, C.B.; Bengtsson, L. Comparison between two extreme NAO winters and consequences on the thermal regime of Lake Vendyurskoe, Karelia. J. Hydrometeorol. 2005, 6, 775–783. [Google Scholar] [CrossRef]
- Bai, X.; Wang, J.; Sellinger, C.; Clites, A.; Assel, R. Interannual variability of Great Lakes ice cover and its relationship to NAO and ENSO. J. Geophys. Res. Oceans 2012, 117, 1–25. [Google Scholar] [CrossRef]
- Kucharski, F.; Parvin, A.; Rodriguez-Fonseca, B.; Farneti, R.; Martin-Rey, M.; Polo, I.; Mohino, E.; Losada, T.; Mechoso, C.R. The Teleconnection of the Tropical Atlantic to Indo-Pacific Sea Surface Temperatures on Inter-Annual to Centennial Time Scales: A Review of Recent Findings. Atmosphere 2016, 7, 29. [Google Scholar] [CrossRef]
- Zamrane, Z.; Turki, I.; Laignel, B.; Mahé, G.; Laftouhi, N.E. Characterization of the Interannual Variability of Precipitation and Streamflow in Tensift and Ksob Basins (Morocco) and Links with the NAO. Atmosphere 2016, 7, 84. [Google Scholar] [CrossRef]
- Wrzesiński, D.; Choiński, A.; Ptak, M.; Skowron, R. Effect of the North Atlantic Oscillation on the Pattern of Lake Ice Phenology in Poland. Acta Geophys. 2015, 63, 1664–1684. [Google Scholar] [CrossRef]
- Scaife, A.A.; Folland, C.K.; Alexander, L.V.; Moberg, A.; Knight, J.R. European climate extremes and the North Atlantic Oscillation. J. Clim. 2008, 21, 72–83. [Google Scholar] [CrossRef]
- Niedźwiedź, T.; Twardosz, R.; Walanus, A. Long-term variability of precipitation series in east central Europe in relation to circulation patterns. Theor. Appl. Climatol. 2009, 98, 337–350. [Google Scholar] [CrossRef]
- Bednorz, E. Synoptic conditions for rapid snowmelt in the Polish-German lowlands. Theor. Appl. Climatol. 2009, 97, 279–286. [Google Scholar] [CrossRef]
- Tomczyk, A.M. Impact of macro-scale circulation types on the occurrence of frosty days in Poland. Bull. Geogr. Phys. Geogr. Ser. 2015, 9, 55–65. [Google Scholar] [CrossRef]
- Pekarova, P.; Pekar, J. Teleconnections of Inter-Annual Streamflow Fluctuation in Slovakia with Arctic Oscillation, North Atlantic Oscillation, Southern Oscillation, and Quasi-Biennial Oscillation Phenomena. Adv. Atmos. Sci. 2007, 24, 655–663. [Google Scholar] [CrossRef]
- Croitoru, A.E.; Drignei, D.; Dragotă, C.S.; Imecs, Z.; Burada, D.C. Sharper detection of winter temperature changes in the Romanian higher-elevations. Glob. Planet. Chang. 2014, 122, 122–129. [Google Scholar] [CrossRef]
- Rust, H.W.; Richling, A.; Bissolli, P.; Ulbrich, U. Linking teleconnection patterns to European temperature—A multiple linear regression model. Meteorol. Z. 2015, 24, 411–423. [Google Scholar] [CrossRef]
- Steirou, E.; Gerlitz, L.; Apel, H.; Merz, B. Links between large-scale circulation patterns and streamflow in Central Europe: A review. J. Hydrol. 2017, 549, 484–500. [Google Scholar] [CrossRef]
- Antonopoulos, V.Z.; Gianniou, S.K. Simulation of water temperature and dissolvedoxygen distribution in Lake Vegoritis, Greece. Ecol. Model. 2003, 160, 39–53. [Google Scholar] [CrossRef]
- Ptak, M.; Nowak, B. Variability of oxygen-thermal conditions in selected lakes in Poland. Ecol. Chem. Eng. S 2016, 23, 639–650. [Google Scholar] [CrossRef]
- Pełechata, A.; Pełechaty, M.; Pukacz, A. Winter temperature and shifts in phytoplankton assemblages in a small Chara-lake. Aquat. Bot. 2015, 124, 10–18. [Google Scholar] [CrossRef]
- Messyasz, B.; Gabka, M.; Barylski, J.; Nowicki, G.; Lamentowicz, L.; Goździcka-Józefiak, A.; Rybak, A.; Dondajewska, R.; Burchardt, L. Phytoplankton, culturable bacteria and their relationships along environmental gradients in a stratified eutrophic lake. Carpathian J. Earth Environ. Sci. 2015, 10, 41–52. [Google Scholar]
- Sobczyński, T.; Joniak, T. The variability and stability of water chemistry in a deep temperate lake: Results of long-term study of eutrophication. Pol. J. Environ. Stud. 2013, 22, 227–237. [Google Scholar]
- Bonk, A.; Tylmann, W.; Amann, B.; Enters, D.; Grosjean, M. Modern limnology and varve-formation processes in lake Żabińskie, northeastern Poland: Comprehensive process studies as a key to understand the sediment record. J. Limnol. 2015, 74, 358–370. [Google Scholar] [CrossRef]
- Winder, M.; Schindler, D.E. Climatic effects on the phenology of lake processes. Glob. Chang. Biol. 2004, 10, 1844–1856. [Google Scholar] [CrossRef]
- Van Cleave, K.; Lenters, J.D.; Wang, J.; Verhamme, E.M. A regime shift in lake superior ice cover, Evaporation, And water temperature following the warm el niño winter of 1997–1998. Limnol. Oceanogr. 2014, 59, 1889–1898. [Google Scholar] [CrossRef]
- Molinero, J.C.; Anneville, O.; Souissi, S.; Lainé, L.; Gerdeaux, D. Decadal changes in water temperature and ecological time series in Lake Geneva, Europe—Relationship to subtropical Atlantic climate variability. Clim. Res. 2007, 34, 15–23. [Google Scholar] [CrossRef]
- Jyväsjärvi, J.; Hämäläinen, H. Profundal benthic invertebrate communities in boreal lakes vary with climate fluctuation. Aquat. Sci. 2015, 77, 261–269. [Google Scholar] [CrossRef]
- Straile, D. Meteorological forcing of plankton dynamics in a large and deep continental European lake. Oecologia 2000, 122, 44–50. [Google Scholar] [CrossRef] [PubMed]
- Livingstone, D.M.; Dokulil, M.T. Eighty years of spatially coherent Austrian lake surface temperatures and their relationship to regional air temperature and the North Atlantic Oscillation. Limnol. Oceanogr. 2001, 46, 1220–1227. [Google Scholar] [CrossRef]
- George, D.G.; Maberly, S.C.; Hewitt, D.P. The influence of the North Atlantic Oscillation on the physical, chemical and biological characteristics of four lakes in the English Lake District. Freshw. Biol. 2004, 49, 760–774. [Google Scholar] [CrossRef]
- Weyhenmeyer, G.A. Synchrony in relationships between the North Atlantic Oscillation and water chemistry among Sweden’s largest lakes. Limnol. Oceanogr. 2004, 49, 1191–1201. [Google Scholar] [CrossRef]
- Blenckner, T.; Adrian, R.; Livingstone, D.M.; Jennings, E.; Weyhenmeyer, G.A.; George, D.G.; Jankowski, T.; Jarvinen, M.; Aonghusa, C.N.; Noges, T.; et al. Large-scale climatic signatures in lakes across Europe: A meta-analysis. Glob. Chang. Biol. 2007, 13, 1314–1326. [Google Scholar] [CrossRef]
- Hernández, A.; Trigo, R.M.; Pla-Rabes, S.; Valero-Garcés, B.L.; Jerez, S.; Rico-Herrero, M.; Vega, J.C.; Jambrina-Enríquez, M.; Giralt, S. Sensitivity of two Iberian lakes to North Atlantic atmospheric circulation modes. Clim. Dyn. 2015, 45, 3403–3417. [Google Scholar] [CrossRef]
- Soja, A.M.; Soja, G. Relations between large scale oscillation patterns and rising water temperatures at Lake Neusiedl. Geophys. Res. Abstr. 2013, 15, EGU2013-5900-1. [Google Scholar]
- Salmaso, N. Influence of atmospheric modes of variability on a deep lake south of the Alps. Clim. Res. 2012, 51, 125–133. [Google Scholar] [CrossRef]
- Skowron, R. The Differentation and the Changeability of Choin Elements of the Thermal Regime of Water in Lakes on Polish Lowland; Wydawnictwo Naukowe Uniwersytetu Mikołaja Kopernika: Toruń, Poland, 2011; p. 346. [Google Scholar]
- Bednorz, E. Synoptic reasons for heavy snowfalls in the Polish-German lowlands. Theor. Appl. Climatol. 2008, 92, 133–140. [Google Scholar] [CrossRef]
- Koźmiński, C.; Michalska, B. Effect of the north Atlantic oscillation on extreme air temperatures at the Polish Baltic Coast. Acta Agrophysica 2010, 16, 79–91. [Google Scholar]
- Vitasse, Y.; Klein, G.; Kirchner, J.W.; Rebetez, M. Intensity, frequency and spatial configuration of winter temperature inversions in the closed La Brevine valley, Switzerland. Theoretical Appl. Climatol. 2017, 130, 1073–1083. [Google Scholar] [CrossRef]
- Barnston, A.G.; Livezey, R.E. Classification, seasonality and persistence of low frequency atmospheric circulation patterns. Mon. Weather Rev. 1987, 115, 1083–1126. [Google Scholar] [CrossRef]
- Woś, A. Climate of Poland in the Second Half of the 20th Century; Wydawnictwo Naukowe Uniwersytetu im. Adama Mickiewicza: Poznań, Poland, 2010. [Google Scholar]
- Gerten, D.; Adrian, R. Differences in the persistency of the North Atlantic Oscillation signal among lakes. Limnol. Oceanogr. 2001, 46, 448–455. [Google Scholar] [CrossRef]
- Livingstone, D.M. Impact of secular climate change on the thermal structure of a large temperate central European lake. Clim. Chang. 2003, 57, 205–225. [Google Scholar] [CrossRef]
- Choiński, A.; Ptak, M.; Skowron, R.; Strzelczak, A. Changes in ice phenology on Polish lakes from 1961–2010 related to location and morphometry. Limnologica 2015, 53, 42–49. [Google Scholar] [CrossRef]
- Mishra, V.; Cherkauer, K.A.; Bowling, L.C. Changing thermal dynamics of lakes in the Great Lakes region: Role of ice cover feedbacks. Glob. Planet. Chang. 2011, 75, 155–172. [Google Scholar] [CrossRef]
- Cydzik, D.; Soszka, H. Atlas Stanu Czystości jezior Polski Badanych w Latach 1979–1983; Instytut Ochrony Środowiska: Warszawa, Poland, 1988. [Google Scholar]
- Wrzesiński, D.; Choiński, A.; Ptak, M. Effect of North Atlantic Oscillation on the hydrological conditions of Lake Morskie Oko (Carphatian Mountains). Bull. Geogr. Phys. Geogr. Ser. 2016, 10, 95–105. [Google Scholar] [CrossRef]
- Girjatowicz, J.P. Effects of the North Atlantic Oscillation on water temperature in southern Baltic coastal lakes. Ann. Limnol. 2011, 47, 73–84. [Google Scholar] [CrossRef]
- Wrzesiński, D.; Choiński, A.; Ptak, M. Effect of the North Atlantic Oscillation on the thermal characteristics of lakes in Poland. Acta Geophys. 2015, 63, 863–883. [Google Scholar] [CrossRef]
- Trusewicz, Z.; Markowski, M.; Barańczuk, J. The influence of the North Atlantic Oscillation on variability of surface temperature of Lake Raduńskie Górne. Limnol. Rev. 2009, 9, 121–128. [Google Scholar]
- Sharov, A.N.; Berezina, N.A.; Nazarova, L.E.; Poliakova, T.N.; Chekryzheva, T.A. Links between biota and climate-related variables in the Baltic region using Lake Onega as an example. Oceanologia 2014, 56, 291–306. [Google Scholar] [CrossRef]
- Bonsal, B.R.; Prowse, T.D.; Duguay, C.R.; Lacroix, M.P. Impacts of large-scale teleconnections on freshwater-ice break/freeze-up dates over Canada. J. Hydrol. 2006, 330, 340–353. [Google Scholar] [CrossRef]
- Soja, G.; Züger, J.; Knoflacher, M.; Kinner, P.; Soja, A.-M. Climate impacts on water balance of a shallow steppe lake in Eastern Austria (Lake Neusiedl). J. Hydrol. 2013, 480, 115–124. [Google Scholar] [CrossRef]
- Kowalczak, P.; Farat, R.; Kepińska-Kasprzak, M.; Kuźnicka, M.; Mager, P. Hierarchia Potrzeb Obszarowych Małej Retencji; Materiały badawcze, Seria, Gospodarka Wodna a Ochrona Wód. 19; IMGW: Warszawa, Poland, 1997. [Google Scholar]
- Choiński, A.; Ławniczak, A.; Ptak, M. Changes in water resources of Polish lakes as influenced by natural and anthropogenic factors. Pol. J. Environ. Stud. 2016, 25, 1883–1890. [Google Scholar] [CrossRef]
- Ptak, M.; Choiński, A.; Strzelczak, A.; Targosz, A. Disappearance of Lake Jelenino since the end of the XVIII century as an effect of anthropogenic transformations of the natural environment. Pol. J. Environ. Stud. 2013, 22, 191–196. [Google Scholar]

| No. | Lake | Area (ha) | Volume (thous.m3) | Depth (m) | |
|---|---|---|---|---|---|
| Average | Max | ||||
| 1 | Sławskie | 822.5 | 42,664.8 | 5.2 | 12.3 |
| 2 | Lubie | 1487.5 | 169,880.5 | 11.6 | 46.2 |
| 3 | Sępoleńskie | 157.5 | 7501.6 | 4.8 | 10.9 |
| 4 | Charzykowskie | 1336 | 134,533.2 | 9.8 | 30.5 |
| 5 | Gardno | 2337.5 | 30,950.5 | 1.3 | 2.6 |
| 6 | Jeziorak | 3152.5 | 141,594.2 | 4.1 | 12.9 |
| 7 | Mikołajskie | 424 | 55,739.7 | 11.2 | 25.9 |
| 8 | Selmęt Wielki | 1207.5 | 99,463.9 | 7.8 | 21.9 |
| 9 | Studzieniczne | 244 | 22,073.6 | 8.7 | 30.5 |
| 10 | Hańcza | 291.5 | 120,364.1 | 38.7 | 106.1 |
| (a) | ||||||||||||||
| Month | January | February | March | April | May | June | July | August | September | October | November | December | ||
| Meteorological Station | ||||||||||||||
| NAO | Chojnice | 0.65 | 0.47 | 0.52 | 0.17 | 0.13 | 0.22 | 0.16 | 0.11 | 0.22 | 0.20 | 0.25 | 0.66 | |
| Gorzów Wlkp | 0.69 | 0.49 | 0.49 | 0.23 | 0.08 | 0.08 | 0.21 | 0.12 | 0.23 | 0.17 | 0.26 | 0.70 | ||
| Koszalin | 0.64 | 0.53 | 0.55 | 0.26 | 0.19 | 0.16 | 0.12 | 0.07 | 0.23 | 0.21 | 0.31 | 0.64 | ||
| Łeba | 0.62 | 0.49 | 0.54 | 0.17 | 0.11 | 0.24 | 0.12 | 0.04 | 0.23 | 0.22 | 0.31 | 0.59 | ||
| Mikołajki | 0.65 | 0.52 | 0.43 | 0.09 | 0.03 | 0.15 | 0.09 | 0.08 | 0.19 | 0.20 | 0.16 | 0.65 | ||
| Olsztyn | 0.66 | 0.52 | 0.46 | 0.10 | 0.04 | 0.20 | 0.11 | 0.01 | 0.19 | 0.24 | −0.11 | 0.47 | ||
| Poznań | 0.69 | 0.49 | 0.47 | 0.16 | 0.08 | 0.04 | 0.16 | 0.02 | 0.19 | 0.16 | 0.19 | 0.68 | ||
| Suwałki | 0.61 | 0.52 | 0.50 | 0.02 | 0.00 | 0.16 | 0.04 | 0.08 | 0.16 | 0.13 | −0.14 | 0.47 | ||
| Świnoujście | 0.68 | 0.50 | 0.55 | 0.30 | 0.09 | 0.24 | 0.19 | 0.13 | 0.27 | 0.24 | 0.32 | 0.65 | ||
| Toruń | 0.67 | 0.52 | 0.45 | 0.17 | 0.10 | 0.23 | 0.13 | 0.04 | 0.20 | 0.10 | 0.16 | 0.65 | ||
| Zielona Góra | 0.66 | 0.47 | 0.41 | 0.21 | 0.08 | 0.04 | 0.17 | 0.07 | 0.26 | 0.18 | 0.19 | 0.58 | ||
| AO | Chojnice | 0.71 | 0.42 | 0.54 | 0.04 | 0.28 | 0.18 | 0.23 | 0.25 | 0.47 | 0.37 | 0.33 | 0.54 | |
| Gorzów Wlkp | 0.67 | 0.42 | 0.51 | 0.05 | 0.30 | 0.06 | 0.21 | 0.27 | 0.55 | 0.35 | 0.29 | 0.54 | ||
| Koszalin | 0.69 | 0.42 | 0.53 | 0.08 | 0.34 | 0.15 | 0.21 | 0.19 | 0.50 | 0.35 | 0.34 | 0.51 | ||
| Łeba | 0.73 | 0.44 | 0.57 | 0.10 | 0.41 | 0.19 | 0.25 | 0.18 | 0.49 | 0.40 | 0.37 | 0.56 | ||
| Mikołajki | 0.68 | 0.46 | 0.52 | 0.10 | 0.19 | 0.11 | 0.21 | 0.22 | 0.46 | 0.37 | 0.31 | 0.52 | ||
| Olsztyn | 0.70 | 0.47 | 0.55 | 0.06 | 0.15 | 0.17 | 0.22 | 0.21 | 0.48 | 0.41 | 0.15 | 0.41 | ||
| Poznań | 0.65 | 0.41 | 0.49 | 0.03 | 0.27 | 0.00 | 0.18 | 0.20 | 0.50 | 0.30 | 0.24 | 0.52 | ||
| Suwałki | 0.68 | 0.47 | 0.57 | 0.09 | 0.09 | 0.13 | 0.21 | 0.22 | 0.42 | 0.33 | 0.13 | 0.43 | ||
| Świnoujście | 0.68 | 0.45 | 0.56 | 0.19 | 0.37 | 0.22 | 0.23 | 0.26 | 0.50 | 0.37 | 0.34 | 0.54 | ||
| Toruń | 0.67 | 0.43 | 0.51 | 0.10 | 0.25 | 0.20 | 0.21 | 0.25 | 0.48 | 0.35 | 0.25 | 0.50 | ||
| Zielona Góra | 0.64 | 0.41 | 0.47 | 0.10 | 0.30 | 0.03 | 0.14 | 0.25 | 0.52 | 0.32 | 0.27 | 0.41 | ||
| EA | Chojnice | 0.27 | 0.36 | 0.28 | 0.43 | 0.29 | 0.19 | 0.31 | 0.33 | 0.63 | 0.57 | 0.53 | 0.04 | |
| Gorzów Wlkp | 0.24 | 0.37 | 0.35 | 0.42 | 0.30 | 0.17 | 0.22 | 0.34 | 0.69 | 0.57 | 0.55 | 0.07 | ||
| Koszalin | 0.26 | 0.33 | 0.26 | 0.36 | 0.34 | 0.27 | 0.37 | 0.38 | 0.64 | 0.56 | 0.48 | 0.09 | ||
| Łeba | 0.30 | 0.31 | 0.23 | 0.45 | 0.37 | 0.25 | 0.39 | 0.32 | 0.61 | 0.50 | 0.41 | –0.04 | ||
| Mikołajki | 0.27 | 0.32 | 0.25 | 0.39 | 0.23 | 0.13 | 0.44 | 0.33 | 0.60 | 0.53 | 0.53 | 0.04 | ||
| Olsztyn | 0.28 | 0.32 | 0.30 | 0.48 | 0.26 | 0.17 | 0.41 | 0.40 | 0.62 | 0.57 | 0.19 | 0.06 | ||
| Poznań | 0.27 | 0.41 | 0.34 | 0.48 | 0.34 | 0.17 | 0.29 | 0.38 | 0.70 | 0.60 | 0.58 | 0.11 | ||
| Suwałki | 0.20 | 0.31 | 0.24 | 0.43 | 0.13 | 0.10 | 0.48 | 0.32 | 0.54 | 0.52 | 0.16 | −0.03 | ||
| Świnoujście | 0.22 | 0.31 | 0.34 | 0.48 | 0.42 | 0.21 | 0.27 | 0.27 | 0.63 | 0.57 | 0.42 | 0.00 | ||
| Toruń | 0.26 | 0.36 | 0.36 | 0.48 | 0.31 | 0.21 | 0.32 | 0.41 | 0.64 | 0.57 | 0.61 | 0.07 | ||
| Zielona Góra | 0.24 | 0.44 | 0.35 | 0.49 | 0.33 | 0.14 | 0.16 | 0.32 | 0.72 | 0.60 | 0.53 | 0.11 | ||
| EAWR | Chojnice | 0.07 | 0.03 | 0.10 | 0.07 | 0.02 | −0.15 | 0.16 | 0.12 | −0.41 | −0.05 | −0.18 | 0.29 | |
| Gorzów Wlkp | 0.03 | −0.03 | 0.09 | 0.15 | 0.09 | 0.00 | 0.20 | 0.11 | −0.31 | −0.06 | −0.16 | 0.30 | ||
| Koszalin | 0.09 | −0.04 | 0.08 | 0.08 | 0.01 | −0.04 | 0.19 | 0.03 | −0.34 | −0.06 | −0.13 | 0.31 | ||
| Łeba | 0.09 | 0.00 | 0.01 | 0.03 | −0.16 | −0.16 | 0.16 | −0.03 | −0.43 | −0.02 | −0.16 | 0.39 | ||
| Mikołajki | 0.06 | −0.02 | 0.05 | −0.16 | −0.16 | −0.20 | 0.06 | 0.04 | −0.44 | −0.15 | −0.30 | 0.25 | ||
| Olsztyn | 0.02 | −0.01 | 0.13 | −0.14 | −0.18 | −0.18 | 0.05 | 0.04 | −0.44 | −0.05 | −0.36 | 0.17 | ||
| Poznań | 0.01 | −0.06 | 0.13 | 0.02 | 0.05 | 0.05 | 0.14 | 0.09 | −0.37 | −0.10 | −0.23 | 0.25 | ||
| Suwałki | 0.11 | −0.03 | 0.11 | −0.18 | −0.23 | −0.26 | 0.01 | −0.01 | −0.50 | −0.18 | −0.39 | 0.14 | ||
| Świnoujście | 0.07 | 0.01 | 0.01 | 0.14 | 0.00 | −0.12 | 0.26 | 0.06 | −0.37 | 0.01 | −0.16 | 0.38 | ||
| Toruń | 0.00 | −0.05 | 0.11 | −0.04 | −0.03 | −0.13 | 0.13 | 0.09 | −0.39 | −0.10 | −0.23 | 0.25 | ||
| Zielona Góra | 0.01 | −0.01 | 0.14 | 0.14 | 0.22 | 0.13 | 0.28 | 0.13 | −0.33 | −0.03 | −0.18 | 0.23 | ||
| SCAND | Chojnice | −0.42 | −0.49 | −0.23 | 0.28 | 0.33 | 0.15 | 0.17 | 0.09 | 0.10 | 0.25 | 0.16 | 0.00 | |
| Gorzów Wlkp | −0.38 | −0.49 | −0.28 | 0.22 | 0.17 | −0.07 | 0.12 | 0.00 | 0.01 | 0.25 | 0.14 | −0.06 | ||
| Koszalin | −0.41 | −0.47 | −0.23 | 0.22 | 0.33 | 0.08 | 0.18 | 0.14 | 0.12 | 0.27 | 0.17 | −0.02 | ||
| Łeba | −0.42 | −0.49 | −0.23 | 0.18 | 0.20 | 0.10 | 0.25 | 0.12 | 0.03 | 0.21 | 0.12 | −0.03 | ||
| Mikołajki | −0.43 | −0.51 | −0.36 | 0.24 | 0.40 | 0.24 | 0.23 | 0.10 | 0.07 | 0.18 | 0.17 | −0.06 | ||
| Olsztyn | −0.45 | −0.50 | −0.19 | 0.25 | 0.46 | 0.23 | 0.19 | 0.10 | 0.02 | 0.17 | 0.18 | −0.03 | ||
| Poznań | −0.35 | −0.46 | −0.25 | 0.21 | 0.23 | −0.10 | 0.15 | 0.03 | 0.07 | 0.30 | 0.21 | −0.02 | ||
| Suwałki | −0.50 | −0.47 | −0.21 | 0.26 | 0.46 | 0.30 | 0.23 | 0.15 | 0.09 | 0.14 | 0.16 | −0.08 | ||
| Świnoujście | −0.40 | −0.51 | −0.36 | 0.07 | 0.03 | −0.02 | 0.17 | 0.12 | 0.12 | 0.27 | 0.11 | −0.05 | ||
| Toruń | −0.42 | −0.48 | −0.27 | 0.22 | 0.31 | 0.07 | 0.17 | 0.09 | 0.06 | 0.17 | 0.19 | −0.07 | ||
| Zielona Góra | −0.34 | −0.44 | −0.29 | 0.18 | 0.11 | −0.16 | 0.07 | −0.05 | 0.02 | 0.28 | 0.12 | 0.05 | ||
| (b) | ||||||||||||||
| Month | January | February | March | April | May | June | July | August | September | October | November | December | ||
| Lakes | ||||||||||||||
| NAO | Sławskie | 0.62 | 0.54 | 0.43 | 0.18 | −0.04 | 0.06 | 0.09 | −0.04 | 0.31 | 0.04 | 0.21 | 0.47 | |
| Lubie | 0.44 | 0.46 | 0.37 | 0.32 | −0.06 | 0.08 | −0.04 | −0.13 | 0.21 | −0.04 | 0.13 | 0.41 | ||
| Gardno | 0.57 | 0.57 | 0.44 | 0.12 | 0.18 | 0.24 | 0.13 | −0.05 | 0.27 | 0.23 | 0.28 | 0.53 | ||
| Sępoleński | 0.54 | 0.42 | 0.49 | 0.20 | −0.07 | 0.16 | 0.08 | 0.08 | 0.27 | −0.06 | 0.18 | 0.43 | ||
| Charzykowskie | 0.62 | 0.44 | 0.41 | 0.26 | −0.03 | 0.11 | 0.08 | 0.07 | 0.31 | −0.03 | 0.29 | 0.40 | ||
| Jeziorak | 0.39 | 0.29 | 0.48 | 0.13 | −0.03 | 0.12 | 0.08 | 0.07 | 0.14 | −0.03 | 0.28 | 0.36 | ||
| Mikołajskie | 0.52 | 0.32 | 0.41 | 0.11 | −0.02 | 0.14 | 0.03 | −0.04 | 0.26 | −0.14 | 0.19 | 0.27 | ||
| Studzieniczne | 0.39 | 0.38 | 0.34 | 0.03 | −0.04 | 0.13 | 0.02 | −0.06 | 0.31 | −0.15 | 0.07 | 0.34 | ||
| Selmęt Wielki | 0.48 | 0.50 | 0.52 | 0.01 | 0.00 | 0.19 | 0.05 | −0.18 | 0.30 | −0.13 | 0.14 | 0.29 | ||
| Hańcza | 0.46 | 0.39 | 0.44 | 0.15 | −0.10 | 0.07 | 0.15 | 0.04 | 0.23 | −0.06 | 0.23 | 0.34 | ||
| AO | Sławskie | 0.54 | 0.57 | 0.53 | 0.34 | 0.32 | 0.04 | 0.10 | 0.24 | 0.56 | 0.18 | 0.30 | 0.26 | |
| Lubie | 0.36 | 0.50 | 0.48 | 0.33 | 0.37 | 0.08 | 0.17 | 0.16 | 0.38 | 0.13 | 0.29 | 0.33 | ||
| Gardno | 0.62 | 0.50 | 0.49 | 0.17 | 0.37 | 0.20 | 0.15 | 0.04 | 0.34 | 0.35 | 0.35 | 0.48 | ||
| Sępoleński | 0.59 | 0.44 | 0.50 | 0.26 | 0.27 | 0.11 | 0.21 | 0.25 | 0.43 | 0.07 | 0.23 | 0.38 | ||
| Charzykowskie | 0.51 | 0.47 | 0.48 | 0.30 | 0.23 | 0.04 | 0.11 | 0.22 | 0.47 | 0.01 | 0.36 | 0.40 | ||
| Jeziorak | 0.45 | 0.36 | 0.52 | 0.21 | 0.26 | 0.06 | 0.18 | 0.25 | 0.42 | 0.17 | 0.29 | 0.42 | ||
| Mikołajskie | 0.46 | 0.37 | 0.44 | 0.27 | 0.30 | 0.09 | 0.24 | 0.10 | 0.41 | −0.02 | 0.25 | 0.26 | ||
| Studzieniczne | 0.39 | 0.43 | 0.43 | 0.22 | 0.24 | 0.06 | 0.21 | 0.08 | 0.34 | −0.10 | 0.28 | 0.27 | ||
| Selmęt Wielki | 0.44 | 0.54 | 0.54 | 0.24 | 0.28 | 0.11 | 0.21 | 0.05 | 0.43 | 0.00 | 0.24 | 0.24 | ||
| Hańcza | 0.43 | 0.44 | 0.44 | 0.31 | 0.26 | 0.06 | 0.25 | 0.16 | 0.41 | 0.00 | 0.22 | 0.27 | ||
| EA | Sławskie | 0.23 | 0.33 | 0.32 | 0.26 | 0.33 | 0.17 | 0.21 | 0.43 | 0.48 | 0.45 | 0.17 | 0.23 | |
| Lubie | 0.41 | 0.23 | 0.24 | 0.37 | 0.30 | 0.23 | 0.35 | 0.42 | 0.39 | 0.45 | 0.19 | 0.18 | ||
| Gardno | 0.43 | 0.27 | 0.30 | 0.21 | 0.26 | 0.13 | 0.39 | 0.24 | 0.45 | 0.47 | 0.42 | 0.19 | ||
| Sępoleński | 0.23 | 0.06 | 0.14 | 0.10 | 0.28 | 0.10 | 0.27 | 0.31 | 0.37 | 0.41 | 0.14 | 0.14 | ||
| Charzykowskie | 0.30 | 0.26 | 0.23 | 0.25 | 0.20 | 0.07 | 0.27 | 0.27 | 0.52 | 0.37 | 0.19 | 0.21 | ||
| Jeziorak | 0.20 | −0.03 | 0.29 | 0.12 | 0.12 | 0.18 | 0.37 | 0.44 | 0.49 | 0.41 | 0.26 | 0.27 | ||
| Mikołajskie | 0.31 | 0.21 | 0.19 | 0.16 | 0.19 | 0.21 | 0.47 | 0.40 | 0.37 | 0.34 | 0.15 | 0.10 | ||
| Studzieniczne | 0.44 | 0.18 | 0.24 | 0.24 | 0.09 | 0.14 | 0.45 | 0.41 | 0.36 | 0.22 | 0.24 | 0.21 | ||
| Selmęt Wielki | 0.50 | 0.13 | 0.16 | 0.22 | 0.17 | 0.14 | 0.50 | 0.50 | 0.33 | 0.31 | 0.20 | 0.26 | ||
| Hańcza | 0.19 | 0.19 | 0.12 | 0.13 | 0.05 | 0.11 | 0.40 | 0.28 | 0.32 | 0.44 | 0.11 | 0.09 | ||
| EAWR | Sławskie | 0.00 | 0.08 | −0.01 | 0.20 | 0.13 | −0.03 | 0.21 | 0.01 | −0.34 | −0.19 | −0.23 | 0.15 | |
| Lubie | −0.22 | 0.10 | 0.12 | 0.24 | 0.08 | −0.22 | 0.21 | 0.01 | −0.23 | −0.29 | −0.14 | 0.26 | ||
| Gardno | 0.04 | 0.11 | −0.05 | 0.01 | 0.04 | −0.23 | 0.16 | −0.01 | −0.37 | −0.13 | −0.15 | 0.30 | ||
| Sępoleński | 0.00 | 0.08 | −0.06 | 0.17 | 0.18 | −0.16 | 0.26 | 0.03 | −0.30 | −0.40 | −0.10 | 0.09 | ||
| Charzykowskie | 0.04 | 0.06 | 0.16 | 0.05 | 0.08 | −0.07 | 0.24 | −0.06 | −0.30 | −0.34 | −0.21 | 0.23 | ||
| Jeziorak | 0.04 | 0.26 | 0.06 | 0.11 | −0.04 | −0.02 | 0.16 | −0.13 | −0.37 | −0.33 | −0.08 | 0.30 | ||
| Mikołajskie | 0.10 | 0.18 | 0.01 | −0.01 | 0.00 | −0.08 | 0.05 | −0.16 | −0.37 | −0.37 | −0.16 | 0.08 | ||
| Studzieniczne | −0.09 | 0.04 | 0.01 | −0.07 | −0.14 | −0.14 | −0.01 | −0.12 | −0.45 | −0.45 | −0.16 | 0.12 | ||
| Selmęt Wielki | −0.04 | 0.20 | 0.00 | 0.00 | 0.00 | −0.15 | 0.01 | −0.18 | −0.37 | −0.30 | −0.24 | 0.00 | ||
| Hańcza | −0.24 | 0.10 | 0.15 | 0.05 | 0.06 | −0.17 | 0.08 | 0.02 | −0.30 | −0.35 | −0.22 | 0.26 | ||
| SCAND | Sławskie | −0.39 | −0.43 | −0.46 | −0.01 | −0.07 | −0.15 | 0.12 | −0.13 | 0.03 | 0.20 | 0.08 | 0.21 | |
| Lubie | −0.21 | −0.50 | −0.59 | 0.02 | −0.05 | −0.18 | 0.07 | 0.02 | 0.22 | 0.18 | 0.02 | 0.18 | ||
| Gardno | −0.32 | −0.47 | −0.36 | 0.10 | 0.16 | 0.26 | 0.34 | 0.23 | 0.16 | 0.17 | 0.20 | 0.19 | ||
| Sępoleński | −0.32 | −0.46 | −0.25 | 0.06 | 0.07 | −0.01 | 0.18 | −0.02 | 0.14 | 0.14 | 0.12 | 0.17 | ||
| Charzykowskie | −0.12 | −0.46 | −0.42 | −0.14 | 0.16 | 0.09 | 0.23 | 0.04 | 0.15 | 0.22 | 0.06 | 0.28 | ||
| Jeziorak | −0.21 | −0.62 | −0.25 | 0.18 | 0.30 | 0.12 | 0.17 | −0.11 | 0.23 | 0.21 | 0.16 | 0.03 | ||
| Mikołajskie | −0.27 | −0.45 | −0.40 | 0.04 | 0.26 | 0.16 | 0.18 | 0.03 | 0.17 | 0.16 | 0.10 | 0.20 | ||
| Studzieniczne | −0.18 | −0.49 | −0.29 | 0.05 | 0.22 | 0.16 | 0.31 | 0.15 | 0.17 | 0.14 | 0.12 | 0.25 | ||
| Selmęt Wielki | −0.25 | −0.64 | −0.36 | 0.05 | 0.23 | 0.10 | 0.22 | 0.02 | 0.16 | 0.10 | 0.08 | 0.22 | ||
| Hańcza | −0.21 | −0.38 | −0.39 | −0.04 | 0.02 | 0.04 | 0.28 | 0.12 | 0.15 | 0.15 | 0.09 | 0.09 | ||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ptak, M.; Tomczyk, A.M.; Wrzesiński, D. Effect of Teleconnection Patterns on Changes in Water Temperature in Polish Lakes. Atmosphere 2018, 9, 66. https://doi.org/10.3390/atmos9020066
Ptak M, Tomczyk AM, Wrzesiński D. Effect of Teleconnection Patterns on Changes in Water Temperature in Polish Lakes. Atmosphere. 2018; 9(2):66. https://doi.org/10.3390/atmos9020066
Chicago/Turabian StylePtak, Mariusz, Arkadiusz M. Tomczyk, and Dariusz Wrzesiński. 2018. "Effect of Teleconnection Patterns on Changes in Water Temperature in Polish Lakes" Atmosphere 9, no. 2: 66. https://doi.org/10.3390/atmos9020066
APA StylePtak, M., Tomczyk, A. M., & Wrzesiński, D. (2018). Effect of Teleconnection Patterns on Changes in Water Temperature in Polish Lakes. Atmosphere, 9(2), 66. https://doi.org/10.3390/atmos9020066






